Bending and Wave Propagation Analysis of Magneto-Electro-Elastic Functionally Graded Porous Microbeams
Abstract
:1. Introduction
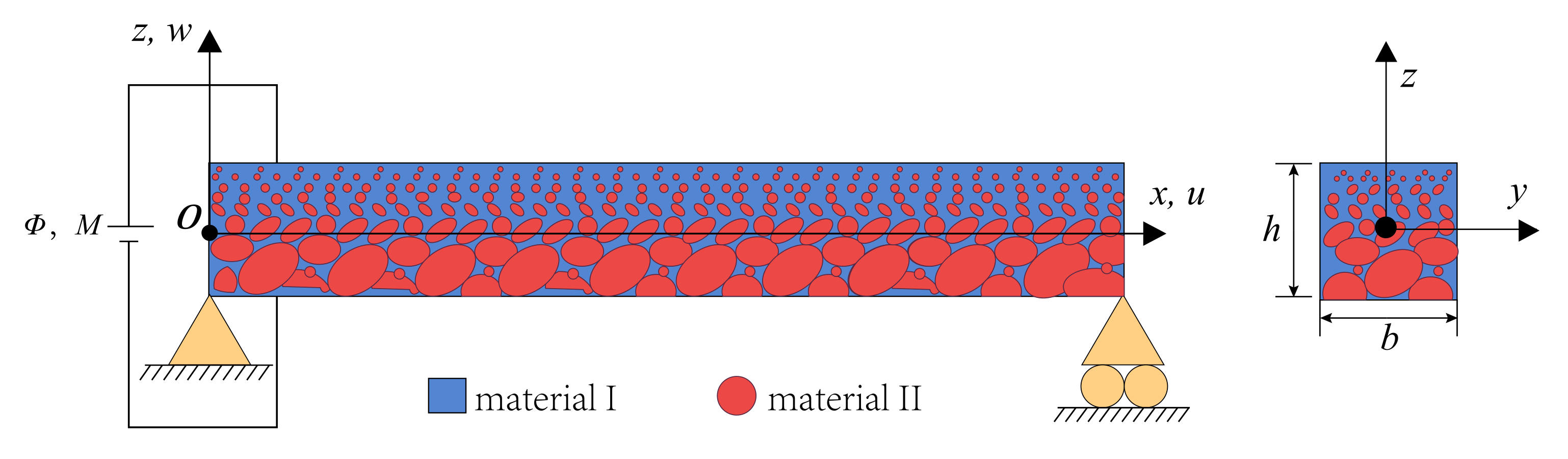
2. Materials and Methods
3. Analytical Solution
3.1. Static Bending
3.2. Wave Propagation
4. Numerical Results
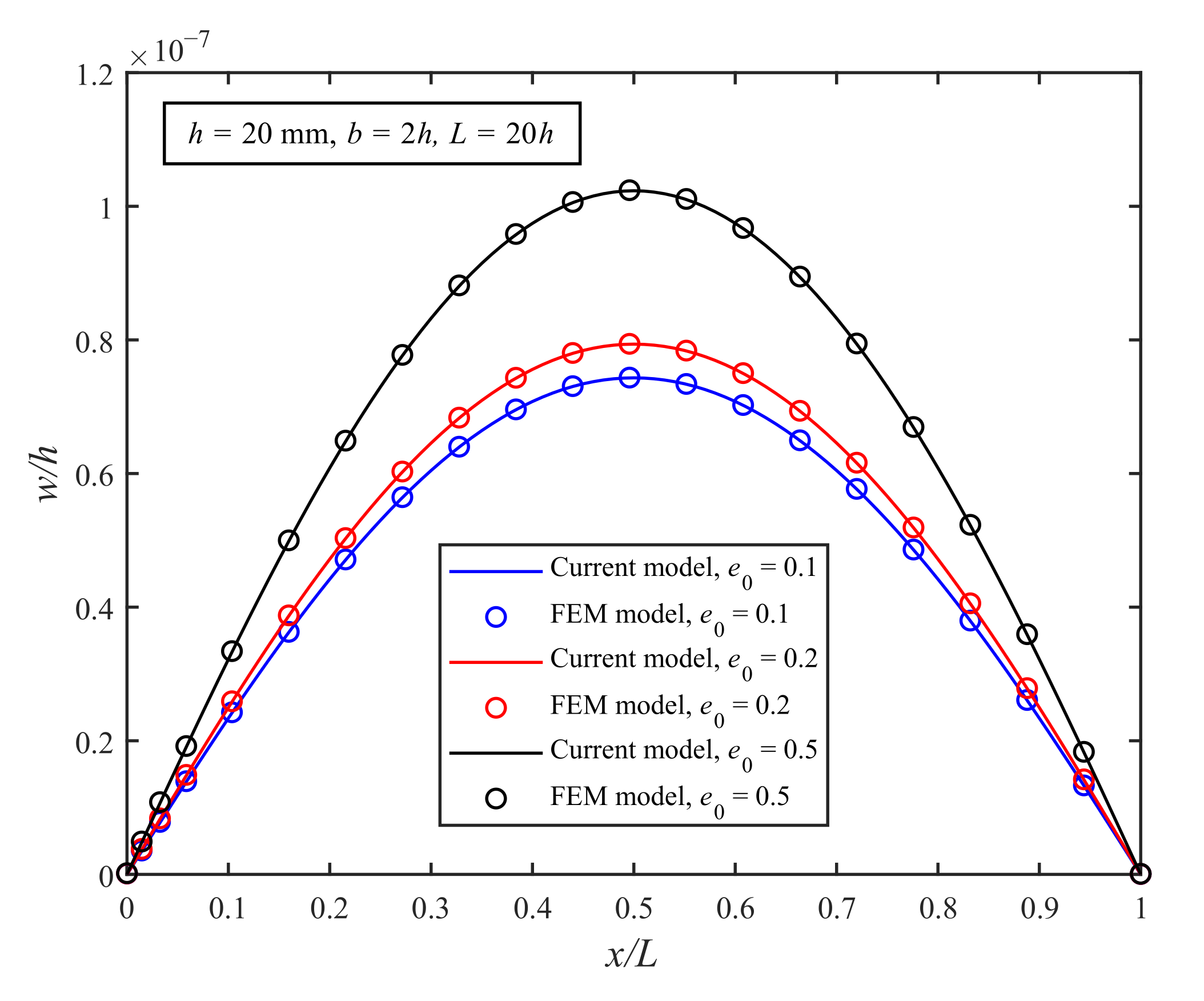
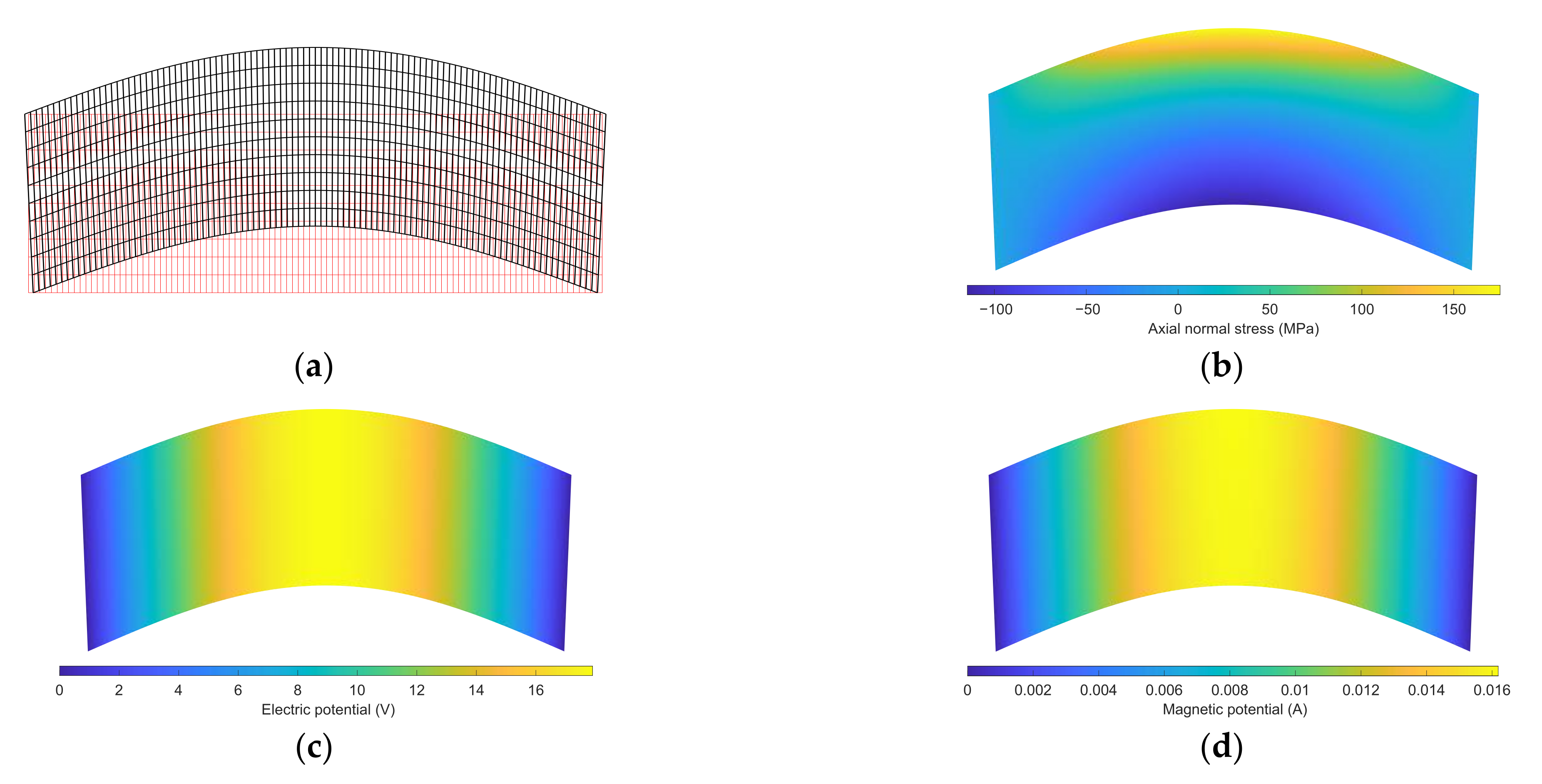
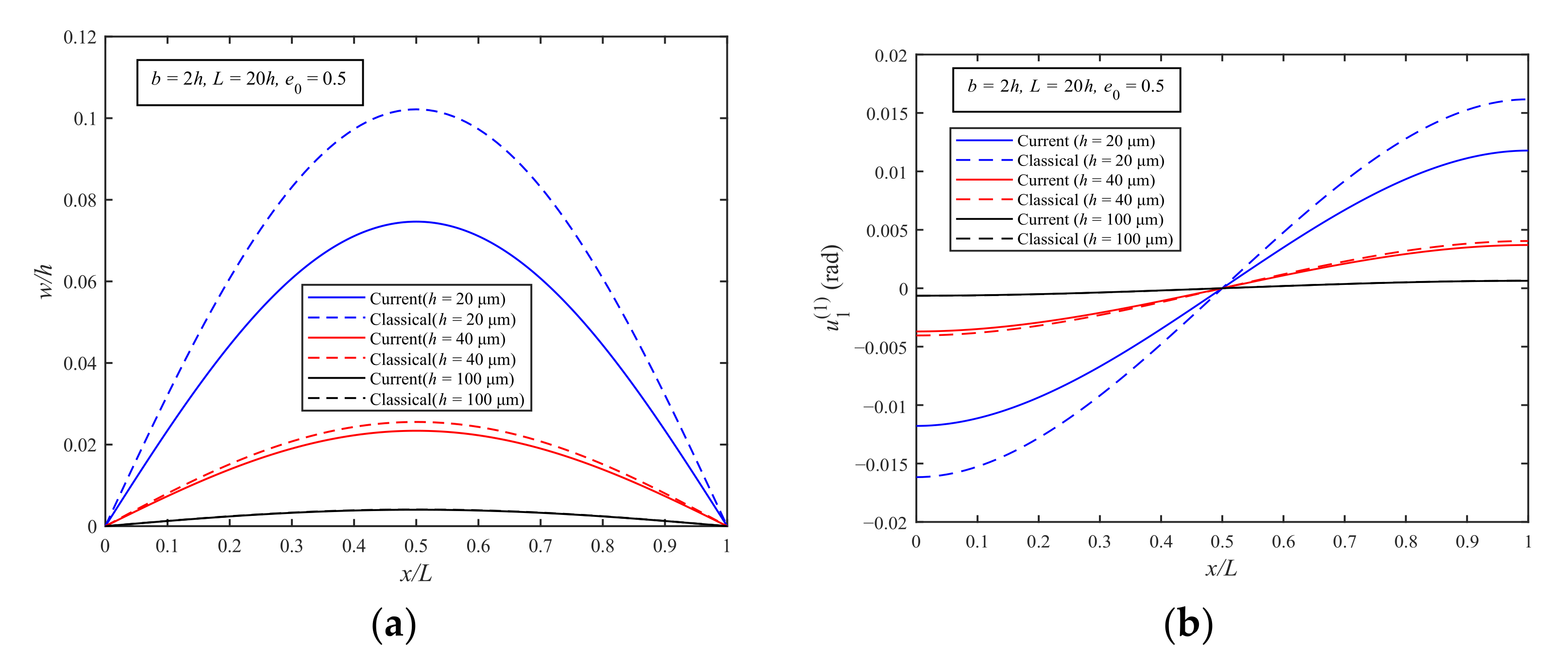
4.1. Static Bending
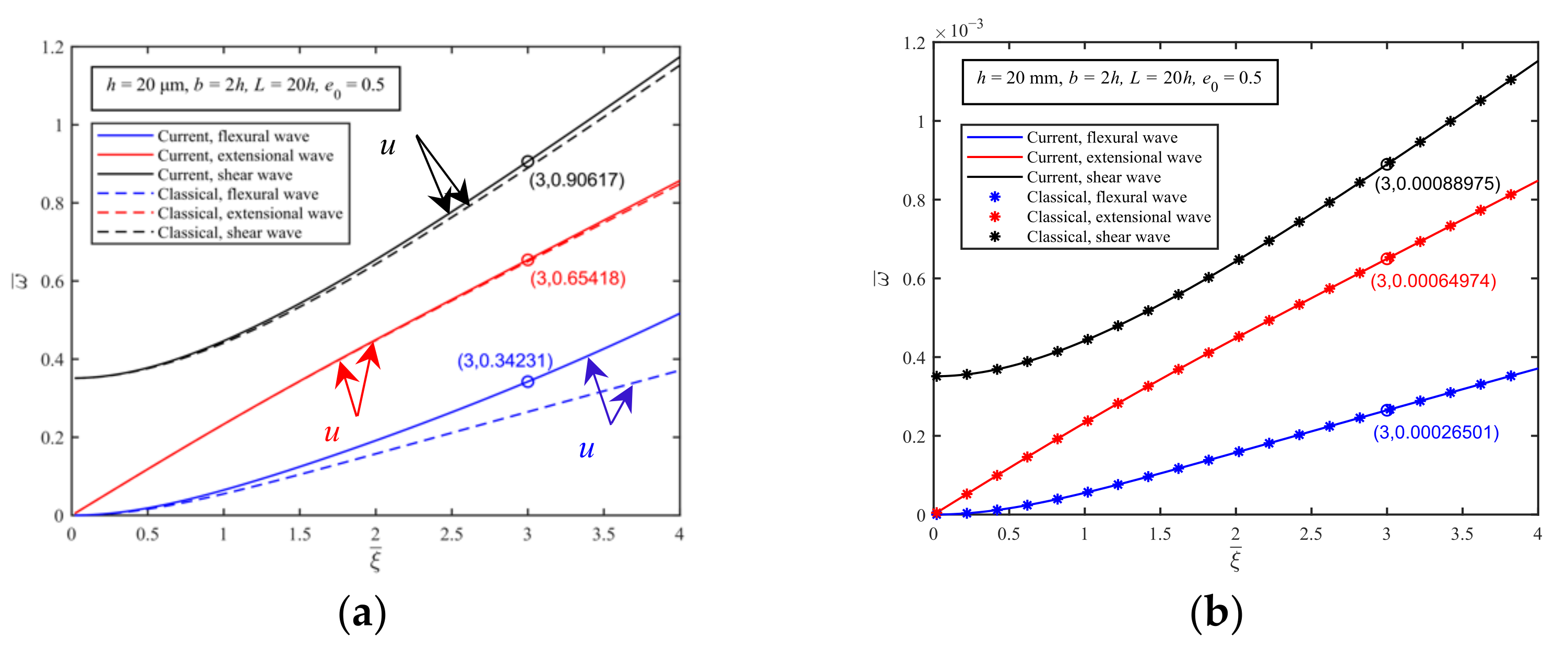
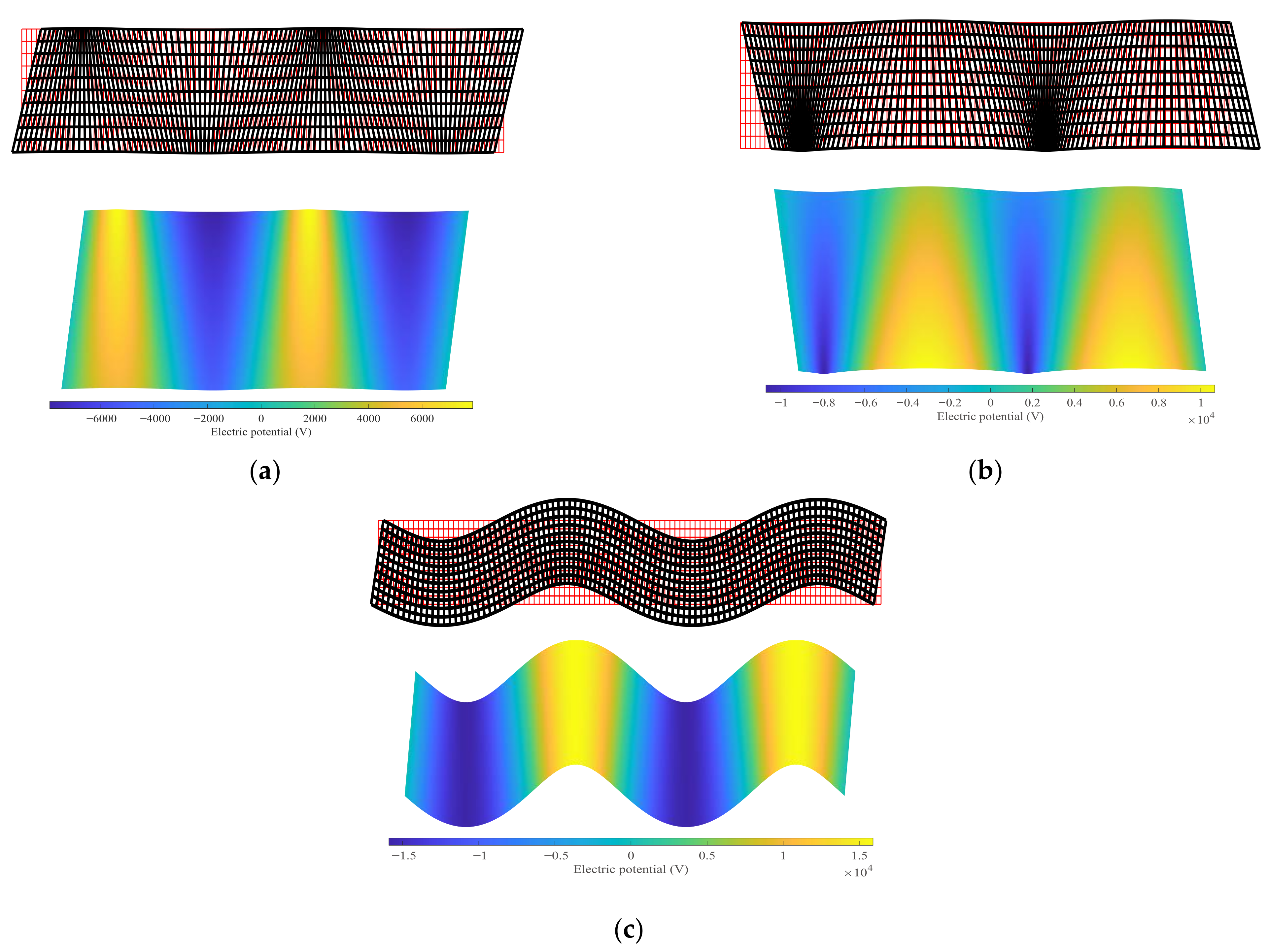
4.2. Wave Propagation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Sahmani, S.; Aghdam, M.M. Nonlocal Strain Gradient Shell Model for Axial Buckling and Postbuckling Analysis of Magneto-Electro-Elastic Composite Nanoshells. Compos. Part. B Eng. 2018, 132, 258–274. [Google Scholar] [CrossRef]
- Farajpour, M.R.; Shahidi, A.R.; Hadi, A.; Farajpour, A. Influence of Initial Edge Displacement on the Nonlinear Vibration, Electrical and Magnetic Instabilities of Magneto-Electro-Elastic Nanofilms. Mech. Adv. Mater. Struct. 2019, 26, 1469–1481. [Google Scholar] [CrossRef]
- Yakhno, V.G. An Explicit Formula for Modeling Wave Propagation in Magneto-Electro-Elastic Materials. J. Electromagn. Waves Appl. 2018, 32, 899–912. [Google Scholar] [CrossRef]
- Reddy, J.N. Microstructure-Dependent Couple Stress Theories of Functionally Graded Beams. J. Mech. Phys. Solids 2011, 59, 2382–2399. [Google Scholar] [CrossRef]
- Taati, E. Analytical Solutions for the Size Dependent Buckling and Postbuckling Behavior of Functionally Graded Micro-Plates. Int. J. Eng. Sci. 2016, 100, 45–60. [Google Scholar] [CrossRef]
- Şimşek, M. Buckling of Timoshenko Beams Composed of Two-Dimensional Functionally Graded Material (2D-FGM) Having Different Boundary Conditions. Compos. Struct. 2016, 149, 304–314. [Google Scholar] [CrossRef]
- Liu, J.; He, B.; Ye, W.; Yang, F. High Performance Model for Buckling of Functionally Graded Sandwich Beams Using a New Semi-Analytical Method. Compos. Struct. 2021, 262, 113614. [Google Scholar] [CrossRef]
- Akbarzadeh, A.H.; Abedini, A.; Chen, Z.T. Effect of Micromechanical Models on Structural Responses of Functionally Graded Plates. Compos. Struct. 2015, 119, 598–609. [Google Scholar] [CrossRef]
- Akbarzadeh, A.; Chen, Z. Thermo-Magneto-Electro-Elastic Responses of Rotating Hollow Cylinders. Mech. Adv. Mater. Struct. 2014, 21, 67–80. [Google Scholar] [CrossRef]
- Zhu, J.; Lai, Z.; Yin, Z.; Jeon, J.; Lee, S. Fabrication of ZrO2–NiCr Functionally Graded Material by Powder Metallurgy. Mater. Chem. Phys. 2001, 68, 130–135. [Google Scholar] [CrossRef]
- Peng, X.; Yan, M.; Shi, W. A New Approach for the Preparation of Functionally Graded Materials via Slip Casting in a Gradient Magnetic Field. Scr. Mater. 2007, 56, 907–909. [Google Scholar] [CrossRef]
- Kiran, M.C.; Kattimani, S.C.; Vinyas, M. Porosity Influence on Structural Behaviour of Skew Functionally Graded Magneto-Electro-Elastic Plate. Compos. Struct. 2018, 191, 36–77. [Google Scholar] [CrossRef]
- Vinyas, M. On Frequency Response of Porous Functionally Graded Magneto-Electro-Elastic Circular and Annular Plates with Different Electro-Magnetic Conditions Using HSDT. Compos. Struct. 2020, 240, 112044. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Jafari, A.; Selvamani, R. Thermal Buckling Analysis of Magneto-Electro-Elastic Porous FG Beam in Thermal Environment. Adv. Nano Res. 2020, 8, 83–94. [Google Scholar] [CrossRef]
- Vinyas, M.; Dineshkumar, H. Large Deflection Analysis of Functionally Graded Magneto-Electro-Elastic Porous Flat Panels. Eng. Comput. 2021, 1–20. [Google Scholar] [CrossRef]
- Sh, E.L.; Kattimani, S.; Vinyas, M. Nonlinear Free Vibration and Transient Responses of Porous Functionally Graded Magneto-Electro-Elastic Plates. Arch. Civ. Mech. Eng. 2022, 22, 38. [Google Scholar] [CrossRef]
- Zhu, C.S.; Fang, X.Q.; Liu, J.X. Surface Energy Effect on Buckling Behavior of the Functionally Graded Nano-Shell Covered with Piezoelectric Nano-Layers under Torque. Int. J. Mech. Sci. 2017, 133, 662–673. [Google Scholar] [CrossRef]
- Qu, Y.; Jin, F.; Zhang, G. Mechanically Induced Electric and Magnetic Fields in the Bending and Symmetric-Shear Deformations of a Microstructure-Dependent FG-MEE Composite Beam. Compos. Struct. 2021, 278, 114554. [Google Scholar] [CrossRef]
- Lam, D.C.C.; Yang, F.; Chong, A.C.M.; Wang, J.; Tong, P. Experiments and Theory in Strain Gradient Elasticity. J. Mech. Phys. Solids 2003, 51, 1477–1508. [Google Scholar] [CrossRef]
- Mcfarland, A.W.; Colton, J.S. Role of Material Microstructure in Plate Stiffness with Relevance to Microcantilever Sensors. J. Micromech. Microeng. 2005, 15, 1060. [Google Scholar] [CrossRef]
- Eringen, A.C. On Differential Equations of Nonlocal Elasticity and Solutions of Screw Dislocation and Surface Waves. J. Appl. Phys. 1983, 54, 4703–4710. [Google Scholar] [CrossRef]
- Toupin, R.A. Elastic Materials with Couple-Stresses. Arch. Ration. Mech. Anal. 1962, 11, 385–414. [Google Scholar] [CrossRef] [Green Version]
- Mindlin, R.D. Influence of Couple-Stresses on Stress Concentrations. Exp. Mech. 1963, 3, 1–7. [Google Scholar] [CrossRef]
- Kolter, W.T. Couple Stresses in the Theory of Elasticity: I and II. Proc. K. Ned. Akad. Wet. B 1964, 67, 17–44. [Google Scholar]
- Mindlin, R.D. Micro-Structure in Linear Elasticity. Arch. Ration. Mech. Anal. 1964, 16, 51–78. [Google Scholar] [CrossRef]
- Mindlin, R.D.; Eshel, N.N. On First Strain-Gradient Theories in Linear Elasticity. Int. J. Solids Struct. 1968, 4, 109–124. [Google Scholar] [CrossRef]
- Polizzotto, C.A. Hierarchy of Simplified Constitutive Models within Isotropic Strain Gradient Elasticity. Eur. J. Mech.—A/Solids 2017, 61, 92–109. [Google Scholar] [CrossRef]
- Altan, B.S.; Aifantis, E.C. On Some Aspects in the Special Theory of Gradient Elasticity. J. Mech. Behav. Mater. 1997, 8, 231–282. [Google Scholar] [CrossRef]
- Yang, F.; Chong, A.C.M.; Lam, D.C.C.; Tong, P. Couple Stress Based Strain Gradient Theory for Elasticity. Int. J. Solids Struct. 2002, 39, 2731–2743. [Google Scholar] [CrossRef]
- Park, S.K.; Gao, X.L. Variational Formulation of a Modified Couple Stress Theory and Its Application to a Simple Shear Problem. Z. Angew. Math. Phys. 2008, 59, 904–917. [Google Scholar] [CrossRef]
- Zhang, G.Y.; Gao, X.L. A New Bernoulli–Euler Beam Model Based on a Reformulated Strain Gradient Elasticity Theory. Math. Mech. Solids 2020, 25, 630–643. [Google Scholar] [CrossRef]
- Qu, Y.L.; Zhang, G.Y.; Fan, Y.M.; Jin, F. A Non-Classical Theory of Elastic Dielectrics Incorporating Couple Stress and Quadrupole Effects: Part I—Reconsideration of Curvature-Based Flexoelectricity Theory. Math. Mech. Solids 2021, 26, 1647–1659. [Google Scholar] [CrossRef]
- Zhang, G.Y.; Gao, X.L.; Zheng, C.Y.; Mi, C.W. A Non-Classical Bernoulli-Euler Beam Model Based on a Simplified Micromorphic Elasticity Theory. Mech. Mater. 2021, 161, 103967. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Barati, M.R. Vibration Analysis of Embedded Biaxially Loaded Magneto-Electrically Actuated Inhomogeneous Nanoscale Plates. J. Vib. Control. 2018, 24, 3587–3607. [Google Scholar] [CrossRef]
- Kiani, A.; Sheikhkhoshkar, M.; Jamalpoor, A.; Khanzadi, M. Free Vibration Problem of Embedded Magneto-Electro-Thermo-Elastic Nanoplate Made of Functionally Graded Materials via Nonlocal Third-Order Shear Deformation Theory. J. Intell. Mater. Syst. Struct. 2018, 29, 741–763. [Google Scholar] [CrossRef]
- Liu, H.; Lv, Z. Vibration Performance Evaluation of Smart Magneto-Electro-Elastic Nanobeam with Consideration of Nanomaterial Uncertainties. J. Intell. Mater. Syst. Struct. 2019, 30, 2932–2952. [Google Scholar] [CrossRef]
- Xiao, W.S.; Gao, Y.; Zhu, H. Buckling and Post-Buckling of Magneto-Electro-Thermo-Elastic Functionally Graded Porous Nanobeams. Microsyst. Technol. 2019, 25, 2451–2470. [Google Scholar] [CrossRef]
- Lim, C.W.; Zhang, G.; Reddy, J.N. A Higher-Order Nonlocal Elasticity and Strain Gradient Theory and Its Applications in Wave Propagation. J. Mech. Phys. Solids 2015, 78, 298–313. [Google Scholar] [CrossRef]
- Şimşek, M. Nonlinear Free Vibration of a Functionally Graded Nanobeam Using Nonlocal Strain Gradient Theory and a Novel Hamiltonian Approach. Int. J. Eng. Sci. 2016, 105, 12–27. [Google Scholar] [CrossRef]
- Li, X.; Li, L.; Hu, Y.; Ding, Z.; Deng, W. Bending, Buckling and Vibration of Axially Functionally Graded Beams Based on Nonlocal Strain Gradient Theory. Compos. Struct. 2017, 165, 250–265. [Google Scholar] [CrossRef]
- Gao, X.L.; Zhang, G.Y. A Microstructure- and Surface Energy-Dependent Third-Order Shear Deformation Beam Model. Z. Angew. Math. Phys. 2015, 66, 1871–1894. [Google Scholar] [CrossRef]
- Yu, T.; Hu, H.; Zhang, J.; Bui, T.Q. Isogeometric Analysis of Size-Dependent Effects for Functionally Graded Microbeams by a Non-Classical Quasi-3D Theory. Thin Walled Struct. 2019, 138, 1–14. [Google Scholar] [CrossRef]
- Zhang, G.Y.; Qu, Y.L.; Gao, X.L.; Jin, F. A Transversely Isotropic Magneto-Electro-Elastic Timoshenko Beam Model Incorporating Microstructure and Foundation Effects. Mech. Mater. 2020, 149, 103412. [Google Scholar] [CrossRef]
- Hong, J.; Wang, S.P.; Zhang, G.Y.; Mi, C.W. On the Bending and Vibration Analysis of Functionally Graded Magneto-Electro-Elastic Timoshenko Microbeams. Crystals 2021, 11, 1206. [Google Scholar] [CrossRef]
- Qu, Y.L.; Li, P.; Zhang, G.Y.; Jin, F.; Gao, X.L. A Microstructure-Dependent Anisotropic Magneto-Electro-Elastic Mindlin Plate Model Based on an Extended Modified Couple Stress Theory. Acta Mech. 2020, 231, 4323–4350. [Google Scholar] [CrossRef]
- Shen, W.; Zhang, G.; Gu, S.; Cong, Y. A Transversely Isotropic Magneto-Electro-Elastic Circular Kirchhoff Plate Model Incorporating Microstructure Effect. Acta Mech. Solida Sin. 2022, 35, 185–197. [Google Scholar] [CrossRef]
- Qu, Y.L.; Jin, F.; Yang, J.S. Magnetically Induced Charge Motion in the Bending of A Beam with Flexoelectric Semiconductor and Piezomagnetic Dielectric Layers. J. Appl. Phys. 2021, 127, 064503. [Google Scholar] [CrossRef]
- Zhu, F.; Ji, S.; Zhu, J.; Qian, Z.; Yang, J. Study on the Influence of Semiconductive Property for the Improvement of Nanogenerator by Wave Mode Approach. Nano Energy 2018, 52, 474–484. [Google Scholar] [CrossRef]
- Shingare, K.B.; Kundalwal, S.I. Static and Dynamic Response of Graphene Nanocomposite Plates with Flexoelectric Effect. Mech. Mater. 2019, 134, 69–84. [Google Scholar] [CrossRef]
- Wang, L.; Liu, S.; Feng, X.; Zhang, C.; Zhu, L.; Zhai, J.; Qin, Y.; Wang, Z.L. Flexoelectronics of Centrosymmetric Semiconductors. Nat. Nanotechnol. 2020, 15, 661–667. [Google Scholar] [CrossRef]
- Sharma, S.; Kumar, A.; Kumar, R.; Talha, M.; Vaish, R. Geometry Independent Direct and Converse Flexoelectric Effects in Functionally Graded Dielectrics: An Isogeometric Analysis. Mech. Mater. 2020, 148, 103456. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Elastic Buckling and Static Bending of Shear Deformable Functionally Graded Porous Beam. Compos. Struct. 2015, 133, 54–61. [Google Scholar] [CrossRef] [Green Version]
- Chen, D.; Yang, J.; Kitipornchai, S. Free and Forced Vibrations of Shear Deformable Functionally Graded Porous Beams. Int. J. Mech. Sci. 2016, 108, 14–22. [Google Scholar] [CrossRef] [Green Version]
- Heshmati, M.; Daneshmand, F. A Study on the Vibrational Properties of Weight-Efficient Plates Made of Material with Functionally Graded Porosity. Compos. Struct. 2018, 200, 229–238. [Google Scholar] [CrossRef]
- Zhang, G.Y.; Gao, X.L. Band Gaps for Wave Propagation in 2-D Periodic Three-Phase Composites with Coated Star-Shaped Inclusions and an Orthotropic Matrix. Compos. Part B Eng. 2020, 182, 107319. [Google Scholar] [CrossRef]
- Mindlin, R.D. High Frequency Vibrations of Piezoelectric Crystal Plates. Int. J. Solids Struct. 1972, 8, 895–906. [Google Scholar] [CrossRef]
- Yang, J. The Mechanics of Piezoelectric Structures; World Scientific: Singapore, 2006. [Google Scholar] [CrossRef]
- Qu, Y.; Jin, F.; Yang, J. Effects of Mechanical Fields on Mobile Charges in a Composite Beam of Flexoelectric Dielectrics and Semiconductors. J. Appl. Phys. 2020, 127, 194502. [Google Scholar] [CrossRef]
- Zhang, G.Y.; Gao, X.L.; Guo, Z.Y. A Non-Classical Model for an Orthotropic Kirchhoff Plate Embedded in a Viscoelastic Medium. Acta Mech. 2017, 228, 3811–3825. [Google Scholar] [CrossRef]
- Han, X.; Pan, E. Fields Produced by Three-Dimensional Dislocation Loops in Anisotropic Magneto-Electro-Elastic Materials. Mech. Mater. 2013, 59, 110–125. [Google Scholar] [CrossRef]
- Kumar, D.; Sarangi, S.; Saxena, P. Universal Relations in Coupled Electro-Magneto-Elasticity. Mech. Mater. 2020, 143, 103308. [Google Scholar] [CrossRef]
- Ma, H.M.; Gao, X.L.; Reddy, J.N. A Microstructure-Dependent Timoshenko Beam Model Based on a Modified Couple Stress Theory. J. Mech. Phys. Solids 2008, 56, 3379–3391. [Google Scholar] [CrossRef]
- Hong, J.; Wang, S.P.; Zhang, G.Y.; Mi, C.W. Bending, Buckling and Vibration Analysis of Complete Microstructure-Dependent Functionally Graded Material Microbeams. Int. J. Appl. Mech. 2021, 13, 2150057. [Google Scholar] [CrossRef]
- Reddy, J.N. Energy Principles and Variational Methods in Applied Mechanics, 2nd ed.; Wiley: New York, NY, USA, 2002. [Google Scholar]
- Gao, X.L.; Mall, S. Variational Solution for a Cracked Mosaic Model of Woven Fabric Composites. Int. J. Solids Struct. 2001, 38, 855–874. [Google Scholar] [CrossRef]
- Yang, J. An Introduction to the Theory of Piezoelectricity; Springer: New York, NY, USA, 2005. [Google Scholar] [CrossRef]
- Li, J.Y. Magnetoelectroelastic Multi-Inclusion and Inhomogeneity Problems and Their Applications in Composite Materials. Int. J. Eng. Sci. 2000, 38, 1993–2011. [Google Scholar] [CrossRef]
- Sih, G.C.; Song, Z.F. Magnetic and Electric Poling Effects Associated with Crack Growth in BaTiO3–CoFe2O4 Composite. Theor. Appl. Fract. Mech. 2003, 39, 209–227. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, R.; Ding, H. Axisymmetric Bending of Functionally Graded Circular Magneto-Electro-Elastic Plates. Eur. J. Mech. A/Solids 2011, 30, 999–1011. [Google Scholar] [CrossRef]
- Zhang, G.Y.; Gao, X.L. Elastic Wave Propagation in 3-D Periodic Composites: Band Gaps Incorporating Microstructure Effects. Compos. Struct. 2018, 204, 920–932. [Google Scholar] [CrossRef]
- Ma, H.M.; Gao, X.L.; Reddy, J.N. A Non-Classical Mindlin Plate Model Based on a Modified Couple Stress Theory. Acta Mechanica 2011, 220, 217–235. [Google Scholar] [CrossRef]


| Physical Parameter | Material I | Material II |
|---|---|---|
| C11 (GPa) | 226 | 4.889 |
| C44 (GPa) | 44.15 | 1.241 |
| e15 (C/m2) | 5.8 | / |
| e31 (C/m2) | −2.2 | / |
| e33 (C/m2) | 9.3 | / |
| s11 (10−9 C2/(N·m2)) | 5.64 | / |
| s33 (10−9 C2/(N·m2)) | 6.35 | / |
| q15 (N/(A·m)) | 275 | / |
| q31 (N/(A·m)) | 290.15 | / |
| q33 (N/(A·m)) | 349.85 | / |
| d11 (10−12 Ns/(V·C)) | 5.38 | / |
| d33 (10−12 Ns/(V·C)) | 2740 | / |
| μ11 (10−6 Ns2/C2) | 297.5 | / |
| μ33 (10−6 Ns2/C2) | 83.5 | / |
| A11 (N) | 11.7484 | 1.4014 |
| A12 (N) | 6.4980 | 0.6903 |
| ρ (kg/m3) | 5550 | 1180 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hong, J.; Wang, S.; Qiu, X.; Zhang, G. Bending and Wave Propagation Analysis of Magneto-Electro-Elastic Functionally Graded Porous Microbeams. Crystals 2022, 12, 732. https://doi.org/10.3390/cryst12050732
Hong J, Wang S, Qiu X, Zhang G. Bending and Wave Propagation Analysis of Magneto-Electro-Elastic Functionally Graded Porous Microbeams. Crystals. 2022; 12(5):732. https://doi.org/10.3390/cryst12050732
Chicago/Turabian StyleHong, Jun, Shaopeng Wang, Xinyuan Qiu, and Gongye Zhang. 2022. "Bending and Wave Propagation Analysis of Magneto-Electro-Elastic Functionally Graded Porous Microbeams" Crystals 12, no. 5: 732. https://doi.org/10.3390/cryst12050732
APA StyleHong, J., Wang, S., Qiu, X., & Zhang, G. (2022). Bending and Wave Propagation Analysis of Magneto-Electro-Elastic Functionally Graded Porous Microbeams. Crystals, 12(5), 732. https://doi.org/10.3390/cryst12050732







