Creating a Domain of Losses in the Laboratory: Effects of Endowment Size
Abstract
1. Introduction
2. Results
2.1. Theoretical Model and Predictions
2.2. Experimental Results
3. Discussion
3.1. General Discussion
3.2. Relationship to Loss Aversion and Market Entry Games
4. Materials and Methods
5. Conclusions
Acknowledgments
Conflicts of Interest
Appendix


References
- Andreoni, J. Warm-glow versus cold-prickle: The effects of positive and negative framing on cooperation in experiments. Q. J. Econ. 1995, 110, 1–21. [Google Scholar] [CrossRef]
- Park, E. Warm-glow versus cold-prickle: A further experimental study of framing effects on free-riding. J. Econ. Behav. Organ. 2000, 43, 405–421. [Google Scholar] [CrossRef]
- Sonnemans, J.; Schram, A.; Offerman, T. Public good provision and public bad prevention: The effect of framing. J. Econ. Behav. Organ. 1998, 34, 143–161. [Google Scholar] [CrossRef]
- Cox, C. Decomposing the effects of negative framing in linear public goods games. Econ. Lett. 2015, 126, 63–65. [Google Scholar] [CrossRef]
- Thaler, R.; Johnson, E. Gambling with the house money and trying to break even: The effects of prior outcomes on risky choice. Manag. Sci. 1990, 36, 643–660. [Google Scholar] [CrossRef]
- Rosenboim, M.; Shavit, T. Whose money is it anyway? Using prepaid incentives in experimental economics to create a natural environment. Exp. Econ. 2012, 15, 145–157. [Google Scholar] [CrossRef]
- Davis, L.R.; Joyce, B.P.; Roelofs, M.R. My money or yours: House money payment effects. Exp. Econ. 2010, 10, 171–178. [Google Scholar] [CrossRef]
- Cherry, T.L.; Frykblom, P.; Shogren, J.F. Hardnose the dictator. Am. Econ. Rev. 2002, 92, 1218–1221. [Google Scholar] [CrossRef]
- Oxoby, R.J.; Spraggon, J. Mine and yours: Property rights in dictator games. J. Econ. Behav. Organ. 2008, 65, 703–713. [Google Scholar] [CrossRef]
- Reinstein, D.; Riener, G. Decomposing desert and tangibility effects in a charitable giving experiment. Exp. Econ. 2012, 15, 229–240. [Google Scholar] [CrossRef]
- Etchart-Vincent, N.; L’Haridon, O. Monetary incentives in the loss domain and behavior toward risk: An experimental comparison of three reward schemes including real losses. J. Risk Uncertain. 2011, 42, 61–83. [Google Scholar] [CrossRef]
- Sorensen, A. Asymmetry, uncertainty, and limits in a binary choice experiment with positive spillovers. J. Econ. Behav. Organ. 2015, 116, 43–55. [Google Scholar] [CrossRef]
- Kahneman, D.; Tversky, A. Prospect Theory: An Analysis of Decision under Risk. Econometrica 1979, 47, 263–291. [Google Scholar] [CrossRef]
- Erev, I.; Eyal, E.; Roth, A. A choice prediction competition for market entry games: An introduction. Games 2010, 1, 117–136. [Google Scholar] [CrossRef]
- Greiner, B. An Online Recruitment System for Economics Experiments. In Forschung und Wissenschaftliches Rechnen; Kremer, K., Macho, V., Eds.; Gesellschaft für wissenschaftliche Datenverarbeitung: Göttingen, Germany, 2004; pp. 79–93. [Google Scholar]
- Fischbacher, U. z-tree: Zurich toolbox for ready-made economics experiments. Exp. Econ. 2007, 10, 171–178. [Google Scholar] [CrossRef]
| 1 | The game in [12] was motivated by the individual vaccination decision, with Option A representing a choice to receive a costly but fully effective vaccine, and Option B representing a choice not to vaccinate and potentially get sick, with one’s likelihood of getting sick increasing with the number of unvaccinated individuals. |
| 2 | and are as in [12], and were determined by a more complex probabilistic contagion model in which the application of interest was individual vaccination decisions. |
| 3 | For sequence 2 columns, the round number corresponds to the round within that sequence. That is, “Round 1” is technically the 11th round in the session, “Round 2” is the 12th round, and so on. |
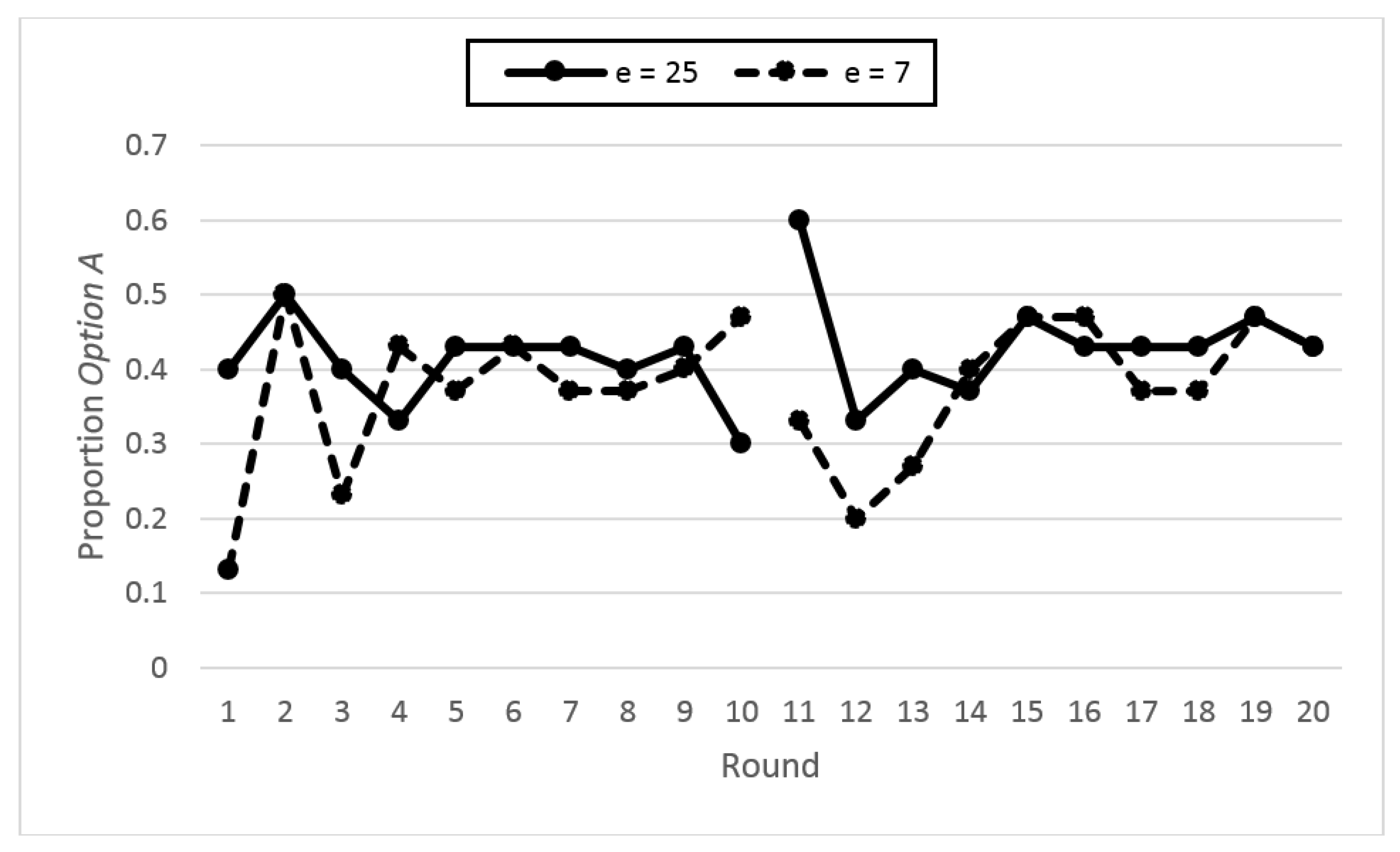
| 4 | This general pattern of individuals choosing Option A more often when e = 25 than when e = 7 in early rounds only is consistent across subject types (High-loss and Low-loss). |
| 5 | In the actual experiments, High-loss subjects were referred to as “Type 1” and Low-loss subjects were referred to as “Type 2”. Subjects knew their own type and the distribution of types within their group. |
| 6 | This was done to encourage subjects to treat each round within a sequence as the same decision task, beginning with an endowment of either 25 ECU or 7 ECU, instead of with their prior earnings or losses built into the next round’s endowment. |
| 7 | Complete experimental instructions and screenshots are included in Appendix. |
| Number of Other Agents who Choose Option B | ||||||
|---|---|---|---|---|---|---|
| 0 | 1 | 2 | 3 | 4 | 5 | |
| Option A Payoff | 18 | 18 | 18 | 18 | 18 | 18 |
| High-loss, Option B payoff | 22.50 | 20.70 | 18.50 | 16.45 | 14.75 | 13.30 |
| Low-loss, Option B payoff | 23.00 | 21.60 | 19.80 | 18.20 | 16.80 | 15.60 |
| Number of Other Agents Who Choose Option B | ||||||
|---|---|---|---|---|---|---|
| 0 | 1 | 2 | 3 | 4 | 5 | |
| Option A Payoff | 0 | 0 | 0 | 0 | 0 | 0 |
| High-loss, Option B payoff | 4.50 | 2.70 | 0.50 | −1.55 | −3.25 | −4.70 |
| Low-loss, Option B payoff | 5.00 | 3.60 | 1.80 | 0.20 | −1.20 | −2.40 |
| e = 25 (S1) | e = 7 (S1) | e = 25 (S2) | e = 7 (S2) | |
|---|---|---|---|---|
| Round 1 | 0.40 | 0.13 | 0.60 | 0.33 |
| Round 2 | 0.50 | 0.50 | 0.33 | 0.20 |
| Round 3 | 0.40 | 0.23 | 0.40 | 0.27 |
| Round 4 | 0.33 | 0.43 | 0.37 | 0.40 |
| Round 5 | 0.43 | 0.37 | 0.47 | 0.47 |
| Round 6 | 0.43 | 0.43 | 0.43 | 0.47 |
| Round 7 | 0.43 | 0.37 | 0.43 | 0.37 |
| Round 8 | 0.40 | 0.37 | 0.43 | 0.37 |
| Round 9 | 0.43 | 0.40 | 0.47 | 0.47 |
| Round 10 | 0.30 | 0.47 | 0.43 | 0.43 |
| All rounds | 0.41 | 0.37 | 0.44 | 0.38 |
| Rounds 1–5 | 0.41 | 0.33 | 0.43 | 0.33 |
| Rounds 6–10 | 0.41 | 0.41 | 0.44 | 0.42 |
| Endowment | Sequence | Type | Constant | |
|---|---|---|---|---|
| All rounds | 0.276 * (0.145) | 0.033 (0.145) | 2.180 *** (0.184) | −1.497 *** (0.200) |
| Round 1 | 1.312 *** (0.425) | 1.003 ** (0.421) | 0.977 ** (0.434) | −2.134 *** (0.465) |
| Round 2 | 0.314 (0.398) | −1.068 *** (0.403) | 0.955 ** (0.415) | −0.470 (0.359) |
| Round 3 | 0.710 * (0.403) | 0.799 (0.400) | 0.686 * (0.413) | −1.393 *** (0.401) |
| Round 4 | −0.333 (0.410) | 0.000 (0.409) | 1.729 *** (0.422) | −0.939 ** (0.380) |
| Round 5 | 0.172 (0.415) | 0.343 (0.416) | 2.086 *** (0.446) | −1.235 *** (0.400) |
| Round 6 | −0.078 (0.396) | 0.078 (0.396) | 1.637 *** (0.421) | −0.789 ** (0.369) |
| Round 7 | 0.420 (0.462) | 0.000 (0.458) | 2.799 *** (0.489) | −1.610 *** (0.446) |
| Round 8 | 0.305 (0.453) | 0.102 (0.451) | 2.638 *** (0.474) | −1.597 *** (0.440) |
| Round 9 | 0.088 (0.419) | 0.263 (0.419) | 2.216 *** (0.456) | −1.149 *** (0.398) |
| Round 10 | −0.484 (0.444) | 0.291 (0.442) | 2.517 *** (0.472) | −1.162 *** (0.407) |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sorensen, A. Creating a Domain of Losses in the Laboratory: Effects of Endowment Size. Games 2018, 9, 13. https://doi.org/10.3390/g9010013
Sorensen A. Creating a Domain of Losses in the Laboratory: Effects of Endowment Size. Games. 2018; 9(1):13. https://doi.org/10.3390/g9010013
Chicago/Turabian StyleSorensen, Andrea. 2018. "Creating a Domain of Losses in the Laboratory: Effects of Endowment Size" Games 9, no. 1: 13. https://doi.org/10.3390/g9010013
APA StyleSorensen, A. (2018). Creating a Domain of Losses in the Laboratory: Effects of Endowment Size. Games, 9(1), 13. https://doi.org/10.3390/g9010013




