Appendix A
Proof of Lemma 1. For any announcement of firm , we define price thresholds and of the lemma, which depend on . Thresholds and depend on and are defined similarly mutatis mutandis. Threshold is defined in such a way that is binding for all and non-binding for . Threshold is defined in such a way that firm is restricted by for all and it breaks if .
If for any , then is never binding and we define . If for any , then is always binding and we define . In the remaining cases, there is a unique price such that so that function is the inverse of function .
If we define . If , we consider . Due to the concavity of , the total profit decreases in on so that any price is suboptimal. Hence, either or . If for any , we define . Otherwise (because ), there is a unique price such that so that function is the -inverse of function .
For , firm is unrestricted so that . Firm is restricted by if so that . Firm breaks if so that . This ends the proof of the lemma expressions for .
Best response is non-decreasing in . By construction, whenever . Hence, is continuous. Finally, is either constant or increases with a slope less than one. Therefore, NE always exists and is unique. Since is non-decreasing in , equilibrium prices are non-decreasing in all announcements .□
Proof of Proposition 1. Consider the second-period best response function
given by Lemma 1:
First, we argue that for any announcement , NE prices satisfy and . Second, we argue that for any announcements and , NE prices satisfy and . This implies that in any SPNE with outcome , it must be that and . Finally, we argue that no announcements and can be an SPNE because each firm has a profitable deviation.
When , so that . Hence, reaction functions and do not intersect when or . Thus, second-period equilibrium prices satisfy .
When , best responses and do not intersect at , because firm is either restricted or breaking so that . Hence, . Then, in turn, . Therefore, can only occur when and .
Suppose firms announce with and . The unique NE of this subgame is . Let firm deviate and announce marginally above . This announcement is binding so that . Firm remains unrestricted so that . As a result, the deviational profit of firm is , which is increasing at . Thus, no SPNE with outcome exists.□
Proof of Proposition 2. According to Proposition 1, an equilibrium where both firms are unrestricted never exists. First, we consider an SPNE where one firm is unrestricted, then we consider an SPNE where neither of them is unrestricted.
Equilibria where one firm is unrestricted.
Let announcements followed by prices be an SPNE outcome, in which and . Then, and firm is either restricted (when so that ) or breaking its announcement (when so that ). We show that in an SPNE, so that must hold.
Suppose
. Then, the best response of firm
is independent of
, and firm
has afv profitable deviation to reduce its announcement to
and save deviational cost
. Hence, it must be that
so that
and firm
is restricted. If firm
deviates to
, its profit is
, the leader’s profit in the Stackelberg game. Therefore,
followed by prices
is a unique SPNE outcome if
is the NE of the subgame, i.e., if
. This occurs when
, where
is defined by
:
In an asymmetric SPNE, price announcement
of
must satisfy two conditions. First, it must be non-binding, i.e.,
. Second, it must be such that firm
does not want to deviate and announce
, i.e.,
. Clearly,
satisfies these two conditions so that these asymmetric SPNE always exist.
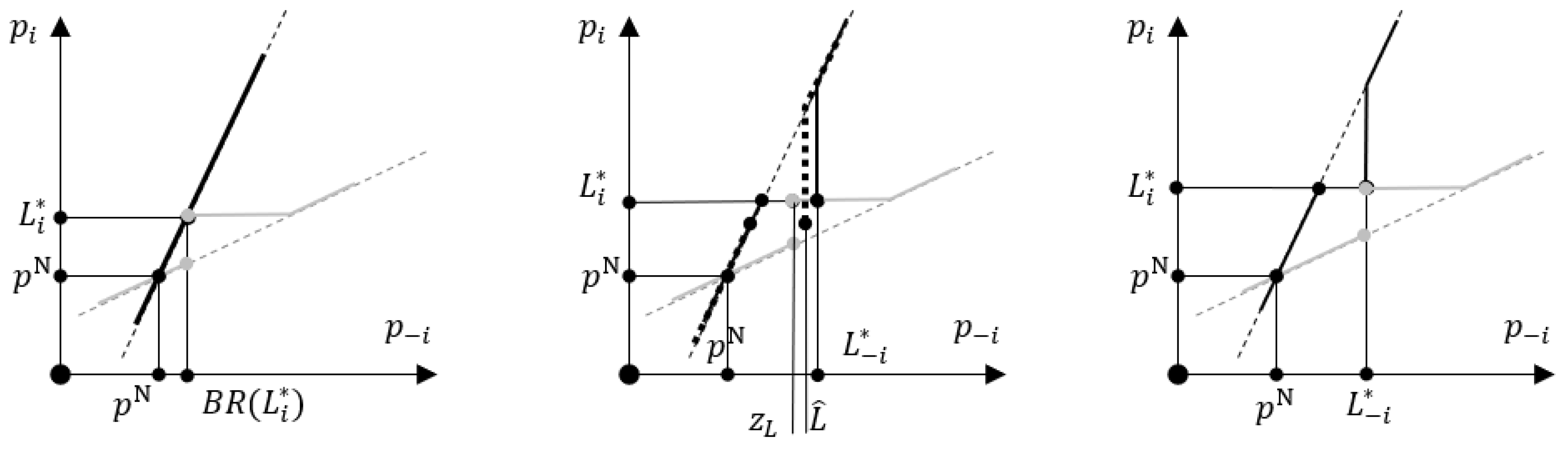
Figure A1 (left) provides a stylized example of firm’ best response functions for the on-path pricing subgame in an asymmetric SPNE. Firm
is unrestricted, and its second-stage best-response function
coincides with
; it is the solid black curve. Firm
is restricted by
, and its second-stage best-response function
is shown by the solid grey curve.
Equilibria where neither of the firms is unrestricted.
Let announcements followed by prices be an SPNE outcome, in which and . Both announcements are binding, and each firm is either restricted (when so that ) or breaking its announcement (when so that ). We show that in an SPNE, so that must hold for both firms.
Suppose
. Then, the best response of firm
is independent of
, and firm
has a profitable deviation to reduce its announcement to
and save deviational cost
. Hence, it must be that
and, similarly,
.
Figure A1.
Firms’ best responses and Nash equilibria in on-path subgames for asymmetric (left picture) and symmetric (right picture) SPNE. Best responses are dashed curves that intersect at , generalized best responses are dotted curves, the second stage best-response functions are solid curves; grey (black) curves are of firm ().
Figure A1.
Firms’ best responses and Nash equilibria in on-path subgames for asymmetric (left picture) and symmetric (right picture) SPNE. Best responses are dashed curves that intersect at , generalized best responses are dotted curves, the second stage best-response functions are solid curves; grey (black) curves are of firm ().
Suppose
and
. Then, the best response of firm
is independent of
, and firm
has a profitable deviation to marginally reduce
to
. Both firms remain restricted. In NE, firm
keeps charging price
, and firm
lowers its price to
. This deviation is profitable because profit of firm
,
, is decreasing in
when
. Hence, it must be that
and, similarly,
. Thus, the necessary SPNE condition is
that must hold for both firms. Since
is increasing in
and has slope less than one, the equilibrium, if it exists, is unique, symmetric, and determined by
where
.
Figure A1 (right) provides a stylized example of firm’ best response functions for the on-path pricing subgame in a symmetric SPNE. Both firms are restricted, their second-stage best-response functions are solid black (for
) and grey (for
) curves.
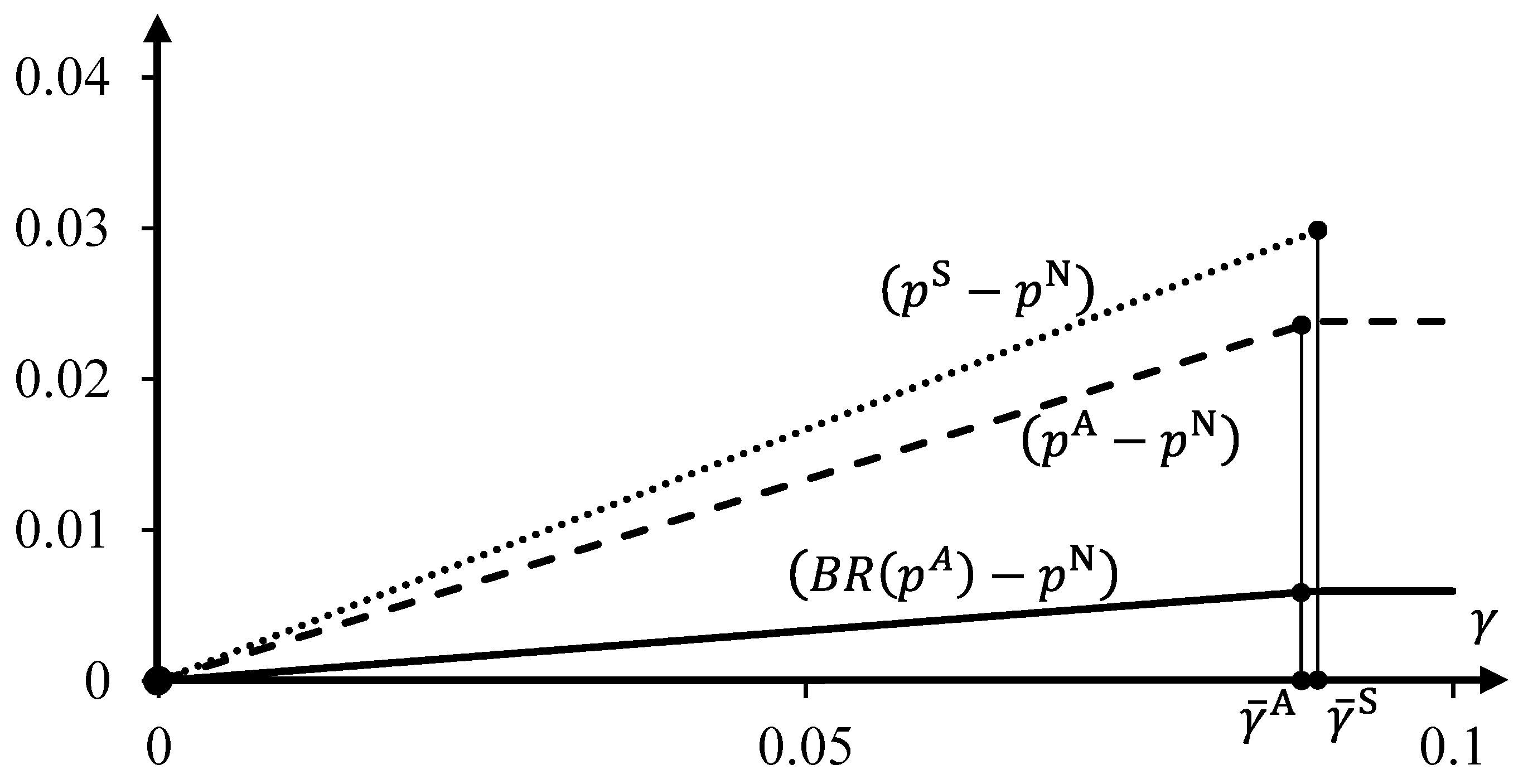
To determine equilibrium existence conditions, let us consider firm . Its equilibrium profit is . Deviating to forces firm to break so that its best response is . Since the best response of firm does not change, NE remains the same, . This deviation only increases the cost of firm and is, therefore, not profitable. Deviating to can either keep firm restricted, if , or make it unrestricted, if . In both cases, firm breaks its announcement and has best response . Profit of firm equals the generalized price-leader profit . Function is assumed concave and is, therefore, maximized by some . The deviation to is not profitable if an only if increases at , i.e., if . Hence, must hold in equilibrium. Therefore, and are the two necessary and sufficient SPNE conditions. Condition implies .
To show that these conditions fail if
is sufficiently large, we write
as
so that
can be written as follows:
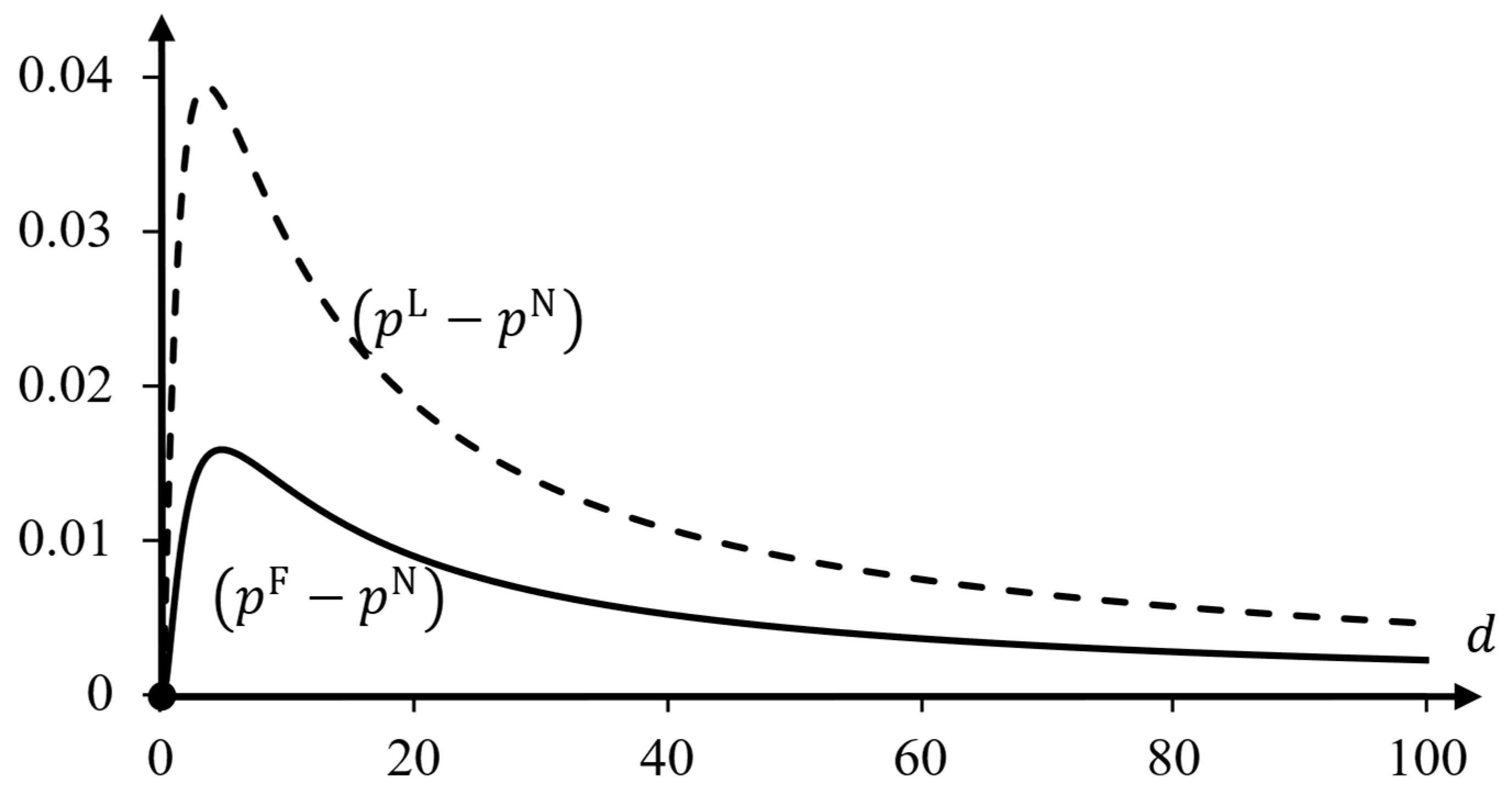
This inequality necessarily fails if . As a result, for large , the solution (if it exists) to the equation is such that , and each firm has a profitable deviation to . Hence there is some such that for any , no SPNE exist, including all symmetric equilibria, in which both firms make binding announcements. This ends the proof.□
Proof of Proposition 3. We consider symmetric and asymmetric SPNE separately.
Asymmetric SPNE.
In an asymmetric SPNE, and . In the proof of Proposition 2, a threshold has been defined in such a way that is not an SPNE announcement for some , because is not NE in the subgame following : firm has a profitable deviation to charge a price . Because any announcement also suffers from this deviation, only SPNE where may exist. Consider such an SPNE.
Let announcements followed by prices be an SPNE where . Announcement is restrictive so that . Firm must be restricted so that . If , a marginal deviation to is profitable because firm remains restricted and its profit is increasing in for . Hence, it must be that . Using , we write it as , Since the left-hand side is continuously increasing in and has a slope less than one in , the solution is unique and is continuously increasing in . Since and , exists for all . This proves part 1 of the proposition.
Symmetric SPNE.
In a symmetric SPNE, where . In the proof of Proposition 2, a threshold is defined in such a way that for any , equation has no solution that satisfies , where is a maximizer of . Thus, is the largest solution to the equation . Assume in the rest of the proof.
Since the left-hand side of the equation is continuously increasing in and has a slope less than one in , its solution is unique and is continuously increasing in . Since , equilibrium condition holds at . By continuity, it holds for where is the smallest solution to the equation . By construction, the symmetric SPNE always exists for , never exist for , and only exist for if . This ends the proof of part 2.
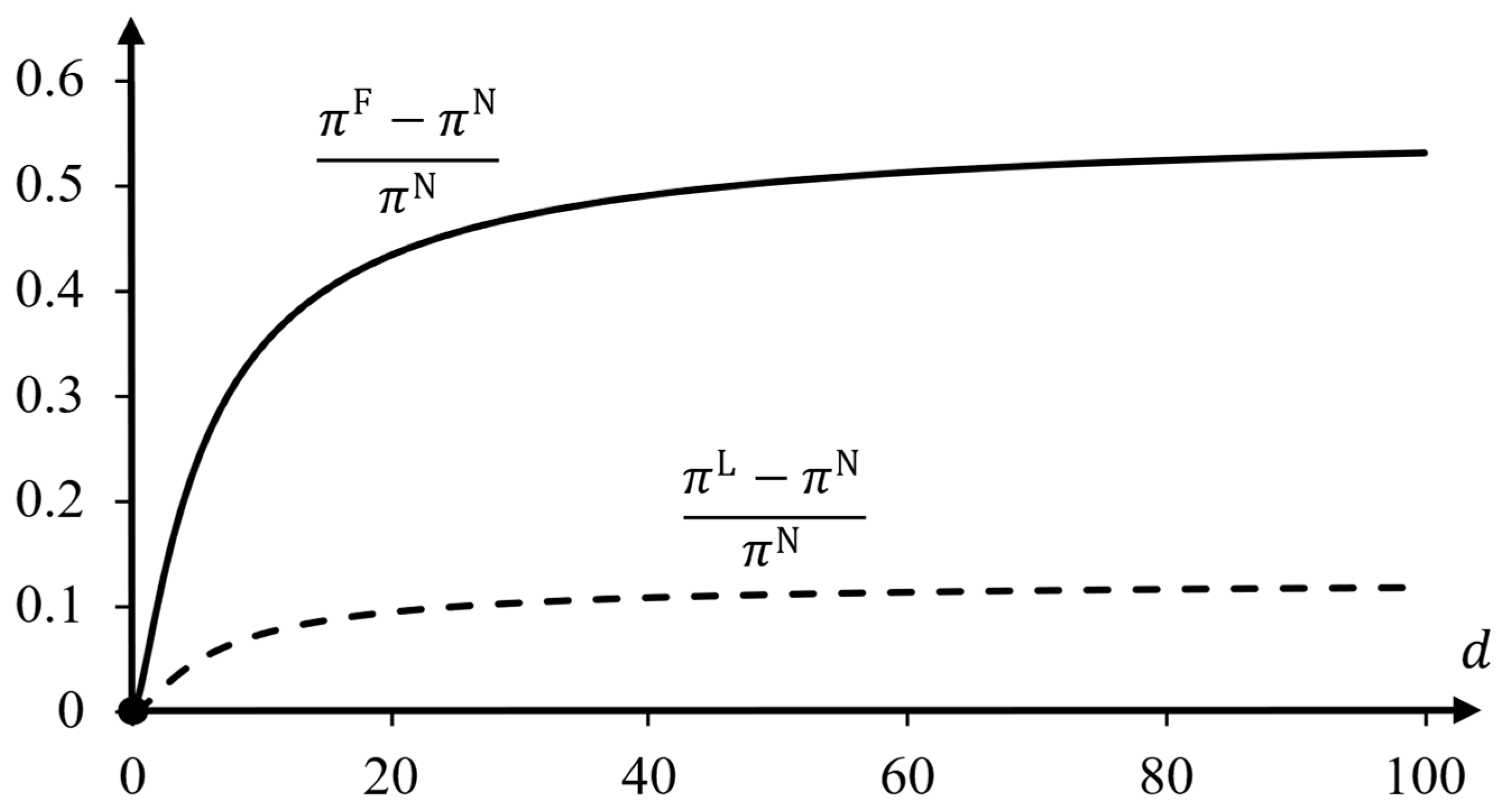
Finally, since and for , it follows that the for .Therefore, , which is the solution to , is always larger than , which is the solution to , i.e., for any . This ends the proof.□
Proof of Lemma 2. Similar to the proof of Lemma 1, for any announcement
, we define price thresholds
,
,
, and
, which depend on
and
, as follows:
If an equality above never holds, we set the corresponding price threshold to either 0 or to
. Thresholds
and
are unique because of the monotonicity of
. Threshold
is uniquely determined by
, where:
because of the monotonicity of
on
:
The inequality follows from
and
for
. Similarly, threshold
is uniquely determined by
.
When so that firm is unrestricted, . When it is restricted, then either if or if . Otherwise, firm breaks its announcement so that .
Firm is restricted when either or , and it breaks the announcement when or . When or , the firm is indifferent between these two options and, therefore, has two best responses. This occurs when .
Best response is non-decreasing in . It has a slope less than 1 for all values of when it is continuous. Therefore, NE always exists, yet is not necessarily unique. Since is piecewise continuous with three intervals of continuity at most, the number of equilibria is finite.□
Proof of Proposition 4. As in the proof of Proposition 2, both firms cannot be unrestricted in an SPNE, and cannot break their announcements. First, we consider an SPNE where only one firm is restricted, then we turn to an SPNE where both firms are restricted.
Equilibria where one firm is restricted.
Let announcements
followed by prices
be an SPNE outcome, in which firm
is restricted so that
, and firm
is unrestricted so that
. Firm
gets profit
, the profit of the Stackelberg leader. Consider a subgame after firm
deviates and announces
. According to the equilibrium selection requirement 2, if
the firm
gets profit
, otherwise firm
is either restricted and gets profit
if
is NE, or firm
breaks its announcement and gets profit
in NE
. Maximizing deviational profit
of firm
w.r.t. deviational announcement
we obtain that the best deviation is
. Therefore,
followed by prices
is an SPNE outcome if
is the NE of the subgame, i.e., if
. Hence, if
where:
then
is an SPNE announcement. The two conditions of Proposition 4 that
must satisfy in an SPNE are the same as in Proposition 2.
Figure A2 (left) provides a stylized example of firm’ best response functions for the on-path pricing subgame in an asymmetric SPNE. The second stage best-response function
is discontinuous and multi-valued at
. As a result of this discontinuity, on-path subgame has two NE:
and
. On path, NE
is played.
Equilibria where both firms are restricted.
Let announcements followed by prices be an SPNE outcome, in which and . Both firms are restricted so that and . Similar to the proof of Proposition 2, we show that .
Suppose, on the contrary, that
, as is shown on
Figure A2 (middle). The subgame has two NE:
and
; NE
is on path. Inequality
is equivalent to
where
is the point of discontinuity of
defined by
. We can now see that firm
has a profitable deviation
. Consider a subgame following announcements
The second stage best-response function
of firm
is shown by a bold dotted black curve. There are two NE in this subgame:
and
; these are the intersections of this dotted black curve
and the solid gray curve
.
According to the equilibrium selection requirement 2, NE of this subgame is
. Profit of firm
in this deviation is
because (1)
decreases with
on
, and (2)
as it can be seen on
Figure A2 (middle). Thus,
must hold for both firms.
Figure A2 (right) presents this case. The on-path subgame has two NE:
and
. On path, NE
is played.
Using function
, which is defined in the proof of Lemma 2:
we write the necessary SPNE conditions
as
and
.
In order to see that the equilibrium is necessarily symmetric, we note that for the relevant range of the arguments, i.e., for
and
so that
,
is decreasing in
(this is shown in the proof of Lemma 2) and is increasing in
. Hence, the equilibrium equation
defines
as a continuously increasing function of
, i.e.,
. Similarly,
. In equilibrium, both conditions
and
must hold. Suppose that there is an asymmetric SPNE
with
. Then, both conditions
and
must hold for
, which contradicts the monotonicity of
. Thus, the equilibrium, if it exists, is necessarily symmetric,
, and is determined by condition
:
Figure A2.
Firms’ best responses and Nash equilibria in on-path subgames for asymmetric (left picture) and symmetric (right picture) SPNE. The middle picture illustrates why cannot happen on path in a symmetric SPNE. Best responses are dashed curves that intersect at , the second stage best-response functions are thick solid curves, is the point of discontinuity of , grey (black) curves are of firm ().
Figure A2.
Firms’ best responses and Nash equilibria in on-path subgames for asymmetric (left picture) and symmetric (right picture) SPNE. The middle picture illustrates why cannot happen on path in a symmetric SPNE. Best responses are dashed curves that intersect at , the second stage best-response functions are thick solid curves, is the point of discontinuity of , grey (black) curves are of firm ().
This condition necessarily fails for . Hence, there is some such that for any , no SPNE exist, including all symmetric equilibria, in which both firms make binding announcements. This ends the proof.□
Proof of Proposition 5. We consider symmetric and asymmetric SPNE separately.
Asymmetric SPNE.
In an asymmetric SPNE, and . In the proof of Proposition 4, a threshold has been defined in such a way that is not an SPNE announcement for some . Consider an SPNE where .
Let announcements
followed by prices
be an SPNE where
and
. Firm
is restricted by
so that
and:
Profit of firm in this SPNE is , which is increasing in for . This implies that if then firm has a profitable deviation (similar to the proof of Proposition 4, we use the equilibrium selection requirement 2 for making this argument). Hence, must hold.
To show that
is well defined and increasing, we consider a function
,
, implicitly defined by:
Function
is well defined on
and is increasing:
because
for
and
so that
, and:
because of the assumption
.
An SPNE announcement is a solution to , i.e., a fixed point of , and by construction. Since , for any there is a unique solution that is increasing in . This proves part 1 of the proposition.
Symmetric SPNE.
In a symmetric SPNE,
, where
satisfies:
In the proof of Proposition 4, a threshold has been defined in such a way that for any , this equation does not define an SPNE announcement . Assume in the rest of the proof.
The symmetric SPNE announcement is the unique solution of and satisfies . The asymmetric SPNE announcement is the unique solution of , and it also satisfies . Since for , it follows that . As a result, , the fixed point of , is always smaller than , the fixed point of : . This proves part 3 of the proposition.
In a symmetric SPNE, firms’ profit is
. It can be seen in
Figure A2 (right), that any deviation to
and a marginal deviation to
result in a subgame with the unique ‘normal’ NE
. Indeed, when
increases, the point of discontinuity of the second-stage best-response function
(solid grey curve) shifts to the left. When
marginally decreases, this point of discontinuity shifts to the right, but the horizontal part of the graph shifts downwards. In both cases,
is the unique NE after these deviations. Since
:
Therefore, neither of these deviations are profitable. Only infra-marginal deviations to that lead to subgames with NE can be profitable. Deviational profit of firm is which increases on . Hence, the most profitable deviation is either if or if .
This implies that if
, then
is an SPNE announcement if and only if:
and if
, then
is an SPNE announcement if and only if:
To show that a symmetric SPNE exists for small values of
, we write:
so that
where
, because
is increasing and
. Hence, the deviation to
is not profitable for all
.
This implies that there exists a such that for all , exists and is an SPNE announcement. This proves part 2 of the proposition.
To prove part 4, we consider functions
and
, and the defining them equations
and
. At
, they result is
, and in derivatives:
due to
(this is the first-order condition that defines
) and
(this is the definition of
). Since
and
are the inverses of
and
correspondingly, their derivatives at
are infinite. Derivatives of the asymmetric equilibrium profits at
are also infinite:
Derivatives of the symmetric equilibrium profits at
are infinite as well:
This ends the proof.□
Cost Thresholds.
For the numerical example used in this paper, cost thresholds are given by: