Measuring the Difficulties in Forming a Coalition Government

Abstract

Electoral thresholds in the context of parliamentary elections are an instrument for preventing the fragmentation of parliaments and facilitate the formation of a coalition government. However, the clauses also introduce distortions and modify the equality of electoral votes in an election. In order to decide to what extent these negative effects can be accepted, it is necessary to measure the difficulties in forming a coalition government and to quantify the effects of electoral thresholds on these difficulties. For this issue, we introduce a concept based on cooperative game theory which takes into account the distribution of seats in parliament and coalition statements of parties.

1. Introduction

An electoral threshold is a provision in a proportional representation system according to which parties with below a certain share of all votes are not taken into account in the allocation of mandates. The justification for these electoral thresholds is that they prevent the fragmentation of parliaments and facilitate the formation of a coalition government [1,2,3].

In Germany, an electoral threshold of 5% exists at the federal level and at the state level.1 For the reasons stated in the first paragraph, this threshold is compatible with the Constitution (German: Grundgesetz) in the view of the Federal Constitutional Court [4]. However, this compatibility does not have to be permanent, and circumstances must be taken into account. At the level of a European election, Germany also had a 5% electoral threshold (European Election Law). In a judgment on 9 November 2011, the Federal Constitutional Court ruled that this threshold is not compatible with the Constitution, because in the European Parliament, no coalition government is formed [5]. Subsequently, the legislature introduced a 3% electoral threshold. This threshold was again rejected by the Federal Constitutional Court [6]. The question of whether and at what level an electoral threshold is compatible with the Constitution is therefore a current problem. An objective measure of when it is more difficult to form a coalition government and how this measure can be used to check whether an electoral threshold is appropriate to the circumstances in parliament is still lacking.

To establish objective measures, cooperative game theory can be used to model party voting power in parliaments. Interestingly, the voting power of a party in a parliament does not necessarily correspond to only its share of votes. The distribution of votes among the other parties is also influential. For example, if party A has 40% of the votes in a parliament and the remaining votes are distributed among an infinite number of other parties, then the voting power of A is very high. However, if only one other party exists (with 60% of the votes), the intuitive assumption is that A’s voting power will be close to zero, since it will be defeated in all votes. To determine the voting power of a party based on the vote shares of all parties, cooperative game theory provides an analytical framework with weighted voting games and voting power indices. Another factor that influences the voting power of a party is its coalition statements. These statements announce preferred coalition partners and excluded coalition parties. The statements have two effects. The initial purpose is to raise the political profile of the party. More specifically, politicians want to increase the attractiveness of their party and influence voters’ behavior. After elections, the statements influence the bargaining strengths of the parties. For example, the exclusion of coalitions might reduce the bargaining power. Some new indices or values of cooperative game theory exist that could measure a party’s voting power in parliaments under consideration of the excluded coalitions. More specifically, Ref. [7] introduced and axiomatized the EC^Sh value (excluded coalitions’ value based on the Shapley [8] value). The EC^Sh value enhances the approaches developed by [9,10] to model player preference for cooperation with some other players. In [11], the value was used to analyze the distributions of power in German coalition governments that were possible with respect to the opinion polls prior to the 2013 federal election. Reference [12] gives some general results on the effects of excluding cooperation in games using the EC^Sh value. In our analysis, in addition, we applied the Holler version [13,14] of the EC^Sh value — the EC^Ho value.

Based on parties’ levels of voting power measured by the EC^Sh value and the EC^Ho value, we applied a measure for concentration — the Herfindahl–Hirschman index [15]—to calculate the concentration of voting power. Our interpretation is that if the concentration is low, we have a parliament in which forming a government or a majority tends to be more difficult.

We performed the following analyses in our approach. First, we considered some theoretical issues with the effect of electoral thresholds on the concentration measure. This was followed by three applications of the concentration measure. First, in a simulation study that randomized election results for five parties, we showed how the concentration of voting power changes on average in all simulations when the electoral threshold is increased step by step. In the following two applications, real election results and coalition statements were considered. The electoral thresholds were constant in both cases. These applications test how our proposed concentration measures would have behaved in a historical context and to what extent they are thus a meaningful measure of the possibility of coalition formation in parliaments. The first application involves the analysis of seat distributions in the parliament of the Weimar Republic between 1918 and 1932. The second application analyzed the distribution of seats in the German federal parliament between 1990 and 2021.

The remainder of this article is structured as follows. Basic definitions of cooperative game theory and our definition of voting power concentration are presented in Section 2. In Section 3, we present our applications. Section 4 concludes the paper.

2. Basic Notation

2.1. Cooperative Game Theory

A TU (transferable utility) game is a pair $\left(N,v\right)$, where $N=\{1,2,...,n\}$ is the non-empty and finite set of players. In our paper, the parties are players. The coalitional function v assigns every subset K of N a certain worth $v\left(K\right),$ which reflects the economic abilities of K (i.e., $v:2^N\to \mathbb{R}$ such that $v\left(\varnothing \right)=0).$ The number of players in N is denoted by n or $\left|N\right|.$

A special case of a TU game are weighted voting games. In these games, a voting body is modeled. Parliaments are voting bodies. A primary task is to determine the characteristic function v. Every party $i\in N$ has a voting weight $w_i\ge 0$ representing the seats of the party.2 The coalition function v assigns the worth one (winning coalition) to a coalition $K\subseteq N$ if more than half of all seats are owned/governed by coalition K. Hence, v is given by:

$$v\left(K\right)=\left\{\begin{array}{ccc}0,\hfill & \mathrm{if}\hfill & \sum _{i\in K}{w}_i\le 0.5·\sum _{i\in N}{w}_i\hfill \\ 1,\hfill & \mathrm{if}\hfill & \sum _{i\in K}{w}_i>0.5·\sum _{i\in N}{w}_i\hfill \end{array}\right.K\subseteq N.$$

(1)

A power index is an operator $\phi$ that assigns (unique) payoff vectors to all games $\left(N,v\right)$ (i.e., uniquely determines a payoff for every party in every TU game). The Shapley value is one power index. To calculate the parties’ Shapley payoffs, rank orders $\rho$ on N are used. The set of these orders is denoted by $RO\left(N\right)$; $n!$ rank orders exist. The set of parties before i in rank order $\rho$, together with party i, is called $K_i\left(\rho \right).$ The Shapley payoff of a party i is [8]:

$${\mathrm{Sh}}_i\left(N,v\right)=\frac{1}{n!}\sum _{\rho \in RO\left(N\right)}v\left(K_i\left(\rho \right)\right)-v\left(K_i\left(\rho \right)\backslash \left\{i\right\}\right).$$

(2)

When applying this value to parliaments, the Shapley value is called the Shapley–Shubik index, and the payoff of party i is interpreted as the voting power of i [16].

Another power index was introduced by [13,14]. They utilized ideas from [17,18]. For calculating the Holler voting power, the set of minimum winning coalitions of $\left(N,v\right)$ is used.3 These coalitions are minimal, in that any party’s defection will reduce the worth of the coalition to zero. They are defined by: $M\left(N,v\right)=\left\{S\subseteq \left.N\right|v\left(S\right)=1\mathrm{and}v\left(T\right)=0\forall T⊊S\right\}.$ The voting power of party i is:

$${\mathrm{Ho}}_i\left(N,v\right)=\frac{C_i\left(N,v\right)}{{\sum }_{j\in N}{C}_j\left(N,v\right)}\mathrm{with}{C}_i\left(N,v\right)=\sum _{K\in M\left(N,v\right),i\in K}v\left(K\right)$$

(3)

whereby $C_i\left(N,v\right)$ measures the number of times a party i is a member of a minimum winning coalition. Since it is common in German parliaments to form coalition governments that are minimum winning coalitions, the Holler power index is an appropriate power index.

The Shapley index and the Holler index differ in two ways. First, voting power with respect to the Holler index considers only minimum winning coalitions. Second, the Holler index weights the marginal contributions of parties equally. Therefore, considering both indexes provides coverage for a variety of approaches to determining voting power and allows us to place our results on broader footing. A more detailed survey of power indices is presented in [20,21,22,23,24].

As mentioned in the Introduction, prior to elections, parties make coalition statements and exclude coalitions with certain parties. These statements prevent coalitions and are modeled below. The set of i’s excluded coalition partners is denoted by $E_i.$ A party excludes only coalitions with single parties; i.e., if party A can cooperate with both party B and party C, it cannot exclude cooperation in a tripartite alliance $\left\{A,B,C\right\}$; $\left|K\right|=1\forall$$K\in E_i.$ The set of coalitions that are not allowed based on $E_i$ is called $X_i,$$X_i:=\left\{\left.K\subseteq N\right|K\backslash \left\{i\right\}\in E_i\right\}$ with $\left|K\right|=2.$ If i does not cooperate with $j,$ we have $X_i\cap X_j=\left\{i,j\right\};$ i.e., if a party i does not cooperate with j, j cannot cooperate with $i.$ All inadmissible coalitions are denoted by $\mathsf{\Gamma }:=\left\{\left.K\subseteq N\right|\exists S\in X_j,j\in N,\mathrm{with}S\subseteq K\right\}.$ Thus, the admissible coalitions in the game $\left(N,v\right)$ are $\mathsf{\Omega }:=\left\{\left.K\subseteq N\right|K\notin \mathsf{\Gamma }\right\}.$ A game with excluded coalitions is a tuple $\left(N,v,\mathsf{\Gamma }\right).$ The primary idea of the EC value is that only admissible coalitions are considered [7]. When calculating the EC value based on the Shapley value, we have:4

$${\mathrm{EC}}_i^{\mathrm{Sh}}\left(N,v,\mathsf{\Gamma }\right)=\frac{1}{n!}\sum _{\rho \in RO\left(N\right),K_i\left(\rho \right)\in \mathsf{\Omega }}v\left(K_i\left(\rho \right)\right)-v\left(K_i\left(\rho \right)\backslash \left\{i\right\}\right).$$

(4)

In the case of $\mathsf{\Gamma }=⌀,$ we have EC${}_i$^Sh$\left(N,v,\mathsf{\Gamma }\right)$ = Sh${}_i$$\left(N,v\right).$ The sum of the payoffs does not have to equal 1, unlike the Shapley value. This expresses the fact that coalition exclusions make it more difficult to find a majority. For example, if two parties, each with more than 25% but less than 50% of the seats in parliament, exclude any coalition with other parties, then the total EC payoffs will be zero.

Similarly, we obtain the Holler version of the EC value by modifying $C_i\left(N,v\right)$:

$$C_i\left(N,v,\mathsf{\Gamma }\right)=\sum _{K\in M\left(N,v\right),i\in K,K\cup \left\{i\right\}\in \mathsf{\Omega }}v\left(K\right).$$

(5)

We obtain [11]:

$${\mathrm{EC}}_i^{\mathrm{Ho}}\left(N,v,\mathsf{\Gamma }\right)=\frac{C_i\left(N,v,\mathsf{\Gamma }\right)}{{\sum }_{j\in N}{C}_j\left(N,v\right)}.$$

(6)

In addition, for this value, the parties’ payoffs need not to sum up to $1.$ Again, in the case of $\mathsf{\Gamma }=⌀,$ we have EC ${}_i^{\mathrm{Ho}}\left(N,v,\mathsf{\Gamma }\right)={\mathrm{Ho}}_i\left(N,v\right).$ Similarly to our explanation of the ${\mathrm{EC}}^{\mathrm{Sh}}$ value, this means that exclusion of coalitions with parties holding seats will lower the concentration of voting power (see next Section).

The payoffs of the two values can be interpreted as a priori voting power in parliaments without the existence of a governing coalition. Decreasing payoffs of the parties mean that their influence on voting results decreases. Due to coalition exclusions, it is possible that $v\left(N\right)=1$ is no longer distributed to the parties. This can be interpreted as a difficulty in forming a governing coalition. The extreme case — ${\sum }_{i\in N}{\mathrm{EC}}_i^{\mathrm{Sh}}\left(N,v,\mathsf{\Gamma }\right)=0$ or ${\sum }_{i\in N}{\mathrm{EC}}_i^{\mathrm{Ho}}\left(N,v,\mathsf{\Gamma }\right)=0,$ respectively — then means that it is no longer possible to form a coalition government.

2.2. Concentration of Voting Power

The idea of our article is that the concentration of the a priori voting power of parties in a parliament operationalizes the capacity of the parliament to act. Concretely, we use the Herfindahl–Hirschman index [15] to determine the concentration of voting power of political parties. If this is low, we have a parliament in which forming a government or a majority tends to be more difficult, and possibly, more parties are represented in a coalition government. In such cases, the electoral threshold can serve to reduce the number of parties in parliament and increase the concentration of voting power.

In other applications, the Herfindahl–Hirschman index is used, for example, in sales markets to determine market penetration. In [26], the Herfindahl–Hirschman index is used to determine the concentration of voting power of the owners of firms. The Herfindahl–Hirschman index has been applied to measure the political competition or the concentration of political power in parliaments based on the seats distribtution of parties in [27,28,29,30]. Maux [31] applied the Herfindahl–Hirschman index to measure the political power within a coalition government after an election.

In our approach, we determine the concentration of voting power in a parliament, $H,$ as:

$$H^{\mathrm{Sh}}={\displaystyle \sum _{i=1}^n}{\left[{\mathrm{EC}}_i^{\mathrm{Sh}}\left(N,v,\mathsf{\Gamma }\right)\right]}^2$$

(7)

respectively

$$H^{\mathrm{Ho}}={\displaystyle \sum _{i=1}^n}{\left[{\mathrm{EC}}_i^{\mathrm{Ho}}\left(N,v,\mathsf{\Gamma }\right)\right]}^2.$$

(8)

Based on Equations (4) and (6) we deduce some first insights on the Herfindahl–Hirschman index:

Corollary 1. 

Iff there is a party $i,$$i\in N,$ with $w_i>0.5·{\sum }_{j\in N}{w}_j$ we have $H^{\mathrm{Sh}}=H^{\mathrm{Ho}}=1$ regardless of the excluded coalitions.

In these cases, finding a majority or forming a coalition government is most readily accomplished; we obtain for party i${\mathrm{EC}}_i^{\mathrm{Ho}}\left(N,v,\mathsf{\Gamma }\right)={\mathrm{EC}}_i^{\mathrm{Sh}}\left(N,v,\mathsf{\Gamma }\right)=1,$ since only coalitions with i are winning coalitions.

Corollary 2. 

Iff there are n parties with $w_i=\frac{1}{n}$ and $E_i=⌀$ for all $i\in N$ we have

$$H^{\mathrm{Ho}}=\stackrel{n}{{\sum }_{i=1}}{\left[{\mathrm{EC}}_i^{\mathrm{Ho}}\left(N,v,\mathsf{\Gamma }\right)\right]}^2=n·{\left(\frac{1}{n}\right)}^2=\frac{1}{n}$$

(9)

respectively:

$$H^{\mathrm{Sh}}=\frac{1}{n}.$$

(10)

In the case of $n\to \infty$, we have $H^{\mathrm{Ho}}\to 0$ and $H^{\mathrm{Sh}}\to 0.$

In this case, all parties are symmetric, and worth is distributed among all parties equally. Hence, we have ${\mathrm{EC}}_i^{\mathrm{Ho}}\left(N,v,\mathsf{\Gamma }\right)={\mathrm{EC}}_i^{\mathrm{Sh}}\left(N,v,\mathsf{\Gamma }\right)=\frac{1}{n}$ for all $i\in N.$

3. Case Studies

3.1. Simulation

With our simulation study, we aim to show the effect of electoral thresholds on the two concentration measures. The exclusion of coalition parties is not considered, since options for this are very diverse.5 To illustrate our simulation, we show two examples:6

Example 1. 

We have five parties with voting results $s_1=0.06,$$s_2=s_3=0.16,$ $s_4=0.17$ and $s_5=0.45.$ The electoral threshold is in a first step $b=0.05$; hence, all five parties are in the parliament, and the distribution of seats corresponds to the voting result. The Shapley payoffs are ${\mathrm{Sh}}_1\left(N,v\right)={\mathrm{Sh}}_2\left(N,v\right)={\mathrm{Sh}}_3\left(N,v\right)={\mathrm{Sh}}_4\left(N,v\right)=0.1$ and ${\mathrm{Sh}}_5\left(N,v\right)=0.6.$ From this, we have $H^{\mathrm{Sh}}=0.4.$ Raising the threshold to $b=0.07$ means that party 1 is no longer represented in the parliament. The seats in parliament are distributed with respect to the voting result of the four bigger parties. With rounding, we obtain $w_2=w_3=0.17,$ $w_4=0.18$ and $w_5=0.48.$ For this seat distribution, we have ${\mathrm{Sh}}_2\left(N,v\right)={\mathrm{Sh}}_3\left(N,v\right)={\mathrm{Sh}}_4\left(N,v\right)=0.1667$, ${\mathrm{Sh}}_5\left(N,v\right)=0.5$ and $H^{\mathrm{Sh}}=0.3333.$ Raising the threshold gives a lower concentration of voting power with respect to the Shapley version of concentration of voting power.

Example 2. 

Five parties are involved with voting results $s_1=0.06,$$s_2=s_3=0.19,$ $s_4=0.26$ and $s_5=0.30.$ Again, the electoral threshold is in the first step $b=0.05$; hence, all five parties are in the parliament, and the distribution of seats corresponds to the voting results. The Shapley payoffs are ${\mathrm{Sh}}_1\left(N,v\right)={\mathrm{Sh}}_2\left(N,v\right)={\mathrm{Sh}}_3\left(N,v\right)=0.1333$ and ${\mathrm{Sh}}_4\left(N,v\right)={\mathrm{Sh}}_5\left(N,v\right)=0.3.$ From this, we have $H^{\mathrm{Sh}}=0.2333$. By raising the threshold to $b=0.07,$ the distribution of seats is (with rounding) $w_2=w_3=0.20,$ $w_4=0.28$ and $w_5=0.32.$ For this seat distribution, we have ${\mathrm{Sh}}_2\left(N,v\right)={\mathrm{Sh}}_3\left(N,v\right)={\mathrm{Sh}}_4\left(N,v\right)=0.1667$, ${\mathrm{Sh}}_5\left(N,v\right)=0.5$ and $H^{\mathrm{Sh}}=0.3333.$ Raising the threshold gives a higher concentration of voting power with respect to the Shapley version of the concentration of voting power.

We simulated election results with five parties 100,000 times. For this, we have random votes $s_i$ assuming a uniform distribution of votes between 0 and 1 with ${\sum }_{i\in N}{s}_i=1.$ For each voting result, we consider electoral thresholds between 0% and 20% in steps of 0.25. For each level of the electoral threshold, we determined which party is represented in parliament and calculate the share of votes in parliament for each party. From this, parties’ Shapley payoffs and Holler payoffs were computed. These were used to calculate the concentration measures $H^{\mathrm{Ho}}$ and $H^{\mathrm{Sh}}.$ Figure 1 shows our average results for all simulations. Concentration with respect to the Holler index starts slightly below that of the Shapley one; both increase exponentially with the electoral threshold. Our results imply that the higher the electoral threshold, the stronger its impact on the concentration of voting power in parliament. Therefore, in our estimation, the legislature should carefully consider the level when introducing electoral thresholds and increase the requirements for consideration as the level of the threshold increases.

With respect to our examples, there is no case where an increase in the threshold results in a reduction of the concentration of voting power with respect to the Holler version of concentration of voting power. For the Shapley version, in 2.611 simulations, an increase in the threshold at some level reduces the concentration of voting power. 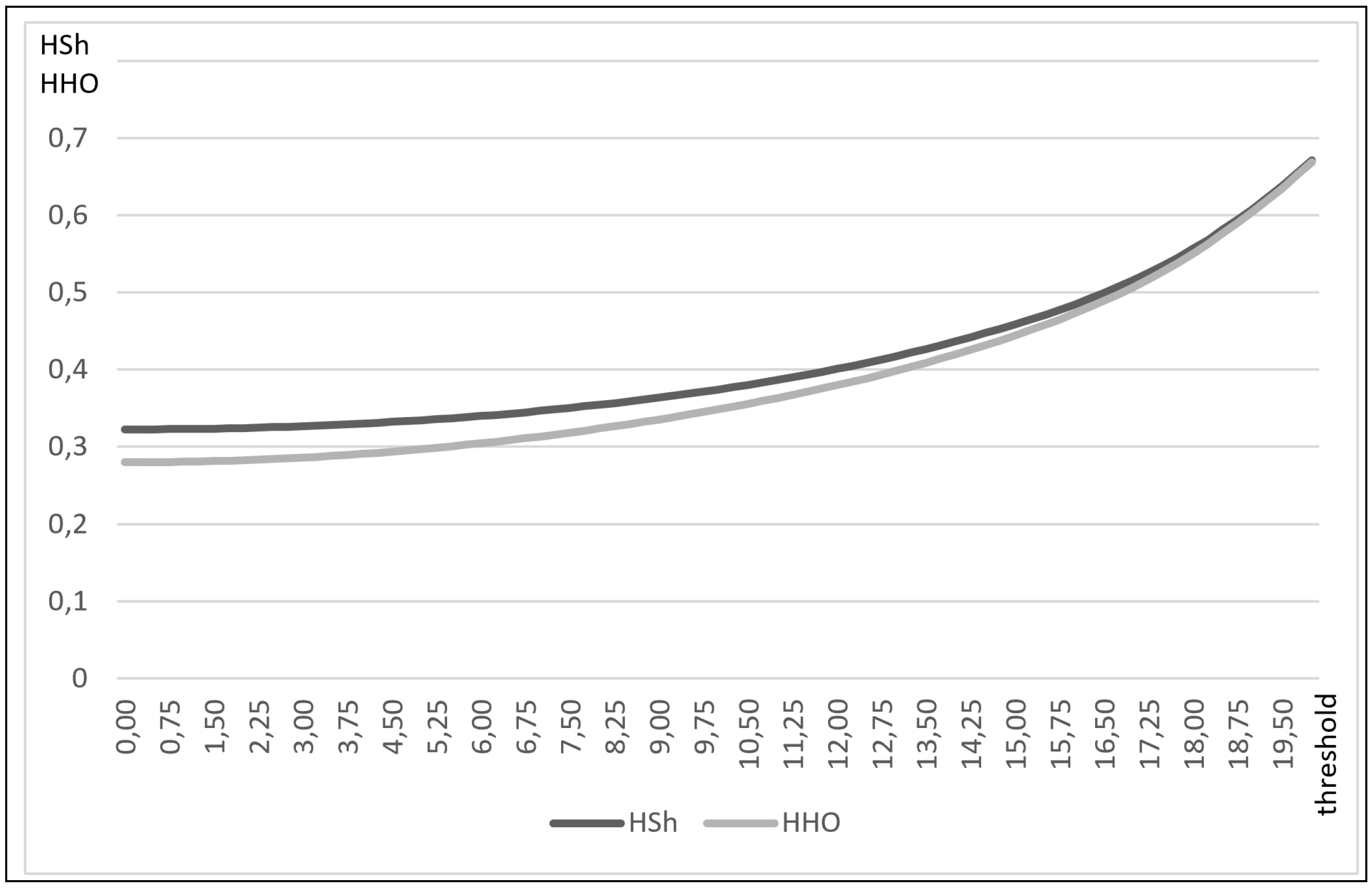Based on these results, the Holler version could be preferred for measuring the difficulties in forming a coalition government if an increase in the threshold should always lead to an increase in voting power concentration.

3.2. Weimar Republic Parliament: 1918–1932

In this section, we apply two concentration measures to the parliament of the Weimar Republic. We consider the seat distribution in parliament and the main excluded coalitions of the parties. As the proportion of parties uninterested in democratic cooperation increased over time, it is generally acknowledged that the difficulties of forming a coalition government increased. We aim to show that the two concentration measures reflect these increasing difficulties.

The electoral system of the Weimar Republic was a proportional representation system. In principle, a party received one seat in the Imperial Diet (German: Reichstag) for every 60,000 votes cast.7. An electoral threshold did not exist. Reforms of the electoral system were discussed throughout the existence of the Weimar Republic. One proposal was to increase the number of votes required per seat to 75,000. In addition, the aggregation of votes at the higher level was suggested to be capped. Other approaches included the introduction of elements of majority voting and electoral thresholds. The introduction of an electoral threshold would have increased the share of seats held by parties on the fringes of the party spectrum due to the high fragmentation of parties in the middle of the party spectrum. Therefore, this idea was rejected in the run-up to the 1930 election [32,33,34,35,36,37]. Decisions in the Imperial Diet were generally made by simple majority (exceptions existed, for example, for constitutional changes).

The entirety of excluded coalitions in the Weimar Republic is quite complex [38,39,40]. We concentrate on the main exclusions that demonstrate the application of our approach—i.e., we focus on the anti-republican parties on the left and right of the party spectrum, respectively, which were not seriously interested in parliamentary work. Concretely, we assume that the KPD (Communist Party of Germany) excluded all coalitions with other parties (with the exception of a possible cooperation with the USPD), and the NSDAP (National Socialist German Workers’ Party)8 excluded all possible coalitions.9 The results of our calculations are shown in

Table 1. Results for the Weimar Republic parliament in 1918–1932.

	1920	May 1924	Dec 1924	1928	1930	Jul 1932	Nov 1932
$H^{\mathrm{Sh}}$	0.042264	0.007370	0.011511	0.013023	0.003115	0	0
$H^{\mathrm{Ho}}$	0.066116	0.003652	0.006036	0.003500	0.004303	0	0

. For the Shapley version of concentration of voting power, the results decrease from the election in 1920 to the election in May 1924. For the next two elections, the results increase a little bit. After this, the values decline, and starting from election in July 1932, the values are zero. Additionally, for $H^{\mathrm{Ho}}$, the highest value was calculated for 1920. Similarly to the Shapley version after this election, the concentration of voting power decreased for the election in May 1924 and increased for the election in December 1924. Differently to $H^{\mathrm{Sh}}$, the results decrease for 1928 and increase for 1930. Finally, both approaches reflect the fact that parliamentary majorities were no longer possible without the KPD and the NSDAP in both elections in 1932. Thus, our approach does well in modeling the problems of the Weimar Republic. The extent to which an electoral threshold would have alleviated the problems remains to be seen, since the parties were particularly fragmented at the center of the party spectrum.

To show the effect of coalitional exclusions, we computed a counterfactual scenario in which no coalitional exclusions existed. The results are shown in

Table 2. Results for Weimar Republic parliament in 1918–1932, variation.

	1920	May 1924	Dec 1924	1928	1930	Jul 1932	Nov 1932
$H^{\mathrm{Sh}}$	0.169079	0.15092	0.176722	0.203582	0.156689	0.327982	0.271113
$H^{\mathrm{Ho}}$	0.110172	0.078634	0.085051	0.067417	0.067698	0.074986	0.076100

. The resulting concentration of voting power was higher than when coalition exclusions were taken into account. The strengthening of the anti-republican parties on the left and right of the party spectrum leads to an increase in the concentration of voting power in this calculation, since the fragmentation of the parliament decreases. Hence, not taking coalition exclusions into account would suggest that coalition formation was not affected toward the end of the Weimar Republic.

More fine-grained modeling of the preferences of parties in the Weimar Republic parliament was applied [41] for the 1919 election and the already undemocratic 1933 election. In their article, the parties are positioned on a spectrum and the values of the winning coalitions are weighted by the distances of the parties. After this, the Banzhaf–Penrose index [42] and intensity function values [43] are applied to them. In principle, the Shapley–Shubik index and the Holler index could also be applied to this weighted values of coalitions. The prerequisite to apply the approach by [41] is a detailed analysis of the positions and distances of the parties in the political spectrum of the Weimar Republic. Moreover, this method assumes that parties are positioned on a linear spectrum. However, cooperation between parties may be impossible despite the political proximity of them — e.g., if one party gets a splinter party (e.g., SPD and USPD during the German Empire, or SPD and WASG in 2004 in Germany).

3.3. German Federal Parliament: 1994–2021

In this final application, we analyze the German federal parliament from 1994 to 2021. Again, we consider the seat distribution in parliament and the main excluded coalitions of parties. As the number of parties in parliament has increased over the years and the AfD, a party with which the other parties do not want to form a coalition, has recently entered the parliament, the latest protracted negotiations to form a coalition government show that the difficulties of forming government have increased here as well. Again, we aim to show that the two concentration measures reflect these increasing difficulties. In addition, it is generally accepted that it was easier to form coalition governments in the period from 1994 to 2021 than at the end of the Weimar Republic — this should also be reflected in the concentration measures.

The election to the Bundestag (Federal Parliament) is regulated by law in the Federal Election Act (German: Bundeswahlgesetz). It is a personalized proportional representation election. Voters elect a direct candidate for their constituency with their so-called first vote. With the second vote, the state list of a party is elected. The distribution of seats in the Bundestag is based on the share of the second vote. If a party receives more direct candidates than the number of seats it is entitled to according to the two-vote share, these are allocated to it as overhang mandates. To compensate for the resulting shift in the proportions in the parliament, the other parties receive compensatory mandates.10 In § 6 (3) of the Federal Election Act, an electoral threshold of 5% is defined. Only parties whose share of the second vote is higher than this threshold are taken into account in the allocation of seats via the state lists. Alternatively, this quorum can be exceeded if a party receives three direct mandates.11 Detailed specifications for the 2021 election are described by [57], for example. The coalition statements for the parties are shown in

Table 3. Excluded coalition partners—German federal parliament.

${\mathit{E}}_{\mathit{CDU}},{\mathit{E}}_{\mathit{FDP}}$	${\mathit{E}}_{\mathit{SPD}},$${\mathit{E}}_{\mathit{Grüne}}$	${\mathit{E}}_{\mathit{Linke}}$	${\mathit{E}}_{\mathit{AfD}}$
{Linke,AfD}	{AfD}	{CDU,FDP,AfD}	{SPD,Grüne,Linke}

. A historical overview and analysis of coalition statements of German parties are presented in [58,59,60,61,62]. Decisions in the Federal Parliament are generally made by simple majority (exceptions existed, for example, for constitutional changes).

The results of our calculations are shown in

Table 4. Results for German federal parliament 1994–2021, variation.

	1994	1998	2002	2005	2009	2013	2017	2021
$H^{\mathrm{Sh}}$	0.083333	0.107778	0.152778	0.071667	0.076389	0.125000	0.015000	0.016111
$H^{\mathrm{Ho}}$	0.259207	0.259207	0.333267	0.067500	0.074059	0.160462	0.005410	0.040000

. The concentration of voting power for both concepts increased from 1994 to 2002. For 2005, both concepts produced their lowest values since 1994. For the elections in 2009 and 2013, the results increased. The last two elections in 2017 and 2021 resulted in low $H^{\mathrm{Ho}}$ and low $H^{\mathrm{Sh}}$. This was due to the higher number of parties in the Bundestag and the existence of coalition exclusions from the parties. In particular, the rather high share of the AfD, combined with the coalition exclusion of the other parties, makes it more difficult to form a government and lowered the results for $H^{\mathrm{Ho}}$ and $H^{\mathrm{Sh}}$. The first three-party coalition in government in 2021 was a result of this development. Compared to the Weimar Republic, however, the results (with limitations in 1920) are higher. This fits with the common opinion that coalition formation was much more difficult toward the end of the Weimar Republic than in the current situation in the German federal parliament. From this perspective, the results for $H^{\mathrm{Ho}}$ and $H^{\mathrm{Sh}}$ seem plausible according to both developments in the German Federal Parliament and compared to the Weimar Republic.

To show the effect of coalition exclusions, we also present an alternative calculation here. The exclusions are shown in Table 5. They are based on the traditional party alliances in the 1990s in Germany, in which a conservative bloc of CDU and FDP opposed the bloc of SPD and Grüne. A coalition with Linke was also ruled out by the SPD and the Grüne. Only the following developments in the last few years allow for fewer exclusions, as shown in Table 3 [63,64]:

• In Thuringia, starting in 2014, the SPD became the junior partner in a coalition government with Linke. In addition, there was the first coalition with SPD and Linke in a western state (Bremen) since 2019.
• CDU and Grüne govern together in Hesse (after elections in 2013 and 2018), Baden-Wuerttemberg (since 2016 the CDU is a junior partner), Saxony-Anhalt (since 2016), Schleswig-Holstein (since 2017), Brandenburg and Saxony (both since 2019). In Saxony and Saxony-Anhalt, cooperation between CDU and Grüne is necessary to form a coalition government.
• Grüne and FDP have been in a coalition government in Schleswig-Holstein (since 2017) and at the federal level since 2021.

As is to be expected, the concentration of voting power decreases as more excluded coalitions are taken into account (see results in Table 6). Hence, in this example as well, the two measures of voting power concentration model the situation appropriately.

Table 5. Excluded coalition partners—German federal parliament.

${\mathit{E}}_{\mathit{CDU}},{\mathit{E}}_{\mathit{FDP}}$	${\mathit{E}}_{\mathit{SPD}},$${\mathit{E}}_{\mathit{Grüne}}$	${\mathit{E}}_{\mathit{Linke}}$	${\mathit{E}}_{\mathit{AfD}}$
{Linke,AfD,Grüne}	{AfD,Linke,FDP}	{CDU,FDP,AfD,SPD,Grüne}	{SPD,Grüne,Linke}

Table 5. Excluded coalition partners—German federal parliament.

${\mathit{E}}_{\mathit{CDU}},{\mathit{E}}_{\mathit{FDP}}$	${\mathit{E}}_{\mathit{SPD}},$${\mathit{E}}_{\mathit{Grüne}}$	${\mathit{E}}_{\mathit{Linke}}$	${\mathit{E}}_{\mathit{AfD}}$
{Linke,AfD,Grüne}	{AfD,Linke,FDP}	{CDU,FDP,AfD,SPD,Grüne}	{SPD,Grüne,Linke}

Table 6. Results for German federal parliament in 1994–2021.

	1994	1998	2002	2005	2009	2013	2017	2021
$H^{\mathrm{Sh}}$	0.015000	0.015000	0.015000	0.005000	0.015000	0.005000	0.002222	0.001111
$H^{\mathrm{Ho}}$	0.074074	0.074074	0.166667	0.005000	0.074074	0.024691	0.001543	0.002500

Table 6. Results for German federal parliament in 1994–2021.

	1994	1998	2002	2005	2009	2013	2017	2021
$H^{\mathrm{Sh}}$	0.015000	0.015000	0.015000	0.005000	0.015000	0.005000	0.002222	0.001111
$H^{\mathrm{Ho}}$	0.074074	0.074074	0.166667	0.005000	0.074074	0.024691	0.001543	0.002500

4. Conclusions

In this article, we presented an approach based on concepts of cooperative game theory that is able to measure the difficulties in forming a coalition government. Our simulation study and the application to two parliaments showed that the approach yields plausible results. In our simulation study with five parties, the concentration of voting power increases on average when the electoral threshold is increased step by step. For the Weimar Republic’s parliament, the two concentration measures reflect the generally acknowledged increase in the difficulty of forming a coalition government. The same result was obtained for the German federal parliament. In addition, the results for the concentration measures for the German federal parliament are higher than our results for the Weimar Republic parliament. This result fits in with the general assessment that forming a coalition government was more difficult in the Weimar Republic than in Germany between 1994 and 2021.

Electoral thresholds are enacted by the legislature and reviewed by courts to reduce these difficulties in forming coalitions. Thus, our approach can be one element to operationalize these difficulties and could be a tool for courts in reviewing electoral thresholds. Of course, the methodology presented should not be used for analysis alone and should be complemented by existing analyses. These analyses include demoscopic analyses, which provide statements on election results, and politological analyses, which examine excluded coalitions between parties. Another line of research could be a comparison of our approach to measure the difficulties in forming a coalition government with other measures used in the literature, such as the number of parties in a coalition government [65,66], the number of seats above majority quorum [67], the margin of victory [68,69] and the volatility of votes shares over time [70]. In addition, our approach could be applied for other analyses in which the difficulties of coalition formation or political competition in parliament are relevant, e.g., budget deficits [27,69,71,72,73,74], tax revenue [75], fiscal performance [30,76,77,78] and expenditure efficiency [29,79,80].

For further research, one objective could be an investigation of flexible electoral thresholds that are determined on the basis of objective criteria. It is conceivable that a certain concentration of voting power in parliament should be set as a target before the election and that this is achieved with a flexible threshold after the election, knowing the election results.12 This would allow electoral thresholds to be removed from the legislature as a political tool.

Methodologically, our analysis was limited, for example, with respect to the modeling of minority governments. In some countries, such as Denmark and Norway, it is common that a government does not have a majority of overall seats in the legislature. This situation could not be modeled satisfactorily with cooperative game theory. Another constraint in our model is that parties can only completely exclude cooperation. In reality, however, more complex constellations exist. Possibly, the approach of overlapping coalitions can be adapted here for better modeling [82]. Additionally, our model could be enhanced by considering situations in which parties make statements that they will not be a junior partner in a coalition government. To model this, decomposition of the n-person game using a $2^n\times 2^n$ matrix could be used [25,83]. In addition, voting power indices that take into account parties’ preferences on coalitions in a more detailed way [41,43] could be the basis for the Herfindahl–Hirschman index.

5. Data

We add a list of supplementary information in

Table 7. Weimar Republic parliament, 1918–1932.

	1920	May 1924	Dec 1924	1928	1930	Jul 1932	Nov 1932
Bay. BB	4	3
Bay BM			5
BVP	21	16	19	17	19	22	20
CNBL				9	19
CSVD					14	3	5
DBP				8	6	2	3
DDP	39	28	32	25
DHP	5	5	4	4	3		1
DL						1
DNVP	71	95	103	73	41	37	51
DSP		4
DStP					20	4	2
DVP	65	45	51	45	30	7	11
KPD	4	62	45	54	77	89	100
KVP					4
Landbund					3	2
Landliste		10	8
NSDAP				12	107	230	196
NSFP		32	14
RDM				23	23	2	1
RLB				3
RVA				2		1
SL				2
SPD	103	100	131	153	143	133	121
TL							1
USPD	83
WBW							2
WP		7	12
Z	64	65	69	61	68	75	70

,

Table 8. Weimar Republic parliament, 1918–1932: abbreviations.

Abb.	Full Party Name
Bay. BB	Bayerischer Bauernbund
Bay BM	Bayerischer Bauern- und Mittelstandsbund
BVP	Bayerische Volkspartei
CNBL	Christlich-Nationale Bauern- und Landvolkpartei
CSVD	Christlich-Sozialer Volksdienst
DBP	Deutsche Bauernpartei
DDP	Deutsche Demokratische Partei
DHP	Deutsch-Hannoversche Partei
DL	Deutsches Landvolk
DNVP	Deutschnationale Volkspartei
DSP	Deutschsoziale Partei
DStP	Deutsche Staatspartei
DVP	Deutsche Volkspartei
KPD	Kommunistische Partei Deutschlands
KVP	Konservative Volkspartei
Landbund	Landbund
Landliste	Landliste
NSDAP	Nationalsozialistische Deutsche Arbeiterpartei
NSFP	Nationalsozialistische Freiheitsbewegung
RDM	Reichspartei des deutschen Mittelstandes
RLB	Reichslandbund
RVA	Reichspartei für Volksrecht und Aufwertung
SL	Sächsisches Landvolk
SPD	Sozialdemokratische Partei Deutschlands
TL	Thüringer Landbund
USPD	Unabhängige Sozialdemokratische Partei Deutschlands
WBW	Württembergischer Bauern- und Weingärtnerbund
WP	Wirtschaftspartei des Deutschen Mittelstandes
Z	Deutsche Zentrumspartei

,

Table 9. German federal parliament, 1994–2021.

	1994	1998	2002	2005	2009	2013	2017	2021
CDU/CSU	294	245	248	226	239	311	246	196
FDP	47	43	47	61	93		80	92
SPD	252	298	251	222	146	193	153	206
Grüne	49	47	55	51	68	63	67	118
PDS/Linke	30	36	2	54	76	64	69	39
AfD							94	83
SSW								1

and

Table 10. German federal parliament, 1994–2021: abbreviations.

Abb.	Full Party Name
CDU/CSU	Christlich Demokratische Union Deutschlands/Christlich-Soziale Union in Bayern
FDP	Freie Demokratische Partei
SPD	Sozialdemokratische Partei Deutschlands
Grüne	Bündnis 90/Die Grünen
PDS/Linke	Partei des Demokratischen Sozialismus/Die Linke
AfD	Alternative für Deutschland
SSW	Südschleswigsche Wählerverband

in order to explain the previous contents.