Do Output-Dependent Prizes Alleviate the Sabotage Problem in Tournaments?
Abstract
1. Introduction
2. The Game-Theoretical Model
2.1. Case (1): Fixed-Prize Tournament
2.2. Case (2): Variable-Prize Tournament Where Prizes Depend on Joint Output
3. Experimental Design and Procedure
4. Empirical Results
4.1. Variables and Descriptives
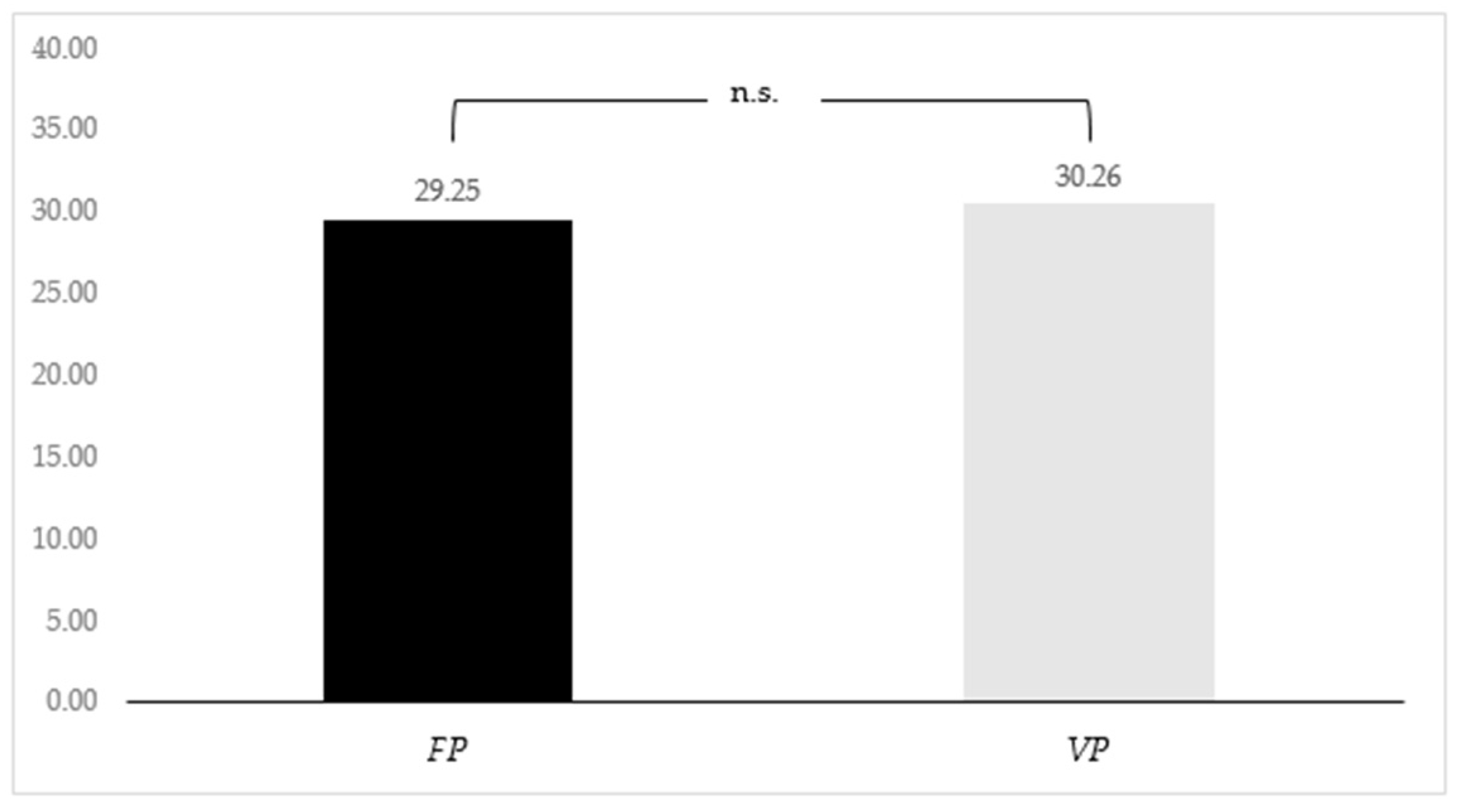
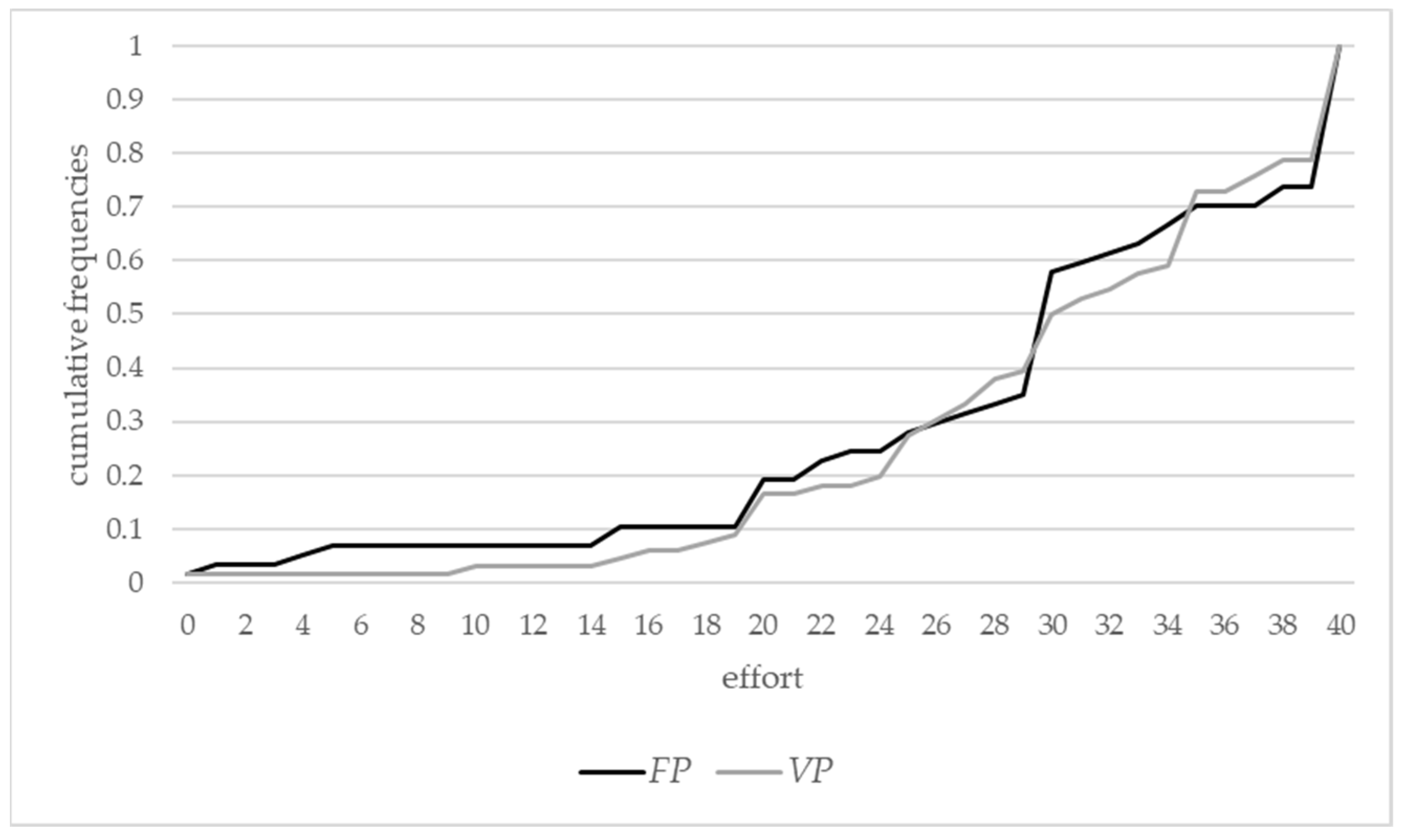
4.2. Effort
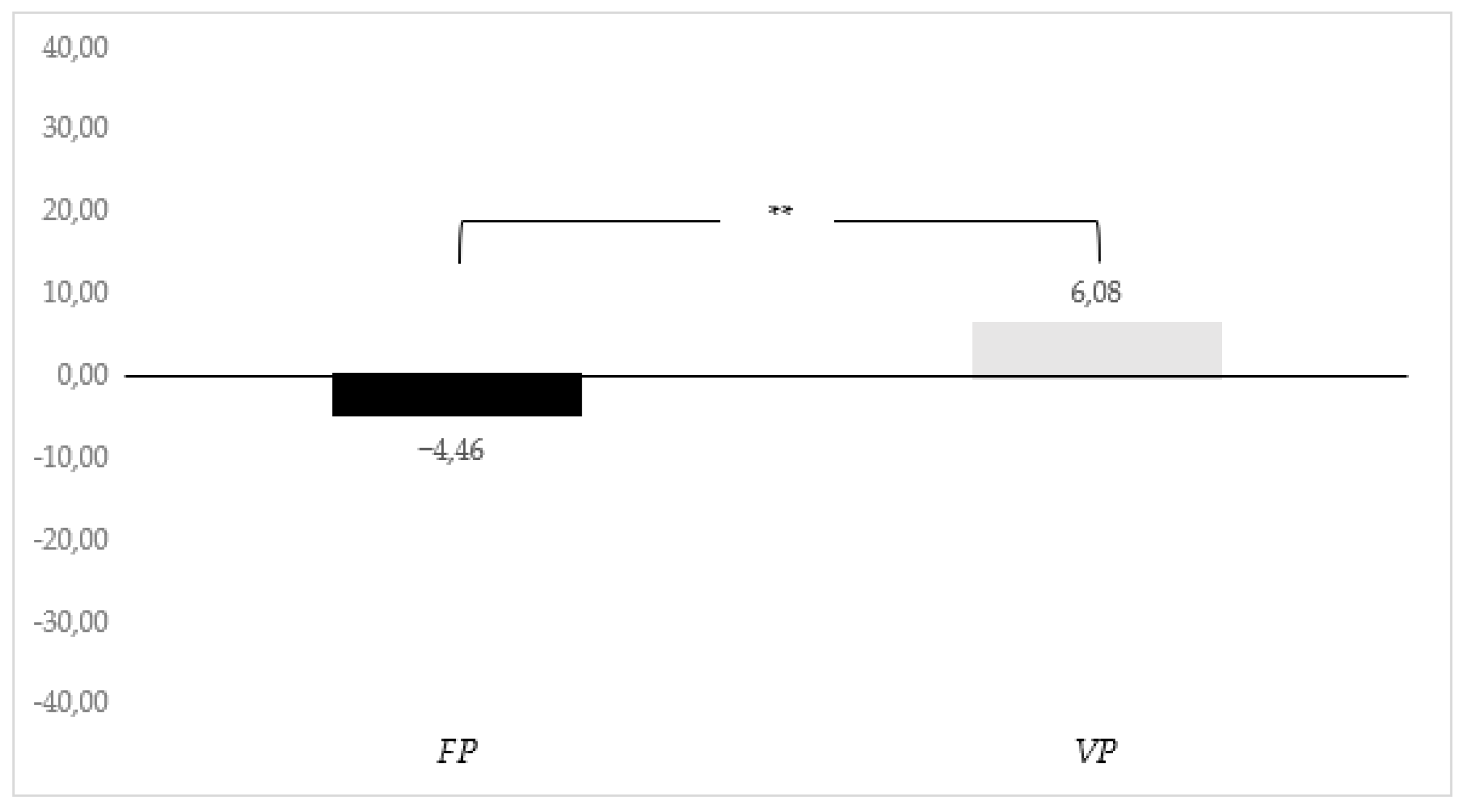
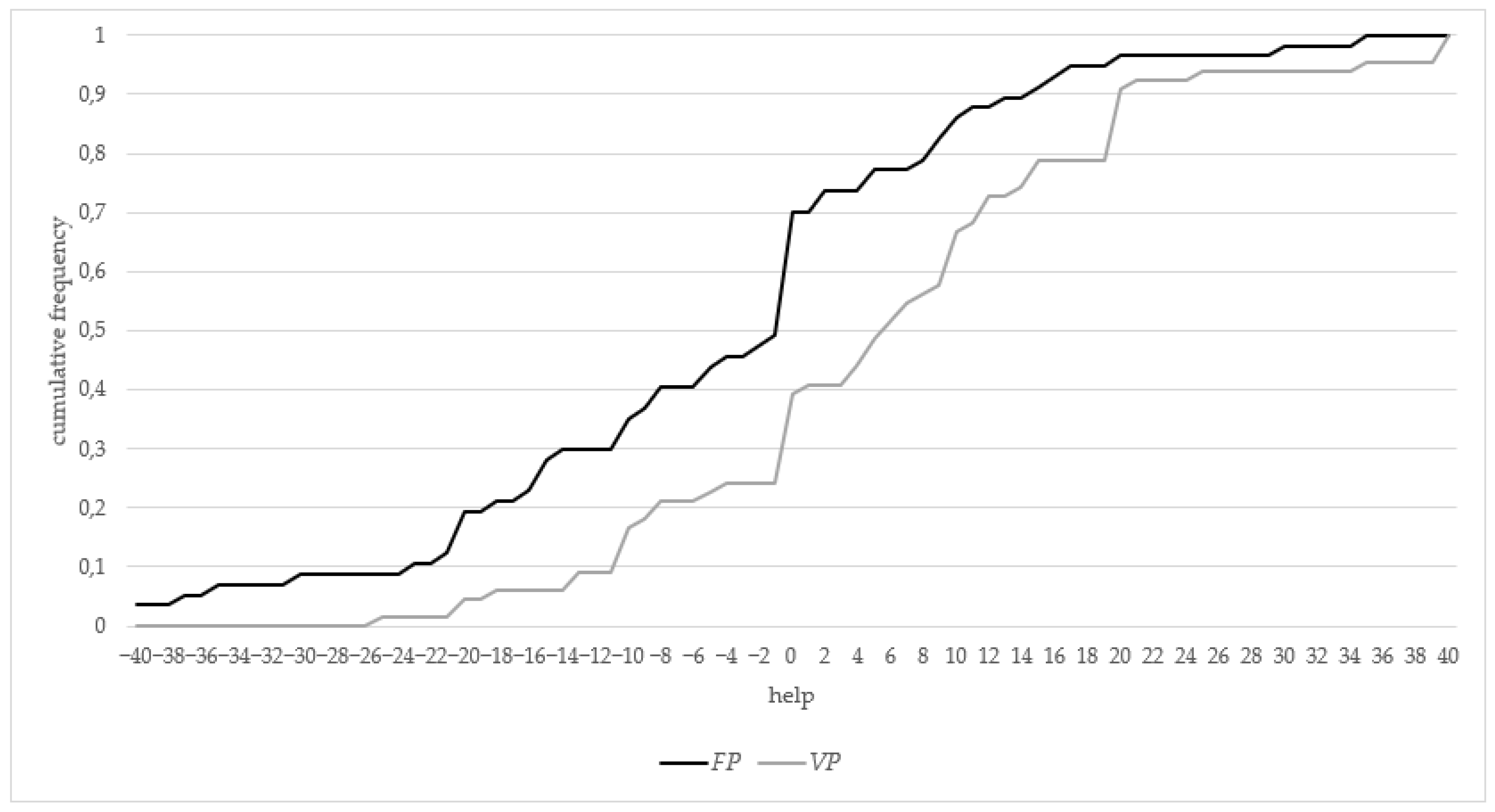
4.3. Help
4.4. Utility Levels
5. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Mathematical Appendix
Appendix B. Supplementary Results
| DV: Effort | Model 1 | Model 2 | Model 3 |
|---|---|---|---|
| VP | 1.012 | 2.101 | 2.047 |
| (1.716) | (1.692) | (1.775) | |
| Help | −0.103 + | −0.090 | |
| (0.058) | (0.643) | ||
| Positional Prefences | −0.432 | ||
| (0.960) | |||
| Risk Aversion | 0.127 | ||
| (0.460) | |||
| Nastiness | 0.096 | ||
| (0.537) | |||
| Female | −1.502 | ||
| (ref. category: male) | (2.086) | ||
| Diverse | 1.743 | ||
| (ref. category: male) | (3.983) | ||
| Extraversion | 1.479 | ||
| (1.133) | |||
| Agreeableness | 0.097 | ||
| (0.981) | |||
| Conscientiousness | −0.393 | ||
| (1.121) | |||
| Neuroticism | 0.645 | ||
| (1.080) | |||
| Openness | 0.871 | ||
| (0.978) | |||
| Constant | 29.246 ** | 28.785 ** | 20.791 ** |
| (1.363) | (1.372) | (7.364) | |
| Observations | 123 | 123 | 123 |
| R-squared | 0.003 | 0.031 | 0.068 |
| DV: Help/Sabotage | Lower Limit = 0 | Upper Limit = 0 |
|---|---|---|
| 5.994 | 5.934 + | |
| (4.607) | (3.280) | |
| Effort | 0.686 | −0.742 ** |
| (0.283) | (0.174) | |
| Positional Prefences | −1.272 | 2.968 * |
| (1.684) | (1.429) | |
| Risk Aversion | 0.180 | 0.819 |
| (0.955) | (0.631) | |
| Nastiness | −1.032 | −0.790 + |
| (0.773) | (0.466) | |
| Female | −5.350 | 6.223 + |
| (ref. category: male) | (4.402) | (3.492) |
| Diverse | omitted | −5.511 |
| (ref. category: male) | (7.186) | |
| Extraversion | −2.308 | 0.074 |
| (2.155) | (1.683) | |
| Agreeableness | 0.383 | −1.744 |
| (2.481) | (1.831) | |
| Conscientiousness | 3.097 | −3.353 |
| (2.715) | (2.237) | |
| Neuroticism | −5.958 ** | −4.192 * |
| (2.187) | (1.713) | |
| Openness | −0.138 | 0.111 |
| (2.413) | (1.332) | |
| Constant | 25.120 | 26.321 + |
| (17.764) | (15.072) | |
| Selection | ||
| 1.027 ** | 0.877 ** | |
| (0.259) | (0.258) | |
| Effort | −0.0089 | −0.021 |
| (0.133) | (0.130) | |
| Positional Prefences | −0.124 | 0.027 |
| (0.141) | (0.140) | |
| Risk Aversion | 0.017 | 0.029 |
| (0.067) | (0.071) | |
| Nastiness | −0.153 * | −0.150 * |
| (0.067) | (0.062) | |
| Female | −0.524 | 0.105 |
| (ref. category: male) | (0.322) | (0.309) |
| Diverse | −5.376 ** | −0.518 |
| (ref. category: male) | (0.487) | 0.854 |
| Extraversion | 0.253 | 0.109 |
| (0.165) | (0.162) | |
| Agreeableness | 0.308 + | 0.151 |
| (0.172) | (0.174) | |
| Conscientiousness | −0.029 | 0.117 |
| (0.164) | (0.170) | |
| Neuroticism | 0.206 | 0.105 |
| (0.158) | (0.149) | |
| Openness | −0.177 | 0.487 |
| (0.122) | (0.126) | |
| Constant | −1.612 | −1.251 |
| (1.172) | (1.163) | |
| Observations | 123 | 123 |
| Prob > Chi² | 0.235 | 0.000 |
| Pseudo R-squared | 0.083 | 0.118 |
Appendix C. Experimental Instructions
- (1)
- Work effort
- (2)
- Sabotage/help
- -
- The other two employees in the group sabotage:
- -
- One of the other two employees in the group sabotages, one helps:
- -
- The other two employees in the group help:
- -
- If none of the other employees in your group choose sabotage or help, your work result will only be affected by the effort you choose and the random number Z.
| Own Effort/Sabotage/Help | Costs (in ECU) | Own Effort/ Sabotage/Help | Costs (in ECU) |
|---|---|---|---|
| 0 | 0.00 | 21 | 5.51 |
| 1 | 0.01 | 22 | 6.05 |
| 2 | 0.05 | 23 | 6.61 |
| 3 | 0.11 | 24 | 7.20 |
| 4 | 0.20 | 25 | 7.81 |
| 5 | 0.31 | 26 | 8.45 |
| 6 | 0.45 | 27 | 9.11 |
| 7 | 0.61 | 28 | 9.80 |
| 8 | 0.80 | 29 | 10.51 |
| 9 | 1.01 | 30 | 11.25 |
| 10 | 1.25 | 31 | 12.01 |
| 11 | 1.51 | 32 | 12.80 |
| 12 | 1.80 | 33 | 13.61 |
| 13 | 2.11 | 34 | 14.45 |
| 14 | 2.45 | 35 | 15.31 |
| 15 | 2.81 | 36 | 16.20 |
| 16 | 3.20 | 37 | 17.11 |
| 17 | 3.61 | 38 | 18.05 |
| 18 | 4.05 | 39 | 19.01 |
| 19 | 4.51 | 40 | 20.00 |
| 20 | 5.00 |
- □
- Help
- □
- Sabotage
- □
- Neither help nor sabotage
- □
- Male
- □
- Female
- □
- Diverse
| 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| □ | □ | □ | □ | □ | □ | □ |
| 1 = not at all willing to take risks | ||||||
| 7 = very risky | ||||||
| Does not apply at all | Fully applicable | ||||
| 1 | 2 | 3 | 4 | 5 | |
| I’m more reserved, distant. | □ | □ | □ | □ | □ |
| I trust others easily, believe in the good in people. | □ | □ | □ | □ | □ |
| I’m comfortable, prone to laziness. | □ | □ | □ | □ | □ |
| I’m relaxed, I don’t let stress get to me. | □ | □ | □ | □ | □ |
| I have little artistic interest. | □ | □ | □ | □ | □ |
| I come out of my shell, I’m social. | □ | □ | □ | □ | □ |
| I tend to criticize others. | □ | □ | □ | □ | □ |
| I do tasks thoroughly. | □ | □ | □ | □ | □ |
| I get nervous and insecure easily. | □ | □ | □ | □ | □ |
| I have an active imagination, am imaginative. | □ | □ | □ | □ | □ |
| 1 | The general marginal probabilities for the different ranks in the tournaments depending on the distribution functions of the error term are presented in Akerlof and Holden [18]. |
References
- Kempf, A.; Ruenzi, S. Tournaments in mutual-fund families. Rev. Finan. Stud. 2008, 21, 1013–1036. [Google Scholar] [CrossRef]
- Backes-Gellner, U.; Pull, K. Tournament compensation systems, employee heterogeneity, and firm performance. Hum. Resour. Manag. 2013, 52, 375–398. [Google Scholar] [CrossRef]
- Casas-Arce, P.; Martinez-Jerez, F.A. Relative performance compensation, contests, and dynamic incentives. Manag. Sci. 2009, 55, 1306–1320. [Google Scholar] [CrossRef]
- Danilov, A.; Harbring, C.; Irlenbusch, B. Helping under a combination of team and tournament incentives. J. Econ. Behav. Org. 2019, 162, 120–135. [Google Scholar] [CrossRef]
- Güth, W.; Levínský, R.; Pull, K.; Weisel, O. Tournaments and piece rates revisited: A theoretical and experimental study of output-dependent prize tournaments. Rev. Econ. Des. 2016, 20, 69–88. [Google Scholar] [CrossRef]
- Lazear, E.P.; Rosen, S. Rank-order tournaments as optimum labor contracts. J. Pol. Econ. 1981, 89, 841–864. [Google Scholar] [CrossRef]
- Kräkel, M. U-type versus J-type Tournaments as Alternative Solutions to the Unverifiability Problem. Labour Econ. 2003, 10, 359–380. [Google Scholar] [CrossRef]
- Schöttner, A. Precision in u-type and j-type tournaments. Schmalenbach Bus. Rev. 2005, 57, 167–192. [Google Scholar] [CrossRef]
- Bevia, C.; Corchón, L.C. Rational sabotage in cooperative production with heterogeneous agents. BE J. Theor. Econ. 2006, 6, 1–27. [Google Scholar] [CrossRef]
- Cason, T.N.; Masters, W.A.; Sheremeta, R.M. Entry into winner-take-all and proportional-prize contests: An experimental study. J. Public Econ. 2010, 94, 604–611. [Google Scholar] [CrossRef]
- Lazear, E.P. Pay equality and industrial politics. J. Pol. Econ. 1989, 97, 561–580. [Google Scholar] [CrossRef]
- Harbring, C.; Irlenbusch, B. Incentives in tournaments with endogenous prize selection. J. Inst. Theor. Econ. 2005, 161, 636–663. [Google Scholar] [CrossRef]
- Harbring, C.; Irlenbusch, B. Sabotage in tournaments: Evidence from a laboratory experiment. Manag. Sci. 2011, 57, 611–627. [Google Scholar] [CrossRef]
- Ayaita, A.; Pull, K. Positional preferences and narcissism: Evidence from ‘money burning’ dictator games. Appl. Econ. Lett. 2022, 29, 267–271. [Google Scholar] [CrossRef]
- Abbink, K.; Sadrieh, A. The pleasure of being nasty. Econ. Lett. 2009, 105, 306–308. [Google Scholar] [CrossRef]
- Rammstedt, B.; Kemper, C.J.; Klein, M.C.; Beierlein, C.; Kovaleva, A. A short scale for assessing the big five dimensions of personality: 10 item big five inventory (BFI-10). Methods Data Anal. 2013, 7, 17. [Google Scholar]
- O’Brien, R.M. A Caution Regarding Rules of Thumb for Variance Inflation Factors. Qual. Quant. 2007, 41, 673–690. [Google Scholar] [CrossRef]
- Akerlof, R.J.; Holden, R.T. The nature of tournaments. Econ. Theory 2012, 51, 289–313. [Google Scholar] [CrossRef]
| Full Sample | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Variables | Measurement | Mean | SD | Min | Max | Mean | SD | Min | Max | Mean | SD | Min | Max |
| Effort | choice variable [0,40] | 29.79 | 9.34 | 0 | 40 | 29.25 | 10.30 | 0 | 40 | 30.26 | 8.47 | 0 | 40 |
| Help | choice variable [−40,40] | 1.20 | 15.88 | −40 | 40 | −4.46 | 15.94 | −40 | 35 | 6.08 | 14.22 | −25 | 40 |
| Risk aversion | # of safe choices in Holt–Laury test [0,10] | 4.85 | 1.89 | 0 | 9 | 4.68 | 1.85 | 0 | 9 | 5.00 | 1.92 | 0 | 9 |
| Positional prefences | # of positional choices in three mini dictator games [0,3] | 1.27 | 1.05 | 0 | 3 | 1.32 | 0.99 | 0 | 3 | 1.23 | 1.11 | 0 | 3 |
| Nastiness | according to joy-of-destruction game [0,8] | 1.50 | 2.54 | 0 | 8 | 1.44 | 2.54 | 0 | 8 | 1.56 | 2.56 | 0 | 8 |
| Female | 0 = other, 1 = female | 0.72 | 0.41 | 0 | 1 | 0.75 | 0.43 | 0 | 1 | 0.70 | 0.46 | 0 | 1 |
| Diverse | 0 = other, 1 = diverse | 0.16 | 0.13 | 0 | 1 | 0.00 | 0.00 | 0 | 0 | 0.30 | 0.17 | 0 | 1 |
| Extraversion | average over 2 items [0,5] | 3.56 | 0.90 | 1 | 5 | 3.69 | 0.77 | 1.5 | 5 | 3.45 | 0.99 | 1 | 5 |
| Agreeableness | average over 2 items [0,5] | 3.38 | 0.83 | 1.5 | 5 | 3.43 | 0.75 | 1.5 | 5 | 3.33 | 0.89 | 1.5 | 5 |
| Conscientiousness | average over 2 items [0,5] | 3.73 | 0.80 | 2 | 5 | 3.83 | 0.78 | 2 | 5 | 3.64 | 0.82 | 2 | 5 |
| Neuroticism | average over 2 items [0,5] | 2.97 | 0.94 | 1 | 5 | 2.92 | 0.90 | 1 | 5 | 3.02 | 0.98 | 1 | 5 |
| Openness | average over 2 items [0,5] | 3.22 | 1.08 | 1 | 5 | 3.27 | 1.10 | 1 | 5 | 3.18 | 1.06 | 1 | 5 |
| N | 123 | 57 | 66 | ||||||||||
| Variables | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1.00 | ||||||||||||
| 2 | Effort | 0.05 | 1.00 | ||||||||||
| 3 | Help | 0.33 ** | −0.14 | 1.00 | |||||||||
| 4 | Risk Aversion | 0.08 | −0.00 | 0.07 | 1.00 | ||||||||
| 5 | Positional Prefences | −0.04 | 0.01 | −0.12 | 0.15 + | 1.00 | |||||||
| 6 | Nastiness | 0.02 | 0.07 | −0.26 ** | −0.06 | 0.45 * | 1.00 | ||||||
| 7 | Female | −0.06 | −0.05 | −0.08 | 0.15 | 0.07 | 0.05 | 1.00 | |||||
| 8 | Diverse | 0.12 | 0.08 | −0.05 | 0.01 | −0.03 | −0.00 | −0.21 * | |||||
| 9 | Extraversion | −0.14 | 0.14 | −0.10 | −0.13 | 0.18 * | 0.26 ** | 0.01 | 0.03 | 1.00 | |||
| 10 | Agreeableness | −0.06 | −0.03 | 0.03 | 0.17 + | 0.06 | −0.17 | 0.17 + | −0.02 | −0.07 | 1.00 | ||
| 11 | Conscientiousness | −0.12 | −0.04 | 0.03 | 0.07 | −0.06 | 0.22 * | 0.17 + | −0.12 | 0.02 | 0.22 * | 1.00 | |
| 12 | Neuroticism | 0.05 | 0.03 | −0.10 | 0.13 | 0.06 | 0.10 | 0.35 ** | −0.17 | −0.17 + | 0.03 | 0.17 + | 1.00 |
| 13 | Openness | −0.04 | 0.12 | −0.06 | 0.04 | 0.04 | −0.17 + | 0.03 | 0.21 | 0.13 | 0.06 | 0.08 | −0.01 |
| Absolute Number of Choices | % | Absolute Number of Choices | % | |
|---|---|---|---|---|
| Help () | 17 | 29.8 | 40 | 60.6 |
| Neither help nor Sabotage () | 12 | 21.1 | 11 | 15.2 |
| Sabotage () | 28 | 49.1 | 16 | 24.2 |
| Total | 57 | 100.0 | 66 | 100.0 |
| FP | VP | ||||||
|---|---|---|---|---|---|---|---|
| Obs. | % | Test Share | Obs. | % | Observable Share | Significance | |
| Panel (A): Participants who chose to neither help nor sabotage are not included. | |||||||
| Help | 17 | 0.38 | 0.38 | 40 | 0.71 | 0.71 | 0.000 |
| Sabotage | 28 | 0.62 | 16 | 0.29 | |||
| Panel (B): Participants who chose to neither help nor sabotage are included in “help”. | |||||||
| Help | 29 | 0.51 | 0.51 | 50 | 0.76 | 0.76 | 0.000 |
| Sabotage | 28 | 0.49 | 16 | 0.24 | |||
| Panel (C): Participants who chose to neither help nor sabotage are included in “sabotage”. | |||||||
| Help | 17 | 0.30 | 0.30 | 40 | 0.61 | 0.61 | 0.000 |
| Sabotage | 40 | 0.70 | 26 | 0.39 | |||
| Treatment | Number of Observations | Rank Sum | Expected |
|---|---|---|---|
| 57 | 2814 | 3534 | |
| 66 | 4812 | 4092 | |
| combined | 123 | 7626 | 7626 |
| Prob>|z| = 0.0002 |
| DV: Help | Model 1 | Model 2 | Model 3 |
|---|---|---|---|
| VP | 10.532 ** | 10.802 ** | 11.387 ** |
| (2.742) | (2.728) | (2.863) | |
| Effort | −0.267 + | −0.217 | |
| (0.143) | (0.148) | ||
| Positional Prefences | 0.184 | ||
| (1.537) | |||
| Risk Aversion | 0.377 | ||
| (0.660) | |||
| Nastiness | −1.634 * | ||
| (0.654) | |||
| Female | −1.695 | ||
| (ref. category: male) | (3.734) | ||
| Diverse | −11.080 * | ||
| (ref. category: male) | (5.168) | ||
| Extraversion | 0.634 | ||
| (1.776) | |||
| Agreeableness | 0.155 | ||
| (2.042) | |||
| Conscientiousness | 0.393 | ||
| (1.665) | |||
| Neuroticism | −1.532 | ||
| (1.493) | |||
| Openness | −0.988 | ||
| (1.478) | |||
| Constant | −4.456 * | 3.351 | 6.835 |
| (2.110) | (4.126) | (13.279) | |
| Observations | 123 | 123 | 123 |
| Adj. R-squared | 0.103 | 0.121 | 0.139 |
| Payoffs | |||
|---|---|---|---|
| Agents | payoff (in ECU) | 16.24 | 18.57 |
| Principals | output units per agent | 25.72 | 39.18 |
| payment to agent (in ECU) | 21.60 | 23.84 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Glökler, T.; Pull, K.; Stadler, M. Do Output-Dependent Prizes Alleviate the Sabotage Problem in Tournaments? Games 2022, 13, 65. https://doi.org/10.3390/g13050065
Glökler T, Pull K, Stadler M. Do Output-Dependent Prizes Alleviate the Sabotage Problem in Tournaments? Games. 2022; 13(5):65. https://doi.org/10.3390/g13050065
Chicago/Turabian StyleGlökler, Thomas, Kerstin Pull, and Manfred Stadler. 2022. "Do Output-Dependent Prizes Alleviate the Sabotage Problem in Tournaments?" Games 13, no. 5: 65. https://doi.org/10.3390/g13050065
APA StyleGlökler, T., Pull, K., & Stadler, M. (2022). Do Output-Dependent Prizes Alleviate the Sabotage Problem in Tournaments? Games, 13(5), 65. https://doi.org/10.3390/g13050065






