The Intermediate Value Theorem and Decision-Making in Psychology and Economics: An Expositional Consolidation

Abstract

On taking the intermediate value theorem (IVT) and its converse as a point of departure, this paper connects the intermediate value property (IVP) to the continuity postulate typically assumed in mathematical economics, and to the solvability axiom typically assumed in mathematical psychology. This connection takes the form of four portmanteau theorems, two for functions and the other two for binary relations, that give a synthetic and novel overview of the subject. In supplementation, the paper also surveys the antecedent literature both on the IVT itself, as well as its applications in economic and decision theory. The work underscores how a humble theorem, when viewed in a broad historical frame, bears the weight of many far-reaching consequences; and testifies to a point of view that the apparently complicated can sometimes be under-girded by a most basic and simple execution.

Cauchy and Bolzano pioneered the transformation of the work of 18th-century analysts into 19th-century rigor. Their work is an example of simultaneous discovery: simultaneous announcement of their program for rigorizing analysis, their definitions of continuous functions and their common desire to prove the intermediate value theorem. Both accepted Lagrange’s goal of reducing the calculus to the “algebraic analysis of finite quantities”.

Grabiner (1984)1

Mathematics is not about anything in particular; it is instead the logically connected study of abstract systems. There were revolutions in thought which changed mathematicians’ views about the nature of mathematical truth, and about what could or should be proved—one such revolution occurred between the 18th and 19th nineteenth centuries: a rejection of the mathematics of powerful techniques and novel results in favor of the mathematics of clear definitions and rigorous proofs.

Grabiner (1974)2

1. Introduction

Lagrange’s 1767 treatise begins by giving prominence to the following statement:

If we have any equation, and if we know two numbers such that, if they are successfully substituted for the unknown in that equation, they give results of opposite signs, the equation will necessarily have at least one real root whose value will be between those two numbers.3

Grabiner [1] makes clear that Lagrange’s theorem was limited to polynomials, and that the intermediate value theorem (IVT) in its modern form was independently discovered and rigorously proved only later by [3,4]. We furnish a version given in [5], and typically taught to incoming undergraduates in mathematics.4

Theorem 1

(Bolzano-Cauchy). Let $a,b\in \mathbb{R},a<b,$ and $f:[a,b]\to \mathbb{R}$ be a function. If f is continuous, and $f\left(a\right)f\left(b\right)<0$, then there exists $c\in \mathbb{R}$ such that $f\left(c\right)=0$.

The theorem is basic and elementary, but this elementary nature notwithstanding, it is now the lens through which the contemporary reader sees the “revolutionary character of Cauchy’s and his contemporaries’ efforts to set mathematical analysis on a rigorous footing, and apply a fundamentally new point of view to the problems and methods of their eighteenth-century forebears”.5 It is understood that the proofs of the theorem are crucial for the “arithmetization of analysis,” as well as the ground on which the quarrel between geometry and algebra can be contested.6

The primary emphasis of this paper, however, is neither epistemological nor historical: it is simply an expositional consolidation based on a distinction between the theorem and the property that the theorem formalizes.7 The intermediate value property (IVP) articulates the raw, and most primitive, intuition furnished by the theorem: any value in the interior of the range is attained in the interior of the domain of an up-and-down function when there are no jumps and no gaps in either its domain or its range. The goal of this paper is to obtain results that connect the IVP to the continuity property in mathematical economics and the solvability property in mathematical psychology, and thereby also to provide an up-to-date exposition of the state of the art concerning it. As such, it is both a critical survey and a contribution to the subject.

Given the recent surveys of the IVT and the associated IVP already available,8 the marginal contribution of the paper needs to be clearly spelled out: taking the framing as given, what is the substance of that which is being framed? The subject rests on two pioneering papers: Eilenberg [16], Rosenthal [17]. Eilenberg was the first to consider an “ordered topological space” and by bringing together the registers of order and topology, the first to consider the representation of binary relations by real-valued functions. In particular, his paper drew attention to the role of hidden behavioral implications in the interplay of the attendant hypotheses of continuity and connectedness.9 Moreover, once the two structures were brought together, it took Rosenthal’s theorem on joint continuity of functions to bring out the subtleties and subdue the Genocchi–Peano counterexamples.10 The literature is by now replete with the usage of the adjective continuous applied to a function, as well as to a binary relation, and this equivalence of different continuity assumptions under seemingly orthogonal behavioral and technical hypotheses continues to be explored in the context of mathematical economics without any reference to the IVP.11 Two other independently pioneering papers, Marschak [29], Nash [30], can be mentioned in this connection: they correctly and completely axiomatized expected utility representation that in hindsight can be seen as making a continuity assumption.12

Somewhat independently, a line of investigation in a similar vein was being pursued by Luce and Tukey [33], Suppes [34] and their followers in the context of decision theory as conceived in mathematical psychology. This work also provided axiomatics guaranteeing the representation of binary relations by real-valued functions, but this time appealing to what was termed solvability notions arising in measurement theory, and again without any explicit reference to the IVP.13 The fact that the IVP is a useful property for a binary relation in measure-theoretic and topological structures, be they of finite or of infinite cardinality, is now being increasingly appreciated in this line of work. Thus [36] write:

Solvability is reminiscent of the intermediate value property of continuous real-valued functions. Without richness conditions such as solvability … axiomatizations become considerably more complex.

(p. 5)

In short, this literature, along with the other economic one, can also be related to Rosenthal’s 1955 theorem and viewed as uplifting of insights and results for functions to binary relations, and to use this to connect the solvability postulate to the IVP, and thereby to effect a synthesis of sorts of unconnected results in mathematical economics and mathematical psychology.14 In a nutshell, in keeping with the theme of understanding the richness of a structure, the connections of the continuity postulate and the solvability to the IVP that we forge, provide a novel overview of the subject summarized in four portmanteau theorems.15 It is the lens through which the equivalence of these postulates for binary relations can now be viewed.

It is perhaps because of its apparently deceptive simplicity that the theorem comes late to ongoing work in mathematical economics and decision theory. If one may paraphrase Sen’s remark regarding topological structures, one may say “Just as it is possible to speak prose without noticing that fact …, it is possible also to be talking about the IVT without sensing IVT or any IVP around us”.16 An outstanding example of this, and also a testimonial to the synthetic treatment offered here, is the reading of Wold’s work as the first application of solvability considerations not in decision theory as studied in mathematical psychology, but in the classical theory of demand as exposited in textbooks of economic theory.17 There are perhaps two additional considerations that need to be adduced. The first concerns the second epigraph: “what can or needs to be proved”–the nature of mathematical truth and rigor that is in operation at a particular point in time. The second involves a first attack on a hitherto novel problem, and so, rather than an inevitably-elusive fuller understanding, investigators typically have to make do with handiest, tailor-made theorems that are then available. A reliance on such theorems for a variety of equilibrium problems in a variety of structures is initially indispensable to give a broad and synthetic overview of the basic problem, but it also obscures the concrete structural details of a particular and concrete specification of it that subsequent investigations can bring to light, and it is here that IVT plays a role.18

It is perhaps worth giving examples of each of these considerations as they pertain to the IVT. As regards the first, it is to be noted that one of the earliest applications of the IVT in mathematical economics is to the neoclassical theory of economic growth where the result was used to show the existence of a balanced growth path in the aggregative Solow–Swan growth model, and where the application went beyond the merely technical to bring out the substantive.19 The essential point, at least as regards the work reported in this paper is concerned, is that a decade earlier, the model was comprehensively investigated with a chapter devoted to this precise (existence) issue, but rather than the IVT, a geometric apparatus and argumentation was used.20 Turning to the second consideration, one may sight the use of the IVT to prove the existence of a Walrasian equilibrium in a two-commodity economy as a simple corollary of the original Bolzano–Cauchy version of the IVT: the essential point here is that the profession had to wait almost two decades for this demonstration.21 In any case, once these pioneering applications of the seventies are in place, the floodgates regarding the IVT and the IVP opened up, and they concerned the theory of games as well as Walrasian and decision theory.

The presentation of the material—the plan of the paper if one prefers—then falls naturally and easily in place into two parts: Section 2 and Section 3 on the old and the available, and Section 4 and Section 5 on the new. More specifically, Section 2 presents an overview of the theorem in so far as it pertains to functions, and also by viewing the IVP in a measure-theoretic context,22 allows a connection to Liapounoff’s 1940 theorem on the range of an atomless vector measure. Despite the material being well-known, the reader hopefully will see some novelty in the arrangement of it.23 In addition to the notion of the up-and-down function abstracted into a binary relation, Section 3 can also be viewed as an abstraction of the notion of equality into an equivalence relation, and it is this that facilitates the formulation of the solvability and restricted solvability properties. This section then turns to the discipline that gave rise to measurement and decision theory:24 Section 4 turns to the new material as categorized under extensions and use of the IVP for functions separately from binary relations, and summarized in four portmanteau theorems, and two main Propositions.25 Section 5 is devoted to the proofs. Section 6 concludes the basics of this essay with three open questions that provide a brief programmatic statement for future work that would allow a reading of the subject that opens the doors for a further investigation. Appendix A contains a selected listing for a reader interested in ongoing work centered around the IVT and IVP, and their extensions.

2. The IVT and Its Generalizations

This section is composed of five parts. The first part surveys the classical theorem of Bolzano and its variants. In the second part, we touch upon the extensions of the IVT for functions with weakened continuity properties. The third and the fourth parts provide an exploratory overview of the conception of the IVT for topological, algebraic, and measure-theoretic registers. Lastly, we conclude with a brief discussion of IVT on finite-dimensional spaces.

2.1. The Classical Theorem

We begin this section by defining the intermediate value property (IVP) for functions defined on subsets of the real line. Let $X\subseteq \mathbb{R}$. A function $f:X\to \mathbb{R}$ has the IVP if for all $x,y\in X,x<y$ and all c lying between $f\left(x\right)$ and $f\left(y\right)$, there exists $z\in [x,y]$ such that $f\left(z\right)=c$.

Bolzano [3] proves the classic intermediate value theorem as stated in the introduction for functions defined on an interval in $\mathbb{R}$. The following theorem is a slightly general version of Bolzano’s theorem; its proof easily follows from Bolzano’s theorem, hence omitted.

Theorem 2.

Every continuous real-valued function defined on an interval in $\mathbb{R}$ has the IVP.

This theorem provides a one-directional relationship between the usual continuity assumption and the IVP, a weak continuity assumption on functions. It is well-known that the converse of this theorem is false without additional assumptions on functions. Sohrab [59] (p. 167) shows that for a class of functions satisfying a weak monotonicity assumption, the two continuity concepts are equivalent.

Definition 1.

Let $a,b\in \mathbb{R},a<b,$ be an interval. A function $f:[a,b]\to \mathbb{R}$ is piecewise monotone if, for some $n>1$, there exists $n-1$ numbers $x_1,\dots ,x_n\in (0,1)$ satisfying $a=x_0<x_1<\dots <x_n=b$ such that for each k such that $1\le k\le n$, f is weakly increasing or weakly decreasing on $[x_{k-1},x_k]$.

Theorem 3

(Sohrab). Let $a,b\in \mathbb{R},a<b,$ and $f:[a,b]\to \mathbb{R}$ be a function that is piecewise monotone. Then, f is continuous if and only if it has the IVP.

Note that every quasi-concave, or quasi-convex, or injective (see [59,60]) function on an interval is piecewise monotone, the following is a corollary of the theorem above.

Corollary 1.

Let $a,b\in \mathbb{R},a<b,$ and $f:[a,b]\to \mathbb{R}$ be a function satisfying any of the following three properties: quasi-concavity, quasi-convexity, or injectivity. Then, f is continuous if and only if it has the IVP.

Sohrab’s theorem and the corollary above provides an equivalence result for different continuity concepts on functions. This result is limited to the one-dimensional setting, but illustrates an equivalence result analogous to the equivalence results on different continuity concepts for various classes of binary relations. There are many results generalizing Bolzano’s theorem to multidimensional settings; see [5,14,61] for three recent surveys. We return to the equivalence of different continuity concepts for functions in a multidimensional setting in Section 4.

2.2. Beyond Continuous Functions

Milgrom and Roberts [62] and Guillerme [63] independently prove a generalization of (an equivalent version of) the IVT by weakening the continuity assumption. We present their result below, as summarized in [64] as a fixed point theorem, but first we provide two weak continuity properties for functions. Let $[a,b]\subset \mathbb{R}$ be an interval. A function $f:[a,b]\to \mathbb{R}$ is continuous but for upward jumps if for all $x\in [a,b]$ and any sequence $x_n$ approaching x from above ($x_n\downarrow x$), ${lim\; inf}_{x_n\downarrow x}f\left(x_n\right)\ge f\left(x\right)$, and for any sequence $x_n$ approaching x from below ($x_n\uparrow x$), ${lim\; sup}_{x_n\uparrow x}f\left(x_n\right)\le f\left(x\right)$.

Theorem 4

(Guillerme–Milgrom–Roberts). If a function $f:[0,1]\to [0,1]$ is continuous but for upward jumps, then it has a fixed point.

Wu [64] proves a generalization of the above theorem for correspondences by using the following weak continuity assumptions on correspondences.26 Let $T:\mathbb{R}\twoheadrightarrow Y$ be a non-empty-valued correspondence, where Y is a metric space. T is said to be upper semicontinuous on the right (RUS) if for any $x\in \mathbb{R}$ and any sequence $x_n\downarrow x$, if a sequence $\left(y_n\right)$ converging to y is such that $y_n\in T\left(x_n\right)\forall n$, then $y\in T\left(x\right)$; T is said to be lower semicontinuous on the left (LLS) if for any sequence $x_n\uparrow x$ and every $y\in T\left(x\right)$, there is a sequence $\left(y_n\right)$ converging to y such that for every n, one has $y_n\in T\left(x_n\right)$.

Theorem 5

(Wu). Let $T:[0,1]\twoheadrightarrow \mathbb{R}$ be an RUS and LLS correspondence. Suppose that $T\left(\right[0,1\left]\right)$ is contained in some bounded set of $\mathbb{R}$ and for any $y\in T\left(0\right)$ we have $y\ge 0$, while for any $y\in T\left(1\right)$ we have $y\le 0$. Then there exists $x\in [0,1]$ such that $0\in T\left(x\right)$.

Ricci [66] proves a further generalization of IVT (which is also related to Amir and DeCastro [67]’s theorem) by allowing additional discontinuities for which we need additional notation and definitions. Assume that $T:X\twoheadrightarrow Y$ is a closed-valued correspondence with non-empty values, where $X,Y$ are two non-empty and compact intervals in $\mathbb{R}$. Denote by $m_T:X\to Y$ and $M_T:X\to Y$ the mappings given by $m_T\left(x\right)=\min T\left(x\right)$ and $M_T\left(x\right)=\max T\left(x\right)$. Note that these two functions are well-defined due to the fact that T has non-empty and closed values. Further, denote by $s_T:X\to Y$ the mapping such that $s_T\left(x\right)$ is the supremum of the maximal connected subset of $T\left(x\right)$, also called component, containing $m_T\left(x\right)$. Note that this function is well-defined because of the properties of the components, and that $m_T\left(x\right)\le s_T\left(x\right)\le M_T\left(x\right)$ and $[m_T\left(x\right),s_T\left(x\right)]\subseteq T\left(x\right)$ for any $x\in X$.

Theorem 6

(Ricci). Let X be a non-empty and compact interval in $\mathbb{R}$ and $T:X\twoheadrightarrow X$ be a correspondence with non-empty and closed values. Assume for all $x_0\in X$,

$$\begin{array}{c}\hfill {\mathrm{limsup}}_{x\uparrow x_0}{s}_T\left(x\right)\le s_T\left(x_0\right)\mathit{and}{\mathrm{limsup}}_{x\downarrow x_0}{m}_T\left(x\right)\ge m_T\left(x_0\right).\end{array}$$

Then there exists $x^{*}\in X$ such that $x^{*}\in T\left(x^{*}\right)$.

These generalized versions of the IVT have been applied in economic theory, we provide references to a selection of them in Appendix A.

2.3. A Topological and Algebraic Conception

In this subsection, we provide a generalization of the IVT for functions that have general domain and range. First, we need the following notation and concepts. We assume throughout this paper that [0, 1] is endowed with the usual topology. An arc in a topological space X is a continuous injective function $m:[0,1]\to X$. A curve in X is the image of an arc $m:[0,1]\to X$. Since an arc m is continuous and injective, it is a bijection from $[0,1]$ to its image $m\left(\right[0,1\left]\right)$.27 A topological space X is connected if there does not exist two non-empty and disjoint open sets $U,V$ in X such that $X=U\cup V$. A subset of X is connected if it is connected under its subspace topology.28 An ordered set X is a set on which a binary relation ≻ is defined with the following properties: (i) $x\succ y\succ z$ implies $x\succ z$ for all $x,y,z\in X$ (transitivity), (ii) $x\nsucc y$ for all $x\in X$ (irreflexivity), (iii) $x\succ y$ or $y\succ x$ for all $x,y\in X,x\ne y$ (total). The order topology on an ordered set X is the topology with a basis consists of the collection of all sets of the following types: for all $a,b\in X$, (i) $(a,b)=\{x\in X:b\succ x\succ a\}$ (open intervals), (ii) $[a_0,b)=\{x\in X:b\succ x\succ a_0,\mathrm{or}x=a_0\}$ where $a_0$ is the smallest element (if any) of X and (iii) $(a,b_0]=\{x\in X:b_0\succ x\succ a,\mathrm{or}x=b_0\}$ where $b_0$ is the largest element (if any) of X (half-open intervals).

Next, we provide an algebraic property of a set that is weaker than a linear space. A set $\mathcal{S}$ is said to be a mixture set if for any $x,y\in \mathcal{S}$ and for any $\mu$ we can associate another element,29 which we write as $x\mu y,$ which is again in $\mathcal{S},$ and where for all $x,y\in \mathcal{S}$ and all $\lambda ,\mu ,$ (S1) $x1y=x$, (S2) $x\mu y=y(1-\mu )x$, (S3) $\left(x\mu y\right)\lambda y=x\left(\lambda \mu \right)y$. In decision theory, it is common to assume that the choice set on which preferences are defined has an algebraic structure instead of a topological structure. Such a setup is introduced by [23] and defined as above. It is easy to see that any convex set is a mixture set, but the converse relationship is false; see [69] for a detailed discussion.

Now, we define three versions of the IVP for functions with general domain and range.

Definition 2.

Let X be a mixture set and Y an ordered set. A function $f:X\to Y$ has the IVP if for all $x,y\in X$ and all c lying between $f\left(x\right)$ and $f\left(y\right)$ (with respect to the order on Y), there exists $\lambda \in [0,1]$ such that $f\left(x\lambda y\right)=c$.

Definition 3.

A real-valued function f on a topological space X has the strong IVP if for all $x,y\in X$, all c lying between $f\left(x\right)$ and $f\left(y\right)$ and all curves $C_{xy}$ connecting x and y, there exists $z\in C_{xy}$ such that $f\left(z\right)=c$.

Definition 4.

Let X be a non-empty set and Y a non-empty ordered set. A function $f:X\to Y$ has the weak IVP if for all $a,b\in X$ and $r\in Y$ lying between $f\left(a\right)$ and $f\left(b\right)$, there exists $c\in X$ such that $f\left(c\right)=r$.

When the domain of a function f is a topological vector space, then the three definitions of the IVP are well defined for f. It follows from the definitions of these three properties that strong IVP implies IVP, and IVP implies weak IVP.

Next, we provide generalizations of the IVP. Different versions of these results are provided in different works in the literature, hence we provide proofs for the convenience of the reader; they all rely on the connectedness assumption.

Proposition 1.

Let X be a mixture sets, Y an ordered set endowed with the order topology and $f:X\to Y$ be a function with the following continuity property: for all $x,y\in X$ and all open sets $U\in Y$, the set $\{\lambda \in [0,1]:f(x\lambda y)\in U\}$ is open in [0, 1]. Then, f satisfies the IVP.

It is easy to observe that if the domain of the function in this proposition is a convex subset of a topological vector space, then the continuity of a function (preimage of every open set is open) implies the continuity assumption above. Hence, this proposition uses both general domain and range, and also a weaker continuity assumption.

Proposition 2.

Let X be a topological space, Y an ordered set endowed with the order topology and $f:X\to Y$ be a continuous function. Then, f satisfies the strong IVP.

Munkres [70] (Theorem 24. 3, p. 154) provides a similar result showing that the function satisfies the weak IVP. The theorem depends not only on the continuity of f but also on the (topological) connectedness property of its domain. Next, we present an equivalence result showing that connectedness of the domain is not only sufficient but also necessary for all continuous functions to have the IVP.

Proposition 3.

Let X be a non-empty topological space and Y be a non-empty ordered set with the order topology. Then, every continuous function $f:X\to Y$ has the weak IVP if and only if X is connected.

Given its trivial nature, the proof is omitted. Note that in this proposition, the weak IVP can be replaced by the strong IVP.

2.4. A Measure-Theoretic Conception

In this subsection, we begin with the strong IVP of measure spaces as defined in Hahn and Rosenthal [57] and Mizel and Martin [58], and connect it with Liapounoff’s 1940 theorem on the range of an atomless vector measure.

Let $(X,\mathcal{A},\mu )$ be a measure space where X is a set, $\mathcal{A}$ a $\sigma$-algebra on $X,$ and a measure, $\mu :\mathcal{A}\to [0,+\infty ]$. Then, following [58], we define the IVP for measure spaces.

Definition 5.

A measure space $(X,\mathcal{A},\mu )$ is said to have the weak IVP if for every real number $a,0\le a\le \mu \left(X\right),$ there exists a measurable subset A of X such that $\mu \left(A\right)=a.$

Definition 6.

A measure space $(X,\mathcal{A},\mu )$ is said to have the strong IVP, if for every measurable subset S of X and every real number $a,0\le a\le \mu \left(S\right),$ there exists a measurable subset A of S such that $\mu \left(A\right)=a$.

It is trivial to observe that for a measure space, the strong intermediate value property implies the weak IVP.

A measurable subset A of X is called an atom if $\mu \left(A\right)\ne 0$ and if every $\mathcal{A}$-measurable subset of A is either of measure 0 or else has the same measure as A. A measure space X is said to be atomless if it has no atoms and a measure is atomless if its support has no atom. Next, we present a result of [57]30 that establishes equivalence between the IVP of a measure space and the atomless property of the measure.

Theorem 7

(Hahn–Rosenthal). A measure space has the strong IVP if and only if it is atom free.

Suppose that ${\mu }_1, {\mu }_2, \dots , {\mu }_n$ are finite atomless measures on $(X,\mathcal{A}).$ We denote the vector of n-measures, $({\mu }_1, {\mu }_2, \dots , {\mu }_n)$ by $\overrightarrow{\mu }.$ Next, we present the famous theorem of Liapounoff [71] on the range of a vector-valued measure31 where the convexity of the range has a similar flavor to the IVT which is followed by a re-statement of an equivalence theorem from Ross [74].

Theorem 8

(Liapounoff). The set $\{\overrightarrow{\mu }S:S\in \mathcal{A}\}$ is convex and compact.

Theorem 9

(Ross). Consider the following statements.

(LT1)

For each $S\in \mathcal{A}$ and $r\in [0,1]$ there is a measurable subset A of S such that $\overrightarrow{\mu }A=r\overrightarrow{\mu }S.$

(LT2)

For each $S\in \mathcal{A}$ there is a $r\in (0,1)$ and a measurable subset A of S such that $\overrightarrow{\mu }A=r\overrightarrow{\mu }S.$

(LT3)

For each $S\in \mathcal{A}$ there is a measurable subfamily ${\left\{A_r\right\}}_{r\in [0,1]}$ such that $A_r\subseteq A_s\subseteq S$ whenever $0\le r\le s\le 1$ and $\overrightarrow{\mu }{A}_r=r\overrightarrow{\mu }S$ for each $r\in [0,1].$

Then, LT3 ⇒ LT1 ⇒ LT2 ⇒ LT3, and each of LT1, LT2, and LT3 is true.

Ross [74] uses the classic Bolzano’s theorem in his proof and shows that convexity of the range of the vector measure follows from any of the three parts (a)–(c) in Theorem (Ross). Next, we connect the theorem of Hahn and Rosenthal [57] to Ross [74].

Proposition 4.

For $n=1,$ each of the three parts (a)–(c) in Theorem (Ross) are equivalent to the strong IVP of a measure space.

The proof is trivial. Since the strong IVP is equivalent to part (a) of Theorem (Ross) which, in turn, is equivalent to parts (b) and (c). This completes the proof.

2.5. More on Finite-Dimensional Spaces

The classical intermediate value theorem is defined on the subsets of the real line. Section 2.3 provides different multi-dimensional extensions of the IVT where the range of the function is an ordered set (such as the real line). There is also a different class of extensions to multi-dimensional setting where the domain and the range of the function have the same dimension and this section is devoted to such class of extensions. The one-dimensional versions of the following three well-known and widely applied theorems in topology are equivalent to the classical intermediate value theorem: the Brouwer fixed point theorem, the Borsuk–Ulam theorem and the Poincaré–Miranda theorem.32

Theorem 10

(Borsuk-Ulam). Let $S^n=\{x\in {\mathbb{R}}^{n+1}:\left|x\right|=1\}$ denote the unit n-sphere in ${\mathbb{R}}^{n+1}$ and $f:S^n\to {\mathbb{R}}^n$ be a continuous function. Then, there exists $x^{*}\in S^n$ such that $f\left(x^{*}\right)=f(-x^{*})$.

Theorem 11

(Brouwer). Let $B^n=\{x\in {\mathbb{R}}^n:\left|x\right|\le 1\}$ denote the unit n-ball in ${\mathbb{R}}^n$ and $f:B^n\to B^n$ be a continuous function. Then, there exists $x^{*}\in S^n$ such that $f\left(x^{*}\right)-x^{*}=0$.

The two theorems above provide a generalization of Bolzano’s theorem as a fixed point theorem. There is very rich literature on generalizations of fixed point theorems, and they are very useful tools not only in economics but also in many other disciplines. A generalization more in the spirit of Bolzano’s theorem is provided by Poincaré–Miranda.

Theorem 12

(Poincaré-Miranda). Let $A={\prod }_{i=1}^n[-a_i,a_i]$, where $a_i\in {\mathbb{R}}_{++}$ for all $i=1,\dots ,n$ and $f=\left(f_1,\dots ,f_n\right):A\to {\mathbb{R}}^n$ be a continuous function such that

$$\begin{array}{cc}\hfill f_i\left(x\right)\ge 0,& \forall x\mathit{such}\mathit{that}{x}_i=-a_i\hfill \\ \hfill f_i\left(x\right)\le 0,& \forall x\mathit{such}\mathit{that}{x}_i=a_i.\hfill \end{array}$$

Then there exists $x^{*}\in K$ such that $f\left(x^{*}\right)=0$.

There are different versions of generalization of Bolzano’s theorem to higher dimensional settings (finite or infinite) in the spirit of Poincaré–Miranda’s theorem; see for example [14,80], and an application of Vrahatis’ result in economics presented in [81]; see Appendix A for details. Moreover, as we provide above for Bolzano’s theorem, there are generalizations of Poincaré–Miranda’s theorem to discontinuous correspondences; on this, see for example, Refs. [5,82,83,84] for some recent surveys.

3. The IVP in Psychology and Economics

Binary relations are the basic tool in many disciplines, including mathematical psychology and mathematical economics. Continuity of a binary relation is usually imposed to enforce enough richness on the structure being investigated so that the mathematical machinery works smoothly.33 In this section, we hinge our discussion around the concept of intermediate valuedness for binary relations as practiced in the field of decision theory and mathematical psychology. In particular, the plan of this section is as follows. The first subsection provides the preliminaries. The second and the third subsection detail the IVP property as practiced in economics and measurement theory literature, respectively.

3.1. Binary Relations: Preliminaries

We begin by listing the preliminaries. Let X be a set. A subset ≿ of $X\times X$ denote a binary relation on $X.$ We denote an element $(x,y)\in \succsim$ as $x\succsim y.$ The asymmetric part ≻ of ≿ is defined as $x\succ y$ if $x\succsim y$ and $y\not\gtrsim x$, and its symmetric part ∼ is defined as $x\sim y$ if $x\succsim y$ and $y\succsim x.$ The inverse of ≿ is defined as $x\precsim y$ if $y\succsim x$. Its asymmetric part ≺ is defined analogously and its symmetric part is ∼. A binary relation ≿ on X is complete if $x\succcurlyeq y$ or $y\succcurlyeq x$ $\forall x,y\in X$, and transitive if $x\succcurlyeq y\succcurlyeq z\Rightarrow x\succcurlyeq z,$ $\forall x,y,z\in X$. We call a subset A of X bounded by ≿ if for all $x,y\in A$ there exists $a,b\in X$ such that $a\succsim x,y$ and $x,y\succsim b$. A relation ≿ is weakly monotonic if for all $x,y\in X$, $x>y$ implies34 $x\succsim y$. A relation ≿ on a set X is order dense if $x\succ y$ implies that there exists $z\in X$ such that $x\succ z\succ y$.

Now, let X be endowed with a topology. A binary relation ≿ on X is continuous if for any $x\in X$, the sets $A_{\succsim }\left(x\right)=\{y\in X|y\succsim x\}$ and $A_{\precsim }\left(x\right)=\{y\in X|y\precsim x\}$ are closed. Moreover, ≿ is graph continuous if it has a closed graph. A relation ≿ on a convex subset X of ${\mathbb{R}}^n$ is separately continuous if the restriction of the relation on any line parallel to a coordinate axis is continuous and linearly continuous if its restriction to any straight line in X is continuous.

Next, we provide continuity assumptions on binary relations that are defined on a mixture set. A topological structure is absent in a mixture set and hence topological properties of a binary relation are defined by using the topology on the unit interval. A binary relation ≿ on a mixture set $\mathcal{S}$ is mixture continuous if for any $x,y,z\in X$, the sets $\{\lambda \in [0,1\left]\right|x\lambda y\succsim z\}$ and $\{\lambda \in [0,1\left]\right|x\lambda y\precsim z\}$ are closed (in [0, 1]). Finally, a binary relation ≿ defined on a mixture set $\mathcal{S}$ is Archimedean if $x,y,z\in X,x\succ y$ implies that there exist $\lambda ,\delta \in (0,1)$ such that $x\lambda z\succ y$ and $x\succ y\delta z$.

3.2. IVP for Binary Relations

In his pioneering work, Wold [45] develops consumer theory and provides a result on numerical representation of binary relations by a continuous function35 based on the following continuity assumption.

Definition 7.

A binary relation ≿ on a subset X of ${\mathbb{R}}^n$ is Wold-continuous if it is order dense and $x\succ z\succ y$ implies that any curve joining x to y meets the indifference class of z.

In a subsequent work, Wold and Jureen [46] use the following weaker continuity assumption.

Definition 8.

A binary relation ≿ on a subset X of ${\mathbb{R}}^n$ is weakly Wold-continuous if it is order dense and $x\succ z\succ y$ implies that the straight line joining x to y meets the indifference class of z.36

This weaker continuity assumption is first used in decision theory independently by Nash [87] and Marschak [29] who provide complete axiomatization of the expected utility theory initiated by von Neumann and Morgenstern [88].37 This continuity assumption has become one of the standard assumptions in decision theory and mathematical psychology.

Comparing Definitions 2 and 3 with Definitions 7 and 8, respectively, shows that weak Wold-continuity has the essence of the IVP and Wold continuity has the essence of strong IVP in the context of a binary relation. Motivated by this observation, we provide a definitions of the strong IVP and IVP for binary relations that are analogous to their counterparts for functions.

Definition 9.

Let X be a non-empty topological space. A binary relation ≿ on X has the strong IVP if for all $x,y,z\in X$ with $x\succsim y\succsim z$ and all curves $C_{xy}$ connecting x and z, there exists $c\in C_{xy}$ such that $c\sim y$.

Next, we introduce a weak version of the IVP for binary relations defined on a mixture set which replaces curves connecting two points by the mixtures of the two points.

Definition 10.

Let X be a non-empty mixture set. A binary relation ≿ on X has the IVP if for all $x,y,z\in X$ with $x\succsim y\succsim z$, there exists $\lambda \in [0,1]$ such that $x\lambda z\sim y$.

Next, we present an intermediate value theorem for a complete and transitive binary relation defined on a connected set–it implies that the continuity postulate characterized by the IVP is implied by the continuity of the relation.

Proposition 5.

Let X be a non-empty and connected topological space. Every complete, transitive and continuous binary relation ≿ on X has the strong IVP.

The following result presents an intermediate value theorem for a complete and transitive binary relation defined on a mixture set.

Proposition 6.

Let X be a non-empty mixture set. Every complete, transitive and mixture continuous binary relation ≿ on X has the IVP.

Note that a convex set in a topological vector space is connected and it is not difficult to show that in the context of a topological vector space, a continuous binary relation is mixture continuous; see [20] for details. Therefore, the following result follows from the two propositions above.

Corollary 2.

Let X be a non-empty convex subset of a topological vector space. Every complete, transitive and continuous binary relation ≿ on X has the strong IVP.

3.3. A Restricted IVP in Measurement Theory

In this subsection, we interpret the restricted solvability axiom in the measurement theory literature as a restricted version of the IVP. The usage of this axiom can be traced back to [33,34] with its full consequences for representation theory brought out in Krantz, Luce, Suppes, and Tversky [35].38

Let $X_1,X_2\dots X_n$ be n non-empty sets where $n\in N,n\ge 2.$ We denote an element in X by $x=(x_1,x_2,\dots ,x_n),$ where $x_i\in X_i,$ for all $1\le i\le n,$ For any $x\in X,$ $x_{-i}$ denotes the element $(x_1,\dots ,x_{i-1},x_{i+1},\dots ,x_n)$ and $(x_i,x_{-i})$ denotes x.

Definition 11.

A relation ≿ on a subset A of $X={\prod }_{i=1}^n{X}_i$ satisfies restricted solvability with respect to the ith component if for any $x,y\in A$ and any $(a_i,y_{-i}),(b_i,y_{-i})\in A$ with $(a_i,y_{-i})\succsim x\succsim (b_i,y_{-i})$, there exists $c_i\in X_i$ with $(c_i,y_{-i})\in A$ such that $x\sim (c_i,y_{-i}).$ When this holds for all $i\in \{1,\dots ,n\}$, the binary relation is said to satisfy restricted solvability.

The following two examples illustrate the definition above.

Example 1.

Let $X={\mathbb{R}}^n,n\ge 2.$ Define ≿ on X as

$$(x_1,x_2,\dots ,x_n)\succsim (y_1,y_2,\dots ,y_n)\iff x_1+x_2+\dots +x_n\ge y_1+y_2+\dots +y_n$$

In this example, $(x_1,x_2,\dots ,x_n)\sim (y_1,y_2,\dots ,y_n)$ iff $x_1+x_2+\dots +x_n=y_1+y_2+\dots +y_n.$ For any given $2n-1$ out of the $2n$ variables, there exists a value for the $2n$-th variable such that the indifference of the binary relation is met. It is easy to verify that Example 1 satisfies restricted solvability for all the components. For any $i\in \{1,\dots ,n\},$ $y_1+\dots +y_{i-1}+a_i+y_{i+1}\dots +y_n\ge x_1+\dots +x_{i-1}+x_i+x_{i+1}\dots +x_n\ge y_1+\dots +y_{i-1}+b_i+y_{i+1}\dots +y_n,$ there exists $c_i\in X_i$ such that $x_1+\dots +x_{i-1}+x_i+x_{i+1}\dots +x_n=y_1+\dots +y_{i-1}+c_i+y_{i+1}\dots +y_n.$

Example 2.

Let $X={\mathbb{R}}^2.$ Define ≿ on X as $(x_1,x_2)\succsim (y_1,y_2)\mathit{iff}{x}_1\ge y_{1}or{x}_1=y_1,x_2\ge y_2$. The relation ≿ is called lexicographic. Now, we show that ≿ does not satisfy restricted solvability. Given $(\frac{1}{2},1)\succsim (\frac{1}{3},0)\succsim (0,1),$ there exists no $x\in \mathbb{R}$ such that, $(\frac{1}{3},0)\sim (x,1).$ Therefore, it is not restricted solvable.

Restricted solvability with respect to the ith component is analogous to the IVP of a binary relation restricted to the ith component while keeping all other components fixed. Hence, we can interpret the restricted solvability as a restricted IVP for binary relations. The following result directly follows from Definitions 10 and 11, and shows that the IVP implies restricted solvability (restricted IVP).

Proposition 7.

If a binary relation satisfies the IVP, then it satisfies restricted solvability.

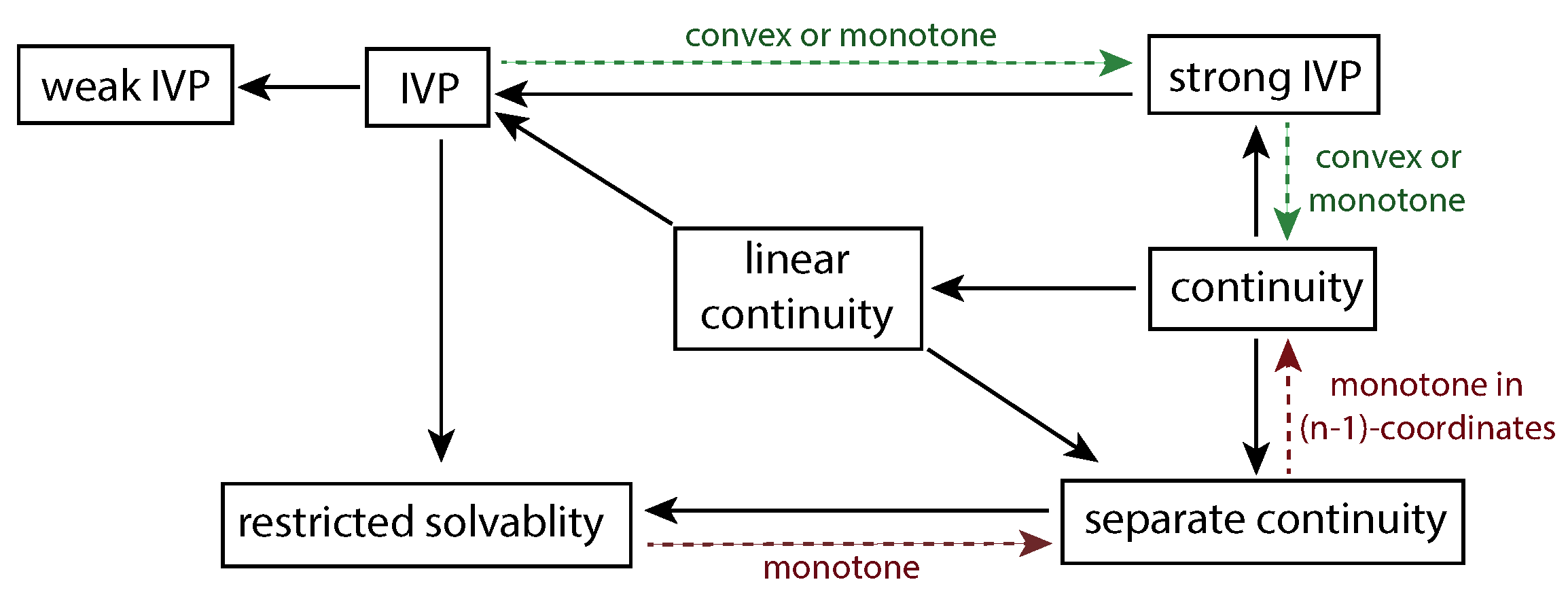
4. The Principal Results

In this section, we provide new results on the relationship among different continuity postulates. The first subsection concerns equivalence results on functions under weak monotonicity or quasi-concavity, or quasi-convexity assumptions. These results not only provide converse of the IVT, but also provide equivalences of various well-known continuity assumptions on functions. In the second subsection, we provide equivalence theorems for binary relations. Most of these equivalences on binary relations are already presented in authors’ recent work. In this subsection, we observe that the IVP (with added order denseness) is analogous to weak Wold-continuity and include the IVP (and strong IVP) in the equivalences. The novelty of these theorems lies in obtaining results that go beyond forging connections that were not brought into salience, hitherto; they give a new perspective on the continuity property.39

4.1. Equivalence Theorems for Functions

For a function on a finite-dimensional Euclidean space, many versions of continuity have been provided: (joint) continuity, linear continuity and separate continuity, in addition to the IVP. A real valued function is linearly continuous if it is continuous on any arbitrary straight line in its domain and it is separately continuous if it is continuous on any straight line in its domain that is parallel to a coordinate axis. The distinction between continuity and separate continuity in each individual variable is to be sure a staple of the first course in real analysis, but linear continuity is perhaps less a part of the vernacular, at least in so far it is current in economics curriculum; see Ciesielski and Miller [92] for a detailed discussion on these different continuity postulates. It follows from their definitions that continuity implies linear continuity, and linear continuity implies separate continuity. Genocchi and Peano [93] (pp. 173–174) provide examples showing that the converse relationships are false.40 There are partial results showing that converse relationships hold under additional assumptions on functions. On this, Kruse and Deely [94] and Young [95] show that for the class of functions satisfying a weak monotonicity assumption, continuity, separate continuity and linear continuity are equivalent. Similarly, Uyanik and Khan [20] show that for the class of quasi-concave, or quasi-convex, functions, linear continuity is equivalent to continuity.41

In this section, we carry this direction forward and provide equivalence theorems among continuity postulates for functions under quasi-concavity, or quasi-convexity or weak monotonicity assumptions. These postulates include, in addition to the three continuity postulates above, the strong IVT, the IVP and the restricted solvability concept for functions that is analogous to Definition 11 for restricted solvability of binary relations.

Definition 12.

Let f be a real-valued function defined on $X\subseteq {\mathbb{R}}^n$. We say f satisfies restricted solvability with respect to the ith component if for any $k\in \mathbb{R}$, $(a_i,y_{-i})\in X$ and $(b_i,y_{-i})\in X$ with $f(a_i,y_{-i})\ge k\ge f(b_i,y_{-i})$, there exists $(c_i,y_{-i})\in X$ such that $f(c_i,y_{-i})=k.$ When this holds for all n-components, the function f is said to satisfy restricted solvability.

Next, we present a result on the relationship between different continuity assumptions on functions.

Proposition 8.

Let $X\subseteq {\mathbb{R}}^n$ be a convex set and $f:X\to \mathbb{R}$ be a function. Then, the following relationships among the continuity postulates are true:

(a)

continuity ⇒ linear continuity ⇒ IVP ⇒ restricted solvability,

(b)

linear continuity ⇒ separate continuity ⇒ restricted solvability,

(c)

continuity ⇒ strong IVP ⇒ IVP ⇒ weak IVP.

Now, we provide two theorems on the equivalence among various continuity postulates on functions under quasi-concavity, or quasi-convexity, or weak monotonicity assumptions.

Theorem 13

(Portmanteau A). Let f be a quasi-concave (or quasi-convex) real-valued function on a convex subset X of ${\mathbb{R}}^n$ that is either open or a polyhedron.42 Then, the following continuity postulates are equivalent: continuity, linear continuity, strong IVP and IVP.

Theorem 14

(Portmanteau B). Let f be a weakly monotone43 real-valued function on a convex subset X of ${\mathbb{R}}^n$ that is bounded by the usual binary relation ≥. Then on intX,44 the following continuity postulates are equivalent: continuity, linearly continuity, separate continuity, restricted solvability, strong IVP and IVP.

The proposition and the two theorems in this subsection not only provide converses of the IVT on multi-dimensional spaces, but also provide portmanteau equivalence results among various continuity postulates for functions defined on subsets of product spaces. Figure 1 illustrates these relationships.

We end this section by illustrating an example showing that the weak IVP provided in Definition 4 does not imply any of the continuity assumptions in Figure 1 even under weak monotonicity and quasi-concavity assumptions.

Example 3.

Let $X={[0,1]}^2$ and $f:X\to \mathbb{R}$ defined as follows: $f\left(x\right)=x_1$ for all x such that $x_2\ne 0$, and $f(x_1,0)=0$ if $x_1<1$ and $f(1,0)=1$. It is easy to observe that f is quasi-concave and weakly monotone, satisfies the weak IVP, but it is discontinuous. By the two equivalence theorems above, this function does not satisfy any of the continuity assumptions in Figure 1 except the weak IVP.

4.2. Equivalence Theorems for Binary Relations

In this subsection, we present a proposition and two equivalence theorems that establish relationships among various continuity postulates, the solvability axiom and IVP for a binary relation under minimal assumptions. These extend the results of Uyanik and Khan [20] and Ghosh, Khan, and Uyanik [21] by integrating the IVP with continuity postulates, which is one of the novel contributions of this survey.45 The proposition provides the relationships without imposing additional assumptions on the binary relation. The first equivalence theorem presents additional relationships forming equivalences for convex binary relations, the second equivalence theorem shows that additional relationships and equivalences are formed under weak monotonicity assumption.

Proposition 9.

Let ≿ be a complete and transitive binary relation on a convex set in ${\mathbb{R}}^n$. Then, the following relationships among the continuity postulates hold.

(a)

Graph continuity ⇔ continuity ⇒ linear continuity ⇔ mixture continuity ⇒ Archimedean and separate continuity and weak Wold continuity and IVP

(b)

Continuity ⇒ Wold-continuity ⇔ strong IVP and order denseness ⇒ weak Wold-continuity ⇔ IVP and order denseness ⇒ Archimedean

(c)

Separate continuity or IVP ⇒ restricted solvability

Next, we present the first equivalence theorem for convex binary relations.

Theorem 15

(Portmanteau C). Let ≿ be a complete, transitive, order dense and convex binary relation on $X\subseteq {\mathbb{R}}^n$ that is either open or a polyhedron. Then the following continuity postulates are equivalent: graph continuity, continuity, mixture continuity, linear continuity, Archimedean, Wold-continuity, weak Wold-continuity, strong IVP and IVP.

We require the weak monotonicity assumption to establish equivalence between restricted solvability, separate continuity and continuity assumptions.46 The following theorem shows that under the weak monotonicity assumption, all continuity postulates for a complete and transitive binary relation we present in this paper are equivalent on a large class of subsets of ${\mathbb{R}}^n$.

Theorem 16

(Portmanteau D). Let ≿ be a complete, transitive, order dense and weakly monotonic binary relation on convex set $X\subseteq {\mathbb{R}}^n$ that is bounded by the usual relation ≥ on ${\mathbb{R}}^n$. Then on intX, the following continuity postulates are equivalent: graph continuity, continuity, mixture continuity, linear continuity, Archimedean, Wold-continuity, weak Wold-continuity, strong IVP, IVP, restricted solvability and separate continuity.

The proposition and the two theorems in this subsection are presented in Uyanik and Khan [20] and Ghosh, Khan, and Uyanik [21] except the relationships concerning the IVP and the strong IVP. Proposition 9 establishes the equivalence of the IVP and weak Wold continuity, as well as the strong IVP and Wold continuity. Therefore, the proofs of Theorems 15 and 16 follow from the two earlier work of the authors and Proposition 9. Figure 2 illustrates the relationship among different continuity postulates.

5. Proofs of the Results

Proof of Proposition 1.

Assume a function $f:X\to Y$ satisfies the continuity property provided in the theorem but fails the IVP. Then, there exists $x,y\in X$ and $c\in Y$ lying between $f\left(x\right)$ and $f\left(y\right)$ such that for all $\lambda \in [0,1]$, $f\left(x\lambda y\right)\ne c$. By the argument in Munkres [70] (p. 86), the sets $Y_{\succ }\left(c\right)=\{a\in Y:a\succ c\}$ and $Y_{\prec }\left(c\right)=\{a\in Y:c\succ a\}$ are open in Y. By the continuity assumption on f, $\{\lambda \in [0,1]:f\left(x\lambda y\right)\in Y_{\succ }\left(c\right)\}$ and $\{\lambda \in [0,1]:f\left(x\lambda y\right)\in Y_{\prec }\left(c\right)\}$ are open in [0, 1]. Since ≻ is transitive, these two sets are disjoint. By assumption that $f\left(x\lambda y\right)\ne c$ for all $\lambda \in [0,1]$ and c lies between $f\left(x\right)$ and $f\left(y\right)$, these two sets are disjoint. This contradicts the connectedness of [0, 1]. Therefore, f satisfies the IVP. □

Proof of Proposition 2.

Assume a function $f:X\to Y$ continuous but fails the strong IVP. Then, there exists $x,y\in X$, a curve $C_{xy}$ connecting x and y, and $c\in Y$ lying between $f\left(x\right)$ and $f\left(y\right)$ such that for all $z\in C_{xy}$, $f\left(z\right)\ne c$. By the argument in Munkres [70] (p. 86), the sets $Y_{\succ }\left(c\right)=\{a\in Y:a\succ c\}$ and $Y_{\prec }\left(c\right)=\{a\in Y:c\succ a\}$ are open in Y. By the continuity of f, $\{z\in C_{xy}:f\left(z\right)\in Y_{\succ }\left(c\right)\}$ and $\{z\in C_{xy}:f\left(z\right)\in Y_{\prec }\left(c\right)\}$ are open in $C_{xy}$. Since ≻ is transitive, these two sets are disjoint. By assumption that $f\left(z\right)\ne c$ for all $z\in C_{xy}$ and c lies between $f\left(x\right)$ and $f\left(y\right)$, these two sets are disjoint. This contradicts the connectedness of $C_{xy}$. Therefore, f satisfies the strong IVP. □

Proof of Proposition 5.

Assume ≿ is continuous but does not satisfy the strong IVP. Then, there exists $x,y,z\in X$ with $x\succsim y\succsim z$ and a curve C connecting x and z (which is induced by a continuous and injective function $m_{xz}:[0,1]\to X$) such that for all $c\in C$, $y\succ c$ or $c\succ y$. Since $[0,1]$ is connected and $m_{xz}$ is continuous, the curve C induced by $m_{xz}$ is a connected subset of X. Define $C_{\succsim }\left(y\right)=\{y^{\prime }\in C|y^{\prime }\succsim y\}$ and $C_{\precsim }\left(y\right)=\{y^{\prime }\in C|y\succsim y^{\prime }\}$. Since ≿ is complete and continuous, $C_{\succsim }\left(y\right)$ and $C_{\precsim }\left(y\right)$ are closed and covers C. Since $x\succ y\succ z$, $C_{\succsim }\left(y\right)$ and $C_{\precsim }\left(y\right)$ are non-empty. Finally, since ≿ is transitive (which implies ≻ is transitive), $C_{\succsim }\left(y\right)$ and $C_{\precsim }\left(y\right)$ are disjoint. This contradicts connectedness of C. Therefore, ≿ satisfy the strong IVP. □

Proof of Proposition 6.

Assume ≿ is mixture continuous but does not satisfy the IVP. Then, there exists $x,y,z\in X$ with $x\succsim y\succsim z$ such that for all $\lambda \in [0,1]$, $x\lambda z\succ y$ or $y\succ x\lambda z$. Note that $[0,1]$ is connected. It follows from completeness and mixture continuity of ≿ that, the sets $L_{\succsim }\left(y\right)=\{\lambda \in [0,1]|x\lambda z\succsim y\}$ and $L_{\precsim }\left(y\right)=\{\lambda \in [0,1]|y\succsim x\lambda z\}$ are closed and covers $[0,1]$. Since $x\succsim y\succsim z$, $L_{\succsim }\left(y\right)$ and $L_{\precsim }\left(y\right)$ are non-empty. It follows from transitivity of ≿ (which implies ≻ is transitive) that $L_{\succsim }\left(y\right)$ and $L_{\precsim }\left(y\right)$ are disjoint. This contradicts connectedness of $[0,1]$. Therefore, ≿ satisfy the IVP. □

Proof of Proposition 8.

Let X be a convex subset of ${\mathbb{R}}^n$ and $f:X\to \mathbb{R}$ a function.

(a)

The statement that continuity implies linear continuity, and that IVP implies restricted solvability follow from their definitions. Next, we show that linear continuity implies IVP. Pick $x,y\in X$ and $c\in \mathbb{R}$ such that $f\left(x\right)<c<f\left(y\right)$. Since f is linearly continuous, it is continuous on the straight line segment $L_{xy}$ connecting x and y. Consider the following sets: $f^{-1}(c,\infty )\cap L_{xy}$ and $f^{-1}(-\infty ,c)\cap L_{xy}$. These sets are non-empty, disjoint and open in $L_{xy}$. If there is no $z\in L_{xy}$ such that $f\left(z\right)=c$, these two sets is an open covering of $L_{xy}$. Since $L_{xy}$ is a connected set, this furnishes us a contradiction. Therefore, f satisfies the IVP.

(b)

The argument that linear continuity implies separate continuity follows from their definitions. Next, we show that separate continuity implies restricted solvability. Separate continuity implies that f is continuous in any line parallel to any coordinate axis, and restricted solvability is implied by the fact that IVP holds on any given index. Hence, Theorem 2 completes the proof.

(c)

The strong IVP implies the IVP, and the IVP implies the weak IVP follow from their definitions. It remains to prove that continuity implies the strong IVP. Pick $x,y\in X$, a curve $C_{xy}$ connecting x and y and $c\in \mathbb{R}$ such that $f\left(x\right)<c<f\left(y\right)$. Consider the following sets: $f^{-1}(c,\infty )\cap C_{xy}$ and $f^{-1}(-\infty ,c)\cap C_{xy}$. These sets are non-empty, disjoint and open in $C_{xy}$. If there is no $z\in C_{xy}$ such that $f\left(z\right)=c$, these two sets are an open covering of $C_{xy}$. Since a curve is a connected set, this furnishes us a contradiction. Therefore, f satisfies the strong IVP.

□

Proof of Theorem 13.

Let X be a convex subset of ${\mathbb{R}}^n$ that is either open or polyhedron and $f:X\to \mathbb{R}$ a quasi-concave function. Proposition 8 provides one-directional relationships. Uyanik and Khan [20] (Theorem 1) shows that linear continuity implies continuity. Hence, it remains to show that IVP implies linear continuity to complete the proof.

Assume f satisfies IVP but fails linear continuity. Then, there exists a straight line L in X such that the restriction of f on L is not continuous. Since f is continuous on L if and only if it is both upper and lower semicontinuous on L, the restriction of f on L fails upper or lower semicontinuity.

First, assume the restriction of f on L is not upper semicontinuous. Then, there exist $x,y\in L$, $c\in \mathbb{R}$ and a convergent sequence ${\lambda }^k\to \lambda$ such that $f\left(x{\lambda }^{k}y\right)\ge c$ for all k and $c>f\left(x\lambda y\right)$. If ${\lambda }^k\le \lambda \le {\lambda }^m$ for some $k,m$, then quasi-concavity of f implies that $f\left(x\lambda y\right)\ge c$. Therefore, either ${\lambda }^n>\lambda$ for all n or ${\lambda }^n<\lambda$ for all n. Assume without loss of generality that ${\lambda }^n>\lambda$ for all n. Then, quasi-convexity of f and ${\lambda }^k\to \lambda$ imply that $f\left(x{\lambda }^{\prime }y\right)\ge c$ for all ${\lambda }^{\prime }\in (\lambda ,{\lambda }^1]$. Next, we show that there exists $\overline{\lambda }\in (\lambda ,{\lambda }^1]$ such that $c=f\left(x\overline{\lambda }y\right)$. If $f\left(x{\lambda }^{\prime }y\right)>c$ for some ${\lambda }^{\prime }\in (\lambda ,{\lambda }^1]$, then $c>f\left(x\lambda y\right)$ and weak IVP imply that there exists $\delta \in (0,1)$ such that $c=f\left(\left(x{\lambda }^{\prime }y\right)\delta \left(x\lambda y\right)\right)=f\left(x(\delta {\lambda }^{\prime }+(1-\delta )\lambda )y\right)$. Otherwise, $f\left(x{\lambda }^{\prime }y\right)=c$ for all ${\lambda }^{\prime }\in (\lambda ,{\lambda }^1]$. Now, it follows from weak IVP of f, and $f\left(x\overline{\lambda }y\right)=c>f\left(x\lambda y\right)$ that there exists $\widehat{\lambda }\in (\lambda ,\overline{\lambda })$ such that $f\left(x\overline{\lambda }y\right)>f\left(x\widehat{\lambda }y\right)>f\left(x\lambda y\right)$. Then, $c=f\left(x\overline{\lambda }y\right)>f\left(x\widehat{\lambda }y\right)$ imply that $c>f\left(x\widehat{\lambda }y\right)$. Then, $\widehat{\lambda }\in (\lambda ,{\lambda }^1]$ contradicts quasi-concavity of f.

Second, assume the restriction of f on L is not lower semicontinuous. Then, there exist $x,y\in X$, $c\in \mathbb{R}$ and a convergent sequence ${\lambda }^k\to \lambda$ such that $c\ge f\left(x{\lambda }^{k}y\right)$ for all k and $f\left(x\lambda y\right)>c$. Assume without loss of generality that there exists a subsequence ${\lambda }^{k_i}$ of ${\lambda }^k$ such that ${\lambda }^{k_i}>\lambda$ for all $i=1,2,\dots$. Next, we show that there exists $\overline{\lambda }\in (\lambda ,{\lambda }^{k_1}]$ such that $c=f\left(x\overline{\lambda }y\right)$. If $c>f\left(x{\lambda }^{k_1}y\right)$, then $f\left(x\lambda y\right)>c$ and weak IVP imply that there exists $\delta \in (0,1)$ such that $c=f\left(\left(x{\lambda }^{k_1}y\right)\delta \left(x\lambda y\right)\right)=f\left(x(\delta {\lambda }^{k_1}+(1-\delta )\lambda )y\right)$. Otherwise, $c=f\left(x{\lambda }^{k_1}y\right)$. Then, it follows from weak IVP of f and $f\left(x\lambda y\right)>c=f\left(x\overline{\lambda }y\right)$ that there exists $\widehat{\lambda }\in (\lambda ,\overline{\lambda })$ such that $f\left(x\lambda y\right)>f\left(x\widehat{\lambda }y\right)>f\left(x\overline{\lambda }y\right)$. Then, $c=f\left(x\overline{\lambda }y\right)<f\left(x\widehat{\lambda }y\right)$ implies that $c<f\left(x\widehat{\lambda }y\right)$. Moreover, quasi-concavity of f implies that for all ${\lambda }^{\prime }\in [\lambda ,\widehat{\lambda }]$, $f\left(x{\lambda }^{\prime }y\right)\ge f\left(x\widehat{\lambda }y\right)$. Since ${\lambda }^{k_i}\to \lambda$, there exists j such that ${\lambda }^{k_j}\in (\lambda ,\widehat{\lambda })$. Then, $c\ge f\left(x{\lambda }^{k_j}y\right)\ge f\left(x\widehat{\lambda }y\right)>c$ furnishes us a contradiction. □

Proof of Theorem 14.

Let X be a convex subset of ${\mathbb{R}}^n$ that is bounded by the usual relation in ${\mathbb{R}}^n$, and $f:X\to \mathbb{R}$ a weakly monotone function. Proposition 8 provides one-directional relationships. Young [95] and Kruse and Deely [94] show that separate continuity implies continuity. Hence, showing that restricted solvability implies separate continuity completes the proof.47 Assume f satisfies restricted solvability but fails separate continuity. Then, there exists a straight line $L_i$ in X that is parallel to a coordinate axis i such that the restriction of f on $L_i$ is not continuous. Since f is continuous on $L_i$ if and only if it is both upper and lower semicontinuous on $L_i$, the restriction of f on $L_i$ fails upper or lower semicontinuity.

First, assume the restriction of f on $L_i$ is not upper semicontinuous. Then, there exist $y\in L_i$, $c\in \mathbb{R}$ and a convergent sequence $y^k\to y$ on the line $L_i$ such that $f\left(y^k\right)\ge c$ for all k and $f\left(y\right)<c$. Then, it follows from weak monotonicity of f and $y^k,y\in L_i$ that $y_i^k>y_i$ for all k. Pick $m\in \mathbb{N}$. Since $f\left(y\right)<c\le f\left(y^m\right)$, there exists $c^{\prime }<c$ such that $f\left(y\right)<c^{\prime }<f\left(y^m\right)$, and hence restricted solvability implies there exists $z\in L_i$ such that $f\left(z\right)=c^{\prime }$. It follows from weak monotonicity that $y_i<z_i<y_i^m$. Then, for all $z_i^{\prime }\in (y_i,z_i]$, $f(z_i^{\prime },z_{-i})\le c^{\prime }<c$. Since $y^k\to y$, there exists $z_i^{\prime }\in (y_i,z_i]$, such that $z_i^{\prime }=y_i^k$ for some k and $f(z_i^{\prime },z_{-i})\ge c$. This yields a contradiction. An analogous argument show that the failure of the restriction of f on $L_i$ being lower semicontinuous yields a contradiction. □

Proof of Proposition 9.

First, note that a careful investigation of the definitions of weak Wold continuity and the IVP shows that weak Wold continuity is equivalent to the IVP and order denseness. Analogously, Wold continuity is equivalent to the strong IVP and order denseness. Moreover, a similar investigation shows that the IVP implies restricted solvability. Next, we show that IVP implies Archimedean property. Towards this end, assume ≿ satisfies the IVP but does not satisfy the Archimedean property. Then, there exists $x,y,z\in X$ such that $x\succ y$ but for all $\lambda \in (0,1)$, $x\lambda z\nsucc y$ (the proof of the case where $x\nsucc y\lambda z$ is analogous). By completeness, $y\succcurlyeq x\lambda z$. Pick $\lambda \in (0,1)$. If $y\succ x\lambda z$, then $x\succ y\succ x\lambda z$ and the IVP imply there exist $\delta \in (0,1)$ such that $y\sim x\delta z$. If $y\sim x\lambda z$, then set $\delta =\lambda$. Then, by transitivity, $x\succ x\delta z$. By order denseness, there exists $\gamma \in (0,1)$ such that $x\succ x\gamma z\succ x\delta z\sim y$. By transitivity, $x\gamma z\succ y$. This furnishes us a contradiction with the assumption that for all ${\lambda }^{\prime }\in (0,1)$, $x{\lambda }^{\prime }z\nsucc y$.

All the remaining relationships are proved in [20,21]. □

6. Conclusions

The perceptive reader may have already noted that the entire investigation hinged on the binary relation being a weak order (complete and transitive). In 1956, Duncan Luce questioned the notion of an indifference curve and noted that “this assumption is contrary to experience and that utility is not perfectly discriminable, as such a theory necessitates”. He then presented a theory that admits intransitive indifferences by proposing semiorders: a “class that can be shown to be substantially equivalent to a utility theory in which there are just noticeable difference functions which state for any value of utility the change in utility so that the change is just noticeable”. We recall the setup of Luce [109] for the convenience of our readers: in what follows, X is a set and P and I two binary relations defined over X.

Theorem 17

(Luce). $(P,I)$ is a semiorder if and only if P is transitive and $(\succ ,\sim )$ is a weak order, the latter defined as being induced on X by a given relation $(P,I)$ as follows:

(i) 

$a\succ b$ if either (a) $aPb$, (b) $aIb$ and there exists $c\in X$ such that $aIc$ and $cPb,$ or (c) $aIb$ and there exists $d\in X$ such that $aPd$ and $dIb,$

(ii) 

If neither $a\succ b$ nor $b\succ a,$ then $a\sim b.$

Luce’s theory has by now attained substantial maturity, and a distinguished following. In Giarlotta and Watson [110], for example, we read:

The concept of semiorder originally appeared in 1914–albeit under a different name–in the work of Fishburn and Monjardet (1992) and Wiener (1914). Nowadays, a semiorder is equivalently defined as either a reflexive and complete relation that is Ferrers and semitransitive (sometimes called a weak semiorder), or an asymmetric relation that is Ferrers and semitransitive (sometimes called a strict semiorder).48

The first open direction of work that we want to single out is whether, and how, the consolidated theory of equivalences between continuity, solvability and intermediate valuedness presented in this paper carries over to a semiorder.

The second direction for further investigation that we single out, and this time from the side of economics, revolves around the notion of a bi-preference. This has recently been given increased life and attention in the work of Giarlotta and his followers: in addition to [28,112,113,114]. However, the point worth noting is that this recent emphasis notwithstanding, bi-preferences entered mathematical economics not only with Sen, Marglin and Feldstein in their Kantian distinctions between the personal and the public preferences,49 but also more directly with the monotonicity assumption in both Walrasian and Cournot–Nash equilibrium theory, as well as in decision theory. The point emphasized in the bi-preference literature relates to an investigation of conditions under which properties of a hard relation imply corresponding properties of a soft relation. This is precisely what is being accomplished by the monotonicity postulate, and so, the open question is whether, and how, the consolidated theory presented in this paper carries over to the setting of bi-preferences.

We conclude as we began, by referring to IVT and IVP for functions and bring to the attention of the reader the the converse of Poincaré–Miranda theorem, or similar extensions of the IVT, for functions mapped from a subset of finite dimensional space to the space itself still remain open.