Modelling Social Dynamics (of Obesity) and Thresholds
Abstract
:1. Introduction
- The agent’s actions depend on the others’ (peers, averages, norms, law, etc.) actions. Since actions are a flow, corresponding social interactions are essentially static yielding a game of the type investigated first in [8] and later in many papers, e.g., in [9,10,11] that introduce moderate social influence as sufficient condition for unique outcomes in static social interaction games. More precisely, assume that the payoff of each player depends on the own action, in our case the own body mass index (BMI) b, and on the average , then moderate social influence (MSI), i.e.,is sufficient for a unique equilibrium of this static game. [12] applies the concept to quantify social multipliers.
- The agent’s preferences are affected by the relation of an individual stock, say wealth, education, weight, etc. vis a vis the same stock held by others. This requires a dynamic analysis, because the evolution of the stock, individually and aggregate, must be analyzed. This set up is considered in [6] in a fairly general form.
- Finally, the socially relevant variable is itself a dynamic process affected by the aggregate of private actions and stocks. In this case an additional differential equation traces the evolution of this ‘norm’, which is affected by the agent’s actions and stocks and which affects the individual preferences. For example how people like to dress will influence the evolution of fashions which in turn influences how people feel in their dress.
2. Social Reference Given by the Average Stock
2.1. Framework
2.2. Applications to Obesity

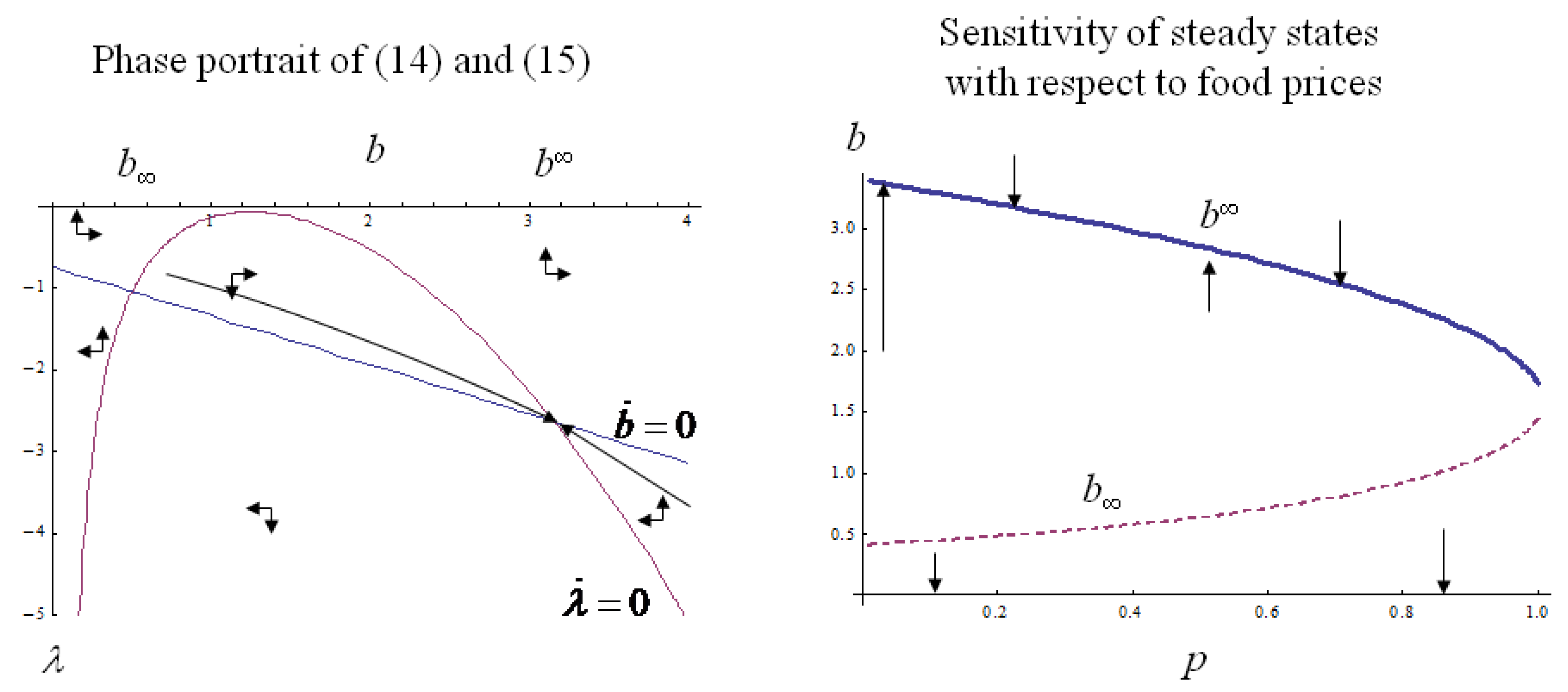
2.3. Other Applications
2.3.1. Labor Market
2.3.2. Wealth Status
2.3.3. Addiction
2.3.4. Fashions
3. Model II—Dynamic Social Norms
- Either or saddlepoint stability, i.e., two eigenvalues are either negative or have negative real parts.
- A pair of complex eigenvalues with non-negative real parts, thus local instability and ; yet the converse does not hold, i.e., , and still allow for saddlepoint stability.
- (where and ) leads to a pair of purely imaginary eigenvalues. Possibly associated with this ‘bifurcation’ are limit cycles. In fact, stable limit cycles exist.
- implies instability, i.e., only one eigenvalue is negative, the other two are positive or have positive real parts.
- Total stability, i.e., all eigenvalues are negative or have negative real parts iff and .
3.1. Application to Obesity
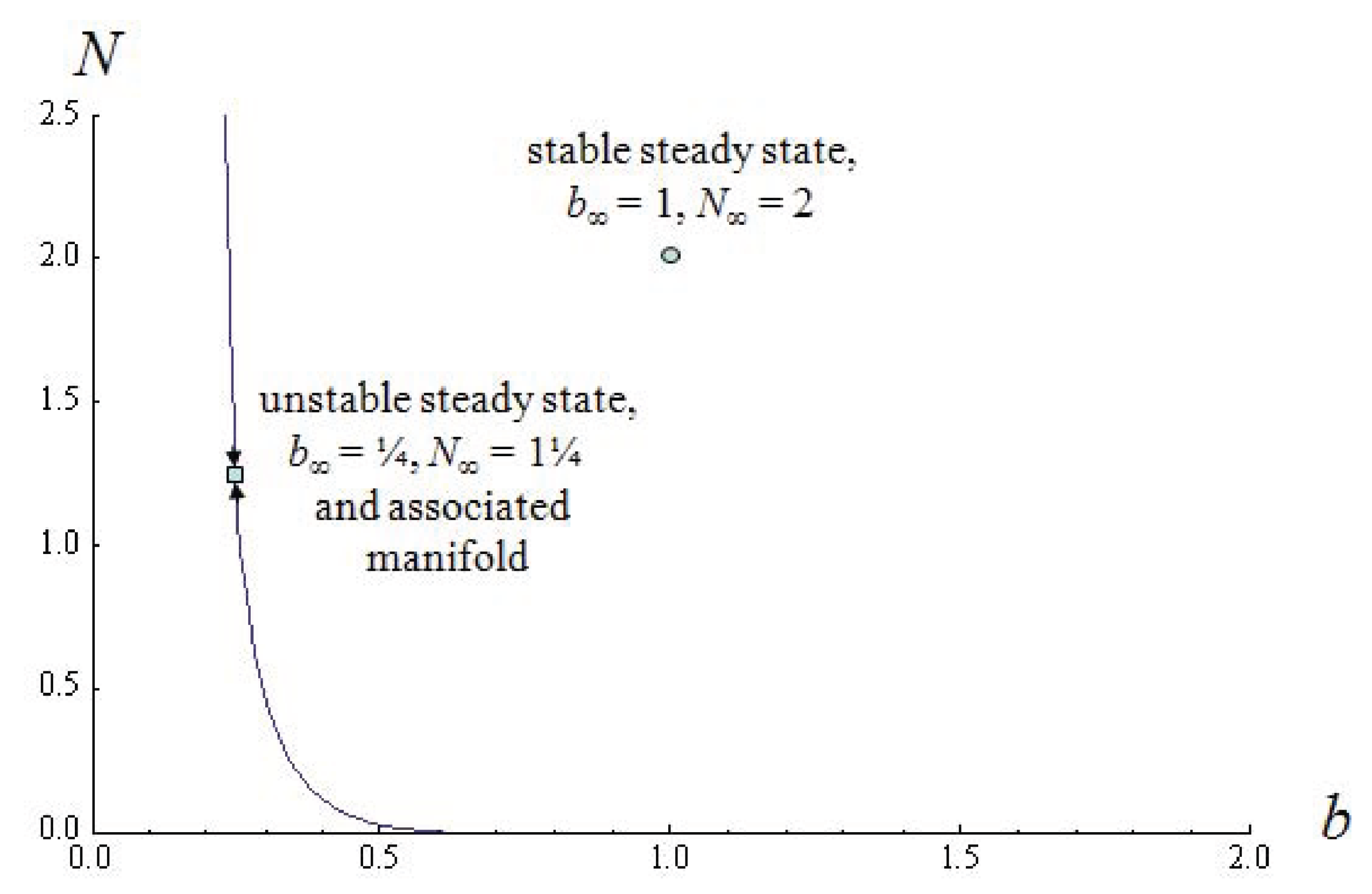
4. Extensions
4.1. Additional (Private) States
Gourmet
Wealth
4.2. Cellular Automata
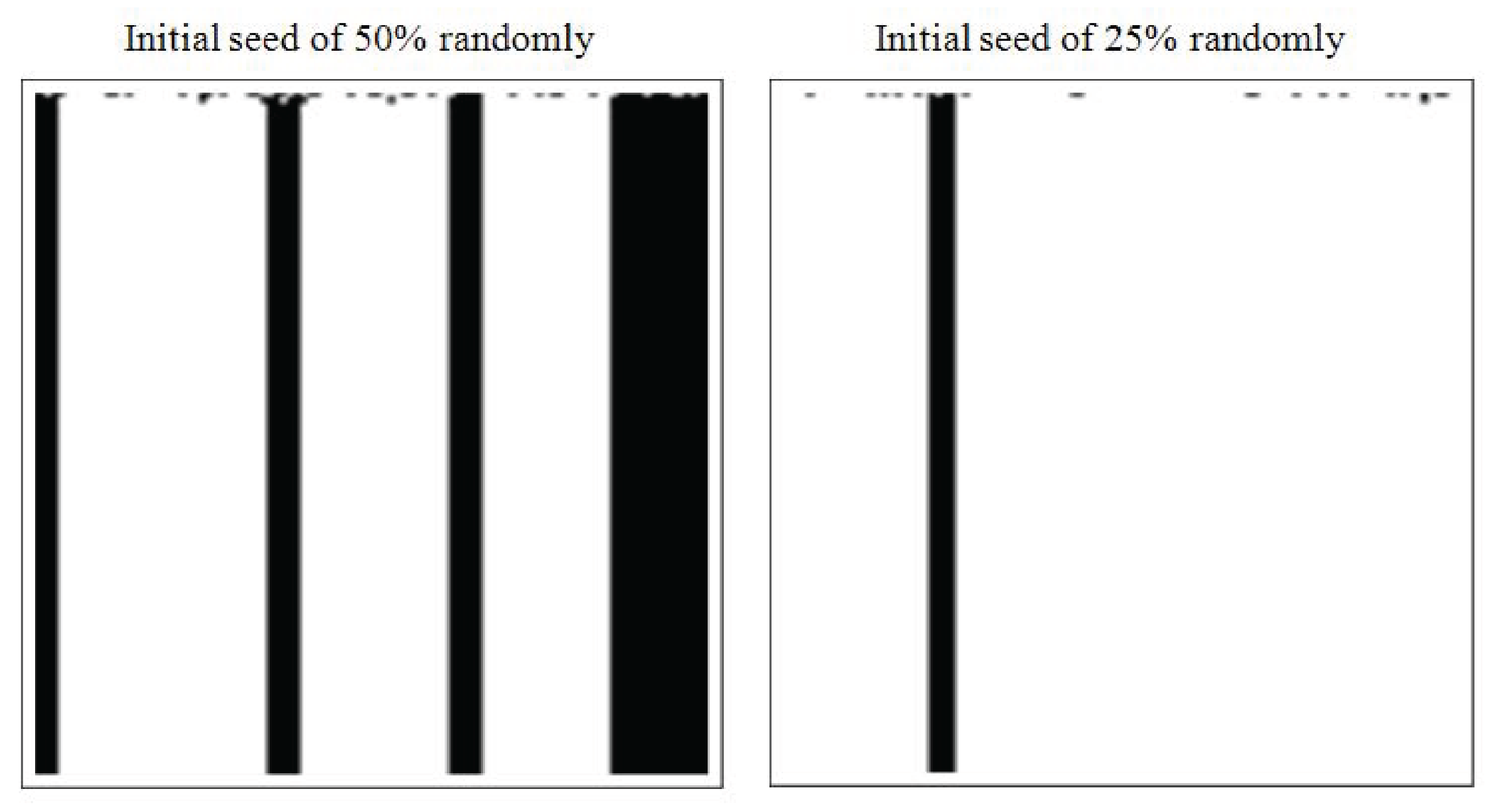
5. Final Remarks
References
- Wirl, F.; Feichtinger, G. History Dependence in Concave Economies. J. Econ. Behav. Organ. 2005, 57, 390–407. [Google Scholar] [CrossRef]
- Durlauf, S.N.; Young, H.P. Social Dynamics; MIT Press Cambridge: Cambridge, MA, USA, 2001. [Google Scholar]
- Burke, M.A.; Heiland, F. Social Dynamics of Obesity. Econ. Inq. 2007, 45, 571–591. [Google Scholar] [CrossRef]
- Cutler, D.M.; Glaeser, E.L.; Shapiro, J.M. Why have Americans Become more Obese? J. Econ. Perspect. 2003, 17, 93–118. [Google Scholar] [CrossRef]
- Cohen-Cole, E.; Fletcher, J.M. Is Obesity Contagious? Social Networks vs. Environmental Factors in the Obesity Epidemic. J. Health Econ. 2008, 27, 1382–1387. [Google Scholar] [CrossRef] [PubMed]
- Fowler, J.H.; Christakis, N.A. Estimating Peer Effects on Health in Social Networks: A Response to Cohen-Cole and Fletcher; Trogdon, Nonnemaker, Pais. J. Health Econ. 2008, 27, 1400–1405. [Google Scholar] [CrossRef] [PubMed]
- Miller, B.J.; Lundgren, J.D. An Experimental Study of the Role of Weight Bias in Candidate Evaluation. Obesity 2010, 18, 712–718. [Google Scholar] [CrossRef] [PubMed]
- Cooper, R.; John, A. Coordinating Coordination Failures in Keynesian Models. Quart. J. Econ. 1988, CII, 441–463. [Google Scholar] [CrossRef]
- Mailath, G.J.; Postlewaite, A. The Social Context of Economic Decisions. J. Eur. Econ. Assoc. 2003, 1, 354–362. [Google Scholar] [CrossRef]
- DeMarzo, P.M.; Vayanos, D.; Zwiebel, J. Persuasion Bias, Social Influence and Unidimensional Opinions. Quart. J. Econ. 2003, 118, 651–667. [Google Scholar] [CrossRef]
- Glaeser, E.L.; Scheinkman, J.A. Non-Market Interaction. In Advances in Economics and Econometrics: Theory and Applications. Eighth World Congress; Dewatripoint, M., Hansen, L.P., Turnovsky, S., Eds.; Cambridge University Press: Cambridge, MA, USA, 2003; Volume 1, pp. 339–369. [Google Scholar]
- Glaeser, E.L.; Sacerdote, B.I.; Scheinkman, J.A. The Social Multiplier. J. Eur. Econ. Assoc. 2003, 1, 345–353. [Google Scholar] [CrossRef]
- Wirl, F. Social Interactions within a Dynamic Competitive Economy. J. Optimiz. Theor. Appl. 2007, 133, 385–400. [Google Scholar] [CrossRef]
- Brekke, K.A.; Kverndokk, S.; Nyborg, K. An Economic Model of Moral Motivation. J. Public Econ. 2003, 87, 1967–1983. [Google Scholar] [CrossRef]
- Krugman, P. History versus Expectations. Quar. J. Econ. 1991, CVI, 651–667. [Google Scholar] [CrossRef]
- Burke, M.A.; Heiland, F. Social Dynamics of Obesity. Econ. Inq. 2007, 45, 571–591. [Google Scholar] [CrossRef]
- Arthur, W.B. Increasing Returns and Path Dependence in the Economy; The University of Michigan Press: Ann Arbor, MI, USA, 1994. [Google Scholar]
- Wirl, F.; Feichtinger, G. History versus Expectations: Increasing Returns or Social Influence? J. Socio-Econo. 2006, 35, 877–888. [Google Scholar] [CrossRef]
- Hof, F.X.; Wirl, F. Wealth Induced Multiple Equilibria in Small Open Economy Versions of the Ramsey Model. Homo Oeconomicus 2008, 25, 1–22. [Google Scholar]
- Barro, R.J.; Sala-i-Martin, X. Economic Growth; Mc Graw Hill: Columbus, OH, USA, 1995. [Google Scholar]
- Becker, G.S.; Murphy, K.M. A Theory of Rational Addiction. J. Polit. Econ. 1988, 96, 675–700. [Google Scholar] [CrossRef]
- Wirl, F. Stability and Limit Cycles in Competitive Equilibria Subject to Adjustment Costs and Dynamic Spillovers. J. Econ. Dynamics Control 2002, 26, 375–398. [Google Scholar] [CrossRef]
- Wirl, F. Stability and Limit Cycles in One-Dimensional Dynamic Optimizations of Competitive Agents with a Market Externality. J. Evol. Econ. 1997, 7, 73–89. [Google Scholar] [CrossRef]
- Dockner, E.; Feichtinger, G. Cyclical Consumption Patterns and Rational Addiction. Amer. Econ. Rev. 1993, 83, 256–263. [Google Scholar]
- Ryder, H.E., Jr.; Heal, G.M. Optimal Growth with Intertemporally Dependent Preferences. Rev. Econ. Stud. 1973, 40, 1–31. [Google Scholar] [CrossRef]
- Wirl, F.; Feichtinger, G. Persistent Cyclical Consumption. Variations on the Becker-Murphy Model on Addiction. Ration. Soc. 1995, 7, 149–159. [Google Scholar] [CrossRef]
- Wolfram, S. Theory and Application of Cellular Automata: Advanced Series on Complex Systems; World Scientific Publishing: Singapore, 1986. [Google Scholar]
- Wolfram, S. Cellular Automata and Complexity: Collected Papers; Addison-Wesley Publishing Company: Reading, MA, USA, 1994. [Google Scholar]
- Wolfram, S. A New Kind of Science; Wolfram Media: Champaign, IL, USA, 2002. [Google Scholar]
- Berninghaus, S.; Schwabe, U. Conventions, Local Interaction, and Automata Networks. J. Evol. Econ. 1996, 6, 297–312. [Google Scholar] [CrossRef]
- Wirl, F. Socio-Economic Typologies of Bureaucratic Corruption and Implications. J. Evol. Econ. 1998, 8, 199–220. [Google Scholar] [CrossRef]
- Arthur, W.B. Inductive Reasoning and Bounded Rationality. Amer. Econ. Rev. 1994, 84, 406–411. [Google Scholar]
- Benabou, R.; Tirole, J. Incentives and Prosocial Behavior. Amer. Econ. Rev. 2006, 96, 1652–1678. [Google Scholar] [CrossRef]
- Bahr, D.B.; Browning, R.C.; Wyatt, H.R.; Hill, J.O. Exploiting Social Networks to Mitigate the Obesity Epidemic. Obesity 2009, 17, 723–728. [Google Scholar] [CrossRef] [PubMed]
Appendix
- 1This is opposite to [13] due a negative valued stock b and positive marginal utility from consumption, πc > 0.
© 2010 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/.)
Share and Cite
Wirl, F.; Feichtinger, G. Modelling Social Dynamics (of Obesity) and Thresholds. Games 2010, 1, 395-414. https://doi.org/10.3390/g1040395
Wirl F, Feichtinger G. Modelling Social Dynamics (of Obesity) and Thresholds. Games. 2010; 1(4):395-414. https://doi.org/10.3390/g1040395
Chicago/Turabian StyleWirl, Franz, and Gustav Feichtinger. 2010. "Modelling Social Dynamics (of Obesity) and Thresholds" Games 1, no. 4: 395-414. https://doi.org/10.3390/g1040395
APA StyleWirl, F., & Feichtinger, G. (2010). Modelling Social Dynamics (of Obesity) and Thresholds. Games, 1(4), 395-414. https://doi.org/10.3390/g1040395




