Caputo Fabrizio Bézier Curve with Fractional and Shape Parameters
Abstract
1. Introduction
2. Caputo Fabrizio Basis Function with Fractional and Shape Parameters
2.1. The Construction of the Caputo Fabrizio Basis Function
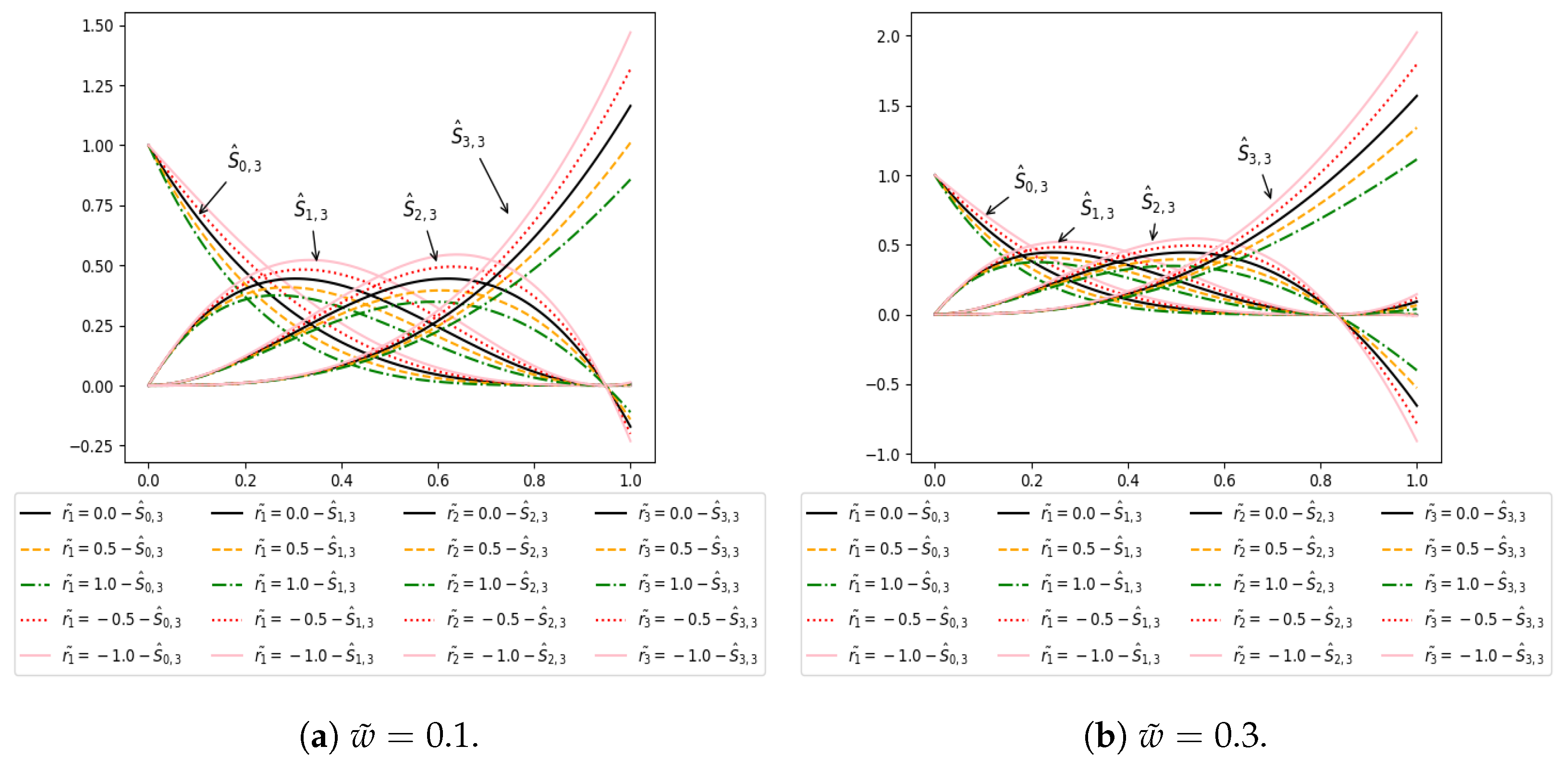
2.2. Properties of Caputo Fabrizio Basis Function
- (i)
- Partition of unity.
- (ii)
- Terminal property.
- (iii)
- Non-negativity.
- (i) Partition of unity.
- (ii) Terminal property.
- (iii) Non-negativity.
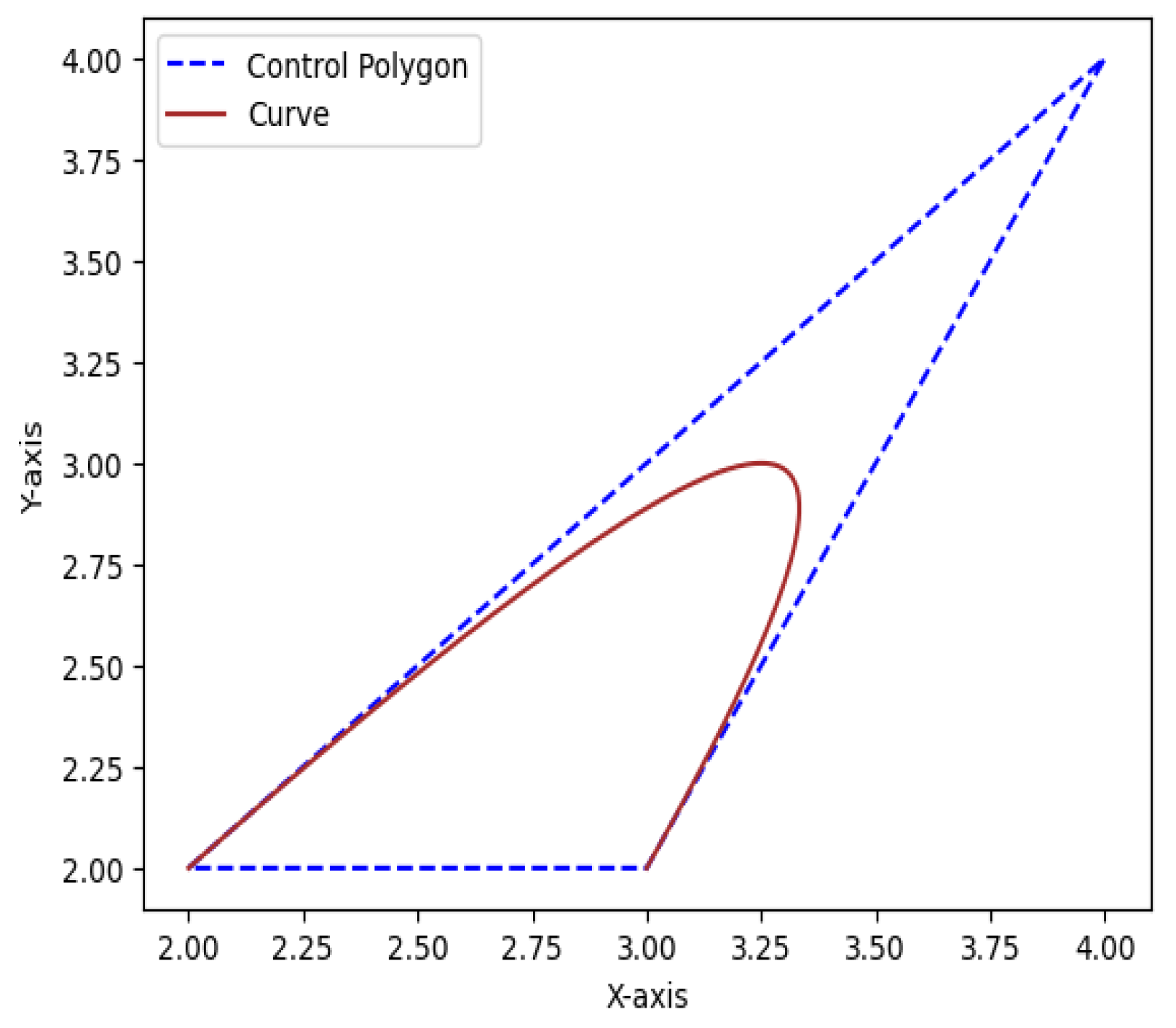
3. Caputo Fabrizio Bézier Curve with Fractional and Shape Parameters
Properties of Caputo Fabrizio Bézier Curve with Fractional and Shape Parameters
- Endpoint terminal.
- Convex hull.
- Symmetry.
- Geometric invariance.
- Shape-adjustable property.
- Curve’s length-adjustable property.
- i. Endpoint terminal.
- ii. Convex hull.
- iii. Symmetry.
- iv. Geometric invariance.
- v. Curve’s shape-adjustable property.
- vi. Curve’s length-adjustable property.
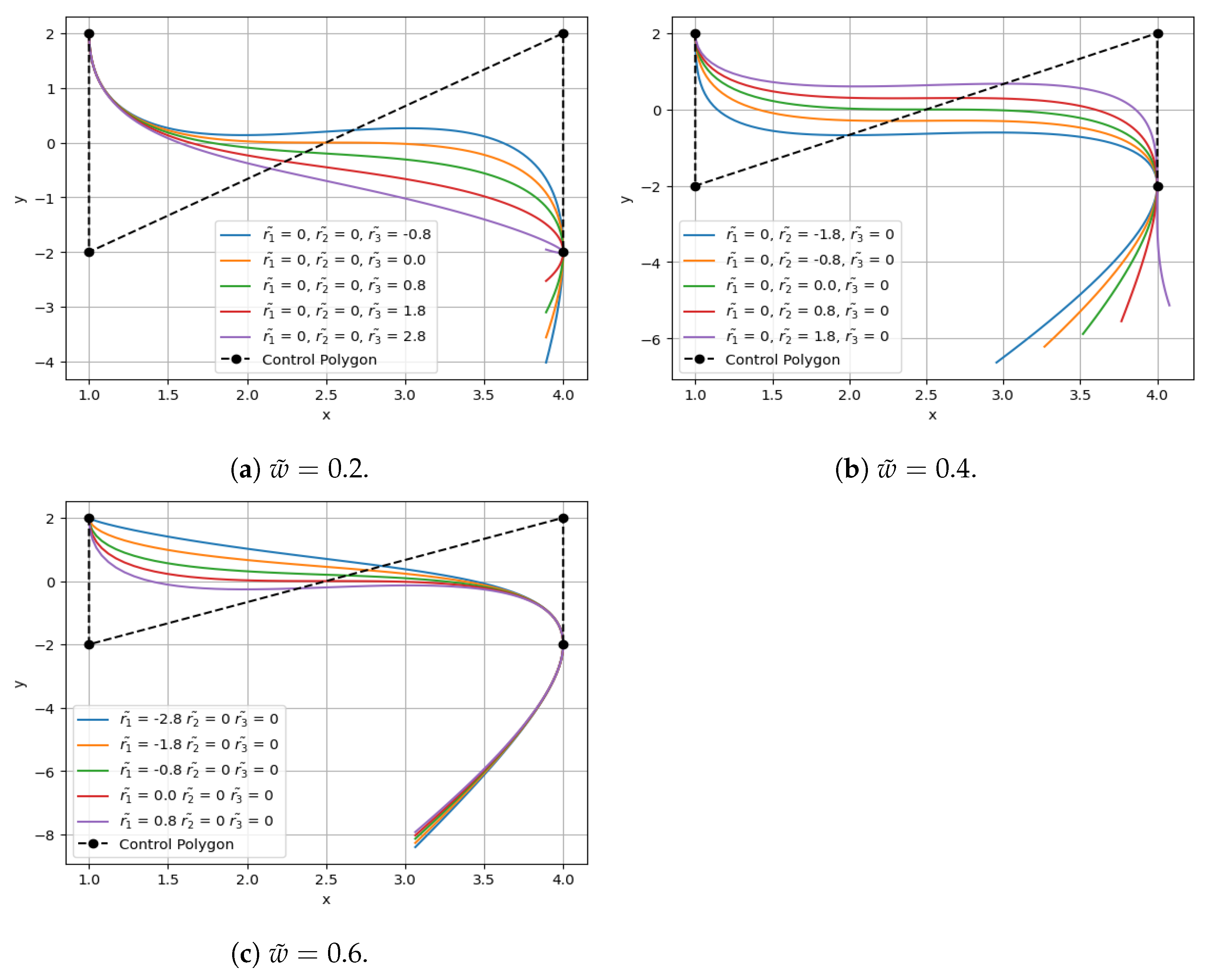
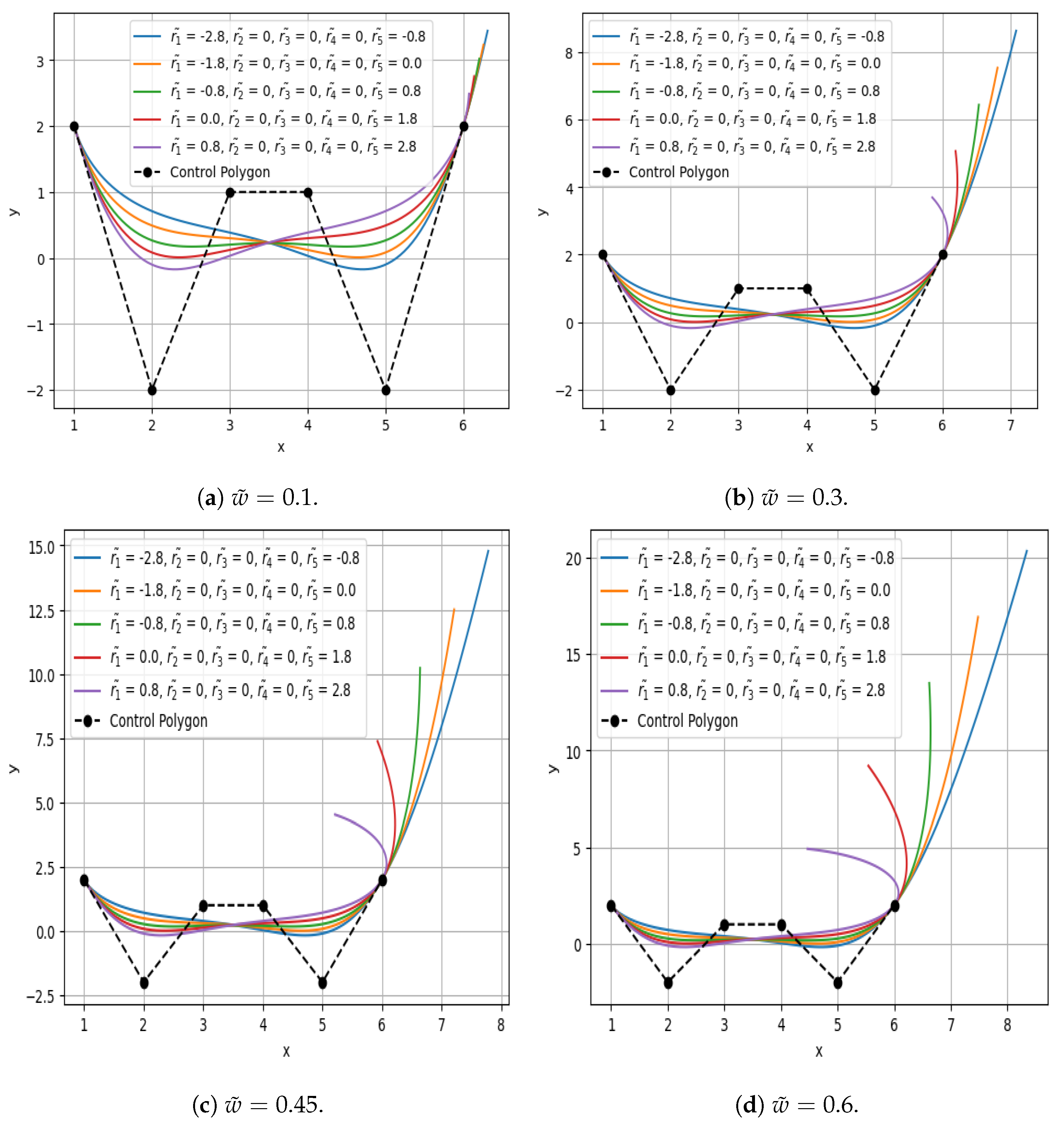
4. The Curve’s Geometric Effect on Fractional and Shape Parameters
5. Construction of Surfaces Using Caputo Fabrizio Bézier Curves
5.1. Surface Revolution
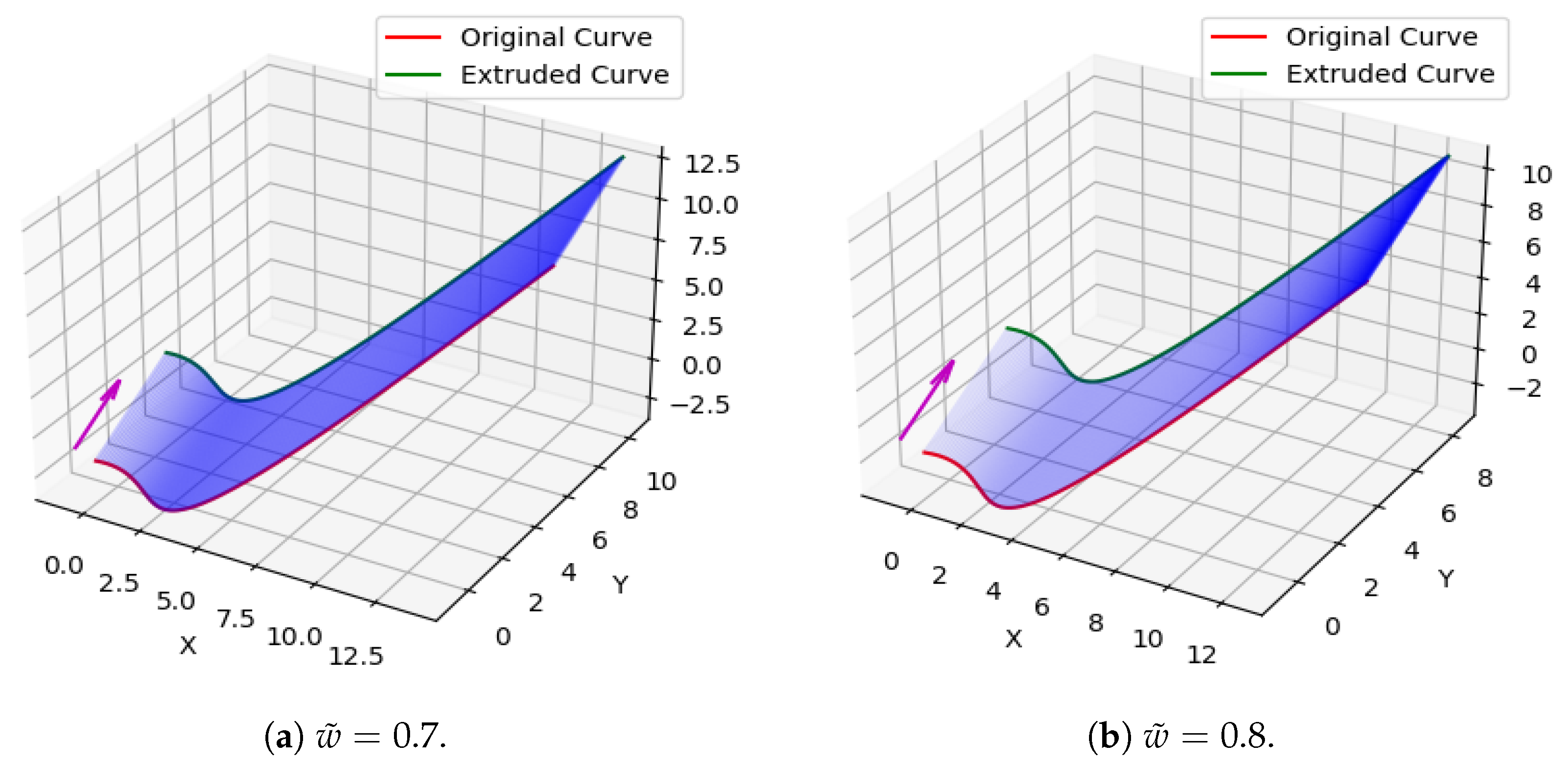
5.2. Extruded Surface
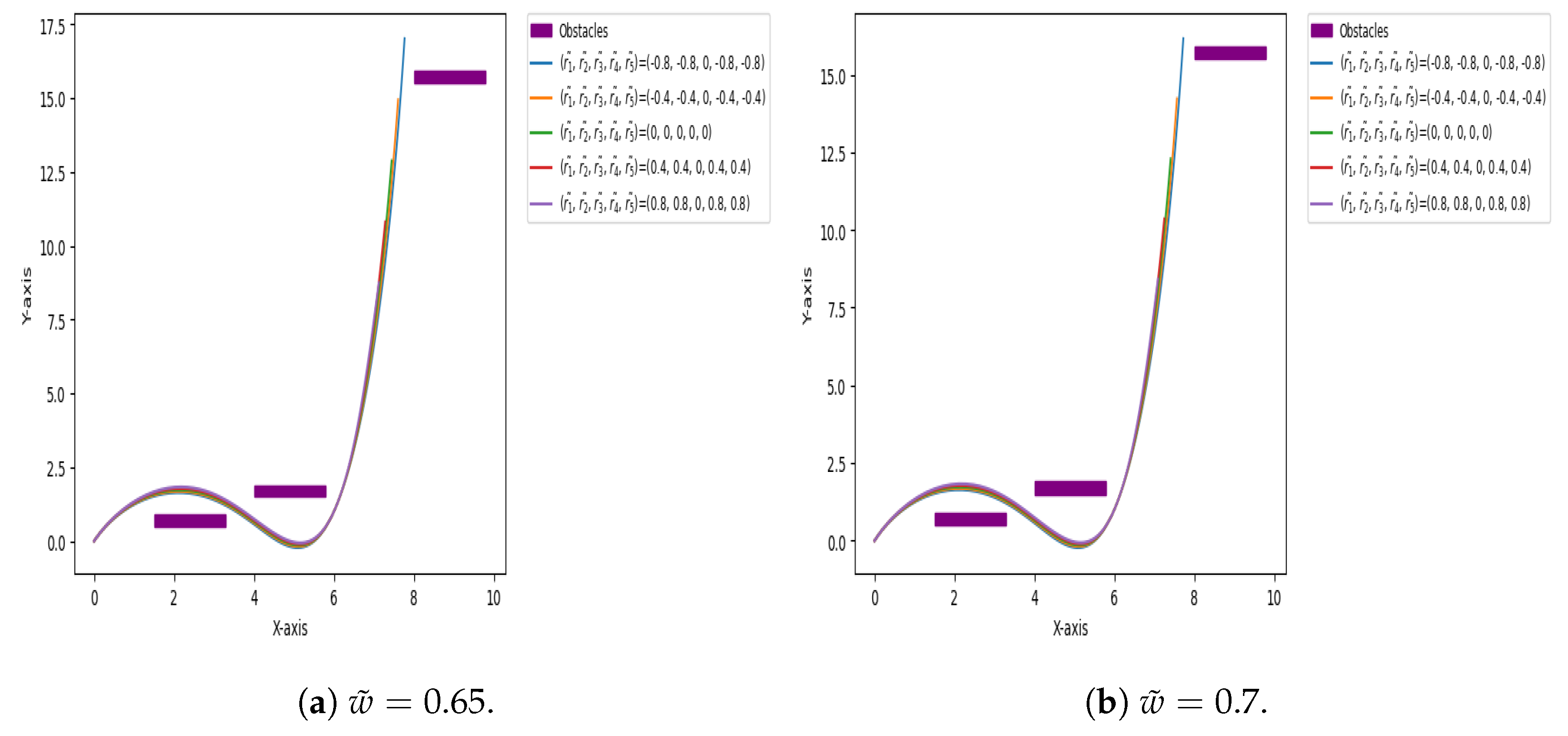
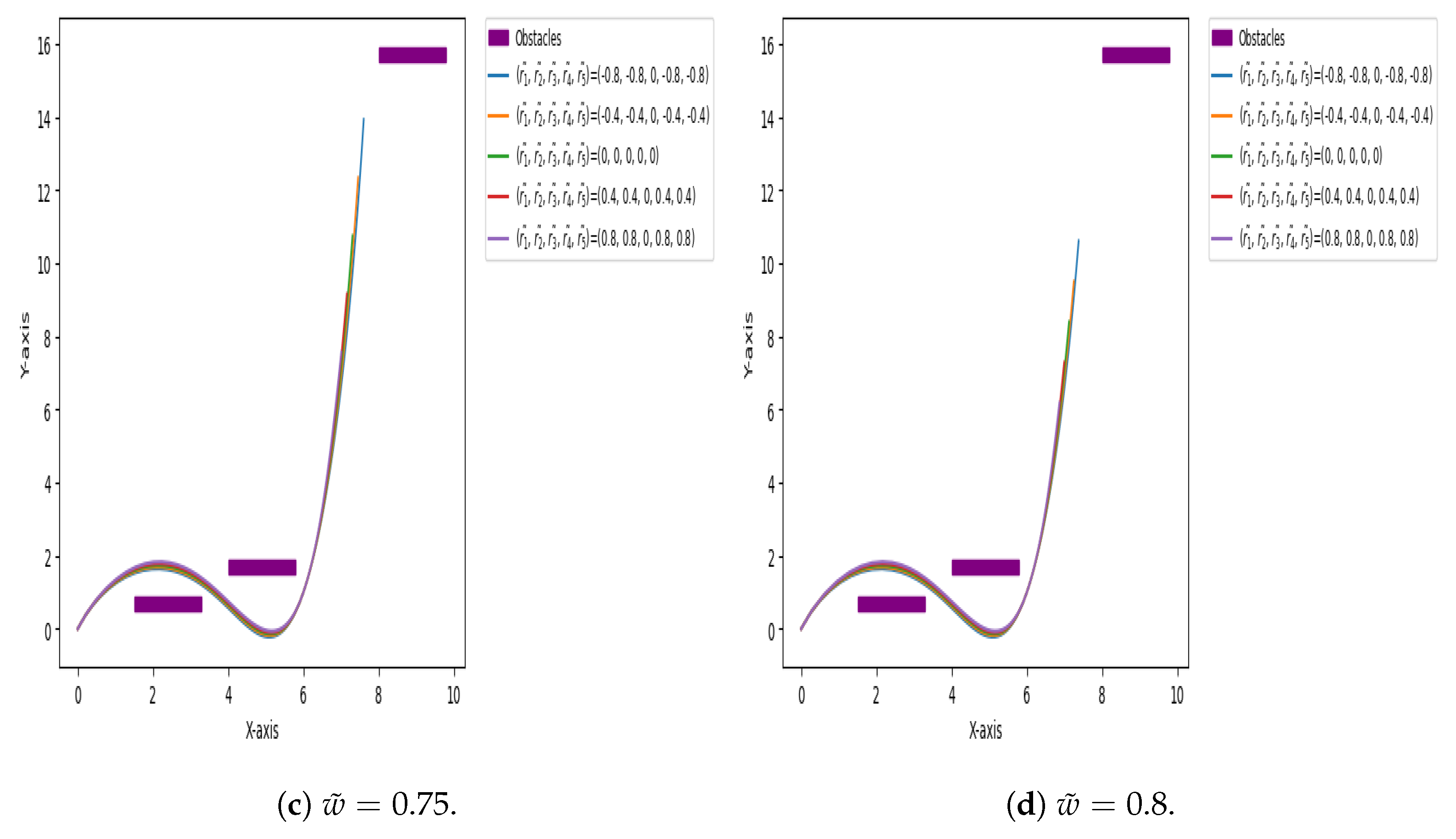
6. Application of Caputo Fabrizio Bézier Curve
- Caputo Fabrizio Bézier curves can be used in molecular dynamics to simulate possible trajectories for a molecule as it travels across a potential energy landscape. In this case, regions of high energy that the molecule must avoid could constitute obstacles.
- Proteins’ smooth folding paths can be modeled using Caputo Fabrizio Bézier curves, which help prevent high-energy misfolded states.
- Caputo Fabrizio Bézier curves can be used in chemical reactions to discover the most efficient path from reactants to products and to navigate potential energy surfaces by avoiding high-energy obstacles.
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Prautzsch, H.; Boehm, W.; Paluszny, M. Bézier and B-Spline Techniques; Springer: Berlin/Heidelberg, Germany, 2002; Volume 6. [Google Scholar]
- Farin, G.E.; Farin, G. Curves and Surfaces for CAGD: A Practical Guide; Morgan Kaufmann: Burlington, MA, USA, 2002. [Google Scholar]
- Wen-Tao, W.; Guo-Zhao, W. Bézier curves with shape parameter. J. Zhejiang-Univ.-Sci. A 2005, 6, 497–501. [Google Scholar] [CrossRef]
- Han, X. Quadratic trigonometric polynomial curves with a shape parameter. Comput. Aided Geom. Des. 2002, 19, 503–512. [Google Scholar] [CrossRef]
- Han, X. Cubic trigonometric polynomial curves with a shape parameter. Comput. Aided Geom. Des. 2004, 21, 535–548. [Google Scholar] [CrossRef]
- Yang, L.; Zeng, X.M. Bézier curves and surfaces with shape parameters. Int. J. Comput. Math. 2009, 86, 1253–1263. [Google Scholar] [CrossRef]
- Hu, G.; Wu, J.; Qin, X. A novel extension of the Bézier model and its applications to surface modeling. Adv. Eng. Softw. 2018, 125, 27–54. [Google Scholar] [CrossRef]
- Maqsood, S.; Abbas, M.; Hu, G.; Ramli, A.L.A.; Miura, K.T. A novel generalization of trigonometric Bézier curve and surface with shape parameters and its applications. Math. Probl. Eng. 2020, 2020, 4036434. [Google Scholar] [CrossRef]
- Ramanantoanina, A.; Hormann, K. New shape control tools for rational Bézier curve design. Comput. Aided Geom. Des. 2021, 88, 102003. [Google Scholar] [CrossRef]
- Bulut, V. Path planning of mobile robots in dynamic environment based on analytic geometry and cubic Bézier curve with three shape parameters. Expert Syst. Appl. 2023, 233, 120942. [Google Scholar] [CrossRef]
- Ammad, M.; Misro, M.Y.; Ramli, A. A novel generalized trigonometric Bézier curve: Properties, continuity conditions and applications to the curve modeling. Math. Comput. Simul. 2022, 194, 744–763. [Google Scholar] [CrossRef]
- Ammad, M.; Misro, M.Y. Construction of local shape adjustable surfaces using quintic trigonometric Bézier curve. Symmetry 2020, 12, 1205. [Google Scholar] [CrossRef]
- Omar, A.; Ahmed, I.; Abuzaiyan, F. The Quadratic Trigonometric Bézier like Curve with two Shape Parameters. J. Pure Appl. Sci. 2023, 22, 215–221. [Google Scholar]
- Ziatdinov, R.; Yoshida, N.; Kim, T.W. Fitting G2 multispiral transition curve joining two straight lines. Comput.-Aided Des. 2012, 44, 591–596. [Google Scholar] [CrossRef]
- Qin, X.; Shen, X.; Yang, Y. A novel extension to the polynomial basis functions describing Bézier curves and surfaces of degree n with multiple shape parameters. Appl. Math. Comput. 2013, 223, 1–16. [Google Scholar] [CrossRef]
- Bashir, U.; Abbas, M.; Ali, J.M. The G2 and C2 rational quadratic trigonometric Bézier curve with two shape parameters with applications. Appl. Math. Comput. 2013, 219, 10183–10197. [Google Scholar] [CrossRef]
- Bibi, S.; Abbas, M.; Miura, K.T.; Misro, M.Y. Geometric modeling of novel generalized hybrid trigonometric Bézier-like curve with shape parameters and its applications. Mathematics 2020, 8, 967. [Google Scholar] [CrossRef]
- Debnath, L. Recent applications of fractional calculus to science and engineering. Int. J. Math. Math. Sci. 2003, 2003, 3413–3442. [Google Scholar] [CrossRef]
- Sebaa, N.; Fellah, Z.E.A.; Lauriks, W.; Depollier, C. Application of fractional calculus to ultrasonic wave propagation in human cancellous bone. Signal Process. 2006, 86, 2668–2677. [Google Scholar] [CrossRef]
- Sun, H.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–231. [Google Scholar] [CrossRef]
- Baleanu, D.; Jajarmi, A.; Mohammadi, H.; Rezapour, S. A new study on the mathematical modelling of human liver with Caputo–Fabrizio fractional derivative. Chaos Solitons 2020, 134, 109705. [Google Scholar] [CrossRef]
- Arora, S.; Mathur, T.; Agarwal, S.; Tiwari, K.; Gupta, P. Applications of fractional calculus in computer vision: A survey. Neurocomputing 2022, 489, 407–428. [Google Scholar] [CrossRef]
- De Oliveira, E.C.; Tenreiro Machado, J.A. A review of definitions for fractional derivatives and integral. Math. Probl. Eng. 2014, 2014, 1–7. [Google Scholar] [CrossRef]
- Li, X. Numerical solution of fractional differential equations using cubic B-spline wavelet collocation method. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 3934–3946. [Google Scholar] [CrossRef]
- Kirmani, S.; Bilal Riaz, M. Shape preserving fractional order KNR C1 cubic spline. Eur. Phys. J. Plus 2019, 134, 319. [Google Scholar] [CrossRef]
- Said Mad Zain, S.A.A.A.; Misro, M.Y.; Miura, K.T. Generalized fractional Bézier curve with shape parameters. Mathematics 2021, 9, 2141. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 2015, 1, 73–85. [Google Scholar]
- Tan, J. The Mechanical Meaning of Bernstein Basis Function. Int. J. Appl. Math. Control. Eng. 2018, 1, 47–54. [Google Scholar]















Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Awais, M.; Kirmani, S.K.N.; Rana, M.; Ahmad, R. Caputo Fabrizio Bézier Curve with Fractional and Shape Parameters. Computers 2024, 13, 206. https://doi.org/10.3390/computers13080206
Awais M, Kirmani SKN, Rana M, Ahmad R. Caputo Fabrizio Bézier Curve with Fractional and Shape Parameters. Computers. 2024; 13(8):206. https://doi.org/10.3390/computers13080206
Chicago/Turabian StyleAwais, Muhammad, Syed Khawar Nadeem Kirmani, Maheen Rana, and Raheel Ahmad. 2024. "Caputo Fabrizio Bézier Curve with Fractional and Shape Parameters" Computers 13, no. 8: 206. https://doi.org/10.3390/computers13080206
APA StyleAwais, M., Kirmani, S. K. N., Rana, M., & Ahmad, R. (2024). Caputo Fabrizio Bézier Curve with Fractional and Shape Parameters. Computers, 13(8), 206. https://doi.org/10.3390/computers13080206






