Tunable Filters Using Defected Ground Structures at Millimeter-Wave Frequencies
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Method
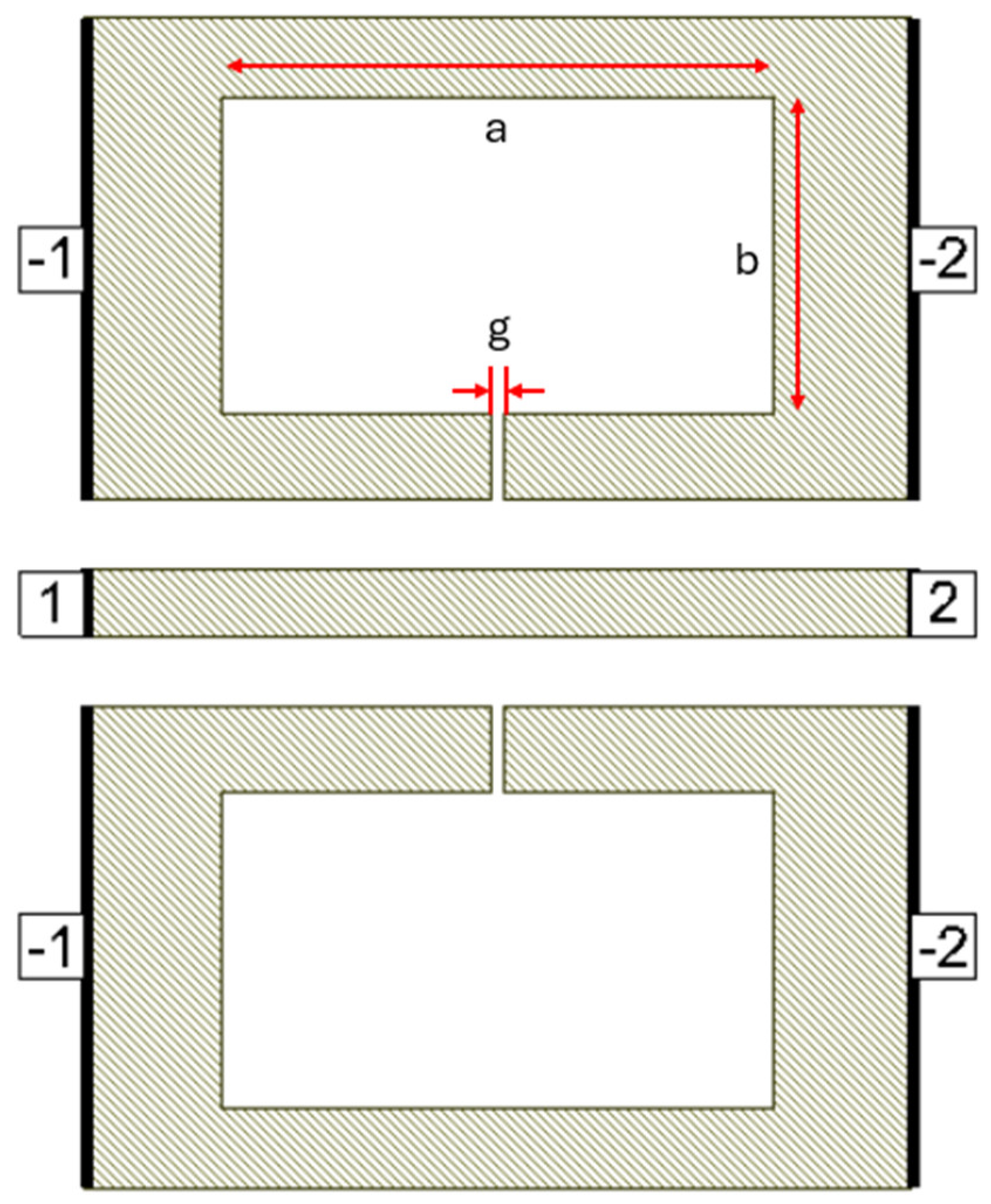
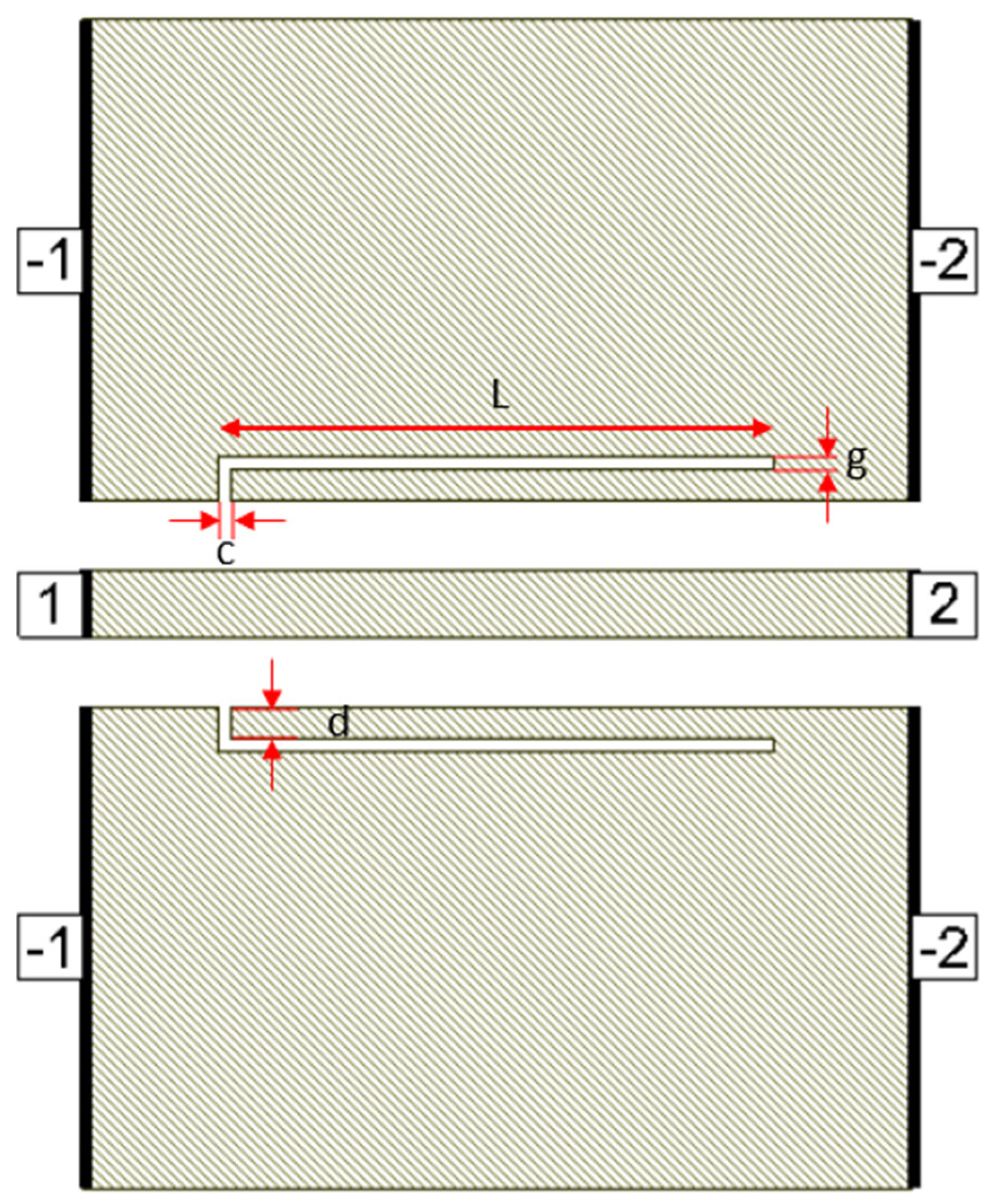
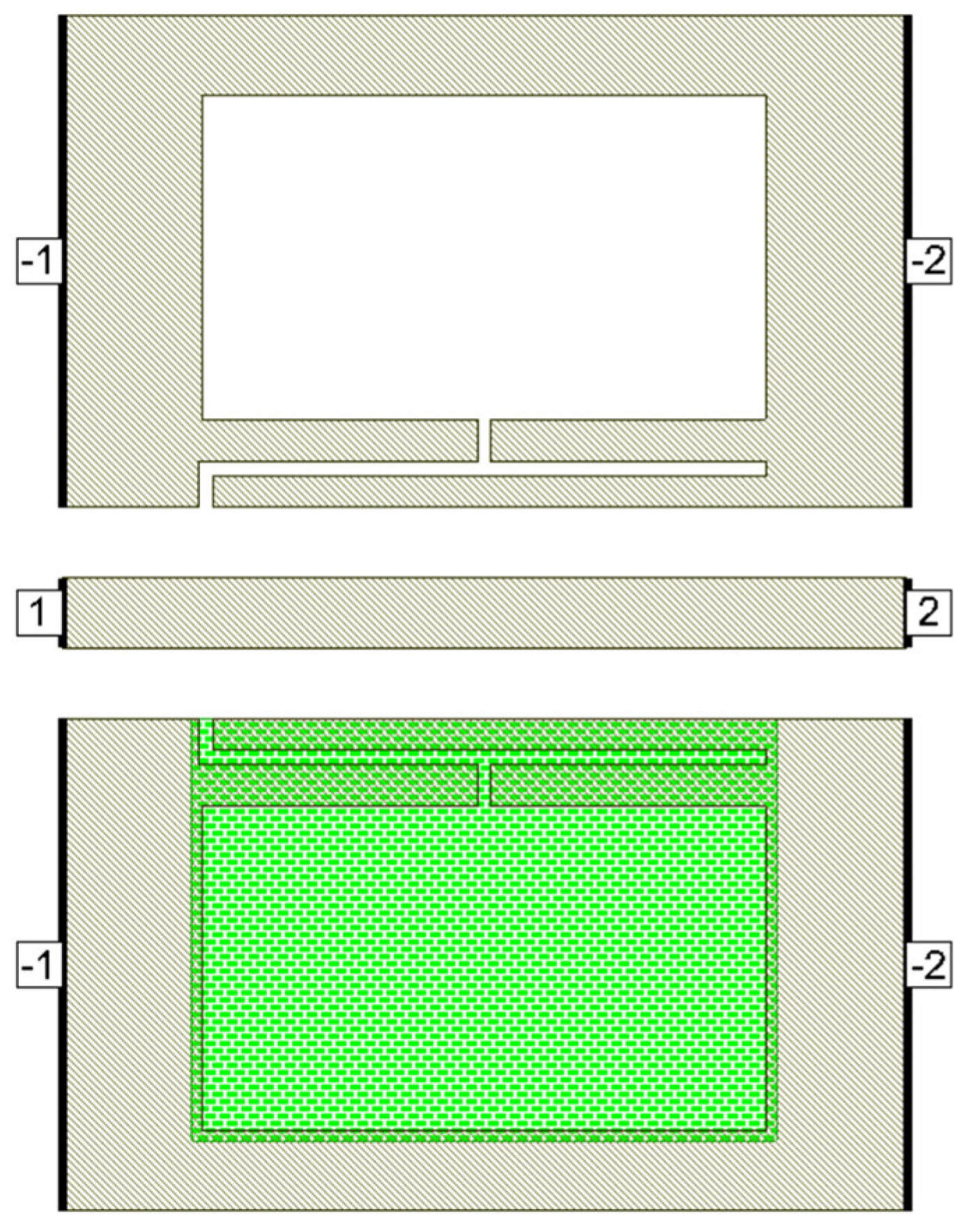
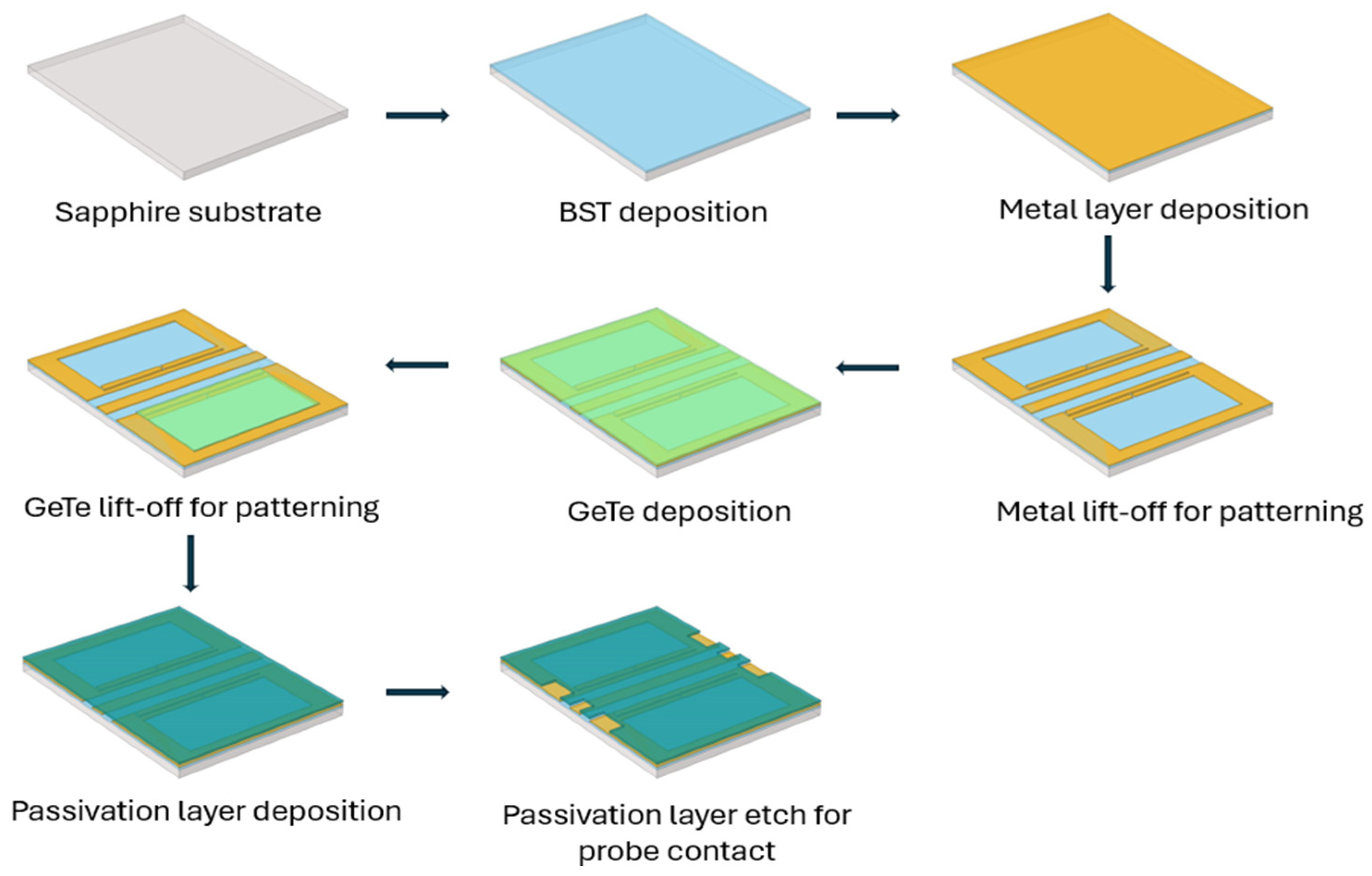
Design and Fabrication of DGS Devices
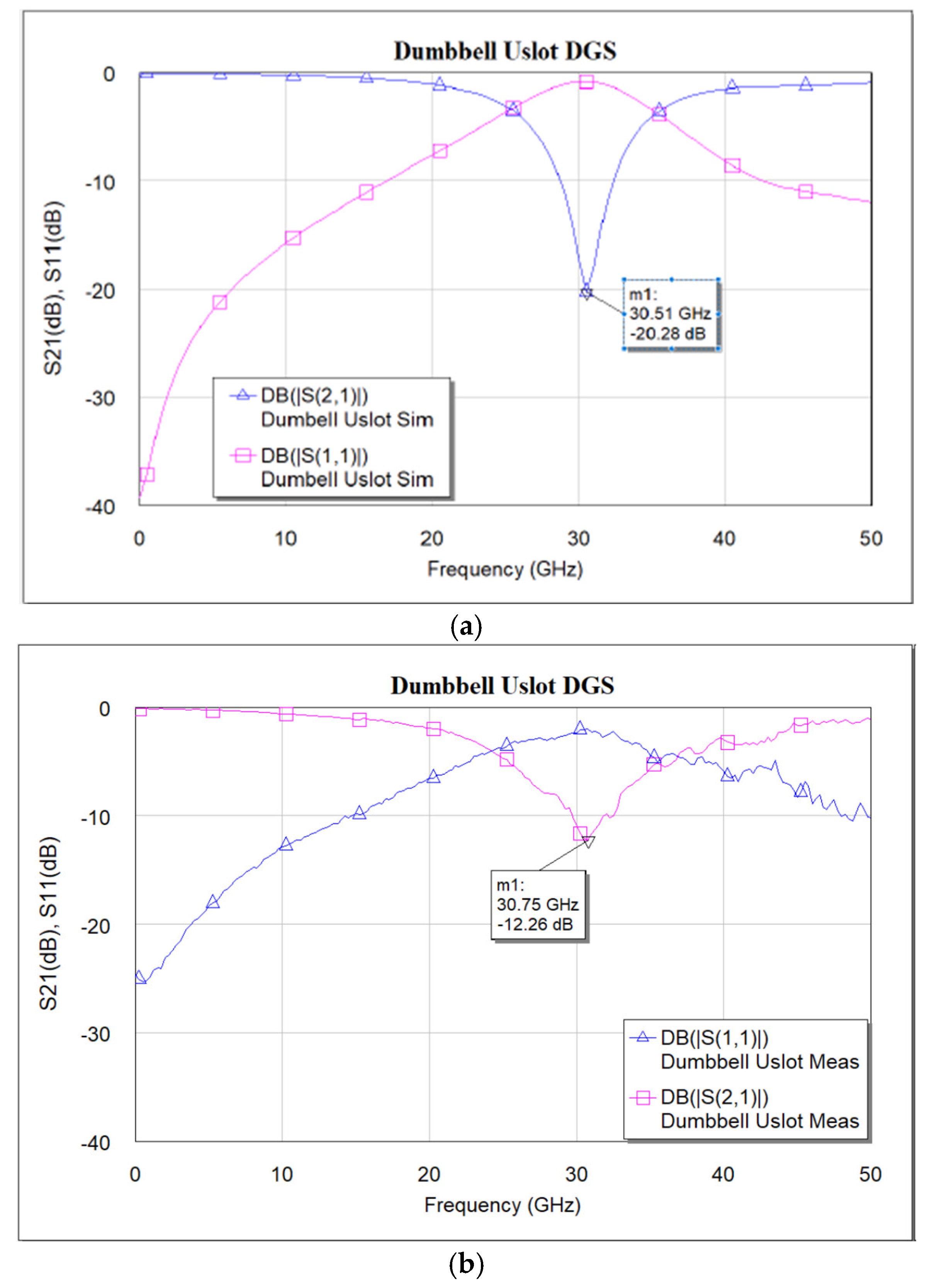
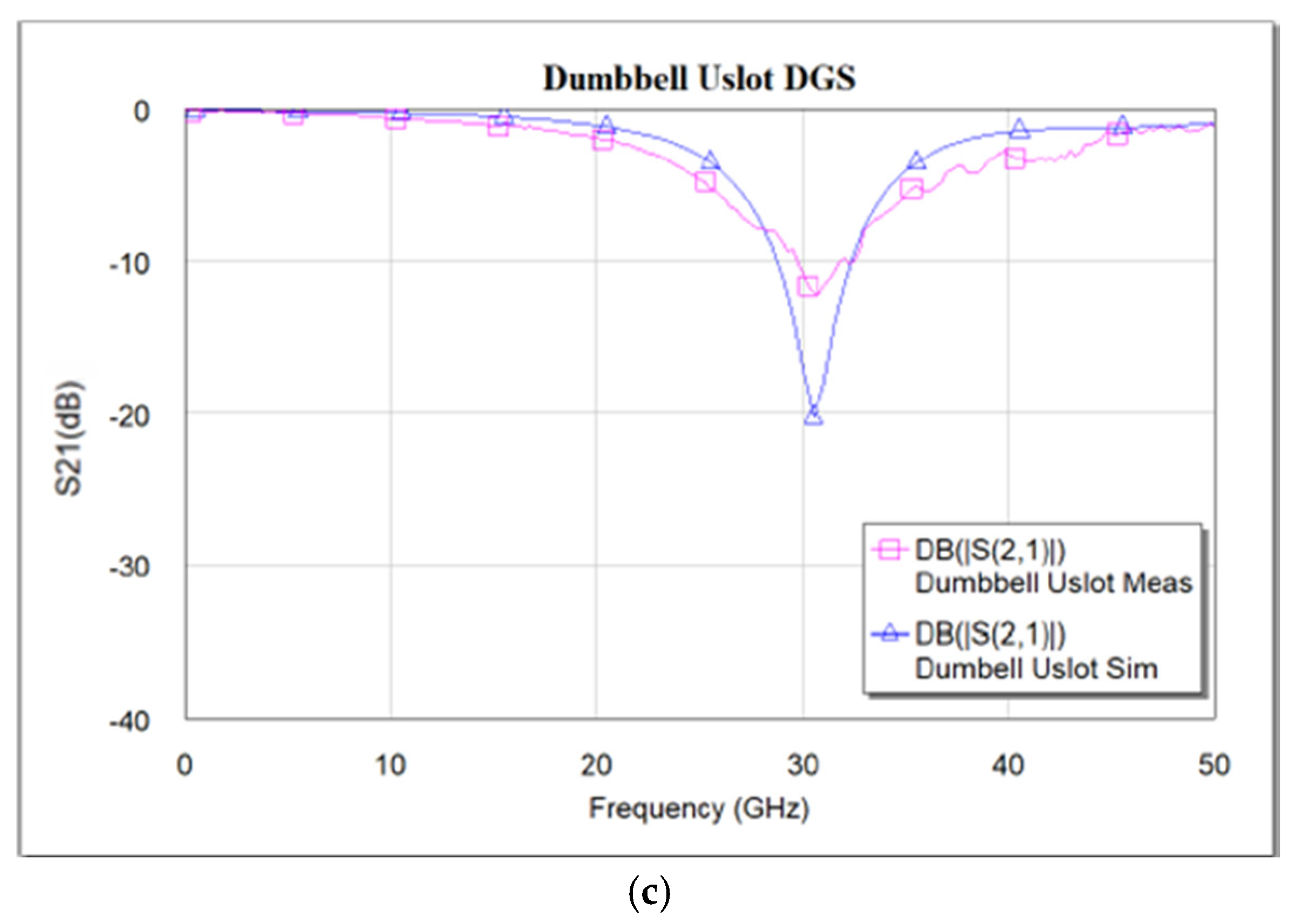
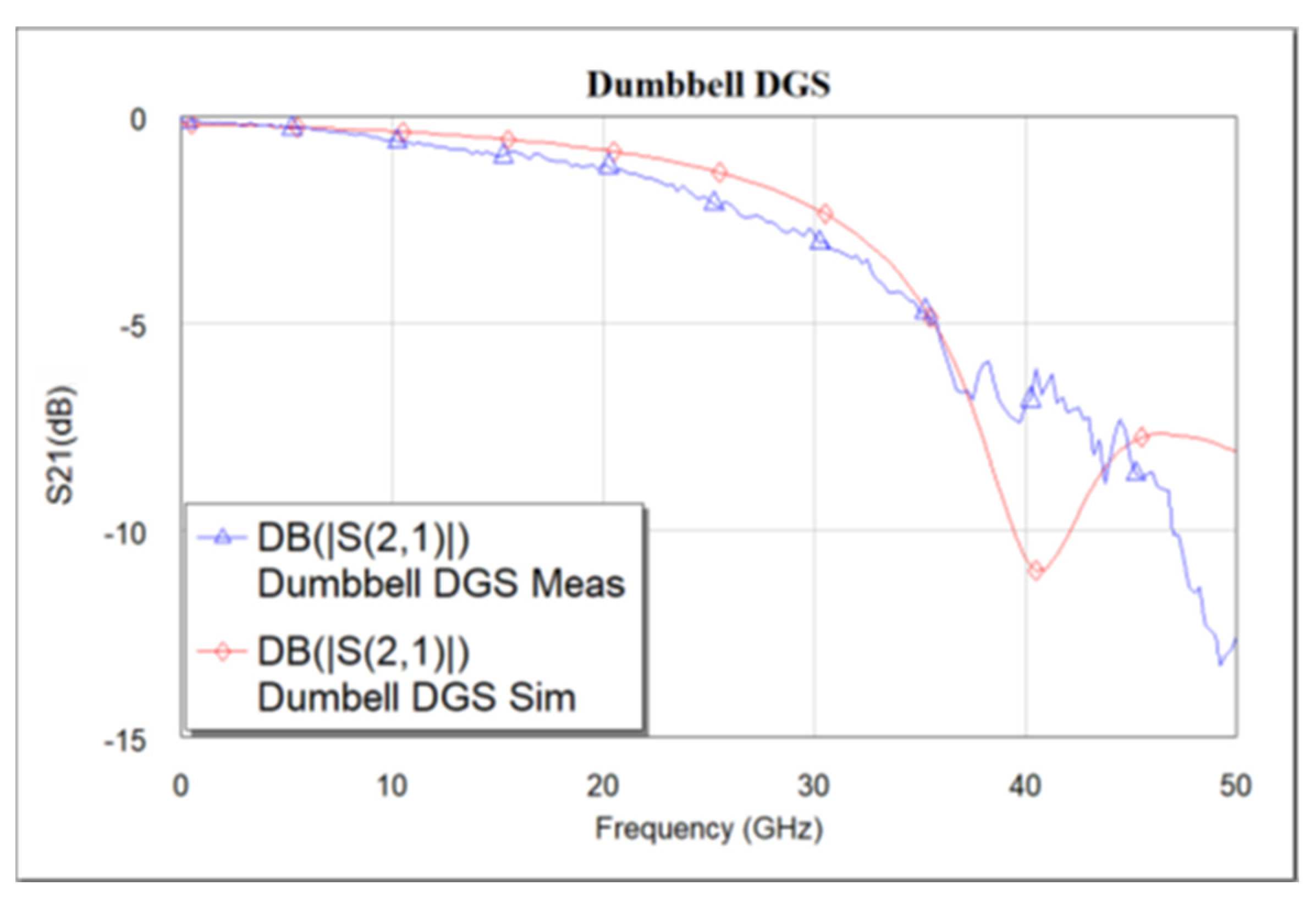
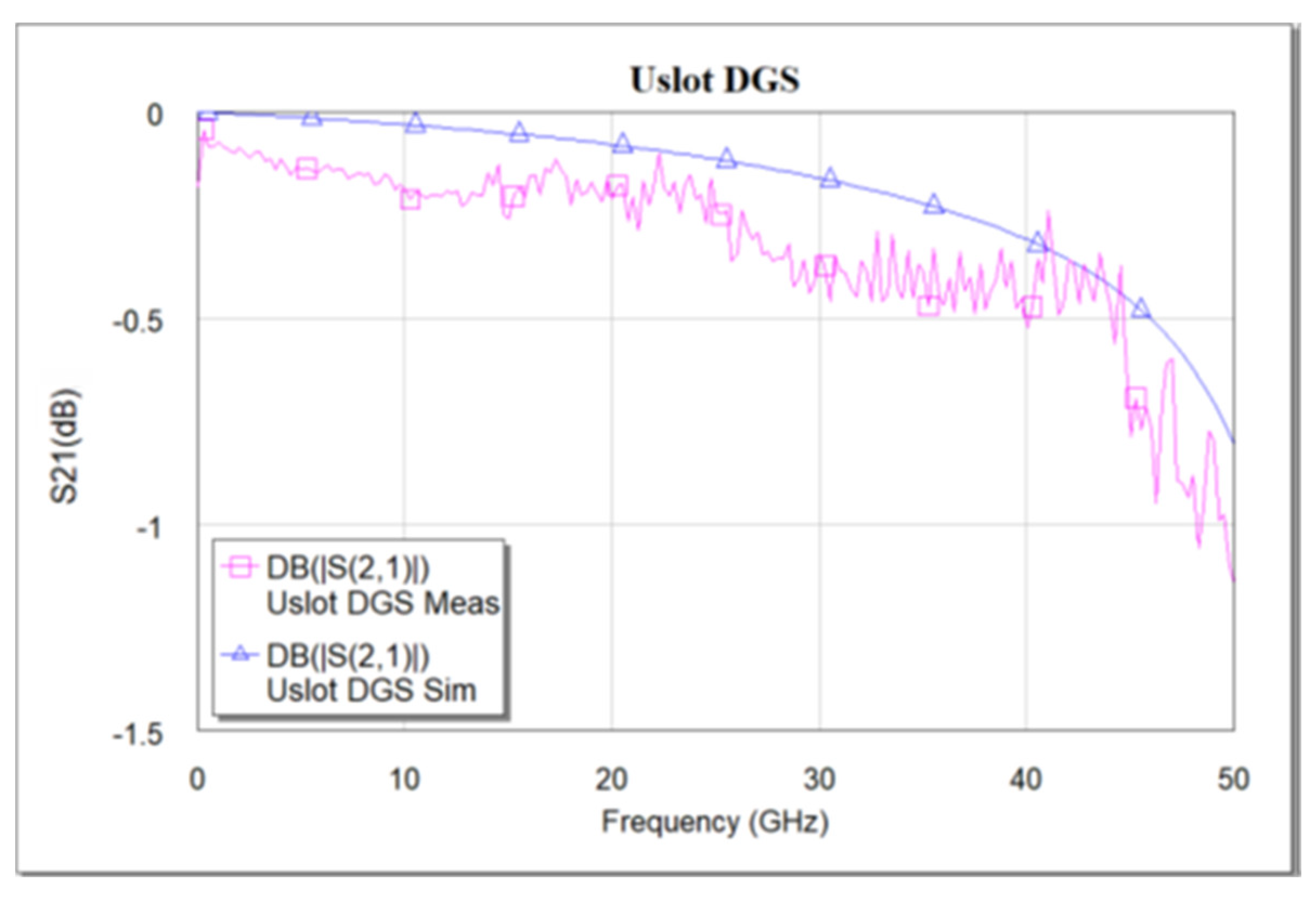
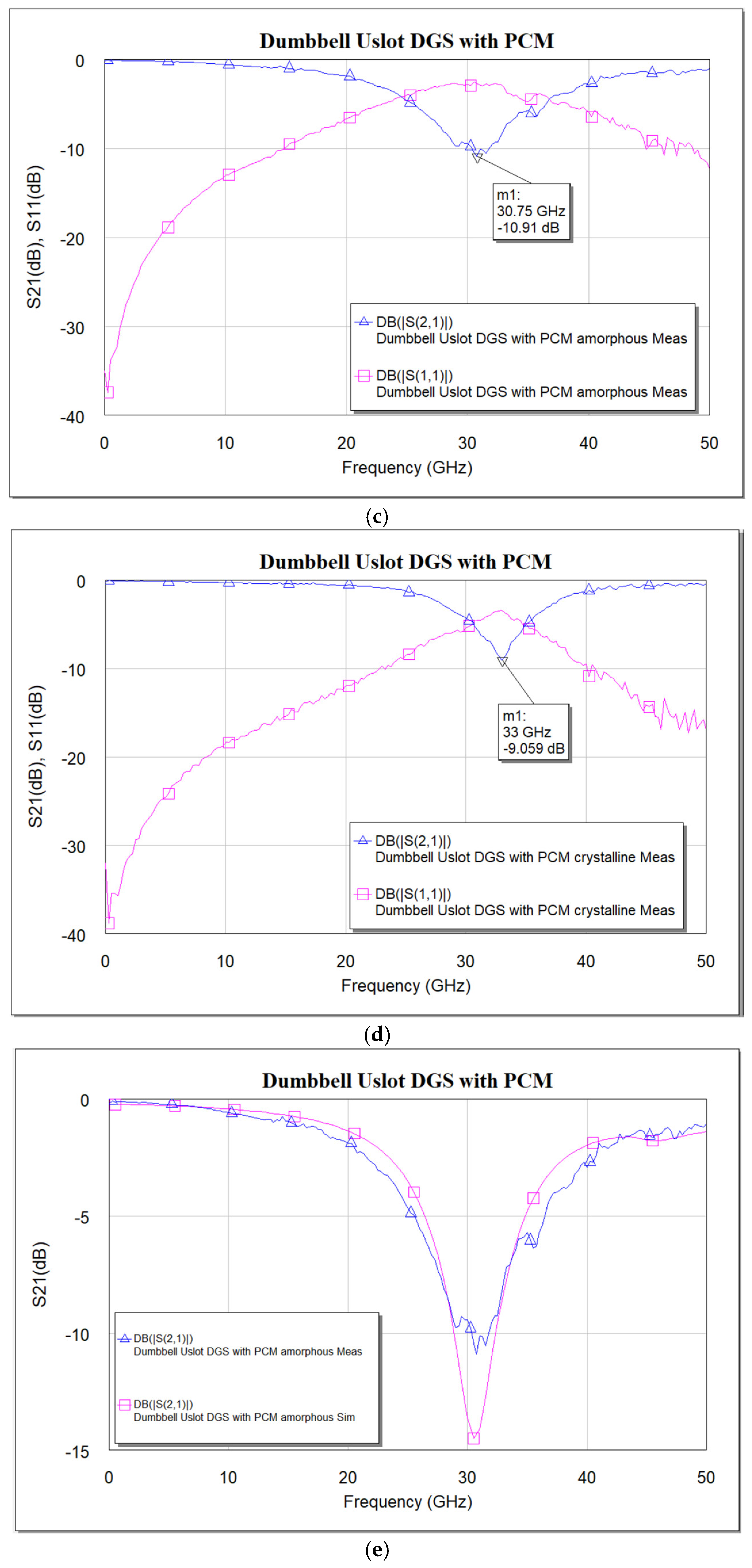
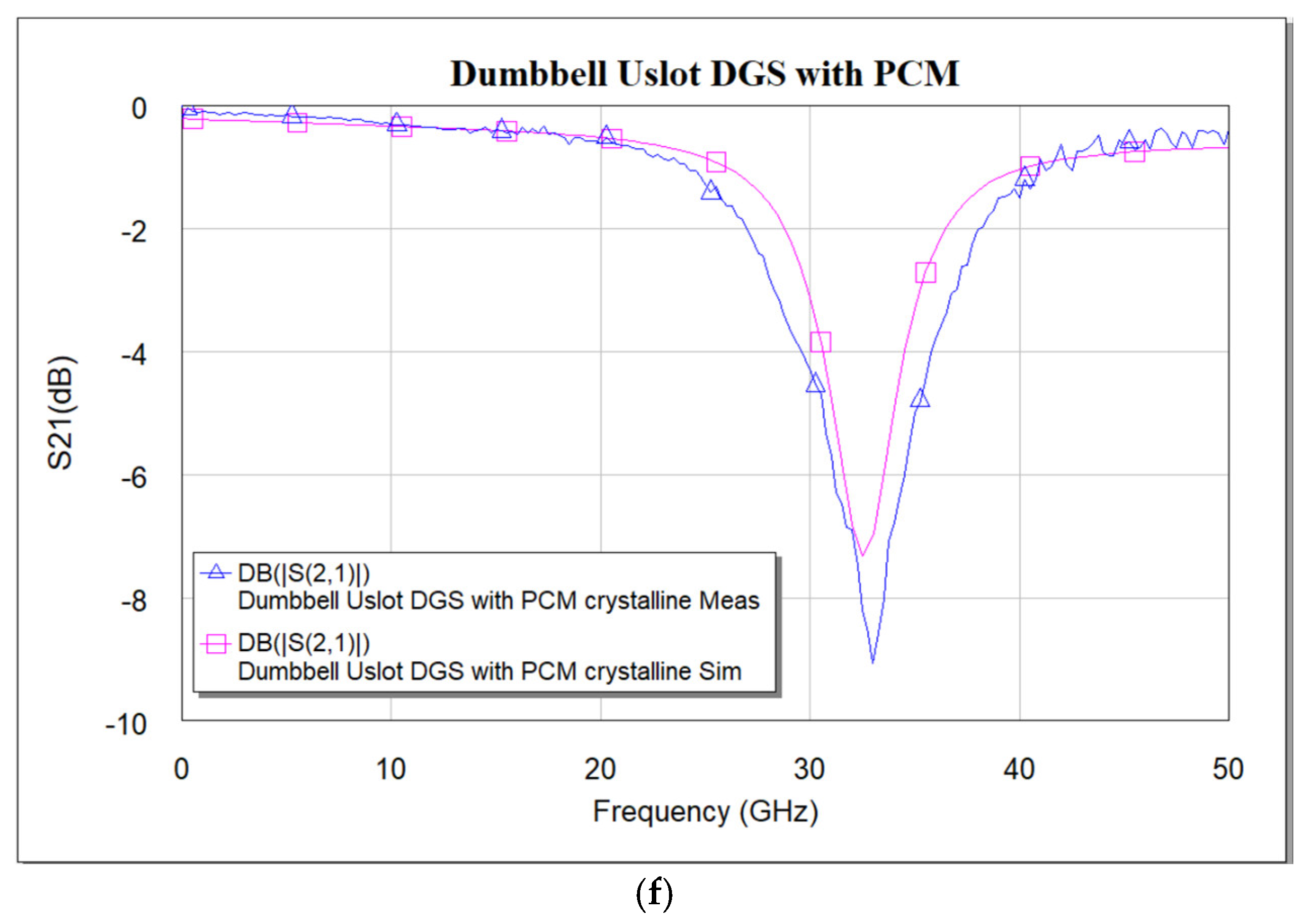
3. Results
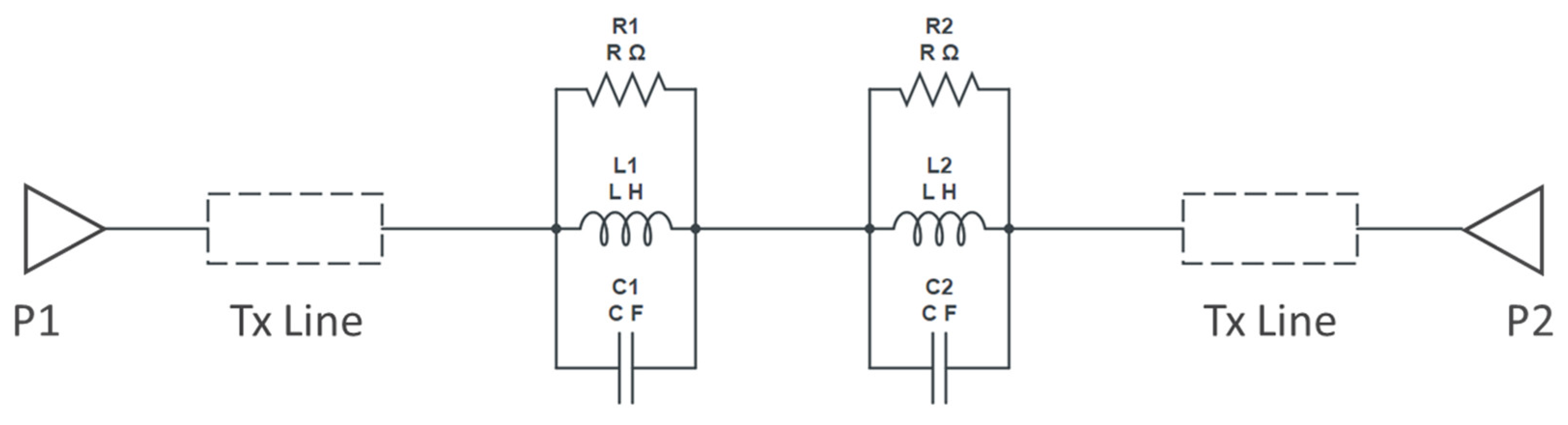
Equivalent Circuit Model
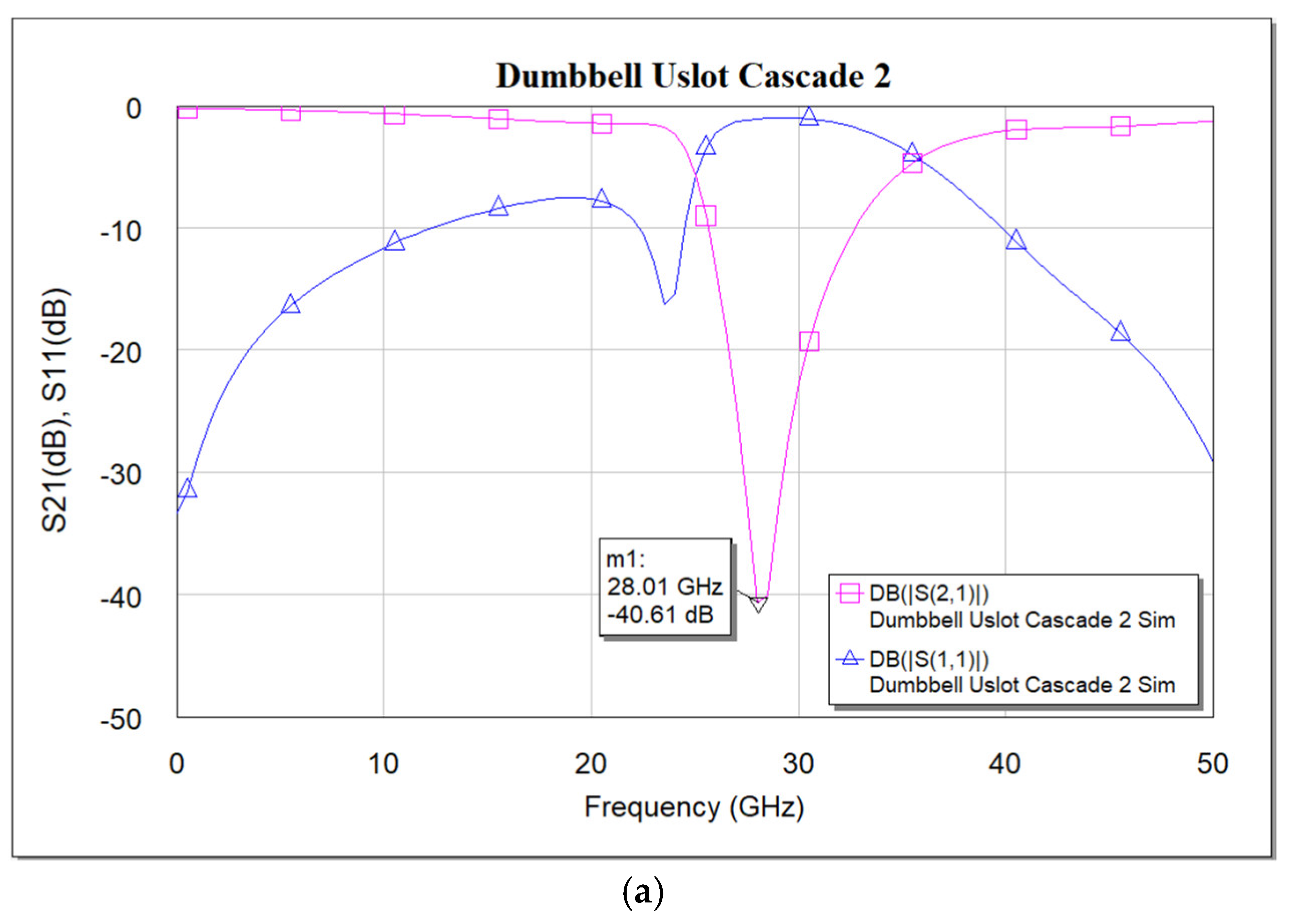
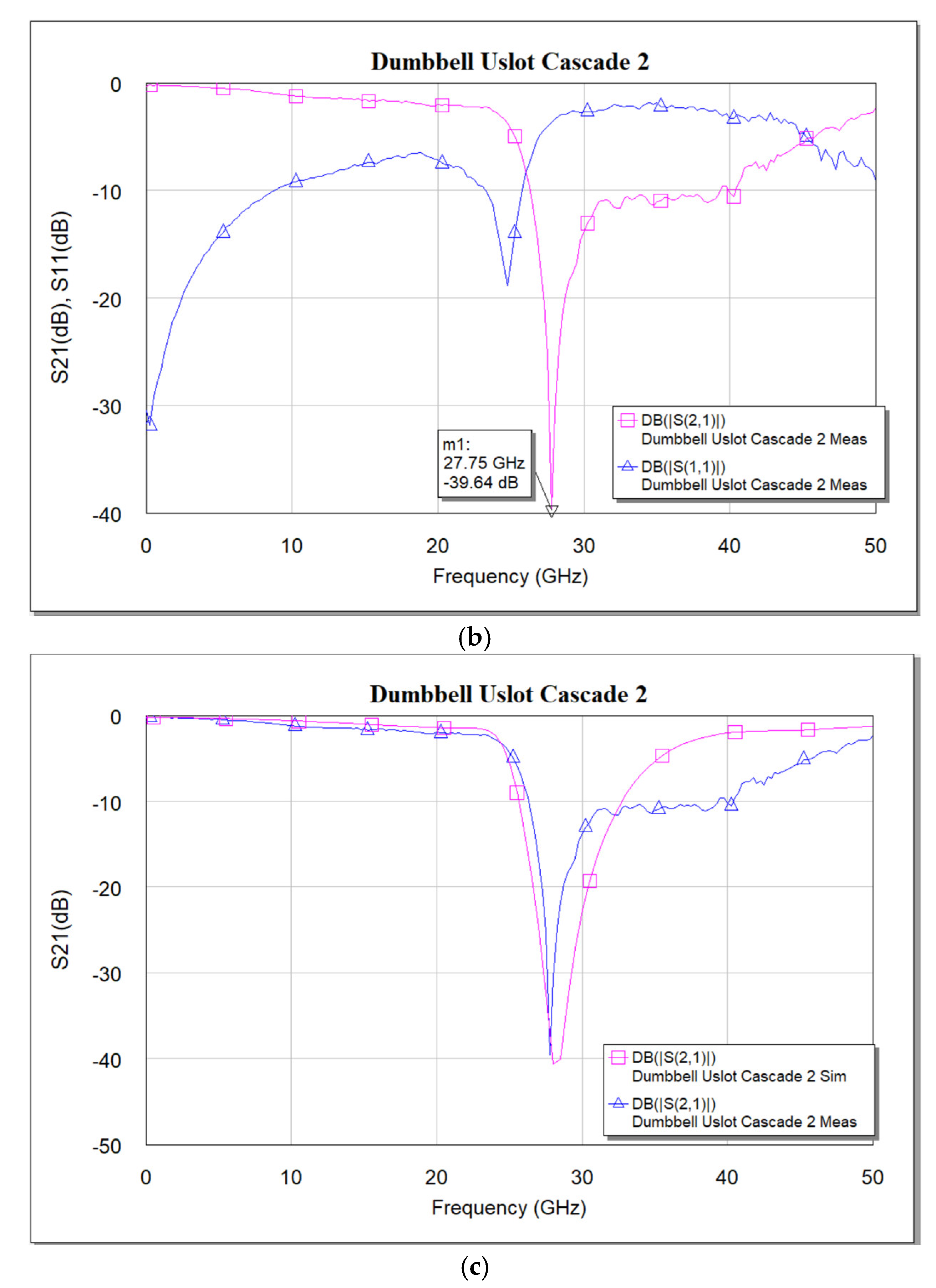
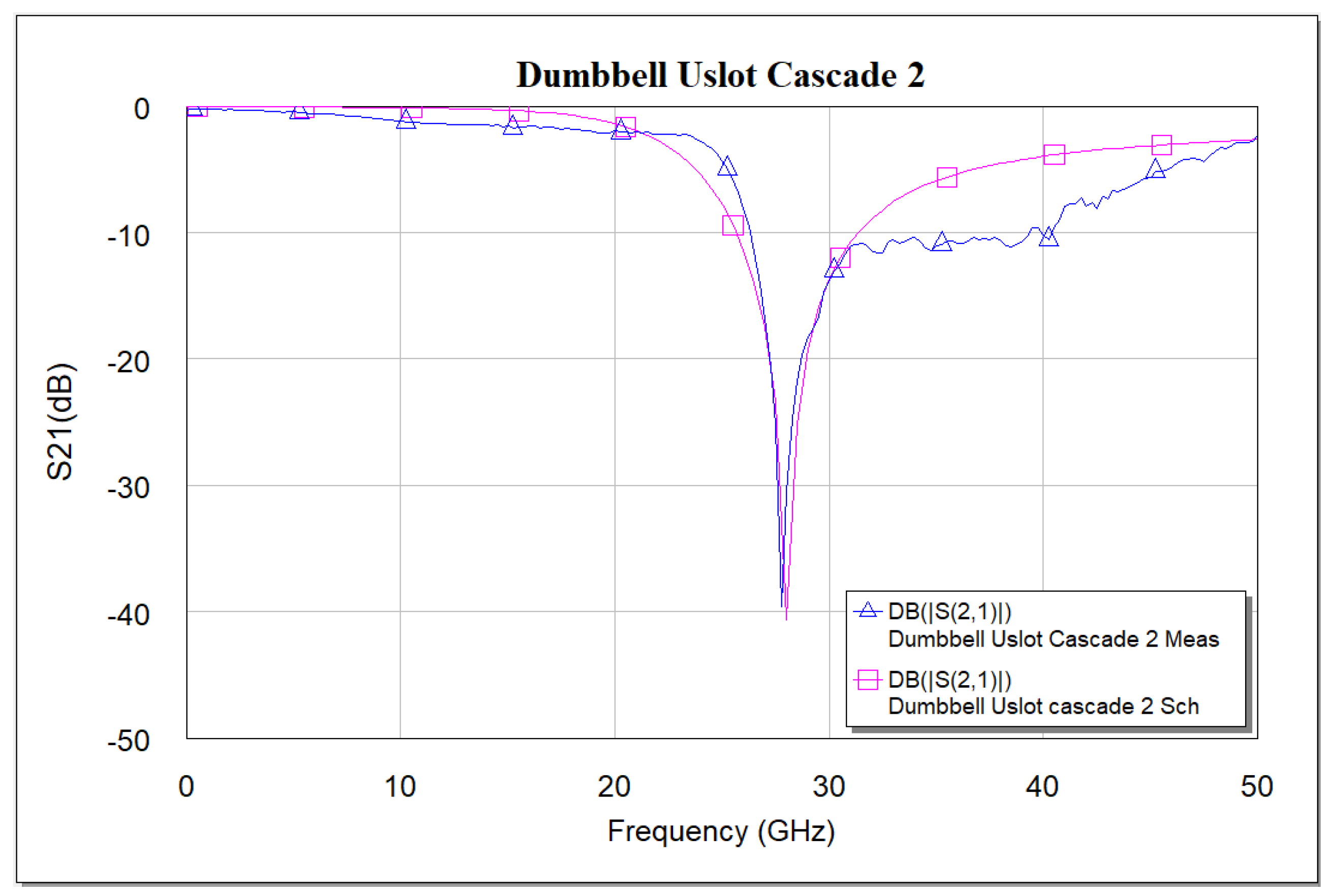
4. Cascading of Dumbbell U-Slot DGS
5. Tunable DGS Filter
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vanukuru, V.N.R.; Velidi, V.K. Millimeter-Wave CMOS 30/80 GHz Sharp-Rejection Dual-Band Bandstop Filters Using TFMS Open-Stepped-Impedance Resonators. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 201–205. [Google Scholar]
- Kim, B.; Lee, J.; Lee, J.; Jung, B.; Chappell, W.J. RF CMOS Integrated On-Chip Tunable Absorptive Bandstop Filter Using Q-Tunable Resonators. IEEE Trans. Electron. Devices 2013, 60, 1730–1737. [Google Scholar] [CrossRef]
- Thapa, S.K.; Chen, B.; Barakat, A.; Pokharel, R.K. A Compact Millimeter-Wave On-chip DGS-based Bandstop Filter with Two Transmission Poles in CMOS Technology. In Proceedings of the 52nd European Microwave Conference (EuMC), Milan, Italy, 27–29 September 2022; pp. 564–567. [Google Scholar]
- Ge, Z.; Chen, L.; Yang, L.; Gómez-García, R.; Zhu, X. On-Chip Millimeter-Wave Integrated Absorptive Bandstop Filter in (Bi)-CMOS Technology. IEEE Electron Device Lett. 2021, 42, 114–117. [Google Scholar] [CrossRef]
- Ge, Z.; Chen, L.; Gómez-García, R.; Zhu, X. Millimeter-Wave Wide-Band Bandpass Filter in CMOS Technology Using a Two-Layered Highpass-Type Approach with Embedded Upper Stopband. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 1586–1590. [Google Scholar] [CrossRef]
- Nan, L.; Mouthaan, K.; Xiong, Y.; Shi, J.; Rustagi, S.C.; Ooi, B. Design Of 60- And 77-GHz Narrow-Bandpass Filters in CMOS Technology. IEEE Trans. Circuits Syst. II Exp. Briefs 2008, 55, 738–742. [Google Scholar] [CrossRef]
- Vetury, R.; Kochhar, A.; Leathersich, J.; Moe, C.; Winters, M.; Shealy, J.; Olsson, R.H. A Manufacturable AlScN Periodically Polarized Piezoelectric Film Bulk Acoustic Wave Resonator (AlScN P3F BAW) Operating in Overtone Mode at X and Ku Band. In Proceedings of the IEEE/MTT-S International Microwave Symposium—IMS, San Diego, CA, USA, 11–16 June 2023; pp. 891–894. [Google Scholar]
- Izhar; Fiagbenu, M.M.A.; Du, X.; Musavigharavi, P.; Deng, Y.; Gunda, A.; Leathersich, J.; Moe, C.; Kochhar, A.; Stach, E.A.; et al. A High Quality Factor, 19-GHz Periodically Poled AlScN BAW Resonator Fabricated in a Commercial XBAW Process. IEEE Trans. Electron. Devices 2024, 71, 5630–5637. [Google Scholar] [CrossRef]
- Hara, M.; Yokoyama, T.; Sakashita, T.; Taniguchi, S.; Iwaki, M.; Nishihara, T.; Ueda, M.; Satoh, Y. Super-High-Frequency Band Filters Configured with Air-Gap-Type Thin-Film Bulk Acoustic Resonators. Jpn. J. Appl. Phys. 2010, 49, 2010. [Google Scholar] [CrossRef]
- Ali, M.; Liu, F.; Watanabe, A.; Raj, P.M.; Sundaram, V.; Tentzeris, M.M.; Tummala, R.R. Miniaturized High-Performance Filters for 5G Small-Cell Applications. In Proceedings of the IEEE 68th Electronic Components and Technology Conference (ECTC), San Diego, CA, USA, 29 May–1 June 2018; pp. 1068–1075. [Google Scholar]
- Takacs, A.; Neculoiu, D.; Vasilache, D.; Muller, A.; Pons, P.; Bary, L.; Calmon, P.; Aubert, H.; Plana, R. Tunable Bandstop and Bandpass MEMS Filters for Millimeter Wave Applications. In Proceedings of the 38th European Microwave Conference, Amsterdam, The Netherlands, 27–31 October 2008; pp. 591–594. [Google Scholar]
- Weng, L.H.; Guo, Y.C.; Shi, X.W.; Chen, X.Q. An Overview on Defected Ground Structure. Prog. Electromagn. Res. B 2008, 7, 173–189. [Google Scholar] [CrossRef]
- Ahn, D.; Park, J.; Kim, C.; Kim, J.; Qian, Y.; Itoh, T. A Design of the Low-Pass Filter using the Novel Microstrip Defected Ground Structure. IEEE Trans. Microw. Theory Tech. 2001, 49, 86–93. [Google Scholar] [CrossRef]
- Annam, K.; Khah, S.K.; Dooley, S.; Cerny, C.; Subramanyam, G. Experimental Design of Bandstop Filters Based on Unconventional Defected Ground Structures. Microw. Opt. Technol. Lett. 2016, 58, 2969–2973. [Google Scholar] [CrossRef]
- Annam, K. Design of Bandstop Filters Using Defected Ground Structures. Master’s Thesis, University of Dayton, Dayton, OH, USA, 2015. [Google Scholar]
- Woo, D.J.; Lee, T.K.; Lee, J.W.; Pyo, C.S.; Choi, W.K. Novel U-slot and V-slot DGS for Bandstop Filter with Improved Q factor. IEEE Trans. Microw. Theory Tech. 2006, 54, 2840–2847. [Google Scholar]
- Lim, J.S.; Kim, C.S.; Lee, Y.T.; Ahn, D.; Nam, S. A Spiral-Shaped Defected Ground Structure for Coplanar Waveguide. IEEE Microw. Wirel. Compon. Lett. 2002, 12, 330–332. [Google Scholar]
- Mandal, M.K.; Sanyal, S. A Novel Defected Ground Structure for Planar Circuits. IEEE Microw. Wirel. Compon. Lett. 2006, 16, 93–95. [Google Scholar] [CrossRef]
- Kiouach, F.; Ghzaoui, M.E.; Das, S.; Islam, T.; Ali, W. Design and Performance Analysis of a Novel Miniaturized Band-Stop Filter for Band Rejection in Sub-6 GHz 5G Spectrum. Phys. Scr. 2024, 99, 105019. [Google Scholar] [CrossRef]
- Varanasi, C.V.; Leedy, K.D.; Tomich, D.H.; Subramanyam, G.; Look, D.C. Improved Photoluminescence of Vertically Aligned ZnO Nanorods Grown on BaSrTiO3 by Pulsed Laser Deposition. Nanotechnology 2009, 20, 385706. [Google Scholar] [CrossRef] [PubMed]
- Varanasi, C.V.; Leedy, K.D.; Tomich, D.H.; Subramanyam, G. Large Area Ba1−xSrxTiO3 Thin Films for Microwave Applications Deposited by Pulsed Laser Ablation. Thin Solid Film. 2009, 517, 2878–2881. [Google Scholar] [CrossRef]
- Park, J.; Kim, C.; Kim, J.; Park, J.; Qian, Y.; Ahn, D.; Itoh, T. Modeling of A Photonic Bandgap and Its Application for The Low-Pass Filter Design. In Proceedings of the Asia Pacific Microwave Conference APMC’99 Microwaves Enter the 21st Century, Singapore, 30 November–3 December 1999; Volume 2, pp. 331–334. [Google Scholar]
- Guo, Y.; Wang, Q. An Improved Parameters Extraction Method for Dumbbell-Shaped Defected Ground Structure. Engineering 2010, 2, 197–200. [Google Scholar] [CrossRef][Green Version]
- Annam, K.; Harvey, M.; Shin, E.; Subramanyam, G. Modified Varactor Device Using Barium Strontium Titanate (Ba0.6Sr0.4TiO3) Thin Films for Low Loss Millimeter Wave Frequency Applications. In Proceedings of the NAECON—IEEE National Aerospace and Electronics Conference, Dayton, OH, USA, 15–18 July 2024; pp. 450–453. [Google Scholar]
- Uher, J.; Hoefer, W.J.R. Tunable Microwave and Millimeter-Wave Band-Pass Filters. IEEE Trans. Microw. Theory Tech. 1991, 39, 643–653. [Google Scholar] [CrossRef]
- Annam, K. Reconfigurable RF/Microwave and Millimeterwave Circuits Using Thin Films of Barium Strontium Titanate and Phase Change Materials. Ph.D. Thesis, University of Dayton, Dayton, OH, USA, 2021. [Google Scholar]
- Annam, K.; Spatz, D.; Shin, E.; Subramanyam, G. Experimental Verification of Microwave Phase Shifters Using Barium Strontium Titanate (BST) Varactors. In Proceedings of the IEEE National Aerospace and Electronics Conference (NAECON), Dayton, OH, USA, 15–19 July 2019; pp. 63–66. [Google Scholar]
- Alemayehu, B.; Annam, K.; Shin, E.; Subramanyam, G. Indium-Doped SnO2 Based Surface Acoustic Wave Gas Sensor with Ba0.6Sr0.4TiO3 Film. Crystals 2024, 14, 366. [Google Scholar] [CrossRef]









Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Annam, K.; Alemayehu, B.; Shin, E.; Subramanyam, G. Tunable Filters Using Defected Ground Structures at Millimeter-Wave Frequencies. Micromachines 2025, 16, 60. https://doi.org/10.3390/mi16010060
Annam K, Alemayehu B, Shin E, Subramanyam G. Tunable Filters Using Defected Ground Structures at Millimeter-Wave Frequencies. Micromachines. 2025; 16(1):60. https://doi.org/10.3390/mi16010060
Chicago/Turabian StyleAnnam, Kaushik, Birhanu Alemayehu, Eunsung Shin, and Guru Subramanyam. 2025. "Tunable Filters Using Defected Ground Structures at Millimeter-Wave Frequencies" Micromachines 16, no. 1: 60. https://doi.org/10.3390/mi16010060
APA StyleAnnam, K., Alemayehu, B., Shin, E., & Subramanyam, G. (2025). Tunable Filters Using Defected Ground Structures at Millimeter-Wave Frequencies. Micromachines, 16(1), 60. https://doi.org/10.3390/mi16010060






