Recent Advances in Precision Diamond Wire Sawing Monocrystalline Silicon
Abstract
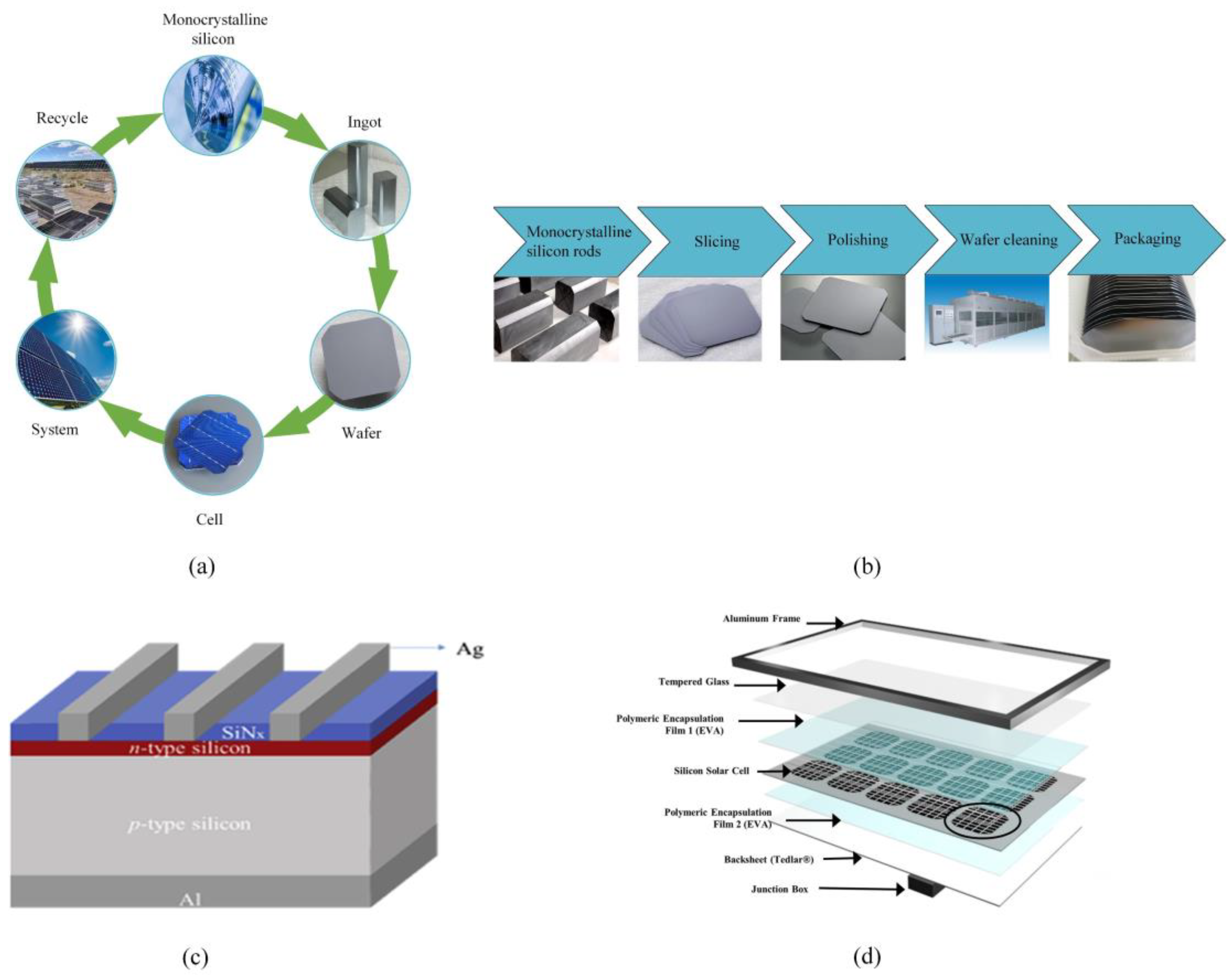
1. Introduction
2. Models and Simulation for DWS
2.1. Mathematical Model
2.2. Finite Element Methods
2.3. Molecular Dynamics Model
2.4. Summary
3. Machining Performance of DWS
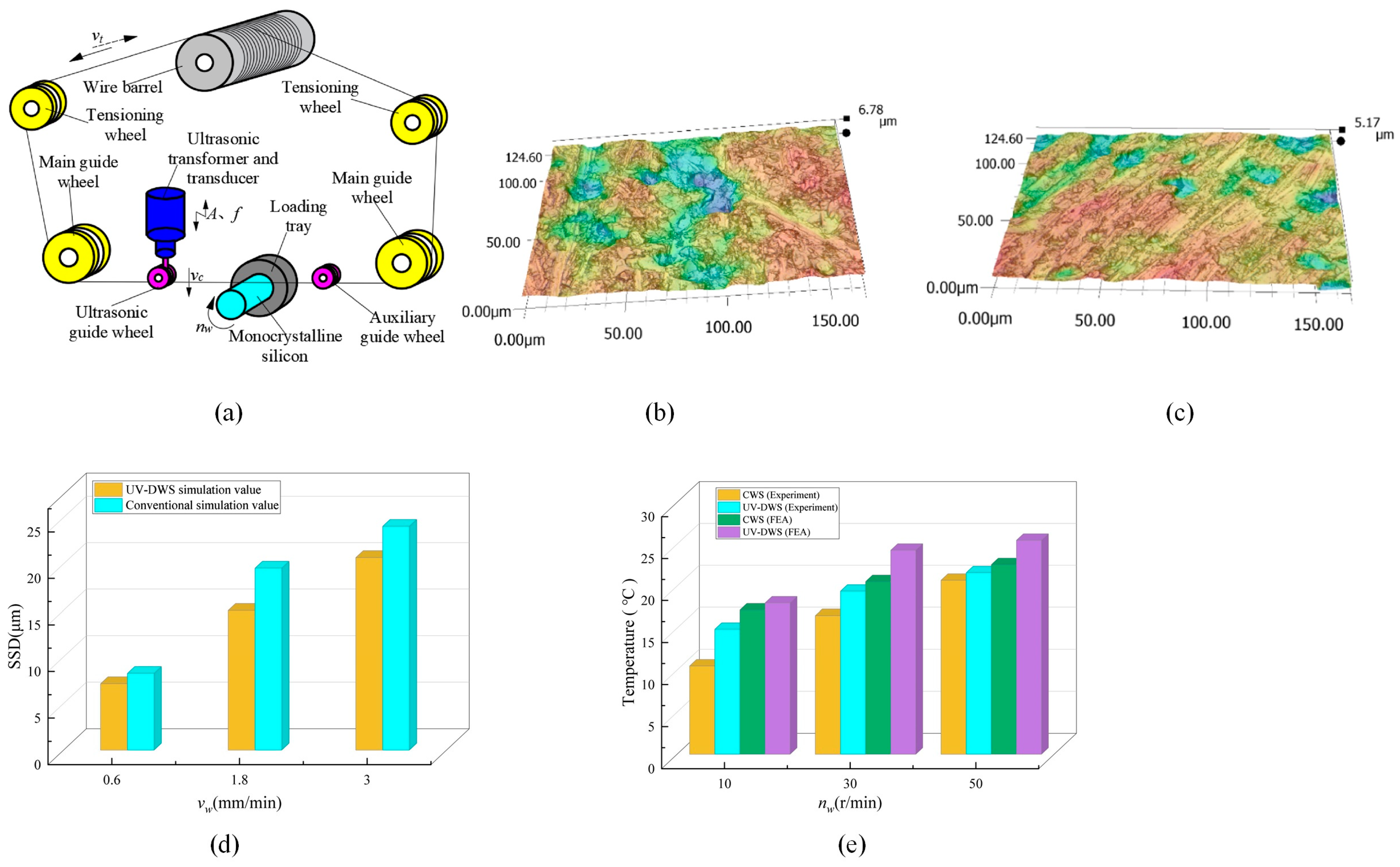
3.1. DWS Equipment
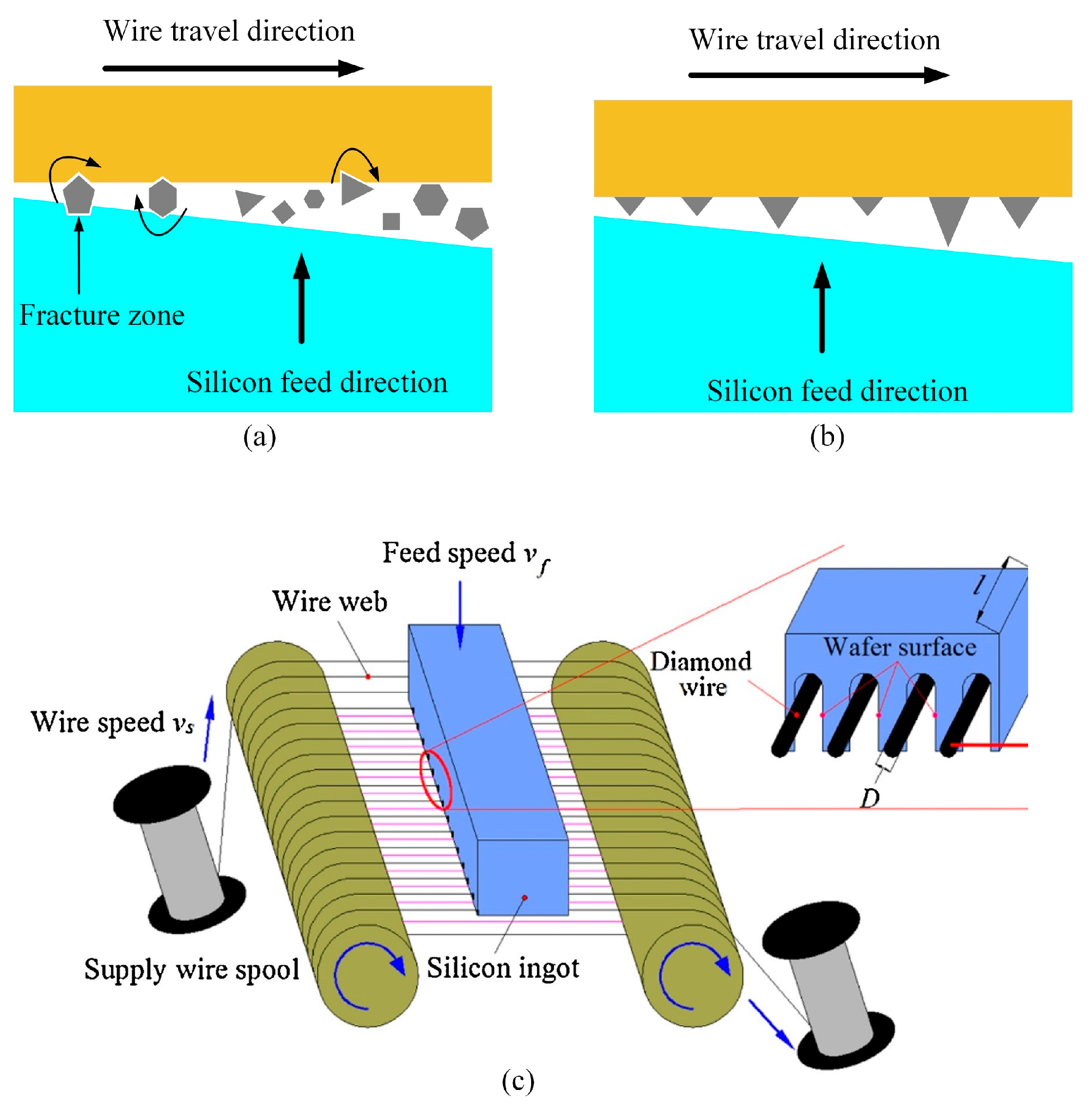
3.2. Material Removal Rate
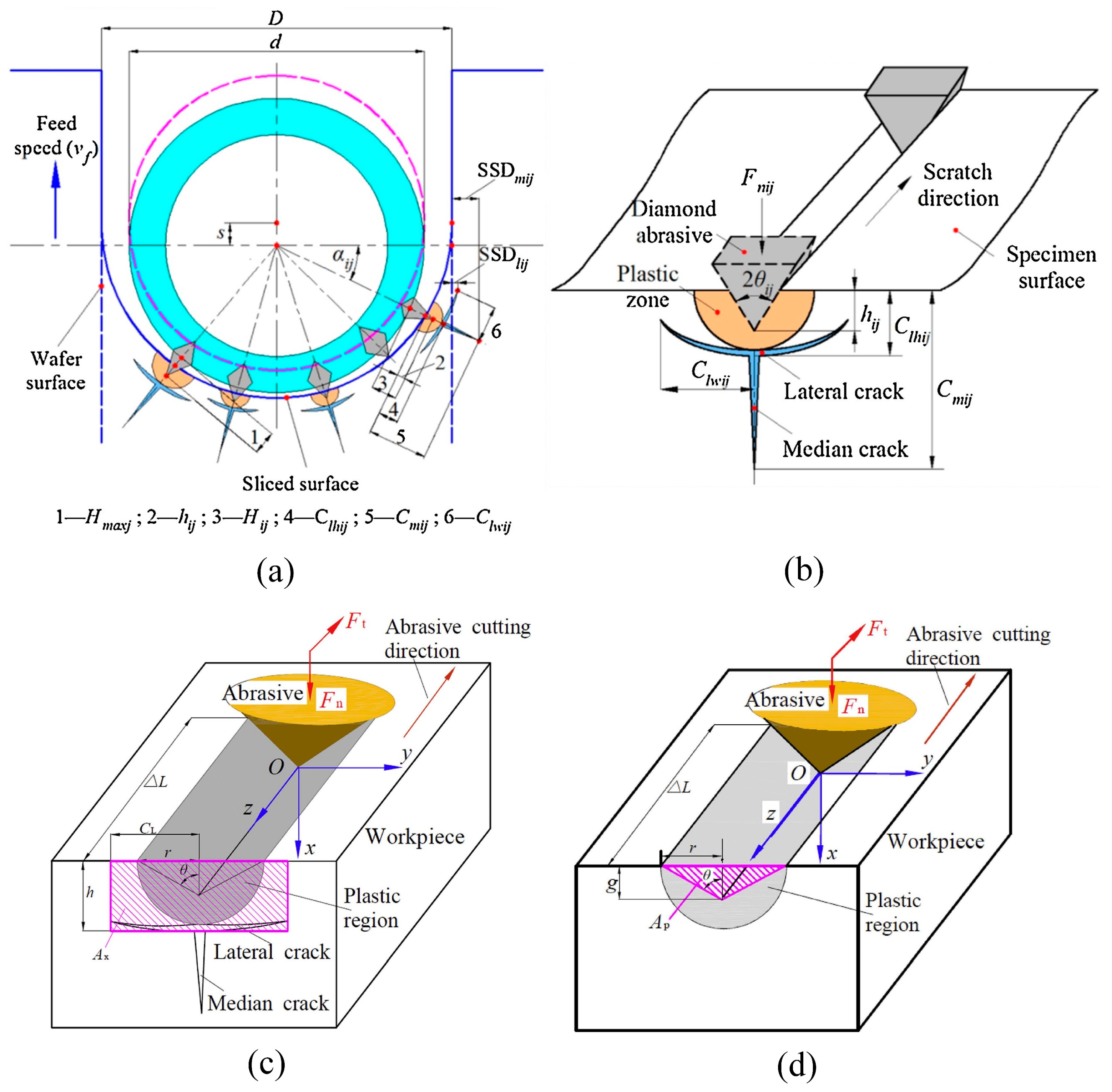
3.3. Surface Morphology and Subsurface Damage
3.4. Summary
4. Hybrid Machining
4.1. Ultrasonic Vibration-Assisted DWS
4.2. Electrical Discharge-Assisted DWS
4.3. Electrochemical -Assisted DWS
4.4. Summary
5. Outlooks
- (1)
- The development of advanced modeling and simulation techniques can aid in the optimization of the cutting process. By utilizing computational models, the complex interrelationships among the cutting tool, the workpiece, and the process parameters can be analyzed [27,110]. Multiple research methods could be combined, such as mathematical modeling with MD, MD with an FEM simulation, or a combination of these three methods. These models can provide insights into material removal mechanisms, stress distributions, and temperature profiles, enabling the prediction and control of surface quality and subsurface damage.
- (2)
- Optimizing the cutting parameters is crucial for achieving greater precision and surface quality. By systematically studying the effects of these process parameters, it becomes possible to understand their complex interplay and identify the optimal settings. Adjusting the wire tension can influence the stability and vibration characteristics of the diamond wire, which in turn affect the cutting process. By understanding the complex interplay between these parameters, it is possible to identify optimal settings that minimize surface roughness and subsurface damage while maximizing productivity.
- (3)
- Combining UV-DWS, ED-DWS, and EC-DWS methods with DWS can enhance the processing of monocrystalline silicon. By combining these methods, the cutting process can be optimized to achieve greater efficiency, better surface quality, and precise control over the cutting parameters. This combination of techniques holds great potential for advancing the DWS of monocrystalline silicon and similar materials. By combining these methods, the cutting process can be optimized to achieve a higher level of efficiency, better surface quality, and precise control over the cutting parameters. Process methods such as laser ultrasound-assisted DWS or a combination of other auxiliary methods may also be introduced in the future to further improve processing quality [189,190]. Non-silicon-based technologies have gained attention due to their unique properties and potential advantages over traditional silicon-based approaches. These technologies offer different characteristics and performance capabilities that may be advantageous in terms of flexibility, energy efficiency, or higher operating frequencies.
- (4)
- Artificial intelligence (AI) technology is growing in various industries. The integration of internal monitoring and feedback systems can enable real-time process control and quality assurance. Machine learning can enable the real-time monitoring of key process parameters and provide feedback for adaptive control [191,192,193]. By incorporating sensors and measurement techniques [194], it becomes possible to monitor key parameters such as the cutting force, temperature, and surface roughness during the cutting process [195,196]. This information can be used to adjust cutting parameters on the fly and ensure consistent and high-quality results.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, Y. Modeling and Experimental Investigation of Monocrystalline Silicon Wafer Cut by Diamond Wire Saw. Eng. Fract. Mech. 2023, 278, 109029. [Google Scholar] [CrossRef]
- Chen, J.; Li, X.; Jia, R.; Tang, Y.; Zhang, D.; Guo, C.; Zhu, H.; Gao, Z. Sulfonate Groups Assisted Texturing for Efficient Monocrystalline Silicon Solar Cells. Sol. Energy Mater. Sol. Cells 2023, 254, 112250. [Google Scholar] [CrossRef]
- Ming, W.; Chen, Z.; Du, J.; Zhang, Z.; Zhang, G.; He, W.; Ma, J.; Shen, F. A Comprehensive Review of Theory and Technology of Glass Molding Process. Int. J. Adv. Manuf. Technol. 2020, 107, 2671–2706. [Google Scholar] [CrossRef]
- Mercaldo, L.V.; Delli Veneri, P. Silicon Solar Cells: Materials, Technologies, Architectures. In Solar Cells and Light Management; Elsevier: Amsterdam, The Netherlands, 2020; pp. 35–57. ISBN 978-0-08-102762-2. [Google Scholar]
- Xu, M.; Xie, P.; Xie, B.-C. Study of China’s Optimal Solar Photovoltaic Power Development Path to 2050. Resour. Policy 2020, 65, 101541. [Google Scholar] [CrossRef]
- Kasap, S.O.; Capper, P. (Eds.) Springer Handbook of Electronic and Photonic Materials; Springer: New York, NY, USA, 2006; ISBN 978-0-387-26059-4. [Google Scholar]
- Deng, R.; Chang, N.L.; Ouyang, Z.; Chong, C.M. A Techno-Economic Review of Silicon Photovoltaic Module Recycling. Renew. Sustain. Energy Rev. 2019, 109, 532–550. [Google Scholar] [CrossRef]
- Fath, P.; Keller, S.; Winter, P.; Jooss, W.; Herbst, W. Status and Perspective of Crystalline Silicon Solar Cell Production. In Proceedings of the 2009 34th IEEE Photovoltaic Specialists Conference (PVSC), Philadelphia, PA, USA, 7–12 June 2009; pp. 002471–002476. [Google Scholar]
- Okil, M.; Salem, M.S.; Abdolkader, T.M.; Shaker, A. From Crystalline to Low-Cost Silicon-Based Solar Cells: A Review. Silicon 2022, 14, 1895–1911. [Google Scholar] [CrossRef]
- Weckend, S.; Wade, A.; Heath, G. End of Life Management: Solar Photovoltaic Panels; NO. NREL/TP-6A20-73852; National Renewable Energy Lab: Golden, CO, USA, 2016; p. 1561525. [Google Scholar]
- Wang, X.; Tian, X.; Chen, X.; Ren, L.; Geng, C. A Review of End-of-Life Crystalline Silicon Solar Photovoltaic Panel Recycling Technology. Sol. Energy Mater. Sol. Cells 2022, 248, 111976. [Google Scholar] [CrossRef]
- Erk, H.F. Wet Etching Technology for Semiconductor and Solar Silicon Manufacturing: Part 2—Process, Equipment and Implementation. ECS Trans. 2010, 27, 1073–1080. [Google Scholar] [CrossRef]
- Li, H.; Gao, Y.; Ge, P.; Bi, W.; Zhang, L. Study on Process Parameters of Fabrication Fine Diameter Electroplated Diamond Wire for Slicing Crystalline Silicon Solar Cell. Int. J. Adv. Manuf. Technol. 2020, 106, 3153–3175. [Google Scholar] [CrossRef]
- Nur’aini, A.; Oh, I. Deep Etching of Silicon Based on Metal-Assisted Chemical Etching. ACS Omega 2022, 7, 16665–16669. [Google Scholar] [CrossRef]
- Negi, S.; Bhandari, R. Silicon Isotropic and Anisotropic Etching for MEMS Applications. Microsyst. Technol. 2013, 19, 203–210. [Google Scholar] [CrossRef]
- Pal, P.; Ashok, A.; Haldar, S.; Xing, Y.; Sato, K. Anisotropic Etching in Low-concentration KOH: Effects of Surfactant Concentration. Micro Amp Nano Lett. 2015, 10, 224–228. [Google Scholar] [CrossRef]
- Naka, Y.; Sugawara, K.; McGreavy, C. Introduction to VLSI Process Engineering; The Society of Chemical Engineers of Japan, Ed.; Springer: Dordrecht, The Netherlands, 1993; ISBN 978-94-010-4682-4. [Google Scholar]
- Arif, M.; Rahman, M.; San, W.Y. A State-of-the-Art Review of Ductile Cutting of Silicon Wafers for Semiconductor and Microelectronics Industries. Int. J. Adv. Manuf. Technol. 2012, 63, 481–504. [Google Scholar] [CrossRef]
- Wang, H.-J.; Yang, T. A Review on Laser Drilling and Cutting of Silicon. J. Eur. Ceram. Soc. 2021, 41, 4997–5015. [Google Scholar] [CrossRef]
- Kumar, A.; Melkote, S.N. Diamond Wire Sawing of Solar Silicon Wafers: A Sustainable Manufacturing Alternative to Loose Abrasive Slurry Sawing. Procedia Manuf. 2018, 21, 549–566. [Google Scholar] [CrossRef]
- Lucca, D.A.; Klopfstein, M.J.; Riemer, O. Ultra-Precision Machining: Cutting with Diamond Tools. J. Manuf. Sci. Eng. 2020, 142, 110817. [Google Scholar] [CrossRef]
- Turchetta, S.; Sorrentino, L.; Bellini, C. A Method to Optimize the Diamond Wire Cutting Process. Diam. Relat. Mater. 2017, 71, 90–97. [Google Scholar] [CrossRef]
- Ke, J.; Chen, X.; Liu, C.; Zhang, J.; Yang, H.; Xu, J. Enhancing the Ductile Machinability of Single-Crystal Silicon by Laser-Assisted Diamond Cutting. Int. J. Adv. Manuf. Technol. 2022, 118, 3265–3282. [Google Scholar] [CrossRef]
- Zhang, Z.; Yan, J.; Kuriyagawa, T. Manufacturing Technologies toward Extreme Precision. Int. J. Extrem. Manuf. 2019, 1, 022001. [Google Scholar] [CrossRef]
- De Oliveira, F.B.; Rodrigues, A.R.; Coelho, R.T.; De Souza, A.F. Size Effect and Minimum Chip Thickness in Micromilling. Int. J. Mach. Tools Manuf. 2015, 89, 39–54. [Google Scholar] [CrossRef]
- Balogun, V.A.; Mativenga, P.T. Impact of Un-Deformed Chip Thickness on Specific Energy in Mechanical Machining Processes. J. Clean. Prod. 2014, 69, 260–268. [Google Scholar] [CrossRef]
- Xiao, H.; Wang, H.; Yu, N.; Liang, R.; Tong, Z.; Chen, Z.; Wang, J. Evaluation of Fixed Abrasive Diamond Wire Sawing Induced Subsurface Damage of Solar Silicon Wafers. J. Mater. Process. Technol. 2019, 273, 116267. [Google Scholar] [CrossRef]
- Yin, Y.; Gao, Y.; Yang, C. Sawing Characteristics of Diamond Wire Cutting Sapphire Crystal Based on Tool Life Cycle. Ceram. Int. 2021, 47, 26627–26634. [Google Scholar] [CrossRef]
- Wilks, J. Performance of Diamonds as Cutting Tools for Precision Machining. Precis. Eng. 1980, 2, 57–72. [Google Scholar] [CrossRef]
- Chung, C.; Tsay, G.D.; Tsai, M.-H. Distribution of Diamond Grains in Fixed Abrasive Wire Sawing Process. Int. J. Adv. Manuf. Technol. 2014, 73, 1485–1494. [Google Scholar] [CrossRef]
- Chung, C.; Nhat, L.V. Generation of Diamond Wire Sliced Wafer Surface Based on the Distribution of Diamond Grits. Int. J. Precis. Eng. Manuf. 2014, 15, 789–796. [Google Scholar] [CrossRef]
- Chung, C.; Le, V.-N. Depth of Cut per Abrasive in Fixed Diamond Wire Sawing. Int. J. Adv. Manuf. Technol. 2015, 80, 1337–1346. [Google Scholar] [CrossRef]
- Nakamura, M.; Sumomogi, T.; Endo, T. Evaluation of Surface and Subsurface Cracks on Nano-Scale Machined Brittle Materials by Scanning Force Microscope and Scanning Laser Microscope. Surf. Coat. Technol. 2003, 169–170, 743–747. [Google Scholar] [CrossRef]
- Bifano, T.G.; Dow, T.A.; Scattergood, R.O. Ductile-Regime Grinding: A New Technology for Machining Brittle Materials. J. Eng. Ind. 1991, 113, 184–189. [Google Scholar] [CrossRef]
- Lawn, B.; Wilshaw, R. Indentation Fracture: Principles and Applications. J. Mater. Sci. 1975, 10, 1049–1081. [Google Scholar] [CrossRef]
- Marshall, D.B.; Lawn, B.R.; Evans, A.G. Elastic/Plastic Indentation Damage in Ceramics: The Lateral Crack System. J. Am. Ceram. Soc. 1982, 65, 561–566. [Google Scholar] [CrossRef]
- Williams, J.A. Engineering Tribology; Cambridge University Press: New York, NY, USA, 2005; ISBN 978-0-521-60988-3. [Google Scholar]
- Liu, T.; Ge, P.; Bi, W.; Gao, Y. Subsurface Crack Damage in Silicon Wafers Induced by Resin Bonded Diamond Wire Sawing. Mater. Sci. Semicond. Process. 2017, 57, 147–156. [Google Scholar] [CrossRef]
- Gao, Y.; Ge, P.; Zhang, L.; Bi, W. Material Removal and Surface Generation Mechanisms in Diamond Wire Sawing of Silicon Crystal. Mater. Sci. Semicond. Process. 2019, 103, 104642. [Google Scholar] [CrossRef]
- Li, S.; Wang, Z.; Wu, Y. Relationship between Subsurface Damage and Surface Roughness of Optical Materials in Grinding and Lapping Processes. J. Mater. Process. Technol. 2008, 205, 34–41. [Google Scholar] [CrossRef]
- Bhagavat, S.; Kao, I. Theoretical Analysis on the Effects of Crystal Anisotropy on Wiresawing Process and Application to Wafer Slicing. Int. J. Mach. Tools Manuf. 2006, 46, 531–541. [Google Scholar] [CrossRef]
- Li, X.; Gao, Y.; Ge, P.; Zhang, L.; Bi, W. The Effect of Cut Depth and Distribution for Abrasives on Wafer Surface Morphology in Diamond Wire Sawing of PV Polycrystalline Silicon. Mater. Sci. Semicond. Process. 2019, 91, 316–326. [Google Scholar] [CrossRef]
- Wu, H.; Melkote, S.N. Effect of Crystal Defects on Mechanical Properties Relevant to Cutting of Multicrystalline Solar Silicon. Mater. Sci. Semicond. Process. 2013, 16, 1416–1421. [Google Scholar] [CrossRef]
- Yin, Y.; Gao, Y.; Wang, L.; Zhang, L.; Pu, T. Analysis of Crack-Free Surface Generation of Photovoltaic Polysilicon Wafer Cut by Diamond Wire Saw. Sol. Energy 2021, 216, 245–258. [Google Scholar] [CrossRef]
- Ming, W.; Zhang, G.; Li, H.; Guo, J.; Zhang, Z.; Huang, Y.; Chen, Z. A Hybrid Process Model for EDM Based on Finite-Element Method and Gaussian Process Regression. Int. J. Adv. Manuf. Technol. 2014, 74, 1197–1211. [Google Scholar] [CrossRef]
- He, W.; Wang, D.; Ming, W.; Ma, J.; Liu, K.; Du, J. Research Progress on Cutting Machining Simulation Technology of Metallic Glasses. Int. J. Adv. Manuf. Technol. 2022, 122, 1167–1193. [Google Scholar] [CrossRef]
- Du, J.; Zhang, H.; He, W.; Ma, J.; Ming, W.; Cao, Y. Simulation and Experimental Study on Surface Formation Mechanism in Machining of SiCp/Al Composites. Appl. Compos. Mater. 2019, 26, 29–40. [Google Scholar] [CrossRef]
- Kumar Dewangan, S.; Kumar Tripathi, M.; Nandan Banjare, P.; Kumar Manoj, M. Temperature Distribution of Friction Stir Welded Al 7075 Alloy Using Finite Element Simulation along with Experimental Validation. Mater. Today Proc. 2023, S2214785323032376. [Google Scholar] [CrossRef]
- He, B.; Wang, H.; Yang, W. Design and Adaptive Finite Element Simulation of Conformal Transformation Optics Devices with Isotropic Materials. Comput. Math. Appl. 2023, 144, 198–209. [Google Scholar] [CrossRef]
- Fu, Y.-F.; Reiner, J. Objective and Automated Calibration of Progressive Damage Models for Finite Element Simulation of Fiber Reinforced Composites. Compos. Struct. 2023, 307, 116618. [Google Scholar] [CrossRef]
- Zhao, M.; Pei, X.; Luo, S.; Yan, M.; Xing, W.; Wang, W.; Liu, S.; Shao, R.; Xu, Z. Research on Mechanical Properties and Failure Mechanism of TRM Composites via Digital Image Correlation Method and Finite Element Simulation. Compos. Struct. 2023, 311, 116798. [Google Scholar] [CrossRef]
- Wei, Y.; Li, Y.; Huang, D.; Zhou, C.; Zhao, J. Fracture Properties of Nanoscale Single-Crystal Silicon Plates: Molecular Dynamics Simulations and Finite Element Method. Eng. Fract. Mech. 2018, 202, 1–19. [Google Scholar] [CrossRef]
- Zhang, L.; Barrett, R.; Cloetens, P.; Detlefs, C.; Sanchez Del Rio, M. Anisotropic Elasticity of Silicon and Its Application to the Modelling of X-Ray Optics. J. Synchrotron Rad. 2014, 21, 507–517. [Google Scholar] [CrossRef]
- Hauch, J.A.; Holland, D.; Marder, M.P.; Swinney, H.L. Dynamic Fracture in Single Crystal Silicon. Phys. Rev. Lett. 1999, 82, 3823–3826. [Google Scholar] [CrossRef]
- Zhu, T.; Li, J.; Yip, S. Atomistic Configurations and Energetics of Crack Extension in Silicon. Phys. Rev. Lett. 2004, 93, 205504. [Google Scholar] [CrossRef]
- Cook, R.F. Strength and Sharp Contact Fracture of Silicon. J. Mater. Sci. 2006, 41, 841–872. [Google Scholar] [CrossRef]
- Cheng, D.; Gao, Y.; Liu, R. Finite Element Analysis on Processing Stress of Polysilicon Cut by Diamond Multi-Wire Saw. Mater. Sci. Semicond. Process. 2021, 131, 105860. [Google Scholar] [CrossRef]
- Wallburg, F.; Kuna, M.; Budnitzki, M.; Schoenfelder, S. A Material Removal Coefficient for Diamond Wire Sawing of Silicon. Wear 2022, 504–505, 204400. [Google Scholar] [CrossRef]
- Bhagavat, S.; Kao, I. A Finite Element Analysis of Temperature Variation in Silicon Wafers during Wiresaw Slicing. Int. J. Mach. Tools Manuf. 2008, 48, 95–106. [Google Scholar] [CrossRef]
- Gao, Y.; Chen, Y.; Ge, P.; Zhang, L.; Bi, W. Study on the Subsurface Microcrack Damage Depth in Electroplated Diamond Wire Saw Slicing SiC Crystal. Ceram. Int. 2018, 44, 22927–22934. [Google Scholar] [CrossRef]
- Wu, C.; Jiang, Z.; Fan, W.; Chen, L. Finite Element Analysis of Multi-Wire Saw Silicon Rods with Consolidated Abrasive Diamonds. Int. J. Adv. Manuf. Technol. 2017, 90, 241–248. [Google Scholar] [CrossRef]
- Huang, H.; Xu, Y.; Luo, G.; Xie, Z.; Ming, W. Molecular Dynamics Study of Laser Interaction with Nanoparticles in Liquids and Its Potential Application. Nanomaterials 2022, 12, 1524. [Google Scholar] [CrossRef]
- Chen, Y.; Guo, X.; Zhang, G.; Cao, Y.; Shen, D.; Li, X.; Zhang, S.; Ming, W. Development of a Hybrid Intelligent Process Model for Micro-Electro Discharge Machining Using the TTM-MDS and Gaussian Process Regression. Micromachines 2022, 13, 845. [Google Scholar] [CrossRef]
- Huang, H.; Yang, W.; Ming, W.; Zhang, G.; Xu, Y.; Zhang, Z. Mechanism of Springback Behavior in Ultra-Thin Glass Molding Process: A Molecular Dynamics Study. J. Non-Cryst. Solids 2022, 596, 121841. [Google Scholar] [CrossRef]
- Beatty, M.F. Mathematical concepts and methods in science and engineering. In Principles of Engineering Mechanics; Plenum Press: New York, NY, USA, 1986; ISBN 978-0-306-42131-0. [Google Scholar]
- Vinogradov, O. Coulomb’s Law Based Interatomic Potential for Iron Crystals in Molecular Statics Applications. Comput. Mater. Sci. 2015, 99, 173–176. [Google Scholar] [CrossRef]
- López-Villellas, L.; Kjelgaard Mikkelsen, C.C.; Galano-Frutos, J.J.; Marco-Sola, S.; Alastruey-Benedé, J.; Ibáñez, P.; Moretó, M.; Sancho, J.; García-Risueño, P. Accurate and Efficient Constrained Molecular Dynamics of Polymers Using Newton’s Method and Special Purpose Code. Comput. Phys. Commun. 2023, 288, 108742. [Google Scholar] [CrossRef]
- Toikka, A.M.; Petrov, A.V. Comparative Analysis of Molecular Interactions in Quaternary Fluid System Performed by Classical and Ab Initio Molecular Dynamics. Mendeleev Commun. 2023, 33, 413–415. [Google Scholar] [CrossRef]
- Badar, M.S.; Shamsi, S.; Ahmed, J.; Alam, M.A. Molecular Dynamics Simulations: Concept, Methods, and Applications. In Transdisciplinarity; Rezaei, N., Ed.; Integrated Science; Springer International Publishing: Cham, Switzerland, 2022; Volume 5, pp. 131–151. ISBN 978-3-030-94650-0. [Google Scholar]
- Li, M.; Guo, X.; Kang, R.; Guo, D.; Zhou, P. Study on the Transformation and Control Mechanism of Amorphous Damage during the Grinding Process of Monocrystalline Silicon Considering Grain Shapes by MD Method. Tribol. Int. 2023, 187, 108720. [Google Scholar] [CrossRef]
- Wang, T.; Cheng, Y.; Liu, Y.; Qu, N.; Lai, Z.; Zhang, X.; Zhu, J. The Mechanics and Design of a Local Crystallization of Amorphous for Carbon Material by Molecular Dynamics Simulation. J. Non-Cryst. Solids 2023, 600, 121991. [Google Scholar] [CrossRef]
- Thomas Parambi, D.G.; Oh, J.M.; Kumar, S.; Sudevan, S.T.; Hendawy, O.M.; Abdelgawad, M.A.; Musa, A.; Al-Sanea, M.M.; Ahmad, I.; Patel, H.; et al. Halogenated Class of Oximes as a New Class of Monoamine Oxidase-B Inhibitors for the Treatment of Parkinson’s Disease: Synthesis, Biochemistry, and Molecular Dynamics Study. Comput. Biol. Chem. 2023, 105, 107899. [Google Scholar] [CrossRef]
- Buchholz, M.; Bungartz, H.-J.; Vrabec, J. Software Design for a Highly Parallel Molecular Dynamics Simulation Framework in Chemical Engineering. J. Comput. Sci. 2011, 2, 124–129. [Google Scholar] [CrossRef]
- Liu, Y.; Wan, W.; Li, Q.; Xiong, Z.; Tang, C.; Zhou, L. Revisiting the Rate-Dependent Mechanical Response of Typical Silicon Structures via Molecular Dynamics. Nanomaterials 2022, 12, 1203. [Google Scholar] [CrossRef]
- Zhang, W.J.; Chen, J.S.; Li, S.; Wu, Y.H.; Zhang, P.L.; Yu, Z.S.; Yue, Z.H.; Chun, Y.; Lu, H. Electronic and Mechanical Properties of Monocrystalline Silicon Doped with Trace Content of N or P: A First-Principles Study. Solid State Sci. 2021, 120, 106723. [Google Scholar] [CrossRef]
- Dai, H.; Li, S.; Chen, G. Comparison of Subsurface Damages on Mono-Crystalline Silicon between Traditional Nanoscale Machining and Laser-Assisted Nanoscale Machining via Molecular Dynamics Simulation. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2018, 414, 61–67. [Google Scholar] [CrossRef]
- Zhu, G.; Chao, D.; Xu, W.; Wu, M.; Zhang, H. Microscale Silicon-Based Anodes: Fundamental Understanding and Industrial Prospects for Practical High-Energy Lithium-Ion Batteries. ACS Nano 2021, 15, 15567–15593. [Google Scholar] [CrossRef]
- Tang, C.Y.; Zhang, L.C.; Mylvaganam, K. Rate Dependent Deformation of a Silicon Nanowire under Uniaxial Compression: Yielding, Buckling and Constitutive Description. Comput. Mater. Sci. 2012, 51, 117–121. [Google Scholar] [CrossRef]
- Rabier, J. On a Plasticity by Partial Dislocations in Silicon at Very High Stress. Mater. Today Proc. 2018, 5, 14705–14711. [Google Scholar] [CrossRef]
- Chavoshi, S.Z.; Xu, S.; Luo, X. Dislocation-Mediated Plasticity in Silicon during Nanometric Cutting: A Molecular Dynamics Simulation Study. Mater. Sci. Semicond. Process. 2016, 51, 60–70. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, J.; Wang, Z.; Hartmaier, A.; Yan, Y.; Sun, T. Interaction between Phase Transformations and Dislocations at Incipient Plasticity of Monocrystalline Silicon under Nanoindentation. Comput. Mater. Sci. 2017, 131, 55–61. [Google Scholar] [CrossRef]
- Yang, T.; Yang, X.; Huang, R.; Liechti, K.M. Rate-Dependent Traction-Separation Relations for a Silicon/Epoxy Interface Informed by Experiments and Bond Rupture Kinetics. J. Mech. Phys. Solids 2019, 131, 1–19. [Google Scholar] [CrossRef]
- Chen, T.; Xu, R.; Li, Q. Effect of Strain Rate on Tensile Strength of Defective Silicon Nanorods. Acta Mech. Solida Sin. 2015, 28, 133–144. [Google Scholar] [CrossRef]
- Ivashchenko, V.I.; Turchi, P.E.A.; Shevchenko, V.I. Simulations of the Mechanical Properties of Crystalline, Nanocrystalline, and Amorphous SiC and Si. Phys. Rev. B 2007, 75, 085209. [Google Scholar] [CrossRef]
- Chandran, L.; Rapaka, S.D.; Eswara Prasad, K.; Annabattula, R.K. Modeling the Failure of Silicon Carbide under High Strain-Rate Compression: A Parametric Study. Mater. Today: Proc. 2023, S2214785323012099. [Google Scholar] [CrossRef]
- Li, W.; Hahn, E.N.; Branicio, P.S.; Yao, X.; Germann, T.C.; Feng, B.; Zhang, X. Defect Reversibility Regulates Dynamic Tensile Strength in Silicon Carbide at High Strain Rates. Scr. Mater. 2022, 213, 114593. [Google Scholar] [CrossRef]
- Zhang, Y.; Cao, P.; Deng, B.; Huang, L.; Shi, Y. Strain Rate-Dependent Tensile Response of Glassy Silicon Nanowires Studied by Accelerated Atomistic Simulations. J. Appl. Phys. 2021, 130, 085105. [Google Scholar] [CrossRef]
- Liu, Y. Molecular Dynamics Simulation Study on the Mechanism of Single Crystal Silicon Diamond Wire Cutting. Ph.D. Thesis, Nanchang University, Nanchang, China, 2022. [Google Scholar]
- Olufayo, O.A.; Abou-El-Hossein, K. Molecular Dynamics Modeling of Nanoscale Machining of Silicon. Procedia CIRP 2013, 8, 504–509. [Google Scholar] [CrossRef]
- Dai, H.; Chen, G.; Li, S.; Fang, Q.; Hu, B. Influence of Laser Nanostructured Diamond Tools on the Cutting Behavior of Silicon by Molecular Dynamics Simulation. RSC Adv. 2017, 7, 15596–15612. [Google Scholar] [CrossRef]
- Skalka, P.; Kotoul, M. Determination of Mechanical and Fracture Properties of Silicon Single Crystal from Indentation Experiments and Finite Element Modelling. Materials 2021, 14, 6864. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.; Basaran, C. A Multiscale Modeling Technique for Bridging Molecular Dynamics with Finite Element Method. J. Comput. Phys. 2013, 253, 64–85. [Google Scholar] [CrossRef]
- Moulins, A.; Ma, L.; Dugnani, R.; Zednik, R.J. Dynamic Crack Modeling and Analytical Stress Field Analysis in Single-Crystal Silicon Using Quantitative Fractography. Theor. Appl. Fract. Mech. 2020, 109, 102693. [Google Scholar] [CrossRef]
- Cervera, M.; Barbat, G.B.; Chiumenti, M.; Wu, J.-Y. A Comparative Review of XFEM, Mixed FEM and Phase-Field Models for Quasi-Brittle Cracking. Arch. Comput. Methods Eng. 2022, 29, 1009–1083. [Google Scholar] [CrossRef]
- Dai, H.; Zhou, Y.; Li, P.; Zhang, Y. Evolution of Nano-Cracks in Single-Crystal Silicon during Ultraprecision Mechanical Polishing. J. Manuf. Process. 2020, 58, 627–636. [Google Scholar] [CrossRef]
- Gurnett, K.; Adams, T. Ultra-Thin Semiconductor Wafer Applications and Processes. III-Vs Review 2006, 19, 38–40. [Google Scholar] [CrossRef][Green Version]
- Dwivedi, S. Fabrication and Manufacturing Process of Solar Cell: Part I. In Green Energy; Tripathi, S.L., Padmanaban, S., Eds.; Wiley: Hoboken, NJ, USA, 2020; pp. 1–37. ISBN 978-1-119-76076-4. [Google Scholar]
- Hara, Y.; Ide, K.; Nishihara, T.; Yokogawa, R.; Nakamura, K.; Ohshita, Y.; Kawatsu, T.; Nagai, T.; Aoki, Y.; Kobayashi, H.; et al. Effect of Sawing Damage on Flexibility of Crystalline Silicon Wafers for Thin Flexible Silicon Solar Cells. Jpn. J. Appl. Phys. 2023, 62, 017001. [Google Scholar] [CrossRef]
- Hardin, C.W.; Qu, J.; Shih, A.J. Fixed Abrasive Diamond Wire Saw Slicing of Single-Crystal Silicon Carbide Wafers. Mater. Manuf. Process. 2004, 19, 355–367. [Google Scholar] [CrossRef]
- Zarepour, H.; Saptaji, K.; Subbiah, S.; Butler, D.L. Novel Diamond-Slurry Wire Sawing Process for Silicon Wafering. In Proceedings of the 29th European Photovoltaic Solar Energy Conference and Exhibition, Amsterdam, The Netherlands, 2–26 September 2014; pp. 773–775. [Google Scholar] [CrossRef]
- Li, S.; Tang, A.; Liu, Y.; Wang, J.; Cui, D.; Landers, R.G. Analytical Force Modeling of Fixed Abrasive Diamond Wire Saw Machining With Application to SiC Monocrystal Wafer Processing. J. Manuf. Sci. Eng. 2017, 139, 041003. [Google Scholar] [CrossRef]
- Li, S.; Wang, J.; Tang, A.; Landers, R.G. Force Modeling of Silicon Monocrystal Wire Saw Machining. In Proceedings of the 2016 International Symposium on Flexible Automation (ISFA), Cleveland, OH, USA, 1–3 August 2016; pp. 127–132. [Google Scholar]
- Teomete, E. Roughness Damage Evolution Due to Wire Saw Process. Int. J. Precis. Eng. Manuf. 2011, 12, 941–947. [Google Scholar] [CrossRef]
- Suzuki, T.; Nishino, Y.; Yan, J. Mechanisms of Material Removal and Subsurface Damage in Fixed-Abrasive Diamond Wire Slicing of Single-Crystalline Silicon. Precis. Eng. 2017, 50, 32–43. [Google Scholar] [CrossRef]
- Wallburg, F.; Kuna, M.; Budnitzki, M.; Schoenfelder, S. Experimental and Numerical Analysis of Scratching Induced Damage during Diamond Wire Sawing of Silicon. Wear 2020, 454–455, 203328. [Google Scholar] [CrossRef]
- Chou, Y.-C.; Tsai, P.-H.; Chen, Y.-C.; Wu, C.-H. Studies of Diamond Wire Sawn Wafers among Different Diamond Size. In Proceedings of the 2013 IEEE 39th Photovoltaic Specialists Conference (PVSC), Tampa, FL, USA, 16–21 June 2013; pp. 502–505. [Google Scholar]
- Albrecht, D.; Möhring, H.-C. Potentials for the Optimization of Sawing Processes Using the Example of Bandsawing Machines. Procedia Manuf. 2018, 21, 567–574. [Google Scholar] [CrossRef]
- Costa, E.C.; Xavier, F.A.; Knoblauch, R.; Binder, C.; Weingaertner, W.L. Effect of Cutting Parameters on Surface Integrity of Monocrystalline Silicon Sawn with an Endless Diamond Wire Saw. Sol. Energy 2020, 207, 640–650. [Google Scholar] [CrossRef]
- Qiu, J.; Liu, C.; Zhang, S. The Machining Accuracy and Surface Roughness of Mono-Crystalline Silicon Regarding Wire Lag and Wire Stiffness of Endless Diamond Wire. Int. J. Adv. Manuf. Technol. 2021, 117, 3253–3265. [Google Scholar] [CrossRef]
- Lai, Z.; Huang, H.; Hu, Z.; Liao, X. Dynamic Model and Machining Mechanism of Wire Sawing. J. Mater. Process. Technol. 2023, 311, 117820. [Google Scholar] [CrossRef]
- Dong, P.; Zhang, J.; Ouyang, C.; Sun, D.; Wu, J. Investigation on Sawing Performance of Diamond Frame Saw Based on Reciprocating Swing in Processing Hard Stone. J. Mater. Process. Technol. 2021, 295, 117171. [Google Scholar] [CrossRef]
- Xu, Z.; Feng, Y.; Pan, L.; Wang, X.; Wang, H.; Jia, X. Influence of Ingot Rocking on the Surface Quality of Multi-Wire Sawing Monocrystalline Silicon Wafers. Int. J. Adv. Manuf. Technol. 2020, 107, 15–24. [Google Scholar] [CrossRef]
- Costa, E.C.; Weingaertner, W.L.; Xavier, F.A. Influence of Single Diamond Wire Sawing of Photovoltaic Monocrystalline Silicon on the Feed Force, Surface Roughness and Micro-Crack Depth. Mater. Sci. Semicond. Process. 2022, 143, 106525. [Google Scholar] [CrossRef]
- Chen, C.-C.A.; Gupta, A. Modeling and Analysis of Wire Motion during Rocking Mode Diamond Wire Sawing of Mono-Crystalline Alumina Oxide Wafer. Int. J. Adv. Manuf. Technol. 2018, 95, 3453–3463. [Google Scholar] [CrossRef]
- Li, Z.; Wang, M.; Cai, Y.; Jia, H. Experimental Study on Surface Topography and Fracture Strength of Worn Saw Wire in Multi-Wire Sawing. Int. J. Adv. Manuf. Technol. 2017, 93, 4125–4132. [Google Scholar] [CrossRef]
- Anspach, O.; Hurka, B.; Sunder, K. Structured Wire: From Single Wire Experiments to Multi-Crystalline Silicon Wafer Mass Production. Sol. Energy Mater. Sol. Cells 2014, 131, 58–63. [Google Scholar] [CrossRef]
- Carton, L.; Riva, R.; Nelias, D.; Fourmeau, M.; Coustier, F.; Chabli, A. Comparative Analysis of Mechanical Strength of Diamond-Sawn Silicon Wafers Depending on Saw Mark Orientation, Crystalline Nature and Thickness. Sol. Energy Mater. Sol. Cells 2019, 201, 110068. [Google Scholar] [CrossRef]
- Teomete, E. Wire Saw Process-Induced Surface Damage Characterization. Arab. J. Sci. Eng. 2013, 38, 1209–1215. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, Z.; Zhang, Y.; Liu, D.; Wu, J.; Huang, Y.; Zhang, G. Study on Machining Characteristics of Magnetically Controlled Laser Induced Plasma Micro-Machining Single-Crystal Silicon. J. Adv. Res. 2021, 30, 39–51. [Google Scholar] [CrossRef]
- Liu, Y.; Guo, H.; Wang, H.; Zhang, Y.; Zhang, Z. Comparative Analysis of Bubbles Behavior in Different Liquids by Laser-Induced Plasma Micromachining Single-Crystal Silicon. Crystals 2022, 12, 286. [Google Scholar] [CrossRef]
- Kray, D.; Schumann, M.; Eyer, A.; Willeke, G.P.; Kubler, R.; Beinert, J.; Kleer, G. Solar Wafer Slicing with Loose and Fixed Grains. In Proceedings of the 2006 IEEE 4th World Conference on Photovoltaic Energy Conference, Waikoloa, HI, USA, 7–12 May 2006; pp. 948–951. [Google Scholar]
- Meinel, B.; Koschwitz, T.; Acker, J. Textural Development of SiC and Diamond Wire Sawed Sc-Silicon Wafer. Energy Procedia 2012, 27, 330–336. [Google Scholar] [CrossRef]
- Kumar, A.; Melkote, S.N.; Kaminski, S.; Arcona, C. Effect of Grit Shape and Crystal Structure on Damage in Diamond Wire Scribing of Silicon. J. Am. Ceram. Soc. 2017, 100, 1350–1359. [Google Scholar] [CrossRef]
- Qiu, J.; Li, X.; Ge, R.; Zhang, S.; Wang, H. Formation Mechanism of Wire Bow and Its Influence on Diamond Wire Saw Process and Wire Cutting Capability. Int. J. Mech. Sci. 2020, 185, 105851. [Google Scholar] [CrossRef]
- Qiu, J. Fundamental Research on Machining Performance of Diamond Wire Sawing and Diamond Wire Electrical Discharge Sawing Quartz Glass. Ceram. Int. 2022, 48, 24332–24345. [Google Scholar] [CrossRef]
- Ming, W.; Zhang, Z.; Wang, S.; Huang, H.; Zhang, Y.; Zhang, Y.; Shen, D. Investigating the Energy Distribution of Workpiece and Optimizing Process Parameters during the EDM of Al6061, Inconel718, and SKD11. Int. J. Adv. Manuf. Technol. 2017, 92, 4039–4056. [Google Scholar] [CrossRef]
- Ming, W.; Cao, C.; Shen, F.; Zhang, Z.; Liu, K.; Du, J.; Jia, H. Numerical and Experimental Study on WEDM of BN-AlN-TiB2 Composite Ceramics Based on a Fusion FEM Model. J. Manuf. Process. 2022, 76, 138–154. [Google Scholar] [CrossRef]
- Liu, Y.; Gao, Y.; Yang, C. Analysis of Sawing Characteristics of Fine Diamond Wire Slicing Multicrystalline Silicon. Diam. Relat. Mater. 2021, 120, 108708. [Google Scholar] [CrossRef]
- Zhang, Z.; Ming, W.; Zhang, G.; Huang, Y.; Wen, X.; Huang, H. A New Method for On-Line Monitoring Discharge Pulse in WEDM-MS Process. Int. J. Adv. Manuf. Technol. 2015, 81, 1403–1418. [Google Scholar] [CrossRef]
- Ming, W.; Ma, J.; Zhang, Z.; Huang, H.; Shen, D.; Zhang, G.; Huang, Y. Soft Computing Models and Intelligent Optimization System in Electro-Discharge Machining of SiC/Al Composites. Int. J. Adv. Manuf. Technol. 2016, 87, 201–217. [Google Scholar] [CrossRef]
- Kumar, A.; Kaminski, S.; Melkote, S.N.; Arcona, C. Effect of Wear of Diamond Wire on Surface Morphology, Roughness and Subsurface Damage of Silicon Wafers. Wear 2016, 364–365, 163–168. [Google Scholar] [CrossRef]
- Ge, M.; Chen, Z.; Wang, P.; Ge, P. Crack Damage Control for Diamond Wire Sawing of Silicon: The Selection of Processing Parameters. Mater. Sci. Semicond. Process. 2022, 148, 106838. [Google Scholar] [CrossRef]
- Kumbera, T.G.; Cherukuri, H.P.; Patten, J.A.; Brand, C.J.; Marusich, T.D. Numerical Simulations of Ductile Machining of Silicon Nitride with a Cutting Tool of Defined Geometry. Mach. Sci. Technol. 2001, 5, 341–352. [Google Scholar] [CrossRef]
- Zareena, A.R.; Veldhuis, S.C. Tool Wear Mechanisms and Tool Life Enhancement in Ultra-Precision Machining of Titanium. J. Mater. Process. Technol. 2012, 212, 560–570. [Google Scholar] [CrossRef]
- Sharma, K.; Mahto, D.; Sen, S.S. In Metal Turning, Effect of Various Parameters on Cutting Tool: A Review. Int. J. Appl. Innov. Eng. Manag. (IJAIEM) 2013, 2, 32–38. [Google Scholar]
- De Oliveira, L.J.; Cabral, S.C.; Filgueira, M. Study Hot Pressed Fe-Diamond Composites Graphitization. Int. J. Refract. Met. Hard Mater. 2012, 35, 228–234. [Google Scholar] [CrossRef]
- Marsh, E.R.; Sommer, E.J.; Deakyne, T.R.S.; Kim, G.A.; Simonson, J.A. Detection of Orientation-Dependent, Single-Crystal Diamond Tool Edge Wear Using Cutting Force Sensors, While Spin-Turning Silicon. Precis. Eng. 2010, 34, 253–258. [Google Scholar] [CrossRef]
- Chung, C.H. Abrasive Distribution of the Fixed Diamond Wire in Wire Sawing Process. Adv. Mater. Res. 2012, 579, 145–152. [Google Scholar] [CrossRef]
- Yin, S.; Xiao, H.; Wu, H.; Wang, C.; Cheung, C.F. Image-Processing-Based Model for the Characterization of Surface Roughness and Subsurface Damage of Silicon Wafer in Diamond Wire Sawing. Precis. Eng. 2022, 77, 263–274. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, B.; Huang, S.; Qian, Z. Study on the Subsurface Damage Depth of Monocrystalline Silicon in Ultrasonic Vibration Assisted Diamond Wire Sawing. Eng. Fract. Mech. 2021, 258, 108077. [Google Scholar] [CrossRef]
- Qiu, J.; Li, X.; Ge, R.; Liu, C. Surface Formation, Morphology, Integrity and Wire Marks in Diamond Wire Slicing of Mono-Crystalline Silicon in the Photovoltaic Industry. Wear 2022, 488–489, 204186. [Google Scholar] [CrossRef]
- Tao, H.; Zeng, Q.; Liu, Y.; Zhao, D.; Lu, X. Effects of Grinding-Induced Surface Topography on the Material Removal Mechanism of Silicon Chemical Mechanical Polishing. Appl. Surf. Sci. 2023, 631, 157509. [Google Scholar] [CrossRef]
- Kang, D.; Park, H.; Choi, D.; Han, H.; Seol, J.; Kang, Y.; Lee, H.-S.; Kim, D. Damage and Residual Layer Analysis of Reactive Ion Etching Textured Multi-Crystalline Silicon Wafer for Application to Solar Cells. Sol. Energy 2022, 233, 111–117. [Google Scholar] [CrossRef]
- Wang, L.; Gao, Y.; Pu, T.; Yin, Y. Fracture Strength of Photovoltaic Silicon Wafers Cut by Diamond Wire Saw Based on Half-Penny Crack System. Eng. Fract. Mech. 2021, 251, 107717. [Google Scholar] [CrossRef]
- Satake, U.; Enomoto, T. Kinematic Analysis of Double-Sided Polishing of Silicon Wafers for Improving Surface Flatness. Procedia CIRP 2023, 117, 56–61. [Google Scholar] [CrossRef]
- Mukherjee, J.; Ranjan, A.; Saxena, A.K.; Das, P.K.; Banerjee, R. Liquid Polycarbosilane Derived SiC Coating on Silicon (111) Wafer for Enhanced Mechanical Properties. Appl. Surf. Sci. 2013, 270, 219–224. [Google Scholar] [CrossRef]
- Gupta, A.; Chen, C.-C.A.; Hsu, H.-W. Study on Diamond Wire Wear, Surface Quality, and Subsurface Damage during Multi-Wire Slicing of c-Plane Sapphire Wafer. Int. J. Adv. Manuf. Technol. 2019, 100, 1801–1814. [Google Scholar] [CrossRef]
- Kang, R.K.; Zeng, Y.F.; Gao, S.; Dong, Z.G.; Guo, D.M. Surface Layer Damage of Silicon Wafers Sliced by Wire Saw Process. Adv. Mater. Res. 2013, 797, 685–690. [Google Scholar] [CrossRef]
- Sekhar, H.; Fukuda, T.; Tanahashi, K.; Takato, H.; Ono, H.; Sampei, Y.; Kobayashi, T. Mechanical Strength Problem of Thin Silicon Wafers (120 and 140 μm) Cut with Thinner Diamond Wires (Si Kerf 120 → 100 μm) for Photovoltaic Use. Mater. Sci. Semicond. Process. 2020, 119, 105209. [Google Scholar] [CrossRef]
- Schumann, M.; Singh, M.; Pérez, T.O.; Riepe, S. Reaching a Kerf Loss Below 100 Μm by Optimizing the Relation between Wire Thickness and Abrasive Size for Multi-Wire Sawing. In Proceedings of the 24th European Photovoltaic Solar Energy Conference, Hamburg, Germany, 21–25 September 2009. [Google Scholar]
- Liu, C.; Qiu, J.; Zhao, S. Tension Analysis and Fluctuation Control of Diamond Multi-Wire Sawing Machine. Int. J. Adv. Manuf. Technol. 2021, 115, 1387–1397. [Google Scholar] [CrossRef]
- Wu, H. Wire Sawing Technology: A State-of-the-Art Review. Precis. Eng. 2016, 43, 1–9. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, R.; Li, S.; Liu, J.; Song, L. Prediction and Verification of Wafer Surface Morphology in Ultrasonic Vibration Assisted Wire Saw (UAWS) Slicing Single Crystal Silicon Based on Mixed Material Removal Mode. Int. J. Adv. Manuf. Technol. 2022, 120, 6789–6806. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, D.; Singh, I.; Rath, D. An Insight into Ultrasonic Vibration Assisted Conventional Manufacturing Processes: A Comprehensive Review. Adv. Mech. Eng. 2022, 14, 16878132221107812. [Google Scholar] [CrossRef]
- Sonia, P.; Jain, J.K.; Saxena, K.K. Influence of Ultrasonic Vibration Assistance in Manufacturing Processes: A Review. Mater. Manuf. Process. 2021, 36, 1451–1475. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, S.; Dong, G.; Su, J.; Qian, Z.; Zhou, J. Theoretical Study on Sawing Force of Ultrasonic Vibration Assisted Diamond Wire Sawing (UAWS) Based on Abrasives Wear. Wear 2022, 496–497, 204291. [Google Scholar] [CrossRef]
- Shen, J.; Zhu, X.; Chen, J.; Tao, P.; Wu, X. Investigation on the Edge Chipping in Ultrasonic Assisted Sawing of Monocrystalline Silicon. Micromachines 2019, 10, 616. [Google Scholar] [CrossRef] [PubMed]
- Yan, L.; Zhang, X.; Li, H.; Zhang, Q. Machinability Improvement in Three-Dimensional (3D) Ultrasonic Vibration Assisted Diamond Wire Sawing of SiC. Ceram. Int. 2022, 48, 8051–8068. [Google Scholar] [CrossRef]
- Wang, J.; Geng, Y.; Li, Z.; Yan, Y.; Luo, X.; Fan, P. Study on the Vertical Ultrasonic Vibration-Assisted Nanomachining Process on Single-Crystal Silicon. J. Manuf. Sci. Eng. 2021, 144, 41013. [Google Scholar] [CrossRef]
- Wang, L.; Gao, Y.; Li, X.; Pu, T.; Yin, Y. Analytical Prediction of Subsurface Microcrack Damage Depth in Diamond Wire Sawing Silicon Crystal. Mater. Sci. Semicond. Process. 2020, 112, 105015. [Google Scholar] [CrossRef]
- Wang, Y.; Li, D.-L.; Ding, Z.-J.; Liu, J.-G.; Wang, R. Modeling and Verifying of Sawing Force in Ultrasonic Vibration Assisted Diamond Wire Sawing (UAWS) Based on Impact Load. Int. J. Mech. Sci. 2019, 164, 105161. [Google Scholar] [CrossRef]
- Wang, W.; Liu, Z.X.; Zhang, W.; Huang, Y.H.; Allen, D.M. Abrasive Electrochemical Multi-Wire Slicing of Solar Silicon Ingots into Wafers. CIRP Ann. 2011, 60, 255–258. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, Y.; Ming, W.; Zhang, Y.; Cao, C.; Zhang, G. A Review on Magnetic Field Assisted Electrical Discharge Machining. J. Manuf. Process. 2021, 64, 694–722. [Google Scholar] [CrossRef]
- Ming, W.; Shen, F.; Zhang, G.; Liu, G.; Du, J.; Chen, Z. Green Machining: A Framework for Optimization of Cutting Parameters to Minimize Energy Consumption and Exhaust Emissions during Electrical Discharge Machining of Al 6061 and SKD 11. J. Clean. Prod. 2021, 285, 124889. [Google Scholar] [CrossRef]
- Ming, W.; Hou, J.; Zhang, Z.; Huang, H.; Xu, Z.; Zhang, G.; Huang, Y. Integrated ANN-LWPA for Cutting Parameter Optimization in WEDM. Int. J. Adv. Manuf. Technol. 2015, 84, 1277–1294. [Google Scholar] [CrossRef]
- Ming, W.; Xie, Z.; Ma, J.; Du, J.; Zhang, G.; Cao, C.; Zhang, Y. Critical Review on Sustainable Techniques in Electrical Discharge Machining. J. Manuf. Process. 2021, 72, 375–399. [Google Scholar] [CrossRef]
- Qiu, J.; Ge, R. High-Performance Electrical Discharge Sawing of Hard and Brittle Monocrystalline Silicon by Electroplated Diamond Wire. Int. J. Adv. Manuf. Technol. 2022, 120, 2031–2046. [Google Scholar] [CrossRef]
- Huang, H.; Zhang, Z.; Ming, W.; Xu, Z.; Zhang, Y. A Novel Numerical Predicting Method of Electric Discharge Machining Process Based on Specific Discharge Energy. Int. J. Adv. Manuf. Technol. 2017, 88, 409–424. [Google Scholar] [CrossRef]
- Ming, W.; Zhang, S.; Zhang, G.; Du, J.; Ma, J.; He, W.; Cao, C.; Liu, K. Progress in Modeling of Electrical Discharge Machining Process. Int. J. Heat. Mass. Transf. 2022, 187, 122563. [Google Scholar] [CrossRef]
- Wu, X.; Li, S. Experimental Investigations of a Hybrid Machining Combining Wire Electrical Discharge Machining (WEDM) and Fixed Abrasive Wire Saw. Int. J. Adv. Manuf. Technol. 2018, 95, 2613–2623. [Google Scholar] [CrossRef]
- Qiu, J.; Lv, J. Machining Accuracy and Force Characteristic of Diamond Wire Sawing and Diamond Wire Electrical Discharge Sawing during Rip Sawing and Cross Sawing. Int. J. Adv. Manuf. Technol. 2023, 126, 697–707. [Google Scholar] [CrossRef]
- Surface Flatness and Roughness of Diamond Wire Electrical Discharge Sawing Mono-Crystalline Silicon with Improved Bath Cooling SpringerLink. Available online: https://link.springer.com/article/10.1007/s00170-022-09155-7 (accessed on 19 June 2023).
- Wang, J.; Jia, Z.; Guo, Y.B. Shape-Cutting of Quartz Glass by Spark Discharge-Assisted Diamond Wire Sawing. J. Manuf. Process. 2018, 34, 131–139. [Google Scholar] [CrossRef]
- Wang, J.; Sun, L.; Jia, Z. Research on Electrochemical Discharge-Assisted Diamond Wire Cutting of Insulating Ceramics. Int. J. Adv. Manuf. Technol. 2017, 93, 3043–3051. [Google Scholar] [CrossRef]
- Aidil, A.R.M.; Minhat, M.; Hussein, N.I.S. Current Research Trends in Wire Electrical Discharge Machining (WEDM): A Review. J. Adv. Manuf. Technol. 2018, 12, 11–24. [Google Scholar]
- Ming, W.; Jia, H.; Zhang, H.; Zhang, Z.; Liu, K.; Du, J.; Shen, F.; Zhang, G. A Comprehensive Review of Electric Discharge Machining of Advanced Ceramics. Ceram. Int. 2020, 46, 21813–21838. [Google Scholar] [CrossRef]
- Sourav, P.S.; Singh, S.; Malik, A. A Review on Micro-Drilling by Electrochemical Discharge Machining. Eng. Res. Express 2023, 5, 022004. [Google Scholar] [CrossRef]
- Rakshit, R.; Das, A.K. A Review on Cutting of Industrial Ceramic Materials. Precis. Eng. 2019, 59, 90–109. [Google Scholar] [CrossRef]
- Wang, J.; Guo, Y.B.; Fu, C.; Jia, Z. Surface Integrity of Alumina Machined by Electrochemical Discharge Assisted Diamond Wire Sawing. J. Manuf. Process. 2018, 31, 96–102. [Google Scholar] [CrossRef]
- Wang, J.; Fu, C.; Jia, Z. Cutting of Hard and Brittle Insulating Materials Using Spark Discharge-Assisted Diamond Wire Sawing. J. Mater. Process. Technol. 2018, 252, 225–232. [Google Scholar] [CrossRef]
- Kuo, K.-Y.; Wu, K.-L.; Yang, C.-K.; Yan, B.-H. Wire Electrochemical Discharge Machining (WECDM) of Quartz Glass with Titrated Electrolyte Flow. Int. J. Mach. Tools Manuf. 2013, 72, 50–57. [Google Scholar] [CrossRef]
- Raugei, M.; Fthenakis, V. Cadmium Flows and Emissions from CdTe PV: Future Expectations. Energy Policy 2010, 38, 5223–5228. [Google Scholar] [CrossRef]
- Aghaei, M. A Review on Comparison between Traditional Silicon Solar Cells and Thin-Film CdTe Solar Cells. In Proceedings of the National Graduate Conference 2012 (NatGrad 2012), Tenaga Nasional University, Putrajaya, Malaysia, 7 November 2012. [Google Scholar]
- Petter Jelle, B.; Breivik, C.; Drolsum Røkenes, H. Building Integrated Photovoltaic Products: A State-of-the-Art Review and Future Research Opportunities. Sol. Energy Mater. Sol. Cells 2012, 100, 69–96. [Google Scholar] [CrossRef]
- Muteri, V.; Cellura, M.; Curto, D.; Franzitta, V.; Longo, S.; Mistretta, M.; Parisi, M.L. Review on Life Cycle Assessment of Solar Photovoltaic Panels. Energies 2020, 13, 252. [Google Scholar] [CrossRef]
- Sinha, B. Trends in Global Solar Photovoltaic Research: Silicon versus Non-Silicon Materials. Curr. Sci. 2011, 100, 654–660. [Google Scholar]
- Manuel, P.; Guizani, M. Broadcasting Algorithms of Carbon Nanotubes. J. Comput. Theor. Nanosci. 2011, 8, 554–562. [Google Scholar] [CrossRef]
- Choi, J.H.; Mao, Y.; Chang, J.P. Development of Hafnium Based High-k Materials—A Review. Mater. Sci. Eng. R: Rep. 2011, 72, 97–136. [Google Scholar] [CrossRef]
- Wang, P.; Zhang, Z.; Hao, B.; Wei, S.; Huang, Y.; Zhang, G. Investigation on Heat Transfer and Ablation Mechanism of CFRP by Different Laser Scanning Directions. Compos. Part. B Eng. 2023, 262, 110827. [Google Scholar] [CrossRef]
- Jiang, Z.; Li, L.; Huang, H.; He, W.; Ming, W. Progress in Laser Ablation and Biological Synthesis Processes: “Top-Down” and “Bottom-Up” Approaches for the Green Synthesis of Au/Ag Nanoparticles. Int. J. Mol. Sci. 2022, 23, 14658. [Google Scholar] [CrossRef]
- Ming, W.; Sun, P.; Zhang, Z.; Qiu, W.; Du, J.; Li, X.; Zhang, Y.; Zhang, G.; Liu, K.; Wang, Y.; et al. A Systematic Review of Machine Learning Methods Applied to Fuel Cells in Performance Evaluation, Durability Prediction, and Application Monitoring. Int. J. Hydrog. Energy 2023, 48, 5197–5228. [Google Scholar] [CrossRef]
- He, W.; Liu, T.; Han, Y.; Ming, W.; Du, J.; Liu, Y.; Yang, Y.; Wang, L.; Jiang, Z.; Wang, Y.; et al. A Review: The Detection of Cancer Cells in Histopathology Based on Machine Vision. Comput. Biol. Med. 2022, 146, 105636. [Google Scholar] [CrossRef]
- He, W.; Li, Z.; Liu, T.; Liu, Z.; Guo, X.; Du, J.; Li, X.; Sun, P.; Ming, W. Research Progress and Application of Deep Learning in Remaining Useful Life, State of Health and Battery Thermal Management of Lithium Batteries. J. Energy Storage 2023, 70, 107868. [Google Scholar] [CrossRef]
- Ming, W.; Shen, F.; Li, X.; Zhang, Z.; Du, J.; Chen, Z.; Cao, Y. A Comprehensive Review of Defect Detection in 3C Glass Components. Measurement 2020, 158, 107722. [Google Scholar] [CrossRef]
- Chavoshi, S.Z.; Goel, S.; Luo, X. Influence of Temperature on the Anisotropic Cutting Behaviour of Single Crystal Silicon: A Molecular Dynamics Simulation Investigation. J. Manuf. Process. 2016, 23, 201–210. [Google Scholar] [CrossRef]
- Zhao, P.; Pan, J.; Zhao, B.; Wu, J. Molecular Dynamics Study of Crystal Orientation Effect on Surface Generation Mechanism of Single-Crystal Silicon during the Nano-Grinding Process. J. Manuf. Process. 2022, 74, 190–200. [Google Scholar] [CrossRef]

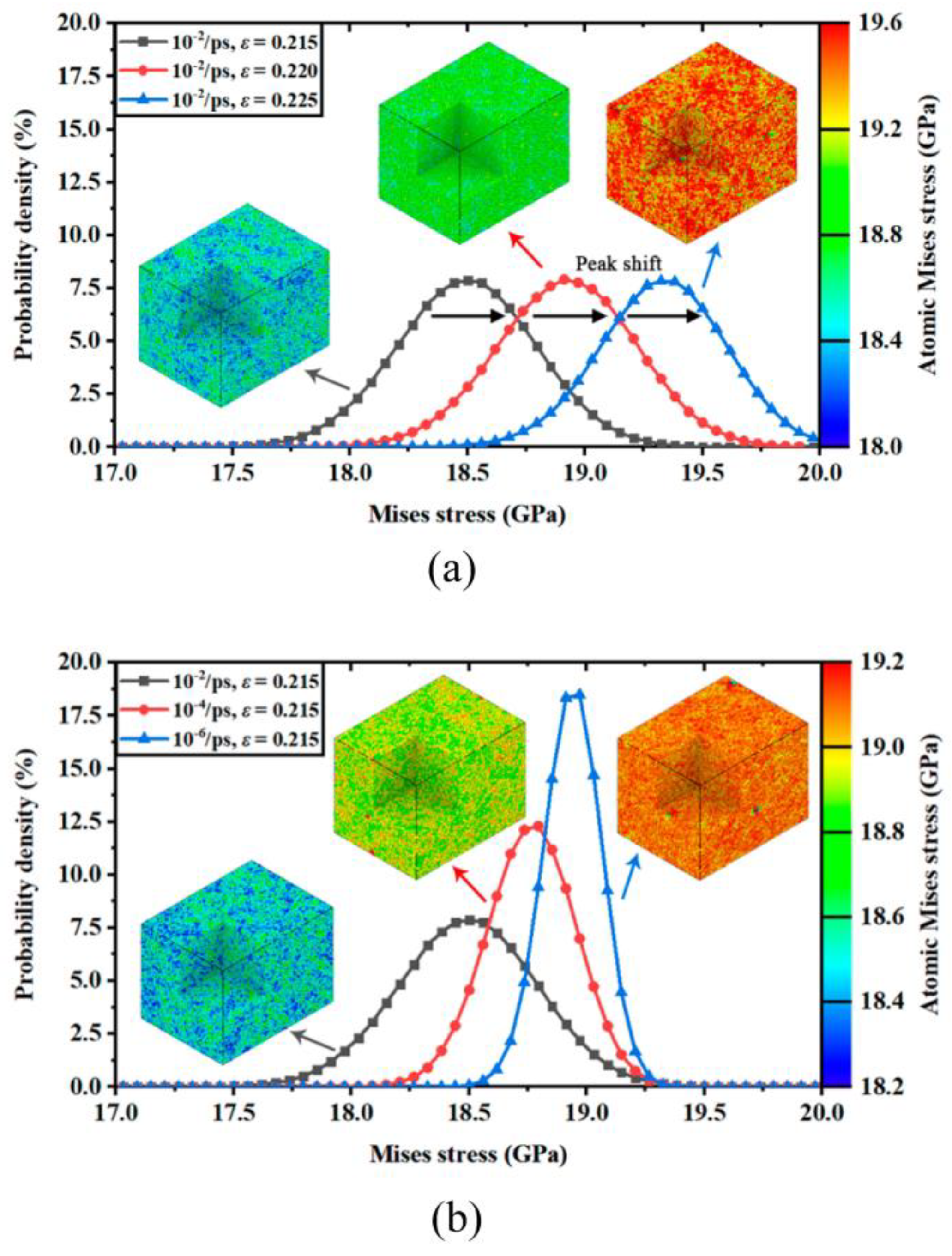



| Types of Models and Simulations | Authors, Year | Purpose | Findings | Remarks |
|---|---|---|---|---|
| Mathematical model | Li et al., 2019 [42] | Based on indentation fracture mechanics, a mathematical model of the influence of process parameters and wire saw parameters was developed. | The areas of brittle cracks produced by the abrasive can affect the surface morphology of the wafer. | Larger feed rates and line speeds increase the cutting efficiency and make it easier to obtain a surface of brittle excised material. |
| Wu et al., 2013 [43] | The effects of crystal defects on the cutting performance of polysilicon were investigated. | At the critical cutting depth of the ductile-brittle transition of the material, there was a significant variation within the particles. | A higher dislocation density is associated with greater fracture toughness and larger critical depth of cut. | |
| Yin et al., 2021 [44] | A mathematical model of DWS was established, and the sawing process was numerically calculated. | The critical ratio of the workpiece feed speed to the saw wire motion speed was obtained with a combination of different parameters. | Increasing the speed of the saw wire movement or decreasing the feed speed of the workpiece is more beneficial to achieving material removal. | |
| MD model | Liu et al., 2022 [74] | The atomic structures of orthocrystalline silicon crystals and silicon nanowires were compared. | Strain rate sensitivities and critical strain rates were obtained for both structures using a rate reactivity model. | A calculation of both rates revealed that the additional surface of THE SiNW reduced the sensitivity of the strain rate. |
| Olufayo et al., 2013 [89] | MD simulation for the atomic visualization of plastic material flow at the tool-workpiece interface during orthogonal cutting. | The simulated MD force and temperature outputs were evaluated to obtain the accuracy of the model. | The MD method can be used to study the atomic reactions on the tool/workpiece surface, revealing the ductile transition response of the nanoprocess. | |
| Dai et al., 2017 [90] | MD simulation of the cutting of monocrystalline silicon with laser-fabricated, nanostructured diamond tools. | The effects of different trench orientations, depths, widths, factors, and shapes on the nanoscale cutting process were investigated. | Groove orientation has a significant effect on the nanoscale cutting process, and cutting with V-shaped grooves can improve material removal. | |
| FEM | Wei et al., 2018 [52] | The thickness and stress strength factors of monocrystalline silicon, as well as the crack extension angle, were studied via MD simulation and FEM, respectively. | The thickness and stress strength factors, as well as the crack extension angle, were obtained via MD simulation and FEM, respectively. | The critical stress strength factors and crack extension angles are clearly dependent on the chiral angle, thickness, and loading angle of the monocrystalline silicon plate. |
| Zhang et al., 2014 [53] | Anisotropic effects in silicon were evaluated using stiffness and flexibility coefficient matrixes. | Proper crystal orientation can improve performance and reduce mechanical bending stress. | For monocrystalline silicon, heat deformation can be approximated by using the isotropic constant Poisson’s ratio. | |
| Skalka et al., 2021 [91] | An FE simulation and optimization procedures were used to determine the cohesive energy density of monocrystalline silicon. | The adhesion energy density was evaluated and the material toughness was determined. | The reliability of the model originates from the comparison of the numerical simulation results with the measured data. |
| Parameters | Authors, Year | Purpose | Findings | Remarks |
|---|---|---|---|---|
| Tension | Albrecht and Möhr-ing, 2018 [107] | The effect on the stability of the sawing process was investigated experimentally and by simulation. | At higher tensions (350 MPa and 400 MPa), saw blade displacement remained essentially the same, while higher tensions resulted in reduced displacement. | Adjusting the saw blade parameter tension during the cutting process does not affect the processing time. |
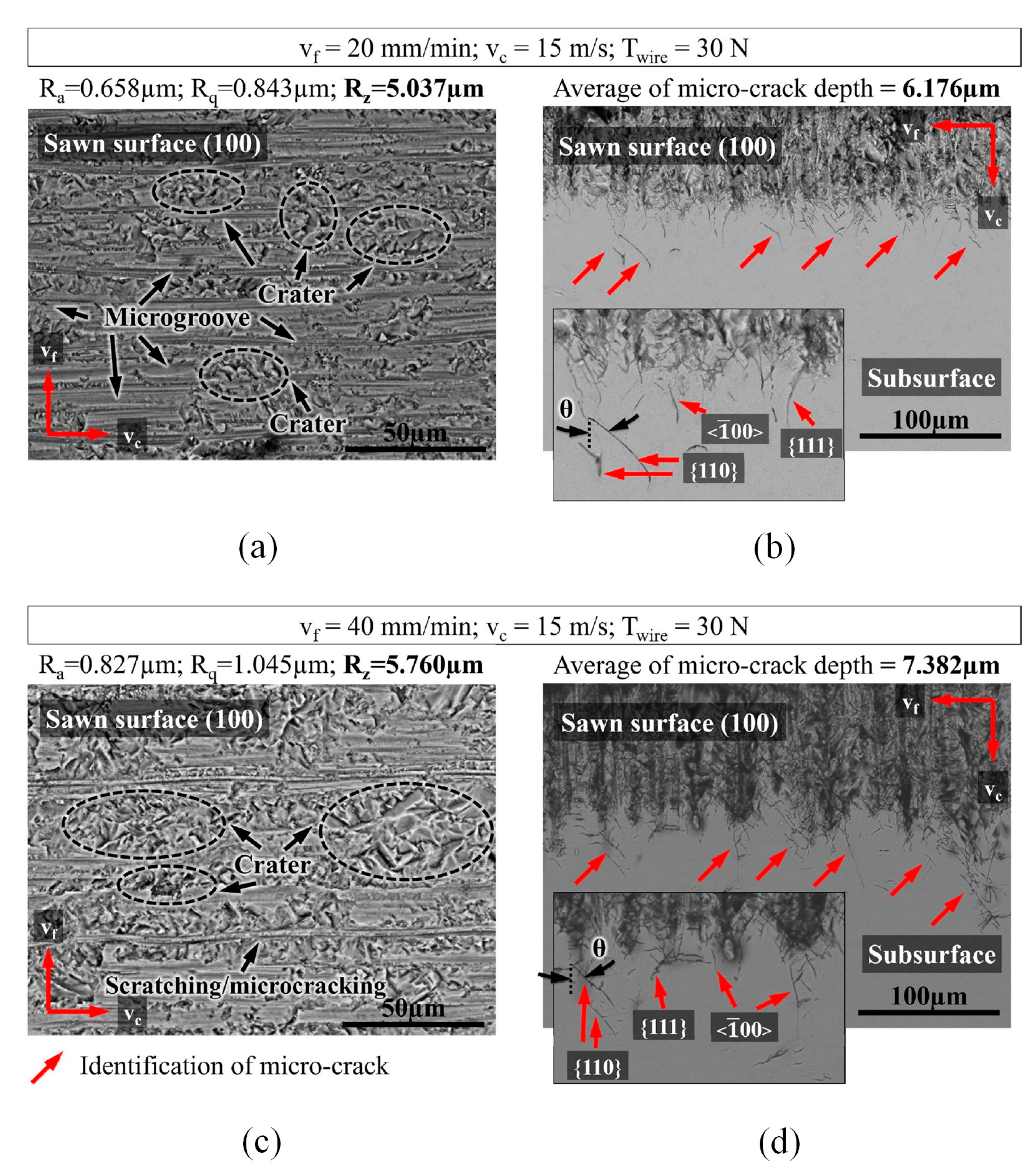
| Cutting speed, feed rate, and wire tension | Costa et al., 2020 [108] | To investigate the effect of DWS on the surface integrity of monocrystalline silicon. | For two wire tensions (Twire) = 30 N, the Sa value increased significantly when compared with the specimens sawn using Twire = 20 N. | The most suitable set of cutting parameters is the lowest feed rate and wire tension and the highest wire cutting speed. |
| Stiffness of wire web, tension, fluctuation of wire, and reciprocating period | Qiu et al., 2021 [109] | To study the factors affecting the machining accuracy of circular diamond rope saws and their mechanisms. | The roughness value of endless wire sawing was Ra = 1.6 µm and that of reciprocating sawing was Ra = 1.254 µm. | Stable tension corresponds to better machining accuracy. |
| Wire speed, feed rate, rocking angle, preload force, and guide roller distance | Lai et al., 2023 [110] | To analyze the effect of machining parameters on sawing force, contact length, and MRR. | Workpiece rocking reduces contact length, with a maximum contact length of about 20% of the workpiece diameter during sawing. | Feed speed, maximum wire feed speed, maximum swing angle and preload force all affect the range of MRR fluctuations. |
| Reciprocating period and sawing arc length | Dong et al., 2021 [111] | A reciprocating oscillating motion pattern was introduced in a cutting frame saw to study the cutting performance of sawing. | The depth of the cut and the distribution of the sawing force depend on the position of the saw blade on the saw surface. | The effect of sawing conditions on sawing force is related to the depth of cut of the cutter head. |
| Types of Hybrid Machining | Authors, Year | Purpose | Findings | Remarks |
|---|---|---|---|---|
| UV-DWS | Wang et al., 2022 [156] | Conducting theoretical research on the cutting force of UV-DWS based on abrasive wear. | A theoretical model of UV-DWS force from single to multiple abrasive grains was developed. | Compared with DWS, UV-DWS can reduce the sawing force and improve the flatness of the workpiece. |
| Wang et al., 2023 [140] | UV-DWS of monocrystalline silicon SSD. | A mathematical model of UV-DWS damage to silicon wafers was developed, and the law of SSD was analyzed. | The UV-DWS monocrystalline wire silicon model verifies that the SSD varies with different sawing parameters. | |
| Wang et al., 2019 [161] | Modeling and validation of UV-DWS cutting force based on impact loading. | The validity of the impact loading was demonstrated using the UV-DWS. | The surface quality of UV-DWS is better than that of DWS. | |
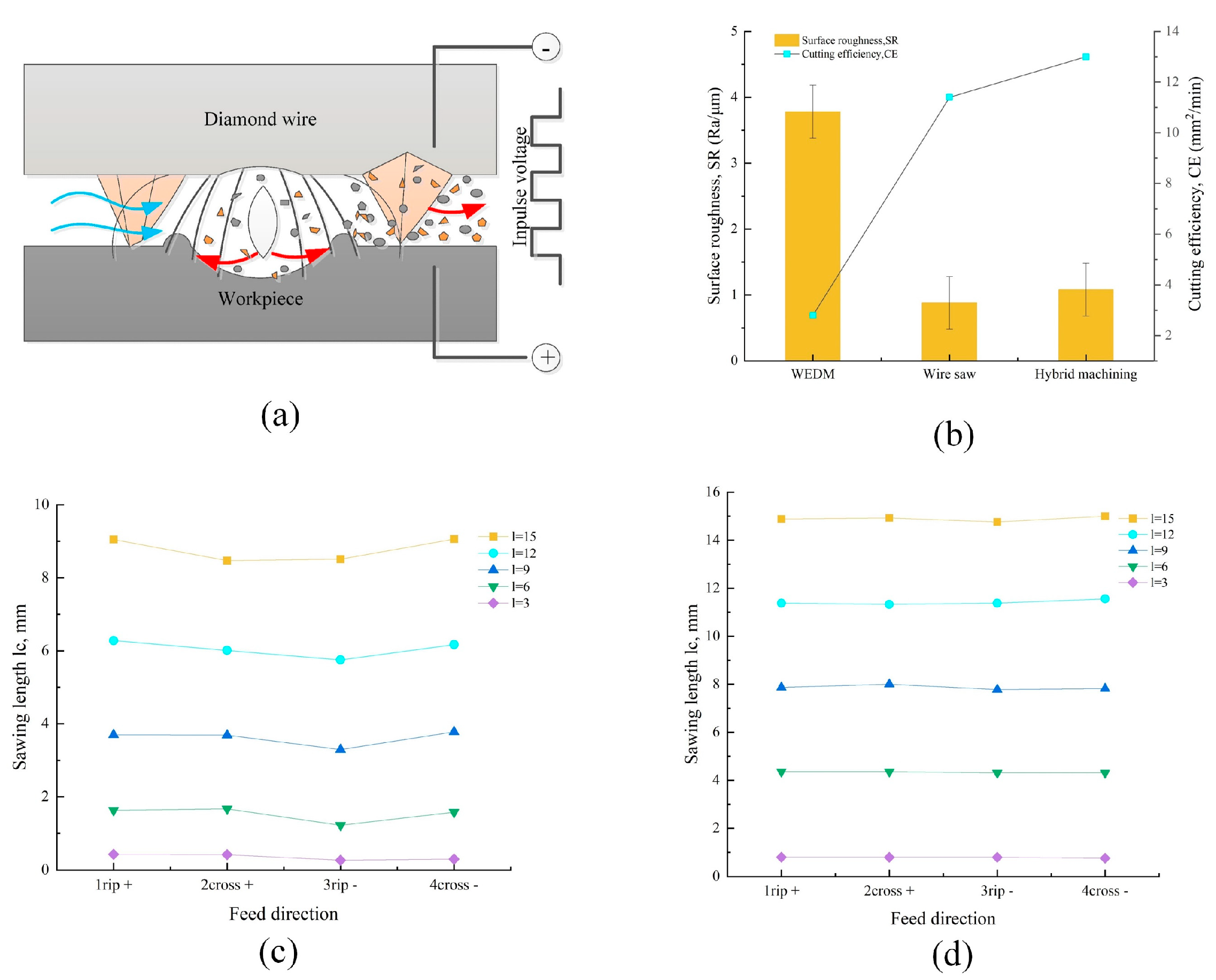
| ED-DWS | Wu et al., 2018 [170] | A pilot study of EDM wire cutting and fixed abrasive wire saw compound machining was conducted. | A composite machining method combining EDM wire cutting and fixed abrasive DWS together was studied. | Compared with fixed abrasive DWS, the hybrid processing method reduces silicon surface scratches. |
| Qiu et al., 2023 [171] | The machining accuracy of DWS and ED-DWS in longitudinal and transverse sawing was compared. | Better machining accuracy and surface quality are achieved with ED-DWS under bath cooling than under jet cooling. | ED-DWS outperforms DWS in terms of machining accuracy and cutting efficiency. | |
| Qiu et al., 2023 [172] | An environmentally improved method of ED-DWS under plating solution cooling conditions was proposed. | Its advantages were compared with those of jet cooling through a series of sawing tests. | The roughness of bath cooling is better than jet cooling, but the fluidity becomes worse and chip removal becomes difficult. | |
| EC-DWS | Wang et al., 2017 [179] | Electrochemical discharge-assisted DWS cutting of hard and brittle materials for surface integrity. | Based on the experimental results, each element of the machined surface was analyzed. | The combination of electrochemical discharge and DWS can improve surface roughness. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, A.; Hu, S.; Zhou, Y.; Wang, H.; Zhang, Z.; Ming, W. Recent Advances in Precision Diamond Wire Sawing Monocrystalline Silicon. Micromachines 2023, 14, 1512. https://doi.org/10.3390/mi14081512
Li A, Hu S, Zhou Y, Wang H, Zhang Z, Ming W. Recent Advances in Precision Diamond Wire Sawing Monocrystalline Silicon. Micromachines. 2023; 14(8):1512. https://doi.org/10.3390/mi14081512
Chicago/Turabian StyleLi, Ansheng, Shunchang Hu, Yu Zhou, Hongyan Wang, Zhen Zhang, and Wuyi Ming. 2023. "Recent Advances in Precision Diamond Wire Sawing Monocrystalline Silicon" Micromachines 14, no. 8: 1512. https://doi.org/10.3390/mi14081512
APA StyleLi, A., Hu, S., Zhou, Y., Wang, H., Zhang, Z., & Ming, W. (2023). Recent Advances in Precision Diamond Wire Sawing Monocrystalline Silicon. Micromachines, 14(8), 1512. https://doi.org/10.3390/mi14081512