Diffractive Achromat with Freeform Slope for Broadband Imaging over a Long Focal Depth
Abstract
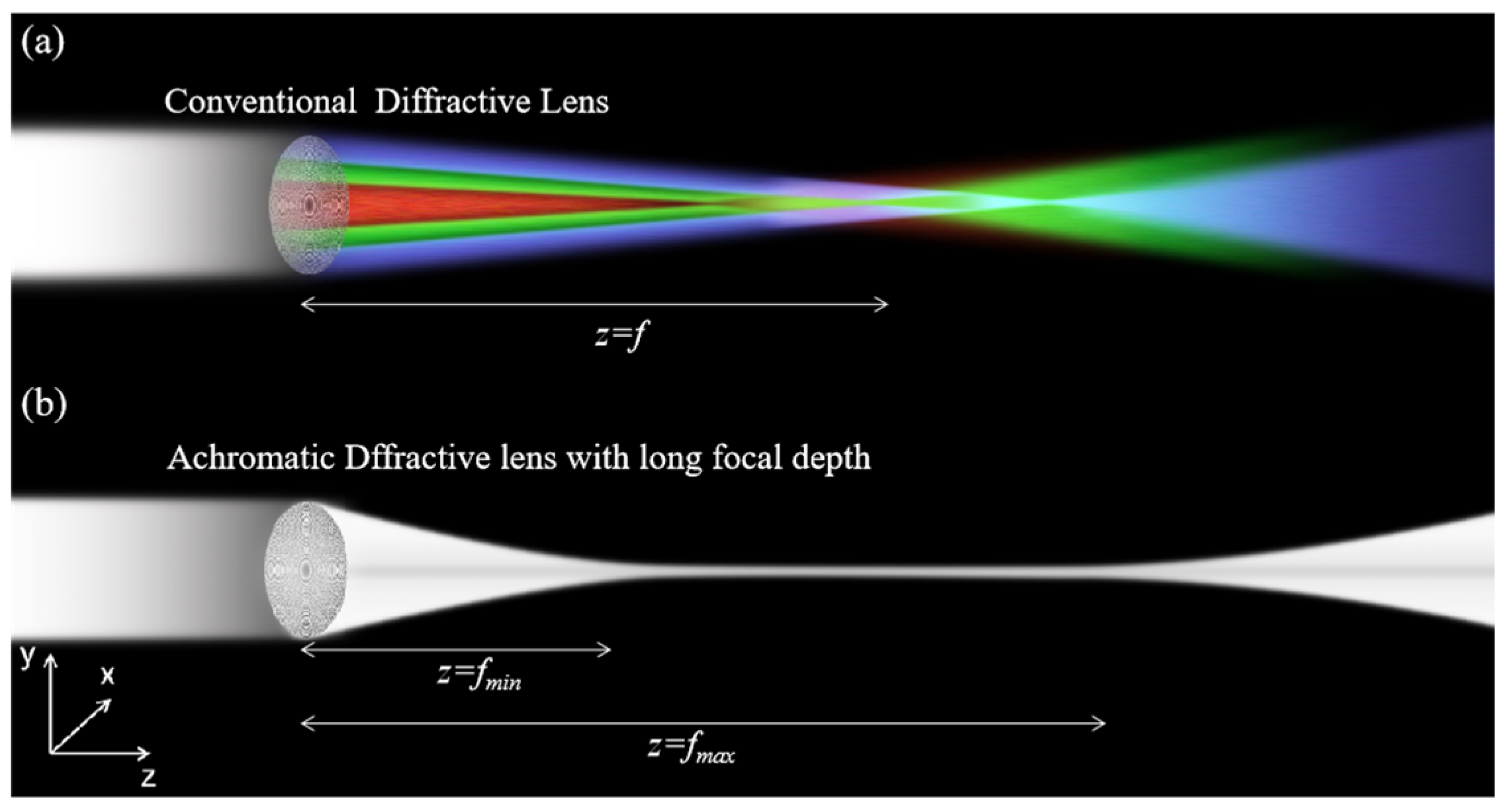
1. Introduction
2. Materials and Methods
2.1. Design
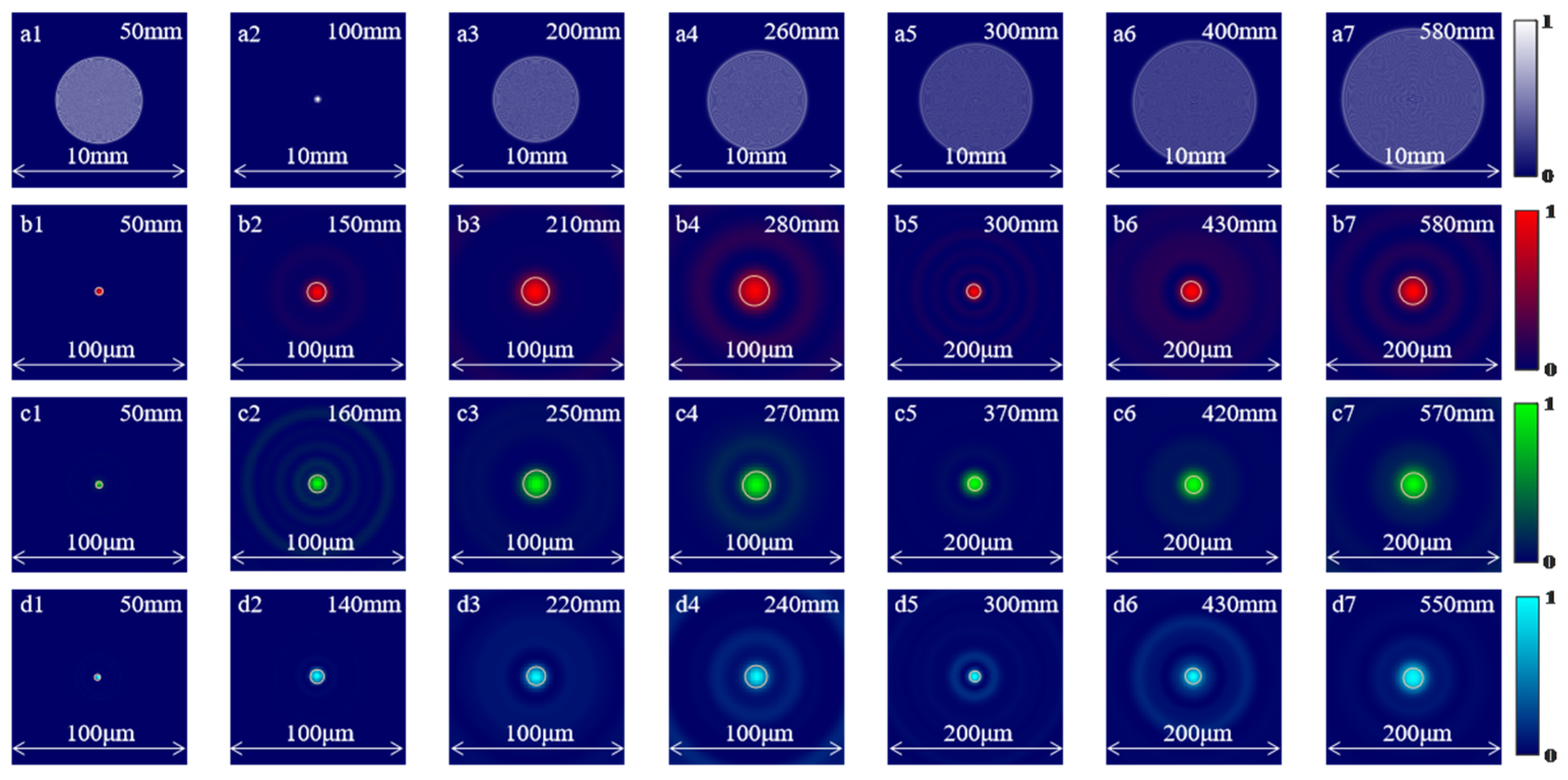
2.2. Simulation Results
3. Results
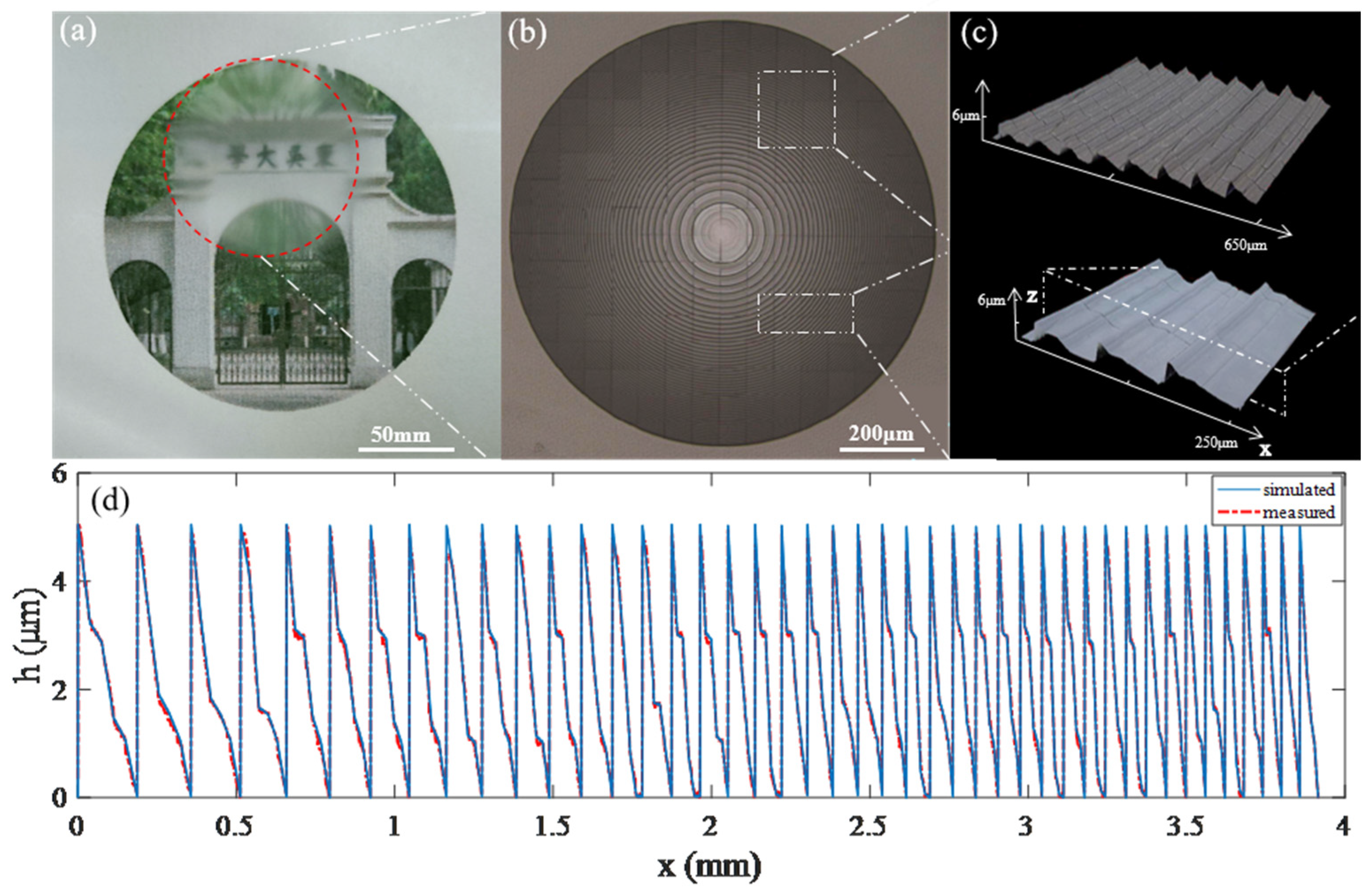
3.1. Fabrication of Long-Focal-Depth Achromatic Diffractive Lens
3.2. The Point Spread Function of Long-Focal-Depth Achromatic Diffractive Lens
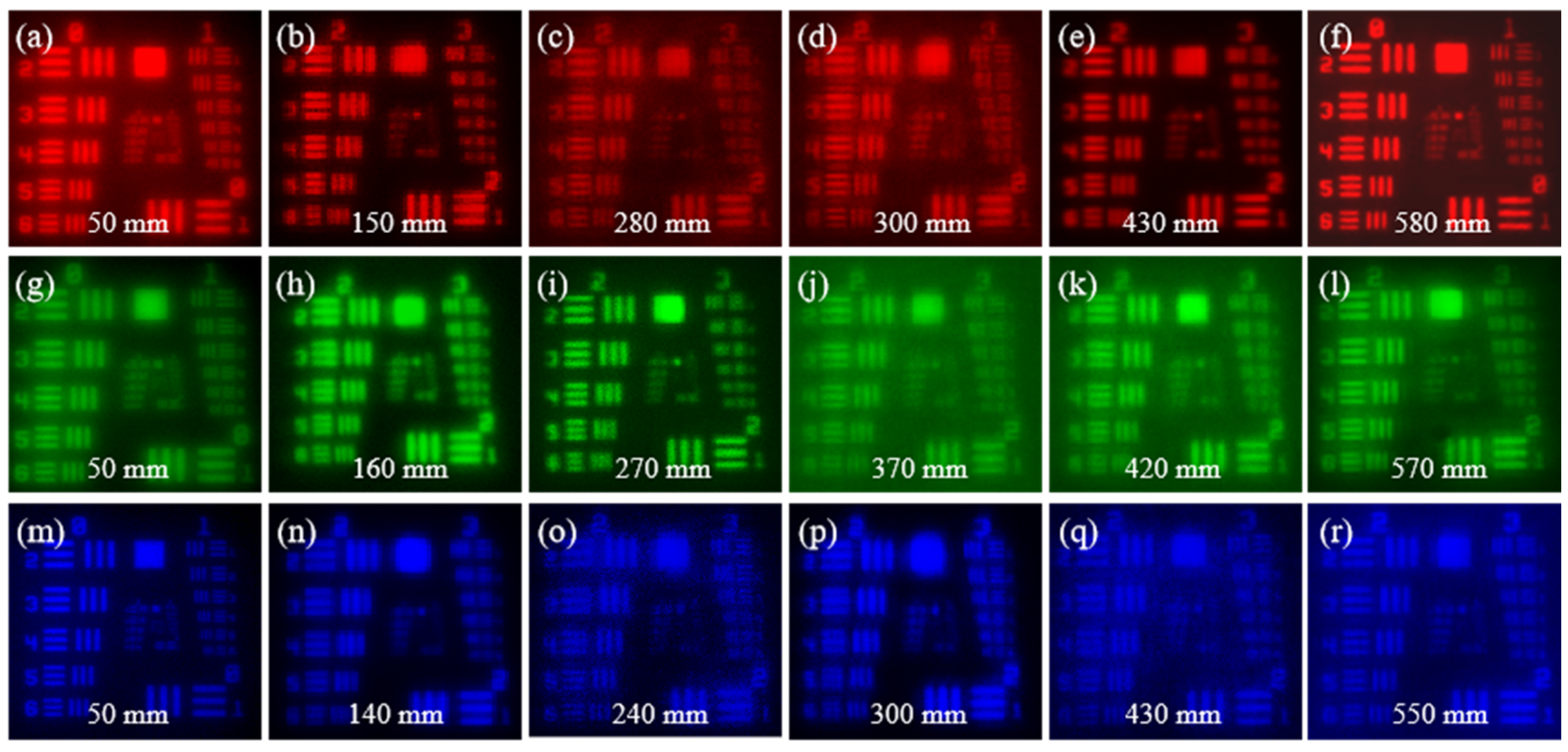
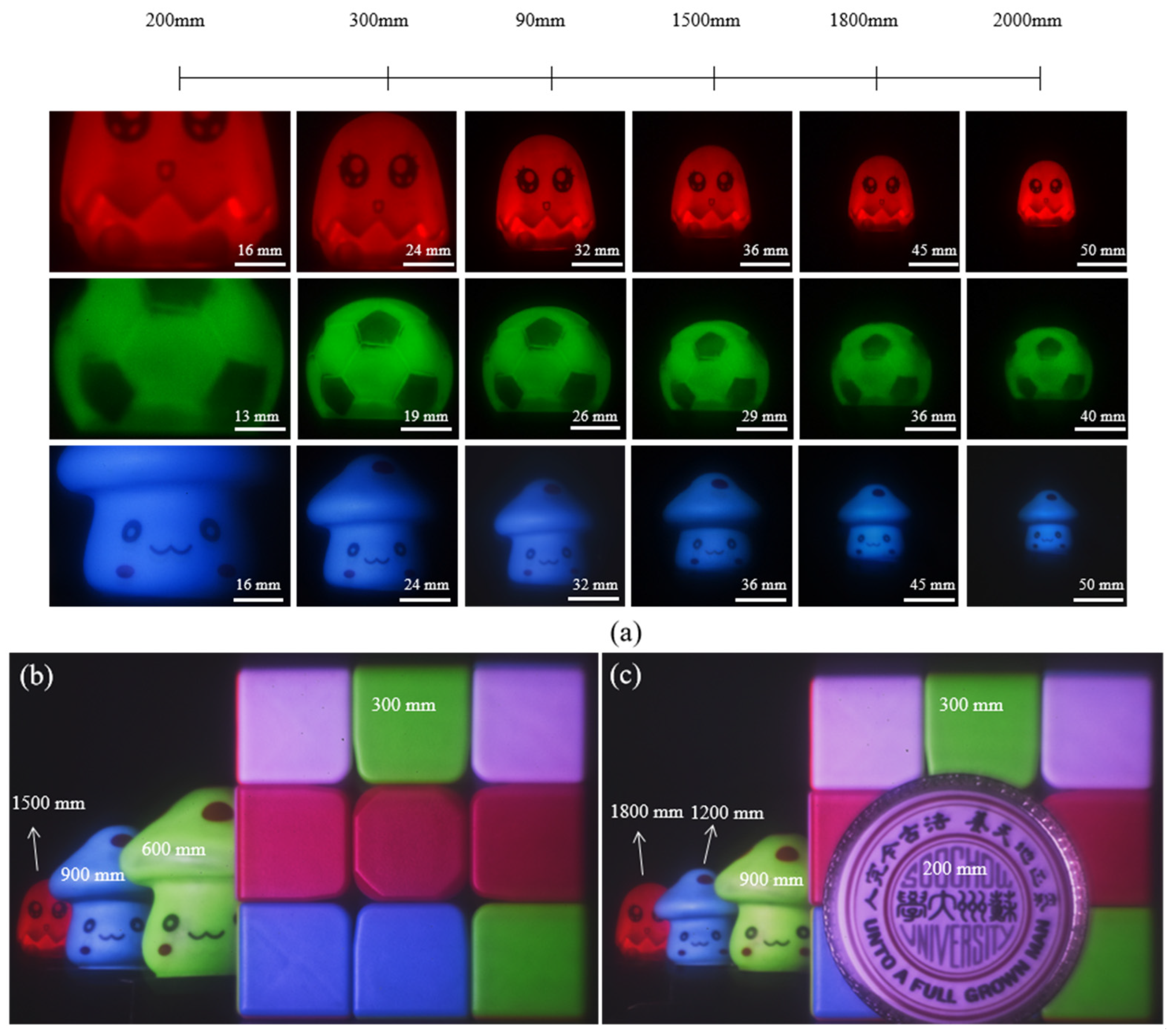
3.3. Characterization of the Long-Focal-Depth Achromatic Diffractive Lens
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Born, M.; Wolf, E. Principle of Optics, 7th ed.; Cambridge University Press: Cambridge, MA, USA, 1999. [Google Scholar] [CrossRef]
- Bradburn, S.; Cathey, W.T.; Dowski, E.R. Realizations of focus invariance in optical–digital systems with wave-front coding. Appl. Opt. 1997, 36, 9157–9166. [Google Scholar] [CrossRef]
- Ilovitsh, A.; Zalevsky, Z. Time multiplexing based extended depth of focus imaging. Opt. Lett. 2015, 41, 183–186. [Google Scholar] [CrossRef]
- Kim, J.; Xing, J.; Nam, H.S.; Song, J.W.; Kim, J.W.; Yoo, H. Endoscopic micro-optical coherence tomography with extended depth of focus using a binary phase spatial filter. Opt. Lett. 2017, 42, 379–382. [Google Scholar] [CrossRef] [PubMed]
- Zhou, F.; Hua, J.; Shi, J.; Qiao, W.; Chen, L. Pixelated Blazed Gratings for High Brightness Multiview Holographic 3D Display. IEEE Photon-Technol. Lett. 2020, 32, 283–286. [Google Scholar] [CrossRef]
- Teng, D.; Xiong, Y.; Liu, L.; Wang, B. Multiview three-dimensional display with continuous motion parallax through planar aligned OLED microdisplays. Opt. Express 2015, 23, 6007–6019. [Google Scholar] [CrossRef] [PubMed]
- Li, K.; Yöntem, A.; Deng, Y.; Shrestha, P.; Chu, D.; Zhou, J.; Yao, J. Full resolution auto-stereoscopic mobile display based on large scale uniform switchable liquid crystal micro-lens array. Opt. Express 2017, 25, 9654–9675. [Google Scholar] [CrossRef]
- McLeod, J.H. The Axicon: A New Type of Optical Element. J. Opt. Soc. Am. 1954, 44, 592–597. [Google Scholar] [CrossRef]
- McLeod, J.H. Axicons and Their Uses. J. Opt. Soc. Am. 1960, 50, 166–169. [Google Scholar] [CrossRef]
- Dowski, E.R.; Cathey, W.T. Extended depth of field through wave-front coding. Appl. Opt. 1995, 34, 1859–1866. [Google Scholar] [CrossRef]
- Wach, H.B.; Dowski, E.R.; Cathey, W.T. Control of chromatic focal shift through wave-front coding. Appl. Opt. 1998, 37, 5359–5367. [Google Scholar] [CrossRef]
- Ojeda-Castaneda, J.; Tepichin, E.; Diaz, A. Arbitrarily high focal depth with a quasioptimum real and positive transmittance apodizer. Appl. Opt. 1989, 28, 2666–2670. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Yang, Q.; Gong, M.; Chen, M.; Long, Z. Metasurface lens with angular modulation for extended depth of focus imaging. Opt. Lett. 2020, 45, 611–614. [Google Scholar] [CrossRef]
- Arbabi, A.; Horie, Y.; Ball, A.J.; Bagheri, M.; Faraon, A. Subwavelength-thick lenses with high numerical apertures and large efficiency based on high-contrast transmitarrays. Nat. Commun. 2015, 6, 7069. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Ma, D.; Li, Z.; Cheng, H.; Choi, D.-Y.; Tian, J.-G.; Chen, S. Aberration-corrected three-dimensional positioning with a single-shot metalens array. Optica 2020, 7, 1706–1713. [Google Scholar] [CrossRef]
- Presutti, F.; Monticone, F. Focusing on bandwidth: Achromatic metalens limits. Optica 2020, 7, 624–631. [Google Scholar] [CrossRef]
- Chen, W.T.; Zhu, A.Y.; Sanjeev, V.; Khorasaninejad, M.; Shi, Z.; Lee, E.; Capasso, F. A broadband achromatic metalens for focusing and imaging in the visible. Nat. Nanotechnol. 2018, 13, 220–226. [Google Scholar] [CrossRef]
- Shen, K.; Duan, Y.; Ju, P.; Xu, Z.; Chen, X.; Zhang, L.; Ahn, J.; Ni, X.; Li, T. On-chip optical levitation with a metalens in vacuum. Optica 2021, 8, 1359. [Google Scholar] [CrossRef]
- Liang, Y.; Liu, H.; Wang, F.; Meng, H.; Guo, J.; Li, J.; Wei, Z. High-Efficiency, Near-Diffraction Limited, Dielectric Metasurface Lenses Based on Crystalline Titanium Dioxide at Visible Wavelengths. Nanomaterials 2018, 8, 288. [Google Scholar] [CrossRef]
- Lalanne, P.; Chavel, P. Metalenses at visible wavelengths: Past, present, perspectives. Laser Photon-Rev. 2017, 11, 1600295. [Google Scholar] [CrossRef]
- Banerji, S.; Meem, M.; Majumder, A.; Vasquez, F.G.; Sensale-Rodriguez, B.; Menon, R. Imaging with flat optics: Metalenses or diffractive lenses? Optica 2019, 6, 805–810. [Google Scholar] [CrossRef]
- Huang, K.; Qin, F.; Liu, H.; Ye, H.; Qiu, C.-W.; Hong, M.; Luk’Yanchuk, B.; Teng, J. Planar Diffractive Lenses: Fundamentals, Functionalities, and Applications. Adv. Mater. 2018, 30, e1704556. [Google Scholar] [CrossRef] [PubMed]
- Xu, N.; Kong, Z.; Tan, Q.; Fu, Y. Multiring pure-phase binary optical elements to extend depth of focus. Appl. Opt. 2018, 57, 9643–9648. [Google Scholar] [CrossRef] [PubMed]
- Veli, M.; Mengu, D.; Yardimci, N.T.; Luo, Y.; Li, J.; Rivenson, Y.; Jarrahi, M.; Ozcan, A. Terahertz pulse shaping using diffractive surfaces. Nat. Commun. 2021, 12, 37. [Google Scholar] [CrossRef] [PubMed]
- Seldowitz, M.A.; Allebach, J.P.; Sweeney, D.W. Synthesis of digital holograms by direct binary search. Appl. Opt. 1987, 26, 2788–2798. [Google Scholar] [CrossRef]
- Sales, T.R.M.; Raguin, D.H. Multiwavelength operation with thin diffractive elements. Appl. Opt. 1999, 38, 3012–3018. [Google Scholar] [CrossRef]
- Kim, G.; Domínguez-Caballero, J.A.; Menon, R. Design and analysis of multi-wavelength diffractive optics. Opt. Express 2012, 20, 2814–2823. [Google Scholar] [CrossRef]
- Banerji, S.; Meem, M.; Majumder, A.; Sensale-Rodriguez, B.; Menon, R. Extreme-depth-of-focus imaging with a flat lens. Optica 2020, 7, 214–217. [Google Scholar] [CrossRef]
- Mohammad, N.; Meem, M.; Shen, B.; Wang, P.; Menon, R. Broadband imaging with one planar diffractive lens. Sci. Rep. 2018, 8, 2799. [Google Scholar] [CrossRef]
- Wang, P.; Mohammad, N.; Menon, R. Chromatic-aberration-corrected diffractive lenses for ultra-broadband focusing. Sci. Rep. 2016, 6, 21545. [Google Scholar] [CrossRef]
- Khorasaninejad, M.; Zhu, A.Y.; Roques-Carmes, C.; Chen, W.T.; Oh, J.; Mishra, I.; Devlin, R.C.; Capasso, F. Polarization-Insensitive Metalenses at Visible Wavelengths. Nano Lett. 2016, 16, 7229–7234. [Google Scholar] [CrossRef]
- Qiao, W.; Huang, W.; Liu, Y.; Li, X.; Chen, L.-S.; Tang, J.-X. Toward Scalable Flexible Nanomanufacturing for Photonic Structures and Devices. Adv. Mater. 2016, 28, 10353–10380. [Google Scholar] [CrossRef]
- Shi, J.; Qiao, W.; Hua, J.; Li, R.; Chen, L. Spatial multiplexing holographic combiner for glasses-free augmented reality. Nanophotonics 2020, 9, 243. [Google Scholar] [CrossRef]
- Wan, W.; Qiao, W.; Pu, D.; Li, R.; Wang, C.; Hu, Y.; Duan, H.; Guo, L.J.; Chen, L. Holographic Sampling Display Based on Metagratings. iScience 2020, 23, 100773. [Google Scholar] [CrossRef]
- Hua, J.; Hua, E.; Zhou, F.; Shi, J.; Wang, C.; Duan, H.; Hu, Y.; Qiao, W.; Chen, L. Foveated glasses-free 3D display with ultrawide field of view via a large-scale 2D-metagrating complex. Light Sci. Appl. 2021, 10, 213. [Google Scholar] [CrossRef]
- Wu, X.; Qiao, W.; Zhu, M.; Ren, J.; Pu, D.; Chen, L. Roll-to-plate additive manufacturing. Opt. Express 2021, 29, 21833–21843. [Google Scholar] [CrossRef]
- Iemmi, C.; Campos, J.; Escalera, J.C.; López-Coronado, O.; Gimeno, R.; Yzuel, M.J. Depth of focus increase by multiplexing programmable diffractive lenses. Opt. Express 2006, 14, 10207–10219. [Google Scholar] [CrossRef]
- Peng, Y.; Fu, Q.; Amata, H.; Su, S.; Heide, F.; Heidrich, W. Computational imaging using lightweight diffractive-refractive optics. Opt. Express 2015, 23, 31393–31407. [Google Scholar] [CrossRef] [PubMed]
- Nikonorov, A.V.; Petrov, M.V.; Bibikov, S.A.; Yakimov, P.Y.; Kutikova, V.V.; Yuzifovich, Y.V.; Morozov, A.A.; Skidanov, R.V.; Kazanskiy, N.L. Toward Ultralightweight Remote Sensing with Harmonic Lenses and Convolutional Neural Networks. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2018, 11, 3338–3348. [Google Scholar] [CrossRef]
- Dun, X.; Ikoma, H.; Wetzstein, G.; Wang, Z.; Cheng, X.; Peng, Y. Learned rotationally symmetric diffractive achromat for full-spectrum computational imaging. Optica 2020, 7, 913–922. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yi, D.; Zhou, F.; Hua, J.; Chen, L.; Qiao, W. Diffractive Achromat with Freeform Slope for Broadband Imaging over a Long Focal Depth. Micromachines 2023, 14, 1401. https://doi.org/10.3390/mi14071401
Yi D, Zhou F, Hua J, Chen L, Qiao W. Diffractive Achromat with Freeform Slope for Broadband Imaging over a Long Focal Depth. Micromachines. 2023; 14(7):1401. https://doi.org/10.3390/mi14071401
Chicago/Turabian StyleYi, Donghui, Fengbin Zhou, Jianyu Hua, Linsen Chen, and Wen Qiao. 2023. "Diffractive Achromat with Freeform Slope for Broadband Imaging over a Long Focal Depth" Micromachines 14, no. 7: 1401. https://doi.org/10.3390/mi14071401
APA StyleYi, D., Zhou, F., Hua, J., Chen, L., & Qiao, W. (2023). Diffractive Achromat with Freeform Slope for Broadband Imaging over a Long Focal Depth. Micromachines, 14(7), 1401. https://doi.org/10.3390/mi14071401






