Exploring the Potential of Flow-Induced Vibration Energy Harvesting Using a Corrugated Hyperstructure Bluff Body
Abstract
1. Introduction
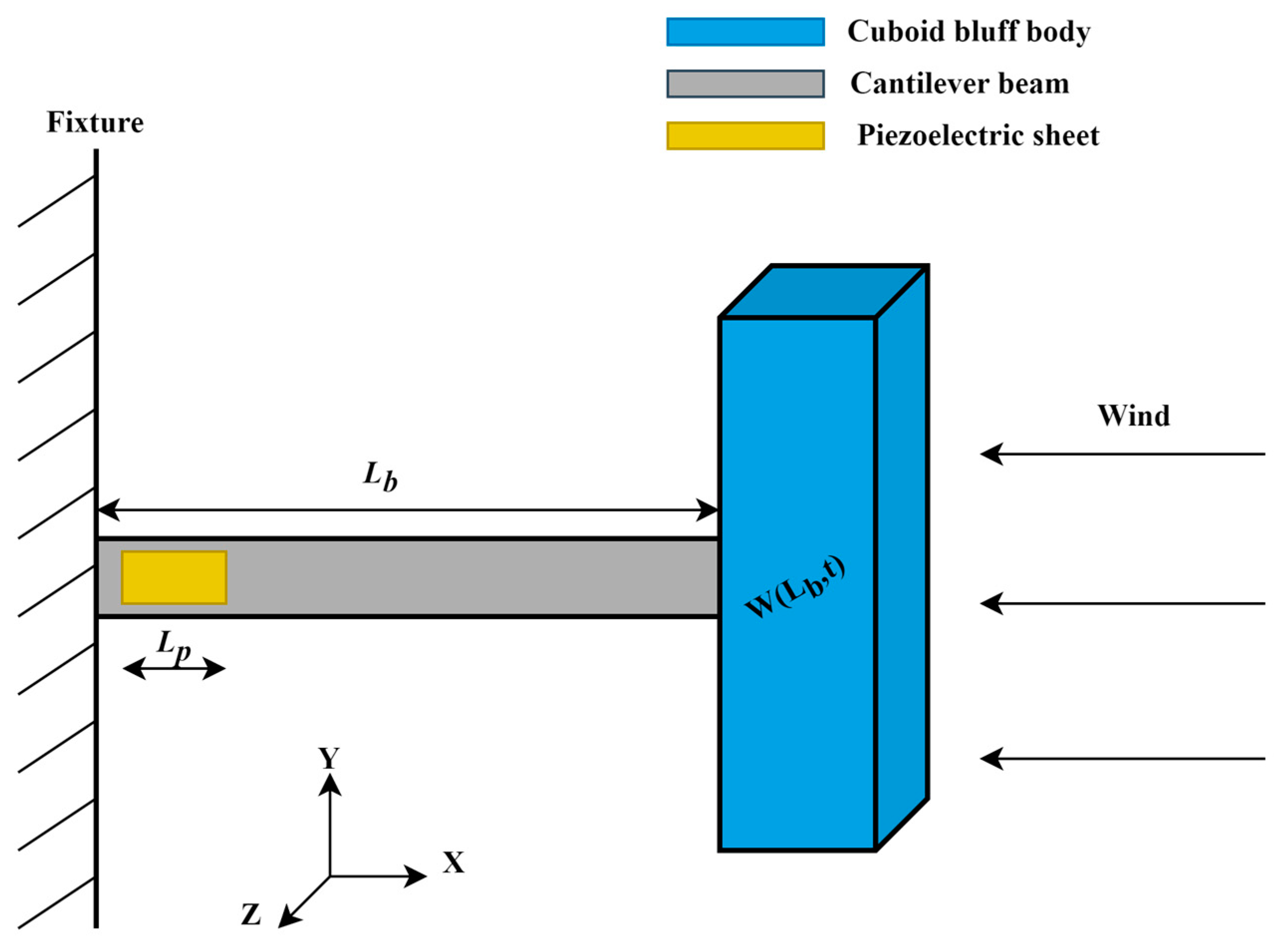
2. Flow-Induced Vibration Harvester Based on a Corrugated Hyperstructure Bluff Body
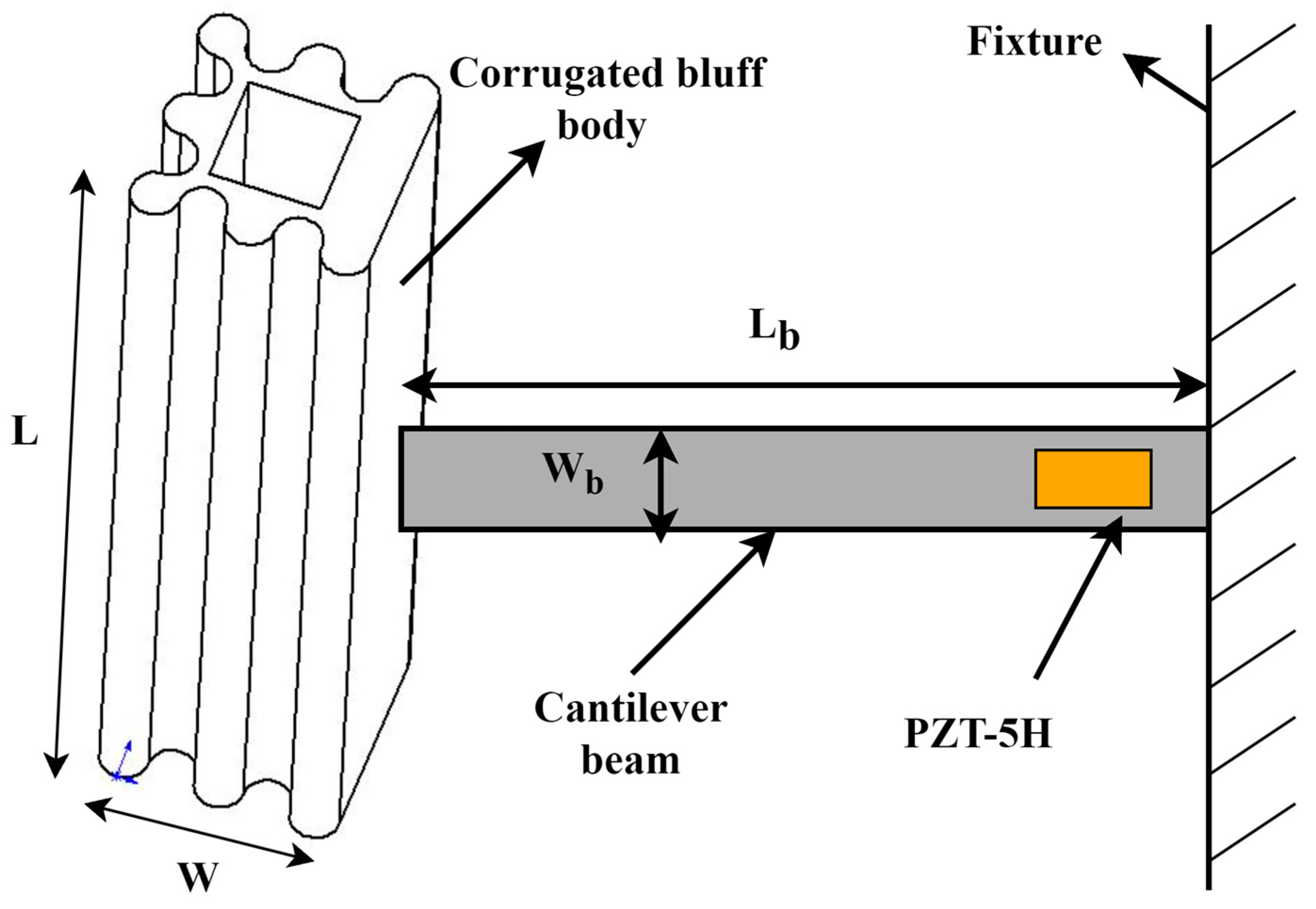
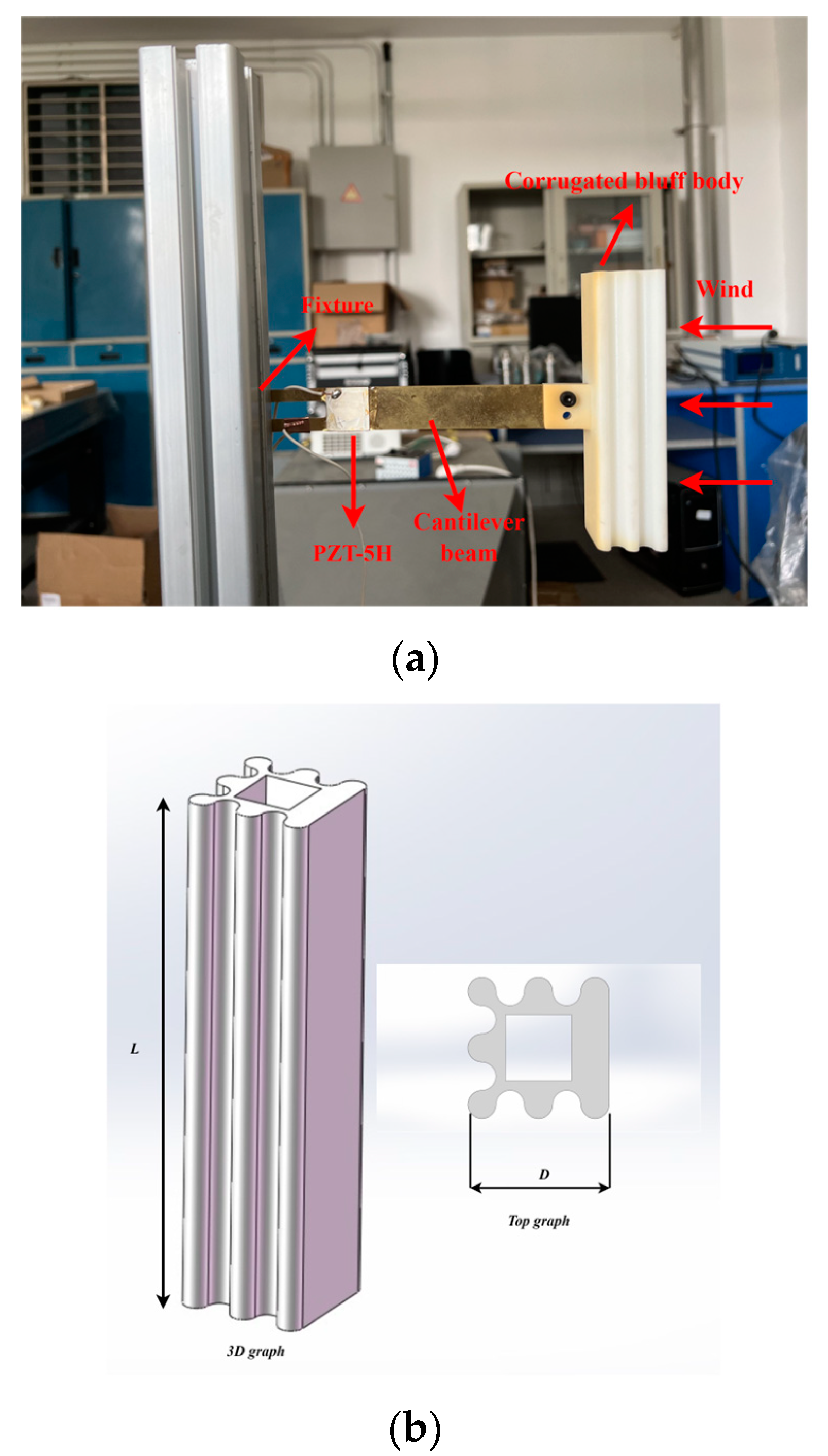
2.1. Structure Design
2.2. Mathematical Model of the Cantilever in the GPEH with a Corrugated Bluff Body
3. CFD Modeling of the Corrugated Bluff Body
3.1. Structure of the Corrugated Bluff Body
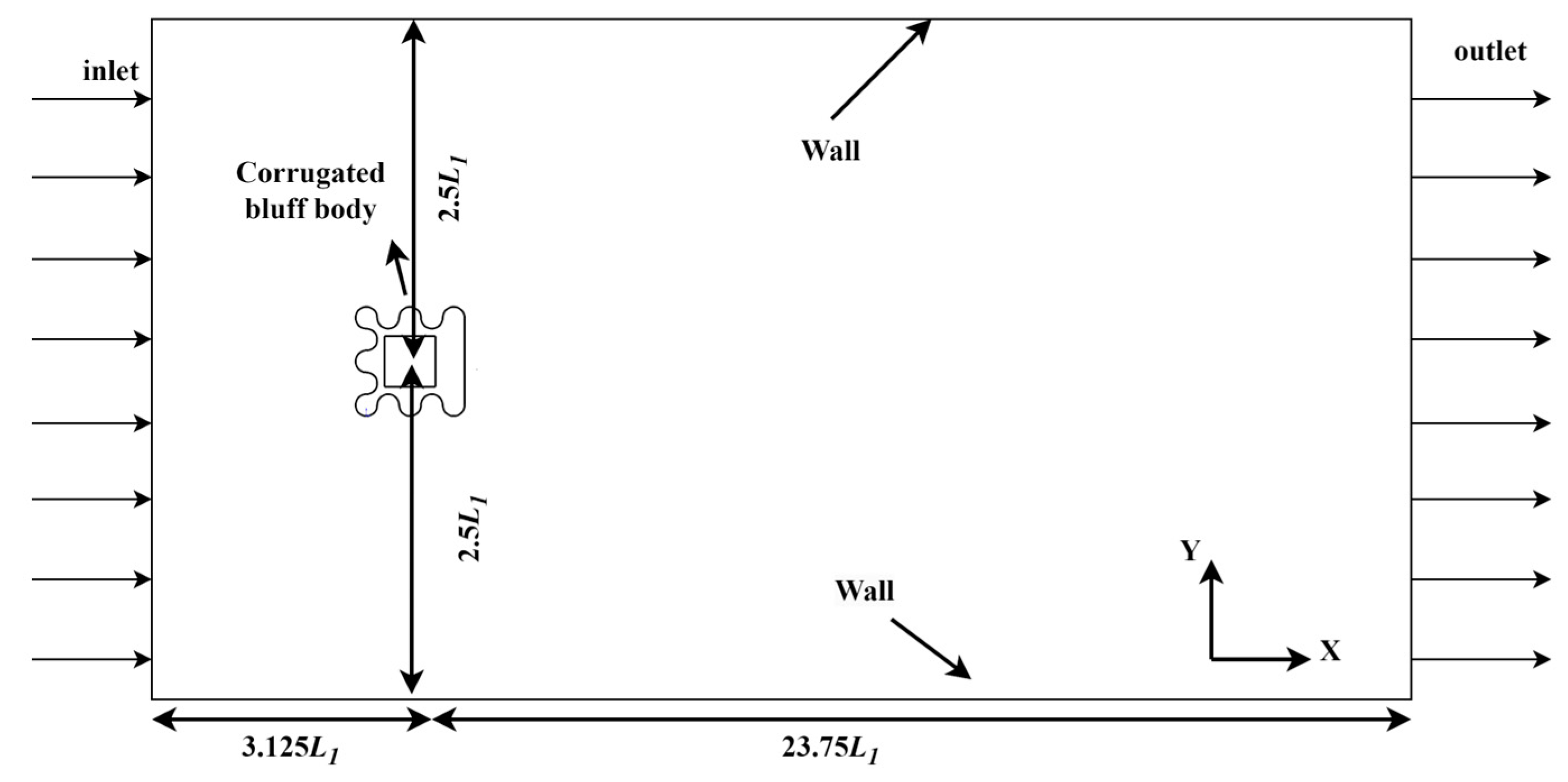
3.2. Two-Dimensional CFD Simulations of the Corrugated Bluff Body
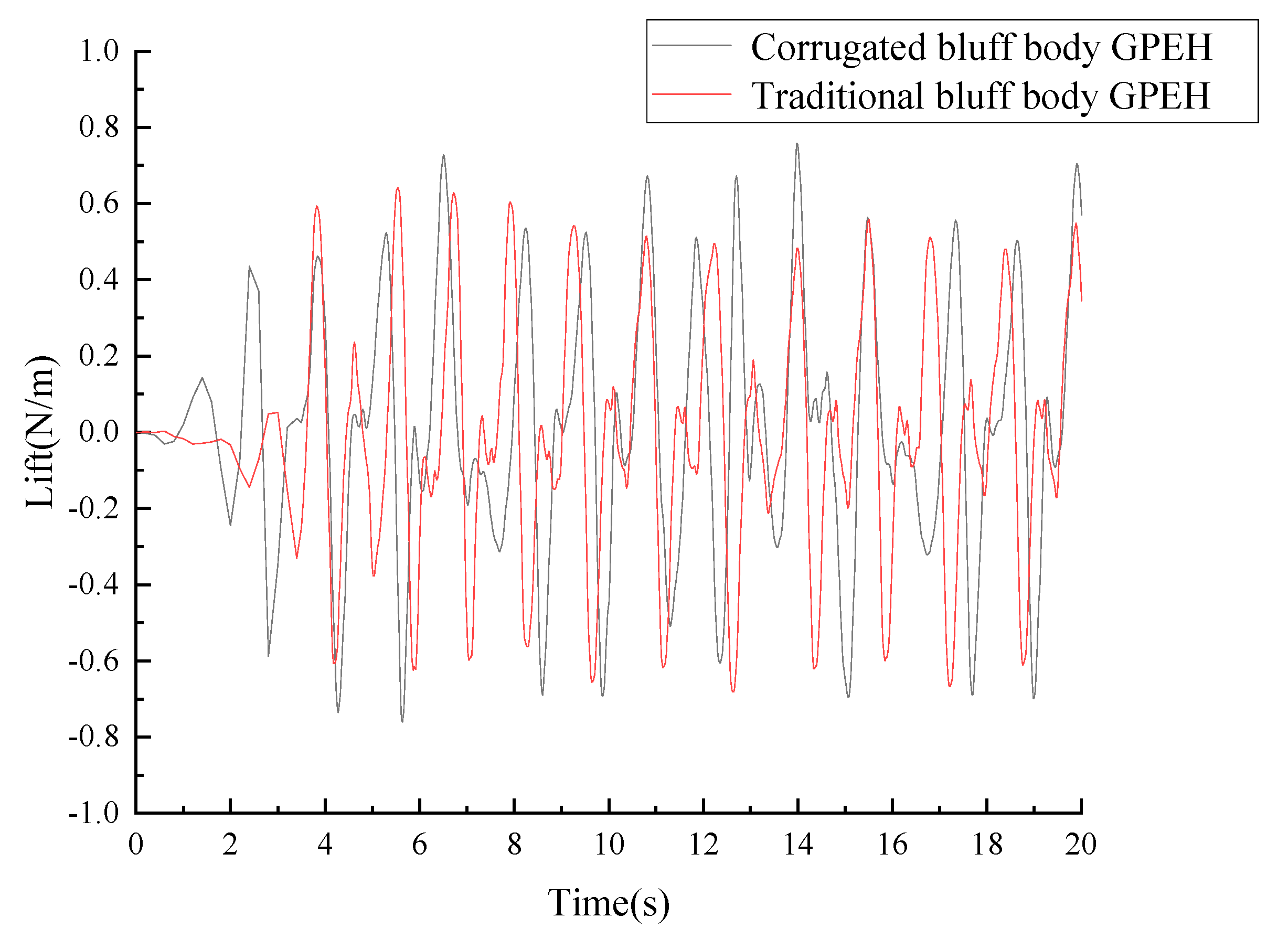
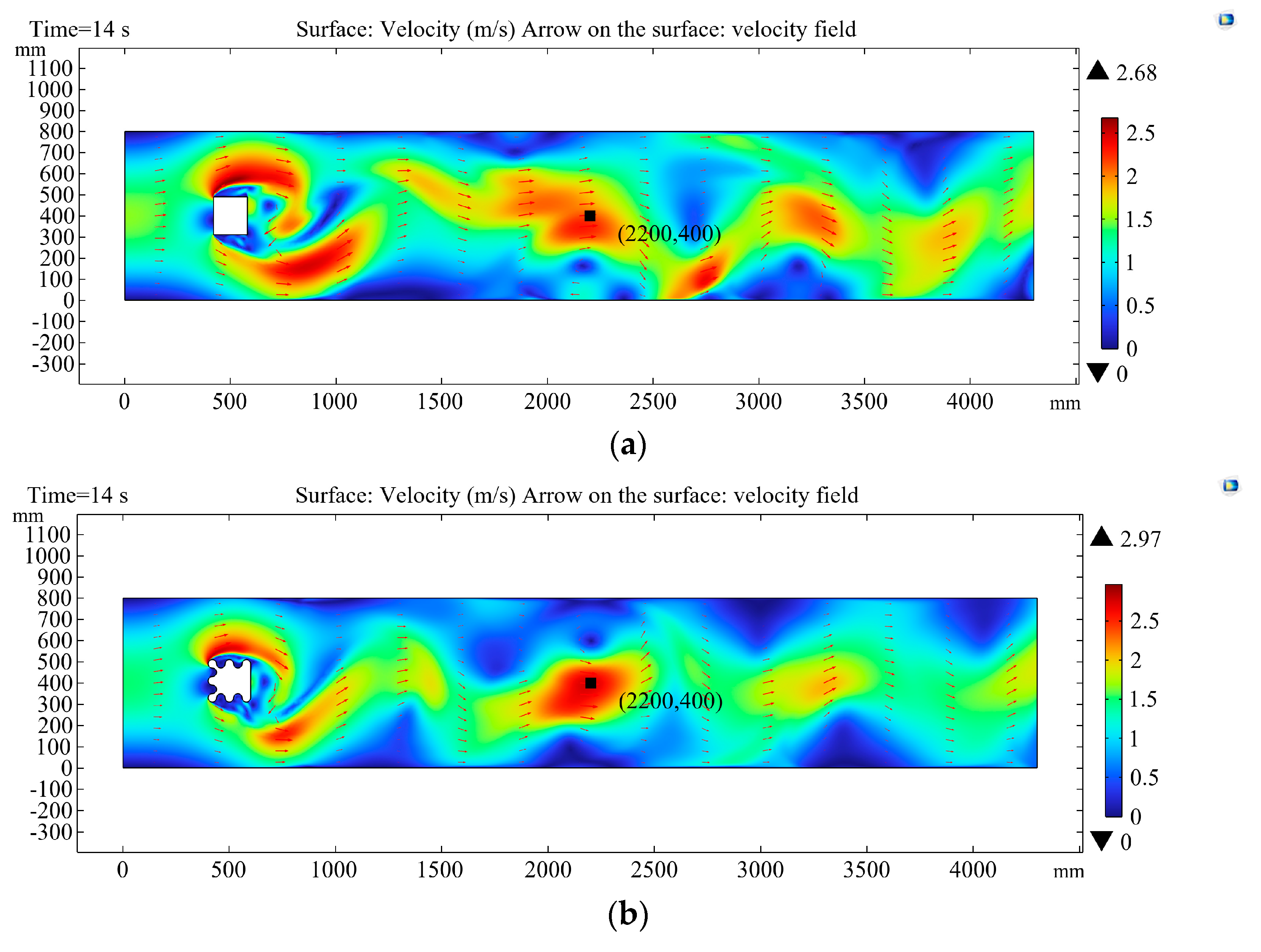
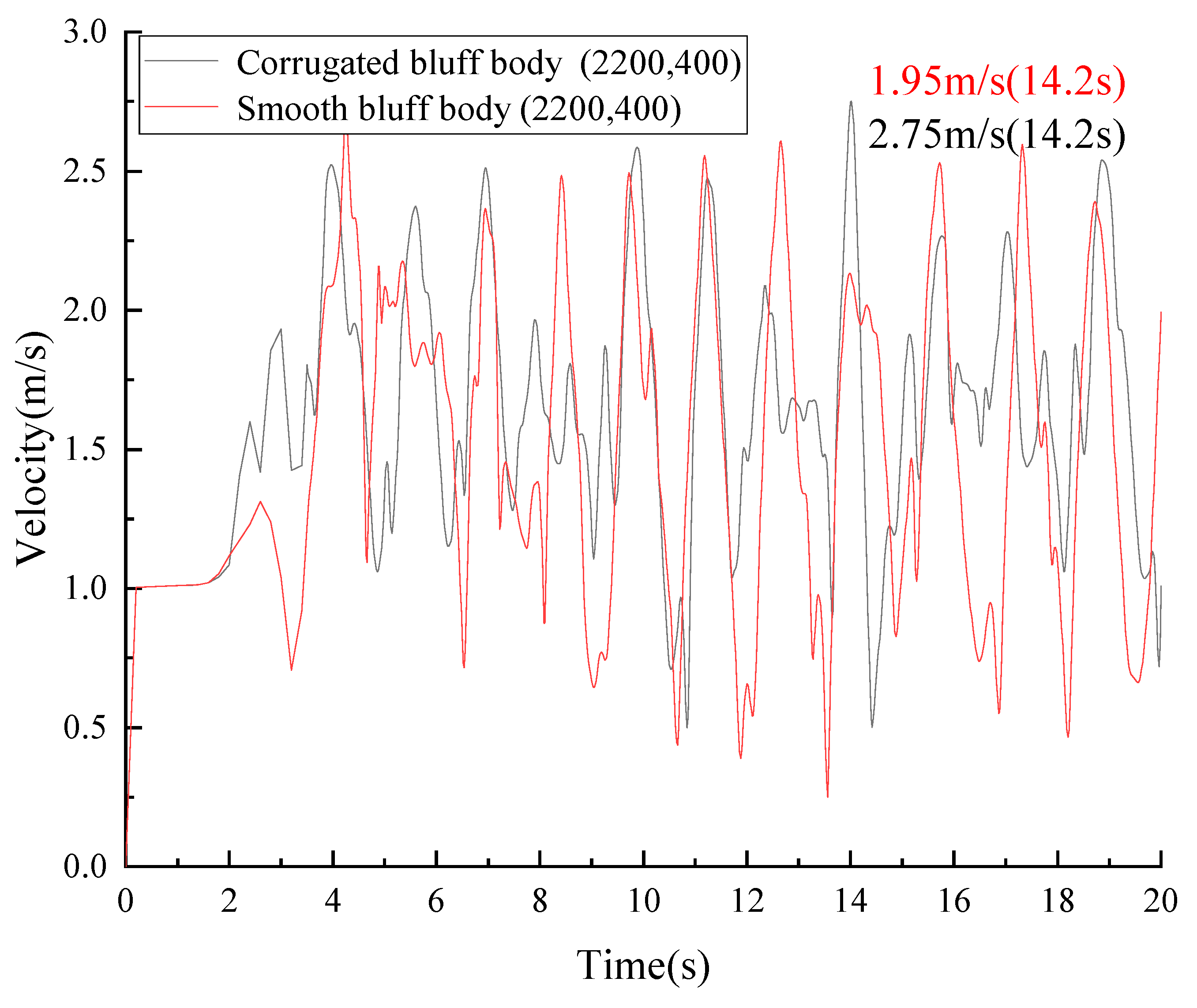
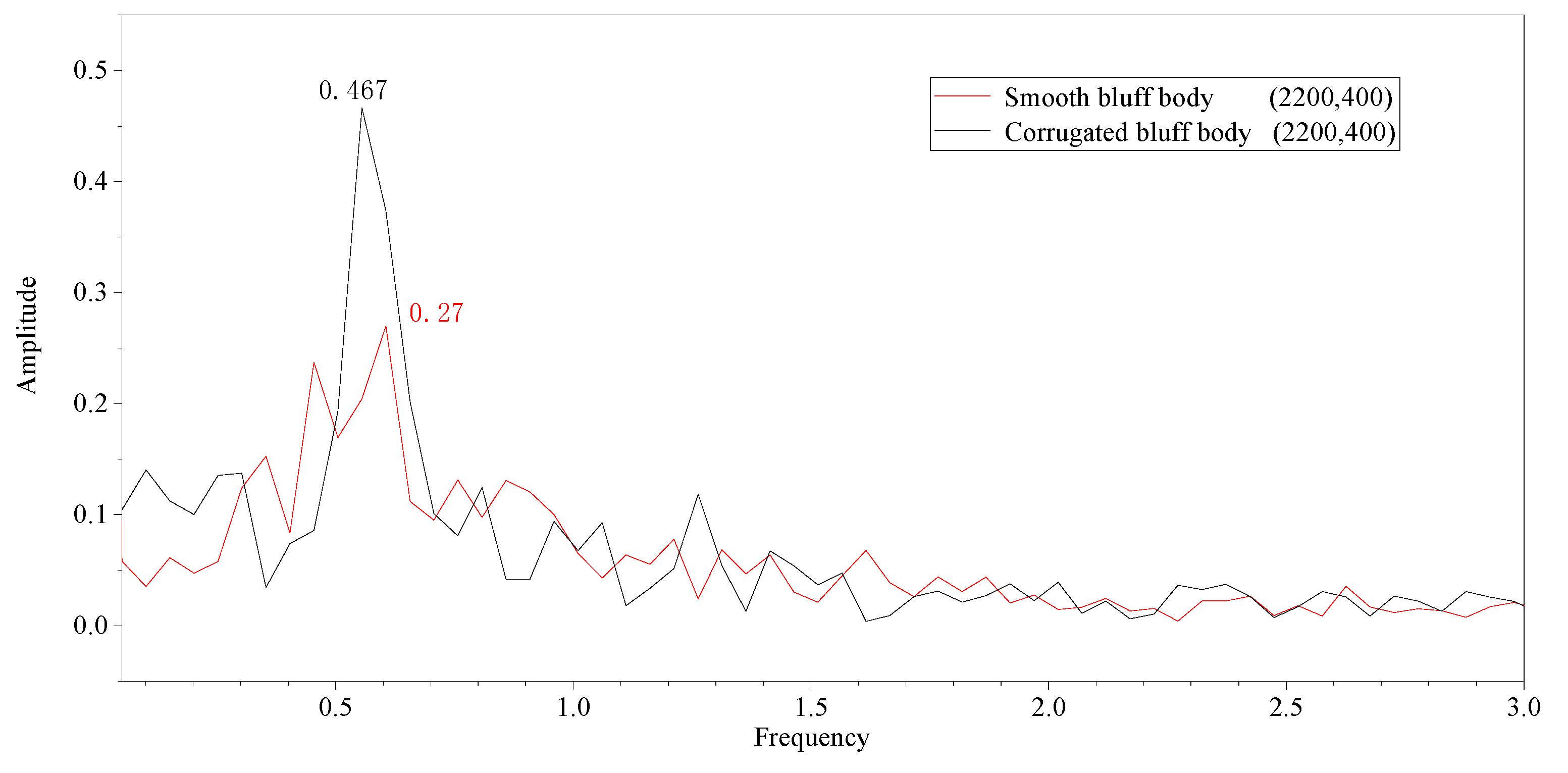
3.3. Validation and Analysis of Simulations
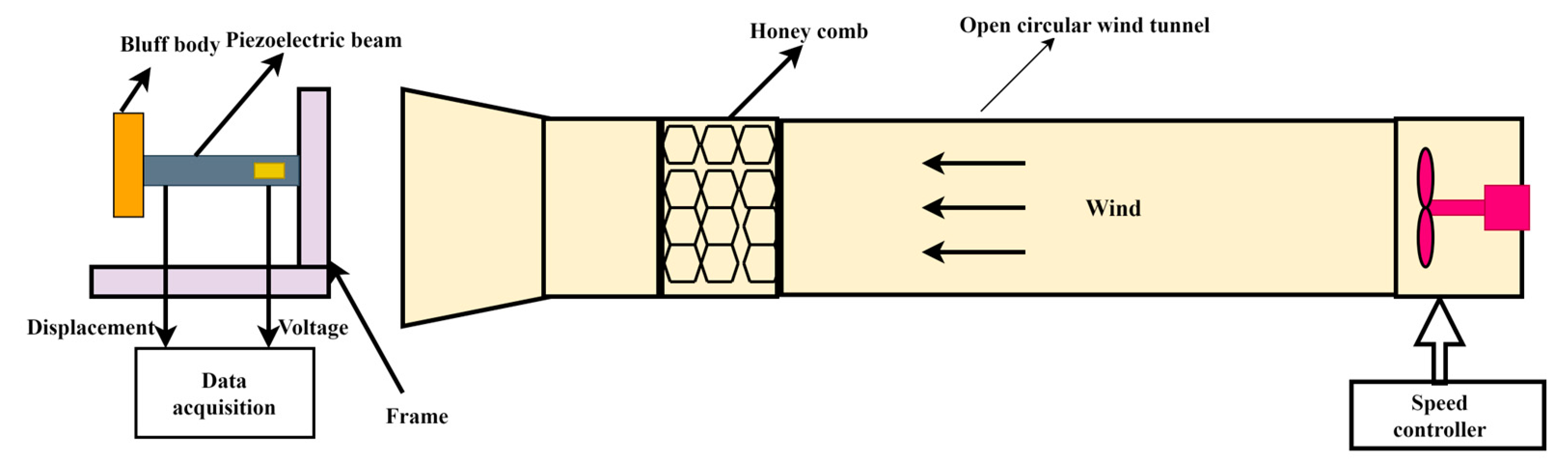
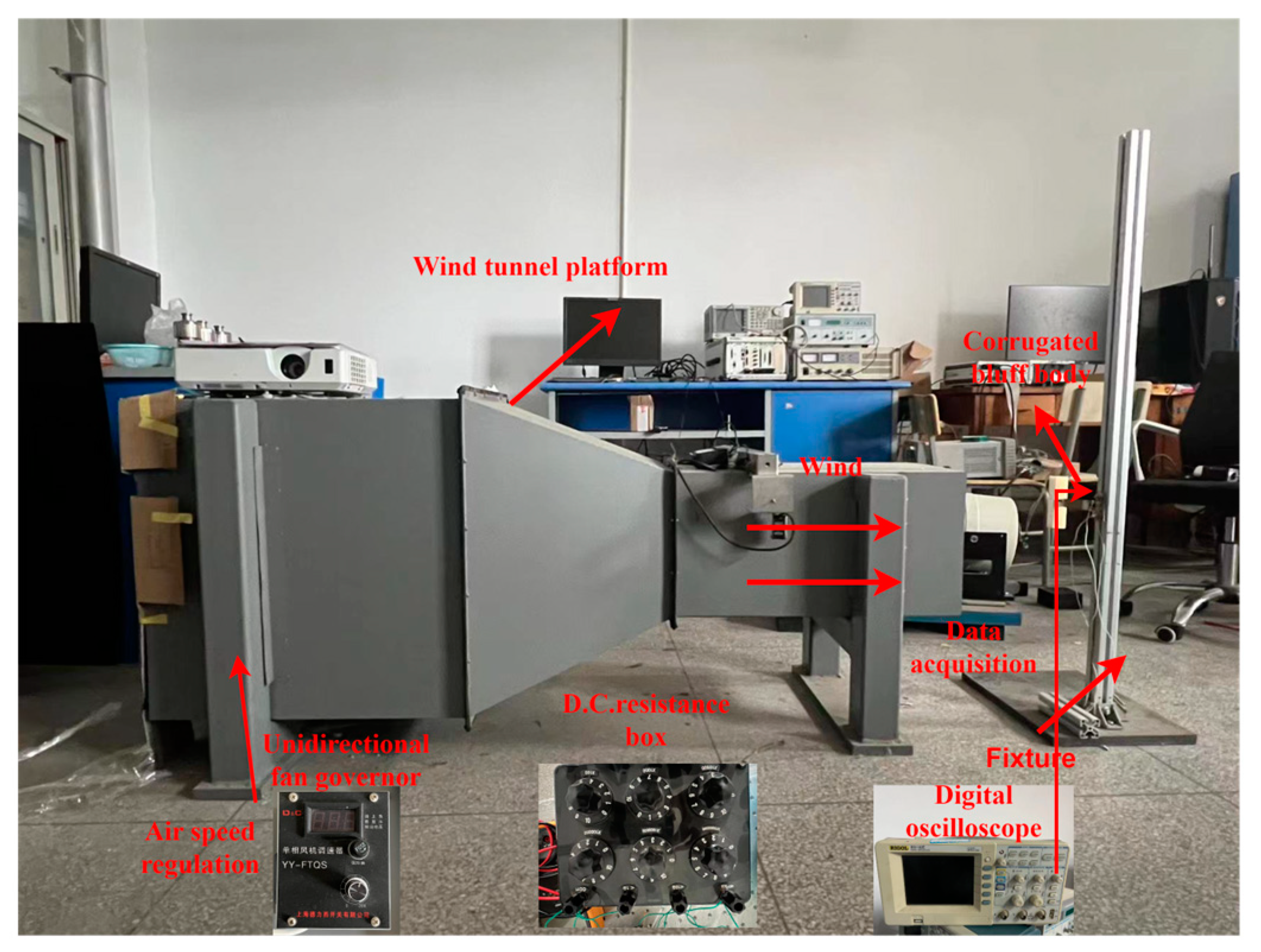
4. Prototypes and Experimental Setup
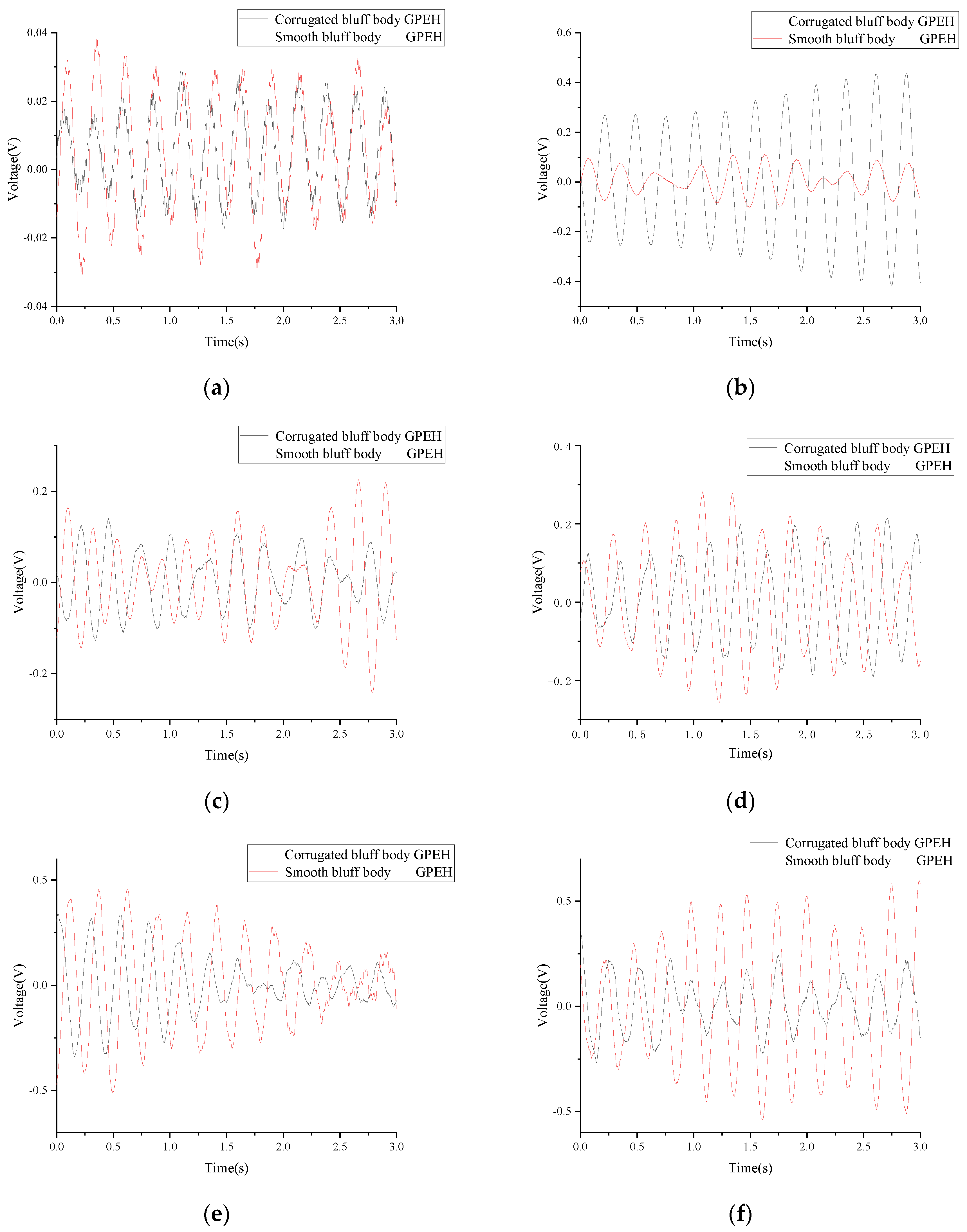
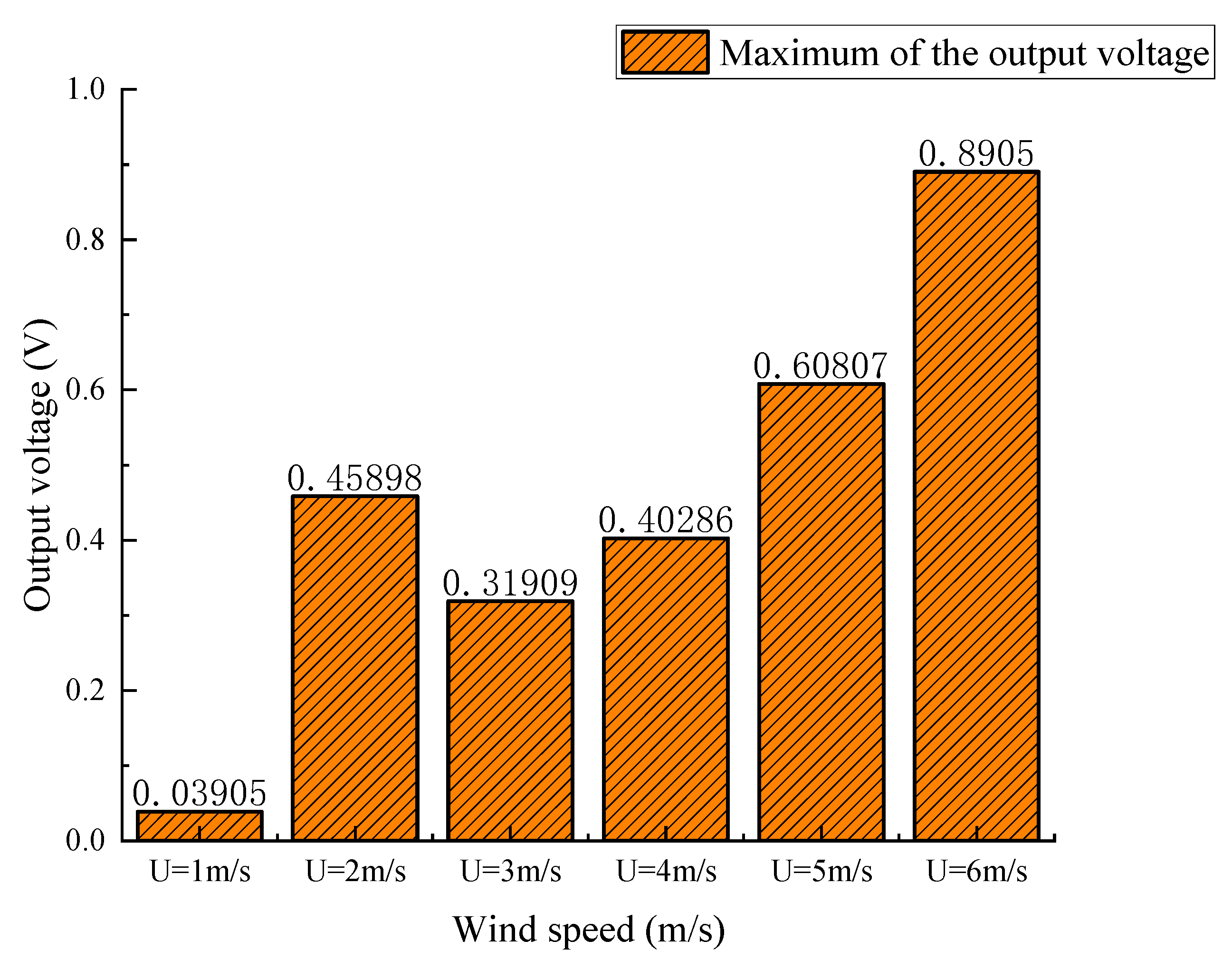
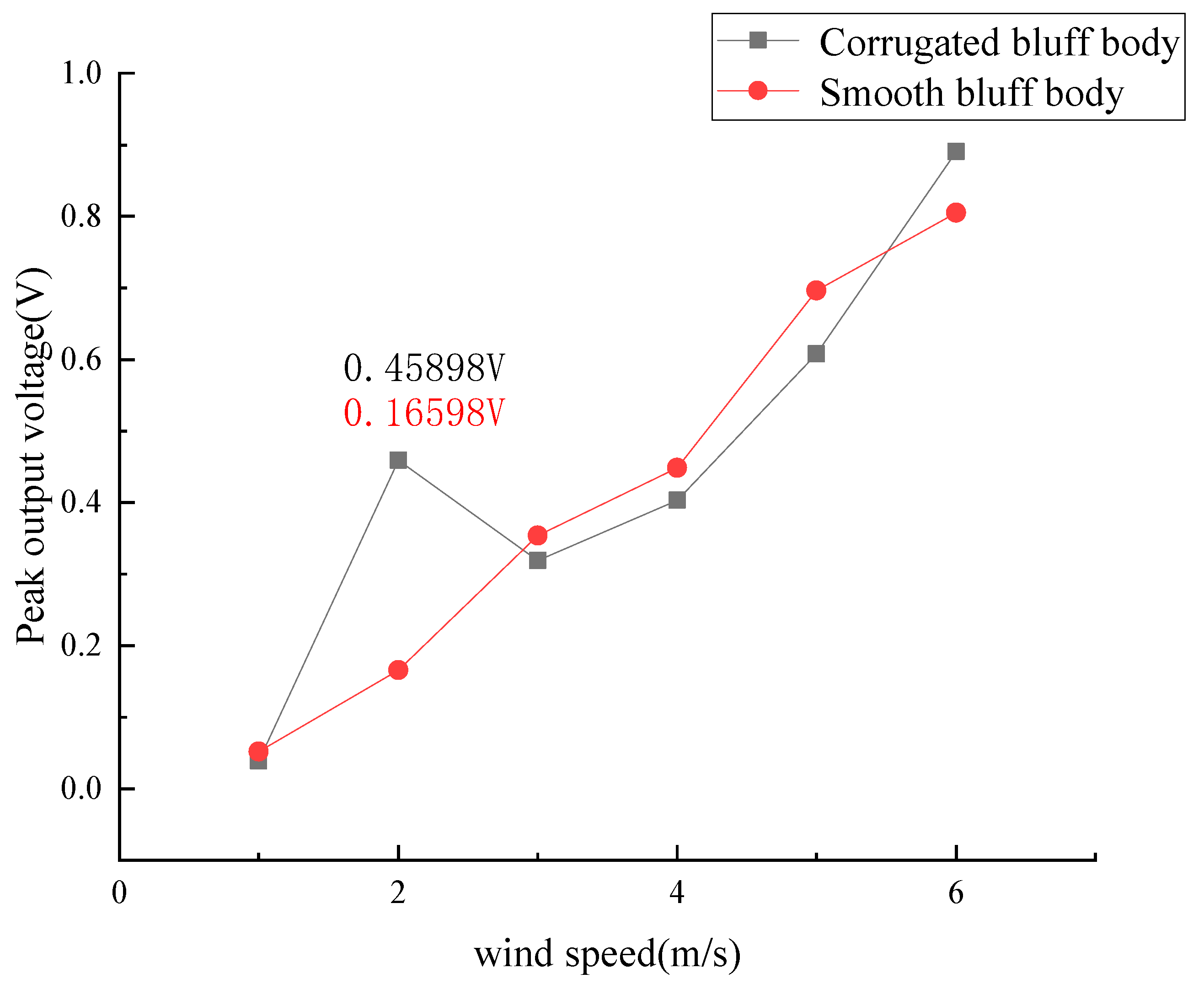
5. Experimental Results and Discussions
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rantz, R.; Halim, M.A.; Xue, T.; Zhang, Q.; Gu, L.; Yang, K.; Roundy, S. Architectures for wrist-worn energy harvesting. Smart Mater. Struct. 2018, 27, 044001. [Google Scholar] [CrossRef][Green Version]
- Zhang, Z.; Wang, S.; Kan, J.; Hu, W.; Chen, Z.; Xu, H. A pneumatic piezoelectric vibration energy harvester based on the compressed air-transducer-structure interaction. Energy Convers. Manag. 2020, 213, 112861. [Google Scholar] [CrossRef]
- Zhao, L.-C.; Zou, H.-X.; Yan, G.; Liu, F.-R.; Tan, T.; Wei, K.-X.; Zhang, W.-M. Magnetic coupling and flextensional amplification mechanisms for high-robustness ambient wind energy harvesting. Energy Convers. Manag. 2019, 201, 112166. [Google Scholar] [CrossRef]
- Kang, W.; Xu, M.; Yao, W.; Zhang, J. Lock-in mechanism of flow over a low-Reynolds-number airfoil with morphing surface. Aerosp. Sci. Technol. 2020, 97, 105647. [Google Scholar] [CrossRef]
- Xu, J.; Bai, J.; Qiao, L.; Zhang, Y. Development of a computational fluid dynamics compatible mathematical model for boundary layer transitional flows in low-disturbance environment. Aerosp. Sci. Technol. 2019, 86, 487–496. [Google Scholar] [CrossRef]
- Xue, D.; Song, B.; Song, W.; Yang, W.; Xu, W.; Wu, T. Computational simulation and free flight validation of body vibration of flapping-wing MAV in forward flight. Aerosp. Sci. Technol. 2019, 95, 105491. [Google Scholar] [CrossRef]
- Zhang, W.; Lv, Z.; Diwu, Q.; Zhong, H. A flutter prediction method with low cost and low risk from test data. Aerosp. Sci. Technol. 2019, 86, 542–557. [Google Scholar] [CrossRef]
- Sun, W.; Jo, S.; Seok, J. Development of the optimal bluff body for wind energy harvesting using the synergetic effect of coupled vortex induced vibration and galloping phenomena. Int. Mech. Sci. 2019, 156, 435–445. [Google Scholar] [CrossRef]
- Wang, J.; Gu, S.; Zhang, C.; Hu, G.; Chen, G.; Yang, K.; Yurchenko, D. Hybrid wind energy scavenging by coupling vortex-induced vibrations and galloping. Energy Convers. Manag. 2020, 213, 112835. [Google Scholar] [CrossRef]
- Wang, J.; Sun, S.; Tang, L.; Hu, G.; Liang, J. On the use of metasurface for Vortex-Induced vibration suppression or energy harvesting. Energy Convers. Manag. 2021, 235, 113991. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, M. Effects of side ratio on energy harvesting from transverse galloping of a rectangular cylinder. Energy 2021, 226, 120420. [Google Scholar] [CrossRef]
- Zhang, M.; Zhang, C.; Abdelkefi, A.; Yu, H.; Gaidai, O.; Qin, X.; Zhu, H.; Wang, J. Piezoelectric energy harvesting from vortex-induced vibration of a circular cylinder: Effect of Reynolds number. Ocean Eng. 2021, 235, 109378. [Google Scholar] [CrossRef]
- Zhang, M.; Abdelkefi, A.; Yu, H.; Ying, X.; Gaidai, O.; Wang, J. Predefined angle of attack and corner shape effects on the effectiveness of square-shaped galloping energy harvesters. Appl. Energy 2021, 302, 117522. [Google Scholar] [CrossRef]





| Parameters | Values | Units |
|---|---|---|
| U | 1 | m/s |
| W1 | 800 | mm |
| L1 | 4300 | mm |
| W2 | 180 | mm |
| L2 | 160 | mm |
| μ | 17.9 × 10−6 | Pa·s |
| ρ | 1.29 | kg/m3 |
| Vinlet | 6 × U × y × (W − y)/W2 × step1 (t [1/s]) | m/s |
| Poutlet | 0 | Pa |
| Parameters | Values | Units |
|---|---|---|
| T | 293.15 | K |
| P | 101,325 | Pa |
| C | 1400 | J/(kg·K) |
| λ | 0.023 | W/(m·K) |
| Properties | Value/Units | Interpretation of Properties |
|---|---|---|
| KP K31 K33 Kt | 0.68 0.38 0.76 0.52 | Coupling factors |
| εTr3 | 3200 | Dielectric constants |
| d31 d33 g31 g33 | −275 × 10−12 C/N 620 × 10−12 C/N 9.7 × 10−3 vm/n 22 × 10−3 vm/n | Piezoelectric constant |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, Y.; Wang, H.; Yang, C.; Sun, H.; Tang, Y.; Zhang, Z. Exploring the Potential of Flow-Induced Vibration Energy Harvesting Using a Corrugated Hyperstructure Bluff Body. Micromachines 2023, 14, 1125. https://doi.org/10.3390/mi14061125
Yuan Y, Wang H, Yang C, Sun H, Tang Y, Zhang Z. Exploring the Potential of Flow-Induced Vibration Energy Harvesting Using a Corrugated Hyperstructure Bluff Body. Micromachines. 2023; 14(6):1125. https://doi.org/10.3390/mi14061125
Chicago/Turabian StyleYuan, Yikai, Hai Wang, Chunlai Yang, Hang Sun, Ye Tang, and Zihao Zhang. 2023. "Exploring the Potential of Flow-Induced Vibration Energy Harvesting Using a Corrugated Hyperstructure Bluff Body" Micromachines 14, no. 6: 1125. https://doi.org/10.3390/mi14061125
APA StyleYuan, Y., Wang, H., Yang, C., Sun, H., Tang, Y., & Zhang, Z. (2023). Exploring the Potential of Flow-Induced Vibration Energy Harvesting Using a Corrugated Hyperstructure Bluff Body. Micromachines, 14(6), 1125. https://doi.org/10.3390/mi14061125








