Impact of the Spatial Domain Size on the Performance of the Ts-VI Triangle Method in Terrestrial Evapotranspiration Estimation
Abstract
:1. Introduction
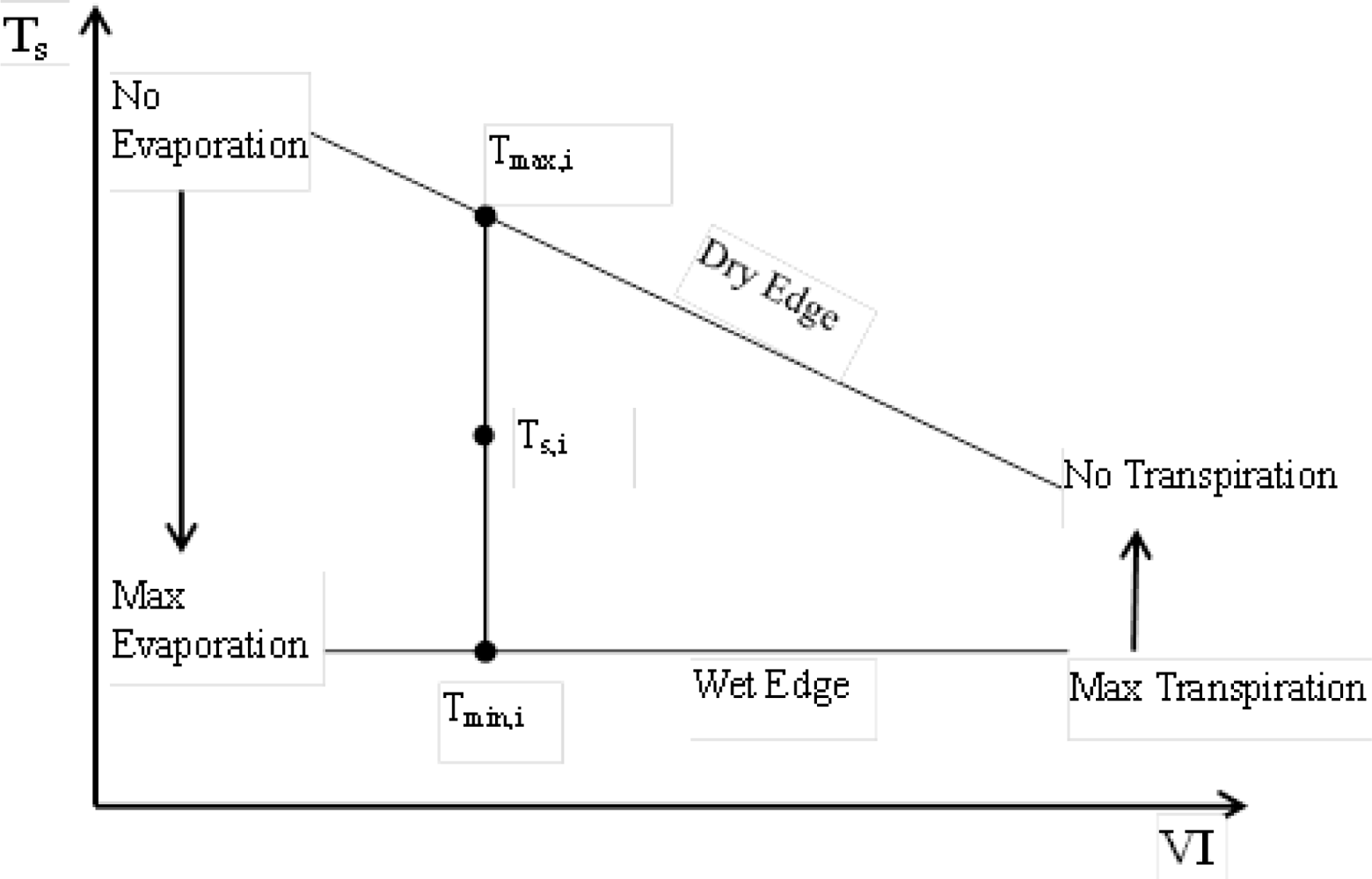
2. Ts-VI Triangle Method
3. Study Site and Data
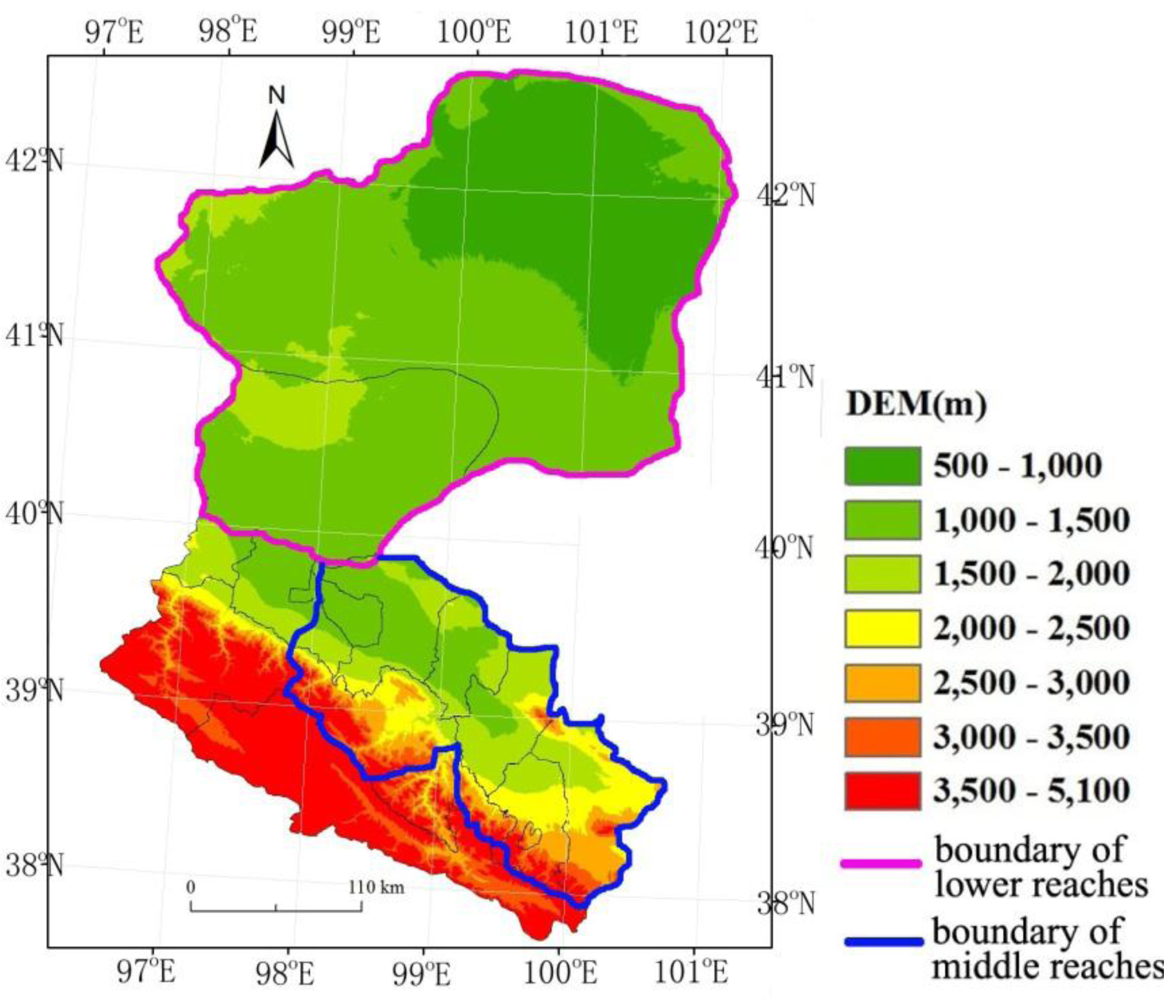
3.1. Area Description
3.2. Moderate Resolution Imaging Spectroradiometer (MODIS) Data
3.3. Meteorological Data
4. Results
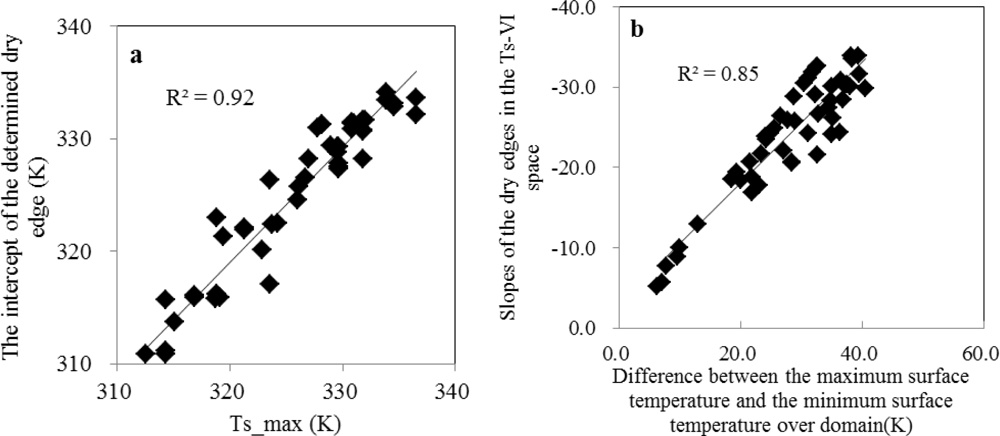
4.1. Comparisons of Dry/Wet Edges Among Different Domain Sizes
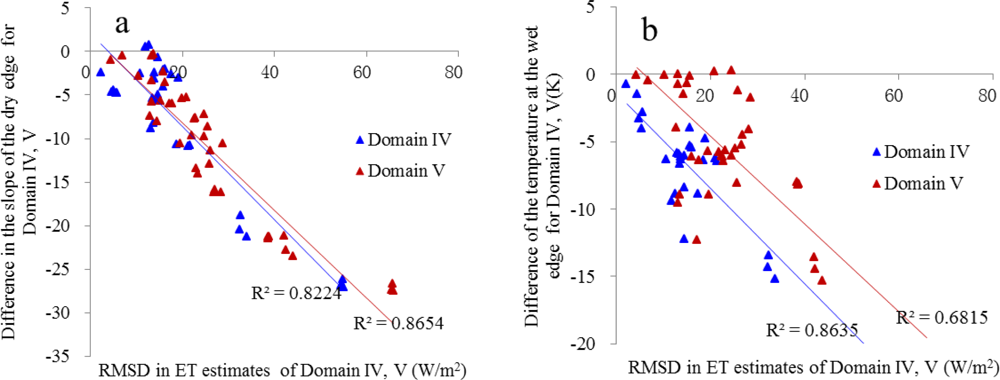
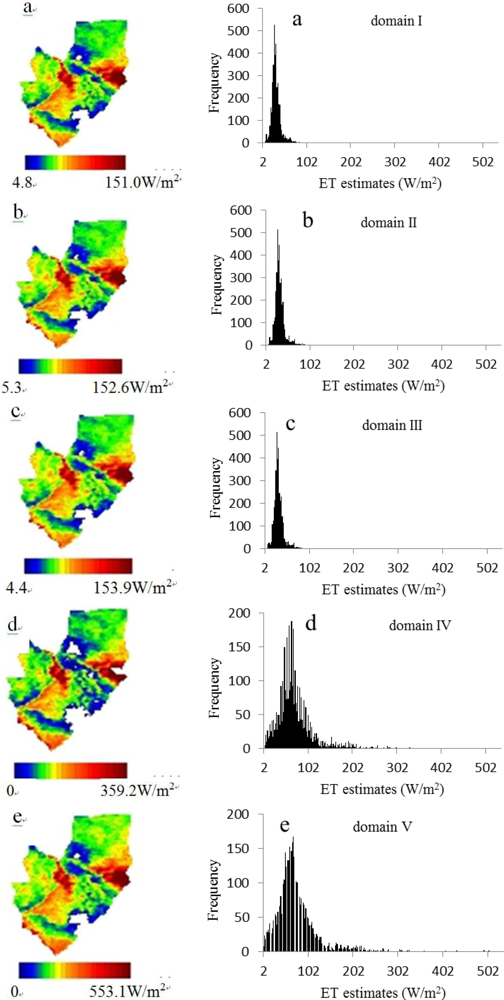
4.2. Variation in ET Estimates with Domain Size
5. Conclusions
Acknowledgments
References
- Norman, J.M.; Kustas, W.P.; Humes, K.S. A two-source approach for estimating soil and vegetation energy fluxes from observation of directional radiometric surface temperature. Agric. For. Meteorol 1995, 77, 263–293. [Google Scholar]
- Bastiaanssen, W.G.M.; Menenti, M.; Feddes, R.A.; Holtslag, A.A.M. A remote sensing surface energy balance algorithm for land (SEBAL) 1. Formulation. J. Hydrol 1998, 213, 198–212. [Google Scholar]
- Kustas, W.P.; Norman, J.M. Evaluation of soil and vegetation heat flux predictions using a simple two-source model with radiometric temperatures for partial canopy cover. Agric. For. Meteorol 1999, 94, 13–29. [Google Scholar]
- Jiang, L.; Islam, S. Estimation of surface evaporation map over southern Great Plains using remote sensing data. Water Resour. Res 2001, 37, 329–340. [Google Scholar]
- Su, Z.B. The surface energy balance system (SEBS) for estimation of turbulent heat fluxes. Hydrol. Earth Syst. Sci 2002, 6, 85–89. [Google Scholar]
- Zhang, R.H.; Sun, X.M.; Wang, W.M.; Xu, J.P.; Zhu, Z.L.; Tian, J. An operational two-layer remote sensing model to estimate surface flux in regional scale: Physical background. Sci. China Ser. D 2005, 48, 225–244. [Google Scholar]
- Allen, R.G.; Tasumi, M.; Trezza, R. Satellite-based energy balance for Mapping Evapotranspiration with Internalized Calibration (METRIC)-model. J. Irrig. Drain Eng 2007, 133, 380–394. [Google Scholar]
- Jiang, L.; Islam, S. A methodology for estimation of surface evapotranspiration over large areas using remote sensing observations. Geophys. Res. Lett 1999, 26, 2773–2776. [Google Scholar]
- Venturini, V.; Bisht, G.; Islam, S.; Jiang, L. Comparison of evaporative fractions estimated from AVHRR and MODIS sensors over South Florida. Remote Sens. Environ 2004, 93, 77–86. [Google Scholar]
- Yang, Y.M.; Su, H.B.; Zhang, R.H.; Tian, J.; Yang, S.Q. Estimation of regional evapotranspiration based on remote sensing: Case study in the Heihe River Basin. J. Appl. Remote Sens. 2012, 6. [Google Scholar] [CrossRef]
- Stisen, S.; Sandholt, I.; Nørgaard, A.; Fensholt, R.; Jensen, K.H. Combining the triangle method with thermal inertia to estimate regional evapotranspiration—Applied to MSG/SEVIRI data in the Senegal River basin. Remote Sens. Environ 2008, 112, 1242–1255. [Google Scholar]
- Tang, R.L.; Li, Z.L.; Tang, B.H. An application of the T(s)-VI triangle method with enhanced edges determination for evapotranspiration estimation from MODIS data in arid and semi-arid regions: Implementation and validation. Remote Sens. Environ 2010, 114, 540–551. [Google Scholar]
- Choi, M.; Kustas, W.P.; Anderson, M.C.; Allen, R.G.; Li, F.Q.; Kjaersgaard, J.H. An intercomparison of three remote sensing-based surface energy balance algorithms over a corn and soybean production region (Iowa, US) during SMACEX. Agric. For. Meteorol 2009, 149, 2082–2097. [Google Scholar]
- Tang, R.L.; Li, Z.L.; Jia, Y.Y.; Li, C.R.; Sun, X.M.; Kustas, W.P.; Anderson, M.C. An intercomparison of three remote sensing-based energy balance models using Large Aperture Scintillometer measurements over a wheat-corn production region. Remote Sens. Environ 2011, 115, 3187–3202. [Google Scholar]
- Su, H.B.; Tian, J.; Chen, S.H.; Zhang, R.H.; Rong, Y.; Yang, Y.M.; Tang, X.Z.; Garcia, J. A New Algorithm to Automatically Determine the Boundary of the Scatter Plot in the Triangle Method for Evapotranspiration Retrieval. Proceedings of 2011 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Vancouver, BC, Canada, 24–29 July 2011.
- Merlin, O.; Duchemin, B.; Hagolle, O; Jacob, F; Coudert, B.; Chehbouni, A.; Dedieu, G.; Garatuza, J.; Kerr, Y. Disaggregation of MODIS surface temperature over an agricultural area using a time series of Formosat-2 images. Remote Sens. Environ 2010, 114, 2500–2512. [Google Scholar]
- Merlin, O.; Rudiger, C.; Al Bitar, A.; Richaume, P.H.; Walker, J.P.; Kerr, Y.H. Disaggregation of SMOS soil moisture in southeastern Australia. IEEE Trans. Geosci. Remote Sens 2012, 50, 1556–1571. [Google Scholar]
- Carlson, T. An Overview of the “Triangle Method” for estimating surface evapotranspiration and soil moisture from satellite imagery. Sensors 2007, 7, 1612–1629. [Google Scholar]
- Zhang, R.H.; Tian, J.; Su, H.B.; Sun, X.M.; Chen, S.H.; Xia, J. Two improvements of an operational two-layer model for terrestrial surface heat flux retrieval. Sensors 2008, 8, 6165–6187. [Google Scholar]
- Chen, X.Y.; Yamaguchi, J.; Chen, Y.S. Scale effect of vegetation-index-based spatial sharpening for thermal imagery: A simulation study by ASTER data. IEEE Geosci. Remote Sens. Lett 2012, 9, 549–553. [Google Scholar]
- Long, D.; Singh, V.P.; Li, Z.L. How sensitive is SEBAL to changes in input variables, domain size and satellite sensor? J. Geophys. Res. 2011, 116. [Google Scholar] [CrossRef]
- Long, D.; Singh, V.P.; Scanlon, B.R. Deriving theoretical boundaries to address scale dependencies of triangle models for evapotranspiration estimation. J. Geophys. Res. 2012, 117. [Google Scholar] [CrossRef]
- Idso, S.B.; Jackson, R.D. Thermal radiation from the atmosphere. J. Geophys. Res 1969, 74, 5397–5403. [Google Scholar]
- Zhang, R.H. Experimental Remote Sensing Modeling and Surface Foundation (in Chinese); Beijing Science Press: Beijing, China, 1996; pp. 105–106. [Google Scholar]
- Zhang, Y.C.; Yu., J.J.; Wang, P.; Fu, G.B. Vegetation response to integrated water management in the Ejina Basin, Northwest China. Hydrol. Process 2011, 25, 3448–3461. [Google Scholar]
- Derber, J.C.; Parish, D.F.; Lord, S.J. The new global operational analysis system at the National Meteorological Center. Weather Forecast 1991, 6, 538–547. [Google Scholar]

| a (K) | b | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| DOY | I | II | III | IV | V | I | II | III | IV | V |
| 96 | 315.9 | 316.0 | 315.7 | 311.1 | 310.9 | −32.7 | −32.5 | −31.8 | −5.6 | −5.2 |
| 112 | 322.1 | 321.9 | 321.3 | 315.9 | 313.7 | −30.4 | −31.2 | −28.8 | −9.9 | −7.7 |
| 121 | 323.0 | 320.1 | 320.2 | 315.8 | 310.8 | −25.6 | −20.6 | −20.7 | −18.6 | −13.0 |
| 144 | 327.3 | 327.5 | 326.5 | 325.7 | 322.4 | −25.7 | −26.4 | −24.2 | −21.7 | −18.4 |
| 152 | 331.3 | 331.3 | 330.9 | 328.2 | 320.1 | −33.9 | −33.9 | −33.6 | −29.1 | −17.7 |
| 163 | 331.4 | 331.5 | 329.3 | 327.8 | 322.5 | −30.2 | −30.8 | −27.4 | −22.0 | −16.8 |
| 176 | 332.9 | 333.1 | 331.6 | 330.8 | 328.2 | −26.1 | −26.7 | −24.2 | −23.5 | −20.7 |
| 192 | 334.1 | 333.5 | 331.7 | 330.7 | 324.5 | −31.6 | −28.4 | −28.3 | −25.9 | −18.7 |
| 224 | 333.6 | 332.1 | 331.4 | 330.9 | 329.4 | −29.8 | −24.3 | −24.1 | −24.8 | −23.8 |
| 231 | 328.8 | 329.3 | 326.3 | 317.1 | 308.9 | −30.3 | −30.1 | −30.1 | −19.4 | −8.9 |
| c (K) | Ts_max(K) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| DOY | I | II | III | IV | V | I | II | III | IV | V |
| 96 | 283.2 | 283.5 | 283.9 | 304.1 | 304.6 | 316.9 | 316.9 | 314.3 | 314.3 | 314.3 |
| 112 | 291.6 | 290.8 | 292.5 | 306.0 | 306.1 | 321.3 | 321.3 | 319.4 | 319.1 | 315.1 |
| 121 | 292.4 | 291.7 | 291.9 | 297.2 | 297.9 | 318.9 | 318.9 | 318.9 | 318.7 | 312.5 |
| 144 | 298.3 | 300.8 | 301.6 | 302.3 | 302.3 | 329.6 | 329.6 | 326.7 | 326.1 | 323.8 |
| 152 | 291.9 | 293.1 | 292.7 | 295.9 | 297.2 | 328.2 | 328.2 | 327.7 | 327.0 | 322.9 |
| 163 | 294.2 | 294.9 | 294.9 | 300.8 | 300.6 | 330.9 | 330.9 | 329.7 | 329.7 | 324.3 |
| 176 | 297.7 | 300.3 | 300.6 | 306.6 | 306.7 | 334.5 | 334.5 | 331.8 | 331.8 | 331.8 |
| 192 | 294.6 | 296.6 | 296.8 | 303.0 | 302.6 | 333.8 | 333.8 | 332.0 | 331.8 | 326.0 |
| 224 | 293.1 | 295.9 | 296.4 | 305.3 | 305.4 | 336.5 | 336.5 | 331.0 | 330.8 | 328.9 |
| 231 | 291.4 | 291.2 | 291.3 | 297.6 | 299.4 | 329.5 | 329.5 | 323.5 | 323.5 | 312.7 |
| a | r | RMSD | MD | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| DOY | I–V | II–V | III–V | IV–V | I–V | II–V | III–V | IV–V | I–V | II–V | III–V | IV–V |
| 96 | 0.9935 | 0.9933 | 0.9940 | 1.0000 | 65.89 | 65.44 | 65.65 | 6.98 | −46.68 | −46.06 | −46.54 | −4.11 |
| 112 | 0.9857 | 0.9862 | 0.9878 | 0.9983 | 42.37 | 43.94 | 42,16 | 15.75 | −20.43 | −22.64 | −21.01 | 12.38 |
| 121 | 0.9816 | 0.9888 | 0.9888 | 0.9941 | 25.40 | 22.80 | 22.53 | 15.29 | −18.01 | −11.46 | −10.99 | 9.41 |
| 144 | 0.9881 | 0.9984 | 0.9989 | 0.9989 | 12.85 | 14.42 | 13.31 | 13.35 | −3.41 | 12.06 | 11.93 | 12.79 |
| 152 | 0.9970 | 0.9975 | 0.9975 | 0.9985 | 26.83 | 28.34 | 26.98 | 26.04 | 20.78 | 23.17 | 21.43 | 23.25 |
| 163 | 0.9959 | 0.9971 | 0.9967 | 0.9993 | 22.86 | 23.26 | 19.56 | 20.89 | 7.64 | 8.84 | 4.72 | 18.85 |
| 176 | 0.9956 | 0.9975 | 0.9973 | 0.9998 | 19.78 | 17.79 | 16.10 | 10.32 | −7.64 | −2.94 | −5.53 | 8.71 |
| 192 | 0.9972 | 0.9976 | 0.9983 | 0.9996 | 25.88 | 24.67 | 21.89 | 24.65 | 7.29 | 11.49 | 6.23 | 21.57 |
| 224 | 0.9964 | 0.9965 | 0.9969 | 0.9999 | 17.23 | 13.33 | 13.63 | 4.32 | −9.77 | −7.59 | −8.78 | 3.69 |
| 231 | 0.9400 | 0.9378 | 0.9489 | 0.9891 | 38.63 | 38.67 | 38.87 | 28.71 | 0.84 | 1.53 | −5.41 | 5.79 |
| average | 0.9871 | 0.9891 | 0.9905 | 0.9977 | 29.77 | 29.27 | 28.07 | 16.63 | −6.94 | −3.36 | −5.40 | 11.23 |
| b | r | RMSD | MD | ||||||
|---|---|---|---|---|---|---|---|---|---|
| DOY | I–IV | II–IV | III–IV | I–IV | II–IV | III–IV | I–IV | II–IV | III–IV |
| 96 | 0.9928 | 0.9926 | 0.9935 | 54.95 | 54.50 | 54.81 | −42.28 | −41.73 | −42.24 |
| 112 | 0.9890 | 0.9895 | 0.9909 | 32.40 | 33.96 | 32.51 | −19.24 | −21.15 | −20.14 |
| 121 | 0.9910 | 0.9991 | 0.9992 | 19.04 | 16.14 | 15.78 | −19.14 | −13.46 | −13.04 |
| 144 | 0.9927 | 0.9997 | 0.9999 | 15.84 | 4.59 | 2.36 | −13.22 | 1.38 | 0.23 |
| 152 | 0.9993 | 0.9996 | 0.9995 | 5.56 | 5.68 | 5.03 | 1.37 | 3.26 | 1.59 |
| 163 | 0.9979 | 0.9986 | 0.9984 | 13.60 | 13.16 | 13.50 | −4.25 | −3.10 | −8.63 |
| 176 | 0.9961 | 0.9979 | 0.9978 | 17.56 | 13.91 | 14.58 | −11.49 | −6.95 | −10.71 |
| 192 | 0.9980 | 0.9982 | 0.9988 | 14.67 | 10.82 | 13.89 | −7.70 | −4.91 | −10.68 |
| 224 | 0.9971 | 0.9973 | 0.9975 | 14.57 | 11.89 | 12.63 | −9.88 | −8.94 | −10.16 |
| 231 | 0.9816 | 0.9808 | 0.9861 | 21.14 | 21.58 | 18.71 | 9.00 | 9.65 | 2.65 |
| average | 0.9936 | 0.9953 | 0.9962 | 20.93 | 18.62 | 18.38 | −11.68 | −8.59 | −11.11 |
| c | r | RMSD | MD | d | r | RMSD | MD | |||
|---|---|---|---|---|---|---|---|---|---|---|
| DOY | I–III | II–III | I–III | II–III | I–III | II–III | DOY | I–II | I–II | I–II |
| 96 | 1.0000 | 1.0000 | 0.68 | 0.54 | −0.37 | 0.32 | 96 | 1.0000 | 0.64 | −0.63 |
| 112 | 0.9999 | 1.0000 | 1.46 | 2.73 | 0.33 | −1.70 | 112 | 1.0000 | 2.04 | 1.96 |
| 121 | 0.9919 | 1.0000 | 1.14 | 0.44 | −1.03 | −0.43 | 121 | 0.9867 | 2.65 | −1.53 |
| 144 | 0.9899 | 0.9998 | 3.34 | 2.66 | −1.12 | 0.49 | 144 | 0.9930 | 2.92 | −2.11 |
| 152 | 1.0000 | 1.0000 | 1.86 | 1.82 | −0.89 | 1.78 | 152 | 0.9999 | 2.48 | −2.15 |
| 163 | 0.9999 | 0.9999 | 4.93 | 5.78 | 3.37 | 4.91 | 163 | 0.9999 | 2.01 | −1.75 |
| 176 | 0.9996 | 0.9999 | 4.98 | 4.14 | −1.89 | 3.16 | 176 | 0.9994 | 5.79 | −5.32 |
| 192 | ,0.9997 | 0.9998 | 5.53 | 5.30 | 0.80 | 4.72 | 192 | 0.9999 | 2.85 | −1.30 |
| 224 | 0.9996 | 0.9999 | 4.83 | 1.36 | −1.23 | 0.77 | 224 | 0.9995 | 2.94 | 0.67 |
| 231 | 0.9994 | 0.9991 | 6.36 | 7.14 | 5.75 | 6.27 | 231 | 1.0000 | 1.21 | −1.12 |
| average | 0.9980 | 0.9998 | 3.51 | 3.19 | 0.37 | 2.03 | average | 0.9978 | 2.55 | −1.33 |
Share and Cite
Tian, J.; Su, H.; Sun, X.; Chen, S.; He, H.; Zhao, L. Impact of the Spatial Domain Size on the Performance of the Ts-VI Triangle Method in Terrestrial Evapotranspiration Estimation. Remote Sens. 2013, 5, 1998-2013. https://doi.org/10.3390/rs5041998
Tian J, Su H, Sun X, Chen S, He H, Zhao L. Impact of the Spatial Domain Size on the Performance of the Ts-VI Triangle Method in Terrestrial Evapotranspiration Estimation. Remote Sensing. 2013; 5(4):1998-2013. https://doi.org/10.3390/rs5041998
Chicago/Turabian StyleTian, Jing, Hongbo Su, Xiaomin Sun, Shaohui Chen, Honglin He, and Linjun Zhao. 2013. "Impact of the Spatial Domain Size on the Performance of the Ts-VI Triangle Method in Terrestrial Evapotranspiration Estimation" Remote Sensing 5, no. 4: 1998-2013. https://doi.org/10.3390/rs5041998
APA StyleTian, J., Su, H., Sun, X., Chen, S., He, H., & Zhao, L. (2013). Impact of the Spatial Domain Size on the Performance of the Ts-VI Triangle Method in Terrestrial Evapotranspiration Estimation. Remote Sensing, 5(4), 1998-2013. https://doi.org/10.3390/rs5041998






