Sparse Regularization Least-Squares Reverse Time Migration Based on the Krylov Subspace Method
Abstract
1. Introduction
2. Methods
2.1. Least-Squares Reverse Time Migration
2.2. Krylov Subspace Inversion Method for LSRTM
| Algorithm 1: FGK projection process |
| (1) Input: observed data , modeling operator , migration operator , initial image (2) Initialize: , where (3) For do: (a) Compute for (b) Set , compute and take (c) Compute and (d) for and set (e) Compute , and take (4) End do . |
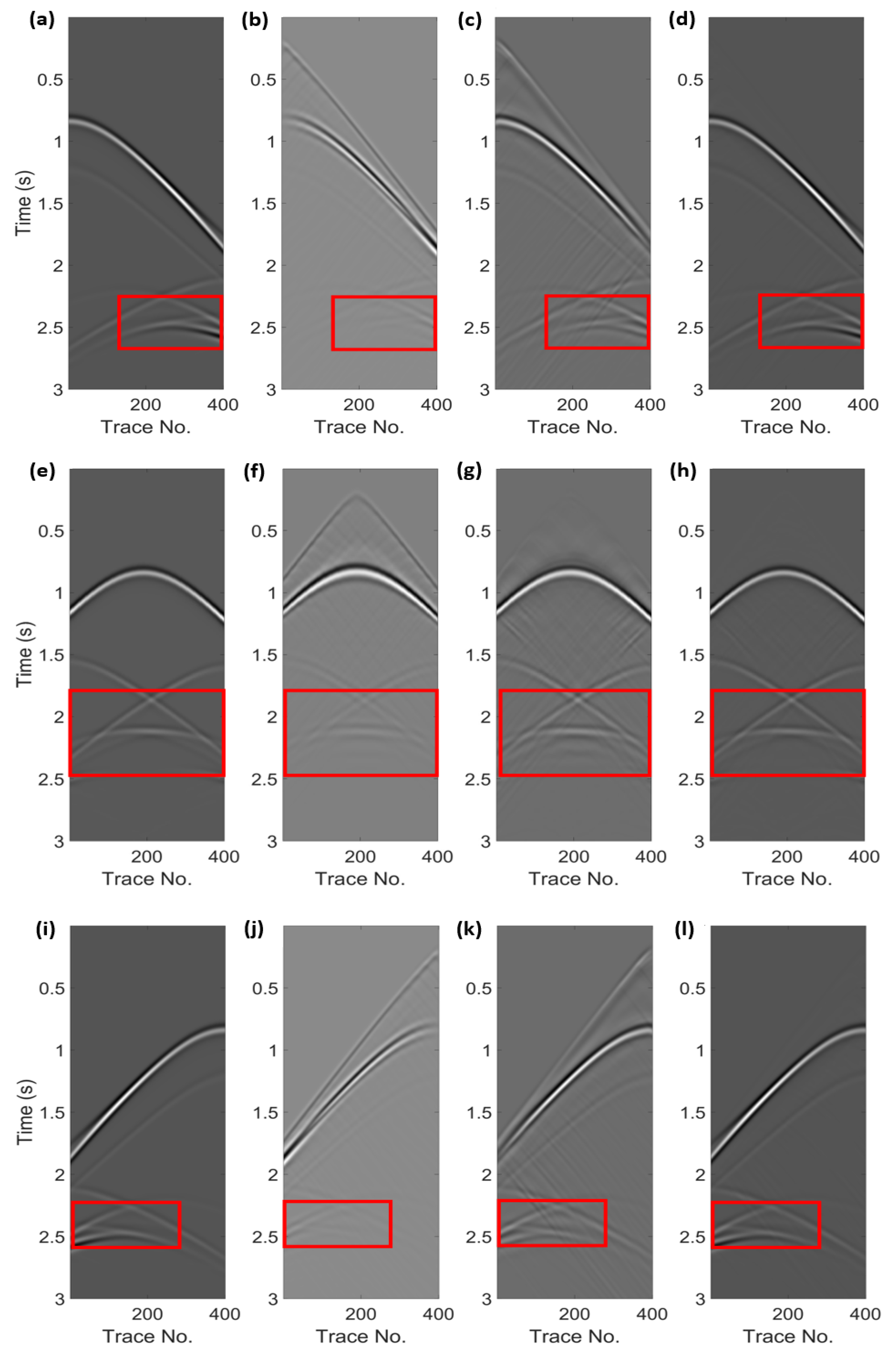
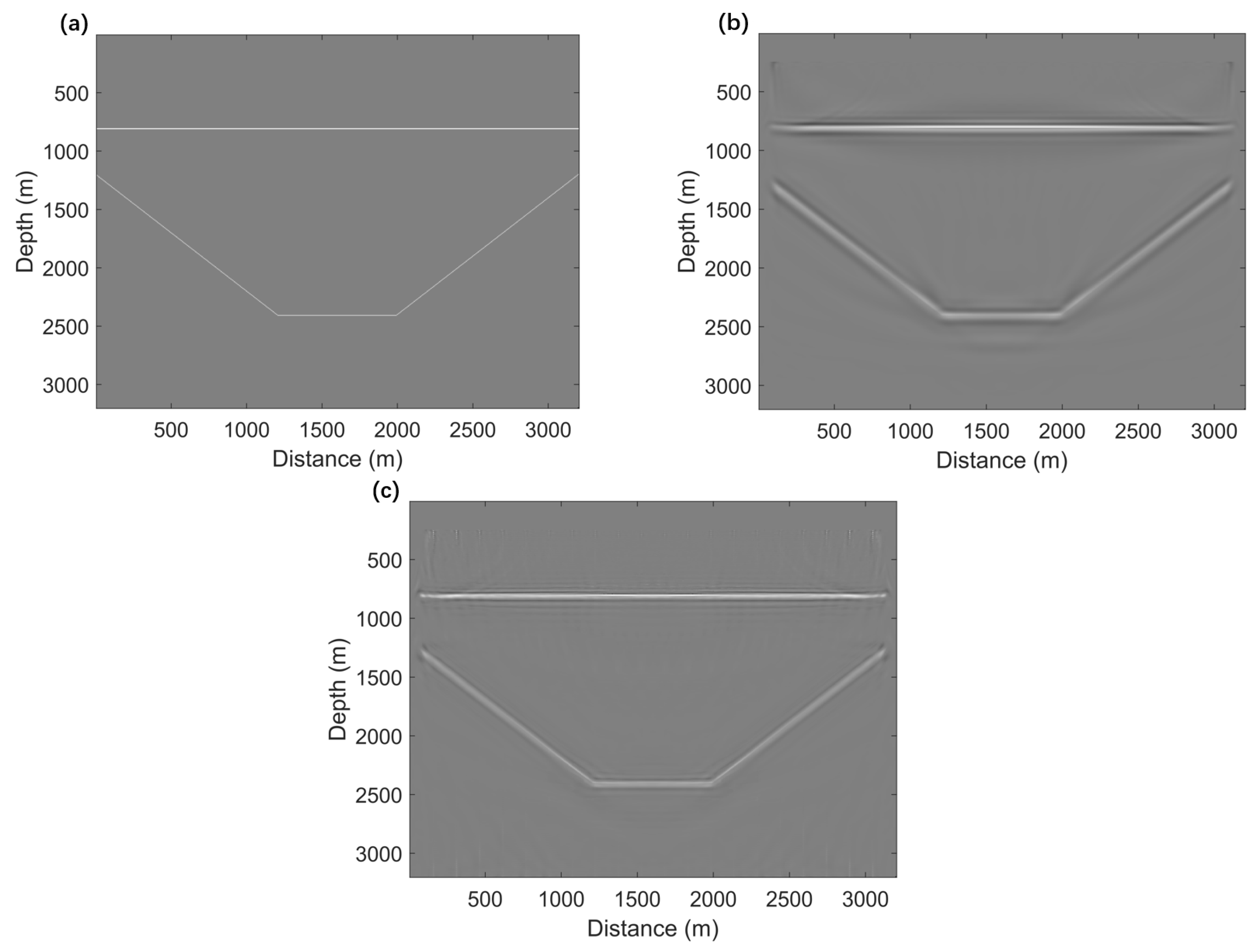
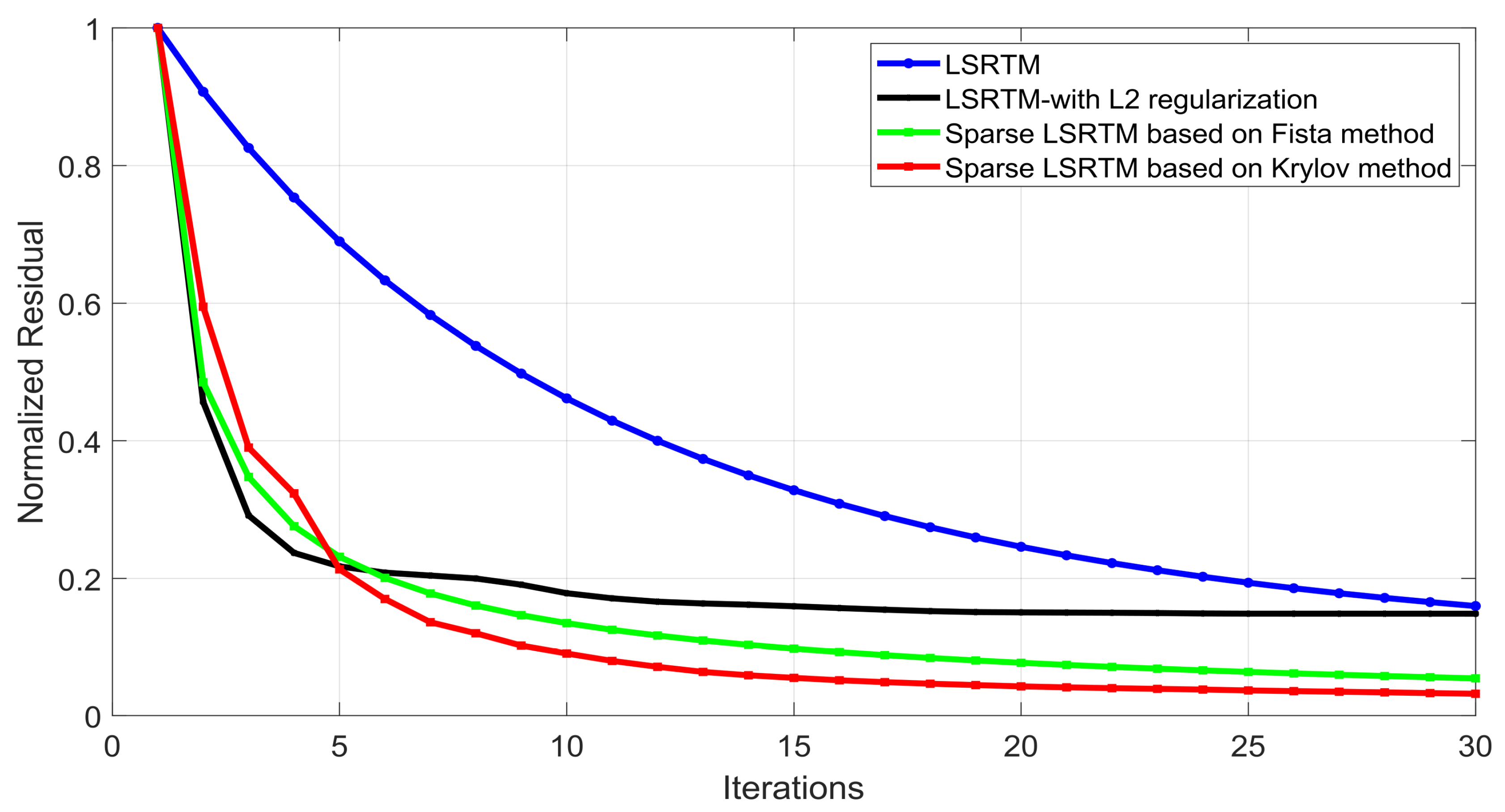
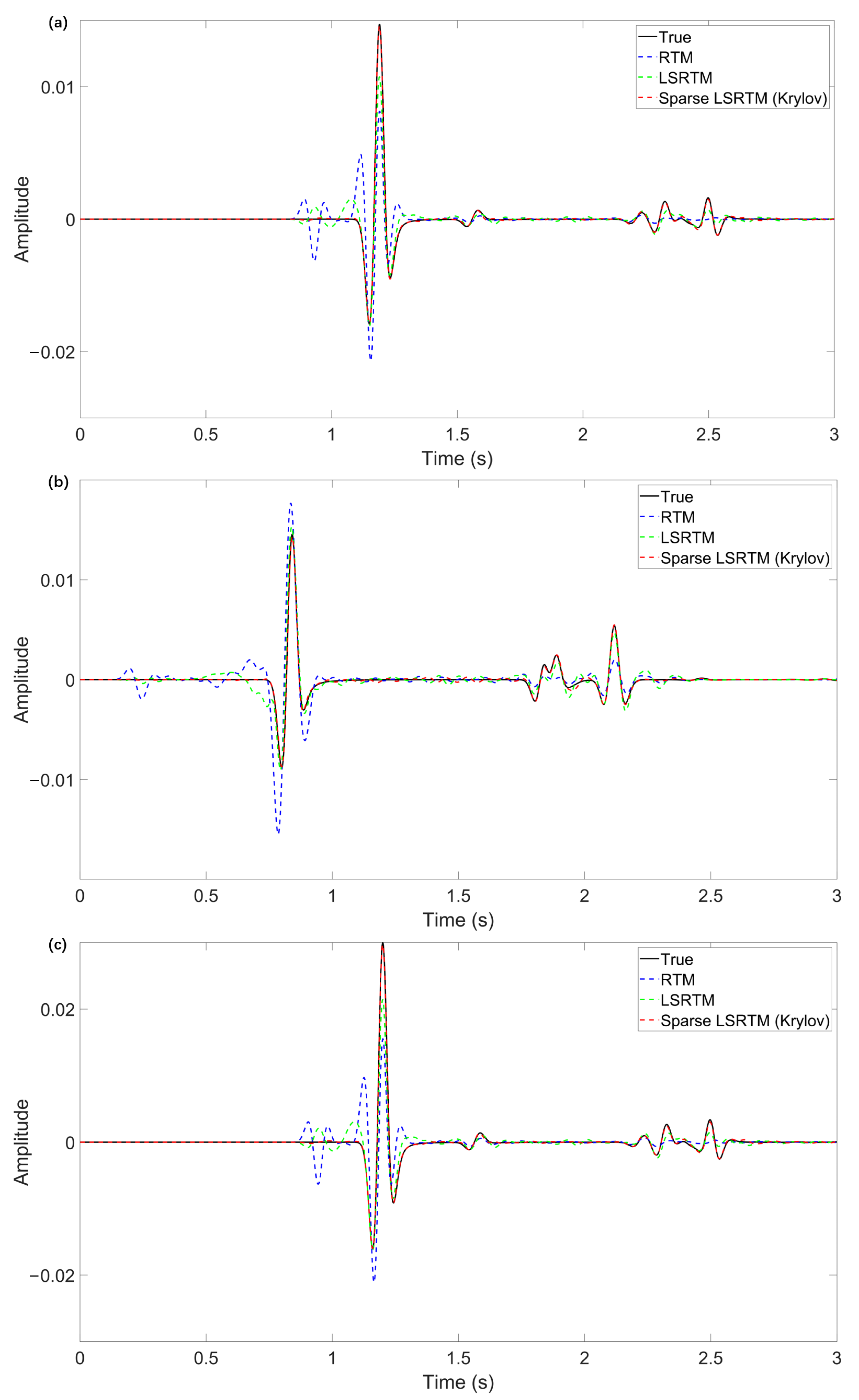
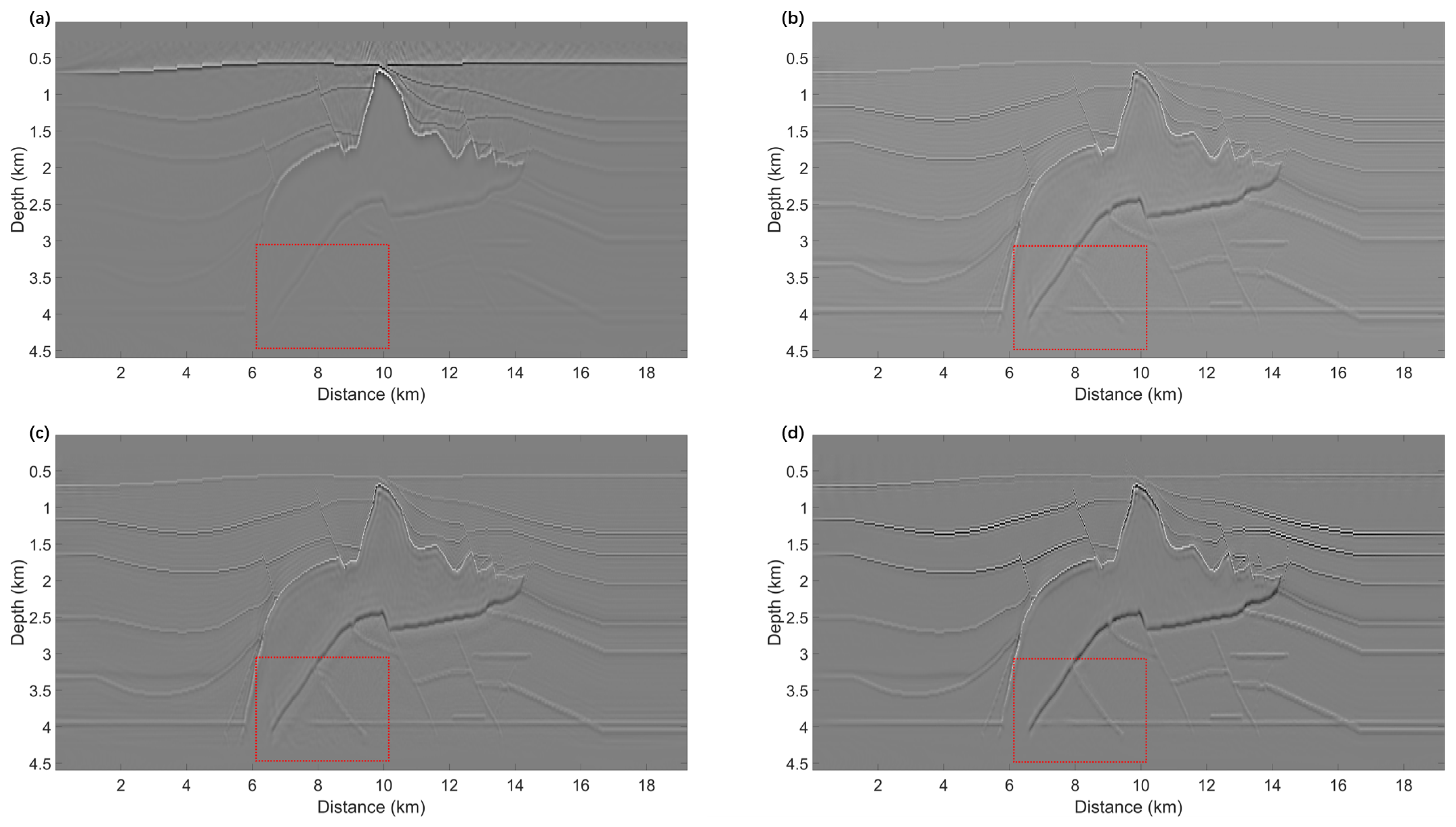
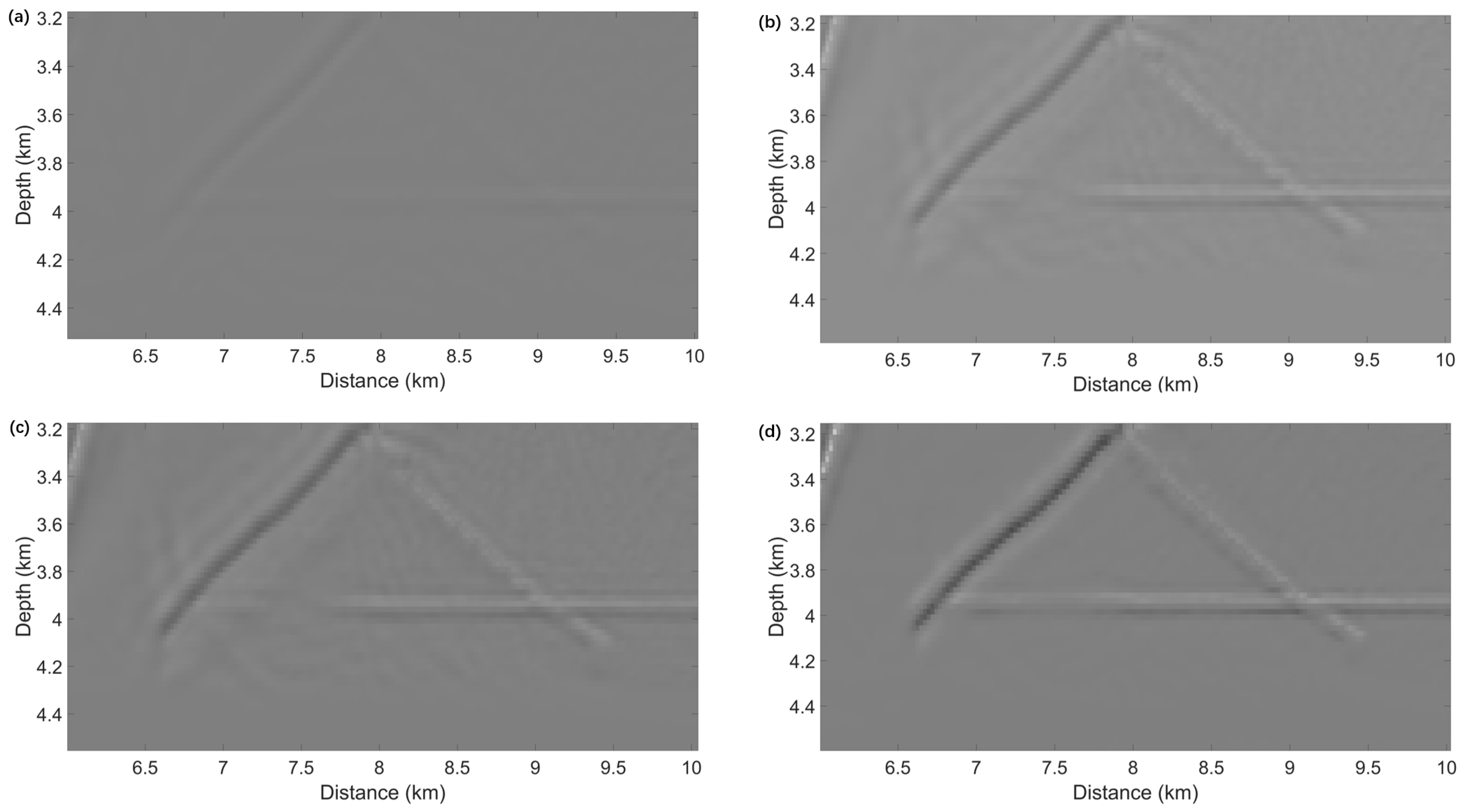
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| (1) Choose a vector (2) For do: (3) Compute for (4) Compute (5) (6) If then Stop (7) (8) End do Through the above loop calculation, the orthogonal vector can be obtained. |
References
- Baysal, E.; Kosloff, D.D.; Sherwood, J. Reverse time migration. Geophysics 1983, 48, 1514–1524. [Google Scholar] [CrossRef]
- McMechan, G.A. Migration by extrapolation of time-dependent boundary values. Geophys. Prospect. 1983, 31, 413–420. [Google Scholar] [CrossRef]
- Whitmore, N.D. Iterative depth migration by backward time propagation. SEG Exp. Abstr. 1983, 382–385. [Google Scholar]
- Yang, K.; Dong, X.; Wang, X.; Zhang, J. Deprimary reverse time migration angle gathers with a stabilized Poynting vector. Geophysics 2022, 87, S267–S279. [Google Scholar] [CrossRef]
- Yang, K.; Zhang, J. The inverse scattering imaging condition for anisotropic reverse time migration. Geophysics 2022, 87, S303–S313. [Google Scholar] [CrossRef]
- Whitmore, N.D.; Crawley, S. Applications of RTM inverse scattering imaging conditions. SEG Exp. Abstr. 2012, SEG-2012-0779. [Google Scholar]
- Liu, F.; Zhang, G.; Morton, S.A.; Leveille, J.P. An effective imaging condition for reverse time migration using wavefield decomposition. Geophysics 2011, 76, S29–S39. [Google Scholar] [CrossRef]
- Yoon, K.; Guo, M.; Cai, J.; Wang, B. 3D RTM angle gathers from source wave propagation direction and dip of reflector. SEG Exp. Abstr. 2011, 3136–3140. [Google Scholar]
- Guo, P.; Guan, H.; McMechan, G.A. Data and model domain up/down wave separation for reverse-time migration with free-surface multiples. Geophys. J. Int. 2020, 223, 77–93. [Google Scholar] [CrossRef]
- Lyu, B.; Nakata, N. Iterative passive-source location estimation and velocity inversion using geometric-mean reverse-time migration and full-waveform inversion. Geophys. J. Int. 2020, 223, 1935–1947. [Google Scholar] [CrossRef]
- Liu, X.; Liu, Y. Deghosting-based reverse time migration with free-surface multiples. Geophys. J. Int. 2019, 216, 1191–1200. [Google Scholar] [CrossRef]
- Sun, D.; Jiao, K.; Cheng, X.; Vigh, D. Compensating for source and receiver ghost effects in full waveform inversion and reverse time migration for marine streamer data. Geophys. J. Int. 2015, 201, 1507–1521. [Google Scholar]
- Schuster, G.T. Least-squares cross-well migration. SEG Exp. Abstr. 1993, 110–113. [Google Scholar]
- Dutta, G. Sparse least-squares reverse time migration using seislets. J. Appl. Geophys. 2017, 136, 142–155. [Google Scholar] [CrossRef]
- Tarantola, A. Inversion of seismic reflection data in the acoustic approximation. Geophysics 1984, 49, 1259–1266. [Google Scholar] [CrossRef]
- Davydenko, M.; Verschuur, E. Full wavefield least-squares reverse time migration. Geophysics 2021, 86, WC67–WC74. [Google Scholar] [CrossRef]
- Feng, Z.; Huang, L. Shear reflectivity compensation in full-waveform inversion using least-squares reverse-time migration. Geophys. J. Int. 2021, 227, 1–15. [Google Scholar] [CrossRef]
- Ren, Z.; Liu, Y.; Sen, M.K. Least-squares reverse time migration in elastic media. Geophys. J. Int. 2017, 208, 1103–1125. [Google Scholar] [CrossRef]
- Rocha, D.; Sava, P. Elastic least-squares reverse time migration using the energy norm. Geophysics 2018, 83, S237–S248. [Google Scholar] [CrossRef]
- Chen, Y.; Dutta, G.; Dai, W.; Schuster, G.T. Q-least-squares reverse time migration with viscoacoustic deblurring filters. Geophysics 2017, 82, S425–S438. [Google Scholar] [CrossRef]
- Qu, Y.; Zhu, J.; Chen, Z.; Huang, C.; Wang, Y.; Liu, C. Q-compensated least-squares reverse time migration with velocity-anisotropy correction based on the first-order velocity-pressure equations. Geophysics 2022, 87, S335–S350. [Google Scholar] [CrossRef]
- Yang, J.; Zhu, H.; McMechan, G.; Zhang, H.; Zhao, Y. Elastic least-squares reverse time migration in vertical transverse isotropic media. Geophysics 2019, 84, S539–S553. [Google Scholar] [CrossRef]
- Qu, Y.; Huang, J.; Li, Z.; Guan, Z.; Li, J. Attenuation compensation in anisotropic least-squares reverse time migration. Geophysics 2017, 82, S411–S423. [Google Scholar] [CrossRef]
- Romero, L.A.; Ghiglia, D.C.; Ober, C.C.; Morton, S.A. Phase encoding of shot records in prestack migration. Geophysics 2000, 65, 426–436. [Google Scholar] [CrossRef]
- Dutta, G.; Giboli, M.; Williamson, P.; Schuster, G.T. Least-squares reverse time migration with factorization-free priorconditioning. SEG Exp. Abstr. 2015, 4270–4275. [Google Scholar]
- Dai, W.; Wang, X.; Schuster, G.T. Least-squares migration of multisource data with a deblurring filter. Geophysics 2011, 76, R135–R146. [Google Scholar] [CrossRef]
- Li, X.; Esser, E.; Herrmann, F.J. Modified Gauss-Newton full-waveform inversion explained—Why sparsity-promoting updates do matter. Geophysics 2016, 81, R125–R138. [Google Scholar] [CrossRef]
- Wu, D.; Yao, G.; Cao, J. Least-squares RTM with L1 norm regularization. J. Geophys. Eng. 2016, 13, 666–673. [Google Scholar] [CrossRef]
- Yao, G.; Wu, B.; Silva, N.d. Least-squares reverse time migration with a multiplicative Cauchy constraint. Geophysics 2022, 87, S151–S167. [Google Scholar] [CrossRef]
- Li, F.; Gao, J.; Gao, Z.; Jiang, X.; Sun, W. Least-squares reverse time migration with sparse regularization in the 2D wavelet domain. Geophysics 2020, 85, S313–S325. [Google Scholar] [CrossRef]
- Dutta, G.; Giboli, M.; Agut, C.; Williamson, P.; Schuster, G.T. Least-squares reverse time migration with local Radon-based preconditioning. Geophysics 2017, 82, S75–S84. [Google Scholar] [CrossRef]
- Zand, T.; Siahkoohi, H.R. Sparsity-promoting least-squares reverse time migration via preconditioned Bregmanized operator splitting. Geophys. Prospect. 2021, 69, 727–744. [Google Scholar] [CrossRef]
- Zand, T.; Ghasemzadeh, H.; Gholami, A.; Malcolm, A. Least-squares reverse time migration with shifted total variation regularization. Geophysics 2023, 88, S59–S69. [Google Scholar] [CrossRef]
- Hansen, P.C. Analysis of discrete ill-posed problems by means of the L-curve. SIAM Rev. 1992, 34, 561–580. [Google Scholar] [CrossRef]
- Jansen, M.; Malfait, M.; Bultheel, A. Generalized cross validation for wavelet thresholding. Signal Process. 1997, 56, 33–44. [Google Scholar] [CrossRef]
- Morikuni, K.; Hayami, K. Inner-iteration Krylov subspace methods for least squares problems. SIAM J. Matrix Anal. Appl. 2013, 34, 1–22. [Google Scholar] [CrossRef]
- Gazzola, S.; Wiaux, Y. Fast nonnegative least squares through flexible Krylov subspaces. SIAM J. Sci. Comput. 2017, 39, A655–A679. [Google Scholar] [CrossRef]
- Chung, J.; Gazzola, S. Computational methods for large-scale inverse problems: A survey on hybrid projection methods. Siam Rev. 2024, 66, 205–284. [Google Scholar] [CrossRef]
- McKenzie, T.; Naheed, N. Deblurring icf images using hybrid iterative regularization techniques. In Proceedings of the 2023 International Conference on Computational Science and Computational Intelligence (CSCI), Las Vegas, NV, USA, 13–15 December 2023; IEEE: New York, NY, USA, 2023; pp. 1271–1274. [Google Scholar]
- Pasha, M.; Kupis, S.; Ahmad, S.; Khan, T. A krylov subspace type method for electrical impedance tomography. ESAIM Math. Model. Numer. Anal. 2021, 55, 2827–2847. [Google Scholar] [CrossRef]
- Gazzola, S.; Landman, M. Krylov methods for inverse problems: Surveying classical, and introducing new, algorithmic approaches. GAMM Mitteilungen 2020, 43, 202000017. [Google Scholar] [CrossRef]
- Chung, J.; Gazzola, S. Flexible Krylov Methods for lp Regularization. SIAM J. Sci. Comput. 2019, 41, S149–S171. [Google Scholar] [CrossRef]
- Guitton, A. Amplitude and kinematic corrections of migrated images for nonunitary imaging operators. Geophysics 2004, 69, 877–1103. [Google Scholar] [CrossRef]
- Xu, S.; Wang, D.; Chen, F.; Lambare, G.; Zhang, Y. Inversion on reflected seismic wave. SEG Exp. Abstr. 2012, 1–7. [Google Scholar]
- Symes, W. Migration velocity analysis and waveform inversion. Geophys. Prospect. 2008, 56, 765–790. [Google Scholar] [CrossRef]
- Beck, A.; Teboulle, M. A fast iterative shrinkage-thresholding algorithm for linear inverse problems. SIAM J. Imaging Sci. 2009, 2, 183–202. [Google Scholar] [CrossRef]
- Gazzola, S.; Nagy, J.G. Generalized Arnoldi-Tikhonov Method for Sparse Reconstruction. SIAM J. Sci. Comput. 2014, 36, B225–B247. [Google Scholar] [CrossRef]
- Gazzola, S.; Novati, P.; Russo, M.R. On Krylov projection methods and Tikhonov regularization. Electron. T. Numer. Ana. 2015, 44, 83–123. [Google Scholar]
- Paige, C.C.; Saunders, M.A. LSQR: An algorithm for sparse linear equations and sparse least squares. ACM T. Math. Softw. 1982, 8, 43–71. [Google Scholar] [CrossRef]
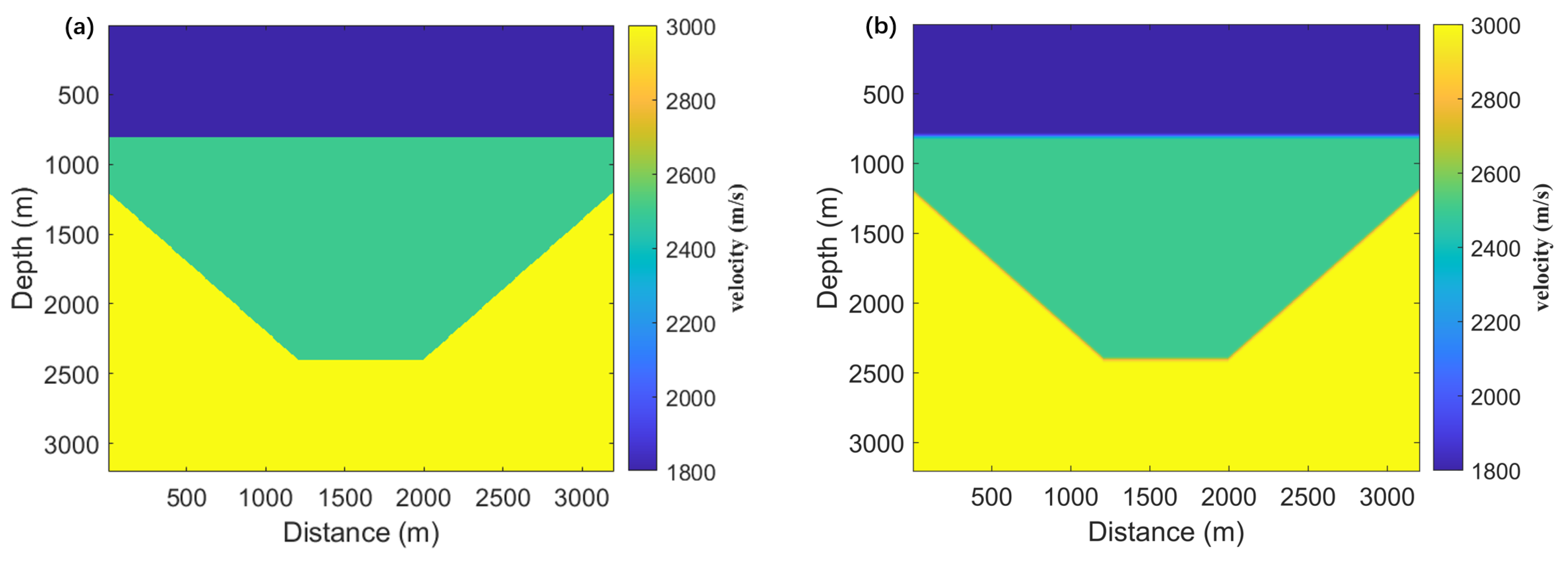


| Model parameter | Mesh | Rectangular grid with a grid size of 400 × 400 and a grid spacing of 8 m |
| Velocity distribution | From 1800 m/s to 3000 m/s, refer to Figure 1 for detail | |
| Observation system | Position | At the shallow surface |
| Source | The Ricker wavelet with a main frequency of 10 Hz excited a total of 20 shots | |
| Seismic forward modeling parameters | Wave field continuation | Finite Difference |
| Propagation time | Propagation time Recorded duration of 3000 ms, sampling interval of 1 ms | |
| Wave equation | Acoustic wave Wave equation, assuming the density is constant | |
| Boundary | Sponge boundary condition |
| Model parameter | Mesh | Rectangular grid with a grid size of 190 × 800 and a grid spacing of 24 m |
| Velocity distribution | From 1500 m/s to 4500 m/s, refer to Figure 6 for detail | |
| Observation system | Position | At the shallow surface |
| Source | The Ricker wavelet with a main frequency of 10 Hz excited a total of 48 shots | |
| Seismic forward modeling parameters | Wave field continuation | Finite Difference |
| Propagation time | Propagation time Recorded duration of 8800 ms, sampling interval of 1 ms | |
| Wave equation | Acoustic wave Wave equation, assuming the density is constant | |
| Boundary | Sponge boundary condition |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, G.; Gong, X.; Wang, S.; Cao, Z.; Xu, Z. Sparse Regularization Least-Squares Reverse Time Migration Based on the Krylov Subspace Method. Remote Sens. 2025, 17, 847. https://doi.org/10.3390/rs17050847
Peng G, Gong X, Wang S, Cao Z, Xu Z. Sparse Regularization Least-Squares Reverse Time Migration Based on the Krylov Subspace Method. Remote Sensing. 2025; 17(5):847. https://doi.org/10.3390/rs17050847
Chicago/Turabian StylePeng, Guangshuai, Xiangbo Gong, Shuang Wang, Zhiyu Cao, and Zhuo Xu. 2025. "Sparse Regularization Least-Squares Reverse Time Migration Based on the Krylov Subspace Method" Remote Sensing 17, no. 5: 847. https://doi.org/10.3390/rs17050847
APA StylePeng, G., Gong, X., Wang, S., Cao, Z., & Xu, Z. (2025). Sparse Regularization Least-Squares Reverse Time Migration Based on the Krylov Subspace Method. Remote Sensing, 17(5), 847. https://doi.org/10.3390/rs17050847






