Approximate Near-Real-Time Assessment of Some Characteristic Parameters of the Spring Ozone Depletion over Antarctica Using Ground-Based Measurements
Abstract
1. Introduction
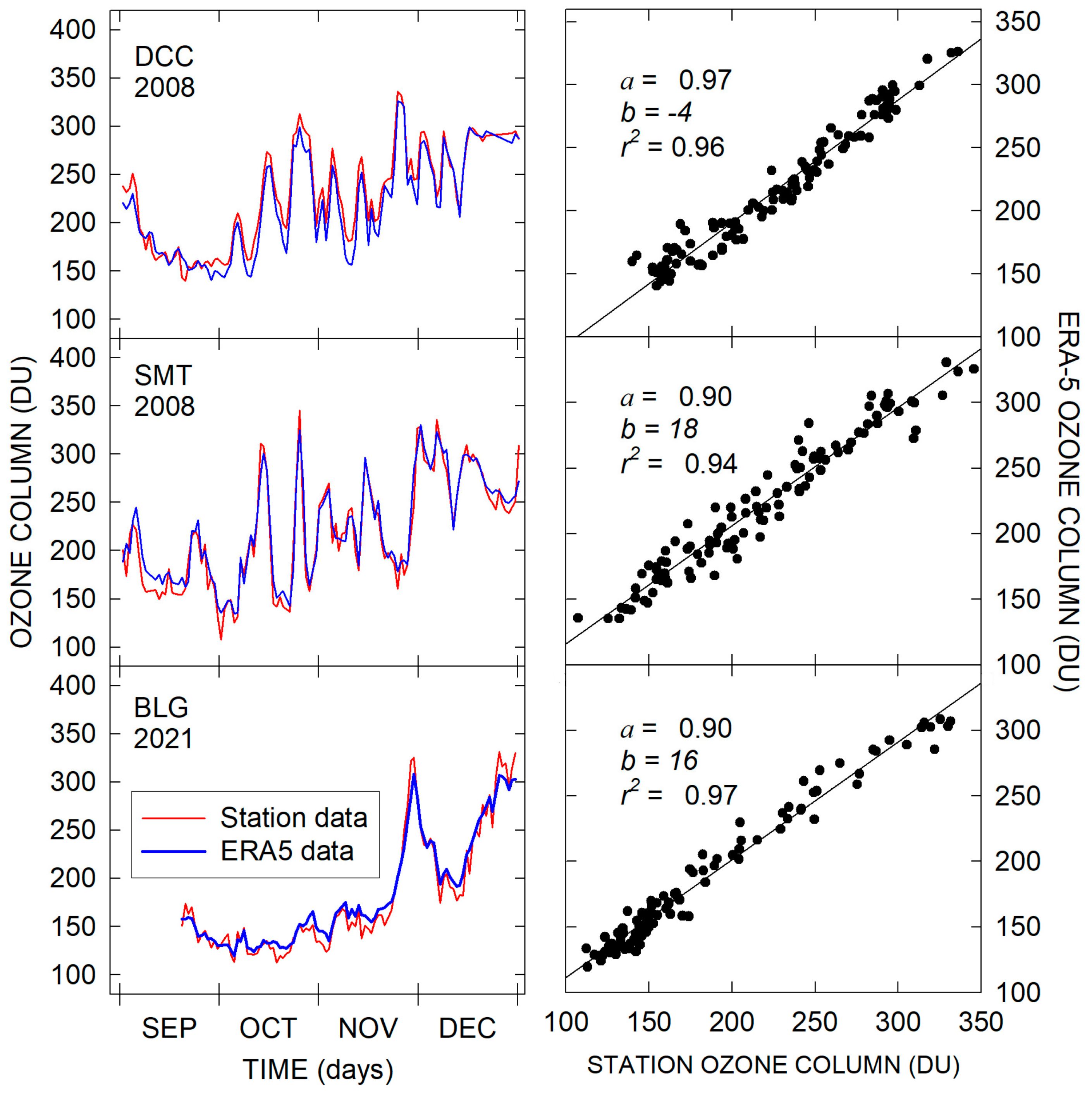
2. Data and Methods
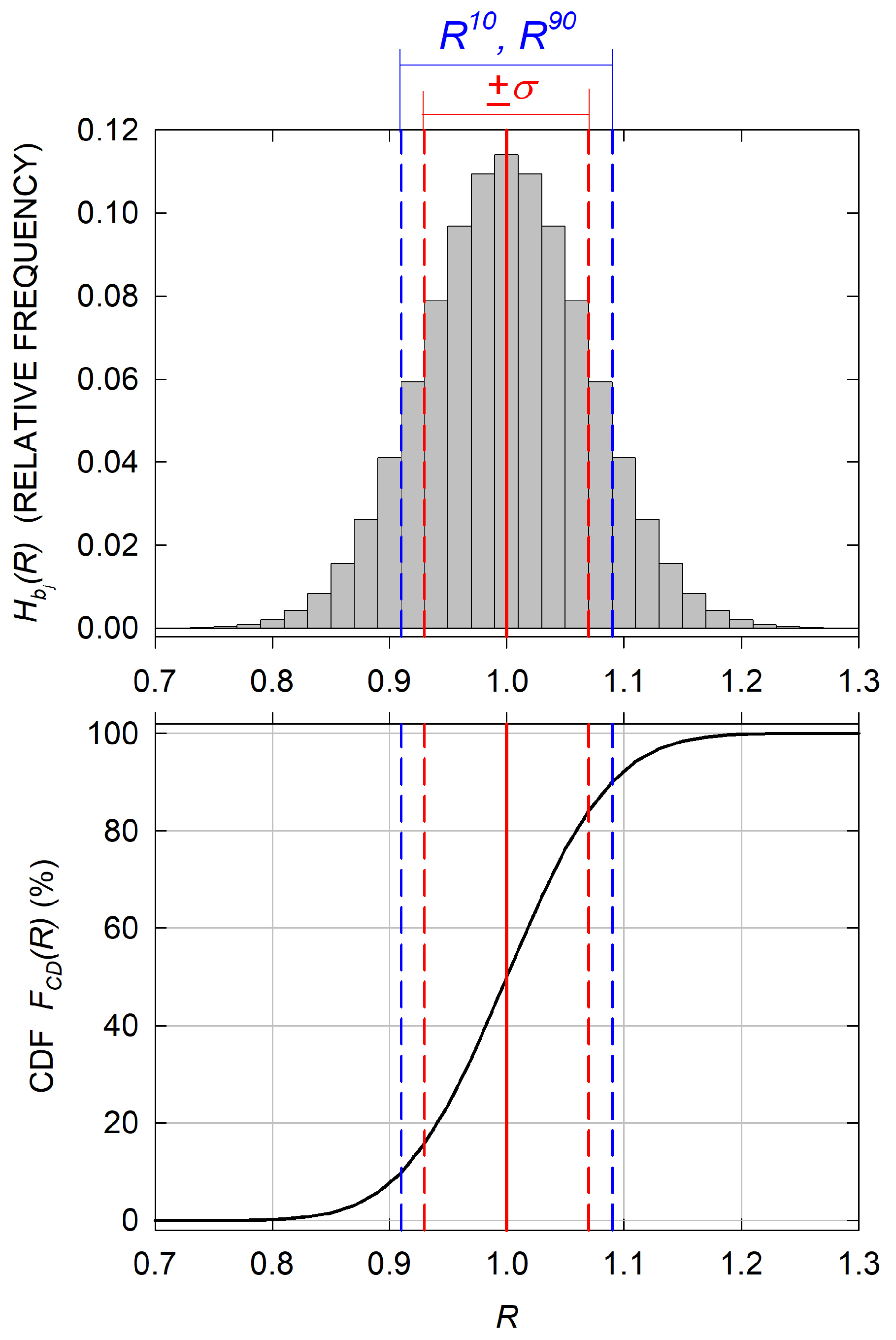
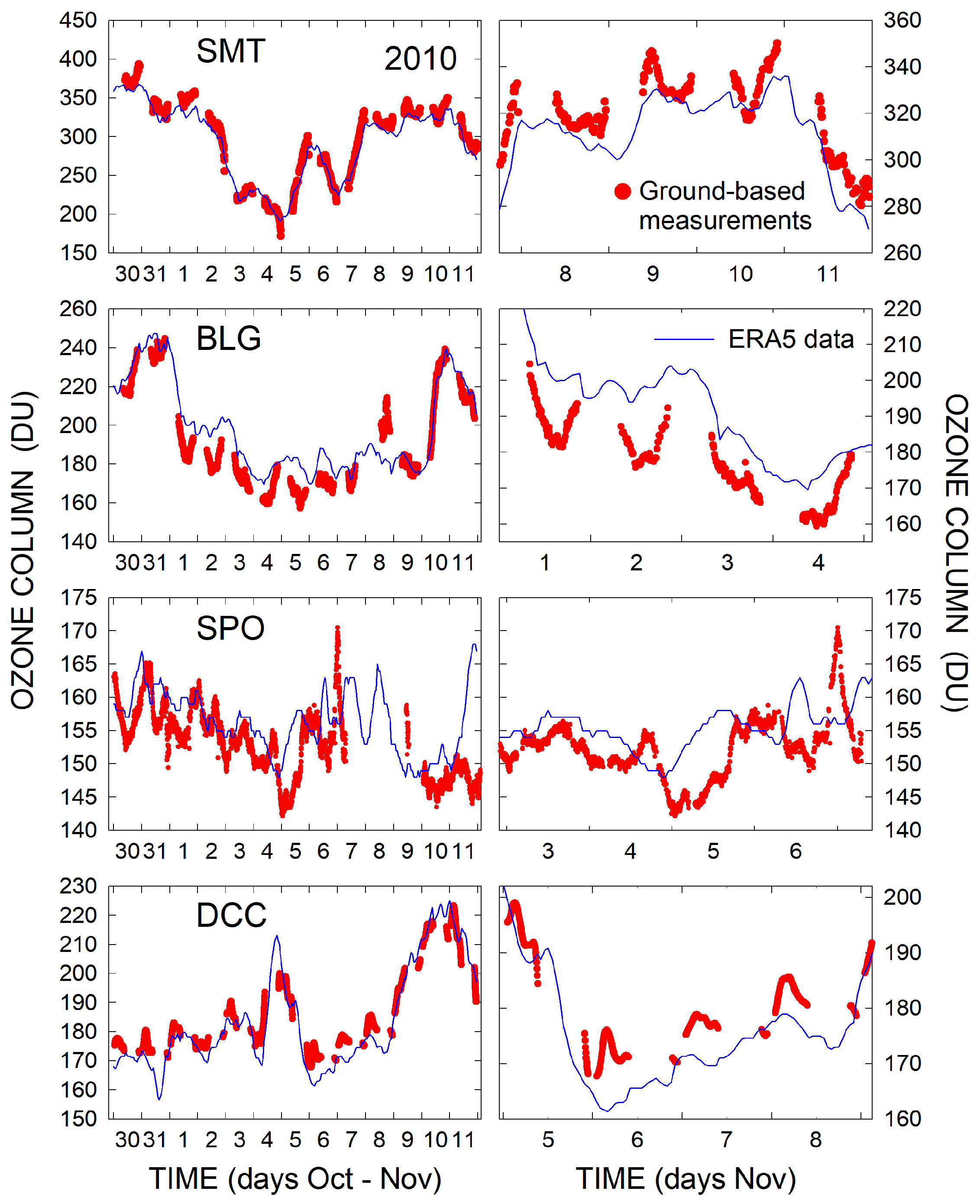
3. Preliminary Data Analysis and Basis of the Study
3.1. Features of the Ozone Column Distributions over Antarctica
3.2. Symmetry in the Zonal Average Ozone Distributions
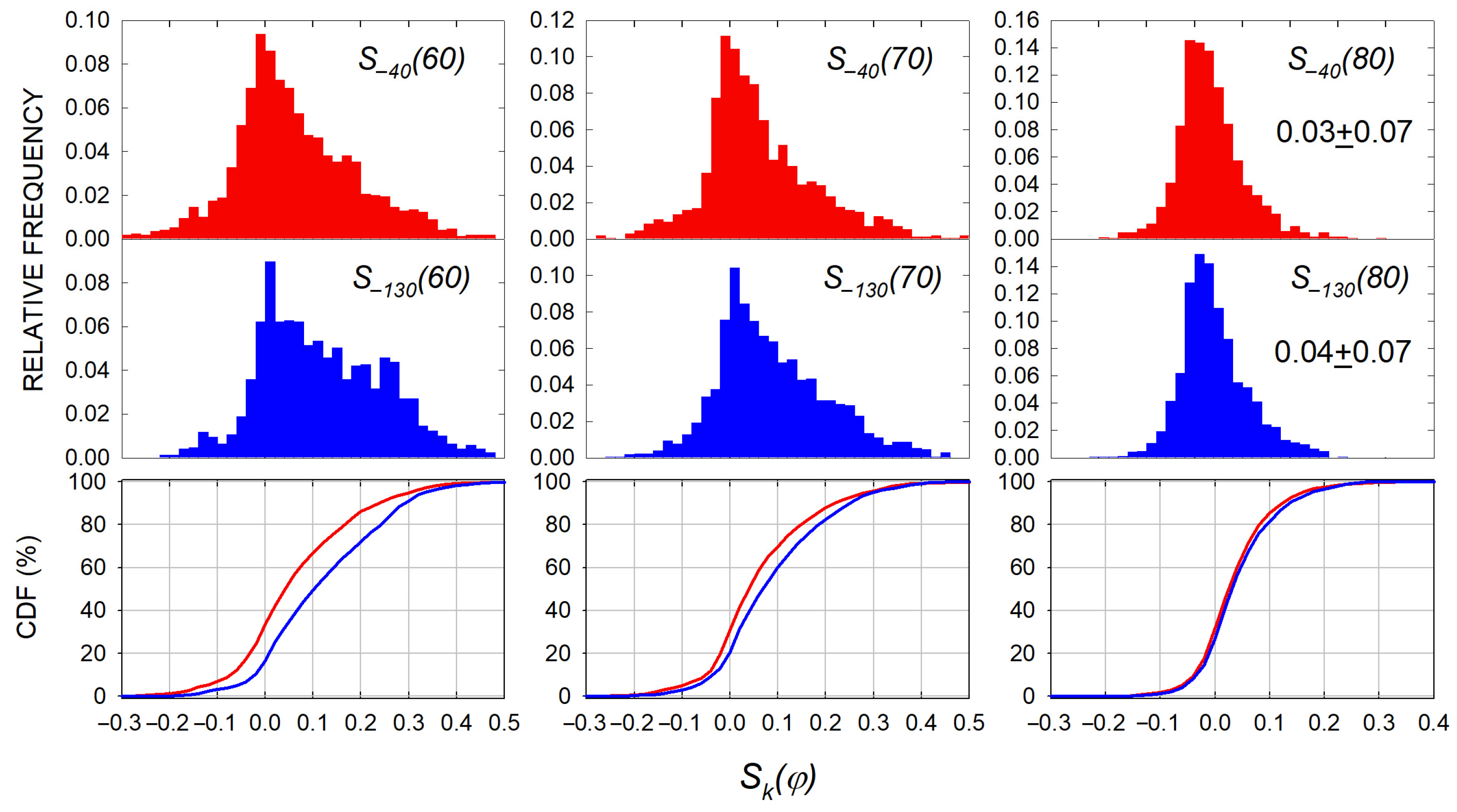
4. Results and Discussion
4.1. Approximation of the Average Ozone Column over Antarctica by the Mean of Station Values
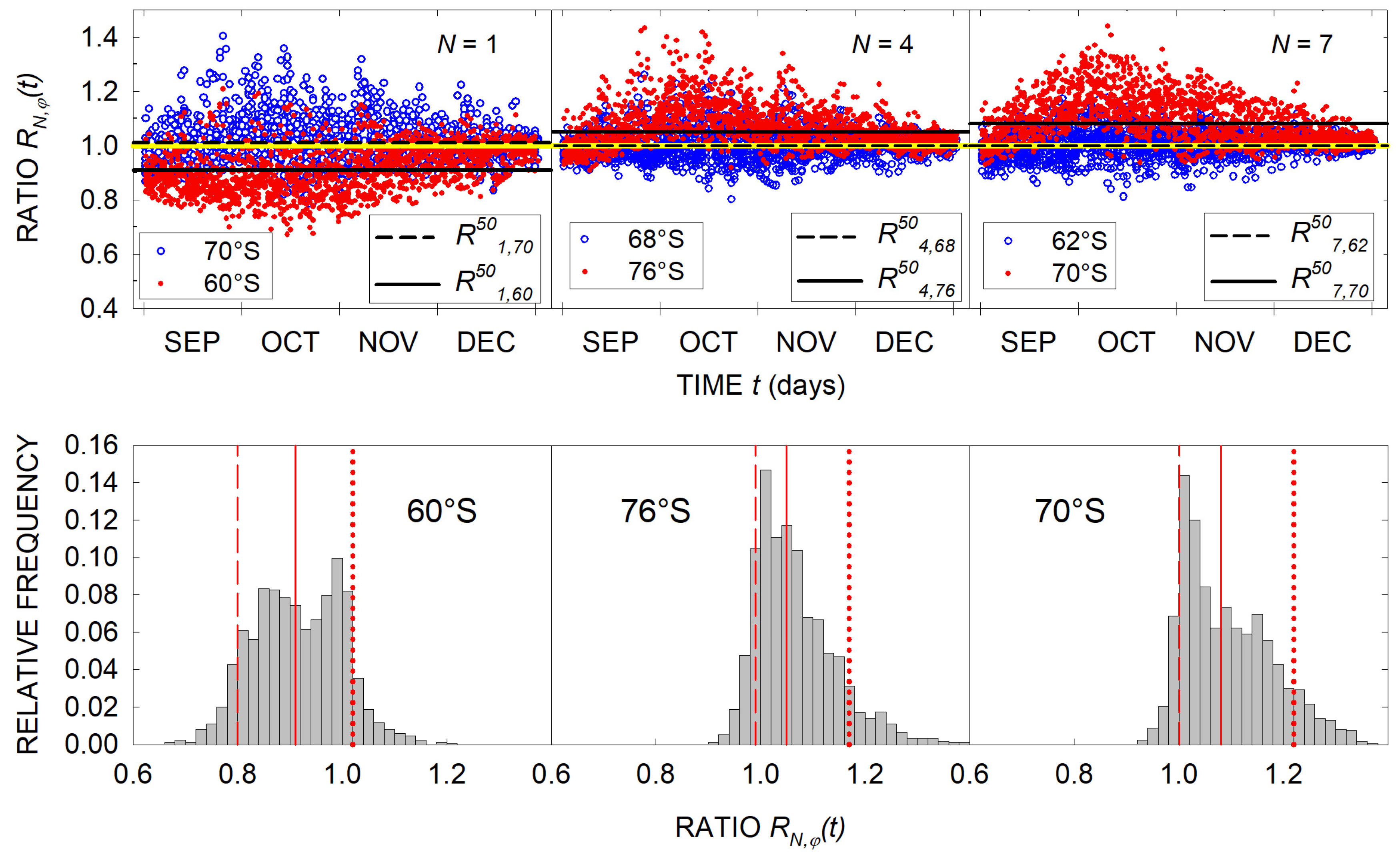
4.2. Determining the Zones
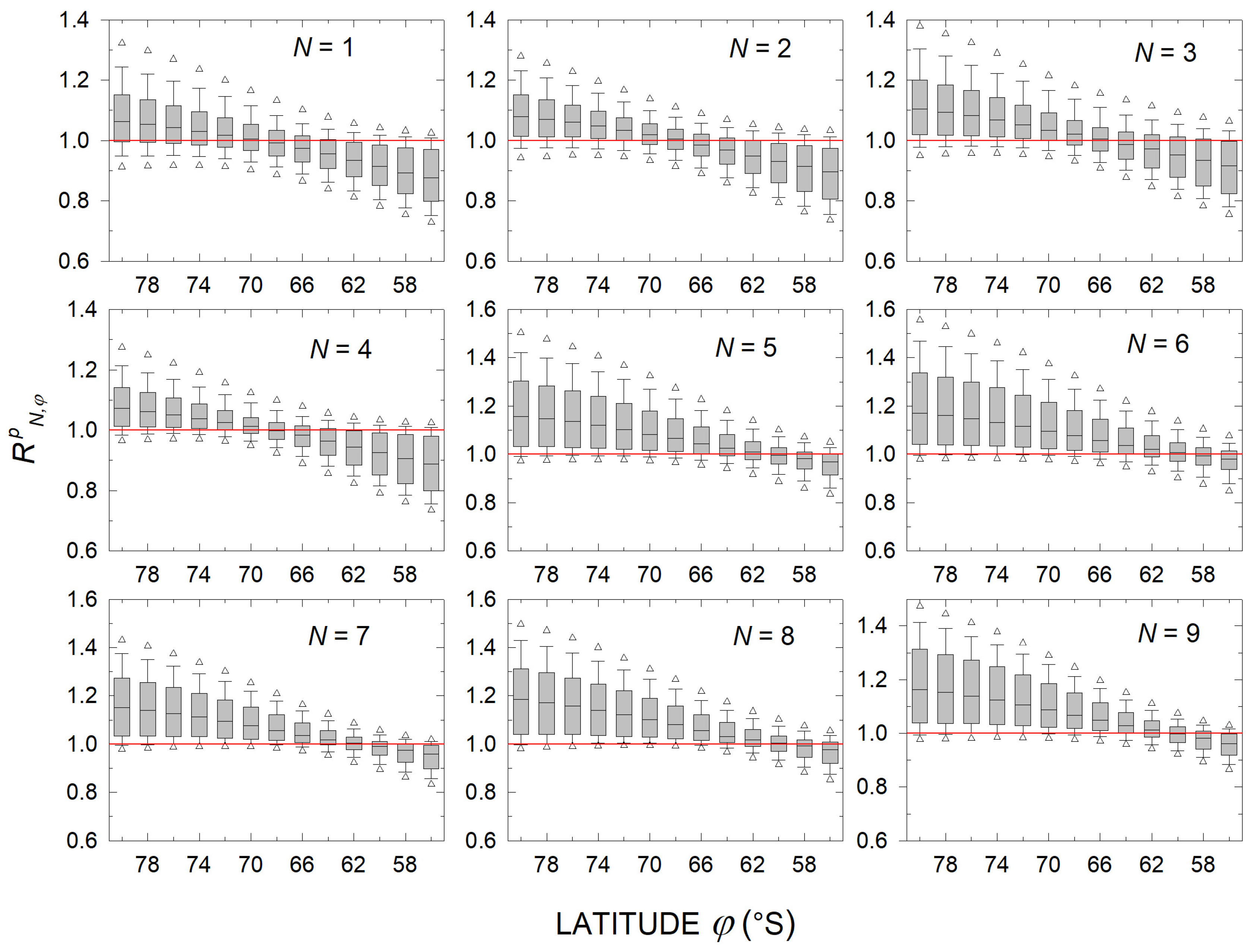



4.3. Deepness of the Ozone Deficit Assessed by the Zonal-Averaged Ozone Column
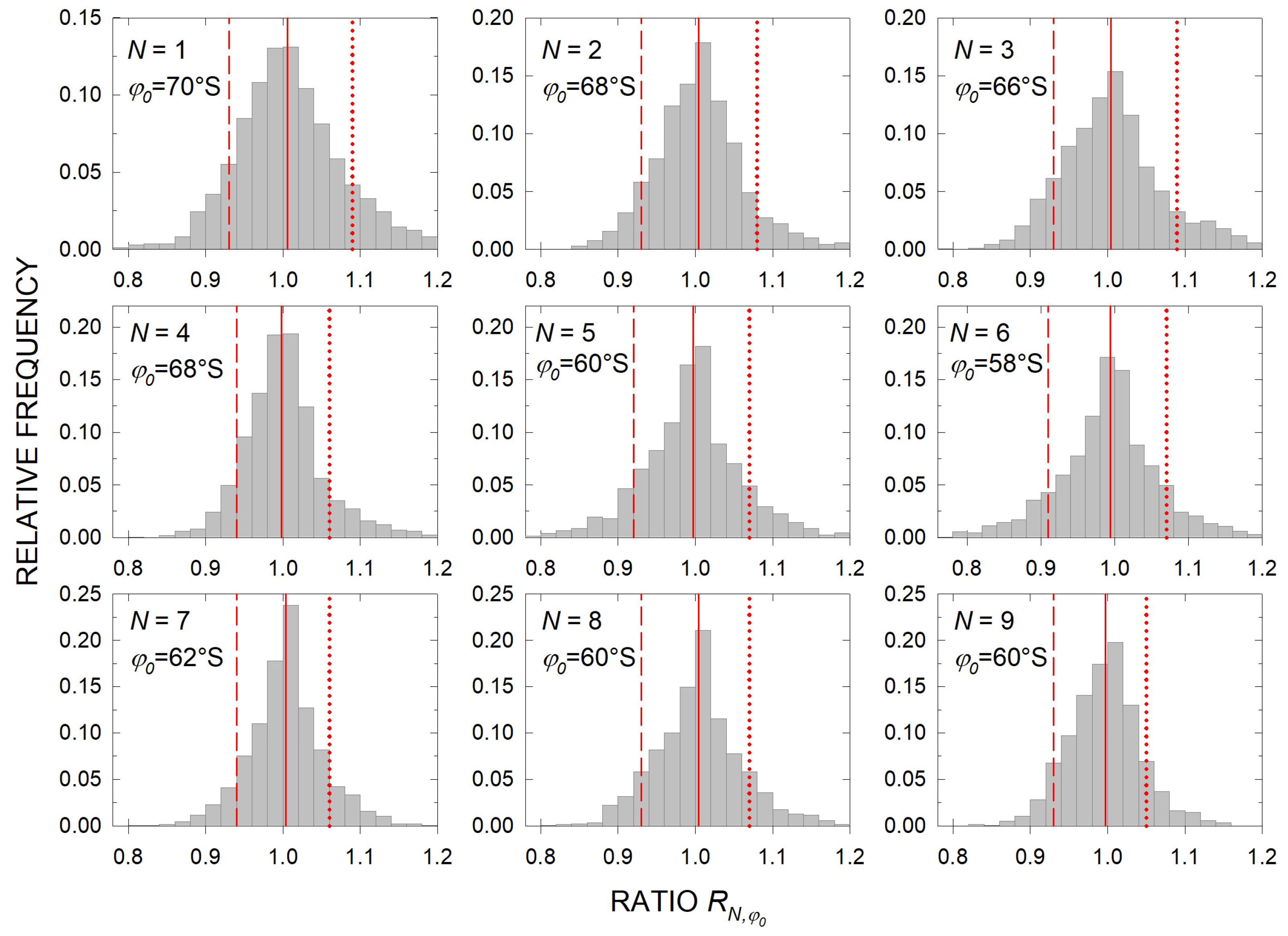
4.4. Estimation of the Ratios Using the Available Ground-Based Dataset
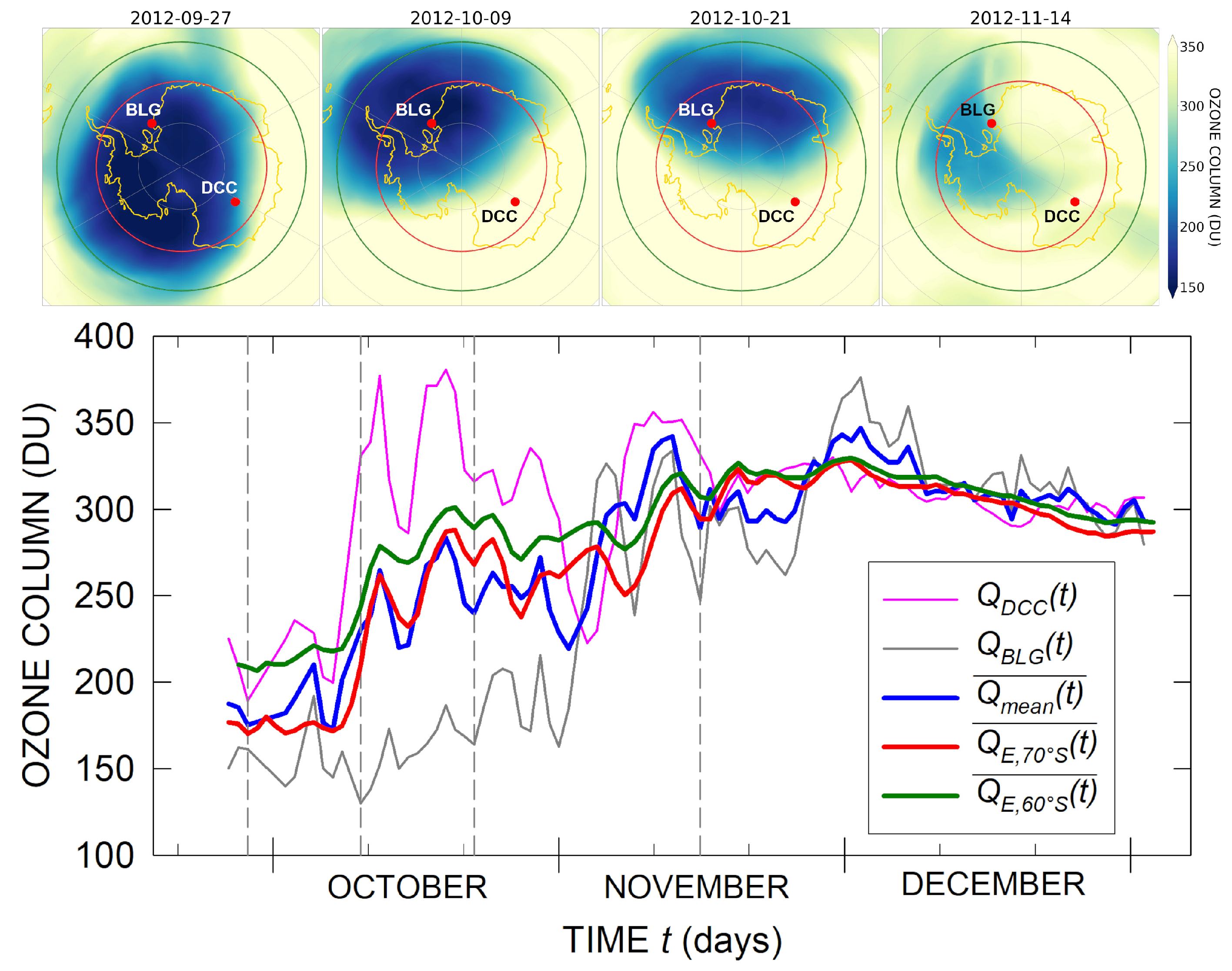
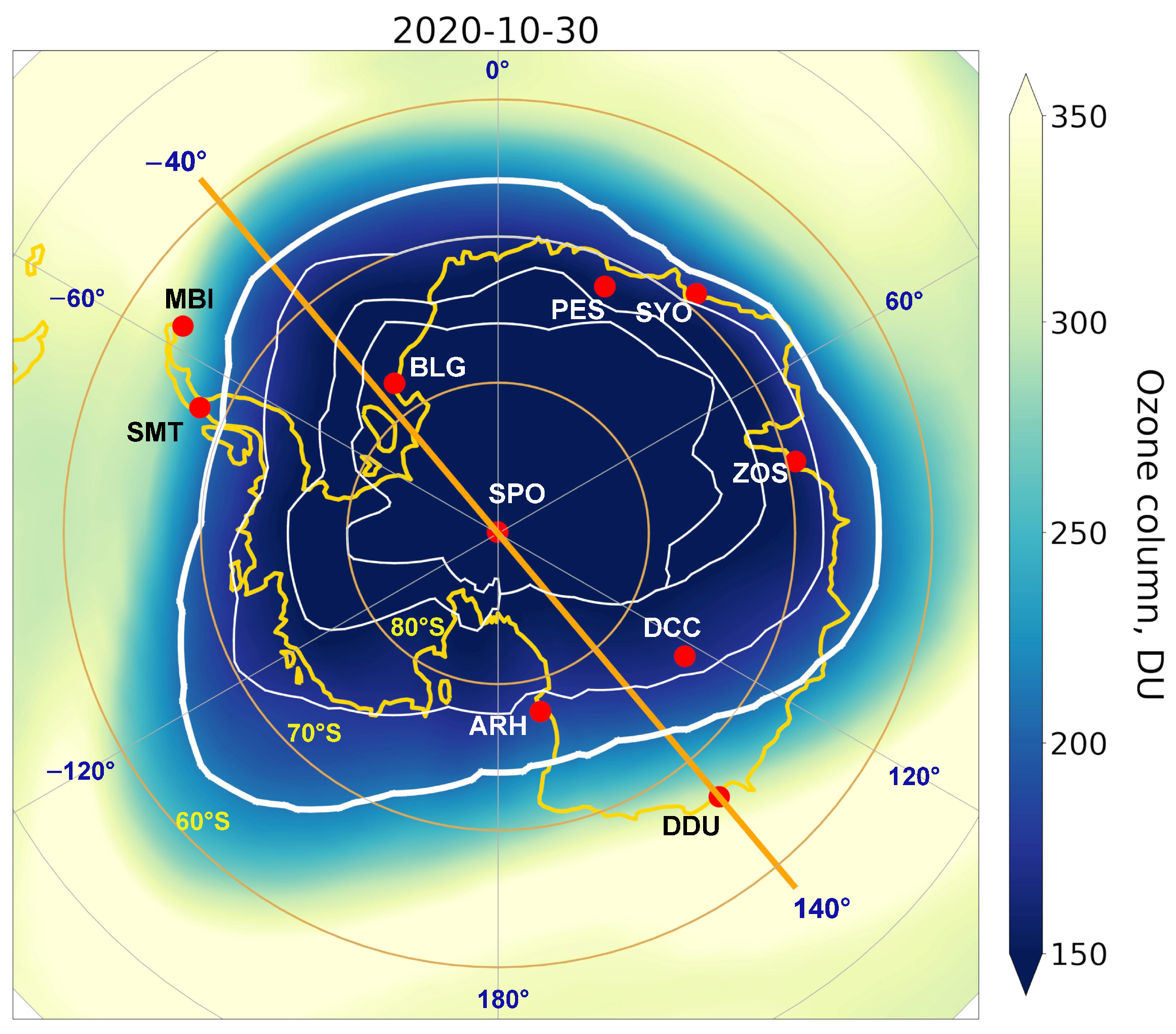
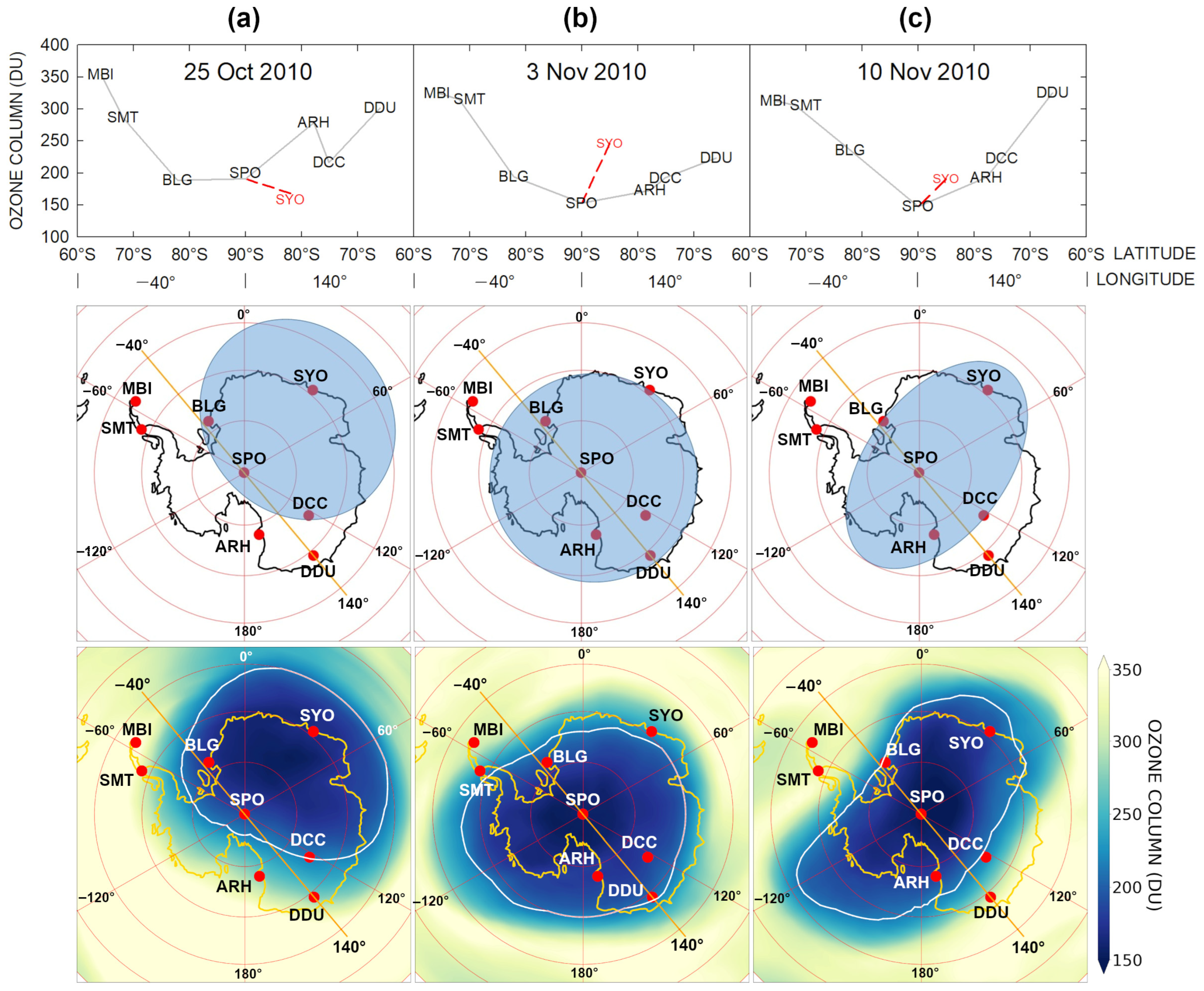
4.5. Reconstruction of the Ozone Hole Position and Shape from Ground-Based Measurements
4.6. A Possible Contribution of Ground-Based Measurements to Studying Short-Term Pulsations in the Ozone Hole
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Station | Number of Measurements | |
|---|---|---|
| PES | ||
| SYO | ||
| ZOS | ||
| DDU | ||
| ARH | ||
| DCC | ||
| SPO | ||
| BLG | ||
| SMT | ||
| MBI |
References
- Bojkov, R. International Ozone Commission: History and Activities, IAMAS Publication Series No. 2., Oberpfaffenhofen, Germany. 2012. Available online: https://www.iamas.org/wp-content/uploads/2019/06/IAMAS-PubSer-No2.pdf (accessed on 22 March 2024).
- Cornu, A. Sur la limite ultraviolette du spectre solaire. Compt. Rendus Acad. Sci. 1879, 88, 1101. [Google Scholar]
- Hartley, W.N. On the absorption spectrum of ozone. J. Chem. Soc. 1881, 39, 57. [Google Scholar] [CrossRef]
- Hartley, W.N. On the absorption of solar rays by atmospheric ozone. J. Chem. Soc. 1881, 39, 111. [Google Scholar] [CrossRef]
- Fabry, C.; Buisson, H. L’absorption de l’ultraviolet par l’ozone et la limite du spectre solaire. J. Phys. 1913, 3, 196–206. [Google Scholar]
- Fabry, C.; Buisson, H. Etude de l’extremite ultra-violette du spectre solaire. J. Phys. 1921, 2, 197–226. [Google Scholar] [CrossRef][Green Version]
- Dobson, G.M.B.; Harrison, D.N.; Lawrence, J. Observations of the amount of ozone in the earth’s atmosphere and its relation to other geophysical conditions. Part III Proc. R. Soc. Lond. 1929, A122, 456–486. [Google Scholar]
- Lindemann, F.A.; Dobson, G.M.B. A theory of Meteors, and the density and temperature of the outer atmosphere to which it leads. Proc. Roy. Soc. Lond. 1922, A102, 411–437. [Google Scholar]
- Chapman, S. A theory of upper atmospheric ozone. Mem. R. Meteorol. Soc. 1930, 3, 103–125. [Google Scholar]
- Brasseur, G.P.; Solomon, S. Aeronomy of the Middle Atmosphere, 5th ed.; Springer: Dordrecht, The Netherlands, 2005; pp. 443–531. [Google Scholar]
- Dobson, G.M.B. Forty years research on atmospheric ozone at Oxford–a history. Appl. Opt. 1968, 7, 387–405. [Google Scholar] [CrossRef]
- Brewer, A.W.; Kerr, J.B. Total ozone measurements in cloudy weather. Pure Appl. Geophys. 1973, 106–108, 928–937. [Google Scholar] [CrossRef]
- Kerr, J.B.; McElroy, C.T.; Olafson, R.A. Measurements of total ozone with the Brewer spectrophotometer. In Proceedings of the Quadrennial Ozone Symposium, Boulder, CO, USA, 4–9 August 1980; London, J., Ed.; National Center for Atmospheric Research: Boulder, CO, USA, 1981; pp. 74–79. [Google Scholar]
- Van Roozendael, M.; Peeters, P.; Roscoe, H.K.; De Backer, H.; Jones, A.E.; Bartlett, L.; Vaughan, G.; Goutail, F.; Pommereau, J.-P.; Kyro, E.; et al. Validation of ground-based visible measurements of total ozone by comparison with Dobson and Brewer spectrophotometers. J. Atmos. Chem. 1998, 29, 55–83. [Google Scholar] [CrossRef]
- Platt, U.; Perner, D. Direct Measurements of Atmospheric CH2O, HNO2, O3, NO2 and SO2 by Differential Optical Absorption in the Near UV. J. Geophys. Res. 1980, 85, 7453–7458. [Google Scholar] [CrossRef]
- Platt, U.; Stutz, J. Differential Optical Absorption Spectroscopy: Principles and Applications; Springer: Weinheim, Germany, 2008; p. 597. [Google Scholar]
- Pommereau, J.P.; Goutail, F. Ozone and NO2 ground-based measurements by visible spectrometry during Arctic winter and spring 1988. Geophys. Res. Lett. 1988, 15, 891–894. [Google Scholar] [CrossRef]
- Bortoli, D.; Ravegnani, F.; Giovanelli, G.; Petritoli, A.; Kostadinov, I. Stratospheric nitrogen dioxide in Antarctic region. In Proceedings of the Quadrennial Ozone Symposium, Kos, Greece, 1–8 June 2004; Zerefos, C.S., Ed.; University of Athens: Athina, Greece, 2004; pp. 940–941. [Google Scholar]
- Wang, Y.; Puķīte, J.; Wagner, T.; Donner, S.; Beirle, S.; Hilboll, A.; Vrekoussis, M.; Richter, A.; Apituley, A.; Piters, A.; et al. Vertical profiles of tropospheric ozone from MAX-DOAS measurements during the CINDI-2 campaign: Part 1—Development of a new retrieval algorithm. J. Geophys. Res. Atmos. 2018, 123, 10637–10670. [Google Scholar] [CrossRef]
- Pfeilsticker, K.; Bösch, H.; Burrows, J.; Butz, A.; Camy-Peyret, C.; Dorf, M.; Gerilowski, K.; Grunow, K.; Gurlit, W.; Naujokat, B.; et al. Balloon-borne DOAS measurements of SCIAMACHY level 1 and 2 products. In Proceedings of the 16th ESA Symposium on European Rocket and Balloon Programmes and Related Research, Sankt Gallen, Switzerland, 2–5 June 2003; Warmbein, B., Ed.; ESA Publications Division: Noordwijk, The Netherlands, 2003; pp. 427–432, ISBN 92-9092-840-9. [Google Scholar]
- Pfeilsticker, K.; Platt, U. Airborne measurements during the Arctic Stratospheric Experiment: Observation of O3 and NO2. Geophys. Res. Lett. 1994, 21, 1375–1378. [Google Scholar] [CrossRef]
- Giovanelli, G.; Bortoli, D.; Petritoli, A.; Castelli, E.; Kostadinov, I.; Ravegnani, F.; Redaelli, G.; Volk, C.M.; Cortesi, U.; Bianchini, G.; et al. Stratospheric minor gas distribution over the Antarctic Peninsula during the APE–GAIA campaign. Int. J. Remote Sens. 2005, 26, 3343–3360. [Google Scholar] [CrossRef]
- Kokhanovsky, A.A.; Rozanov, V.V. The uncertainties of satellite DOAS total ozone retrieval for a cloudy sky. Atmos. Res. 2008, 87, 27–36. [Google Scholar] [CrossRef]
- Qian, Y.; Luo, Y.; Zhou, H.; Yang, T.; Xi, L.; Si, F. First Retrieval of Total Ozone Columns from EMI-2 Using the DOAS Method. Remote Sens. 2023, 15, 1665. [Google Scholar] [CrossRef]
- Gelaro, R.; McCarty, W.; Suárez, M.J.; Todling, R.; Molod, A.; Takacs, L.; Randles, C.A.; Darmenov, A.; Bosilovich, M.G.; Reichle, R.; et al. The Modern-Era Retrospective Analysis for Research and Applications, Version 2 (MERRA-2). J. Clim. 2017, 30, 5419–5454. [Google Scholar] [CrossRef]
- Hersbach, H.; Bell, B.; Berrisford, P.; Hirahara, S.; Horányi, A.; Muñoz-Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Schepers, D.; et al. The ERA5 global reanalysis. Q. J. R. Meteorol. Soc. 2020, 146, 1999–2049. [Google Scholar] [CrossRef]
- Chubachi, S. Preliminary result of ozone observations at Syowa station from February 1982 to January 1983. Mem. Nati. Inst. Polar Res. Spec. Issue 1984, 34, 13–19. [Google Scholar]
- Farman, J.C.; Gardiner, B.G.; Shanklin, J.D. Large losses of total ozone in Antarctica reveals seasonal ClOx/NOx interactions. Nature 1985, 315, 207–210. [Google Scholar] [CrossRef]
- Crook, J.A.; Gillett, N.P.; Keeley, S.P.E. Sensitivity of Southern Hemisphere climate to zonal asymmetry in ozone. Geophys. Res. Lett. 2008, 35, L07806. [Google Scholar] [CrossRef]
- Bian, L.G.; Lin, Z.; Zheng, X.-D.; Ma, Y.-F.; Lu, L.-H. Trend of Antarctic ozone hole and its influencing factors. Adv. Clim. Chang. Res. 2012, 3, 68–75. [Google Scholar] [CrossRef]
- WMO. Scientific Assessment of Ozone Depletion: 2018, Global Ozone Research and Monitoring Project Report, No. 58; WMO: Geneva, Switzerland, 2018; Chapter 4. [Google Scholar]
- Sibira, E.E.; Radionov, V.F.; Rusina, E.N. Results of Long-term Observations of Total Ozone in Antarctica and over the Atlantic and Southern Oceans. Russ. Meteorol. Hydrol. 2020, 45, 161–168. [Google Scholar] [CrossRef]
- Hartmann, D.L. The Antarctic ozone hole and the pattern effect on climate sensitivity. Proc. Natl. Acad. Sci. USA 2022, 119, e2207889119. [Google Scholar] [CrossRef]
- Kessenich, H.E.; Seppälä, A.; Rodger, C.J. Potential drivers of the recent large Antarctic ozone holes. Nat. Commun. 2023, 14, 7259. [Google Scholar] [CrossRef]
- Zhu, L.; Wu, Z. Climatic influence of the Antarctic ozone hole on the East Asian winter precipitation. Clim. Atmos. Sci. 2024, 7, 184. [Google Scholar] [CrossRef]
- Park, H.; Heath, D.F. Nimbus 7 SBUV/TOMS Calibration for the Ozone Measurement. In Atmospheric Ozone; Zerefos, C.S., Ghazi, A., Eds.; Springer: Dordrecht, The Netherlands, 1985. [Google Scholar] [CrossRef]
- Fleig, A.J.; Bhartia, P.K.; Wellemeyer, C.G.; Silberstein, D.S. Seven years of total ozone from the TOMS instrument-A report on data quality. Geophys. Res. Lett. 1986, 13, 1355–1358. [Google Scholar] [CrossRef]
- Bracher, A.; Lamsal, L.N.; Weber, M.; Bramstedt, K.; Coldewey-Egbers, M.; Burrows, J.P. Global satellite validation of SCIAMACHY O3 columns with GOME WFDOAS. Atmos. Chem. Phys. 2005, 5, 2357–2368. [Google Scholar] [CrossRef]
- Levelt, P.F.; Van den Oord, G.H.J.; Dobber, M.R.; Malkki, A.; Visser, H.; de Vries, J. The ozone monitoring instrument. IEEE Trans. Geosci. Remote Sens. 2006, 44, 1093–1101. [Google Scholar] [CrossRef]
- Toth, G.; Hillger, D. A brief history of ozone-monitoring satellites and instruments. Orbit 2011, 91, 12–17. Available online: https://rammb.cira.colostate.edu/dev/hillger/pdf/A_brief_history_of_ozone-monitoring_satellites_and_instruments.pdf (accessed on 15 September 2024).
- Kramarova, N.A.; Nash, E.R.; Newman, P.A.; Bhartia, P.K.; McPeters, R.D.; Rault, D.F.; Seftor, C.J.; Xu, P.Q.; Labow, G.J. Measuring the Antarctic ozone hole with the new Ozone Mapping and Profiler Suite (OMPS). Atmos. Chem. Phys. 2014, 14, 2353–2361. [Google Scholar] [CrossRef]
- Kokhanovsky, A.; Gascoin, S.; Arnaud, L.; Picard, G. Retrieval of Snow Albedo and Total Ozone Column from Single-View MSI/S-2 Spectral Reflectance Measurements over Antarctica. Remote Sens. 2021, 13, 4404. [Google Scholar] [CrossRef]
- Kokhanovsky, A.A.; Brell, M.; Segl, K.; Bianchini, G.; Lanconelli, C.; Lupi, A.; Petkov, B.; Picard, G.; Arnaud, L.; Stone, R.S.; et al. First Retrievals of Surface and Atmospheric Properties Using EnMAP Measurements over Antarctica. Remote Sens. 2023, 15, 3042. [Google Scholar] [CrossRef]
- Molina, M.J.; Rowland, F.S. Stratospheric sink for chlorofluoromethanes: Chlorine atom catalysed destruction of ozone. Nature 1974, 249, 810–812. [Google Scholar] [CrossRef]
- Crutzen, P.J.; Arnold, F. Nitric acid cloud formation in the cold Antarctic stratosphere: A major cause for the springtime’ ozone hole. Nature 1986, 324, 651–655. [Google Scholar] [CrossRef]
- Rowland, F.S. Stratospheric ozone depletion. Phil. Trans. R. Soc. B 2006, 361, 769–790. [Google Scholar] [CrossRef]
- Paul, A.; Newman, P.A.; Kawa, S.R.; Nash, E.R. On the size of the Antarctic ozone hole. Geophys. Res. Lett. 2004, 31, L21104. [Google Scholar]
- Grytsai, A.V.; Evtushevsky, O.M.; Agapitov, O.V.; Klekociuk, A.R.; Milinevsky, G.P. Structure and long-term change in the zonal asymmetry in Antarctic total ozone during spring. Ann. Geophys. 2007, 25, 361–374. [Google Scholar] [CrossRef][Green Version]
- Evtushevsky, O.M.; Grytsai, A.V.; Klekociuk, A.R.; Milinevsky, G.P. Total ozone and tropopause zonal asymmetry during the Antarctic spring. J. Geophys. Res. 2008, 113, D00B06. [Google Scholar] [CrossRef]
- Ialongo, I.; Sofieva, V.; Kalakoski, N.; Tamminen, J.; Kyrölä, E. Ozone zonal asymmetry and planetary wave characterization during Antarctic spring. Atmos. Chem. Phys. 2012, 12, 2603–2614. [Google Scholar] [CrossRef]
- Grytsai, A.; Klekociuk, A.; Milinevsky4, G.; Evtushevsky, O.; Stone, K. Evolution of the eastward shift in the quasi-stationary minimum of the Antarctic total ozone column. Atmos. Chem. Phys. 2017, 17, 1741–1758. [Google Scholar] [CrossRef]
- Yu, R.; Reshetnyk, V.; Grytsai, A.; Milinevsky, G.; Evtushevsky, O.; Klekociuk, A.; Shi, Y. Current trends in the zonal distribution and asymmetry of ozone in Antarctica based on satellite measurements. Ukr. Antarct. J. 2024, 22, 24–39. [Google Scholar] [CrossRef]
- Manney, G.L.; Santee, M.L.; Rex, M.; Livesey, N.J.; Pitts, M.C.; Veefkind, P.; Nash, E.R.; Wohltmann, I.; Lehmann, R.; Froidevaux, L.; et al. Unprecedented arctic ozone loss in 2011. Nature 2011, 478, 469–475. [Google Scholar] [CrossRef]
- Petkov, B.H.; Vitale, V.; Di Carlo, P.; Drofa, O.; Mastrangelo, D.; Smedley, A.R.D.; Diémoz, H.; Siani, A.M.; Fountoulakis, I.; Webb, A.R.; et al. An unprecedented Arctic ozone depletion event during spring 2020 and its impacts across Europe. J. Geophys. Res. Atmos. 2023, 128, e2022JD037581. [Google Scholar] [CrossRef]
- WOUDC. World Ozone and Ultraviolet Data Centre. 2024. Available online: https://woudc.org (accessed on 1 March 2024).
- Petkov, B.; Vitale, V.; Tomasi, C.; Bonafé, U.; Scaglione, S.; Flori, D.; Santaguida, R.; Gausa, M.; Hansen, G.; Colombo, T. Narrow-band filter radiometer for ground-based measurements of global UV solar irradiance and total ozone. Appl. Opt. 2006, 45, 4383–4395. [Google Scholar] [CrossRef]
- ECMWF. European Centre for Medium-Range Weather Forecasts, ERA-5 Reanalyses [Registration Required]. 2024. Available online: https://cds.climate.copernicus.eu/datasets/reanalysis-era5-single-levels?tab=overview (accessed on 1 February 2024).
- Brandt, S. Statistical and Computational Methods in Data Analysis, 2nd ed.; North-Holland Publishing Company: Amsterdam, The Netherlands, 1970. [Google Scholar]
- Fioletov, V.; Zhao, X.; Abboud, I.; Brohart, M.; Ogyu, A.; Sit, R.; Lee, S.C.; Petropavlovskikh, I.; Miyagawa, K.; Johnson, B.J.; et al. Total ozone variability and trends over the South Pole during the wintertime. Atmos. Chem. Phys. 2023, 23, 12731–12751. [Google Scholar] [CrossRef]
- Čížková, K.; Láska, K.; Metelka, L.; Staněk, M. Assessment of spectral UV radiation at Marambio Base, Antarctic Peninsula. Atmos. Chem. Phys. 2023, 23, 4617–4636. [Google Scholar] [CrossRef]
- Orte, P.F.; Wolfram, E.; Salvador, J.; Mizuno, A.; Bègue, N.; Bencherif, H.; Bali, J.L.; D’Elia, R.; Pazmiño, A.; Godin-Beekmann, S.; et al. Analysis of a southern sub-polar short-term ozone variation event using a millimetre-wave radiometer. Ann. Geophys. 2019, 37, 613–629. [Google Scholar] [CrossRef]
- Nichol, S.E.; Valenti, C. Intercomparison of total ozone measured at low sun angles by the Brewer and Dobson spectrophotometers at Scott Base, Antarctica. Geophys. Res. Lett. 1993, 20, 2051–2054. [Google Scholar] [CrossRef]


| Station | GAW ID | Coordinates | Instrument | Gaps |
|---|---|---|---|---|
| Princess Elisabeth | PES | 71.95°S 23.35°E | Brewer MK III 100 | 2008–2010, 2013, 2016 September–October 2012, 2014, 2015, 2019, 2020, 2021 September–November 2011, 2017, 2018, 2022 December 2022 |
| Syowa | SYO | 69.01°S 39.58°E | Dobson Beck 119, 122 Brewer MK III 252 | ― |
| Zhong Shan | ZOS | 69.37°S 76.38°E | Brewer MK IV 74 Brewer MK III 193 | 2008–2010 September–November 2011 September–October 2012 |
| Dumont D’Urville | DDU | 66.67°S 140.02°E | SAOZ CP200_NMOS 1024 17 | ― |
| Arrival Heights | ARH | 77.83°S 166.67°E | Dobson Back 17 | December 2009 November–December 2014 October–December 2017 September–November 2019 |
| Dome Concordia 1 | DCC | 75.10°S 123.35°E | UV-RAD | 2009, 2016–2019 September 2010, 2015 November 2015 |
| South Pole | SPO | 89.99(7)°S 24.8°W | Dobson Beck 80, 82 Brewer MK III 21, 85 | September 2008–2010, 2012–2018, 2021 |
| Belgrano 1 | BLG | 77.87°S 34.62°W | Brewer MK IV 99 | 2018–2020 September 2014 November–December 2017 |
| San Martin 1 | SMT | 68.13°S 67.10°W | Brewer MK IV 162 | 2011–2014, 2018, 2020 September 2017 September 2022 |
| Marambio | MBI | 64.24°S 56.62°W | Dobson Beck 99 Brewer MK III 199 | November–December 2021 |
| Percentage of Cases (%) | ||||
|---|---|---|---|---|
| 60.0 | 46.0 | |||
| 72.5 | 61.0 | 77.5 | 72.0 | |
| 85.0 | 72.0 | 88.0 | 82.0 | |
| Stations Composing the Patterns | Median | 10th and 90th Percentiles | Mean | Standard Deviation | ||
|---|---|---|---|---|---|---|
| 1 | BLG, DCC | 70°S | 1.01 | 0.93, 1.09 | 1.01 | ±0.07 |
| 2 | BLG, ARH | 68°S | 1.00 | 0.93, 1.08 | 1.00 | ±0.06 |
| 3 | BLG, DCC, ARH | 66°S | 1.00 | 0.93, 1.09 | 1.01 | ±0.07 |
| 4 | BLG, DCC, ARH, SPO | 68°S | 1.00 | 0.94, 1.06 | 1.00 | ±0.05 |
| 5 | SMT, BLG, SPO, DCC, DDU | 60°S | 1.00 | 0.92, 1.07 | 1.00 | ±0.06 |
| 6 | MBI, BLG, SPO, DCC, DDU | 58°S | 0.99 | 0.90, 1.07 | 0.99 | ±0.07 |
| 7 | SMT, BLG, SPO, DCC, ARH, DDU, PES | 62°S | 1.00 | 0.95, 1.06 | 1.00 | ±0.05 |
| 8 | SMT, BLG, SPO, DCC, ARH, DDU, ZOS | 60°S | 1.00 | 0.93, 1.07 | 1.00 | ±0.06 |
| 9 | MBI, SMT, BLG, PES, SYO, ZOS, DDU, ARH, DCC, SPO | 60°S | 1.00 | 0.94, 1.05 | 1.00 | ±0.05 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petkov, B.H.; Vitale, V.; Carlo, P.D.; Ochoa, H.A.; Gulisano, A.; Coronato, I.L.; Láska, K.; Kostadinov, I.; Lupi, A.; Mazzola, M.; et al. Approximate Near-Real-Time Assessment of Some Characteristic Parameters of the Spring Ozone Depletion over Antarctica Using Ground-Based Measurements. Remote Sens. 2025, 17, 507. https://doi.org/10.3390/rs17030507
Petkov BH, Vitale V, Carlo PD, Ochoa HA, Gulisano A, Coronato IL, Láska K, Kostadinov I, Lupi A, Mazzola M, et al. Approximate Near-Real-Time Assessment of Some Characteristic Parameters of the Spring Ozone Depletion over Antarctica Using Ground-Based Measurements. Remote Sensing. 2025; 17(3):507. https://doi.org/10.3390/rs17030507
Chicago/Turabian StylePetkov, Boyan H., Vito Vitale, Piero Di Carlo, Héctor A. Ochoa, Adriana Gulisano, Iona L. Coronato, Kamil Láska, Ivan Kostadinov, Angelo Lupi, Mauro Mazzola, and et al. 2025. "Approximate Near-Real-Time Assessment of Some Characteristic Parameters of the Spring Ozone Depletion over Antarctica Using Ground-Based Measurements" Remote Sensing 17, no. 3: 507. https://doi.org/10.3390/rs17030507
APA StylePetkov, B. H., Vitale, V., Carlo, P. D., Ochoa, H. A., Gulisano, A., Coronato, I. L., Láska, K., Kostadinov, I., Lupi, A., Mazzola, M., Cavaliere, A., Frangipani, C., Verazzo, G., & Pulimeno, S. (2025). Approximate Near-Real-Time Assessment of Some Characteristic Parameters of the Spring Ozone Depletion over Antarctica Using Ground-Based Measurements. Remote Sensing, 17(3), 507. https://doi.org/10.3390/rs17030507








