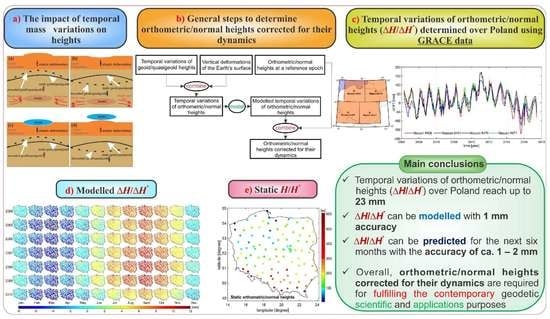
Contribution of GRACE Satellite Mission to the Determination of Orthometric/Normal Heights Corrected for Their Dynamics—A Case Study of Poland
Abstract
1. Introduction
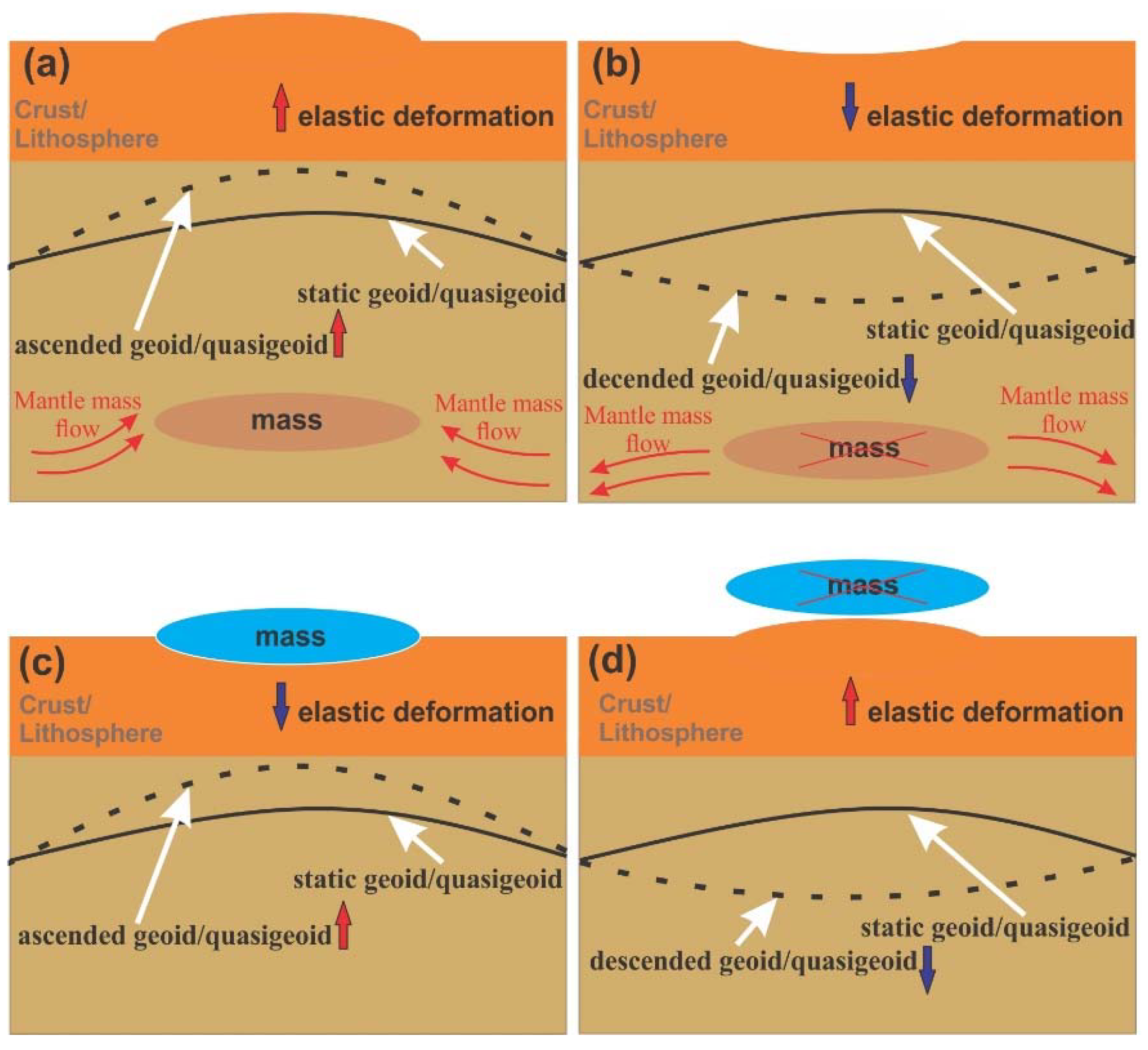
2. The Impact of TMVES on Heights
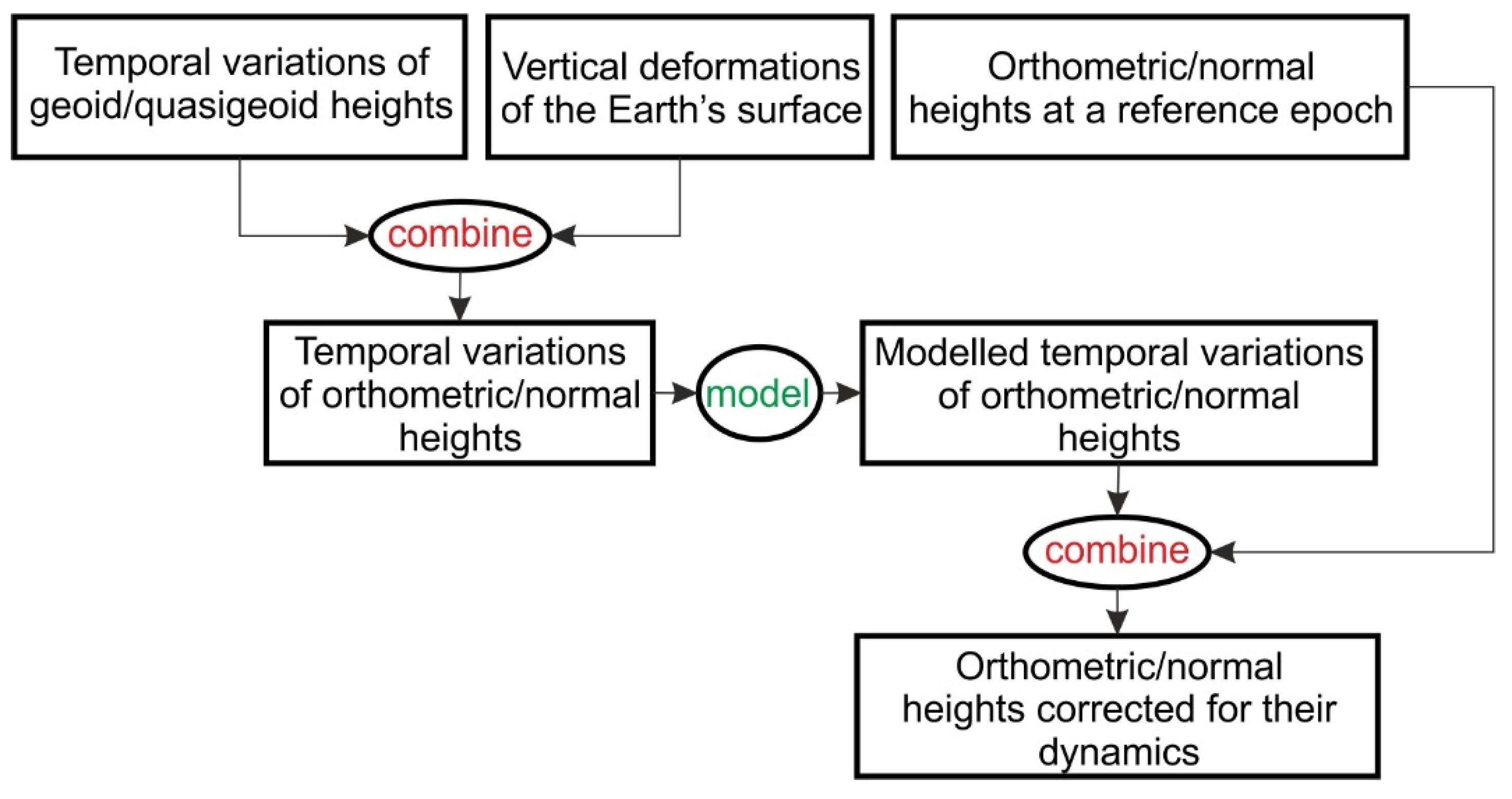
3. The Determination of Orthometric/Normal Heights Corrected for Their Dynamics
- (1)
- Determination of H(t0)/H*(t0).
- (2)
- Estimation of ∆H/∆H* over the time interval including t0 and if possible, t, by combining ∆N/∆ζ and ∆h. Then, developing the model of ∆H/∆H* and calculating ∆HM(t)/∆H*M(t) from this model.
- (3)
- Determination of H(t)/H*(t) at t as a sum of H(t0)/H*(t0) and ∆HM(t)/∆H*M(t) obtained from step 2.
4. A Case Study
4.1. Orthometric/Normal Heights at Reference Epoch
4.2. Temporal Variations of Orthometric/Normal Heights
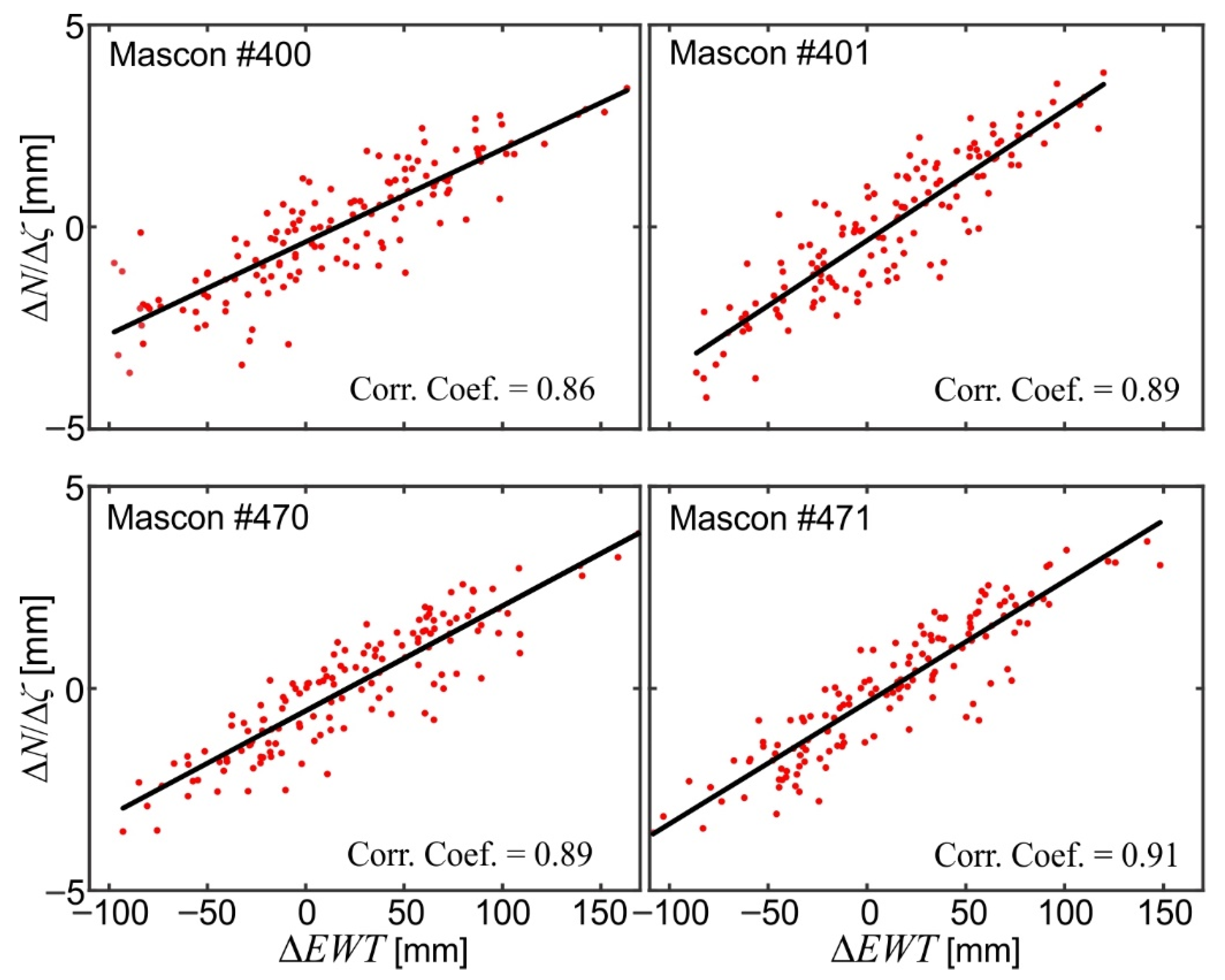
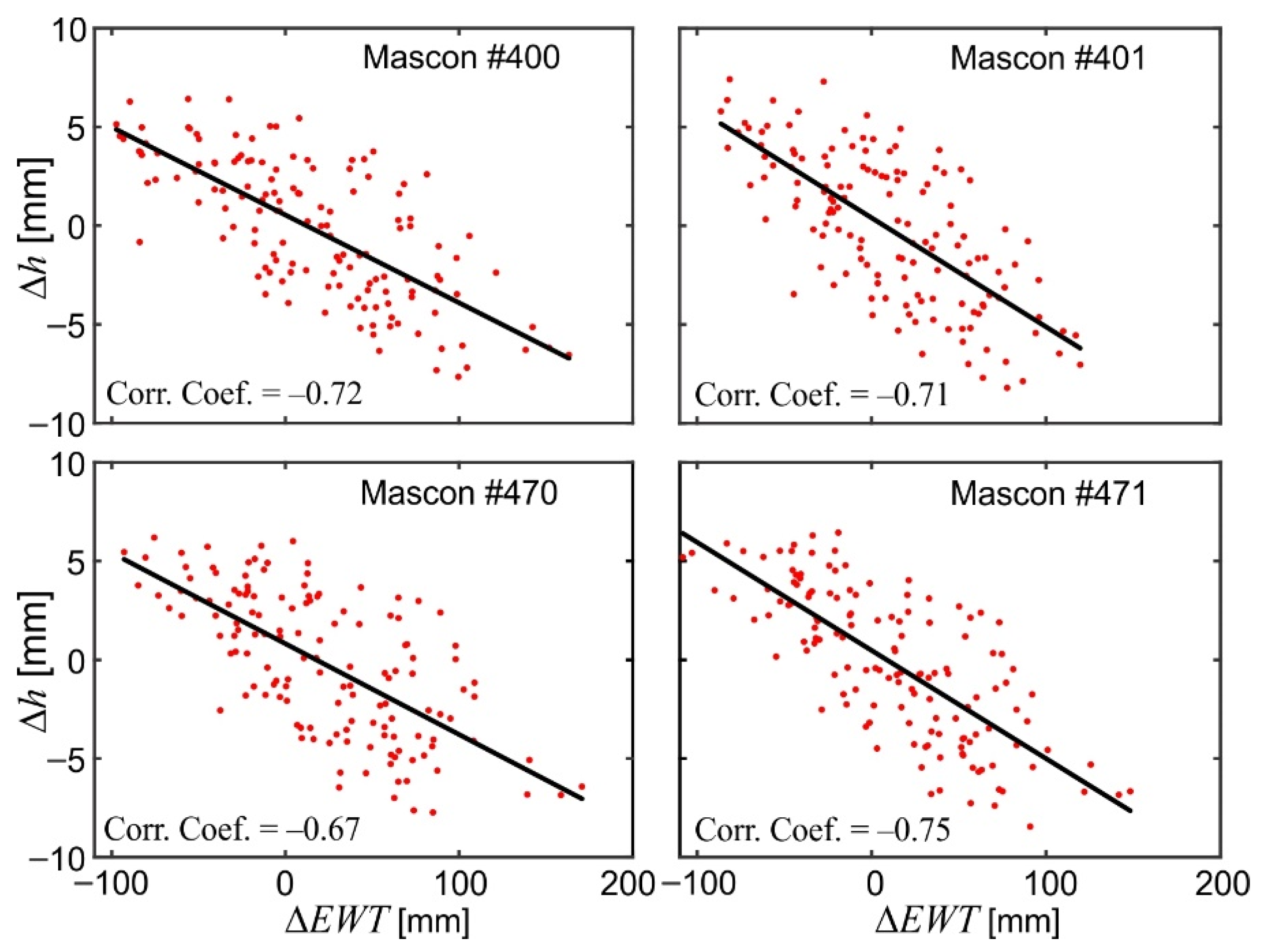
4.2.1. The Estimation of ∆H/∆H*
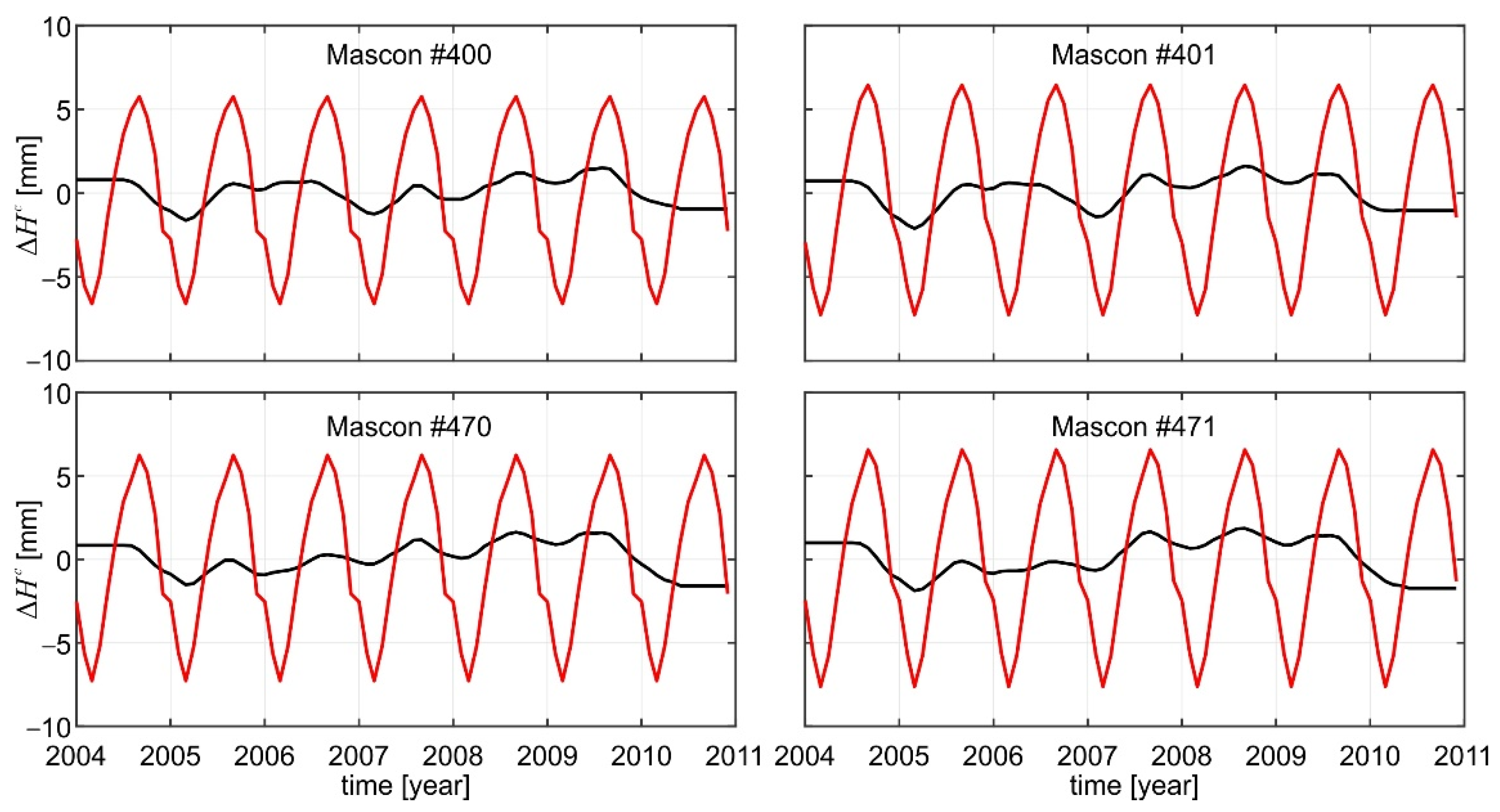
4.2.2. The Analysis and Modelling of ΔH/ΔH* and Determining ΔHM/ΔH*M
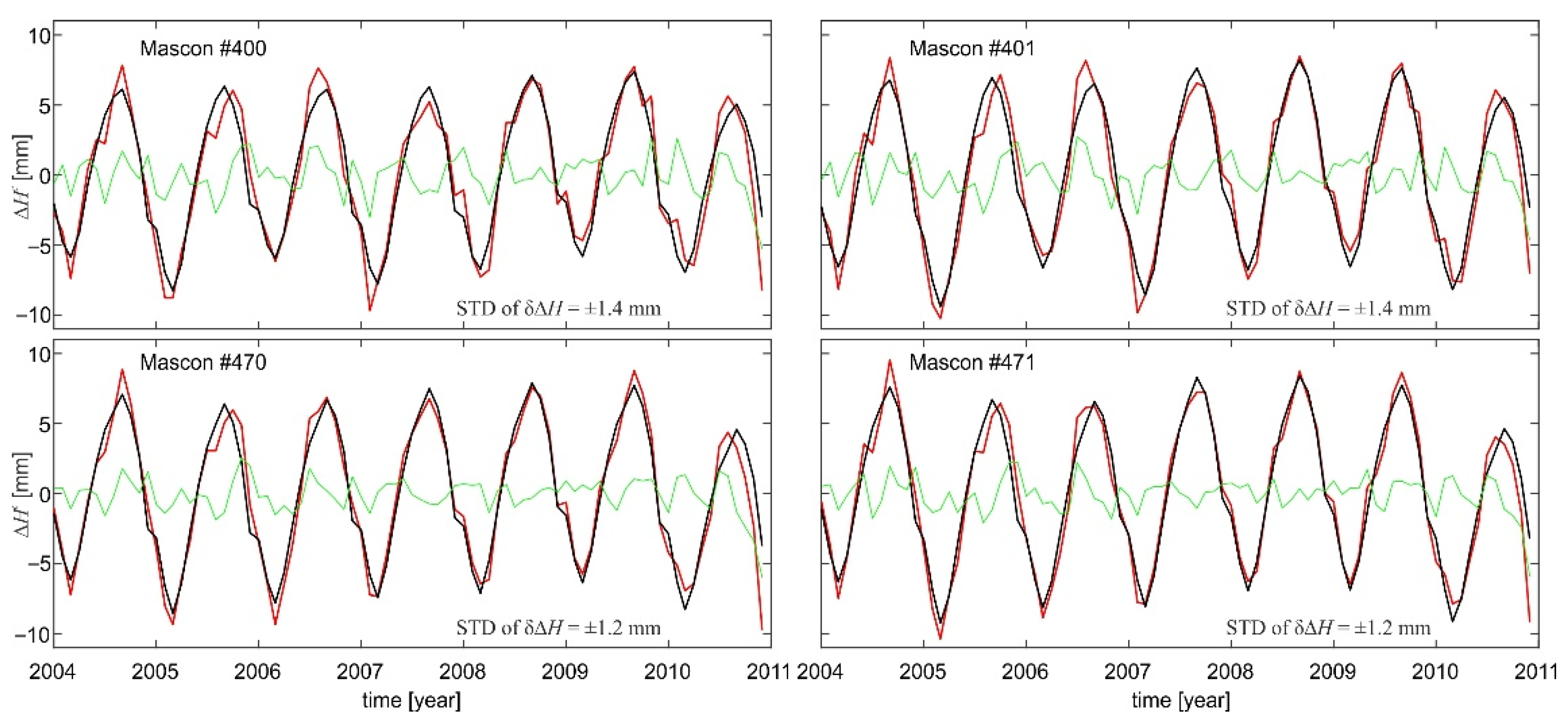
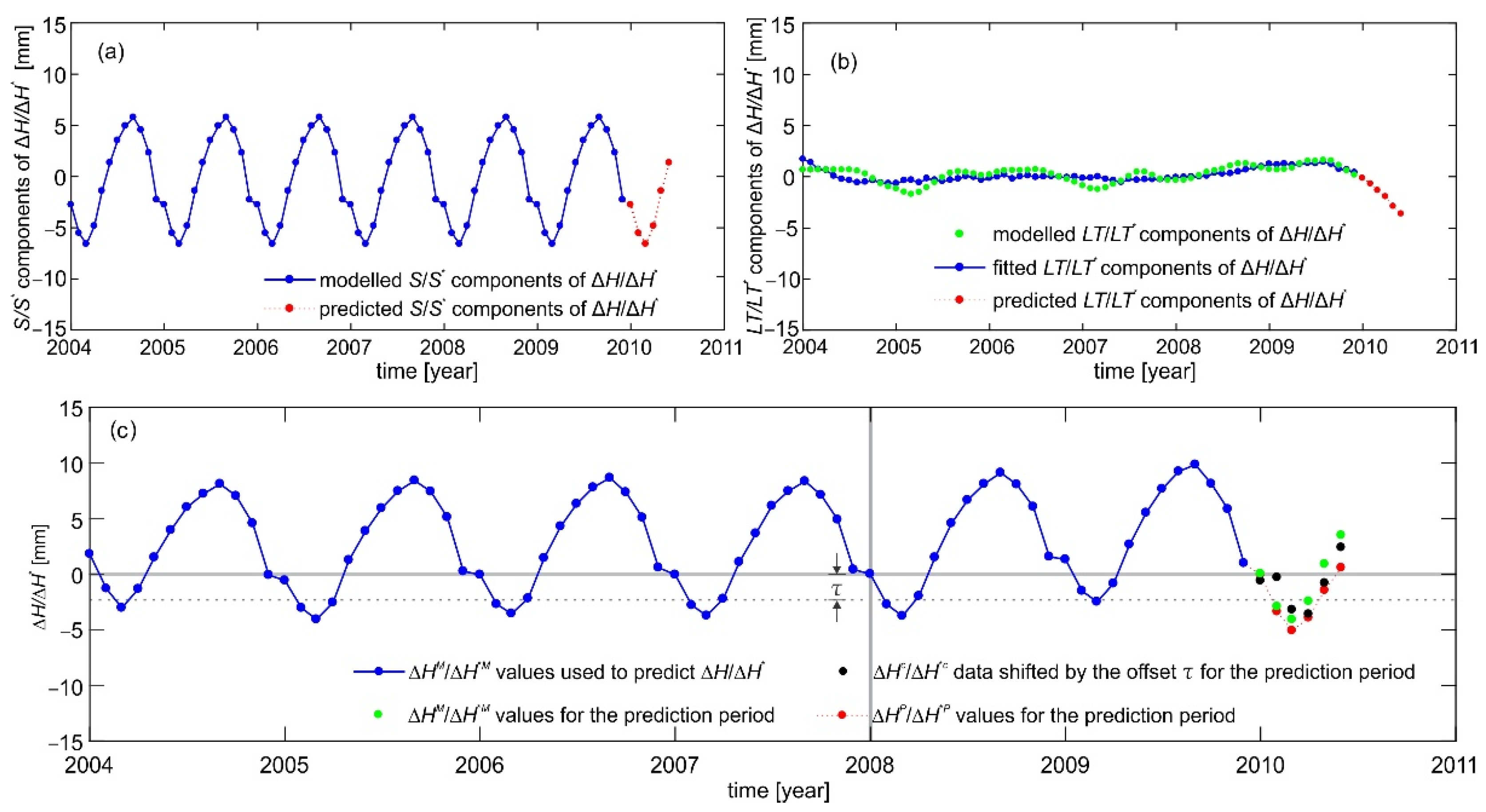
4.2.3. The Prediction of ΔHM/ΔH*M
4.3. The Determination of H(t)/H*(t)
5. Conclusions
- Temporal variations of orthometric/normal heights ΔH/ΔH* for the period from April 2002 to August 2016, obtained as a combination of temporal variations of geoid/quasigeoid heights ΔN/Δζ and vertical deformations of the Earth’s surface Δh reach up to 23 mm.
- The major part of the signal, i.e., ca. 66%, of ΔH/ΔH* results from Δh, while its remaining part is due to ΔN/Δζ.
- The ΔH/ΔH* are strongly correlated with ΔEWT (correlation coefficients of −0.79 ± 0.03).
- The use of the seasonal decomposition method makes possible modelling ΔH/ΔH* with one millimetre accuracy at the confidence level of 97%; it also makes possible predicting them for the next six months with the accuracy of ca. 1–2 mm.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Farahani, H.H.; Klees, R.; Slobbe, C. Data requirements for a 5-mm quasi-geoid in the Netherlands. Stud. Geophys. Geod. 2017, 61, 675–702. [Google Scholar] [CrossRef]
- Foroughi, I.; Vaníček, P.; Kingdon, R.W.; Sheng, M.; Afrasteh, Y.; Novák, P.; Santos, M.C. Sub-centimetre geoid. J. Geod. 2018, 93, 849–868. [Google Scholar] [CrossRef]
- Ellmann, A.; Märdla, S.; Oja, T. The 5 mm geoid model for Estonia computed by the least squares modified Stokes’s formula. Surv. Rev. 2019, 52, 352–372. [Google Scholar] [CrossRef]
- Drewes, H.; Kuglitsch, F.; Adám, J.; Rózsa, S. The Geodesist’s handbook 2016. J. Geod. 2016, 90, 907–1205. [Google Scholar] [CrossRef]
- Dach, R.; Lutz, S.; Walser, P.; Fridez, P. Bernese GNSS Software Version 5.2. User Manual; Astronomical Institute, University of Bern, Bern Open Publishing: Bern, Switzerland, 2015; ISBN 978-3-906813-05-9. [Google Scholar] [CrossRef]
- Mäkinen, J.; Saaranen, V. Determination of post-glacial land uplift from the three precise levellings in Finland. J. Geod. 1998, 72, 516–529. [Google Scholar] [CrossRef]
- Fotopoulos, G. An Analysis on the Optimal Combination of Geoid, Orthometric and Ellipsoidal Height Data. Ph.D. Thesis, UCGE Reports No. 20185. Department of Geomatics Engineering, University of Calgary, Calgary, AB, Canada, 2003. [Google Scholar]
- Fuhrmann, T.; Westerhaus, M.; Zippelt, K.; Heck, B. Vertical displacement rates in the Upper Rhine Graben area derived from precise levelling. J. Geod. 2014, 88, 773–787. [Google Scholar] [CrossRef]
- Lyon, T.J.; Filmer, M.S.; Featherstone, W.E. On the use of repeat leveling for the determination of vertical land motion: Artifacts, aliasing, and extrapolation errors. J. Geophys. Res. Solid Earth 2018, 123, 7021–7039. [Google Scholar] [CrossRef]
- Blewitt, G.; Lavallée, D.; Clarke, P.; Nurutdinov, K. A new global mode of Earth deformation: Seasonal cycle detected. Science 2001, 294, 2342–2345. [Google Scholar] [CrossRef]
- Bevis, M.; Alsdorf, D.; Kendrick, E.; Fortes, L.P.; Forsberg, B.; Smalley, R., Jr.; Becker, J. Seasonal fluctuations in the mass of the Amazon River system and Earth’s elastic response. Geophys. Res. Lett. 2005, 32, L16308. [Google Scholar] [CrossRef]
- van Dam, T.; Wahr, J.; Lavallée, D. A comparison of annual vertical crustal displacements from GPS and Gravity Recovery and Climate Experiment (GRACE) over Europe. J. Geophys Res. 2007, 112, B03404. [Google Scholar] [CrossRef]
- Heki, K. Dense GPS array as a new sensor of seasonal changes of surface loads. In The State of the Planet: Frontiers and Challenges in Geophysics; Geophysical Monograph Series; Sparks, R.S.J., Hawkesworth, C.J., Eds.; AGU: Washington, DC, USA, 2004; Volume 150, pp. 177–196. [Google Scholar]
- Davis, J.L.; Elósegui, P.; Mitrovica, J.X.; Tamisiea, M.E. Climate-driven deformation of the solid earth from GRACE and GPS. Geophys. Res. Lett. 2004, 31, L24605. [Google Scholar] [CrossRef]
- Steckler, M.S.; Nooner, S.L.; Akhter, S.H.; Chowdhury, S.K.; Bettadpur, S.; Seeber, L.; Kogan, M.G. Modeling Earth deformation from monsoonal flooding in Bangladesh using hydrographic, GPS, and Gravity Recovery and Climate Experiment (GRACE) data. J. Geophys. Res. Solid Earth 2010, 115, B08407. [Google Scholar] [CrossRef]
- Nahmani, S.; Bock, O.; Bouin, M.N.; Santamaría-Gómez, A.; Boy, J.P.; Collilieux, X.; Métivier, L.; Panet, I.; Genthon, P.; De Linage, C.; et al. Hydrological deformation induced by the West African Monsoon: Comparison of GPS, GRACE and loading models. J. Geophys. Res. Solid Earth 2012, 117, B05409. [Google Scholar] [CrossRef]
- Fu, Y.; Freymueller, J.T.; Jensen, T. Seasonal hydrological loading in southern Alaska observed by GPS and GRACE. Geophys. Res. Lett. 2012, 39, L15310. [Google Scholar] [CrossRef]
- Liu, R.L.; Li, J.; Fok, H.S.; Shum, C.K.; Li, Z. Earth surface deformation in the North China plain detected by joint analysis of GRACE and GPS data. Sensors 2014, 14, 19861–19876. [Google Scholar] [CrossRef]
- Pan, Y.; Shen, W.B.; Hwang, C.; Liao, C.; Zhang, T.; Zhang, G. Seasonal Mass Changes and Crustal Vertical Deformations Constrained by GPS and GRACE in Northeastern Tibet. Sensors 2016, 16, 1211. [Google Scholar] [CrossRef]
- Rajner, M.; Liwosz, T. Studies of crustal deformation due to hydrological loading on GPS height estimates. Geod. Cartogr. 2011, 60, 135–144. [Google Scholar] [CrossRef][Green Version]
- Godah, W.; Ray, J.D.; Szelachowska, M.; Krynski, J. The Use of National CORS Networks for Determining Temporal Mass Variations within the Earth’s System and for Improving GRACE/GRACE–FO Solutions. Remote Sens. 2020, 12, 3359. [Google Scholar] [CrossRef]
- Godah, W.; Szelachowska, M.; Ray, J.D.; Krynski, J. Comparison of vertical deformation of the Earth’s surface obtained using GRACE-based GGMS and GNNS data—A case study of South-Eastern Poland. Acta Geodyn. Geomater. 2020, 17, 169–176. [Google Scholar] [CrossRef]
- Birhanu, Y.; Bendick, R. Monsoonal loading in Ethiopia and Eritrea from vertical GPS displacement time series. J. Geophys. Res. Solid Earth 2015, 120, 7231–7238. [Google Scholar] [CrossRef]
- Tapley, B.D.; Bettadpur, S.; Watkins, M.; Reigber, C. The gravity recovery and climate experiment: Mission overview and early results. Geophys Res. Lett. 2004, 31, L09607. [Google Scholar] [CrossRef]
- Rangelova, E. A Dynamic Geoid Model for Canada, Report No. 20261. Ph.D. Thesis, Department of Geomatics Engineering, University of Calgary, Calgary, AB, Canada, 2007. [Google Scholar]
- Rangelova, E.; Fotopoulos, G.; Sideris, M.G. Implementing a dynamic geoid as a vertical datum for orthometric heights in Canada. In Gravity, Geoid and Earth Observation. International Association of Geodesy Symposia; Mertikas, S.P.P., Ed.; Springer: Berlin/Heidelberg, Germany, 2010; Volume 135, pp. 295–302. [Google Scholar] [CrossRef]
- Rangelova, E.; Sideris, M.G. Contributions of terrestrial and GRACE data to the study of the secular geoid changes in North America. J. Geodyn. 2008, 46, 131–143. [Google Scholar] [CrossRef]
- Krynski, J.; Kloch-Główka, G.; Szelachowska, M. Analysis of time variations of the gravity field over Europe obtained from GRACE data in terms of geoid height and mass variations. In Earth on the Edge: Science for a Sustainable Planet. International Association of Geodesy Symposia; Rizos, C., Willis, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; Volume 139, pp. 365–370. [Google Scholar] [CrossRef]
- Godah, W.; Szelachowska, M.; Krynski, J. On the analysis of temporal geoid height variations obtained from GRACE-based GGMs over the area of Poland. Acta Geophys. 2017, 65, 713–725. [Google Scholar] [CrossRef]
- Godah, W.; Szelachowska, M.; Krynski, J. Investigation of geoid height variations and vertical displacements of the Earth surface in the context of the realization of the modern vertical reference system—A case study for Poland, In International Symposium on Gravity, Geoid and Height Systems 2016, International Association of Geodesy Symposia; Vergos, G., Pail, R., Barzaghi, R., Eds.; Springer: Cham, Switzerland, 2017; Volume 148, pp. 135–141. [Google Scholar] [CrossRef]
- Godah, W.; Szelachowska, M.; Krynski, J. On the estimation of physical height changes using GRACE satellite mission data—A case study of Central Europe. Geod. Cartogr. 2017, 66, 211–226. [Google Scholar] [CrossRef][Green Version]
- Godah, W.; Szelachowska, M.; Krynski, J. Application of the PCA/EOF method for the analysis and modelling of temporal variations of geoid heights over Poland. Acta Geod. Et Geophys. 2018, 53, 93–105. [Google Scholar] [CrossRef]
- Zhang, X.; Jin, S.; Lu, X. Global Surface Mass Variations from Continuous GPS Observations and Satellite Altimetry Data. Remote Sens. 2017, 9, 1000. [Google Scholar] [CrossRef]
- Öztürk, Z.E.; Godah, W.; Abbak, R.A. Estimation of physical height changes from GRACE satellite mission data and WGHM over Turkey. Acta Geod. Geophys. 2020, 55, 301–317. [Google Scholar] [CrossRef]
- Godah, W.; Szelachowska, M.; Krynski, J.; Ray, J.D. Assessment of Temporal Variations of Orthometric/Normal Heights Induced by Hydrological Mass Variations over Large River Basins Using GRACE Mission Data. Remote Sens. 2020, 12, 3070. [Google Scholar] [CrossRef]
- Sanchez, L.; Sideris, M.G. Vertical datum unification for the International Height Reference System (IHRS). Geophys. J. Int. 2017, 209, 570–586. [Google Scholar] [CrossRef]
- Purkhauser, A.F.; Pail, R. Next generation gravity missions: Near-real time gravity field retrieval strategy. Geophys. J. Int. 2019, 217, 1314–1333. [Google Scholar] [CrossRef]
- Mehlstäubler, T.E.; Grosche, G.; Lisdat, C.; Schmidt, P.O.; Denker, H. Atomic clocks for geodesy. Rep. Prog. Phys. 2018, 81, 064401. [Google Scholar] [CrossRef] [PubMed]
- Torge, W.; Müller, J. Geodesy, 4th ed.; Walter de Gruyter: Berlin, Germany; Boston, MA, USA, 2012. [Google Scholar]
- Barthelmes, F. Definition of Functionals of the Geopotential and Their Calculation from Spherical Harmonic Models: Theory and Formulas Used by the Calculation Service of the International Centre for Global Earth Models (ICGEM). In GFZ German Research Centre for Geosciences; Helmholtz-Zentrum Potsdam: Potsdam, Germany, 2013; p. 32. Available online: http://icgem.gfzpotsdam.de (accessed on 4 August 2022).
- Makridakis, S.; Wheelwright, S.C.; Hyndman, R.J. Forecasting: Methods and Applications, 3rd ed.; Wiley: New York, NY, USA, 1998; ISBN 978-0-471-53233-0. [Google Scholar]
- Jolliffe, I. Principal Component Analysis; Springer: New York, NY, USA, 2002. [Google Scholar]
- Pavlis, N.K.; Holmes, S.A.; Kenyon, S.C.; Factor, J.K. The development and evaluation of the earth gravitational model 2008 (EGM2008). J. Geophys. Res. 2012, 117, B04406. [Google Scholar] [CrossRef]
- Szelachowska, M.; Krynski, J. GDQM-PL13—The new gravimetric quasigeoid model for Poland. Geoinf. Issues 2014, 6, 5–19. [Google Scholar] [CrossRef]
- Dziennik Ustaw Rzeczypospolitej Polskiej. The Regulation of the Council of Ministers Concerning the National Spatial Reference System. 2012. Available online: https://isap.sejm.gov.pl/isap.nsf/download.xsp/WDU20120001247/O/D20121247.pdf (accessed on 1 August 2022).
- Krynski, J.; Rogowski, J.B. National Report of Poland to EUREF 2014. In Proceedings of the Symposium of the IAG Subcommission for Europe (EUREF), Vilnius, Lithuania, 4–6 June 2014; Available online: http://www.euref-iag.net/symposia/2014Vilnius/05-20-p-Poland.pdf (accessed on 4 August 2022).
- Heiskanen, W.A.; Moritz, H. Physical Geodesy; W.H. Freeman and Company: San Francisco, CA, USA, 1967. [Google Scholar]
- Bettadpur, S. Gravity Recovery and Climate Experiment Level-2 Gravity Field Product User Handbook; Center for Space Research at The University of Texas at Austin: Austin, TX, USA, 2018; Available online: https://podaac-tools.jpl.nasa.gov/drive/files/allData/grace/docs/L2-UserHandbook_v4.0.pdf (accessed on 1 August 2022).
- Dziewonski, A.M.; Anderson, D.L. Preliminary reference Earth model. Phys. Earth Planet. Inter. 1981, 25, 297–356. [Google Scholar] [CrossRef]
- Godah, W. IGiK–TVGMF: A MATLAB package for computing and analysing temporal variations of gravity/mass functionals from GRACE satellite based global geopotential models. Comput. Geosci. 2019, 123, 47–58. [Google Scholar] [CrossRef]
- Swenson, S.; Chambers, D.; Wahr, J. Estimating geocenter variations from a combination of GRACE and ocean model output. J. Geophys. Res. Solid Earth 2008, 113, B08410. [Google Scholar] [CrossRef]
- Cheng, M.K.; Tapley, B.D.; Ries, J.C. Deceleration in the Earth’s oblateness. J. Geophys. Res. 2013, 118, 740–747. [Google Scholar] [CrossRef]
- Kusche, J.; Schmidt, R.; Petrovic, S.; Rietbroek, R. Decorrelated GRACE time-variable gravity solutions by GFZ, and their validation using a hydrological model. J. Geod. 2009, 83, 903–913. [Google Scholar] [CrossRef]
- Vestøl, O.; Ågren, J.; Steffen, H.; Tarasov, L. NKG2016LU: A new land uplift model for Fennoscandia and the Baltic Region. J. Geod. 2019, 93, 1759–1779. [Google Scholar] [CrossRef]
- MATLAB; Version 8.6 (Matlab R2015b); The MathWorks Inc.: Natick, MA, USA, 2015.


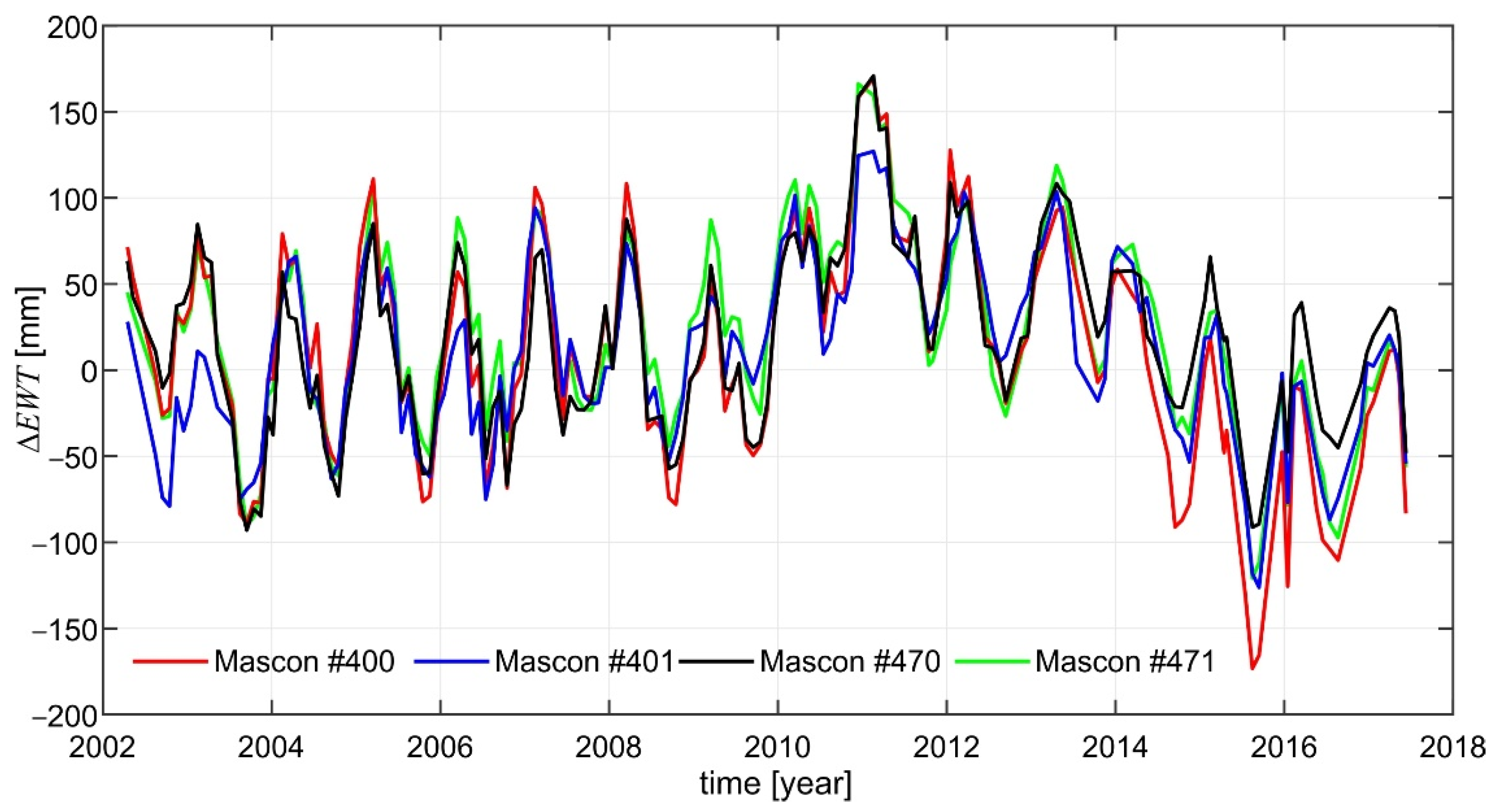


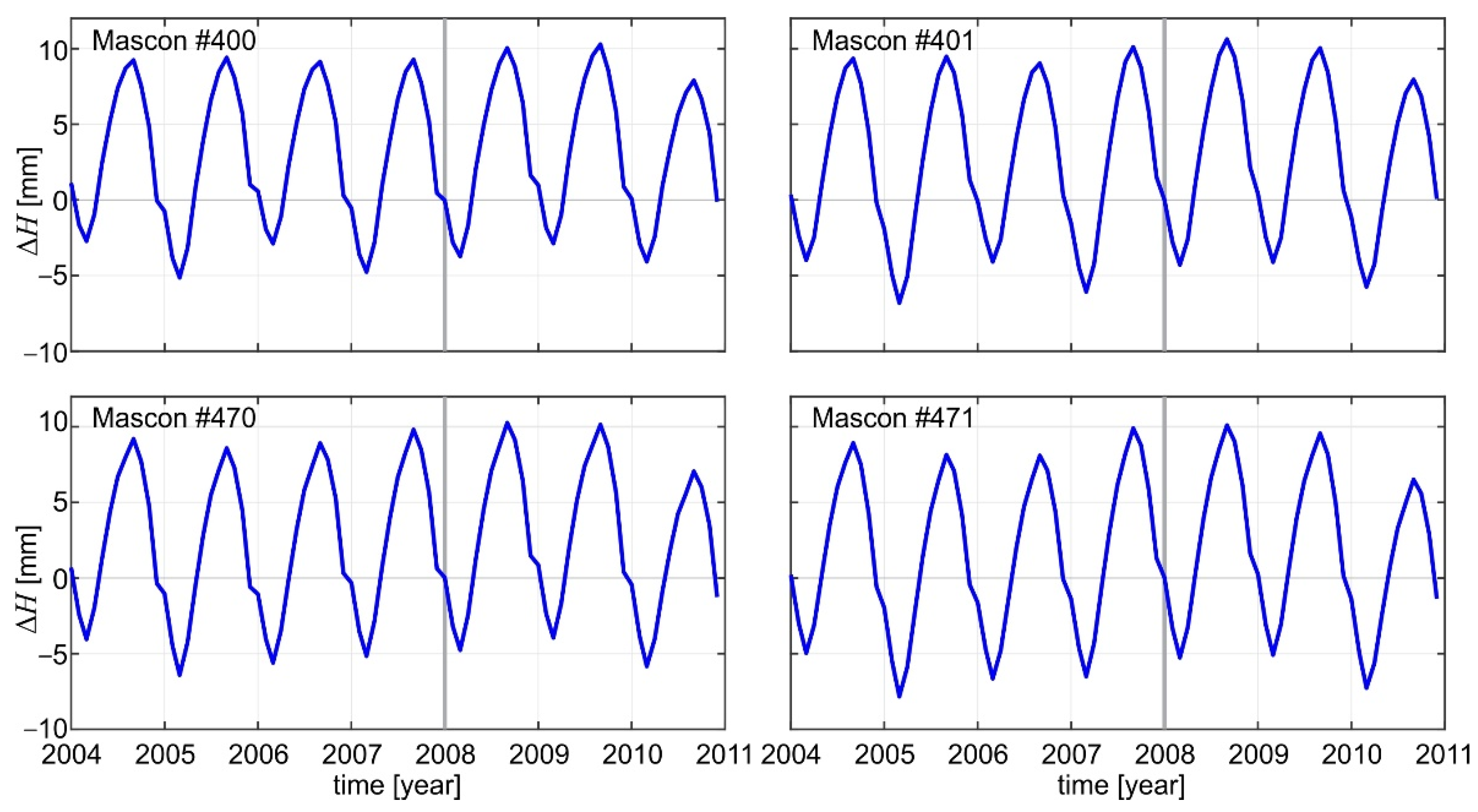


| Mascon | Month | δP1 | δP2 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Min | Max | Mean | Std | Min | Max | Mean | Std | ||
| #400 | 1st | 0.2 | −1.6 | −0.7 | 0.6 | 1.8 | −2.2 | −0.9 | 1.6 |
| 2nd | 0.7 | −1.3 | −0.7 | 0.8 | 2.3 | −2.6 | −0.5 | 2.0 | |
| 3rd | 1.1 | −1.4 | −0.4 | 1.1 | 2.5 | −2.9 | −0.4 | 2.4 | |
| 4th | 0.6 | −2.1 | −0.9 | 1.1 | 1.4 | −3.7 | −1.1 | 2.3 | |
| 5th | 2.5 | −0.9 | 0.7 | 1.2 | 2.3 | −2.6 | 0.5 | 2.0 | |
| 6th | 2.4 | −1.1 | 0.7 | 1.2 | 1.9 | −2.2 | 0.3 | 1.5 | |
| #401 | 1st | 0.6 | −1.1 | −0.4 | 0.6 | 1.3 | −2.3 | −0.7 | 1.2 |
| 2nd | 0.9 | −1.1 | −0.5 | 0.8 | 2.6 | −2.1 | −0.4 | 1.8 | |
| 3rd | 1.1 | −1.3 | −0.3 | 1.0 | 2.5 | −2.2 | −0.3 | 2.1 | |
| 4th | 0.7 | −1.5 | −0.4 | 0.9 | 1.9 | −2.5 | −0.5 | 2.0 | |
| 5th | 2.1 | −1.0 | 0.6 | 1.1 | 2.4 | −2.3 | 0.6 | 1.9 | |
| 6th | 2.2 | −0.5 | 1.0 | 1.0 | 2.3 | −1.5 | 0.9 | 1.4 | |
| #470 | 1st | −1.1 | −2.9 | −1.9 | 0.7 | 0.1 | −4.0 | −2.0 | 1.6 |
| 2nd | −1.0 | −2.6 | −1.9 | 0.6 | 0.1 | −3.6 | −1.4 | 1.5 | |
| 3rd | −1.0 | −2.1 | −1.6 | 0.3 | 0.3 | −3.1 | −1.2 | 1.4 | |
| 4th | −1.0 | −1.7 | −1.3 | 0.3 | 0.5 | −2.8 | −1.3 | 1.4 | |
| 5th | −0.4 | −1.3 | −0.8 | 0.3 | 1.1 | −3.0 | −1.2 | 1.7 | |
| 6th | 0.5 | −0.9 | −0.1 | 0.5 | 1.4 | −2.9 | −1.0 | 1.8 | |
| #471 | 1st | −1.4 | −3.1 | −2.2 | 0.7 | −0.5 | −4.2 | −2.3 | 1.5 |
| 2nd | −1.7 | −3.3 | −2.6 | 0.7 | −0.6 | −4.4 | −2.3 | 1.5 | |
| 3rd | −1.7 | −2.7 | −2.3 | 0.4 | −0.4 | −3.8 | −2.0 | 1.2 | |
| 4th | −1.9 | −2.3 | −2.1 | 0.2 | −0.6 | −3.4 | −2.1 | 1.2 | |
| 5th | −1.2 | −1.7 | −1.4 | 0.2 | 0.0 | −2.9 | −1.8 | 1.3 | |
| 6th | −0.5 | −1.1 | −0.7 | 0.2 | 0.7 | −2.9 | −1.3 | 1.5 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szelachowska, M.; Godah, W.; Krynski, J. Contribution of GRACE Satellite Mission to the Determination of Orthometric/Normal Heights Corrected for Their Dynamics—A Case Study of Poland. Remote Sens. 2022, 14, 4271. https://doi.org/10.3390/rs14174271
Szelachowska M, Godah W, Krynski J. Contribution of GRACE Satellite Mission to the Determination of Orthometric/Normal Heights Corrected for Their Dynamics—A Case Study of Poland. Remote Sensing. 2022; 14(17):4271. https://doi.org/10.3390/rs14174271
Chicago/Turabian StyleSzelachowska, Malgorzata, Walyeldeen Godah, and Jan Krynski. 2022. "Contribution of GRACE Satellite Mission to the Determination of Orthometric/Normal Heights Corrected for Their Dynamics—A Case Study of Poland" Remote Sensing 14, no. 17: 4271. https://doi.org/10.3390/rs14174271
APA StyleSzelachowska, M., Godah, W., & Krynski, J. (2022). Contribution of GRACE Satellite Mission to the Determination of Orthometric/Normal Heights Corrected for Their Dynamics—A Case Study of Poland. Remote Sensing, 14(17), 4271. https://doi.org/10.3390/rs14174271