Longitudinal Structure in the Altitude of the Sporadic E Observed by COSMIC in Low-Latitudes
Abstract
:1. Introduction
2. Data
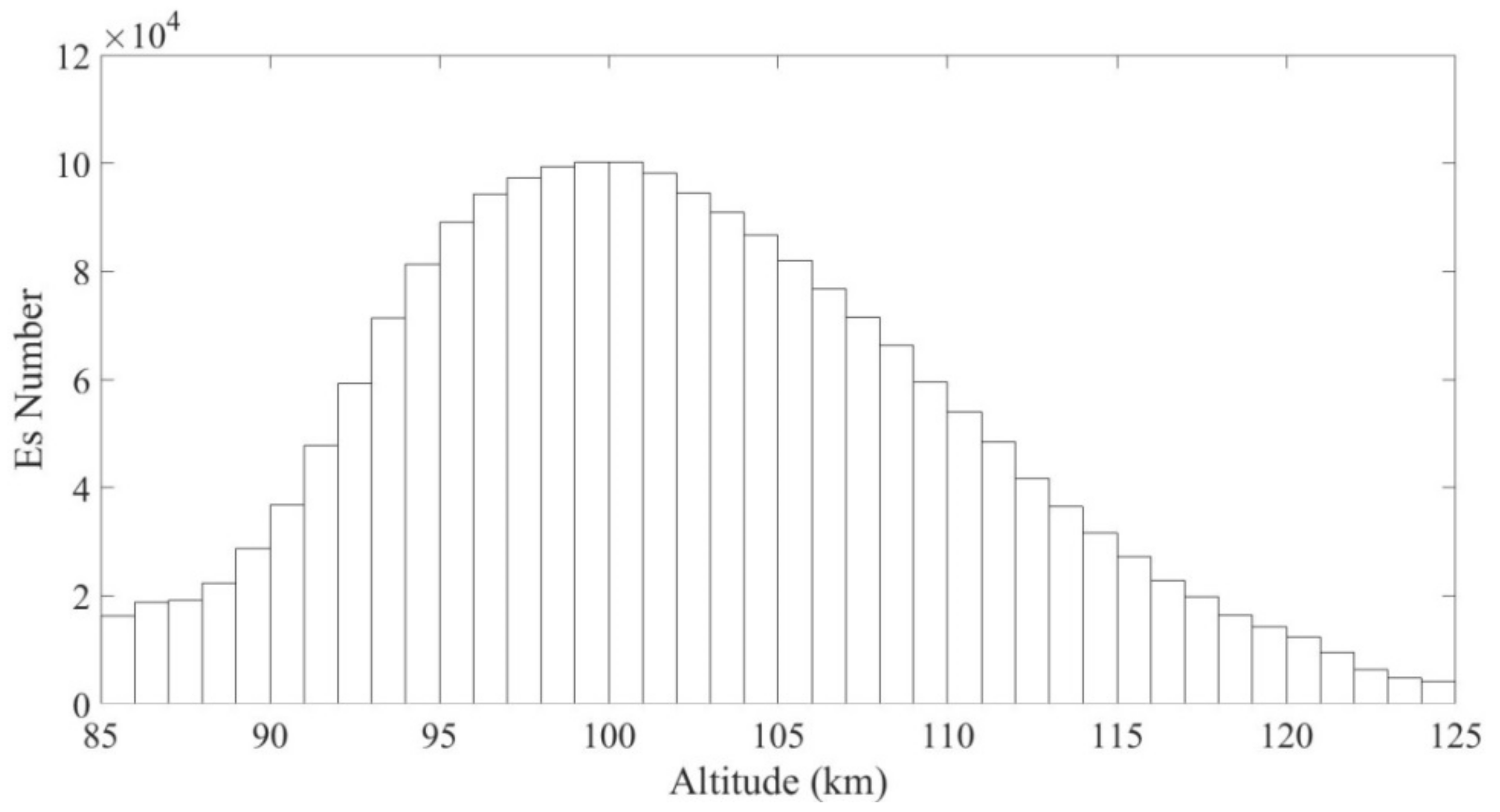
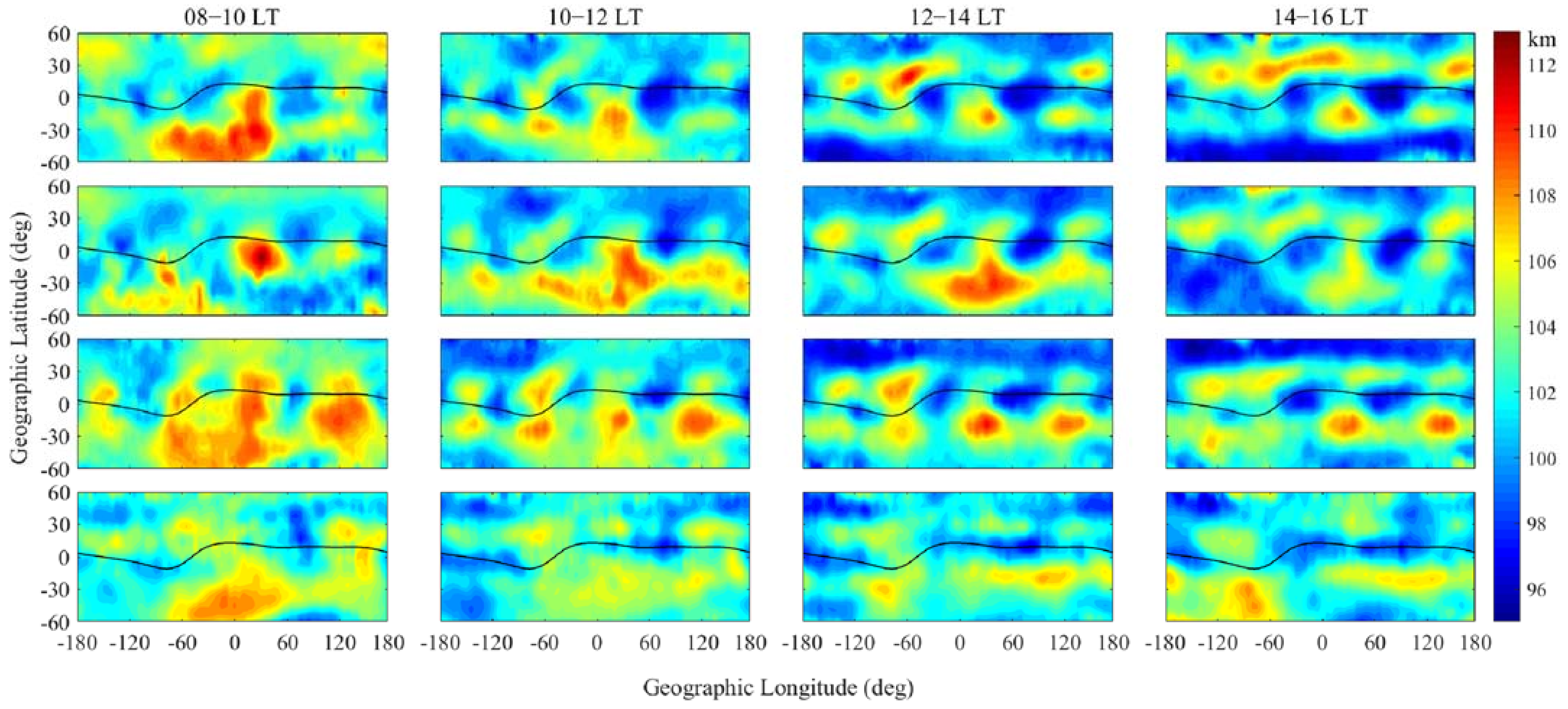
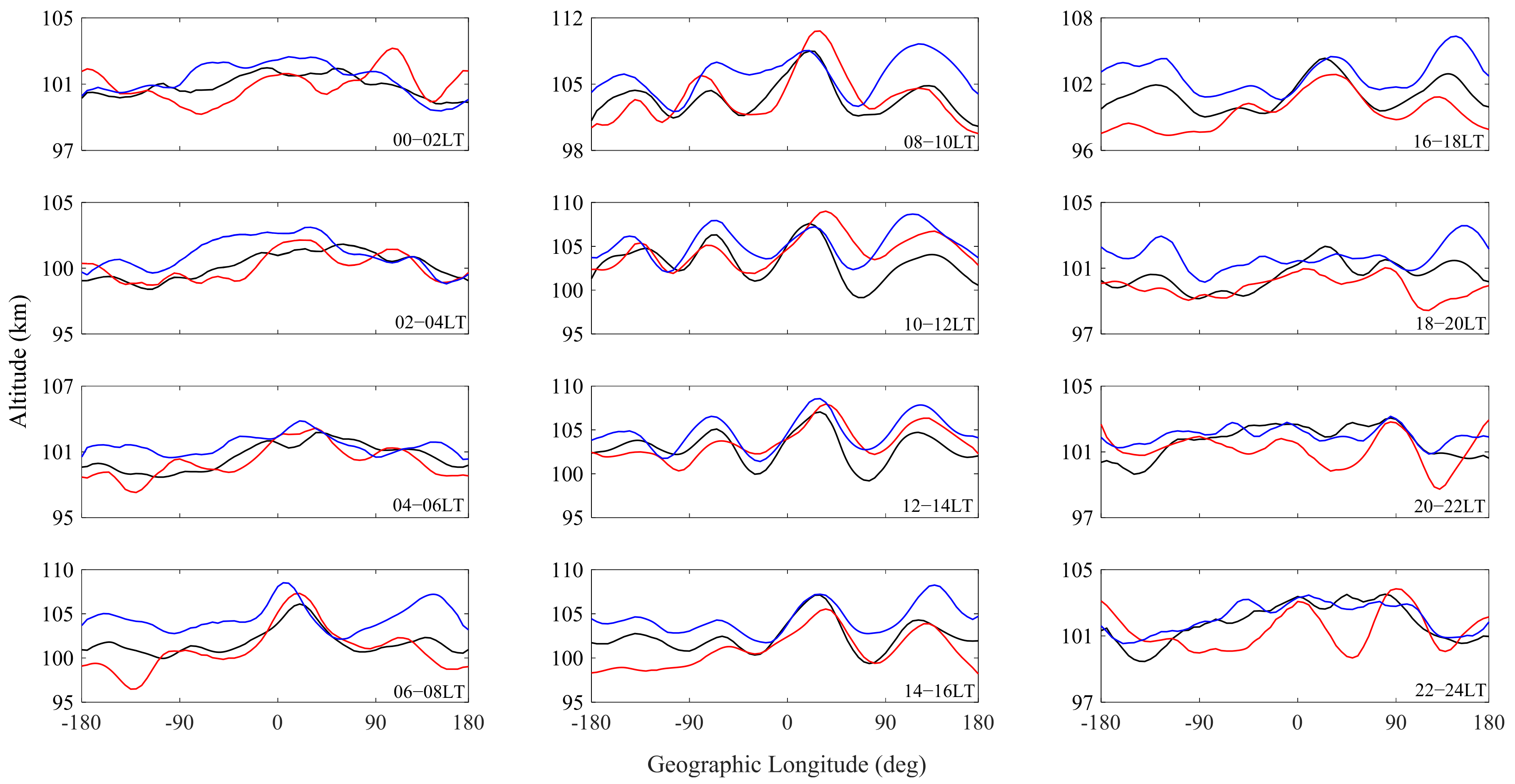
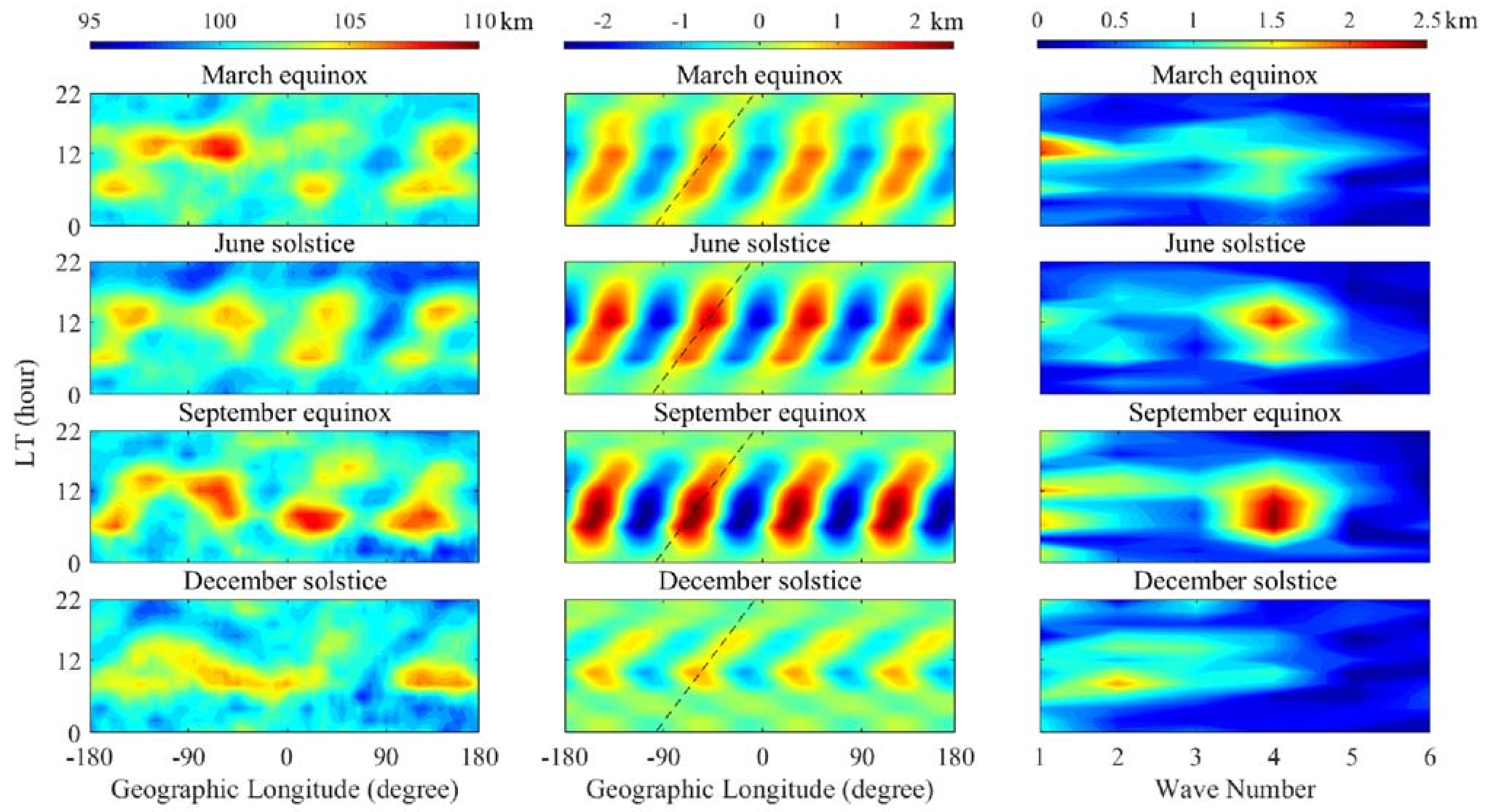
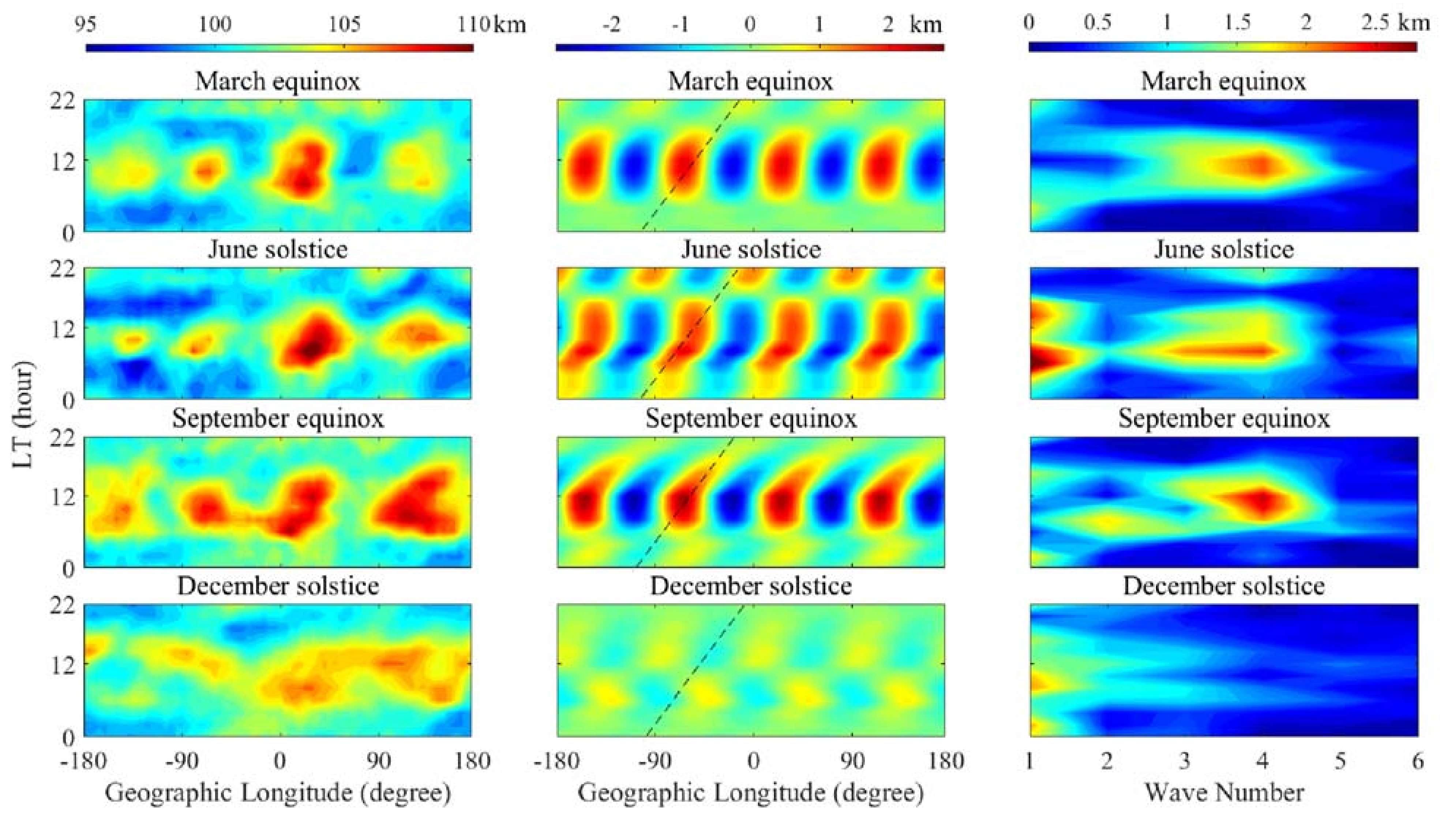
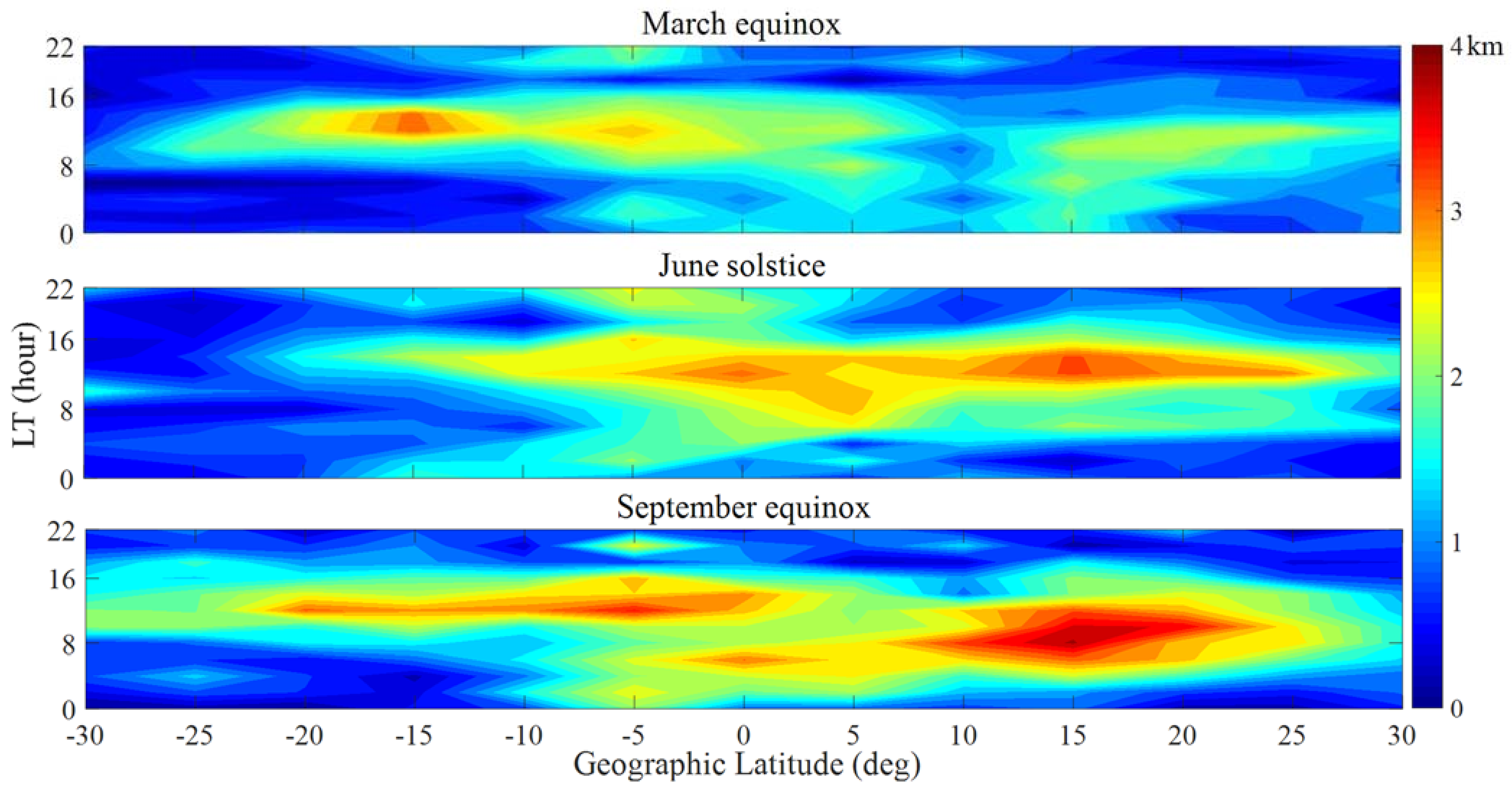
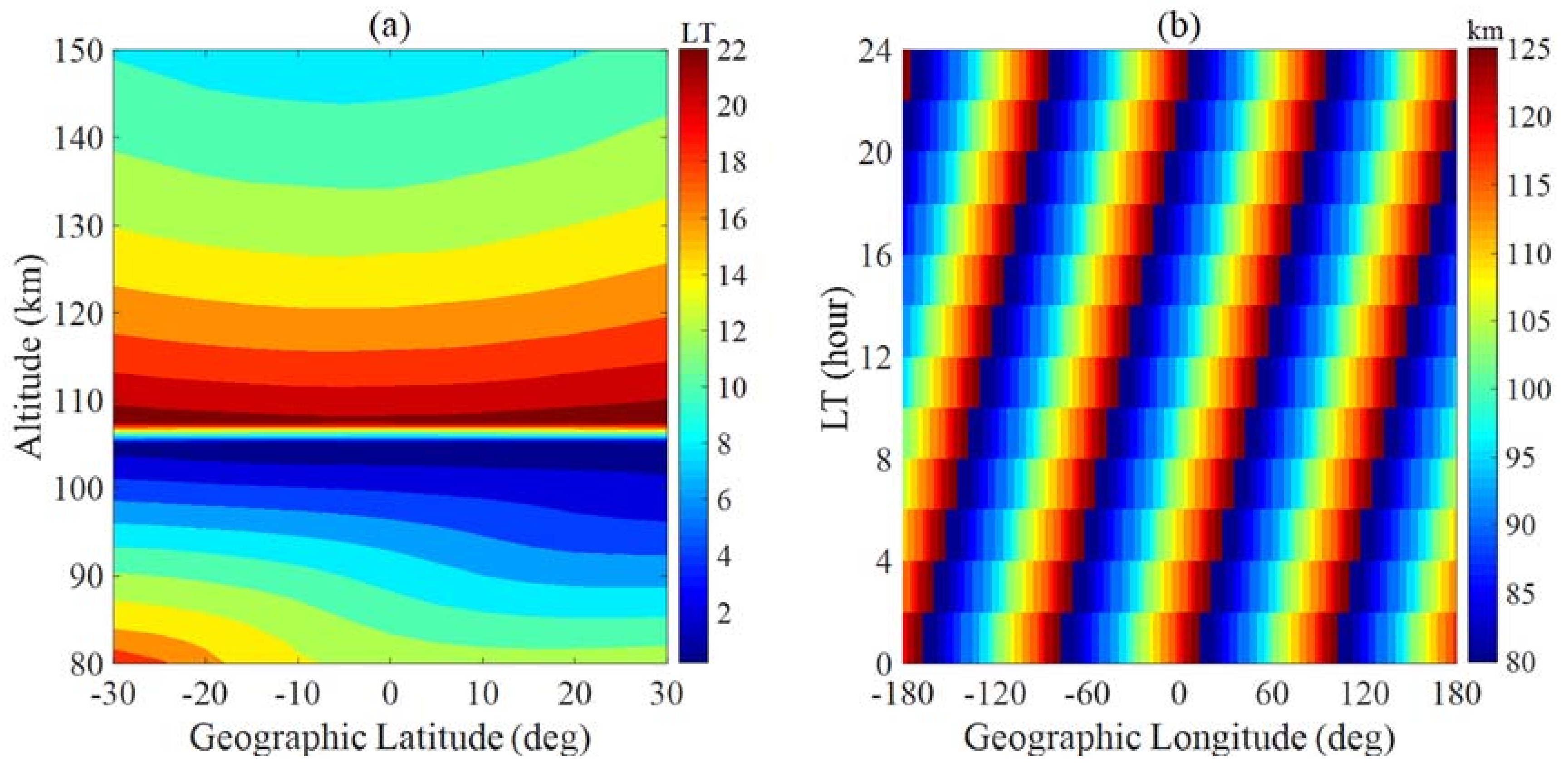
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pavelyev, A.G.; Liou, Y.A.; Wickert, J.; Schmidt, T.; Liu, S.F. Effects of the ionosphere and solar activity on radio occultation signals: Application to CHAllenging Minisatellite Payload satellite observations. J. Geophys. Res. Space Phys. 2007, 112, 112. [Google Scholar] [CrossRef] [Green Version]
- Wu, D.L.; Ao, C.O.; Hajj, G.A.; de La Torre Juarez, M.; Mannucci, A.J. Sporadic Emorphology from GPS-CHAMP radio occultation. J. Geophys. Res. Space Phys. 2005, 110. [Google Scholar] [CrossRef] [Green Version]
- Arras, C.; Wickert, J.; Beyerle, G.; Heise, S.; Schmidt, T.; Jacobi, C. A global climatology of ionospheric irregularities derived from GPS radio occultation. Geophys. Res. Lett. 2008, 35. [Google Scholar] [CrossRef]
- Chu, Y.H.; Brahmanandam, P.S.; Wang, C.Y.; Su, C.L.; Kuong, R.M. Coordinated sporadic E layer observations made with Chung-Li 30MHz radar, ionosonde and FORMOSAT-3/COSMIC satellites. J. Atmos. Solar Terr. Phys. 2011, 73, 883–894. [Google Scholar] [CrossRef]
- Chu, Y.H.; Wang, C.Y.; Wu, K.H.; Chen, K.T.; Tzeng, K.J.; Su, C.L.; Feng, W.; Plane, J. Morphology of sporadicElayer retrieved from COSMIC GPS radio occultation measurements: Wind shear theory examination. J. Geophys. Res. Space Phys. 2014, 119, 2117–2136. [Google Scholar] [CrossRef]
- Arras, C.; Jacobi, C.; Wickert, J. Semidiurnal tidal signature in sporadic E occurrence rates derived from GPS radio occultation measurements at higher midlatitudes. Ann. Geophys. 2009, 27, 2555–2563. [Google Scholar] [CrossRef] [Green Version]
- Haldoupis, C. A Tutorial Review on Sporadic E Layers. In Aeronomy of the Earth’s Atmosphere and Ionosphere; Springer International Publishing: Berlin, Germany, 2011; pp. 381–394. [Google Scholar]
- Cai, X.; Yuan, T.; Eccles, J.V.; Raizada, S. Investigation on the Distinct Nocturnal Secondary Sodium Layer Behavior Above 95 km in Winter and Summer Over Logan, UT (41.7° N, 112°W) and Arecibo Observatory, PR (18.3° N, 67°W). J. Geophys. Res. Space Phys. 2019, 124, 9610–9625. [Google Scholar] [CrossRef]
- Friedman, J.; Gonzalez, S.A.; Tepley, C.A.; Zhou, Q.; Sulzer, M.P.; Collins, S.C.; Grime, B.W. Simultaneous atomic and ion layer enhancements observed in the mesopause region over Arecibo during the Coqui II Sounding Rocket Campaign. Geophys. Res. Lett. 2000, 27, 449–452. [Google Scholar] [CrossRef] [Green Version]
- Yuan, T.; Wang, J.; Cai, X.; Sojka, J.; Rice, D.; Oberheide, J.; Criddle, N. Investigation of the seasonal and local time variations of the high-altitude sporadic Na layer (Nas) formation and the associated midlatitude descendingElayer (Es) in lowerEregion. J. Geophys. Res. Space Phys. 2014, 119, 5985–5999. [Google Scholar] [CrossRef]
- Haldoupis, C.; Pancheva, D. Planetary waves and midlatitude sporadic E layers: Strong experimental evidence for a close relationship. J. Geophys. Res. Space Phys. 2002, 107, A6. [Google Scholar] [CrossRef]
- Haldoupis, C.; Pancheva, D.; Singer, W.; Meek, C.; Macdougall, J. An explanation for the seasonal dependence of midlatitude sporadic E layers. J. Geophys. Res. Space Phys. 2007, 112, A6. [Google Scholar] [CrossRef]
- Arras, C.; Jacobi, C.; Wickert, J.; Heise, S.; Schmidt, T. Analysis of sporadic E variability derived from GPS radio occultation measurements and possible links to dynamics. In Proceedings of the EGU General Assembly Conference, Vienna, Austria, 21 April 2009. [Google Scholar]
- Forbes, J.M.; Zhang, X.; Palo, S.; Russell, J.; Mertens, C.J.; Mlynczak, M. Tidal variability in the ionospheric dynamo region. J. Geophys. Res. Space Phys. 2008, 113. [Google Scholar] [CrossRef]
- Haldoupis, C.; Pancheva, D. Terdiurnal tidelike variability in sporadic E layers. J. Geophys. Res. Space Phys. 2006, 111, A7. [Google Scholar] [CrossRef]
- Haldoupis, C.; Pancheva, D.; Mitchell, N.J. A study of tidal and planetary wave periodicities present in midlatitude sporadic E layers. J. Geophys. Res. Space Phys. 2004, 109, A2. [Google Scholar] [CrossRef]
- Christakis, N.; Haldoupis, C.; Zhou, Q.; Meek, C. Seasonal variability and descent of mid-latitude sporadic E layers at Arecibo. Ann. Geophys. 2009, 27, 923–931. [Google Scholar] [CrossRef] [Green Version]
- Mathews, J. Sporadic E: Current views and recent progress. J. Atmos. Solar Terr. Phys. 1998, 60, 413–435. [Google Scholar] [CrossRef]
- Haldoupis, C.; Meek, C.; Christakis, N.; Pancheva, D.; Bourdillon, A. Ionogram height–time–intensity observations of descending sporadic E layers at mid-latitude. J. Atmos. Sol. Terr. Phys. 2006, 68, 539–557. [Google Scholar] [CrossRef] [Green Version]
- Hagan, M.E.; Forbes, J.M. Migrating and nonmigrating diurnal tides in the middle and upper atmosphere excited by tropospheric latent heat release. J. Geophys. Res. Space Phys. 2002, 107, D24. [Google Scholar] [CrossRef]
- Lühr, H.; Rother, M.; Häusler, K.; Alken, P.; Maus, S. The influence of nonmigrating tides on the longitudinal variation of the equatorial electrojet. J. Geophys. Res. Space Phys. 2008, 113, A8. [Google Scholar] [CrossRef]
- Hartman, W.A.; Heelis, R. Longitudinal variations in the equatorial vertical drift in the topside ionosphere. J. Geophys. Res. Space Phys. 2007, 112, 112. [Google Scholar] [CrossRef]
- Li, G.; Ning, B.; Liu, L.; Zhao, B.; Yue, X.; Su, S.-Y.; Venkatraman, S. Correlative study of plasma bubbles, evening equatorial ionization anomaly, and equatorial prereversalE×Bdrifts at solar maximum. Radio Sci. 2008, 43. [Google Scholar] [CrossRef]
- Wan, W.; Liu, L.; Pi, X.; Zhang, M.-L.; Ning, B.; Xiong, J.; Ding, F. Wavenumber-4 patterns of the total electron content over the low latitude ionosphere. Geophys. Res. Lett. 2008, 35, 150–152. [Google Scholar] [CrossRef]
- Lin, C.H.; Hsiao, C.C.; Liu, J.Y.; Liu, C.H. Longitudinal structure of the equatorial ionosphere: Time evolution of the four-peaked EIA structure. J. Geophys. Res. Space Phys. 2007, 112, A12. [Google Scholar] [CrossRef]
- Liu, Z.; Fang, H.; Hoque, M.M.; Weng, L.; Yang, S.; Gao, Z. A New Empirical Model of NmF2 Based on CHAMP, GRACE, and COSMIC Radio Occultation. Remote Sens. 2019, 11, 1386. [Google Scholar] [CrossRef] [Green Version]
- Oberheide, J.; Forbes, J.M. Thermospheric nitric oxide variability induced by nonmigrating tides. Geophys. Res. Lett. 2008, 35, 35. [Google Scholar] [CrossRef]
- Häusler, K.; Lühr, H.; Rentz, S.; Köhler, W. A statistical analysis of longitudinal dependences of upper thermospheric zonal winds at dip equator latitudes derived from CHAMP. J. Atmos. Solar Terr. Phys. 2007, 69, 1419–1430. [Google Scholar] [CrossRef]
- Liu, Z.; Fang, H.; Yue, X.; Lyu, H. Wavenumber-4 Patterns of the Sporadic E Over the Middle- and Low-Latitudes. J. Geophys. Res. Space Phys. 2021, 126, 029238. [Google Scholar] [CrossRef]
- Niu, J. Relationship Between Wavenumber 4 Pattern of Sporadic E Layer Intensity and Eastward Propagating Diurnal Tide With Zonal Wavenumber 3 in Low Latitude Region. J. Geophys. Res. Space Phys. 2021, 126, 028985. [Google Scholar] [CrossRef]
- Schreiner, W.; Rocken, C.; Sokolovskiy, S.; Syndergaard, S.; Hunt, D. Estimates of the precision of GPS radio occultations from the COSMIC/FORMOSAT-3 mission. Geophys. Res. Lett. 2007, 34, 545–559. [Google Scholar] [CrossRef] [Green Version]
- Brahmanandam, P.S.; Uma, G.; Liu, J.Y.; Chu, Y.H.; Devi, N.S.M.P.L.; Kakinami, Y. Global S4 index variations observed using FORMOSAT-3/COSMIC GPS RO technique during a solar minimum year. J. Geophys. Res. Space Phys. 2012, 117, A9. [Google Scholar] [CrossRef]
- Yue, X.; Schreiner, W.S.; Zeng, Z.; Kuo, Y.-H.; Xue, X. Case study on complex sporadic E layers observed by GPS radio occultations. Atmos. Meas. Tech. 2015, 8, 225–236. [Google Scholar] [CrossRef] [Green Version]
- Yue, X.; Schreiner, W.S.; Pedatella, N.M.; Kuo, Y. Characterizing GPS radio occultation loss of lock due to ionospheric weather. Space Weather. 2016, 14, 285–299. [Google Scholar] [CrossRef] [Green Version]
- Yu, B.; Xue, X.; Yue, X.; Yang, C.; Yu, C.; Dou, X.; Ning, B.; Hu, L. The global climatology of the intensity of the ionospheric sporadic E layer. Atmos. Chem. Phys. Discuss. 2019, 19, 4139–4151. [Google Scholar] [CrossRef] [Green Version]
- Yu, B.; Xue, X.; Scott, C.J.; Wu, J.; Yue, X.; Feng, W.; Chi, Y.; Marsh, D.R.; Liu, H.; Dou, X.; et al. Interhemispheric transport of metallic ions within ionospheric sporadic E layers by the lower thermospheric meridional circulation. Atmos. Chem. Phys. Discuss. 2021, 21, 4219–4230. [Google Scholar] [CrossRef]
- Qiu, L.; Zuo, X.; Yu, T.; Sun, Y.; Qi, Y. Comparison of global morphologies of vertical ion convergence and sporadic E oc-currence rate. Adv. Space Res. 2019, 63, 3606–3611. [Google Scholar] [CrossRef]
- Arras, C.; Wickert, J. Estimation of ionospheric sporadic E intensities from GPS radio occultation measurements. J. Atmos. Solar Terr. Phys. 2018, 171, 60–63. [Google Scholar] [CrossRef]
- Häusler, K.; Luhr, H. Nonmigrating tidal signals in the upper thermospheric zonal wind at equatorial latitudes as observed by CHAMP. Ann. Geophys. 2009, 27, 2643–2652. [Google Scholar] [CrossRef] [Green Version]
- Abdu, M.A.; Macdougall, J.W.; Batista, I.S.; Sobral, J.H.A.; Jayachandran, P.T. Equatorial evening prereversal electric field enhancement and sporadic E layer disruption: A manifestation of E and F region coupling. J. Geophys. Res. Space Phys. 2003, 108, A6. [Google Scholar] [CrossRef] [Green Version]
- Resende, L.C.A.; Batista, I.S.; Denardini, C.M.; Batista, P.; Carrasco, A.J.; Andrioli, V.F.; Moro, J. The influence of tidal winds in the formation of blanketing sporadic e-layer over equatorial Brazilian region. J. Atmos. Solar Terr. Phys. 2018, 171, 64–71. [Google Scholar] [CrossRef]
- Resende, L.C.A.; Arras, C.; Batista, I.S.; Denardini, C.M.; Bertollotto, T.O.; Moro, J. Study of sporadic E layers based on GPS radio occultation measurements and digisonde data over the Brazilian region. Ann. Geophys. 2018, 36, 587–593. [Google Scholar] [CrossRef] [Green Version]
- Resende, L.C.A.; Batista, I.S.; Denardini, C.M.; Batista, P.; Carrasco, A.J.; Andrioli, V.D.F.; Moro, J. Simulations of blanketing sporadic E-layer over the Brazilian sector driven by tidal winds. J. Atmos. Solar Terr. Phys. 2017, 154, 104–114. [Google Scholar] [CrossRef]
- Carrasco, A.J.; Batista, I.S.; Abdu, M.A. Simulation of the sporadic E layer response to prereversal associated evening vertical electric field enhancement near dip equator. J. Geophys. Res. 2007, 112, A6. [Google Scholar]
- Cai, X.; Yuan, T.; Eccles, J.V.; Pedatella, N.M.; Xi, X.; Ban, C.; Liu, A.Z. A Numerical Investigation on the Variation of Sodium Ion and Observed Thermospheric Sodium Layer at Cerro Pachón, Chile During Equinox. J. Geophys. Res. Space Phys. 2019, 124, 10395–10414. [Google Scholar] [CrossRef]
- Cai, X.; Yuan, T.; Eccles, J.V. A Numerical Investigation on Tidal and Gravity Wave Contributions to the Summer Time Na Variations in the Midlatitude E Region. J. Geophys. Res. Space Phys. 2017, 122, 577. [Google Scholar] [CrossRef]
- Haldoupis, C.; Shalimov, S. On the altitude dependence and role of zonal and meridional wind shears in the generation of E region metal ion layers. J. Atmos. Solar Terr. Phys. 2021, 214, 105537. [Google Scholar] [CrossRef]
- Shinagawa, H.; Miyoshi, Y.; Jin, H.; Fujiwara, H. Global distribution of neutral wind shear associated with sporadic E layers derived from GAIA. J. Geophys. Res. Space Phys. 2017, 122, 4450–4465. [Google Scholar] [CrossRef]
- Liu, Y.; Zhou, C.; Tang, Q.; Li, Z.; Song, Y.; Qing, H.; Ni, B.; Zhao, Z.; Li, Z. The seasonal distribution of sporadic E layers observed from radio occultation measurements and its relation with wind shear measured by TIMED/TIDI. Adv. Space Res. 2018, 62, 426–439. [Google Scholar] [CrossRef]
- Oberheide, J.; Forbes, J.; Zhang, X.; Bruinsma, S.L. Climatology of upward propagating diurnal and semidiurnal tides in the thermosphere. J. Geophys. Res. Space Phys. 2011, 116, 116. [Google Scholar] [CrossRef]
- Nygrén, T.; Jalonen, L.; Oksman, J.; Turunen, T. The role of electric field and neutral wind direction in the formation of sporadic E-layers. J. Atmos. Terr. Phys. 1984, 46, 373–381. [Google Scholar] [CrossRef]
- Jin, H.; Miyoshi, Y.; Fujiwara, H.; Shinagawa, H. Electrodynamics of the formation of ionospheric wave number 4 longitudinal structure. J. Geophys. Res. Space Phys. 2008, 113, 113. [Google Scholar] [CrossRef]
- Förster, M.; Cnossen, I. Upper atmosphere differences between northern and southern high latitudes: The role of magnetic field asymmetry. J. Geophys. Res. Space Phys. 2013, 118, 5951–5966. [Google Scholar] [CrossRef] [Green Version]
- Plane, J. Atmospheric Chemistry of Meteoric Metals. Chem. Rev. 2003, 103, 4963–4984. [Google Scholar] [CrossRef] [PubMed]
- Bishop, R.L.; Earle, G.D.; Larsen, M.F.; Swenson, C.M.; Carlson, C.G.; Roddy, P.A.; Fish, C.; Bullett, T.W. Sequential observations of the local neutral wind field structure associated with E region plasma layers. J. Geophys. Res. Space Phys. 2005, 110, 04309. [Google Scholar] [CrossRef]
- Yeh, W.-H.; Liu, J.-Y.; Huang, C.-Y.; Chen, S.-P. Explanation of the sporadic-Elayer formation by comparing FORMOSAT-3/COSMIC data with meteor and wind shear information. J. Geophys. Res. Atmos. 2014, 119, 4568–4579. [Google Scholar] [CrossRef]
- Dalakishvili, G.; Didebulidze, G.; Todua, M. Formation of sporadic E (Es) layer by homogeneous and inhomogeneous horizontal winds. J. Atmos. Solar Terr. Phys. 2020, 209, 105403. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Li, Q.; Fang, H.; Gao, Z. Longitudinal Structure in the Altitude of the Sporadic E Observed by COSMIC in Low-Latitudes. Remote Sens. 2021, 13, 4714. https://doi.org/10.3390/rs13224714
Liu Z, Li Q, Fang H, Gao Z. Longitudinal Structure in the Altitude of the Sporadic E Observed by COSMIC in Low-Latitudes. Remote Sensing. 2021; 13(22):4714. https://doi.org/10.3390/rs13224714
Chicago/Turabian StyleLiu, Zhendi, Qingfeng Li, Hanxian Fang, and Ze Gao. 2021. "Longitudinal Structure in the Altitude of the Sporadic E Observed by COSMIC in Low-Latitudes" Remote Sensing 13, no. 22: 4714. https://doi.org/10.3390/rs13224714
APA StyleLiu, Z., Li, Q., Fang, H., & Gao, Z. (2021). Longitudinal Structure in the Altitude of the Sporadic E Observed by COSMIC in Low-Latitudes. Remote Sensing, 13(22), 4714. https://doi.org/10.3390/rs13224714





