Sustainability Analysis of Supply Chains with Fashion Products under Alternative Power Structures and Loss-Averse Supplier
Abstract
:1. Introduction
2. Literature Review
3. The Model
- 1.
- is an increasing function of q;
- 2.
- is a decreasing function of q, ;
- 3.
- is a decreasing function of q, .
4. Sustainability Analysis
4.1. Loss-Neutral Supplier
- 1.
- , for any ;
- 2.
- , for any .
4.2. Loss-Averse Supplier
- 1.
- is increasing in when ; and
- 2.
- is decreasing in when .
- 1.
- , when ;
- 2.
- , when .
- 1.
- in Scenario SA,
- (a)
- , when ;
- (b)
- , when ;
- 2.
- in Scenario RA,
- (a)
- , when ;
- (b)
- , when .
5. Conclusions
- Supply chain sustainability is increasing (decreasing) in the buy-back price in the supplier-as-the-leader (retailer-as-the-leader) scenario.
- With loss neutral supplier, the buy-back contract results in a lower sustainability than the wholesale price contract.
- Supplier’s loss-averseness helps to improve supply chain sustainability.
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
References
- Sarkar, B.; Saren, S.; Sinha, D.; Hur, S. Effect of Unequal Lot Sizes, Variable Setup Cost, and Carbon Emission Cost in a Supply Chain Model. Math. Probl. Eng. 2015. [Google Scholar] [CrossRef]
- Sarkar, B.; Saren, S.; Sarkar, M.; Seo, Y.W. A Stackelberg Game Approach in an Integrated Inventory Model with Carbon-Emission and Setup Cost Reduction. Sustainability 2016, 8, 1244. [Google Scholar] [CrossRef]
- Sarkar, B.; Ganguly, B.; Sarkar, M.; Pareek, S. Effect of variable transportation and carbon emission in a three-echelon supply chain model. Trans. Res. Part E 2016, 91, 112–128. [Google Scholar] [CrossRef]
- European Commission (EC). Environmental Impact of Products. Available online: http://ec.europa.eu/environment/ipp/pdf/eipro_report.pdf (accessed on 26 February 2017).
- Shen, B.; Zheng, J.; Chow, P.; Chow, K. Perception of fashion sustainability in online community. J. Text. Inst. 2014, 9, 971–979. [Google Scholar] [CrossRef]
- Sarkar, B. Supply Chain Coordination with Variable Backorder, Inspections, and Discount Policy for Fixed Lifetime Products. Math. Probl. Eng. 2016. [Google Scholar] [CrossRef]
- United States Fashion Industry Association (USFIA). 2016. Available online: http://www.usfashionindustry.com/pdf_files/USFIA-2016-Fashion-Industry-Benchmarking-Study.pdf (accessed on 26 February 2017).
- Xue, W.; Demirag, O.C.; Niu, B.Z. Supply chain performance and consumer surplus under alternative structures of channel dominance. Eur. J. Oper. Res. 2014, 239, 130–145. [Google Scholar] [CrossRef]
- Choi, T.M.; Chiu, C.H. Mean-downside-risk and mean-variance newsvendor models: Implications for sustainable fashion retailing. Int. J. Prod. Econ. 2012, 135, 552–560. [Google Scholar] [CrossRef]
- Li, J.; Choi, T.M.; Cheng, T.C.E. Mean variance analysis of fast fashion supply chains with returns policy. IEEE Trans. Syst. Man Cybern. Syst. 2014, 44, 422–434. [Google Scholar] [CrossRef]
- Choi, T.M. Optimal return service charging policy for a fashion mass customization program. Serv. Sci. 2013, 5, 56–68. [Google Scholar] [CrossRef]
- Zhang, Y.; Donohue, K.; Cui, T.H. Contract preferences and performance for the loss-averse supplier: Buyback vs. revenue sharing. Manag. Sci. 2015, 62, 1734–1754. [Google Scholar] [CrossRef]
- Cachon, G. Supply chain coordination with contracts. Handb. Oper. Res. Manag. Sci. 2003, 11, 227–339. [Google Scholar]
- Dong, C.; Shen, B.; Chow, P.S.; Yang, L.; Ng, C.T. Sustainability investment under cap-and-trade regulation. Ann. Oper. Res. 2016, 240, 509–531. [Google Scholar] [CrossRef]
- Wang, K.; Zhao, Y.; Cheng, Y.; Choi, T.M. Cooperation or competition? Channel choice for a remanufacturing fashion supply chain with government subsidy. Sustainability 2014, 6, 7292–7310. [Google Scholar] [CrossRef]
- Shen, B. Sustainable Fashion Supply Chain: Lessons from H&M. Sustainability 2014, 6, 6239–6249. [Google Scholar]
- Shen, B.; Li, Q. Impact of returning unsold products in retail outsourcing fashion supply chain: A sustainability analysis. Sustainability 2015, 7, 1172–1185. [Google Scholar] [CrossRef]
- Wang, F.; Zhuo, X.; Niu, B.; Rosen, M.A. Sustainability analysis and buy-back coordination in a fashion supply chain with price competition and demand uncertainty. Sustainability 2017, 9, 25. [Google Scholar] [CrossRef]
- Choi, S.C. Price competition in a channel structure with a common retailer. Mark. Sci. 1991, 10, 271–296. [Google Scholar] [CrossRef]
- Ferguson, M.E. When to commit in a serial supply chain with forecast updating. Nav. Res. Logist. 2003, 50, 917–936. [Google Scholar] [CrossRef]
- Ferguson, M.E.; DeCroix, G.A.; Zipkin, P. Commitment decisions with partial information updating. Nav. Res. Logist. 2005, 52, 780–795. [Google Scholar] [CrossRef]
- Wang, Y.; Zipkin, P. Agents’ incentives under buy-back contracts in a two-stage supply chain. Int. J. Prod. Econ. 2009, 120, 525–539. [Google Scholar] [CrossRef]
- Wang, J.C.; Lau, A.H.L.; Lau, H.S. Practical and effective contracts for the dominant retailer of a newsvendor product with price-sensitive demand. Int. J. Prod. Econ. 2012, 138, 46–54. [Google Scholar] [CrossRef]
- Wang, Y.Y.; Wang, J.C.; Shou, B. Pricing and effort investment for a newsvendor-type product. Eur. J. Oper. Res. 2013, 229, 422–432. [Google Scholar] [CrossRef]
- Donohue, K.L. Efficient supply chain contracts for fashion goods with forecast updating and two production modes. Manag. Sci. 2000, 46, 1397–1411. [Google Scholar] [CrossRef]
- Yang, D.; Choi, T.M.; Xiao, T.; Cheng, T.C.E. Coordinating a two-supplier and one-retailer supply chain with forecast updating. Automatica 2011, 47, 1317–1329. [Google Scholar] [CrossRef]
- Chiu, C.H.; Choi, T.M.; Tang, C.S. Price, rebate, and returns supply contracts for coordinating supply chains with price-dependent demands. Prod. Oper. Manag. 2011, 20, 81–91. [Google Scholar] [CrossRef]
- Zhao, Y.; Choi, T.M.; Cheng, T.C.E.; Sethi, S.; Wang, S. Buyback contracts with price-dependent demands: Effects of demand uncertainty. Eur. J. Oper. Res. 2014, 239, 663–673. [Google Scholar] [CrossRef]
- Kurata, H.; Yue, X. Trade promotion mode choice and information sharing in fashion retail supply chains. Int. J. Prod. Econ. 2008, 114, 507–519. [Google Scholar] [CrossRef]
- Liang, C.; Cakanyildirim, M.; Sethi, S.P. Analysis of Product Rollover Strategies in the Presence of Strategic Customers. Manag. Sci. 2014, 60, 1033–1056. [Google Scholar] [CrossRef]
- Lariviere, M.A. A Note on Probability Distributions with Increasing Generalized Failure Rate. Oper. Res. 2006, 53, 602–604. [Google Scholar] [CrossRef]
- Wang, Y.; Niu, B.; Guo, P. The Comparison of two vertical outsourcing structures under push and pull contracts. Prod. Oper. Manag. 2014, 23, 610–625. [Google Scholar] [CrossRef]
- Sarkar, B. An EOQ model with delay in payments and stock dependent demand. Appl. Math. Comput. 2012, 218, 8295–8308. [Google Scholar]
- Sarkar, B. An EOQ model with delay in payments and time varying deterioration rate. Math. Comput. Model. 2012, 55, 367–377. [Google Scholar] [CrossRef]
- Sarkar, B. An inventory model with reliability in an imperfect production process. Appl. Math. Comput. 2012, 218, 4881–4891. [Google Scholar] [CrossRef]
- Sarkar, B. A production-inventory model with probabilistic deterioration in two-echelon supply chain management. Appl. Math. Model. 2013, 37, 3138–3151. [Google Scholar] [CrossRef]
- Sarkar, B.; Majumder, A. Integrated vender-buyer supply chain model with vendor’s setup cost reduction. Appl. Math. Comput. 2013, 224, 362–371. [Google Scholar]



| Author(s) | Sustainability | Supply Chain Management | Structure Power | Attitude to Loss (Risk) |
|---|---|---|---|---|
| Cachon [13] | √ | |||
| Choi [19] | √ | |||
| Choi and Chiu [9] | √ | √ | ||
| Choi [11] | √ | √ | ||
| Chiu et al. [27] | √ | |||
| Dong et al. [14] | √ | √ | ||
| Donohue [25] | √ | |||
| Ferguson [20] | √ | |||
| Ferguson et al. [21] | √ | |||
| Li et al. [10] | √ | √ | ||
| Sarkar et al. [1] | √ | √ | ||
| Sarkar et al. [2] | √ | √ | ||
| Sarkar et al. [3] | √ | √ | ||
| Sarkar [6] | √ | |||
| Shen [16] | √ | |||
| Shen and Li [17] | √ | √ | ||
| Shen et al. [5] | √ | |||
| Wang et al. [18] | √ | √ | ||
| Wang et al. [23] | √ | |||
| Wang et al. [15] | √ | |||
| Wang and Zipkin [22] | √ | √ | ||
| Wang et al. [24] | √ | |||
| Xue et al. [8] | √ | |||
| Yang et al. [26] | √ | |||
| Zhao et al. [28] | √ | |||
| Zhang et al. [12] | √ | √ |
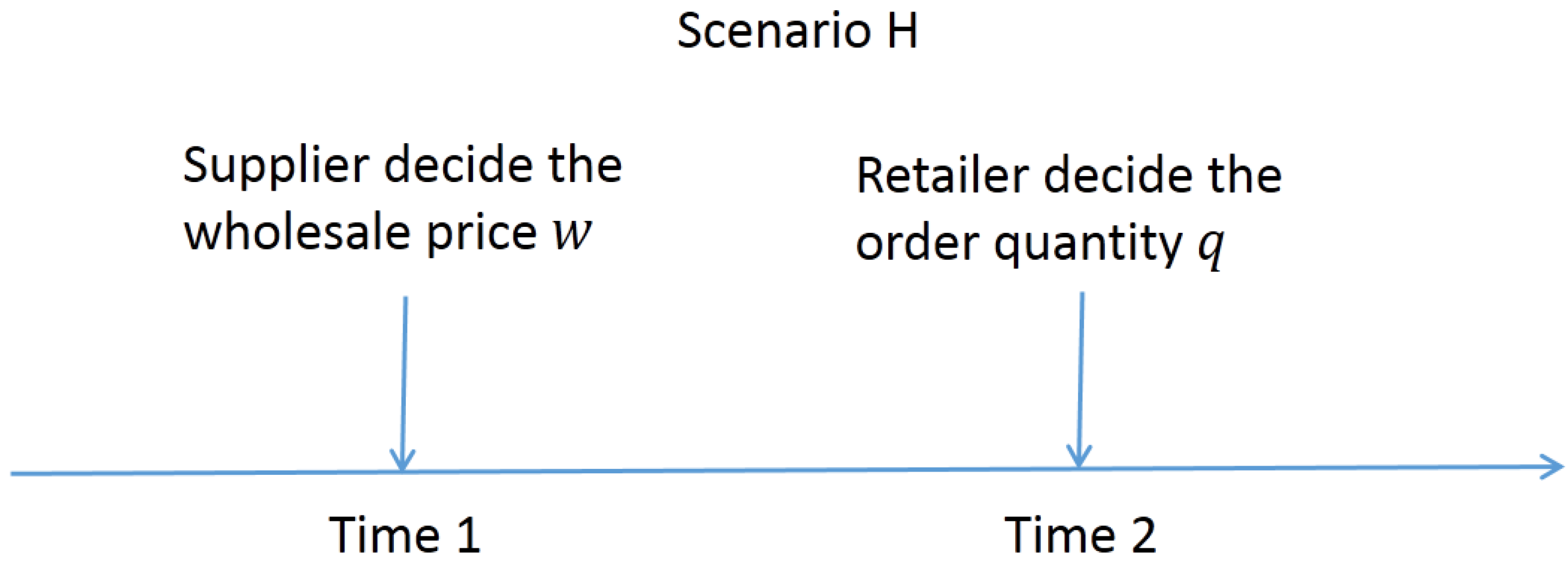
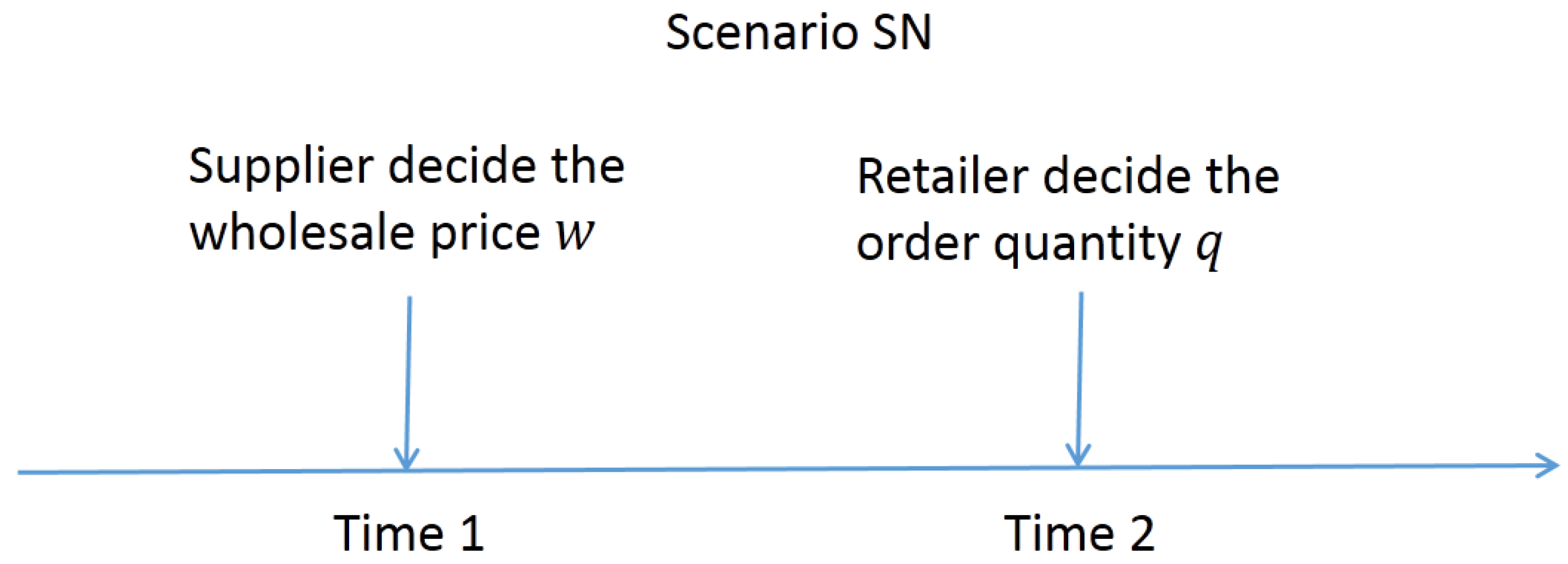
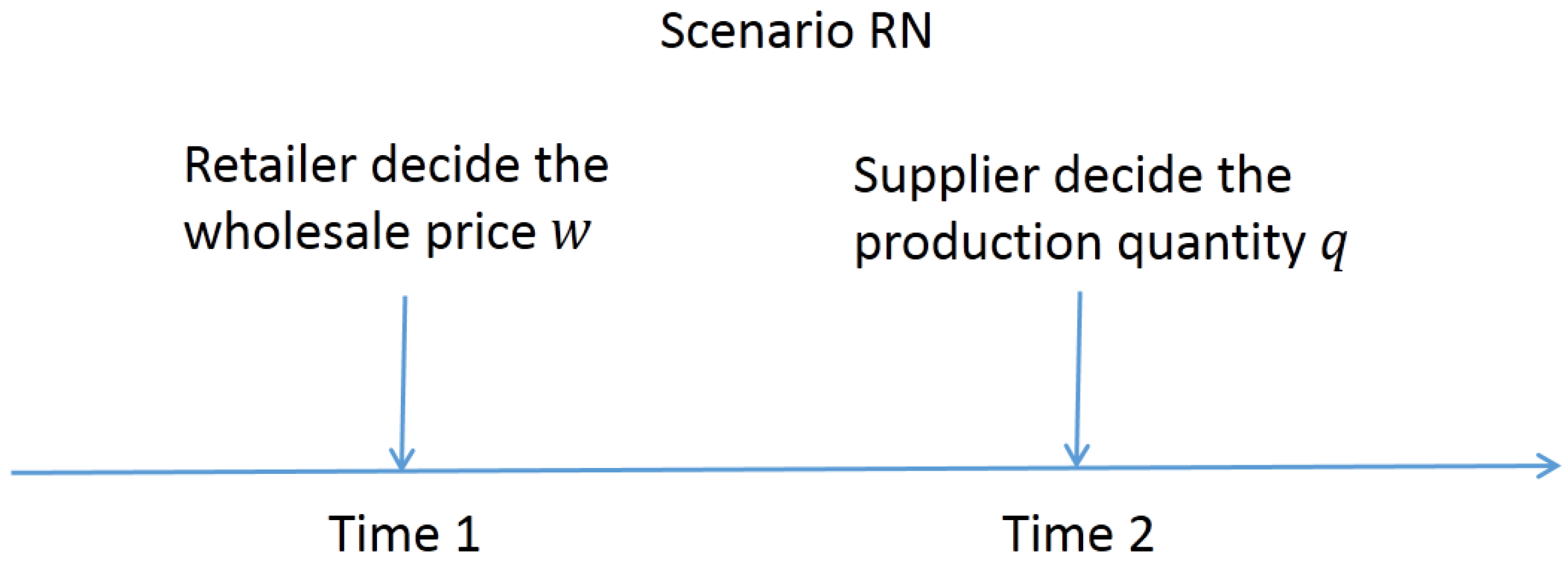
| Supplier-as-a-Leader | Retailer-as-a-Leader | |
|---|---|---|
| entry Loss-Neutral Supplier | Scenario SN | Scenario RN |
| entry Loss-Averse Supplier | Scenario SA | Scenario RA |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niu, B.; Chen, L.; Zhang, J. Sustainability Analysis of Supply Chains with Fashion Products under Alternative Power Structures and Loss-Averse Supplier. Sustainability 2017, 9, 995. https://doi.org/10.3390/su9060995
Niu B, Chen L, Zhang J. Sustainability Analysis of Supply Chains with Fashion Products under Alternative Power Structures and Loss-Averse Supplier. Sustainability. 2017; 9(6):995. https://doi.org/10.3390/su9060995
Chicago/Turabian StyleNiu, Baozhuang, Lei Chen, and Jie Zhang. 2017. "Sustainability Analysis of Supply Chains with Fashion Products under Alternative Power Structures and Loss-Averse Supplier" Sustainability 9, no. 6: 995. https://doi.org/10.3390/su9060995
APA StyleNiu, B., Chen, L., & Zhang, J. (2017). Sustainability Analysis of Supply Chains with Fashion Products under Alternative Power Structures and Loss-Averse Supplier. Sustainability, 9(6), 995. https://doi.org/10.3390/su9060995




