Estimating the Total Economic Value of Cultivated Flower Land in Taiwan
Abstract
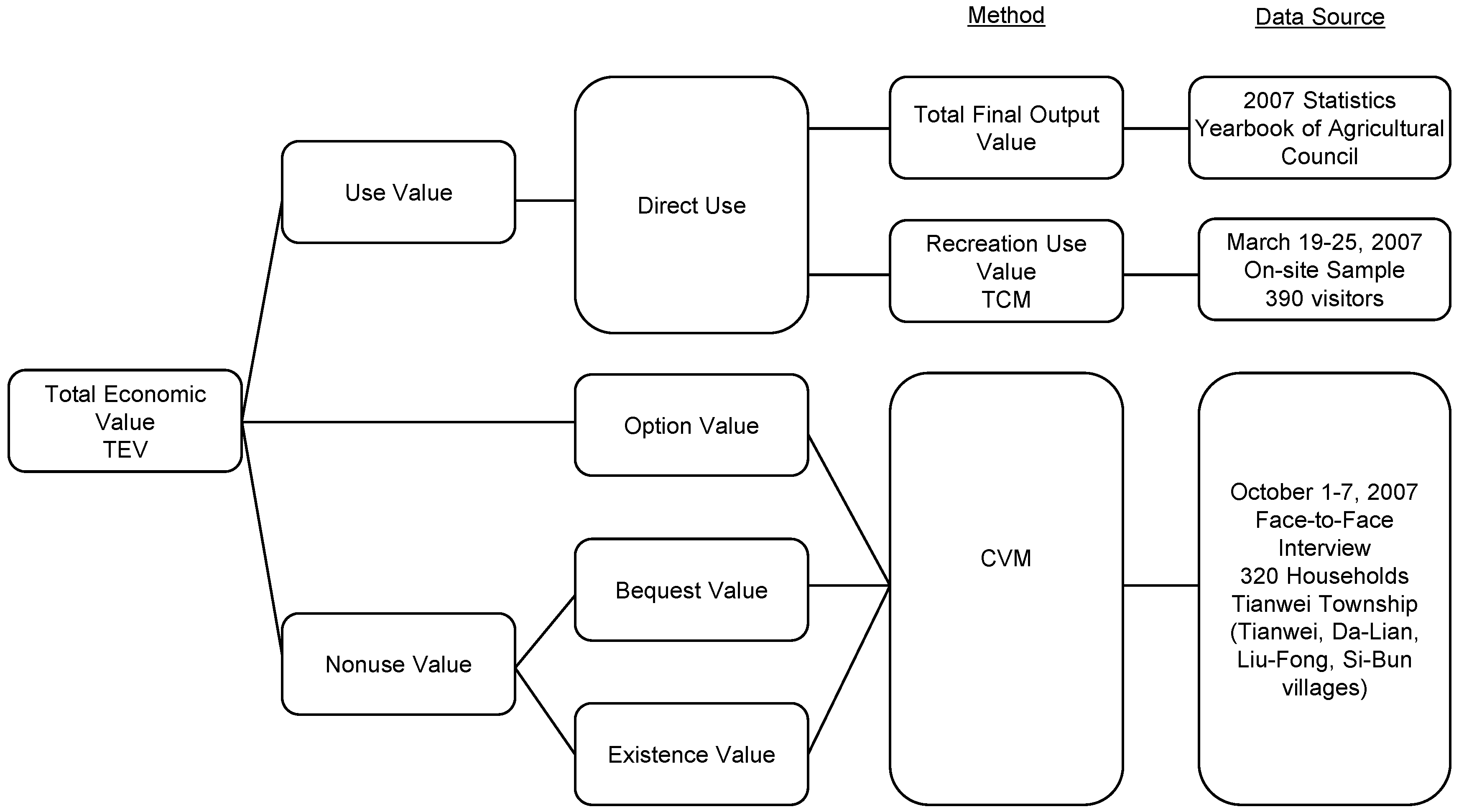
:1. Introduction
2. Materials and Methods
2.1. Travel Cost Method
2.2. Contingent Valuation Method
2.3. Data and Variables
| Variable | Definition | Mean | S.D. |
|---|---|---|---|
| TRIPS | Number of observed trips for individual visits to Tianwei Highway Garden | 4.82 | 6.20 |
| COST | Total round-trip travel costs to Tianwei Highway Garden (NT dollars) | 1311 | 1301 |
| SCOST | Total round-trip travel costs to the substitute site, Taichung Lavender Forest | 1704 | 1327 |
| GENDER | Male, 1; female, 0 | 0.39 | 0.49 |
| MARITAL | Marital status of visitor: married, 1; otherwise, 0 | 0.55 | 0.50 |
| AGE | Age of visitor | 34.59 | 12.32 |
| EDU | Educated years of visitor | 13.94 | 3.14 |
| LINCOME | Log of monthly income | 4.43 | 0.22 |
| HOLIDAY | Dummy, 1, if visitor went to Tianwei Highway Garden on Saturday or Sunday; 0, otherwise | 0.75 | 0.43 |
| LIVE | Number of years residents have lived in their current location | 16.37 | 5.38 |
| VILLAGE1 | Dummy, 1, if a resident lives in Da-Lian; 0, otherwise | ||
| VILLAGE2 | Dummy, 1, if a resident lives in Tianwei; 0, otherwise | ||
| VILLAGE3 | Dummy, 1, if a resident lives in Liu-Fong; 0, otherwise |
3. Results and Discussion
3.1. Recreational Benefits
| Variable | Coefficient | t value |
|---|---|---|
| INT | 4.0196 | (6.662) |
| COST | −0.0006 ※ | (−10.1899) *** |
| SCOST | 0.0004 | (8.149) *** |
| GENDER | −0.0369 | (−0.627) |
| MARITAL | 0.4831 | (6.369) *** |
| AGE | −0.0118 | (−4.030) *** |
| EDU | −0.0068 | (−8.9797) *** |
| LINCOME | −0.4223 | (−3.3361) *** |
| HOLIDAY | 0.6037 | (7.4261) *** |
| Log likelihood function | −15,690 | |
| Chi-squared | 466 |
3.2. Option, Bequest, and Existence Values
| Variable | Log-normal | Weibull | Gamma | Exponential |
|---|---|---|---|---|
| INT | 1.61 (0.86) | 3.24 (1.98) | 2.67 (1.41) | 2.82 (1.30) |
| AGE | −0.004 (0.46) | −0.01 (1.48) * | −0.01 (1.08) | −0.01 (0.98) |
| EDU | 0.01 (0.31) | 0.02 (0.71) | 0.02 (0.60) | 0.02 (0.53) |
| LNINCOME | 0.47 (2.39) ** | 0.31 (1.84) * | 0.37 (1.90) ** | 0.36 (1.60) * |
| LIVE | 0.01 (0.98) | 0.02 (2.48) ** | 0.01 (1.62) * | 0.01 (1.60) * |
| VILLAGE1 | 0.02 (0.10) | 0.17 (0.90) | 0.11 (0.48) | 0.10 (0.39) |
| VILLAGE 2 | 0.08 (0.39) | 0.55 (2.87) ** | 0.44 (1.60) * | 0.43 (1.69) * |
| VILLAGE 3 | −0.40 (1.62) * | −0.13 (0.62) | −0.23 (0.87) | −0.23 (0.81) |
| Scale | 0.93 (15.76) *** | 0.73 (14.13) *** | 0.80 (7.24) *** | 1 (31.40) *** |
| Log-likelihood | −253.60 | −252.47 | −252.23 | −260.94 |
| Log-likelihood ratio | 12.03 * | 25.38 *** | 14.66 ** | 14.50 ** |
| WTP(NT$) | 638.48 | 768.08 | 723.14 | 673.06 |
| Variable | Log-normal | Weibull | Gamma | Exponential |
|---|---|---|---|---|
| INT | 1.64 (0.87) | 3.28 (1.99) | 2.64 (1.40) | 2.85 (1.30) |
| AGE | −0.004 (0.48) | −0.01 (1.50) * | −0.01 (1.07) | −0.01 (1.00) |
| EDU | 0.01 (0.26) | 0.02 (0.64) | 0.02 (0.52) | 0.02 (0.46) |
| LNINCOME | 0.46 (2.36) ** | 0.31 (1.81) * | 0.37 (1.91) ** | 0.36 (1.58) * |
| LIVE | 0.01 (1.07) | 0.02 (2.57) ** | 0.01 (1.68) * | 0.01 (1.68) * |
| VILLAGE1 | 0.0004 (0.00) | 0.15 (0.78) | 0.42 (1.56) * | 0.07 (0.27) |
| VILLAGE 2 | 0.08 (0.39) | 0.56 (2.87) ** | 0.44 (1.60) * | 0.43 (1.69) * |
| VILLAGE 3 | −0.40 (1.62)* | −0.13 (0.61) | −0.23 (0.91) | −0.23 (0.81) |
| Scale | 0.92 (15.82) *** | 0.73 (14.20) *** | 0.81 (7.95) *** | 1 (31.52) *** |
| Log-likelihood | −254.31 | −253.34 | −252.99 | −260.94 |
| Log-likelihood ratio | 10.61 | 23.63 *** | 13.11 * | 12.67 * |
| WTP(NT$) | 635.02 | 763.75 | 714.40 | 668.61 |
| Variable | Log-normal | Weibull | Gamma | Exponential |
|---|---|---|---|---|
| INT | 1.70 (0.89) | 3.42 (2.03) | 2.57 (1.30) | 3.03 (1.37) |
| AGE | −0.01 (0.62) | −0.01 (1.74) * | −0.01 (1.12) | −0.01 (1.23) |
| EDU | 0.01 (0.19) | 0.01 (0.47) | 0.02 (0.37) | 0.01 (0.32) |
| LNINCOME | 0.47 (2.33) ** | 0.31 (1.78) * | 0.39 (1.94) ** | 0.36 (1.56) * |
| LIVE | 0.01 (1.07) | 0.02 (2.58) ** | 0.01 (1.54) * | 0.01 (1.72) * |
| VILLAGE1 | −0.01 (0.05) | 0.14 (0.69) | 0.04 (0.20) | 0.06 (0.22) |
| VILLAGE 2 | 0.05 (0.21) | 0.52 (2.63) ** | 0.33 (1.14) | 0.40 (1.54) * |
| VILLAGE 3 | −0.42 (1.69) * | −0.17 (0.77) | −0.30 (1.13) | −0.27 (0.93) |
| Scale | 0.93 (15.69) *** | 0.74 (14.10) *** | 0.85 (8.18) *** | 1 (27.60) *** |
| Log-likelihood | −252.95 | −252.67 | −252.14 | −260.40 |
| Log-likelihood ratio | 13.32 * | 24.97 *** | 14.81 ** | 15.56 ** |
| WTP(NT$) | 636.96 | 767.95 | 702.47 | 676.48 |
| WTP/person | Population | Total Value (NT$ million) | |
|---|---|---|---|
| Total Output Value | 1441 | ||
| Recreational Value | NT$8271.00 | 2.147 million visitors | 17,757 |
| Option Value | NT$768.08 | 6672 residents | 5.125 |
| Bequest Value | NT$767.95 | 6672 residents | 5.124 |
| Existence Value | NT$763.75 | 6672 residents | 5.096 |
4. Conclusions
Author Contributions
Appendix
Questionnaire Example
- Option Value: A measure to conserve Tianwei Highway Garden, which would allow you to visit or not to visit for an activity in the future.
- Existence Value: Knowing Tianwei Highway Garden through the news or other resources, you are glad that Tianwei Highway Garden exists and allows you or others to visit it.
- Bequest Value: The value that you would like to attribute to Tianwei Highway Garden for future generations.
- (1)
- Would you like to pay NT$500 to maintain the option value of Tianwei Highway Garden?
- □
- Yes; would you like to pay NT$1000 to maintain the option value of Tianwei Highway Garden?
- □
- No; would you like to pay NT$250 to maintain the option value of Tianwei Highway Garden?
- (2)
- Would you like to pay NT$100 to maintain the existence value of Tianwei Highway Garden?
- □
- Yes; would you like to pay NT$200 to maintain the option value of Tianwei Highway Garden?
- □
- No; would you like to pay NT$50 to maintain the option value of Tianwei Highway Garden?
- (3)
- Would you like to pay NT$300 to maintain the bequest value of Tianwei Highway Garden?
- □
- Yes; would you like to pay NT$600 to maintain the option value of Tianwei Highway Garden?
- □
- No; would you like to pay NT$150 to maintain the option value of Tianwei Highway Garden?
Conflicts of Interest
References
- Romstad, E.; Vatn, A.; RøStad, P.K.; Søyland, V. Multifunctional Agriculture: Implications for Policy Design; Report No. 21; Department of Economics and Social Sciences, Agricultural University of Norway: Akershus, Norway, 2000; ISSN 0802-9210. [Google Scholar]
- Organization for Economic Co-operation and Developmen (OECD). Multifunctionality: Towards an Analytical Framework; OECD: Paris, France, 2001. [Google Scholar]
- European Commission (EC). Safeguarding the Multifunctional Role of Agriculture: Which Instruments? EC: Brussels, Belgium, 1999. [Google Scholar]
- Miškolci, S. Multifunctional agriculture: Evaluation of non-production benefits using the analytical hierarchy process. Agric. Econ. 2008, 54, 322–332. [Google Scholar]
- Randall, A.; Stoll, J. Existence values in a total valuation framework. In Managing Air Quality and Scenic Resources at National Parks and Wilderness Areas; Row, R.D., Chestnut, L.G., Eds.; Westview Press: Boulder, CO, USA, 1983. [Google Scholar]
- Peterson, G.L.; Sorg, C.F. Toward the Measurement of Total Economic Value; USDA Forest Service, Rocky Mountain Forest and Range Experiment Station: Fort Collins, CO, USA, 1987. [Google Scholar]
- Pearce, D.W.; Turner, R.K. Economics of Natural Resources and the Environment; Harvester Wheatsheaf: London, UK, 1990. [Google Scholar]
- Pearce, D.W. Economic Values and the Natural World; Earthscan: London, UK, 1993. [Google Scholar]
- Freeman, M.A. The Measurement of Environmental and Resource Values: Theory and Methods; Resources for the Future: Washington, DC, USA, 1993. [Google Scholar]
- Whitehead, J.C. Total economic values for coastal and marine wildlife: Specification, validity, and valuation issues. Mar. Resour. Econ. 1993, 8, 119–132. [Google Scholar]
- Bateman, I.J.; Langford, I.H. Non-users’ willingness to pay for a national park: an application and critique of the contingent valuation method. Reg. Stud. 1997, 31, 571–582. [Google Scholar] [CrossRef]
- Krutilla, J.V. Conservation reconsidered. Am. Econ. Rev. 1967, 57, 777–786. [Google Scholar]
- Pearce, D.W.; Moran, D. The Economic Value of Biodiversity; Earthscan Publication Ltd.: London, UK, 1994. [Google Scholar]
- Lazo, J.K.; McClelland, G.H.; Schulze, W.D. Economic theory and psychology of non-use values. Land Econ. 1997, 73, 358–371. [Google Scholar] [CrossRef]
- Weisbrod, B.A. Collective-consumption services of individual-consumption goods. Q. J. Econ. 1964, 78, 471–478. [Google Scholar] [CrossRef]
- Lee, C.-K.; Han, S.-Y. Estimating the use and preservation values of national parks’ tourism resources using a contingent valuation method. Tourism Manag. 2002, 23, 531–540. [Google Scholar] [CrossRef]
- Olsen, G. Option value. Aus. J. Agric. Econ. 1975, 19, 197–209. [Google Scholar]
- Togridou, A.; Hovardas, T.; Pantis, J.D. Determinants of visitors’ willingness to pay for the National Marine Park of Zakynthos, Greece. Ecol. Econ. 2006, 60, 308–319. [Google Scholar] [CrossRef]
- Plottu, E.; Plottu, B. The concept of total economic value of environment: A reconsideration within a hierarchical rationality. Ecol. Econ. 2007, 61, 52–61. [Google Scholar] [CrossRef]
- Loomis, J.; Kent, P.; Strange, L.; Fausch, K.; Covich, A. Measuring the total economic value of restoring ecosystem services in an impaired river basin: Results from a contingent valuation survey. Ecol. Econ. 2000, 33, 103–117. [Google Scholar] [CrossRef]
- Parumog, G.M.; Cal, C.P.; Mizokami, S. Using travel cost and contingent valuation methodologies in valuing externalities of urban road development: An application in valuing damages to cultural heritage. J. Eastern Asia Soc. Transp. Stud. 2003, 5, 2948–2961. [Google Scholar]
- Zander, K.K.; Signorello, G.; de Salvo, M.; Fandini, G.; Drucker, A.G. Assessing the total economic value of threatened livestock breeds in Italy: Implications for conservation policy. Ecol. Econ. 2013, 93, 219–229. [Google Scholar] [CrossRef]
- Yang, W.; Chang, J.; Xu, B.; Peng, C.; Ge, Y. Ecosystem service value assessment for constructed wetlands: A case study in Hangzhou, China. Ecol. Econ. 2008, 68, 116–125. [Google Scholar] [CrossRef]
- Boyd, J.; Banzhaf, S. What are Ecosystem Services? The Need for Standardized Environmental Accounting Units. Ecol. Econ. 2007, 63, 616–626. [Google Scholar] [CrossRef]
- Pruckner, J.G. Agricultural landscape cultivation in Austria: An application of CVM. Eur. Agric. Econ. 1995, 22, 173–190. [Google Scholar]
- Poor, P.J.; Smith, J.M. Travel cost analysis of a cultural heritage site: the case of historic St. Mary’s city of Maryland. J. Cult. Econ. 2004, 28, 217–229. [Google Scholar] [CrossRef]
- Yung, E.H.K.; Yu, P.L.H.; Chan, E.H.W. Economic Valuation of Historic Properties: Review and Recent Developments. Prop. Manag. 2013, 31, 335–358. [Google Scholar]
- Loomis, J.B.; Walsh, R.G. Recreation Economic Decisions: Comparing Benefits and Costs; Venture Publishing, Inc.: Edmonton, AB, Canada, 1997. [Google Scholar]
- Fix, P.; Loomis, J. Comparison the Economics Value of Mountain Biking Estimated Using Revealed and Stated Preference. J. Environ. Plan. Manag. 1998, 41, 227–236. [Google Scholar] [CrossRef]
- Siderelis, C.; Moore, R. Outdoor recreation net benefits of rail-trails. J. Leisure Res. 1995, 27, 344–359. [Google Scholar]
- Carson, R.T.; Flores, E.N.; Martin, M.K.; Wright, L.J. Contingent Valuation and Revealed Preference Methodologies: Comparing the estimates for qusi-public goods. Land Econ. 1996, 72, 80–89. [Google Scholar] [CrossRef]
- Common, M.; Bull, T.; Stoeckl, N. The travel cost method: An empirical investigation of Randall’s Difficulty. Aust. J. Agric. Resource Econ. 1999, 43, 457–477. [Google Scholar] [CrossRef]
- Randall, A.A. Difficulty with the travel cost method. Land Econ. 1994, 70, 88–96. [Google Scholar] [CrossRef]
- Bockstael, N.E.; Strand, I.E.; Hanemann, W.M. Time and the recreational demand model. Am. J. Agric. Econ. 1987, 69, 293–302. [Google Scholar] [CrossRef]
- U.S. Water Resources Council. Economic and Environmental Principles and Guidelines for Water and Related Land Resources Implementation Studies; U.S. Government Printing Office: Washington, DC, USA, 1983.
- McConnell, K.E.; Strand, I. Measuring the cost of time in recreational demand analysis: An application to sport fishing. Am. J. Agric. Econ. 1981, 63, 153–156. [Google Scholar] [CrossRef]
- Rosenthal, D.H. The necessity for substitute prices in recreation demand analysis. Am. J. Agric. Econ. 1987, 69, 828–837. [Google Scholar] [CrossRef]
- Chakraborty, K.; Keith, J.E. Estimating the recreation demand and economic value of mountain biking in Moab, Utah: An application of count data models. J. Environ. Plan. Manag. 2000, 43, 461–469. [Google Scholar] [CrossRef]
- Hellerstein, D.; Mendelsohn, R. A theoretical foundation for count data models. Am. J. Agric. Econ. 1993, 75, 604–611. [Google Scholar] [CrossRef]
- Creel, M.D.; Loomis, J.B. Theory and empirical advantages of truncated count data estimators for analysis of deer hunting in California. Am. J. Agric. Econ. 1990, 72, 434–441. [Google Scholar] [CrossRef]
- Shaw, D. On-site samples’ regression: Problem of non-negative integers, truncation and endogenous stratification. J. Econ. 1988, 37, 211–223. [Google Scholar] [CrossRef]
- Englin, J.; Shonkwiler, J.S. Estimating social welfare using count data models: An application to long-run recreation demand under conditions of endogenous stratification and truncation. Rev. Econ. Stat. 1995, 77, 104–112. [Google Scholar] [CrossRef]
- Englin, J.; Shonkwiler, J.S. Modeling recreation demand in the presence of unobservable travel costs: Toward a travel price model. J. Environ. Econ. Manag. 1995, 29, 368–377. [Google Scholar] [CrossRef]
- Grogger, J.T.; Carson, R.T. Models for truncated counts. J. Appl. Econ. 1991, 6, 225–238. [Google Scholar] [CrossRef]
- Bishop, R.C.; Heberlein, T.A. Measuring values of extramarket foods: Are indirect measures biased? Am. J. Agric. Econ. 1979, 61, 926–930. [Google Scholar] [CrossRef]
- Mitchell, R.C.; Carson, R.T. Using Surveys to Value Public Goods: The Contingent Valuation Method; Resource for the Future: Washington, DC, USA, 1989. [Google Scholar]
- Cameron, T.A.; James, M.D. Efficient Estimation Methods for Closed Ended Contingent Valuation Survey. Rev. Econ. Stat. 1987, 69, 269–276. [Google Scholar] [CrossRef]
- Cummings, R.G.; Brookshire, D.S.; Schulze, W.D. Valuing Environmental Goods: A State of the Arts Assessment of the Contingent Valuation Method; Roweman and Allanheld: Totowa, NJ, USA, 1986. [Google Scholar]
- Veronesi, M.; Alberini, A.; Cooper, J.C. Implication of bid design and willingness-to-pay distribution for starting point bias in double-bounded dichotomous choice contingent valuation surveys. Environ. Resour. Econ. 2011, 49, 199–215. [Google Scholar] [CrossRef]
- Haab, T.C.; McConnell, K.E. Valuing Environmental and Natural Resources; Edward Elgar Publishing, Inc.: Cheltenham, UK, 2002. [Google Scholar]
- Walsh, R.G.; Loomis, J.B.; Gillman, R.A. Valuing option, existence and bequest demands for wilderness. Land Econ. 1984, 60, 14–29. [Google Scholar] [CrossRef]
- Kanninen, B.J. Bias in discrete response contingent valuation. J. Environ. Econ. Manag. 1995, 28, 114–125. [Google Scholar] [CrossRef]
- Park, J.H.; MacLachlan, D.L. Estimating willingness to pay with exaggeration bias-corrected contingent valuation method. Mark. Sci. 2008, 27, 691–698. [Google Scholar] [CrossRef]
- Johannesson, M.; Liljas, B.; Johansson, P.O. An experimental comparison of dichotomous choice contingent valuation question and real purchase decisions. Appl. Econ. 1998, 30, 643–647. [Google Scholar] [CrossRef]
- Lee, W.S.; Graefe, A.R.; Hwang, D. Willingness to pay for an ecological park experience. Asia Pac. J. Tour. Res. 2012, 18, 288–302. [Google Scholar] [CrossRef]
- Scarpa, R.; Bateman, I. Efficiency gains afforded by improved bid design versus follow-up valuation questions in discrete-choice CV studies. Land Econ. 2000, 76, 299–311. [Google Scholar] [CrossRef]
- Alberini, A. Efficiency vs. bias of willingness to pay estimates: Bivariate and interval-data models. J. Environ. Econ. Manag. 1995, 29, 169–180. [Google Scholar] [CrossRef]
- Hanemann, W.M.; Loomis, J.; Kanninen, B. Statistical efficiency of double-bounded dichotomous choice contingent valuation. Am. J. Agric. Econ. 1991, 73, 1255–1263. [Google Scholar] [CrossRef]
- Carson, R.D.; Wilks, L.; Imber, D. Valuing the preservation of Australia’s Kakadu Conservation Zone. Oxf. Econ. Pap. 1994, 46, 727–749. [Google Scholar]
- Bateman, I.J.; Langford, I.H.; Jones, A.P.; Kerr, G.N. Bound and path effects in double and triple bounded dichotomous choice contingent valuation. Resour. Energy Econ. 2001, 23, 191–213. [Google Scholar] [CrossRef]
- Kerr, G.N. Dichotomous choice contingent valuation probability distributions. Aus. J. Agric. Resour. Econ. 2000, 44, 233–252. [Google Scholar] [CrossRef]
- Imber, D.; Stevenson, G.; Wilks, L. A Contingent Valuation Survey of the Kakadu Conservation Zone; Research Paper No. 3; Resource Assessment Commission, Commonwealth Government Printer: Canberra, Australia, 1991.
- Nelson, W. Applied Life Data Analysis; John Wiley: New York, NY, USA, 1982. [Google Scholar]
- León, C.J. Double bounded survival values for preserving the landscape of natural parks. J. Environ. Manag. 1996, 46, 103–118. [Google Scholar] [CrossRef]
- Council of Agriculture. Agricultural Statistics Yearbook; Council of Agriculture: Taipei, Taiwan, 2007.
- Huang, C.H.; Huang, Y.H.; Lin, W.L.; Hsiao, P.H. Estimating recreational benefits and environmental effects for the amenities of flowers industry. Adv. Manag. 2011, 4, 60–65. [Google Scholar]
- Stacy, E.W. A generalization of the Gamma distribution. Ann. Math. Stat. 1962, 33, 1187–1192. [Google Scholar] [CrossRef]
- Cooper, J.C.; Hanemann, M.; Signorello, G. One-and-one-half-bound Dichotomous Choice Contingent Valuation. Rev. Econ. Stat. 2002, 84, 742–750. [Google Scholar] [CrossRef]
- Stoeckl, N.; Farr, N.; Larson, S.; Adams, V.M.; Kubiszewski, I.; Esparon, M.; Costanza, R. A new approach to the pfoblem of overlapping values: A case study in Australia’s Great Barrier Reef. Ecosyst. Serv. 2014, 10, 61–78. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, C.-H.; Wang, C.-H. Estimating the Total Economic Value of Cultivated Flower Land in Taiwan. Sustainability 2015, 7, 4764-4782. https://doi.org/10.3390/su7044764
Huang C-H, Wang C-H. Estimating the Total Economic Value of Cultivated Flower Land in Taiwan. Sustainability. 2015; 7(4):4764-4782. https://doi.org/10.3390/su7044764
Chicago/Turabian StyleHuang, Chin-Huang, and Chiung-Hsia Wang. 2015. "Estimating the Total Economic Value of Cultivated Flower Land in Taiwan" Sustainability 7, no. 4: 4764-4782. https://doi.org/10.3390/su7044764
APA StyleHuang, C.-H., & Wang, C.-H. (2015). Estimating the Total Economic Value of Cultivated Flower Land in Taiwan. Sustainability, 7(4), 4764-4782. https://doi.org/10.3390/su7044764





