A Polynomial Chaos Expansion Method for Mechanical Properties of Flexoelectric Materials Based on the Isogeometric Finite Element Method
Abstract
:1. Introduction
- 1.
- IGA-FEM is employed to analyze mechanical properties of flexoelectric materials.
- 2.
- PCE is adapted to accelerate mechanical properties analysis for flexoelectric materials with different single uncertain parameters.
2. The PCE Fundamental Theory
3. Theory of Flexoelectricity
4. IGA-FEM Discretization of the Flexoelectric Fourth-Order Partial Differential Equation
5. Numerical Examples
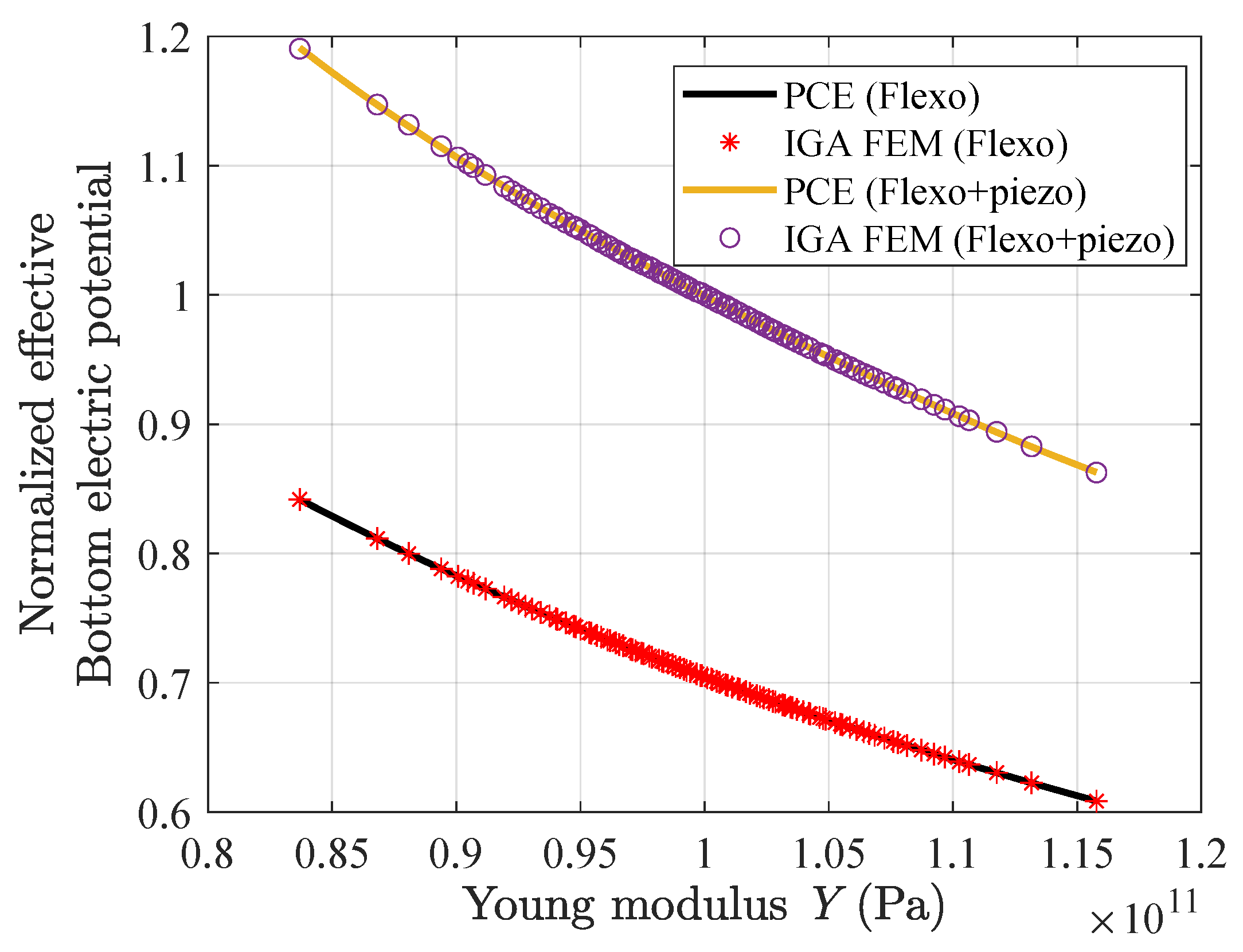
5.1. Model Validation
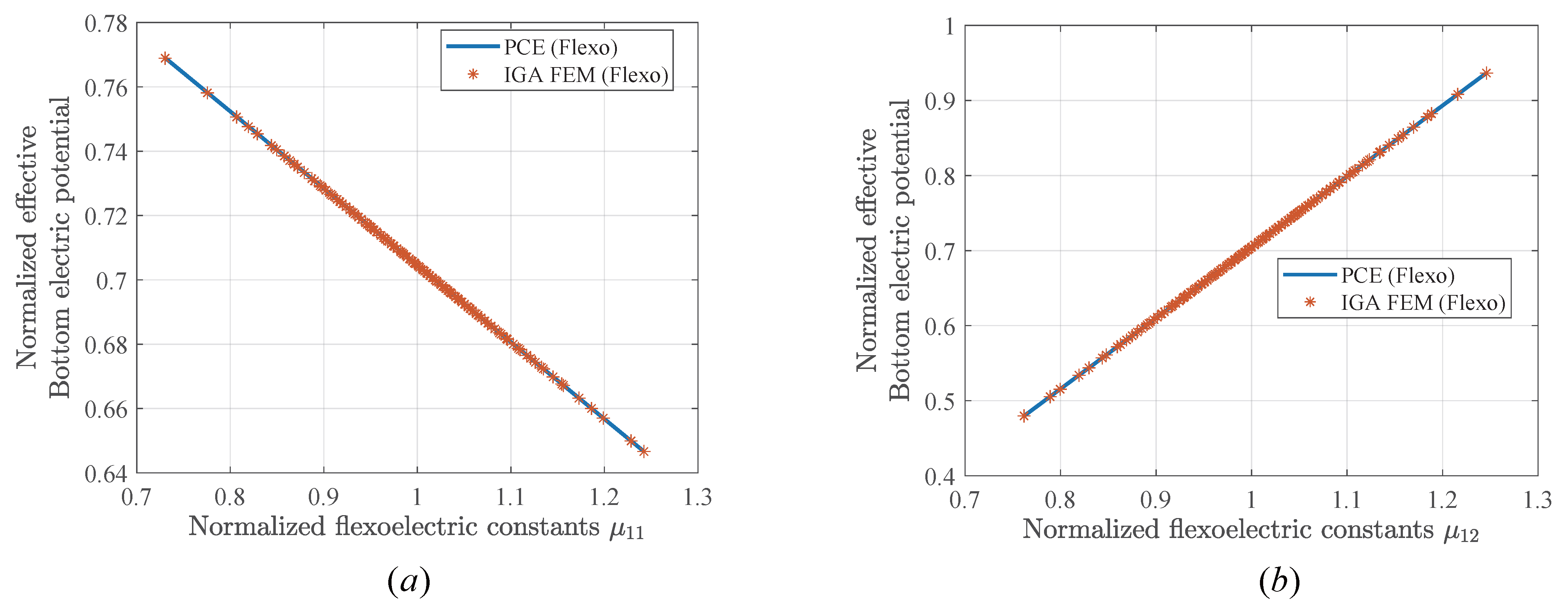
5.2. Verification of Surrogate Models for Mechanical Properties of Flexoelectric Materials
6. Conclusions
- 1.
- The B-spline shape functions satisfying the continuity requirement are employed to discretize the governing equations.
- 2.
- The IGA-FEM reduces the need for repeated meshing in uncertainty quantification while maintaining geometric accuracy.
- 3.
- The truncated pyramid model was chosen as the object of study to obtain the mechanical properties of the flexoelecteric material.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mashkevich, V.; Tolpygo, K. Electrical, optical and elastic properties of diamond type crystals. Sov. Phys. JETP 1957, 5, 435–439. [Google Scholar]
- Ahmadpoor, F.; Sharma, P. Flexoelectricity in two-dimensional crystalline and biological membranes. Nanoscale 2015, 7, 16555–16570. [Google Scholar] [CrossRef]
- Ahmadpoor, F.; Lahmer, T.; Zhuang, X.; Zi, G.; Rabczuk, T. Detection of material interfaces using a regularized level set method in piezoelectric structures. Inverse Probl. Sci. Eng. 2016, 24, 153–176. [Google Scholar] [CrossRef]
- Yudin, P.; Tagantsev, A. Fundamentals of flexoelectricity in solids. Nanotechnology 2013, 24, 432001. [Google Scholar] [CrossRef]
- Nguyen, T.D.; Mao, S.; Yeh, Y.W.; Purohit, P.K.; McAlpine, M.C. Nanoscale flexoelectricity. Adv. Mater. 2013, 25, 946–974. [Google Scholar] [CrossRef] [PubMed]
- Zubko, P.; Catalan, G.; Tagantsev, A.K. Flexoelectric effect in solids. Annu. Rev. Mater. Res. 2013, 43, 387–421. [Google Scholar] [CrossRef]
- Vinyas, M.; Nischith, G.; Loja, M.A.R.; Ebrahimi, F.; Duc, N.D. Numerical analysis of the vibration response of skew magneto-electro-elastic plates based on the higher-order shear deformation theory. Compos. Struct. 2019, 214, 132–142. [Google Scholar] [CrossRef]
- Zhao, Q.; Liu, Y.; Wang, L.; Yang, H.; Cao, D. Design method for piezoelectric cantilever beam structure under low frequency condition. Int. J. Pavement Res. Technol. 2018, 11, 153–159. [Google Scholar] [CrossRef]
- Majdoub, M.S.; Sharma, P.; Cagin, T. Enhanced size-dependent piezoelectricity and elasticity in nanostructures due to the flexoelectric effect. Phys. Rev. B 2008, 77, 125424. [Google Scholar] [CrossRef]
- Abdollahi, A.; Peco, C.; Millán, D.; Arroyo, M.; Arias, I. Computational evaluation of the flexoelectric effect in dielectric solids. J. Appl. Phys. 2014, 116, 093502. [Google Scholar] [CrossRef]
- Blatman, G. Adaptive Sparse Polynomial Chaos Expansions for Uncertainty Propagation and Sensitivity Analysis. Ph.D. Thesis, Blaise Pascal University, Aubiere, France, 2009. [Google Scholar]
- Kumar, Y.A.; Mani, G.; Pallavolu, M.R.; Sangaraju, S.; Reddy, N.R.; Selvaraj, M.; Alfakeer, M.; Bahajjaj, A.A.A.; Ouladsmane, M.; Rao, S.S.R.; et al. Facile synthesis of efficient construction of tungsten disulfide/iron cobaltite nanocomposite grown on nickel foam as a battery-type energy material for electrochemical supercapacitors with superior performance. J. Colloid Interface Sci. 2022, 609, 434–446. [Google Scholar] [CrossRef]
- Moniruzzaman, M.; Kumar, Y.A.; Pallavolu, M.R.; Arbi, H.M.; Alzahmi, S.; Obaidat, I. Two-dimensional core-shell structure of cobalt-doped@MnO2 nanosheets grown on nickel foam as a binder-free battery-type electrode for supercapacitor application. Nanomaterials 2022, 12, 3187. [Google Scholar] [CrossRef]
- Pallavolu, M.R.; Kumar, Y.A.; Reddy, N.M.; Dhananjaya, M.; Al-Asbahi, B.A.; Sreedhar, A.; Joo, S.W. Design and synthesis of highly efficient Nitrogen-doped carbon nano-onions for asymmetric supercapacitors. J. Alloy. Compd. 2022, 918, 165609. [Google Scholar] [CrossRef]
- Hurtado, J.; Barbat, A. Monte Carlo techniques in computational stochastic mechanics. Arch. Comput. Methods Eng. 1998, 5, 3–29. [Google Scholar] [CrossRef]
- Honda, R. Stochastic BEM with spectral approach in elastostatic and elastodynamic problems with geometrical uncertainty. Eng. Anal. Bound. Elem. 2005, 29, 415–427. [Google Scholar] [CrossRef]
- Liu, W.K.; Belytschko, T.; Mani, A. Random field finite elements. Int. J. Numer. Methods Eng. 1986, 23, 1831–1845. [Google Scholar] [CrossRef]
- Kamiński, M. Stochastic perturbation approach to engineering structure vibrations by the finite difference method. J. Sound Vib. 2002, 251, 651–670. [Google Scholar] [CrossRef]
- Kamiński, M. On generalized stochastic perturbation-based finite element method. Commun. Numer. Methods Eng. 2006, 22, 23–31. [Google Scholar] [CrossRef]
- Zhang, B.Y.; Ni, Y.Q. A hybrid sequential sampling strategy for sparse polynomial chaos expansion based on compressive sampling and Bayesian experimental design. Comput. Methods Appl. Mech. Eng. 2021, 386, 114130. [Google Scholar] [CrossRef]
- Kersaudy, P.; Sudret, B.; Varsier, N.; Picon, O.; Wiart, J. A new surrogate modeling technique combining Kriging and polynomial chaos expansions–Application to uncertainty analysis in computational dosimetry. J. Comput. Phys. 2015, 286, 103–117. [Google Scholar] [CrossRef]
- Novak, L.; Novak, D. Polynomial chaos expansion for surrogate modelling: Theory and software. Beton-Stahlbetonbau 2018, 113, 27–32. [Google Scholar] [CrossRef]
- Wan, H.P.; Ren, W.X.; Todd, M.D. Arbitrary polynomial chaos expansion method for uncertainty quantification and global sensitivity analysis in structural dynamics. Mech. Syst. Signal Process. 2020, 142, 106732. [Google Scholar] [CrossRef]
- Rajabi, M.M.; Ataie-Ashtiani, B.; Simmons, C.T. Polynomial chaos expansions for uncertainty propagation and moment independent sensitivity analysis of seawater intrusion simulations. J. Hydrol. 2015, 520, 101–122. [Google Scholar] [CrossRef]
- Guo, X.; Dias, D.; Claudio, C.; Laurent, P.; Pierre, B. Reliability analysis of embankment dam sliding stability using the sparse polynomial chaos expansion. Eng. Struct. 2018, 174, 295–307. [Google Scholar] [CrossRef]
- Hariri, A.; Mohammad, A.; Claudio, C.; Sudret, B. Polynomial chaos expansion for uncertainty quantification of dam engineering problems. Eng. Struct. 2020, 203, 109631. [Google Scholar] [CrossRef]
- Matthies, H.G.; Keese, A. Galerkin methods for linear and nonlinear elliptic stochastic partial differential equations. Comput. Methods Appl. Mech. Eng. 2005, 194, 1295–1331. [Google Scholar] [CrossRef]
- Cao, G.; Yu, B.; Chen, L.; Yao, W. Isogeometric dual reciprocity BEM for solving non-Fourier transient heat transfer problems in FGMs with uncertainty analysis. Int. J. Heat Mass Transf. 2023, 203, 123783. [Google Scholar] [CrossRef]
- Le Maître, O.; Knio, O.M. Spectral Methods for Uncertainty Quantification with Applications to Computational Fluid Dynamics; Springer: Dordrecht, The Netherlands, 2010. [Google Scholar] [CrossRef]
- Blatman, G.; Sudret, B. Sparse polynomial chaos expansions and adaptive stochastic finite elements using a regression approach. Comptes Rendus Mec. 2008, 336, 518–523. [Google Scholar] [CrossRef]
- Palar, P.S.; Tsuchiya, T.; Parks, G.T. Multi-fidelity non-intrusive polynomial chaos based on regression. Comput. Methods Appl. Mech. Eng. 2016, 305, 579–606. [Google Scholar] [CrossRef]
- Zhou, Y.; Lu, Z.; Cheng, K.; Shi, Y. An expanded sparse Bayesian learning method for polynomial chaos expansion. Mech. Syst. Signal Process. 2019, 128, 153–171. [Google Scholar] [CrossRef]
- Hughes, T.J.; Cottrell, J.A.; Bazilevs, Y. Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput. Methods Appl. Mech. Eng. 2005, 194, 4135–4195. [Google Scholar] [CrossRef]
- Ghasemi, H.; Park, H.S.; Alajlan, N.; Rabczuk, T. A computational framework for design and optimization of flexoelectric materials. Int. J. Comput. Methods 2020, 17, 1850097. [Google Scholar] [CrossRef]
- Li, H.; Zhao, J.; Guo, X.; Cheng, Y.; Xu, Y.; Yuan, X. Sensitivity analysis of flexoelectric materials surrogate model based on the isogeometric finite element method. Front. Phys. 2022, 10, 1343. [Google Scholar] [CrossRef]
- Schillinger, D.; Dede, L.; Scott, M.A.; Evans, J.A.; Borden, M.J.; Rank, E.; Hughes, T.J. An isogeometric design-through-analysis methodology based on adaptive hierarchical refinement of NURBS, immersed boundary methods, and T-spline CAD surfaces. Comput. Methods Appl. Mech. Eng. 2012, 249, 116–150. [Google Scholar] [CrossRef]
- Chen, L.; Lian, H.; Xu, Y.; Li, S.; Liu, Z.; Atroshchenko, E.; Kerfriden, P. Generalized isogeometric boundary element method for uncertainty analysis of time-harmonic wave propagation in infinite domains. Appl. Math. Model. 2023, 114, 360–378. [Google Scholar] [CrossRef]
- Evans, J.A.; Hughes, T.J. Isogeometric divergence-conforming B-splines for the unsteady Navier–Stokes equations. J. Comput. Phys. 2013, 241, 141–167. [Google Scholar] [CrossRef]
- Chen, L.; Liu, C.; Zhao, W.; Liu, L. An isogeometric approach of two dimensional acoustic design sensitivity analysis and topology optimization analysis for absorbing material distribution. Comput. Methods Appl. Mech. Eng. 2018, 336, 507–532. [Google Scholar] [CrossRef]
- Chen, L.; Lian, H.; Liu, Z.; Chen, H.; Atroshchenko, E.; Bordas, S. Structural shape optimization of three dimensional acoustic problems with isogeometric boundary element methods. Comput. Methods Appl. Mech. Eng. 2019, 355, 926–951. [Google Scholar] [CrossRef]
- Chen, L.; Cheng, R.; Li, S.; Lian, H.; Zheng, C.; Bordas, S.P. A sample-efficient deep learning method for multivariate uncertainty qualification of acoustic–vibration interaction problems. Comput. Methods Appl. Mech. Eng. 2022, 393, 114784. [Google Scholar] [CrossRef]
- Chen, L.; Lu, C.; Lian, H.; Liu, Z.; Zhao, W.; Li, S.; Chen, H.; Bordas, S.P. Acoustic topology optimization of sound absorbing materials directly from subdivision surfaces with isogeometric boundary element methods. Comput. Methods Appl. Mech. Eng. 2020, 362, 112806. [Google Scholar] [CrossRef]
- Lu, C.; Chen, L.; Luo, J.; Chen, H. Acoustic shape optimization based on isogeometric boundary element method with subdivision surfaces. Eng. Anal. Bound. Elem. 2023, 146, 951–965. [Google Scholar] [CrossRef]
- Jiang, F.; Chen, L.; Wang, J.; Miao, X.; Chen, H. Topology optimization of multimaterial distribution based on isogeometric boundary element and piecewise constant level set method. Comput. Methods Appl. Mech. Eng. 2022, 390, 114484. [Google Scholar] [CrossRef]
- Jiang, F.; Zhao, W.; Chen, L.; Zheng, C.; Chen, H. Combined shape and topology optimization for sound barrier by using the isogeometric boundary element method. Eng. Anal. Bound. Elem. 2021, 124, 124–136. [Google Scholar] [CrossRef]
- Liu, C.; Chen, L.; Zhao, W.; Chen, H. Shape optimization of sound barrier using an isogeometric fast multipole boundary element method in two dimensions. Eng. Anal. Bound. Elem. 2017, 85, 142–157. [Google Scholar] [CrossRef]
- Jahanbin, R.; Rahman, S. Stochastic isogeometric analysis in linear elasticity. Comput. Methods Appl. Mech. Eng. 2020, 364, 112928. [Google Scholar] [CrossRef]
- Liu, Z.; Yang, M.; Cheng, J.; Tan, J. A new stochastic isogeometric analysis method based on reduced basis vectors for engineering structures with random field uncertainties. Appl. Math. Model. 2021, 89, 966–990. [Google Scholar] [CrossRef]
- Xiu, D.; Karniadakis, G.E. The Wiener–Askey polynomial chaos for stochastic differential equations. SIAM J. Sci. Comput. 2002, 24, 619–644. [Google Scholar] [CrossRef]
- Cao, L.; Liu, J.; Jiang, C.; L, G. Optimal sparse polynomial chaos expansion for arbitrary probability distribution and its application on global sensitivity analysis. Comput. Methods Appl. Mech. Eng. 2022, 399, 115368. [Google Scholar] [CrossRef]
- Hauseux, P.; Hale, J.S.; Bordas, S.P. Accelerating Monte Carlo estimation with derivatives of high-level finite element models. Comput. Methods Appl. Mech. Eng. 2017, 318, 917–936. [Google Scholar] [CrossRef]
- Ghasemi, H.; Park, H.S.; Rabczuk, T. A level-set based IGA formulation for topology optimization of flexoelectric materials. Comput. Methods Appl. Mech. Eng. 2017, 313, 239–258. [Google Scholar] [CrossRef]
- Chen, L.; Lian, H.; Natarajan, S.; Zhao, W.; Chen, X.; Bordas, S.P.A. Multi-frequency acoustic topology optimization of sound-absorption materials with isogeometric boundary element methods accelerated by frequency-decoupling and model order reduction techniques. Comput. Methods Appl. Mech. Eng. 2022, 395, 114997. [Google Scholar] [CrossRef]
- Chen, L.; Lian, H.; Liu, Z.; Gong, Y.; Zheng, C.J.; Bordas, S.P.A. Bi-material topology optimization for fully coupled structural-acoustic systems with isogeometric FEM–BEM. Eng. Anal. Bound. Elem. 2022, 135, 182–195. [Google Scholar] [CrossRef]
- Chen, L.; Wang, Z.; Peng, X.; Yang, J.; Wu, P.; Lian, H. Modeling pressurized fracture propagation with the isogeometric BEM. Geomech. Geophys. Geo-Energy Geo-Resour. 2021, 7, 51. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, Y.; Lian, H.; Atroshchenko, E.; Ding, C.; Bordas, S.P.A. Seamless integration of computer-aided geometric modeling and acoustic simulation: Isogeometric boundary element methods based on Catmull-Clark subdivision surfaces. Adv. Eng. Softw. 2020, 149, 102879. [Google Scholar] [CrossRef]
- Chen, L.; Li, H.; Guo, Y.; Chen, P.; Atroshchenko, E.; Lian, H. Uncertainty quantification of mechanical property of piezoelectric materials based on isogeometric stochastic FEM with generalized nth-order perturbation. Eng. Comput. 2023, 1–21. [Google Scholar] [CrossRef]
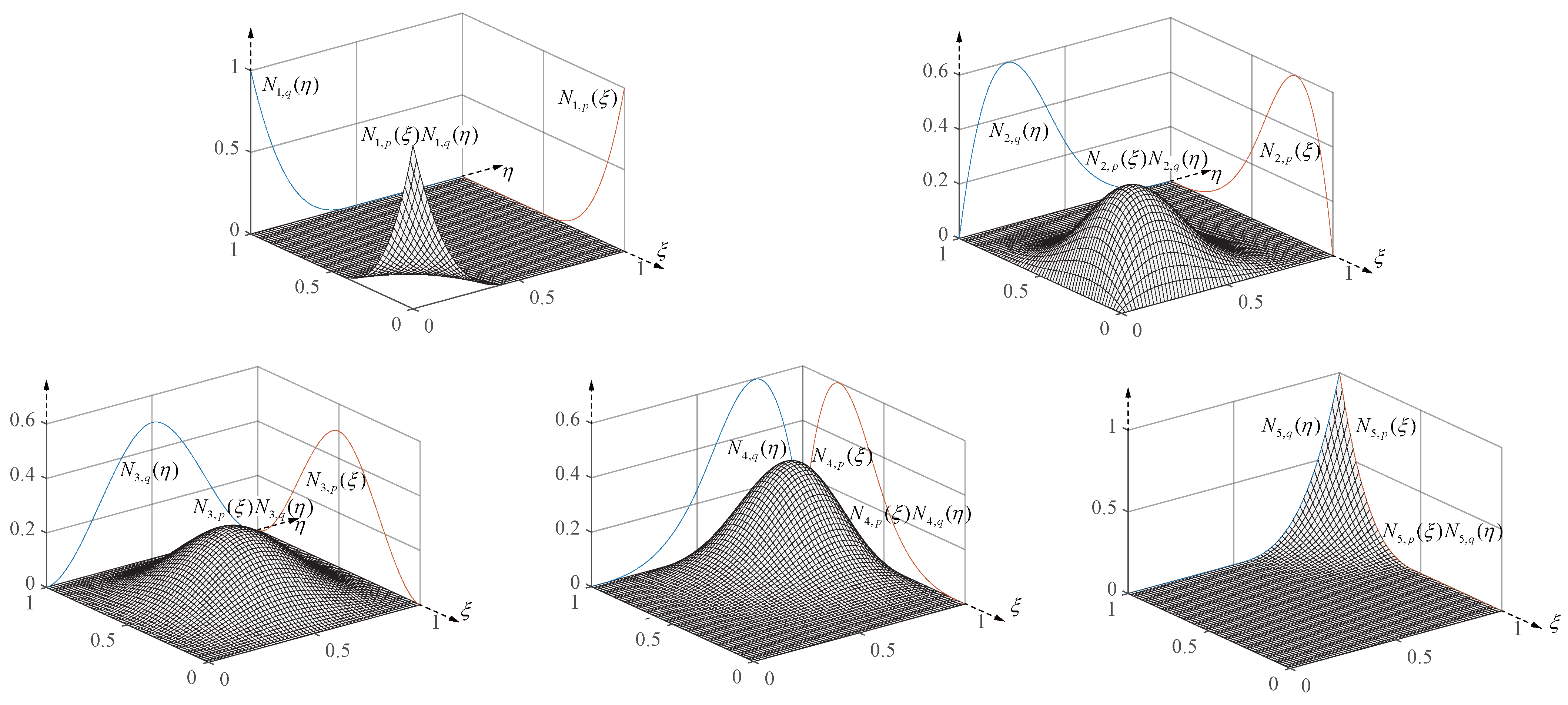

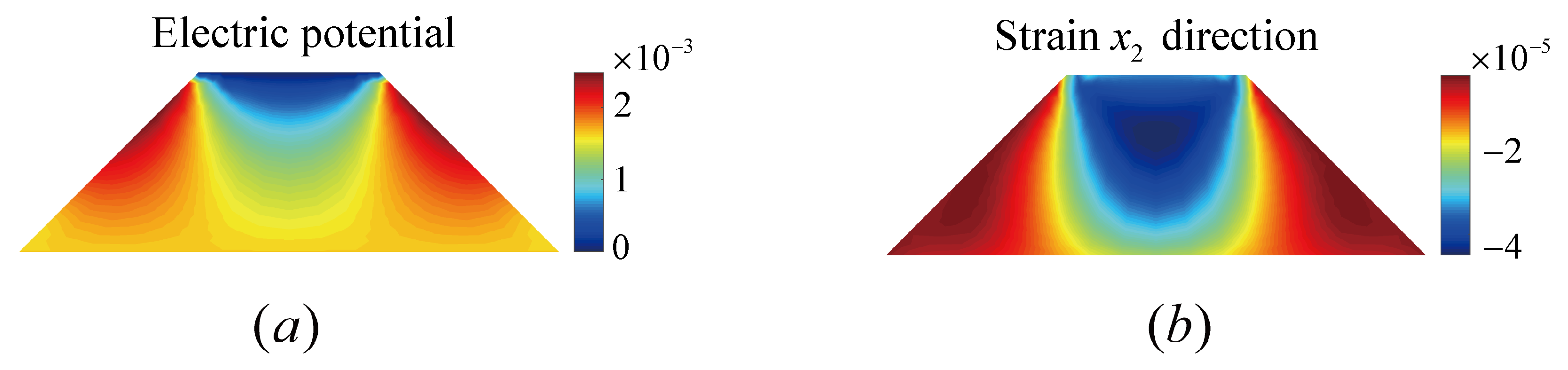

| Distribution of Random Parameter | Type | Interval |
|---|---|---|
| Uniform | Legendre | |
| Normal | Hermite | |
| Beta | Jacobi | |
| Gamma | Laguerre |
| 1 | 1 |
| 2 | r |
| 3 | |
| 4 | |
| 5 | |
| 6 |
| Type | Symbol | Magnitude | Unit |
|---|---|---|---|
| Upper edge width | 750 | ||
| Lower edge width | 2250 | ||
| Thickness | h | 750 | |
| Distributed force | F | 6 |
| Random Input Parameters | Expected Values | Coefficient of Variation | The Input Parameters’ Limitations: |
|---|---|---|---|
| [Lower, Upper] | |||
| Young’s modulus Y | 1 × 10 | 0.06 | [8.20 × 10, 1.18 × 10] |
| Uniformly distributed force F | 6 × 10 | 0.06 | [4.92 × 10, 7.08 × 10] |
| Flexoelectric constant / | 1 × 10 | 0.1 | [7.00 × 10, 1.30 × 10] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Zhao, J.; Li, H.; Huang, Y.; Yuan, X. A Polynomial Chaos Expansion Method for Mechanical Properties of Flexoelectric Materials Based on the Isogeometric Finite Element Method. Sustainability 2023, 15, 3417. https://doi.org/10.3390/su15043417
Chen L, Zhao J, Li H, Huang Y, Yuan X. A Polynomial Chaos Expansion Method for Mechanical Properties of Flexoelectric Materials Based on the Isogeometric Finite Element Method. Sustainability. 2023; 15(4):3417. https://doi.org/10.3390/su15043417
Chicago/Turabian StyleChen, Leilei, Juan Zhao, Haozhi Li, Yajun Huang, and Xiaohui Yuan. 2023. "A Polynomial Chaos Expansion Method for Mechanical Properties of Flexoelectric Materials Based on the Isogeometric Finite Element Method" Sustainability 15, no. 4: 3417. https://doi.org/10.3390/su15043417
APA StyleChen, L., Zhao, J., Li, H., Huang, Y., & Yuan, X. (2023). A Polynomial Chaos Expansion Method for Mechanical Properties of Flexoelectric Materials Based on the Isogeometric Finite Element Method. Sustainability, 15(4), 3417. https://doi.org/10.3390/su15043417






