Performance Evaluation of Solar Chimney Power Plants with Bayburt Stone and Basalt on the Ground as Natural Energy Storage Material
Abstract
:1. Introduction
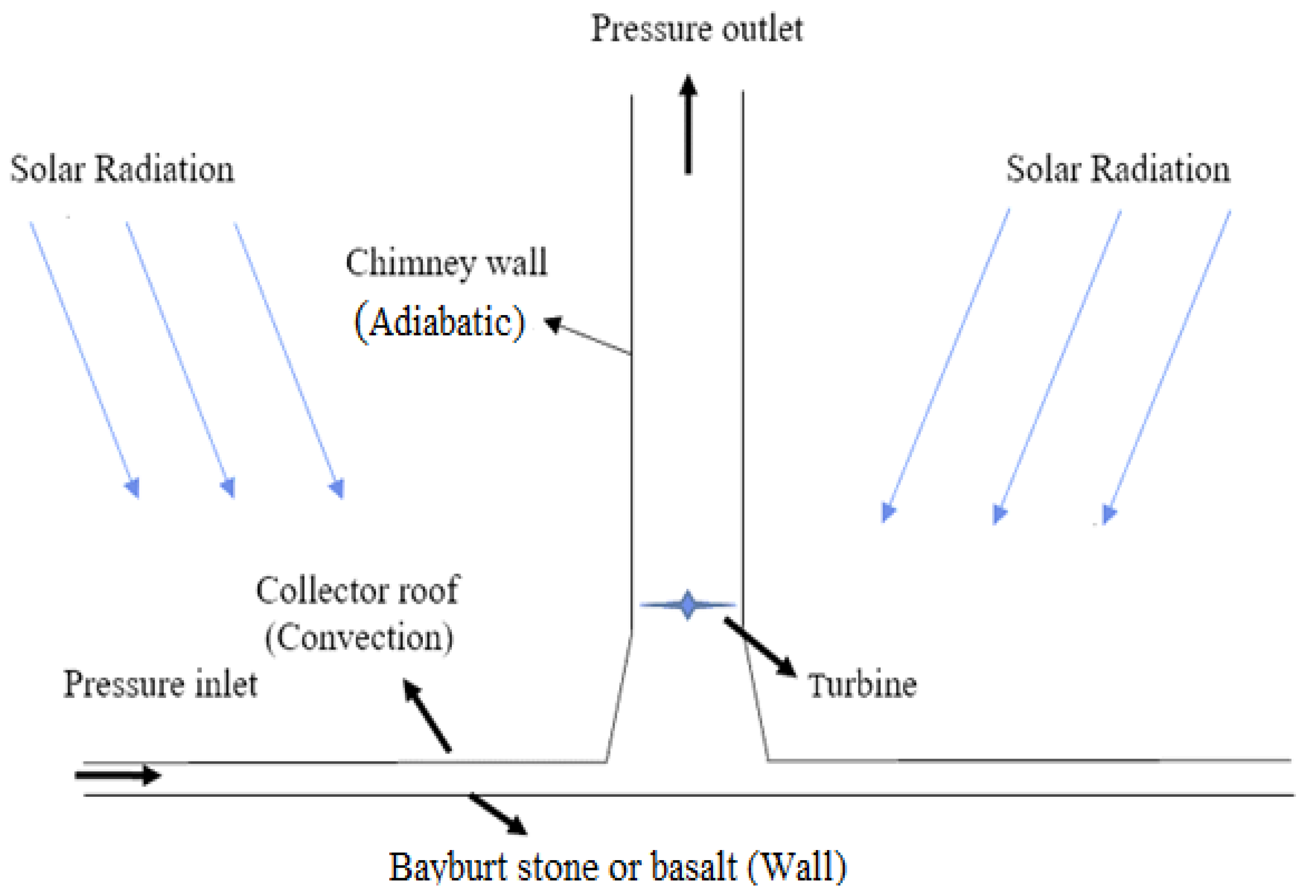
2. CFD Model and System Details
- The flow regime is constant in all cases, 3D and turbulent throughout the system.
- Environmental conditions are constant during each simulation.
- Air, which is the system fluid, is incompressible.
- Boussinesq approximation is used for density variation.
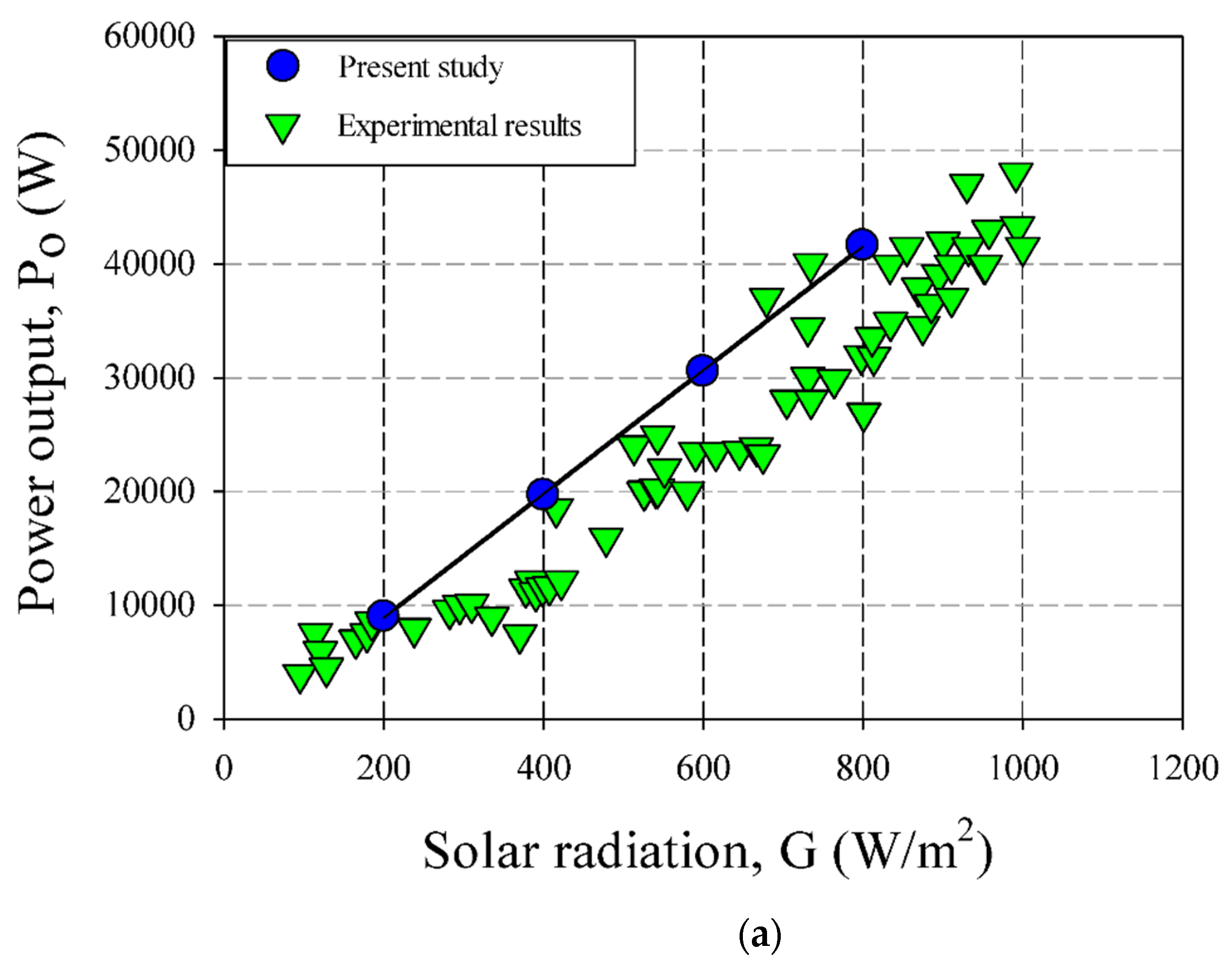
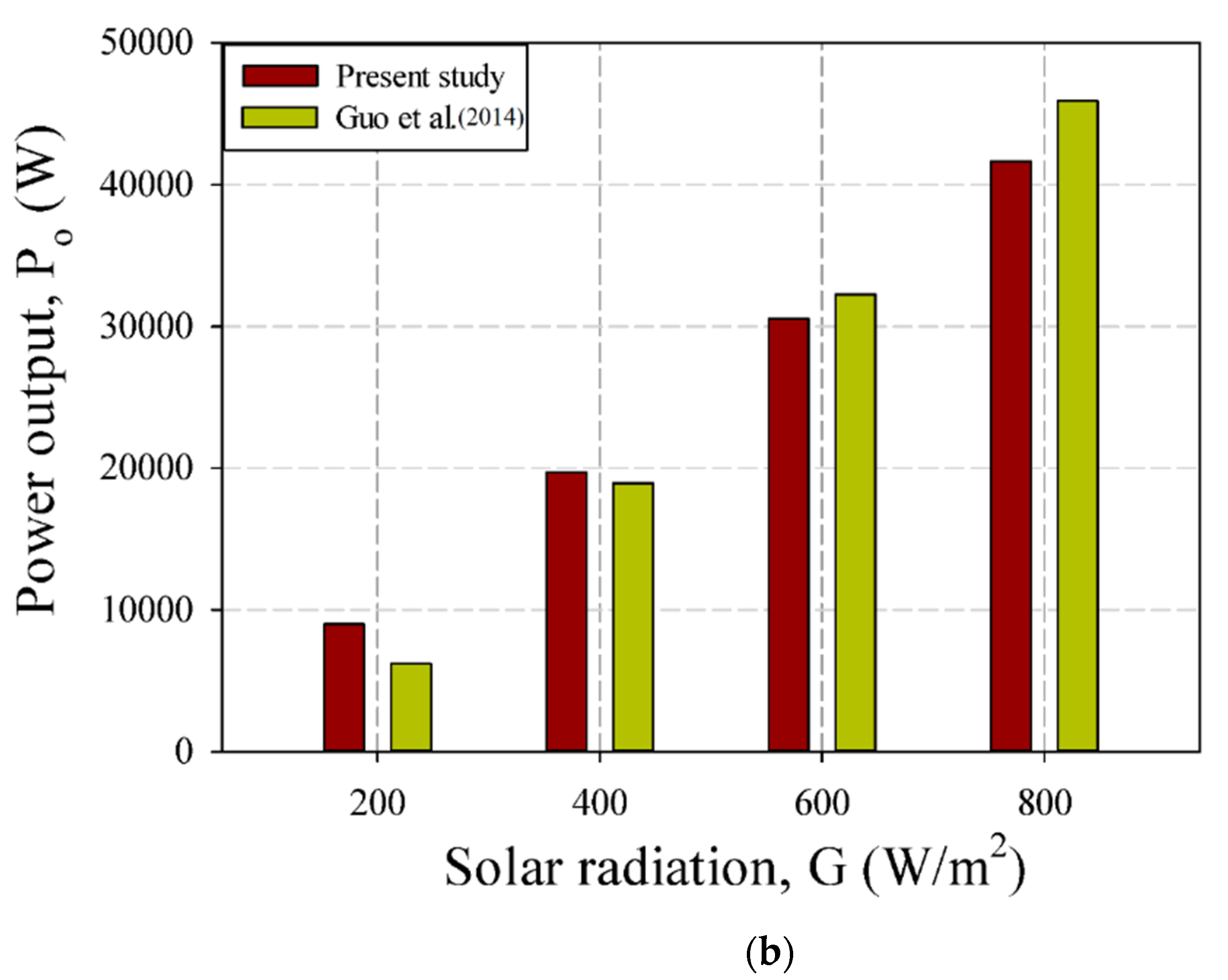
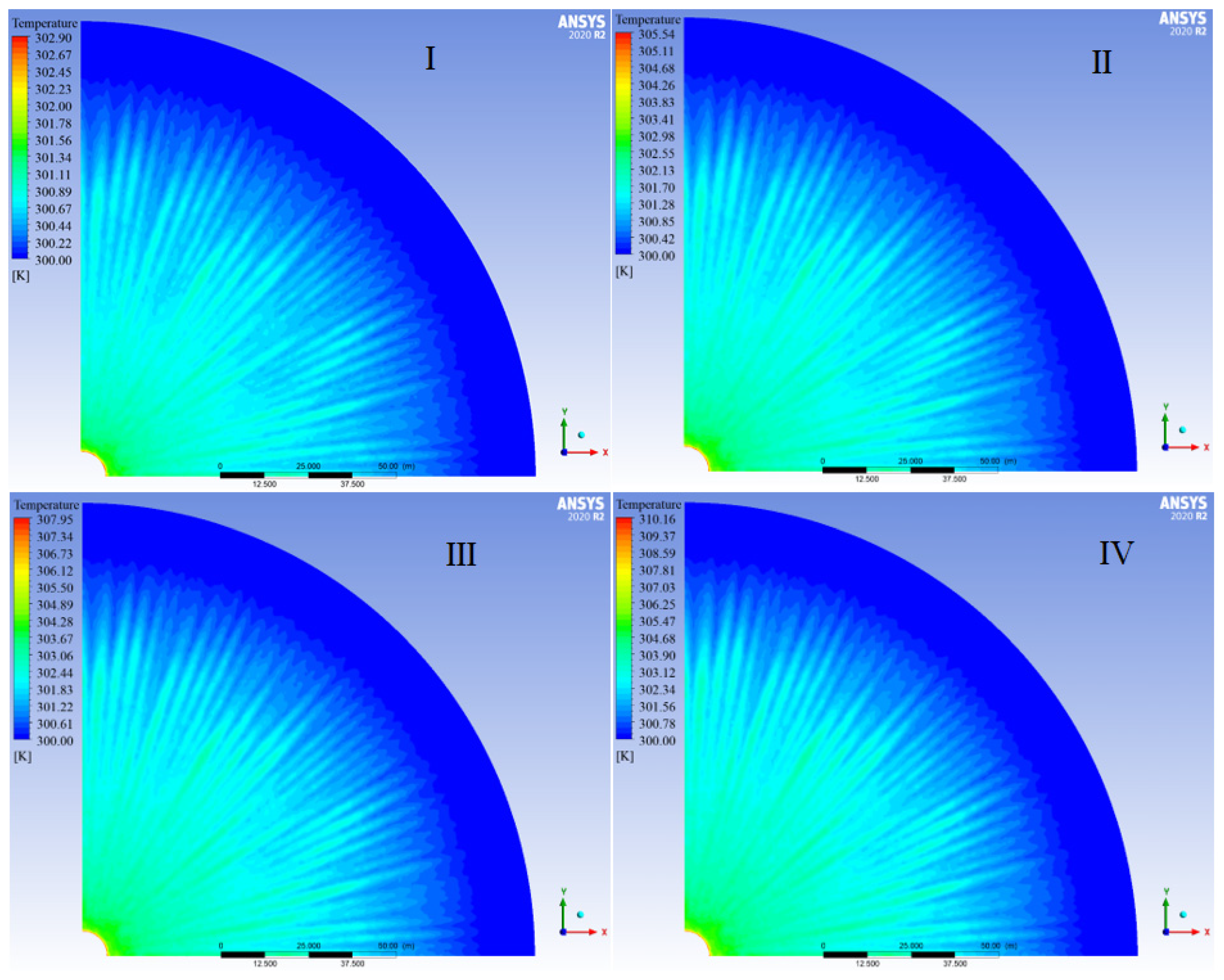
3. Results and Discussion
4. Conclusions
- DO SRTA and RNGTM give consistent results for SCPP.
- The level of solar radiation has a strong effect on the performance of the system.
- The increase in outdoor temperature negatively affects the temperature rise in the system.
- The use of Bayburt stone and basalt for energy storage on the ground shows a similar effect on the system.
- When the storage material is Bayburt stone, the temperature rise in the system is 67.58 K at an outdoor temperature of 290 K. The temperature rise is 64.62 K when the outdoor temperature is 300 K.
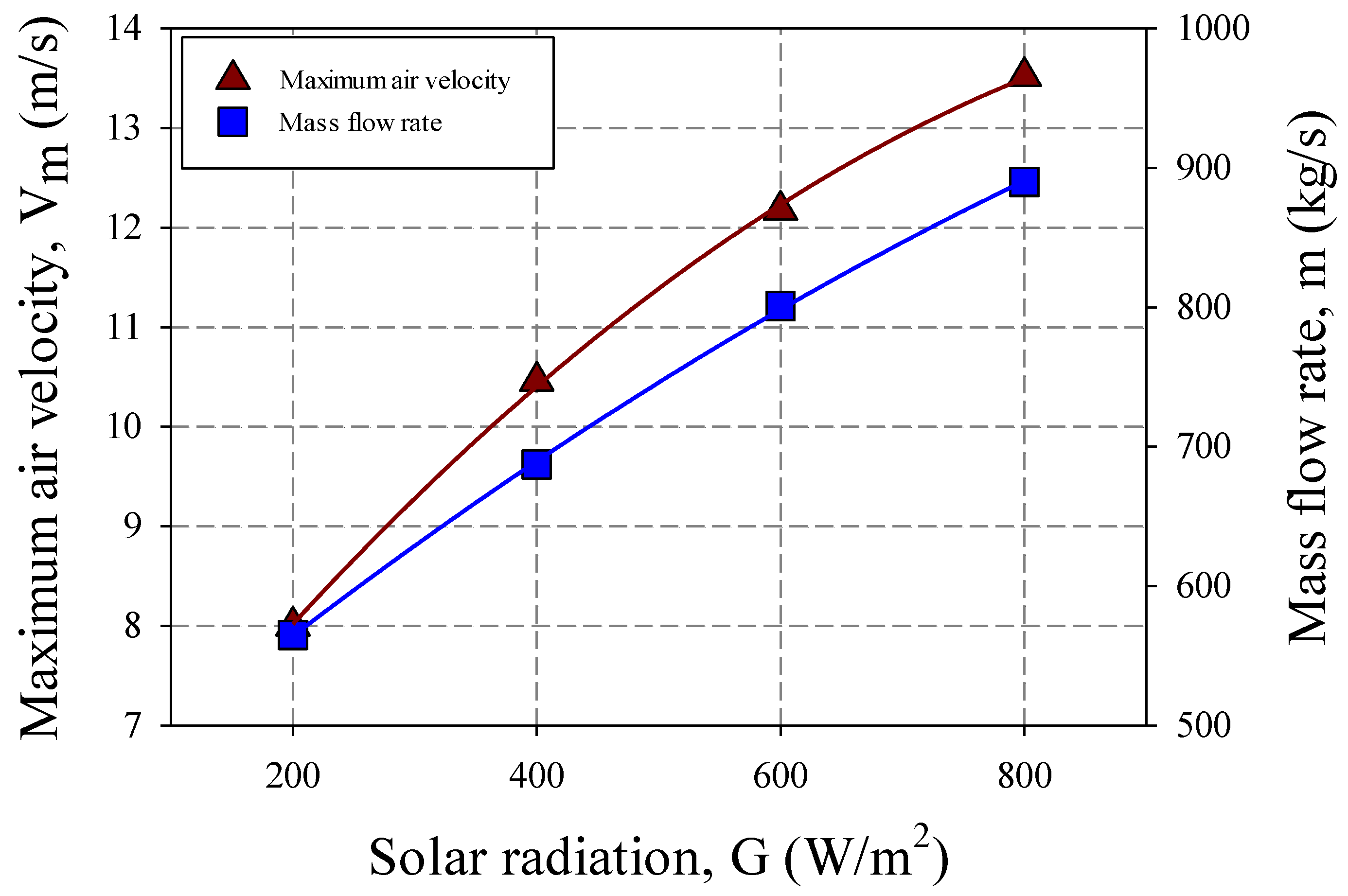
- At solar radiation of 800 W/m2, the maximum airflow rate in the system is 13.519 m/s when the outdoor temperature is 300 K and Bayburt stone is used.
- Solar radiation positively supports the mass flow rate of the system. Compared to 200 W/m2, at 800 W/m2, the mass flow rate increases by 70% and becomes 960.68 kg/s with Bayburt stone.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Collector area (m2) | |
| The specific heat of system air (J/kg·K) | |
| Gravity constant (m/s2) | |
| G | Radiation intensity (W/m2) |
| Po | PO (W) |
| T | Temp. (K) |
| Pt | Pressure near turbine position (Pa) |
| Heat transf. rate (W) | |
| Volume flow rate (m3/s) | |
| rt | TPD (turbine pressure drop) rate |
| SCPPs | Solar chimney power plant system |
| MPP | Manzanares power plant |
| RNG | RNG turbulence model |
| SRTA | Solar ray tracing algorithm |
| α | Outdoors |
| β | Thermal expansion coefficient (1/K) |
| ρ | Density (kg/m3) |
References
- Young, C.H.; Riffat, S.B.; Cuce, E. High capacity energy efficiency solar glass. In Proceedings of the Fourteenth International Conference on Sustainable Energy Technologies 2015, Nottingham, UK, 25–27 August 2015. [Google Scholar]
- Arnaoutakis, G.E.; Katsaprakakis, D.A. Concentrating Solar Power Advances in Geometric Optics, Materials and System Integration. Energies 2021, 14, 6229. [Google Scholar] [CrossRef]
- Schlaich, J.R.; Bergermann, R.; Schiel, W.; Weinrebe, G. Design of commercial solar updraft tower systems—Utilization of solar induced convective flows for power generation. J. Sol. Energy Eng. 2005, 127, 117–124. [Google Scholar] [CrossRef]
- Mullett, L.B. The solar chimney—Overall efficiency, design and performance. Int. J. Ambient. Energy 1987, 8, 35–40. [Google Scholar] [CrossRef]
- Haaf, W. Solar chimneys: Part ii: Preliminary test results from the Manzanares pilot plant. Int. J. Sustain. Energy 1984, 2, 141–161. [Google Scholar] [CrossRef]
- Tayebi, T.; Djezzar, M.; Gouidmi, H. 3D numerical study of flow in a solar chimney power plant system. J. Sci. Technol. 2018, 3, 55–59. [Google Scholar]
- Dhahri, A.; Omri, A.; Orfi, J. Numerical study of a solar chimney power plant. Res. J. Appl. Sci. Eng. Technol. 2014, 8, 1953–1965. [Google Scholar] [CrossRef]
- Cuce, E.; Sen, H.; Cuce, P.M. Numerical performance modelling of solar chimney power plants: Influence of chimney height for a pilot plant in Manzanares, Spain. Sustain. Energy Technol. Assess. 2020, 39, 100704. [Google Scholar] [CrossRef]
- Hu, S.; Leung, D.Y.; Chan, J.C. Impact of the geometry of divergent chimneys on the power output of a solar chimney power plant. Energy 2017, 120, 1–11. [Google Scholar] [CrossRef]
- Cuce, E.; Cuce, P.M. Performance assessment Energy Research Journal of solar chimneys: Part 2—Impacts of slenderness value and collector slope on power output. Energy Res. J. 2019, 10, 20–26. [Google Scholar] [CrossRef]
- Sen, H.; Cuce, E. Dynamic pressure distributions in solar chimney power plants: A numerical research for the pilot plant in Manzanares, Spain. WSSET Newsl. 2020, 12, 2. [Google Scholar]
- Li, J.Y.; Guo, P.H.; Wang, Y. Effects of collector radius and chimney height on power output of a solar chimney power plant with turbines. Renew. Energy 2012, 47, 21–28. [Google Scholar] [CrossRef]
- Karimipour Fard, P.; Beheshti, H. Performance enhancement and environmental impact analysis of a solar chimney power plant: Twenty-four-hour simulation in climate condition of isfahan province, Iran. Int. J. Eng. 2017, 30, 1260–1269. [Google Scholar]
- Rajput, S.R.; Nigam, D.S.R.; Sen, D.M. Integrated solar heat and wind power plant: Design and performance. Int. J. Eng. Sci. Manag. 2017, 7, 407–423. [Google Scholar]
- Ming, T.; de Richter, R.K.; Meng, F.; Pan, Y.; Liu, W. Chimney shape numerical study for solar chimney power generating systems. Int. J. Energy Res. 2013, 37, 310–322. [Google Scholar] [CrossRef]
- Das, P.; Chandramohan, V.P. 3D numerical study on estimating flow and performance parameters of solar updraft tower (SUT) plant: Impact of divergent angle of chimney, ambient temperature, solar flux and turbine efficiency. J. Clean. Prod. 2020, 256, 120353. [Google Scholar] [CrossRef]
- Cuce, E.; Saxena, A.; Cuce, P.M.; Sen, H.; Guo, S.; Sudhakar, K. Performance assessment of solar chimney power plants with the impacts of divergent and convergent chimney geometry. Int. J. Low-Carbon Technol. 2021, 16, 704–714. [Google Scholar] [CrossRef]
- Hassan, A.; Ali, M.; Waqas, A. Numerical investigation on performance of solar chimney power plant by varying collector slope and chimney diverging angle. Energy 2018, 142, 411–425. [Google Scholar] [CrossRef]
- Weli, R.B.; Atrooshi, S.A.; Schwarze, R. Investigation of the performance parameters of a sloped collector solar chimney model–An adaptation for the North of Iraq. Renew. Energy 2021, 176, 504–519. [Google Scholar] [CrossRef]
- Cuce, E.; Cuce, P.M.; Sen, H.; Sudhakar, K.; Berardi, U.; Serencam, U. Impacts of Ground Slope on Main Performance Figures of Solar Chimney Power Plants: A Comprehensive CFD Research with Experimental Validation. Int. J. Photoenergy 2021, 2021, 6612222. [Google Scholar] [CrossRef]
- Cuce, P.M.; Cuce, E.; Sen, H. Improving electricity production in solar chimney power plants with sloping ground design: An extensive CFD research. J. Sol. Energy Res. Updates 2020, 7, 122–131. [Google Scholar] [CrossRef]
- Attig-Bahar, F.; Sahraoui, M.; Guellouz, M.S.; Kaddeche, S. Effect of the ground heat storage on solar chimney power plant performance in the South of Tunisia: Case of Tozeur. Sol. Energy 2019, 193, 545–555. [Google Scholar] [CrossRef]
- Sedighi, A.A.; Deldoost, Z.; Karambasti, B.M. Effect of thermal energy storage layer porosity on performance of solar chimney power plant considering turbine pressure drop. Energy 2020, 194, 116859. [Google Scholar] [CrossRef]
- Ming, T.; Liu, W.; Pan, Y.; Xu, G. Numerical analysis of flow and heat transfer characteristics in solar chimney power plants with energy storage layer. Energy Convers. Manag. 2008, 49, 2872–2879. [Google Scholar] [CrossRef]
- Xu, G.; Ming, T.; Pan, Y.; Meng, F.; Zhou, C. Numerical analysis on the performance of solar chimney power plant system. Energy Convers. Manag. 2011, 52, 876–883. [Google Scholar] [CrossRef]
- Senbeto, M. Numerical simulations of solar chimney power plant with thermal storage. Int. J. Eng. Res. Technol. IJERT 2020, 9, 103–106. [Google Scholar]
- Guo, P.; Wang, Y.; Li, J.; Wang, Y. Thermodynamic analysis of a solar chimney power plant system with soil heat storage. Appl. Therm. Eng. 2016, 100, 1076–1084. [Google Scholar] [CrossRef]
- ANSYS FLUENT Theory Guide. November 2013. Available online: http://www.pmt.usp.br/academic/martoran/notasmodelosgrad/ANSYS%20Fluent%20Theory%20Guide%2015.pdf (accessed on 11 May 2022).
- Tingzhen, M.; Wei, L.; Guoling, X.; Yanbin, X.; Xuhu, G.; Yuan, P. Numerical simulation of the solar chimney power plant systems coupled with turbine. Renew. Energy 2008, 33, 897–905. [Google Scholar] [CrossRef]
- Haaf, W.; Friedrich, K.; Mayr, G.; Schlaich, J. Solar chimneys part I: Principle and construction of the pilot plant in Manzanares. Int. J. Sol. Energy 1983, 2, 3–20. [Google Scholar] [CrossRef]
- Pandey, M.; Padhi, B.N.; Mishra, I. Performance analysis of a waste heat recovery solar chimney for nocturnal use. Eng. Sci. Technol. Int. J. 2021, 24, 1–10. [Google Scholar] [CrossRef]
- Abdelmohimen, M.A.; Algarni, S.A. Numerical investigation of solar chimney power plants performance for Saudi Arabia weather conditions. Sustain. Cities Soc. 2018, 38, 1–8. [Google Scholar] [CrossRef]
- Cuce, P.M. Box type solar cookers with sensible thermal energy storage medium: A comparative experimental investigation and thermodynamic analysis. Sol. Energy 2018, 166, 432–440. [Google Scholar] [CrossRef]
- Chen, W. Analysis of heat transfer and flow in the solar chimney with the sieve-plate thermal storage beds packed with phase change capsules. Renew. Energy 2020, 157, 491–501. [Google Scholar] [CrossRef]
- Setareh, M. Comprehensive mathematical study on solar chimney powerplant. Renew. Energy 2021, 175, 470–485. [Google Scholar] [CrossRef]
- Guo, P.H.; Li, J.Y.; Wang, Y. Numerical simulations of solar chimney power plant with radiation model. Renew. Energy 2014, 62, 24–30. [Google Scholar] [CrossRef]
- Cuce, E.; Cuce, P.M.; Carlucci, S.; Sen, H.; Sudhakar, K.; Hasanuzzaman, M.; Daneshazarian, R. Solar chimney power plants: A review of the concepts, designs and performances. Sustainability 2022, 14, 1450. [Google Scholar] [CrossRef]




| Design Aspect | Value |
|---|---|
| Coll. height | 1.85 m |
| Coll. diameter | 244.0 m |
| Chim. diameter | 10.16 m |
| Chim. height | 194.6 m |
| Ground layer thick | 2 m |
| Feature | Glass | Bayburt Stone | Basalt | Chimney |
|---|---|---|---|---|
| Dens. (kg∙m−3) | 2700 | 2370 | 2695 | 2100 |
| Thermal cond. (W∙m−1K−1) | 0.78 | 0.59 | 1.71 | 1.4 |
| Specific heat (J∙kg−1K−1) | 840 | 714.4 | 920 | 880 |
| Transmissivity | 0.9 | Non-transp. | Non-transp. | Non-transp. |
| Absorptivity | 0.04 | 0.8 | 0.8 | 0.6 |
| Index of refraction | 1 | 1 | 1 | 1 |
| Emissivity | 0.1 | 0.9 | 0.9 | 0.71 |
| Thickness (m) | 0.004 | 2 | 2 | 0.00125 |
| Incoming Solar Intensity (W∙m−2) | 600–800 |
|---|---|
| Outdoor pressure (Pa) | 92,930 |
| Outdoor temperature (K) | 300 |
| Outdoor air dens. (kg/m3) | 1.0795 |
| Gravity const.(m/s2) | 9.81 |
| Thermal cond. (W/mK) | 0.0264 |
| Gas constant (J/kgK) | 287 |
| Kin. viscosity (m/s2) | 1.8 × 10−5 |
| Heat capacity (J/kgK) | 1006.24 |
| TPD ratio | 2/3 |
| Stefan Boltzmann const. (W/m2K4) | 0.5667 × 10−7 |
| Cell No. | Element Size (m) | Max Air Velocity (m/s) | % Difference |
|---|---|---|---|
| 1.08 m | 1 | 13.56 | - |
| 1.43 m | 0.885 | 13.55 | 0.07 |
| 1.78 m | 0.8 | 13.546 | 0.03 |
| Ground Storage Material | Solar Radiation, G (W/m2) | PO, Po (W) |
|---|---|---|
| Bayburt stone | 800 | 41,397 |
| 600 | 30,184 | |
| 400 | 19,067 | |
| 200 | 8430 | |
| Basalt | 800 | 41,427 |
| 600 | 30,185 | |
| 400 | 19,103 | |
| 200 | 8451 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cuce, P.M.; Cuce, E.; Alshahrani, S.; Saboor, S.; Sen, H.; Veza, I.; Saleel, C.A. Performance Evaluation of Solar Chimney Power Plants with Bayburt Stone and Basalt on the Ground as Natural Energy Storage Material. Sustainability 2022, 14, 10960. https://doi.org/10.3390/su141710960
Cuce PM, Cuce E, Alshahrani S, Saboor S, Sen H, Veza I, Saleel CA. Performance Evaluation of Solar Chimney Power Plants with Bayburt Stone and Basalt on the Ground as Natural Energy Storage Material. Sustainability. 2022; 14(17):10960. https://doi.org/10.3390/su141710960
Chicago/Turabian StyleCuce, Pinar Mert, Erdem Cuce, Saad Alshahrani, Shaik Saboor, Harun Sen, Ibham Veza, and C. Ahamed Saleel. 2022. "Performance Evaluation of Solar Chimney Power Plants with Bayburt Stone and Basalt on the Ground as Natural Energy Storage Material" Sustainability 14, no. 17: 10960. https://doi.org/10.3390/su141710960
APA StyleCuce, P. M., Cuce, E., Alshahrani, S., Saboor, S., Sen, H., Veza, I., & Saleel, C. A. (2022). Performance Evaluation of Solar Chimney Power Plants with Bayburt Stone and Basalt on the Ground as Natural Energy Storage Material. Sustainability, 14(17), 10960. https://doi.org/10.3390/su141710960










