Upgrading Strategy, Warranty Policy and Pricing Decisions for Remanufactured Products Sold with Two-Dimensional Warranty

Abstract

The environmental sustainability and business benefits of end-of-life products have led to worldwide growth in the remanufacturing market. However, customers are often sceptical about the quality and durability of remanufactured products. To ensure a risk-free customer experience, dealers carry out some upgrading actions on the most critical components and offer a reasonable warranty period on products at the time of resale. It is crucial for dealers to consider customers’ attitudes and preferences when deciding on an upgrading strategy, warranty coverage, and sales price for remanufactured products. This paper aims to establish an equilibrium between customers’ expected costs and dealers’ expected profit for remanufactured products sold with a two-dimensional warranty and post-warranty service. In our model, customers make their decisions based on cost–benefit balance, whereas dealers make decisions that maximise their profit margin. Owing to the nature of the conflict between the dealer and customers, a Stackelberg game model is developed to optimise the upgrade strategy, warranty policy, and pricing decisions for remanufactured products. The Karush–Kuhn–Tucker (KKT) optimality condition of the lower-level problem is used to solve the model. Finally, a numerical example is provided to illustrate its applicability.

1. Introduction

Recent technological advances have increased the lifespan of consumable products. A longer life for products will enable businesses to generate new revenue streams and contribute to a circular economy for a more sustainable future. Remanufacturing is a new trend in the field of environmentally friendly and resource-saving materials. It refers to the process of returning used products (i.e., end-of-life products) to ‘like-new’ conditions by rebuilding or repairing major components that are close to failure [1]. Remanufacturing offers many benefits to both dealers and customers. It provides an opportunity for dealers to make a profit from the remanufacturing/recycling of used products (along with selling new products to customers), reduce their energy and water consumption, and lower their greenhouse gas emissions. On the other side, as remanufacturing is less expensive than manufacturing a new product, it will offer more affordable prices to customers with lower purchasing power [2].

Despite all the above-mentioned benefits, there are some challenges obstructing the growth of the remanufacturing market. One of the most remarkable problems is that as customers have little information about the past life and/or maintenance history of remanufactured products, they are often sceptical about their quality and durability. Wahjudi et al. [3] reported that the risk attitude of customers and the selling price of remanufactured products are two most important factors affecting purchase intention. To ensure a risk-free customer experience, dealers carry out some upgrading actions on the most critical elements, as well as offering a reasonable warranty on remanufactured products before returning them to the market. Warranties—including base and extended warranties—play a very important role in reassuring customers about the durability and performance of products. Nowadays, a variety of remanufactured products such as toner/printer cartridges, automobile parts, and electronic components are sold with different types of warranty. In general, the warranty policies for industrial products can be categorised into two types, i.e., one-dimensional (1D) and two-dimensional (2D). The main difference between 1D and 2D warranties is that the former is characterised by an interval, called the warranty period, while the latter is described by a region in a two-dimensional plane, with one axis representing the product age (in terms of number of years) and the other one representing product usage (in terms of, for instance, frequency of use).

With the rapidly increasing number of end-of-life products, it is crucial for dealers to have a thorough understanding of the risks and complexities associated with major engineering and marketing decisions in the remanufacturing business. The upgrading strategy, warranty policy, and selling price are three strategic decisions that can be critical to the success of any remanufacturing system. Determining the optimal level of these three control variables is very complex, as it involves consideration of several technical and economic parameters. Upgrading end-of-life products excessively and/or offering long warranty periods for upgraded items—regardless of affordability—may make the remanufacturing markets less competitive, and, eventually, will lead some customers to opt for lower priced products. A review of the literature shows that a lot of research has been dedicated to optimising the upgrading strategy and 1D warranty policy of remanufactured or refurbished products. In what follows, some of the literature relevant to this subject will be reviewed:

Shafiee et al. [4] acknowledged uncertainties associated with the remanufacturing process and determined an optimal upgrading strategy for remanufactured products sold with a failure free warranty (FRW). Under such a policy, items are replaced/repaired free of cost to customers so long as they are under warranty. Saidi-Mehrabad et al. [5] presented two upgrading methods to improve the reliability of remanufactured items, namely, a virtual age model and a screening test method. Jalali-Naini and Shafiee [6] proposed an optimisation framework to determine the upgrading strategy as well as selling price of remanufactured products sold with a warranty. Shafiee et al. [7] performed a stochastic cost–benefit analysis to evaluate the efficiency of investments made by dealers to improve the reliability of remanufactured products sold with a FRW policy. Shafiee et al. [8] developed a stochastic model to determine the optimal upgrading strategy for used products having an increasing failure rate (IFR) and according to given profit structures. Shafiee and Chukova [9] presented a three-parameter optimisation model to determine the optimal strategy for dealers in terms of upgrading, warranty length, and sale price for remanufactured products. Su and Wang [10] proposed a profit-maximisation model to determine the optimal upgrading strategy and preventive maintenance (PM) policy for remanufactured products sold with a non-renewing FRW policy. Kim et al. [11] determined the optimal upgrading strategy as well as the frequency of PM actions for remanufactured products such that dealers’ total expected costs were minimised. Liao et al. [12] studied a warranty policy for remanufactured products and presented three marketing settings to examine the impact of warranties on consumer behaviour as well as manufacturers’ profit. Otieno and Liu [13] presented a framework to optimise the warranty period for remanufactured electrical products such that the manufacturer’s total expected profit was maximised. Alqahtani and Gupta [14] proposed an optimal warranty policy for sensor embedded remanufactured products such that, simultaneously, the cost incurred by remanufacturers was minimised and customer confidence regarding the purchase of remanufactured products was maximised. Kim et al. [15] determined an optimal sequential inspection schedule for remanufactured products after the expiration of the non-renewing FRW policy such that the expected maintenance cost for customers during the life cycle of the product was minimised. At each inspection, the failure rate of the product was reduced proportionally. Darghouth et al. [16] developed a cost minimisation model to optimise upgrading strategies, as well as a periodic PM policy for remanufactured products sold with a FRW policy. The model was used to assess whether performing PM actions during the warranty period, according to a specific maintenance strategy, is worthwhile in terms of cost reduction. In another study, Darghouth and Chelbi [17] developed a decision model to determine the optimal upgrading strategy, warranty period and PM effort for second-hand products such that the dealer’s total expected profit—which was a function of the product’s age and sales volume—was maximised. Tang et al. [18] proposed a Stackelberg game model to examine pricing and warranty decisions for remanufactured products in a two-period, closed-loop supply chain system consisting of a manufacturer and a retailer. Zhu and Yu [19] presented a Stackelberg game model to analyse the impact of warranty efficiency on customer confidence in terms of purchasing remanufactured products in a closed-loop supply chain system. The results of the study showed that consumer confidence in purchasing remanufactured products increased the demand for both new and remanufactured products. Cao et al. [20] investigated the optimal trade-in and warranty period for dealers who sell both new and remanufactured products taking into account carbon tax and trade-in subsidies policies. Two theoretical models were presented, namely, TIA, which offers trade-in services to all products, and TIR, which offers trade-in service to only remanufactured products.

In contrast with the vast literature on 1D warranties, 2D warranties for remanufactured products have received very little attention to date. Shafiee et al. [21] developed statistical analysis models to estimate dealers’ expected warranty costs for remanufactured products sold with a 2D FRW policy. Dealers’ costs were formulated as a function of product reliability, past age and usage, servicing strategy and conditions and terms of the warranty policy. Su and Wang [22] proposed mathematical models to determine the optimal upgrading strategy for remanufactured items sold with a 2D warranty such that dealers’ total expected servicing costs (including upgrading cost and warranty servicing cost) were minimised. In another work, Su and Wang [23] developed a bivariate failure modelling framework to evaluate the effect of reliability improvement actions on the age/usage of end-of-life products sold with a 2D FRW policy. The optimal reliability improvement policy was determined to minimise the total expected servicing cost per unit sale from the dealer’s perspective. Alqahtani and Gupta [24] proposed a discrete-event simulation model to evaluate different 2D warranty policies for remanufactured products. They caried out pairwise comparison tests along with one-way analyses of variance (ANOVA) to assess the impact of warranty and PM on the remanufacturer’s total cost. In another study, Wang et al. [25] proposed a modelling approach to investigate the effect of customer usage heterogeneity, upgrading process and PM actions on a remanufactured product’s reliability as well as the corresponding expected 2D warranty cost to the dealer.

From the above reviewed papers, it can be concluded that most of the literature on the subject is devoted to studying the warranty decision-making problem for remanufactured products from the dealer’s perspective. However, customers’ preferences regarding warranty services and product prices play an important role in remanufacturers’ marketing strategy and dealers’ profit margins. Moreover, an analysis of the remanufacturing markets reveals that warranty options for remanufactured products are very limited and almost non-negotiable. For example, certified pre-owned (CPO) warranties in the remanufactured automobile market are limited to only one year of operation and 12,000 miles of usage beyond the base warranty period, where price is non-negotiable. However, customers with different usage patterns may desire a variety of options for warranty coverage, type of warranty policy, quality, and price of the services. To shift from remanufacturer-led markets to customer-led markets, there is an essential need for remanufacturers to design customised warranty plans that will best suit customer preferences and, at the same time, boost sales volume and increase the profitability. Therefore, considering customers as the market leaders and then optimising dealer benefits based on customer preferences would be more insightful to decision-makers, helping them formulate the transactions in a win-win manner in the remanufacturing market.

In the present study, a Stackelberg game-theoretic decision-making approach is presented to establish an equilibrium between customers’ expected costs and dealer’s expected profit for remanufactured products sold with a 2D warranty and post-warranty service. In this model, the purchasing price of an end-of-life item, as well as the dealer’s upgrading costs, are assumed to be functions of the product’s age and usage. To evaluate the effect of upgrading actions on the failure rate of a product, a reliability improvement factor approach is proposed. The sales volume of the remanufactured product is formulated as a function of its selling price and the expenses that customers have to bear after the warranty period expires. Also, customers are given this option to decide on the best upgrade level and warranty coverage in order to minimise their expected expenses during the period of product use. Consequently, the dealer will maximise his/her expected profit under the upgrade strategy and warranty policy chosen by the customers.

The rest of this paper is organised as follows. Section 2 presents the model assumptions and notation. Section 3 describes the components of the model including customers’ expected costs and dealers’ expected profit. Section 4 develops a customer-dealer game theoretic model and proposes a solution approach to solve the problem. In Section 5, a numerical example is provided. Finally, some conclusions are discussed in Section 6.

2. Model Assumptions and Notation

In this Section, we present the assumptions and notation used in our model formulation.

Assumptions

• The base-warranty of an end-of-life product has expired when it is sold by a user to a dealer.
• The purchasing price of an end-of-life product depends on the reliability of the product, which is considered to be dependent on its age and usage.
• Each end-of-life product is disassembled into parts, and after an appropriate upgrading process, the parts will be reused.
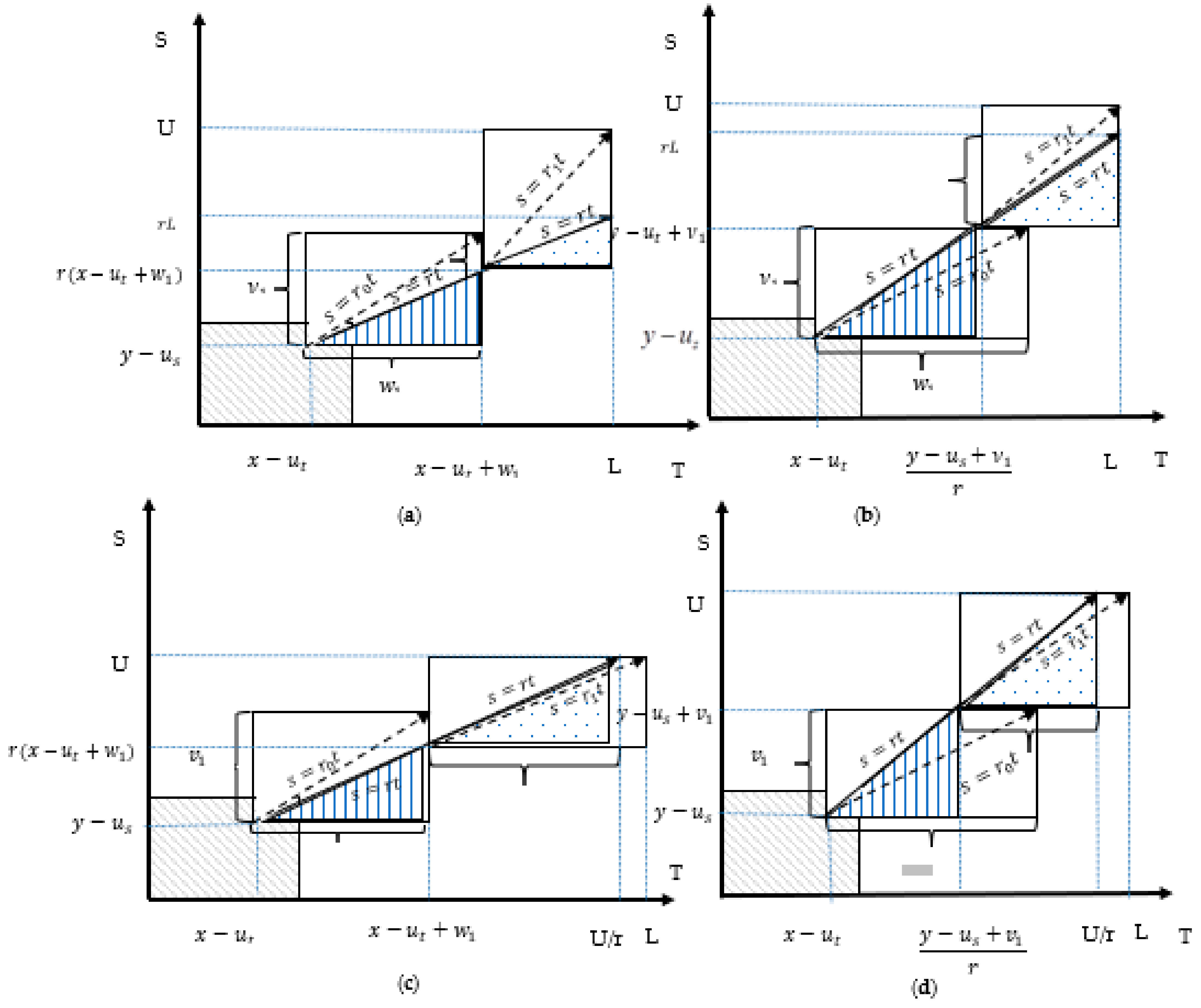
• The warranty coverage offered by the dealer for remanufactured products is two-dimensional (2D), i.e., both the product’s age and usage will be used to characterise the warranty.
• Customers will have the option to choose the upgrading strategy as well as the warranty policy for the products that they purchase.
• The number of product failures during a given operation period is assumed to follow a non-homogeneous Poisson process (NHPP).
• All the product failures during warranty are repaired minimally by the dealer with no charge to the consumer.
• The time to repair a faulty item is considerably smaller than the mean inter-failure time, so the repair time is assumed to be negligible.
• Customers are considered to be the market leader, aiming to minimise their expected costs during the remaining life of the product by choosing the most affordable upgrading strategy and warranty policy.
• The dealer is considered to be the market follower, aiming to maximise his/her profit margin based on customer preferences about the upgrading and warranty.
• The demand for the remanufactured product is a nonlinear function of its selling price, as well as the expected warranty servicing costs.

3. Components of the Model

In this section, we present mathematical equations with which to derive the product purchasing price from an end-user, the expected cost of upgrading actions carried out by the dealer, the dealer’s expected cost of warranty, price of upgrading and warranty services for the customers, and the expected cost of failure rectification after the expiration of the warranty period until the product is disposed of.

3.1. The Purchasing Price of an End-of-Life Product from an End-User

As stated in the assumptions, the purchasing price of an end-of-life product from an end-user depends on the reliability performance of that product, which, in turn, is a function of the product’s age and past usage. In this paper, we assume that the purchasing price of a product from an end-user (c_p) is a decreasing function of the product’s age (x) and/or past usage (y); this is given by:

$$c_p=c_{sv}+\left(p_0-c_{sv}\right)\times b; 0\le b\le 1$$

(1)

where p₀ is the sale price of the new product, c_sv is the salvage value of the product at the end of its life (i.e., either at age L or usage U, whichever comes first), and b has a Beta distribution with parameters a₁ and a₂, that are dependent on x and y. The Beta distribution parameters a₁ and a₂ are modelled by the following equations [7]:

$$a_1={\alpha }_1{x}^{{\beta }_1}; a_2={\alpha }_2{y}^{-{\beta }_2}; 0 \le x \le L, 0 \le y \le U,$$

(2)

where α₁, α₂, β₁, and β₂ are constant parameters that must be chosen such that the value of b declines with an increase of the product’s age (x) and/or usage (y). In practice, these parameters are determined using an appropriate regression model. As parameter b has a Beta distribution, the purchase price of a product with age x and usage y from an end-user can be expressed by:

$$c_p={{\displaystyle \int }}_0^1\left[c_{sv}+\left(p_0-c_{sv}\right)\times b\right]\times B\left(b\right)d,$$

(3)

where B(b) represents the probability density function (PDF) of Beta distribution and is given by:

$$B\left(b\right)=\frac{\mathsf{\Gamma }\left(a_1+a_2\right)}{\mathsf{\Gamma }\left(a_1\right)\times \mathsf{\Gamma }\left(a_2\right)}{b}^{a_1-1}{\left(1-b\right)}^{a_2-1}; 0 \le b \le 1,$$

(4)

where $\mathsf{\Gamma }\left(.\right)$ is the Gamma function defined by $\mathsf{\Gamma }\left(z\right)={{\displaystyle \int }}_0^{\infty }{x}^{z-1}{e}^{-x}dx$. Note that when the product is new, it will be sold at the price of a new product, i.e., $b=1$. In the case when the product has reached its maximum life, it will be disposed of with a salvage value of c_sv, i.e., $b=0$.

3.2. The Upgrading Cost

The product upgrading process consists of a collection of tasks that are carried out by the dealer to improve the reliability and safety of the product before selling it to a new customer. Overhauling, replacing deteriorated parts with a new or younger items, non-destructive testing (NDT), and cleaning and painting are some examples of upgrading tasks. These tasks usually restore the condition of the product to somewhere between that of a ‘new’ product and a ‘pre-upgrade’ condition (i.e., the condition before the system was upgraded). The effect of an upgrading action on the reliability of products can be modelled using some classical approaches, such as the virtual age approach, which was introduced by Kijima et al. [26], the improvement factor approach, which was introduced by Malik [27], the probabilistic approach, which was introduced by Brown and Proschan [28], etc. In this paper, the failure rate modification/reduction approach—as presented in [7]—will be used.

Let T and S represent two random variables describing, respectively, the time and the usage to failure of the product. The joint probability density function (PDF) and cumulative distribution function (CDF) of random variables T and S are denoted by f(t,s) and F(t,s), respectively. We assume that the product has undergone minimal repairs during its past life, represented by Ω₀ = [0, x] × [0, y]. Therefore, the failure/repair process of the product follows a non-homogeneous Poisson process (NHPP) with a rate of occurrence of failures (ROCOF) of r(t,s) = f(t,s)/[1 − F(t,s)]. At age x and usage y, the product is subjected to an upgrading action with the upgrade level u = (u_t, u_s), where 0 ≤ u_t ≤ x and 0 ≤ u_s ≤ y. We assume the failure rate function after upgrading is expressed by r(t,s;u) = r (t − γu_t, s − τu_s), where γ and τ ∈ [0, 1] represent the effectiveness of the upgrading process on the age and usage, respectively.

The upgrade level u can be a decision variable chosen by either dealers or customers, or both. In general, the higher the upgrade level, the better the condition of the product after upgrading but the higher the expense of the upgrading process. The cost of upgrading a product with past age x and past usage y by a level of u = (u_t, u_s) is modelled by a nonlinear function, given by Equation (5):

$$c_u\left(x,y\right)=c_s+c_u\times {\left(b\right)}^{\xi }{u}_t^{\psi }{u}_s^{\phi }$$

(5)

where c_s is the fixed set-up cost of the upgrading process, and parameters c_u, ζ, ψ and φ are non–negative constant values that can be estimated by means of an appropriate regression model. As the parameter b is assumed to have a Beta distribution, the expected upgrading cost will be modelled by:

$$c_u\left(x,y\right)={{\displaystyle \int }}_0^1(c_s+c_u\times (b{)}^{\xi }{u}_t^{\psi }{u}_s^{\phi })\times B\left(b\right)db$$

(6)

3.3. Dealer’s Expected Costs during the Warranty Period

We assume that customers have a usage rate of r during the warranty period. Customers’ usage rate is considered as a random variable having a probability density function (PDF) and a cumulative distribution function, i.e., (CDF) of g_R(.) and G_R(.), respectively.

Let E [N_w(u_t, u_s, w₁, v₁)] represent the expected number of repairs carried out by the dealer within the 2D warranty coverage region, i.e., Ω_w = [x − u_t, x − u_t + w₁] × [y − u_s, y − u_s + v₁]. The warranty coverage regions for two cases of (i) r < r₀, and (ii) r ≥ r₀, where r₀ = v₁/w₁, are shown in Figure 1. While 1D warranties have a certain expiration time, in 2D cases, the warranty coverage begins from the point that the product is upgraded and expires at an unknown time depending on the customers’ usage rate. The usage rate r varies from one customer to another, but will remain constant for a given customer. When the warranty coverage expires, the usage of the product during the warranty coverage period changes from y − u_s to s = rt (see Figure 1a). On the other hand, if the warranty coverage expires due to usage, then the age of the product during the warranty coverage period will change from x − u_t to (y − u_s + v₁)/r (see Figure 1b). Therefore,

$$E\left[N_w\left(u_t,u_s,w_1,v_1,\left|r\right.\right)\right]=\left\{\begin{array}{c}{{\displaystyle \int }}_{x-u_t}^{x-u_t+w_1}{{\displaystyle \int }}_{y-u_s}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt, r<r_0\\ {{\displaystyle \int }}_{x-u_t}^{\frac{y-u_s+v_1}{r}}{{\displaystyle \int }}_{y-u_s}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt,r\ge r_0\end{array}\right.$$

(7)

Given that the PDF of the usage rate is represented by g_R(.), the expected number of repairs carried out by the dealer within the 2D warranty coverage region is estimated by:

$$\begin{array}{cc}E\left[N_w\left(u_t,u_s,w_1,v_1,\right)\right]=\hfill & {{\displaystyle \int }}_0^{r_0}\left({{\displaystyle \int }}_{x-u_t}^{x-u_t+w_1}{{\displaystyle \int }}_{y-u_s}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt\right)g_R\left(r\right)dr+\\ & {{\displaystyle \int }}_{r_0}^{\infty }\left({{\displaystyle \int }}_{x-u_t}^{\frac{y-u_s+v_1}{r}}{{\displaystyle \int }}_{y-u_s}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt\right)g_R\left(r\right)dr\end{array}$$

(8)

where γ and τ represent the effectiveness of the upgrading process in reducing the product’s age and usage, respectively. γ and τ are assumed to follow a uniform distribution with the PDFs of, respectively, U(γ) and U(τ) on the interval [0, 1]. Therefore,

$$\begin{array}{cc}E\left[N_w\left(u_t,u_s,w_1,v_1\right)\right]\hfill & \\ \hfill = & {{\displaystyle \int }}_0^1{{\displaystyle \int }}_0^1\left\{\begin{array}{c}{{\displaystyle \int }}_0^{r_0}\left({{\displaystyle \int }}_{x-u_t}^{x-u_t+w_1}{{\displaystyle \int }}_{y-u_s}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt\right)g_R\left(r\right)dr\\ +{{\displaystyle \int }}_{r_0}^{\infty }\left({{\displaystyle \int }}_{x-u_t}^{\frac{y-u_s+v_1}{r}}{{\displaystyle \int }}_{y-u_s}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt\right)g_R\left(r\right)dr\end{array}\right\}U\left(\gamma \right)U\left(\tau \right)d\gamma d\tau \end{array}$$

(9)

Let c_r represent the average cost of a repair action carried out by the dealer during warranty coverage. Then, the total expected cost of the dealer associated with repair services during warranty coverage, E [c_w(u_t, u_s, w₁, v₁)] is given by:

$$E\left[c_w\left(u_t,u_s,w_1,v_1\right)\right]=c_r\times E\left[N_w\left(u_t,u_s,w_1,v_1\right)\right]$$

(10)

3.4. The Expected Price of Upgrading and Warranty for Customers

The expected price that customers have to pay for warranty coverage and upgrading services depends on the total cost of repairs during the warranty period as well as the cost associated with upgrade process. In addition, the dealer’s profit for these services should also be included when pricing the product for resale. Therefore, the expected price of the warranty policy and upgrading strategy for the new user, E [p_u,w(u_t, u_s, w₁, v₁, δ_u,w)], is expressed by:

$$E[p_{u,w}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)]=\left(1+{\delta }_{u,w}\right)\left(c_u\left(x,y\right)+E\left[c_w\left(u_t,u_s,w_1,v_1\right)\right]\right)$$

(11)

where δ_u,w represents the percentage of the dealer’s profit margin from offering the warranty and upgrading services to customers, c_u(x,y) is the expected upgrade cost which is given by Equation (6), and E [c_w(u_t, u_s, w₁, v₁)] is the total expected cost of the dealer due to repair services during warranty coverage, which is given by Equation (10). Therefore, the expected selling price of the remanufactured product to customers is given by:

$$E\left[P\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)\right]=c_p+E\left[p_{u,w}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)\right]$$

(12)

where c_p and E [p_u,w(u_t, u_s, w₁, v₁, δ_u,w)] are given by Equations (3) and (11), respectively.

3.5. The Dealer’s Expected Cost for Post-Warranty Services

Let E [N_pw(u_t, u_s, w₁, v₁)] represent the expected number of repairs carried out by the dealer during the post-warranty coverage period. The post-warranty regions, Ω_pw, for four cases are shown in Figure 2, including two cases for r < r₁, where r₁ = [U − (y − u_s + v₁)]/[L − (x − u_t + w₁)] (see Figure 2a,b) and two cases for r ≥ r₁ (see Figure 2c,d). Therefore,

$$\begin{array}{cc}E\left[N_{pw}\right(u_t,u_s,w_1,\hfill & v_1\left|r\right.\left)\right]=\hfill \\ & \left\{\begin{array}{c}{{\displaystyle \int }}_{x-u_t+w_1}^L{{\displaystyle \int }}_{r\left(x-u_t+w_1\right)}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt+{{\displaystyle \int }}_{x-u_t+w_1}^{\frac{U}{r}}{{\displaystyle \int }}_{r\left(x-u_t+w_1\right)}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt, r<r_1\hfill \\ {{\displaystyle \int }}_{\frac{y-u_s+v_1}{r}}^L{{\displaystyle \int }}_{y-u_s+v_1}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt+{{\displaystyle \int }}_{\frac{y-u_s+v_1}{r}}^{\frac{U}{r}}{{\displaystyle \int }}_{y-u_s+v_1}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt, r\ge r_1\end{array}\right.\end{array}$$

(13)

The expected number of repairs carried out by the dealer within the 2D post-warranty coverage period is estimated by:

$$\begin{array}{c}E\left[N_{pw}\left(u_t,u_s,w_1,v_1\right)\right]={{\displaystyle \int }}_0^{r_1}\left({{\displaystyle \int }}_{x-u_t+w_1}^L{{\displaystyle \int }}_{r\left(x-u_t+w_1\right)}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt+{{\displaystyle \int }}_{\frac{y-u_s+v_1}{r}}^L{{\displaystyle \int }}_{y-u_s+v_1}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt\right)g_R\left(r\right)dr\\ +{{\displaystyle \int }}_{r_1}^{\infty }\left({{\displaystyle \int }}_{x-u_t+w_1}^{\frac{U}{r}}{{\displaystyle \int }}_{r\left(x-u_t+w_1\right)}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt+{{\displaystyle \int }}_{\frac{y-u_s+v_1}{r}}^{\frac{U}{r}}{{\displaystyle \int }}_{y-u_s+v_1}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt\right)g_R\left(r\right)dr\end{array}$$

(14)

As parameters γ and τ are assumed to follow a uniform distribution with the PDFs of U(γ) and U(τ), we have:

$$\begin{array}{c}E\left[N_{pw}\left(u_t,u_s,w_1,v_1\right)\right]={{\displaystyle \int }}_0^1{{\displaystyle \int }}_0^1\left\{{{\displaystyle \int }}_0^{r_1}\right({{\displaystyle \int }}_{x-u_t+w_1}^L{{\displaystyle \int }}_{r\left(x-u_t+w_1\right)}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt+{{\displaystyle \int }}_{\frac{y-u_s+v_1}{r}}^L{{\displaystyle \int }}_{y-u_s+v_1}^{rt}\\ r\left(t-\gamma u_t,s-\tau u_s\right)dsdt)g_R\left(r\right)dr+{{\displaystyle \int }}_{r_1}^{\infty }({{\displaystyle \int }}_{x-u_t+w_1}^{\frac{U}{r}}{{\displaystyle \int }}_{r\left(x-u_t+w_1\right)}^{rt}r\left(t-\gamma u_t,s-\tau u_s\right)dsdt+{{\displaystyle \int }}_{\frac{y-u_s+v_1}{r}}^{\frac{U}{r}}{{\displaystyle \int }}_{y-u_s+v_1}^{rt}\\ r\left(t-\gamma u_t,s-\tau u_s\right)dsdt\left)g_R\left(r\right)dr\right\}U\left(\gamma \right)U\left(\tau \right)d\gamma d\tau \end{array}$$

(15)

Therefore, the total expected cost of the dealer associated with repair services during post-warranty coverage, E [c_pw(u_t, u_s, w₁, v₁)], is given by:

$$E\left[c_{pw}\left(u_t,u_s,w_1,v_1\right)\right]=c_r\times E\left[N_{pw}\left(u_t,u_s,w_1,v_1\right)\right]$$

(16)

3.6. The Expected Price of Post-Warranty Service for Customers

The expected price that customers have to pay for post-warranty service depends on the total cost of repairs covered by the dealer within the 2D post-warranty coverage period. In addition, the dealer’s profit for this service should also be included when pricing the product for resale. Therefore, the expected price of the post-warranty policy for customers, E [p_pw(u_t, u_s, w₁, v₁, δ_pw)], is expressed by:

$$E[p_{pw}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)]=\left(1+{\delta }_{pw}\right)\left(E\left[c_{pw}\left(u_t,u_s,w_1,v_1,\right)\right]\right)$$

(17)

where ${\delta }_{pw}$ represents the percentage of the dealer’s profit margin from offering the post-warranty service to customers and E [c_pw(u_t, u_s, w₁, v₁)] is the total expected cost of the dealer associated with repair services during the post-warranty period, which is given by Equation (16).

4. The Proposed Model and Solution Approach

4.1. The Customer–Dealer Stackelberg Game Model

In actual environments, it is necessary for dealers to consider customers’ attitudes and preferences about upgrading and warranty services when pricing remanufactured products. In this study, customers are given this option to choose the best upgrading strategy and warranty coverage to minimise their costs during the period of product use. Therefore, the decision variables for customers include: warranty coverage w₁, v₁ and upgrade level u = (u_t, u_s). The customer uses the product until the maximum age limit L or usage limit U is reached. The expected cost of customers during the product use is obtained by summing the expected selling price of the product in the region ${\Omega }_w$ and the expected price of post-warranty coverage ${\Omega }_{pw}$. Therefore, we have:

$$E\left[C_{customer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]=E\left[P\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)\right]+E\left[p_{pw}\left(u_t,u_s,w_1,v_1,{\delta }_{pw}\right)\right]$$

(18)

where E [P(u_t, u_s, w₁, v₁, δ_u,w)] and E [p_pw(u_t, u_s, w₁, v₁, δ_pw)] are given by Equations (12) and (17), respectively.

According to the decision of the customer, the dealer will maximise his/her expected profit margin considering the sales volume (demand) for the remanufactured product. We assume that product demand is a power function of its selling price and customers’ expected post-warranty expenses [29]. Therefore, the demand function can be modelled as follows:

$$\begin{gathered} d\left(E\left[P\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)\right],E\left[p_{pw}\left(u_t,u_s,w_1,v_1,{\delta }_{pw}\right)\right]\right)= \\ {k}_1{\left(E\left[P\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)\right]\right)}^{-\omega }\times {\left(E\left[p_{pw}\left(u_t,u_s,w_1,v_1,{\delta }_{pw}\right)\right]+k_2\right)}^{-v} \end{gathered}$$

(19)

where E [P(u_t, u_s, w₁, v₁, δ_u,w)] and E [p_pw(u_t, u_s, w₁, v₁, δ_pw)] are given by Equations (12) and (17), respectively; k₁ > 0 is a constant amplitude factor; k₂ > 0 is a constant for the post-warranty price, which allows for non-zero demand when there is no post-warranty coverage; $\omega >1$ is a constant parameter of the selling price elasticity; and $\mu >1$ is a constant parameter of the post-warranty price elasticity.

The dealer’s expected profit can be expressed in terms of the profit margin from offering the warranty and upgrading services and the post-warranty services. Thus:

$$\begin{gathered} E\left[Profi{t}_{dealer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]= \\ \left({\delta }_{u,w}\left(c_u\left(x,y\right)+E\left[c_w\left(u_t,u_s,w_1,v_1\right)\right]\right)+{\delta }_{pw}E\left[c_{pw}\left(u_t,u_s,w_1,v_1\right)\right]\right)\times \\ d\left(E\left[P\left(u_t,u_s,w_1,v_1,{\delta }_{u,w}\right)\right],E\left[p_{pw}\left(u_t,u_s,w_1,v_1,{\delta }_{pw}\right)\right]\right) \end{gathered}$$

(20)

where c_u(x,y), E[c_w(u_t, u_s, w₁, v₁)], E[c_pw(u_t, u_s, w₁, v₁)] and d(E[P(u_t, u_s, w₁, v₁, δ_u,w)], E[p_pw(u_t, u_s, w₁, v₁, δ_pw)]) are given by Equations (6), (10), (16) and (19), respectively.

Now, a Stackelberg game-theoretic decision-making model is presented to establish an equilibrium between the customer’s expected costs and the dealer’s expected profit. In this model, the customer is the leader and the dealer is the follower. The mathematical formulation of the model is as follows:

$$Leader: minE\left[C_{customer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]$$

(21)

$$Follower: maxE\left[Profi{t}_{dealer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]$$

(22)

$$\mathit{Subject}\mathit{to}: \begin{array}{c}{\delta }_{u,w}\ge 0 ; \\ {\delta }_{pw}\ge 0 ;\\ 0\le u_t\le x ;\\ 0\le u_s\le y ;\\ x-u_t\le w_1\le L ;\\ y-u_s\le v_1\le U\end{array}$$

(23)

where $E\left[{\mathrm{C}}_{customer}\left(u_t, u_s, w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]$ in Equation (21) is given by Equation (18), $E\left[{\mathrm{Profit}}_{dealer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]$ in Equation (22) is given by Equation (20), and Equation (23) represents the model constraints.

4.2. The Solution Approach

One efficient approach to solve non-linear bi-level programming problems is to replace the lower level problem with Karush–Kuhn–Tucker (KKT) optimality conditions. For further reading, the readers can refer to some useful references in the literature, e.g., [30]. The optimisation problem in Equations (21)–(23) can be solved by applying the method introduced in [31]. This method uses the KKT optimality conditions of the lower-level problem where the non-linear, bi-level programming problem is transformed into a corresponding single level programming. Therefore, the model formulation can be rewritten as follows:

$$min E\left[C_{customer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]$$

(24)

$$\begin{array}{l}\mathit{Subject}\mathit{to}:\\ \frac{\partial E\left[Profi{t}_{dealer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]}{\partial {\delta }_{u,w}}=-{\lambda }_1\end{array}$$

(25)

$$\frac{\partial E\left[Profi{t}_{dealer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]}{\partial {\delta }_{pw}}=-{\lambda }_2$$

(26)

$${\lambda }_1{\delta }_{u,w}=0$$

(27)

$${\lambda }_2{\delta }_{pw}=0$$

(28)

$${\lambda }_i\ge 0 , i=1,2$$

(29)

$$\begin{array}{c}{\delta }_{u,w}\ge 0 ; \\ {\delta }_{pw}\ge 0 ;\\ 0\le u_t\le x ;\\ 0\le u_s\le y ;\\ x-u_t\le w_1\le L ;\\ y-u_s\le v_1\le U\end{array}$$

(30)

where $E\left[C_{customer}\left(u_t,u_s,w_1,v_1,{\delta }_{u,w},{\delta }_{pw}\right)\right]$ in Equation (24) is given by Equation (18), ${\lambda }_{1 },{\lambda }_2\ge 0$ are dual variables, and the constraints in Equation (30) are same as the constraints presented in Equation (23).

5. Numerical Example

In this section, a numerical example is presented to illustrate the efficiency of the proposed model. Consider a dealer who sells remanufactured products of different ages with a FRW policy. Assume that the maximum life of the product is L = 10 years and U = 10 (×10⁵) miles, whichever comes first. The product is subject to random failures with the time to first failure following the Weibull distribution with $r\left(t,s\right)=\frac{{\beta }_3}{{{\alpha }_3}^{{\beta }_3}}\frac{{\beta }_4}{{{\alpha }_4}^{{\beta }_4}}{\left(t\right)}^{{\beta }_3-1}{\left(s\right)}^{{\beta }_4-1}$. The effectiveness of the upgrading process in terms of age and usage reduction, γ and τ respectively, are considered as two uniformly distributed random variables. The usage rate is assumed to be distributed according to a Gamma distribution with $g\left(r\right)=\frac{{\left(\lambda r\right)}^{\left(\rho -1\right)}\lambda exp\left(-\lambda r\right)}{\mathsf{\Gamma }\left(\rho \right)}$, where $\mathsf{\Gamma }\left(\rho \right)$ is the gamma function which is given by $\mathsf{\Gamma }\left(\rho \right)={{\displaystyle \int }}_0^{\infty }{z}^{\rho -1}{e}^{-z}dz$. We assume that u_t, u_s, w₁ and v₁ are decision variables to be determined such that the customer’s expected cost is minimised, and δ_u,w and δ_pw are decision variables to be determined such that the dealer’s expected profit is maximised.

Table 1. A summary of the model input parameter values.

Product’s Maximum Lifetime	L = 10 Years, U = 10 (×105) Miles
Sale price of a new product	p0 = 2500
Disposal or salvage value of a product	Csv = 100
Parameters of the Beta distribution	a1= x, a2 = 1/y, α1 = 1, α2 = 1, β1 = 1, β2 = 1
Parameters of the Weibull distribution	α3 = 1/0.345, α4 = 1/0.354, β3 = 2, β4 = 2
Parameters of the upgrade action cost	cs = 10, cu = 500, ζ = 0.2, ψ = 0.25, φ = 0.25
Parameters of the Uniform distribution	[0, 1]
Parameters of the Gamma function	λ = 1, ρ = 4
Parameters of the demand function	k1 = 1011, ω = 1.15, µ = 1.15, k2 = 0.01
Expected cost of a repair action at any point of time	cr = 100

presents a summary of the input parameter values used in the analysis.

Based on the values of the parameters reported in Table 1, the model was solved and the results were obtained for a product with an age of 1 year and a usage of 2.5 (×10⁵ km) miles. It was found that the minimum expected cost for customers during product use, i.e., E* (C_customer) = 3679, will be achieved when the customer chooses the upgrading strategy of (u_t* = 0.89, u*_s = 2.49) and warranty coverage of (w₁* = 2.14 years, v₁* = 4.06 × 10⁵ miles). In such a case, if the dealer chooses the percentage of the profit margin from offering the warranty coverage and upgrade services as δ_u,w* = 1.49, and from the post-warranty services as δ_pw* = 1.11, the demand for the remanufactured product will be estimated to be 3098, the optimal selling price of the product to be 1839, and the optimal expected profit of the dealer to be 4,956,658. The customer’s optimal strategy, the dealer’s optimal strategy, the upgrade cost, the expected number of repairs during the warranty period as well as the expected number of failures during the post-warranty period are all presented in

Table 2. Customer’s optimal strategy (u_t*, u_s*, w₁*, v₁*), dealer’s optimal strategy (δ_u,w*, δ_pw*), and their optimal objective functions for a product with past age of x = 1 and different past usages.

	x = 1
	y = 0.5	y = 1	y = 2	y = 2.5	y = 3	y = 3.5	y = 4
ut*	0	0.78	0.68	0.89	0.98	0.28	0.14
us*	0	0.03	1.93	2.49	2.96	3.49	3.89
w1*	0.80	2.86	1.90	2.14	2.18	1.38	0.95
v1*	9.47	8.15	6.06	4.06	3.17	3.00	2.10
δu,w*	4.05	2.08	1.38	1.49	1.40	1.87	0.83
δpw*	1.59	1.16	1.28	1.11	1.08	0.97	2.35
cp	1700	1300	900	785	700	633	580
cu(x,y)	10.03	178	393	417	418	303	250
E[Nw (ut*, us*, w1*, v1*)]	1.47	2.28	0.32	0.05	0.01	−0.02	−0.02
E[cw (ut*, us*, w1*, v1*)]	147	228	32	5.1	1.88	−2.40	−2.42
E[pu,w (ut*, us*, w1*, v1*, δu,w*)]	795	1257	1015	1053	1011	865	831
E[P(ut*, us*, w1*, v1*, δu,w*)]	2495	2557	1915	1839	1711	1498	1411
E[Npw (ut*, us*, w1*, v1*)]	9.63	11.8	8.39	8.71	8.40	7.60	7.67
E[cpw (ut*, us*, w1*, v1*)]	963	1183	839	871	840	760	767
E[ppw (ut*, us*, w1*, v1*, δpw*)]	2495	2557	1915	1839	1748	1498	1411
d(E[P(ut*, us*, w1*, v1*, δu,w*)], E[ppw (ut*, us*, w1*, v1*, δpw*)])	1537	1452	2822	3098	3568	4962	5695
E[Profitdealer (ut*, us*, w1*, v1*, δu,w*, δpw*)]	3,334,949	3,230,301	4,702,092	4,956,658	5,356,424	6,468,434	6,992,316
E[Ccustomer (ut*, us*, w1*, v1*, δu,w*, δpw*)]	4990	5114	3831	3679	3460	2997	2823

.

The following observations can be made from the results in Table 2:

(1)

As the past usage of the remanufactured product increases, the customer’s expected cost decreases and, as a result, demand for the product increases. This also results in an increase in the dealer’s expected profit. At lower usage levels, the rate of cost decrease and the rate of demand increase are lower than those at higher usage levels. This means that between two products having same age, customers will prefer the one with larger/longer past usage because it is less expensive. Moreover, the upgrading cost is lower compared to the purchasing price from an end user.

(2)

Considering the product’s reliability at the time of purchase, it is found that the reliability decreases with an increase in the product’s usage. Therefore, the purchasing price from an end user also decreases.

(3)

The upgrading cost increases with an increase in the upgrade level and decreases with an increase in the product’s reliability.

6. Conclusions

Recent technological advances, on one hand, have increased the lifespans of products and, on the other hand, have significantly contributed to the growth of remanufacturing markets in terms of sales value and volume. However, the remanufacturing market faces some challenges which need to be addressed. One of the most significant problems is that customers are usually sceptical about the quality and durability of remanufactured products due to a lack of information about the past life and/or maintenance history of the product in question. To provide peace of mind for customers, dealers carry out some upgrading actions on the most critical components, as well as offering a reasonable warranty on remanufactured products at the point of resale. Applying excessive upgrades to end-of-life products and/or offering long warranty periods on upgraded items—regardless of buyer affordability—may make the remanufacturing market less competitive, and eventually, will lead some of customers to shift to lower priced products. Therefore, to maximise sales volumes and profit margins, it is necessary for dealers to consider customers’ attitudes and preferences when deciding on an upgrading strategy, warranty coverage and sales price for remanufactured products.

In this paper, we proposed a Stackelberg game-theoretic decision-making model to manage the conflict of interests between customers and dealers in the remanufacturing markets. Our model established a win–win equilibrium between customers’ expected costs and the dealer’s expected profit for remanufactured products sold with a two-dimensional warranty and post-warranty service. The customers were considered to be the market leader, with the dealer acting as the follower. The purchasing price of a product from an end-user and the upgrade cost were modelled as functions of the product’s reliability. Demand for the product was also formulated as a nonlinear function of its selling price, as well as the expected servicing costs during the two-dimensional warranty period. Customers were given the option to decide on the best upgrading strategy and warranty coverage to minimise their expected cost during the period of product use. Consequently, the dealer attempted to maximise his/her expected profit via the upgrade strategy and warranty policy chosen by the customer.

The proposed model was tested on a numerical example to examine its effectiveness. The results revealed that incorporating customer preferences in terms of upgrading strategy, warranty policy and selling price into an integrated optimisation model would lower ownership costs for customers and, at the same time, increase sales volumes for remanufactured product as well as dealers’ profit margins. Additionally, the reliability of the remanufactured product was found to be an influencing factor in upgrading cost and expected warranty expenses.

The provided numerical example was generic, and therefore, can be applied to a wide range of applications. Nevertheless, to fully validate the results, the authors are working towards applying the models to a real-life system (remanufactured automobile engines). The results will be published elsewhere in the near future. Finally, a few assumptions were made which may not be suitable for certain applications, e.g., the usage rate was considered to remain constant for a given customer. Some of these assumptions will be reexamined in future work.