A Quality Decision Model Considering the Delay Effects in a Dual-Channel Supply Chain

Abstract

Quality is not only the basis for business survival and development but also a key issue that cannot be ignored in supply chain management decisions. In practice, the impact of quality on goodwill does not show an immediate effect, and there is a dynamic delayed effect. Therefore, we developed a dynamic model that considers the delayed effect of quality on goodwill. Firstly, we constructed a delayed differential equation for the effect of quality on goodwill based on the Nerlove–Arrow model for a two-channel supply chain in a competitive environment and studied the dynamic quality decision problem of manufacturers and retailers under the delay effect. Secondly, we constructed the manufacturer and retailer Hamilton functions based on the principles of being of great value, solving and comparing the optimal product quality level, having an optimal service quality level, product goodwill, and overall profit of the supply chain under both decentralized and centralized decision modes, and investigated the effect of delay time on the profit and quality decisions of supply chain members. The conclusions show that: (i) delay time is an important reference for supply chain members when choosing the decision mode, and the overall profit size of the supply chain has different relationships with the different values of delay time taken into account with the two decision scenarios. (ii) Adopting a centralized decision mode can motivate manufacturers and retailers to improve the quality level, which in turn promotes the sales of products and the accumulation of brand goodwill.

1. Introduction

In the twenty-first century, competition is no longer between companies, but between supply chains. Supply chain management promotes efficiency improvement and superiority at the enterprise level, but is it influenced by product quality at the micro level? The effective use of quality management can significantly affect the overall performance of the supply chain and the performance of a single enterprise, and quality has become one of the key indicators of supply chain operational performance as the competitive landscape of the supply chain evolves and reshapes [1,2,3]. Product quality, for example, indicates a country’s comparative advantage or a company’s productivity advantage, as well as determining the supply chain system’s current and future benefits. When consumer experience is integrated into quality management, the focus of supply chain management shifts and organizations should pay attention not just to product quality but also to service quality. Product quality is concerned with a product’s technical performance, whereas service quality is concerned with the customer experience. The supply chain should not only focus on meeting consumers’ basic functional needs (basic quality), but also encourage constant iteration and updating of products and services in order to meet consumers’ individualized needs (attractive quality) [4].

Supply chain product and service quality control is an important aspect of supply chain management and the embodiment of supply chain competitiveness [5]. The increasing complexity and incomplete information of the supply chain make quality management break through the boundary of individual enterprises and extend to all links of the supply chain, while quality problems in any upstream link will cause hidden product quality problems. For example, “The People’s Daily” hailed Three Squirrels as the new era of the “reform business card” due to frequent quality problems that have appeared, several times, to be in a product crisis, where complaints about moldy, spoiled products on social media were screened, for the reason being that upstream suppliers do not strictly enforce product quality control, production, or testing systems and standards. Additionally, the supply chain’s concealed difficulties are not just a problem for Three Squirrels; other companies employing the same OEM production model are experiencing significant product quality issues as well [6]. Especially in a highly competitive market, the final product quality of the supply chain depends not only on the product quality management of suppliers but also on the service quality control of retailers in the consumer market. For example, due to a quality testing issue with their vehicles gateway control module, FAW-Volkswagen had to recall 453,791 domestic Audi Q5L vehicles and 368 imported Audi SQ5s on 27 January 2022. This was the third recall, affecting around 470,000 Audi cars. Audi’s quality was inevitably questioned as a result of the frequent recalls. Audi China’s overall sales of 701,289 units declined 3.6 percent year on year in 2021, lagging behind Mercedes-Benz and BMW in terms of both total sales and growth [7]. Elsewhere, fierce external competition has put pressure on corporate quality management strategies and tools, and businesses have realized that long-term survival necessitates not only continuous quality improvement to satisfy their direct customers, but also leading the formation of new and effective competencies by stimulating new consumer demands in a changing global market environment. HEMA FRESH STORE is an exemplary company in quality control. It is committed to connecting upstream farmers and downstream customers with information technology, logistics, and shared data to develop an integrated fresh food supply chain, starting with a supply chain mindset. It has built a professional cross-functional supply chain operation team from the top-down and set accurate specific requirements for land, water, and transportation channels in minute details, considerably enriching commodities, ensuring food security, and maintaining freshness. For example, the products of “7 Fresh”, a key brand of HEMA FRESH STORE, are sourced directly from the production base by the team and delivered to customers with a full cold chain. Overall, the key to its success is its expertise in the supply chain, value chain management, and an integrated supply chain management system to ensure quality and safety [8].

Product quality and service quality are often identified as intrinsic indicators that affect a company, while the outward indicator that affects a company’s survival and growth is corporate goodwill [9]. Goodwill is the external expression of a company’s capability, that primarily refers to the market’s comprehensive assessment of the firm’s long-term commercial performance ability, as well as the centralized embodiment of the firm’s core competitiveness, with the important value of lowering market transaction risks and costs [10,11]. The most important source of goodwill is the company’s capacity to meet consumer needs with product functionalities, quality product services, and marketable product designs, as well as the market’s opinion or contentment with the company. Quality does not happen overnight but requires continuous quality improvement and management based on the response of the demand market. Quality improvement is also becoming a powerful competitive tool in the marketplace, with manufacturers and retailers actively pursuing different quality strategies to seek better cooperation on quality and gain a greater market viability; however, quality improvement is not immediate, and there is a delayed effect of quality on goodwill. This is because, in the process of transferring business flow, logistics and info, ration flow in the supply chain, product and service information feedback is evaluated and promoted only after consumers have purchased and experienced the products, so there is a certain time lag between the evaluation of quality improvement and the reflection of goodwill.

With the maturation of e-commerce technology, an increasing number of suppliers are launching electronic direct sales channels based on existing merchant channels. Faced with the threat of suppliers’ direct sales channels invading traditional offline markets, retailers have begun to provide services that online stores cannot (such as a shopping environment, touchable products, the sustainability of enthusiastic shopping guide services, and so on), eventually forming a unique type of multi-channel supply chain model called the dual-channel supply chain model. A large number of scholars have also emphasized the importance of dual-channel supply chain models to supply chain management and the impact of dual-channel supply chain models on supply chain members’ profitability. This paper uses the competitive dual-channel supply chain as the research object to get closer to the actual dynamic development of the supply chain. It also considers the importance of studying the decision problem of dynamic product quality level and service quality level in the dual-channel supply chain scenario under the delay effect.

The main goals of this paper’s research are to locate product quality and service quality in the same framework from a dynamic perspective, and to investigate the decision problems of product quality and service quality levels in a dual-channel supply chain, in order to assist supply chain or business managers in adjusting their strategies flexibly under various scenarios. Firstly, the delayed differential equations representing the effects of product and service quality on goodwill are established using the Nerlove–Arrow model. Secondly, the Hamilton functions of manufacturers and retailers are constructed based on the principle of great value to identify optimal decisions for the product quality level, service quality level, and service quality cost-sharing rate under different decision-making situations, and to investigate the conditions for adopting various approaches. Finally, numerical analysis is used to examine the impact of delay time on supply chain profit, product goodwill, product quality level, and service quality level.

The following is the scope of this paper’s work. Section 2 contains a survey of the literature on pertinent research areas. The basic assumptions and notational descriptions are introduced in Section 3, Section 4 and Section 5 and build decentralized and centralized decision models for dual-channel supply chains, respectively, to solve for the manufacturer’s ideal product quality level, the retailer’s optimal service quality level, and the product’s optimal goodwill. The numerical analysis presented in Section 6 is used to verify the prior theoretical results. The three conclusions reached throughout the paper are listed and some recommendations for deeper research and expansion is presented in Section 7.

2. Literature Review

In conjunction with the research in this paper, this section deals with the literature on dual-channel supply chains, delay effects, and quality.

2.1. Dual-Channel Supply Chain Study

Scholars have paid close attention to the dual-channel supply chain issue as more stores and even manufacturers implement it. Zhou et al. (2019) [12] used a two-channel supply chain screening model to investigate the corresponding pricing decisions of dominant suppliers and retailers involved in asymmetric operational information, concluding that suppliers can earn greater profits without paying information rents under certain conditions. In addition, Peng (2019) [13] suggested that the simultaneous implementation of the store channel and online channel can bring more convenience or lower shipping costs for customers, further bringing channel synergies and providing customer satisfaction. As a result, in order to manage and maximize the advantages of both online and store channels, many dual-channel retailers, such as Walmart, Suning Appliance, Uniqlo, and 7 Eleven, have opened up the BOPS channel [14,15]. Many scholars have viewed the dual-channel supply chain as a special case of the multi-channel approach to study the pricing decisions, quality decisions, and coordination method decisions of a supply chain with a dual-channel supply chain. Tang and Yang (2020) [16] suggested that suppliers have found that adding new channels enhances corporate profits, and many move down the value chain to sell products directly to consumers through online channels in the context of the widespread popularity of e-commerce platforms. Pei and Swimberghe (2021) [17] studied the dual-channel supply chain coordination approach and proposed that this approach, in which manufacturers combine rebates and volume discounts from offline consumers, helps to mitigate O2O channel competition and increase the profitability of all parties in the supply chain. Dai (2022) [18] investigated the impact of information sharing scenarios on strategy formulation (wholesale price and product quantity) and the agility effects of dual-channel supply chain members based on a predictive decision model. Li and Mizuno (2022) [19] developed a decision model using stochastic dynamic programming to study three possible power structures between manufacturers and retailers in a dual-channel supply chain, with the aim that firms can maximize total expected discount profits within the plan by adjusting pricing and inventory decisions in a timely manner. From this, it can be seen that more scholars have taken a dual-channel structure as the research object to establish the supply chain model, and also clarify the impact of a dual-channel structure in the profit enhancement of the supply chain. In order to be closer to the actual dynamic development of the supply chain, this paper also takes the competitive dual-channel supply chain as the research object.

2.2. Research on Supply Chain Quality Issues

Interestingly, the development of supply chain management stems from this root of quality management [20], and as a result, there is more academic attention placed on the issue of supply chain quality.

Some scholars have used game theory models, equilibrium decision models, and other perspectives to study quality decisions. Prasenjit and Tarun (2020) [21] characterized suppliers’ component quality decisions based on a programmatic game-theoretic model to explore quality decisions under product differentiation and information asymmetry. Fan et al. (2020) [22] studied the cost-of-quality decision problem in a two-tier supply chain, in which upstream manufacturers and downstream retailers shared the product liability costs caused by quality defects, and showed that the expected share of liability costs can have an impact on product quality, pricing decisions, and on the profitability of the supply chain members and the entire supply chain. More importantly, recent works have proposed novel approaches for modeling product quality. For example, Li and Mishra (2021) [23] took the perspective of the relationship between suppliers and users in the product and service supply chain and analyzed the impact of product obsolescence on downstream after-sales service contracts. Yang et al. (2021) [24] studied manufacturers who provide after-sales services and e-retailers who share demand information and examined manufacturers’ optimal decisions on sales models and service levels, as well as the retailers’ demand for information-sharing strategies. Qiu et al. (2022) [25] investigated the dynamic decision problem in a two-tier decentralized supply chain consisting of suppliers and retailers. The results showed that consumers’ initial reference quality, reference quality effect strength and memory parameters are important variables influencing the decision, and retailers should attract potential consumers to purchase products offline with dynamic reference quality effects.

Among these, under the research object of the dual-channel supply chain, supply chain quality issues are mainly divided into product quality issues (manufacturers) and service quality issues (retailers).

On the one hand, product quality has been used as one of the key indicators of supply chain operational performance, and some scholars have studied the issue of the product quality level in supply chains. Sarkar et al. (2020) [26] discussed a three-tier supply chain model among the cooperative participants. The cooperation between the three participants increased the total profit under the cooperative advertising system to improve the quality of the product to be sold. Ullah and Sarkar (2020) [27] proposed that every product has a fixed lifetime characteristic and that no company would not care about the relationship between the product and a supply chain cost reduction. Ma et al. (2020) [28] incorporated static reference quality effects into a pricing model for remanufactured products to investigate the impact of different quality reference standards on supply chain decisions. Zhou et al. (2020) [29] developed a dynamic model including product quality reference effects and service quality reference effects, and used differential game theory to obtain optimal decisions for product quality and service quality decisions under different decision scenarios. Lejarza and Baldea (2020) [30] built on an existing quality decay model with an inventory production and distribution planning framework to introduce a rolling horizon scheme to consider the impact of environmental conditions across the supply chain on product quality. Immediately after, Lejarza and Baldea (2022) [31] developed a computationally efficient optimization framework for the perishable product supply chain to help supply chain members control multiple quality attributes of their products in their production and distribution plans to meet stricter safety regulations, evolving consumer quality requirements, and the need for more competitive markets.

On the other hand, particular emphasis is placed on the fact that the products we study are mainly general physical products; however, how to effectively transfer products to consumers also requires studying the quality of services that match these products, product services that include after-sales services, value-added services, extended warranty services, and other services (e.g., warranty services, experience services, etc.) [32]. Jamkhaneh et al. (2022) [33] suggested that service quality is an important tool for service firms to improve their reputation and improving the quality of logistics services facilitates the improvidence of logistics service quality helps firms to minimize transaction costs when searching for company information and alternatives in competition. Meanwhile, Lin and Wang (2022) [34] studied the influence of user feedback, service duration, and service quality level on service provider decision making. Li et al. (2021) [35] considered the interest relationship between retailers, service providers, and manufacturers in the supply chain, and analyzed not only the way supply chain members make product and service decisions, but also explored the vertical channel integration strategies of retailers under different business models. Some scholars have also studied the service quality problem from different modeling ideas. Malik and Kim (2020) [36] developed a model of the relationship between process quality and productivity, to find the optimal solution for the decision variables of order quantity, delivery time, and minimum cost, by considering the storage space and budget constraints of the supplier and the customer service level constraints of the buyer. Nobil et al. (2020) [37] and Malik and Sarkar (2020) [38] proposed a corresponding quality management model by considering practical constraints such as the production cycle time and the available budget for storage space. Afsahi and Shafiee (2020) [39] studied the basic service decision problem of a manufacturer by the stochastic optimization approach and analyzed the manufacturer’s service delivery strategy. Huang et al. (2021) [40] considered the service decision problem between two manufacturers and two service providers facing product competition, while Kuppusamy et al. (2021) [41] considered the product and service decision problem in the electric vehicle supply chain and investigated the supplier’s selection strategy for buyers and the supplier’s pricing decision for infrastructure services. Liu et al. (2021) [42] considered the product pricing and service level decision with an oligopolistic manufacturer (providing value-added services through an e-platform) and an e-platform operator.

Collectively, there are two main components of supply chain quality decisions, which are divided into product quality decisions and service quality decisions. Most scholars focus on product quality or service quality as the decision variables to study supply chain quality issues, and only a few scholars (e.g., Li et al. (2021) [35], Kuppusamy et al. (2021) [41]., Liu et al. (2021) [42]) have studied both product and service in the same framework, and more importantly, their studies are based on a static perspective and do not consider the dynamic nature of supply chain decision making.

2.3. Study on Delay Effects

Product quality and service quality both play a crucial role in improving organizational performance and competitiveness in the dual-channel supply chain. Product quality appears to be the foundation for an enterprise’s existence and growth, whereas service quality can efficiently deliver products to people; however, there is a time lag between product and service quality feedback and goodwill. As a result, from the dynamic perspective of the delay effect, it is more realistic to explore the supply chain quality decision problem [43]. There are a large number of scholars who have introduced the delay effect into the field of supply chain management for research. Chen et al. (2020) [44] proposed an equilibrium model for analyzing automotive supply chain networks under uncertain payment delays, and the study demonstrated that the supply chain networks make decisions on transaction volume, price, profit allocation, and payment delay strategies under a dynamic scenario of payment delays. Gago-Rodríguez et al. (2021) [45] considered the extent to which asymmetry in the bargaining power among supply chain members moderates the impact of set-up delay costs on negotiation outcomes. Zhang and Chen (2022) [46] considered the application of the delay effect in the field of supply chain finance, and studied the optimal operational and financing portfolio strategies for capital-constrained, closed-loop supply chains under fully and partially delayed payment scenarios.

In addition, based on the dynamic nature of the delay effect, many scholars have constructed various models of supply chain dynamics. Chen et al. (2017) [47] and Yu et al. (2018) [48] introduced the advertising delay effect into supply chain decision making and established a dynamic model of brand reputation with time delay and a model of product sales volume considering the brand reputation to study the dynamic cooperative advertising problem considering the delay effect. Guo et al. (2019) [49], from the perspective of supply chain product marketing, considered the impact on product goodwill when both the manufacturer and retail marketing efforts had delay effects, and constructed a dynamic differential model based on double delay effects. Ahmed et al. (2021) [50] developed a synergic inventory model that enhances inventory management performance by considering the cycle time of monitoring the supply chain and the fraction of stages with an optimistic inventory. Sawik (2022) [51] considered a multi-portfolio approach and a scenario-based stochastic MIP (mixed integer planning) model to study delayed regional disruption dilemmas in supply chains under duration.

See

Table 1. Comparison of recent relevant literature research points.

Literature	Publish Time	Product Quality as a Decision Variable	Service Quality as a Decision Variable	Using Differential Gaming Methods	Consider the Delay Effect
Chen et al. [47]	2017	no	no	yes	yes
Huang et al. [48]	2018	yes	yes	no	no
Guo et al. [49]	2019	no	no	yes	yes
Hu [43]	2019	no	no	yes	yes
Malik and Kim [36]	2020	yes	yes	no	no
Ahmed et al. [50]	2021	no	no	yes	yes
Li and Mishra [23]	2021	yes	yes	no	no
Prasenjit and Tarun [21]	2021	yes	no	yes	no
Kuppusamy [41]	2021	yes	yes	yes	no
Qiu et al. [25]	2022	yes	no	yes	no
Lin and Wang [34]	2022	no	yes	no	yes
This paper	2022	yes	yes	yes	yes

for a summary of the main research problems raised in the current relevant research literature. The existing study on supply chain quality issues has the following weaknesses, as evidenced by the literature review: firstly, while the topic of supply chain product quality and service quality plan has piqued the interest of many academics, the combination of product quality and service quality strategy has been researched from the perspective of the supply chain. There is not a lot of literature out there. Secondly, although some scholars have considered the problem of equilibrium strategies for product and service supply chains, this part of the research is mostly set up in a static framework, and the dynamic characteristics of supply chains have not been considered and there is less literature available. From the perspective of dynamics, it is obvious that constructing models and methods from a static architecture is not enough, and it is difficult to provide appropriate decision advice for supply chain quality management. Thirdly, there is less literature on the related research on the delayed utility of quality on goodwill, and the current research on the delay effect is mostly applied to the decision problems of the supply chain such as transaction volume, price, financing strategy, profit allocation and a payment delay strategy, and no research has yet considered the delay effect between the evaluation of quality improvement on goodwill reflection in the dual-channel supply chain.

Given this, based on the above literature, this paper further innovates to expand the scope of the study. First, the delayed effect of product quality and service quality on the impact of goodwill in a dual-channel supply chain is considered. Second, based on the Nerlove–Arrow model, we constructed delayed differential equations for the impact of quality on goodwill from a dynamic perspective, and studied the dynamic product quality level and service quality level issues of delayed effects. Third, the optimal decisions of goodwill, product quality level, and service quality level for maximizing supply chain profit was investigated under decentralized decision making and centralized decision making, respectively.

3. Model Development

“Go to the physical stores set up by Suning and Jing-dong to experience the products and place orders at the corresponding online channels” has become the shopping norm in the consumer market, and the rapid development of electronic information technology, with differences in online and offline channels between price, service and other new competitive factors, is resulting in competition in the supply chain in the original simple upstream and downstream model with an added horizontal competition between channels and coordination. The National Sporting Goods Association (NSGA) has called on upstream suppliers to not use a dual-channel sales model to avoid channel conflicts between manufacturers’ online channels and their retailers’ physical channels. In the environment of a dual-channel structure, although it has caused a serious squeeze on the channel demand of some distributors and eaten into the fundamental interests of traditional channel traders, based on literature combing, it can be seen that a large number of scholars believe that under reasonable pricing decisions, benefit contracts and information symmetry, the benefits brought by dual-channel structure can offset the disadvantages brought to downstream retailers.

In addition, from the perspective of model construction, it has a clearer logic to study the dynamic quality decision problem of each member of the supply chain in a dual-channel environment, thus, we took a dual-channel supply chain as the research object and considered the existence of a supply chain system consisting mainly of manufacturers and retailers, where the manufacturers can sell their products through dual channels (online and offline), with the online channel referring to the manufacturer’s electronic direct sales channel and the offline channel being where the manufacturer sells their products to retailers and relies on the traditional channels of independent retailers to complete product sales. Additionally, this paper further designs the relevant symbols and parameters, as shown in

Table 2. Relevant symbols and parameters.

Symbol	Description	Symbol	Description
$z(t)$	The manufacturer’s product quality level at time t, which is the decision variable.	${\rho }_{MF}$	Marginal profit of offline channels for manufacturers.
$f(t)$	The service quality level of retailers at time t, which is a decision variable.	${\rho }_R$	Marginal profit for retailers.
$\varphi (t)$	The proportion of the manufacturer bearing the retailer’s service quality cost at time t, which is a decision variable.	$d(t)$	The market demand at time t.
$G(t)$	The goodwill of the product at the time t.	$a_i$	The potential sales volume in the market before the improvement of product quality and service quality, $a_i>0$. $i=1$ indicates offline channel and $i=2$ indicates online channel.
$d_z$	Delay in manufacturer’s improvement of product quality, which in turn affects product goodwill.	$b$	The sensitivity coefficient of product goodwill to product market demand, $b>0$.
$d_s$	Retailers improve service quality, which in turn affects the delay of product goodwill.	${\theta }_1$	The sensitivity coefficient of the retailer’s service quality level to the product market demand, ${\theta }_1>0$.
$\gamma$	The extent to which improvements in product quality affect the rate of change in goodwill.	${\theta }_2$	The sensitivity coefficient of the manufacturer’s product quality level to the product market demand, ${\theta }_2>0$.
$\beta$	The extent to which improvements in service quality affect the rate of change in goodwill.	$k_1$	The manufacturer’s quality cost coefficient, $k_1>0$.
$\lambda$	Discount rate.	$k_2$	The retailer’s quality cost coefficient, $k_2>0$.
${\rho }_{MO}$	Marginal profit of online channels for manufacturers.	$\delta$	The decay rate of product goodwill, $\delta >0$.

.

In the dual-channel supply chain system, product quality $z(t)$ and the proportion of service quality costs borne by the manufacturer to the retailer $\varphi (t)$ are the decision variables of the manufacturer, while service quality level $f(t)$ is the decision variable of the retailer. Among them, both the manufacturer’s product quality decision and the retailer’s service quality decision have two paths of action on market demand: first, product quality (service quality) directly affects market demand, and second, product quality (service quality) indirectly affects market demand through the dynamic delayed effect on the firm’s goodwill. The proportion of the manufacturer’s share of the retailer’s service quality costs affects the market demand directly or indirectly mainly through the retailer’s service quality level. As shown in Figure 1.

Further, this paper proposes four hypotheses:

Hypothesis 1.

There is a supply chain system with a manufacturer and a retailer. The manufacturer has two sales channels: online through an e-commerce platform or offline through a retailer. Both online and offline sales are selling the same type of product (same quality, size, color, etc.); however, product service varies by channel, and we assume that online product service is 0 compared to offline. This is because offline service is mainly reflected in three aspects compared to online: first, the image and touch-ability of the products displayed offline, second, consumers who buy offline can enjoy the hospitality and professional explanation of the service staff, and third, the physical environment offline is more friendly and experiential.

Hypothesis 2.

Manufacturers’ product quality level and retailers’ service quality level jointly positively affect product goodwill. Based on Nerlove and Arrow [52], a delay differential equation of quality level affecting product goodwill is constructed:

$$\{\begin{array}{l}\dot{G}(t)=\gamma z(t-d_z)+\beta f(t-d_s)-\delta G(t)\\ G(0)=G_0\end{array}$$

(1)

Hypothesis 3.

Based on the above analysis, traditional and online channel demand functions are constructed by combining the literature [53]. Traditional channel demand ($d_t$) was influenced by product quality, goodwill, and service quality. Online channel demand ($d_e$) was influenced by product goodwill, and product quality. Their functions are as follows:

$$\{\begin{array}{l}{d}_t(t)=a_1+bG(t)+{\theta }_{2}z(t)+{\theta }_{1}f(t)\\ d_e(t)=a_2+bG(t)+{\theta }_{2}z(t)\end{array}$$

(2)

Hypothesis 4.

The quality improvement cost is an increasing function of the quality level, and the second derivative is greater than 0, that is: $c_m^{″}(z)>0$,$c_r^{″}(f)>0$, and the quality improvement cost functions of manufacturers and retailers are as shown in Equation (3):

$$\{\begin{array}{l}{c}_m=\frac{1}{2}{k}_2{z}^2(t)\\ c_r=\frac{1}{2}{k}_1{f}^2(t)\end{array}$$

(3)

This paper also assumes that the supply chain members make their decisions with their respective profit optimality within an infinite time horizon. In summary, the net discounted profit function for the manufacturer, the retailer, and the entire supply chain can be obtained as:

$$J_M={\displaystyle \underset{0}{\overset{\infty }{\int }}{e}^{-\lambda t}\{(d_e(t){\rho }_{MO}+d_t{\rho }_{MF})-\frac{1}{2}{k}_2{z}^2(t)-\frac{1}{2}\varphi k_1{f}^2(t)\}}dt$$

(4)

$$J_R={\displaystyle \underset{0}{\overset{\infty }{\int }}{e}^{-\lambda t}\{{\rho }_R{d}_t(t)-\frac{1}{2}(1-\varphi )k_1{f}^2(t)\}}dt$$

(5)

$$J_{MR}={\displaystyle \underset{0}{\overset{\infty }{\int }}{e}^{-\lambda t}\{(d_t(t)p_R+d_e(t){\rho }_{MO}+d_t(t){\rho }_{MF})-\frac{1}{2}{k}_2{z}^2(t)-\frac{1}{2}{k}_1{f}^2(t)\}}dt$$

(6)

4. Decentralized Decision Making

As independent individuals, manufacturers and retailers make decisions on product quality level and service quality level, respectively, based on the principle of their own best interests. At the same time, to expand the market scale, manufacturers encourage retailers to improve their service level and promise to share a certain proportion of the cost-of-service quality improvement.

Proposition 1.

In the decentralized decision scenario, the manufacturer’s optimal product quality level and the retailer’s optimal service quality level are:

$$z^{\ast }(t)=\frac{{\theta }_2({\rho }_{MF}+{\rho }_{MO})}{k_2}+\frac{({\rho }_{MF}+{\rho }_{MO})b\gamma }{k_2(\lambda +\delta )}{e}^{\delta d_z}$$

(7)

$$f^{\ast }(t)=\frac{{\theta }_1{\rho }_R}{(1-\varphi )k_1}+\frac{{\rho }_{R}b\beta }{(1-\varphi )k_1(\lambda +\delta )}{e}^{\delta d_s}$$

(8)

The optimal goodwill of the product is:

$$G^{\ast }(t)=\frac{(1-e^{-\delta t})}{\delta }\left\{\gamma [\frac{{\theta }_2({\rho }_{MF}+{\rho }_{MO})}{k_2}+\frac{({\rho }_{MF}+{\rho }_{MO})b\gamma }{k_2(\lambda +\delta )}{e}^{\delta d_z}]+\beta [\frac{{\theta }_1{\rho }_R}{(1-\varphi )k_1}+\frac{{\rho }_{R}b\beta }{(1-\varphi )k_1(\lambda +\delta )}{e}^{\delta d_s}]\right\}+G_0{e}^{-\delta t}$$

(9)

The optimal proportion for manufacturers to share the service quality cost of retailers is:

When ${\theta }_1{\rho }_{MF}+({\rho }_{MO}+{\rho }_{MF})\frac{b\beta }{\delta +\lambda }>\frac{1}{2}[{\theta }_1{\rho }_R+\frac{{\rho }_{R}b\beta }{(\lambda +\delta )}{e}^{\delta d_s}]$ the manufacturer’s share of the retailer’s cost of service quality is:

$$\varphi =\frac{{\theta }_1{\rho }_{MF}+({\rho }_{MO}+{\rho }_{MF})\frac{b\beta }{\delta +\lambda }-\frac{1}{2}[{\theta }_1{\rho }_R+\frac{{\rho }_{R}b\beta }{(\lambda +\delta )}{e}^{\delta d_s}]}{{\theta }_1{\rho }_{MF}+({\rho }_{MO}+{\rho }_{MF})\frac{b\beta }{\delta +\lambda }+\frac{1}{2}[{\theta }_1{\rho }_R+\frac{{\rho }_{R}b\beta }{(\lambda +\delta )}{e}^{\delta d_s}]}$$

(10)

When ${\theta }_1{\rho }_{MF}+({\rho }_{MO}+{\rho }_{MF})\frac{b\beta }{\delta +\lambda }\le \frac{1}{2}[{\theta }_1{\rho }_R+\frac{{\rho }_{R}b\beta }{(\lambda +\delta )}{e}^{\delta d_s}]$ the manufacturer’s share of the retailer’s cost of service quality is zero, it is denoted as $\varphi =0$.

Proof (see Appendix A for the reasoning and proof process).

According to Proposition 1, when there is service cooperation, the optimal profits of the manufacturers and retailers are:

$$\{\begin{array}{l}{J}_M=\frac{1}{\lambda }[(a_2+{\theta }_2{z}^{\ast }(t)){\rho }_{MO}+(a_1+{\theta }_2{z}^{\ast }(t)+{\theta }_1{f}^{\ast }(t)){\rho }_{MF}]-\frac{1}{2}{k}_2{z}^{\ast }{}^2(t)-\frac{1}{2}\varphi k_1{f}^{\ast }{}^2(t)\hspace{0.17em}+(b{\rho }_{MO}+b{\rho }_{MF})\frac{\gamma z^{\ast }(t)+\beta f^{\ast }(t)}{\lambda \delta }\\ +(b{\rho }_{MO}+b{\rho }_{MF})(G_0-\frac{\gamma z^{\ast }(t)+\beta f^{\ast }(t)}{\delta })\frac{1}{\delta +\lambda }\\ J_R=\frac{1}{\lambda }[p_R(a_1+{\theta }_2{z}^{\ast }(t)+{\theta }_1{f}^{\ast }(t))-\frac{1}{2}(1-\varphi )k_1{f}^{\ast }{}^2(t)]+{\rho }_{R}b\frac{\gamma z^{\ast }(t)+\beta f^{\ast }(t)}{\delta \lambda }+\frac{{\rho }_{R}b}{\delta +\lambda }(G_0-\frac{\gamma z^{\ast }(t)+\beta f^{\ast }(t)}{\delta })\end{array}$$

(11)

Inference 1.

Four laws can be inferred from decentralized decision making: (1) the greater the marginal profit (${\rho }_R$, ${\rho }_{MO}$ and ${\rho }_{MF}$) the higher the level of product quality offered by the producer and the level of service quality provided by the retailer, but at the cost of higher quality improvement costs. (2) When the marginal profit ratio of the supplier and the demander meets ${\theta }_1{\rho }_{MF}+b({\rho }_{MO}+{\rho }_{MF})\frac{\beta }{\delta +\lambda }>\frac{1}{2}[{\theta }_1{\rho }_R+\frac{{\rho }_{R}b\beta }{(\lambda +\delta )}{e}^{\delta d_s}]$, the manufacturer is willing to bear the cost of service quality improvement, and the proportion is positively correlated with the level of service quality. (3) The service quality improvement sharing ratio is positively associated with the manufacturer’s marginal profit, while the service quality improvement sharing ratio is adversely related to the retailer’s marginal profit. (4) When the delay time of retailers’ service quality improvement affects goodwill increases, manufacturers will reduce the sharing proportion of service improvement.

Inference 1 shows that the size of the marginal profit has a great impact on the quality improvement decision-making of the enterprise. If the marginal profit is greater, the enterprise has a higher enthusiasm to improve the quality level. The larger the manufacturer’s marginal profit, the more actively it will support retailers to improve the service quality level. On the contrary, if the marginal profit is smaller, this will reduce the support of the service quality level. Manufacturers bear part of the cost-of-service quality improvement, which reduces the economic burden of downstream retailers to a certain extent and encourages them to improve service quality. A longer delay time for retailers to improve service quality and affect goodwill, will produce more improvement costs and bring more economic returns, which will reduce the enthusiasm of manufacturers to undertake service quality improvement to a certain extent.

Inference 2.

Under decentralized decision-making, the discount rate$\lambda$is negatively correlated with the manufacturer’s product quality level and the retailer’s service quality level, respectively, and the attenuation rate$\delta$is positively correlated with the manufacturer’s product quality level and the retailer’s service quality level (meeting the conditions:$\lambda +\delta >\frac{1}{d_s}$,$\lambda +\delta >\frac{1}{d_z}$, respectively).

Inference 3.

Under decentralized decision-making, if the delay time is longer, the corresponding manufacturer’s optimal product quality level and retailer’s optimal service quality level will be higher. The manufacturer’s optimal profit function decreases as product quality level delay time increases but increases as service quality level delay time increases; on the other hand, the retailer’s optimal profit function decreases as the service quality level delay time increases, but increases as product quality level delay time increases.

Inference 3 shows that the longer the time it takes for the level of product quality to affect goodwill, the more improvements the manufacturer will make in product development, technology introduction, and other areas, raising the company’s costs, despite an increased market demand, lowering relative profits. Meanwhile, product quality influences market demand, and improving product quality helps the merchant raise sales revenue, hence increasing profits; however, despite the ability to raise the level of service quality, a longer delay in the level of service quality impacting goodwill, increases the cost burden and reduces the profit earned by the retailer owing to the delay effect.

5. Centralized Decision-Making

Under centralized decision-making, manufacturers and retailers are regarded as two departments in an enterprise, which maximize the profits of the supply chain by designing the optimal product quality $z(t)$ and service quality $f(t)$.

Proposition 2.

Under centralized decision-making, the manufacturer’s optimal product quality level and the retailer’s optimal service quality level are:

$$\{\begin{array}{l}{z}^{\ast \ast }(t)=\frac{{\theta }_2({\rho }_R+{\rho }_{MF}+{\rho }_{MO})}{k_2}+\frac{b({\rho }_R+{\rho }_{MF}+{\rho }_{MO})\gamma }{k_2(\lambda +\delta )}{e}^{\delta d_z}\\ f^{\ast }{}^{\ast }(t)=\frac{{\theta }_1({\rho }_R+{\rho }_{MF})}{k_1}+\frac{b({\rho }_R+{\rho }_{MF}+{\rho }_{MO})\beta e^{\delta d_s}}{k_1(\lambda +\delta )}\end{array}$$

(12)

The optimal goodwill of the product is:

$$G^{\ast }{}^{\ast }(t)=\frac{(1-e^{-\delta t})}{\delta }\left\{\begin{array}{l}\gamma [\frac{{\theta }_2({\rho }_R+{\rho }_{MF}+{\rho }_{MO})}{k_2}+\frac{b({\rho }_R+{\rho }_{MF}+{\rho }_{MO})\gamma }{k_2(\lambda +\delta )}{e}^{\delta d_z}]\\ +\beta [\frac{{\theta }_1({\rho }_R+{\rho }_{MF})}{k_1}+\frac{b({\rho }_R+{\rho }_{MF}+{\rho }_{MO})\beta e^{\delta d_s}}{k_1(\lambda +\delta )}]\end{array}\right\}+G_0{e}^{-\delta t}$$

(13)

Proof (see Appendix B for the reasoning and proof process).

From Proposition 2. the optimal profit of the whole supply chain can be obtained as:

$$\begin{array}{l}{J}_{MR}=\frac{1}{\lambda }[(a_1+{\theta }_2{z}^{\ast \ast }(t)+{\theta }_1{f}^{\ast \ast }(t))({\rho }_R+{\rho }_{MF})+(a_2+{\theta }_2{z}^{\ast \ast }(t)){\rho }_{MO}-\frac{1}{2}{k}_2{z}^{\ast \ast }{}^2(t)-\frac{1}{2}{k}_1{f}^{\ast \ast }{}^2(t)]\\ \hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}+b({\rho }_{MO}+{\rho }_R+{\rho }_{MF})\{\frac{1}{\lambda \delta }(\gamma z^{\ast \ast }(t)+\beta f^{\ast \ast }(t))+\frac{1}{\lambda +\delta }[G_0-(\gamma z^{\ast \ast }(t)+\beta f^{\ast \ast }(t))\frac{1}{\delta }]\}\end{array}$$

(14)

Proposition 3.

The manufacturer’s optimal product quality level, retailer’s optimal service quality level, product optimal goodwill, and market demand under centralized decision-making are greater than the corresponding values under decentralized decision-making, namely,$z^{\ast \ast }(t)>z^{\ast }(t)$,$f^{\ast \ast }(t)>f^{\ast }(t)$,$G^{\ast \ast }(t)>G^{\ast }(t)$and$D^{\ast \ast }(t)>D^{\ast }(t)$.

Proof (see Appendix C for the reasoning and proof process).

Proposition 3 shows that the centralized decision-making model of the supply chain can encourage manufacturers and retailers to improve the quality level, and then promote the sales of products and the accumulation of brand goodwill.

Proposition 4:

(1) 

In the case of a delay time satisfaction relationship$d_s\in RS1$, then$d_z\ge 0$, the overall profit of the supply chain under centralized decision-making is not greater than that under decentralized decision-making, and decentralized decision-making is the optimal decision-making mode of the supply chain.

(2) 

When the delay time satisfies the relationship$d_s\in RS2$, if$f_{10}\le f_{11}$, then$d_z\in RZ1$; alternatively, when the delay time satisfies the relationship$d_s\in RS2$, if$f_{11}<f_{10}<f_{12}$, then$d_z\in RZ3$; in the case of the delay time satisfaction relationship$d_s\in RS2$, if$f_{12}\le f_{10}$, then$d_z>0$, it can be observed that the overall profit of the supply chain under decentralized decision-making is not less than that under centralized decision-making, and the supply chain should adopt decentralized decision-making mode.

(3) 

When the delay time satisfies the relationship$d_s\in RS2$, if$f_{10}\le f_{11}$, then$d_z\in RZ2$; alternatively, when the delay time satisfies the relationship$d_s\in RS2$, if$f_{11}<f_{10}<f_{12}$, then$d_z\in RZ4$, the overall profit of the supply chain under decentralized decision-making is less than that under centralized decision-making, and the supply chain should adopt a centralized decision-making mode.

(4) 

In the case of delay time satisfaction relationship$d_s\in RS3$, if$f_{10}\le f_{12}$, then$d_z\in RZ5$, the overall profit of the supply chain under decentralized decision-making is not greater than that under centralized decision-making, and the supply chain should adopt a centralized decision-making mode.

(5) 

In the case of delay time satisfaction$d_s\in RS3$, if$f_{10}\le f_{12}$, then$d_z\in RZ6$, or in the case of delay time satisfaction$d_s\in RS3$, if$f_{10}>f_{12}$, then$d_z>0$, the overall profit of the supply chain under decentralized decision-making is greater than that under centralized decision-making, and the supply chain should adopt a decentralized decision-making mode.

$$J_{MR}-J_M-J_R\{\begin{array}{ll}\le 0& d_s\in RS1\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z\ge 0\\ \le 0& d_s\in RS2,f_{10}\le f_{11}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z\in RZ1\\ >0& d_s\in RS2,f_{10}\le f_{11}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z\in RZ2\\ \le 0& d_s\in RS2,f_{11}<f_{10}<f_{12}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z\in RZ3\\ >0& d_s\in RS2,f_{11}<f_{10}<f_{12}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z\in RZ4\\ \le 0& d_s\in RS2,f_{12}\le f_{10}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z>0\\ \ge 0& d_s\in RS3,f_{10}\le f_{12}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z\in RZ5\\ <0& d_s\in RS3,f_{10}\le f_{12}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z\in RZ6\\ <0& d_s\in RS3,f_{10}>f_{12}\hspace{0.17em}\mathrm{and}\hspace{0.17em}{d}_z>0\end{array}$$

(15)

Proof (see Appendix D for the reasoning and proof process).

Proposition 4 shows that delay time provides a basis for supply chain members to choose decision-making methods. There are different value ranges for the delay time of the impact of product quality level on goodwill and the delay time of the impact of service quality level on goodwill. In different ranges, there are different relationships between the overall profit of the supply chain under the two decision-making situations. When supply chain members take centralized decisions, if the manufacturers and retailers can sign a reasonable profit distribution agreement, the optimal profits of the manufacturers and retailers will be “Pareto improved”.

6. Numerical Analysis

Through numerical experiments, this paper analyzes the differences between decentralized decision-making and centralized decision-making in the three aspects of supply chain profit, product goodwill and quality level, to verify the previous theoretical results.

It is assumed that the values of various parameters in the model are: discount rate $\lambda =0.1$, attenuation $\delta =0.01$, marginal profit ${\rho }_{MF}=10$, ${\rho }_{MO}=11$, and ${\rho }_R=4$, influencing factors $b=1.2$, ${\theta }_2=3$, ${\theta }_1=2$, $\gamma =2$, $\beta =1$, $k_2=1$, $k_1=1$, the initial value of brand reputation $G(0)=2$, and potential sales volume in the market $a_1=2$, and $a_2=3$.

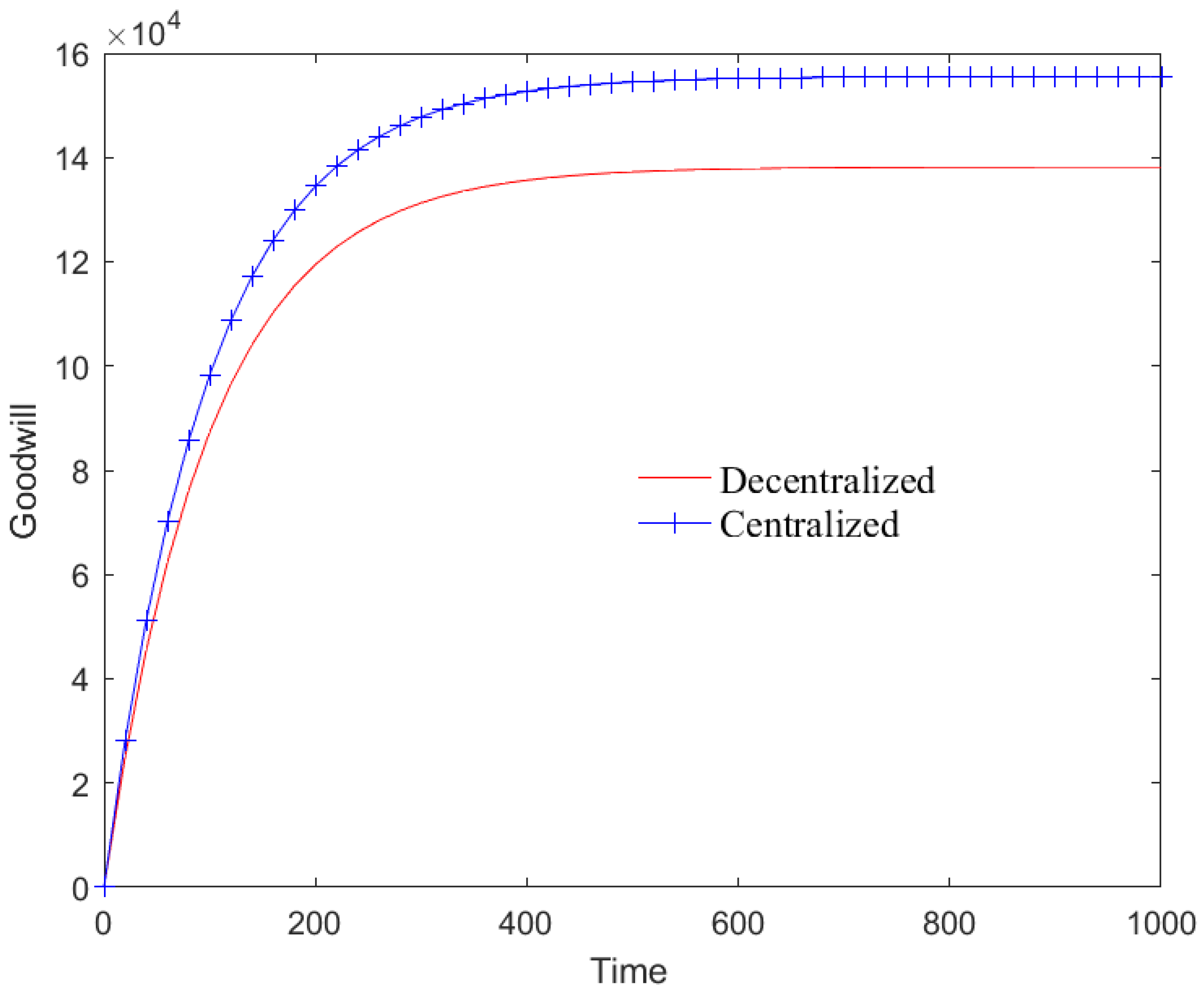
Firstly, according to the benchmark parameters, take $d_z=1$, $d_s=1$, $t\in [0,200]$, and draw the optimal trajectory of product goodwill under a centralized decision and decentralized decision, as shown in Figure 2.

As can be seen from Figure 2, with the passage of time, the product goodwill under centralized decision-making is higher than that under decentralized decision-making, and the gap between goodwill values in the two cases is becoming larger and larger and tends to be stable.

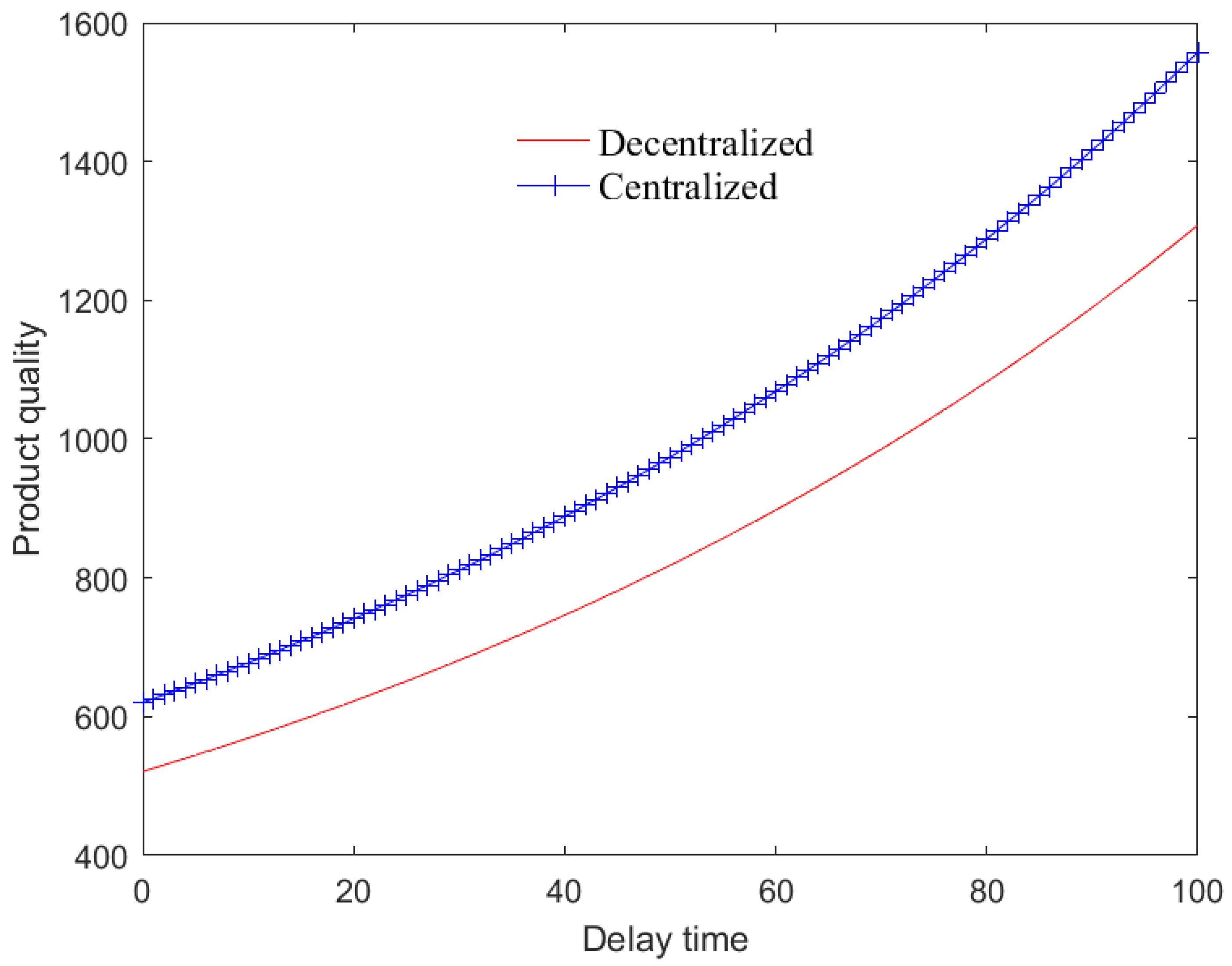
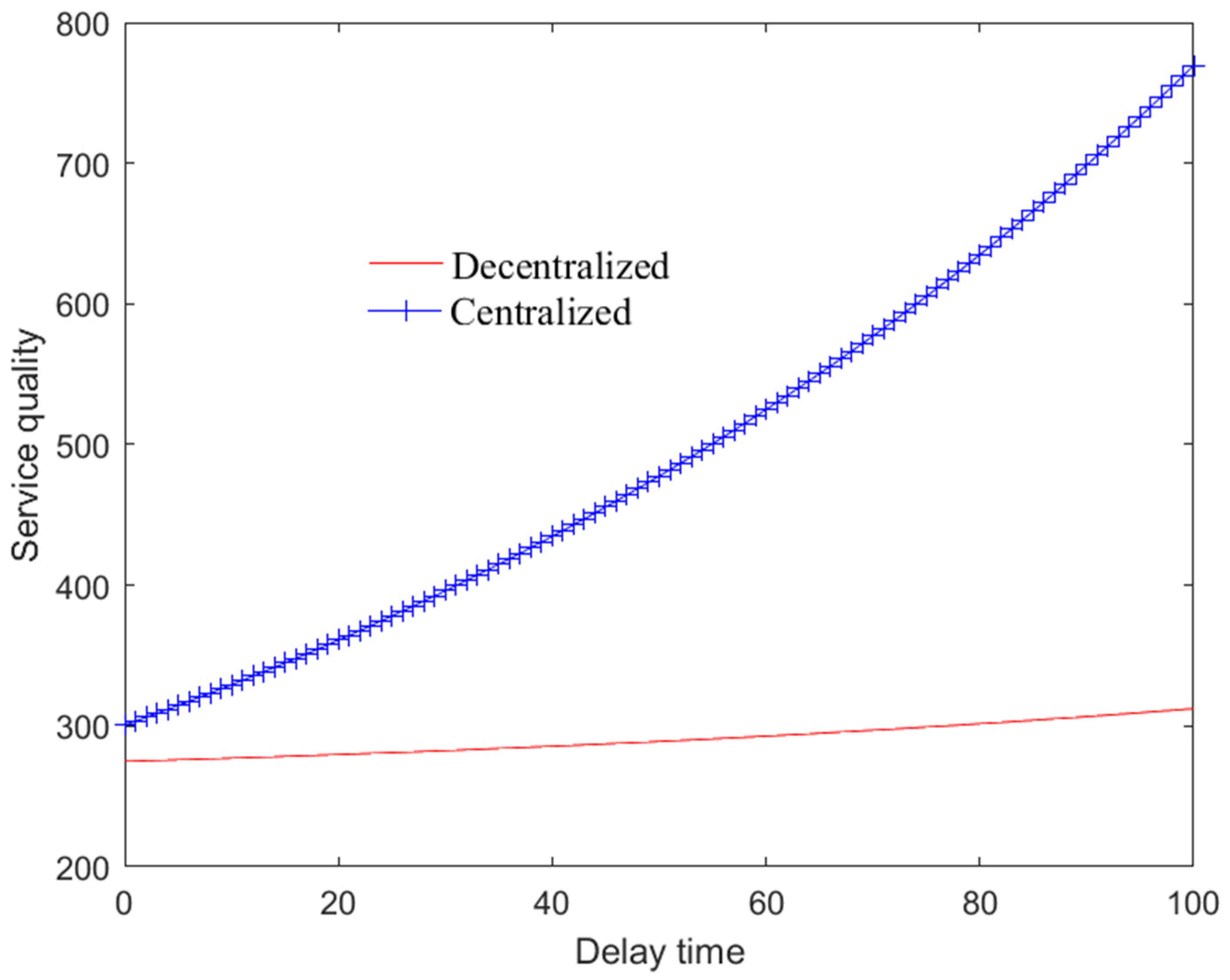
Secondly, according to the benchmark parameters, the effects of delay time on the manufacturer’s product quality level and retailer’s service quality level under decentralized and centralized decision-making are drawn, as shown in Figure 3 and Figure 4. Among them, the abscissa of Figure 3 and Figure 4 are the delay time when product quality affects goodwill and the delay time when service quality affects goodwill, respectively.

It can be seen from Figure 3 and Figure 4 that the product quality level of manufacturers and the service quality level of retailers under centralized decision-making are higher than the corresponding values of decentralized decision-making. The longer the delay time of the impact of the manufacturer’s product quality on goodwill under the two decisions, the higher the degree of improvement of the manufacturer, indicating that the delay time can encourage the manufacturer to make more product quality improvements and improve the level of product quality, similarly, the longer the delay time of the impact of service quality on goodwill, the more incentive for retailers to improve service quality levels.

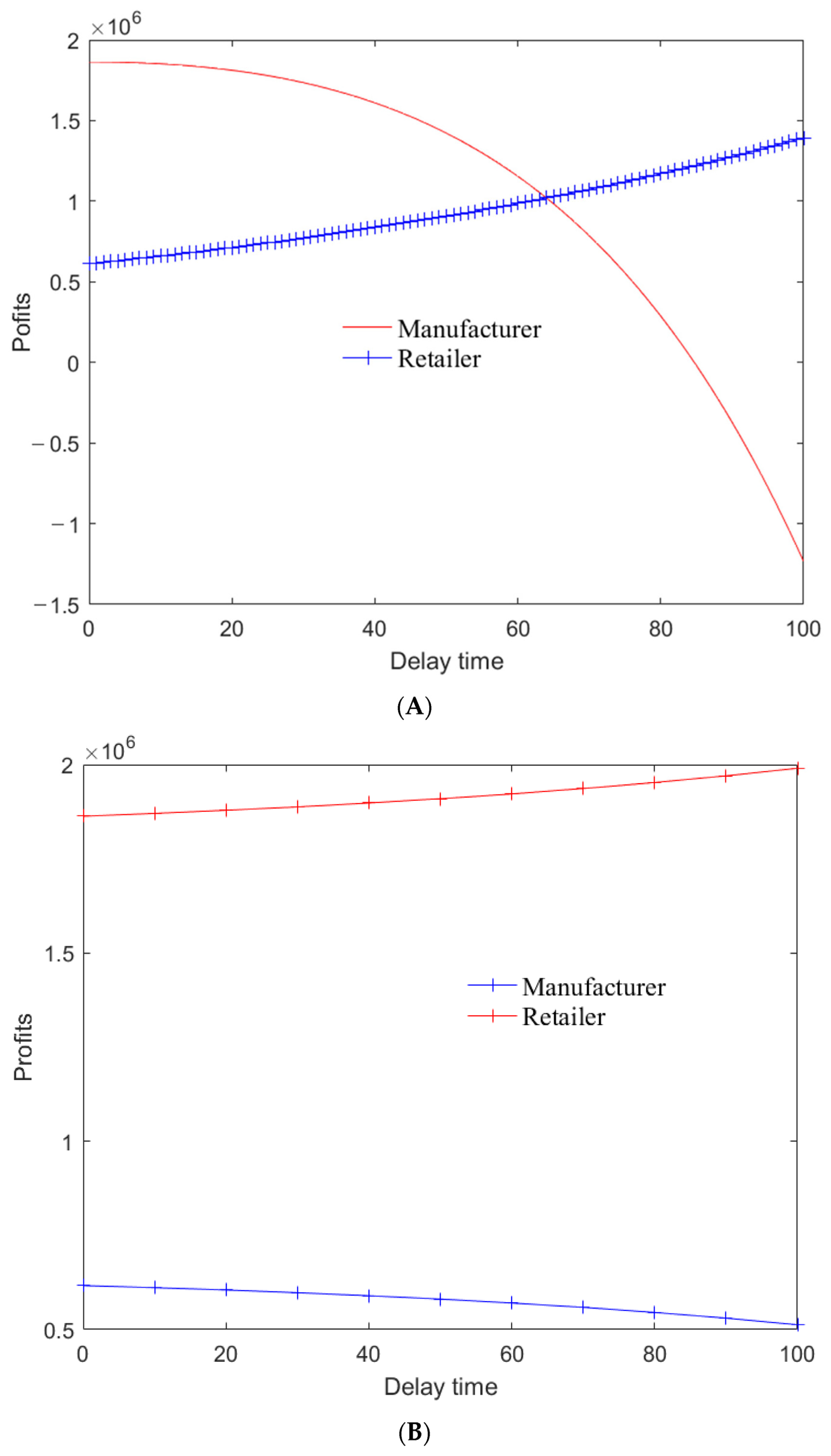
Further, under decentralized decision-making, the change in the manufacturer and retailer profits with delay time $d_z$ ($d_s=1$, $d_z\in [0,100]$), are shown in Figure 5A, and the change in the manufacturer and retailer profits with delay time $d_s$ ($d_z=1$, $d_s\in [0,100]$), are shown in Figure 5B.

It can be seen from Figure 5 that under decentralized decision-making, the manufacturer’s profit decreases with the increase in product quality delay time, and the retailer’s profit increases with the increase of product quality delay time. Therefore, the product quality delay effect is beneficial to the retailer and unfavorable to the manufacturer; under decentralized decision-making, the manufacturer’s profit increases with the increase of service quality delay time, and the retailer’s profit decreases with the increase of service quality delay time. Therefore, it can be concluded that the service quality delay effect is unfavorable to the retailer and beneficial to the manufacturer.

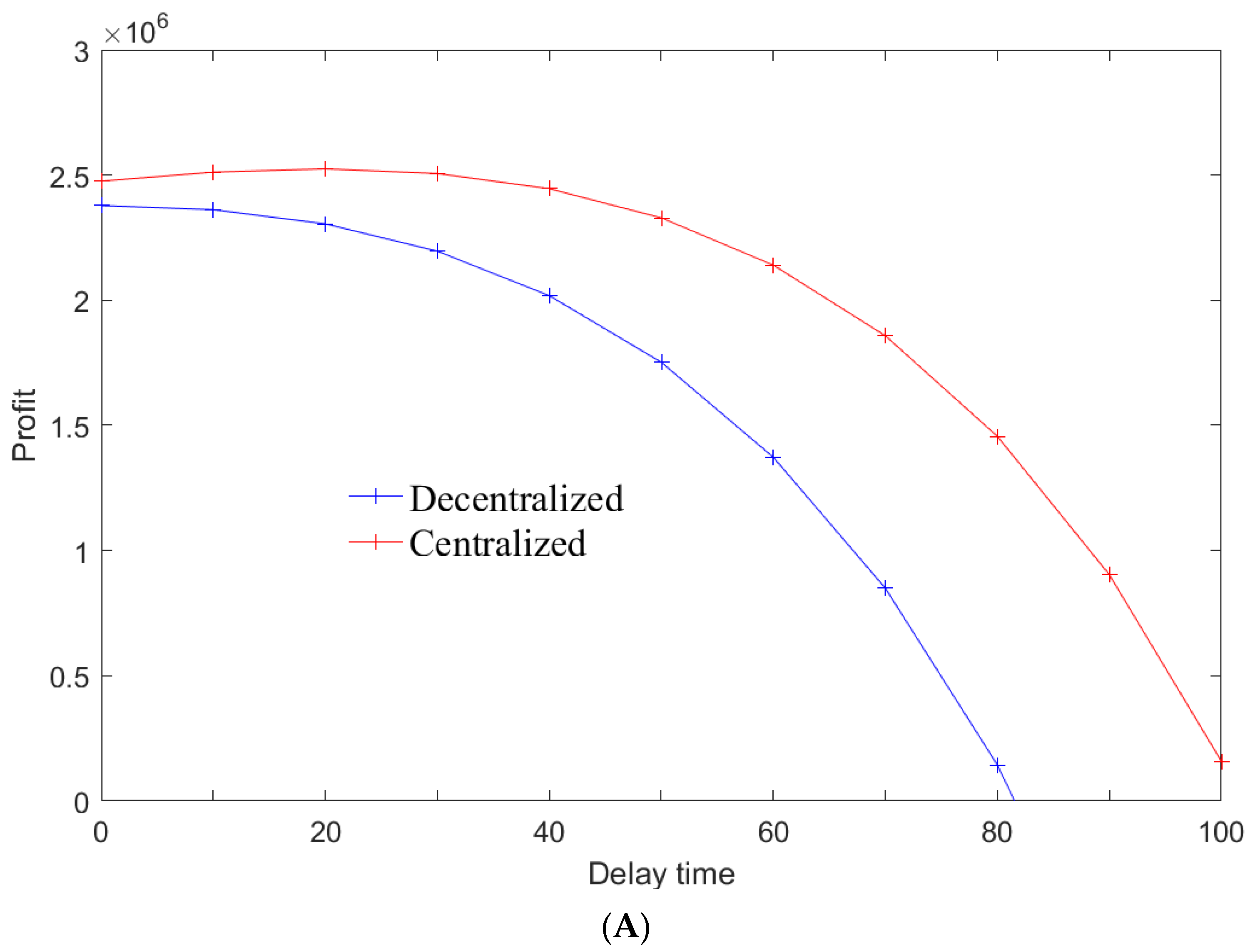
Finally, the change of supply chain profit with product quality delay time under two decision-making situations are drawn as $d_s=1$, $d_z\in [0,100]$ (as Figure 6A); then, the change of supply chain profit with service quality delay time under two decision-making situations is drawn as $d_z=1$, $d_s\in [0,100]$ (as Figure 6B).

It can be seen from Figure 6A that centralized decision-making will promote an improvement of the manufacturer’s product quality and improve the product quality level, but at the same time, it will also lead to an increase in the manufacturer’s cost and reduce the profit of the whole supply chain. Therefore, the profit obtained by decentralized decision-making is greater than that from centralized decision-making.

As can be seen from Figure 6B, the increase in delay time means more improvement in service quality under centralized decision-making, increasing the cost for manufacturers and retailers under centralized decision-making. Therefore, supply chain members will need to take a decentralized decision-making approach.

7. Conclusions and Discussion

In this paper, we propose a differential game model based on delay effects for a two-channel supply chain in a competitive scenario to investigate the dynamic quality decision dilemma between producers and retailers under delay effects. We examine and compare the values of decision variables such as product quality, service quality, goodwill, and supply chain profit in the supply chain under decentralized and centralized decision making, as well as the influence of delay time on the profit and quality levels of supply chain members. The general conclusions are as follows:

7.1. Impact of Corporate Profits on Quality Decisions

Under decentralized decision-making, the influence of corporate profits on quality decisions is reflected in two ways. First, the level of product quality controlled by the manufacturer positively influences market demand, which in turn helps to increase the retailer’s sales revenue, making it more profitable. Second, marginal profit is closely linked to a firm’s service quality improvement. The higher a retailer’s marginal profit, the higher the improvement costs and the longer the economic return cycle, which will, to some extent, reduce the manufacturer’s motivation to improve service quality. From the manufacturer’s perspective, when the manufacturer’s marginal profit is higher, they are willing to share a larger proportion of the service quality improvement cost with the retailer; on the other hand, when the manufacturer’s marginal profit is lower, they are willing to share a smaller proportion of the service quality improvement cost with the retailer. From the perspective of the entire supply chain, the manufacturer bears a portion of the service quality enhancement expenditures, which lessens the economic burden on downstream retailers and enhances the supply chain’s overall service quality level.

Under centralized decision making, the manufacturer and the retailer are considered as two divisions within a firm, and they are both subordinated to the profit maximization decision of the supply chain. Therefore, the relationship between the profit and quality decisions of manufacturers and retailers is mainly based on their profit-sharing contracts, which, if rationalized, will lead to a “Pareto improvement” in the optimal profits of manufacturers and retailers.

7.2. Impact of Delay Effects on Quality Decisions

Delay time is an important reference for supply chain members to choose the decision method. There are different ranges of delay time for the impact of quality on goodwill, and the overall profit size of the supply chain in the two decision scenarios has different relationships with different delay time values.

Among them, under decentralized decision-making, there are two conclusions. First, the longer the delay in the level of product quality affecting goodwill, the more improvement costs the manufacturer faces in product development, technology introduction, etc. The incremental corporate profits caused by quality are then insufficient to cover the costs of inefficient product quality improvement, causing heavy operating pressure. Second, the longer the delay in the level of service quality affecting goodwill, the heavier the cost burden faced by improving the level of service quality, but when the manufacturer’s marginal profit is at a high level, to expand the market size of the product, the manufacturer will actively relieve the cost burden for the retailer and increase the proportion of quality cost improvement to the retailer. Under the centralized decision, to reasonably reduce the negative impact of the delay effect on enterprise profit, the manufacturer and retailer will implement local optimization and the overall optimization of quality improvement through agreement in a joint way, to finally meet the overall goal of dual-channel supply chain unity. As a result, from the perspective of enterprises, they must choose their cooperation approach based on the quality delay reflection features of their supply chain. The supply chain centralized decision-making strategy is the preferable alternative for the quality of the dual-channel supply chain from the perspective of the supply chain as a whole, in the external environment of severe competition and unpredictability. The “win–win” concept of collaborative management is primarily used to continuously optimize the coordination of interests within and outside the manufacturer and suppliers, focusing more on seamless business integration between entities and emphasizing the rational allocation of resources in the supply chain.

7.3. Impact of Decision-Making Approach on Quality, Profit, and Goodwill Decisions

For product quality and service quality, the optimal values obtained by centralized decision-making are higher than the corresponding values for decentralized decision-making, indicating that the adoption of a centralized decision-making model in the supply chain provides incentives for manufacturers and retailers to improve quality levels. However, the product quality of manufacturers and the service quality of retailers are also affected by the discount rate ($\lambda$) and the decay rate ($\delta$), which ($\lambda$) is negatively related to the product quality of manufacturers and the service quality of retailers, while ($\delta$) is positively related to the product quality of manufacturers and the service quality of retailers, respectively.

In terms of goodwill, the optimal value obtained through centralized decision-making is higher than the equivalent value obtained through decentralized decision-making, and the overall trend of product goodwill varies as an increasing function over time and eventually tends to a stable value, regardless of the decision-making scenario. This suggests that the supply chain’s centralized decision-making approach can incentivize manufacturers and merchants to enhance quality, boosting product sales and brand goodwill. Manufacturers and retailers seek joint quality control benefits based on appropriate profit and risk allocation methods, and implement a division of labor, complementary advantages, strong alliances, and benefit-sharing, according to the centralized decision-making model.

For the overall profit of the supply chain, the magnitude of the overall profit in both cases is related to the product quality improvement delay time and the service quality improvement delay time. Specifically, when the product quality delay time increases, the manufacturer’s optimal profit decreases while the retailer’s optimal profit increases; when the service quality delay time increases, the manufacturer’s optimal profit increases while the retailer’s optimal profit decreases, the product quality delay time is negatively related to the manufacturer’s optimal profit and positively related to the retailer’s optimal profit. Additionally, the service quality delay time is positively related to the manufacturer’s optimal profit and negatively related to the manufacturer’s optimal profit that is negatively correlated with the manufacturer’s optimal profit.

This paper studies the quality decision problem in the dual-channel supply chain from a dynamic perspective based on the gap point of existing research; however, due to limited time and resources, there is still a need for deeper research and expansion. First, in the selection of the research object, the research in this paper is only based on the dual-channel supply chain, but in the actual market, it is more common to have a multi-channel structure supply chain model, which can be used as a research object in the future. Second, this paper ignores the behavioral characteristics of consumer demand behavior on quality decision making, but in real life, many products are directly eliminated by the consumer market, forming irreversible product selection, and enterprises cannot make up for the demand through quality improvement. Third, this paper does not greatly consider the influence of competition and cooperation behaviors among decision-makers on quality decision making and future research needs to pay more attention to the competition and cooperation behavioral factors of decision-makers.