Allocation of Environmental Impacts in Circular and Cascade Use of Resources—Incentive-Driven Allocation as a Prerequisite for Cascade Persistence
Abstract
1. Introduction
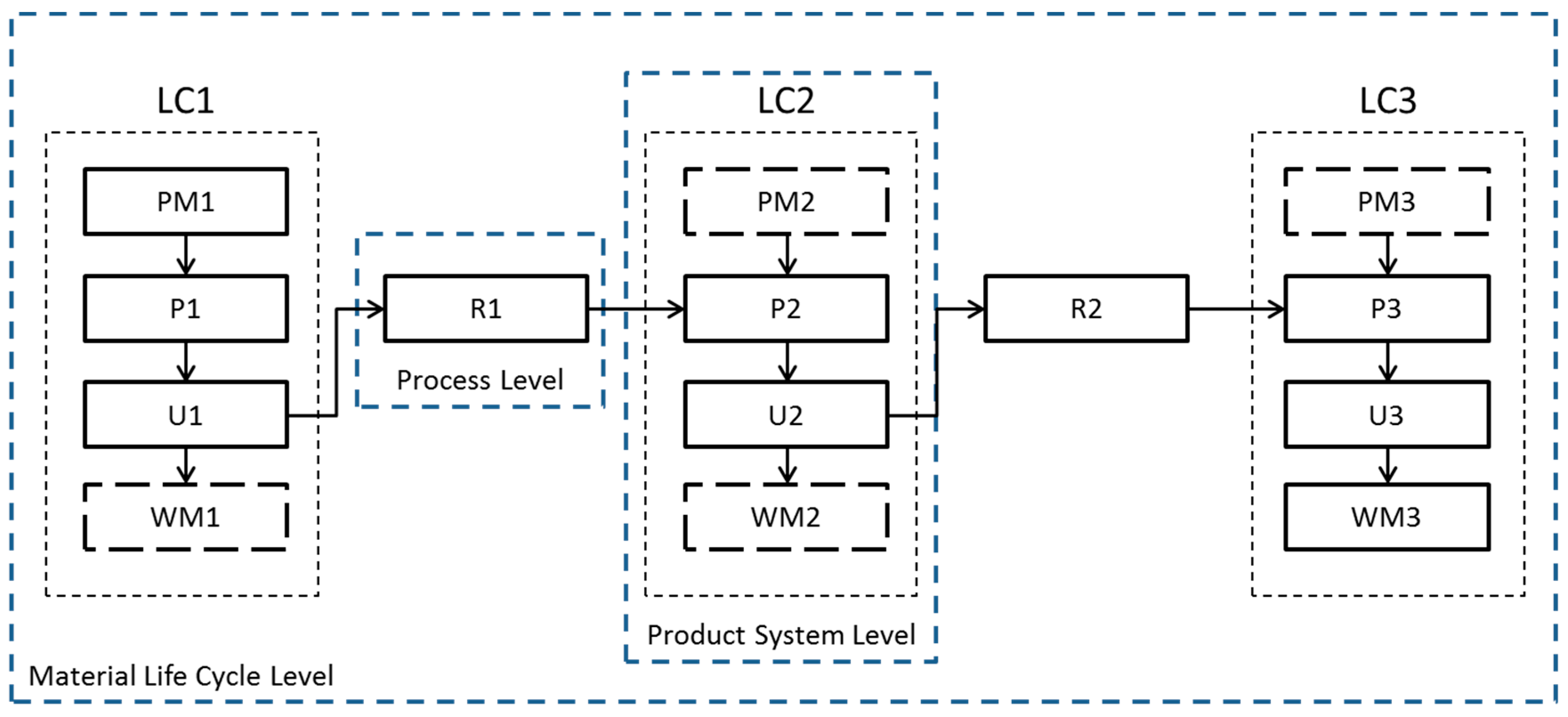
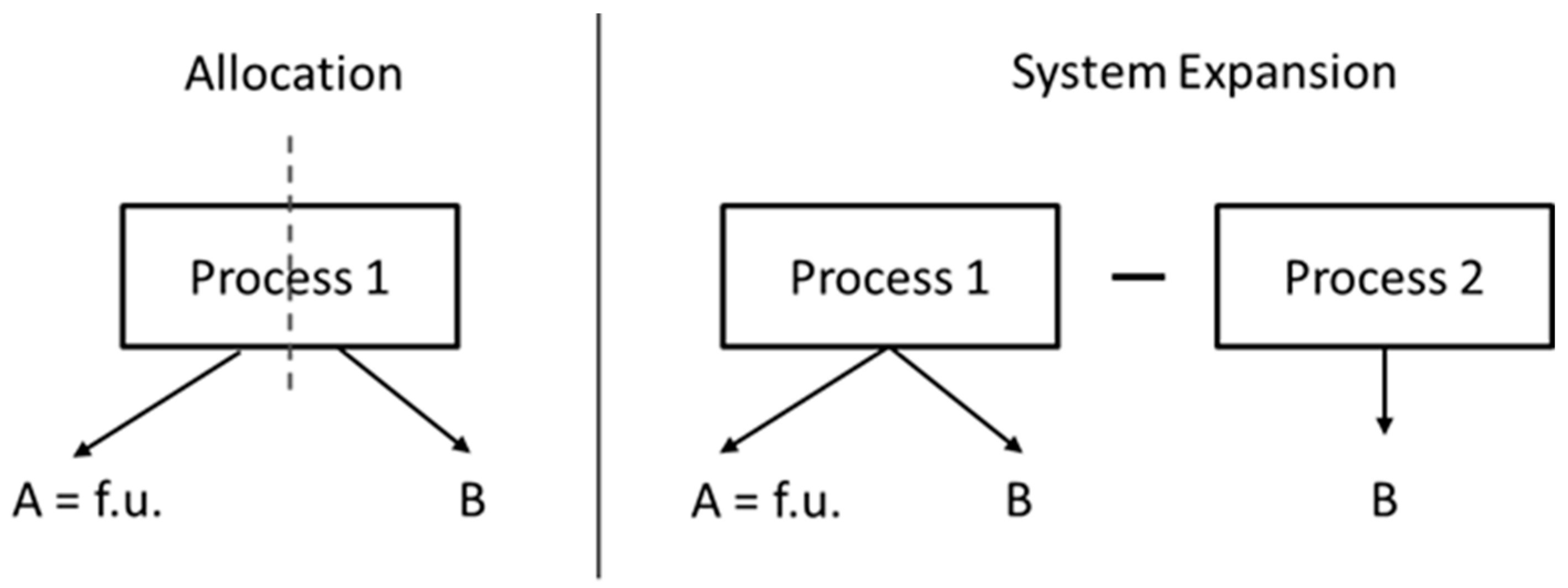
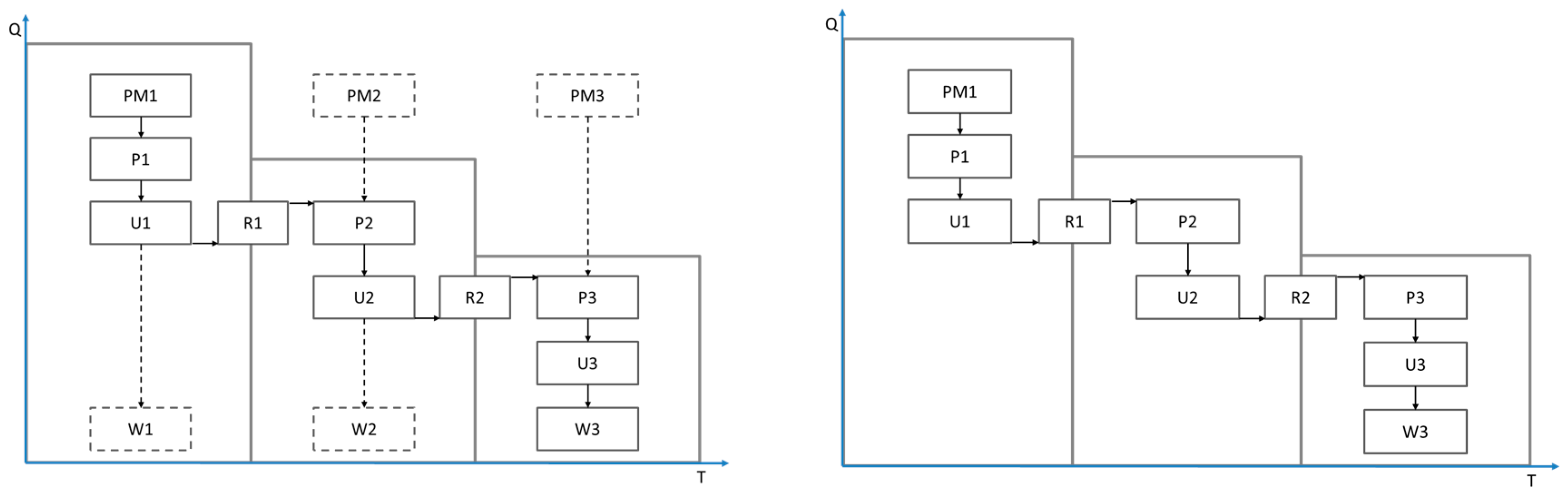
2. Background
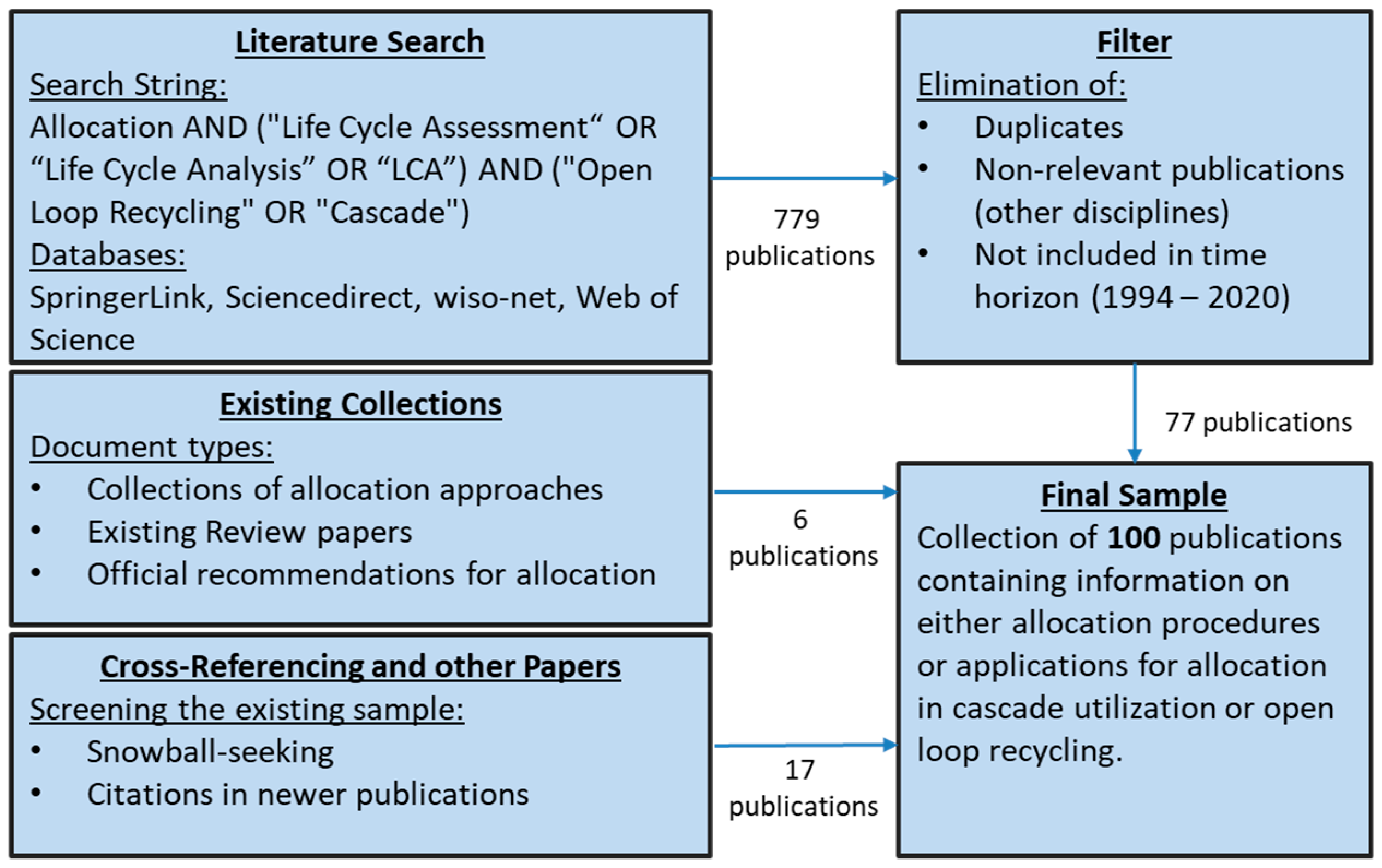
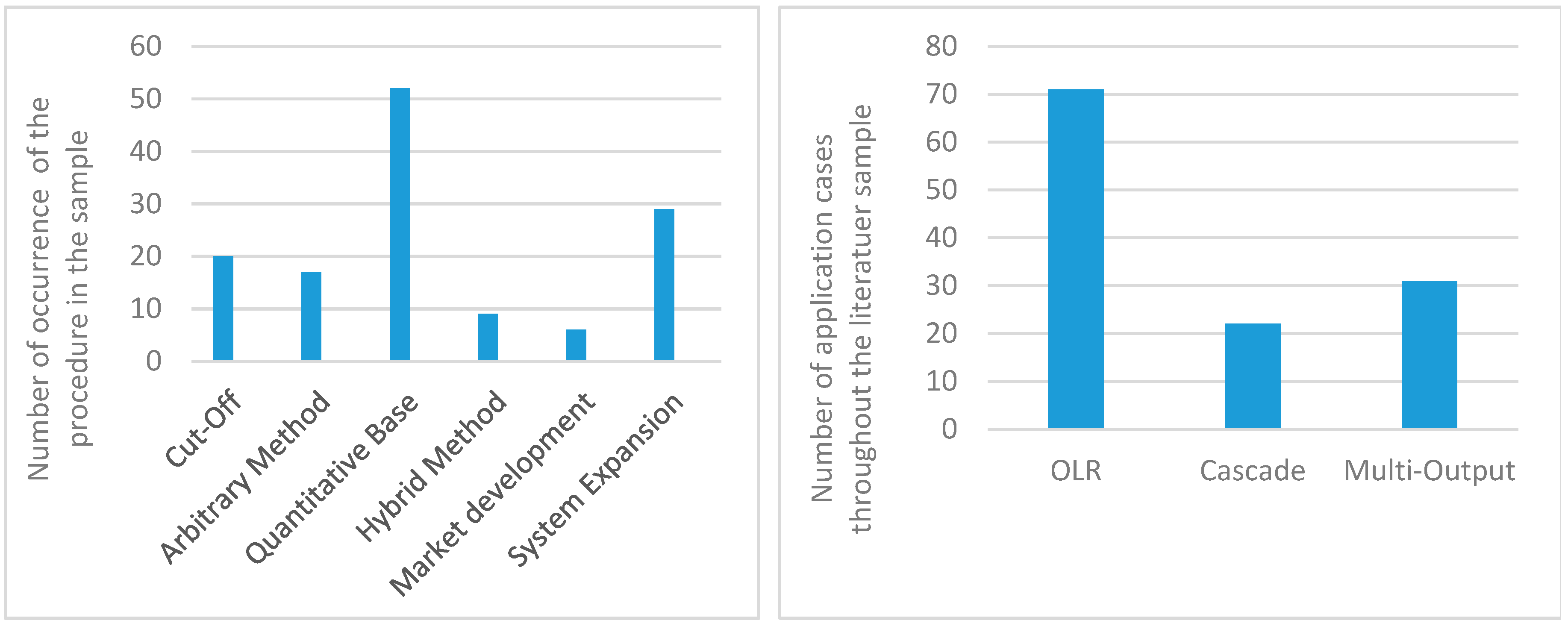
3. Systematic Literature Review
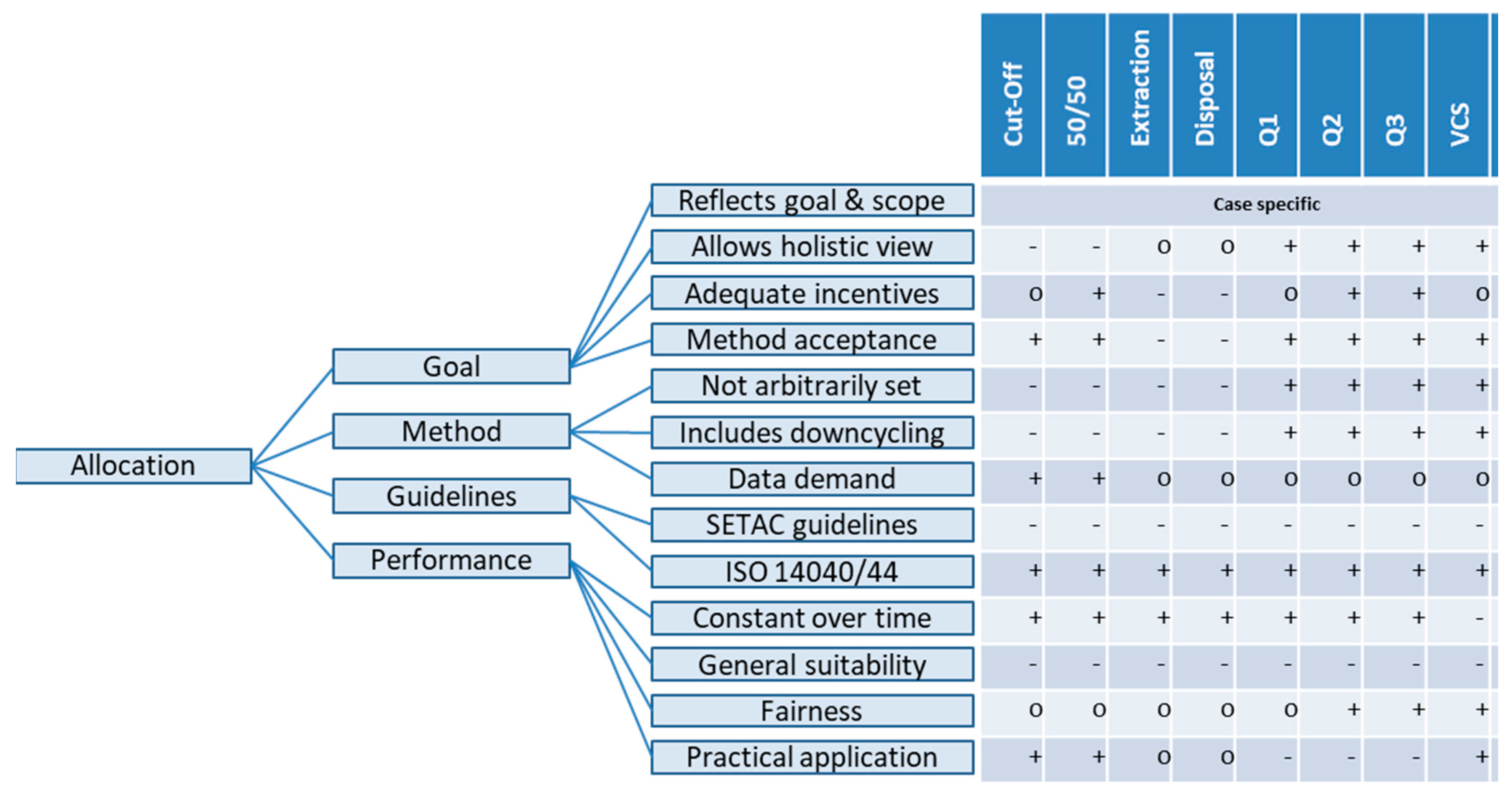
4. Evaluation of the Allocation Procedures
- Institutional guidelines or standards: Besides ISO 14040 and 14040 [9,13], which should form the base of any LCA study, there is a series of more detailed guidelines, such as theSociety of Environmental Toxicology and Chemistry (SETAC) recommendations for allocation in LCA [17], which recommend alignment of goal and scope and procedure applied, re-evaluation of the order of allocation preference as presented in ISO 14044, and an application of industry-specific allocation procedures.
- Goal and scope of the study: The appropriateness with respect to the LCA study’s goal and scope is an indispensable criterion. A holistic view is taken by considering the whole cascade, including upstream and downstream processes from the process in focus. Incentives influence cascade participants in their decision on whether to enter a cascade or not. Although there is no direct effect on environmental burden distribution, incentives can be seen as a prerequisite for cascade use in general and have to be taken into account when choosing allocation procedures. The overall acceptance of the chosen procedure is crucial in order to justify the decision. This includes transparency of the procedure, as well as consensus from all actors involved [113].
- Methodological principles: Basing procedures on a well-founded approach, reflecting the underlying relationships, is advantageous. An intuitively preferable way is the inclusion of inherent quality change, mostly representing downcycling [2].
- Overall performance: A key requirement for the high performance of an allocation procedure is time invariance. This means that factors defining allocation are not changing over (shorter) time. Besides, double-counting must be avoided. General suitability to all possible situations and fairness with respect to all actors involved can be seen as further performance factors. The practical application should not be hindered by any factors necessary for the allocation procedure. Another factor is data demand [2,8,24].
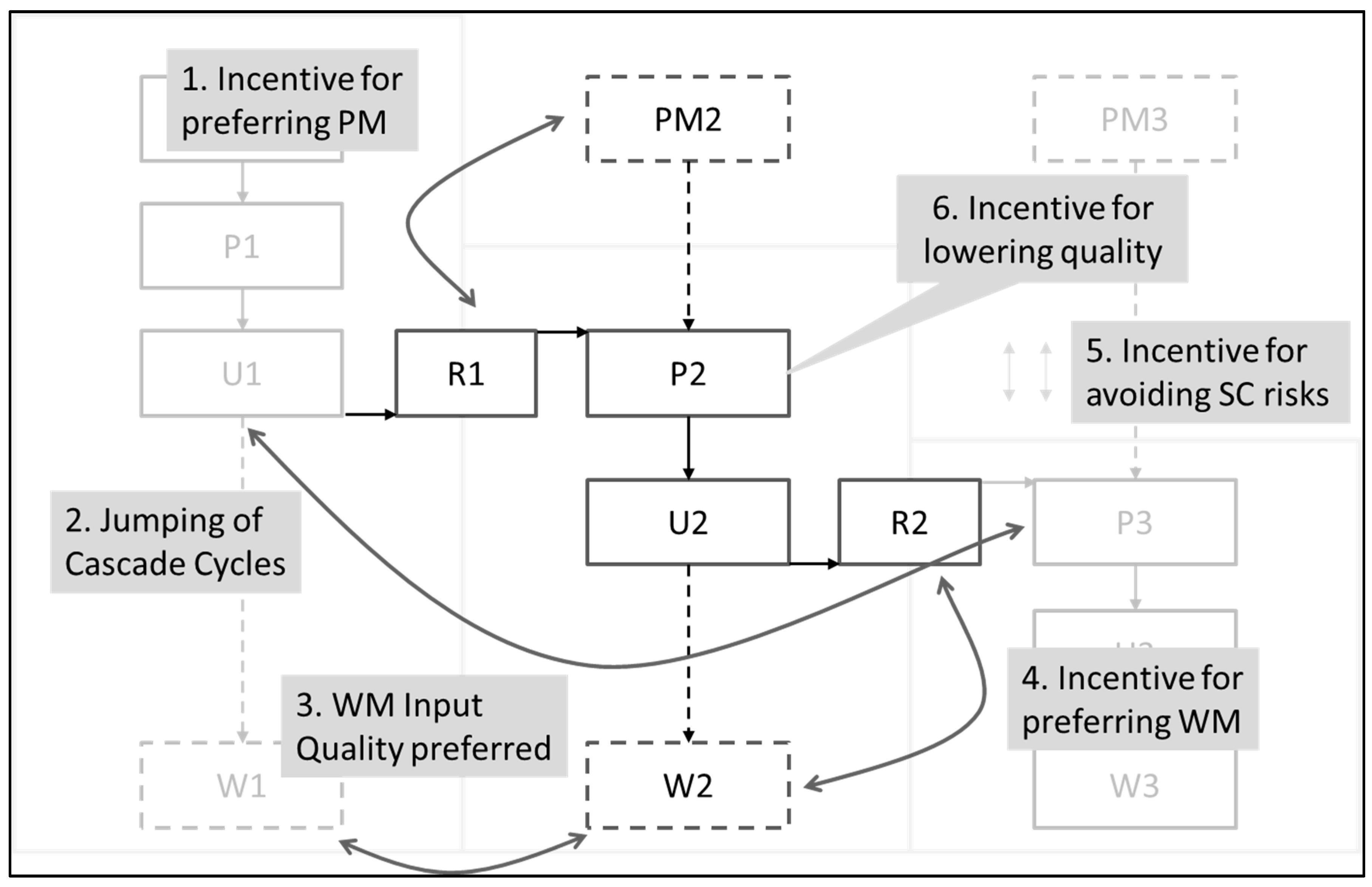
5. Incentives as an Additional Key Criterion for Allocation Procedures in Cascades
- Aspect 1.
- An incentive in place for preferring a primary material over the secondary material supplied by the upstream part of a cascade This may be the case for primary material being offered at a lower price, in better quality, or in a logistically more advantageous way than secondary material.
- Aspect 2.
- An incentive for leaving out a cascade step. This is the case if, for example, an upstream cascade participant sees the advantage of selling his material to a non-neighboring participant further downstream. An example from practice is energetic utilization being economically more advantageous (e.g., caused by a framework/regulation), favoring waste to energy utilization.
- Aspect 3.
- The incentive for the waste management participant to prefer or also accept an input material of higher quality and thus giving the incentive to deliver so.
- Aspect 4.
- Material leaving a cascade step may be preferred to be fed to waste management instead of to a further recycling step. This may be the case due to low revenues from giving material to downstream cascade participants in combination with the low cost of disposal of waste.
- Aspect 5.
- Incentives to not enter a cascade by transaction costs, lock-in effects, or the insecurities linked to relying on others delivering or buying material to achieve their own company goals. These insecurities may include supply, demand, or quality fluctuations or inflexibility in supply and demand.
- Aspect 6.
- Lack of incentives to keep material quality high along the process chain inside a participant’s production, which causes the material to be less useful for all downstream processes, which is not reflected in market prices.
5.1. A Cooperative Game Theory Based Concept for an Optimal Constellation
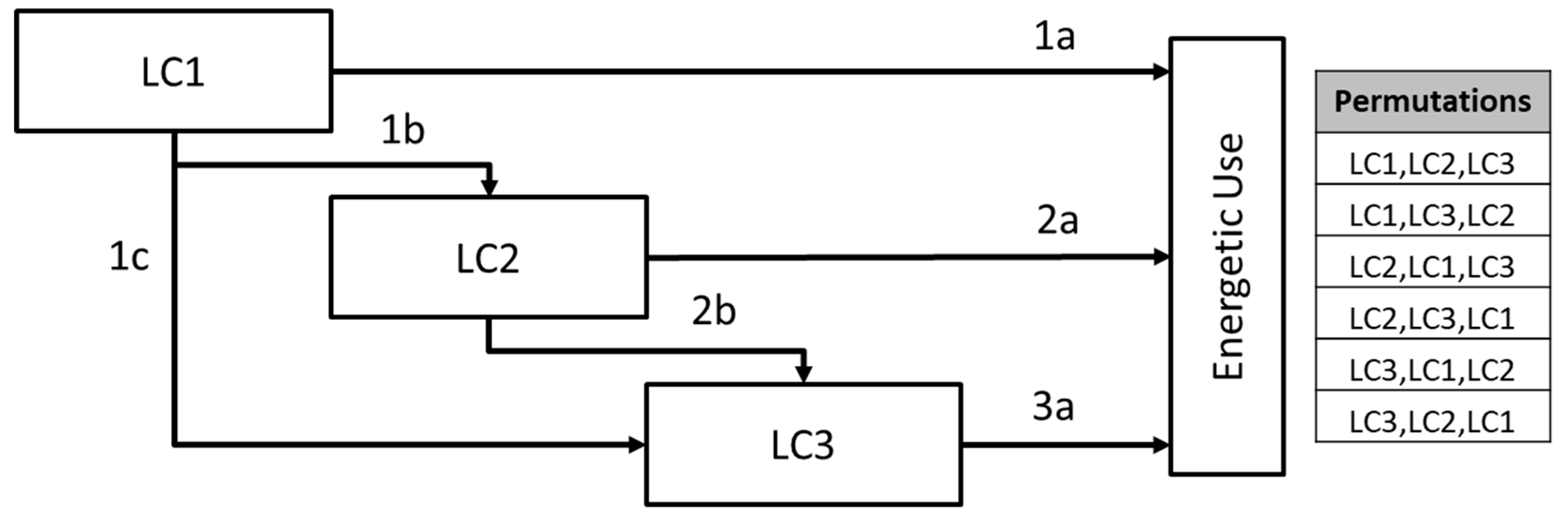
5.2. The Shapley Value for Fair Allocation in the Cascades
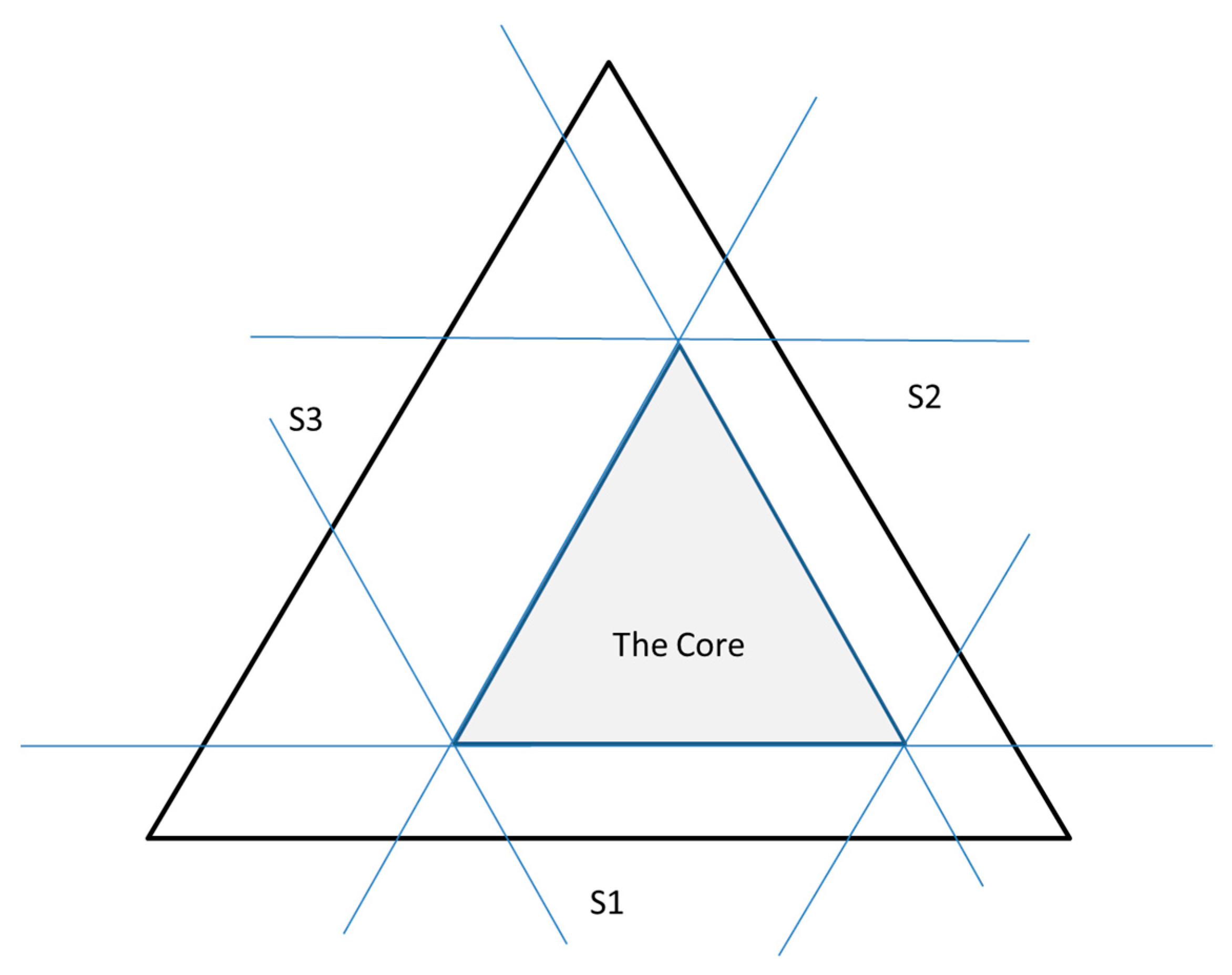
5.3. The Core as a Procedure Incorporating Incentives for a Cascade to Form
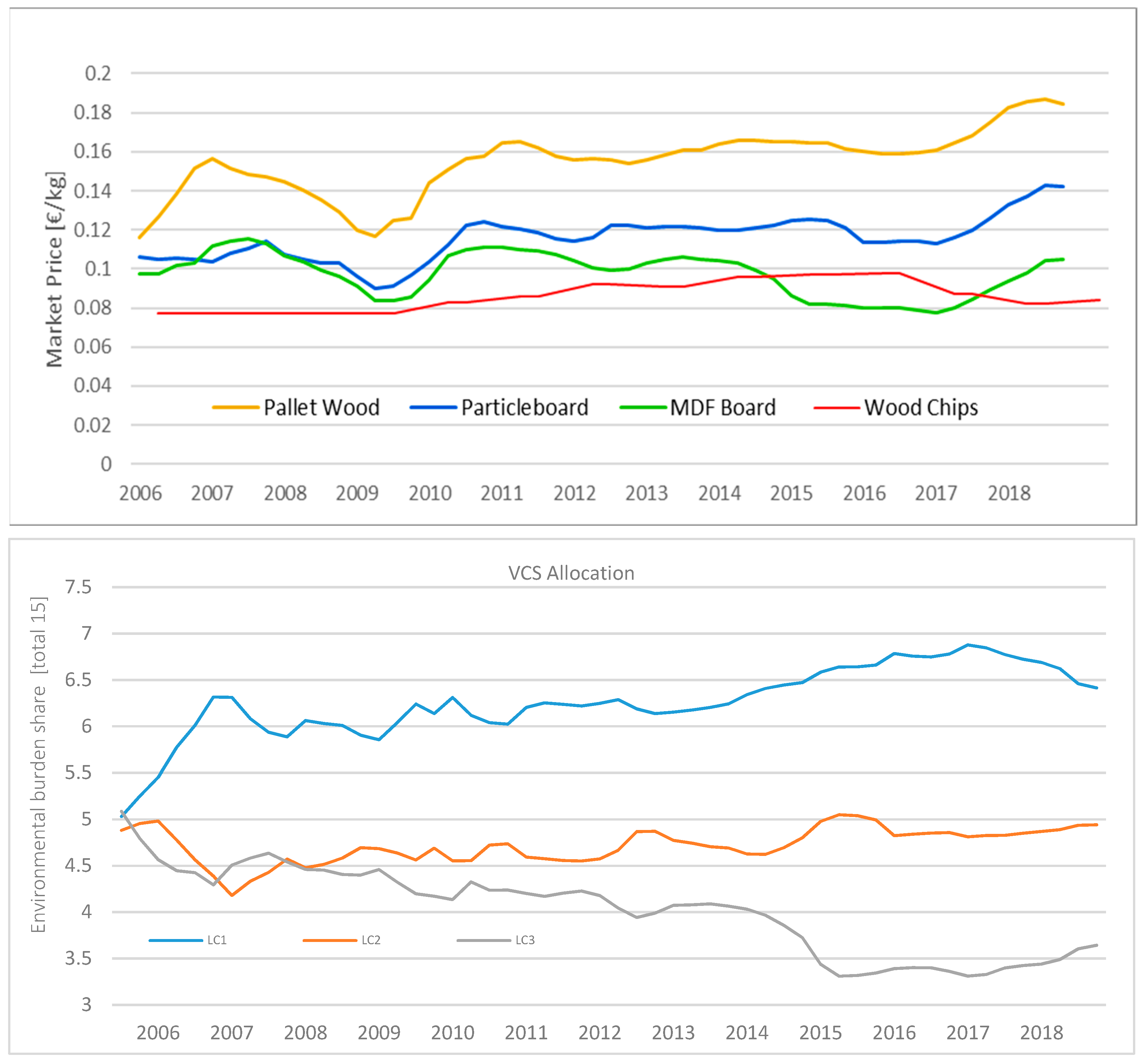
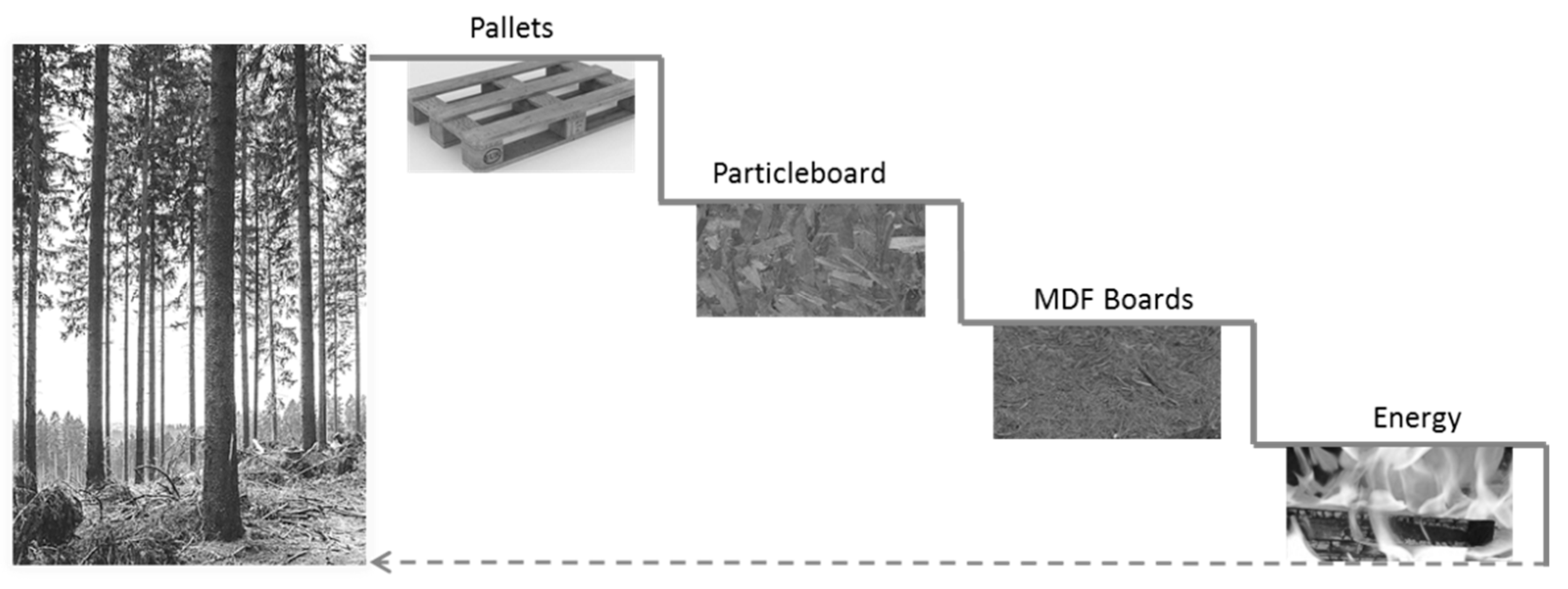
6. Holistic Evaluation Results from the Case Study of a Wood Cascade
7. Discussion
Author Contributions
Funding
Conflicts of Interest
References
- Suh, S.; Weidema, B.; Schmidt, J.H.; Heijungs, R. Generalized Make and Use Framework for Allocation in Life Cycle Assessment. J. Ind. Ecol. 2010, 14, 335–353. [Google Scholar] [CrossRef]
- Kim, S.; Hwang, T.; Lee, K.M. Allocation for Cascade Recycling System LCA Methodology Allocation for Cascade Recycling System. Int. J. Life Cycle Assess. 1997, 2, 217–222. [Google Scholar]
- Sirkin, T.; ten Houten, M. The cascade chain: A theory and tool for achieving resource sustainability with applications for product design. Resour. Conserv. Recycl. 1994, 10, 213–276. [Google Scholar] [CrossRef]
- Vis, M.; Mantau, U.; Allen, B.; Essel, R.; Reichenbach. CASCADES: Study on the Optimised Cascading Use of Wood; Institute for European Environmental Policy Publications Office: Luxembourg, 2016; ISBN 978-92-79-57725-3. [Google Scholar]
- Kosmol, J.; Kanthak, J. UBA Glossar zum Ressourcenschutz; Umweltbundesamt: Dessau-Roßlau, Germany, 2012. [Google Scholar]
- Mettke, A. Ökologische Prozessbetrachtungen—RC-Beton; TU: Cottbus, Germany, 2010. [Google Scholar]
- Ahmadi, L.; Young, S.B.; Fowler, M.; Fraser, R.A.; Achachlouei, M.A. A cascaded life cycle: Reuse of electric vehicle lithium-ion battery packs in energy storage systems. Int. J. Life Cycle Assess. 2017, 22, 111–124. [Google Scholar] [CrossRef]
- Ekvall, T.; Tillman, A.-M. Open-loop recycling: Criteria for allocation procedures. Int. J. Life Cycle Assess. 1997, 2, 155–162. [Google Scholar] [CrossRef]
- DIN EN ISO 14044—2018: Environmental Management—Life Cycle Assessment—Requirements and Guidelines; Deutsches Institut für Normung e.V. (DIN). Available online: https://www.beuth.de/de/norm/din-en-iso-14044/279938986 (accessed on 26 May 2020).
- Zamagni, A.; Guinée, J.; Ekvall, T.; Bersani, R. Critical Review of the Current Research Needs and Limitations Related To ISO Practice; The Italian National Agency on new Technologies, Energy and the Environment: Rome, Italy, 2008. [Google Scholar]
- European Commission; Joint Research Centre. ILCD Handbook: General Guide for Life Cycle Assessment: Detailed Guidance; Publications Office of the European Union: Luxembourg, 2010; ISBN 978-92-79-19092-6. [Google Scholar]
- Gaudreault, C. Methods for Allocation in Life Cycle Assessment and Carbon Footprint Studies of Paper Products; NCASI Nactioan Council for Air and Stream Improvement: Montreal, QC, Canada, 2012. [Google Scholar]
- ISO 14040—2006: Environmental Management—Life Cycle Assessment—Principles and Framework. International Standards Organization. Available online: https://www.iso.org/standard/37456.html (accessed on 26 May 2020).
- ISO ISO/TR 14049:2012-06 Umweltmanagement—Ökobilanz—Beispiele zur Anwendung von ISO 14044 zur Festlegung des Ziels und des Untersuchungsrahmens sowie zur Sachbilanz. International Standards Organization. Available online: https://www.beuth.de/de/technische-regel/iso-tr-14049/154234934 (accessed on 26 May 2020).
- Höglmeier, K.; Steubing, B.; Weber-Blaschke, G.; Richter, K. LCA-based optimization of wood utilization under special consideration of a cascading use of wood. J. Environ. Manag. 2015, 152, 158–170. [Google Scholar] [CrossRef] [PubMed]
- Guinée, J.B.; Heijungs, R.; Huppes, G. Economic allocation: Examples and derived decision tree. Int. J. Life Cycle Assess. 2004, 9, 23–33. [Google Scholar] [CrossRef]
- Lundie, S.; Ciroth, A.; Huppes, G. Inventory Methods in LCA: Towards Consistency and Improvement; UNEP-SETAC Life cycle Inventory (LCI) Programme: Nairobi, Kenya, 2007. [Google Scholar]
- Azapagic, A.; Clift, R. Allocation of environmental burdens in multiple-function systems. J. Clean. Prod. 1999, 7, 101–119. [Google Scholar] [CrossRef]
- Chen, C.; Habert, G.; Bouzidi, Y.; Jullien, A.; Ventura, A. LCA allocation procedure used as an incitative method for waste recycling: An application to mineral additions in concrete. Resour. Conserv. Recycl. 2010, 54, 1231–1240. [Google Scholar] [CrossRef]
- Cherubini, F.; Strømman, A.H.; Ulgiati, S. Influence of allocation methods on the environmental performance of biorefinery products—A case study. Resour. Conserv. Recycl. 2011, 55, 1070–1077. [Google Scholar] [CrossRef]
- Dubreuil, A.; Young, S.B.; Atherton, J.; Gloria, T.P. Metals recycling maps and allocation procedures in life cycle assessment. Int. J. Life Cycle Assess. 2010, 15, 621–634. [Google Scholar] [CrossRef]
- Van der Harst, E.; Potting, J.; Kroeze, C. Comparison of different methods to include recycling in LCAs of aluminium cans and disposable polystyrene cups. Waste Manag. 2016, 48, 565–583. [Google Scholar] [CrossRef]
- Werner, F. Recycling of Used Wood—Inclusion of End of Life Options in LCA; Eidgenössische Materialprüfungs- und Forschungsanstalt (EMPA) Report: Duebendorf, Switzerland, 2000. [Google Scholar]
- Ardente, F.; Cellura, M. Economic Allocation in Life Cycle Assessment: The State of the Art and Discussion of Examples. J. Ind. Ecol. 2011, 16, 387–398. [Google Scholar] [CrossRef]
- Brandenburg, M.; Govindan, K.; Sarkis, J.; Seuring, S. Quantitative models for sustainable supply chain management: Developments and directions. Eur. J. Oper. Res. 2014, 233, 299–312. [Google Scholar] [CrossRef]
- Seuring, S.; Gold, S. Conducting content-analysis based literature reviews in supply chain management. Supply Chain Manag. Int. J. 2012, 17, 544–555. [Google Scholar] [CrossRef]
- Nicholson, A.L.; Olivetti, E.A.; Gregory, J.R.; Field, F.R.; Kirchain, R.E. End-of-life LCA allocation methods: Open loop recycling impacts on robustness of material selection decisions. In Proceedings of the 2009 IEEE International Symposium on Sustainable Systems and Technology, ISSST ’09 in Cooperation with 2009 IEEE International Symposium on Technology and Society, Tempe, AZ, USA, 18–20 May 2009; pp. 1–6. [Google Scholar] [CrossRef]
- Johnson, J.X.; McMillan, C.A.; Keoleian, G.A. Evaluation of Life Cycle Assessment Recycling Allocation Methods. J. Ind. Ecol. 2013, 17, 700–711. [Google Scholar] [CrossRef]
- Schrijvers, D.L.; Loubet, P.; Sonnemann, G. Developing a systematic framework for consistent allocation in LCA. Int. J. Life Cycle Assess. 2016, 1–18. [Google Scholar] [CrossRef]
- Allacker, K.; Mathieux, F.; Pennington, D.; Pant, R. The search for an appropriate end-of-life formula for the purpose of the European Commission Environmental Footprint initiative. Int. J. Life Cycle Assess. 2017, 22, 1441–1458. [Google Scholar] [CrossRef]
- Rehberger, M.; Hiete, M. Allocation procedures for generic cascade use cases—An Evaluation using Monte Carlo Analysis. In Proceedings of the 69. BHT Freiberger Universitätsforum Tagungsband “Future Materials–Safe Resources Supply–Circular Economy”, Freiberg, Germany, 6–8 June 2019. [Google Scholar]
- Allacker, K.; Mathieux, F.; Manfredi, S.; Pelletier, N.; De Camillis, C.; Ardente, F.; Pant, R. Allocation solutions for secondary material production and end of life recovery: Proposals for product policy initiatives. Resour. Conserv. Recycl. 2014, 88, 1–12. [Google Scholar] [CrossRef]
- Andreola, F.; Barbieri, L.; Corradi, A.; Ferrari, A.M.; Lancellotti, I.; Neri, P. Recycling of EOL CRT glass into ceramic glaze formulations and its environmental impact by LCA approach. Int. J. Life Cycle Assess. 2007, 12, 448–454. [Google Scholar] [CrossRef]
- Avadí, A. Screening LCA of French organic amendments and fertilisers. Int. J. Life Cycle Assess. 2020, 25, 698–718. [Google Scholar] [CrossRef]
- Azapagic, A.; Clift, R. Allocation of environmental burdens in co-product systems: Product-related burdens (Part 1). Int. J. Life Cycle Assess. 1999, 4, 357–369. [Google Scholar]
- Bobba, S.; Mathieux, F.; Ardente, F.; Blengini, G.A.; Cusenza, M.A.; Podias, A.; Pfrang, A. Life Cycle Assessment of repurposed electric vehicle batteries: An adapted method based on modelling energy flows. J. Energy Storage 2018, 19, 213–225. [Google Scholar] [CrossRef]
- Boguski, T.K.; Hunt, R.G.; Franklin, W.E. General mathematical models for LCI recycling. Resour. Conserv. Recycl. 1994, 12, 147–163. [Google Scholar]
- Borg, M.; Paulsen, J.; Trinius, W. Proposal of a method for allocation in building-related environmental LCA based on economic parameters. Int. J. Life Cycle Assess. 2001, 6, 219. [Google Scholar]
- Botas, J.A.; Moreno, J.; Espada, J.J.; Serrano, D.P.; Dufour, J. Recycling of used lubricating oil: Evaluation of environmental and energy performance by LCA. Resour. Conserv. Recycl. 2017, 125, 315–323. [Google Scholar] [CrossRef]
- Broeren, M.L.M.; Kuling, L.; Worrell, E.; Shen, L. Environmental impact assessment of six starch plastics focusing on wastewater-derived starch and additives. Resour. Conserv. Recycl. 2017, 127, 246–255. [Google Scholar] [CrossRef]
- Civancik-Uslu, D.; Puig, R.; Ferrer, L.; Fullana-i-Palmer, P. Influence of end-of-life allocation, credits and other methodological issues in LCA of compounds: An in-company circular economy case study on packaging. J. Clean. Prod. 2019, 212, 925–940. [Google Scholar] [CrossRef]
- Cobo, S.; Dominguez-Ramos, A.; Irabien, A. From linear to circular integrated waste management systems: A review of methodological approaches. Resour. Conserv. Recycl. 2017. [Google Scholar] [CrossRef]
- Corrado, S.; Sala, S. Bio-Economy Contribution to Circular Economy. In Designing Sustainable Technologies, Products and Policies; Benetto, E., Gericke, K., Guiton, M., Eds.; Springer International Publishing: Cham, Switzerland, 2018; pp. 49–59. ISBN 978-3-319-66980-9. [Google Scholar]
- Ekvall, T. A market-based approach to allocation at open-loop recycling. Resour. Conserv. Recycl. 2000, 29, 91–109. [Google Scholar] [CrossRef]
- Ekvall, T.; Finnveden, G. Allocation in ISO 14041—A critical review. J. Clean. Prod. 2001, 9, 197–208. [Google Scholar] [CrossRef]
- Ekvall, T.; Weidema, B.P. System boundaries and input data in consequential life cycle inventory analysis. Int. J. Life Cycle Assess. 2004, 9, 161–171. [Google Scholar] [CrossRef]
- Escamilla-Alvarado, C.; Poggi-Varaldo, H.M.; Ponce-Noyola, M.T. Bioenergy and bioproducts from municipal organic waste as alternative to landfilling: A comparative life cycle assessment with prospective application to Mexico. Environ. Sci. Pollut. Res. 2017, 24, 25602–25617. [Google Scholar] [CrossRef] [PubMed]
- Fernández-Dacosta, C.; Stojcheva, V.; Ramirez, A. Closing carbon cycles: Evaluating the performance of multi-product CO 2 utilisation and storage configurations in a refinery. J. CO2 Util. 2018, 23, 128–142. [Google Scholar] [CrossRef]
- Ferreira, J.; Domnigos, I.; Antunes, P. Allocation of Environmental Loads in Recycling - A model based in qualitative value of recycled material. Environ. Conscious Manuf. 2001, 4193, 116–123. [Google Scholar]
- Finnveden, G.; Hauschild, M.Z.; Ekvall, T.; Guinée, J.; Heijungs, R.; Hellweg, S.; Koehler, A.; Pennington, D.; Suh, S. Recent developments in Life Cycle Assessment. J. Environ. Manag. 2009, 91, 1–21. [Google Scholar] [CrossRef]
- Forte, A.; Zucaro, A.; Faugno, S.; Basosi, R.; Fierro, A. Carbon footprint and fossil energy consumption of bio-ethanol fuel production from Arundo donax L. crops on marginal lands of Southern Italy. Energy 2018, 150, 222–235. [Google Scholar] [CrossRef]
- Frees, N. Crediting Aluminium Recycling in LCA by Demand or by Disposal. Int. J. Life Cycle Assess. 2008, 13, 212–218. [Google Scholar] [CrossRef]
- Frischknecht, R. Allocation in life cycle inventory analysis for joint production. Int. J. Life Cycle Assess. 2000, 5, 85–95. [Google Scholar] [CrossRef]
- Frischknecht, R. LCI modelling approaches applied on recycling of materials in view of environmental sustainability, risk perception and eco-efficiency. Int. J. Life Cycle Assess. 2010, 15, 666–671. [Google Scholar] [CrossRef]
- Garofalo, P.; D’Andrea, L.; Tomaiuolo, M.; Venezia, A.; Castrignanò, A. Environmental sustainability of agri-food supply chains in Italy: The case of the whole-peeled tomato production under life cycle assessment methodology. J. Food Eng. 2017, 200, 1–12. [Google Scholar] [CrossRef]
- Heijungs, R.; Frischknecht, R. A special view on the nature of the allocation problem. Int. J. Life Cycle Assess. 1998, 3, 321–332. [Google Scholar] [CrossRef]
- Helmdach, D.; Yaseneva, P.; Heer, P.K.; Schweidtmann, A.M.; Lapkin, A.A. A Multiobjective Optimization Including Results of Life Cycle Assessment in Developing Biorenewables-Based Processes. ChemSusChem 2017, 10, 3632–3643. [Google Scholar] [CrossRef] [PubMed]
- Hermansson, F.; Janssen, M.; Svanström, M. Prospective study of lignin-based and recycled carbon fibers in composites through meta-analysis of life cycle assessments. J. Clean. Prod. 2019, 223, 946–956. [Google Scholar] [CrossRef]
- Hohenthal, C.; Leon, J.; Dobon, A.; Kujanpää, M.; Meinl, G.; Ringman, J.; Hortal, M.; Forsström, U. The ISO 14067 approach to open-loop recycling of paper products: Making it operational. J. Clean. Prod. 2019, 224, 264–274. [Google Scholar] [CrossRef]
- Iacovidou, E.; Velis, C.A.; Purnell, P.; Zwirner, O.; Brown, A.; Hahladakis, J.; Millward-Hopkins, J.; Williams, P.T. Metrics for optimising the multi-dimensional value of resources recovered from waste in a circular economy: A critical review. J. Clean. Prod. 2017, 166, 910–938. [Google Scholar] [CrossRef]
- Ilagan, E.R.; Tan, R.R. Simultaneous allocation and data reconciliation procedure in life cycle inventory analysis using fuzzy mathematical programming. Asia Pac. J. Chem. Eng. 2011, 6, 794–800. [Google Scholar] [CrossRef]
- Jungmeier, G.; Werner, F.; Jarnehammar, A.; Hohenthal, C.; Richter, K. LCA Case Studies Allocation in LCA of Wood-based Products. Int. J. 2002, 7, 369–375. [Google Scholar]
- Kim, S.; Overcash, M. LCA Methodology Allocation Procedure in Multi-Output Process: An Illustration of ISO 14041. Int. J. Life Cycle Assess. 2000, 5, 221–228. [Google Scholar]
- Klöpffer, W. Allocation rule for open-loop recycling in life cycle assessment. Int. J. Life Cycle Assess. 1996, 1, 27–31. [Google Scholar] [CrossRef]
- Koffler, C.; Florin, J. Tackling the Downcycling Issue—A Revised Approach to Value-Corrected Substitution in Life Cycle Assessment of Aluminum (VCS 2.0). Sustainability 2013, 5, 4546–4560. [Google Scholar] [CrossRef]
- Koffler, C.; Finkbeiner, M. Are we still keeping it “real”? Proposing a revised paradigm for recycling credits in attributional life cycle assessment. Int. J. Life Cycle Assess. 2018, 23, 181–190. [Google Scholar] [CrossRef]
- Li, Z.; Du, H.; Xiao, Y.; Guo, J. Carbon footprints of two large hydro-projects in China: Life-cycle assessment according to ISO/TS 14067. Renew. Energy 2017, 114, 534–546. [Google Scholar] [CrossRef]
- Lindfors, L.G. Nordic Guidelines on Life-cycle Assessment; Nordic Council of Ministers: London, UK, 1995; ISBN 92-9120-692-X. [Google Scholar]
- Marinković, S.; Dragaš, J.; Ignjatović, I.; Tošić, N. Environmental assessment of green concretes for structural use. J. Clean. Prod. 2017, 154, 633–649. [Google Scholar] [CrossRef]
- Martin, M.; Svensson, N.; Eklund, M. Who gets the benefits? An approach for assessing the environmental performance of industrial symbiosis. J. Clean. Prod. 2015, 98, 263–271. [Google Scholar] [CrossRef]
- Marvuglia, A.; Cellura, M.; Heijungs, R. Toward a solution of allocation in life cycle inventories: The use of least-squares techniques. Int. J. Life Cycle Assess. 2010, 15, 1020–1040. [Google Scholar] [CrossRef]
- Matsuno, Y.; Daigo, I.; Adachi, Y. LCA Methodology Application of Markov Chain Model to Calculate the Average Number of Times of Use of a Material in Society. Int. J. 2007, 12, 34–39. [Google Scholar]
- McLaren, J.; Parkinson, S.; Jackson, T. Modelling material cascades - Frameworks for the environmental assessment of recycling systems. Resour. Conserv. Recycl. 2000, 31, 83–104. [Google Scholar] [CrossRef]
- Medeiros, D.L.; Tavares, A.O.; Rapôso, Á.; Kiperstok, A. Life cycle assessment in the furniture industry: The case study of an office cabinet. Int. J. Life Cycle Assess. 2017, 22, 1823–1836. [Google Scholar] [CrossRef]
- Mehr, J.; Vadenbo, C.; Steubing, B.; Hellweg, S. Environmentally optimal wood use in Switzerland—Investigating the relevance of material cascades. Resour. Conserv. Recycl. 2018, 131, 181–191. [Google Scholar] [CrossRef]
- Morão, A.; de Bie, F. Life Cycle Impact Assessment of Polylactic Acid (PLA) Produced from Sugarcane in Thailand. J. Polym. Environ. 2019, 27, 2523–2539. [Google Scholar] [CrossRef]
- Nakano, K.; Ando, K.; Takigawa, M.; Hattori, N. Life cycle assessment of wood-based boards produced in Japan and impact of formaldehyde emissions during the use stage. Int. J. Life Cycle Assess. 2018, 23, 957–969. [Google Scholar] [CrossRef]
- Nakatani, J. Life cycle inventory analysis of recycling: Mathematical and graphical frameworks. Sustainability 2014, 6, 6158–6169. [Google Scholar] [CrossRef]
- Oldfield, T.L.; White, E.; Holden, N.M. The implications of stakeholder perspective for LCA of wasted food and green waste. J. Clean. Prod. 2018, 170, 1554–1564. [Google Scholar] [CrossRef]
- Olofsson, J.; Börjesson, P. Residual biomass as resource – Life-cycle environmental impact of wastes in circular resource systems. J. Clean. Prod. 2018, 196, 997–1006. [Google Scholar] [CrossRef]
- Parajuli, R.; Knudsen, M.T.; Djomo, S.N.; Corona, A.; Birkved, M.; Dalgaard, T. Environmental life cycle assessment of producing willow, alfalfa and straw from spring barley as feedstocks for bioenergy or biorefinery systems. Sci. Total Environ. 2017, 586, 226–240. [Google Scholar] [CrossRef]
- Parajuli, R.; Dalgaard, T.; Birkved, M. Can farmers mitigate environmental impacts through combined production of food, fuel and feed? A consequential life cycle assessment of integrated mixed crop-livestock system with a green biorefinery. Sci. Total Environ. 2018, 619–620, 127–143. [Google Scholar] [CrossRef]
- Paras, M.K.; Pal, R. Application of Markov chain for LCA: A study on the clothes ‘reuse’ in Nordic countries. Int. J. Adv. Manuf. Technol. 2018, 94, 191–201. [Google Scholar] [CrossRef]
- Paraskevas, D.; Kellens, K.; Dewulf, W.; Duflou, J.R. 10. 1 Closed and Open Loop Recycling of Aluminium: A Life Cycle Assessment Perspective. In Proceedings of the 11th Global Conference on Sustainable Manufacturing - Innovative Solutions; Seilger, G., Ed.; Universitätsverlag der TU: Berlin, Germany, 2013; pp. 305–310. [Google Scholar]
- Pawelzik, P.; Carus, M.; Hotchkiss, J.; Narayan, R.; Selke, S.; Wellisch, M.; Weiss, M.; Wicke, B.; Patel, M.K.K. Critical aspects in the life cycle assessment (LCA) of bio-based materials—Reviewing methodologies and deriving recommendations. Resour. Conserv. Recycl. 2013, 73, 211–228. [Google Scholar] [CrossRef]
- Perez-Gallardo, J.R.; Azzaro-Pantel, C.; Astier, S. A Multi-objective Framework for Assessment of Recycling Strategies for Photovoltaic Modules based on Life Cycle Assessment. Waste Biomass Valorization 2018, 9, 147–159. [Google Scholar] [CrossRef]
- Rasmussen, F.; Birkved, M.; Birgisdóttir, H. Upcycling and Design for Disassembly—LCA of buildings employing circular design strategies. IOP Conf. Ser. Earth Environ. Sci. 2019, 225, 012040. [Google Scholar] [CrossRef]
- Reale, F.; Buttol, P.; Cortesi, S.; Mengarelli, M.; Masoni, P.; Scalbi, S.; Zamagni, A. Dealing with LCA modeling for the end of life of mechatronic products. Environ. Eng. Manag. J. 2015, 14, 1691–1704. [Google Scholar] [CrossRef]
- Rice, P.; O’Brien, D.; Shalloo, L.; Holden, N.M. Evaluation of allocation methods for calculation of carbon footprint of grass-based dairy production. J. Environ. Manag. 2017, 202, 311–319. [Google Scholar] [CrossRef] [PubMed]
- Richa, K.; Babbitt, C.W.; Nenadic, N.G.; Gaustad, G. Environmental trade-offs across cascading lithium-ion battery life cycles. Int. J. Life Cycle Assess. 2017, 22, 66–81. [Google Scholar] [CrossRef]
- Risse, M.; Weber-Blaschke, G.; Richter, K. Resource efficiency of multifunctional wood cascade chains using LCA and exergy analysis, exemplified by a case study for Germany. Resour. Conserv. Recycl. 2017, 126, 141–152. [Google Scholar] [CrossRef]
- Sandin, G.; Peters, G.M. Environmental impact of textile reuse and recycling—A review. J. Clean. Prod. 2018, 184, 353–365. [Google Scholar] [CrossRef]
- Seghetta, M.; Goglio, P. Life Cycle Assessment of Seaweed Cultivation Systems. In Biofuels from Algae; Spilling, K., Ed.; Methods in Molecular Biology; Springer: New York, NY, USA, 2018; Volume 1980, pp. 103–119. ISBN 978-1-4939-9415-1. [Google Scholar]
- Sfez, S.; De Meester, S.; Vlaeminck, S.E.; Dewulf, J. Improving the resource footprint evaluation of products recovered from wastewater: A discussion on appropriate allocation in the context of circular economy. Resour. Conserv. Recycl. 2019, 148, 132–144. [Google Scholar] [CrossRef]
- Shen, L.; Worrell, E.; Patel, M.K. Open-loop recycling: A LCA case study of PET bottle-to-fibre recycling. Resour. Conserv. Recycl. 2010, 55, 34–52. [Google Scholar] [CrossRef]
- Sommerhuber, P.F.; Wenker, J.L.; Rüter, S.; Krause, A. Life cycle assessment of wood-plastic composites: Analysing alternative materials and identifying an environmental sound end-of-life option. Resour. Conserv. Recycl. 2017, 117, 235–248. [Google Scholar] [CrossRef]
- Song, X.; Pettersen, J.B.; Pedersen, K.B.; Røberg, S. Comparative life cycle assessment of tailings management and energy scenarios for a copper ore mine: A case study in Northern Norway. J. Clean. Prod. 2017, 164, 892–904. [Google Scholar] [CrossRef]
- Suter, F.; Steubing, B.; Hellweg, S. Life Cycle Impacts and Benefits of Wood along the Value Chain: The Case of Switzerland: Life Cycle Impacts and Benefits of Wood. J. Ind. Ecol. 2017, 21, 874–886. [Google Scholar] [CrossRef]
- Timonen, K.; Sinkko, T.; Luostarinen, S.; Tampio, E.; Joensuu, K. LCA of anaerobic digestion: Emission allocation for energy and digestate. J. Clean. Prod. 2019, 235, 1567–1579. [Google Scholar] [CrossRef]
- Toniolo, S.; Mazzi, A.; Pieretto, C.; Scipioni, A. Allocation strategies in comparative life cycle assessment for recycling: Considerations from case studies. Resour. Conserv. Recycl. 2017, 117, 249–261. [Google Scholar] [CrossRef]
- Visintin, P.; Xie, T.; Bennett, B. A large-scale life-cycle assessment of recycled aggregate concrete: The influence of functional unit, emissions allocation and carbon dioxide uptake. J. Clean. Prod. 2020, 248, 119243. [Google Scholar] [CrossRef]
- Vladimirov, V.; Bica, I. Methodology and calculation model for recycling of composite construction products. E3s Web Conf. 2019, 85, 07016. [Google Scholar] [CrossRef]
- Vogtländer, J.; Brezet, H.; Hendriks, C. Allocation in Recycling Systems. Int. J. Life Cycle Assess. 2001, 6, 344–355. [Google Scholar] [CrossRef]
- Weidema, B. Avoiding Co-Product Allocation in Life-Cycle Assessment. J. Ind. Ecol. 2001, 4, 11–33. [Google Scholar] [CrossRef]
- Weidema, B. Market Information in Life Cycle Assessment; Environmental Project No. 863 2003; Danish Environmental Protection Agency: Copenhagen, Denmark, 2003. [Google Scholar]
- Werner, F. Recycling of used wood—Inclusion of end-of-life options in LCA. Life Cycle Assess. For. For. Prod. Achiev. COST Action E9 Work. Group 2001, 3, 1–24. [Google Scholar]
- Werner, F.; Althaus, H.-J.; Richter, K.; Scholz, R.W. Post-consumer waste wood in attributive product LCA. Int. J. Life Cycle Assess. 2007, 12, 160–172. [Google Scholar] [CrossRef]
- Williams, T.G.J.L.; Heidrich, O.; Sallis, P.J. A case study of the open-loop recycling of mixed plastic waste for use in a sports-field drainage system. Resour. Conserv. Recycl. 2010, 55, 118–128. [Google Scholar] [CrossRef]
- Xia, B.; Ding, T.; Xiao, J. Life cycle assessment of concrete structures with reuse and recycling strategies: A novel framework and case study. Waste Manag. 2020, 105, 268–278. [Google Scholar] [CrossRef]
- Yan, M.; Holden, N.M. Life cycle assessment of multi-product dairy processing using Irish butter and milk powders as an example. J. Clean. Prod. 2018, 198, 215–230. [Google Scholar] [CrossRef]
- Yang, J.; Gu, F.; Guo, J. Environmental feasibility of secondary use of electric vehicle lithium-ion batteries in communication base stations. Resour. Conserv. Recycl. 2020, 156, 104713. [Google Scholar] [CrossRef]
- Zhang, Y.; Luo, W.; Wang, J.; Wang, Y.; Xu, Y.; Xiao, J. A review of life cycle assessment of recycled aggregate concrete. Constr. Build. Mater. 2019, 209, 115–125. [Google Scholar] [CrossRef]
- Pauliuk, S.; Majeau-Bettez, G.; Mutel, C.L.; Steubing, B.; Stadler, K. Lifting Industrial Ecology Modeling to a New Level of Quality and Transparency: A Call for More Transparent Publications and a Collaborative Open Source Software Framework: Open Source Software for Industrial Ecology. J. Ind. Ecol. 2015, 19, 937–949. [Google Scholar] [CrossRef]
- Werner, F.; Scholz, R. Ambiguities in decision-oriented Life Cycle Inventories. Int. J. Life Cycle Assess. 2002, 6, 9. [Google Scholar] [CrossRef]
- Müller, Eva Ursula Price Information Website for wood materials until January 2015. Available online: https://factsandfigures.fnr.de/ (accessed on 26 May 2020).
- Rademacher, J. Wood Pricing Index Website of the HPE; Industry Association for Wooden Packaging, Pallets and Export Packaging: Düsseldorf, Germany, 2019. [Google Scholar]
- Lenz Kaminholz-Wissen Website for Wood material prices Waldbröl. 2019. Available online: http://www.kaminholz-wissen.de/ (accessed on 26 May 2020).
- Holler, M.; Illing, G. Einführung in die Spieltheorie; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Tijs, S.H.; Driessen, T.S.H. Game Theory and Cost Allocation Problems. Manag. Sci. 1986, 32, 1015–1028. [Google Scholar] [CrossRef]
- Rogerson, W. Intertemporal Cost Allocation and Investment Decisions. J. Political Econ. 2008, 116, 931–950. [Google Scholar] [CrossRef]
- Young, H.P. Producer Incentives in Cost Allocation. Econometrica 1985, 53, 757. [Google Scholar] [CrossRef]
- Zucca, R. A Cooperative Game Theory Approach for Cost Allocation in Complex Water Resource Systems; Università degli Studi di Cagliari: Cagliari, Italy, 2010. [Google Scholar]
- Shapley, L.S. A Value for n-person Games. In Contributions to the Theory of Games, Volume II; Princeton University Press: Princeton, NJ, USA, 1953; pp. 307–317. ISBN 0-691-07935-8. [Google Scholar]
- Roth, A.E.; Verrecchia, R.E. The Shapley Value As Applied to Cost Allocation: A Reinterpretation. J. Account. Res. 1979, 17, 295. [Google Scholar] [CrossRef]
- Hiete, M.; Ludwig, J.; Schultmann, F. Intercompany Energy Integration: Adaptation of Thermal Pinch Analysis and Allocation of Savings. J. Ind. Ecol. 2012, 16, 689–698. [Google Scholar] [CrossRef]
- Van Campen, T.; Hamers, H.; Husslage, B.; Lindelauf, R. A new approximation method for the Shapley value applied to the WTC 9/11 terrorist attack. Soc. Netw. Anal. Min. 2018, 8. [Google Scholar] [CrossRef]
- Gillies, D.B. Some Theorems on n-Person Games. Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 1953. [Google Scholar]
- Shapley, L.S.; Shubik, M. The assignment game I: The core. Int. J. Game Theory 1971, 1, 111–130. [Google Scholar] [CrossRef]
- DIN EN 13698-1:2004-01 Produktspezifikation für Paletten—Teil 1: Herstellung von 800 mm×1200 mm-Flachpaletten aus Holz. Deutsches Institut für Normung e.V. (DIN); Available online: https://www.beuth.de/de/norm/din-en-13698-1/60040228 (accessed on 26 May 2020).
- Wood Pricing Data for December 2016 from Forstbetriebsgemeinschaft Amberg-Schnaittenbach w.V (a German regional forestry association). Available online: https://fbg-amberg.de/. (accessed on 26 May 2020).
- Probst, T. Plasticker Marktbericht für Primär- und Sekundärkunststoffe—Market Report for Primary and Secondary Plastics; R. Verhoeven: Kaarst, Germany, 2018. [Google Scholar]


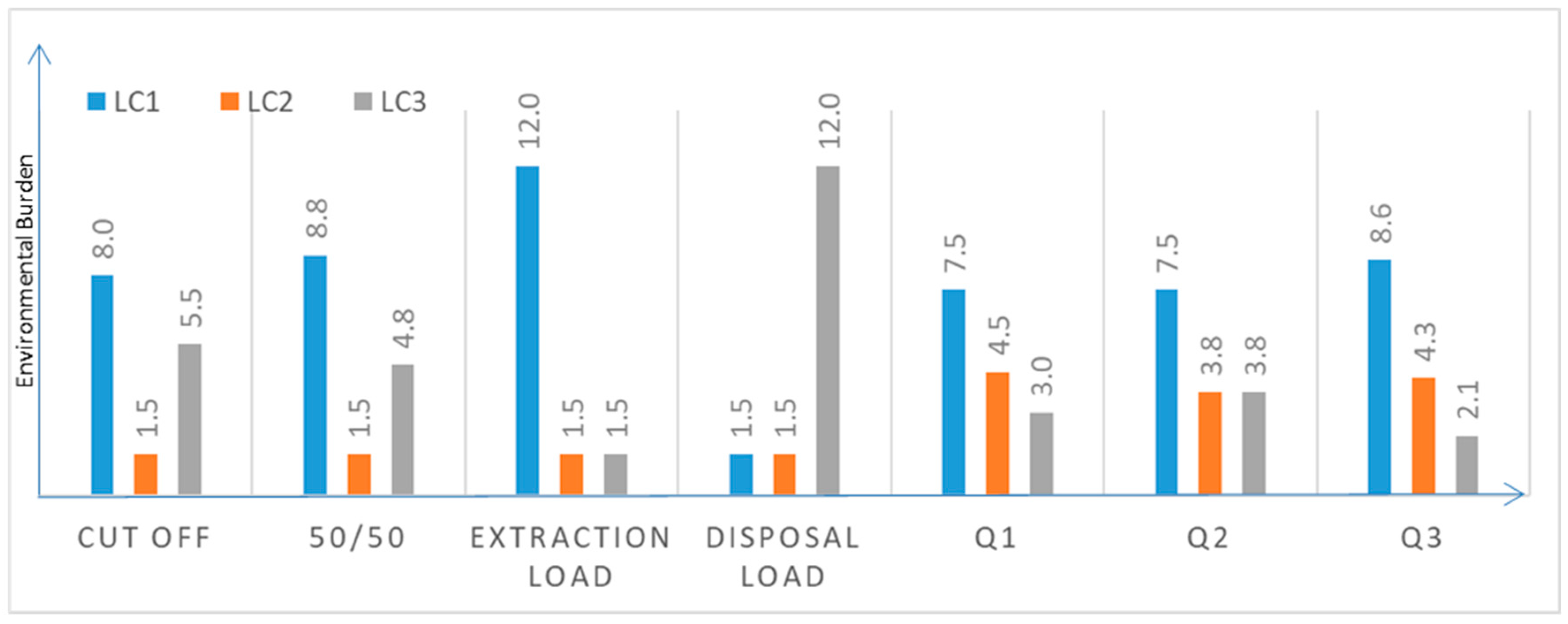
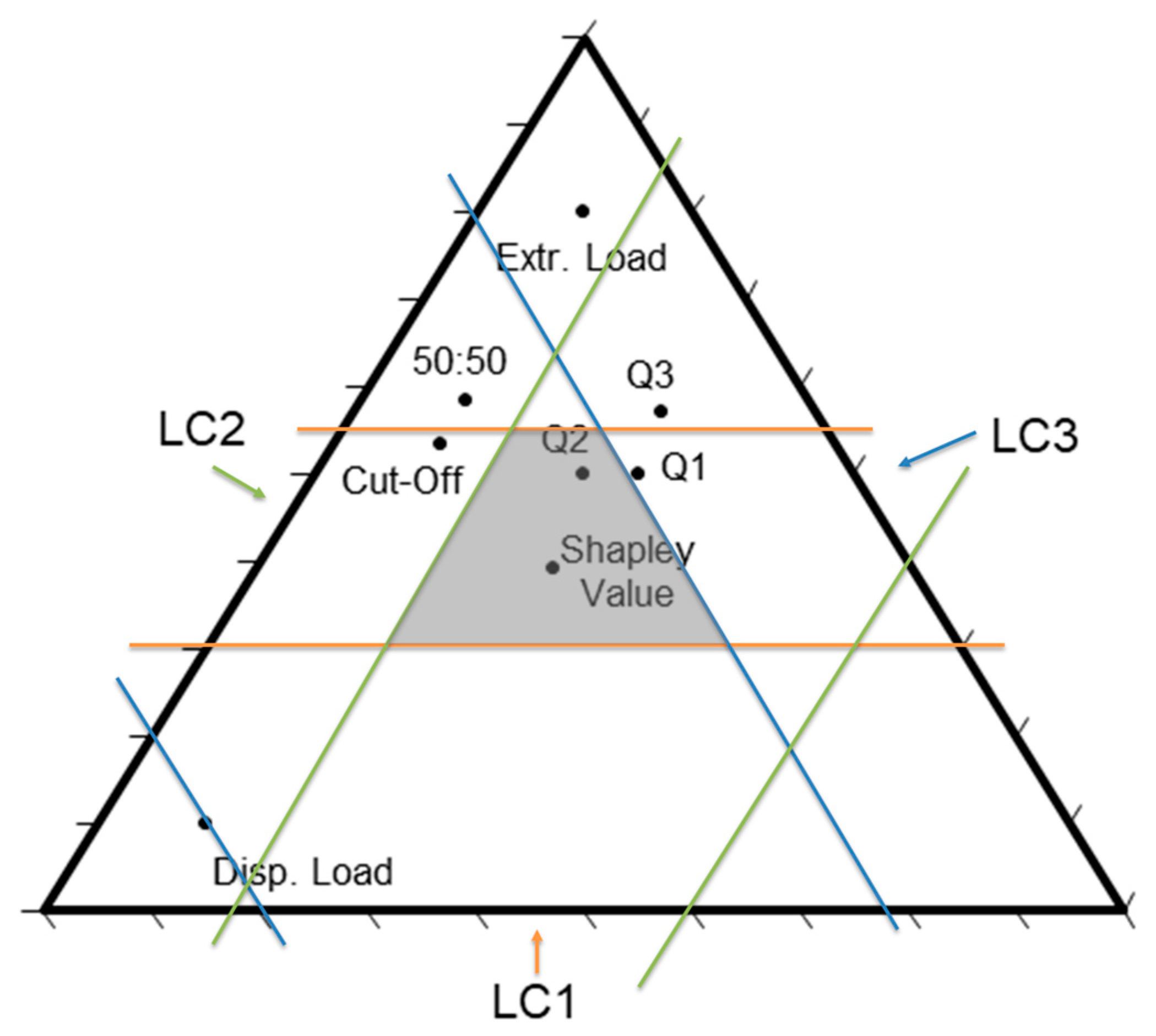
| Cut-Off | |
| 50:50 | |
| Extraction load | |
| Disposal load | |
| Quality degradation 1st option | |
| Quality degradation 2nd option | |
| Quality degradation 3rd option | |
| Value corrected substitution | |
| Environmental burden accredited to the i-th cascade cycle | |
| Recycling burdens from the i-1-th cascade cycle | |
| Environmental burden of the primary material input into the i-th cascade cycle | |
| Environmental burden of production in the i-th cascade cycle | |
| Environmental burden of product use in the i-th cascade cycle | |
| Environmental burden of waste management in the i-th cascade cycle | |
| Environmental burden accredited to recycling in the i-th cascade cycle | |
| Material price in the i-th cascade cycle | |
| Quality of the material at the beginning of the i-th cascade cycle | |
| Quality of the material at the beginning of the last cascade cycle |
| Publication | Allocation Type | Application Case | Type | ||||||
|---|---|---|---|---|---|---|---|---|---|
| CO | AM | QB | HM | MD | SE | ||||
| [7] | Ahmadi et al. 2017 | X | Li-ion batteries | Cascade | |||||
| [32] | Allacker et al. 2014 | X | General | OLR | |||||
| [30] | Allacker et al. 2017 | X | X | X | General | Cascade, OLR | |||
| [33] | Andreola et al. 2007 | X | CRT glass | OLR | |||||
| [24] | Ardente and Cellura 2011 | X | Food, Buildings, Biofuels | OLR | |||||
| [34] | Avadí 2020 | X | Compost | OLR | |||||
| [18] | Azapagic and Clift 1999b | X | X | General | Multi-output | ||||
| [35] | Azapagic and Clift 1999a | X | Boron production | Multi-output | |||||
| [36] | Bobba et al. 2018 | X | X | Li-ion batteries | OLR | ||||
| [37] | Boguski et al. 1994 | X | General | OLR | |||||
| [38] | Borg et al. 2001 | X | Building area | OLR | |||||
| [39] | Botas et al. 2017 | X | Lubricating oil | Multi-output | |||||
| [40] | Broeren et al. 2017 | X | Starch | Multi-output | |||||
| [19] | Chen et al. 2010 | X | Concrete | OLR | |||||
| [20] | Cherubini et al. 2011 | X | X | X | Biorefinery | Multi-output | |||
| [41] | Civancik-Uslu et al. 2019 | X | X | X | Packaging | OLR | |||
| [42] | Cobo et al. 2017 | X | X | General | OLR | ||||
| [43] | Corrado and Sala 2018 | X | X | Bioeconomy | Multi-output | ||||
| [21] | Dubreuil et al. 2010 | X | Metals | OLR | |||||
| [8] | Ekvall and Tillman 1997 | X | X | X | X | General | OLR | ||
| [44] | Ekvall 2000 | X | X | Paperboard, Newspaper | OLR | ||||
| [45] | Ekvall and Finnveden 2001 | X | X | X | X | General | Multi-output, OLR | ||
| [46] | Ekvall and Weidema 2004 | X | X | General | Multi-output, OLR | ||||
| [47] | Escamilla-Alvarado et al. 2017 | X | General | Multi-output, OLR | |||||
| [48] | Fernández-Dacosta et al. 2018 | X | X | CO2 | Cascade | ||||
| [49] | Ferreira et al. 2001 | X | X | Wood cascade | Cascade | ||||
| [50] | Finnveden et al. 2009 | X | General | Multi-output, OLR | |||||
| [51] | Forte et al. 2018 | X | Bioethanol | Multi-output | |||||
| [52] | Frees 2008 | X | X | Aluminum | OLR | ||||
| [53] | Frischknecht 2000 | X | CHP | Multi-output | |||||
| [54] | Frischknecht 2010 | X | X | Plastics, Metals | OLR | ||||
| [55] | Garofalo et al. 2017 | X | Tomatoes | Multi-output | |||||
| [12] | Gaudreault et al. 2012 | X | X | X | X | X | General | OLR | |
| [16] | Guinée et al. 2004b | X | X | Chemicals, Aluminum | Multi-output, OLR | ||||
| [56] | Heijungs and Frischknecht 1998 | X | Fuel production | Cascade | |||||
| [57] | Helmdach et al. 2017 | X | Bioeconomy | Multi-output | |||||
| [58] | Hermansson et al. 2019 | X | Lignin carbon fiber | Multi-output | |||||
| [15] | Höglmeier et al. 2015 | X | Wood | Cascade | |||||
| [59] | Hohenthal et al. 2019 | X | Paperboard | Cascade, OLR | |||||
| [60] | Iacovidou et al. 2017 | X | X | General | OLR | ||||
| [61] | Ilagan and Tan 2011 | X | Aluminum | OLR | |||||
| [28] | Johnson et al. 2013 | X | X | X | Metals | OLR | |||
| [62] | Jungmeier et al. 2002 | X | Wood | Cascade | |||||
| [2] | Kim et al. 1997 | X | General | Cascade | |||||
| [63] | Kim and Overcash 2000 | X | Ammonia | Multi-output | |||||
| [64] | Klöpffer 1996 | X | X | X | General | OLR | |||
| [65] | Koffler and Florin 2013 | X | Aluminum | OLR | |||||
| [66] | Koffler and Finkbeiner 2018 | X | X | X | General | Cascade | |||
| [67] | Li et al. 2017 | X | Hydropower | Multi-output | |||||
| [68] | Lindfors 1995 | X | X | X | General | OLR | |||
| [69] | Marinković et al. 2017 | X | Concrete recycling | OLR | |||||
| [70] | Martin et al. 2015 | X | X | X | EIP | Cascade | |||
| [71] | Marvuglia et al. 2010 | X | General | Multi-output, OLR | |||||
| [72] | Matsuno et al. 2007 | X | Steel | OLR | |||||
| [73] | McLaren et al. 2000 | X | General | Cascade | |||||
| [74] | Medeiros et al. 2017 | X | Furniture | Cascade | |||||
| [75] | Mehr et al. 2018 | Wood | Cascade | ||||||
| [76] | Morão and de Bie 2019 | X | X | Biocomoposites | Multi-output | ||||
| [77] | Nakano et al. 2018 | X | Wood products | Cascade | |||||
| [78] | Nakatani 2014 | X | General | Multi-output, OLR | |||||
| [27] | Nicholson et al. 2009 | X | X | X | General | OLR | |||
| [79] | Oldfield et al. 2018 | X | X | Food | Cascade | ||||
| [80] | Olofsson and Börjesson 2018 | X | General | OLR | |||||
| [81] | Parajuli et al. 2017 | X | Barley | Multi-output | |||||
| [82] | Parajuli et al. 2018 | X | Crops, livestock | Multi-output | |||||
| [83] | Paras and Pal 2018 | X | Clothing | OLR | |||||
| [84] | Paraskevas et al. 2013 | X | Aluminum | OLR | |||||
| [85] | Pawelzik et al. 2013 | X | Biomaterials | Cascade | |||||
| [86] | Perez-Gallardo et al. 2018 | X | X | PV modules | OLR | ||||
| [87] | Rasmussen et al. 2019 | X | Building area | OLR | |||||
| [88] | Reale et al. 2015 | X | X | Mechatronic products | OLR | ||||
| [89] | Rice et al. 2017 | X | Dairy products | Multi-output | |||||
| [90] | Richa et al. 2017 | X | Li-ion batteries | Cascade | |||||
| [91] | Risse et al. 2017 | X | Wood | Cascade | |||||
| [92] | Sandin and Peters 2018 | X | X | X | X | Textile | OLR | ||
| [29] | Schrijvers et al. 2016 | X | X | X | General | OLR | |||
| [93] | Seghetta and Goglio 2018 | X | X | Seaweed | Multi-output | ||||
| [94] | Sfez et al. 2019 | X | Sewage sludge | Cascade | |||||
| [95] | Shen et al. 2010 | X | X | X | PET bottle | OLR | |||
| [96] | Sommerhuber et al. 2017 | X | Wood-plastic composites | OLR | |||||
| [97] | Song et al. 2017 | X | Ore | Multi-output | |||||
| [1] | Suh et al. 2010 | X | X | General | OLR | ||||
| [98] | Suter et al. 2017 | X | Wood | OLR | |||||
| [99] | Timonen et al. 2019 | X | X | Bioeconomy | Multi-output | ||||
| [100] | Toniolo et al. 2017 | X | X | X | Plastic products | OLR | |||
| [22] | van der Harst et al. 2016b | X | X | X | X | X | Aluminum, Polystyrene | OLR | |
| [101] | Visintin et al. 2020 | X | X | X | Concrete | OLR | |||
| [102] | Vladimirov and Bica 2019 | X | X | Construction composites | OLR | ||||
| [103] | Vogtländer et al. 2001 | X | General | Cascade | |||||
| [104] | Weidema 2001 | X | General | Multi-output | |||||
| [105] | Weidema 2003 | X | General | Multi-output | |||||
| [23] | Werner 2000 | X | Aluminum | OLR | |||||
| [106] | Werner 2001 | X | X | X | X | Used wood | OLR | ||
| [107] | Werner et al. 2007 | X | X | X | X | Wood | Cascade | ||
| [108] | Williams et al. 2010 | X | Plastics | OLR | |||||
| [109] | Xia et al. 2020 | X | Concrete | OLR | |||||
| [110] | Yan and Holden 2018 | X | Dairy products | Multi-output | |||||
| [111] | Yang et al. 2020 | X | X | Li-ion batteries | Cascade | ||||
| [10] | Zamagni et al. 2008 | X | X | X | X | X | General | Multi-output, OLR | |
| [112] | Zhang et al. 2019 | X | X | X | Concrete | OLR | |||
| i | EPMi | ERi | EPi | EUi | EWi | MPi | Qi |
|---|---|---|---|---|---|---|---|
| 1 | 8 | 1.5 | 1 | 1 | 4 | 0.18 | 0.57 |
| 2 | 5 | 1.5 | 1 | 1 | 4 | 0.14 | 0.29 |
| 3 | 2 | 0 | 1 | 1 | 4 | 0.10 | 0.14 |
| π Permutations | δ Cost of the Coalitions | ||
|---|---|---|---|
| LC1 | LC2 | LC3 | |
| LC1,LC2,LC3 | 14.0 | 3.5 | 3.5 |
| LC1,LC3,LC2 | 14.0 | 3.5 | 3.5 |
| LC2,LC1,LC3 | 6.5 | 11.0 | 3.5 |
| LC2,LC3,LC1 | 6.5 | 11.0 | 3.5 |
| LC3,LC1,LC2 | 9.5 | 3.5 | 8.0 |
| LC3,LC2,LC1 | 6.5 | 6.5 | 8.0 |
| Mean | 9.5 | 6.5 | 5.0 |
| Input Values (Costs of coalitions) | |
|---|---|
| Scenario | Env. Burden |
| LC1 | 14.0 |
| LC2 | 11.0 |
| LC3 | 8.0 |
| LC1+LC2 | 17.5 |
| LC1+LC3 | 17.5 |
| LC2+LC3 | 14.5 |
| LC1+LC2+LC3 | 21.0 |
| Rationality | Marginality | ||
|---|---|---|---|
| c(LC1) | ≤14 | c(LC1) ≥ c(N)-c(LC2+LC3) | =6.5 |
| c(LC2) | ≤11 | c(LC2) ≥ c(N)-c(LC1+LC3) | =3.5 |
| c(LC3) | ≤8 | c(LC3) ≥ c(N)-c(LC2+LC1) | =3.5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rehberger, M.; Hiete, M. Allocation of Environmental Impacts in Circular and Cascade Use of Resources—Incentive-Driven Allocation as a Prerequisite for Cascade Persistence. Sustainability 2020, 12, 4366. https://doi.org/10.3390/su12114366
Rehberger M, Hiete M. Allocation of Environmental Impacts in Circular and Cascade Use of Resources—Incentive-Driven Allocation as a Prerequisite for Cascade Persistence. Sustainability. 2020; 12(11):4366. https://doi.org/10.3390/su12114366
Chicago/Turabian StyleRehberger, Max, and Michael Hiete. 2020. "Allocation of Environmental Impacts in Circular and Cascade Use of Resources—Incentive-Driven Allocation as a Prerequisite for Cascade Persistence" Sustainability 12, no. 11: 4366. https://doi.org/10.3390/su12114366
APA StyleRehberger, M., & Hiete, M. (2020). Allocation of Environmental Impacts in Circular and Cascade Use of Resources—Incentive-Driven Allocation as a Prerequisite for Cascade Persistence. Sustainability, 12(11), 4366. https://doi.org/10.3390/su12114366