Pricing and Low-Carbon Investment Decisions in an Emission Dependent Supply Chain under a Carbon Labelling Scheme
Abstract
1. Introduction
- (1)
- What is the impact of a carbon labelling scheme on the key decisions of supply chain players?
- (2)
- Considering consumers’ environmental awareness, is it profitable for a manufacturer to invest in low-carbon production?
- (3)
- If yes, how do the manufacturer and the retailer make decisions (the price and the product carbon footprint) for the carbon-labelled products?
- (1)
- The decision-making model without considering a carbon labelling scheme (denoted Model NL);
- (2)
- The manufacturer is subject to a carbon labelling scheme (denoted Model LNR);
- (3)
- The manufacturer invests in carbon footprint reduction to mitigate the impact of a carbon labelling scheme (denoted Model LR).
2. Literature Review
3. Problem Notations and Assumption
4. Analytical Models
4.1. Manufacturer and Retailer Pricing Decisions without a Carbon Labelling Scheme (Model NL)
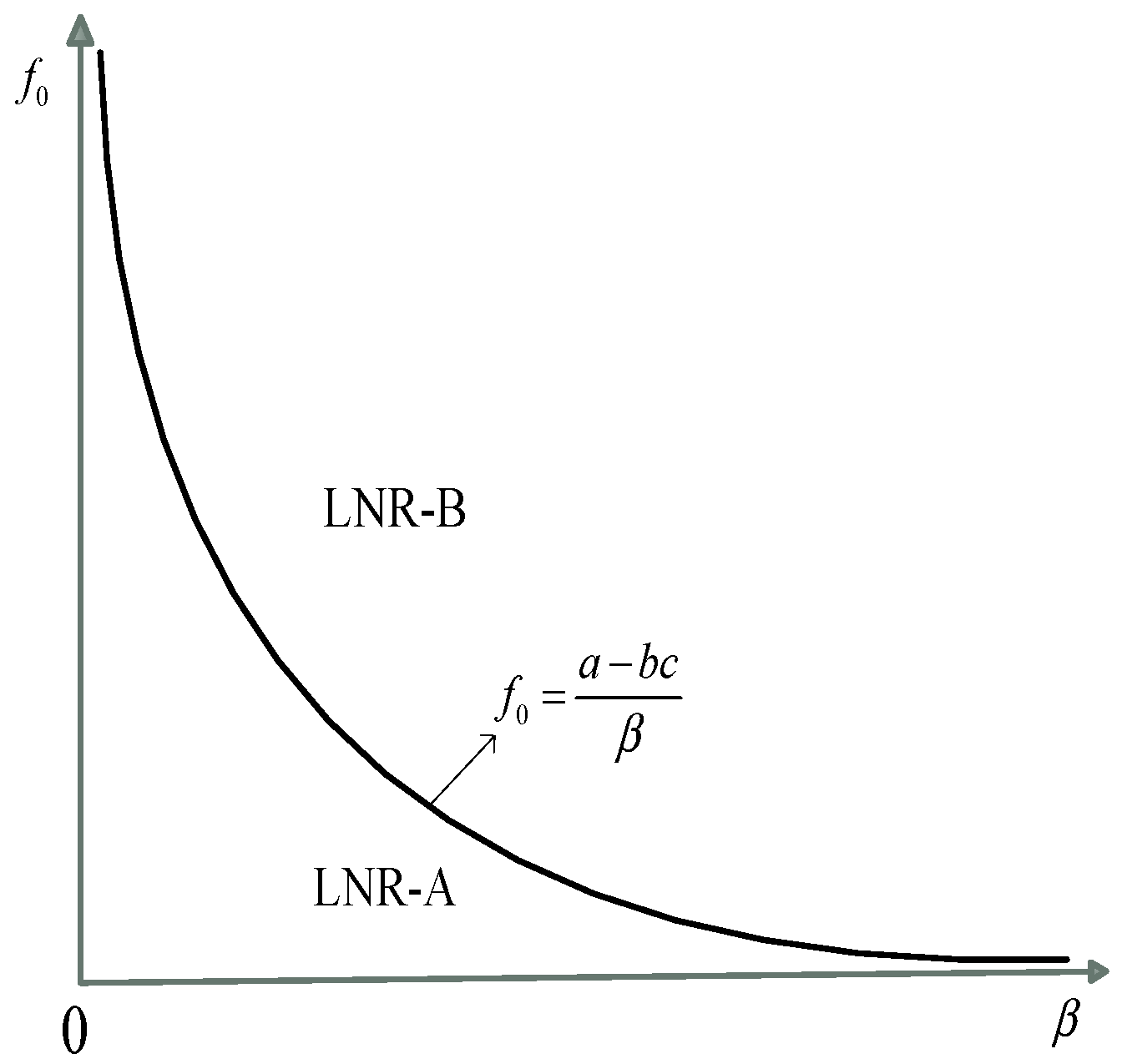
4.2. Manufacturer and Retailer’s Pricing Decisions with Carbon Labelling Scheme (Model LNR)
- (1)
- LNR-A (i.e., ),
- (2)
- LNR-B (i.e., ), which can be viewed as a function of “” and “” and is shown in Figure 2.
- (1)
- In region LNR-A,,,,,,;
- (2)
- To ensure positive market demand, the initial product carbon footprint must satisfy. Therefore, in region LNR-B where, the market demand is zero, which means the supply chain members exit the market. This occurs because consumer environmental awareness is very high and the product with high initial carbon footprint will be rejected from the market.
4.3. Manufacturer and Retailer Pricing and PCF-Reduction Investment Decisions with a Carbon Labelling Scheme (Model LR)
- (1)
- LR-1-A (i.e., , ),
- (2)
- LR-1-B (i.e., , ),
- (3)
- LR-1-C (i.e., , ),
- (4)
- LR-2-A (i.e., , ), and
- (5)
- LR-2-B (i.e., , ), which can be viewed as a function of “” and “” and shown in Figure 3.
- (1)
- In region LR-1-A and region LR-2-A,,,,,,,;
- (2)
- In region LR-1-B,,,,,,,;
- (3)
- In region LR-1-C and region LR-2-B, the supply chain members exit the market.
- (1)
- Only when,,,,. Otherwise,,,,;
- (2)
- Only when,. Otherwise,;
- (3)
- Only when,. Otherwise,.
5. Comparisons of the Equilibrium Outcomes among the Three Models
5.1. Model LNR vs. Model NL
- (1)
- When,,,,,and;
- (2)
- When, the chain members exit the market.
- (1)
- When the initial product carbon footprint is smaller than the threshold value (), the optimal wholesale price, the retail price, the market demand, the total carbon emissions, and the manufacturer’s and retailer’s profits are lower than those without a carbon labelling scheme.
- (2)
- When the initial product carbon footprint is larger than the threshold value (), the chain members exit the market.
5.2. Model LR vs. Model LNR
- (1)
- Whenand, orand,,,,,,;
- (2)
- Whenand,,,,①,,;Note ①: When,, otherwise.
- (3)
- Whenand, the chain members will not exit the market.
- (1)
- When the initial product carbon footprint and the level of consumer environmental awareness are relatively small ( and ) or the initial product carbon footprint is relatively small () and the level of consumer environmental awareness is relatively high (), both the manufacturer and the retailer can raise their prices. The market demands and the manufacturer’s and retailer’s profits will be increased relative to the scenario without investment in PCF-reduction, and total carbon emissions will be reduced because of the manufacturer’s investment in PCF-reduction.
- (2)
- When the initial product carbon footprint is intermediate () and the level of consumer environmental awareness is relatively small (), both the manufacturer and the retailer can raise their prices. The market demand and the manufacturer’s and retailer’s profits will be increased relative to the situation without investment in PCF-reduction. When the initial product carbon footprint is relatively small, total carbon emissions will be reduced; otherwise, total carbon emissions will be increased.
- (3)
- When the level of consumers’ environmental awareness is relatively high () and the initial product carbon footprint is intermediate (), the chain members will not exit the market.
6. Numerical Examples
- (1)
- In Model LNR (the manufacturer does not make an investment in PCF-reduction under a carbon labelling scheme), when the initial product carbon footprint or the level of consumer environmental awareness is relatively small (lower), the equilibrium wholesale price, the retail price, the market demand, the total carbon emissions, and the manufacturer’s and retailer’s profits will decrease with increasing consumer environmental awareness; otherwise, the chain members will exit the market.
- (2)
- In Model LR (the manufacturer makes an investment in PCF-reduction under a carbon labelling scheme), when the initial product carbon footprint is very small, the equilibrium wholesale price, the retail price, the market demand, and the retailer’s profits are the same as the equilibrium outcomes in Model NL (no carbon labelling). In addition, the product carbon footprint and the total carbon emissions are reduced to zero, and the manufacturer’s profit is reduced slightly owing to the investment in PCF-reduction; however, it is higher than that of Model NL. Interestingly, when the initial product carbon footprint is at the intermediate level and the level of consumer environmental awareness is very high, the equilibrium outcomes are the same as when the initial product carbon footprint is very small. More importantly, the chain members do not exit the market, in contrast to Model LNR. However, when the initial product carbon footprint and the level of consumer environmental awareness is very large (high), the chain members also exit the market.
- (3)
- Total carbon emissions in Model LNR and Model LNR are lower than that in Model NL, which means that total carbon emissions will decrease after the government implements the carbon labelling scheme, regardless of whether the manufacturer makes an investment in PCF-reduction.
- (4)
- Compared to Model NL, the manufacturer’s profit will surely decrease and the retailer’s profit may or may not decrease after the government implements the carbon labelling scheme.
7. Conclusions and Suggestions for Further Research
- (1)
- Regardless of whether or not the manufacturer makes an investment in PCF-reduction under the carbon labelling scheme, the total carbon emissions are lower than without the carbon labelling scheme. The conclusion may illustrate the role of a carbon labelling scheme in combating global climate change. Furthermore, the total carbon emissions are highly related to consumer environmental awareness.
- (2)
- Under a carbon labelling scheme, the manufacturer’s and retailer’s optimal decisions depend on two critical values (the initial product carbon footprint and the level of consumer environmental awareness). Given that the manufacturer makes an investment in PCF-reduction, when the initial product carbon footprint is very small, the product carbon footprint can be reduced to near zero, and the optimal pricing strategies can remain the same as the benchmark without a carbon labelling scheme. When the initial product carbon footprint is intermediate and the consumer environmental-awareness level is relatively low, both should also lower their prices. In addition, there is a threshold. When the initial product carbon footprint is smaller than the threshold, the product carbon footprint will decrease with increasing consumer environmental awareness, However, when the initial product carbon footprint is larger than the threshold (), the product carbon footprint will be increased with increasing consumer environmental awareness.
- (3)
- Under a carbon labelling scheme, the manufacturer’s profit is lower than without the carbon labelling scheme. In addition, the retailer’s profit under the carbon labelling scheme may remain unchanged or be lower than without the carbon labelling scheme; this will depend on whether the manufacturer makes an investment in PCF-reduction. This conclusion may explain why the voices of the retailers participating in carbon labelling schemes have been louder than those of the manufacturers in the years since carbon labelling schemes have been implemented.
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
- (1)
- When , to ensure , we obtain ; therefore, the optimal product carbon footprint . Substituting this equation into , we obtain the optimal wholesale price . Substituting both into , we obtain . If , that is , the optimal product carbon footprint , , and . If , that is , the optimal product carbon footprint . However, the market demand is negative under this condition; therefore, the chain members must exit the market.
- (2)
- When , if , then ; if , then . Furthermore, comparing the two profit functions, we note that ; therefore, can attain the maximum value at and when . Otherwise, the manufacturer’s profit is negative, and the chain members must exit the market.
- (1)
- , , ,, ,;
- (2)
- ,,,. □
References
- Boardman, B. Carbon labelling: Too complex or will it transform our buying? Significance 2008, 5, 168–171. [Google Scholar] [CrossRef]
- Cohen, M.; Vandenbergh, M.P. The potential role of carbon labeling in a green economy. Energy Econ. 2012, 34, S53–S63. [Google Scholar] [CrossRef]
- Wu, P.; Feng, Y.; Pienaar, J.; Xiad, B. A review of benchmarking in carbon labelling schemes for building materials. J. Clean. Prod. 2015, 109, 108–117. [Google Scholar] [CrossRef]
- Jeroen, C.; Berghab, V.; Truffer, B.; Kallis, G. Environmental innovation and societal transitions: Introduction and overview. Environ. Innov. Soc. Transit. 2011, 1, 1–23. [Google Scholar]
- Hornibrook, S.; May, C.; Fearne, A. Sustainable development and the consumer: Exploring the role of carbon labelling in retail supply chains. Bus. Strategy Environ. 2015, 24, 266–276. [Google Scholar] [CrossRef]
- Liu, T.; Wang, Q.; Su, B. A review of carbon labeling: Standards, implementation, and impact. Renew. Sustain. Energy Rev. 2016, 53, 68–79. [Google Scholar] [CrossRef]
- Tan, M.; Tan, R.; Khoo, H. Prospects of carbon labelling—A life cycle point of view. J. Clean. Prod. 2014, 72, 76–88. [Google Scholar] [CrossRef]
- Brenton, P.; Edwards-Jones, G.; Jensen, M.F. Carbon labelling and low-income country exports: A review of the development issues. Dev. Policy Rev. 2009, 27, 243–267. [Google Scholar] [CrossRef]
- Caro, F.; Corbett, C.J.; Tan, T.; Zuidwijk, R. Double counting in supply chain carbon footprinting. Manuf. Serv. Oper. Manag. 2013, 15, 545–558. [Google Scholar] [CrossRef]
- Ji, J.; Zhang, Z.; Yang, L. Carbon emission reduction decisions in the retail-/dual-channel supply chain with consumers’ preference. J. Clean. Prod. 2017, 141, 852–867. [Google Scholar] [CrossRef]
- Bocken, N.M.P.; Allwood, J.M. Strategies to reduce the carbon footprint of consumer goods by influencing stakeholders. J. Clean. Prod. 2012, 35, 118–129. [Google Scholar] [CrossRef]
- Burnetas, A.; Ritchken, P. Option pricing with downward-sloping demand curves: The case of supply chain options. Manag. Sci. 2005, 51, 566–580. [Google Scholar] [CrossRef]
- Sajadieh, M.; Jokar, M. Optimizing shipment, ordering and pricing policies in a two-stage supply chain with price-sensitive demand. Transp. Res. Part E Logist. Transp. Rev. 2009, 45, 564–571. [Google Scholar] [CrossRef]
- Xue, M.; Tang, W.; Zhang, J. Supply chain pricing and coordination with markdown strategy in the presence of conspicuous consumers. Int. Trans. Oper. Res. 2016, 23, 1051–1065. [Google Scholar] [CrossRef]
- Ghosh, D.; Shah, J. Supply chain analysis under green sensitive consumer demand and cost sharing contract. Int. J. Prod. Econ. 2015, 164, 319–329. [Google Scholar] [CrossRef]
- Darby, M.R.; Karni, E. Free competition and the optimal amount of fraud. J. Law Econ. 1973, 16, 67–88. [Google Scholar] [CrossRef]
- Hogan, L.; Thorpe, S. Issues in Food Miles and Carbon Labeling; Australian Bureau of Agricultural and Resource Economics: Canberra, Australia, 2009.
- Karl, H.; Orwat, C. Economic aspects of environmental labeling. In The International Yearbook of Environmental and Resource Economics 1999/2000; Folmer, H., Tietenberg, T.H., Eds.; Edward Elgar: Cheltenham, UK, 1999. [Google Scholar]
- Loch, C.; Wu, Y. Social preferences and supply chain performance: An experimental study. Manag. Sci. 2008, 54, 1835–1849. [Google Scholar] [CrossRef]
- Young, W.; Hwang, K.; McDonald, S.; Oates, C.J. Sustainable consumption: Green consumer behavior when purchasing products. Sustain. Dev. 2010, 18, 20–31. [Google Scholar] [CrossRef]
- Blengini, G.; Shields, D. Green labels and sustainability reporting: Overview of the building products supply chain in Italy. Manag. Environ. Qual. 2010, 21, 77–493. [Google Scholar]
- Tang, C.; Zhou, S. Research advances in environmentally and socially sustainable operations. Eur. J. Oper. Res. 2012, 223, 585–594. [Google Scholar] [CrossRef]
- Vanclay, J.; Shortiss, J.; Aulsebrook, S. Customer response to carbon labelling of groceries. J. Consum. Policy 2011, 34, 153–160. [Google Scholar] [CrossRef]
- Zhao, R.; Zhong, S. Carbon labelling influences on consumers’ behaviour: A system dynamics approach. Ecol. Indic. 2015, 51, 98–106. [Google Scholar] [CrossRef]
- Baddeley, S.; Cheng, P.; Wolfe, R. Trade Policy Implications of Carbon Labels on Food; Canadian Agricultural Trade Policy and Competitiveness Research Network Commissioned Paper. Available online: https://ageconsearch.umn.edu/bitstream/122740/2/Commissioned%20Paper%202011-04%20Wolfe%20-%202.pdf (accessed on 16 April 2018).
- Upham, P.; Dendler, L.; Bleda, M. Carbon labelling of grocery products: Public perceptions and potential emissions reductions. J. Clean. Prod. 2011, 19, 348–355. [Google Scholar] [CrossRef]
- Schaefer, F.; Michael, B. Opportunities and challenges of carbon footprint, climate or CO2 labelling for horticultural products. Erwerbs-Obstbau 2014, 56, 73–80. [Google Scholar] [CrossRef]
- Yenipazarli, A. Managing new and remanufactured products to mitigate environmental damage under emissions regulation. Eur. J. Oper. Res. 2016, 249, 117–130. [Google Scholar] [CrossRef]
- Palmer, K.; Oates, W.; Portney, P. Tightening environmental standards: The benefit-cost or the no-cost paradigm? J. Econ. Perspect. 1995, 9, 119–132. [Google Scholar] [CrossRef]
- Pagell, M.; Wu, Z. Building a more complete theory of sustainable supply chain management using case studies of 10 exemplars. J. Supply Chain Manag. 2009, 45, 37–56. [Google Scholar] [CrossRef]
- King, A.; Lenox, M. Exploring the locus of profitable pollution reduction. Manag. Sci. 2002, 48, 289–299. [Google Scholar] [CrossRef]
- Yalabik, B.; Fairchild, R. Customer, regulatory, and competitive pressure as drivers of environmental innovation. Int. J. Prod. Econ. 2011, 131, 519–527. [Google Scholar] [CrossRef]
- Sundarakani, B.; De Souza, R.; Goh, M.; Wagner, S.; Manikandan, S. Modeling carbon footprints across the supply chain. Int. J. Prod. Econ. 2010, 128, 43–50. [Google Scholar] [CrossRef]
- Jiang, Y.; Klabjan, D. Optimal Emissions Reduction Investment under Greenhouse Gas Emissions Regulations; Working Paper; Northwestern University: Evanston, IL, USA, 2012. [Google Scholar]
- Chang, X.; Xia, H.; Zhu, H. Production decisions in a hybrid manufacturing–remanufacturing system with carbon cap and trade mechanism. Int. J. Prod. Econ. 2015, 162, 160–173. [Google Scholar] [CrossRef]
- Benjaafar, S.; Li, Y.; Daskin, M. Carbon footprint and the management of supply chains: Insights from simple models. IEEE Trans. Autom. Sci. Eng. 2013, 10, 99–116. [Google Scholar] [CrossRef]
- Chen, X.; Benjaafar, S.; Elomri, A. The carbon-constrained EOQ. Oper. Res. Lett. 2013, 41, 172–179. [Google Scholar] [CrossRef]
- Guenther, M.; Saunders, C.; Tait, P. Carbon labeling and consumer attitudes. Carbon Manag. 2012, 3, 445–455. [Google Scholar] [CrossRef]
- Hartikainen, H.; Roininen, T.; Katajajuuri, J. Finnish consumer perceptions of carbon footprints and carbon labelling of food products. J. Clean. Prod. 2014, 73, 285–293. [Google Scholar] [CrossRef]
- Nishino, N.; Akai, K.; Tamura, H. Product differentiation and consumer’s purchase decision-making under carbon footprint scheme. Procedia CIRP 2014, 16, 116–121. [Google Scholar] [CrossRef][Green Version]
- Leng, M.; Parlar, M. Game-theoretic analyses of decentralized assembly supply chains: Non-cooperative equilibria vs. coordination with cost-sharing contracts. Eur. J. Oper. Res. 2010, 204, 96–104. [Google Scholar] [CrossRef]
- Jamali, M.; Rasti-Barzoki, M. A game theoretic approach for green and non-green product pricing in chain-to-chain competitive sustainable and regular dual-channel supply chains. J. Clean. Prod. 2018, 170, 1029–1043. [Google Scholar] [CrossRef]
- Du, S.; Zhu, J.; Jiao, H. Game-theoretical analysis for supply chain with consumer preference to low carbon. Int. J. Prod. Res. 2015, 53, 3753–3768. [Google Scholar] [CrossRef]
- Yang, H.; Zhang, X.; Meng, Q. Stackelberg games and multiple equilibrium behaviors on networks. Transp. Res. Part B Methodol. 2007, 41, 841–861. [Google Scholar] [CrossRef]
- Wu, C.; Zhao, Q.; Xi, M. A retailer-supplier supply chain model with trade credit default risk in a supplier-Stackelberg game. Comput. Ind. Eng. 2017, 112, 568–575. [Google Scholar] [CrossRef]
- Chua, F.; Vasnani, N.; Pacio, L. A Stackelberg game in multi-period planning of make-to-order production system across the supply chain. J. Manuf. Syst. 2018, 46, 231–246. [Google Scholar] [CrossRef]

| Symbols and Notation | Explanation |
|---|---|
| the unit wholesale price, a decision variable | |
| the unit retail price, a decision variable | |
| the product carbon footprint (PCF) after the manufacturer makes an investment in PCF-reduction, a decision variable | |
| the market demand | |
| the basic market scale of consumers | |
| the sensitivity of demand to the retailer’s price | |
| the unit production cost | |
| the initial product carbon footprint before the manufacturer makes an investment in PCF-reduction | |
| the sensitivity of consumers to the PCF, here we can interpret it as the consumer environmental-awareness level | |
| the cost factor when the manufacturer makes an investment in PCF-reduction | |
| total carbon emissions | |
| the manufacturer’s profit | |
| the retailer’s profit |
| Parameters | Model LNR | Model LR | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.5 | 1 | 39.8 | 49.6 | 9.9 | 4.9 | 195 | 97.5 | 40 | 0 | 50 | 10 | 0 | 198 | 100 |
| 4 | 39 | 48.5 | 9.5 | 4.8 | 180.5 | 90.3 | 40 | 0 | 50 | 10 | 0 | 198 | 100 | |
| 7 | 38.3 | 47.3 | 9.1 | 4.7 | 166.5 | 83.3 | 40 | 0 | 50 | 10 | 0 | 198 | 100 | |
| 10 | 37.5 | 46.3 | 8.8 | 4.4 | 153.1 | 76.6 | 40 | 0 | 50 | 10 | 0 | 198 | 100 | |
| 4.5 | 1 | 37.3 | 46.6 | 8.9 | 39.9 | 157.5 | 78.8 | 38. | 3.9 | 47.4 | 9 | 40.6 | 160 | 81.3 |
| 4 | 31 | 36.5 | 5.5 | 24.8 | 60.5 | 30.3 | 34.8 | 2.7 | 42 | 5.5 | 14.7 | 80.7 | 53.8 | |
| 7 | 24.3 | 26.4 | 2.1 | 9.6 | 9 | 4.5 | 38.1 | 0.5 | 47.2 | 9.1 | 4.8 | 38.5 | 82.3 | |
| 10 | N/A | N/A | N/A | N/A | N/A | N/A | 40 | 0 | 50 | 10 | 0 | 198 | 100 | |
| 8.5 | 1 | 35.8 | 43.6 | 7.9 | 66.9 | 124 | 62 | 36 | 8 | 44 | 8 | 64 | 126 | 64 |
| 4 | 23 | 24.5 | 1.5 | 12.8 | 4.5 | 2.3 | 24 | 8 | 26 | 2 | 16 | 6 | 4 | |
| 7 | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | |
| 10 | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | N/A | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, Y.; Sun, H.; Jia, F.; Koh, L. Pricing and Low-Carbon Investment Decisions in an Emission Dependent Supply Chain under a Carbon Labelling Scheme. Sustainability 2018, 10, 1238. https://doi.org/10.3390/su10041238
Cheng Y, Sun H, Jia F, Koh L. Pricing and Low-Carbon Investment Decisions in an Emission Dependent Supply Chain under a Carbon Labelling Scheme. Sustainability. 2018; 10(4):1238. https://doi.org/10.3390/su10041238
Chicago/Turabian StyleCheng, Yonghong, Hui Sun, Fu Jia, and Lenny Koh. 2018. "Pricing and Low-Carbon Investment Decisions in an Emission Dependent Supply Chain under a Carbon Labelling Scheme" Sustainability 10, no. 4: 1238. https://doi.org/10.3390/su10041238
APA StyleCheng, Y., Sun, H., Jia, F., & Koh, L. (2018). Pricing and Low-Carbon Investment Decisions in an Emission Dependent Supply Chain under a Carbon Labelling Scheme. Sustainability, 10(4), 1238. https://doi.org/10.3390/su10041238





