Author Contributions
Conceptualization, R.F.P., R.M. and M.R.; methodology, R.F.P. and R.M.; software, R.F.P., R.M. and R.B.; validation, R.M., M.R. and V.C.; formal analysis, R.F.P. and R.M.; investigation, R.F.P., R.M. and R.B.; resources, M.R. and G.G.; data curation, R.F.P. and R.B.; writing—original draft preparation, R.F.P., R.M., R.B., M.R. and V.C.; writing—review and editing, R.F.P., R.M., R.B., M.R., V.C. and G.G.; visualization, R.F.P., R.M. and R.B.; supervision, M.R., V.C. and G.G. All authors have read and agreed to the published version of the manuscript.
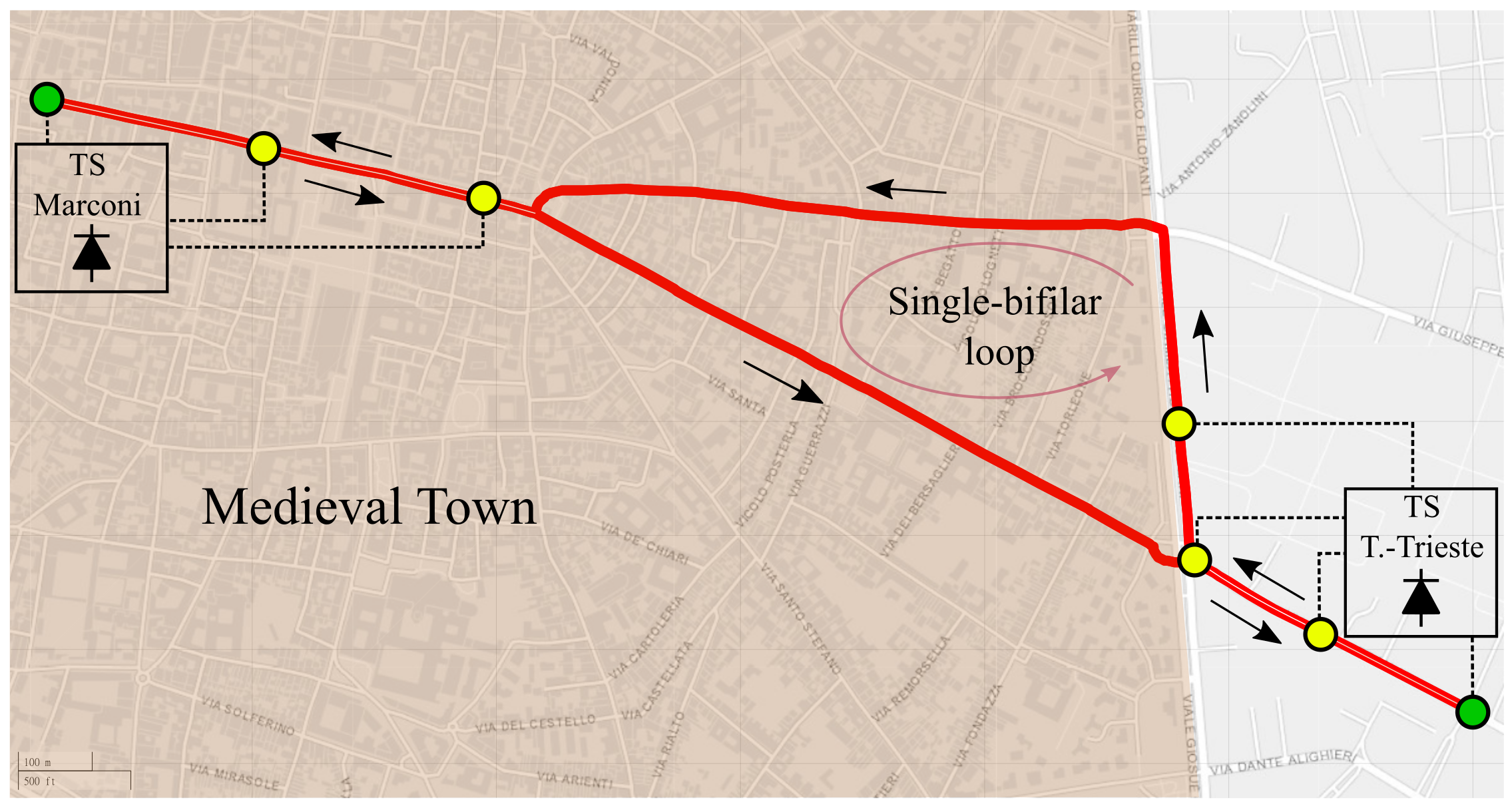
Figure 1.
Topology of FS MTT. Green and yellow circles represent the location of feeders and reinforcement feeders, respectively. Dashed lines represent the connection with the TSs. Arrows indicate the trolleybuses’ travel direction.
Figure 1.
Topology of FS MTT. Green and yellow circles represent the location of feeders and reinforcement feeders, respectively. Dashed lines represent the connection with the TSs. Arrows indicate the trolleybuses’ travel direction.
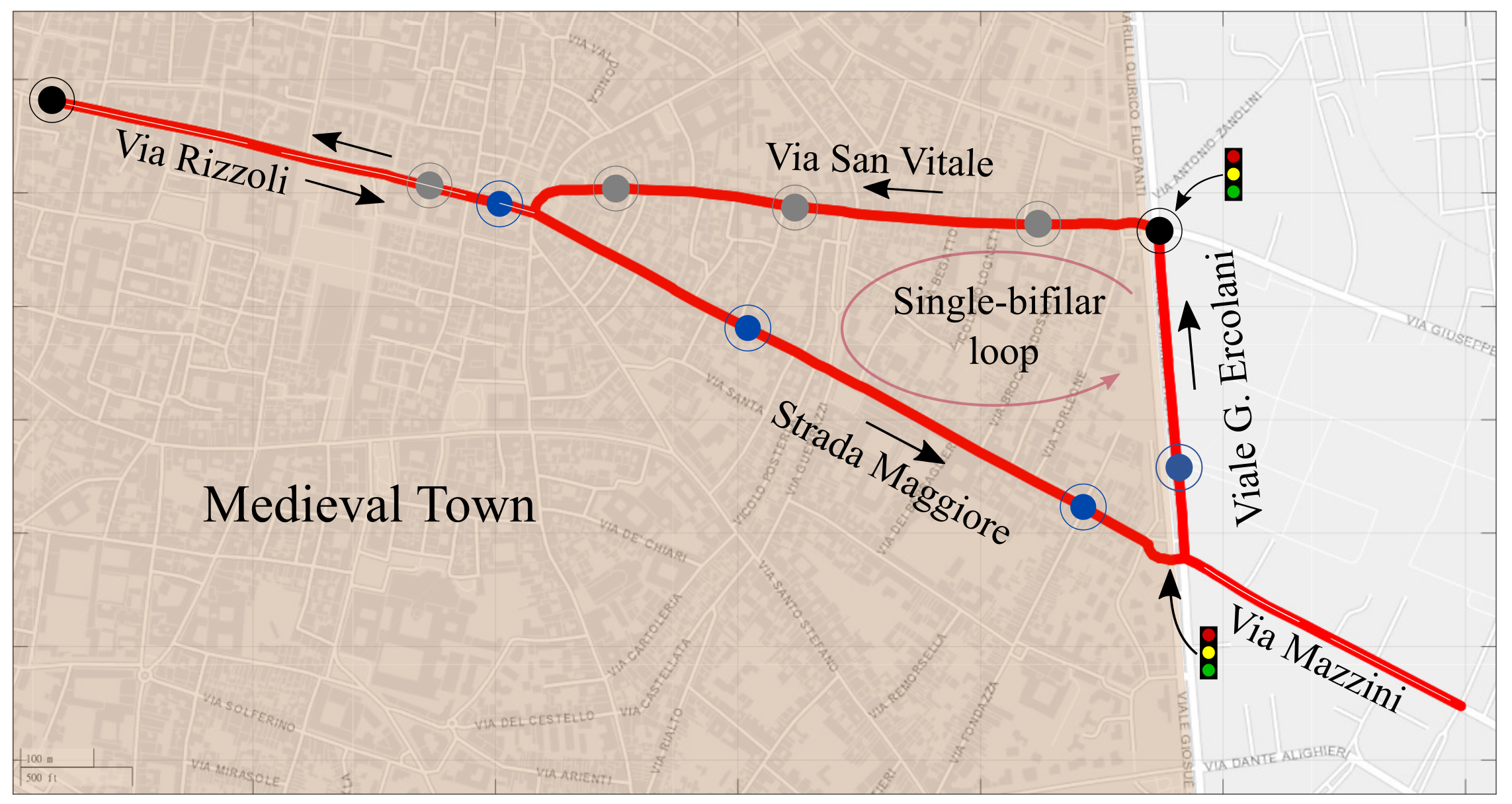
Figure 2.
Bus stops of line 14 in FS MTT. Initial and final stops for outward and return journey (black points); intermediate stops for outward journey (blue); intermediate stops for return journey (grey). Arrows indicate the trolleybuses’ travel direction.
Figure 2.
Bus stops of line 14 in FS MTT. Initial and final stops for outward and return journey (black points); intermediate stops for outward journey (blue); intermediate stops for return journey (grey). Arrows indicate the trolleybuses’ travel direction.
Figure 3.
Bus stops scheme for line 14. Initial and final stops (black points) for outward (top) and return (bottom) journeys; intermediate stops for outward journey (blue); intermediate stops for return journey (grey).
Figure 3.
Bus stops scheme for line 14. Initial and final stops (black points) for outward (top) and return (bottom) journeys; intermediate stops for outward journey (blue); intermediate stops for return journey (grey).
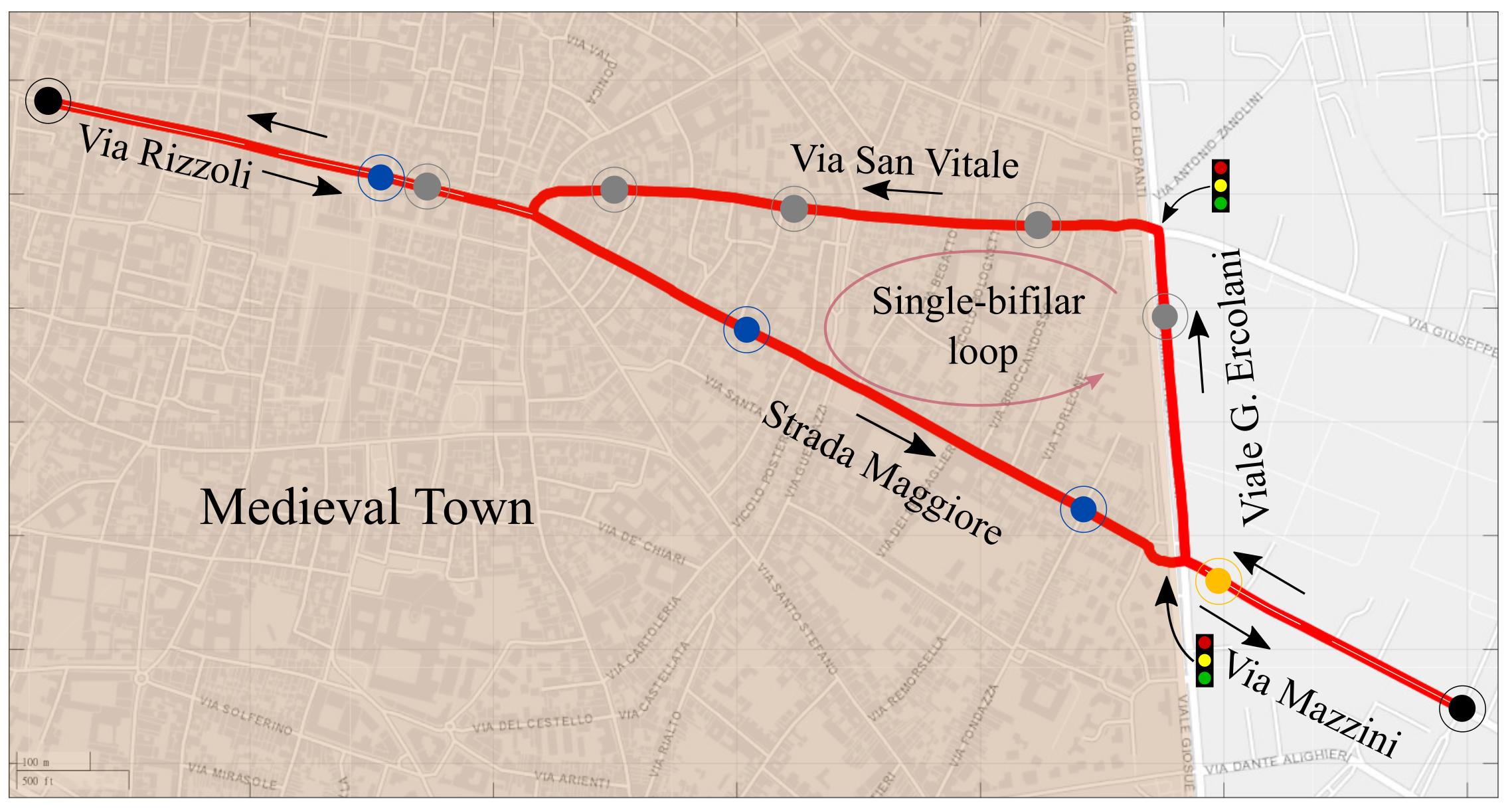
Figure 4.
Bus stops of line 15 in FS MTT. Initial and final stops for outward and return journey (black points); intermediate stops for outward journey (blue); intermediate stops for return journey (grey); common stop for outward and return journey (yellow). Arrows indicate the trolleybuses’ travel direction.
Figure 4.
Bus stops of line 15 in FS MTT. Initial and final stops for outward and return journey (black points); intermediate stops for outward journey (blue); intermediate stops for return journey (grey); common stop for outward and return journey (yellow). Arrows indicate the trolleybuses’ travel direction.
Figure 5.
Bus stops scheme for line 15. Initial and final stops (black points) for outward (top) and return (bottom) journeys; intermediate stops for outward journey (blue); intermediate stops for return journey (grey); common stop for outward and return journey (yellow).
Figure 5.
Bus stops scheme for line 15. Initial and final stops (black points) for outward (top) and return (bottom) journeys; intermediate stops for outward journey (blue); intermediate stops for return journey (grey); common stop for outward and return journey (yellow).
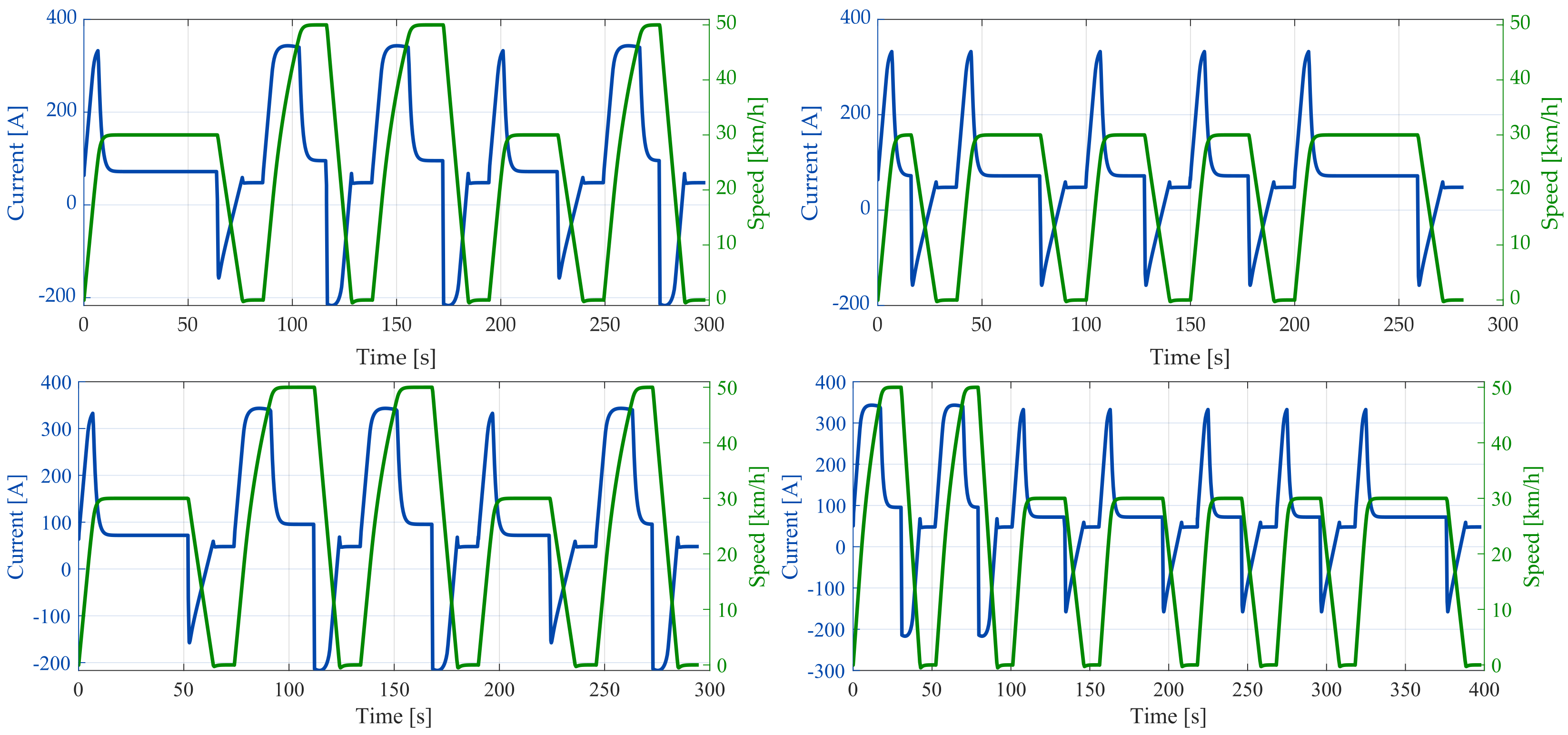
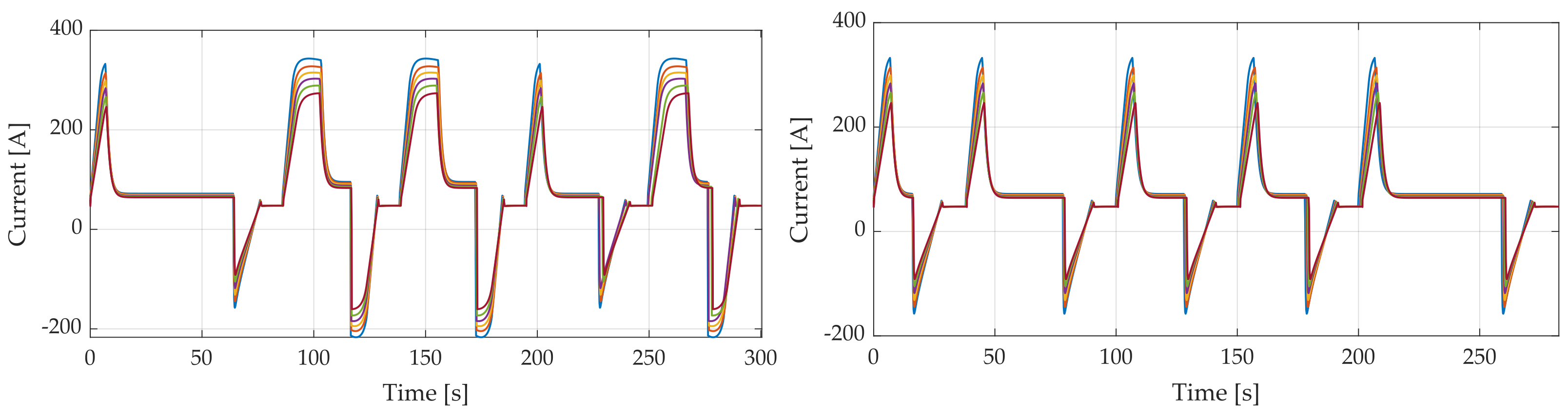
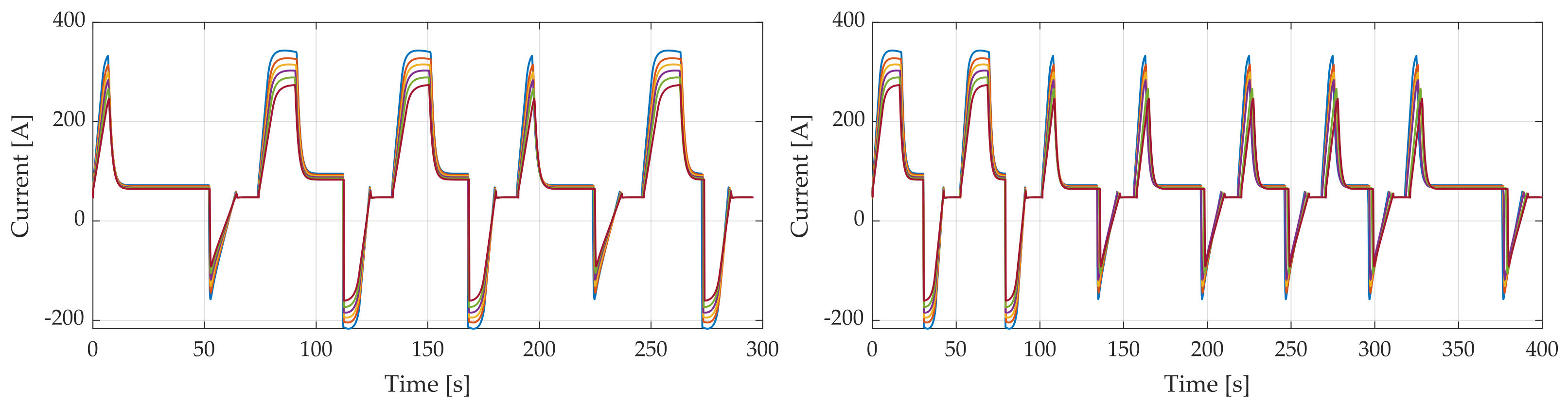
Figure 6.
Traction diagram speed (green traces) and currents (blue traces) of conventional trolleybuses covering the paths of line 14 (upper figures) and line 15 (bottom figures). Outward journeys are on the left side; return journeys on the right side.
Figure 6.
Traction diagram speed (green traces) and currents (blue traces) of conventional trolleybuses covering the paths of line 14 (upper figures) and line 15 (bottom figures). Outward journeys are on the left side; return journeys on the right side.
Figure 7.
Traction diagram speed (green traces) and currents (blue traces) of IMC trolleybuses covering the paths of line 14 (upper figures) and line 15 (bottom figures). Outward journeys are on the left side; return journeys on the right side.
Figure 7.
Traction diagram speed (green traces) and currents (blue traces) of IMC trolleybuses covering the paths of line 14 (upper figures) and line 15 (bottom figures). Outward journeys are on the left side; return journeys on the right side.
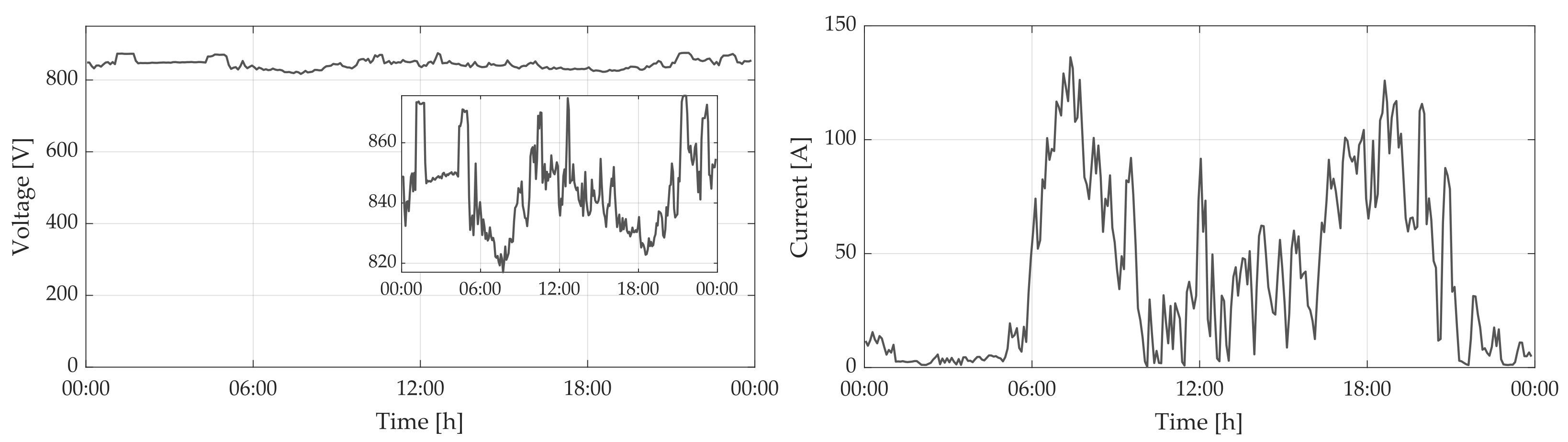
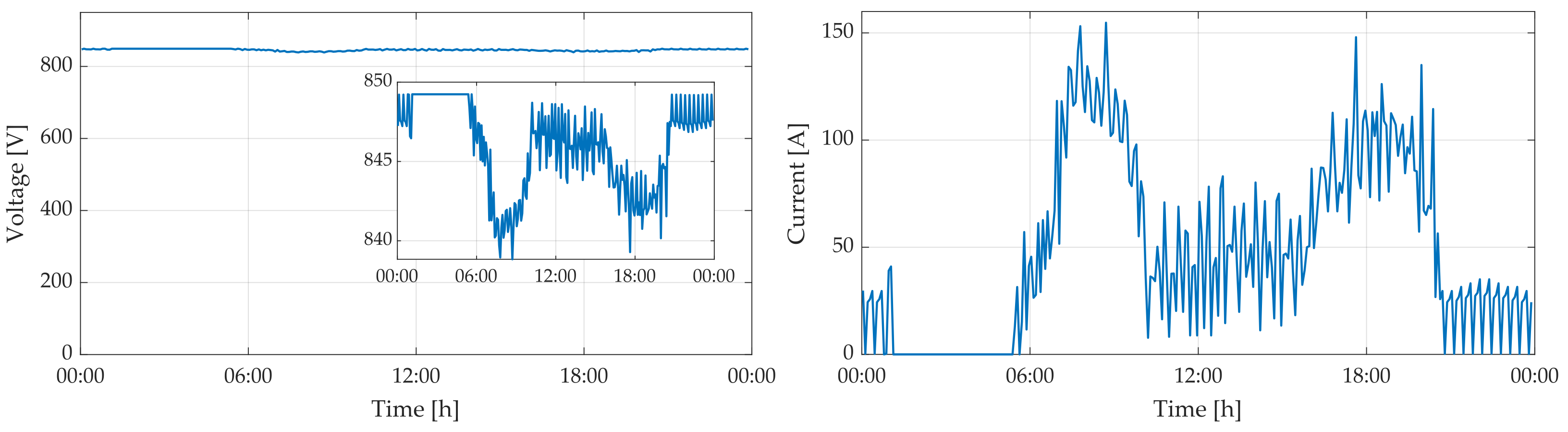
Figure 8.
Voltage (left) and current (right) measurements in TS T-T in an average window of 5 s. The plots cover a 24-h simulation scenario.
Figure 8.
Voltage (left) and current (right) measurements in TS T-T in an average window of 5 s. The plots cover a 24-h simulation scenario.
Figure 9.
Voltage (left) and current (right) measurements in TS T-T in an average window of 5 min. The plots cover a 24-h simulation scenario.
Figure 9.
Voltage (left) and current (right) measurements in TS T-T in an average window of 5 min. The plots cover a 24-h simulation scenario.
Figure 10.
Voltage (left) and current (right) measurements in TS T-T in an average window of 15 min. The plots cover a 24-h simulation scenario.
Figure 10.
Voltage (left) and current (right) measurements in TS T-T in an average window of 15 min. The plots cover a 24-h simulation scenario.
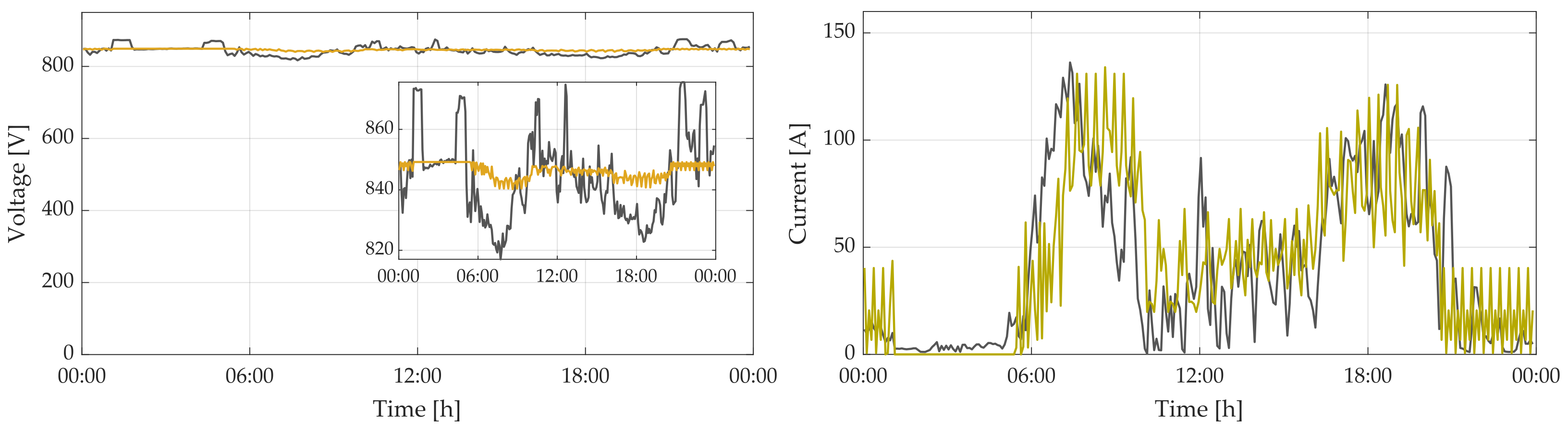
Figure 11.
Measurement (black) and simulations (yellow) of voltage (left) and current (right) in TS T-T in an average window of 5 min. The plots cover a 24-h simulation scenario.
Figure 11.
Measurement (black) and simulations (yellow) of voltage (left) and current (right) in TS T-T in an average window of 5 min. The plots cover a 24-h simulation scenario.
Figure 12.
Measurement (black) and simulations (yellow) of voltage (left) and current (right) in TS T-T in an average window of 15 min. The plots cover a 24-h simulation scenario.
Figure 12.
Measurement (black) and simulations (yellow) of voltage (left) and current (right) in TS T-T in an average window of 15 min. The plots cover a 24-h simulation scenario.
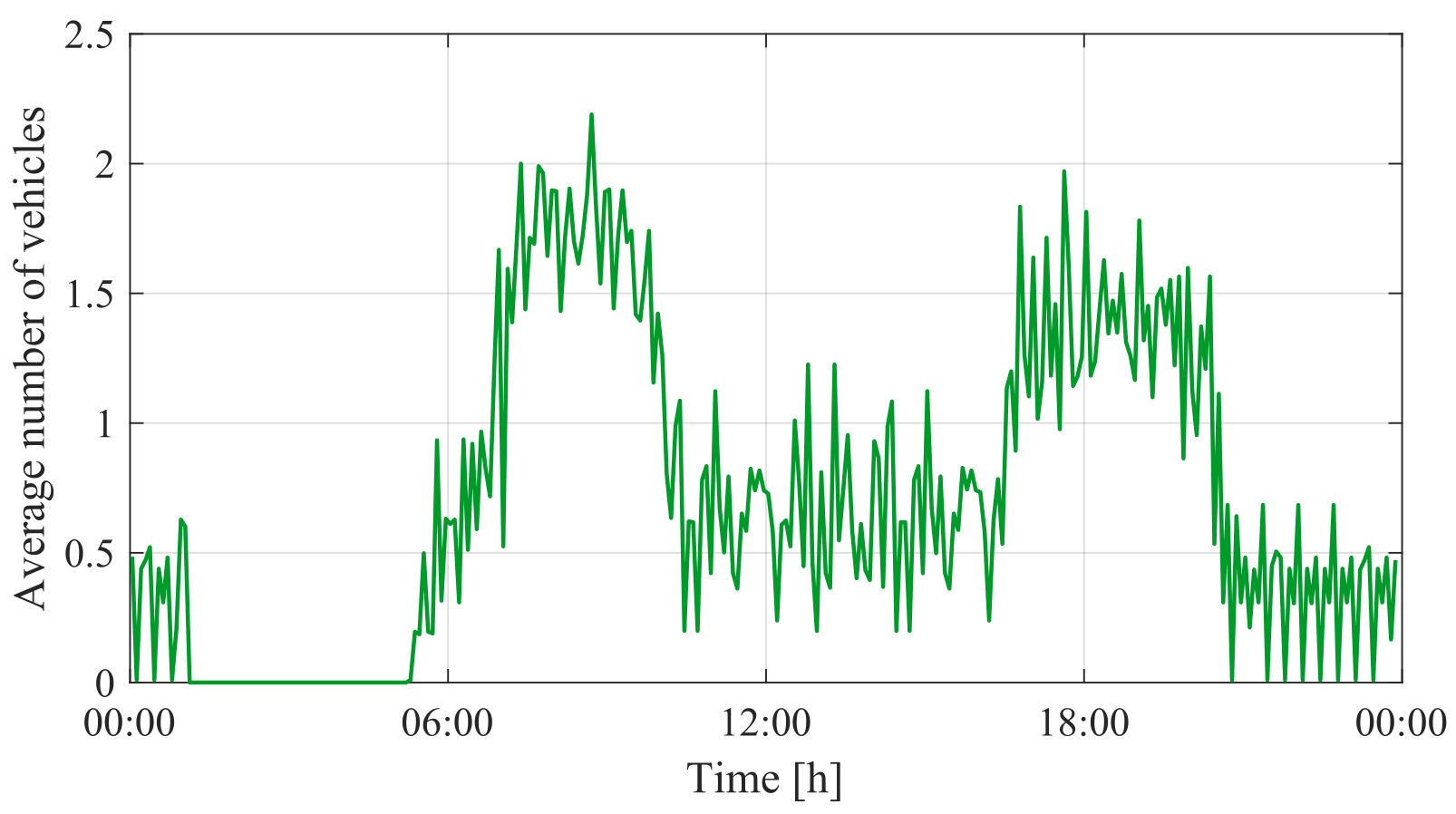
Figure 13.
Number of trolleybuses operating in FS MTT in function of the time (5 min averaged). The plot covers a 24-h simulation scenario.
Figure 13.
Number of trolleybuses operating in FS MTT in function of the time (5 min averaged). The plot covers a 24-h simulation scenario.
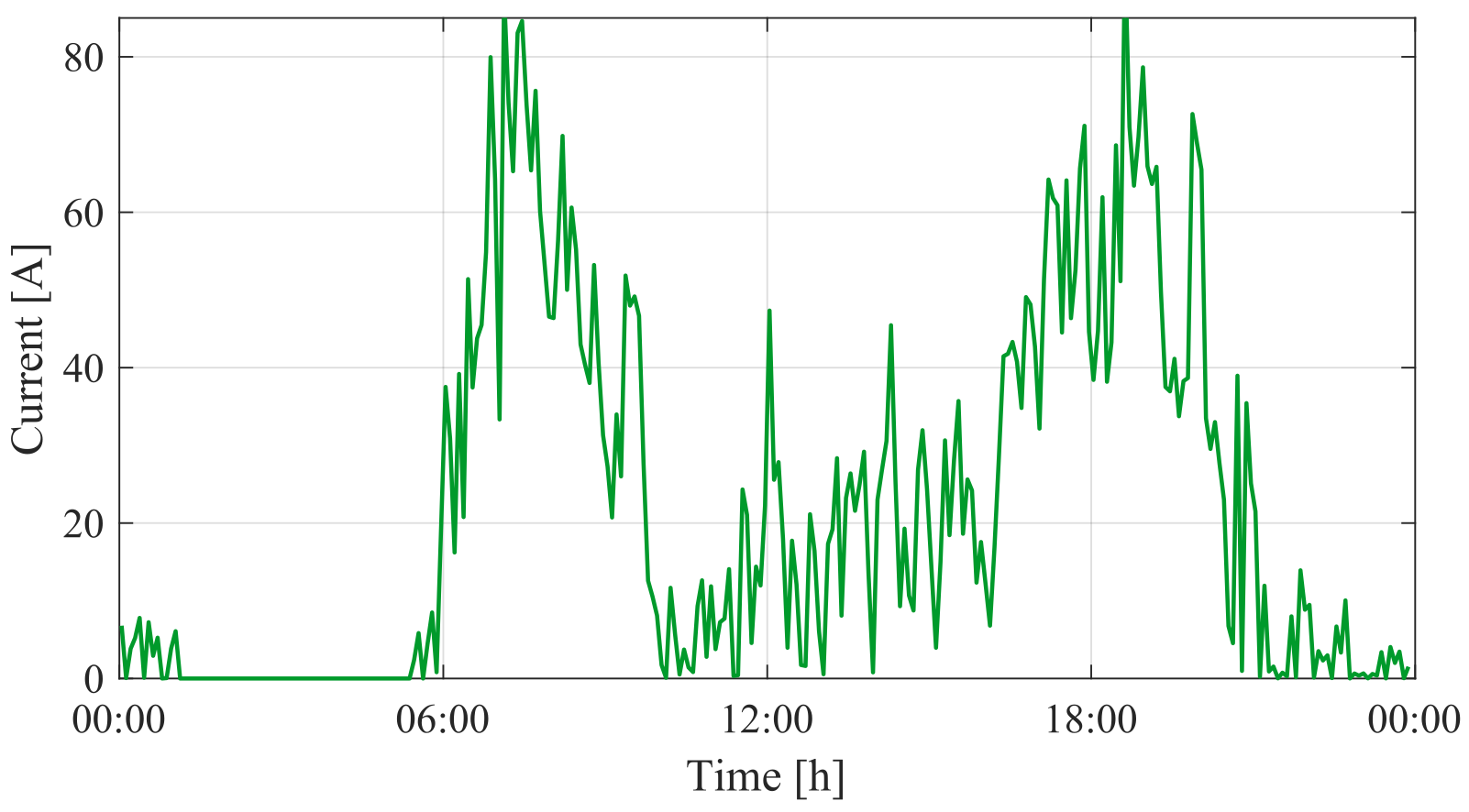
Figure 14.
Current supplied by the TS T-T divided by the number of vehicles in operation according to the period of the day. The plot covers a 24-h simulation scenario.
Figure 14.
Current supplied by the TS T-T divided by the number of vehicles in operation according to the period of the day. The plot covers a 24-h simulation scenario.
Figure 15.
Estimated weight of the trolleybuses according to the period of the day. The plot covers the period from 04:00 to 24:00.
Figure 15.
Estimated weight of the trolleybuses according to the period of the day. The plot covers the period from 04:00 to 24:00.
Figure 16.
Current of conventional trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 14. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 16.
Current of conventional trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 14. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 17.
Current of conventional trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 15. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 17.
Current of conventional trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 15. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 18.
Current of IMC trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 14. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 18.
Current of IMC trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 14. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 19.
Current of IMC trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 15. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 19.
Current of IMC trolleybuses according to the vehicle’s weight covering the outgoing (left) and return (right) journeys of line 15. The weights start at 30 t (upper blue line) and decrease to 20 t (bottom red line) with 2 t steps.
Figure 20.
Voltage (left) and current (right) in TS T-T in an average window of 5 min. Measurements (black), simulation results with gross weight (yellow), and results of the weight-based simulation (blue). The plots cover a 24-h simulation scenario.
Figure 20.
Voltage (left) and current (right) in TS T-T in an average window of 5 min. Measurements (black), simulation results with gross weight (yellow), and results of the weight-based simulation (blue). The plots cover a 24-h simulation scenario.
Figure 21.
Voltage (left) and current (right) in TS T-T in an average window of 15 min. Measurements (black), simulation results with gross weight (yellow), and results of the weight-based simulation (blue). The plots cover a 24-h simulation scenario.
Figure 21.
Voltage (left) and current (right) in TS T-T in an average window of 15 min. Measurements (black), simulation results with gross weight (yellow), and results of the weight-based simulation (blue). The plots cover a 24-h simulation scenario.
Figure 22.
Weight-based simulation results of voltage (left) and current (right) in TS M in an average window of 5 min. The plots cover a 24-h simulation scenario.
Figure 22.
Weight-based simulation results of voltage (left) and current (right) in TS M in an average window of 5 min. The plots cover a 24-h simulation scenario.
Figure 23.
Voltage along the catenary for the four considered scenarios. Voltage maximum (dot-dashed lines) and minimum (dashed lines), mean (upper solid lines), and 95% central inter-percentile range (filled areas above bottom solid lines). Results for the simulations of: 24 h (left), 07:00 to 10:00 (right), and 17:00 to 20:00 (bottom).
Figure 23.
Voltage along the catenary for the four considered scenarios. Voltage maximum (dot-dashed lines) and minimum (dashed lines), mean (upper solid lines), and 95% central inter-percentile range (filled areas above bottom solid lines). Results for the simulations of: 24 h (left), 07:00 to 10:00 (right), and 17:00 to 20:00 (bottom).
Figure 24.
RMS currents (solid lines) along the catenary and its mean value (dashed lines) for the four considered scenarios. Results for the simulations of: 07:00 to 10:00 (left), and 17:00 to 20:00 (right).
Figure 24.
RMS currents (solid lines) along the catenary and its mean value (dashed lines) for the four considered scenarios. Results for the simulations of: 07:00 to 10:00 (left), and 17:00 to 20:00 (right).
Figure 25.
Maximum and minimum currents along the catenary (left), the 95% inter-percentile range (right), and maximum RMS currents in each position during the day (bottom) for the four considered scenarios.
Figure 25.
Maximum and minimum currents along the catenary (left), the 95% inter-percentile range (right), and maximum RMS currents in each position during the day (bottom) for the four considered scenarios.
Table 1.
Line 14—Outward journey. Names of the trolleybuses’ stops and traffic light on the final route.
Table 1.
Line 14—Outward journey. Names of the trolleybuses’ stops and traffic light on the final route.
| Path Number | Stops Names | Length |
|---|
| 1–2 | Ugo Bassi–Rizzoli | |
| 2–3 | Rizzoli–Strada Maggiore | |
| 3–4 | Strada Maggiore–Torleone | |
| 4–5 | Torleone–Porta Maggiore | |
| 5–6 | Porta Maggiore–Traffic light | |
Table 2.
Line 14—Return journey. Names of the trolleybuses’ stops and traffic light on the initial route.
Table 2.
Line 14—Return journey. Names of the trolleybuses’ stops and traffic light on the initial route.
| Path Number | Stops Names | Length |
|---|
| 6–7 | Traffic light–Porta San Vitale | |
| 7–8 | Porta San Vitale–San Vitale | |
| 8–9 | San Vitale–Due Torri | |
| 9–10 | Due Torri–Rizzoli | |
| 10–1 | Rizzoli–Ugo Bassi | |
Table 3.
Line 15—Outward journey. Names of the trolleybuses’ stops.
Table 3.
Line 15—Outward journey. Names of the trolleybuses’ stops.
| Path Number | Stops Names | Length |
|---|
| 1–2 | Ugo Bassi–Rizzoli | |
| 2–3 | Rizzoli–Strada Maggiore | |
| 3–4 | Strada Maggiore–Torleone | |
| 4–5 | Torleone–Porta Maggiore | |
| 5–6 | Porta Maggiore–Albertoni | |
Table 4.
Line 15—Return journey. Names of the trolleybuses’ stops.
Table 4.
Line 15—Return journey. Names of the trolleybuses’ stops.
| Path Number | Stops Names | Length |
|---|
| 6–7 | Albertoni–Porta Maggiore | |
| 7–8 | Porta Maggiore–Porta San Vitale | |
| 8–9 | Porta San Vitale–Porta San Vitale | |
| 9–10 | Porta San Vitale–San Vitale | |
| 10–11 | San Vitale–Due Torri | |
| 11–12 | Due Torri–Rizzoli | |
| 12–1 | Rizzoli–Ugo Bassi | |
Table 5.
Conventional trolleybus specifications.
Table 5.
Conventional trolleybus specifications.
| Description | Parameter |
|---|
| Curb weight | |
| Gross weight | |
| Frontal area | |
| Drag coefficient | |
| Rolling resistance coefficient | |
| Maximum acceleration | |
| Motor power | |
Table 6.
On-board trolleybus battery specifications.
Table 6.
On-board trolleybus battery specifications.
| Description | Parameter |
|---|
| Battery module weight | |
| Nominal capacity | |
| Useful capacity (10–90% state of charge) | |
| Energy content | |
| Energy content (10–90% state of charge) | |
| Nominal voltage | |
Table 7.
Daily energy for the TS T-T and the TS M considering the four simulation scenarios.
Table 7.
Daily energy for the TS T-T and the TS M considering the four simulation scenarios.
| | Measurements | BC | IMC|1 | IMC|2 | IMC|3 |
|---|
| TS T-T | | | | | |
| TS M | – | | | | |
| Total | – | | | | |