A Compendium of Magnetic Nanoparticle Essentials: A Comprehensive Guide for Beginners and Experts
Abstract
1. Introduction
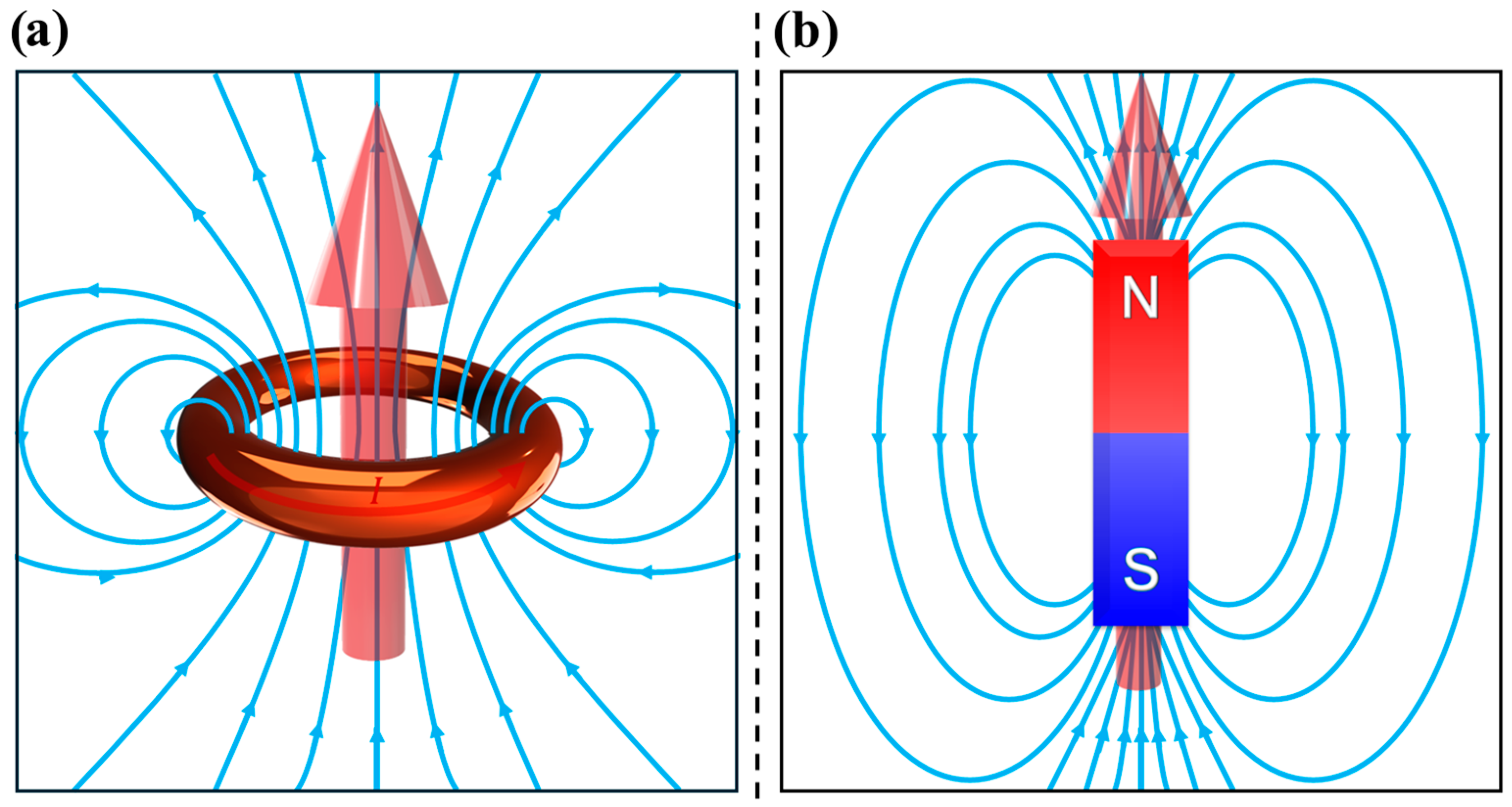
2. Magnetism Fundamental Quantities
3. Types of Magnetic Materials
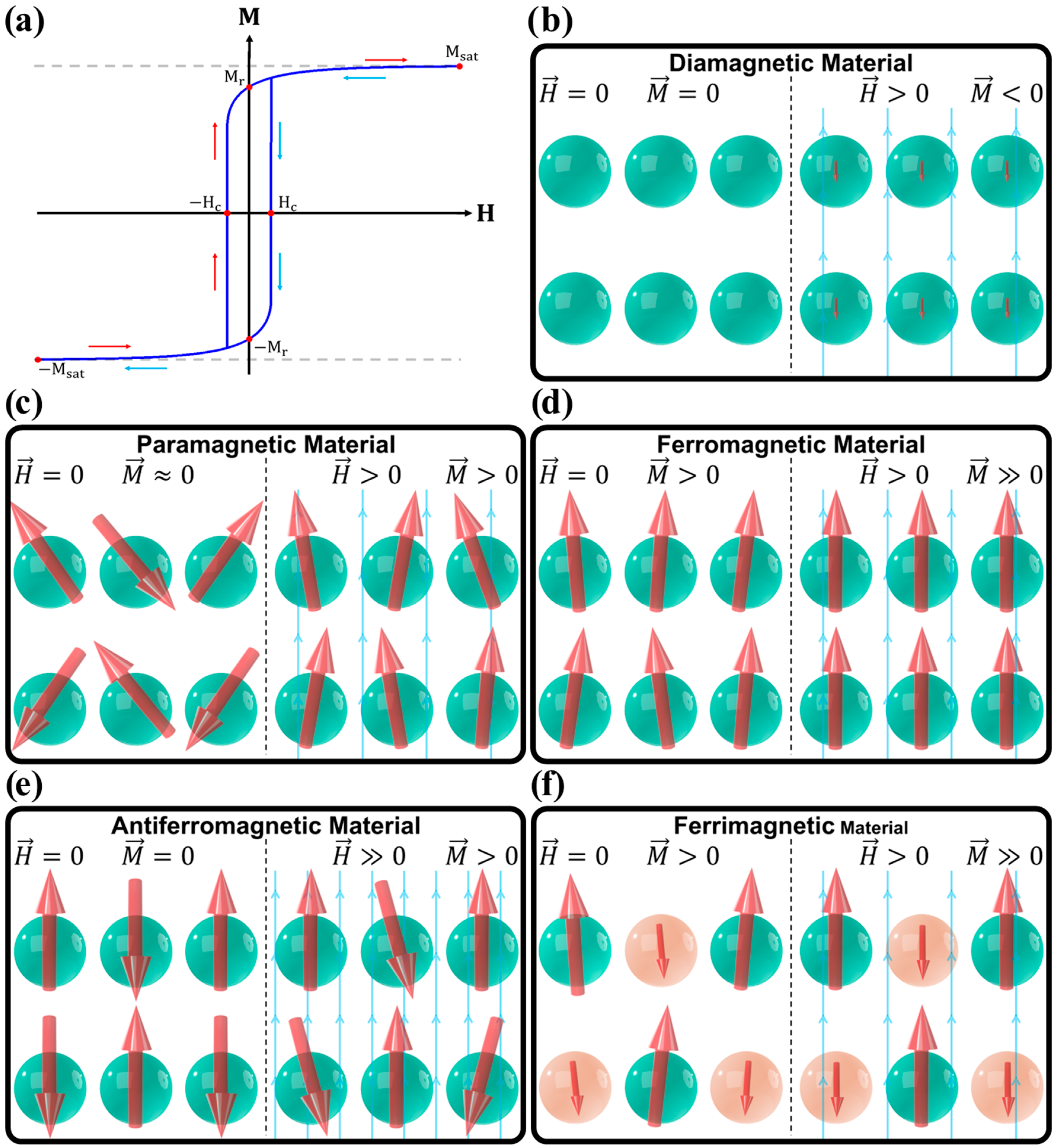
3.1. Diamagnetic Materials
3.2. Paramagnetic Materials
3.3. Ferromagnetic Materials
3.4. Antiferromagnetic Materials
3.5. Ferrimagnetic Materials
4. Nanomagnetism
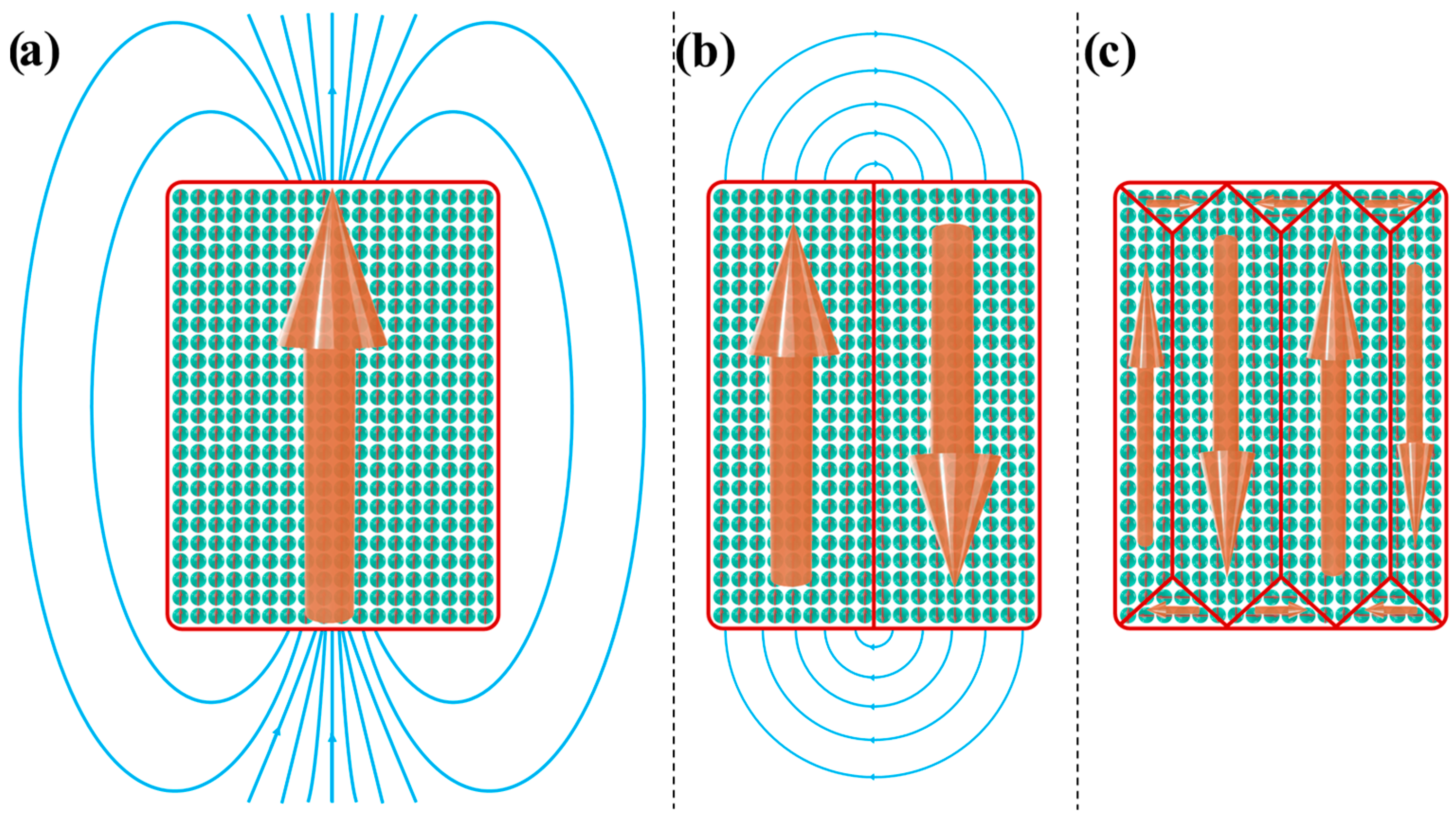
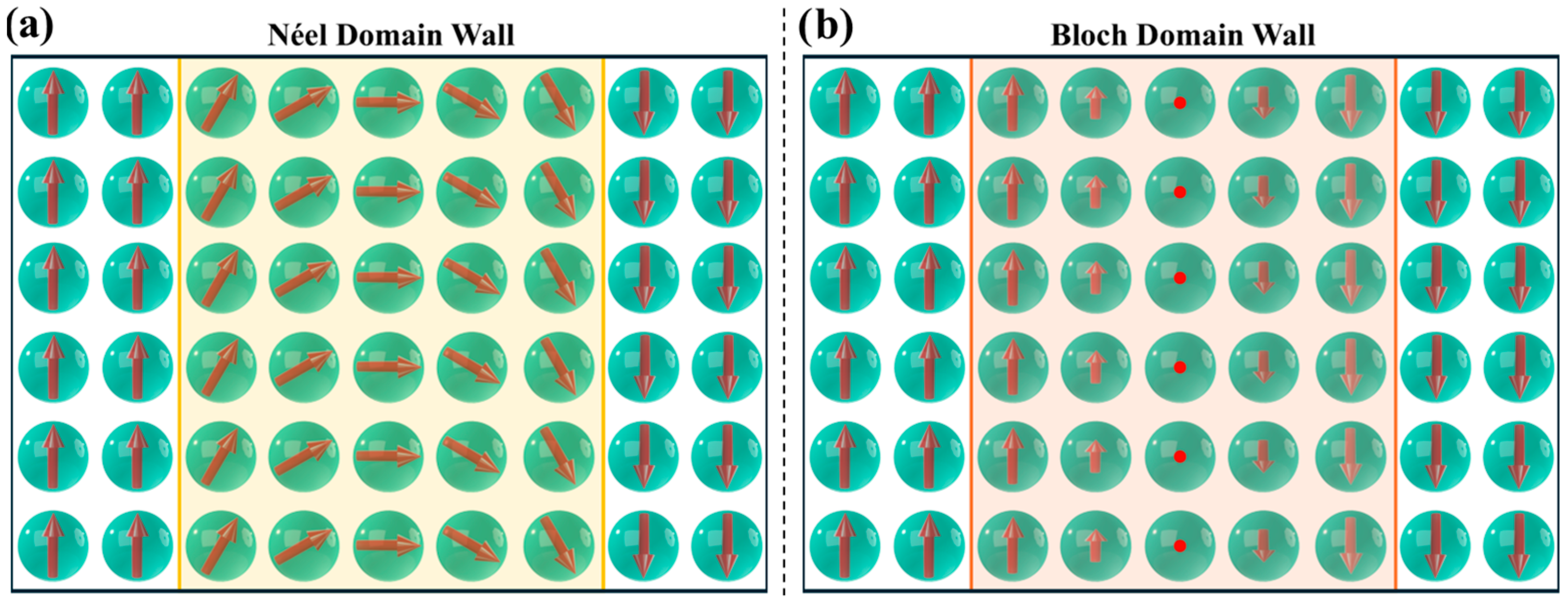
4.1. Magnetic Domains
4.2. Single Domain MNPs
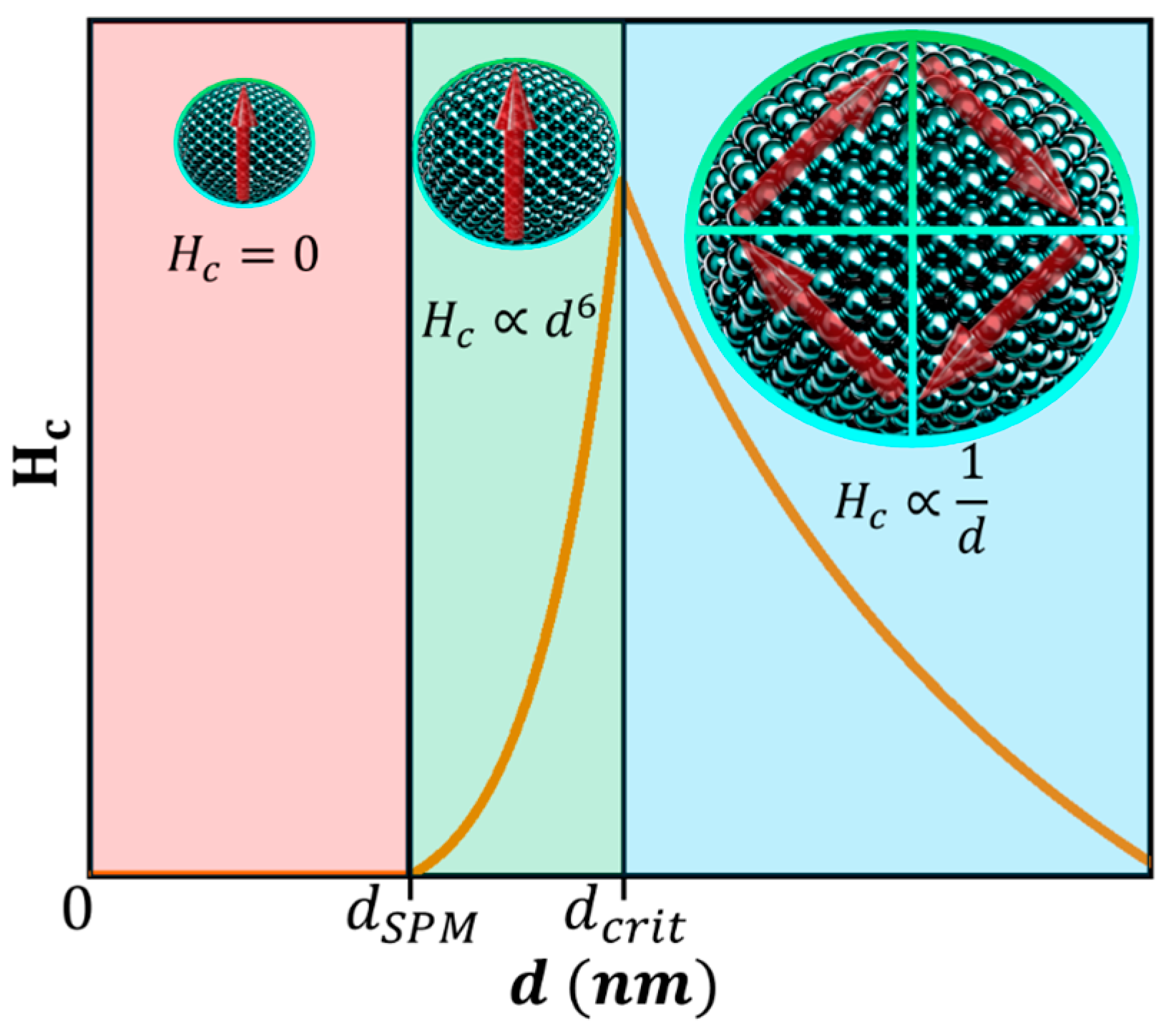
4.3. Superparamagnetism
4.4. Superferromagnetism
4.5. Most Popular MNPs
4.5.1. Metallic MNPs
4.5.2. Oxide Based MNPs
4.6. Surface Effects
4.6.1. Surface Spin Disorder
4.6.2. Enhanced Surface Magnetic Anisotropy
4.6.3. Surface Reactivity and Degradation
4.6.4. Magnetic Dead Layer
4.6.5. Exchange Bias
5. Routine Characterization Techniques for MNPs
5.1. XRD
5.2. Raman Spectroscopy
5.3. Transmission Electron Microscopy and EDS
5.4. Magnetometry
Additional Considerations Regarding Magnetometry
6. Magnetic Induced Heating
6.1. Magnetic Hyperthermia
6.2. Self-Regulated Heating
7. Concluding Remarks
Funding
Conflicts of Interest
References
- Laurent, S.; Forge, D.; Port, M.; Roch, A.; Robic, C.; Vander Elst, L.; Muller, R.N. Magnetic iron oxide nanoparticles: Synthesis, stabilization, vectorization, physicochemical characterizations, and biological applications. Chem. Rev. 2008, 108, 2064–2110. [Google Scholar]
- Roduner, E. Size matters: Why nanomaterials are different. Chem. Soc. Rev. 2006, 35, 583–592. [Google Scholar] [CrossRef] [PubMed]
- Kudr, J.; Haddad, Y.; Richtera, L.; Heger, Z.; Cernak, M.; Adam, V.; Zitka, O. Magnetic nanoparticles: From design and synthesis to real world applications. Nanomaterials 2017, 7, 243. [Google Scholar] [CrossRef]
- Lu, A.H.; Salabas, E.E.; Schüth, F. Magnetic nanoparticles: Synthesis, protection, functionalization, and application. Angew. Chem. Int. Ed. 2007, 46, 1222–1244. [Google Scholar] [CrossRef] [PubMed]
- Reddy, L.H.; Arias, J.L.; Nicolas, J.; Couvreur, P. Magnetic nanoparticles: Design and characterization, toxicity and biocompatibility, pharmaceutical and biomedical applications. Chem. Rev. 2012, 112, 5818–5878. [Google Scholar] [CrossRef] [PubMed]
- Wu, W.; He, Q.; Jiang, C. Magnetic iron oxide nanoparticles: Synthesis and surface functionalization strategies. Nanoscale Res. Lett. 2008, 3, 397–415. [Google Scholar] [CrossRef]
- Sun, C.; Lee, J.S.H.; Zhang, M. Magnetic nanoparticles in MR imaging and drug delivery. Adv. Drug Deliv. Rev. 2008, 60, 1252–1265. [Google Scholar] [CrossRef]
- Gupta, A.K.; Gupta, M. Synthesis and surface engineering of iron oxide nanoparticles for biomedical applications. Biomaterials 2005, 26, 3995–4021. [Google Scholar] [CrossRef]
- Hua, M.; Zhang, S.; Pan, B.; Zhang, W.; Lv, L.; Zhang, Q. Heavy metal removal from water/wastewater by nanosized metal oxides: A review. J. Hazard. Mater. 2012, 211, 317–331. [Google Scholar] [CrossRef]
- Xu, C.; Sun, S. New forms of superparamagnetic nanoparticles for biomedical applications. Adv. Drug Deliv. Rev. 2013, 65, 732–743. [Google Scholar] [PubMed]
- Gao, J.; Gu, H.; Xu, B. Multifunctional magnetic nanoparticles: Design, synthesis, and biomedical applications. Acc. Chem. Res. 2009, 42, 1097–1107. [Google Scholar] [CrossRef]
- Ansari, M.J.; Kadhim, M.M.; Hussein, B.A.; Lafta, H.A.; Kianfar, E. Synthesis and stability of magnetic nanoparticles. Bionanoscience 2022, 12, 627–638. [Google Scholar] [CrossRef]
- Shahbazi, R.; Behbahani, F.K. Synthesis, modifications, and applications of iron-based nanoparticles. Mol. Divers. 2024, 28, 4515–4552. [Google Scholar] [CrossRef] [PubMed]
- Selim, M.M.; El-Safty, S.; Tounsi, A.; Shenashen, M. A review of magnetic nanoparticles used in nanomedicine. APL Mater. 2024, 12, 010601. [Google Scholar] [CrossRef]
- Khanna, L.; Verma, N.K.; Tripathi, S.K. Burgeoning tool of biomedical applications-Superparamagnetic nanoparticles. J. Alloys Compd. 2018, 752, 332–353. [Google Scholar] [CrossRef]
- Stiufiuc, G.F.; Stiufiuc, R.I. Magnetic Nanoparticles: Synthesis, Characterization, and Their Use in Biomedical Field. Appl. Sci. 2024, 14, 1623. [Google Scholar] [CrossRef]
- Veloso, S.R.S.; Vázquez-González, M.; Spuch, C.; Freiría-Martínez, L.; Comís-Tuche, M.; Iglesias-Martínez-Almeida, M.; Rivera-Baltanás, T.; Hilliou, L.; Amorim, C.O.; Amaral, V.S. An Injectable Composite Co-Assembled Dehydropeptide-Based Magnetic/Plasmonic Lipogel for Multimodal Cancer Therapy. Adv. Funct. Mater. 2024, 34, 2402926. [Google Scholar] [CrossRef]
- Pacheco, A.R.F.; Barros, A.M.; Amorim, C.O.; Amaral, V.S.; Coutinho, P.J.G.; Rodrigues, A.R.O.; Castanheira, E.M.S. Elastic Liposomes Containing Calcium/Magnesium Ferrite Nanoparticles Coupled with Gold Nanorods for Application in Photothermal Therapy. Nanomaterials 2024, 14, 679. [Google Scholar] [CrossRef] [PubMed]
- Veloso, S.R.S.; Andrade, R.G.D.; Gomes, V.; Amorim, C.O.; Amaral, V.S.; Salgueiriño, V.; Coutinho, P.J.G.; Ferreira, P.M.T.; Correa-Duarte, M.A.; Castanheira, E.M.S. Oxidative precipitation synthesis of calcium-doped manganese ferrite nanoparticles for magnetic hyperthermia. Int. J. Mol. Sci. 2022, 23, 14145. [Google Scholar] [CrossRef]
- Horta, A.C.; Amorim, C.O.; Soares, S.F.; Bañobre-López, M.; Daniel-da-Silva, A.L.; Trindade, T.; Amaral, J.S. High yttrium retention in magnetite nanoparticles functionalized with hybrid silica-dextran shells. Nano-Struct. Nano-Objects 2023, 36, 101065. [Google Scholar] [CrossRef]
- Horta, A.C.; André, P.; Amaral, J.S.; Amorim, C.O. Curie temperature control in Zn-Fe ferrite superparamagnetic nanoparticles. J. Magn. Magn. Mater. 2024, 610, 172497. [Google Scholar] [CrossRef]
- Veloso, S.R.S.; Nereu, S.F.; Amorim, C.O.; Amaral, V.S.; Correa-Duarte, M.A.; Castanheira, E.M.S. Eco-friendly synthesis of fluorescent cobalt-doped manganese ferrites for thermo-therapeutic applications. Mater. Today Commun. 2024, 39, 108822. [Google Scholar] [CrossRef]
- Cardoso, B.D.; Rodrigues, A.R.O.; Bañobre-López, M.; Almeida, B.G.; Amorim, C.O.; Amaral, V.S.; Coutinho, P.J.G.; Castanheira, E.M.S. Magnetoliposomes based on shape anisotropic calcium/magnesium ferrite nanoparticles as nanocarriers for doxorubicin. Pharmaceutics 2021, 13, 1248. [Google Scholar] [CrossRef]
- Cardoso, B.D.; Rodrigues, A.R.O.; Almeida, B.G.; Amorim, C.O.; Amaral, V.S.; Castanheira, E.M.S.; Coutinho, P.J.G. Stealth magnetoliposomes based on calcium-substituted magnesium ferrite nanoparticles for curcumin transport and release. Int. J. Mol. Sci. 2020, 21, 3641. [Google Scholar] [CrossRef] [PubMed]
- Cardoso, B.D.; Fernandes, D.E.M.; Amorim, C.O.; Amaral, V.S.; Coutinho, P.J.G.; Rodrigues, A.R.O.; Castanheira, E.M.S. Magnetoliposomes with Calcium-Doped Magnesium Ferrites Anchored in the Lipid Surface for Enhanced DOX Release. Nanomaterials 2023, 13, 2597. [Google Scholar] [CrossRef] [PubMed]
- Pereira, D.S.M.; Cardoso, B.D.; Rodrigues, A.R.O.; Amorim, C.O.; Amaral, V.S.; Almeida, B.G.; Queiroz, M.-J.R.P.; Martinho, O.; Baltazar, F.; Calhelha, R.C. Magnetoliposomes containing calcium ferrite nanoparticles for applications in breast cancer therapy. Pharmaceutics 2019, 11, 477. [Google Scholar] [CrossRef]
- Nogueira, J.; Soares, S.F.; Amorim, C.O.; Amaral, J.S.; Silva, C.; Martel, F.; Trindade, T.; Daniel-da-Silva, A.L. Magnetic driven nanocarriers for pH-responsive doxorubicin release in cancer therapy. Molecules 2020, 25, 333. [Google Scholar] [CrossRef] [PubMed]
- Veloso, S.R.S.; Martins, J.A.; Hilliou, L.; Amorim, C.O.; Amaral, V.S.; Almeida, B.G.; Jervis, P.J.; Moreira, R.; Pereira, D.M.; Coutinho, P.J.G. Dehydropeptide-based plasmonic magnetogels: A supramolecular composite nanosystem for multimodal cancer therapy. J. Mater. Chem. B 2020, 8, 45–64. [Google Scholar] [CrossRef]
- Veloso, S.R.S.; Tiryaki, E.; Spuch, C.; Hilliou, L.; Amorim, C.O.; Amaral, V.S.; Coutinho, P.J.G.; Ferreira, P.M.T.; Salgueiriño, V.; Correa-Duarte, M.A. Tuning the drug multimodal release through a co-assembly strategy based on magnetic gels. Nanoscale 2022, 14, 5488–5500. [Google Scholar] [CrossRef]
- Veloso, S.R.S.; Marta, E.S.; Rodrigues, P.V.; Moura, C.; Amorim, C.O.; Amaral, V.S.; Correa-Duarte, M.A.; Castanheira, E.M.S. Chitosan/alginate nanogels containing multicore magnetic nanoparticles for delivery of doxorubicin. Pharmaceutics 2023, 15, 2194. [Google Scholar] [CrossRef]
- Rauwel, E.; Al-Arag, S.; Salehi, H.; Amorim, C.O.; Cuisinier, F.; Guha, M.; Rosario, M.S.; Rauwel, P. Assessing cobalt metal nanoparticles uptake by cancer cells using live Raman spectroscopy. Int. J. Nanomed. 2020, 15, 7051–7062. [Google Scholar] [CrossRef] [PubMed]
- Mishra, S.; Yadav, M.D. Magnetic nanoparticles: A comprehensive review from synthesis to biomedical frontiers. Langmuir 2024, 40, 17239–17269. [Google Scholar] [CrossRef] [PubMed]
- Losito, D.W.; Souza, N.I.N.; Martins, T.S.; Britos, T.N.; Schumacher, M.L.; Haddad, P.S. A review of superparamagnetic nanoparticles applications and regulatory aspects in medicine and environmental areas. J. Mater. Sci. 2024, 59, 1–31. [Google Scholar] [CrossRef]
- Mura, S.; Nicolas, J.; Couvreur, P. Stimuli-responsive nanocarriers for drug delivery. Nat. Mater. 2013, 12, 991–1003. [Google Scholar] [CrossRef] [PubMed]
- Lei, S.; He, J.; Gao, P.; Wang, Y.; Hui, H.; An, Y.; Tian, J. Magnetic particle imaging-guided hyperthermia for precise treatment of cancer: Review, challenges, and prospects. Mol. Imaging Biol. 2023, 25, 1020–1033. [Google Scholar] [CrossRef]
- Chang, F.; Davies, G.-L. From 0D to 2D: Synthesis and bio-application of anisotropic magnetic iron oxide nanomaterials. Prog. Mater. Sci. 2024, 144, 101267. [Google Scholar] [CrossRef]
- Veloso, S.R.S.; Andrade, R.G.D.; Castanheira, E.M.S. Review on the advancements of magnetic gels: Towards multifunctional magnetic liposome-hydrogel composites for biomedical applications. Adv. Colloid. Interface Sci. 2021, 288, 102351. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.-Y.; Hu, S.-H.; Liu, T.-Y.; Liu, D.-M.; Chen, S.-Y. Magnetic-sensitive behavior of intelligent ferrogels for controlled release of drug. Langmuir 2006, 22, 5974–5978. [Google Scholar] [CrossRef]
- Zrinyi, M. Intelligent polymer gels controlled by magnetic fields. Colloid. Polym. Sci. 2000, 278, 98–103. [Google Scholar] [CrossRef]
- Fuhrer, R.; Athanassiou, E.K.; Luechinger, N.A.; Stark, W.J. Crosslinking metal nanoparticles into the polymer backbone of hydrogels enables preparation of soft, magnetic field-driven actuators with muscle-like flexibility. Small 2009, 5, 383–388. [Google Scholar] [CrossRef]
- Satarkar, N.S.; Hilt, J.Z. Magnetic hydrogel nanocomposites for remote controlled pulsatile drug release. J. Control. Release 2008, 130, 246–251. [Google Scholar] [CrossRef]
- Häring, M.; Schiller, J.; Mayr, J.; Grijalvo, S.; Eritja, R.; Díaz Díaz, D. Magnetic gel composites for hyperthermia cancer therapy. Gels 2015, 1, 135–161. [Google Scholar] [CrossRef] [PubMed]
- Weeber, R.; Hermes, M.; Schmidt, A.M.; Holm, C. Polymer architecture of magnetic gels: A review. J. Phys. Condens. Matter 2018, 30, 063002. [Google Scholar] [CrossRef]
- Du, Y.; Lai, P.T.; Leung, C.H.; Pong, P.W.T. Design of superparamagnetic nanoparticles for magnetic particle imaging (MPI). Int. J. Mol. Sci. 2013, 14, 18682–18710. [Google Scholar] [CrossRef]
- Niraula, G.; Wu, C.; Yu, X.; Malik, S.; Verma, D.S.; Yang, R.; Zhao, B.; Ding, S.; Zhang, W.; Sharma, S.K. The Curie temperature: A key playmaker in self-regulated temperature hyperthermia. J. Mater. Chem. B 2023, 12, 286–331. [Google Scholar] [CrossRef]
- Rosensweig, R.E. Heating magnetic fluid with alternating magnetic field. J. Magn. Magn. Mater. 2002, 252, 370–374. [Google Scholar] [CrossRef]
- Suriyanto Ng, E.Y.K.; Kumar, S.D. Physical mechanism and modeling of heat generation and transfer in magnetic fluid hyperthermia through Néelian and Brownian relaxation: A review. Biomed. Eng. Online 2017, 16, 36. [Google Scholar] [CrossRef]
- Karami, M.H.; Abdouss, M.; Maleki, B. The state of the art metal nanoparticles in drug delivery systems: A comprehensive review. Nanomed. J. 2024, 11, 222. [Google Scholar]
- Patri, S.; Thanh, N.T.K.; Kamaly, N. Magnetic iron oxide nanogels for combined hyperthermia and drug delivery for cancer treatment. Nanoscale 2024, 16, 15446–15464. [Google Scholar] [CrossRef]
- Zhang, Y.-F.; Lu, M. Advances in magnetic induction hyperthermia. Front. Bioeng. Biotechnol. 2024, 12, 1432189. [Google Scholar] [CrossRef]
- Oliveira, F.M.; Paštika, J.; Ayaz, I.; Mazánek, V.; Plutnarová, I.; Zeng, L.; Olsson, E.; Amorim, C.O.; Melle-Franco, M.; Gusmão, R. Alkaline water electrolysis performance of mixed cation metal phosphorous trichalcogenides. Mater. Today Energy 2024, 39, 101468. [Google Scholar] [CrossRef]
- Soares, S.F.; Amorim, C.O.; Amaral, J.S.; Trindade, T.; Daniel-da-Silva, A.L. On the efficient removal, regeneration and reuse of quaternary chitosan magnetite nanosorbents for glyphosate herbicide in water. J. Environ. Chem. Eng. 2021, 9, 105189. [Google Scholar] [CrossRef]
- Soares, S.F.; Rocha, M.J.; Ferro, M.; Amorim, C.O.; Amaral, J.S.; Trindade, T.; Daniel-da-Silva, A.L. Magnetic nanosorbents with siliceous hybrid shells of alginic acid and carrageenan for removal of ciprofloxacin. Int. J. Biol. Macromol. 2019, 139, 827–841. [Google Scholar] [CrossRef]
- Campos, V.; Marques, D.G.; Nogueira, J.; Amorim, C.O.; Daniel-da-Silva, A.L.; Trindade, T. Magnetic nanosorbents of γ-polyglutamic acid for removing a β-blocker from water. J. Environ. Chem. Eng. 2023, 11, 110498. [Google Scholar] [CrossRef]
- Santos, G.T.A.D.; Estrada, A.C.; Amorim, C.O.; Amaral, J.S.; Deuermeier, J.; Duarte, A.C.; Santos, P.S.M. Hybrid nanocomposites of Fe3O4/SiO2-EDTA: Holistic comparison of one-step and two-step modification methods. Powder Technol. 2024, 444, 120046. [Google Scholar] [CrossRef]
- Afonso, E.L.; Carvalho, L.; Fateixa, S.; Amorim, C.O.; Amaral, V.S.; Vale, C.; Pereira, E.; Silva, C.M.; Trindade, T.; Lopes, C.B. Can contaminated waters or wastewater be alternative sources for technology-critical elements? The case of removal and recovery of lanthanides. J. Hazard. Mater. 2019, 380, 120845. [Google Scholar] [CrossRef] [PubMed]
- Gupta, V.K.; Nayak, A. Cadmium removal and recovery from aqueous solutions by novel adsorbents prepared from orange peel and Fe2O3 nanoparticles. Chem. Eng. J. 2012, 180, 81–90. [Google Scholar] [CrossRef]
- Ali, I. New generation adsorbents for water treatment. Chem. Rev. 2012, 112, 5073–5091. [Google Scholar] [CrossRef] [PubMed]
- Liong, M.; Lu, J.; Kovochich, M.; Xia, T.; Ruehm, S.G.; Nel, A.E.; Tamanoi, F.; Zink, J.I. Multifunctional inorganic nanoparticles for imaging, targeting, and drug delivery. ACS Nano 2008, 2, 889–896. [Google Scholar] [CrossRef] [PubMed]
- Chandra, V.; Park, J.; Chun, Y.; Lee, J.W.; Hwang, I.-C.; Kim, K.S. Water-dispersible magnetite-reduced graphene oxide composites for arsenic removal. ACS Nano 2010, 4, 3979–3986. [Google Scholar] [CrossRef] [PubMed]
- Qasem, N.A.; Mohammed, R.H.; Lawal, D.U. Removal of heavy metal ions from wastewater: A comprehensive and critical review. Npj Clean Water 2021, 4, 1–15. [Google Scholar] [CrossRef]
- Rathi, B.S.; Kumar, P.S.; Vo, D.-V.N. Critical review on hazardous pollutants in water environment: Occurrence, monitoring, fate, removal technologies and risk assessment. Sci. Total Environ. 2021, 797, 149134. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Kong, J. Novel magnetic Fe3O4@ C nanoparticles as adsorbents for removal of organic dyes from aqueous solution. J. Hazard. Mater. 2011, 193, 325–329. [Google Scholar] [CrossRef]
- Rocher, V.; Siaugue, J.-M.; Cabuil, V.; Bee, A. Removal of organic dyes by magnetic alginate beads. Water Res. 2008, 42, 1290–1298. [Google Scholar] [CrossRef] [PubMed]
- Calcagnile, P.; Fragouli, D.; Bayer, I.S.; Anyfantis, G.C.; Martiradonna, L.; Cozzoli, P.D.; Cingolani, R.; Athanassiou, A. Magnetically driven floating foams for the removal of oil contaminants from water. ACS Nano 2012, 6, 5413–5419. [Google Scholar] [CrossRef] [PubMed]
- Sun, X.; Huang, Y.; Nikles, D.E. FePt and CoPt magnetic nanoparticles film for future high density data storage media. Int. J. Nanotechnol. 2004, 1, 328–346. [Google Scholar] [CrossRef]
- Sun, S. Recent advances in chemical synthesis, self-assembly, and applications of FePt nanoparticles. Adv. Mater. 2006, 18, 393–403. [Google Scholar] [CrossRef]
- Reiss, G.; Hütten, A. Applications beyond data storage. Nat. Mater. 2005, 4, 725–726. [Google Scholar] [CrossRef]
- Chen, W.D.; Kohll, A.X.; Nguyen, B.H.; Koch, J.; Heckel, R.; Stark, W.J.; Ceze, L.; Strauss, K.; Grass, R.N. Combining data longevity with high storage capacity—Layer-by-layer DNA encapsulated in magnetic nanoparticles. Adv. Funct. Mater. 2019, 29, 1901672. [Google Scholar] [CrossRef]
- Zhang, H.; Liu, Y.; Sun, S. Synthesis and assembly of magnetic nanoparticles for information and energy storage applications. Front. Phys. China 2010, 5, 347–356. [Google Scholar] [CrossRef]
- Thomson, T.; Abelmann, L.; Groenland, H. Magnetic data storage: Past present and future. In Magnetic Nanostructures in Modern Technology: Spintronics, Magnetic MEMS and Recording; Springer: Berlin/Heidelberg, Germany, 2008; pp. 237–306. [Google Scholar]
- Galloway, J.M.; Talbot, J.E.; Critchley, K.; Miles, J.J.; Bramble, J.P. Developing biotemplated data storage: Room temperature biomineralization of L10 CoPt magnetic nanoparticles. Adv. Funct. Mater. 2015, 25, 4590–4600. [Google Scholar] [CrossRef]
- Phan, M.-H.; Alonso, J.; Khurshid, H.; Lampen-Kelley, P.; Chandra, S.; Stojak Repa, K.; Nemati, Z.; Das, R.; Iglesias, Ó.; Srikanth, H. Exchange bias effects in iron oxide-based nanoparticle systems. Nanomaterials 2016, 6, 221. [Google Scholar] [CrossRef] [PubMed]
- Shinjo, T. Nanomagnetism and Spintronics; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Lukaszew, R.A. Handbook of Nanomagnetism: Applications and Tools; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Ma, Z.; Mohapatra, J.; Wei, K.; Liu, J.P.; Sun, S. Magnetic nanoparticles: Synthesis, anisotropy, and applications. Chem. Rev. 2021, 123, 3904–3943. [Google Scholar] [CrossRef] [PubMed]
- Martins, N.M.R.; Martins, L.M.; Amorim, C.O.; Amaral, V.S.; Pombeiro, A.J.L. Solvent-free microwave-induced oxidation of alcohols catalyzed by ferrite magnetic nanoparticles. Catalysts 2017, 7, 222. [Google Scholar] [CrossRef]
- Fernandes, R.J.C.; Magalhães, C.A.B.; Amorim, C.O.; Amaral, V.S.; Almeida, B.G.; Castanheira, E.M.S.; Coutinho, P.J.G. Magnetic nanoparticles of zinc/calcium ferrite decorated with silver for photodegradation of dyes. Materials 2019, 12, 3582. [Google Scholar] [CrossRef] [PubMed]
- Martins, N.M.R.; Martins, L.M.; Amorim, C.O.; Amaral, V.S.; Pombeiro, A.J.L. First-row-transition ion metals (II)-EDTA functionalized magnetic nanoparticles as catalysts for solvent-free microwave-induced oxidation of alcohols. Catalysts 2017, 7, 335. [Google Scholar] [CrossRef]
- Baig, R.B.N.; Varma, R.S. Magnetically retrievable catalysts for organic synthesis. Chem. Commun. 2013, 49, 752–770. [Google Scholar] [CrossRef]
- Zhu, H.; Zhang, S.; Huang, Y.-X.; Wu, L.; Sun, S. Monodisperse MxFe3–xO4 (M = Fe, Cu, Co, Mn) nanoparticles and their electrocatalysis for oxygen reduction reaction. Nano Lett. 2013, 13, 2947–2951. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.; Ai, J.; Zhang, H. The mechanism of degradation of bisphenol A using the magnetically separable CuFe2O4/peroxymonosulfate heterogeneous oxidation process. J. Hazard. Mater. 2016, 309, 87–96. [Google Scholar] [CrossRef] [PubMed]
- Park, J.; Kang, E.; Son, S.U.; Park, H.M.; Lee, M.K.; Kim, J.; Kim, K.W.; Noh, H.-J.; Park, J.-H.; Bae, C.J. Monodisperse nanoparticles of Ni and NiO: Synthesis, characterization, self-assembled superlattices, and catalytic applications in the Suzuki coupling reaction. Adv. Mater. 2005, 17, 429–434. [Google Scholar] [CrossRef]
- Lin, F.; Doong, R. Bifunctional Au−Fe3O4 heterostructures for magnetically recyclable catalysis of nitrophenol reduction. J. Phys. Chem. C 2011, 115, 6591–6598. [Google Scholar] [CrossRef]
- Abu-Reziq, R.; Alper, H.; Wang, D.; Post, M.L. Metal supported on dendronized magnetic nanoparticles: Highly selective hydroformylation catalysts. J. Am. Chem. Soc. 2006, 128, 5279–5282. [Google Scholar] [CrossRef] [PubMed]
- Koo, K.N.; Ismail, A.F.; Othman, M.H.D.; Bidin, N.; Rahman, M.A. Preparation and characterization of superparamagnetic magnetite (Fe3O4) nanoparticles: A short review. Malays. J. Fundam. Appl. Sci. 2019, 15, 23–31. [Google Scholar] [CrossRef]
- Jun, B.-H. Nanotechnology for Bioapplications; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Blundell, S. Magnetism in Condensed Matter; OUP Oxford: Oxford, UK, 2001. [Google Scholar]
- Coey, J.M.D. Magnetism and Magnetic Materials; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Siegmann, H.C.; Stöhr, J. Magnetism: From Fundamentals to Nanoscale Dynamics; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Spaldin, N.A. Magnetic Materials: Fundamentals and Applications; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Soler, M.A.G.; Paterno, L.G. Magnetic Nanomaterials. Nanostructures; Elsevier: Amsterdam, The Netherlands, 2017; pp. 147–186. [Google Scholar]
- Fermon, C.; Van de Voorde, M. Nanomagnetism: Applications and Perspectives; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Mugiraneza, S.; Hallas, A.M. Tutorial: A beginner’s guide to interpreting magnetic susceptibility data with the Curie-Weiss law. Commun. Phys. 2022, 5, 95. [Google Scholar] [CrossRef]
- Rumble, J. CRC Handbook of Chemistry and Physics; CRC: Boca Raton, FL, USA, 2017. [Google Scholar]
- Hedayatnasab, Z.; Abnisa, F.; Daud, W.M.A.W. Review on magnetic nanoparticles for magnetic nanofluid hyperthermia application. Mater. Des. 2017, 123, 174–196. [Google Scholar] [CrossRef]
- Guimarães, A.P.; Guimarães, A.P. The basis of nanomagnetism. In Principles of Nanomagnetism; Springer: Cham, Switzerland, 2017; pp. 1–23. [Google Scholar]
- Binns, C. Nanomagnetism: Fundamentals and Applications; Newnes: Oxford, UK, 2014; Volume 6. [Google Scholar]
- Amorim, C.O.; Figueiras, F.; Amaral, J.S.; Vaghefi, P.M.; Tavares, P.B.; Correia, M.R.; Baghizadeh, A.; Alves, E.; Rocha, J.; Amaral, V.S. Peculiar Magnetoelectric Coupling in BaTiO3: Fe113 ppm Nanoscopic Segregations. ACS Appl. Mater. Interfaces 2015, 7, 24741–24747. [Google Scholar] [CrossRef]
- de Almeida, A.A.; Fabris, F.; da Silva, G.S.; Pirota, K.R.; Knobel, M.; Muraca, D. Control of Anisotropy and Magnetic Hyperthermia Effect by Addition of Cobalt on Magnetite Nanoparticles. ACS Appl. Mater. Interfaces 2024. [Google Scholar] [CrossRef]
- Kuz’min, M.D.; Richter, M.; Yaresko, A.N. Factors determining the shape of the temperature dependence of the spontaneous magnetization of a ferromagnet. Phys. Rev. B—Condens. Matter Mater. Phys. 2006, 73, 100401. [Google Scholar] [CrossRef]
- Devesa, S.; Amorim, C.O.; Belo, J.H.; Araújo, J.P.; Teixeira, S.S.; Graça, M.P.F.; Costa, L.C. Comprehensive Characterization of Bi1.34Fe0.66Nb1.34O6.35 Ceramics: Structural, Morphological, Electrical, and Magnetic Properties. Magnetochemistry 2024, 10, 79. [Google Scholar] [CrossRef]
- Kuz’min, M.D.; Tishin, A.M. Temperature dependence of the spontaneous magnetisation of ferromagnetic insulators: Does it obey the 3/2–5/2–β law? Phys. Lett. A 2005, 341, 240–243. [Google Scholar] [CrossRef]
- Muscas, G.; Concas, G.; Laureti, S.; Testa, A.M.; Mathieu, R.; De Toro, J.A.; Cannas, C.; Musinu, A.; Novak, M.A.; Sangregorio, C. The interplay between single particle anisotropy and interparticle interactions in ensembles of magnetic nanoparticles. Phys. Chem. Chem. Phys. 2018, 20, 28634–28643. [Google Scholar] [CrossRef]
- Ondeck, C.L.; Habib, A.H.; Ohodnicki, P.; Miller, K.; Sawyer, C.A.; Chaudhary, P.; McHenry, M.E. Theory of magnetic fluid heating with an alternating magnetic field with temperature dependent materials properties for self-regulated heating. J. Appl. Phys. 2009, 105, 07B324. [Google Scholar] [CrossRef]
- Berndt, T.; Muxworthy, A.R.; Paterson, G.A. Determining the magnetic attempt time τ0, its temperature dependence, and the grain size distribution from magnetic viscosity measurements. J. Geophys. Res. Solid Earth 2015, 120, 7322–7336. [Google Scholar] [CrossRef]
- McNerny, K.L.; Kim, Y.; Laughlin, D.E.; McHenry, M.E. Chemical synthesis of monodisperse γ-Fe–Ni magnetic nanoparticles with tunable Curie temperatures for self-regulated hyperthermia. J. Appl. Phys. 2010, 107, 09A312. [Google Scholar] [CrossRef]
- Hernando, A.; Crespo, P.; García, M.A. Metallic magnetic nanoparticles. Sci. World J. 2005, 5, 972–1001. [Google Scholar] [CrossRef] [PubMed]
- Carvalho, J.P.F.; Vieira, T.; Silva, J.C.; Soares, P.I.P.; Ferreira, N.M.; Amorim, C.O.; Teixeira, S.S.; Graça, M.P.F. Potassium Ferrite for Biomedical Applications. Materials 2023, 16, 3880. [Google Scholar] [CrossRef] [PubMed]
- Natividad, E.; Castro, M.; Goglio, G.; Andreu, I.; Epherre, R.; Duguet, E.; Mediano, A. New insights into the heating mechanisms and self-regulating abilities of manganite perovskite nanoparticles suitable for magnetic fluid hyperthermia. Nanoscale 2012, 4, 3954–3962. [Google Scholar] [CrossRef] [PubMed]
- Nogués, J.; Sort, J.; Langlais, V.; Skumryev, V.; Suriñach, S.; Muñoz, J.S.; Baró, M.D. Exchange bias in nanostructures. Phys. Rep. 2005, 422, 65–117. [Google Scholar] [CrossRef]
- Shaterabadi, Z.; Nabiyouni, G.; Goya, G.F.; Soleymani, M. The effect of the magnetically dead layer on the magnetization and the magnetic anisotropy of the dextran-coated magnetite nanoparticles. Appl. Phys. A 2022, 128, 631. [Google Scholar] [CrossRef]
- Jin, L.; Jia, C.; Lindfors-Vrejoiu, I.; Zhong, X.; Du, H.; Dunin-Borkowski, R.E. Direct Demonstration of a Magnetic Dead Layer Resulting from A-Site Cation Inhomogeneity in a (La, Sr) MnO3 Epitaxial Film System. Adv. Mater. Interfaces 2016, 3, 1600414. [Google Scholar] [CrossRef]
- Curiale, J.; Granada, M.; Troiani, H.E.; Sánchez, R.D.; Leyva, A.G.; Levy, P.; Samwer, K. Magnetic dead layer in ferromagnetic manganite nanoparticles. Appl. Phys. Lett. 2009, 95, 043106. [Google Scholar] [CrossRef]
- Knobel, M.; Nunes, W.C.; Socolovsky, L.M.; De Biasi, E.; Vargas, J.M.; Denardin, J.C. Superparamagnetism and other magnetic features in granular materials: A review on ideal and real systems. J. Nanosci. Nanotechnol. 2008, 8, 2836–2857. [Google Scholar] [CrossRef] [PubMed]
- Millan, A.; Urtizberea, A.; Silva, N.J.O.; Palacio, F.; Amaral, V.S.; Snoeck, E.; Serin, V. Surface effects in maghemite nanoparticles. J. Magn. Magn. Mater. 2007, 312, L5–L9. [Google Scholar] [CrossRef]
- Kolhatkar, A.G.; Jamison, A.C.; Litvinov, D.; Willson, R.C.; Lee, T.R. Tuning the magnetic properties of nanoparticles. Int. J. Mol. Sci. 2013, 14, 15977–16009. [Google Scholar] [CrossRef]
- D’Ippolito, V.; Andreozzi, G.B.; Bersani, D.; Lottici, P.P. Raman fingerprint of chromate, aluminate and ferrite spinels. J. Raman Spectrosc. 2015, 46, 1255–1264. [Google Scholar] [CrossRef]
- Zhang, W.; Yu, X.; Li, H.; Dong, D.; Zuo, X.; Wu, C. Magnetic nanoparticles with low Curie temperature and high heating efficiency for self-regulating temperature hyperthermia. J. Magn. Magn. Mater. 2019, 489, 165382. [Google Scholar] [CrossRef]
- Joudeh, N.; Linke, D. Nanoparticle classification, physicochemical properties, characterization, and applications: A comprehensive review for biologists. J. Nanobiotechnol. 2022, 20, 262. [Google Scholar] [CrossRef]
- Mourdikoudis, S.; Pallares, R.M.; Thanh, N.T.K. Characterization techniques for nanoparticles: Comparison and complementarity upon studying nanoparticle properties. Nanoscale 2018, 10, 12871–12934. [Google Scholar] [CrossRef]
- Faraji, M.; Yamini, Y.; Rezaee, M.j.j. Magnetic nanoparticles: Synthesis, stabilization, functionalization, characterization, and applications. J. Iran. Chem. Soc. 2010, 7, 1–37. [Google Scholar] [CrossRef]
- Kefeni, K.K.; Msagati, T.A.M.; Mamba, B.B. Ferrite nanoparticles: Synthesis, characterisation and applications in electronic device. Mater. Sci. Eng. B 2017, 215, 37–55. [Google Scholar] [CrossRef]
- Rezaei, B.; Yari, P.; Sanders, S.M.; Wang, H.; Chugh, V.K.; Liang, S.; Mostufa, S.; Xu, K.; Wang, J.; Gómez-Pastora, J. Magnetic nanoparticles: A review on synthesis, characterization, functionalization, and biomedical applications. Small 2024, 20, 2304848. [Google Scholar] [CrossRef] [PubMed]
- Ali, A.; Shah, T.; Ullah, R.; Zhou, P.; Guo, M.; Ovais, M.; Tan, Z.; Rui, Y. Review on recent progress in magnetic nanoparticles: Synthesis, characterization, and diverse applications. Front. Chem. 2021, 9, 629054. [Google Scholar] [CrossRef]
- Galinetto, P.; Albini, B.; Bini, M.; Mozzati, M.C. Raman spectroscopy in zinc ferrites nanoparticles. Raman Spectrosc. 2018, 223, 228. [Google Scholar]
- Amorim, C.O.; Goncalves, J.N.; Tavares, D.S.; Fenta, A.S.; Lopes, C.B.; Pereira, E.; Trindade, T.; Correia, J.G.; Amaral, V.S. Ultra sensitive quantification of Hg2+ sorption by functionalized nanoparticles using radioactive tracker spectroscopy. Microchem. J. 2018, 138, 418–423. [Google Scholar] [CrossRef]
- Amorim, C.O.; Gonçalves, J.N.; Amaral, V.S. Exploiting Radioactive Isotopes: From Pollutant Tracking to Solid State studies using a combined ab initio and PAC approach. Eur. J. Inorg. Chem. 2020, 2020, 1822–1833. [Google Scholar] [CrossRef]
- Cramer, C.J.; Truhlar, D.G. Density functional theory for transition metals and transition metal chemistry. Phys. Chem. Chem. Phys. 2009, 11, 10757–10816. [Google Scholar] [PubMed]
- Carpino, F.; Zborowski, M.; Williams, P.S. Quadrupole magnetic field-flow fractionation: A novel technique for the characterization of magnetic nanoparticles. J. Magn. Magn. Mater. 2007, 311, 383–387. [Google Scholar] [CrossRef]
- Holzwarth, U.; Gibson, N. The Scherrer equation versus the “Debye-Scherrer equation”. Nat. Nanotechnol. 2011, 6, 534. [Google Scholar] [CrossRef] [PubMed]
- Mos, Y.M.; Vermeulen, A.C.; Buisman, C.J.N.; Weijma, J. X-Ray Diffraction of Iron Containing Samples: The Importance of a Suitable Configuration. Geomicrobiol. J. 2018, 35, 511–517. [Google Scholar] [CrossRef]
- Fernandes, T.; Nogueira, H.I.S.; Amorim, C.O.; Amaral, J.S.; Daniel-da-Silva, A.L.; Trindade, T. Chemical Strategies for Dendritic Magneto-plasmonic Nanostructures Applied to Surface-Enhanced Raman Spectroscopy. Chem.–A Eur. J. 2022, 28, e202202382. [Google Scholar] [CrossRef]
- Nekvapil, F.; Bunge, A.; Radu, T.; Cinta Pinzaru, S.; Turcu, R. Raman spectra tell us so much more: Raman features and saturation magnetization for efficient analysis of manganese zinc ferrite nanoparticles. J. Raman Spectrosc. 2020, 51, 959–968. [Google Scholar] [CrossRef]
- Amorim, C.O.; Sloyan, K.; Correia, M.R.; Lourenco, A.; Dahlem, M.S.; Amaral, V.S. Constrained titanohematite formation at BTO/Fe interfaces deposited by RF-sputtering. J. Alloys Compd. 2020, 828, 154244. [Google Scholar] [CrossRef]
- Shaikh, J.; Ankola, D.D.; Beniwal, V.; Singh, D.; Kumar, M.N.V.R. Nanoparticle encapsulation improves oral bioavailability of curcumin by at least 9-fold when compared to curcumin administered with piperine as absorption enhancer. Eur. J. Pharm. Sci. 2009, 37, 223–230. [Google Scholar] [CrossRef]
- Kesharwani, P.; Jain, K.; Jain, N.K. Dendrimer as nanocarrier for drug delivery. Prog. Polym. Sci. 2014, 39, 268–307. [Google Scholar] [CrossRef]
- Danaei, M.; Dehghankhold, M.; Ataei, S.; Hasanzadeh Davarani, F.; Javanmard, R.; Dokhani, A.; Khorasani, S.; Mozafari, M.R. Impact of particle size and polydispersity index on the clinical applications of lipidic nanocarrier systems. Pharmaceutics 2018, 10, 57. [Google Scholar] [CrossRef] [PubMed]
- Wohlfarth, E.P. The temperature dependence of the magnetic susceptibility of spin glasses. Phys. Lett. A 1979, 70, 489–491. [Google Scholar] [CrossRef]
- Tournus, F.; Tamion, A. Magnetic susceptibility curves of a nanoparticle assembly II. Simulation and analysis of ZFC/FC curves in the case of a magnetic anisotropy energy distribution. J. Magn. Magn. Mater. 2011, 323, 1118–1127. [Google Scholar] [CrossRef]
- de Almeida, A.A.; De Biasi, E.; Mansilla, M.V.; Valdés, D.P.; Troiani, H.E.; Urretavizcaya, G.; Torres, T.E.; Rodríguez, L.M.; Fregenal, D.E.; Bernardi, G.C. Magnetic hyperthermia experiments with magnetic nanoparticles in clarified butter oil and paraffin: A thermodynamic analysis. J. Phys. Chem. C 2020, 124, 27709–27721. [Google Scholar] [CrossRef]
- Lavorato, G.C.; Lima, E., Jr.; Tobia, D.; Fiorani, D.; Troiani, H.E.; Zysler, R.D.; Winkler, E.L. Size effects in bimagnetic CoO/CoFe2O4 core/shell nanoparticles. Nanotechnology 2014, 25, 355704. [Google Scholar] [CrossRef]
- Nunes, W.C.; Folly, W.S.D.; Sinnecker, J.P.; Novak, M.A. Temperature dependence of the coercive field in single-domain particle systems. Phys. Rev. B—Condens. Matter Mater. Phys. 2004, 70, 014419. [Google Scholar] [CrossRef]
- Amorim, C.O.; Mohseni, F.; Dumas, R.K.; Amaral, V.S.; Amaral, J.S. A geometry-independent moment correction method for the MPMS3 SQUID-based magnetometer. Meas. Sci. Technol. 2021, 32, 105602. [Google Scholar] [CrossRef]
- Stamenov, P.; Coey, J.M.D. Sample size, position, and structure effects on magnetization measurements using second-order gradiometer pickup coils. Rev. Sci. Instrum. 2006, 77, 015106. [Google Scholar] [CrossRef]
- Sawicki, M.; Stefanowicz, W.; Ney, A. Sensitive SQUID magnetometry for studying nanomagnetism. Semicond. Sci. Technol. 2011, 26, 064006. [Google Scholar] [CrossRef]
- Buchner, M.; Höfler, K.; Henne, B.; Ney, V.; Ney, A. Tutorial: Basic principles, limits of detection, and pitfalls of highly sensitive SQUID magnetometry for nanomagnetism and spintronics. J. Appl. Phys. 2018, 124, 161101. [Google Scholar] [CrossRef]
- Rao, P.A.; Rao, K.S.; Raju, T.R.K.P.; Kapusetti, G.; Choppadandi, M.; Varma, M.C.; Rao, K.H. A systematic study of cobalt-zinc ferrite nanoparticles for self-regulated magnetic hyperthermia. J. Alloys Compd. 2019, 794, 60–67. [Google Scholar]
- Zhang, W.; Zuo, X.; Niu, Y.; Wu, C.; Wang, S.; Guan, S.; Silva, S.R.P. Novel nanoparticles with Cr3+ substituted ferrite for self-regulating temperature hyperthermia. Nanoscale 2017, 9, 13929–13937. [Google Scholar] [CrossRef] [PubMed]
- Rajan, A.; Sahu, N.K. Review on magnetic nanoparticle-mediated hyperthermia for cancer therapy. J. Nanoparticle Res. 2020, 22, 319. [Google Scholar] [CrossRef]
- Atkinson, W.J.; Brezovich, I.A.; Chakraborty, D.P. Usable frequencies in hyperthermia with thermal seeds. IEEE Trans. Biomed. Eng. 1984, 31, 70–75. [Google Scholar] [CrossRef] [PubMed]
- Oskoui, P.R.; Rezvani, M. Revolution in Cancer Treatment Methods: Perspective Review of Factors Affecting the Final Results of Nanoparticles Used in Magnetic Fluid Hyperthermia. Health Sci. Rev. 2025; in press. [Google Scholar] [CrossRef]
- Herrero de la Parte, B.; Rodrigo, I.; Gutiérrez-Basoa, J.; Iturrizaga Correcher, S.; Mar Medina, C.; Echevarría-Uraga, J.J.; Garcia, J.A.; Plazaola, F.; García-Alonso, I. Proposal of new safety limits for in vivo experiments of magnetic hyperthermia antitumor therapy. Cancers 2022, 14, 3084. [Google Scholar] [CrossRef]
- Kwok, M.K.Y.; Maley, C.C.J.; Dworkin, A.; Hattersley, S.; Southern, P.; Pankhurst, Q.A. Nonspecific eddy current heating in magnetic field hyperthermia. Appl. Phys. Lett. 2023, 122, 240502. [Google Scholar] [CrossRef]
- Lee, J.-H.; Kim, B.; Kim, Y.; Kim, S.-K. Ultra-high rate of temperature increment from superparamagnetic nanoparticles for highly efficient hyperthermia. Sci. Rep. 2021, 11, 4969. [Google Scholar] [CrossRef] [PubMed]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amorim, C.O. A Compendium of Magnetic Nanoparticle Essentials: A Comprehensive Guide for Beginners and Experts. Pharmaceutics 2025, 17, 137. https://doi.org/10.3390/pharmaceutics17010137
Amorim CO. A Compendium of Magnetic Nanoparticle Essentials: A Comprehensive Guide for Beginners and Experts. Pharmaceutics. 2025; 17(1):137. https://doi.org/10.3390/pharmaceutics17010137
Chicago/Turabian StyleAmorim, Carlos O. 2025. "A Compendium of Magnetic Nanoparticle Essentials: A Comprehensive Guide for Beginners and Experts" Pharmaceutics 17, no. 1: 137. https://doi.org/10.3390/pharmaceutics17010137
APA StyleAmorim, C. O. (2025). A Compendium of Magnetic Nanoparticle Essentials: A Comprehensive Guide for Beginners and Experts. Pharmaceutics, 17(1), 137. https://doi.org/10.3390/pharmaceutics17010137





