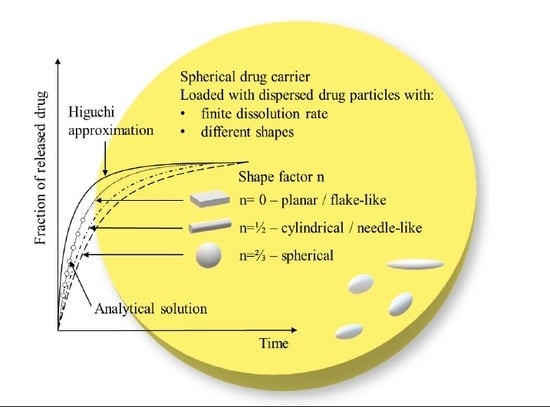
Drug Release from a Spherical Matrix: Theoretical Analysis for a Finite Dissolution Rate Affected by Geometric Shape of Dispersed Drugs
Abstract
1. Introduction
2. Methods
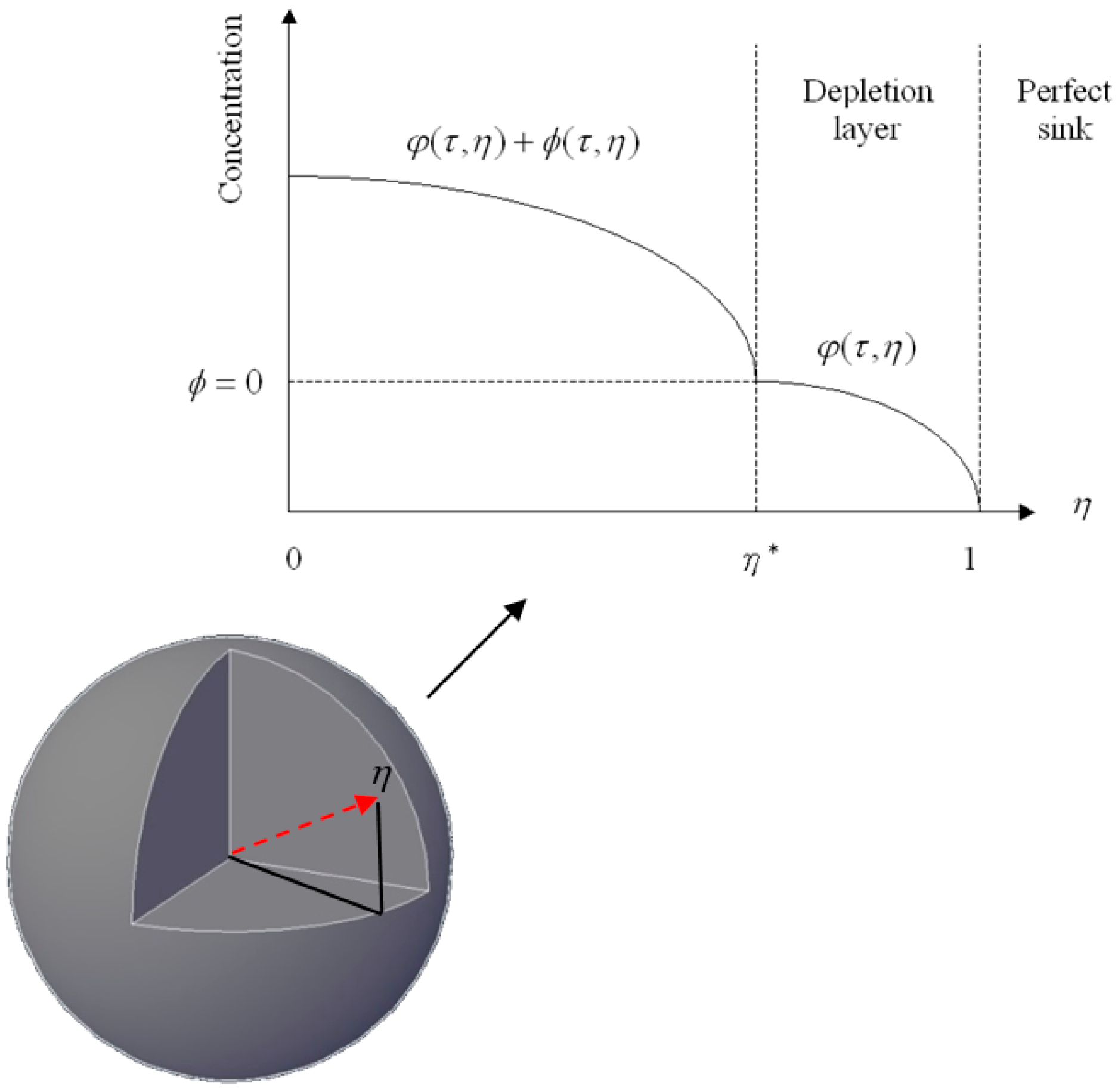
2.1. Mathematical Formulation
2.2. Critical Time for the Formation of a Surface Depletion Zone
2.3. Special Case of Planar Drugs
2.4. Numerical Solutions
2.5. Higuchi’s Model
3. Results and Discussion
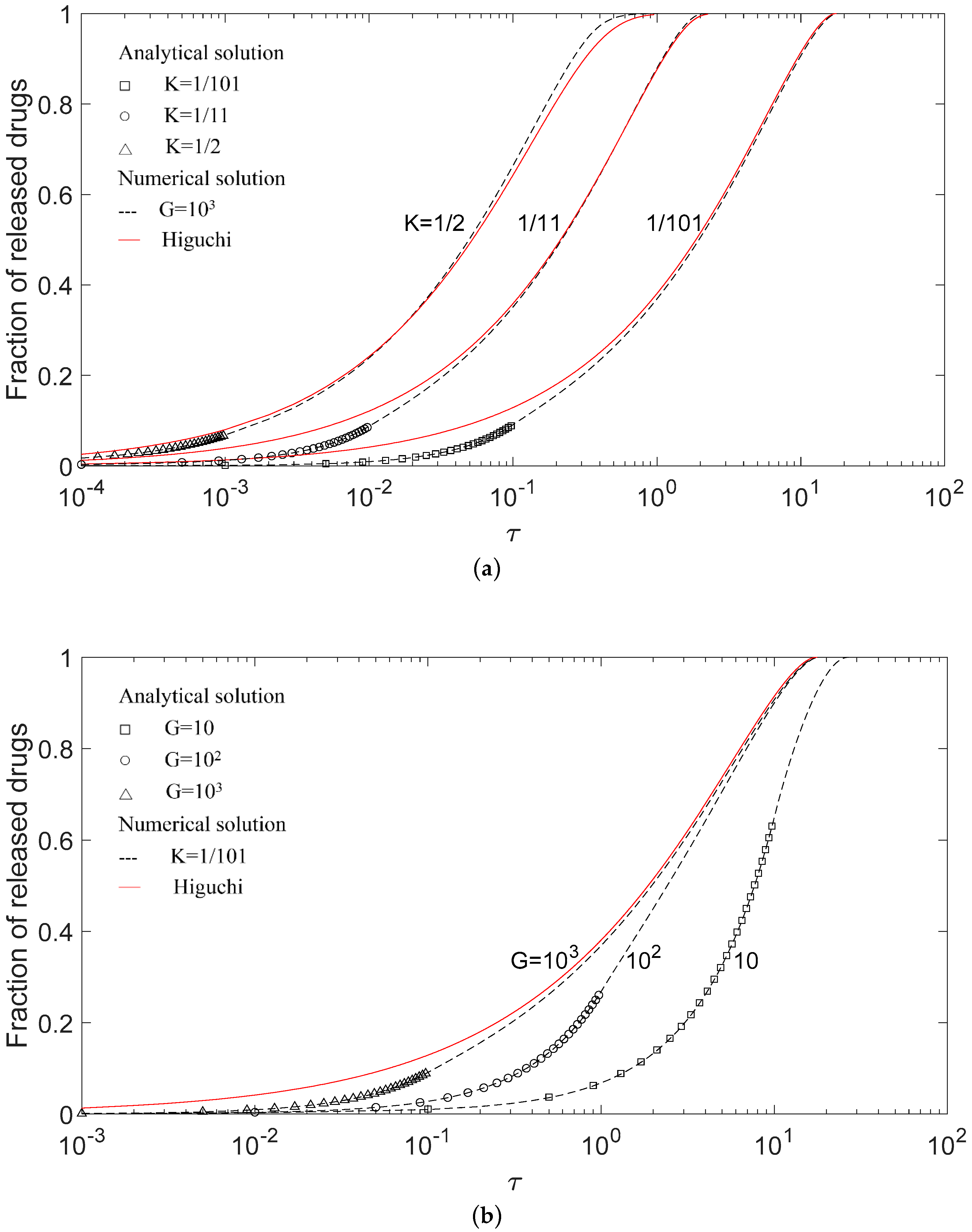
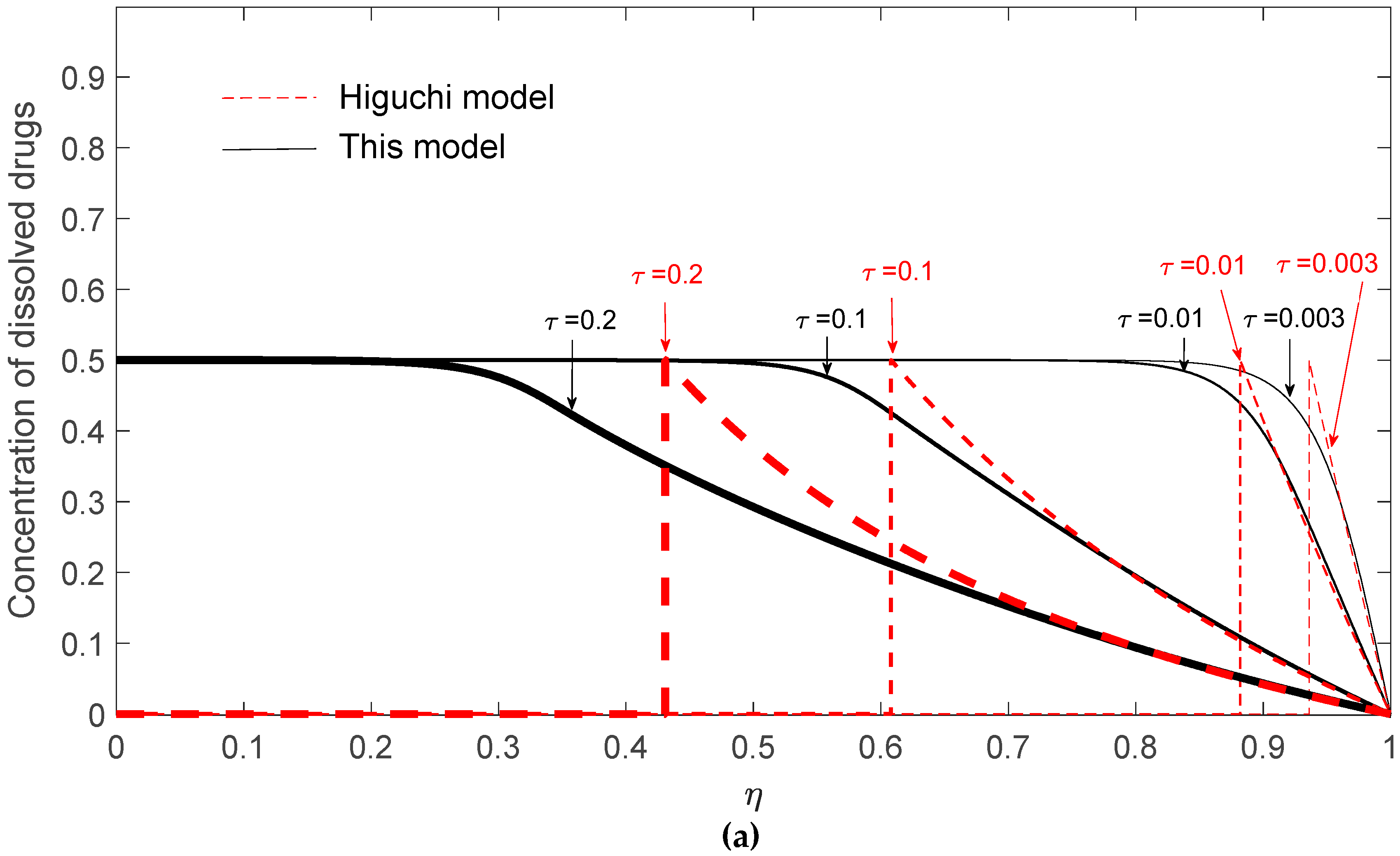
3.1. Validation of the Model
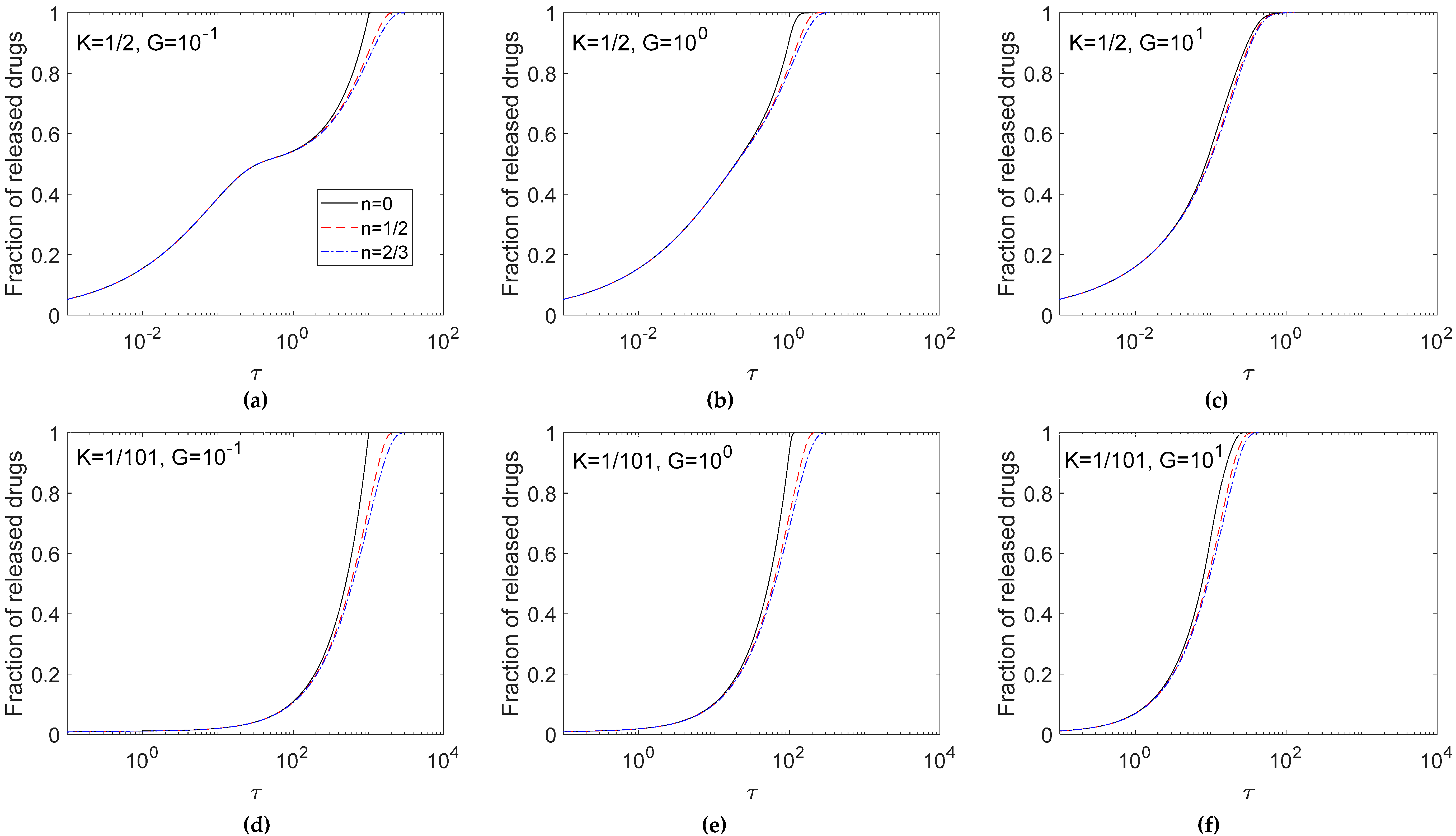
3.2. Effects of the Drug Shape Factor
3.3. Identification of Constant Release Rate Region
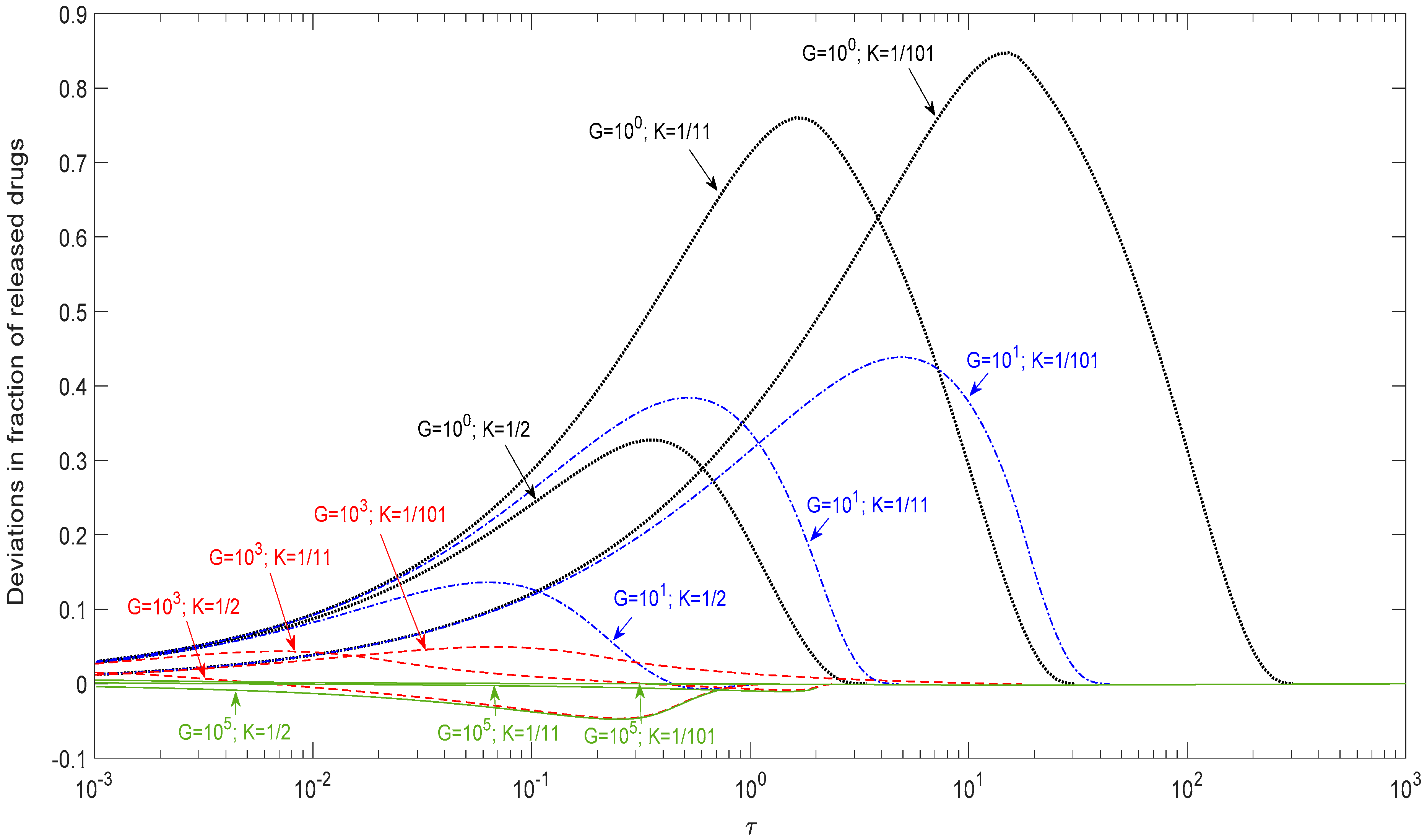
3.4. Evaluation of Possible Deviation by Higuchi Approximation
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| A | drug surface area per volume unit sphere, 1/cm |
| Ao | initial drug surface area per volume unit sphere, 1/cm |
| C(r,t) | dissolved drug concentration, g/mL |
| Ca(r,t) | un-dissolved drug concentration, g/mL |
| Cao | initial un-dissolved drug concentration, g/mL |
| Cs | solubility of drug in matrix, g/mL |
| Ct | initial total drug concentration Ct = Cao + Cs, g/mL |
| D | diffusivity of drug in matrix, cm2/s |
| G | ratio of diffusion rate to dissolution rate kAoro2/D |
| K | ratio of drug solubility to initial total drug concentration Cs/Ct |
| k | dissolution rate constant, 1/(cm2·s) |
| n | shape factor of a drug particle |
| r | radial coordinate from the left of a sphere, cm |
| ro | radius of a sphere, cm |
| r* | moving front, cm |
| t | time, s |
| u | unit step function |
| Greek symbols | |
| φ(η,τ) | dimensionless dissolved concentration C/Ct |
| ϕ(η,τ) | dimensionless un-dissolved concentration Ca/Ct |
| η | dimensionless radius r/ro |
| η* | dimensionless moving front η/ro |
| τ | dimensionless time Dt/ |
| τc | dimensionless critical time |
| τs | dimensionless starting time of constant release rate region |
References
- Siepmann, J.; Peppas, N.A. Modeling of drug release from delivery systems based on hydroxypropyl methylcellulose (HPMC). Adv. Drug Deliver. Rev. 2001, 48, 139–157. [Google Scholar] [CrossRef]
- Wang, F.; Saidel, G.M.; Gao, J. A mechanistic model of controlled drug release from polymer millirods: Effects of excipients and complex binding. J. Control. Rel. 2007, 119, 111–120. [Google Scholar] [CrossRef] [PubMed]
- Lin, L.Y.; Lee, N.S.; Zhu, J.; Nyström, A.M.; Pochan, D.J.; Dorshow, R.B.; Wooley, K.L. Tuning core vs. shell dimensions to adjust the performance of nanoscopic containers for the loading and release of doxorubicin. J. Control. Rel. 2011, 152, 37–48. [Google Scholar] [CrossRef] [PubMed]
- Higuchi, T. Rate of release of medicaments from ointment bases containing drugs in suspension. J. Pharm. Sci. 1961, 50, 874–875. [Google Scholar] [CrossRef] [PubMed]
- Higuchi, T. Mechanism of sustained-action medication: Theoretical analysis of rate of release of solid drugs dispersed in solid matrices. J. Pharm. Sci. 1963, 52, 1145–1149. [Google Scholar] [CrossRef]
- Fan, L.T.; Singh, S.K. Diffusion-controlled release. In Controlled Release: A Quantitative Treatment; Springer-Verlag: Berlin/Heidelberg, Germany, 1989; pp. 9–88. [Google Scholar]
- Siepmann, J.; Siepmann, F. Mathematical modeling of drug delivery. Int. J. Pharm. 2008, 364, 328–343. [Google Scholar] [CrossRef]
- Siepmann, J.; Siepmann, F. Modeling of diffusion controlled drug delivery. J. Control. Rel. 2012, 161, 351–362. [Google Scholar] [CrossRef]
- Siepmann, J.; Gopferich, A. Mathematical modeling of bioerodible, polymer drug delivery systems. Adv. Drug Deliver. Rev. 2001, 48, 229–247. [Google Scholar] [CrossRef]
- Castillo, M.E.; Morin, P. On a dissolution–diffusion model. Existence, uniqueness, regularity and simulations. Comput. Math. Appl. 2015, 70, 1887–1905. [Google Scholar] [CrossRef]
- Siepmann, J.; Siepmann, F. Mathematical modeling of drug dissolution. Int. J. Pharm. 2013, 453, 12–24. [Google Scholar] [CrossRef]
- Manhas, V.; Guyot, Y.; Kerckhofs, G.; Chai, Y.C.; Geris, L. Computational modelling of local calcium ions release from calcium phosphate-based scaffolds. Biomech. Model Mechanobiol. 2017, 16, 425–438. [Google Scholar] [CrossRef]
- Lindstrom, F.T.; Ayres, J.W. Diffusion model for drug release from suspensions II: Release to a perfect sink. J. Pharm. Sci. 1977, 66, 662–668. [Google Scholar] [CrossRef]
- Ayres, J.W.; Lindstrom, F.T. Diffusion model for drug release from suspensions I: Theoretical considerations. J. Pharm. Sci. 1977, 66, 654–662. [Google Scholar] [CrossRef] [PubMed]
- Gurny, R.; Doelker, E.; Peppas, N.A. Modelling of sustained release of water-soluble drugs from porous, hydrophobic polymers. Biomaterials 1982, 3, 27–32. [Google Scholar] [CrossRef]
- Peppas, N.A. A model of dissolution-controlled solute release from porous drug delivery polymeric systems. J. Biomed. Mater. Res. 1983, 17, 1079–1087. [Google Scholar] [CrossRef] [PubMed]
- Harland, R.S.; Dubernet, C.; Benoit, J.P.; Peppas, N.A. A model of dissolution-controlled, diffusional drug release from non-swellable polymeric microspheres. J. Control. Rel. 1988, 7, 207–215. [Google Scholar] [CrossRef]
- Lin, Y.S.; Tsay, R.Y. The effects of the finite dissolution rate on the drug release of spherical particles loaded with uniformly dispersed drugs. J. Chin. Inst. Chem. Engrs. 2004, 35, 667–676. [Google Scholar]
- Frenning, G. Theoretical investigation of drug release from planar matrix systems: Effects of a finite dissolution rate. J. Control. Rel. 2003, 92, 331–339. [Google Scholar] [CrossRef]
- Frenning, G. Theoretical analysis of the release of slowly dissolving drugs from spherical matrix systems. J. Control. Rel. 2004, 95, 109–117. [Google Scholar] [CrossRef]
- Frenning, G.; Brohede, U.; Stromme, M. Finite element analysis of the release of slowly dissolving drugs from cylindrical matrix systems. J. Control. Rel. 2005, 107, 320–329. [Google Scholar] [CrossRef]
- Cabrera, M.I.; Luna, J.A.; Grau, R.J.A. Modeling of dissolution-diffusion controlled drug release from planar polymeric systems with finite dissolution rate and arbitrary drug loading. J. Membr. Sci. 2006, 280, 693–704. [Google Scholar] [CrossRef]
- Cabrera, M.I.; Grau, R.J.A. A generalized integral method for solving the design equations of dissolution/diffusion-controlled drug release from planar, cylindrical and spherical matrix devices. J. Membr. Sci. 2007, 293, 1–14. [Google Scholar] [CrossRef]
- Noyes, A.A.; Whitney, W.R. The rate of solution of solid substances in their own solutions. J. Am. Chem. Soc. 1897, 19, 930–934. [Google Scholar] [CrossRef]
- Frenning, G.; Stromme, M. Drug release modeled by dissolution, diffusion, and immobilization. Int. J. Pharm. 2003, 250, 137–145. [Google Scholar] [CrossRef]
- Blagoeva, R.; Nedev, A. A problem for drug release from 2D polymeric systems. Mech. Res. Commun. 2008, 35, 344–349. [Google Scholar] [CrossRef]
- Frenning, G. Modelling drug release from inert matrix systems: From moving-boundary to continuous-field descriptions. Int. J. Pharm. 2011, 418, 88–99. [Google Scholar] [CrossRef]
- Lee, P.I. Modeling of drug release from matrix systems involving moving boundaries: Approximate analytical solutions. Int. J. Pharm. 2011, 418, 18–27. [Google Scholar] [CrossRef]
- Nuxoll, E. Added release time in diffusion/dissolution coupled release. Int. J. Pharm. 2015, 494, 195–204. [Google Scholar] [CrossRef]
- Skeel, R.D.; Berzins, M. A method for the spatial discretization of parabolic equations in one space variable. SIAM J. Sci. Stat. Comput. 1990, 11, 1–32. [Google Scholar] [CrossRef]
- Chandrasekaran, S.K.; Paul, D.R. Dissolution-controlled transport from dispersed matrixes. J. Pharm. Sci. 1982, 71, 1399–1402. [Google Scholar] [CrossRef]


| K | G | Time Region of Constant Release Rate (τs–τc) | n | Release Rate | Coverage of Constant Release Rate (%) | |
|---|---|---|---|---|---|---|
| Range | Middle | |||||
| 1/2 | 10−1 | 0.91–10 | 0 | 5.02 × 10−2–4.97 × 10−2 | 4.97 × 10-2 | 45.77 |
| 1/2 | 4.84 × 10−2–2.65 × 10−2 | 3.75 × 10-2 | 33.17 | |||
| 2/3 | 4.78 × 10−2–2.36 × 10−2 | 3.57 × 10−2 | 30.99 | |||
| 100 | 0.59–1 | 0 | 4.75 × 10−1–4.70 × 10−1 | 4.73 × 10−1 | 20.66 | |
| 1/2 | 3.77 × 10−1–2.84 × 10−1 | 3.31 × 10−1 | 13.36 | |||
| 2/3 | 3.54 × 10−1–2.56 × 10−1 | 3.05 × 10−1 | 12.31 | |||
| 101 | Negligible–0.1 | 0 | - | - | - | |
| 1/2 | - | - | - | |||
| 2/3 | - | - | - | |||
| 1/101 | 10−1 | 0.91–1000 | 0 | 9.94 × 10−4–9.84 × 10−4 | 9.89 × 10−4 | 98.28 |
| 1/2 | 9.94 × 10−4–5.19 × 10−4 | 7.57 × 10−4 | 73.92 | |||
| 2/3 | 9.94 × 10−4–4.62 × 10−4 | 7.28 × 10−4 | 69.39 | |||
| 100 | 0.61–100 | 0 | 9.40 × 10−3–9.30 × 10−3 | 9.35 × 10−3 | 92.60 | |
| 1/2 | 9.38 × 10−3–5.21 × 10−3 | 7.30 × 10−3 | 71.29 | |||
| 2/3 | 9.38 × 10−3–4.65 × 10−3 | 7.02 × 10−3 | 67.13 | |||
| 101 | 0.2–10 | 0 | 6.52 × 10−2–6.46 × 10−2 | 6.49 × 10−2 | 66.88 | |
| 1/2 | 6.49 × 10−2–4.57 × 10−2 | 5.53 × 10−2 | 53.98 | |||
| 2/3 | 6.48 × 10−2–4.24 × 10−2 | 5.36 × 10−2 | 51.85 | |||
| G | 100 | 101 | 103 | 105 | |
|---|---|---|---|---|---|
| K | |||||
| 1/2 | 32.7 | 13.6 | −4.6 | −4.7 | |
| 1/11 | 76.0 | 38.4 | 4.3 | −1.1 | |
| 1/101 | 84.7 | 43.9 | 4.9 | 0.5 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, Y.-S.; Tsay, R.-Y. Drug Release from a Spherical Matrix: Theoretical Analysis for a Finite Dissolution Rate Affected by Geometric Shape of Dispersed Drugs. Pharmaceutics 2020, 12, 582. https://doi.org/10.3390/pharmaceutics12060582
Lin Y-S, Tsay R-Y. Drug Release from a Spherical Matrix: Theoretical Analysis for a Finite Dissolution Rate Affected by Geometric Shape of Dispersed Drugs. Pharmaceutics. 2020; 12(6):582. https://doi.org/10.3390/pharmaceutics12060582
Chicago/Turabian StyleLin, Yung-Sheng, and Ruey-Yug Tsay. 2020. "Drug Release from a Spherical Matrix: Theoretical Analysis for a Finite Dissolution Rate Affected by Geometric Shape of Dispersed Drugs" Pharmaceutics 12, no. 6: 582. https://doi.org/10.3390/pharmaceutics12060582
APA StyleLin, Y.-S., & Tsay, R.-Y. (2020). Drug Release from a Spherical Matrix: Theoretical Analysis for a Finite Dissolution Rate Affected by Geometric Shape of Dispersed Drugs. Pharmaceutics, 12(6), 582. https://doi.org/10.3390/pharmaceutics12060582