Partial Solvation Parameters of Drugs as a New Thermodynamic Tool for Pharmaceutics
Abstract
:1. Introduction
2. Theory of the Partial Solvation Parameter (PSP) Approach
2.1. Definitions
2.2. Mixture Thermodynamics
2.3. Surface Energy Components and Wetting Phenomena
3. Materials and Experimental Methods
3.1. Materials
3.2. Differential Scanning Calorimetry
3.3. Inverse Gas Chromatography
3.4. Solubility Measurements and X-ray Analysis of Residual Solid
3.5. Software Used for LSER Calculation and Statistical Data Evaluation
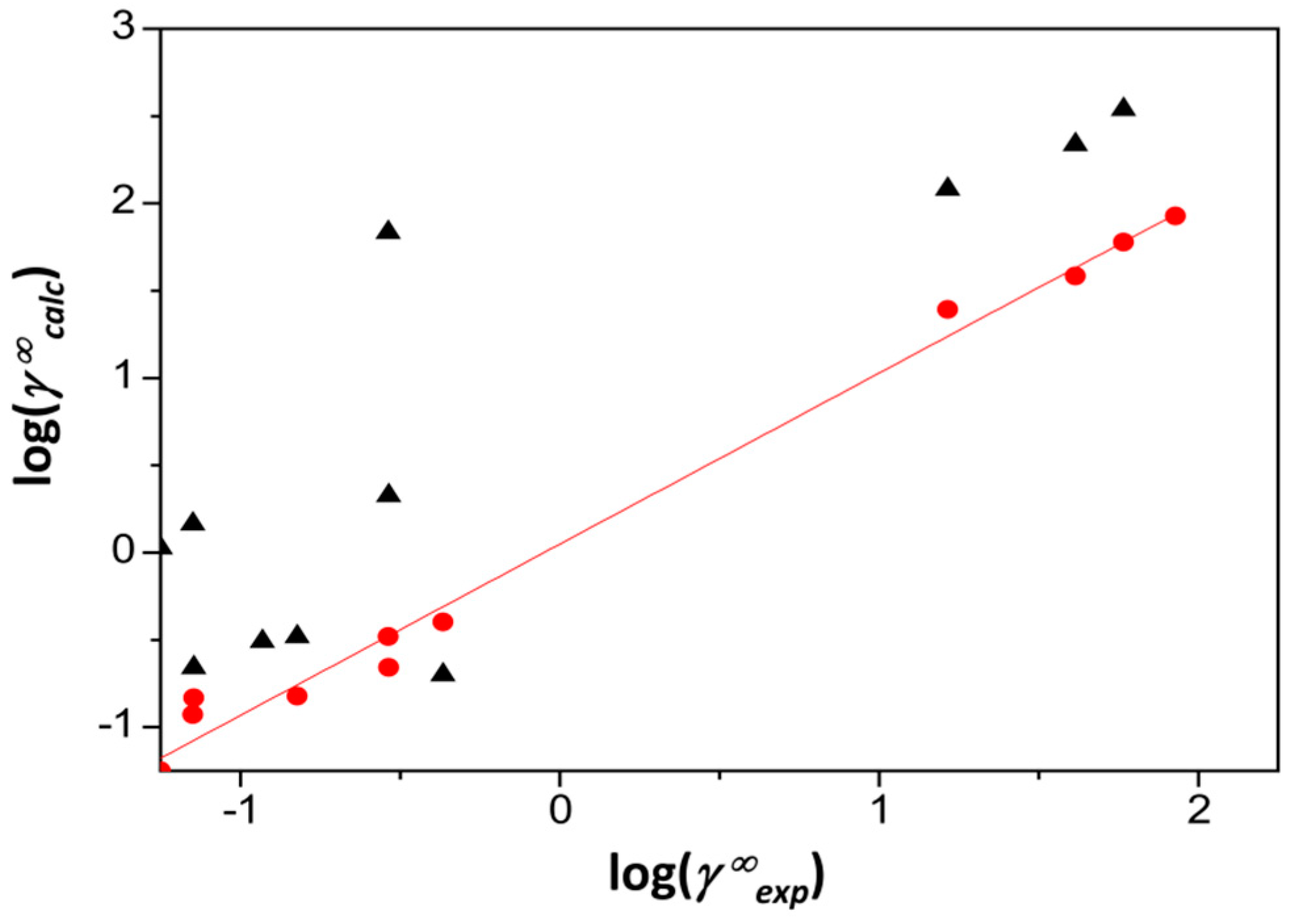
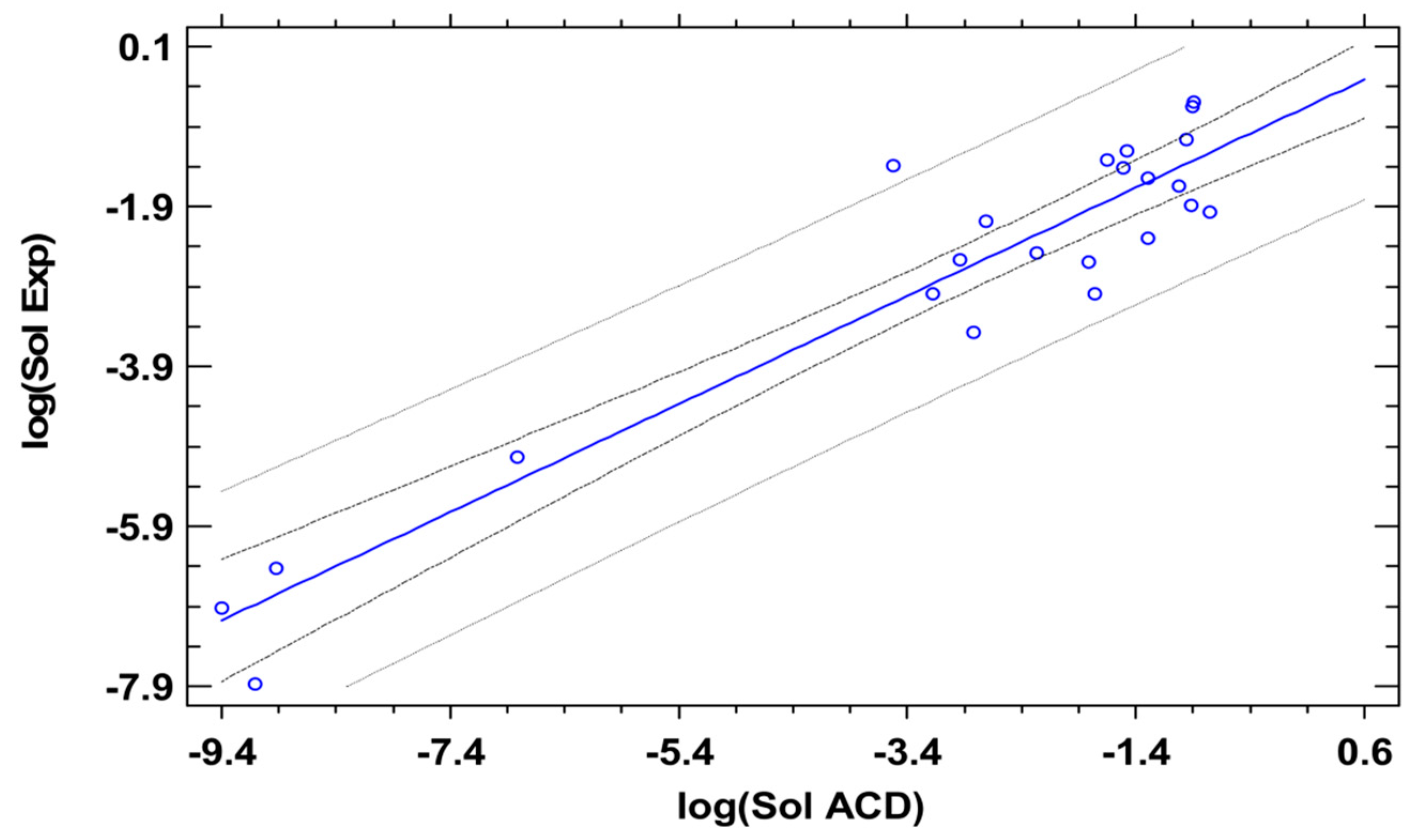
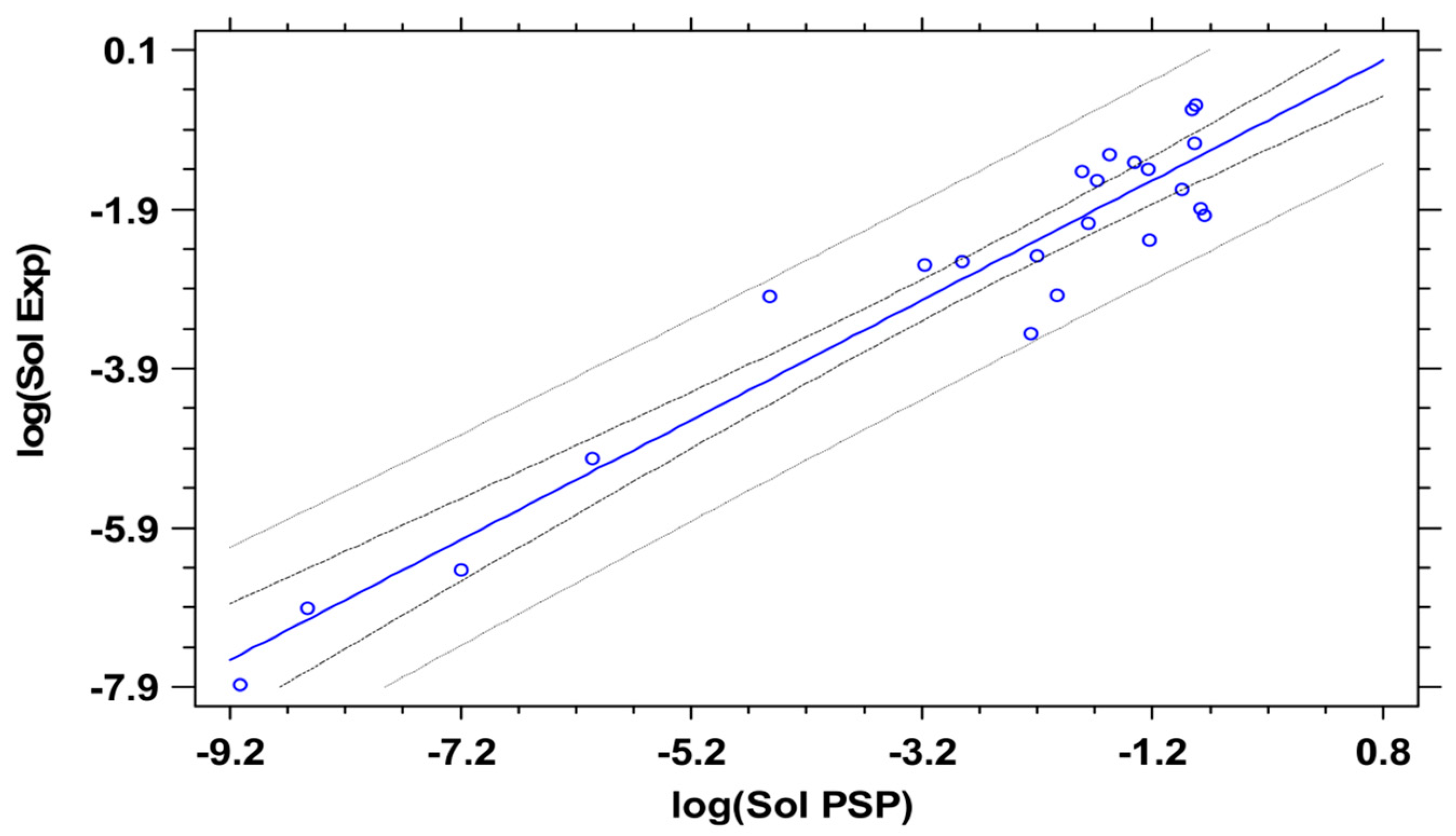
4. Results and Discussion
4.1. PSP of Solvents
4.2. Applications to Pharmaceutical Drugs
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Hansen, C.M. Hansen Solubility Parameters. A User’s Handbook; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Abbott, S.; Yamamoto, H.; Hansen, C.M. Hansen Solubility Parameters in Practice. Complete with Software, Data and Examples, 3rd ed.; Version 3.1.20; Hansen-Solubility.com: Ipswich, UK, 2010. [Google Scholar]
- Jankovic, S.; Tsakiridou, G.; Ditzinger, F.; Koehl, N.J.; Price, D.J.; Ilie, A.R.; Kalantzi, L.; Kimpe, K.; Holm, R.; Nair, A.; et al. Application of the solubility parameter concept to assist with oral delivery of poorly water-soluble drugs—A PEARRL Review. J. Pharm. Pharmacol. 2018. [Google Scholar] [CrossRef] [PubMed]
- Louwerse, M.J.; Maldonado, A.G.; Rousseau, S.; Moreau-Masselon, C.; Roux, B.; Rothenberg, G. Revisiting Hansen solubility parameters by including thermodynamics. Chem. Phys. Chem. 2017, 18, 2999–3006. [Google Scholar] [CrossRef] [PubMed]
- Abraham, M.H. Scales of solute hydrogen-bonding: Their construction and application to physico-chemical and biochemical processes. Chem. Soc. Rev. 1993, 22, 73–83. [Google Scholar] [CrossRef]
- Abraham, M.H.; Ibrahim, A.; Zissimos, A.M. Determination of sets of solute descriptors from chromatographic measurements J. Chromatogr. A 2004, 1037, 29–47. [Google Scholar] [CrossRef]
- Endo, S.; Watanabe, N.; Ulrich, N.; Bronner, G.; Goss, K.-U. UFZ-LSER Database v 2.1 [Internet]. Leipzig. Germany. Helmholtz Centre for Environmental Research-UFZ. 2015. Available online: https://www.ufz.de/index.php?en=31698&contentonly=1&m=0&lserd_data[mvc]=Public/start (accessed on 15 May 2018).
- Abraham, M.H.; Chadha, H.S.; Whiting, G.S.; Mitchell, R.C. Hydrogen bonding. 32. An analysis of water-octanol and water-alkane partitioning and the ΔlogP parameter of Seiler. J. Pharm. Sci. 1994, 83, 1085–1100. [Google Scholar] [CrossRef] [PubMed]
- Abraham, M.H.; Chadha, H.S.; Dixon, J.; Leo, A.J. Hydrogen bonding. 39. The partition of solutes between water and various alcohols. Phys. Org. Chem. 1994, 7, 712–716. [Google Scholar] [CrossRef]
- Abraham, M.H.; Zissimos, A.M.; Acree, W.E., Jr. Partition of solutes into wet and dry ethers; an LFER analysis. New J. Chem. 2003, 27, 1041–1044. [Google Scholar] [CrossRef]
- Abraham, M.H.; Joelle, L. The correlation and prediction of the solubility of compounds in water using an amended solvation energy relationship. J. Pharm. Sci. 1999, 88, 868–880. [Google Scholar] [CrossRef]
- Burns, S.T.; Khaledi, M.G. Rapid determination of liposome-water partition coefficients (KIw) using liposome electrokinetic chromatography. J. Pharm. Sci. 2002, 91, 1601–1612. [Google Scholar] [CrossRef]
- Niederquell, A.; Kuentz, M. Biorelevant drug solubility enhancement modeled by a linear solvation energy relationship. J. Pharm. Sci. 2018, 107, 503–506. [Google Scholar] [CrossRef]
- Abraham, M.H. Human intestinal absorption—Neutral molecules and ionic species. J. Pharm. Sci. 2014, 103, 1956–1966. [Google Scholar] [CrossRef] [PubMed]
- Platts, J.A.; Abraham, M.H.; Zhao, Y.H.; Hersey, A.; Ijaz, L.; Butina, D. Correlation and prediction of a large blood–brain distribution data set—An LFER study. Eur. J. Med. Chem. 2001, 36, 719–730. [Google Scholar] [CrossRef]
- Panayiotou, C. Redefining solubility parameters: The partial solvation parameters. Phys. Chem. Chem. Phys. 2012, 14, 3882–3908. [Google Scholar] [CrossRef]
- Panayiotou, C. Partial solvation parameters and mixture thermodynamics. Phys. Chem. B 2012, 116, 7302–7321. [Google Scholar] [CrossRef]
- Panayiotou, C. Inverse gas chromatography and partial solvation parameters. Chromatogr. A 2012, 1251, 194–207. [Google Scholar] [CrossRef]
- Panayiotou, C.; Mastrogeorgopoulos, S.; Aslanidou, D.; Avgidou, M.; Hatzimanikatis, V.J. Redefining solubility parameters: Bulk and surface properties from unified molecular descriptors. Chem. Thermodyn. 2017, 111, 207–2020. [Google Scholar] [CrossRef]
- Mastrogeorgopoulos, S.; Hatzimanikatis, V.; Panayiotou, C. Toward a simple predictive molecular thermodynamic model for bulk phases and interfaces. Ind. Eng. Chem. Res. 2017, 56, 10900–10910. [Google Scholar] [CrossRef]
- Panayiotou, C. Thermodynamic characterization of polymers. Polymer 2018, 136, 47–61. [Google Scholar] [CrossRef]
- Klamt, A.; Eckert, F. COSMO-RS: A novel and efficient method for the a priori prediction of thermophysical data of liquids. Fluid Phase Equilib. 2000, 172, 43–72. [Google Scholar] [CrossRef]
- Klamt, A. COSMO-RS from Quantum Chemistry to Fluid Phase Thermodynamics and Drug Design; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- COSMObase Ver. C30_1401; COSMOlogic GmbH & Co. K.G.: Leverkusen, Germany, 2014.
- Klamt, A.; Schüürmann, G.J. COSMO: A new approach to dielectric screening in solvents with explicit expressions for the screening energy and its gradient. J. Chem. Soc. Perkin Trans. II 1993, 2, 799–805. [Google Scholar] [CrossRef]
- Schäfer, A.; Klamt, A.; Sattel, D.; Lohrenz, J.C.W.; Eckert, F. COSMO Implementation in TURBOMOLE: Extension of an efficient quantum chemical code towards liquid systems. Phys. Chem. Chem. Phys. 2000, 2, 2187–2193. [Google Scholar] [CrossRef]
- Lin, S.T.; Sandler, S.I. A priori phase equilibrium prediction from a segment contribution solvation model. Ind. Eng. Chem. Res. 2002, 41, 899–913. [Google Scholar] [CrossRef]
- Grensemann, H.; Gmehling, J. Performance of a conductor-like screening model for real solvents model in comparison to classical group contribution methods. Ind. Eng. Chem. Res. 2005, 44, 1610–1624. [Google Scholar] [CrossRef]
- Pye, C.C.; Ziegler, T.; van Lenthe, E.; Louwen, J.N. An implementation of the conductor-like screening model of solvation within the Amsterdam density functional package—Part II. COSMO for real solvents. Can. J. Chem. 2009, 87, 790–797. [Google Scholar] [CrossRef]
- Huang, S.H.; Radosz, M. Equation of state for small, large, polydisperse, and associating molecules. Ind. Eng. Chem. Res. 1990, 29, 2284–2294. [Google Scholar] [CrossRef]
- Gross, J.; Sadowski, G. Perturbed-chain SAFT: An equation of state based on a perturbation theory for chain molecules. Ind. Eng. Chem. Res. 2001, 40, 1244–1260. [Google Scholar] [CrossRef]
- Panayiotou, C.; Pantoula, M.; Stefanis, E.; Tsivintzelis, I.; Economou, I.G. Nonrandom hydrogen-bonding model of fluids and their mixtures. 1. Pure fluids. Ind. Eng. Chem. Res. 2004, 43, 6592–6606. [Google Scholar] [CrossRef]
- Panayiotou, C.; Tsivintzelis, I.; Economou, I. Nonrandom hydrogen-bonding model of fluids and their mixtures. 2. Multicomponent mixtures. Ind. Eng. Chem. Res. 2007, 46, 2628–2636. [Google Scholar] [CrossRef]
- Panayiotou, C.; Aslanidou, D. Partial solvation parameters and the equation-of-state approach. Fluid Phase Equilibr. 2015, 406, 101–115. [Google Scholar] [CrossRef]
- Abrams, D.S.; Prausnitz, J.M. Statistical thermodynamics of liquid mixtures: A new expression for the excess Gibbs energy of partly or completely miscible systems. AIChE J. 1975, 21, 116–128. [Google Scholar] [CrossRef]
- Macedo, E.A.; Weidlich, U.; Gmehling, J.; Rasmussen, P. Vapor-liquid equilibriums by UNIFAC group contribution. Revision and extension. 3. Ind. Eng. Chem. Process Des. Dev. 1983, 22, 676–678. [Google Scholar] [CrossRef]
- Staverman, A.J. The entropy of high polymer solutions. Generalization of formulae. Recl. Trav. Chim. Pays-Bas 1950, 69, 163–174. [Google Scholar] [CrossRef]
- Guggenheim, E.A. Mixtures; Oxford University Press: Oxford, UK, 1951. [Google Scholar]
- Flory, P.J. Principles of Polymer Chemistry; Cornell University Press: Ithaca, NY, USA, 1953. [Google Scholar]
- Hildebrand, J.; Scott, R.L. The Solubility of Nonelectrolytes, 3rd ed.; Rheinhold: New York, NY, USA, 1950. [Google Scholar]
- Veytsman, B.A. Are lattice models valid for fluids with hydrogen bonds? J. Phys. Chem. 1990, 94, 8499–8500. [Google Scholar] [CrossRef]
- Panayiotou, C.; Sanchez, I.C. Hydrogen bonding in fluids: An equation-of-state approach. J. Phys. Chem. 1991, 95, 10090–10097. [Google Scholar] [CrossRef]
- Missopolinou, D.; Panayiotou, C. Hydrogen-bonding cooperativity and competing inter- and intramolecular associations: A unified approach. J. Phys. Chem. A 1998, 102, 3574–3581. [Google Scholar] [CrossRef]
- Kramer, A.; Thodos, G. Adaptation of the Flory-Huggins theory for modeling supercritical solubilities of solids. Ind. Eng. Chem. Res. 1988, 27, 1506–1510. [Google Scholar] [CrossRef]
- Prausnitz, J.M.; Lichtentaler, R.N.; de Azevedo, E.G. Molecular Thermodynamics of Fluid-Phase Equilibria; Prentice-Hall: Upper Saddle River, NJ, USA, 1999. [Google Scholar]
- Yalkowsky, S.H.; Banerjee, S. Aqueous Solubility: Methods of Estimation for Organic Compounds; Marcel Dekker: New York, NY, USA, 1992. [Google Scholar]
- Van Oss, C.J.; Chaudhury, M.K.; Good, R. Interfacial Lifshitz-van der Waals and polar interactions in macroscopic systems. J. Chem. Rev. 1988, 88, 927–941. [Google Scholar] [CrossRef]
- Panayiotou, C. Handbook of Surface and Colloid Chemistry, 4th ed.; Birdi, K., Ed.; CRC Press: Boca Raton, FL, USA, 2016; pp. 601–622. [Google Scholar]
- Wyttenbach, N.; Alsenz, J.; Grassman, O. Miniaturized Assay for Solubility and Residual Solid Screening (SORESOS) in Early Drug Development. Pharm. Res. 2007, 24, 888–898. [Google Scholar] [CrossRef]
- Dorris, G.M.; Gray, D.G. Adsorption of n-alkanes at zero surface coverage on cellulose paper and wood fibers. J. Colloid Interface Sci. 1980, 77, 353. [Google Scholar] [CrossRef]
- Otte, A.; Carvajal, M.E. Assessment of Milling-Induced Disorder of Two Pharmaceutical Compounds. J. Pharm. Sci. 2011, 100, 1793–1804. [Google Scholar] [CrossRef]
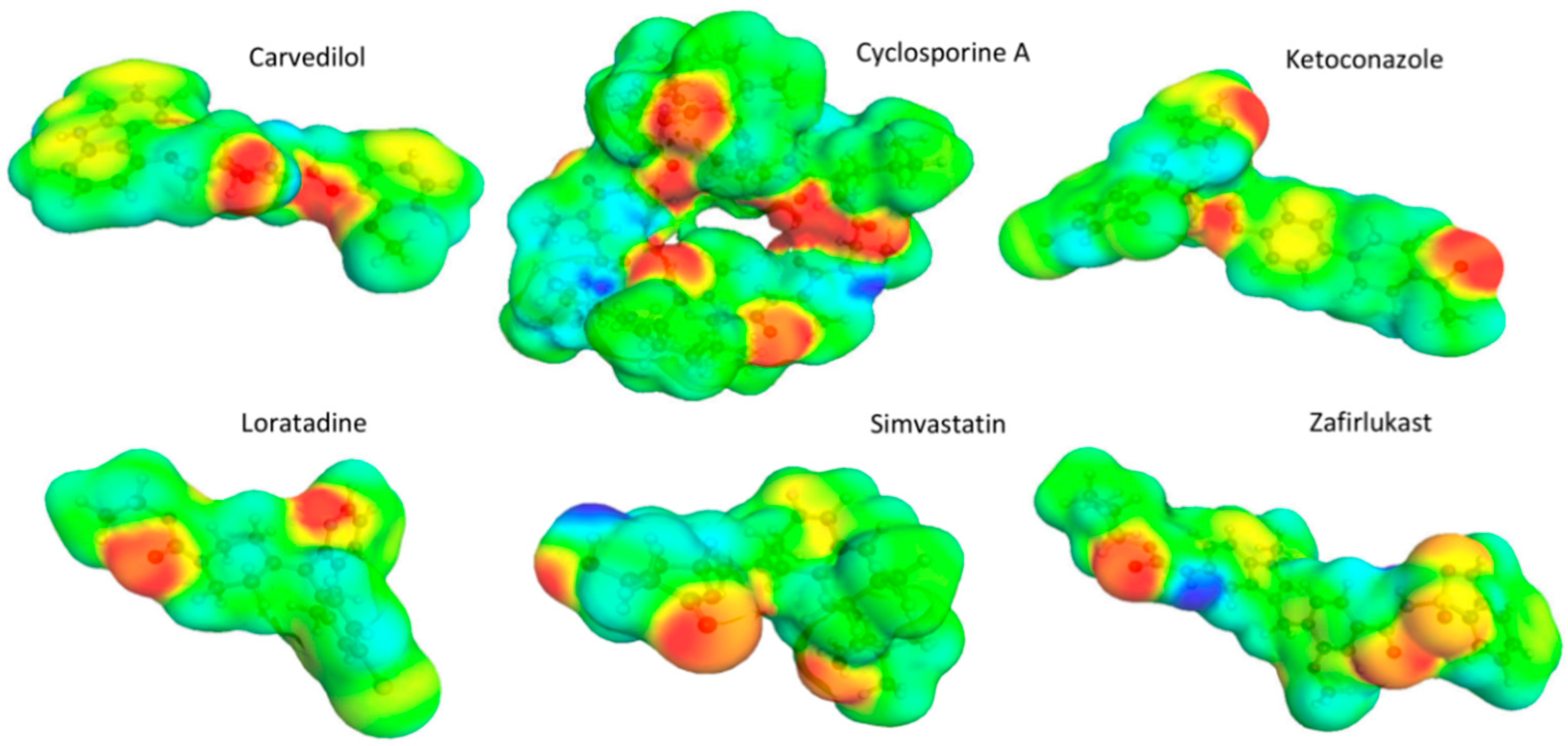

| Compound | Gradient (A:B) (%) | Detection Wavelength (nm) |
|---|---|---|
| Carvedilol | 90:10 | 241 |
| Cyclosporine A | 50:50 | 210 |
| Ketoconazole | 90:10 | 220 |
| Loratadine | 90:10 | 270 |
| Simvastatin | 30:70 | 230 |
| Zafirlukast | 40:60 | 225 |
| Solvent | Vx | E | S | A | B |
|---|---|---|---|---|---|
| n-Hexane | 0.954 | ||||
| n-Heptane | 1.095 | ||||
| n-Octane | 1.236 | ||||
| n-Nonane | 1.377 | ||||
| n-Decane | 1.518 | ||||
| Cyclohexane | 0.845 | 0.310 | 0.010 | ||
| Carbon tetrachloride | 0.739 | 0.460 | 0.380 | ||
| Benzene | 0.716 | 0.610 | 0.520 | ||
| Toluene | 0.857 | 0.600 | 0.520 | ||
| Ethylbenzene | 0.998 | 0.610 | 0.510 | ||
| Acetone | 0.547 | 0.180 | 0.703 | 0.493 | |
| Methyl ethyl ketone | 0.688 | 0.170 | 0.697 | 0.510 | |
| Ethyl acetate | 0.747 | 0.110 | 0.618 | 0.450 | |
| n-Butyl acetate | 1.028 | 0.071 | 0.597 | 0.449 | |
| Tetrahydrofuran | 0.622 | 0.291 | 0.524 | 0.479 | |
| 1,4-Dioxane | 0.681 | 0.330 | 0.744 | 0.635 | |
| Chloroform | 0.617 | 0.430 | 0.490 | 0.150 | |
| Dichloromethane | 0.494 | 0.390 | 0.574 | 0.100 | |
| Methanol | 0.308 | 0.280 | 0.439 | 0.430 | 0.470 |
| Ethanol | 0.449 | 0.250 | 0.419 | 0.370 | 0.480 |
| 1-Propanol | 0.590 | 0.240 | 0.420 | 0.370 | 0.480 |
| 1-Butanol | 0.731 | 0.220 | 0.420 | 0.370 | 0.480 |
| 1-Octanol | 1.295 | 0.200 | 0.421 | 0.370 | 0.480 |
| Isopropanol | 0.590 | 0.210 | 0.366 | 0.330 | 0.560 |
| 2-Butanol | 0.731 | 0.220 | 0.360 | 0.330 | 0.560 |
| Cyclohexanol | 0.904 | 0.461 | 0.557 | 0.320 | 0.570 |
| Phenol | 0.775 | 0.808 | 0.890 | 0.600 | 0.300 |
| Ethylene glycol | 0.508 | 0.400 | 0.900 | 0.580 | 0.780 |
| Acetonitrile | 0.404 | 0.241 | 0.900 | 0.040 | 0.330 |
| Water | 0.167 | 0.000 | 0.450 | 0.820 | 0.350 |
| Parameter | Carvedilol | Cyclosporine A | Ketoconazole | Loratadine | Simvastatin | Zafirlukast |
|---|---|---|---|---|---|---|
| LSER | ||||||
| Vx | 3.10 | 10.02 | 3.72 | 2.87 | 3.43 | 4.23 |
| E | 3.08 | 4.23 | 3.14 | 2.19 | 1.35 | 3.64 |
| S(ACD) | 3.00 | 10.16 | 3.76 | 2.09 | 2.29 | 4.09 |
| S(PSP) | 3.19 | 7.72 | 2.75 | 2.96 | 4.28 | 4.66 |
| A(ACD) | 0.62 | 1.25 | 0.00 | 0.00 | 0.31 | 0.85 |
| A(PSP) | 0.50 | 0.7 ± 0.1 | 0.00 | 0.00 | 0.20 | 0.71 |
| B(ACD) | 2.09 | 7.61 | 2.22 | 1.14 | 1.45 | 2.13 |
| B(PSP) | 1.45 | 4.5 | 0.7 ± 0.3 | 0.6 ± 0.2 | 0.7 ± 0.2 | 2.1 |
| Drug | Tm (K) | ΔHm (kJ/mol) |
|---|---|---|
| Carvedilol | 388.15 ± 0.12 | 47.36 ± 0.37 |
| Cyclosporine A | n.a. * | n.a. * |
| Ketoconazole | 422.29 ± 0.01 | 53.16 ± 0.59 |
| Loratadine | 407.29 ± 0.05 | 27.97 ± 0.18 |
| Simvastatin | 412.21 ± 0.06 | 29.16 ± 0.83 |
| Zafirlukast | 467.95 ± 0.1 | 19.6 ± 1.7 |
| Drug | γd (mJ/m2) | γhb (mJ/m2) | γtot (mJ/m2) * | γa | γb |
|---|---|---|---|---|---|
| Carvedilol | 47.83 | 5.13 | 52.96 | 1.51 | 4.37 |
| Cyclosporine A | 13.19 | 1.09 | 14.28 | 0.21 | 1.38 |
| Ketoconazole | 45.86 | 0.00 | 45.86 | 0.00 | 1.84 |
| Loratadine | 41.6 | 0.00 | 41.60 | 0.00 | 1.78 |
| Simvastatin | 57.36 | 2.64 | 60.00 | 0.71 | 2.47 |
| Zafirlukast | 49.43 | 5.64 | 55.07 | 1.64 | 4.85 |
| Drug | σd (MPa0.5) | σp (MPa0.5) | σtot * (MPa0.5) | σhb (MPa0.5) | σGa (MPa0.5) | σGb (MPa0.5) |
|---|---|---|---|---|---|---|
| Carvedilol | 19.75 | 9.91 | 23.55 | 8.14 | 3.92 | 6.68 |
| Cyclosporine A | 18.04 | 8.44 | 21.12 | 7.03 | 2.54 | 6.44 |
| Ketoconazole | 19.52 | 8.45 | 21.27 | 0.00 | 0.00 | 4.26 |
| Loratadine | 19.11 | 9.87 | 21.51 | 0.00 | 0.00 | 4.45 |
| Simvastatin | 17.82 | 10.65 | 20.96 | 2.89 | 2.30 | 4.31 |
| Zafirlukast | 19.60 | 10.33 | 23.92 | 9.01 | 4.03 | 6.94 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niederquell, A.; Wyttenbach, N.; Kuentz, M.; Panayiotou, C. Partial Solvation Parameters of Drugs as a New Thermodynamic Tool for Pharmaceutics. Pharmaceutics 2019, 11, 17. https://doi.org/10.3390/pharmaceutics11010017
Niederquell A, Wyttenbach N, Kuentz M, Panayiotou C. Partial Solvation Parameters of Drugs as a New Thermodynamic Tool for Pharmaceutics. Pharmaceutics. 2019; 11(1):17. https://doi.org/10.3390/pharmaceutics11010017
Chicago/Turabian StyleNiederquell, Andreas, Nicole Wyttenbach, Martin Kuentz, and Costas Panayiotou. 2019. "Partial Solvation Parameters of Drugs as a New Thermodynamic Tool for Pharmaceutics" Pharmaceutics 11, no. 1: 17. https://doi.org/10.3390/pharmaceutics11010017
APA StyleNiederquell, A., Wyttenbach, N., Kuentz, M., & Panayiotou, C. (2019). Partial Solvation Parameters of Drugs as a New Thermodynamic Tool for Pharmaceutics. Pharmaceutics, 11(1), 17. https://doi.org/10.3390/pharmaceutics11010017






