Enhancing the Estimation of Stem-Size Distributions for Unimodal and Bimodal Stands in a Boreal Mixedwood Forest with Airborne Laser Scanning Data
Abstract
:1. Introduction
2. Materials and Methods
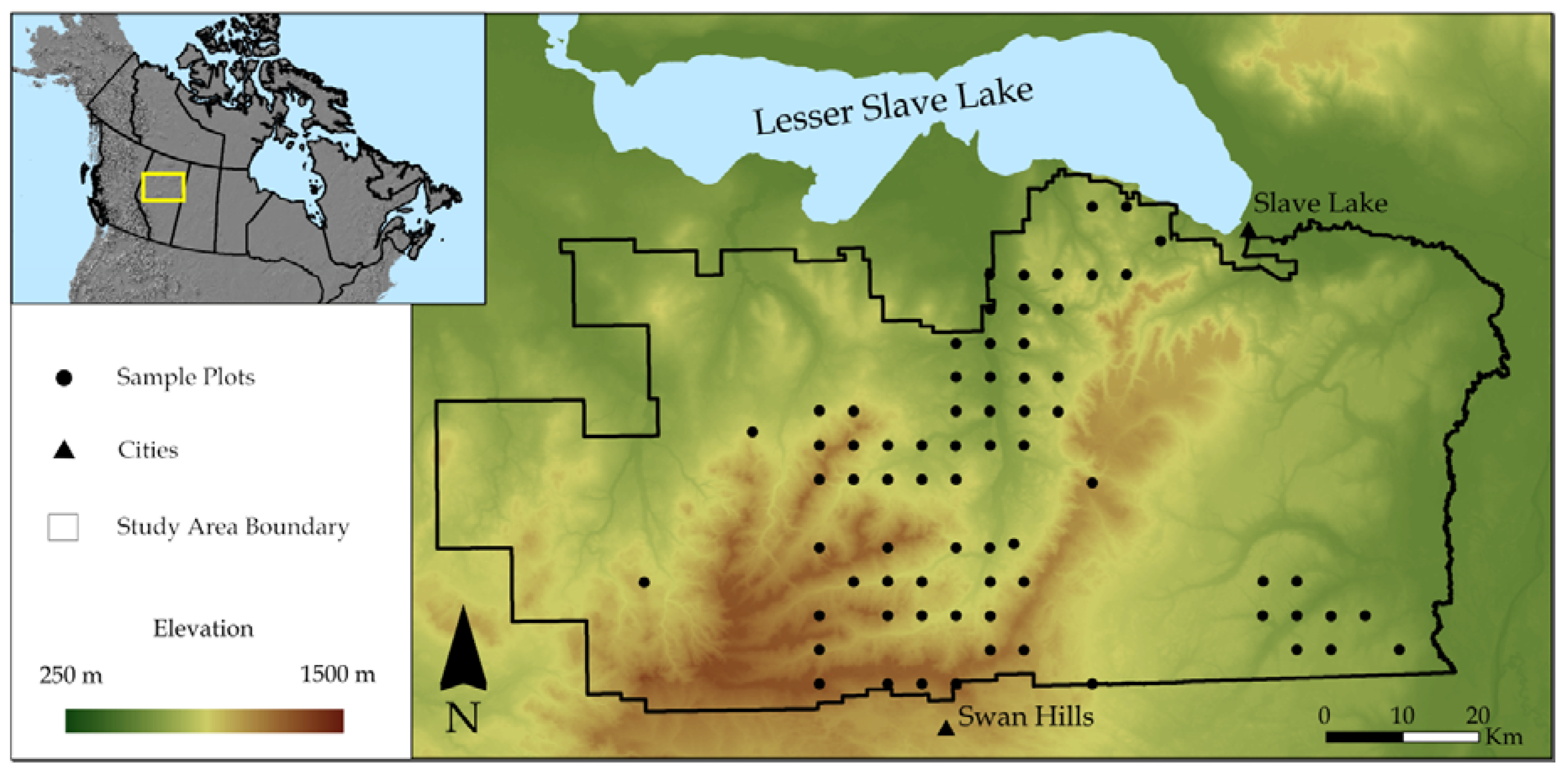
2.1. Study Area
2.2. Ground Plot Data
2.3. ALS Data and Metrics
2.4. Analysis Approach
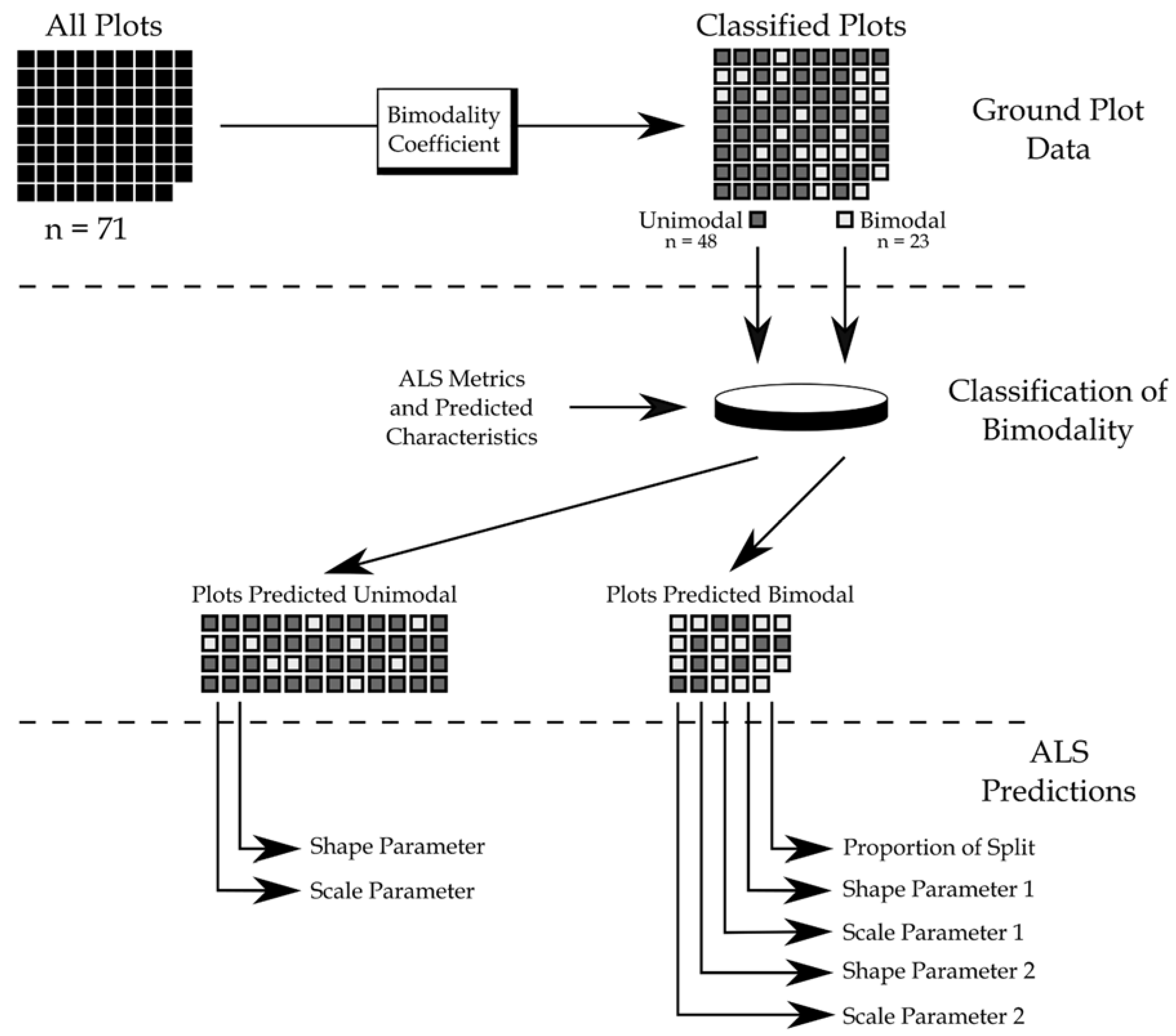
2.5. Differentiation of Modality in Stem Size Distributions
2.6. Accuracy of Modality Differentiation
2.7. Predictive Modeling of SSD Parameters Using ALS Metrics
2.8. Evaluation of SSD Parameters Using the Error Index (EI)
3. Results
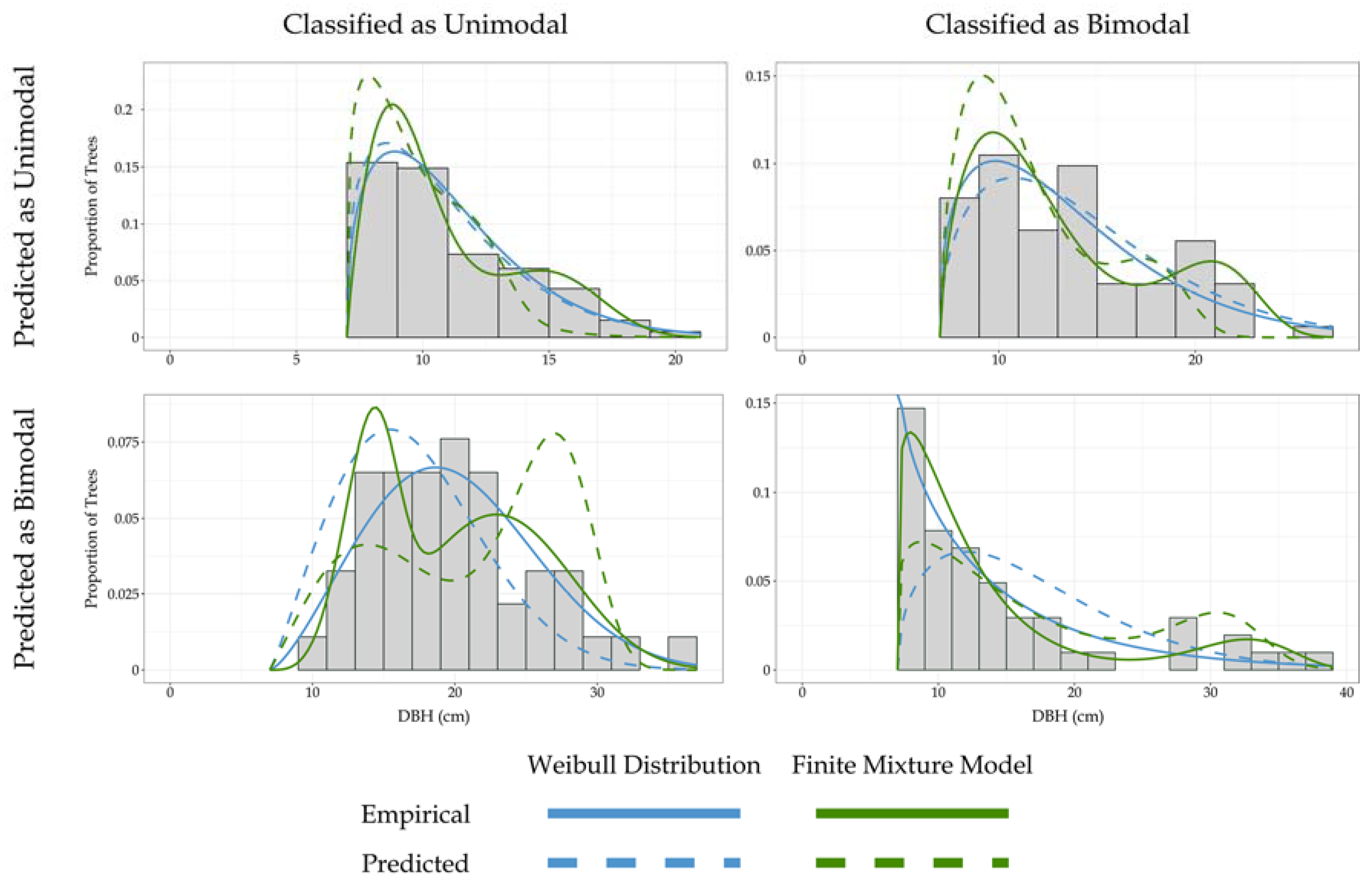
3.1. Differentiation of Modality in Stem Size Distributions
3.2. Predictive Modeling of SSD Parameters Using ALS Metrics
3.3. Accuracy of Predicted Distributions
4. Discussion
4.1. Differentiation of Modality in Stem Size Distributions
4.2. Predictive Modeling of SSD Parameters Using ALS Metrics
4.3. Accuracy of Predicted SSDs
4.4. Model Application
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Hyyppä, J.; Hyyppä, H.; Leckie, D.; Gougeon, F.; Yu, X.; Maltamo, M. Review of methods of small-footprint airborne laser scanning for extracting forest inventory data in boreal forests. Int. J. Rem. Sens. 2008, 29, 1339–1366. [Google Scholar] [CrossRef]
- Taubert, F.; Hartig, F.; Dobner, H.-J.; Huth, A. On the challenge of fitting tree size distributions in ecology. PLoS ONE 2013, 8, e58036. [Google Scholar] [CrossRef] [PubMed]
- Gobakken, T.; Næsset, E. Estimation of diameter and basal area distributions in coniferous forest by means of airborne laser scanner data. Scand. J. For. Res. 2004, 19, 529–542. [Google Scholar] [CrossRef]
- Hetemäki, L.; Mery, G.; Holopainen, M.; Hyyppä, J.; Vaario, L.-M.; Yrjälä, K. Implications of Technological Development to Forestry; IUFRO (International Union of Forestry Research Organizations) Secretariat: Vienna, Austria, 2010; Volume 25, ISBN 3-901347-93-3. [Google Scholar]
- Nduwayezu, J.; Mafoko, G.; Mojeremane, W.; Mhaladi, L. Vanishing multipurpose indigenous trees in Chobe and Kasane forest reserves of Botswana. Resour. Environ. 2015, 5, 167–172. [Google Scholar]
- Landsberg, J.; Mäkelä, A.; Sievänen, R.; Kukkola, M. Analysis of biomass accumulation and stem size distributions over long periods in managed stands of Pinus sylvestris in Finland using the 3-PG model. Tree Physiol. 2005, 25, 781–792. [Google Scholar] [CrossRef] [PubMed]
- Garcıa, O. What is a diameter distribution? In Proceedings of the Symposium on Integrated Forest Management Information Systems; International Union of Forest Research Organizations: Tsukuba, Japan, 1992; pp. 11–29. [Google Scholar]
- Brandt, J.; Flannigan, M.; Maynard, D.; Thompson, I.; Volney, W. An introduction to Canada’s boreal zone: ecosystem processes, health, sustainability, and environmental issues. Environ. Rev. 2013, 21, 207–226. [Google Scholar] [CrossRef]
- Coomes, D.A.; Duncan, R.P.; Allen, R.B.; Truscott, J. Disturbances prevent stem size-density distributions in natural forests from following scaling relationships. Ecol. Lett. 2003, 6, 980–989. [Google Scholar] [CrossRef]
- Freeman, J.B.; Dale, R. Assessing bimodality to detect the presence of a dual cognitive process. Behav. Res. Methods 2013, 45, 83–97. [Google Scholar] [CrossRef] [PubMed]
- Podlaski, R. Suitability of the selected statistical distributions for fitting diameter data in distinguished development stages and phases of near-natural mixed forests in the Świętokrzyski National Park (Poland). For. Ecol. Manag. 2006, 236, 393–402. [Google Scholar] [CrossRef]
- Maltamo, M.; Gobakken, T. Predicting tree diameter distributions. In Forestry Applications of Airborne Laser Scanning; Springer: Dordrecht, The Netherlands, 2014; pp. 177–191. [Google Scholar]
- Zhang, L.; Gove, J.H.; Liu, C.; Leak, W.B. A finite mixture of two Weibull distributions for modeling the diameter distributions of rotated-sigmoid, uneven-aged stands. Can. J. For. Res. 2001, 31, 1654–1659. [Google Scholar] [CrossRef]
- Liu, C.; Zhang, L.; Davis, C.J.; Solomon, D.S.; Gove, J.H. A finite mixture model for characterizing the diameter distributions of mixed-species forest stands. For. Sci. 2002, 48, 653–661. [Google Scholar]
- Thomas, V.; Oliver, R.; Lim, K.; Woods, M. LiDAR and Weibull modeling of diameter and basal area. For. Chron. 2008, 84, 866–875. [Google Scholar] [CrossRef]
- Kane, V.R.; Bakker, J.D.; McGaughey, R.J.; Lutz, J.A.; Gersonde, R.F.; Franklin, J.F. Examining conifer canopy structural complexity across forest ages and elevations with LiDAR data. Can. J. For. Res. 2010, 40, 774–787. [Google Scholar] [CrossRef]
- Franklin, J.F.; Spies, T.A.; Van Pelt, R.; Carey, A.B.; Thornburgh, D.A.; Berg, D.R.; Lindenmayer, D.B.; Harmon, M.E.; Keeton, W.S.; Shaw, D.C. Disturbances and structural development of natural forest ecosystems with silvicultural implications, using Douglas-fir forests as an example. For. Ecol. Manag. 2002, 155, 399–423. [Google Scholar] [CrossRef]
- Bailey, R.L.; Dell, T. Quantifying diameter distributions with the Weibull function. For. Sci. 1973, 19, 97–104. [Google Scholar]
- Myung, I.J. Tutorial on maximum likelihood estimation. J. Math. Psychol. 2003, 47, 90–100. [Google Scholar] [CrossRef]
- Packalén, P.; Maltamo, M. Estimation of species-specific diameter distributions using airborne laser scanning and aerial photographs. Can. J. For. Res. 2008, 38, 1750–1760. [Google Scholar] [CrossRef]
- Penner, M.; Woods, M.; Pitt, D.G. A comparison of airborne laser scanning and image point cloud derived tree size class distribution models in boreal Ontario. Forests 2015, 6, 4034–4054. [Google Scholar] [CrossRef]
- Eid, T.; Gobakken, T.; Næsset, E. Comparing stand inventories for large areas based on photo-interpretation and laser scanning by means of cost-plus-loss analyses. Scand. J. For. Res. 2004, 19, 512–523. [Google Scholar] [CrossRef]
- White, J.C.; Coops, N.C.; Wulder, M.A.; Vastaranta, M.; Hilker, T.; Tompalski, P. Remote sensing technologies for enhancing forest inventories: A review. Can. J. Rem. Sens. 2016, 42, 619–641. [Google Scholar] [CrossRef]
- Næsset, E. Predicting forest stand characteristics with airborne scanning laser using a practical two-stage procedure and field data. Rem. Sens. Environ. 2002, 80, 88–99. [Google Scholar] [CrossRef]
- Næsset, E. Airborne laser scanning as a method in operational forest inventory: Status of accuracy assessments accomplished in Scandinavia. Scand. J. For. Res. 2007, 22, 433–442. [Google Scholar] [CrossRef]
- Van Leeuwen, M.; Nieuwenhuis, M. Retrieval of forest structural parameters using LiDAR remote sensing. Eur. J. For Res. 2010, 129, 749–770. [Google Scholar] [CrossRef]
- Maltamo, M.; Packalén, P.; Yu, X.; Eerikäinen, K.; Hyyppä, J.; Pitkänen, J. Identifying and quantifying structural characteristics of heterogeneous boreal forests using laser scanner data. For. Ecol. Manag. 2005, 216, 41–50. [Google Scholar] [CrossRef]
- Kao, D.L.; Kramer, M.G.; Love, A.L.; Dungan, J.L.; Pang, A.T. Visualizing distributions from multi-return lidar data to understand forest structure. Cartogr. J. 2005, 42, 35–47. [Google Scholar] [CrossRef]
- Maltamo, M.; Suvanto, A.; Packalén, P. Comparison of basal area and stem frequency diameter distribution modelling using airborne laser scanner data and calibration estimation. For. Ecol. Manag. 2007, 247, 26–34. [Google Scholar] [CrossRef]
- Natural Regions Committee Natural Regions and Subregions of Alberta. Compiled by DJ Downing and WW Pettapiece; Government of Alberta: Edmonton, Canada, 2006.
- Forest Management Branch. Permanent Sample Plot (PSP) Field Procedures Manual; Alberta Sustainable Resource Development, Public Lands and Forest Division: Edmonton, Canada, 2005.
- Zhang, L.; Packard, K.C.; Liu, C. A comparison of estimation methods for fitting Weibull and Johnson’s SB distributions to mixed spruce fir stands in northeastern North America. Can. J. For. Res. 2003, 33, 1340–1347. [Google Scholar] [CrossRef]
- SAS Institute. SAS/STAT User’s Guide; SAS Institute Inc.: Cary, NC, USA, 1989. [Google Scholar]
- Ellison, A.M. Effect of seed dimorphism on the density-dependent dynamics of experimental populations of Atriplex triangularis (Chenopodiaceae). Am. J. Bot. 1987, 1280–1288. [Google Scholar] [CrossRef]
- Axelsson, P. DEM generation from laser scanner data using adaptive TIN models. Int. Arch. Photogramm. Rem. Sens. 2000, XXXIII, 110–117. [Google Scholar]
- McGaughey, R.J. FUSION/LDV: Software for LIDAR Data Analysis and Visualization; US Department of Agriculture, Forest Service, Pacific Northwest Research Station: Seattle, WA, USA, 2009; p. 123.
- R Core Team. R: A language and environment for statistical computing; R Foundation for Statistical Computing: Vienna, Austria, 2016. [Google Scholar]
- Roussel, J.R.; Auty, D. lidR: Airborne LiDAR Data Manipulation and Visualization for Forestry Applications. R package. Available online: https://github.com/Jean-Romain/lidR (accessed on 31 January 2018).
- Kane, V.R.; McGaughey, R.J.; Bakker, J.D.; Gersonde, R.F.; Lutz, J.A.; Franklin, J.F. Comparisons between field-and LiDAR-based measures of stand structural complexity. Can. J. For. Res. 2010, 40, 761–773. [Google Scholar] [CrossRef]
- Tompalski, P. Wykorzystanie wskaźników przestrzennych 3D w analizach cech roślinności miejskiej na podstawie danych z lotniczego skanowania laserowego. Archiwum Fotogrametrii, Kartografii i Teledetekcji 2012, 23. [Google Scholar]
- Van Ewijk, K.Y.; Treitz, P.M.; Scott, N.A. Characterizing forest succession in Central Ontario using LiDAR-derived indices. Photogramm. Engin. Rem. Sens. 2011, 77, 261–269. [Google Scholar] [CrossRef]
- Tompalski, P.; Coops, N.C.; White, J.C.; Wulder, M.A. Enriching ALS-derived area-based estimates of volume through tree-level downscaling. Forests 2015, 6, 2608–2630. [Google Scholar] [CrossRef]
- Bouvier, M.; Durrieu, S.; Fournier, R.A.; Renaud, J.-P. Generalizing predictive models of forest inventory attributes using an area-based approach with airborne LiDAR data. Rem. Sens. Environ. 2015, 156, 322–334. [Google Scholar] [CrossRef]
- Popescu, S.C.; Zhao, K. A voxel-based lidar method for estimating crown base height for deciduous and pine trees. Rem. Sens. Environ. 2008, 112, 767–781. [Google Scholar] [CrossRef]
- Van Aardt, J.A.; Wynne, R.H.; Oderwald, R.G. Forest volume and biomass estimation using small-footprint lidar-distributional parameters on a per-segment basis. For. Sci. 2006, 52, 636–649. [Google Scholar]
- Aronoff, S. Classification accuracy: A user approach. Photogramm. Eng. Rem. Sens. 1982, 48, 1299–1307. [Google Scholar]
- McGarrigle, E.; Kershaw, J.A., Jr.; Lavigne, M.B.; Weiskittel, A.R.; Ducey, M. Predicting the number of trees in small diameter classes using predictions from a two-parameter Weibull distribution. Forestry 2011, 84, 431–439. [Google Scholar] [CrossRef]
- Bollandsås, O.M.; Maltamo, M.; Gobakken, T.; Næsset, E. Comparing parametric and non-parametric modelling of diameter distributions on independent data using airborne laser scanning in a boreal conifer forest. Forestry 2013, 86, 493–501. [Google Scholar] [CrossRef]
- Maltamo, M.; Næsset, E.; Bollandsås, O.M.; Gobakken, T.; Packalén, P. Non-parametric prediction of diameter distributions using airborne laser scanner data. Scand. J. For. Res. 2009, 24, 541–553. [Google Scholar] [CrossRef]
- Reynolds, M.R.; Burk, T.E.; Huang, W.-C. Goodness-of-fit tests and model selection procedures for diameter distribution models. For. Sci. 1988, 34, 373–399. [Google Scholar]
- Palahí, M.; Pukkala, T.; Trasobares, A. Modelling the diameter distribution of Pinus sylvestris, Pinus nigra and Pinus halepensis forest stands in Catalonia using the truncated Weibull function. Forestry 2006, 79, 553–562. [Google Scholar] [CrossRef]
- Isenburg, M. Lastools-efficient LiDAR processing software. Available online: lastools.org (accessed on 10 October 2017).
- Borders, B.E.; Wang, M.; Zhao, D. Problems of scaling plantation plot diameter distributions to stand level. For. Sci. 2008, 54, 349–355. [Google Scholar]
- Siipilehto, J.; Lindeman, H.; Vastaranta, M.; Yu, X.; Uusitalo, J. Reliability of the predicted stand structure for clear-cut stands using optional methods: Airborne laser scanning-based methods, smartphone-based forest inventory application Trestima and pre-harvest measurement tool EMO. Silva Fennica 2016, 50. [Google Scholar] [CrossRef]
- Magnussen, S.; Renaud, J.-P. Multidimensional scaling of first-return airborne laser echoes for prediction and model-assisted estimation of a distribution of tree stem diameters. Ann. For. Sci. 2016, 73, 1089–1098. [Google Scholar] [CrossRef]
- Lundholm, A. Evaluating Inventory Methods for Estimating Stem Diameter Distributions in Micro Stands Derived from Airborne Laser Scanning. Master’s Thesis, Sveriges lantbruksuniversitet, Umeå, Sweden, 2014. [Google Scholar]

| Characteristic | Minimum | 1st Quartile | Median | 3rd Quartile | Maximum | Mean | Std. Deviation |
|---|---|---|---|---|---|---|---|
| Lorey’s Mean Height (m) | 6.13 | 12.71 | 15.99 | 19.83 | 28.39 | 16.27 | 4.97 |
| Quadratic Mean Diameter (cm) | 3.88 | 11.00 | 13.48 | 17.08 | 25.42 | 14.14 | 4.72 |
| Age | 27.6 | 53.27 | 70.19 | 126.75 | 197.49 | 85.34 | 43.69 |
| Total Volume (m3) | 16.99 | 154.1 | 248.2 | 348.8 | 809.1 | 280.83 | 181.96 |
| Density (N/ha) | 1075 | 1875 | 2650 | 3138 | 8325 | 2644 | 1194.65 |
| Characteristic | Collection Year | |
|---|---|---|
| 2006 | 2007–2008 | |
| Sensor | Optech ALTM 3100 | |
| Flying Height | 1250 m | 1400 m |
| Flight Speed | 160 kts | |
| Pulse Repetition Frequency | 50 kHz | 70 kHz |
| Scan Frequency | 30 Hz | 33 Hz |
| Scan Angle | 50° | |
| Beam Divergence | 0.3 mrad | |
| Average Point Density | 1.5 pts/m2 | |
| Metric | Description | Source | Category |
|---|---|---|---|
| P05 | Height of the 5th percentile of returns | McGaughey 2014 [36] | Height |
| P25 | Height of the 25th percentile of returns | McGaughey 2014 [36] | Height |
| P50 | Height of the 50th percentile of returns | McGaughey 2014 [36] | Height |
| P75 | Height of the 75th percentile of returns | McGaughey 2014 [36] | Height |
| P95 | Height of the 95th percentile of returns | McGaughey 2014 [36] | Height |
| Std. Dev. | Standard deviation of return heights | McGaughey 2014 [36] | Variability of Heights |
| Variance | Variance of return heights | McGaughey 2014 [36] | Variability of Heights |
| IQ | Interquartile range of return heights | McGaughey 2014 [36] | Variability of Heights |
| Skewness | Skewness of return heights | McGaughey 2014 [36] | Variability of Heights |
| Kurtosis | Kurtosis of return heights | McGaughey 2014 [36] | Variability of Heights |
| AAD | Average absolute deviation of return heights | McGaughey 2014 [36] | Variability of Heights |
| Median | Median of return heights | McGaughey 2014 [36] | Variability of Heights |
| % First Returns Above 2 m | Percent of first returns above 2 meters | McGaughey 2014 [36] | Cover |
| % All Returns Above 2 m | Percent of all returns above 2 meters | McGaughey 2014 [36] | Cover |
| 0.5 m–2 m Return Proportion | Proportion of returns between 0.5 and 2 m | McGaughey 2014 [36] | Cover |
| 2 m–5 m Return Proportion | Proportion of returns between 2 and 5 m | McGaughey 2014 [36] | Cover |
| 5 m–10 m Return Proportion | Proportion of returns between 5 and 10 m | McGaughey 2014 [36] | Cover |
| 10 m–20 m Return Proportion | Proportion of returns between 10 and 20 m | McGaughey 2014 [36] | Cover |
| Rumple | Ratio of canopy surface area to plot area | Kane et al. 2010 [39] | Structure |
| Filling Ratio | Proportion of returns in voxels under the canopy | Tompalski 2012 [40] | Structure |
| VCI | Vertical complexity index—distribution of abundance of returns in specified height bins | Van Ewijk et al. 2011 [41] | Structure |
| Vertical Rumple | Measure of variance of vertical structure as a function of filled voxels in point cloud | Tompalski et al. 2015 [42] | Structure |
| LAD CV | Coefficient of variation of leaf area density—vertical dispersion of foliage density through the canopy | Bouvier et al. 2015 [43] | Structure |
| Differentiation | Source | Quantified as |
|---|---|---|
| Uneven-aged stands | Zhang et al. 2001 [13] | Std. dev. of ages (SDA) |
| Mixed-species stands | Liu et al. 2002 [14] | % Dominant species |
| Density/Height Ratio | Thomas et al. 2008 [15] | N/top height (D/H) |
| Multilayered | Podlaski 2010 [11] | Std. dev. of heights (SDH) |
| Varied diameters | Maltamo and Gobakken 2014 [12] | Std. dev. of DBHs (SDDBH) |
| Differentiation | Overall Accuracy | ALS Prediction Accuracy | ||
|---|---|---|---|---|
| Adj. r2 | % RMSE | |||
| Plot Data | SDA | 59.2 | - | - |
| % Dominant Species | 59.2 | - | - | |
| D/H | 64.8 | - | - | |
| SDH | 67.6 | - | - | |
| SDDBH | 70.4 | - | - | |
| ALS Predictions | SDA | 66.2 | 0.059 | 88.4 |
| % Dominant Species | 63.4 | 0.155 | 27.3 | |
| D/H | 74.7 * | 0.600 | 43.8 | |
| SDH | 67.6 | 0.694 | 25.7 | |
| SDDBH | 74.7 * | 0.640 | 31.2 | |
| ALS Metrics | Variance | 77.5 * | - | - |
| Kurtosis | 46.5 | - | - | |
| Canopy Relief Ratio | 57.8 | - | - | |
| % All Returns >2 m. | 63.4 | - | - | |
| Filling Ratio | 66.2 | - | - | |
| Rumple | 74.7 * | - | - | |
| Parameter | Prediction Accuracy | ||
|---|---|---|---|
| Adj. r2 | % RMSE | ||
| Unimodal | Shape | 0.3925 | 23.26 |
| Scale | 0.6271 | 30.39 | |
| Bimodal | Shape1 | 0.5497 | 30.25 |
| Scale1 | 0.5898 | 32.86 | |
| Shape2 | 0.2019 | 33.93 | |
| Scale2 | 0.5203 | 29.81 | |
| % over breakpoint | 0.5389 | 42.91 | |
| Ground Fstimates (EIG) | ALS Predictions (EIALS) | |
|---|---|---|
| Mean EI (unimodal plots) | 25.29 | 42.86 |
| Mean EI (bimodal plots) | 34.30 | 62.21 |
| Mean EI (all plots) | 28.20 | 49.13 |
| Mean EI (unimodal Weibull on all plots) | 31.24 | 51.31 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mulverhill, C.; Coops, N.C.; White, J.C.; Tompalski, P.; Marshall, P.L.; Bailey, T. Enhancing the Estimation of Stem-Size Distributions for Unimodal and Bimodal Stands in a Boreal Mixedwood Forest with Airborne Laser Scanning Data. Forests 2018, 9, 95. https://doi.org/10.3390/f9020095
Mulverhill C, Coops NC, White JC, Tompalski P, Marshall PL, Bailey T. Enhancing the Estimation of Stem-Size Distributions for Unimodal and Bimodal Stands in a Boreal Mixedwood Forest with Airborne Laser Scanning Data. Forests. 2018; 9(2):95. https://doi.org/10.3390/f9020095
Chicago/Turabian StyleMulverhill, Christopher, Nicholas C. Coops, Joanne C. White, Piotr Tompalski, Peter L. Marshall, and Todd Bailey. 2018. "Enhancing the Estimation of Stem-Size Distributions for Unimodal and Bimodal Stands in a Boreal Mixedwood Forest with Airborne Laser Scanning Data" Forests 9, no. 2: 95. https://doi.org/10.3390/f9020095
APA StyleMulverhill, C., Coops, N. C., White, J. C., Tompalski, P., Marshall, P. L., & Bailey, T. (2018). Enhancing the Estimation of Stem-Size Distributions for Unimodal and Bimodal Stands in a Boreal Mixedwood Forest with Airborne Laser Scanning Data. Forests, 9(2), 95. https://doi.org/10.3390/f9020095







