An Open-Source Tree Bucking Optimizer Based on Dynamic Programming
Abstract
1. Introduction
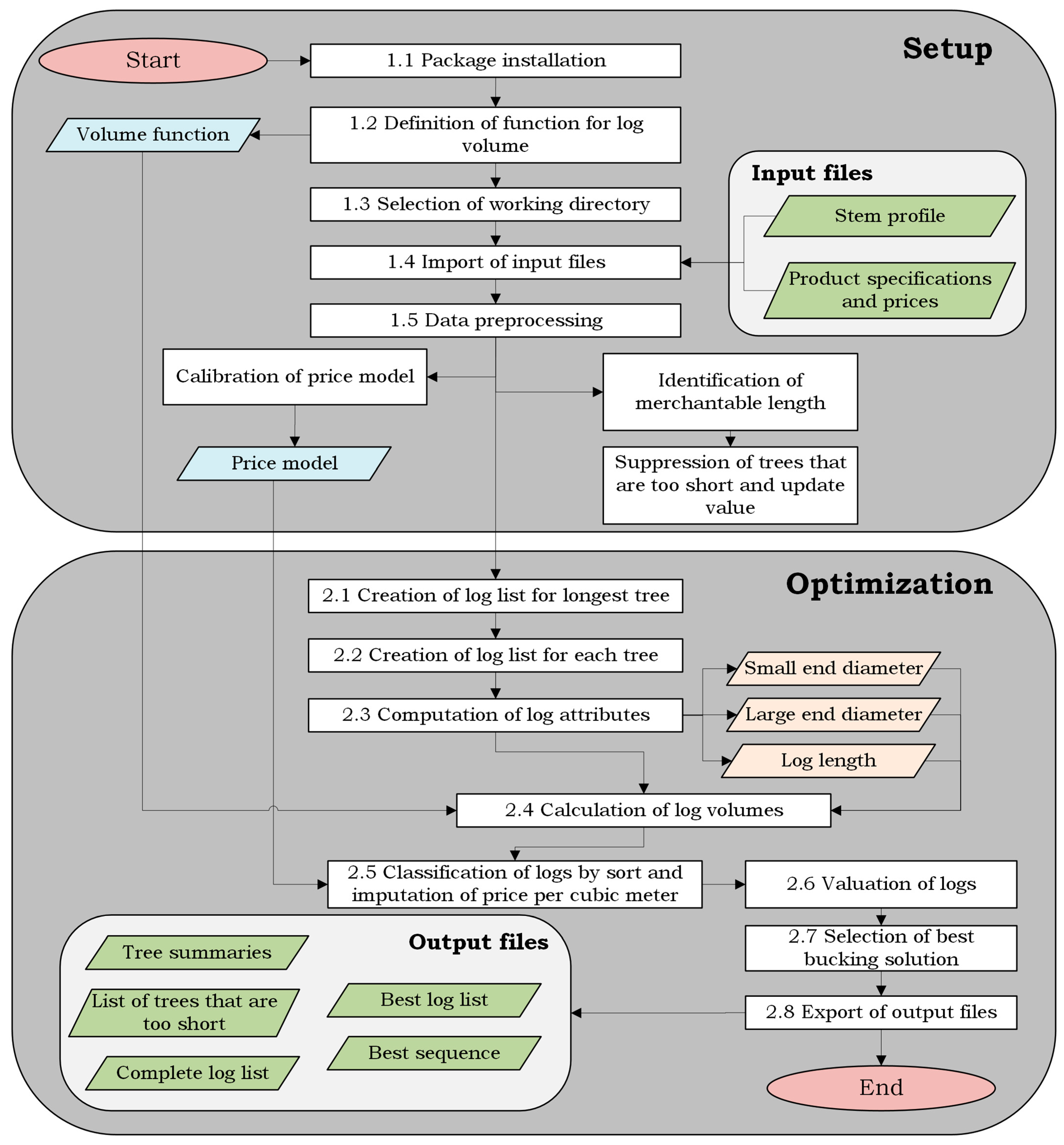
2. Development and Implementation
2.1. Dynamic Programming
2.2. Implementation in R and Objective Function Definition
2.3. Optimization Algorithm
2.3.1. Input Files
2.3.2. Classification Model
2.3.3. Creation of Log Combinations
2.3.4. Classification of Logs and Attribution of Value
2.3.5. Selection of the Best Bucking Solution
2.3.6. Output
3. Validation
4. Discussion
- The volume calculation is based on the volume formula proposed by Dykstra [30], but it can be adapted to others, depending on the jurisdiction and regulation. The volume determination chosen was shown to impact the volume results [35]. This may affect the bucking solution found by the algorithm, depending on the log scaling formula or table used.
- Sometimes, mill specifications allow for random lengths rather than fixed lengths for logs: in this case, with BuckR, it is mandatory to code a series of products with different lengths of a fixed interval and a common value per cubic meter.
- The code can be adapted to process more than one species (in such cases, we recommend the calibration of a log classifier for each species).
- BuckR can be modified to consider stem defects and sweep criteria that are commonly used for the bucking of high-quality hardwoods or softwoods and is shown to impact bucking [8].
- The application of bucking optimization in harvesters implies decision-making with partial information about the stem profile: for each tree, an early bucking solution is calculated based on the profile measured at the bottom of the stem, which is later revised and corrected with supplemental data on the tree shape. This situation is not covered by the current version of our algorithm.
- Finally, a more demanding upgrade would imply the bucking of trees with large, marketable branches, a common issue in hardwoods [24].
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Marshall, H.D. Log Merchandizing Model Used in Mechanical Harvesting. In Handbook of Operations Research in Natural Resources; Springer: Boston, MA, USA, 2007; pp. 379–389. [Google Scholar]
- Pnevmaticos, S.M.; Mann, S.H. Dynamic Programming in Tree Bucking. For. Prod. J. 1972, 22, 26–30. [Google Scholar]
- Kivinen, V.-P. A Genetic Algorithm Approach to Tree Bucking Optimization. For. Sci. 2004, 50, 696–710. [Google Scholar] [CrossRef]
- Faaland, B.; Briggs, D. Log Bucking and Lumber Manufacturing Using Dynamic Programming. Manag. Sci. 1984, 30, 245–458. [Google Scholar] [CrossRef]
- Lembersky, M.R.; Chi, U.H. Weyerhaeuser Decision Simulator Improves Timber Profits. Interfaces 1986, 16, 6–15. [Google Scholar] [CrossRef]
- Murphy, G.E. An Economic Analysis of Final Log Manufacturing Locations in the Steep Terrain Radiata Pine Plantations of New Zealand. Ph.D. Dissertation, Oregon State University, Corvallis, OR, USA, 1987. [Google Scholar]
- Wang, J.; LeDoux, C.B.; McNeel, J. Optimal Tree-Stem Bucking of Northeastern Species of China. For. Prod. J. 2004, 54, 45–52. [Google Scholar]
- Pickens, J.B.; Lee, A.; Lyon, G.W. Optimal Bucking of Northern Hardwoods. North. J. Appl. For. 1992, 9, 149–152. [Google Scholar] [CrossRef]
- Sessions, J.; Garland, J. Olsen Testing Computer-Aided Bucking at the Stump. J. For. 1989, 87, 43–46. [Google Scholar]
- Laroze, A.J. Development and Comparison of Stand-Level Bucking Optimization Methods. Ph.D. Dissertation, Oregon State University, Corvallis, OR, USA, 1994. [Google Scholar]
- Laroze, A.J.; Greber, B.J. Using Tabu Search To Generate Stand-Level, Rule-Based Bucking Patterns. For. Sci. 1997, 43, 157–169. [Google Scholar] [CrossRef]
- Rust, J. Dynamic Programming. In The New Palgrave Dictionary of Economics; Macmillan Publishers Ltd., Ed.; Palgrave Macmillan UK: London, UK, 2018; pp. 3133–3158. ISBN 978-1-349-95188-8. [Google Scholar]
- Liu, J. Optimal Bucking Hardwood Species in Central Appalachia. Master’s Thesis, West Virginia University, Morgantown, WV, USA, 2006. [Google Scholar]
- Olivera, A.; Visser, R. Using the Harvester On-Board Computer Capability to Move towards Precision Forestry. NZ J. For. 2016, 60, 3–7. [Google Scholar]
- Uusitalo, J. Introduction to Forest Operations and Technology; JVP Forest Systems Oy: Tampere, Finland, 2010; ISBN 978-952-92-5269-5. [Google Scholar]
- Palander, T.; Mutanen, J.; Kärhä, K.; Sorsa, J.-A.; Räsänen, T. Multiple-Criteria Stem Bucking (Picea abies L. Karst.) for Maximizing Monetary Value of Timber Trade. Int. J. Adv. Eng. Res. Sci. 2018, 5, 183–191. [Google Scholar] [CrossRef]
- Lundbäck, M.; Häggström, C.; Nordfjell, T. Worldwide Trends in Methods for Harvesting and Extracting Industrial Roundwood. Int. J. For. Eng. 2021, 32, 202–215. [Google Scholar] [CrossRef]
- Labelle, E.R.; Breinig, L.; Sycheva, E. Exploring the Use of Harvesters in Large-Diameter Hardwood-Dominated Stands. Forests 2018, 9, 424. [Google Scholar] [CrossRef]
- Silversides, C.R. Mechanized Forestry, World War II to the Present. For. Chron. 1984, 60, 231–235. [Google Scholar] [CrossRef]
- Gellerstedt, S.; Dahlin, B. Cut-To-Length: The Next Decade. J. For. Eng. 1999, 10, 17–24. [Google Scholar]
- Labelle, E.R.; Huß, L. Creation of Value through a Harvester On-Board Bucking Optimization System Operated in a Spruce Stand. Silva Fenn. 2018, 52. [Google Scholar] [CrossRef]
- Kärhä, K.; Änäkkälä, J.; Hakonen, O.; Palander, T.; Sorsa, J.-A.; Räsänen, T.; Moilanen, T. Analyzing the Antecedents and Consequences of Manual Log Bucking in Mechanized Wood Harvesting. Mech. Mater. Sci. Eng. 2017, 12, 1–15. [Google Scholar]
- Boston, K.; Murphy, G.E. Value Recovery from Two Mechanized Bucking Operations in the Southeastern United States. South. J. Appl. For. 2003, 27, 259–263. [Google Scholar] [CrossRef]
- Millet, J.; Oldemanet, A.A.; Back, F.; Guénette, S. The Architecture of Trees in Temperate Regions: Its History, Concepts, and Uses; Jeanne Millet: Montréal, QC, Canada, 2025; ISBN 978-2-9820900-4-0. [Google Scholar]
- Pickens, J.B.; Lyon, G.W.; Lee, A.; Frayer, W.E. HW-Buck: A Computerized Hardwood Bucking Decision Simulator. In Proceedings of the General Technical Report SE-74; U.S. Department of Agriculture, Forest Service: Charleston, SC, USA, 1991; pp. 213–216. [Google Scholar]
- Wang, J.; Liu, J.; LeDoux, C.B. A Three-Dimensional Bucking System for Optimal Bucking of Central Appalachian Hardwoods. Int. J. For. Eng. 2009, 20, 10. [Google Scholar] [CrossRef]
- Bellman, R. On the Theory of Dynamic Programming. Proc. Natl. Acad. Sci. USA 1952, 38, 716–719. [Google Scholar] [CrossRef]
- Eddy, S.R. What Is Dynamic Programming? Nat. Biotechnol. 2004, 22, 909–910. [Google Scholar] [CrossRef]
- Prendes, C.; Acuna, M.; Canga, E.; Ordoñez, C.; Cabo, C. Optimal Bucking of Stems from Terrestrial Laser Scanning Data to Maximize Forest Value. Scand. J. For. Res. 2023, 38, 174–188. [Google Scholar] [CrossRef]
- Dykstra, D.P. Chapter Ten - Dynamic Programming. In Mathematical Programming. In Mathematical Programming for Natural Resources Management; McGraw-Hill Series on Computer Communications: New York, NY, USA, 1984; pp. 287–310. [Google Scholar]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2021. [Google Scholar]
- Skogforsk StandForD 2010-Modern Communication with Forest Machines. Available online: https://www.skogforsk.se/cd_20210625001813/contentassets/1a68cdce4af1462ead048b7a5ef1cc06/stanford2010-info-english-2021.zip (accessed on 5 May 2025).
- Therneau, T.; Atkinson, B. rpart: Recursive Partitioning and Regression Trees. R Package Version 4.1.21. Available online: https://CRAN.R-project.org/package=rpart (accessed on 5 May 2025).
- Kershaw, J.A.; Ducey, M.J.; Beers, T.W.; Husch, B. Forest Mensuration, 5th ed.; Wiley/Blackwell: Chichester, UK; Hoboken, NJ, USA, 2017; ISBN 978-1-118-90203-5. [Google Scholar]
- Hohmann, F.; Ligocki, A.; Frerichs, L. Harvester Measuring System for Trunk Volume Determination: Comparison with the Real Trunk Volume and Applicability in the Forest Industry. Bull. Transilv. Univ. Braşov Spec. Issue 2017, 10, 27–34. [Google Scholar]

| Program Name | HW-Buck | HW-Buck 2 | NECO | CAHO |
|---|---|---|---|---|
| Related publication | Pickens et al., 1991 [25] | Pickens et al., 1991 [25] | Wang et al., 2004 [7] | Wang et al., 2009 [26] |
| Geographic region or forest | Northern Hardwoods, Northeastern USA | Northern Hardwoods, Northeastern USA | Northeastern China | Central Appalachian Forests, Eastern USA |
| Availability | Online https://hardwoodbucking.mtu.edu/downloads.htm (accessed on 5 May 2025) | Through personal communication with first author | Not online | Through personal communication with first author |
| Inclusion of quality factors | Yes | Yes | Yes | Yes |
| Mathematical approach | Dynamic programming | Dynamic programming | Network analysis | Dynamic programming |
| Unit system | Imperial | Imperial | Metric | Metric |
| Log scaling rules | Scribner Decimal C and International ¼-Inch Log Rule | Scribner Decimal C and International ¼-Inch Log Rule | According to China Wood Standardization Committee | Doyle International ¼-Inch Log Rule |
| Goal of the program | Train buckers by “playing” | Train buckers by “playing” | Evaluation of actual bucking | Evaluate actual bucking and train operators |
| Customizable | No | Partly (markets (with restrictions) but not log scaling rules) | Unknown | Partly |
| Input File | Name of the Variable | Description | Data Type in R |
|---|---|---|---|
| Tree data | Tree.No | Sequential number of trees (1 to x) | Factor |
| ID.Tree | Alphanumeric variable for identification of study trees | Factor | |
| SP | Tree species | Factor | |
| LG | Vertical position from the first cross-cut in 1 cm increments (cm) | Numeric | |
| DG | Diameter for each vertical position (mm) | Numeric | |
| DBH | DBH of study tree (cm) | Numeric | |
| HT | Total height of study tree (m) | Numeric | |
| Product specifications | ProductID | Sequential number of products (1 to x) | Factor |
| SP | Tree species | Factor | |
| L | Requested log length (including overlength, cm) | Numeric | |
| SEDmin | Minimum diameter of product (mm) | Numeric | |
| SEDmax | Maximum diameter of product (mm) | Numeric | |
| VAM | Value of product (unit per cubic meter) | Numeric | |
| cat | Category of product (“saw”, “pulp” or “waste”) | Factor |
| Product ID | Log Length (cm) | Minimum Small-End Diameter (mm) | Maximum Small-End Diameter (mm) | Value per Cubic Meter |
|---|---|---|---|---|
| 1 | 300 | 200 | 499 | 90 |
| 2 | 300 | 500 | 2000 | 0 |
| 3 | 450 | 200 | 499 | 100 |
| 4 | 45 | 500 | 2000 | 125 |
| 5 | 600 | 200 | 499 | 115 |
| 6 | 600 | 500 | 799 | 150 |
| 7 | 600 | 800 | 2000 | 200 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bennemann, C.; Lussier, J.-M.; Labelle, E.R. An Open-Source Tree Bucking Optimizer Based on Dynamic Programming. Forests 2025, 16, 780. https://doi.org/10.3390/f16050780
Bennemann C, Lussier J-M, Labelle ER. An Open-Source Tree Bucking Optimizer Based on Dynamic Programming. Forests. 2025; 16(5):780. https://doi.org/10.3390/f16050780
Chicago/Turabian StyleBennemann, Caroline, Jean-Martin Lussier, and Eric R. Labelle. 2025. "An Open-Source Tree Bucking Optimizer Based on Dynamic Programming" Forests 16, no. 5: 780. https://doi.org/10.3390/f16050780
APA StyleBennemann, C., Lussier, J.-M., & Labelle, E. R. (2025). An Open-Source Tree Bucking Optimizer Based on Dynamic Programming. Forests, 16(5), 780. https://doi.org/10.3390/f16050780








