The Influence of the Root Diameter of Cunninghamia lanceolata (Chinese Fir) on the Strength and Deformation Behavior of Sand

Abstract

This study used triaxial tests to examine the impact of the root diameter of Cunninghamia lanceolata (Chinese fir) on the mechanical behavior of sand, including stress–strain development, strength, volumetric strain, and failure envelope. It also revealed the reinforcement mechanisms of roots with different diameters based on root–soil interactions. The results showed the following: (1) The addition of roots significantly enhanced sand strength and reduced volumetric deformation. The average peak strength increased by 31.8%, while the average peak volumetric strain decreased by 34.3%. (2) Roots provided additional cohesion and increased the friction angle of the sand, causing the failure envelope to shift upward and deflect. (3) Smaller-diameter roots improved the mechanical properties of sand more significantly, leading to higher peak strength, shear strength parameters, and smaller volumetric deformation. As the root diameter increased from 1 mm to 5 mm, the peak strength ratio decreased from 1.78 to 1.13, and the peak volumetric strain increased from 0.48 to 0.79. (4) Smaller-diameter roots, which form denser networks, allowing more roots to resist loads, and have a higher elastic modulus providing greater tensile stress, also possess higher tensile strength and critical sliding tensile stress, making them less likely to fail, thereby making the mechanical reinforcement of sand more significant.

1. Introduction

With the increasing frequency and intensity of extreme weather events, shallow landslide disasters are becoming more common, exacerbating regional soil erosion and ecological degradation [1]. Sand is a critical soil type for slopes, covering approximately 4,990,200,000 hectares globally and accounting for 31% of the total land area [2]. Its loose structure, low cohesion, and poor erosion resistance make it highly susceptible to slope failures [3,4], as exemplified by sandy soil landslides in the Anning River Basin of Southwest China [5], Shikoku Island in Japan [6], and Jinbu-myeon in South Korea [3]. Effective slope soil stabilization is essential for preventing such landslides and ensuring the stability of infrastructure. Traditional methods, such as retaining walls and soil nails, while effective, are often costly and can pose environmental challenges [7]. Vegetated slope protection has emerged as a sustainable bioengineering method that offers a more economical and environmentally friendly alternative [8,9,10]. This approach utilizes the natural root systems of plants to reinforce soil structure, enhance its mechanical properties, and reduce erosion and landslide risk [11,12,13]. Understanding the role of roots in improving soil strength and stability is crucial for the development of effective vegetated slope protection techniques, particularly in sandy areas.

One of the most important contributions of vegetation in improving slope stability and mitigating soil erosion is the mechanical reinforcement provided by roots embedded in the soil [14,15,16]. Many studies have been conducted to investigate the mechanical reinforcement of slopes by roots through field and laboratory shear tests [17,18,19,20,21,22]. This reinforcement is widely recognized for providing additional soil strength, with the degree of reinforcement largely depending on the tensile strength of the roots and the extent of the root area crossing the shear plane [23,24,25].

Rainfall, being one of the main triggering factors for shallow landslides [26], can cause shallow soil layers to become saturated during intense or prolonged events [27,28,29]. The mechanical reinforcement provided by roots is one of the dominant mechanisms determining the stability of vegetation-covered saturated shallow slopes [30,31]. Recently, some researchers [32,33,34,35] have turned their attention to the mechanical reinforcement effect of roots in saturated soils. Ensuring sample saturation and accurately monitoring pore water pressure development during deformation and failure in direct shear tests presents significant challenges [32,36]. Triaxial tests have become the preferred method for investigating the influence of roots on the mechanical properties of saturated soils. Related studies indicate that the strength of saturated soils is enhanced by roots, with the mechanical properties significantly influenced by the root content [33,35] and arrangement [32,34].

Vegetation roots exhibit significant natural variability [37], with root content and root diameter in soil varying significantly with soil depth [37,38], soil type [39], vegetation type [40], and the plant growth cycle [41]. Root diameter determined the mechanical properties of the roots themselves, including tensile strength, ultimate tensile strain, and elastic modulus [40,42]. Additionally, root diameter was a key factor influencing the friction strength at the root–soil interface [43,44]. These characteristics directly affected the mechanical reinforcement provided by the roots to the soil [25,45,46]. Therefore, root diameter is a critical factor to consider when studying the mechanical reinforcement mechanisms of roots on soil. However, there is limited research on how root diameter impacts soil mechanical behavior.

This study conducted a series of triaxial tests on the root–soil composites consisting of Cunninghamia lanceolata roots and saturated sand with varying root diameters (1–5 mm). It systematically analyzed the effects of root diameter on the stress–strain relationship, strength, failure envelope, and volumetric strain of the sand. Additionally, uniaxial tensile tests on root mechanical properties and friction strength tests at the root–soil interface were performed to reveal the mechanical reinforcement mechanisms of sand by roots of different diameters based on root–soil interactions. The research findings will provide elaborate guidelines for understanding the mechanical reinforcement mechanisms of different root diameters in the sand and defining the mechanical response following root reinforcement. They will also offer a partial basis for developing constitutive relationships that predict the mechanical behavior of root-reinforced sand, which will be helpful in designing and assessing effective vegetative slope protection techniques.

2. Materials and Methods

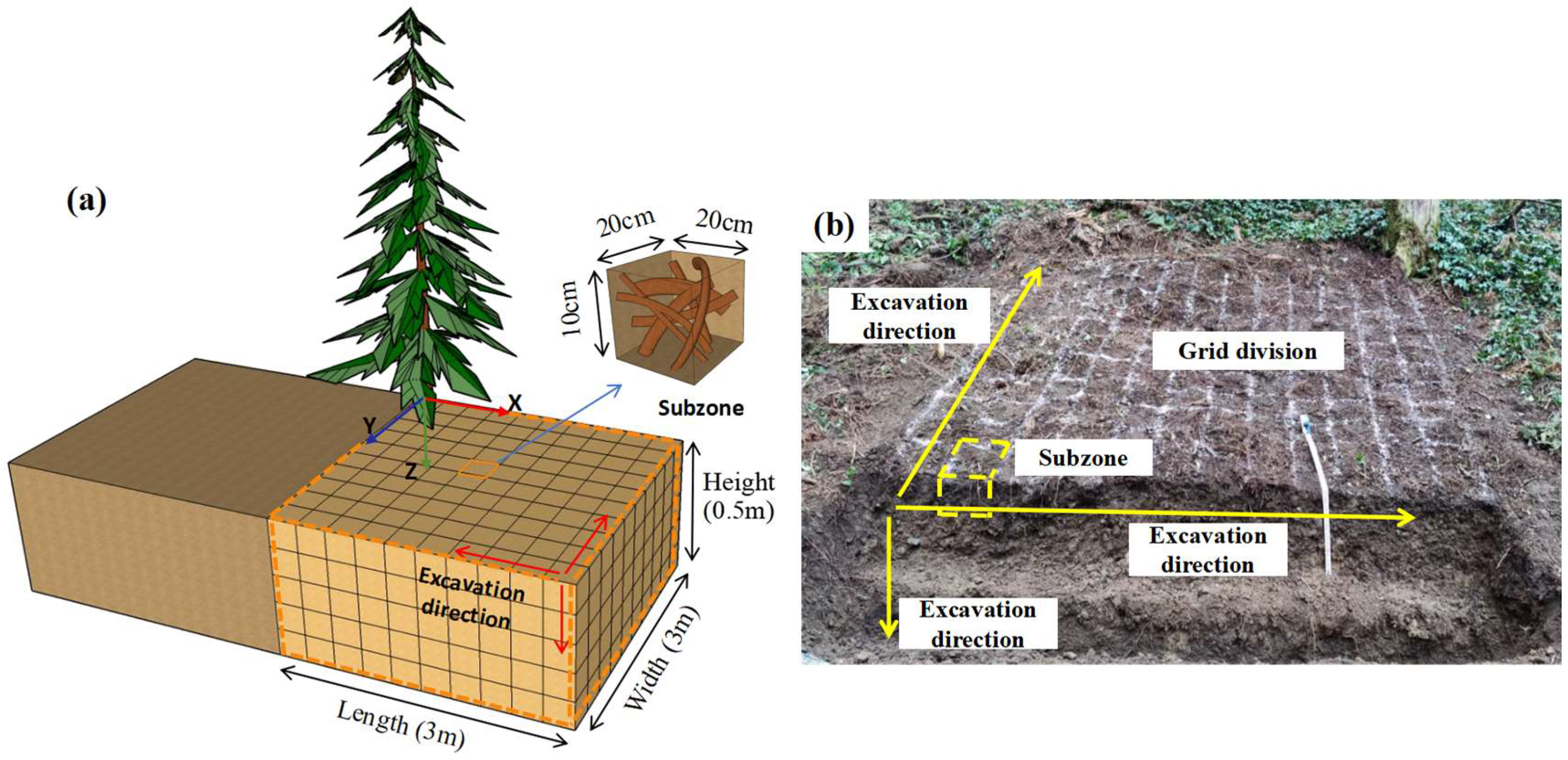
2.1. Study Area and Field Investigations

2.1.1. Study Area

The roots and soil samples used in this study were collected from the Longchi National Forest Park in the Longxi River Basin, as shown in Figure 1. The region is prone to geological hazards like landslides, collapses, and debris flows, particularly with shallow landslides prevalent along debris flow channels and roadsides [47]. The Cunninghamia lanceolata tree (Cupressaceae, Chinese fir), one of the dominant species in the study area, plays a vital role in soil and water conservation, water source preservation, and climate regulation [13]. It primarily grows at altitudes between 1500 and 3000 m, and its roots were used in this study [48].

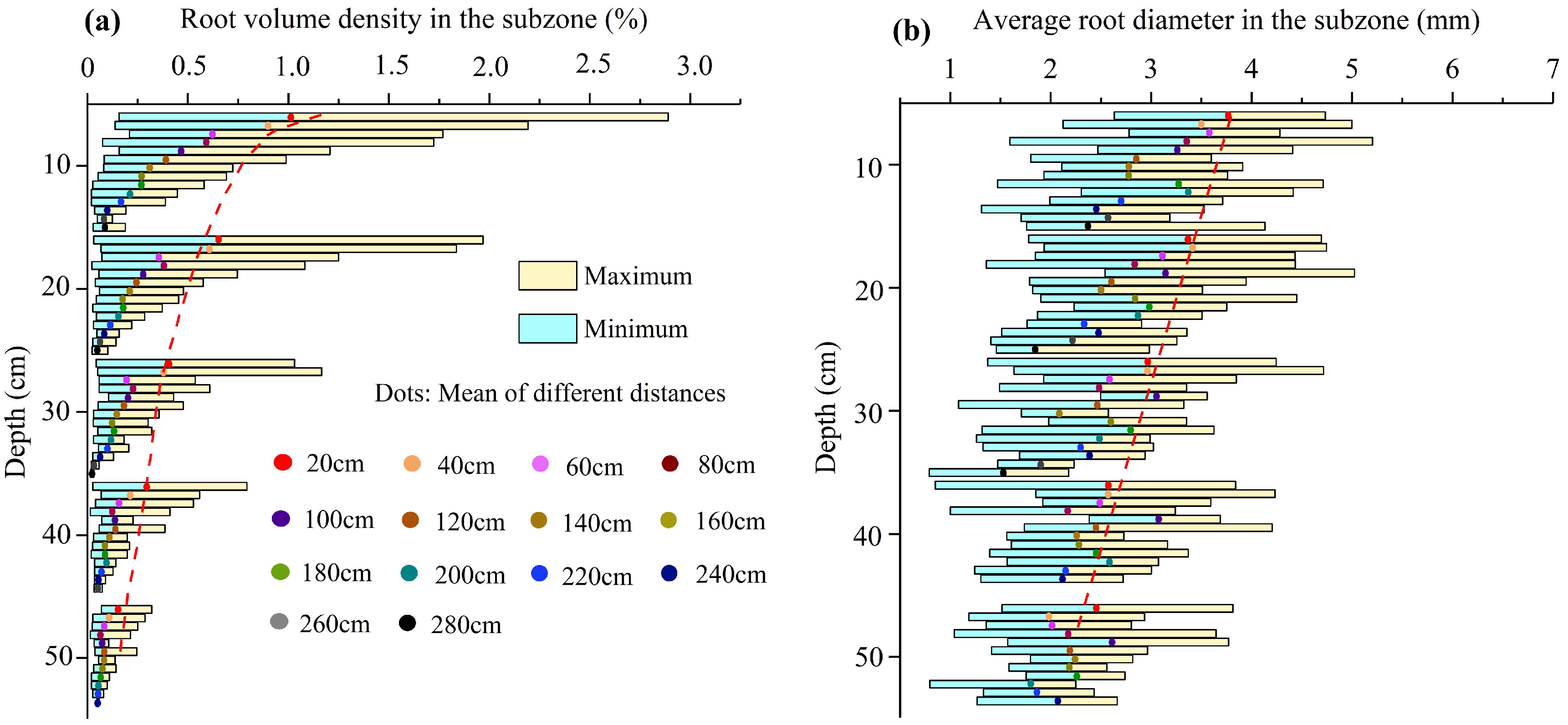
2.1.2. Root Field Investigation

To determine the root diameter and root content in the triaxial tests of root–soil composites, a field survey was conducted to measure the average root diameter and root volume density of Cunninghamia lanceolata tree using the profile excavation method. A sample tree with a breast height diameter of 425 ± 5 mm was selected at 103°34′15″ E longitude and 31°3′33″ N latitude. An area covering 1/4 of the downhill root growth area, measuring 3 m in length and width, was excavated. The excavation area was marked with white powder and divided into a grid of 20 cm × 20 cm squares (Figure 2a). Excavation was carried out in 10 cm increments vertically until the maximum root growth depth was reached (Figure 2b). Roots from each sub-zone were collected, placed in labeled plastic bags, vacuum-sealed, and stored in a refrigerator at 4 °C. The diameter and volume of the roots in each sub-zone were measured, and the average root diameter (D_ave) and root volume density (RVD) were calculated for each sub-zone, as shown in Equations (1) and (2), respectively.

$$D_{\mathrm{ave}}={\displaystyle \frac{{\displaystyle \sum _{\mathrm{i}=1}^n{D}_i}}{n}}$$

(1)

$$RVD={\displaystyle \frac{V_r}{V_{all}}}$$

(2)

where D_i represents the diameter of each root within the sub-zone (mm); n is the number of roots within the sub-zone, V_r is the total volume of roots within the sub-zone (mm³), and V_all is the volume of the sub-zone, which is 4 × 10³ cm³ in this study.

As shown in Figure 3, both root volume density and average root diameter decrease with increasing soil depth. This pattern is consistent with the field survey results of Cunninghamia lanceolata roots by Alam et al. (2020) [48]. Additionally, the root volume density in the 1125 tested sub-zones predominantly ranges from 0 to 2.4%, and the average root diameter is mainly between 1 mm and 5 mm. Studies by Meng et al. (2020) [49] and Alam et al. (2022) [35] have demonstrated that higher root content in the soil significantly enhances its mechanical reinforcement. This study focuses on the influence of root diameter on the mechanical behavior of sand. To emphasize the mechanical reinforcement effect of roots on soil, a higher root volume density of 2.4% was used in the triaxial tests, with root diameters selected at 1 mm, 2 mm, 3 mm, 4 mm, and 5 mm.

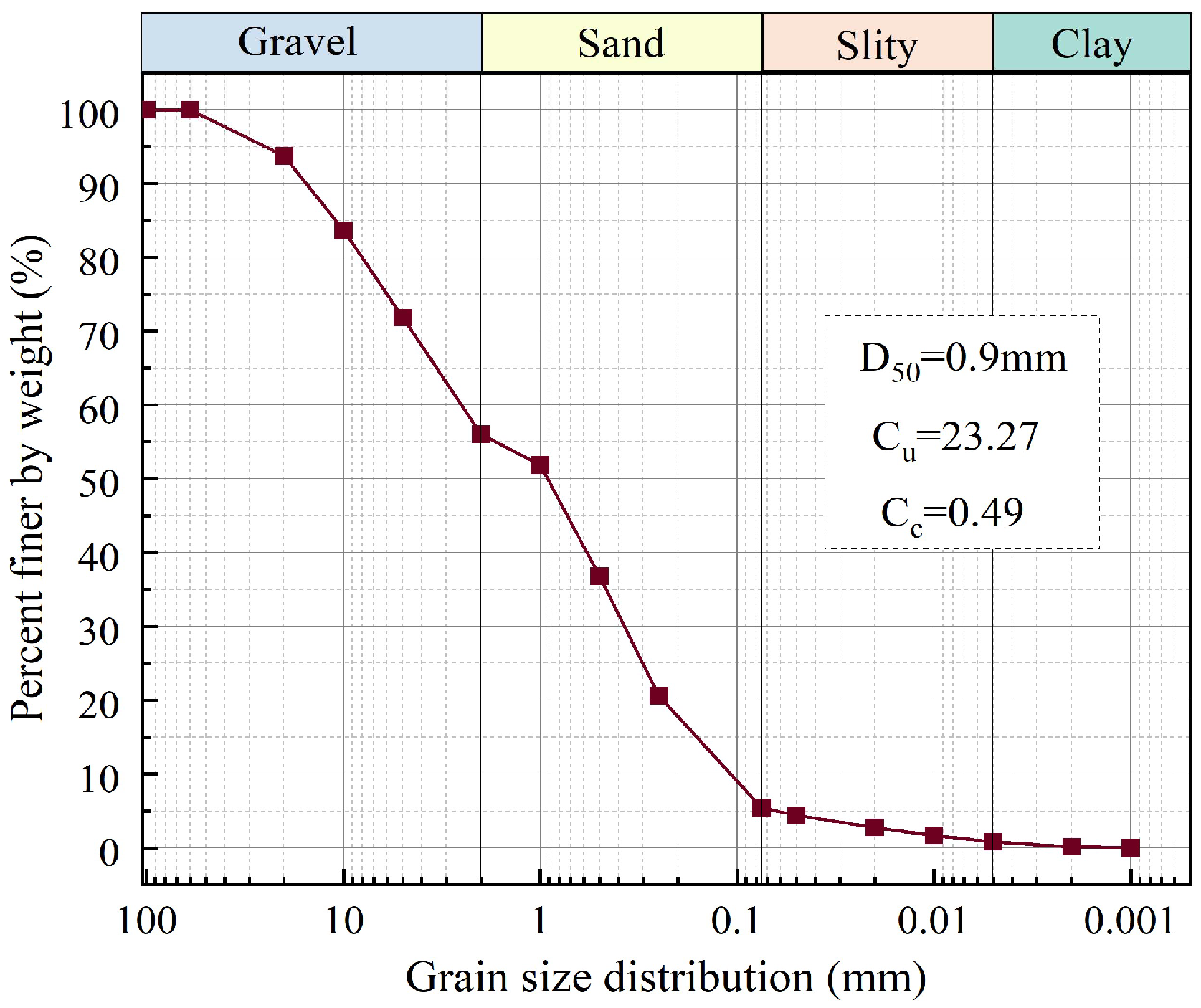
2.2. Soil and Root Properties

2.2.1. Soil

The soil was collected from the depth 0.35 m to 0.6 m in the root excavation area. The collected soil was dried at a temperature of 105 ± 5 °C for 24 h and then sieved through a 2 mm mesh to prepare it for the experiment. The particle size distribution of the dried soil samples was determined using both the sieving method and laser particle size analysis (<0.25 mm). The particle size distribution curve is shown in Figure 4. According to the ASTM 2017 soil classification method, the experimental soil is classified as poorly graded sand (Group: SP). The in situ soil moisture content and dry density, as well as the physical parameters of the experimental soil, were tested according to GB/T 50123-2019 [50]. The test results are shown in

Table 1. Properties of the sand for test.

Maximum Dry Density (g·cm−3)	Minimum Dry Density (g·cm−3)	Unit Weight (g·cm−3)	Field Dry Density (g·cm−3)	Soil Water Content (%)	Plastic Limit (%)	Liquid Limit (%)
2.10	1.41	2.65	1.62	10	13.6	21.23

.

2.2.2. Roots

The M.T.S. Bionix servo-hydraulic system was used for the tensile tests of Cunninghamia lanceolata roots with different diameters, with a tensile rate of 1 mm/min, as shown in Figure 5. To prevent the clamps from damaging the roots, the ends of the segments were hardened with synthetic resin. The peak stress point of tensile stress–strain curve represents the tensile strength of the roots (Equation (3)), and the tensile stress corresponding to 1% strain represents the elastic modulus of the roots (E_r). As shown in

Table 2. Tensile mechanical properties of roots with different diameters.

Mechanical Parameters	Fitted Curve	R2
Maximum tensile force	$F_t=19.96D_r^{1.57}$	0.96
Tensile strength	$T_r=25.41D_r^{-0.43}$	―
Elastic modulus	$E_r=1604.75D_r^{-1.34}$	0.99

, there is a significant positive correlation between the maximum tensile force (F_t) and the root diameter, which is described by a power function (Equation (4)) [40,42,51]. Combining this with Equation (3), the relationship between the tensile strength and the root diameter is given by Equation (5), where tensile strength decreases as diameter increases [51,52]. Additionally, the analysis shows that the elastic modulus of the roots decreases following a power law as the diameter increases [51].

$$T_r={\displaystyle \frac{F_t}{\pi \cdot D_r^2/4}}$$

(3)

$$F_t=a{(D_r)}^b$$

(4)

$$T_r={\displaystyle \frac{4}{\pi }}a{(D_r)}^{b-2}$$

(5)

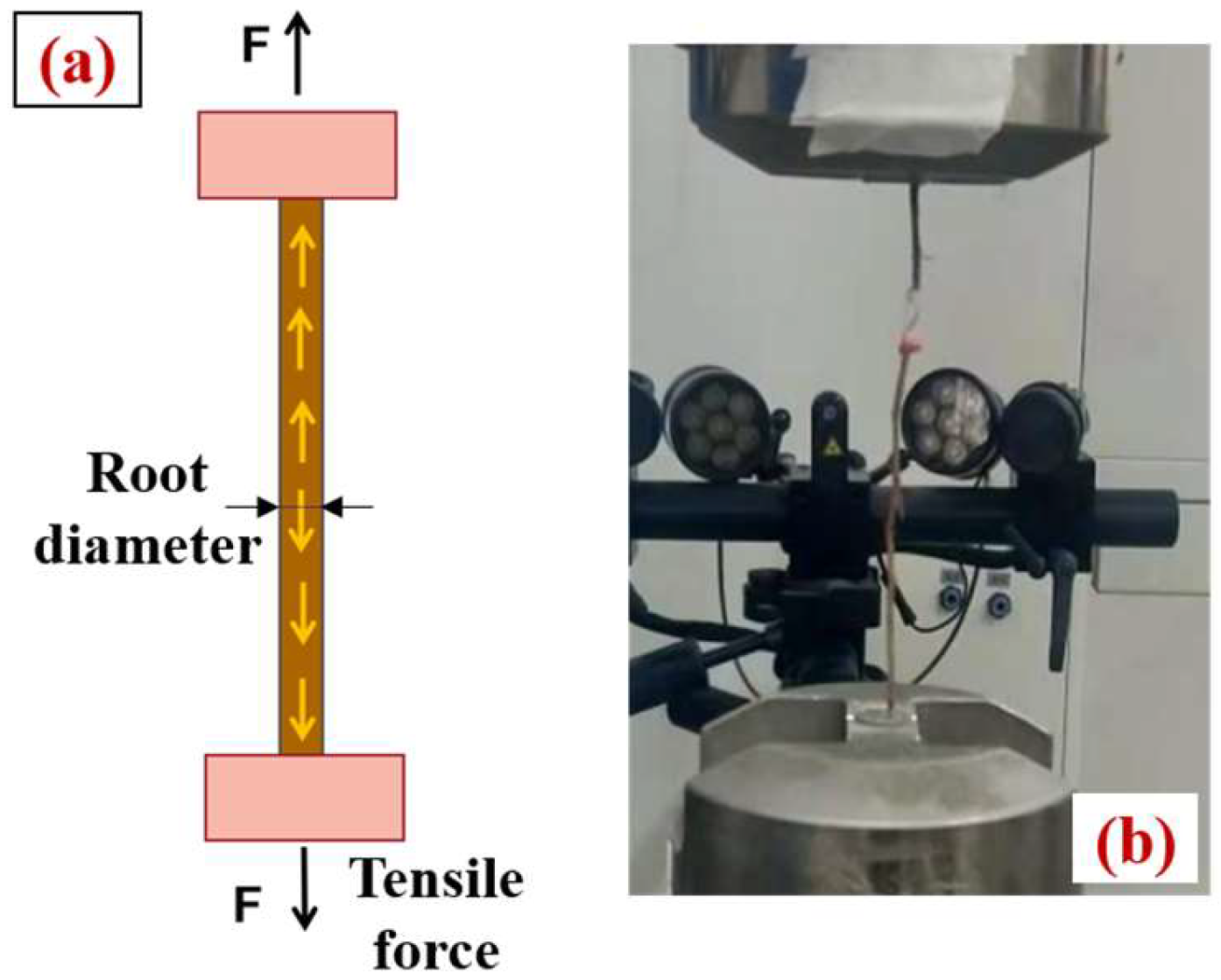
where T_r is tensile strength of roots (MPa), F_t is the maximum tensile force during the root tensile process (N), D_r is the diameter of the root (mm), and a and b are fitting parameters.

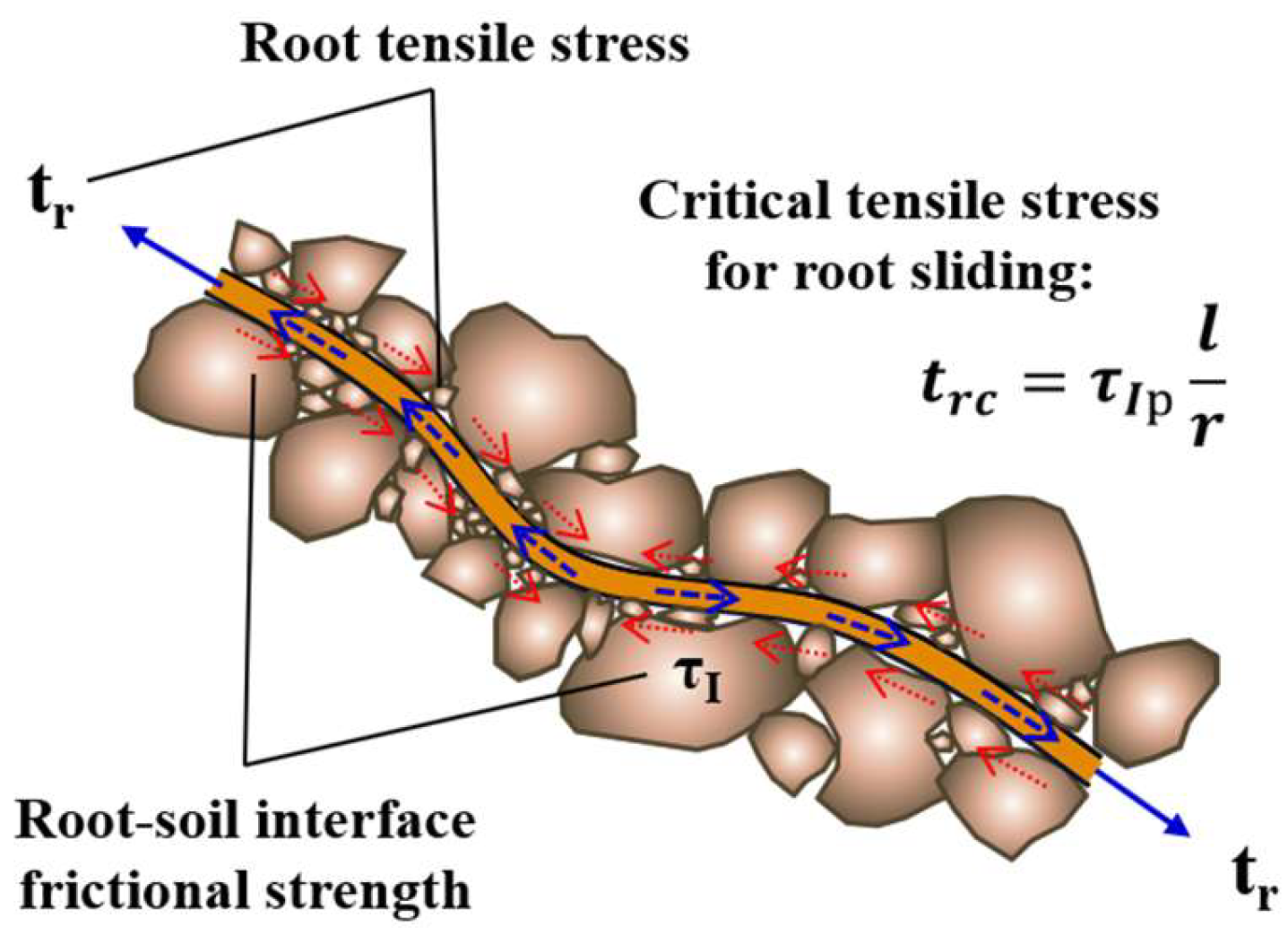
2.2.3. Root–Soil Interface Friction Strength

The friction strength tests between roots of different diameters and sand were conducted using a custom-designed root-pulling testing apparatus developed by the Chengdu Mountain Institute [53]. The pulling rate was set at 1 mm/min, and the pulling strain was 20%. This apparatus can apply different normal stresses to the pull-out specimens to calculate the friction strength parameters of the root–soil interface, as shown in Figure 6. The peak point of the shear stress–strain curve represented the friction strength of the root–soil interface (Equation (6)). Studies by Mickovski et al. (2010) [54] and Ning et al. (2023) [1] have shown that the friction strength of the root–soil interface conforms to Coulomb’s law (Equation (7)).

Table 3. Frictional strength parameters at the root–soil interface for different root diameters.

Root Diameter	Peak Frictional Strength Parameters
	cIp	φIp	R2
1 mm	2.00	37.95	0.93
2 mm	1.99	38.66	0.90
3 mm	2.26	39.35	0.93
4 mm	2.36	40.36	0.94
5 mm	2.55	41.35	0.95

presents the friction strength parameters of the root–soil interface for various root diameters. Overall, larger root diameters correlate with higher friction strength at the root–soil interface, with corresponding friction strength parameters also increasing, consistent with the conclusions of Mickovski et al. (2010) [54] and Lin et al. (2024) [12]. Furthermore, the friction strength parameters at the root–soil interface (c_Ip and φ_Ip) show a significant positive linear correlation with root diameter (Figure 7).

$${\tau }_I={\displaystyle \frac{F_p}{\pi \cdot D_r\cdot (l_0-l_p)}}$$

(6)

$${\tau }_{Ip}=c_{Ip}+{\sigma }_n\tan {\phi }_{Ip}$$

(7)

where τ_Ip represents the peak shear stress at the root–soil interface (kPa); F_p is the maximum pull-out force during the root-pulling process (N), which is equal in magnitude but opposite in direction to the frictional resistance on the roots; and σ_n, c_Ip, and φ_Ip represent the normal stress (kPa), peak cohesion (kPa), and peak friction angle (°) at the root–soil interface, respectively.

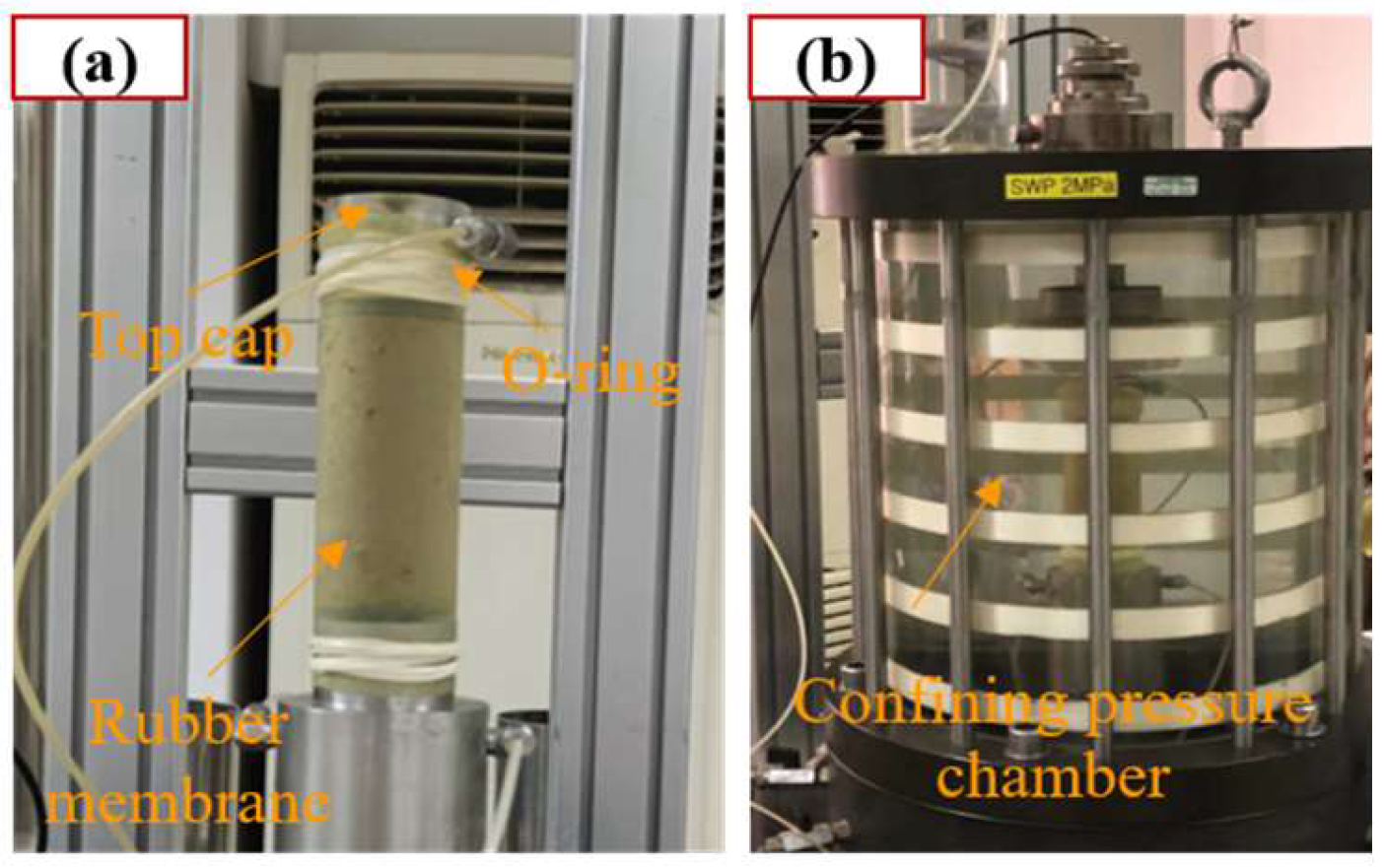
2.3. Preparation of Triaxial Specimens

All specimens were reconstituted to match the in situ soil moisture content (10%) and dry density (1.62 g/cm³). The specimen for triaxial testing had dimensions of 50 mm in diameter and 100 mm in height. According to GB/T50123-2019 [50], the bare soil specimen was prepared by mixing distilled water with sieved dry soil to achieve in situ moisture content. After placing the specimen in a sealed container for 24 h to ensure uniform moisture distribution, the soil was compacted into a mold in three layers to reach the desired dry density. For the rooted specimens depicted in Figure 8, roots were trimmed to 40 mm to prevent stress concentration at specimen boundaries [34,35]. The root volume density (the ratio of total root volume to sample volume) for the samples with different root diameters was 2.4%. To ensure uniformity, the required roots for each sample were divided into three equal portions. Each portion was then thoroughly mixed with one-third of the required soil and compacted layer by layer to prepare the root–soil composite samples [35].

2.4. Apparatus and Testing Procedure

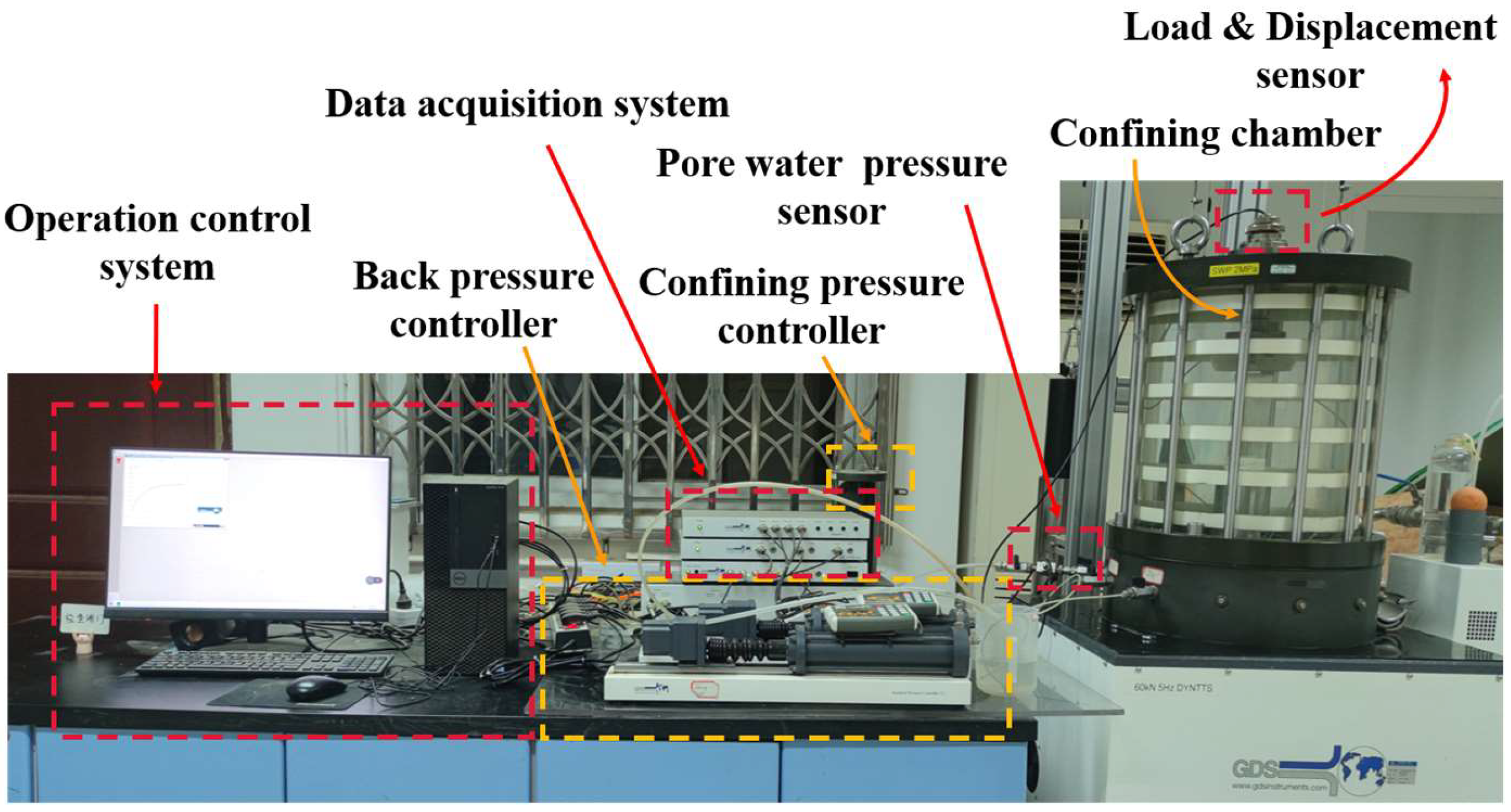
2.4.1. Test Apparatus

This research tested the mechanical properties of sand and root–soil composites under different drainage conditions using the GDS triaxial testing apparatus, as shown in Figure 9. The equipment includes an operating system, GDSLAB control software v2.8.2, data acquisition system, pressure controllers, a pressure chamber, loading devices, and various sensors. The sampling frequency for test data is 0.5 Hz.

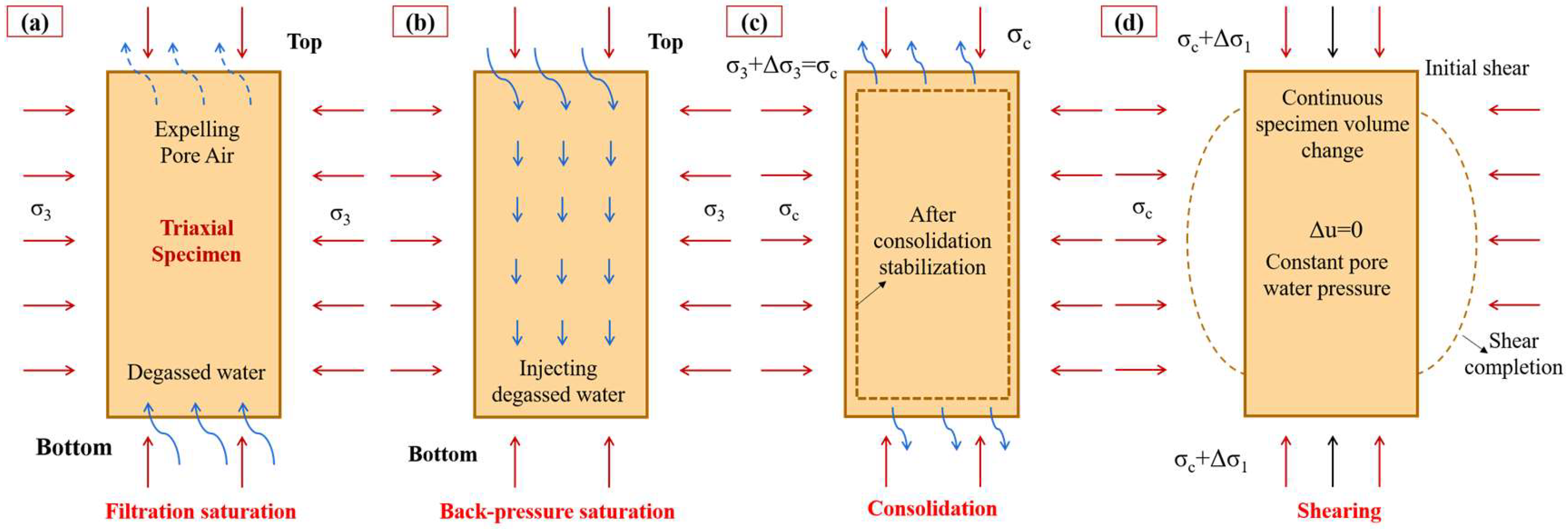
2.4.2. Testing Procedure

According to GB/T50123-2019 [50], the triaxial compression test involved four stages: sample installation (Figure 10), saturation, consolidation, and shearing, as shown in Figure 11. The detailed steps are as follows:

(1) Specimen installation: Filter paper and porous stones were placed at the top and bottom of the specimen, which was enclosed in a rubber membrane and carefully positioned on the pressure chamber base. The top cap was then secured with the rubber membrane, and O-rings were used to seal the connections between the membrane, the chamber base, and the top cap;

(2) Saturation: To achieve complete saturation (B > 0.95), both filtration and back-pressure methods were used. Filtration started from the toe to the top with a confining pressure of 10 kPa and a differential back-pressure of 5 kPa. For back-pressure saturation, the confining pressure was kept at 8 kPa higher than the back-pressure, both gradually increasing and maintaining for 0.5 h;

(3) Consolidation: During this stage, the specimen is consolidated stably under the target confining pressure. The excess pore water pressure rapidly increased with the rise in confining pressure and then gradually dissipated under consolidation, while the specimen continuously compressed and expelled water. The process was monitored by measuring the excess pore water pressure and expelled water volume (back volume). Consolidation stability was achieved when the excess pore water pressure dissipated by 98% and the back volume change was less than 5 mm³ within 5 min. To avoid unloading rebound, the confining pressure was kept higher than the sum of the pressure difference at saturation and the B-detection stage increment. Eight initial confining pressures were used: 15, 30, 50, 100, 150, 200, 300, and 400 kPa;

(4) Shearing: The stress path applied in the triaxial test was the consolidation–drainage (CD). During the test, the excess pore water pressure of the specimen remained constant, and the continuous volume changes were reflected by the increase and decrease in the back pressure volume. The loading rate was set at 0.01 mm/min, and shearing was terminated at 20% axial strain in both tests.

3. Results

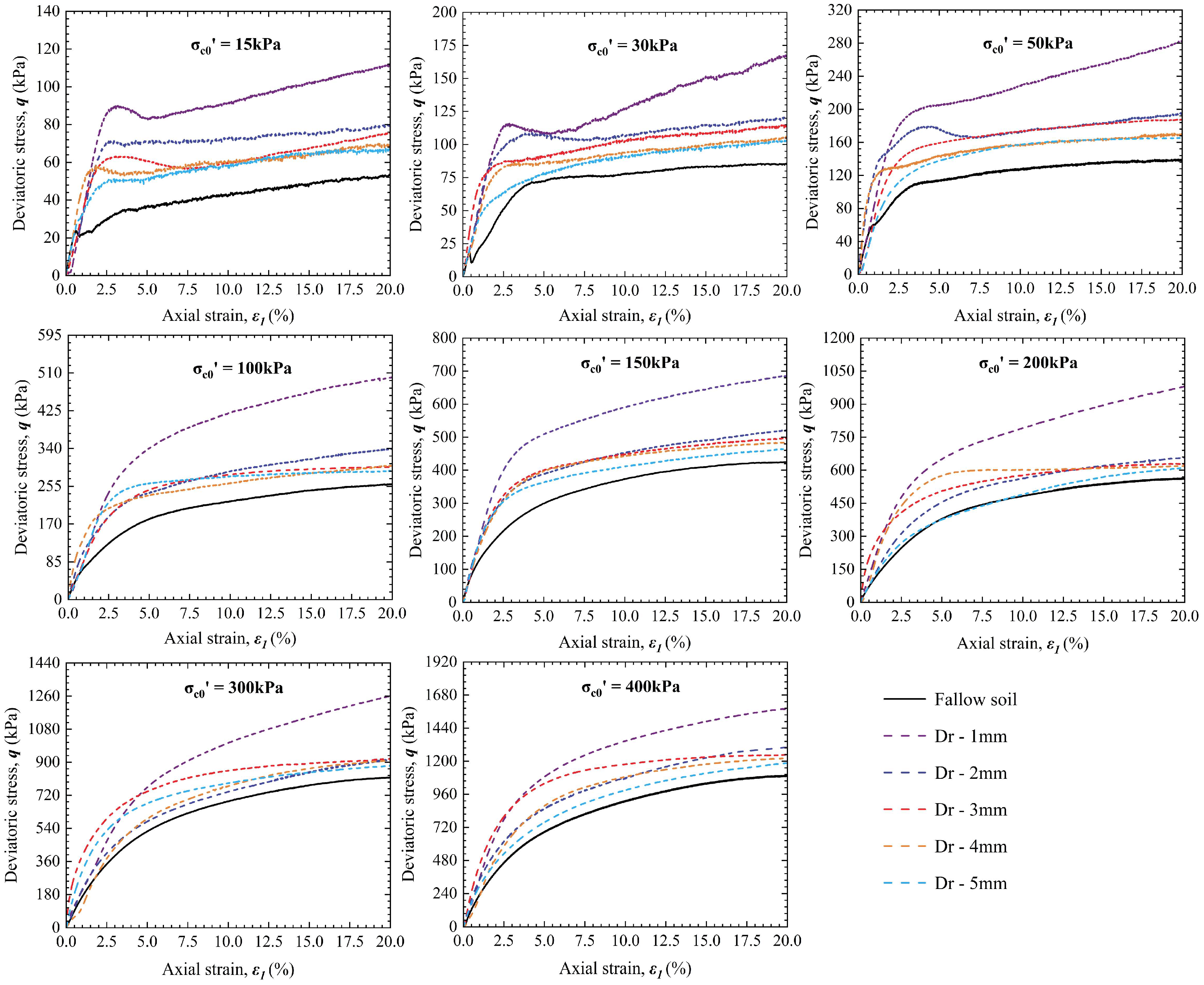
3.1. Stress–Strain Behavior

As illustrated in Figure 12, overall, all samples exhibited strain-hardening responses, with deviatoric stress (the difference between the major principal stress σ₁ and minor principal stress σ₃) increasing with axial displacement during the shear failure process. The deviatoric stress of the root–soil composite samples was higher than that of the bare soil samples. Furthermore, the stress–strain behavior of root–soil composites was directly influenced by root diameter. Under the same confining pressure, smaller root diameters resulted in higher deviatoric stress and more pronounced hardening responses during the deformation process. Moreover, the stress–strain behavior of the root–soil composites was related to confining pressure, with higher confining pressures leading to greater deviatoric stress and a more significant hardening response. When the confining pressures were 15, 30, and 50 kPa, the deviatoric stress of the root–soil composites rapidly increased during the initial shearing phase (an axial strain of 1%–5%) before slightly decreasing. With further shearing, the deviatoric stress continued to increase, exhibiting overall strain-hardening characteristics. When the confining pressure exceeded 50 kPa, the deviatoric stress consistently increased with axial strain until deformation stabilized, showing significant strain-hardening characteristics.

The volumetric strain (ε_v, a measure of the specimen’s volume change) of the triaxial specimen is defined as positive for compression and negative for expansion, as calculated in Equation (8). A higher absolute value indicates a greater volume change in the specimen. As depicted in Figure 13, the peak volumetric strain of the specimens increased with rising confining pressure. The root–soil composite consistently exhibited a smaller volumetric strain compared to the soil. Both the roots and the confining pressure significantly influenced the volumetric deformation behavior of the specimens. At confining pressures of 15, 30, and 50 kPa, the root–soil composite specimens initially exhibited slight dilation (an axial strain of 1%–5%). As shearing progressed, the volume decreased, and the specimens contracted. This behavior corresponded to the deviator stress–axial strain response of the root–soil composite specimens. The peak strength observed in the early stages of shearing was related to the volumetric deformation of the root–soil composite.

$${\epsilon }_v={\displaystyle \frac{\Delta V}{V_0-\Delta V_C}}$$

(8)

where V₀ is the sample’s initial volume, mm, and ∆V and ΔV_C denote the sample’s volume change during shearing and after isotropic consolidation, respectively, both in mm.

3.2. Strength Characteristics

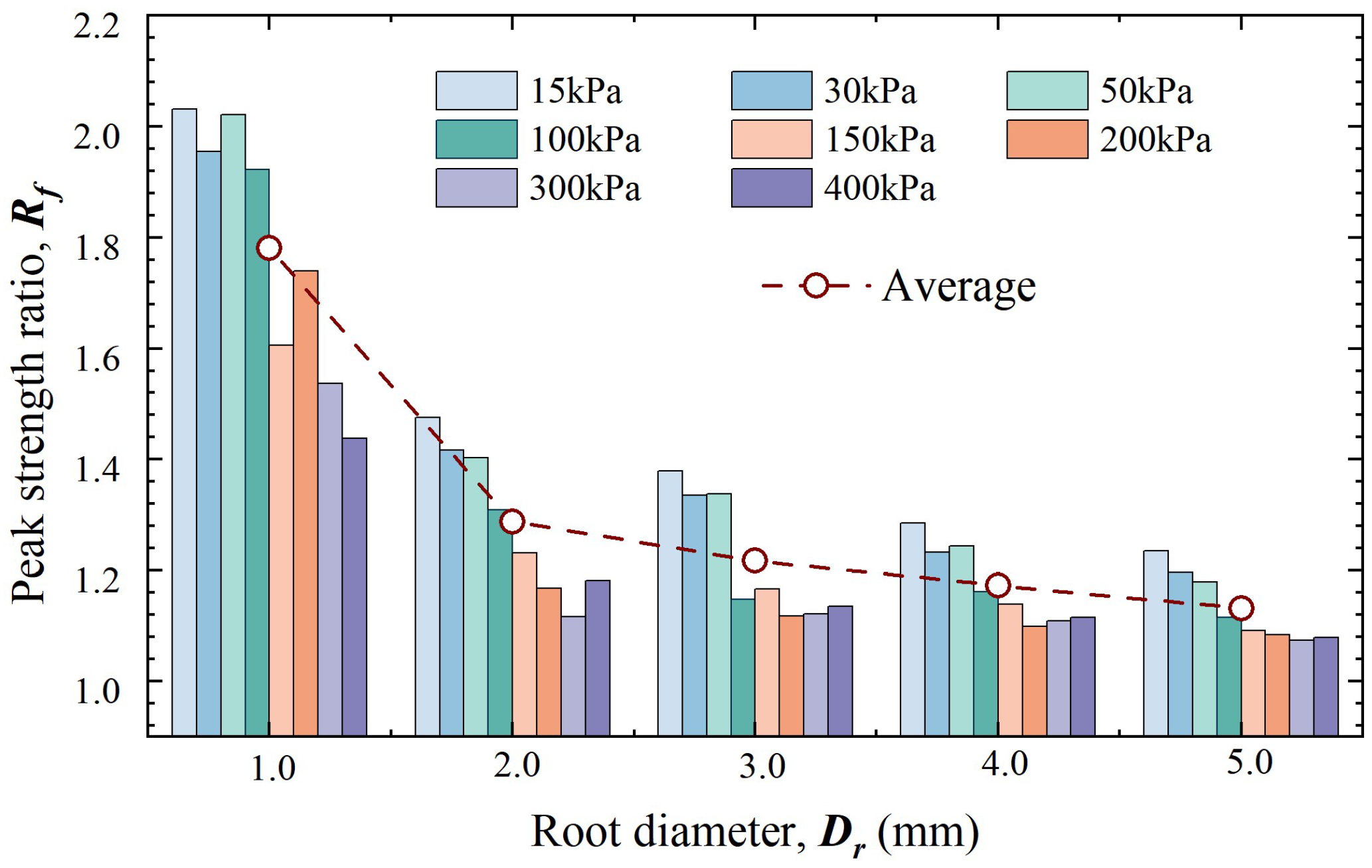
3.2.1. Characteristic Strength Ratio

The peak strengths of root–soil composites and bare soil are shown in

Table 4. Peak strength of root–soil composites (kPa).

Confining Pressure (kPa)	Bare Soil	Soil + 1 mm Roots	Soil + 2 mm Roots	Soil + 3 mm Roots	Soil + 4 mm Roots	Soil + 5 mm Roots
15	55.39	112.53	81.75	76.39	71.20	68.40
30	86.94	169.93	123.14	116.07	107.18	104.07
50	140.59	284.13	197.25	188.08	174.98	165.76
100	260.95	501.60	341.50	299.49	302.96	291.14
150	427.65	686.75	526.86	498.83	487.18	466.80
200	565.15	982.95	659.62	631.37	621.33	612.40
300	821.11	1261.81	916.78	921.02	911.08	882.26
400	1100.53	1582.34	1300.73	1249.05	1226.82	1187.52

. The strength ratio parameter (R_f) was introduced to quantify the effect of roots on the shear strength of sand, as described in Equation (9) [55,56]. Figure 14 illustrates the characteristic strength ratios of root–soil composites with varying root diameters under eight levels of confining pressure. Generally, the addition of roots increased the peak strength of sand under different confining pressures, with an average increase of 31.8%. However, larger root diameters were associated with a lower peak strength ratio in the root–soil composites at the same confining pressure. The average peak strength ratio of root–soil composites was observed to decrease from 1.78 to 1.13 as the root diameter increased from 1 mm to 5 mm. Specifically, the addition of 1 mm roots increased sand strength by 78.1%, 2 mm roots increased sand strength by 28.8%, 3 mm roots increased sand strength by 21.7%, 4 mm roots increased sand strength by 17.3%, and 5 mm roots increased sand strength by 13.2%. These findings indicate that the addition of roots significantly improves the strength of sand and enhances slope stability, with smaller-diameter roots exerting a more pronounced effect on the strengthening of sand and the stabilizing of slopes.

$$R_f={\displaystyle \frac{{(q)}_{rf}}{{(q)}_{sf}}}$$

(9)

where the peak deviatoric stresses, (q)_rf for the root–soil composite and (q)_sf for the bare soil specimens, are identified at the peak points on the deviatoric stress vs. axial strain curves [57].

3.2.2. Shear Strength Parameters

In accordance with the Mohr–Coulomb strength criterion, Mohr circles (stress trajectories on any plane under the action of principal stresses σ₁ and σ₃) and failure envelopes (the limiting stress state at failure) for bare soil and root–soil composites were plotted in the σ-τ space, as shown in Figure 15. The failure envelope of the bare soil passed through the origin, indicating that the cohesion of the saturated sand was zero and that the shear strength was solely provided by the frictional strength between soil particles. In contrast, the failure envelope of the root–soil composite did not pass through the origin, indicating that the addition of roots elevated the soil’s failure envelope and provided an additional cohesion component to the soil. This finding is consistent with the results of previous related studies [58,59]. Furthermore, the roots not only provided additional cohesion but also enhanced the frictional strength of the soil.

According to the Mohr–Coulomb failure criterion, the shear strength parameters of the soil, including cohesion (c′) and internal friction angle (φ′), are given by Equation (10). This equation can be employed to calculate the shear strength parameters of root–soil composites [23,25,45].

Table 5. Effective shear strength parameters of root–soil composites.

Shear Strength Parameters c′ (kPa), φ′ (°)	Bare Soil	Roots-Soil Composite
		Dr—1 mm	Dr—2 mm	Dr—3 mm	Dr—4 mm	Dr—5 mm
c′	0.00	20.30	8.85	6.23	5.12	4.71
Δcr′	—	20.30	8.85	6.23	5.12	4.71
φ′	35.45	41.40	37.44	37.07	36.89	36.38
Δφr′	—	5.96	1.99	1.63	1.44	0.93

shows the shear strength parameters of bare soil and root–soil composites with varying root diameters. The cohesion and internal friction angle of root–soil composite specimens with varying root diameters were significantly higher than those of bare soil. Specifically, the addition of roots provided an additional cohesion with an average value of 9.04 kPa. This improvement can be attributed to the interlocking effect between roots and soil particles, which results in the formation of a more stable composite structure and a notable increase in the initial shear strength. Moreover, the addition of roots resulted in a considerable improvement in the internal friction angle of the sand, with an average increase of 2.39°. Additionally, the root diameter had a pronounced impact on the shear strength parameters of the root–soil composite. Smaller root diameters corresponded to higher cohesion and internal friction angle values of the root–soil composite. The additional cohesion and additional internal friction angle provided by the roots exhibited an inverse relationship with the root diameter. As the root diameter increased from 1 mm to 5 mm, the additional cohesion decreased from 20.3 kPa to 4.71 kPa, and the additional internal friction angle decreased from 5.96° to 0.93°, representing reductions of 76.8% and 12.1%, respectively.

$$\tau =c^{\prime }+\sigma \tan {\phi }^{\prime }$$

(10)

where τ and σ represent the soil shear strength and normal stress, respectively, both in kPa. Subscript ‘r’ was added before the aforementioned parameters to denote the corresponding strength parameters of the root–soil composite.

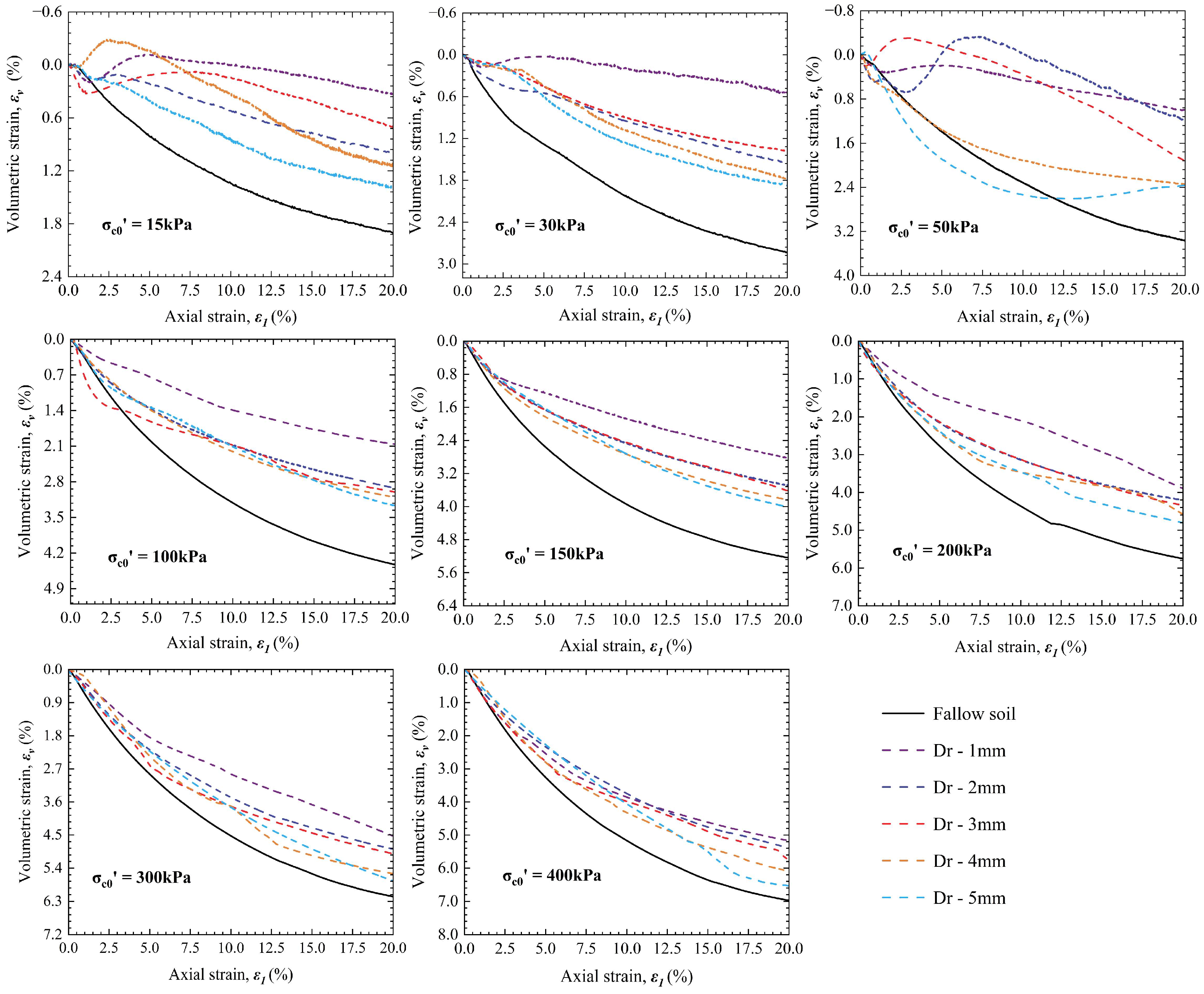
3.3. Volumetric Deformation Behaviour

The volumetric strains at peak strength for root–soil composites and bare soil are shown in

Table 6. The volumetric strain ratio at peak strength of root–soil composites (%).

Confining Pressure (kPa)	Bare Soil	Soil + 1 mm Roots	Soil + 2 mm Roots	Soil + 3 mm Roots	Soil + 4 mm Roots	Soil + 5 mm Roots
15	1.89	0.33	1.00	0.71	1.12	1.39
30	2.83	0.56	1.55	1.37	1.78	1.88
50	3.34	1.00	1.19	1.92	2.32	2.36
100	4.42	2.06	2.92	3.00	3.10	3.25
150	5.23	2.82	3.48	3.61	3.78	3.98
200	5.75	3.90	4.20	4.25	4.58	4.80
300	6.17	4.53	4.87	4.96	5.54	5.75
400	6.95	5.14	5.39	5.75	6.07	6.52

. The volumetric strain ratio at peak strength (V_f) was used to measure the effect of roots on soil volumetric deformation, as shown in Equation (11). Changes in root diameter significantly influenced the volumetric deformation behavior of the root–soil composite. As shown in Figure 13, under the same confining pressure, the volumetric strain of the root–soil composite decreased with smaller root diameters. This finding suggests that the smaller the root diameter, the more pronounced the restriction effect of the roots on the soil’s volumetric strain. The influence of root diameter on the volumetric strain ratio at peak strength for the root–soil composite is illustrated in Figure 16. Overall, as the root diameter increased, the peak volumetric strain ratio of the root–soil composite exhibited a gradual increase. For instance, when the confining pressure was 100 kPa, the root diameter increased from 1 mm to 5 mm, resulting in an increase in the peak strain ratio from 0.47 to 0.74. Furthermore, the average peak volumetric strain ratio increased from 0.48 to 0.79 as the root diameter rose from 1 mm to 5 mm. These results indicate that root addition significantly reduced the volumetric deformation of sand, thereby enhancing its resistance to deformation and mitigating slope failure. This improvement was more pronounced with smaller-diameter roots. Coupled with the analysis of soil strength, plants with smaller and denser roots demonstrate more prominent mechanical protective effects in vegetation-based slope protection projects.

$$V_f={\displaystyle \frac{{({\epsilon }_v)}_{rf}}{{({\epsilon }_v)}_{sf}}}$$

(11)

where (ε_v)_rf and (ε_v)_sf represent the volumetric strains at peak strength of the root–soil composite and bare soil specimens, respectively, identified at the peak points of the volumetric strain–axial strain curves.

4. Discussion

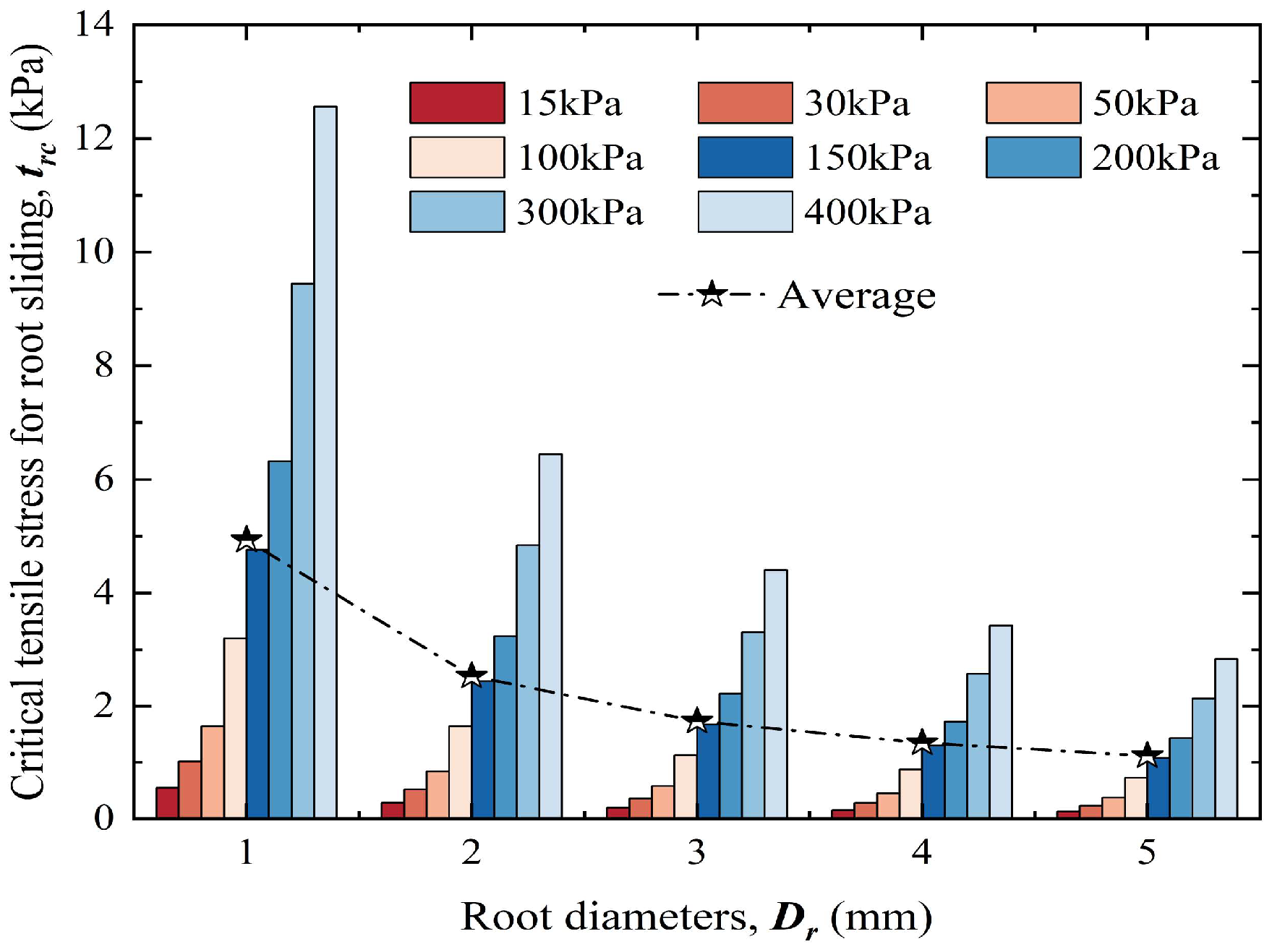
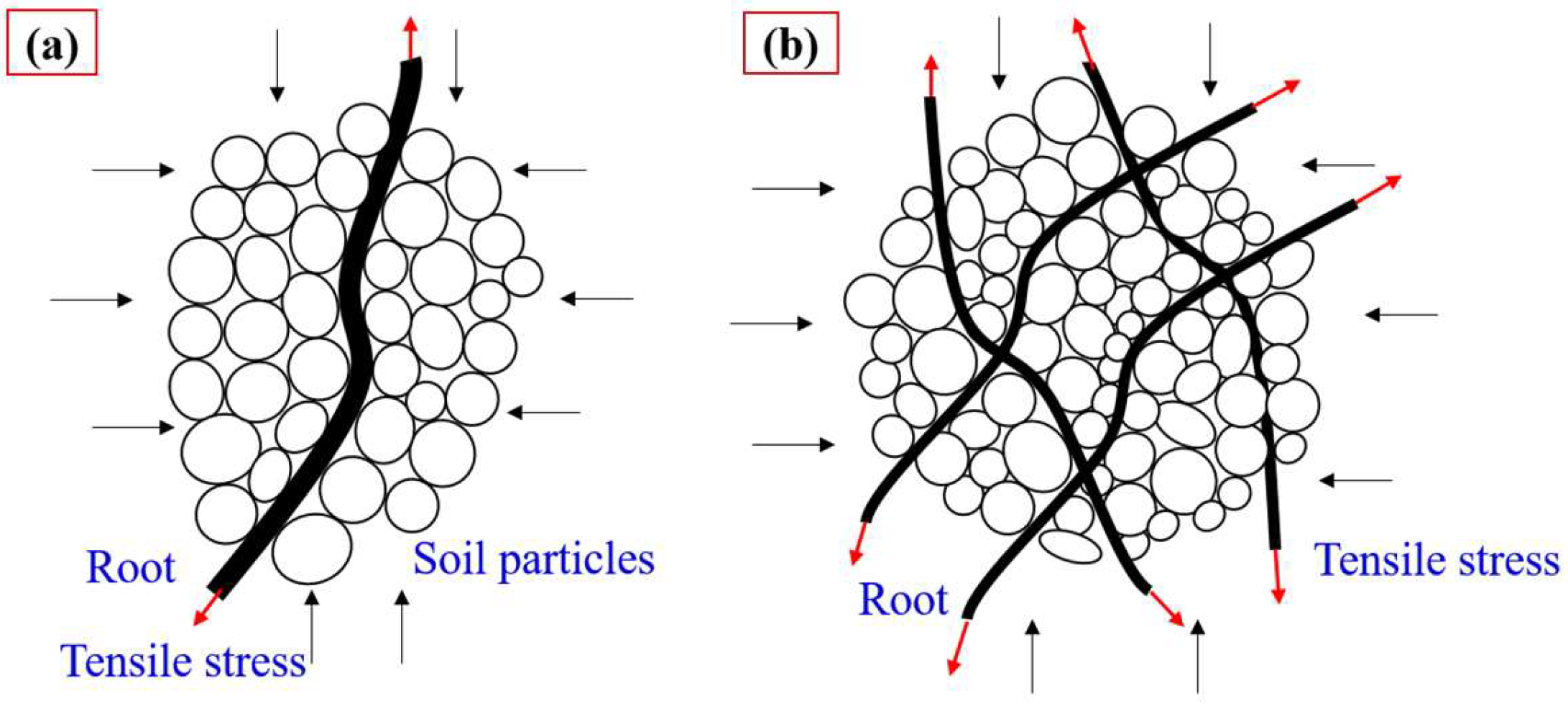
4.1. The Root–Soil Interaction for Different Root Diameters

The findings indicate that roots enhance the strength of sand, the failure envelope, and its resistance to deformation. Root diameter plays a critical role in these mechanical properties, with smaller root diameters showing a greater capacity to improve the mechanical behavior of sand. The mechanism through which root diameter influences the soil mechanical properties is complex, involving factors such as root mechanical properties, root–soil interface bonding, and the structure of the root–soil composite. Under external loads, the relative deformation or deformation trends between roots and soil result in frictional forces at the root–soil interface, activating and utilizing the tensile properties of the roots to constrain soil deformation and share external loads [25,45,46]. In this process, root failure in the soil can be categorized into two types: (1) when the root–soil interface bond is strong and frictional force is sufficient, roots may experience fracture failure due to tensile stress surpassing their strength; (2) if the frictional force at the root–soil interface is insufficient to fully mobilize tensile stress, root slippage failure may occur.

Michalowski and Zhao (1996) [60] proposed a threshold criterion based on fiber aspect ratio (Equation (13)) to determine the conditions under which fiber fracture failure occurs in soil. If the aspect ratio exceeds this threshold, fracture failure will occur; otherwise, fiber slippage may occur. In this study, the theoretical thresholds for root fracture failure exceeded the aspect ratios of all root–soil composites tested. Consequently, no roots experienced fracture failure during the deformation and failure stages, with root tensile stress being controlled by soil–root interface friction. This theoretical conclusion was consistent with observations in triaxial tests, where no root fractures occurred under different confining pressures and stress paths.

$$\eta ={\displaystyle \frac{l}{2r}}$$

(12)

$$\eta <{\displaystyle \frac{1}{2}}{\displaystyle \frac{T_r}{(c_{Ip}+{\sigma }_n\tan {\phi }_{Ip})}}$$

(13)

where η is the aspect ratio of the fiber, l is the fiber length, r is the fiber radius, and T_r is the tensile strength of the root.

Previous studies have shown that the tensile strength of roots is a crucial parameter in determining the effectiveness of root reinforcement [36], and this parameter is directly used to evaluate the shear strength of root–soil composites [23,25]. However, in the majority of studies on the strength of root–soil composites [33,34,36,49], root fracture has not been observed. In such cases, where the aspect ratio of the root in the soil is below the threshold for root fracture (Equation (13)), the shear strength of root–soil composites is not directly related to the tensile strength of roots. Conversely, the potential failure mode of roots is sliding failure, where the maximum tensile stress that roots can mobilize is referred to as the critical sliding tensile stress. A higher critical sliding tensile stress indicates greater resistance to root failure and a more pronounced improvement in the mechanical properties of the soil. As shown in Figure 17, when the cumulative tensile stress of the roots caused the shear stress at the root–soil interface to exceed the peak friction strength, sliding failure occurred at the root–soil interface, and the tensile stress of the roots rapidly decreased. Based on the stress equilibrium of roots in the soil, the critical tensile stress for root sliding was quantitatively calculated using Equation (14).

$$t_{rc}={\tau }_{Ip}{\displaystyle \frac{l}{r}}$$

(14)

where t_rc is the critical tensile stress for root sliding.

4.2. The Mechanism of Root Diameter’s Influence on Sand Strength

The peak deviatoric stress of the root–soil composite was generally higher than that of the bare soil under different confining pressures. Correspondingly, the peak shear strength parameters and residual strength parameters of the root–soil composite were also greater than those of the bare soil. These findings are consistent with previous studies on fiber-reinforced soils [61,62] and root-reinforced soils [32,33,35]. However, it is noteworthy that, under the same volume root content, the shear strength of the root–soil composite exhibited an inverse decrease with increasing root diameter. The effect of root diameter on the shear strength of the root–soil composite is rarely considered in current research. To our knowledge, only Meng et al. (2020) [49] considered root diameter differences in the strength of unsaturated clay–root composites. However, their study did not control for a single variable, as both root diameter and root content varied. This made it difficult to determine whether the observed differences in shear strength were due to root diameter or root content. In fiber-reinforced soils, the direct impact of fiber diameter on soil strength has rarely been studied, mainly because fiber materials typically have very small diameters, usually on the order of 0.01 mm [63,64,65]. Instead, research has focused more on the influence of fiber length [63,65,66] on soil strength.

Based on the analysis in Section 4.1, the root–soil interaction enables roots to mobilize their tensile stress to bear part of the external load, thereby enhancing soil strength. According to Table 2, both the elastic modulus and tensile strength of roots decreased following a power function with increasing root diameter. This result indicated that the smaller the root diameter, the greater the elastic modulus of the root, which meant that under the same tensile strain, smaller-diameter roots could generate higher tensile stress. This implied that in a root–soil composite, smaller-diameter roots could provide higher tensile stress at the initial stage of loading to resist external loads, thereby increasing the initial stiffness of the root–soil composite as root diameter decreased (Figure 12). As the root–soil composite deformed further, the tensile strain of the roots increased, with smaller-diameter roots still exerting higher tensile stress than larger-diameter roots to share external loads. In addition, Table 3 presents the interface friction strength between roots of different diameters and the soil, while the critical tensile stress during root sliding for different diameters, calculated using Equation (14), is shown in Figure 18. The critical tensile stress for root sliding in the root–soil composite decreased with increasing root diameter, indicating that larger-diameter roots were more prone to sliding failure.

In the soil, not all roots undergo sliding failure simultaneously, but rather, a combination of root tension and root sliding coexist [1,35,46]. From the above analysis, it can be inferred that both the elastic modulus of roots and critical tensile stress for root sliding decreased with increasing root diameter. As a result, regardless of whether root sliding failure occurs, smaller-diameter roots mobilize higher tensile stress and achieve a more comprehensive utilization of the root’s tensile properties. This allows the roots to share more external loads and significantly enhances the soil’s strength. On the other hand, under the same root content, smaller diameters imply a greater number of roots involved in load-bearing, distributed more densely within the soil (Figure 19). This forms a tighter root network, further improving the soil’s strength.

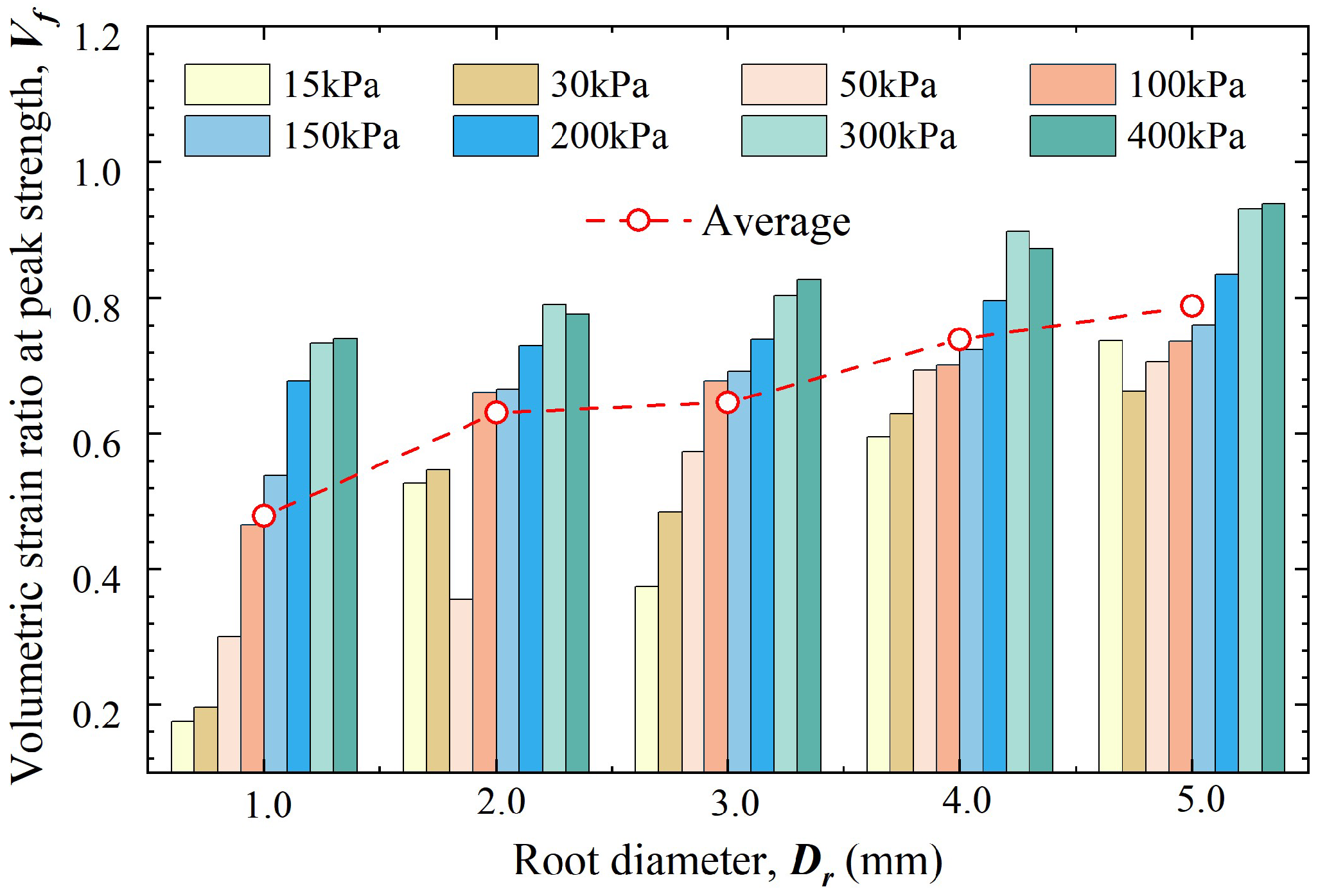
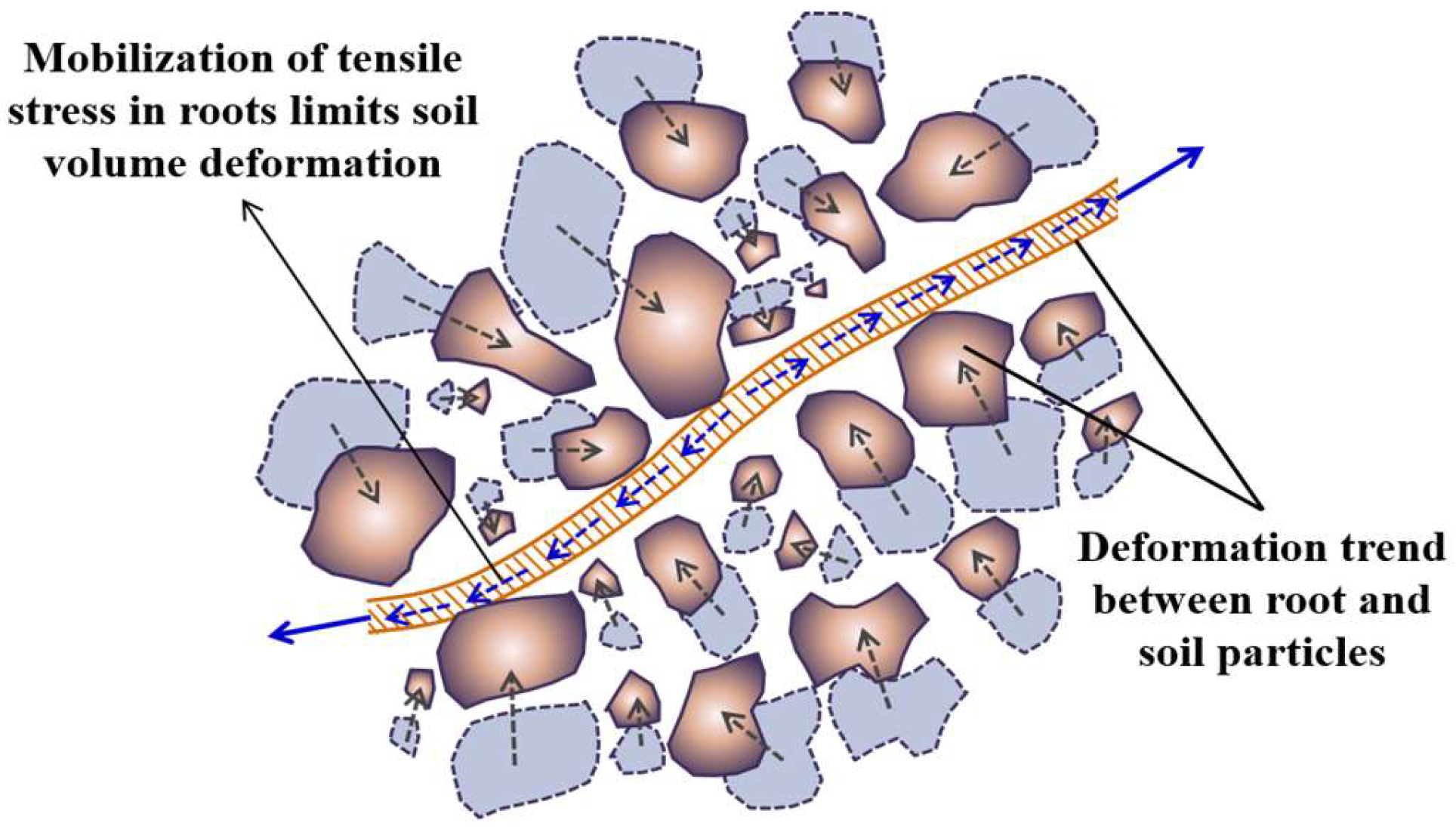
4.3. The Influence of Root Diameter on the Volumetric Deformation of Sand

The addition of roots restricted the shear contraction deformation of the soil, resulting in a volumetric strain ratio at peak strength of the root–soil composite of less than 1. This result was consistent with the findings of Foresta et al. (2020) [33], Jiang et al. (2022) [34], and Alam et al. (2022) [35]. The analysis suggested that due to the significant difference in mechanical properties between roots and soil, relative deformation tends to occur between the soil and roots under external loading. The root–soil interaction mobilizes the tensile properties of the roots to resist this relative deformation, thereby limiting the contraction deformation of the soil (Figure 20).

The elastic modulus and tensile strength of small-diameter roots is higher than that of large-diameter roots (Table 2). At the same root volume content, smaller root diameters result in a greater number of roots and a more compact fiber network in the soil. Therefore, smaller root diameters allow the roots to mobilize greater tensile stress in the soil, leading to a stronger restriction on soil deformation. This results in a decrease in the volumetric strain ratio and dilatancy angle corresponding to the peak strength as the root diameter decreases.

5. Conclusions

This study conducted triaxial tests to investigate how varying root diameters (1, 2, 3, 4, 5 mm) impact the mechanical behavior of saturated sand under different confining pressures (15, 30, 50, 100, 150, 200, 300, 400 kPa) and drainage conditions (CD, CU). The specific conclusions are as follows:

(1)

Root addition significantly increased the strength of sand, with an average increase of 31.8% observed for roots with diameters ranging from 1 mm to 5 mm. Smaller root diameters had a more pronounced strengthening effect on sand, as the increase in strength increased from 13.2% to 78.1% as the root diameter decreased from 5 mm to 1 mm;

(2)

Roots provide significant additional cohesion to the sand and increase its internal friction angle, thereby raising and reversing the failure envelope of the sand. The cohesion and internal friction angle of the root–soil composite decrease as the root diameter increases. As the root diameter increased from 1 mm to 5 mm, both the additional cohesion provided by the roots and the internal friction angle decreased by 76.8% and 12.1%, respectively;

(3)

The addition of roots significantly reduced the volumetric deformation of sand, with the average volumetric strain of the root–soil composites being only 0.66 times that of bare soil. A positive correlation was observed between root diameter and the volumetric strain of the root–soil composites. As the root diameter increased from 1 mm to 5 mm, the volumetric strain ratio increased from 0.48 to 0.79. This suggests that smaller root diameters more effectively limit volumetric deformation, thereby significantly enhancing the sand’s resistance to deformation;

(4)

Smaller root diameters more effectively enhance the mechanical properties of sand. They form a denser network, enabling more roots to resist external loads, which strengthens the composite and improves its deformation resistance. Additionally, smaller roots have a higher elastic modulus, resulting in greater tensile stress. Their higher tensile strength and sliding resistance make them less prone to failure, further enhancing the composite’s overall strength and resistance to deformation. Therefore, selecting plants with smaller and denser roots is an effective strategy for vegetative slope protection.