Individual Tree Diameter and Height Growth Models for 30 Tree Species in Mixed-Species and Uneven-Aged Forests of Mexico
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area and Data
2.2. Procedures for Developing the Species-Specific Individual-Tree Growth Equations
2.3. Model Comparison
3. Results
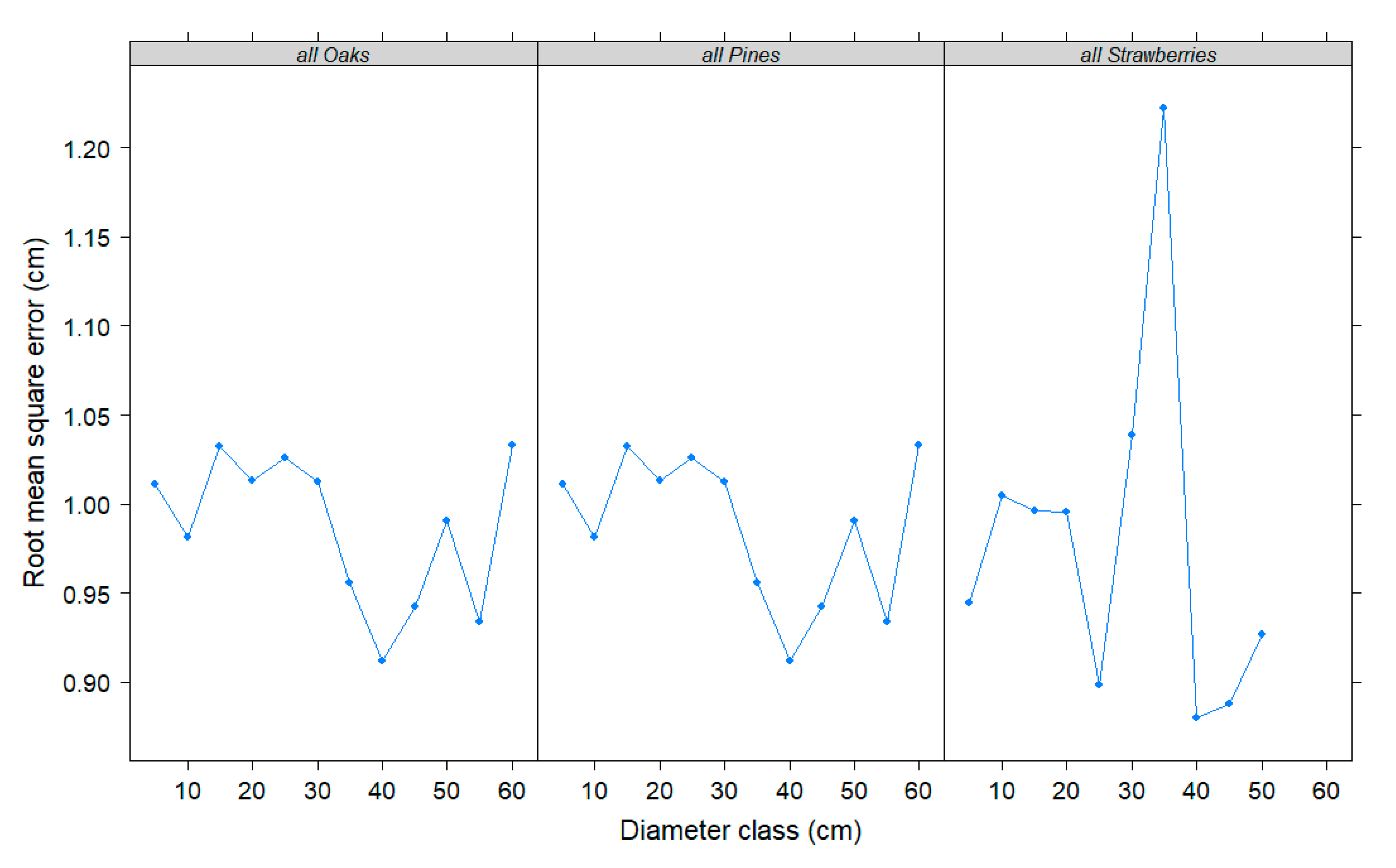
3.1. Diameter Growth Equations
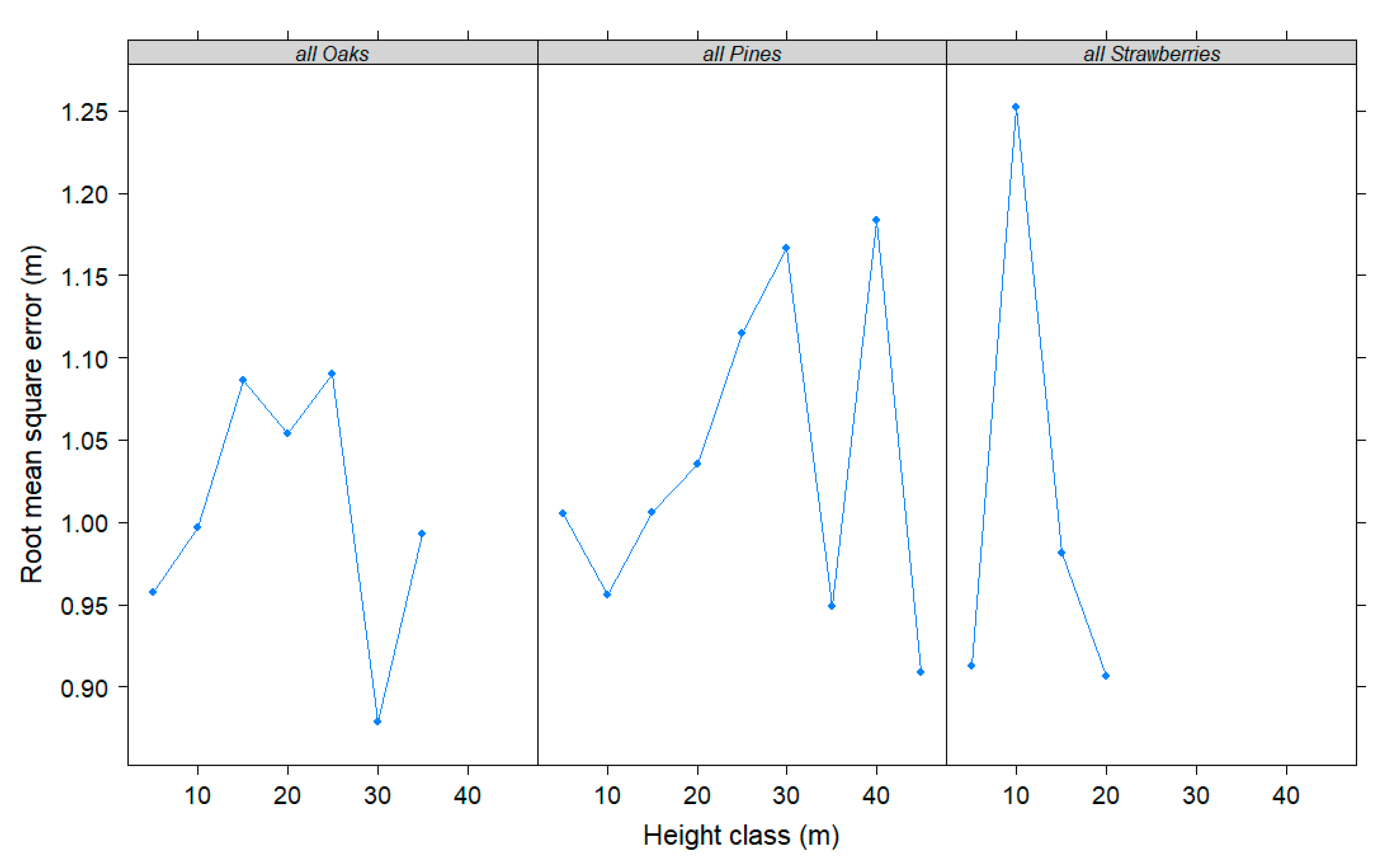
3.2. Height Growth Equations
4. Discussion
5. Conclusions
Author Contributions
Acknowledgments
Funding
Conflicts of Interest
References
- Tenzin, J.; Tenzin, K.; Hasenauer, H. Individual tree basal area increment models for broadleaved forests in Bhutan. For. Int. J. For. Res. 2017, 90, 367–380. [Google Scholar] [CrossRef]
- Perin, J.; Claessens, H.; Lejeune, P.; Brostaux, Y.; Hébert, J. Distance independent tree basal area growth models for Norway spruce Douglas-fir and Japanese larch in Southern Belgium. Eur. J. For. Res. 2016, 136, 193–204. [Google Scholar] [CrossRef]
- Quiñonez-Barraza, G.; Zhao, D.; Héctor, M.; Corral-Rivas, J.J. Considering neighborhood effects improves individual dbh growth models for natural mixed-species forests in Mexico. Ann. For. Sci. 2018, 75, 78. [Google Scholar] [CrossRef]
- Pretzsch, H. Forest Dynamics, Growth and Yield; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Lhotka, J.M.; Loewenstein, E.F. An individual-tree diameter growth model for managed uneven-aged oak-shortleaf pine stands in the Ozark Highlands of Missouri, USA. For. Ecol. Manag. 2011, 261, 770–778. [Google Scholar] [CrossRef]
- Andreassen, K.; Tomter, S.M. Basal area growth models for individual trees of Norway spruce, Scots pine, birch and other broadleaves in Norway. For. Ecol. Manag. 2003, 180, 11–24. [Google Scholar] [CrossRef]
- Burkhart, H.E.; Tomé, M. Growth and Yield Models for Uneven-Aged Stands. In Modeling Forest Trees and Stands, 1st ed.; Springer: New York, NY, USA, 2012; pp. 339–361. [Google Scholar]
- Kiviste, A.; González, J.G.Á.; Alboreca, A.R.; González, A.D.R. Funciones de Crecimiento de Aplicación en el ámbito Forestal, 1st ed.; Instituto Nacional de Investigación y Tecnología Agraria y Alimentaría (INIA): Madrid, España, 2002. [Google Scholar]
- Vanclay, J.K. Modelling Forest Growth and Yield: Applications to Mixed Tropical Forests, 1st ed.; CAB International: Wallingford, UK, 1994. [Google Scholar]
- Martin, G.L.; Ek, A.R. A comparison of competition measures and growth models for predicting plantation red pine diameter and height growth. For. Sci. 1984, 30, 731–743. [Google Scholar]
- Monserud, R.A.; Sterba, H. A basal area increment model for individual trees growing in even-and uneven-aged forest stands in Austria. For. Ecol. Manag. 1996, 80, 57–80. [Google Scholar] [CrossRef]
- Hökkä, H.; Groot, A. An individual-tree basal area growth model for black spruce in second-growth peatland stands. Can. J. For. Res. 1999, 29, 621–629. [Google Scholar] [CrossRef]
- Contreras, M.A.; Affleck, D.; Chung, W. Evaluating tree competition indices as predictors of basal area increment in western Montana forests. For. Ecol. Manag. 2011, 262, 1939–1949. [Google Scholar] [CrossRef]
- Russel, M.B.; Weiskittel, A.R. Comparing strategies for modeling individual-tree height and heigth-to-crown base increment in mixed-species Acadian forests of northeastern North America. Eur. J. For. Res. 2014, 133, 1121–1135. [Google Scholar] [CrossRef]
- Zhang, Z.; Papaik, M.J.; Wang, X.; Hao, Z.; Ye, J.; Lin, F. The effect of tree size, neighborhood competition and environment on tree growth in an old-growth temperate forest. J. Plant Ecol. 2017, 10, 970–980. [Google Scholar] [CrossRef]
- Canham, C.D.; Papaik, M.J.; Uriarte, M.; Mc Williams, W.H.; Jenkins, J.C.; Twery, M.J. Neighborhood analyses of canopy tree competition along environmental gradients in New England forests. Ecol. Appl. 2006, 16, 540–554. [Google Scholar] [CrossRef]
- Fien, E.K.P.; Fravera, S.; Teets, A.; Weiskittel, A.R.; Hollinger, D.Y. Drivers of individual tree growth and mortality in an uneven-aged, mixed-species conifer forest. For. Ecol. Manag. 2019, 449, 117446. [Google Scholar] [CrossRef]
- Aakala, T.; Fraver, S.; D’Amato, A.W.; Palik, B.J. Influence of competition and age on tree growth in structurally complex old-growth forests in northern Minnesota, USA. For. Ecol. Manag. 2013, 308, 128–135. [Google Scholar] [CrossRef]
- Franklin, J.F.; Spies, T.A.; Van Pelt, R.; Carey, A.B.; Thornburgh, D.A.; Berg, D.R.; Chen, J. Disturbances and structural development of natural forest ecosystems with silvicultural implications, using Douglas-fir forests as an example. For. Ecol. Manag. 2002, 155, 399–423. [Google Scholar] [CrossRef]
- Zhao, D.; Borders, B.; Wilson, M.; Rathbun, S.L. Modeling neighborhood effects on the growth and survival of individual trees in a natural temperate species-rich forest. Ecol. Model 2006, 196, 90–102. [Google Scholar] [CrossRef]
- Roberts, S.D.; Harrington, C.A. Individual tree growth response to variable-density thinning in coastal Pacific Northwest forests. For. Ecol. Manag. 2008, 255, 2771–2781. [Google Scholar] [CrossRef]
- Woodall, C.; Fiedler, C.; Milner, K. Intertree competition in uneven-aged ponderosa pine stands. Can. J. For. Res. 2003, 33, 1719–1726. [Google Scholar] [CrossRef]
- Soares, P.; Tomé, M. GLOBTREE: An individual tree growth model for Eucalyptus globulus in Portugal. In Modelling Forest Systems, 1st ed.; Amaro, A., Reed, D., Soares, P., Eds.; CAB International: Wallingford, UK, 2003; pp. 97–110. [Google Scholar]
- Larocque, G.R.; Luckai, N.; Adhikary, S.N.; Groot, A.; Bell, F.W.; Sharma, M. Competition theory-science and application in mixed forest stands: review of experimental and modelling methods and suggestions for future research. Environ. Rev. 2013, 21, 71–84. [Google Scholar] [CrossRef]
- Porté, A.; Bartelink, H.H. Modelling mixed forest growth: A review of models for forest management. Ecol. Model 2002, 150, 141–188. [Google Scholar] [CrossRef]
- González-Elizondo, M.S.; González-Elizondo, M.; Márquez-Linares, M.A. Vegetación y Ecorregiones de Durango, 1st ed.; Instituto Politécnico Nacional: México DF, México, 2007. [Google Scholar]
- Simental-Cano, B.; López-Sánchez, C.A.; Wehenkel, C.; Vargas-Larreta, B.; Álvarez-González, J.G.; Corral-Rivas, J.J. Species-specific and regional volume models for 12 forest species in Durango, Mexico. Rev. Chapingo Ser. Cie. 2017, 23, 155–171. [Google Scholar] [CrossRef]
- Corral-Rivas, S.; Álvarez-González, J.G.; Corral-Rivas, J.J.; López-Sánchez, C.A. Characterization of diameter structures of natural forests of northwest of Durango, Mexico. Rev. Chapingo Ser. Cie. 2015, 21, 221–236. [Google Scholar] [CrossRef]
- Corral-Rivas, S.; Álvarez-González, J.G.; Crecente-Campo, F.; Corral-Rivas, J.J. Local and generalized height-diameter models with random parameters for mixed, uneven-aged forests in Northwestern Durango, Mexico. For. Ecosyst. 2014, 1, 6. [Google Scholar] [CrossRef]
- Silva-Flores, R.; Pérez-Verdín, G.; Wehenkel, C. Patterns of Tree Species Diversity in Relation to Climatic Factors on the Sierra Madre Occidental, Mexico. PLoS ONE 2014, 9, e105034. [Google Scholar] [CrossRef]
- Corral-Rivas, J.J.; González, J.G.Á.; González, A.D.R.; Von Gadow, K. Compatible height and site index models for five pine species in El Salto, Durango (Mexico). For. Ecol. Manag. 2004, 201, 145–160. [Google Scholar] [CrossRef]
- Vargas-Larreta, B.; Corral-Rivas, J.; Aguirre-Calderón, Ó.; Nagel, J. Modelos de crecimiento de árbol individual: Aplicación del Simulador BWINPro7. Madera Bosques 2010, 16, 81–104. [Google Scholar] [CrossRef]
- Castillo López, A.; Vargas-Larreta, B.; Corral Rivas, J.J.; Nájera Luna, J.A.; Cruz Cobos, F.; Hernández, F.J. Modelo compatible altura-índice de sitio para cuatro especies de pino en Santiago Papasquiaro, Durango. Rev. Mex. Cienc. For. 2013, 4, 89–103. [Google Scholar]
- Valles-Gándara, A.G.; Valadez-Castro, R.C. Evaluación de índices de competencia independientes de la distancia para predecir el crecimiento de bosques mezclados en San Dimas, Durango. Rev. Chapingo Ser. Cie. 2006, 12, 39–44. [Google Scholar]
- Corral, J.R.; Álvarez, J.G.; Aguirre, O.; Hernandez, F. The effect of competition on individual tree basal area growth in mature stands of Pinus cooperi Blanco in Durango (Mexico). Eur. J. For. Res. 2005, 124, 133–142. [Google Scholar]
- Corral-Rivas, J.J.; Diéguez-Aranda, U.; Castedo, F.; Corral-Rivas, S. A merchantable volume system for major pine species in El Salto, Durango (Mexico). For. Ecol. Manag. 2007, 238, 118–129. [Google Scholar] [CrossRef]
- Vargas-Larreta, B.; Corral-Rivas, J.J.; Aguirre-Calderón, O.A.; López-Martínez, J.O.; Santos-Posadas, H.M.; Zamudio-Sánchez, F.J.; Aguirre-Calderón, C.G. SiBiFor: Forest Biometric System for forest management in Mexico. Rev. Chapingo Ser. Cie. 2017, 23, 437–455. [Google Scholar]
- Crecente-Campo, F.; Corral-Rivas, J.J.; Vargas-Larreta, B.; Wehenkel, C. Can random components explain differences in the height–diameter relationship in mixed uneven-aged stands? Ann. For. Sci. 2014, 71, 51–70. [Google Scholar] [CrossRef]
- Corral Rivas, J.J.; Larreta, V.; Calderón, A.; Alberto, O. Guía Para el Establecimiento de Sitios de Investigación Forestal y de Suelos en Bosques del Estado de Durango, 1st ed.; Editorial de la Universidad Juárez del Estado de Durango: Durango, México, 2009. [Google Scholar]
- Wykoff, W.R. A basal area increment model for individual conifers in the Northern Rocky Mountains. For. Sci. 1990, 36, 1077–1104. [Google Scholar]
- Wykoff, W.R.; Crookston, N.L.; Stage, A.R. User’s Guide to the Stand Prognosis Model, 1st ed.; US Department of Agriculture, Forest Service, Intermountain Forest and Range Experiment Station: Utah, UT, USA, 1982. [Google Scholar]
- Schröder, J.; Gadow, K.V. Testing a new competition index for Maritime pine in northwestern Spain. Can. J. For. Res. 1999, 29, 280–283. [Google Scholar] [CrossRef]
- Krajicek, J.E.; Brinkman, K.A.; Gingrich, S.F. Crown competition-a measure of density. For. Sci. 1961, 7, 35–42. [Google Scholar]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria; Available online: https://www.R-project.org/ (accessed on 20 June 2019).
- Myers, R.H. Classical and modern regression with applications, 1st ed.; Duxbury Press: Belmont, CA, USA, 1990; Volume 2. [Google Scholar]
- Crecente-Campo, F.; Soares, P.; Tomé, M.; Diéguez-Aranda, U. Modelling annual individual-tree growth and mortality of Scots pine with data obtained at irregular measurement intervals and containing missing observations. For. Ecol. Manag. 2010, 260, 1965–1974. [Google Scholar] [CrossRef]
- Hann, D.W. An adjustable predictor of crown profile for stand-grown Douglas-fir trees. For. Sci. 1999, 45, 217–225. [Google Scholar]
- Park, R.E. Estimation with heteroscedastic error terms. Econometrica (pre-1986) 1966, 34, 888. [Google Scholar] [CrossRef]
- Neter, J.; Wasserman, W.; Kutner, M.H. Applied Linear Models, Regression, Analysis of Variance and Experimental Designs, 3rd ed.; Burr Ridge Irwin: Boston, MA, USA, 1990. [Google Scholar]
- Huang, S.; Price, D.; Titus, S.J. Development of ecoregion-based height–diameter models for white spruce in boreal forests. For. Ecol. Manag. 2000, 129, 125–141. [Google Scholar] [CrossRef]
- Lujan-Soto, J.E.; Corral-Rivas, J.J.; Aguirre-Calderón, O.A.; Gadow, K.V. Grouping forest tree species on the Sierra Madre Occidental, Mexico. Allg. Forst. Jagdztg. 2015, 186, 63–71. [Google Scholar]
- Zhang, L.; Peng, C.; Dang, Q. Individual-tree basal area growth models for jack pine and black spruce in northern Ontario. For. Chron. 2004, 80, 366–374. [Google Scholar] [CrossRef]
- Baluarte, J.B.; Alvarez, J.G. Modelamiento del crecimiento del tornillo (Cedrelinga catenaeformis) en plantaciones en Jenarno Herrera, Departamento de Loreto, Perú. Folio Amazonica 2015, 24, 33–57. [Google Scholar]
- Sánchez-Gómez, D.; Zavala, M.A.; Van Schalkwijk, D.B.; Urbieta, I.R.; Valladares, F. Rank reversals in tree growth along tree size, competition and climatic gradients for four forest canopy dominant species in Central Spain. Ann. For. Sci. 2008, 65, 605. [Google Scholar] [CrossRef]
- Das, A. The effect of size and competition on tree growth rate in old-growth coniferous forests. Can. J. For. Res. 2012, 42, 1983–1995. [Google Scholar] [CrossRef]
- Russo, S.E.; Wiser, S.K.; Coomes, D.A. Growth-size scaling relationships of woody plant species differ from predictions of the Metabolic Ecology Model. Ecol. Lett. 2007, 10, 889–901. [Google Scholar] [CrossRef]
- Stephenson, N.L.; Das, A.J.; Condit, R.; Russo, S.E.; Baker, P.J.; Beckman, N.G.; Coomes, D.A.; Lines, E.R.; Morris, W.K.; Rüger, N.; et al. Rate of tree carbon accumulation increases continuously with tree size. Nature 2014, 507, 90–93. [Google Scholar] [CrossRef]
- Gómez-Aparicio, L.; Garcia-Valdes, R.; Ruiz-Benito, P.; Zavala, M.A. Disentangling the relative importance of climate, size, and competition on tree growth in Iberian forests: Implications for forest management under global change. Glob. Change Biol. 2011, 17, 2400–2414. [Google Scholar] [CrossRef]
- Holzwarth, F.; Kahl, A.; Bauhus, J.; Wirth, C. Many ways to die – partitioning tree mortality dynamics in a near-natural mixed deciduous forest. J. Ecol. 2013, 101, 220–230. [Google Scholar] [CrossRef]
- Fraver, S.; D’Amato, A.W.; Bradford, J.B.; Jonsson, B.G.; Jönsson, M.; Esseen, P.A. Tree growth and competition in an old-growth Picea abies forest of boreal Sweden: Influence of tree spatial patterning. J. Veg. Sci. 2014, 25, 374–385. [Google Scholar] [CrossRef]
- Bowman, D.M.J.S.; Brienen, R.J.W.; Gloor, E.; Phillips, O.L.; Prior, L.D. Detecting trends in tree growth: Not so simple. Trends Plant Sci. 2013, 18, 11–17. [Google Scholar] [CrossRef]
- Simard, S.W.; Zimonick, B.J. Neighborhood size effects on mortality, growth and crown morphology of paper birch. For. Ecol. Manag. 2005, 214, 251–265. [Google Scholar]
- King, D.A.; Davies, S.J.; Nur Supardi, M.N.; Tan, S. Tree growth is related to light interception and wood density in two mixed dipterocarp forests of Malaysia. Funct. Ecol. 2005, 19, 445–453. [Google Scholar]
- Metz, J.; Seidel, D.; Schall, P.; Scheffer, D.; Schulze, E.D.; Ammer, C. Crown modeling by terrestrial laser scanning as an approach to assess the effect of aboveground intra-and interspecific competition on tree growth. For. Ecol. Manag. 2013, 310, 275–288. [Google Scholar]
- Tomé, M.; Burkhart, H.E. Distance-dependent competition measures for predicting growth of individual trees. For. Sci. 1989, 35, 816–831. [Google Scholar]
- Biging, G.S.; Dobbertin, M. Evaluation of competition indices in individual tree growth models. For. Sci. 1995, 41, 360–377. [Google Scholar]
- Canham, C.D.; LePage, P.T.; Coates, K.D. A neighborhood analysis of canopy tree competition: Effects of shading versus crowding. Can. J. For. Res. 2004, 34, 778–787. [Google Scholar]
- Coates, K.D.; Canham, C.D.; LePage, P.T. Above- versus below-ground competitive effects and responses of a guild of temperate tree species. J. Ecol. 2009, 97, 118–130. [Google Scholar]
- Pretzsch, H.; Bielak, K.; Block, J.; Bruchwald, A.; Dieler, J.; Ehrhart, H.-P.; Kohnle, U.; Nagel, J.; Spellmann, H.; Zasada, M.; et al. Productivity of mixed versus pure stands of oak (Quercus petraea (Matt.) Liebl. and Quercus robur L.) and European beech (Fagus sylvatica L.) along an ecological gradient. Eur. J. For. Res. 2013, 132, 263–280. [Google Scholar]
- Keddy, P.A. Studying Competition. In Competition, 2nd ed.; Usher, M.B., DeAngelis, D.L., Eds.; Springer Science & Business Media: Louisiana, LA, USA, 2001; pp. 1–58. [Google Scholar]
- Adame, P.; Hynynen, J.; Canellas, I.; del Río, M. Individual-tree diameter growth model for rebollo oak (Quercus pyrenaica Willd.) coppices. For. Ecol. Manag. 2008, 255, 1011–1022. [Google Scholar]
- Pukkala, T.; Lahde, E.; Laiho, O. Growth and yield models for uneven-sized forest stands in Finland. For. Ecol. Manag. 2009, 258, 207–216. [Google Scholar]
- Álvarez-Taboada, M.F.; Barrio-Anta, M.; Gorgoso-Varela, J.; Alvarez-González, J.G. Influence of the competition on the section growth in Pinus radiata D. Don. Inv. Agrar.-Sist. Rec. F. 2003, 12, 25–35. [Google Scholar]
- Corral-Rivas, J.J.; González-Elizondo, M.S.; Lujan-Soto, J.E.; Gadow, K.V. Effects of density and structure on production in the communal forests of the Mexican Sierra Madre Occidental. South. For. J. For. Sci. 2019, 81, 1–10. [Google Scholar] [CrossRef]
- Vargas-Larreta, B.; Castedo-Dorado, F.; Álvarez-González, J.G.; Barrio-Anta, M.; Cruz-Cobos, F. A generalized height-diameter model with random coefficients for uneven-aged stands in El Salto, Durango (Mexico). Forestry 2009, 84, 445–462. [Google Scholar] [CrossRef]
- Abetz, P. Zur Standraumregulierung in Mischbeständen und Auswahl von Zukunftsbäumen. AFZ 1974, 29, 871–873. [Google Scholar]
- Monserud, R.A.; Rehfeldt, G.E. Genetic and environmental components of variation of site index in inland Douglas-fir. For. Sci. 1990, 36, 1–9. [Google Scholar]
- Hasenauer, H.; Burkhart, H.E.; Sterba, H. Variation in potential volume yield of loblolly pine plantations. For. Sci. 1994, 40, 162–176. [Google Scholar]
- Sánchez-Salguero, R.; Linares, J.C.; Camarero, J.J.; Madriga-Gonzalez, J.; Hevia, A.; Sanchez-Miranda, A.; Ballesteros-Canovas, J.A.; Alfaro-Sanchez, C.; Garcia-Cervignon, A.K.; Bigler, C.; et al. Disentangling the effects of competition and climate on individual tree growth: A retrospective and dynamic approach in Scots pine. For. Ecol. Manag. 2015, 358, 12–25. [Google Scholar] [CrossRef]
- Bigelow, S.W.; Runkle, J.R.; Oswald, E.M. Competition, Climate, and Size Effects on Radial Growth in an Old-Growth Hemlock Forest. Forests 2020, 11, 52. [Google Scholar] [CrossRef]

| Variable | 2007 | 2012 | ||||||
|---|---|---|---|---|---|---|---|---|
| Mean | Max | Min | SD | Mean | Max | Min | SD | |
| N | 623.78 | 2264.00 | 120.00 | 281.30 | 624.18 | 2152.00 | 144.00 | 275.91 |
| G | 21.16 | 53.63 | 3.15 | 8.20 | 23.31 | 58.52 | 3.92 | 8.98 |
| QMD | 21.32 | 34.10 | 12.40 | 4.28 | 22.34 | 36.00 | 13.40 | 4.44 |
| H0 | 16.82 | 29.50 | 5.20 | 4.80 | 18.18 | 31.60 | 5.80 | 5.19 |
| V | 188.55 | 604.21 | 12.04 | 106.19 | 221.04 | 709.20 | 16.57 | 124.28 |
| dbh | 17.98 | 72.52 | 6.75 | 9.96 | 19.19 | 74.58 | 7.16 | 10.26 |
| S | 8 | 15 | 1 | 2 | 8 | 16 | 1 | 2 |
| PAI | 6.50 | 21.66 | 0.24 | 4.40 | ||||
| Species | n | 2007 | 2012 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Mean | SD | Mean | SD | ||||||||||
| dbh1 | h1 | dbh1 | h1 | dbh2 | h2 | AAI-dbh | AAI-h | dbh2 | h2 | AAI-dbh | AAI-h | ||
| Abies durangensis | 134 | 25.16 | 16.16 | 15.05 | 7.90 | 26.45 | 17.74 | 1.29 | 1.58 | 15.25 | 8.20 | 0.94 | 1.65 |
| Alnus firmifolia | 425 | 15.05 | 9.27 | 8.32 | 4.01 | 16.49 | 10.86 | 1.44 | 1.59 | 8.63 | 4.40 | 1.01 | 1.50 |
| Arbutus arizonica | 592 | 14.84 | 5.57 | 8.09 | 2.16 | 15.92 | 6.32 | 1.08 | 0.75 | 8.34 | 2.38 | 0.81 | 0.87 |
| Arbutus bicolor | 698 | 14.91 | 5.69 | 8.24 | 2.16 | 16.15 | 6.61 | 1.23 | 0.93 | 8.69 | 2.57 | 0.92 | 1.05 |
| Arbutus madrensis | 846 | 15.60 | 5.73 | 8.14 | 2.09 | 16.82 | 6.62 | 1.22 | 0.89 | 8.56 | 2.50 | 0.92 | 1.06 |
| Arbutus tessellata | 387 | 15.60 | 5.64 | 7.96 | 2.17 | 16.76 | 6.51 | 1.16 | 0.87 | 8.18 | 2.56 | 0.89 | 1.24 |
| Arbutus xalapensis | 947 | 14.76 | 5.90 | 7.71 | 2.40 | 15.81 | 6.78 | 1.05 | 0.88 | 8.03 | 2.81 | 0.91 | 1.02 |
| Cupressus lusitánica | 315 | 23.78 | 13.16 | 19.67 | 7.95 | 25.09 | 14.35 | 1.32 | 1.19 | 20.43 | 8.47 | 1.23 | 1.19 |
| Juniperus deppeana | 2896 | 15.52 | 7.00 | 9.02 | 2.87 | 16.77 | 7.82 | 1.26 | 0.82 | 9.29 | 3.02 | 0.91 | 0.87 |
| Pinus arizonica | 7618 | 17.99 | 10.74 | 9.27 | 4.88 | 19.12 | 11.95 | 1.13 | 1.22 | 9.59 | 5.18 | 0.84 | 1.07 |
| Pinus cooperi | 2770 | 20.49 | 13.11 | 10.25 | 5.67 | 22.32 | 14.52 | 1.82 | 1.41 | 10.79 | 5.74 | 1.24 | 1.18 |
| Pinus durangensis | 11,760 | 18.92 | 13.23 | 10.80 | 5.61 | 20.33 | 14.68 | 1.41 | 1.45 | 11.25 | 5.94 | 1.06 | 1.32 |
| Pinus engelmannii | 751 | 23.24 | 14.56 | 11.77 | 6.17 | 24.65 | 15.66 | 1.41 | 1.10 | 11.99 | 6.26 | 1.14 | 1.19 |
| Pinus herrerae | 1766 | 25.01 | 16.62 | 14.09 | 6.62 | 26.54 | 18.43 | 1.53 | 1.81 | 14.49 | 6.85 | 1.23 | 1.65 |
| Pinus leiophylla | 2161 | 21.09 | 12.89 | 10.37 | 5.54 | 22.51 | 14.04 | 1.43 | 1.15 | 10.78 | 5.80 | 1.02 | 1.15 |
| Pinus lumholtzii | 1458 | 16.95 | 10.50 | 7.68 | 4.28 | 17.98 | 11.54 | 1.03 | 1.04 | 7.77 | 4.41 | 0.77 | 1.14 |
| Pinus strobiformis | 2550 | 17.74 | 11.54 | 9.33 | 4.72 | 19.29 | 12.91 | 1.54 | 1.37 | 9.66 | 4.92 | 0.99 | 1.17 |
| Pinus teocote | 3953 | 19.00 | 11.95 | 10.36 | 5.11 | 20.43 | 13.14 | 1.43 | 1.18 | 10.68 | 5.40 | 1.00 | 1.25 |
| Quercus arizonica | 179 | 19.15 | 9.47 | 7.79 | 3.94 | 20.07 | 10.91 | 0.92 | 1.44 | 7.95 | 4.37 | 0.76 | 1.58 |
| Quercus crassifolia | 2186 | 17.47 | 8.14 | 10.33 | 3.85 | 18.46 | 9.07 | 0.99 | 0.93 | 10.65 | 4.20 | 0.87 | 1.04 |
| Quercus durifolia | 255 | 18.50 | 7.75 | 11.02 | 3.70 | 19.70 | 8.44 | 1.21 | 0.69 | 11.18 | 3.84 | 0.96 | 0.91 |
| Quercus emoryi | 171 | 14.54 | 8.28 | 8.49 | 2.63 | 15.67 | 9.40 | 1.13 | 1.12 | 8.45 | 3.01 | 0.75 | 1.09 |
| Quercus fulva | 327 | 18.76 | 9.38 | 8.58 | 3.92 | 19.72 | 10.42 | 0.96 | 1.04 | 8.65 | 4.20 | 0.93 | 1.25 |
| Quercus jonesii | 222 | 16.19 | 6.76 | 8.52 | 3.74 | 17.24 | 7.62 | 1.04 | 0.86 | 8.82 | 3.98 | 0.76 | 0.84 |
| Quercus laeta | 389 | 17.67 | 9.19 | 9.64 | 3.88 | 18.57 | 10.28 | 0.90 | 1.10 | 9.82 | 4.28 | 0.77 | 1.31 |
| Quercus mcvaughii | 870 | 14.67 | 5.96 | 6.93 | 2.02 | 15.72 | 6.69 | 1.05 | 0.73 | 7.01 | 2.17 | 0.73 | 0.73 |
| Quercus radiata | 158 | 15.65 | 7.78 | 8.72 | 4.04 | 16.90 | 8.48 | 1.25 | 0.70 | 9.06 | 4.25 | 0.83 | 0.80 |
| Quercus rugosa | 896 | 18.60 | 8.78 | 11.29 | 3.56 | 19.97 | 9.58 | 1.37 | 0.80 | 11.75 | 3.82 | 1.05 | 0.95 |
| Quercus sideroxyla | 7373 | 19.30 | 9.50 | 12.26 | 4.33 | 20.60 | 10.56 | 1.30 | 1.05 | 12.65 | 4.65 | 1.04 | 1.20 |
| Quercus urbanii | 105 | 18.97 | 6.11 | 8.41 | 1.79 | 20.38 | 6.59 | 1.41 | 0.48 | 8.73 | 1.92 | 1.03 | 0.40 |
| All pines | 34,885 | 19.21 | 12.45 | 10.51 | 5.56 | 20.59 | 13.79 | 1.38 | 1.33 | 10.90 | 5.85 | 1.03 | 1.25 |
| All oaks | 13,228 | 18.36 | 8.82 | 11.27 | 4.12 | 19.56 | 9.80 | 1.20 | 0.98 | 11.62 | 4.44 | 0.98 | 1.13 |
| All strawberries | 3470 | 15.10 | 5.73 | 8.02 | 2.21 | 16.25 | 6.60 | 1.15 | 0.87 | 8.37 | 2.59 | 0.90 | 1.04 |
| Species | n | Estimated Parameters | RMSE (cm) | R2 | |||
|---|---|---|---|---|---|---|---|
| a0 | a1 | a2 | a3 | ||||
| Abies durangensis | 134 | 1.2745 | 0.9422 | ns | −0.0013 | 0.88 | 0.997 |
| Alnus firmifolia | 425 | 1.3368 | 0.9453 | ns | −0.002 | 0.97 | 0.987 |
| Arbutus arizonica | 592 | 1.2053 | 0.9589 | ns | ns | 0.79 | 0.991 |
| Arbutus bicolor | 698 | 1.2017 | 0.9619 | ns | −0.001 | 0.83 | 0.991 |
| Arbutus madrensis | 846 | 1.1737 | 0.9658 | 0.0013 | ns | 0.85 | 0.99 |
| Arbutus tessellata | 387 | 1.2073 | 0.9626 | ns | ns | 0.87 | 0.989 |
| Arbutus xalapensis | 947 | 1.1680 | 0.9721 | ns | ns | 0.87 | 0.988 |
| Cupressus lusitanica | 315 | 1.1516 | 0.9735 | ns | −0.0007 | 0.98 | 0.998 |
| Juniperus deppeana | 2896 | 1.2959 | 0.9421 | 0.0008 | −0.0017 | 0.87 | 0.991 |
| Pinus arizonica | 7618 | 1.1711 | 0.9618 | 0.0018 | −0.0007 | 0.78 | 0.993 |
| Pinus cooperi | 2770 | 1.3175 | 0.9251 | 0.0045 | −0.0027 | 1.11 | 0.99 |
| Pinus durangensis | 11,760 | 1.2015 | 0.9567 | 0.002 | −0.001 | 0.96 | 0.993 |
| Pinus engelmannii | 751 | 1.2143 | 0.9689 | −0.0026 | 0.001 | 1.12 | 0.991 |
| Pinus herrerae | 1766 | 1.1624 | 0.9729 | ns | 0.0005 | 1.16 | 0.994 |
| Pinus leiophylla | 2161 | 1.222 | 0.9509 | 0.0021 | −0.0014 | 0.95 | 0.992 |
| Pinus lumholtzii | 1458 | 1.2734 | 0.9320 | 0.0025 | −0.0019 | 0.77 | 0.99 |
| Pinus strobiformis | 2550 | 1.2577 | 0.9402 | 0.0027 | −0.0009 | 0.93 | 0.991 |
| Pinus teocote | 3953 | 1.2358 | 0.9431 | 0.0031 | −0.0012 | 0.94 | 0.992 |
| Quercus arizonica | 179 | 1.1084 | 0.9931 | −0.003 | 0.0014 | 0.75 | 0.991 |
| Quercus crassifolia | 2186 | 1.1858 | 0.9598 | 0.0012 | −0.001 | 0.83 | 0.994 |
| Quercus durifolia | 255 | 1.3001 | 0.9386 | ns | −0.0017 | 0.94 | 0.993 |
| Quercus emoryi | 171 | 1.5079 | 0.882 | 0.0048 | −0.0066 | 0.62 | 0.995 |
| Quercus fulva | 327 | 1.3912 | 0.9165 | 0.0013 | −0.0037 | 0.91 | 0.989 |
| Quercus jonesii | 222 | 1.1978 | 0.9526 | ns | −0.0026 | 0.71 | 0.994 |
| Quercus laeta | 389 | 1.2017 | 0.9593 | ns | −0.0011 | 0.75 | 0.994 |
| Quercus mcvaughii | 870 | 1.2704 | 0.9333 | 0.0023 | −0.0012 | 0.73 | 0.989 |
| Quercus radiata | 158 | 1.2433 | 0.9278 | 0.0069 | −0.0026 | 0.74 | 0.993 |
| Quercus rugosa | 896 | 1.2257 | 0.954 | 0.0015 | −0.0013 | 0.96 | 0.993 |
| Quercus sideroxyla | 7373 | 1.258 | 0.9438 | 0.0022 | −0.0018 | 0.97 | 0.994 |
| Quercus urbanii | 105 | 1.2524 | 0.9547 | ns | ns | 0.99 | 0.987 |
| All pines | 34,885 | 1.2085 | 0.9516 | 0.0025 | −0.0010 | 0.96 | 0.992 |
| All oaks | 13,228 | 1.2328 | 0.9495 | 0.0018 | −0.0015 | 0.93 | 0.994 |
| All strawberries | 3470 | 1.1875 | 0.9659 | ns | −0.0004 | 0.85 | 0.990 |
| Species | n | Estimated Parameters | RMSE (m) | R2 | |||
|---|---|---|---|---|---|---|---|
| b0 | b1 | b2 | b3 | ||||
| Abies durangensis | 134 | 1.3983 | 0.8883 | 0.0054 | ns | 1.64 | 0.960 |
| Alnus firmifolia | 425 | 1.6787 | 0.8847 | ns | −0.0019 | 1.48 | 0.887 |
| Arbutus arizonica | 592 | 1.2969 | 0.8920 | 0.0049 | ns | 0.87 | 0.867 |
| Arbutus bicolor | 698 | 1.2665 | 0.9044 | 0.0082 | −0.0022 | 1.03 | 0.840 |
| Arbutus madrensis | 846 | 1.2893 | 0.9273 | ns | ns | 1.05 | 0.824 |
| Arbutus tessellata | 387 | 1.5365 | 0.8387 | 0.0087 | −0.0090 | 1.22 | 0.773 |
| Arbutus xalapensis | 947 | 1.2214 | 0.9441 | 0.0041 | ns | 0.99 | 0.876 |
| Cupressus lusitanica | 315 | 1.2178 | 0.9759 | −0.0018 | 0.0002 | 1.10 | 0.983 |
| Juniperus deppeana | 2896 | 1.3426 | 0.8885 | 0.0047 | −0.0023 | 0.87 | 0.918 |
| Pinus arizonica | 7618 | 1.3660 | 0.8813 | 0.0072 | −0.0017 | 1.03 | 0.961 |
| Pinus cooperi | 2770 | 1.5429 | 0.8528 | 0.0049 | −0.0021 | 1.16 | 0.959 |
| Pinus durangensis | 11,760 | 1.3379 | 0.9096 | 0.0034 | −0.0006 | 1.31 | 0.952 |
| Pinus engelmannii | 751 | 1.3622 | 0.8881 | 0.0054 | −0.0018 | 1.19 | 0.964 |
| Pinus herrerae | 1766 | 1.6105 | 0.8488 | 0.0047 | −0.0022 | 1.64 | 0.943 |
| Pinus leiophylla | 2161 | 1.3041 | 0.9095 | 0.0042 | −0.0013 | 1.14 | 0.962 |
| Pinus lumholtzii | 1458 | 1.3825 | 0.8874 | 0.0041 | −0.0012 | 1.15 | 0.932 |
| Pinus strobiformis | 2550 | 1.3900 | 0.8924 | 0.0038 | −0.0009 | 1.16 | 0.944 |
| Pinus teocote | 3953 | 1.2745 | 0.9115 | 0.0051 | ns | 1.23 | 0.948 |
| Quercus arizonica | 179 | 1.4913 | 0.8536 | 0.0081 | −0.0045 | 1.56 | 0.872 |
| Quercus crassifolia | 2186 | 1.2162 | 0.9275 | 0.0044 | ns | 1.01 | 0.943 |
| Quercus durifolia | 255 | 1.2883 | 0.9023 | 0.0049 | −0.0030 | 0.90 | 0.945 |
| Quercus emoryi | 171 | 1.2861 | 0.9079 | 0.0078 | −0.0039 | 1.06 | 0.875 |
| Quercus fulva | 327 | 1.2640 | 0.8933 | 0.0074 | ns | 1.23 | 0.914 |
| Quercus jonesii | 222 | 1.2470 | 0.9241 | ns | ns | 0.83 | 0.956 |
| Quercus laeta | 389 | 1.2588 | 0.9217 | 0.0048 | ns | 1.29 | 0.909 |
| Quercus mcvaughii | 870 | 1.2981 | 0.8996 | 0.0035 | ns | 0.73 | 0.887 |
| Quercus radiata | 158 | 1.2473 | 0.9186 | ns | −0.0029 | 0.79 | 0.966 |
| Quercus rugosa | 896 | 1.2068 | 0.9290 | 0.0048 | −0.0015 | 0.93 | 0.940 |
| Quercus sideroxyla | 7373 | 1.2952 | 0.9087 | 0.0046 | −0.0014 | 1.18 | 0.935 |
| Quercus urbanii | 105 | 1.3727 | 1.0010 | −0.0190 | ns | 0.37 | 0.962 |
| All pines | 34,885 | 1.3512 | 0.8957 | 0.0050 | −0.0010 | 1.23 | 0.956 |
| All oaks | 13,228 | 1.2560 | 0.9156 | 0.0050 | −0.0013 | 1.10 | 0.938 |
| All strawberries | 3470 | 1.2800 | 0.9207 | 0.0043 | −0.0018 | 1.03 | 0.843 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Briseño-Reyes, J.; Corral-Rivas, J.J.; Solis-Moreno, R.; Padilla-Martínez, J.R.; Vega-Nieva, D.J.; López-Serrano, P.M.; Vargas-Larreta, B.; Diéguez-Aranda, U.; Quiñonez-Barraza, G.; López-Sánchez, C.A. Individual Tree Diameter and Height Growth Models for 30 Tree Species in Mixed-Species and Uneven-Aged Forests of Mexico. Forests 2020, 11, 429. https://doi.org/10.3390/f11040429
Briseño-Reyes J, Corral-Rivas JJ, Solis-Moreno R, Padilla-Martínez JR, Vega-Nieva DJ, López-Serrano PM, Vargas-Larreta B, Diéguez-Aranda U, Quiñonez-Barraza G, López-Sánchez CA. Individual Tree Diameter and Height Growth Models for 30 Tree Species in Mixed-Species and Uneven-Aged Forests of Mexico. Forests. 2020; 11(4):429. https://doi.org/10.3390/f11040429
Chicago/Turabian StyleBriseño-Reyes, Jaime, José Javier Corral-Rivas, Raúl Solis-Moreno, Jaime Roberto Padilla-Martínez, Daniel José Vega-Nieva, Pablito Marcelo López-Serrano, Benedicto Vargas-Larreta, Ulises Diéguez-Aranda, Gerónimo Quiñonez-Barraza, and Carlos Antonio López-Sánchez. 2020. "Individual Tree Diameter and Height Growth Models for 30 Tree Species in Mixed-Species and Uneven-Aged Forests of Mexico" Forests 11, no. 4: 429. https://doi.org/10.3390/f11040429
APA StyleBriseño-Reyes, J., Corral-Rivas, J. J., Solis-Moreno, R., Padilla-Martínez, J. R., Vega-Nieva, D. J., López-Serrano, P. M., Vargas-Larreta, B., Diéguez-Aranda, U., Quiñonez-Barraza, G., & López-Sánchez, C. A. (2020). Individual Tree Diameter and Height Growth Models for 30 Tree Species in Mixed-Species and Uneven-Aged Forests of Mexico. Forests, 11(4), 429. https://doi.org/10.3390/f11040429











