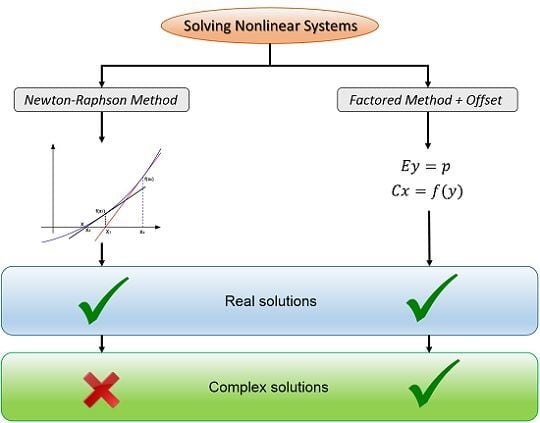
Offset-Assisted Factored Solution of Nonlinear Systems
Abstract
:1. Introduction
2. Review of the Factored Solution Method
- Step 0: Given an initial guess, , set .
- Step 1:
- 1.1. Compute λ by solving the linear system,
- 1.2. Obtain from,
- Step 2: Perform the one-to-one nonlinear transformation, , and obtain the (trivial) inverse of the diagonal Jacobian matrix, .
- Step 3:
- 3.1. Obtain by solving,
- 3.2. Update .
- 3.3. Convergence check: if , then stop; else, return to Step 1.
3. Limitations of the Factored Method When Real Negative or Complex Solutions Are Involved
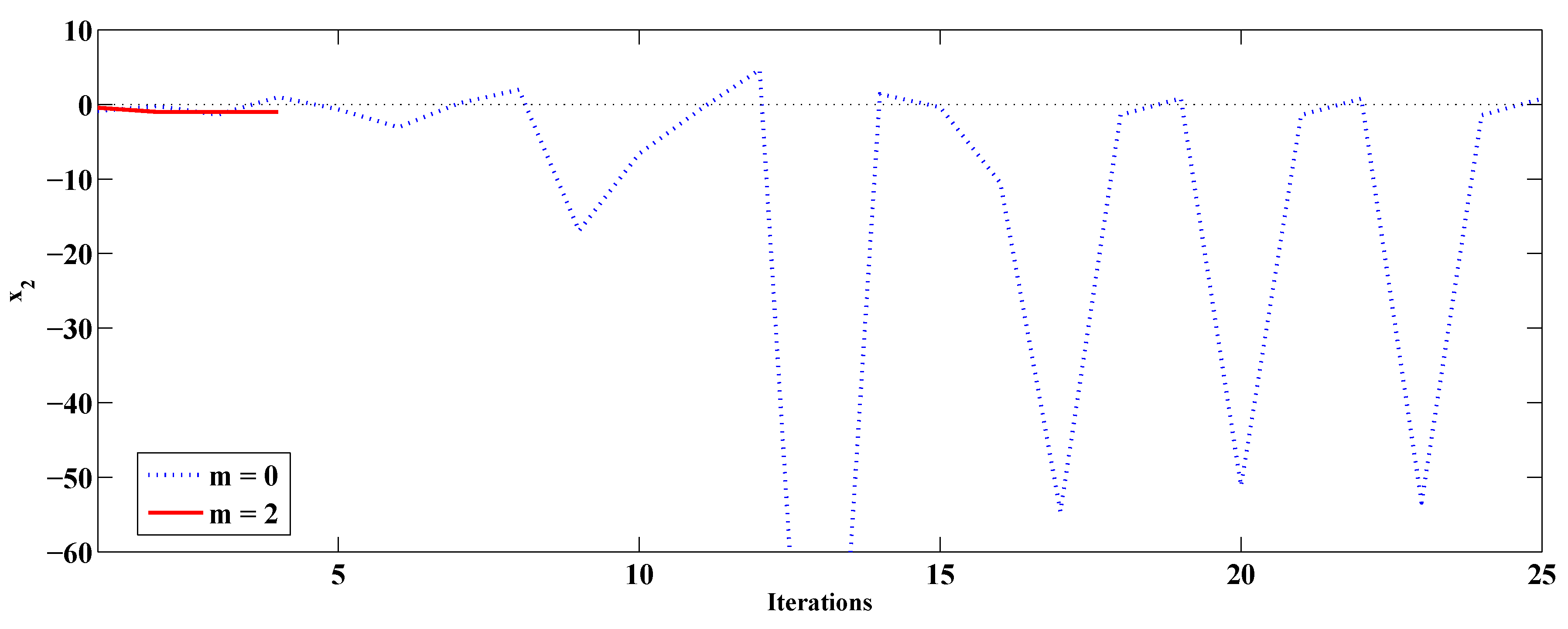
3.1. Negative Real Solutions
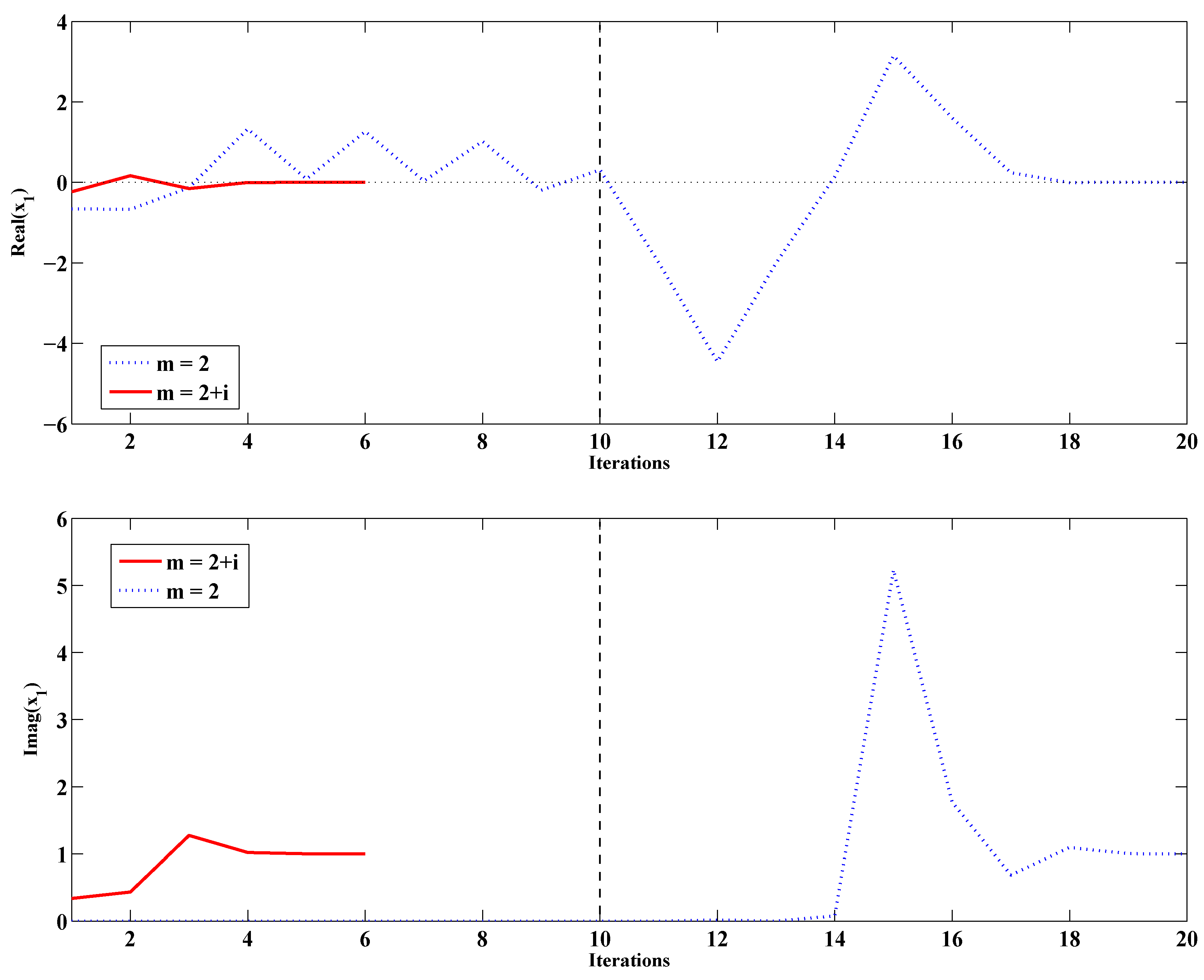
3.2. Complex Solutions
4. Generalized Offset-Assisted Factored Solution
5. Case Studies
5.1. Fourth-Order Polynomial System
| Color | ||
|---|---|---|
| 1 | ||
| 0.6344 | ||
| i | i | |
| - | - |

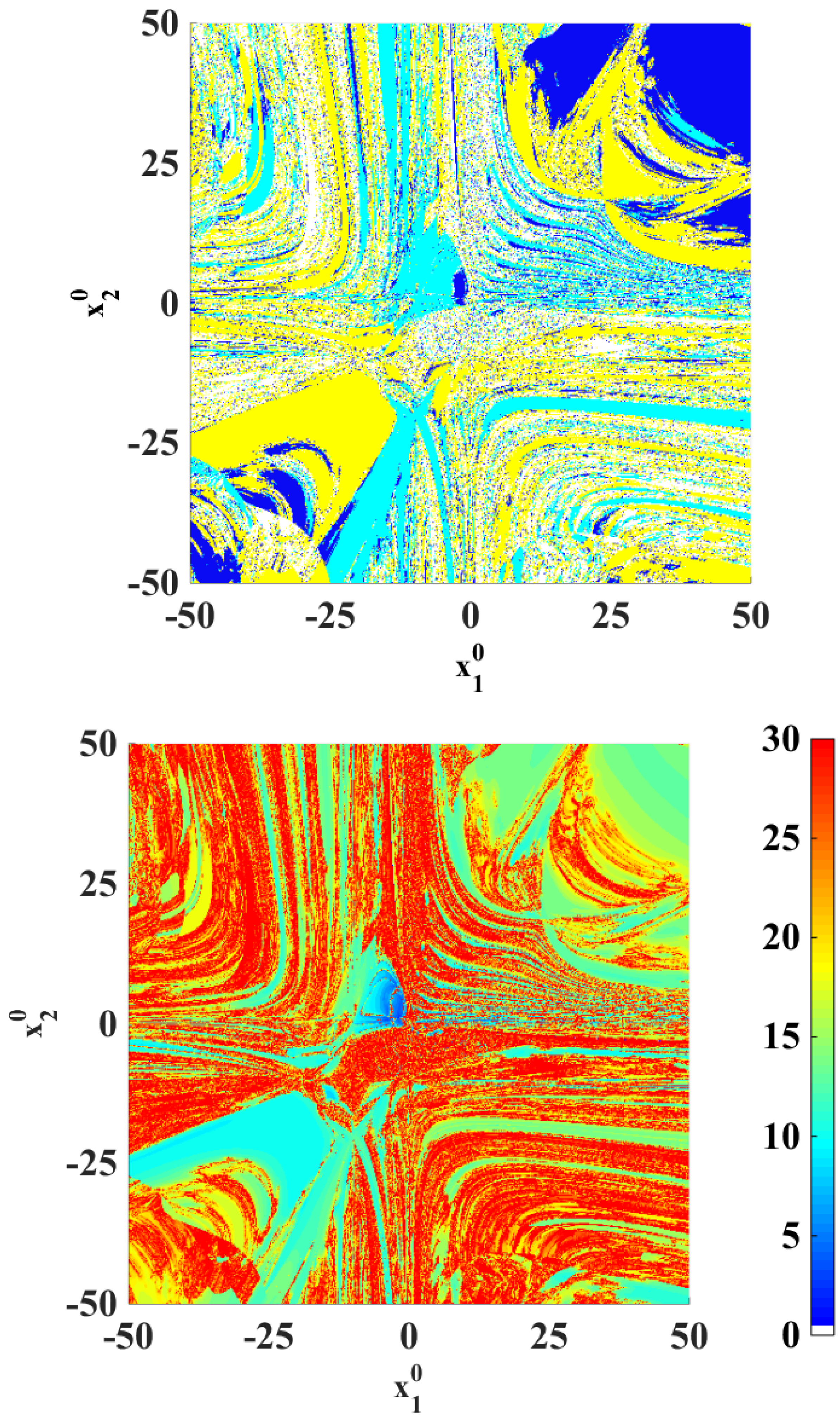
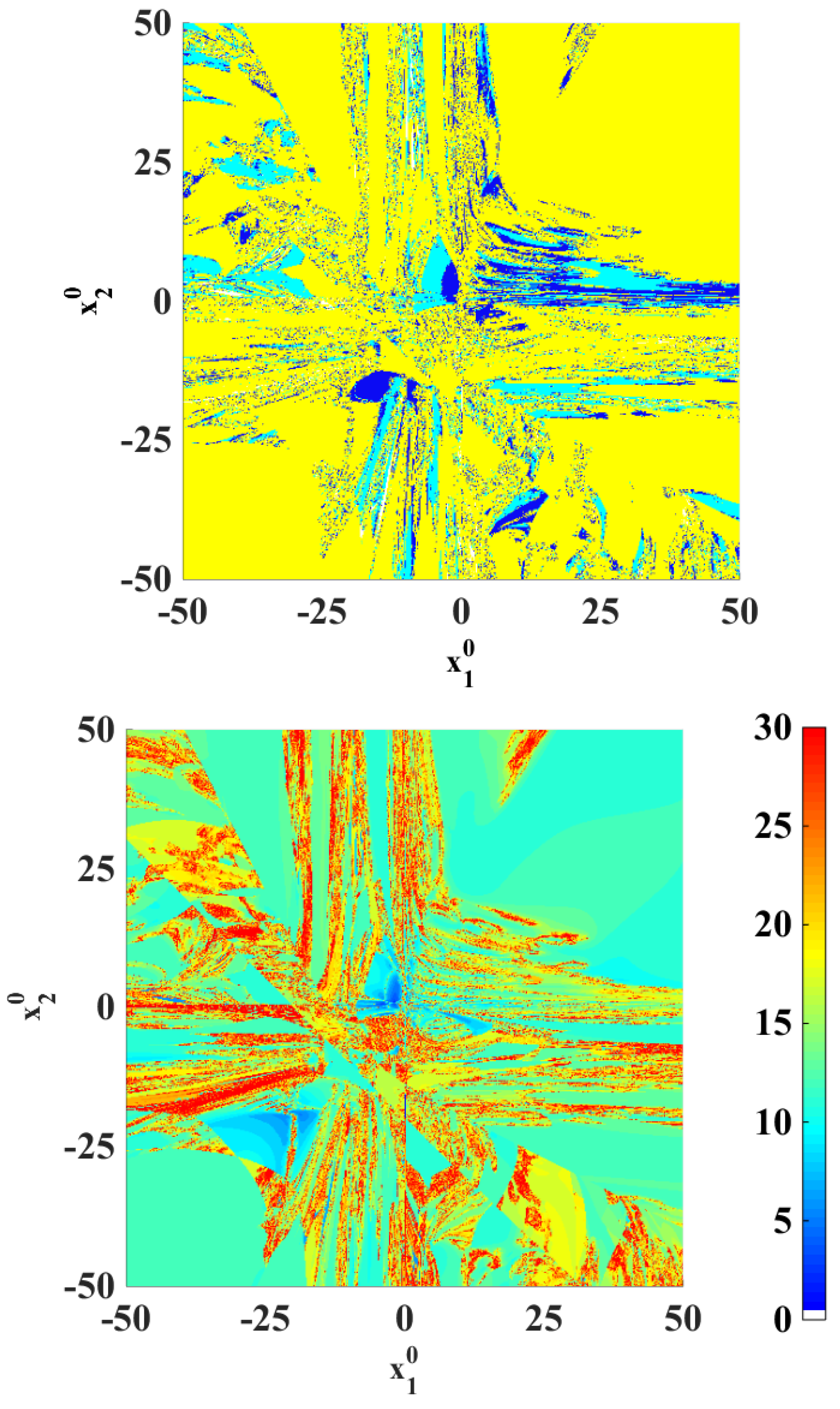
5.2. Kelley’s Synthetic System
| Color | ||
|---|---|---|
| 1 | ||
| 1 | 1 | |
| 1.3311 | ||
| 3.5129 | ||
| - | - |
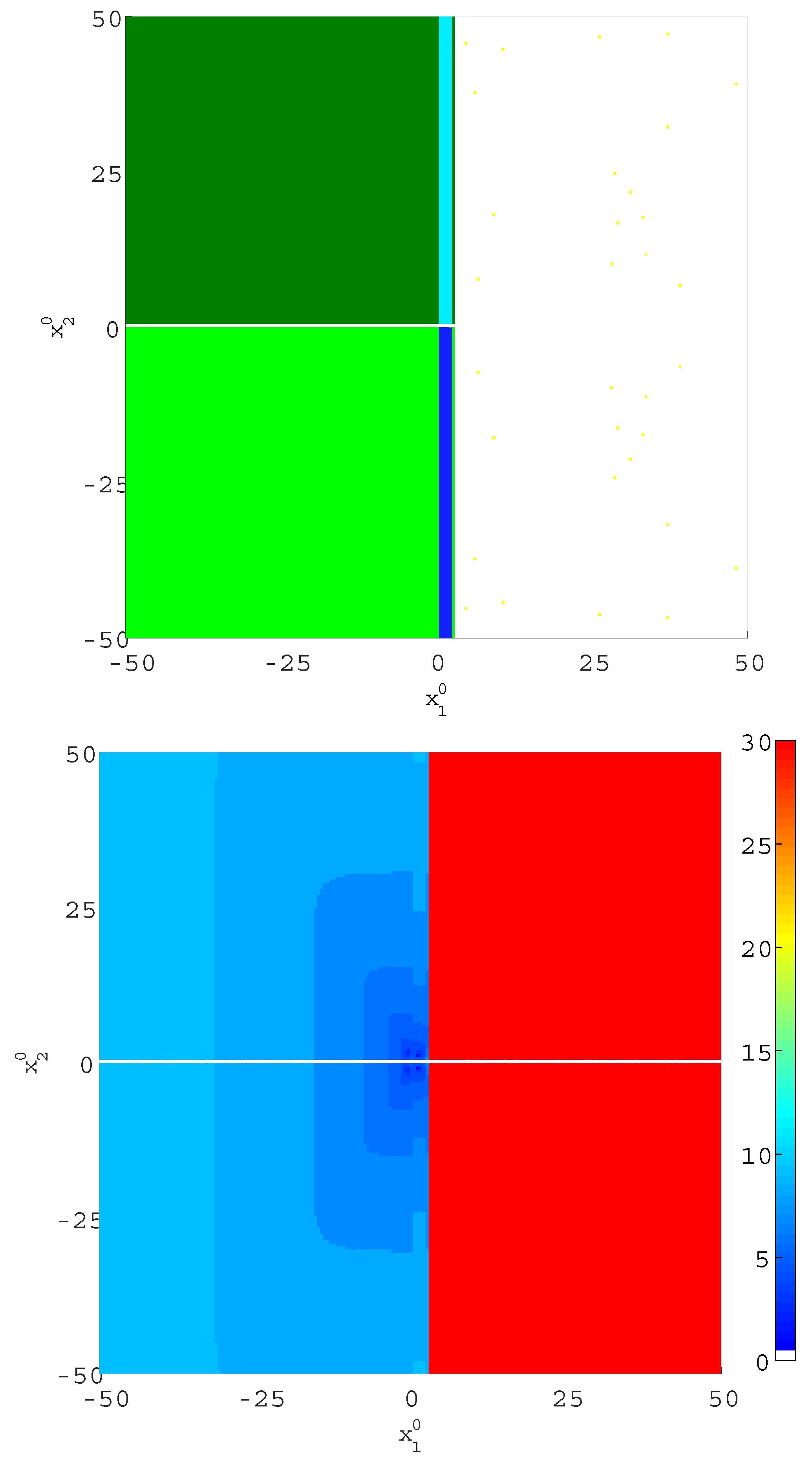
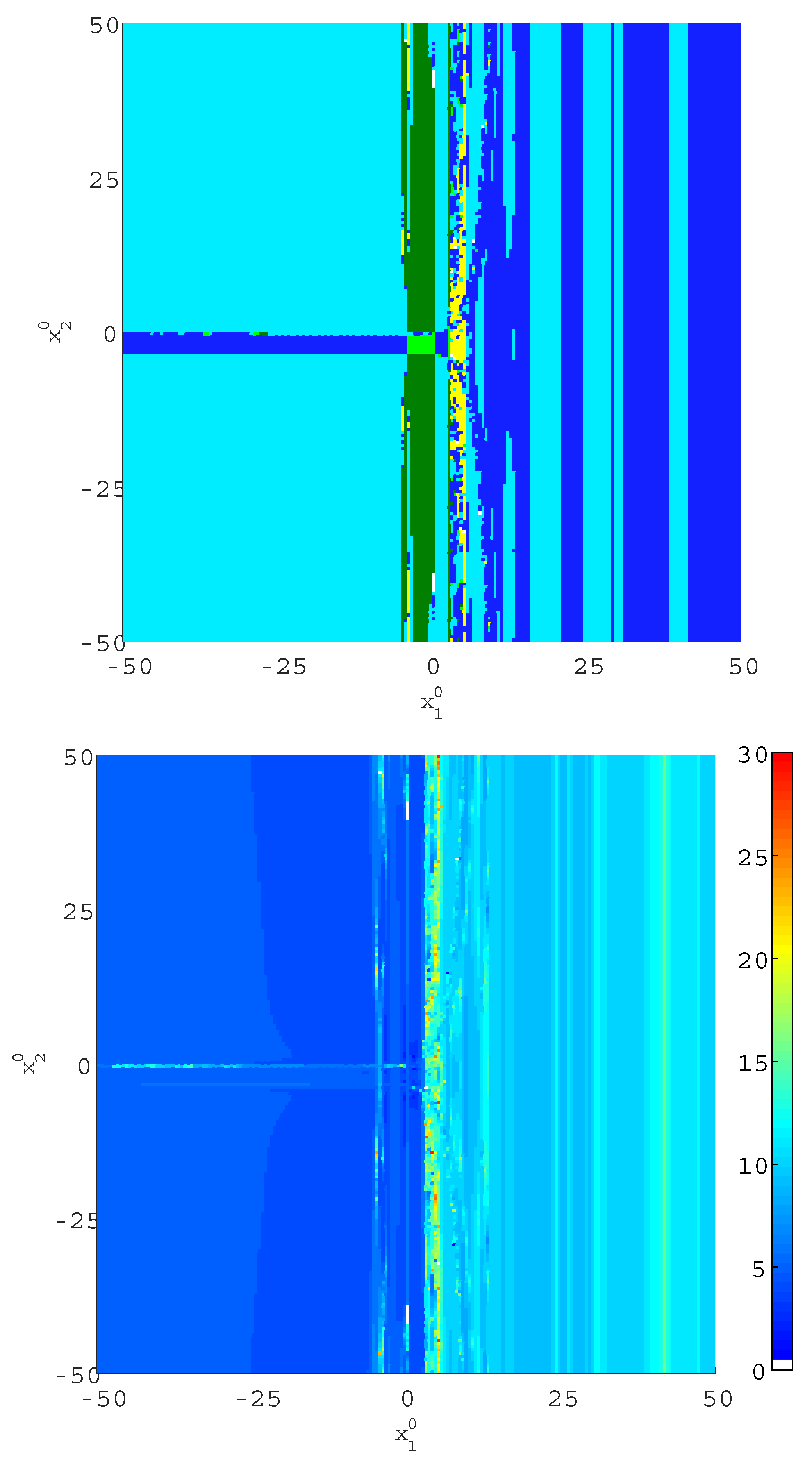
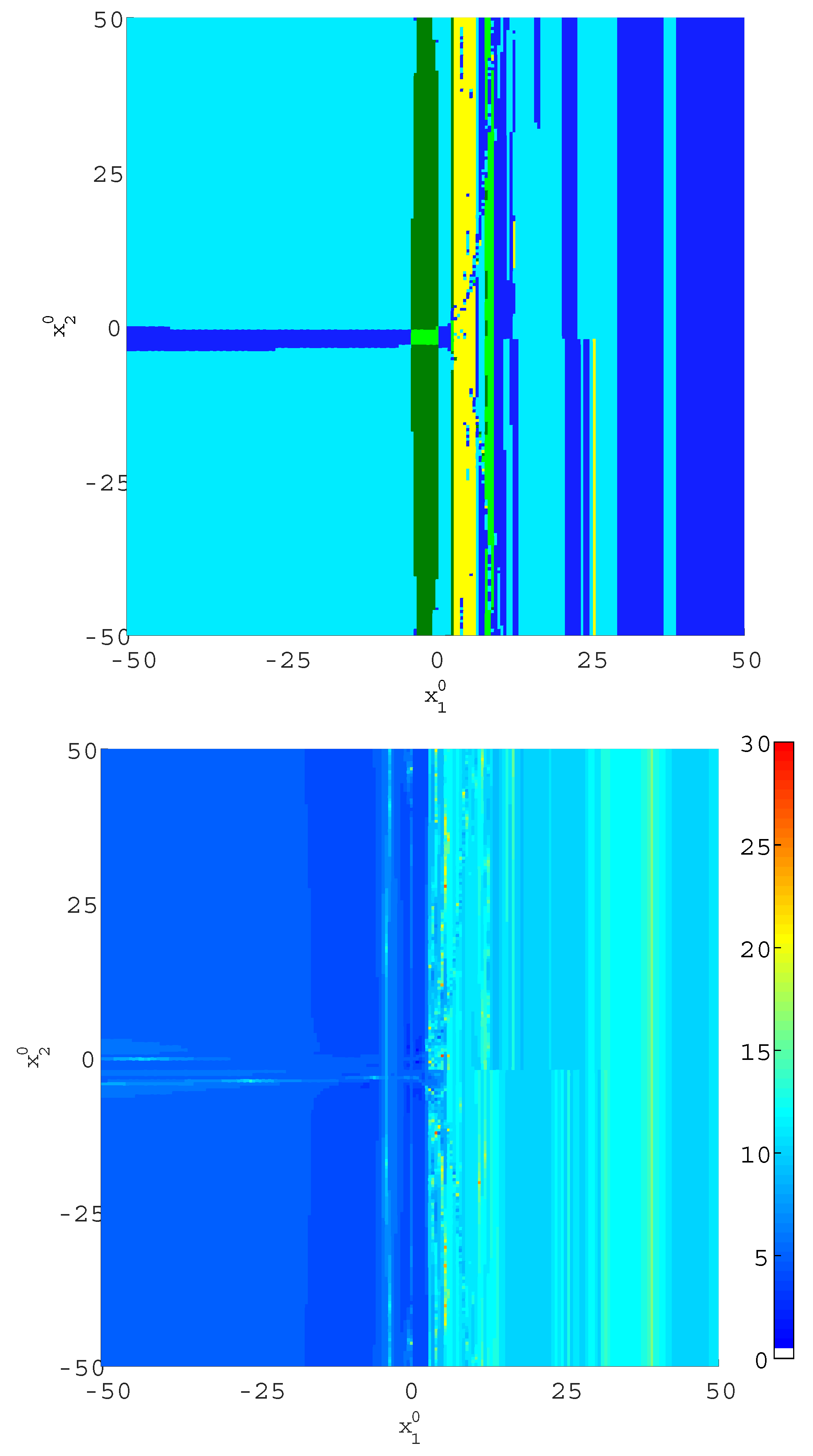
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kuo, M. Solution of nonlinear equations. IEEE Trans. Comput. 1968, 17, 897–898. [Google Scholar] [CrossRef]
- Rice, J. Numerical Methods, Software, and Analysis; Academic Press: New York, NY, USA, 1993. [Google Scholar]
- Ortega, J.M.; Rheinboldt, W.C. Iterative Solution of Nonlinear Equations in Several Variables; Academic Press: New York, NY, USA, 1970; (also published by SIAM, Philadelphia, 2000). [Google Scholar]
- Kelley, C.T. Iterative Methods for Linear and Nonlinear Equations; SIAM: Philadelphia, PA, USA, 1995. [Google Scholar]
- Deuflhard, P. Newton Methods for Nonlinear Problems; Springer-Verlag: Heidelberg, Germany, 2004. [Google Scholar]
- Kelley, C.T. Solving Nonlinear Equations with Newton’s Method, Fundamentals of Algorithms; SIAM: Philadelphia, PA, USA, 2003. [Google Scholar]
- Judd, K.L. Numerical Methods in Economics; MIT Press: Boston, MA, USA, 1998. [Google Scholar]
- Gómez-Expósito, A. Factored Solution of Nonlinear Equation Systems. Proc. R. Soc. A 2014. [Google Scholar] [CrossRef]
- Gómez-Expósito, A.; Gómez-Quiles, C. Factorized Load Flow. IEEE Trans. Power Syst. 2013, 28, 4607–4614. [Google Scholar] [CrossRef]
- Gómez-Expósito, A.; Gómez-Quiles, C.; Vargas, W. Factored Solution of Infeasible Load Flow Cases. In Proceedings of the Power Systems Computation Conference (PSCC), Wroclaw, Poland, 18–22 August 2014.
- Sandberg, I.W.; Willson, A.N. Existence and Uniqueness of Solutions for the Equations of Nonlinear DC Networks. SIAM J. Appl. Math. 1972, 22, 173–186. [Google Scholar] [CrossRef]
- Rheinboldt, W. Some Nonlinear Test Problems. Available online: http://folk.uib.no/ssu029/Pdf_file/Testproblems/testprobRheinboldt03.pdf (accessed on 22 December 2015).
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ruiz-Oltra, J.M.; Gómez-Quiles, C.; Gómez-Expósito, A. Offset-Assisted Factored Solution of Nonlinear Systems. Algorithms 2016, 9, 2. https://doi.org/10.3390/a9010002
Ruiz-Oltra JM, Gómez-Quiles C, Gómez-Expósito A. Offset-Assisted Factored Solution of Nonlinear Systems. Algorithms. 2016; 9(1):2. https://doi.org/10.3390/a9010002
Chicago/Turabian StyleRuiz-Oltra, José M., Catalina Gómez-Quiles, and Antonio Gómez-Expósito. 2016. "Offset-Assisted Factored Solution of Nonlinear Systems" Algorithms 9, no. 1: 2. https://doi.org/10.3390/a9010002
APA StyleRuiz-Oltra, J. M., Gómez-Quiles, C., & Gómez-Expósito, A. (2016). Offset-Assisted Factored Solution of Nonlinear Systems. Algorithms, 9(1), 2. https://doi.org/10.3390/a9010002