An Algorithm for Producing Fuzzy Negations via Conical Sections
Abstract
:1. Introduction
2. Preliminaries
Fuzzy Negation
- (1)
- (2)
- A fuzzy negationis called strong if the following property is met,
- (3)
- (1)
- is a continuous fuzzy negation,
- (2)
- is a strictly decreasing fuzzy negation,
- (3)
- is a continuous fuzzy negation if and only ifis a strictly decreasing fuzzy negation. In both cases.
- (1)
- is a strong negationisconjugate with classical negation,there exists, such that.
3. Main Results
Production of Fuzzy Negations via Conical Sections
4. Special Cases
4.1. Strong Negation via Circle
4.2. Strong Negation via Line
4.3. Strong Negation via Ellipse
4.4. Strong Negation of Hyperbola
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Baczyński, M.; Balasubramaniam, J. Fuzzy Implications; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Baczyński, M.; Beliakov, G.; Bustince, S.H.; Pradera, A. Advances in Fuzzy Implication Functions; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar] [CrossRef]
- Souliotis, G.; Papadopoulos, B. Fuzzy Implications Generating from Fuzzy Negations. ICANN 2018. In Proceedings of the 27th International Conference on Artificial Neural Networks, Rhodes, Greece, 4–7 October 2018. [Google Scholar] [CrossRef]
- Gottwald, S. A Treatise on Many-Valued Logics; Research Studies Press: Baldock, UK, 2001. [Google Scholar]
- Klir, G.J.; Yuan, B. Fuzzy Sets and Fuzzy Logic. Theory and Applications; Prentice Hall: Upper Saddle River, NJ, USA, 1995. [Google Scholar]
- Nguyen, H.T.; Walker, E.A. A First Course in Fuzzy Logic, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Fodor, J.C.; Roubens, M. Fuzzy Preference Modelling and Multicriteria Decision Support; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1994. [Google Scholar]
- Mayor, G. Sugeno’s negations and t-norms. Mathw. Soft Comput. 1994, 1, 93–98. [Google Scholar]
- Jabari, S.; Zhang, Y. Very High Resolution Satellite Image Classification Using Fuzzy Rule-Based Systems. Algorithms 2013, 6, 762–781. [Google Scholar] [CrossRef]
- Yang, E. Fixpointed Idepontent Uninorm (Based) Logics. Mathematics 2019, 7, 107. [Google Scholar] [CrossRef]
- Bogiatzis, A.; Papadopoulos, B. Thresholding Adaptive Neuro-Fuzzy Inference System Trained with Fuzzy Inclusion and Entropy Measures. Symmetry 2019, 11, 286. [Google Scholar] [CrossRef]

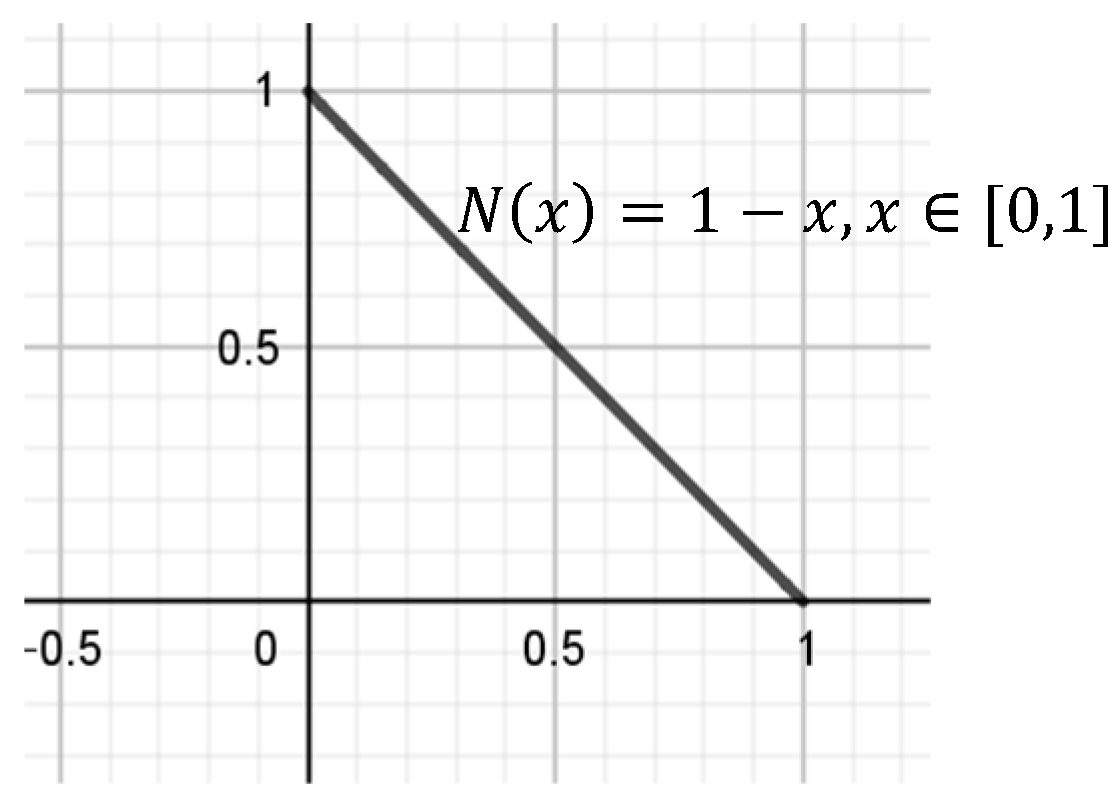
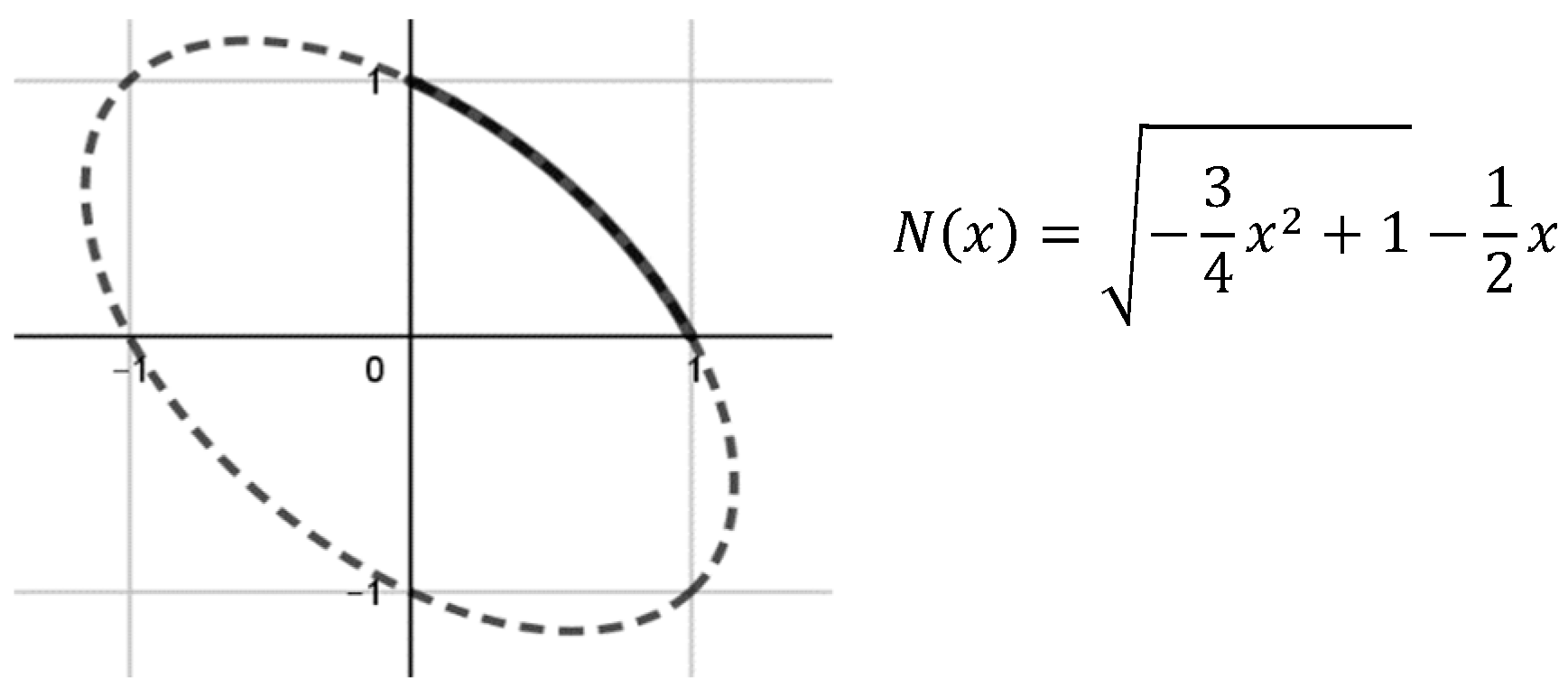

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Souliotis, G.; Papadopoulos, B. An Algorithm for Producing Fuzzy Negations via Conical Sections. Algorithms 2019, 12, 89. https://doi.org/10.3390/a12050089
Souliotis G, Papadopoulos B. An Algorithm for Producing Fuzzy Negations via Conical Sections. Algorithms. 2019; 12(5):89. https://doi.org/10.3390/a12050089
Chicago/Turabian StyleSouliotis, Georgios, and Basil Papadopoulos. 2019. "An Algorithm for Producing Fuzzy Negations via Conical Sections" Algorithms 12, no. 5: 89. https://doi.org/10.3390/a12050089
APA StyleSouliotis, G., & Papadopoulos, B. (2019). An Algorithm for Producing Fuzzy Negations via Conical Sections. Algorithms, 12(5), 89. https://doi.org/10.3390/a12050089





