Optimal Sliding Mode Control for an Active Suspension System Based on a Genetic Algorithm
Abstract
1. Introduction
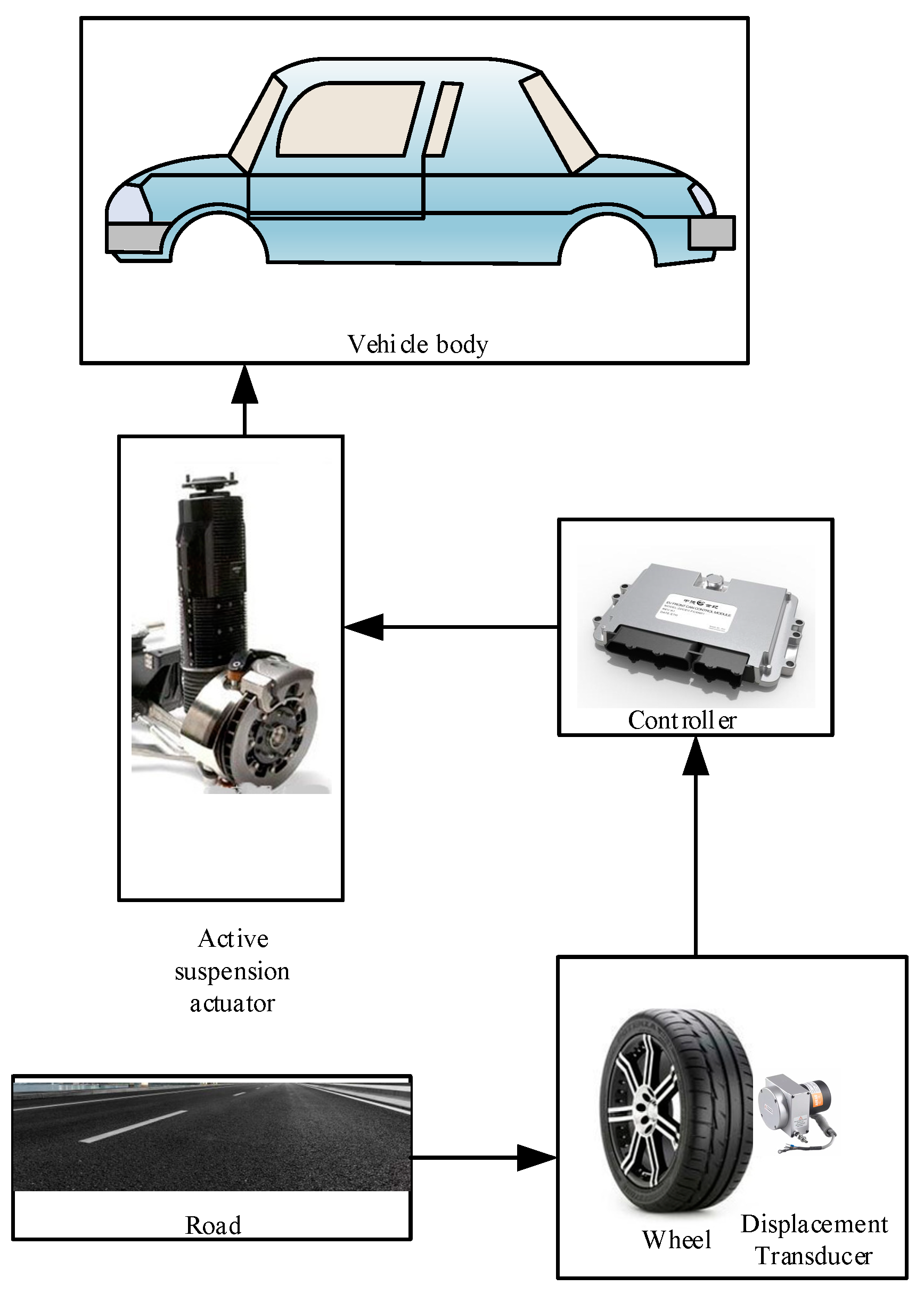
2. The Structure and Control Principle of the Active Suspension System
3. Modeling of the Active Suspension System
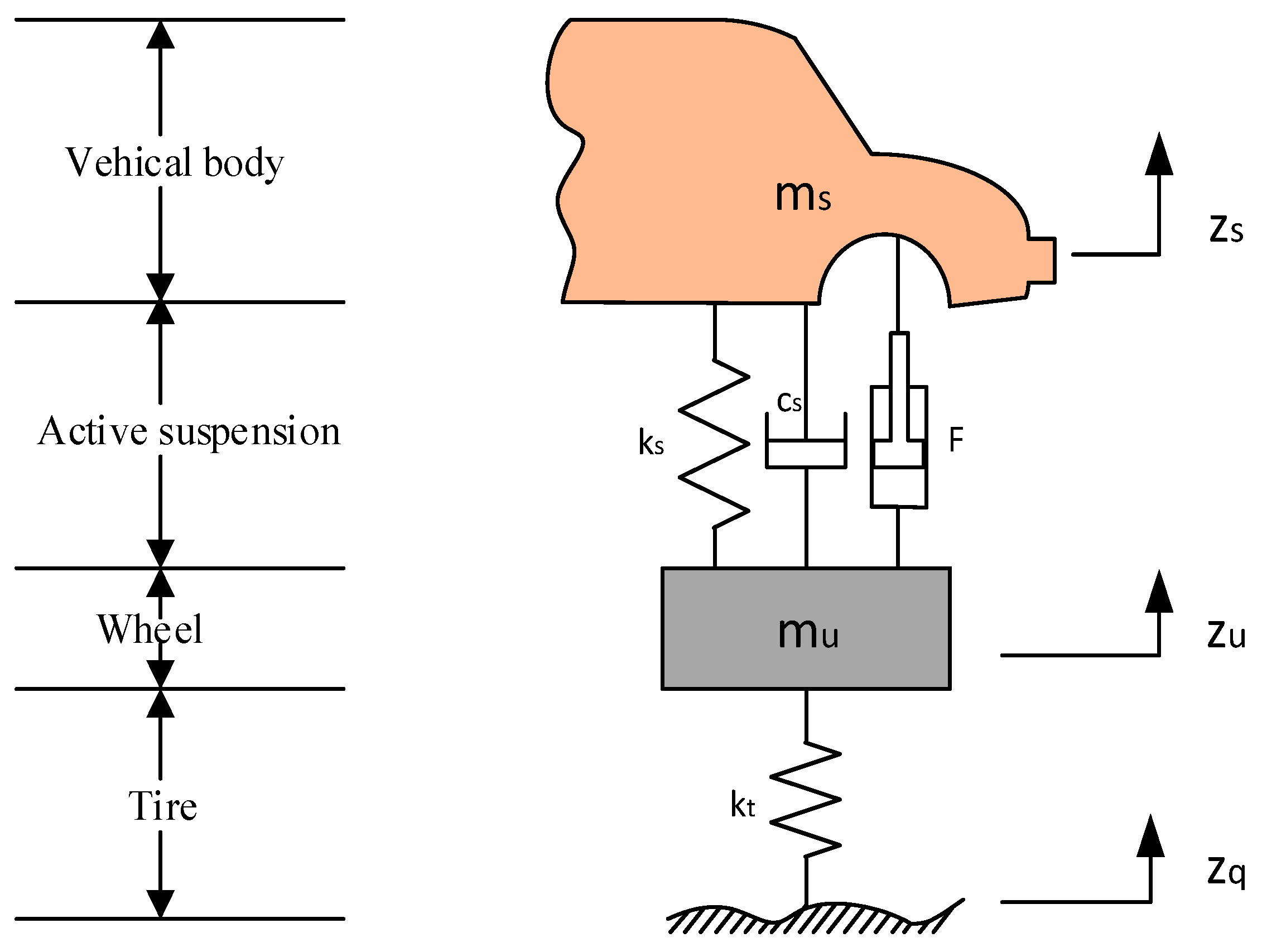
3.1. Dynamic Model of Quarter Car Active Suspension System
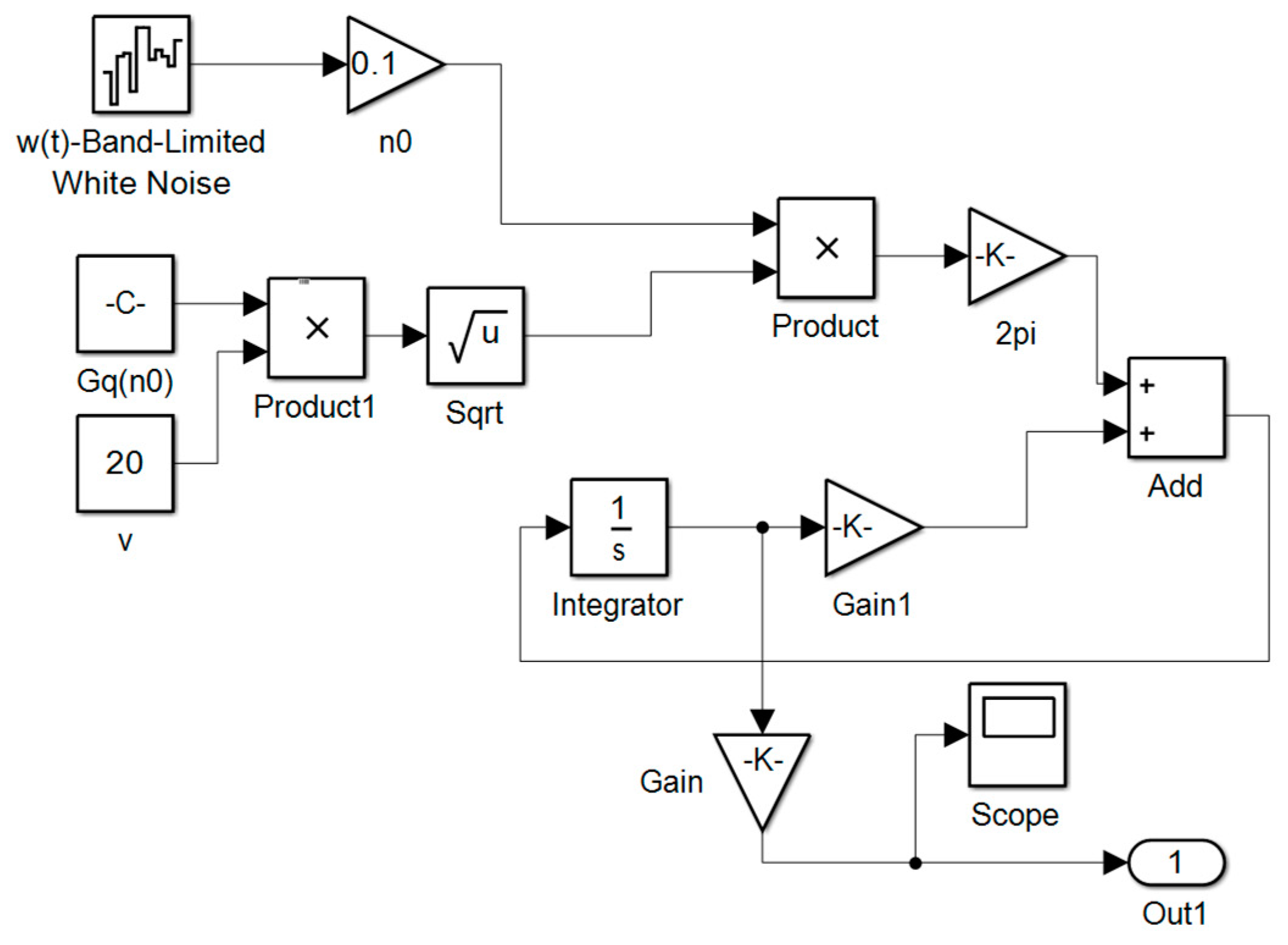
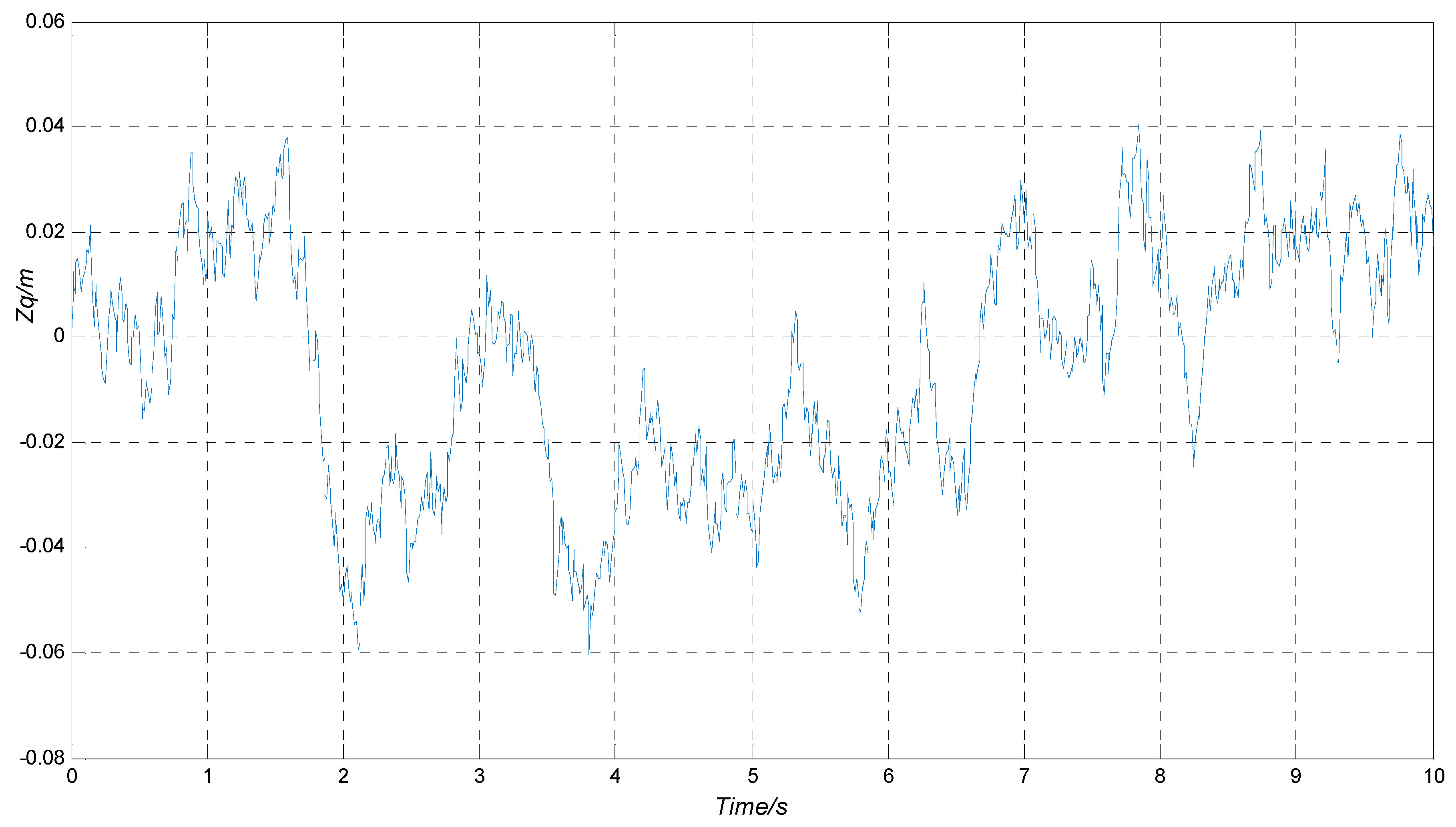
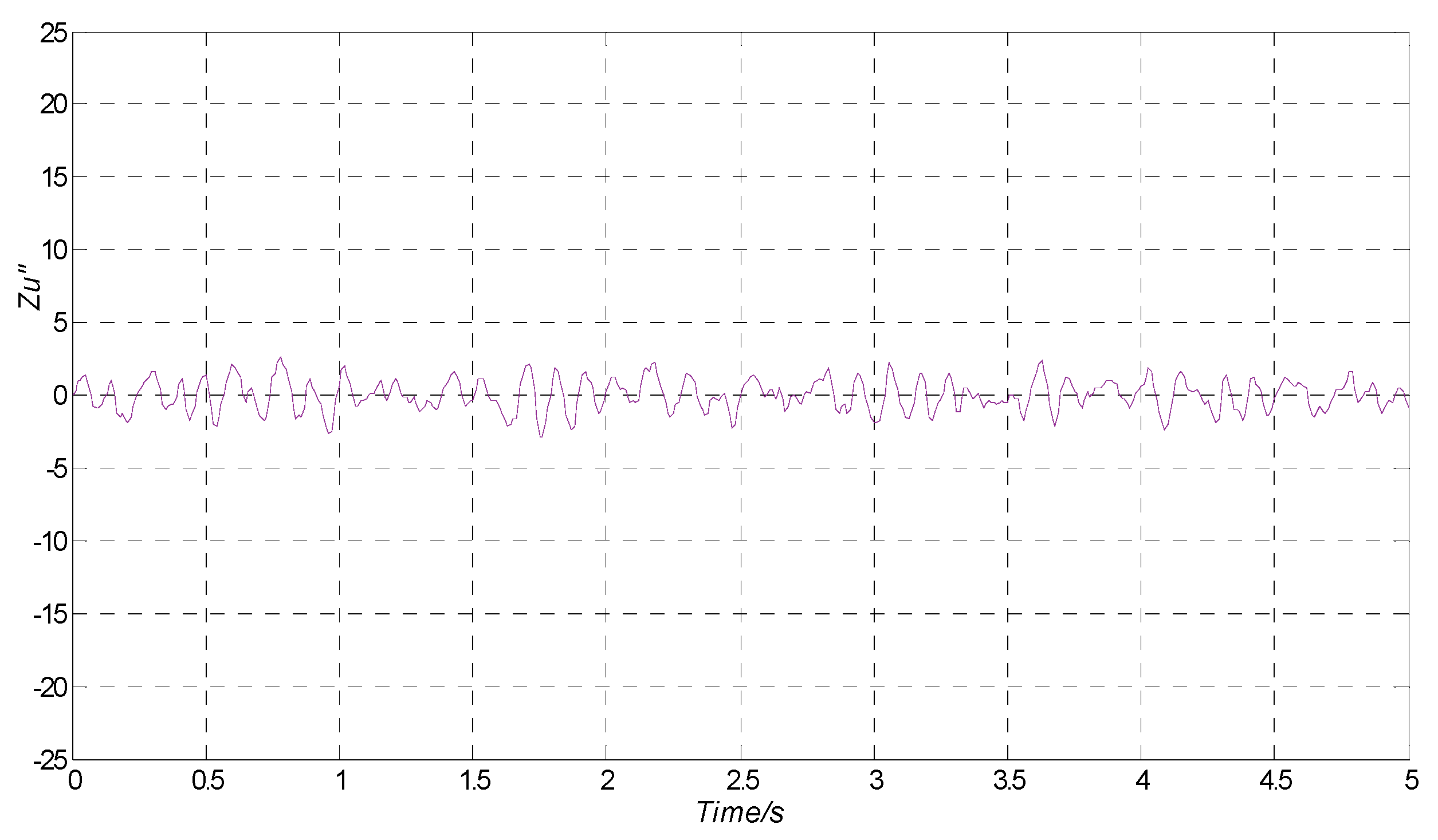
3.2. Random Road Inputs Model
3.3. Evaluation Index of the Suspension System
4. The OSMC Controller Design
4.1. Design of Sliding Surface
4.2. Establishment of the Control Law
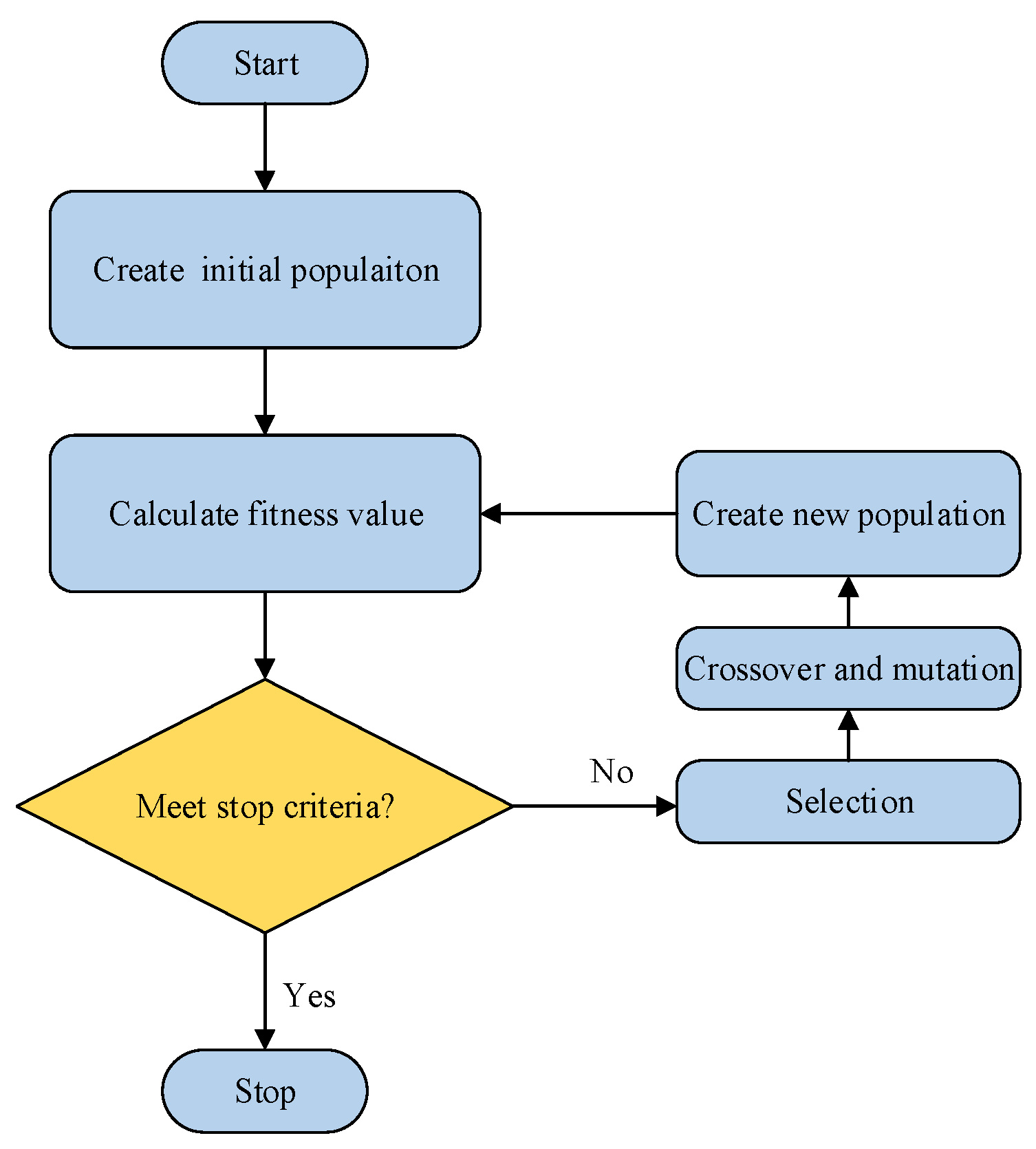
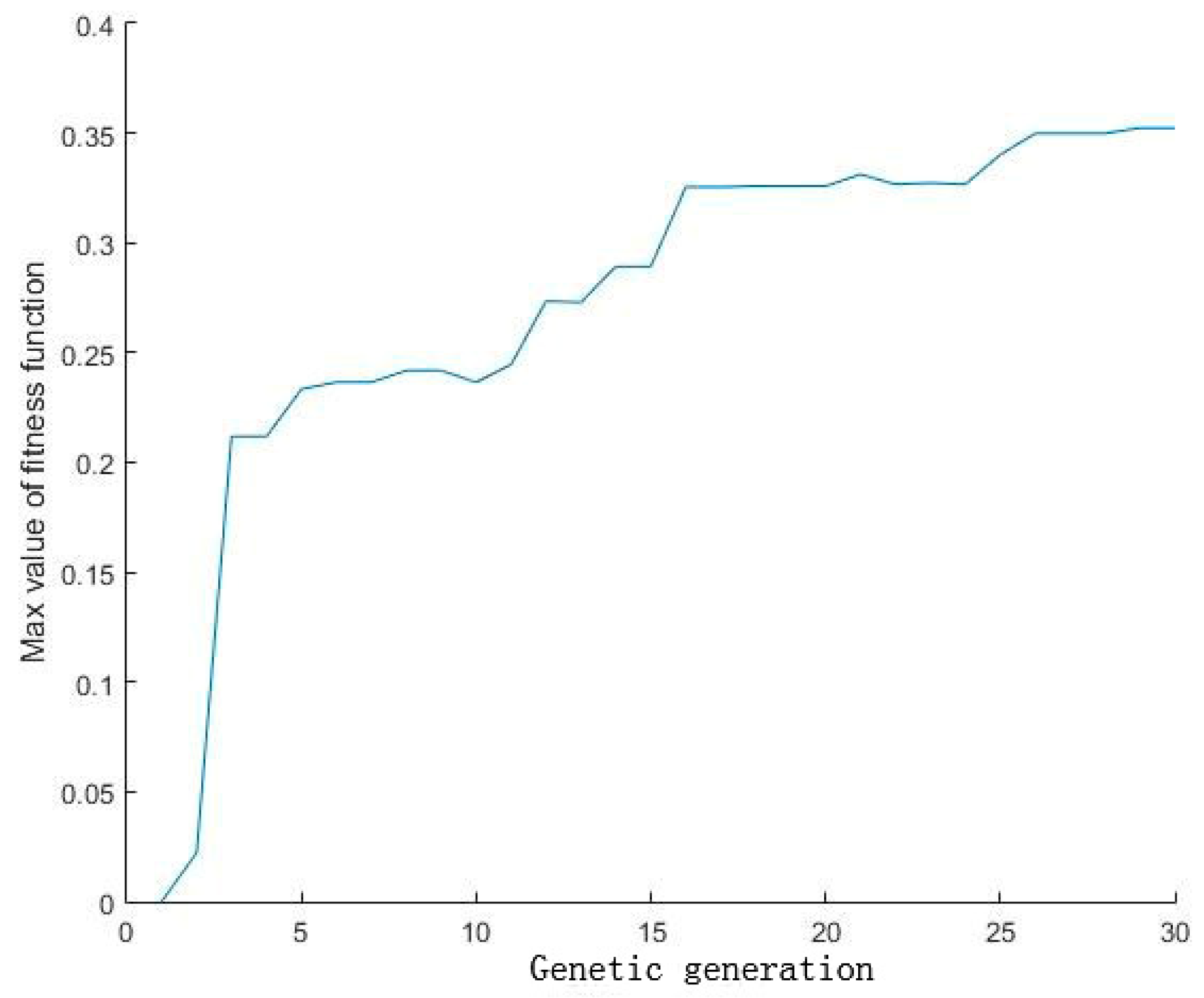
4.3. Optimal Tuning of the Switching Function Based on GA
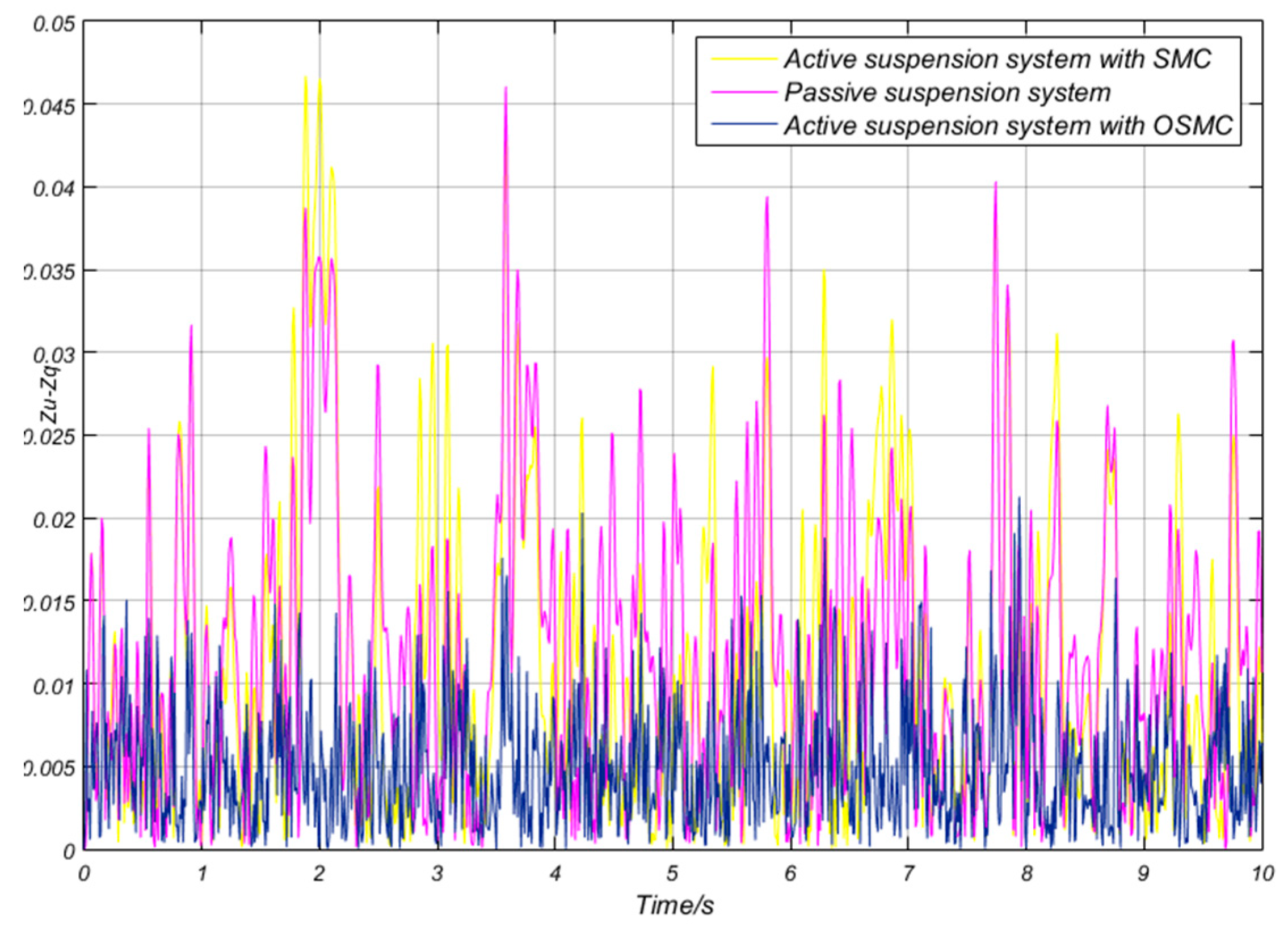
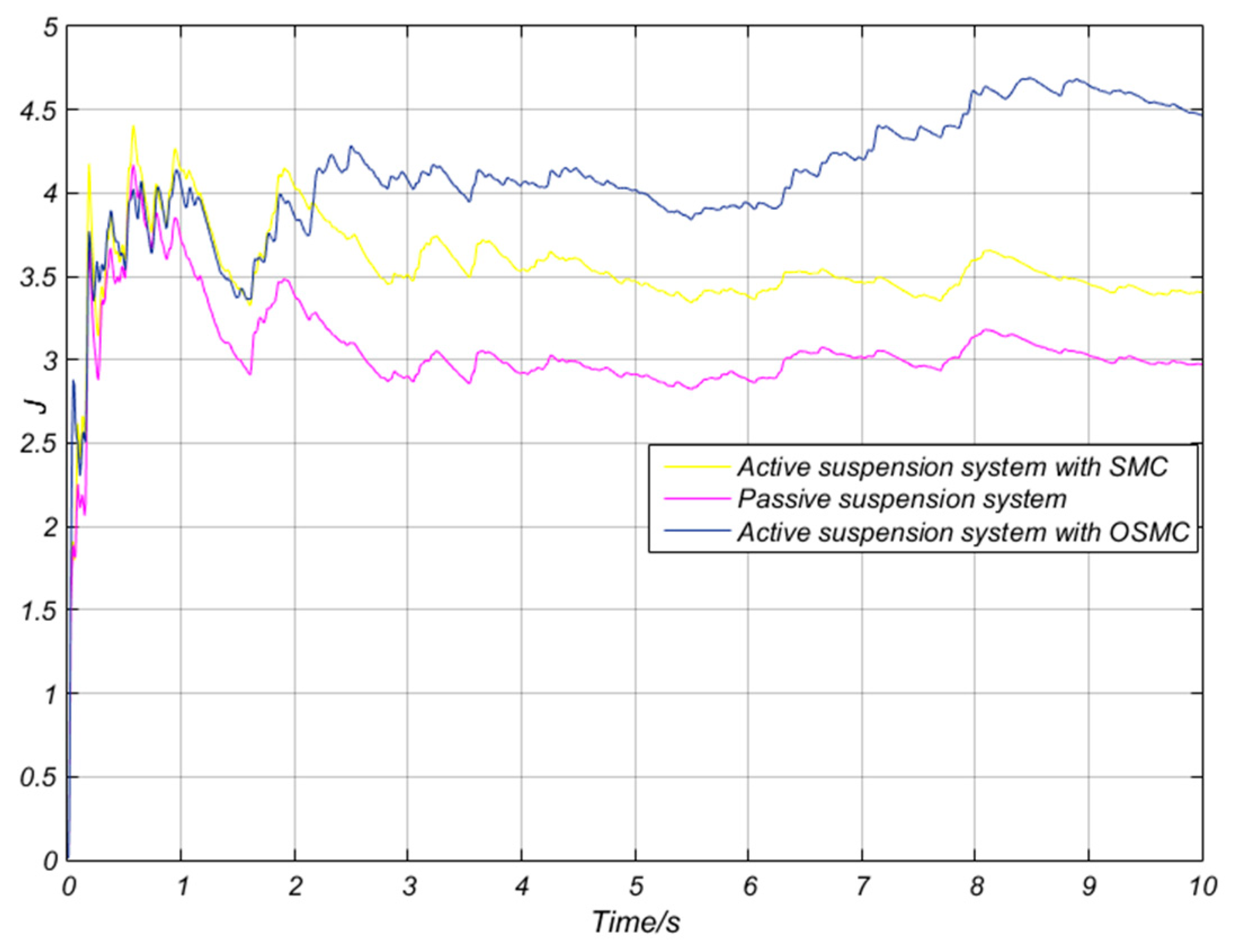
5. Simulations and Analysis
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Cui, M.; Geng, L. Random Modeling and Control of Nonlinear Active Suspension. Math. Probl. Eng. 2017, 2017, 4045796. [Google Scholar] [CrossRef]
- Su, X. Master-Slave Control for Active Suspension Systems with Hydraulic Actuator Dynamics. IEEE Access 2017, 5, 3612–3621. [Google Scholar] [CrossRef]
- Mohammadi, Y.; Ganjefar, S. Quarter car active suspension system: Minimum time controller design using singular perturbation method. Int. J. Control Autom. Syst. 2017, 15, 2538–2550. [Google Scholar] [CrossRef]
- Wang, P.; Wang, Q.; Xu, X.; Chen, N. Fractional Critical Damping Theory and Its Application in Active Suspension Control. Shock Vib. 2017, 2017, 2738976. [Google Scholar] [CrossRef]
- Huang, Y.; Na, J.; Wu, X.; Gao, G. Approximation-Free Control for Vehicle Active Suspensions with Hydraulic Actuator. IEEE Trans. Ind. Electron. 2018, 65, 7258–7267. [Google Scholar] [CrossRef]
- Bououden, S.; Chadli, M. A robust predictive control design for nonlinear active suspension systems. Asian J. Control 2016, 18, 122–132. [Google Scholar] [CrossRef]
- Kumar, V.; Rana, K.P.S.; Kumar, J.; Mishra, P. Self-tuned robust fractional order fuzzy PD controller for uncertain and nonlinear active suspension system. Neural Comput. Appl. 2018, 30, 1827–1843. [Google Scholar] [CrossRef]
- Deepika, D.; Narayan, S.; Kaur, S. Robust finite time integral sliding mode tracker for nth-order non-affine non-linear system with uncertainty and disturbance estimator. Math. Comput. Simul. 2018, 156, 364–376. [Google Scholar] [CrossRef]
- Bharali, J.; Garg, N. Efficient Ride Quality and Road Holding Improvement for Active Suspension System. In Proceedings of the 14th IEEE India Council International Conference (INDICON), Roorkee, India, 15–17 December 2017. [Google Scholar]
- Torchani, B.; Sellami, A.; Mhiri, R.; Gareia, G. Study of Comparative Saturated Sliding Mode Control and LQR Controller. J. Mech. Eng. Autom. 2011, 1, 245–249. [Google Scholar]
- Ayadi, A.; Smaoui, M.; Aloui, S. Adaptive sliding mode control with moving surface: Experimental validation for electropneumatic system. Mech. Syst. Signal Process. 2018, 109, 27–44. [Google Scholar] [CrossRef]
- Mohamed, G.; Sofiane, A.A.; Nicolas, L. Adaptive super twisting extended state observer based sliding mode control for diesel engine air path subject to matched and unmatched disturbance. IEEE Trans. Ind. Electron. 2018, 65, 6901–6911. [Google Scholar] [CrossRef]
- Benamor, A.; Messaoud, H. Robust adaptive sliding mode control for uncertain systems with unknown time-varying delay input. ISA Trans. 2018, 79, 1–12. [Google Scholar] [CrossRef]
- Devika, K.B.; Thomas, S. Sliding mode controller design for MIMO nonlinear systems: A novel power rate reaching law approach for improved performance. J. Frankl. Inst. Eng. Appl. Math. 2018, 355, 5082–5098. [Google Scholar]
- Ozer, H.O.; Hacioglu, Y.; Yagiz, N. High order sliding mode control with estimation for vehicle active suspensions. Trans. Inst. Meas. Control 2017, 40, 1457–1470. [Google Scholar] [CrossRef]
- Amini, M.R.; Shahbakhti, M.; PanA, S. Daptive Discrete Second-Order sliding mode control with Application to Nonlinear Automotive Systems. J. Dyn. Syst. Meas. Control. 2018, 140, 121010. [Google Scholar] [CrossRef]
- Liu, J. Simulation of Sliding Mode Control Based on MATLAB; Tsinghua University Press: Beijing, China, 2015; pp. 4–25. [Google Scholar]
- Tuan, L.A.; Cuong, H.M.; van Trieu, P. Adaptive neural network sliding mode control of shipboard container cranes considering actuator backlash. Mech. Syst. Signal Process. 2018, 112, 233–250. [Google Scholar] [CrossRef]
- Alves, U.N.L.; Garcia, J.P.F.; Teixeira, M.; Garcia, S.C.; Rodrigues, F.B. Sliding mode control for active suspension system with data acquisition delay. Math. Probl. Eng. 2014, 2014, 529293. [Google Scholar] [CrossRef]
- Yuvapriya, T.; Lakshmi, P.; Rajendiran, S. Vibration suppression in full car active suspension system using fractional order sliding mode controller. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 217. [Google Scholar] [CrossRef]
- Repecho, V.; Biel, D.; Olm, J.M. Robust sliding mode control of a DC/DC Boost converter with switching frequency regulation. J. Frankl. Inst. 2018, 355, 5367–5383. [Google Scholar] [CrossRef]
- Ahmadi, M.A.; Zendehboudi, S.; Dusseault, M.B. Evolving simple-to-use method to determine water–oil relative permeability in petroleum reservoirs. J. Pet. 2016, 2, 67–78. [Google Scholar] [CrossRef]
- Mitchell, M. An Introduction to Genetic Algorithms; MIT Press: Cambridge, MA, USA, 1996; Volume 32. [Google Scholar]
- Pankaj, R.; Vineet, S.; Om, P. Determination of Stabilizing Parameter of Fractional Order PID Controller Using Genetic Algorithm. Int. J. Comput. Eng. Manag. 2012, 15, 24–32. [Google Scholar]
- Nabavi, S.; Zhang, L. Design and Optimization of Piezoelectric MEMS Vibration Energy Harvesters Based on Genetic Algorithm. IEEE Sens. J. 2017, 17, 7372–7382. [Google Scholar] [CrossRef]
- Gad, S.; Metered, H.; Bassuiny, A.; Ghany, A. Multi-objective genetic algorithm fractional-order PID controller for semi-active magnetorheologically damped seat suspension. J. Vib. Control 2016, 34, 71–80. [Google Scholar] [CrossRef]
- Almeida, J.H.S.; Ribeiro, M.L. Stacking sequence optimization in composite tubes under internal pressure based on genetic algorithm accounting for progressive damage. Compos. Struct. 2017, 178, 20–26. [Google Scholar] [CrossRef]
- Koch, G.; Pellegrini, E.; Spirk, S.; Lohmann, B. Design and Modeling of a Quarter-Vehicle Test Rig for Active Suspension Control; Technical Reports on Automatic Control; Lehrstuhl für Regelungstechnik: Garching bei München, Germany, 2010; Volume TRAC-5. [Google Scholar]
- Dong, X.; Zhao, D.; Yang, B.; Han, C. Fractional-order control of active suspension actuator based on parallel adaptive clonal selection algorithm. J. Mech. Sci. Technol. 2016, 30, 2769–2781. [Google Scholar] [CrossRef]
- Wang, D.; Zhao, D.; Gong, M.; Yang, B. Nonlinear Predictive Sliding Mode Control for Active Suspension System. Shock Vib. 2018, 2018, 8194305. [Google Scholar] [CrossRef]
- Wang, J. Design Methods of Holographic Optimal Sliding Mode Controllers for Vehicle Active Suspensions. J. Traffic Transp. Eng. 2016, 3, 72–83. [Google Scholar]
- Aly, M.F.; Nassef, A.O.; Hamza, K. Multi-objective design of vehicle suspension systems via a local diffusion genetic algorithm for disjoint Pareto frontiers. Optim. Eng. 2015, 47, 706–717. [Google Scholar] [CrossRef]
- Tran, H.K.; Nguyen, T.N. Flight Motion Controller Design using Genetic Algorithm for a Quadcopter. Meas. Control 2018, 51, 59–64. [Google Scholar] [CrossRef]
- Gupta, R.K.; Bhunia, A.K.; Roy, D. A GA based penalty function technique for solving constrained redundancy allocation problem of series system with interval valued reliability of components. J. Comput. Appl. Math. 2009, 232, 275–284. [Google Scholar] [CrossRef]
- Shian, C.; Feng, Q. A method to determine the weight coefficient of vehicle suspension LQG control. J. Vib. Shock 2008, 2, 65–68. [Google Scholar]
- Yuan, G.A.O.; Wei, X.U.; Huili, L.A.N. Fuzzy sliding mode control for nonlinear vehicle suspension based on differential geometry. J. Hefei Univ. Technol. (Nat. Sci.) 2014, 3, 266–271. [Google Scholar]





| Symbol | Value | Unit |
|---|---|---|
| 0.1 | ||
| 0.2 | ||
| ν | 20 | |
| 0.0005 |
| Parameter | Value |
|---|---|
| Population size | 100 |
| Crossover probability | 0.5 |
| Mutation probability | 0.02 |
| Maximum number of generations | 30 |
| Symbol | Value | Unit | Symbol | Value | Unit |
|---|---|---|---|---|---|
| 42 | 8.2 | \ | |||
| 343 | 0.0148 | \ | |||
| 1.2 | 0.06 | \ | |||
| 200 | 29.63 | \ | |||
| 20 | 20000 | \ | |||
| 2.538 | \ | 100 | \ |
| Index | OSMC | SMC | Passive System |
|---|---|---|---|
| 1.838 | 2.222 | 2.001 | |
| 0.01066 | 0.00591 | 0.006425 | |
| 0.00745 | 0.009969 | 0.003582 | |
| 2.974 | 3.408 | 4.466 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, C.; Liu, X.; Chen, W.; Xu, F.; Cao, B. Optimal Sliding Mode Control for an Active Suspension System Based on a Genetic Algorithm. Algorithms 2018, 11, 205. https://doi.org/10.3390/a11120205
Zhou C, Liu X, Chen W, Xu F, Cao B. Optimal Sliding Mode Control for an Active Suspension System Based on a Genetic Algorithm. Algorithms. 2018; 11(12):205. https://doi.org/10.3390/a11120205
Chicago/Turabian StyleZhou, Chen, Xinhui Liu, Wei Chen, Feixiang Xu, and Bingwei Cao. 2018. "Optimal Sliding Mode Control for an Active Suspension System Based on a Genetic Algorithm" Algorithms 11, no. 12: 205. https://doi.org/10.3390/a11120205
APA StyleZhou, C., Liu, X., Chen, W., Xu, F., & Cao, B. (2018). Optimal Sliding Mode Control for an Active Suspension System Based on a Genetic Algorithm. Algorithms, 11(12), 205. https://doi.org/10.3390/a11120205





