Zirconium Carbide Produced by Spark Plasma Sintering and Hot Pressing: Densification Kinetics, Grain Growth, and Thermal Properties
Abstract
:1. Introduction
2. Materials and Experiment
2.1. Starting Powders
2.2. Consolidation of Zirconium Carbide Powder
2.3. Characterization of Processed Specimens
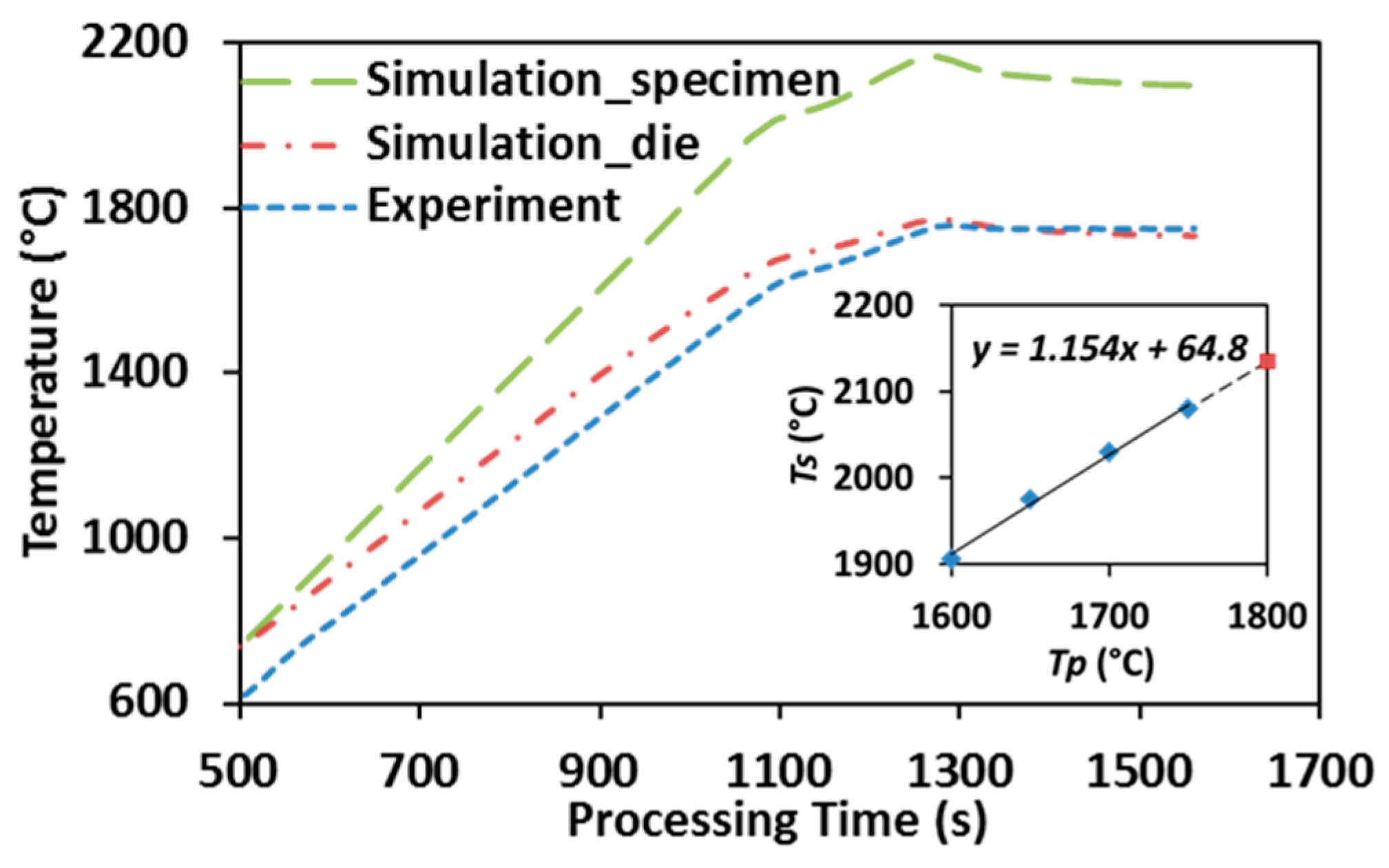
2.4. Temperature Evolution in SPS of ZrC
2.5. Measurement of Thermal Properties
3. Discussion
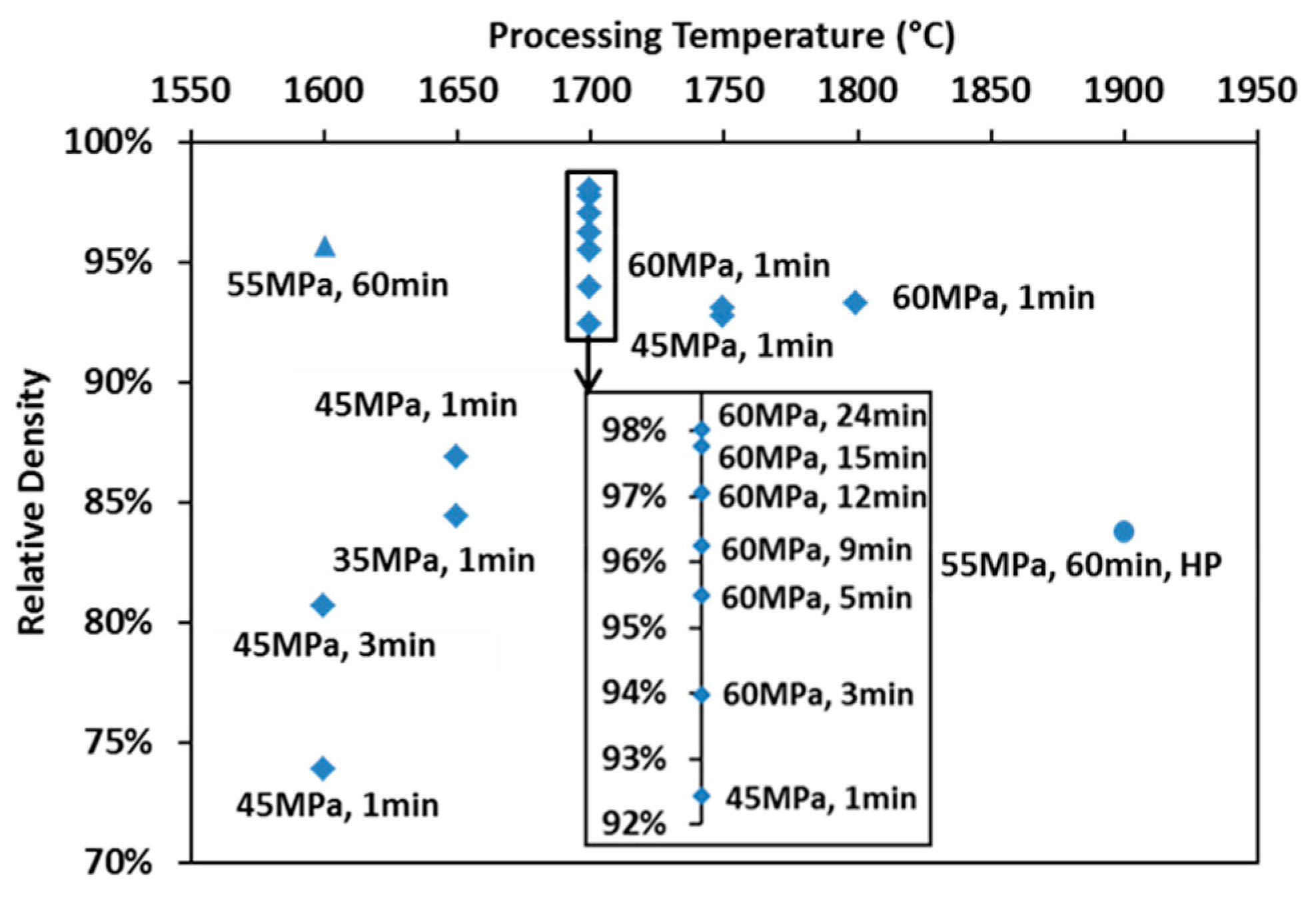
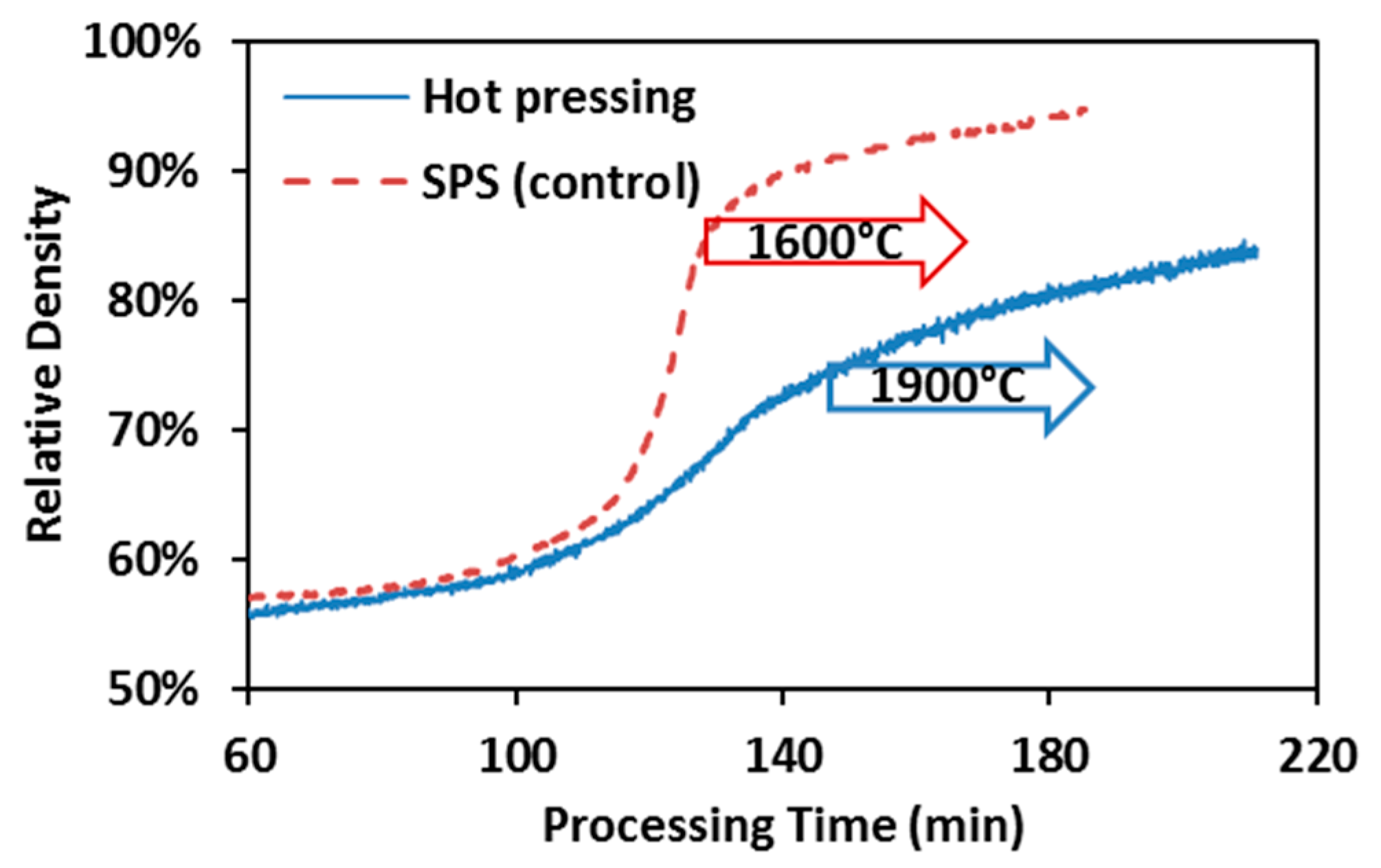
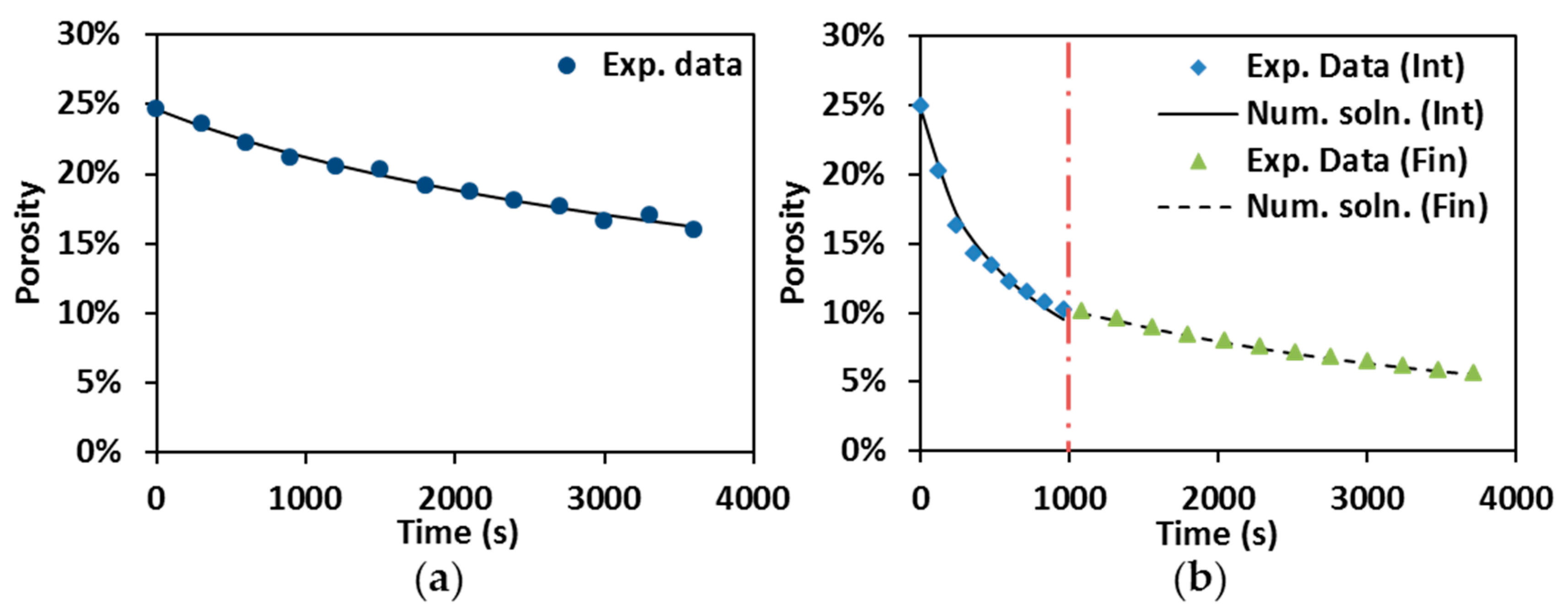
3.1. Densification Kinetics
3.2. Temperature Evolution in SPS of ZrC
3.3. Densification Mechanisms in SPS and Hot Pressing of ZrC
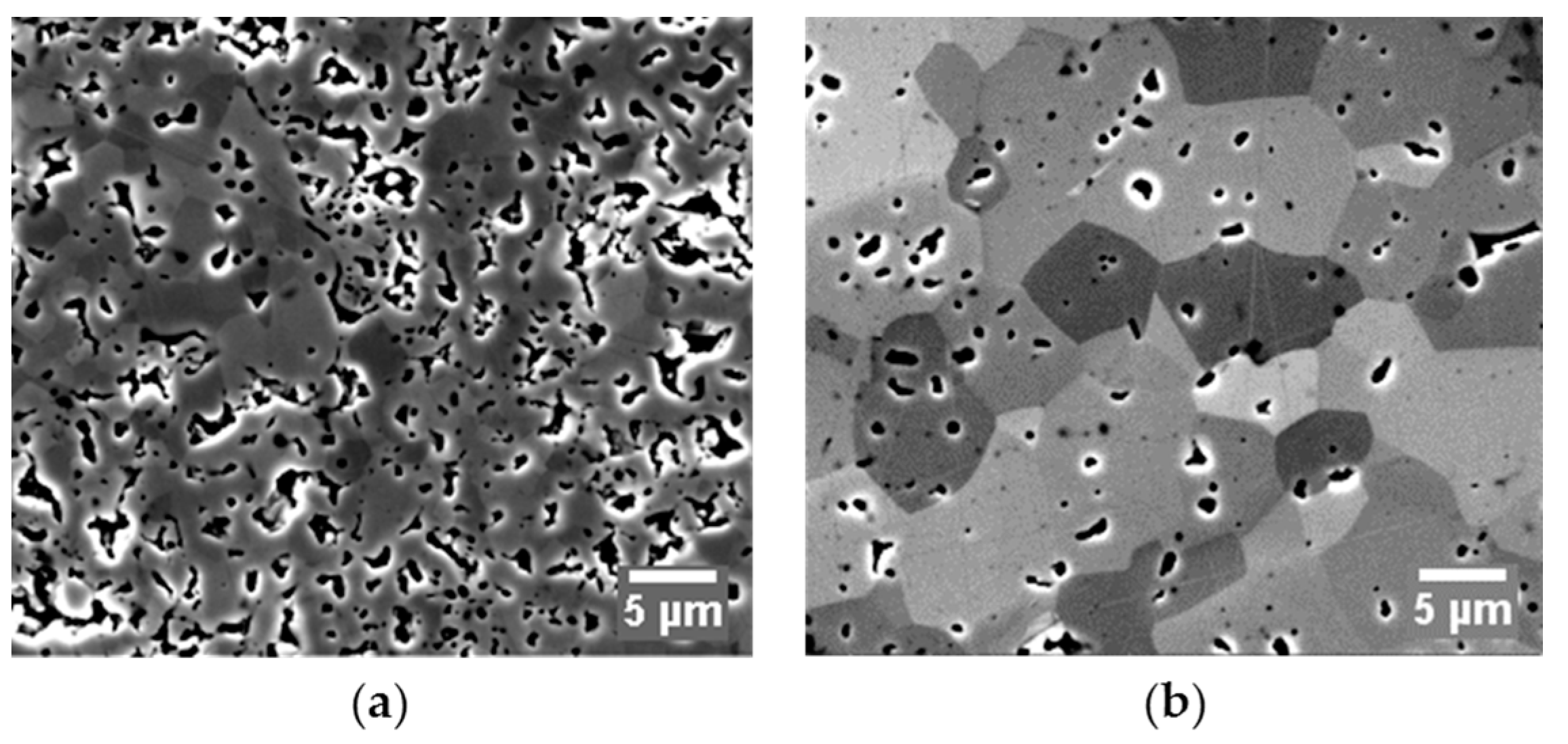
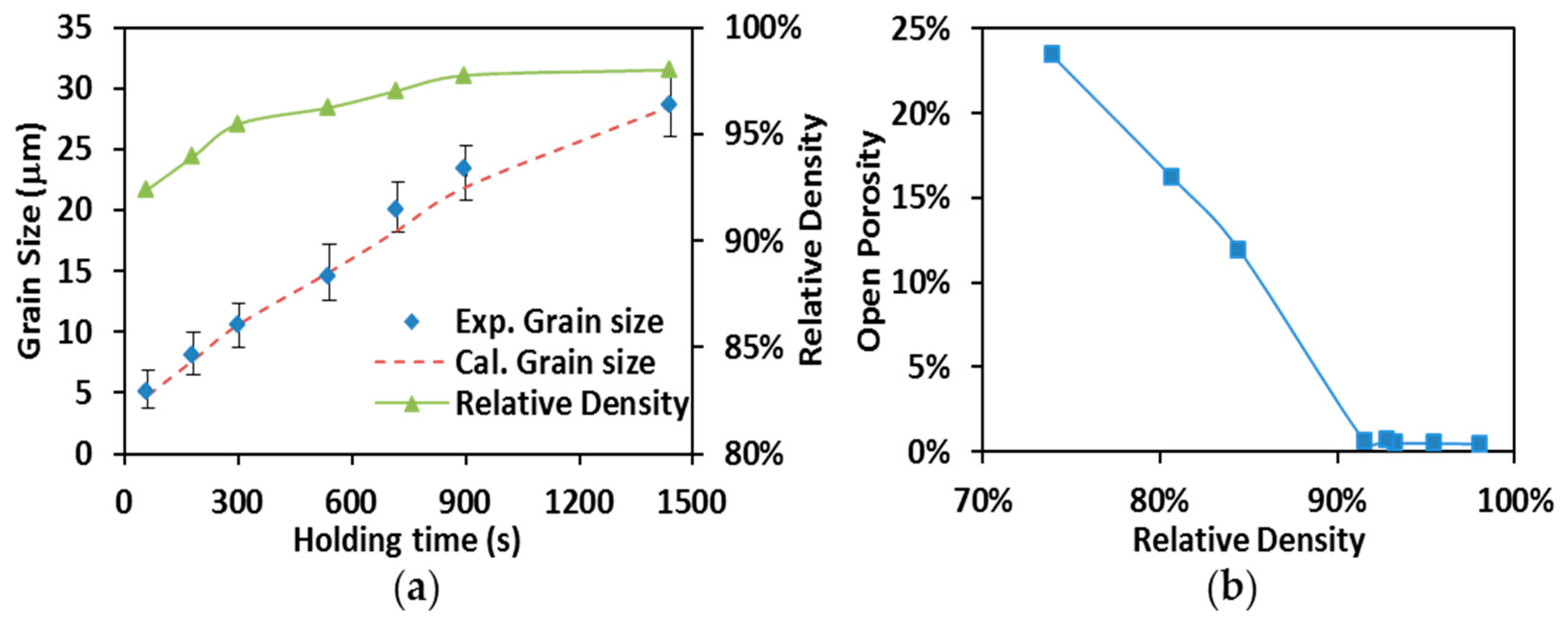
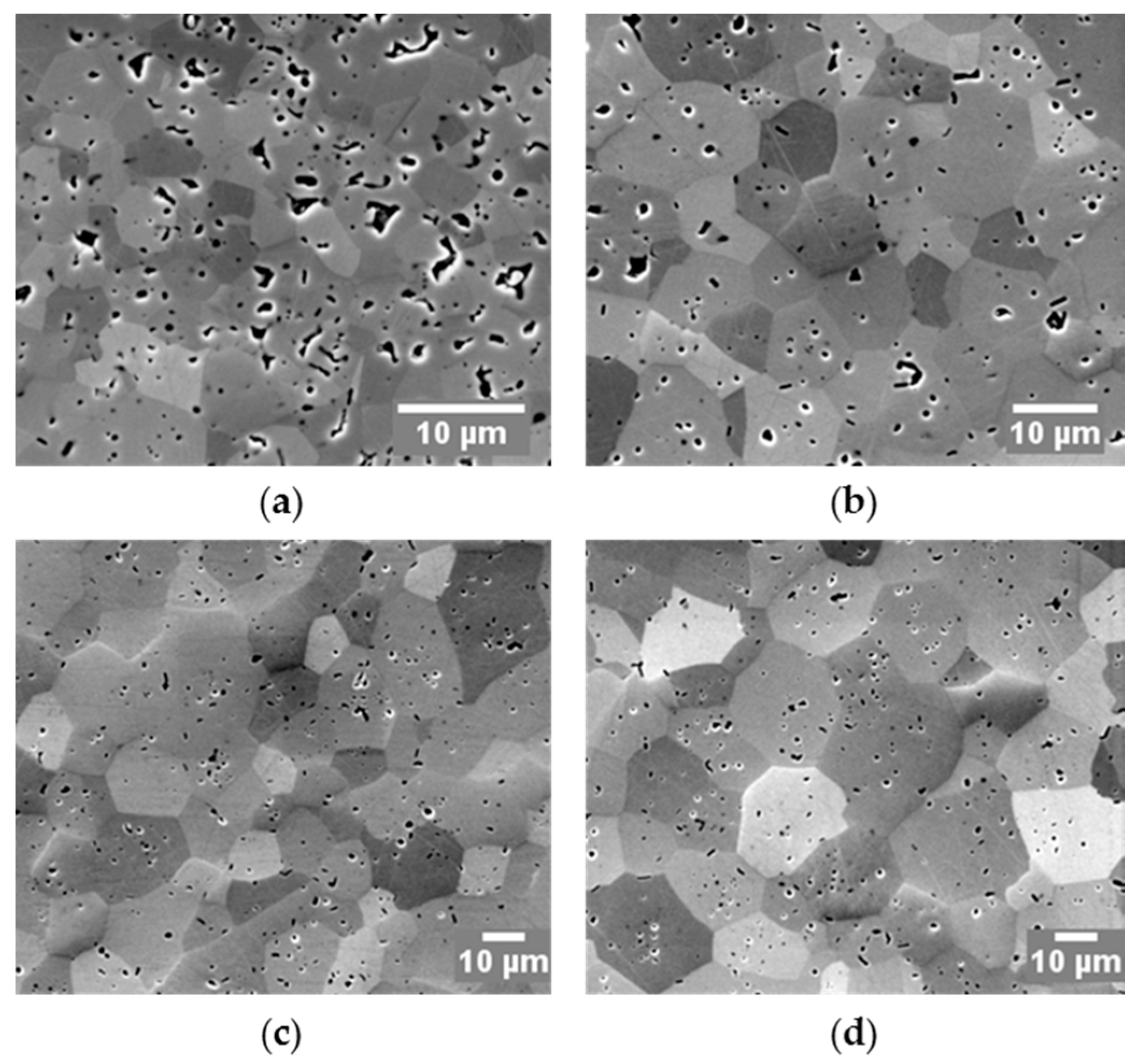
3.4. Grain Growth and Microstructures of SPS Processed Specimens
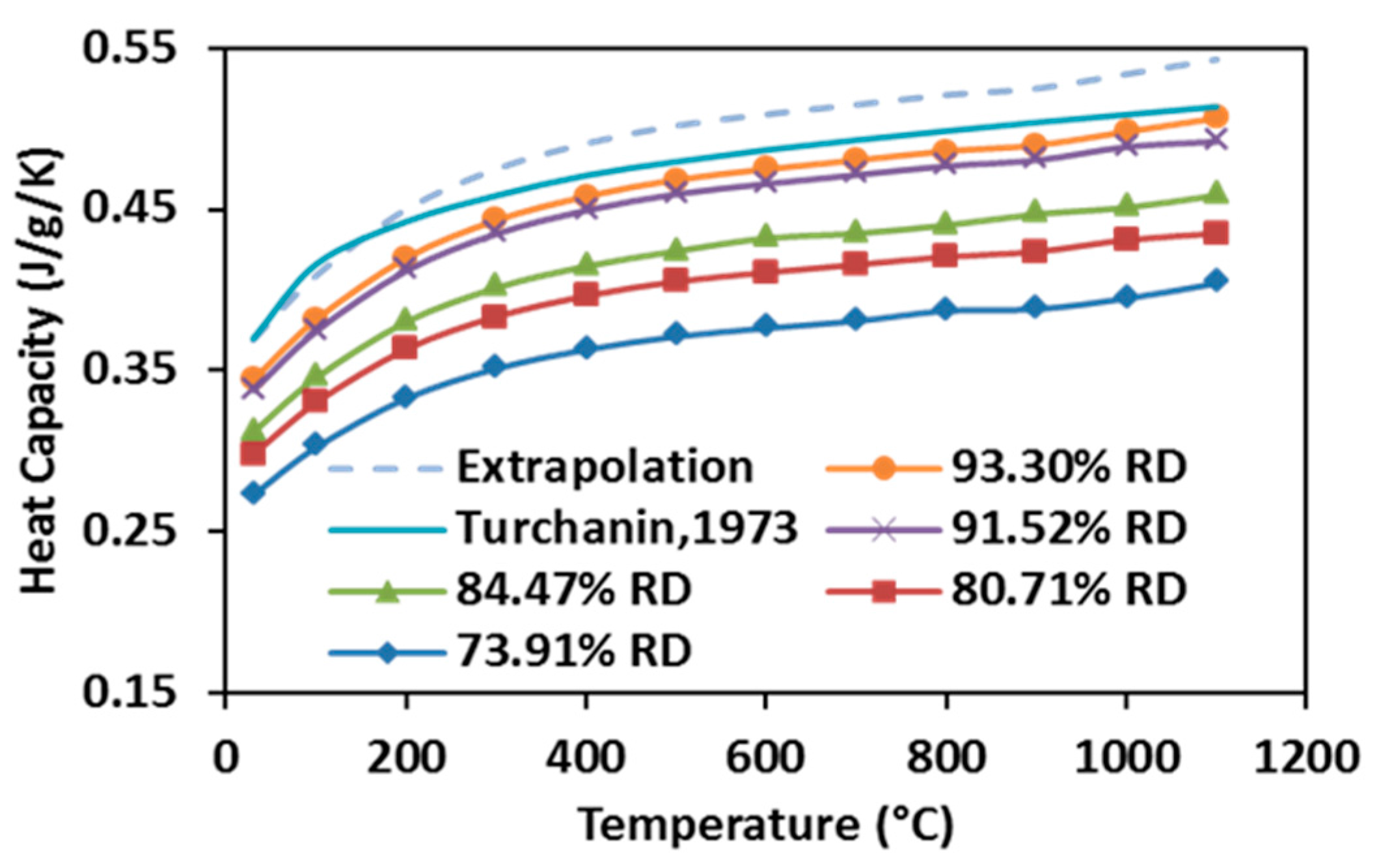
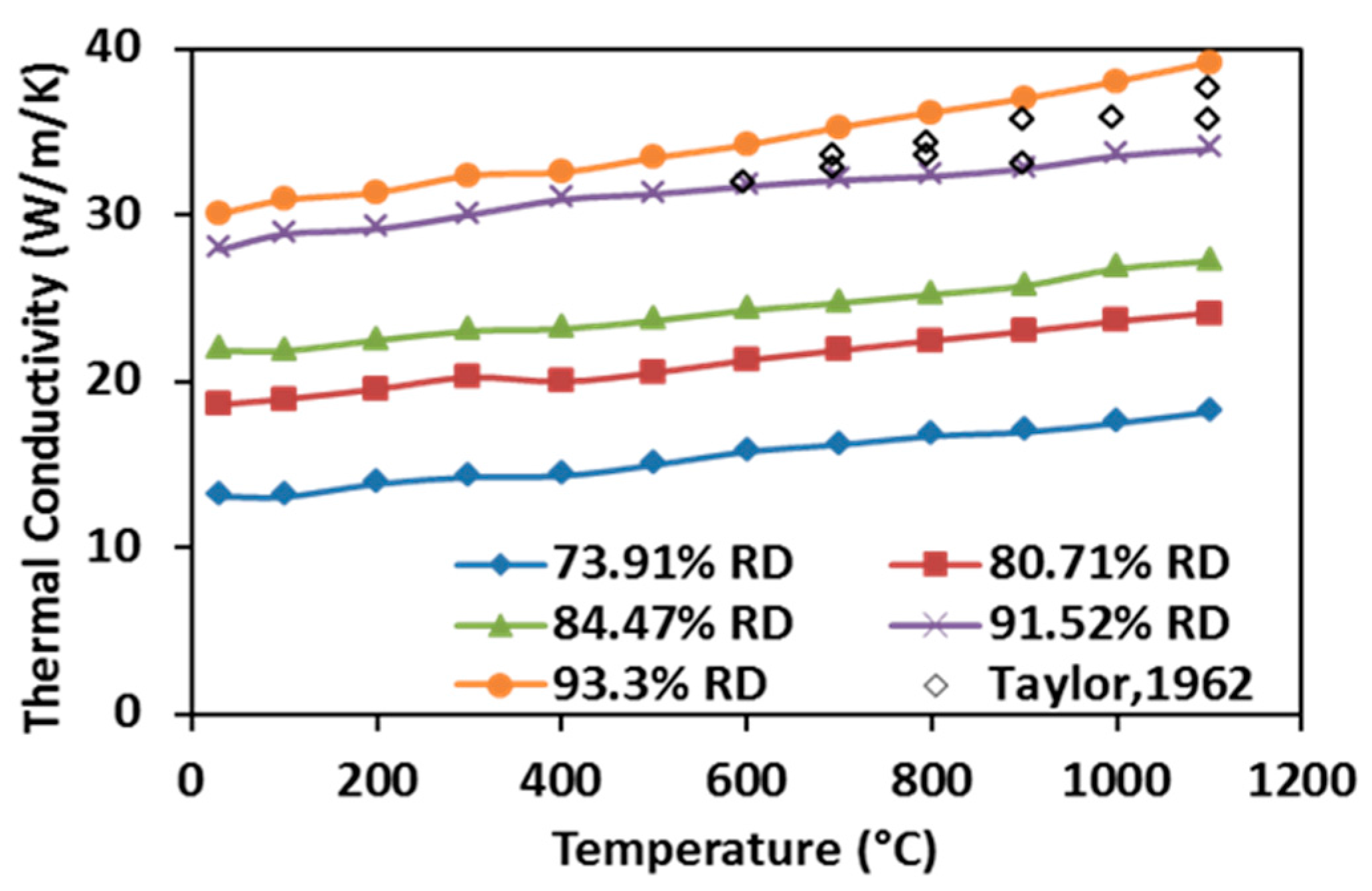
3.5. Thermal Properties of SPS-Processed Specimens
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Munir, Z.A.; Anselmi-Tamburini, U.; Ohyanagi, M. The effect of electric field and pressure on the synthesis and consolidation of materials: A review of the spark plasma sintering method. J. Mater. Sci. 2006, 41, 763–777. [Google Scholar] [CrossRef]
- Orru, R.; Licheri, R.; Locci, A.M.; Cincotti, A.; Cao, G.C. Consolidation/synthesis of materials by electric current activated/assisted sintering. Mater. Sci. Eng. R. 2009, 63, 127–287. [Google Scholar] [CrossRef]
- Munir, Z.A.; Quach, D.V.; Ohyanagi, M. Electric current activation of sintering: A review of the pulsed electric current sintering process. J. Am. Ceram. Soc. 2011, 94, 1–19. [Google Scholar] [CrossRef]
- Guillon, O.; Gonzalez-Julian, J.; Dargatz, B.; Kessel, T.; Schierning, G.; Räthel, J.; Herrmann, M. Field-assisted sintering technology/spark plasma sintering: Mechanisms, materials, and technology developments. Adv. Eng. Mater. 2014, 16, 830–849. [Google Scholar] [CrossRef]
- Khaleghi, E.; Lin, Y.S.; Meyers, M.A.; Olevsky, E.A. Spark plasma sintering of tantalum carbide. Scr. Mater. 2010, 63, 577–580. [Google Scholar] [CrossRef]
- Bellosi, A.; Monteverde, F.; Sciti, D. Fast Densification of ultra-high-temperature ceramics by spark plasma sintering. Int. J. Appl. Ceram. Technol. 2006, 3, 32–40. [Google Scholar] [CrossRef]
- Li, W.; Olevsky, E.A.; Khasanov, O.L.; Back, C.A.; Izhvanov, O.L.; Opperman, J.; Khalifa, H.E. Spark plasma sintering of agglomerated vanadium carbide powder. Ceram. Int. 2015, 41, 3748–3759. [Google Scholar] [CrossRef]
- Wei, X.; Back, C.; Izhvanov, O.; Khasanov, O.; Haines, C.; Olevsky, E. Spark plasma sintering of commercial zirconium carbide powders: Densification behavior and mechanical properties. Materials 2015, 8, 6043–6061. [Google Scholar] [CrossRef]
- Mizuguchi, T.; Guo, S.; Kagawa, Y. Transmission electron microscopy characterization of spark plasma sintered ZrB2 ceramic. Ceram. Int. 2010, 36, 943–946. [Google Scholar] [CrossRef]
- Giuntini, D.; Wei, X.; Maximenko, A.L.; Li, W.; Ilyina, A.M.; Olevsky, E.A. Initial stage of free pressureless spark-plasma sintering of vanadium carbide: Determination of surface diffusion parameters. Int. J. Refract. Met. Hard Mater. 2013, 41, 501–506. [Google Scholar] [CrossRef]
- Olevsky, E.; Bogachev, I.; Maximenko, A. Spark-plasma sintering efficiency control by inter-particle contact area growth: A viewpoint. Scr. Mater. 2013, 69, 112–116. [Google Scholar] [CrossRef]
- Narayan, J. Grain growth model for electric field-assisted processing and flash sintering of materials. Scr. Mater. 2013, 68, 785–788. [Google Scholar] [CrossRef]
- Ryu, H.J.; Lee, Y.W.; Cha, S.I.; Hong, S.H. Sintering behaviour and microstructures of carbides and nitrides for the inert matrix fuel by spark plasma sintering. J. Nucl. Mater. 2006, 352, 341–348. [Google Scholar] [CrossRef]
- Vasudevamurthy, G.; Knight, T.W.; Roberts, E.; Adams, T.M. Laboratory production of zirconium carbide compacts for use in inert matrix fuels. J. Nucl. Mater. 2008, 374, 241–247. [Google Scholar] [CrossRef]
- Katoh, Y.; Vasudevamurthy, G.; Nozawa, T.; Snead, L.L. Properties of zirconium carbide for nuclear fuel applications. J. Nucl. Mater. 2013, 441, 718–742. [Google Scholar] [CrossRef]
- Spivak, I.I.; Klimenko, V.V. Densification kinetics in the hot pressing and recrystallization of carbides. Sov. Powder Metall. Met. Ceram. 1973, 12, 883–887. [Google Scholar]
- Bulychev, V.P.; Andrievskii, R.A.; Nezhevenko, L.B. The sintering of zirconium carbide. Sov. Powder Metall. Met. Ceram. 1977, 16, 273–276. [Google Scholar] [CrossRef]
- Barnier, P.; Brodhag, C.; Thevenot, F. Hot-pressing kinetics of zirconium carbide. J. Mater. Sci. 1986, 21, 2547–2552. [Google Scholar] [CrossRef]
- Minhaga, E.; Scott, W.D. Sintering and mechanical-properties of ZrC-ZrO2 composites. J. Mater. Sci. 1988, 23, 2865–2870. [Google Scholar] [CrossRef]
- Wang, X.G.; Guo, W.M.; Kan, Y.M.; Zhang, G.J.; Wang, P.L. Densification behavior and properties of hot-pressed ZrC ceramics with Zr and graphite additives. J. Eur. Ceram. Soc. 2011, 31, 1103–1111. [Google Scholar] [CrossRef]
- Zhao, L.; Jia, D.; Duan, X.; Yang, X.; Zhou, Y. Pressureless sintering of ZrC-based ceramics by enhancing powder sinterability. Int. J. Refract. Met. Hard Mater. 2011, 29, 516–521. [Google Scholar] [CrossRef]
- Sciti, D.; Guicciardi, S.; Nygren, M. Spark plasma sintering and mechanical behaviour of ZrC-based composites. Scr. Mater. 2008, 59, 638–641. [Google Scholar] [CrossRef]
- Gendre, M.; Maître, A.; Trolliard, G. A study of the densification mechanisms during spark plasma sintering of zirconium (oxy-)carbide powders. Acta Mater. 2010, 58, 2598–2609. [Google Scholar] [CrossRef]
- Gendre, M.; Maître, A.; Trolliard, G. Synthesis of zirconium oxycarbide (ZrCxOy) powders: Influence of stoichiometry on densification kinetics during spark plasma sintering and on mechanical properties. J. Eur. Ceram. Soc. 2011, 31, 2377–2385. [Google Scholar] [CrossRef]
- Núñez-González, B.; Ortiz, A.L.; Guiberteau, F.; Nygren, M.; Shaw, L. Improvement of the spark-plasma-sintering kinetics of ZrC by high-energy call-milling. J. Am. Ceram. Soc. 2012, 95, 453–456. [Google Scholar] [CrossRef]
- Xie, J.; Fu, Z.; Wang, Y.; Lee, W.W.; Niihara, K. Synthesis of nanosized zirconium carbide powders by a combinational method of sol–gel and pulse current heating. J. Eur. Ceram. Soc. 2014, 34, 13.e1–13.e7. [Google Scholar] [CrossRef]
- Antou, G.; Pradeilles, N.; Gendre, M.; Maître, A. New approach of the evolution of densification mechanisms during Spark Plasma Sintering: Application to zirconium (oxy-)carbide ceramics. Scr. Mater. 2015, 101, 103–106. [Google Scholar] [CrossRef]
- Olevsky, E.A. Theory of sintering: From discrete to continuum. Mater. Sci. Eng. R. 1998, 23, 41–100. [Google Scholar] [CrossRef]
- Antou, G.; Mathieu, G.; Trolliard, G.; Maître, A. Spark plasma sintering of zirconium carbide and oxycarbide: Finite element modeling of current density, temperature, and stress distributions. J. Mater. Res. 2009, 24, 404–412. [Google Scholar] [CrossRef]
- Taylor, R.E. Thermal conductivity of zirconium carbide at high temperatures. J. Am. Ceram. Soc. 1962, 45, 353–354. [Google Scholar] [CrossRef]
- Grossman, L.N. High-temperature thermophysical properties of zirconium carbide. J. Am. Ceram. Soc. 1965, 48, 236–242. [Google Scholar] [CrossRef]
- Olevsky, E.A.; Garcia-Cardona, C.; Bradbury, W.L.; Haines, C.D.; Martin, D.G.; Kapoor, D. Fundamental aspects of spark plasma sintering: II. Finite element analysis of scalability. J. Am. Ceram. Soc. 2012, 95, 2414–2422. [Google Scholar] [CrossRef]
- Giuntini, D.; Olevsky, E.A.; Garcia-Cardona, C.; Maximenko, A.L.; Yurlova, M.S.; Haines, C.D. Localized overheating phenomena and optimization of spark-plasma sintering tooling design. Materials 2013, 6, 2612–2632. [Google Scholar] [CrossRef]
- Meyers, M.A.; Chawla, K.K. Mechanical Behavior of Materials; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Wei, X.; Giuntini, D.; Maximenko, A.L.; Haines, C.D.; Olevsky, E.A. Experimental investigation of electric contact resistance in spark plasma sintering tooling setup. J. Am. Ceram. Soc. 2015, 98, 3553–3560. [Google Scholar] [CrossRef]
- Yovanovich, M.M. Four decades of research on thermal contact, gap and joint resistance in microelectronics. IEEE. Trans. Compon. Pack. Technol. 2005, 28, 182–206. [Google Scholar] [CrossRef]
- Anselmi-Tamburini, U.; Gennari, S.; Garay, J.E.; Munir, Z.A. Fundamental investigations on the spark plasma sintering/synthesis process: II. Modeling of current and temperature distributions. Mater. Sci. Eng. A 2005, 394, 139–148. [Google Scholar] [CrossRef]
- Parker, W.J.; Jenkins, R.J.; Butler, C.P.; Abbott, G.L. Flash method of determining thermal diffusivity, heat capacity, and thermal conductivity. J. Appl. Phys. 1961, 32, 1679–1684. [Google Scholar] [CrossRef]
- Giuntini, D.; Raethel, J.; Herrmann, M.; Michaelis, A.; Olevsky, E.A. Advancement of tooling for spark plasma sintering. J. Am. Ceram. Soc. 2015, 98, 3529–3537. [Google Scholar] [CrossRef]
- Kelly, J.P.; Graeve, O.A. Mechanisms of pore formation in high-temperature carbides: Case study of TaC prepared by spark plasma sintering. Acta Mater. 2015, 84, 472–483. [Google Scholar] [CrossRef]
- Coble, R.L. Sintering crystalline solids. I. Intermediate and final state diffusion models. J. Appl. Phys. 1961, 32, 787–792. [Google Scholar] [CrossRef]
- Gifkins, R.C. Grain-boundary sliding and its accommodation during creep and superplasticity. Metall. Trans. A 1976, 7, 1225–1232. [Google Scholar] [CrossRef]
- Weertman, J. Steady-state creep of crystals. J. Appl. Phys. 1957, 28, 1185–1189. [Google Scholar] [CrossRef]
- Chen, I.W.; Wang, X.H. Sintering dense nanocrystalline ceramics without final-stage grain growth. Nature 2000, 404, 168–171. [Google Scholar] [CrossRef] [PubMed]
- Chaim, R. Densification mechanisms in spark plasma sintering of nanocrystalline ceramics. Mater. Sci. Eng. A 2007, 443, 25–32. [Google Scholar] [CrossRef]
- Djohari, H.; Martínez-Herrera, J.I.; Derby, J.J. Transport mechanisms and densification during sintering: I. Viscous flow versus vacancy diffusion. Chem. Eng. Sci. 2009, 64, 3799–3809. [Google Scholar] [CrossRef]
- Andrievskii, R.A.; Khormov, Y.F.; Alekseeva, I.S. Self-diffusion of carbon and metal atoms in zirconium and niobium carbides (in Russian). Fiz. Metal. Metalloved. 1971, 32, 664–667. [Google Scholar]
- Sarian, S. Diffusion of carbon through zirconium monocarbide. J. Appl. Phys. 1967, 38, 1794–1798. [Google Scholar] [CrossRef]
- Storms, E.K. The Refractory Carbides; Academic Press: New York, NY, USA, 1967. [Google Scholar]
- Turchanin, A.G.; Guseva, E.A.; Fesenko, V.V. Thermodynamic properties of refractory carbides in the temperature range 0–3000° K. Sov. Powder Metall. Met. Ceram. 1973, 12, 215–217. [Google Scholar] [CrossRef]
- Anderson, K.R.; Groza, J.R.; Fendorf, M.; Echer, C.J. Surface oxide debonding in field assisted powder sintering. Mater. Sci. Eng. A 1999, 270, 278–282. [Google Scholar] [CrossRef]
- Groza, J.R.; Zavaliangos, A. Sintering activation by external electrical field. Mater. Sci. Eng. A 2000, 287, 171–177. [Google Scholar] [CrossRef]


| Parameters | Values |
|---|---|
| Heat capacity, (J/kg/K) | |
| Thermal conductivity, (J/m/K) | |
| Electric conductivity, (S/m) |
| Parameters | Intermediate Stage | Final Stage | ||||
|---|---|---|---|---|---|---|
| m | Q (kJ/mol) | A0 | m | Q (kJ/mol) | A0 | |
| Hot pressing Control SPS | 0.382 | 653 | 5.92 × 10−6 | N/A | N/A | N/A |
| 0.403 | 563 | 6.58 × 10−6 | 0.403 | 576 | 6.58 × 10−6 | |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, X.; Back, C.; Izhvanov, O.; Haines, C.D.; Olevsky, E.A. Zirconium Carbide Produced by Spark Plasma Sintering and Hot Pressing: Densification Kinetics, Grain Growth, and Thermal Properties. Materials 2016, 9, 577. https://doi.org/10.3390/ma9070577
Wei X, Back C, Izhvanov O, Haines CD, Olevsky EA. Zirconium Carbide Produced by Spark Plasma Sintering and Hot Pressing: Densification Kinetics, Grain Growth, and Thermal Properties. Materials. 2016; 9(7):577. https://doi.org/10.3390/ma9070577
Chicago/Turabian StyleWei, Xialu, Christina Back, Oleg Izhvanov, Christopher D. Haines, and Eugene A. Olevsky. 2016. "Zirconium Carbide Produced by Spark Plasma Sintering and Hot Pressing: Densification Kinetics, Grain Growth, and Thermal Properties" Materials 9, no. 7: 577. https://doi.org/10.3390/ma9070577
APA StyleWei, X., Back, C., Izhvanov, O., Haines, C. D., & Olevsky, E. A. (2016). Zirconium Carbide Produced by Spark Plasma Sintering and Hot Pressing: Densification Kinetics, Grain Growth, and Thermal Properties. Materials, 9(7), 577. https://doi.org/10.3390/ma9070577








