Effect of Admixtures on the Yield Stresses of Cement Pastes under High Hydrostatic Pressures
Abstract
:1. Introduction
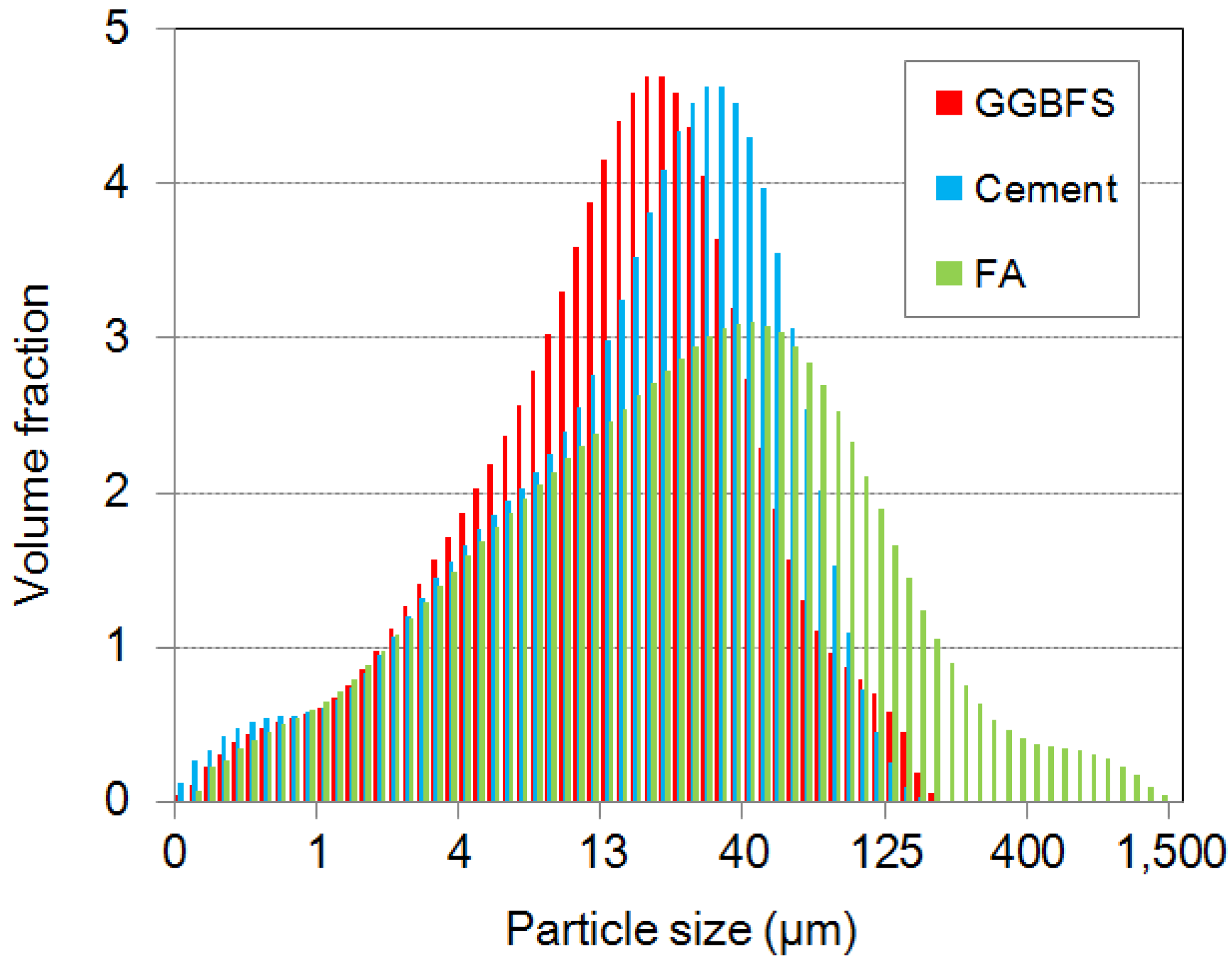
2. Sample Preparation
3. Experimental Section
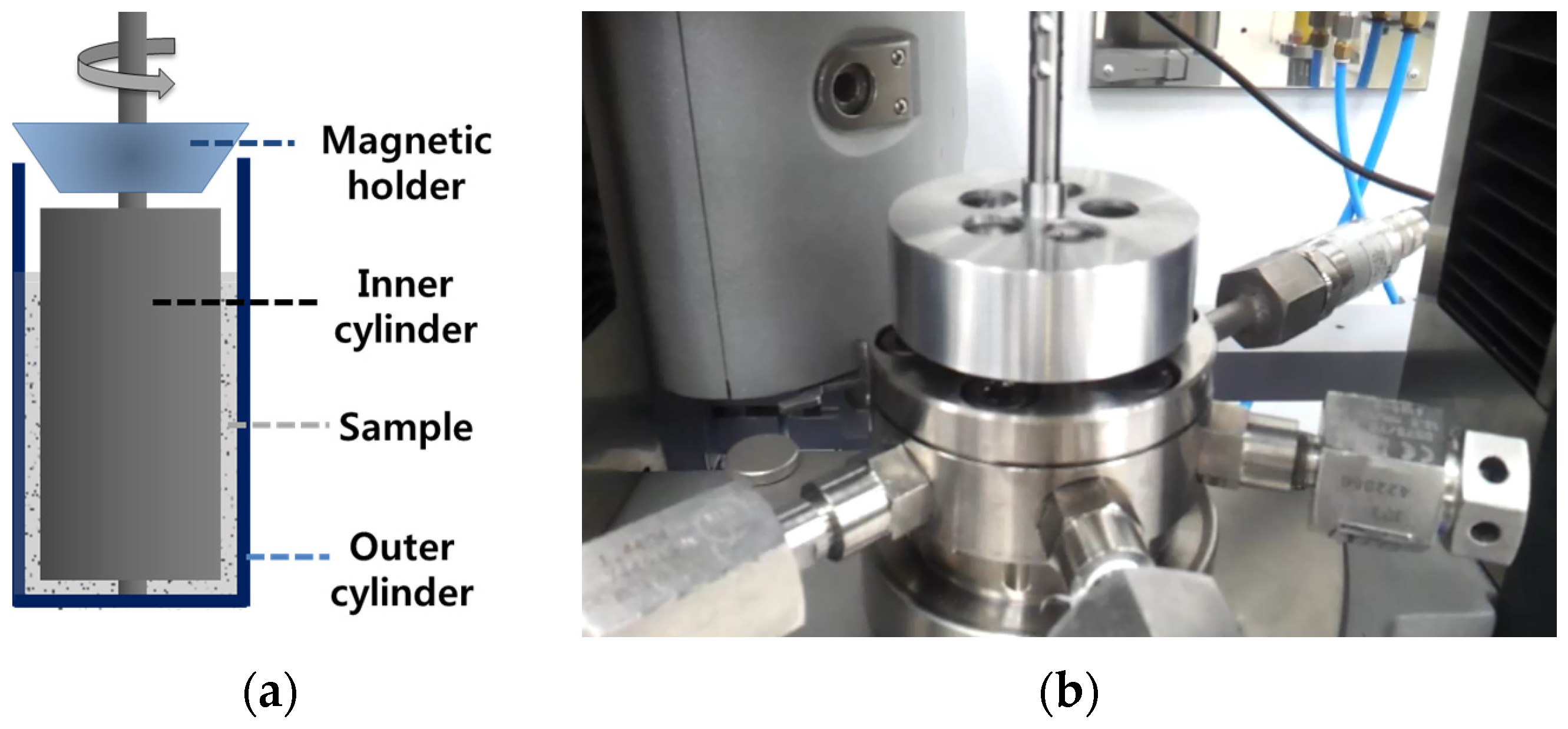
3.1. Instrument for Rheological Measurement under High Pressure
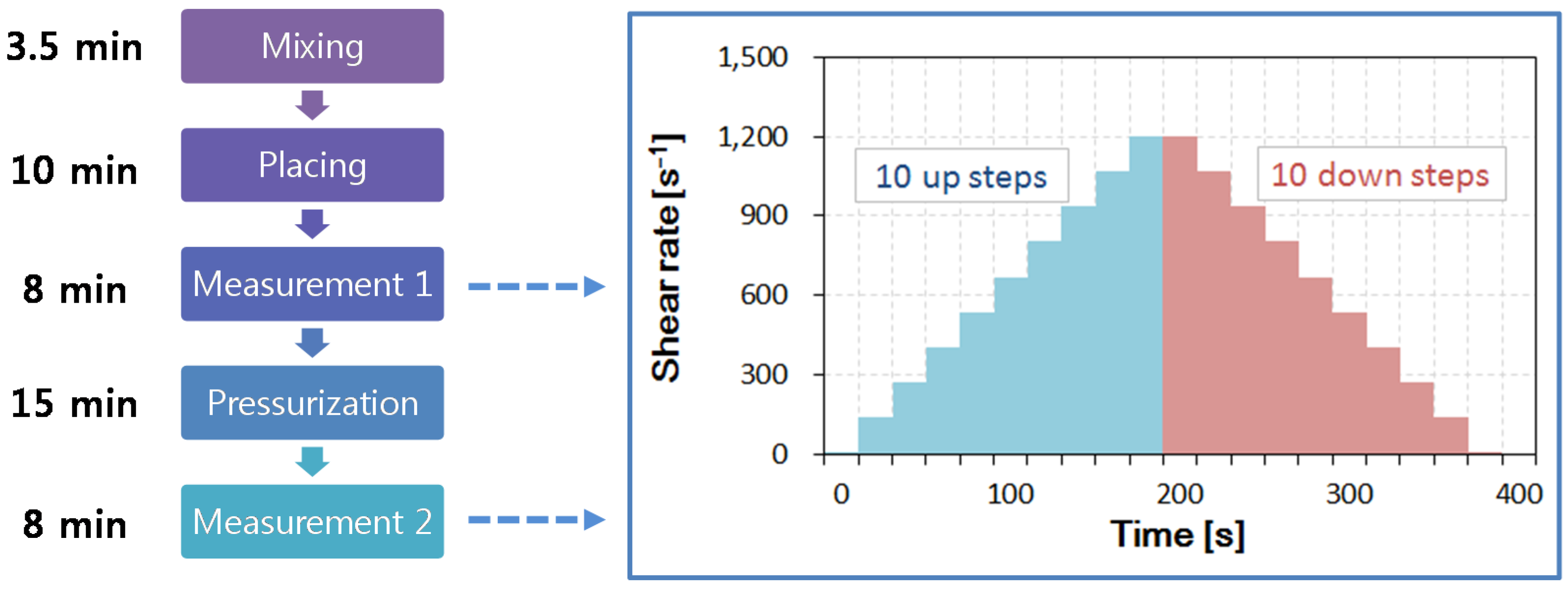
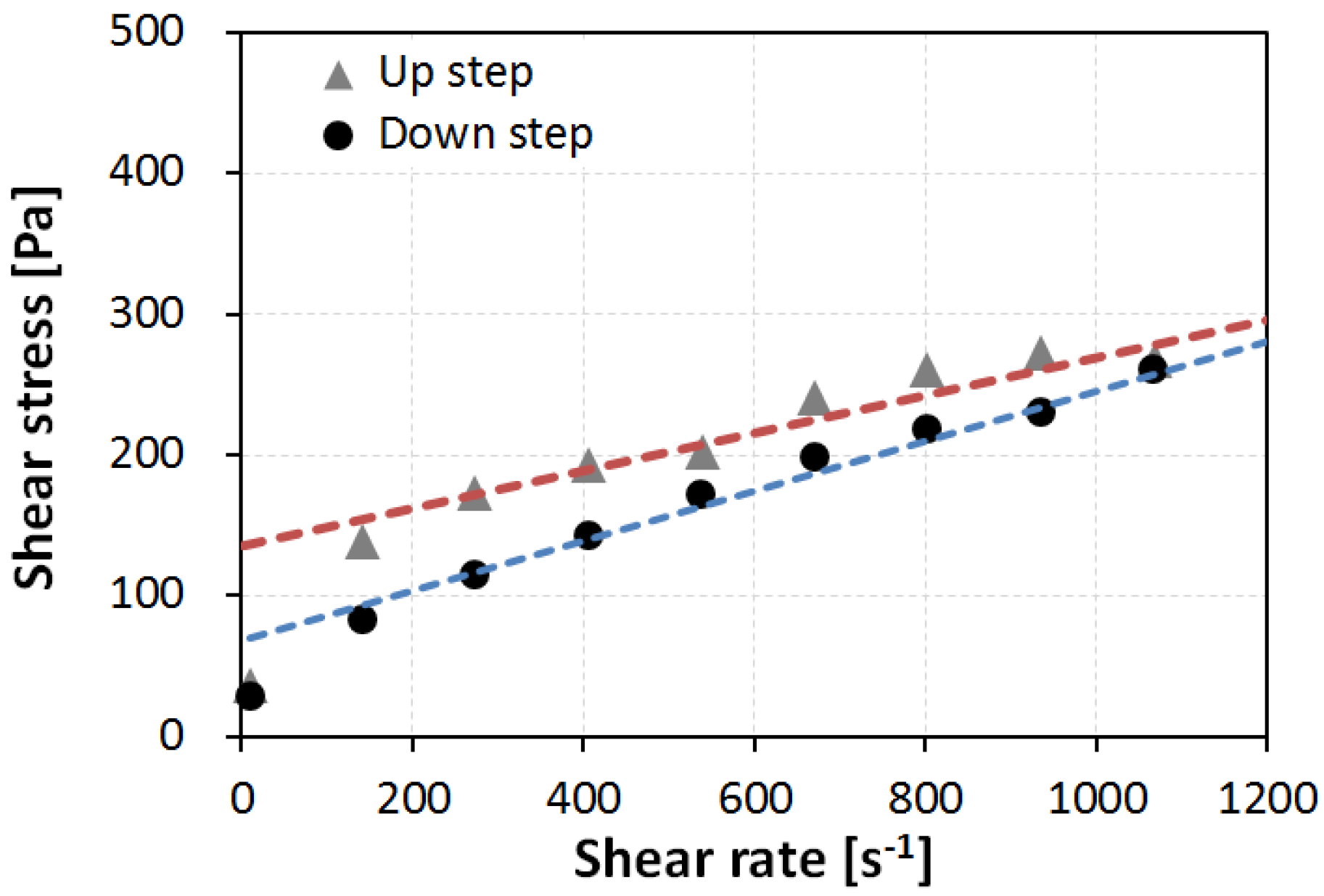
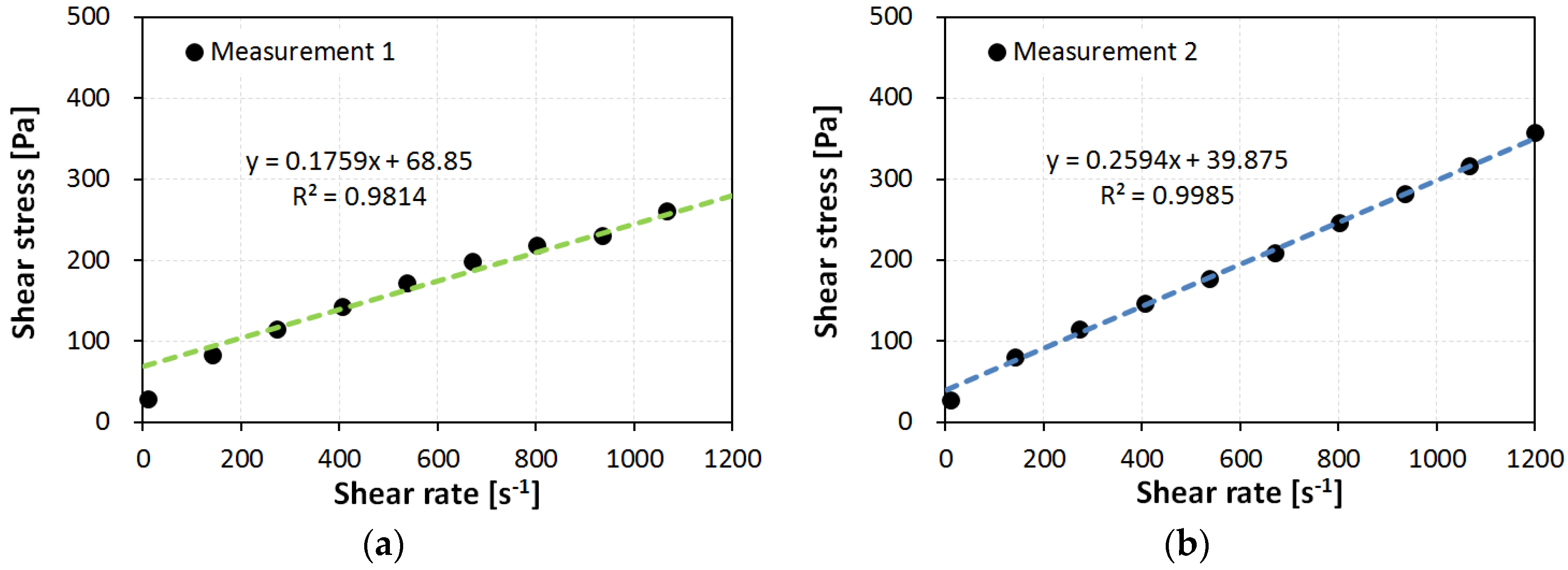
3.2. Rheological Measurements
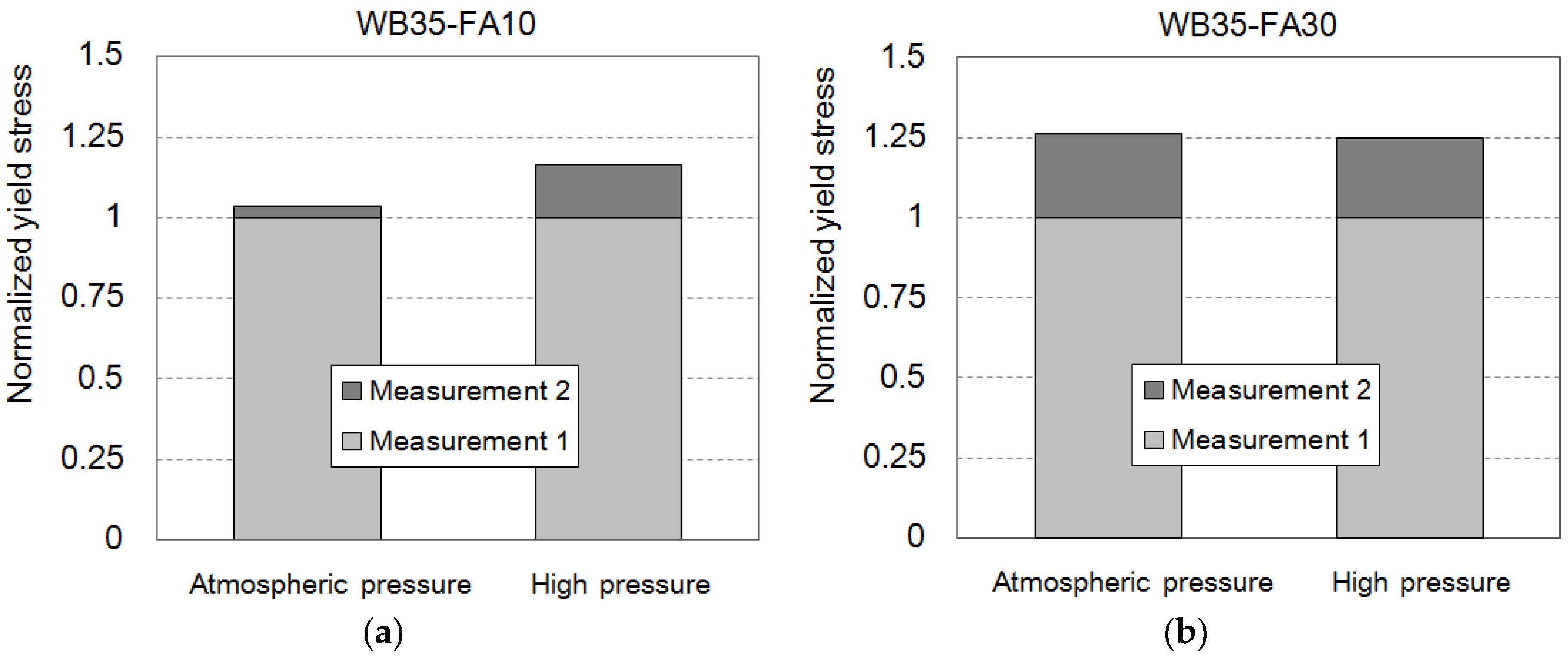
4. Results and Discussion
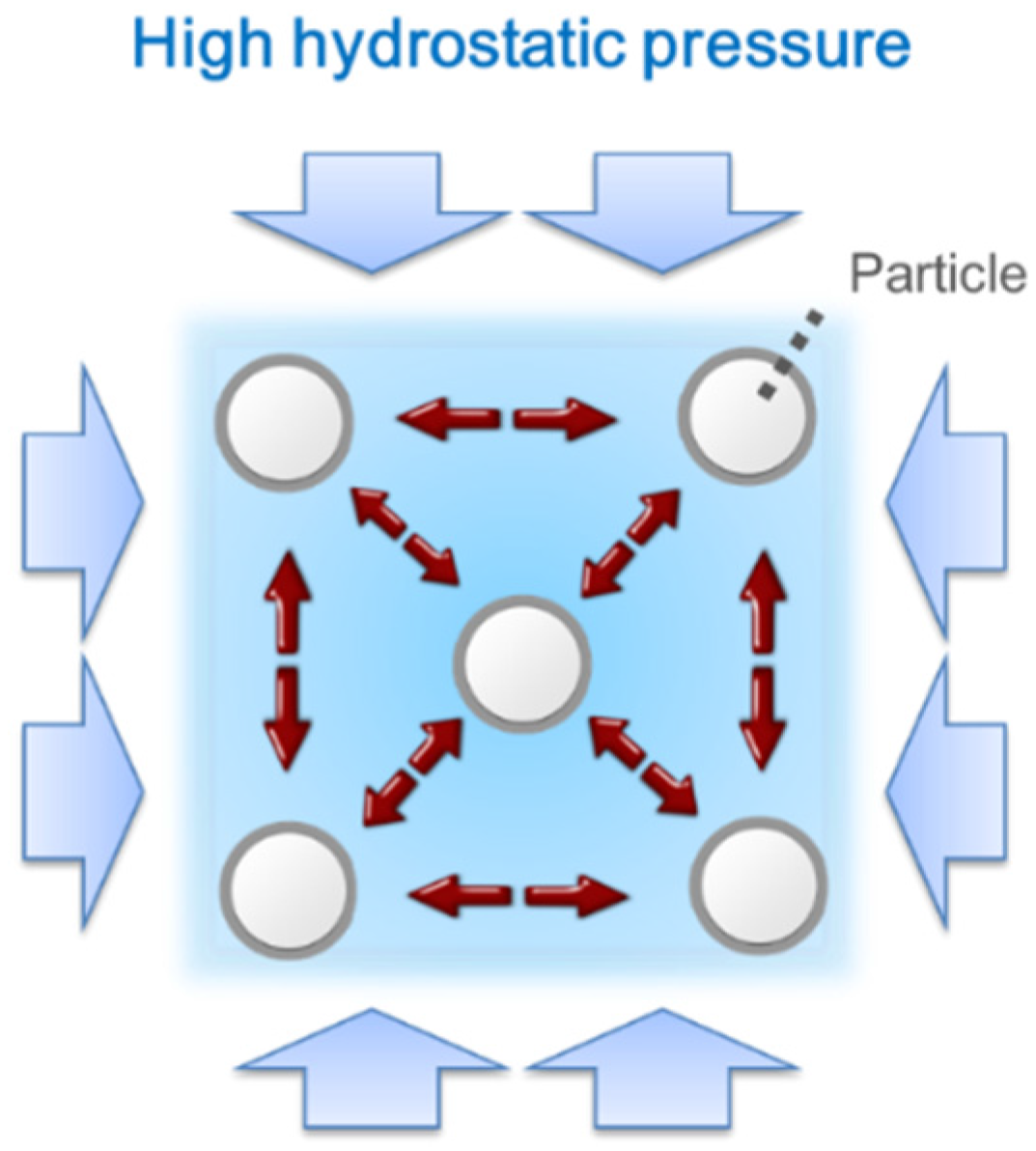
4.1. Microstructural State
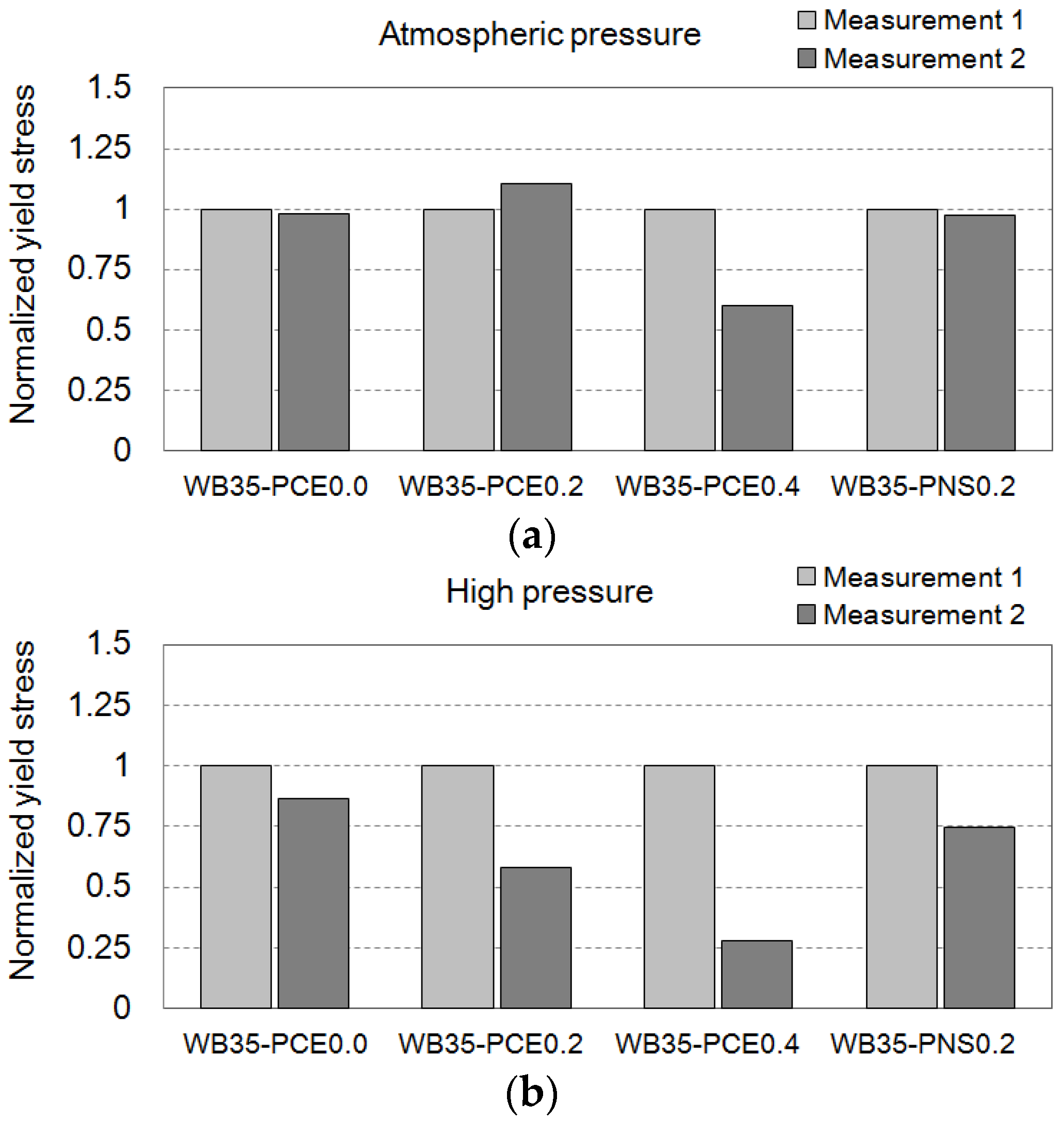
4.2. Effect of Superplasticizer
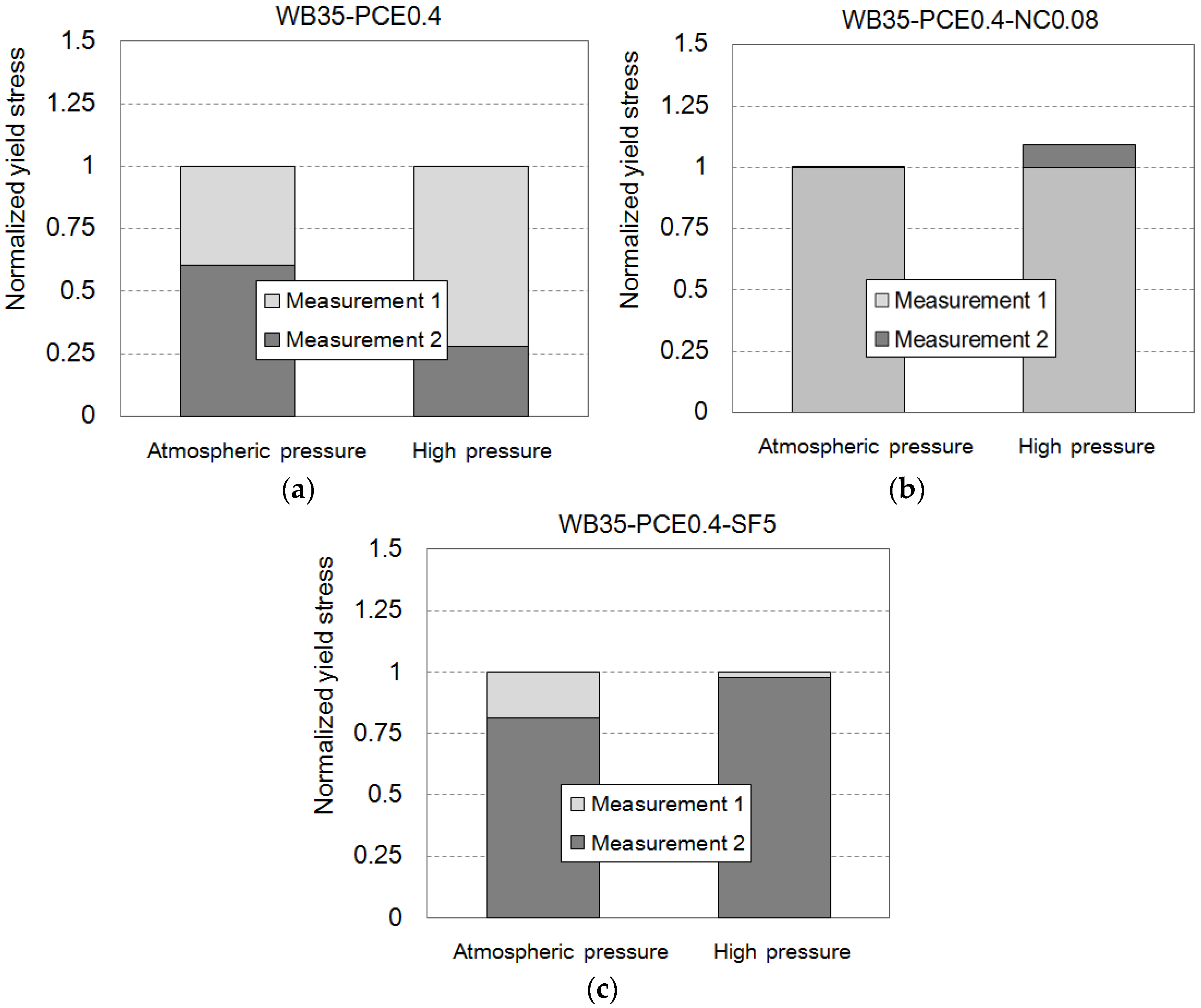
4.3. Effect of Nanoclay and Silica Fume
4.4. Effect of Supplementary Cementitious Materials
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Guptill, N.R.; Akers, D.J.; Kelsey, R.A.; Pierce, J.S.; Bognacki, C.; King, J.C.; Reinhart, P.E. Placing Concrete by Pumping Methods; American Concrete Institute: Farmington Hills, MI, USA, 1998; p. 25. [Google Scholar]
- Recommendations for Practices of Placing Concrete by Pumping Methods; Architectural Institute of Japan: Tokyo, Japan, 2009; p. 266. (In Japanese)
- Kwon, S.H.; Park, C.K.; Jeong, J.H.; Jo, S.D.; Lee, S.H. Prediction of Concrete Pumping: Part I—Development of New Tribometer for Analysis of Lubricating Layer. ACI Mater. J. 2013, 110, 647–655. [Google Scholar]
- Kwon, S.H.; Park, C.K.; Jeong, J.H.; Jo, S.D.; Lee, S.H. Prediction of Concrete Pumping: Part II—Analytical Prediction and Experimental Verification. ACI Mater. J. 2013, 110, 657–667. [Google Scholar]
- Ahmed, R.M.; Takach, N.E.; Khan, U.M.; Taoutaou, S.; James, S.; Saasen, A.; Godøy, R. Rheology of foamed cement. Cem. Concr. Res. 2009, 39, 353–361. [Google Scholar] [CrossRef]
- Rosquoët, F.; Alexis, A.; Khelidj, A.; Phelipot, A. Experimental study of cement grout: Rheological behavior and sedimentation. Cem. Concr. Res. 2003, 33, 713–722. [Google Scholar] [CrossRef]
- Kaplan, D.; de Larrard, F.; Sedran, T. Design of Concrete Pumping Circuit. ACI Mater. J. 2005, 102, 110–117. [Google Scholar]
- Feys, D.; Khayat, K.H.; Perez-Schell, A.; Khatib, R. Development of a tribometer to characterize lubrication layer properties of self-consolidating concrete. Cem. Concr. Compos. 2014, 54, 40–52. [Google Scholar] [CrossRef]
- Ko, J.H.; Moon, H.J.; Seok, W.K.; Park, S.J. A Study on the 1:1 Full Scale Core Wall Mock-up Test of High Strength Concrete Performed by Testing Pumpability (in Korean). J. Architec. Inst. Korea 2008, 24, 203–210. [Google Scholar]
- Ouchi, M.; Sakue, J. Self-Compactability of Fresh Concrete in Terms of Dispersion and Coagulation of Particles of Cement Subject to Pumping. In Proceedings of the 3rd North American Conference on the Design and Use of Self-Consolidating Concrete, Chicago, IL, USA, 10–12 November 2008.
- Kim, Y.G.; Kim, S.H.; Ji, S.W.; Yoo, T.D.; Seo, C.H. A Study on the Properties Variation of Concrete According to Pumping on the Field Placing. In Proceedings of the Semi-Annual Conference of Architectural Institute of Korea, Kangwon, Korea, 29–30 October 2004. (In Korean)
- Feys, D.; de Schutter, G.; Verhoeven, R. Parameters influencing pressure during pumping of self-compacting concrete. Mater. Struct. 2013, 46, 533–555. [Google Scholar] [CrossRef]
- Kim, J.H.; Beacraft, M.; Shah, S.P. Effect of mineral admixtures on formwork pressure of self-consolidating concrete. Cem. Concr. Compos. 2010, 32, 665–671. [Google Scholar] [CrossRef]
- Kawashima, S.; Kim, J.H.; Corr, D.J.; Shah, S.P. Study of the mechanisms underlying the fresh-state response of cementitious materials modified with nanoclays. Constr. Build. Mater. 2012, 36, 749–757. [Google Scholar] [CrossRef]
- Ferron, R.P.; Gregori, A.; Sun, Z.; Shah, S.P. Rheological Method to Evaluate Structural Buildup in Self-Consolidating Concrete Cement Pastes. ACI Mater. J. 2007, 104, 242–250. [Google Scholar]
- Kim, J.H.; Jang, H.R.; Yim, H.J. Sensitivity and accuracy for rheological simulation of cement-based materials. Comput. Concr. 2015, 6, 903–919. [Google Scholar] [CrossRef]
- Krieger, I.M.; Dougherty, T.J. A Mechanism for Non-Newtonian Flow in Suspensions of Rigid Spheres. J. Rheol. 1959, 3, 137–152. [Google Scholar] [CrossRef]
- Struble, L.; Sun, G.K. Viscosity of Portland cement paste as a function of concentration. Adv. Cem. Based Mater. 1995, 2, 62–69. [Google Scholar] [CrossRef]
- Wildemuth, C.R.; Williams, M.C. Viscosity of suspensions modeled with a shear-dependent maximum packing fraction. Rheol. Acta 1984, 23, 627–635. [Google Scholar] [CrossRef]
- Yim, H.J.; Kim, J.H.; Shah, S.P. Cement particle flocculation and breakage monitoring under Couette flow. Cem. Concr. Res. 2013, 53, 36–43. [Google Scholar] [CrossRef]
- Flatt, R.J.; Bowen, P. Yodel: A yield stress model for suspensions. J. Am. Ceram. Soc. 2006, 89, 1244–1256. [Google Scholar] [CrossRef]
- Kashani, A.; San Nicolas, R.; Qiao, G.G.; van Deventer, J.S.; Provis, J.L. Modelling the yield stress of ternary cement–slag–fly ash pastes based on particle size distribution. Powder Tech. 2014, 266, 203–209. [Google Scholar] [CrossRef]
- Ferraris, C.F.; Obla, K.H.; Hill, R. The influence of mineral admixtures on the rheology of cement paste and concrete. Cem. Concr. Res. 2001, 31, 245–255. [Google Scholar] [CrossRef]
- Tregger, N.A.; Pakula, M.E.; Shah, S.P. Influence of clays on the rheology of cement pastes. Cem. Concr. Res. 2010, 40, 384–391. [Google Scholar] [CrossRef]
- Bentz, D.P.; Ferraris, C.F.; Galler, M.A.; Hansen, A.S.; Guynn, J.M. Influence of particle size distributions on yield stress and viscosity of cement–fly ash pastes. Cem. Concr. Res. 2012, 42, 404–409. [Google Scholar] [CrossRef]
- Coussot, P.; Ancey, C. Rheophysical classification of concentrated suspensions and granular pastes. Phys. Rev. E 1999, 59, 4445–4457. [Google Scholar] [CrossRef]
| Label | w/b [%] | Superplasticizer [%] | Nanoclay [%] | Silica Fume [%] | Fly Ash [%] | GGBFS [%] |
|---|---|---|---|---|---|---|
| WB35-PCE0.0 | 35 | - | - | - | - | - |
| WB35-PCE0.2 | 35 | 0.2 (PCE) | - | - | - | - |
| WB35-PCE0.4 | 35 | 0.4 (PCE) | - | - | - | - |
| WB35-PNS0.2 | 35 | 0.2 (PNS) | - | - | - | - |
| WB35-PCE0.4 | 35 | 0.4 (PCE) | - | - | - | - |
| WB35-PCE0.4-NC0.08 | 35 | 0.4 (PCE) | 0.08 | - | - | - |
| WB35-PCE0.4-SF5 | 35 | 0.4 (PCE) | - | 5 | - | - |
| WB35-FA10 | 35 | - | - | - | 10 | - |
| WB35-FA20 | 35 | - | - | - | 20 | - |
| WB35-FA30 | 35 | - | - | - | 30 | - |
| WB35-BS10 | 35 | - | - | - | - | 10 |
| WB35-BS20 | 35 | - | - | - | - | 20 |
| WB35-BS30 | 35 | - | - | - | - | 30 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yim, H.J.; Kim, J.H.; Kwon, S.H. Effect of Admixtures on the Yield Stresses of Cement Pastes under High Hydrostatic Pressures. Materials 2016, 9, 147. https://doi.org/10.3390/ma9030147
Yim HJ, Kim JH, Kwon SH. Effect of Admixtures on the Yield Stresses of Cement Pastes under High Hydrostatic Pressures. Materials. 2016; 9(3):147. https://doi.org/10.3390/ma9030147
Chicago/Turabian StyleYim, Hong Jae, Jae Hong Kim, and Seung Hee Kwon. 2016. "Effect of Admixtures on the Yield Stresses of Cement Pastes under High Hydrostatic Pressures" Materials 9, no. 3: 147. https://doi.org/10.3390/ma9030147
APA StyleYim, H. J., Kim, J. H., & Kwon, S. H. (2016). Effect of Admixtures on the Yield Stresses of Cement Pastes under High Hydrostatic Pressures. Materials, 9(3), 147. https://doi.org/10.3390/ma9030147






