Optimising Embodied Carbon in Axial Tension Piles: A Comparative Study of Concrete, Steel, and Timber Piles Using a Hybrid Genetic Approach
Abstract
1. Introduction
1.1. Background
1.2. Aims and Objectives
- Introduce a robust optimisation algorithm that is capable of producing pile designs with the lowest embodied carbon for different soil conditions and pile capacities.
- Deploy the optimisation algorithm to discover the optimal design of six different pile types; concrete solid, concrete hollow, steel pipe, steel universal column (UC) sections, timber circular, and timber square, in a range of common soil types.
- Compare the characteristics of optimal tension piles and optimal compression piles, in order to provide generalised design guidance.
- Apply the optimisation algorithm to an existing case study to assess the potential carbon savings for a built structure, to support future endeavours.
1.3. Analysis Setup
- (a)
- Deploy the optimisation algorithm to produce optimised tension piles in undrained clay soil: Concrete, steel, and timber piles with capacities up to 3 MN were designed for use in undrained clay conditions. The optimal design parameters for each material type were determined and compared.
- (b)
- Deploy the optimisation algorithm to produce optimised tension piles in loose sand: Concrete, steel, and timber piles were designed for capacities up to 3 MN in loose sand conditions, and the optimal design parameters were compared.
- (c)
- Comparative analysis: A broad discussion is provided comparing the optimal design options for compression and tension piles in different soil types.
- (d)
- Case study: A real-world case study of an existing tension pile design is presented in detail. The parameters from the built piles were fed into the optimisation algorithm to generate an alternative optimal pile design.
2. Methodology
2.1. Pile Capacities and Soil Types
- Structural capacity: the pile resistance as a structural element subjected to pure tensile stresses is within a safe limit.
- Geotechnical capacity: the factored pile’s frictional resistance is less than the applied tensile load.
2.1.1. Structural Capacity
- -
- = concrete tensile capacity, steel tensile capacity, and timber tensile capacity.
- -
- and = steel cross-sectional area and timber cross-sectional area
- -
- = characteristic compressive strength of timber and steel.
- -
- = partial factor for timber strength = 1.3 [43]
- -
- = partial factor for steel strength = 1.3 [43]
- -
- n = reduction factor for timber class 3, submerged in water = 0.8 [42]
2.1.2. Geotechnical Capacity
- -
- , , and = the pile’s length, diameter, and radius.
- -
- , G, and E = Poisson’s ratio, shear modulus of elasticity, and Young’s modulus of elasticity.
- -
- and = soil effective stress and pile–soil friction angle.
- -
- = the average shear stress (shaft friction) mobilised along the pile shaft in sand and clay soils.
- -
- = soil and pile friction coefficients.
- -
- α = adhesion factor for undrained clay.
2.1.3. Tested Soil Types
2.2. Embodied Carbon Model
2.2.1. LCA Approach
- -
- TEC = total embodied carbon (kgCO2e)
- -
- = mass of the construction material (kg)
- -
- = embodied carbon factor for a given material (kgCO2e/kg), as shown in Table 2.
2.2.2. Embodied Carbon Factors
2.3. The Optimisation Algorithm
2.3.1. Algorithm Definition
- In the first stage, the GA explored the full design space using a large initial population (500 individuals) and standard genetic operators (uniform selection, arithmetic crossover, Gaussian mutation). The design space was defined by various design variables with bounds shown in Table 3, corresponding to a multi-dimensional design space with approximately 106 potential design combinations. Range partitions were not discretised a priori but sampled continuously within the GA framework.
- Once the GA converged to a promising solution after 50 stall generations, a refined local search was applied using MATLAB’s fmincon solver, which performed constrained nonlinear optimisation. This step improved the local accuracy of the solution by fine-tuning within a narrower design range around the best GA result.
2.3.2. Section Constraints
3. Results and Discussion
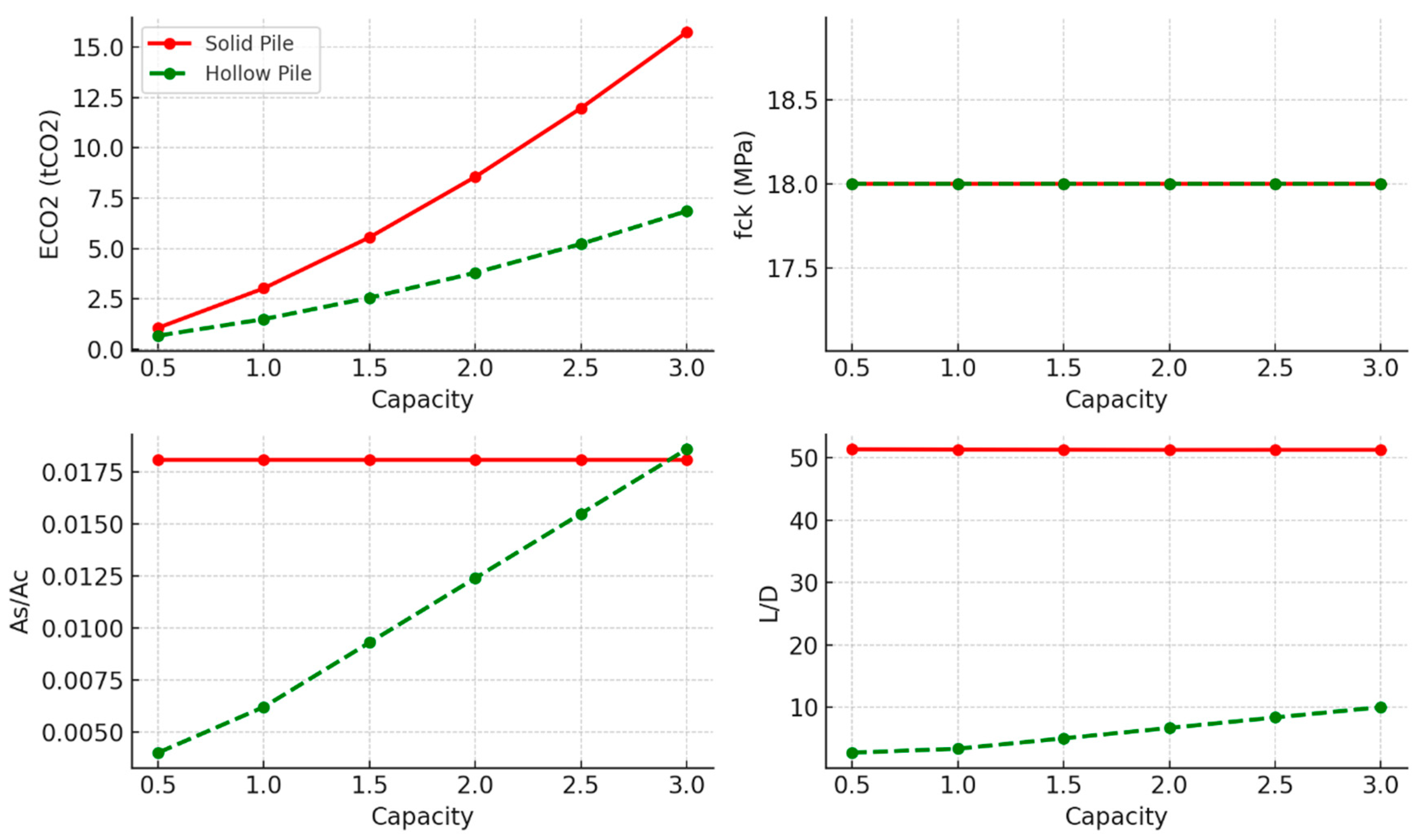
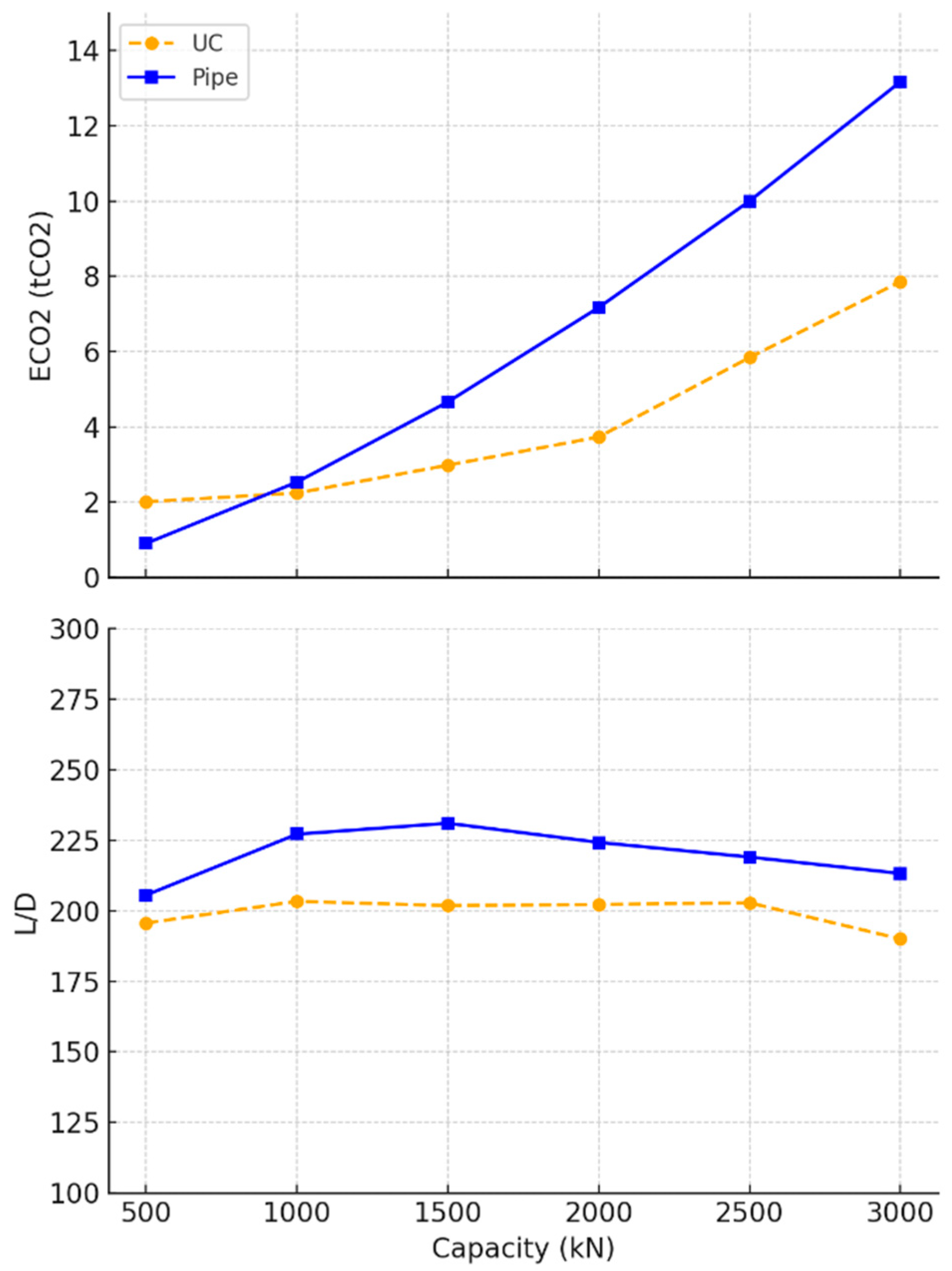
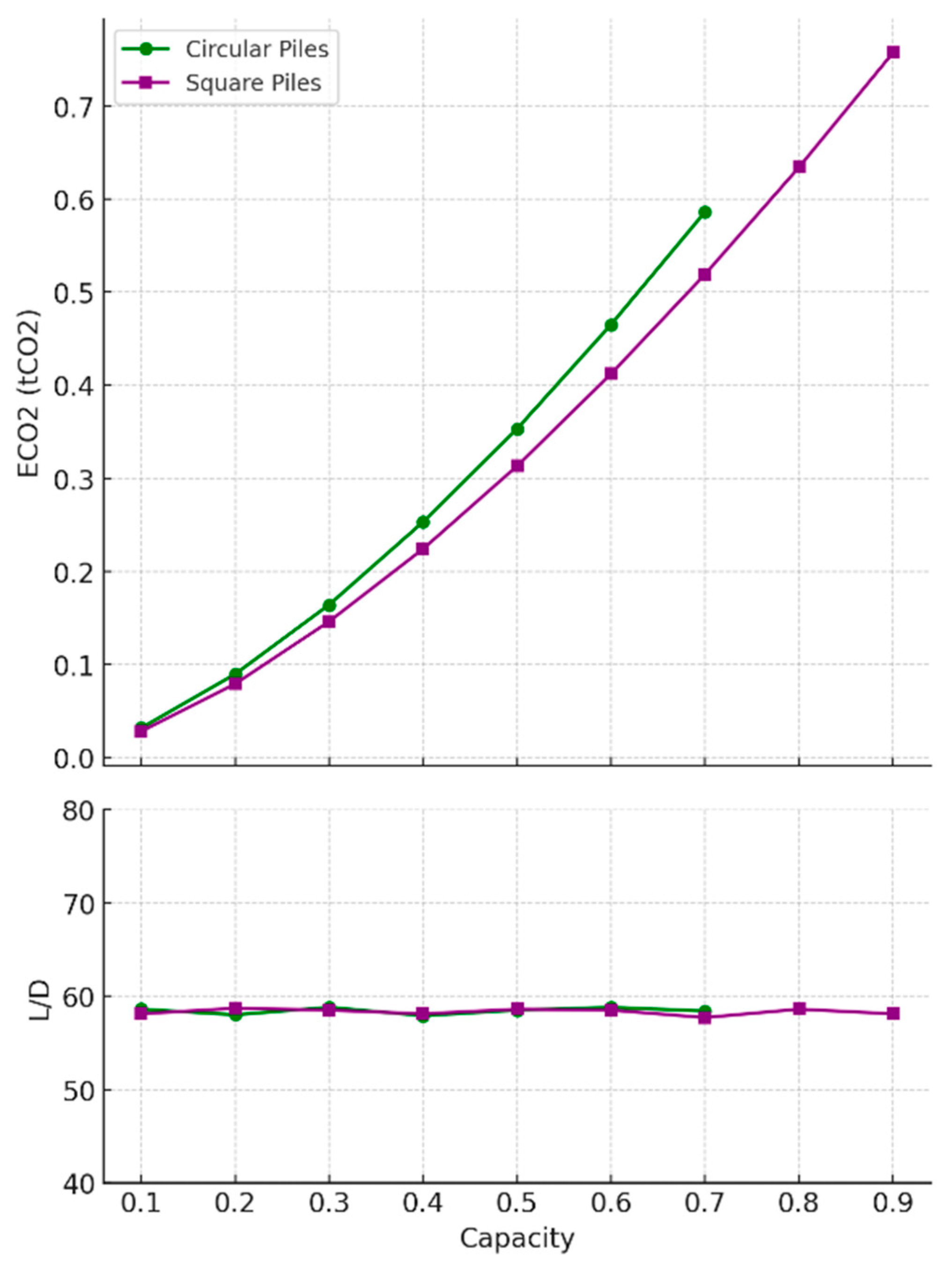
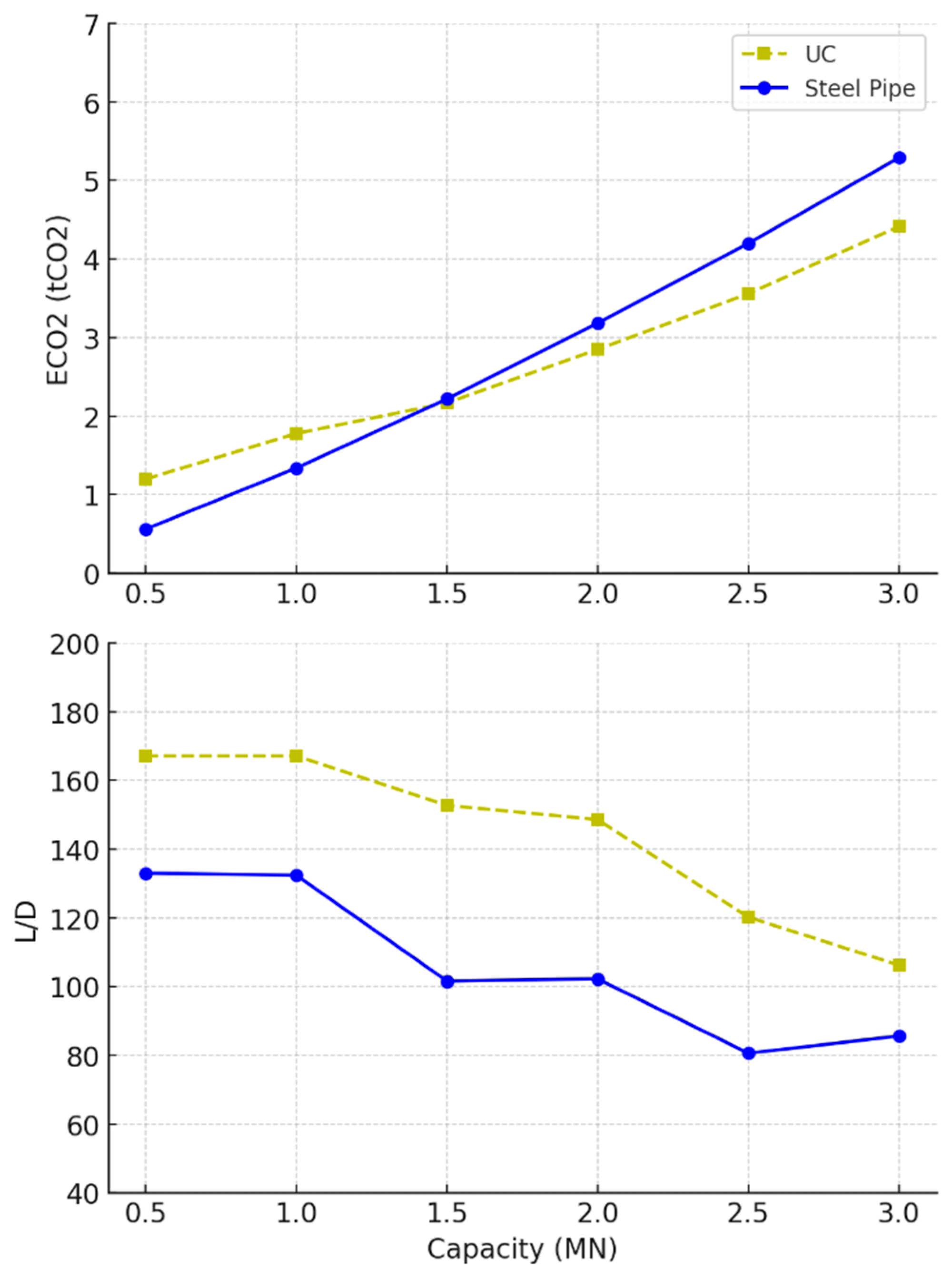
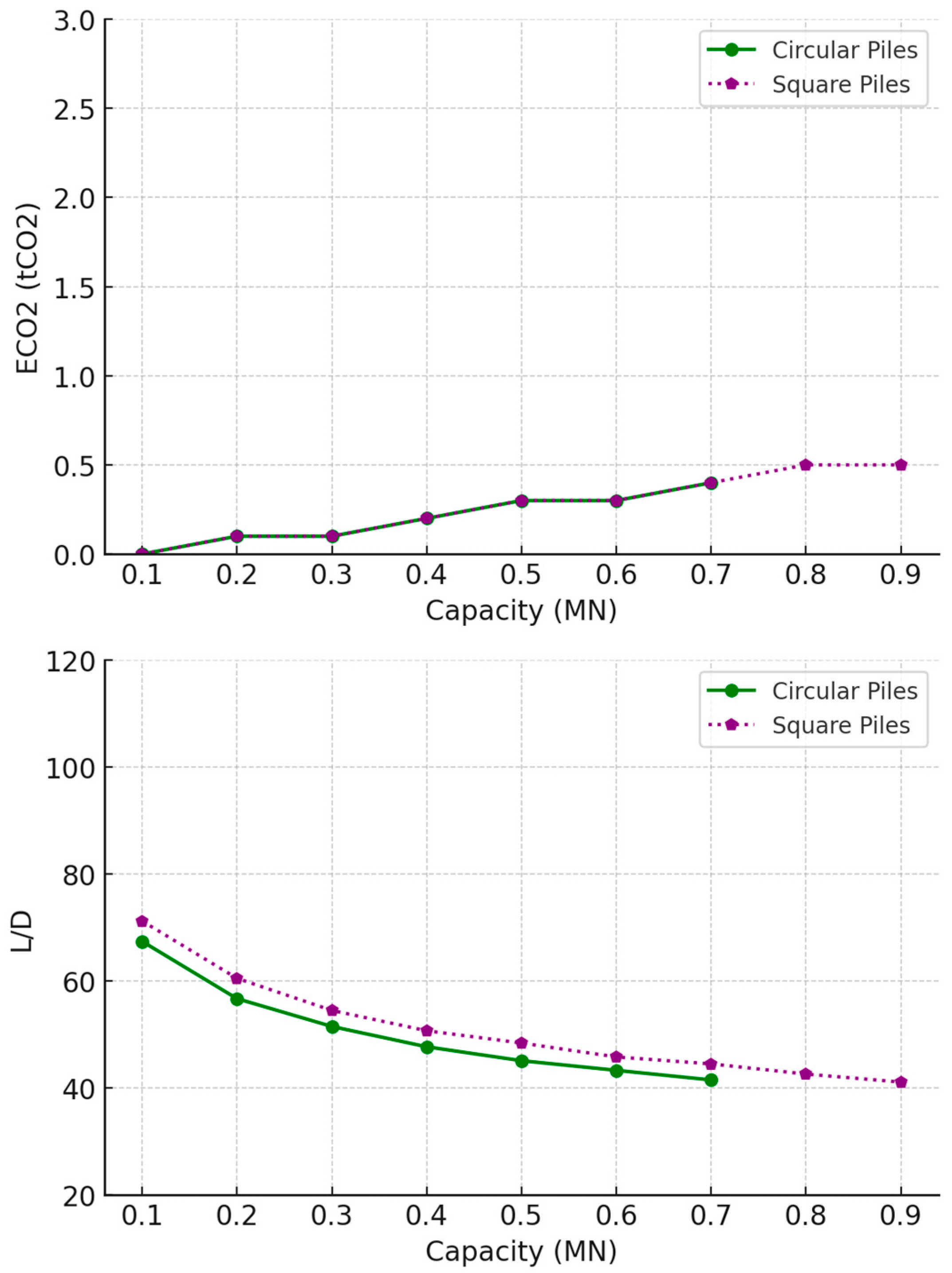
3.1. Undrained Clay Soil
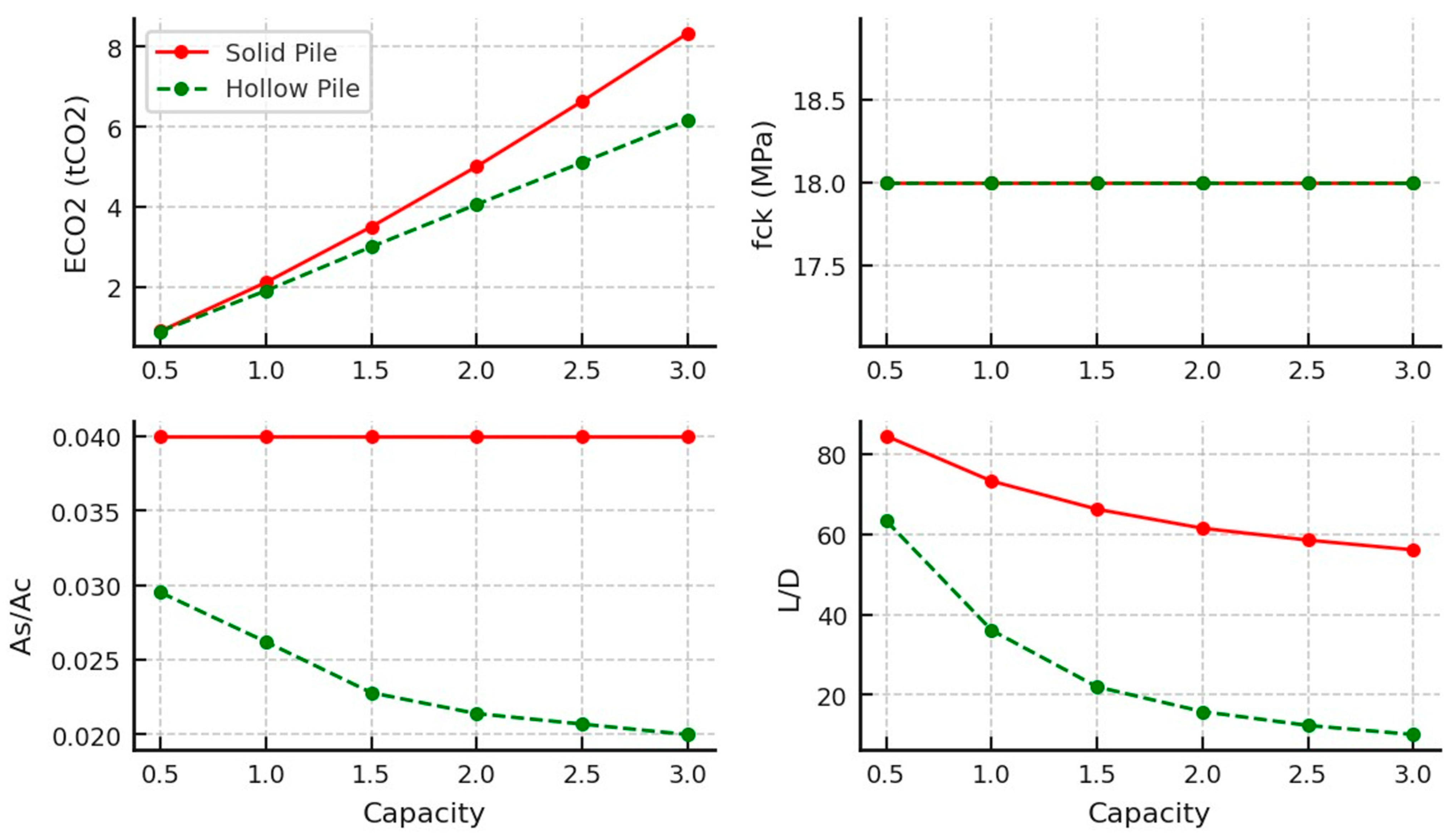
3.2. Sand Soil
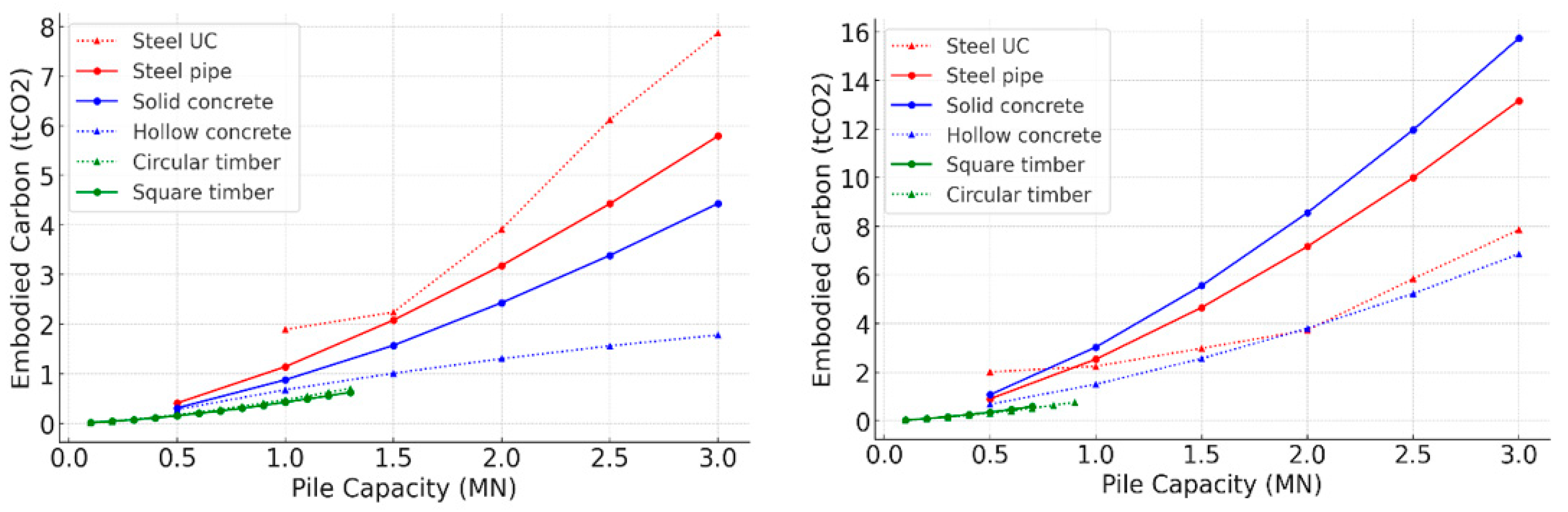
3.3. Tension vs. Compression Piles
3.3.1. Tension vs. Compression Piles in Undrained Clay
3.3.2. Tension vs. Compression Piles in Loose Sand
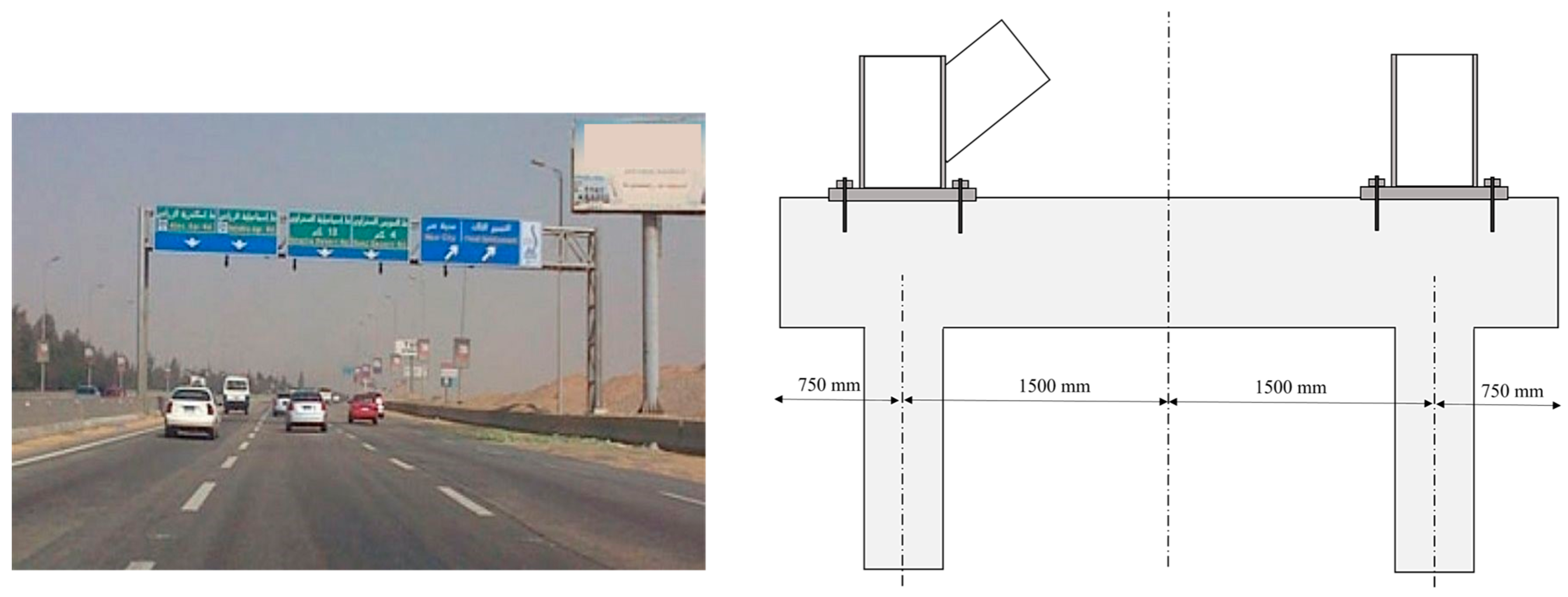
4. Case Study
4.1. Case Description
4.2. Soil Profile and Pile Design
4.3. Pile Optimisation Results and Discussion
- Availability of timber piles: timber piles were shown to be the most sustainable option in this study, especially for lower-capacity pile designs. However, it is crucial to recognise that timber sections are not universally accessible across all regions. In countries with limited forest resources, especially those with arid climates, the availability of piling-grade timber can be a significant limitation [65]. Furthermore, timber prices and logistical challenges associated with transportation and procurement may limit the feasibility of their widespread adoption, especially in regions where alternative materials, such as concrete or steel, are more readily available and economically viable. Another issue remains the unsuitability of timber sections for soils with changing GWT levels, as timber becomes vulnerable to decomposition and loss of strength.
- Industry practices: feedback from contractors reveals that many companies typically adopt off-the-shelf pile designs, with standardised section dimensions and reinforcement specifications. These designs are often conservative to account for the uncertainties associated with varying soil conditions and profiles across different locations. While this approach enhances the robustness of pile designs, it presents a challenge to the adoption of sustainability-focused designs during the early stages of project planning. The industry’s reliance on conservative designs may hinder the transition to more sustainable practices, particularly when optimising for embodied carbon reduction.
- Culture: social factors also play a critical role in the selection of piling solutions. However, social acceptance is difficult to quantify and can vary significantly between regions, clients, and contractors. What is deemed an acceptable or preferable solution in one geographical area or by one contractor may not be viewed similarly elsewhere. This subjectivity adds another layer of complexity when implementing sustainable design options, as local preferences and perceptions can significantly impact decision-making.
- Uncertainties: the embodied carbon of structures varies across different locations due to regional disparities in transportation logistics, material sourcing, and availability. In countries with longer supply chains or limited local resources, higher emissions may result from transporting materials over greater distances, while countries with abundant local materials can reduce embodied carbon significantly. The embodied carbon outcomes presented in this study are sensitive to the emission factors applied, particularly for materials like steel, where variability across product types and production routes is significant. While our primary findings are robust—especially those related to geometric efficiency and optimisation logic—the relative ranking of designs with high steel content may shift under different carbon factor assumptions. We acknowledge this uncertainty and encourage sensitivity testing for project-specific applications.
5. Conclusions
- Tension piles in undrained clay were shown to be more emitting than those in loose sand. This is believed to be due to the nature of sandy soil, which exhibits higher surface friction resistance than undrained clay, a main factor that influences the capacity of tension piles.
- Regarding undrained clay, the optimisation results indicate that while timber piles are restricted to lower capacity applications, square designs exhibit slightly superior environmental performance, though the difference between square and circular sections remains minimal. Hollow concrete piles and UC steel sections demonstrated greater material efficiency and sustainability compared with solid concrete and steel pipe piles, particularly in terms of reducing embodied carbon. The observed efficiency of hollow concrete piles aligns with findings reported in the literature [66].
- In loose sand, timber piles, though limited to lower capacities, are the most sustainable options for low-capacity piles, with minimal differences in embodied carbon and L/D ratios between circular and square designs. Both concrete and steel piles exhibited more compact, material-efficient designs due to steeper declines in their optimal L/D ratios compared with the designs for use on undrained clay. Regarding high-capacity piles, UC steel piles outperformed other pile types in terms of embodied carbon.
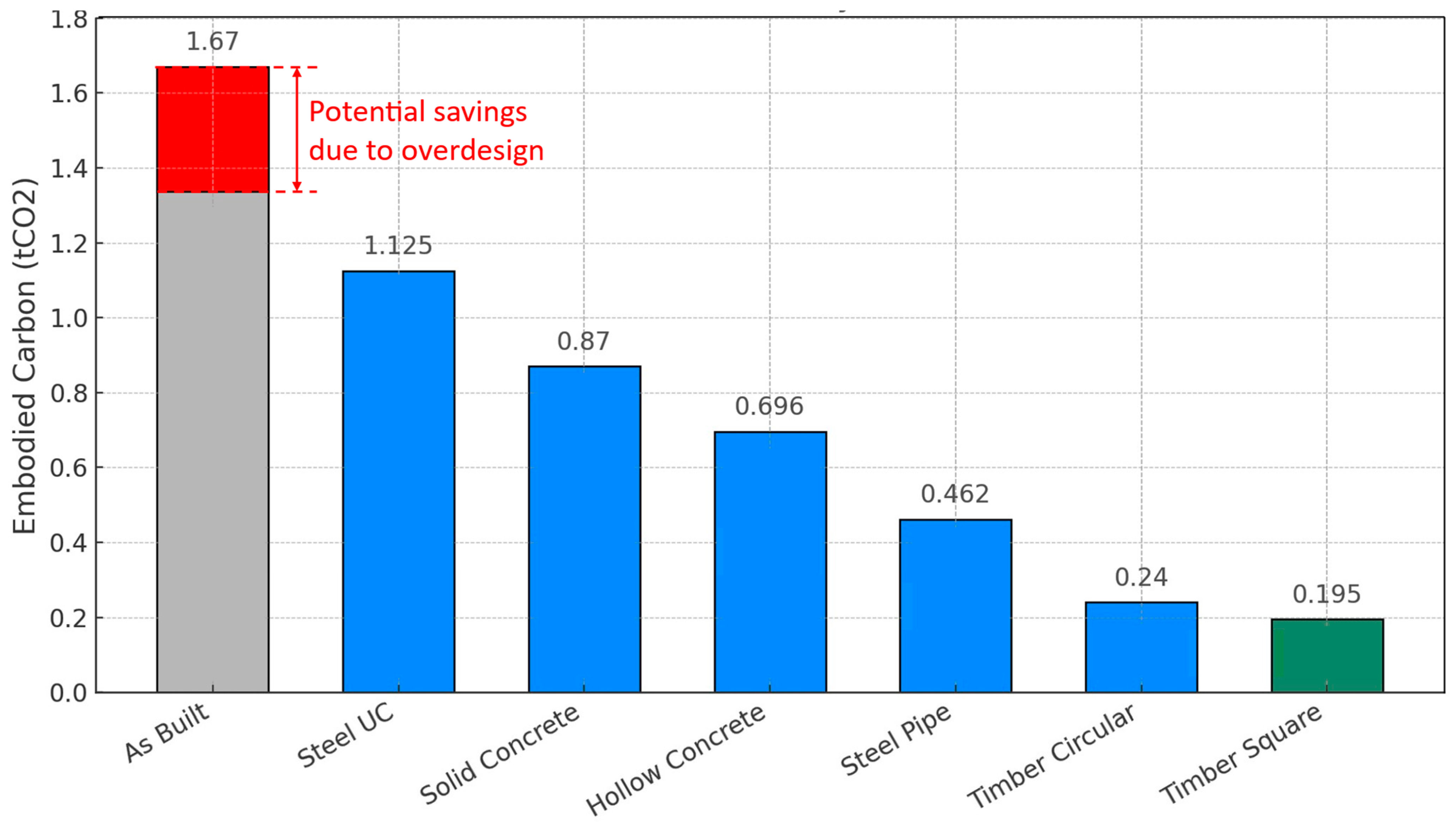
- The optimisation tool was applied to an existing case study for a large crossroad signpost and demonstrated significant potential for reducing the embodied carbon in pile foundations, with timber, steel, and hollow concrete piles offering substantial carbon savings. However, practical challenges such as the limited availability of timber, conservative industry practices, and varying social acceptance across regions must be addressed to facilitate the widespread adoption of these sustainable designs in real-world construction.
- The findings of this study are specific to the selected input soil properties, and variations in soil types may yield different optimal pile designs, as demonstrated in the case study. Nonetheless, the conceptual optimisation technique employed remains applicable across diverse soil conditions, offering flexibility and adaptability for future design scenarios. Designers are therefore encouraged to adapt the proposed optimisation approach to their local contexts and materials data rather than directly relying on the specific findings presented in this paper.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- United Nations (UN) Report Buildings and Construction Sector—Key Facts and Figures. Available online: https://www.un.org/ (accessed on 2 May 2025).
- Hong, T.; Ji, C.; Jang, M.; Park, H. Assessment Model for Energy Consumption and Greenhouse Gas Emissions during Building Construction. J. Manag. Eng. 2014, 30, 226–235. [Google Scholar] [CrossRef]
- Regona, M.; Yigitcanlar, T.; Hon, C.; Teo, M. Artificial Intelligence and Sustainable Development Goals: Systematic Literature Review of the Construction Industry. Sustain. Cities Soc. 2024, 105499. [Google Scholar] [CrossRef]
- Gauch, H.L.; Hawkins, W.; Ibell, T.; Allwood, J.M.; Dunant, C.F. Carbon vs. Cost Option Mapping: A Tool for Improving Early-Stage Design Decisions. Autom. Constr. 2022, 136, 104178. [Google Scholar] [CrossRef]
- Fang, D.; Brown, N.; De Wolf, C.; Mueller, C. Reducing Embodied Carbon in Structural Systems: A Review of Early-Stage Design Strategies. J. Build. Eng. 2023, 76, 107054. [Google Scholar] [CrossRef]
- Sahab, M.G.; Ashour, A.F.; Toropov, V.V. Cost Optimisation of Reinforced Concrete Flat Slab Buildings. Eng. Struct. 2005, 27, 313–322. [Google Scholar] [CrossRef]
- Faddoul, Y.; Sirivivatnanon, V. Sustainable Concrete Structures by Optimising Structural and Concrete Mix Design. Aust. J. Struct. Eng. 2025, 26, 1–9. [Google Scholar] [CrossRef]
- Jayasinghe, A.; Orr, J.; Ibell, T.; Boshoff, W.P. Minimising Embodied Carbon in Reinforced Concrete Beams. Eng. Struct. 2021, 242, 112590. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, X. Sustainable Design of Reinforced Concrete Structural Members Using Embodied Carbon Emission and Cost Optimization. J. Build. Eng. 2021, 44, 102940. [Google Scholar] [CrossRef]
- Georgiou, E.; Raffin, M.; Colquhoun, K.; Borrion, A.; Campos, L.C. The Significance of Measuring Embodied Carbon Dioxide Equivalent in Water Sector Infrastructure. J. Clean. Prod. 2019, 216, 268–276. [Google Scholar] [CrossRef]
- Berndt, M.L. Influence of Concrete Mix Design on CO2 Emissions for Large Wind Turbine Foundations. Renew. Energy 2015, 83, 608–614. [Google Scholar] [CrossRef]
- Zolotuchin, S.N.; Novikova, K.K.; Kim, M.S. New Method for the Construction of Pile Foundations in Low-Rise Constructions Using Micro-Piles in the Knocked-out Wells. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2018. [Google Scholar]
- Kayabekir, A.E.; Arama, Z.A.; Bekdaş, G.; Nigdeli, S.M.; Geem, Z.W. Eco-Friendly Design of Reinforced Concrete Retaining Walls: Multi-Objective Optimization with Harmony Search Applications. Sustainability 2020, 12, 6087. [Google Scholar] [CrossRef]
- Balasbaneh, A.T.; Marsono, A.K. Applying Multi-Criteria Decision-Making on Alternatives for Earth-Retaining Walls: LCA, LCC, and S-LCA. Int. J. Life Cycle Assess. 2020, 25, 2140–2153. [Google Scholar] [CrossRef]
- Abushama, K.; Hawkins, W.; Pelecanos, L.; Ibell, T. Minimising the Embodied Carbon of Reinforced Concrete Piles Using a Multi-Level Modelling Tool with a Case Study. Structures 2023, 58, 105476. [Google Scholar] [CrossRef]
- Abushama, K.; Hawkins, W.; Pelecanos, L.; Ibell, T. Embodied Carbon Optimisation of Concrete Pile Foundations and Comparison of the Performance of Different Pile Geometries. Eng. Struct. 2024, 310, 118109. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, F.; Jia, S. Embodied Energy and Carbon Emissions Analysis of Geosynthetic Reinforced Soil Structures. J. Clean. Prod. 2022, 370, 133510. [Google Scholar] [CrossRef]
- Yazdani, F.; AliPanahi, P.; Sadeghi, H. A Comparative Study of Environmental and Economic Assessment of Vegetation-Based Slope Stabilization with Conventional Methods. J. Environ. Manag. 2024, 359, 121002. [Google Scholar] [CrossRef]
- Sandanayake, M.; Zhang, G.; Setunge, S. Environmental Emissions at Foundation Construction Stage of Buildings: Two Case Studies. Build. Environ. 2016, 95, 189–198. [Google Scholar] [CrossRef]
- Teodosio, B.; Wasantha, P.L.; Yaghoubi, E.; Guerrieri, M.; Fragomeni, S.; van Staden, R. Environmental, Economic, and Serviceability Attributes of Residential Foundation Slabs: A Comparison between Waffle and Stiffened Rafts Using Multi-Output Deep Learning. J. Build. Eng. 2023, 80, 107983. [Google Scholar] [CrossRef]
- Danziger, F.A.; Danziger, B.R.; Pacheco, M.P. The Simultaneous Use of Piles and Prestressed Anchors in Foundation Design. Eng. Geol. 2006, 87, 163–177. [Google Scholar] [CrossRef]
- Zhou, J.J.; Gong, X.N.; Wang, K.H.; Zhang, R.H.; Yan, J.J. Testing and Modeling the Behavior of Pre-Bored Grouting Planted Piles under Compression and Tension. Acta Geotech. 2017, 12, 1061–1075. [Google Scholar] [CrossRef]
- Dickin, E.A.; Leung, C.F. Performance of Piles with Enlarged Bases Subject to Uplift Forces. Can. Geotech. J. 1990, 27, 546–556. [Google Scholar] [CrossRef]
- Shanker, K.; Basudhar, P.K.; Patra, N.R. Uplift Capacity of Single Piles: Predictions and Performance. Geotech. Geol. Eng. 2007, 25, 151–161. [Google Scholar] [CrossRef]
- Arsoy, S.; Duncan, J.M.; Barker, R.M. Performance of Piles Supporting Integral Bridges. Transp. Res. Rec. 2002, 1808, 162–167. [Google Scholar] [CrossRef]
- Liang, F.; Zheng, H.; Zhang, H. On the Pile Tension Capacity of Scoured Tripod Foundation Supporting Offshore Wind Turbines. Appl. Ocean. Res. 2020, 102, 102323. [Google Scholar] [CrossRef]
- Russo, G. Experimental Investigations and Analysis on Different Pile Load Testing Procedures. Acta Geotech. 2013, 8, 17–31. [Google Scholar] [CrossRef]
- Zhang, G.; Wang, L.; Wang, Y. Pile Reinforcement Mechanism of Soil Slopes. Acta Geotech. 2017, 12, 1035–1046. [Google Scholar] [CrossRef]
- Boulanger, R.W.; Kutter, B.L.; Brandenberg, S.J.; Singh, P.; Chang, D. Pile Foundations in Liquefied and Laterally Spreading Ground during Earthquakes: Centrifuge Experiments & Analyses; University of California, Center for Geotechnical Modeling: Davis, CA, USA, 2003. [Google Scholar]
- Rainer, J. Accuracy of Pile Capacity Assessment on the Basis of Piling Reports. E3S Web Conf. 2019, 97, 04029. [Google Scholar] [CrossRef]
- Lacasse, S.; Nadim, F.; Langford, T.; Knudsen, S.; Yetginer, G.L.; Guttormsen, T.R.; Eide, A. Model Uncertainty in Axial Pile Capacity Design Methods. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 6 May 2013; p. OTC-24066. [Google Scholar]
- Bueno Aguado, M.; Escolano Sánchez, F.; Sanz Pérez, E. Model Uncertainty for Settlement Prediction on Axially Loaded Piles in Hydraulic Fill Built in Marine Environment. J. Mar. Sci. Eng. 2021, 9, 63. [Google Scholar] [CrossRef]
- Levacher, D.R.; Sieffert, J.G. Tests on Model Tension Piles. J. Geotech. Eng. 1984, 110, 1735–1748. [Google Scholar] [CrossRef]
- Alawneh, A. Tension Piles in Sand: A Method Including Degradation of Shaft Friction during Pile Driving. Transp. Res. Rec. 1999, 1663, 41–49. [Google Scholar] [CrossRef]
- Fazeres-Ferradosa, T.; Chambel, J.; Taveira-Pinto, F.; Rosa-Santos, P.; Taveira-Pinto, F.V.; Giannini, G.; Haerens, P. Scour Protections for Offshore Foundations of Marine Energy Harvesting Technologies: A Review. J. Mar. Sci. Eng. 2021, 9, 297. [Google Scholar] [CrossRef]
- Ikbarieh, A.; Izadifar, M.; Abu-Farsakh, M.Y.; Voyiadjis, G.Z. A Parametric Study of Embankment Supported by Geosynthetic Reinforced Load Transfer Platform and Timber Piles Tip on Sand. Transp. Geotech. 2023, 38, 100901. [Google Scholar] [CrossRef]
- Al-Rawabdeh, A.M.; Vinod, J.S.; Liu, M.D.; McCarthy, T.J.; Redwood, S. Large-scale laboratory testing of helical piles: Effect of the shape. Geotech. Geol. Eng. 2024, 42, 1675–1692. [Google Scholar] [CrossRef]
- Abushama, K.; Hawkins, W.; Pelecanos, L.; Ibell, T. Optimising the Embodied Carbon of Laterally Loaded Piles Using a Genetic Algorithm and Finite Element Simulation. Results Eng. 2024, 24, 103188. [Google Scholar] [CrossRef]
- Abushama, K.; Hawkins, W.; Pelecanos, L.; Ibell, T. Optimisation of Embodied Carbon and Construction Cost of Concrete, Steel and Timber Piles. Dev. Built Environ. 2025, 22, 100656. [Google Scholar] [CrossRef]
- BS EN 1992-1-1:2023; British Standards Institution Eurocode 2: Design of Concrete Structures. BSI: London, UK, 2008.
- BS EN 1993; British Standards Institute Eurocode 3—Design of Steel Structures. BSI: London, UK, 2005.
- EN 1995-1-1; British Standards Institute Eurocode 5 Design of Timber Structures: Part 1-1 General Rules and Rules for Buildings. BSI: London, UK, 2004.
- BS EN 1991-1-1; British Standards Institute 1-1: 2002 Eurocode 1: Actions on Structures—General Actions—Densities, Self-Weight, Imposed Loads for Bulidings. BSI: London, UK, 2002.
- Alawneh, A.S.; Husein Malkawi, A.I.; Al-Deeky, H. Tension Tests on Smooth and Rough Model Piles in Dry Sand. Can. Geotech. J. 1999, 36, 746–753. [Google Scholar] [CrossRef]
- Lehane, B.M.; Jardine, R.J.; Bond, A.J.; Frank, R. Mechanisms of Shaft Friction in Sand from Instrumented Pile Tests. J. Geotech. Eng. 1993, 119, 19–35. [Google Scholar] [CrossRef]
- De Nicola, A.; Randolph, M.F. Tensile and Compressive Shaft Capacity of Piles in Sand. J. Geotech. Eng. 1993, 119, 1952–1973. [Google Scholar] [CrossRef]
- Kulhawy, F.H.; Phoon, K.K. Drilled Shaft Side Resistance in Clay Soil to Rock. In Design and Performance of Deep Foundations: Piles and Piers in Soil and Soft Rock; ASCE: Reston, VA, USA, 1993; pp. 172–183. [Google Scholar]
- Phoon, K.K.; Kulhawy, F.H. Characterization of geotechnical variability. Can. Geotech. J. 1999, 36, 612–624. [Google Scholar] [CrossRef]
- Knappett, J.; Craig, R.F. Craig’s Soil Mechanics; CRC Press LLC: Boca Raton, FL, USA, 2012. [Google Scholar]
- Athena Sustainable Materials Institute. Athena Impact Estimator for Buildings [Software], Version 5.4; Athena Sustainable Materials Institute: Ottawa, Canada, 2020.
- Royal Institution of Chartered Surveyors (RICS). Whole Life Carbon Assessment for the Built Environment; RICS: London, UK, 2017. [Google Scholar]
- Gibbons, O.P.; Orr, J.J.; Archer-Jones, C.; Arnold, W.; Green, D. How to Calculate Embodied Carbon; Institution of Structural Engineers ISTRUCTE: London, UK, 2022. [Google Scholar]
- BS EN 15978-1; Sustainability of Construction Works. Assessment of Environmental Performance of Buildings. BSI: London, UK, 2011.
- Hammond, G.P.; Jones, C.I. Embodied Energy and Carbon in Construction Materials. Proc. Proc. Inst. Civ. Eng.-Energy 2008, 161, 87–98. [Google Scholar] [CrossRef]
- Miles, J. Genetic Algorithms for Design. In Advances of Soft Computing in Engineering; Springer: Vienna, Austria, 2010; pp. 1–56. [Google Scholar]
- Kanyilmaz, A.; Tichell, P.R.N.; Loiacono, D. A Genetic Algorithm Tool for Conceptual Structural Design with Cost and Embodied Carbon Optimization. Eng. Appl. Artif. Intell. 2022. [Google Scholar] [CrossRef]
- Feng, C.W.; Liu, L.; Burns, S.A. Using Genetic Algorithms to Solve Construction Time-Cost Trade-off Problems. J. Comput. Civ. Eng. 1997, 11, 184–189. [Google Scholar] [CrossRef]
- Kim, G.H.; Yoon, J.E.; An, S.H.; Cho, H.H.; Kang, K.I. Neural network model incorporating a genetic algorithm in estimating construction costs. Build. Environ. 2004, 39, 1333–1340. [Google Scholar] [CrossRef]
- Aidy, A.; Rady, M.; Mashhour, I.M.; Mahfouz, S.Y. Structural Design Optimization of Flat Slab Hospital Buildings Using Genetic Algorithms. Buildings 2022, 12, 2195. [Google Scholar] [CrossRef]
- Hwang, J.H.; Lyu, Y.D.; Chung, M.C. Optimizing Pile Group Design Using a Real Genetic Approach. In ISOPE International Ocean and Polar Engineering Conference; ISOPE: Mountain View, CA, USA, 2011; pp. 491–499. [Google Scholar]
- Chan, C.M.; Zhang, L.M.; Ng, J.T. Optimization of Pile Groups Using Hybrid Genetic Algorithms. J. Geotech. Geoenvironmental Eng. 2009, 135, 497–505. [Google Scholar] [CrossRef]
- Viguier, J.; Bourreau, D.; Bocquet, J.F.; Pot, G.; Bléron, L.; Lanvin, J.D. Modelling Mechanical Properties of Spruce and Douglas Fir Timber by Means of X-Ray and Grain Angle Measurements for Strength Grading Purpose. Eur. J. Wood Wood Prod. 2017, 75, 527–541. [Google Scholar] [CrossRef]
- Drewniok, M.P.; Azevedo, J.M.; Dunant, C.F.; Allwood, J.M.; Cullen, J.M.; Ibell, T.; Hawkins, W. Mapping Material Use and Embodied Carbon in UK Construction. Resour. Conserv. Recycl. 2023, 197, 107056. [Google Scholar] [CrossRef]
- Steel Construction Institute. Tata Steel Steel Building Design: Design Data. In Accordance with Eurocodes and the UK National Annexes; Steel Construction Institute, Print: Ascot, UK, 2013. [Google Scholar]
- Leefers, L.A.; Vasievich, J.M. Timber Resources and Factors Affecting Timber Availability and Sustainability for Kinross, Michigan. 2010. Available online: http://michiganwoodbiofuels.org/sites/default/files/Attachment%201_Feedstock%20Inventory%20report_v3_01072011.pdf (accessed on 2 May 2025).
- Lalicata, L.M.; Stallebrass, S.E.; McNamara, A.; Panchal, J.P. Design Method for the ‘impression Pile’. Proc. Inst. Civ. Eng.-Geotech. Eng. 2022, 175, 75–85. [Google Scholar] [CrossRef]

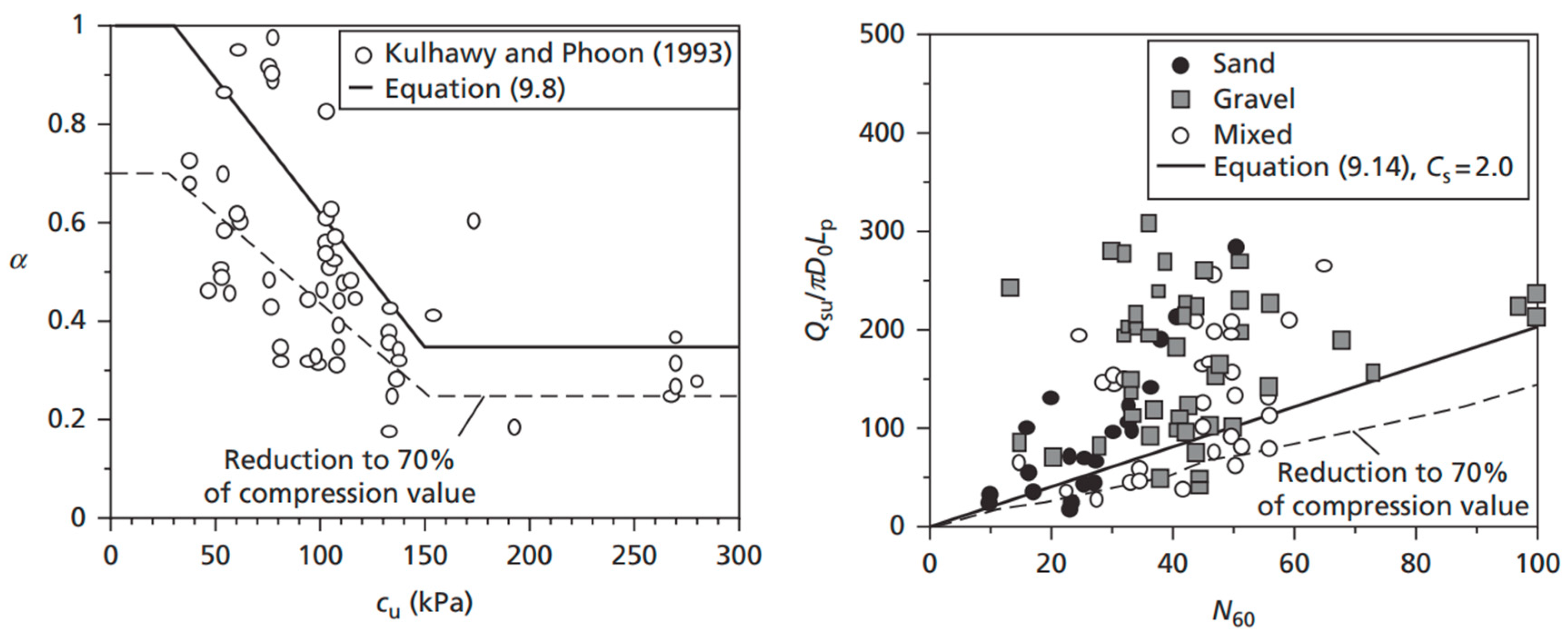
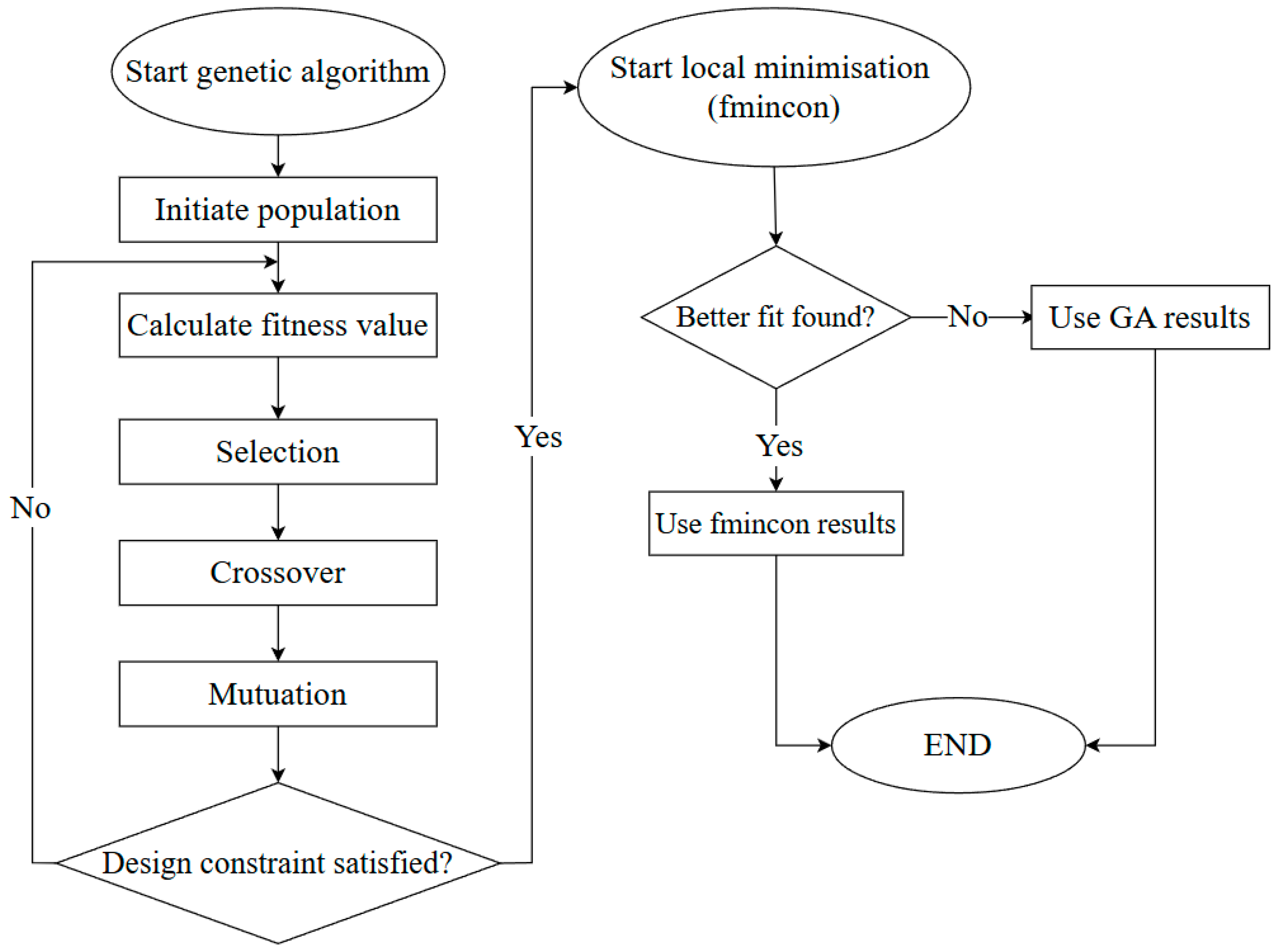
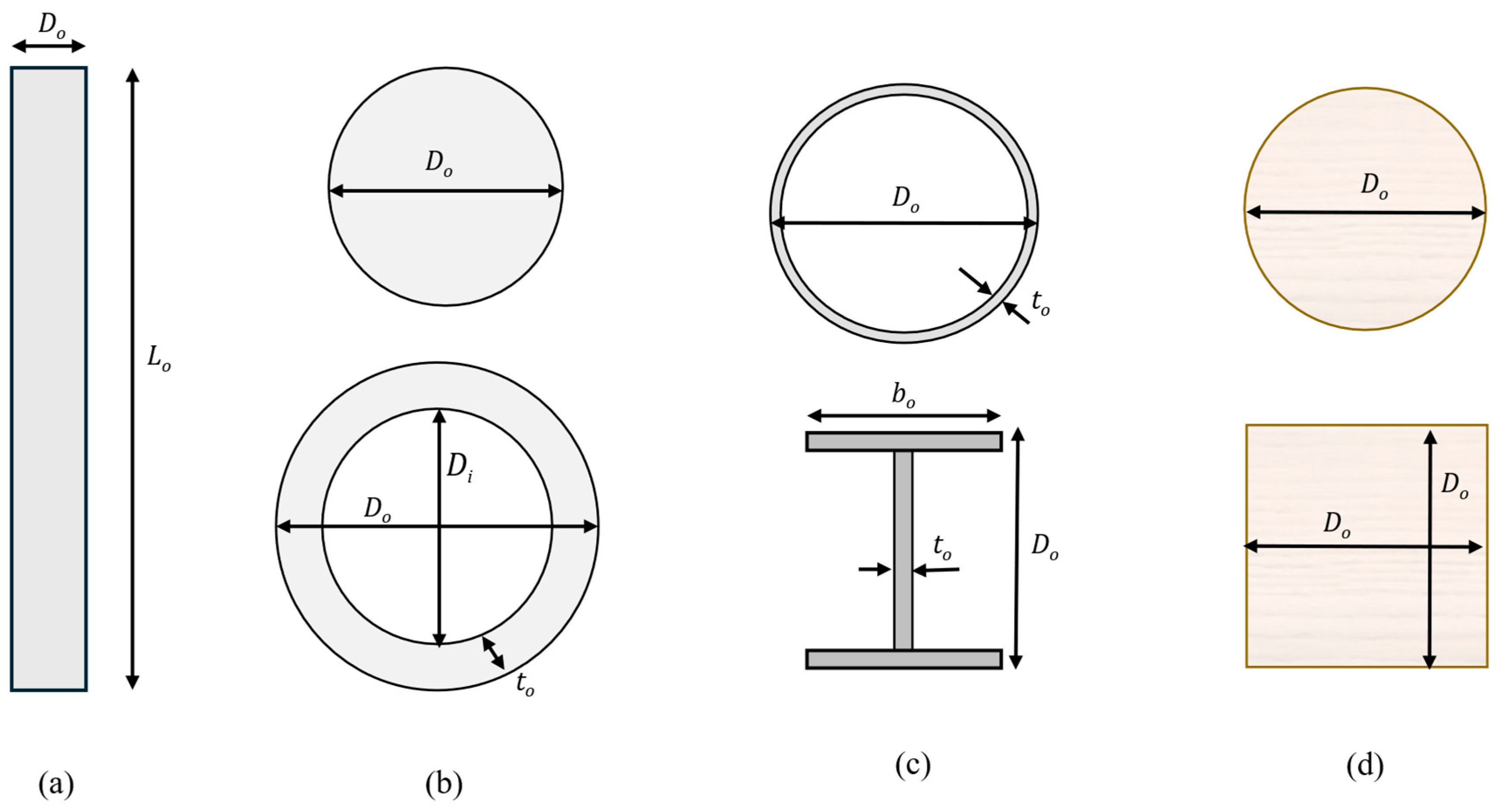

| Soil Type | Property | Symbol | Value (Unit) |
|---|---|---|---|
| Undrained clay | Unit weight | 18 (kN/m3) | |
| Undrained shear strength | cu | 80 + 1.5z * (kPa) | |
| Poisson’s ratio | v | 0.2 | |
| Shear modulus | G | 5000 + 500z * (kPa) | |
| Adhesion factor | α | 0.5 | |
| Dry loose sand | Unit weight | 15 (kN/m3) | |
| Angle of internal friction | φ′ | 32° | |
| Pile–soil interface angle | δ | 24° | |
| Poisson’s ratio | v | 0.2 | |
| Shear modulus | G | 10 MPa |
| Material | A1–A3 (kgCO2e/kg) | A4 (kgCO2e/kg) | A5 (kgCO2e/kg) | Assumptions |
|---|---|---|---|---|
| In situ cast concrete | 0.082 + 0.002fck | 0.005 ** | 0.053 | Linear regression of the ICE inventory [15] |
| Reinforced steel bars | 1.99 | 0.032 * | 0.053 | Worldwide steel of low recycled content |
| Construction steel | 1.55 | 0.032 * | 0.01 | Worldwide open steel sections |
| Timber | 0.263 | 0.032 * | 0.01 | Studwork, softwood |
| Sections | Optimised Design Variables | Symbol | Range/Value | Unit |
|---|---|---|---|---|
| Solid concrete and hollow concrete bored piles | Length | Lo | [1–300] | m |
| Outer diameter | Do | [0.1–3] | m | |
| Inner diameter (hollow concrete) | Di | [0.1–3] | m | |
| Concrete thickness | to | [0.1–D/2] | m | |
| Concrete grade | fcko | [12–60] | MPa | |
| Reinforcement ratio | As/Ac | [0.004–0.04] | - | |
| Circular and square timber driven piles | Length | Lo | [1–12] * | m |
| Diameter or width | Do | [0.05–0.45] | m | |
| Timber grade [62] | Douglas fir C24 = 18 | MPa | ||
| Steel pipe and steel universal columndriven piles | Length | Lo | [1–300] | m |
| Section diameter/height | Do | [0.1–3] | m | |
| Pipe or web thickness | to | [0.001–0.05] [63] | m | |
| Flange width | bo | [0.15–0.48] | m | |
| Steel grade | S355 = 355 | MPa |
| Capacity (MN) | Clayey Soil | Sandy Soil | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Solid | Hollow | Solid | Hollow | |||||||
| Lo (m) | Do (m) | Lo (m) | Do (m) | Di (m) | Lo (m) | Do (m) | Lo (m) | Do (m) | Di (m) | |
| 0.5 | 18.5 | 0.6 | 4.2 | 1.6 | 1.4 | 21.1 | 0.25 | 19.0 | 0.4 | 0.2 |
| 1 | 26.2 | 0.6 | 10.0 | 2.0 | 1.8 | 25.1 | 0.3 | 19.8 | 0.6 | 0.4 |
| 1.5 | 32.1 | 0.6 | 13.4 | 2.0 | 1.8 | 27.8 | 0.4 | 19.3 | 0.9 | 0.7 |
| 2 | 37.0 | 0.7 | 5.0 | 2.0 | 1.8 | 29.9 | 0.5 | 19.0 | 1.2 | 1.0 |
| 2.5 | 41.4 | 0.8 | 16.7 | 2.0 | 1.8 | 31.6 | 0.5 | 18.8 | 1.5 | 1.3 |
| 3 | 45.3 | 0.9 | 20.0 | 2.0 | 1.8 | 33.1 | 0.6 | 18.7 | 1.9 | 1.7 |
| (MN) | Clayey Soil | Sandy Soil | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Pipe Section | UC Section | Pipe Section | UC Section | |||||||
| Lo (m) | Do (m) | to (mm) | Lo (m) | Section | Lo (m) | Do (m) | to (mm) | Lo (m) | Section | |
| 0.5 | 39.8 | 0.19 | 4.0 | 30.2 | 152 × 152 × 37 | 25 | 0.19 | 4.0 | 21.8 | 152 × 152 × 23 |
| 1 | 50.3 | 0.22 | 5.0 | 32.0 | 152 × 152 × 51 | 29.7 | 0.22 | 5.0 | 25.4 | 152 × 152 × 23 |
| 1.5 | 68.9 | 0.32 | 6.0 | 41.0 | 203 × 203 × 46 | 32.9 | 0.32 | 6.0 | 31.0 | 203 × 203 × 46 |
| 2 | 79.6 | 0.36 | 8.0 | 41.3 | 203 × 203 × 52 | 36.4 | 0.36 | 8.0 | 31.7 | 203 × 203 × 100 |
| 2.5 | 89.0 | 0.41 | 8.0 | 51.6 | 254 × 254 × 73 | 37.4 | 0.46 | 8.0 | 31.9 | 257 × 254 × 167 |
| 3 | 97.4 | 0.46 | 8.0 | 58.0 | 305 × 305 × 79 | 39.1 | 0.46 | 10 | 32.7 | 305 × 305 × 18 |
| Capacity (MN) | Clayey Soil | Sandy Soil | ||||||
|---|---|---|---|---|---|---|---|---|
| Round Pile | Square Pile | Round Pile | Square Pile | |||||
| Lo (m) | Do (m) | Lo (m) | Do (m) | Lo (m) | Do (m) | Lo (m) | Do (m) | |
| 0.1 | 9.8 | 0.17 | 8.7 | 0.15 | 11.6 | 0.17 | 10.7 | 0.15 |
| 0.2 | 13.9 | 0.24 | 12.3 | 0.21 | 13.5 | 0.24 | 12.7 | 0.21 |
| 0.3 | 17.1 | 0.29 | 15.1 | 0.26 | 14.9 | 0.29 | 14.1 | 0.26 |
| 0.4 | 19.7 | 0.34 | 17.4 | 0.30 | 16.1 | 0.34 | 15.1 | 0.30 |
| 0.5 | 22.0 | 0.38 | 19.5 | 0.33 | 17.0 | 0.38 | 16.0 | 0.33 |
| 0.6 | 24.1 | 0.41 | 21.4 | 0.37 | 17.8 | 0.41 | 1.7 | 0.37 |
| 0.7 | 26.0 | 0.45 | 23.1 | 0.40 | 18.9 | 0.45 | 17.4 | 0.39 |
| 0.8 | - | - | 24.7 | 0.42 | - | - | 18.0 | 0.42 |
| 0.9 | - | - | 26.2 | 0.45 | - | - | 18.0 | 0.45 |
| Soil Parameter [Symbol] (Unit) | Layer 1—Sand | Layer 2—Silty Clay |
|---|---|---|
| Layer depth [z] (m) | [0–25] | [25–42] |
| Unit weight [γ] (kN/m3) | 19 | 20 |
| Young’s modulus [E] (MPa) | [25–50] | [25–30] |
| Effective cohesion [c] (kPa) | 0 | [20–30] |
| Effective friction angle [φ] (Degrees) | [32–36] | 20 |
| Pile Type | Design | Embodied Carbon |
|---|---|---|
| As-built design | L = 18 m D = 0.5 m fck = 25 MPa As/Ac = 1.5% | Reference value |
| Solid concrete | L = 20 m D = 0.25 m fck = 25 MPa As/Ac = 4% | −47.9% |
| Hollow concrete | L = 17 m Do = 0.3 m Di = 0.1 fck = 25 MPa As/Ac = 2.4% | −58.3% |
| Steel pipe | L = 23.62 D = 0.2 m t = 3 mm | −72.3% |
| Steel UC | L = 16 m Section = 203 × 203 × 46 | −32.6% |
| Circular timber | L = 16.5 D = 0.35 Douglas fir C24 | −85.6% |
| Square timber | L = 15.5 D = 0.3 Douglas fir C24 | −88.3% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abushama, K.; Hawkins, W.; Pelecanos, L.; Ibell, T. Optimising Embodied Carbon in Axial Tension Piles: A Comparative Study of Concrete, Steel, and Timber Piles Using a Hybrid Genetic Approach. Materials 2025, 18, 2160. https://doi.org/10.3390/ma18092160
Abushama K, Hawkins W, Pelecanos L, Ibell T. Optimising Embodied Carbon in Axial Tension Piles: A Comparative Study of Concrete, Steel, and Timber Piles Using a Hybrid Genetic Approach. Materials. 2025; 18(9):2160. https://doi.org/10.3390/ma18092160
Chicago/Turabian StyleAbushama, Kareem, Will Hawkins, Loizos Pelecanos, and Tim Ibell. 2025. "Optimising Embodied Carbon in Axial Tension Piles: A Comparative Study of Concrete, Steel, and Timber Piles Using a Hybrid Genetic Approach" Materials 18, no. 9: 2160. https://doi.org/10.3390/ma18092160
APA StyleAbushama, K., Hawkins, W., Pelecanos, L., & Ibell, T. (2025). Optimising Embodied Carbon in Axial Tension Piles: A Comparative Study of Concrete, Steel, and Timber Piles Using a Hybrid Genetic Approach. Materials, 18(9), 2160. https://doi.org/10.3390/ma18092160







