Flexural Behavior of Orthotropic Steel–LUHPC Composite Bridge Decks: Experimental and Numerical Study
Abstract
:1. Introduction
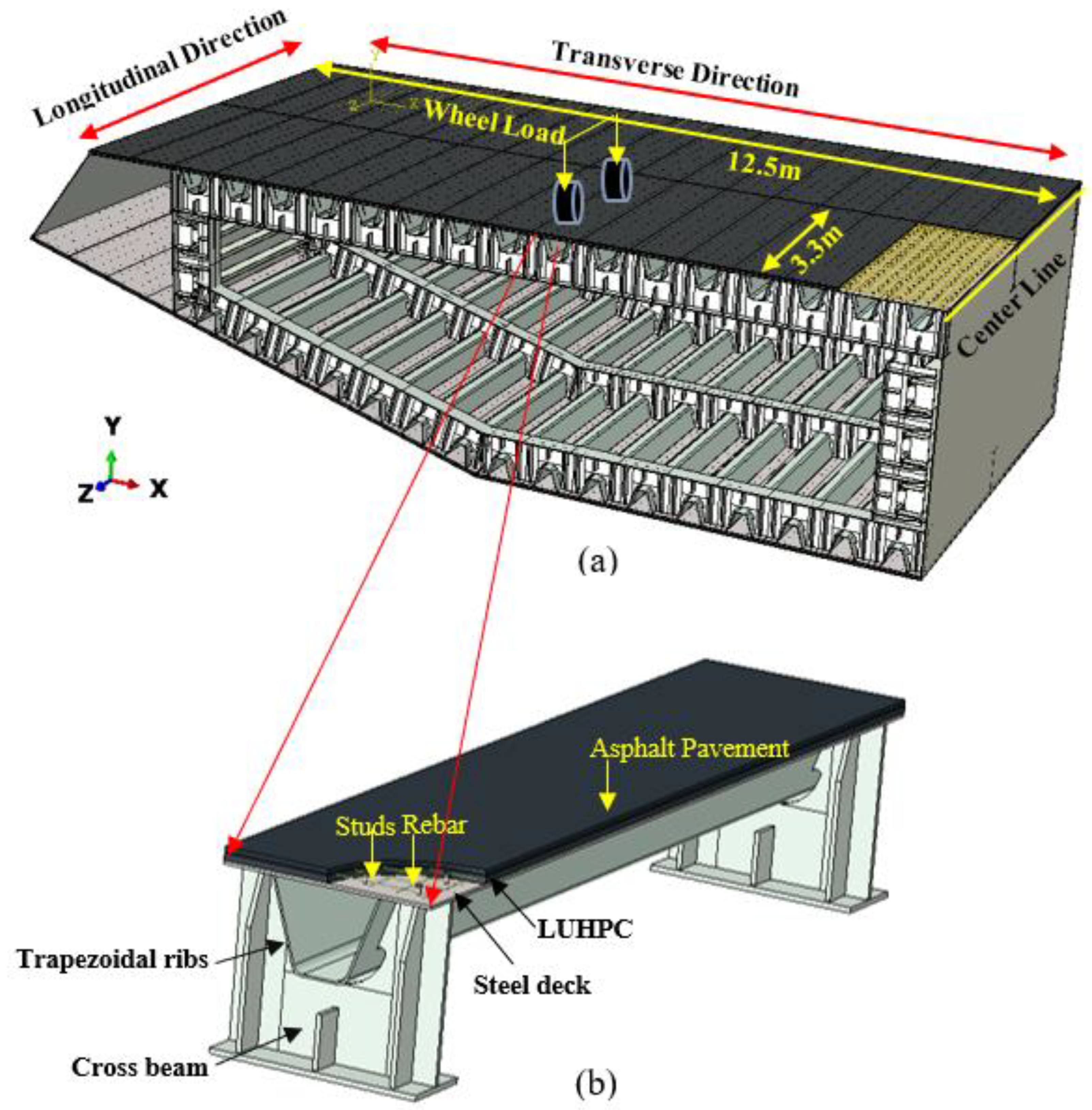
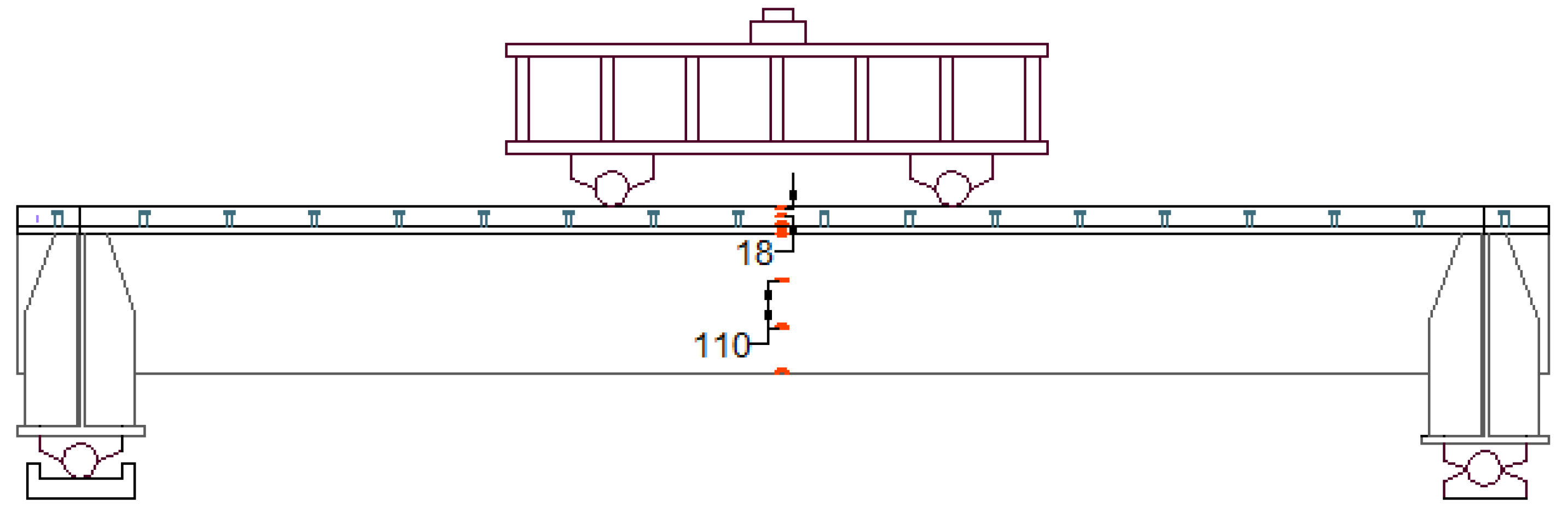
2. Experimental Program
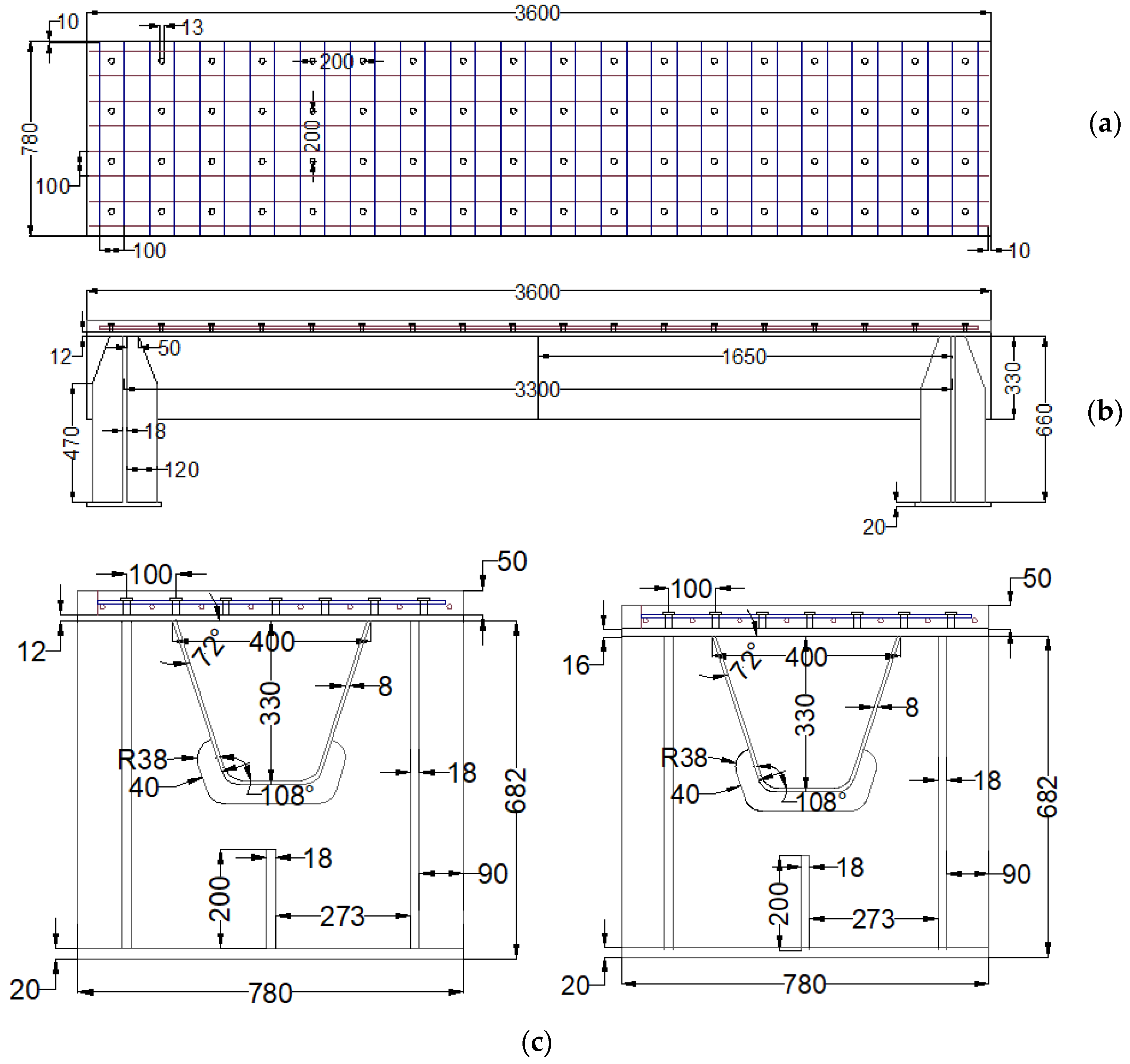
2.1. Specimens for the Flexural Tests
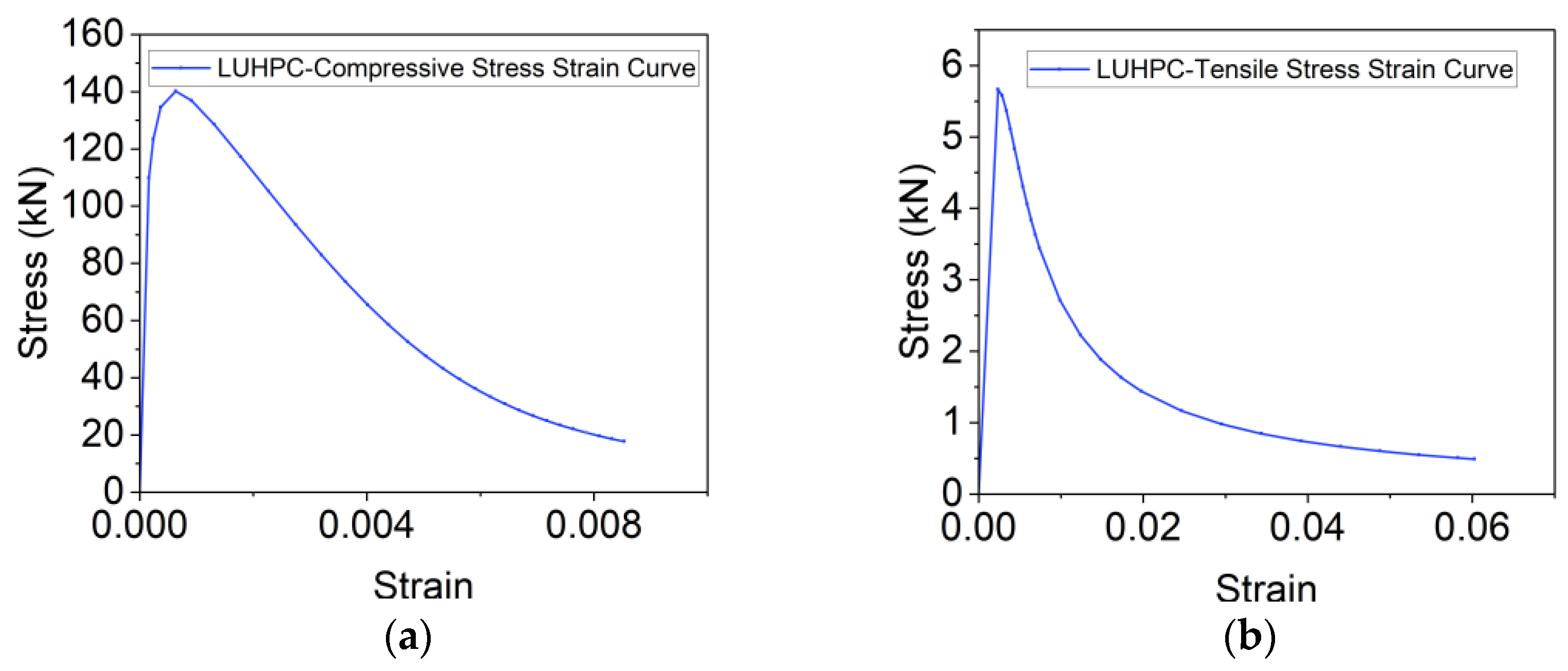
2.2. Material Properties of LUHPC and UHPC
2.3. Fabrication of Specimens
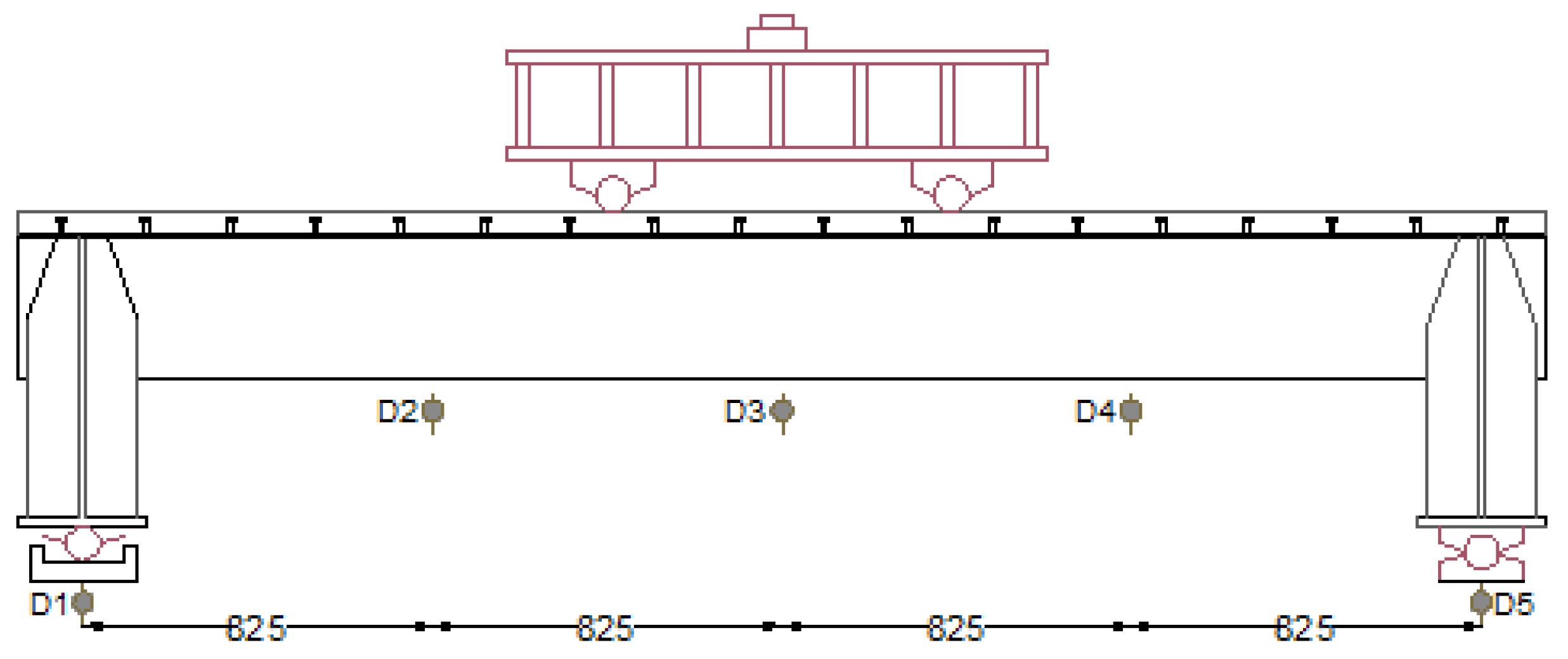
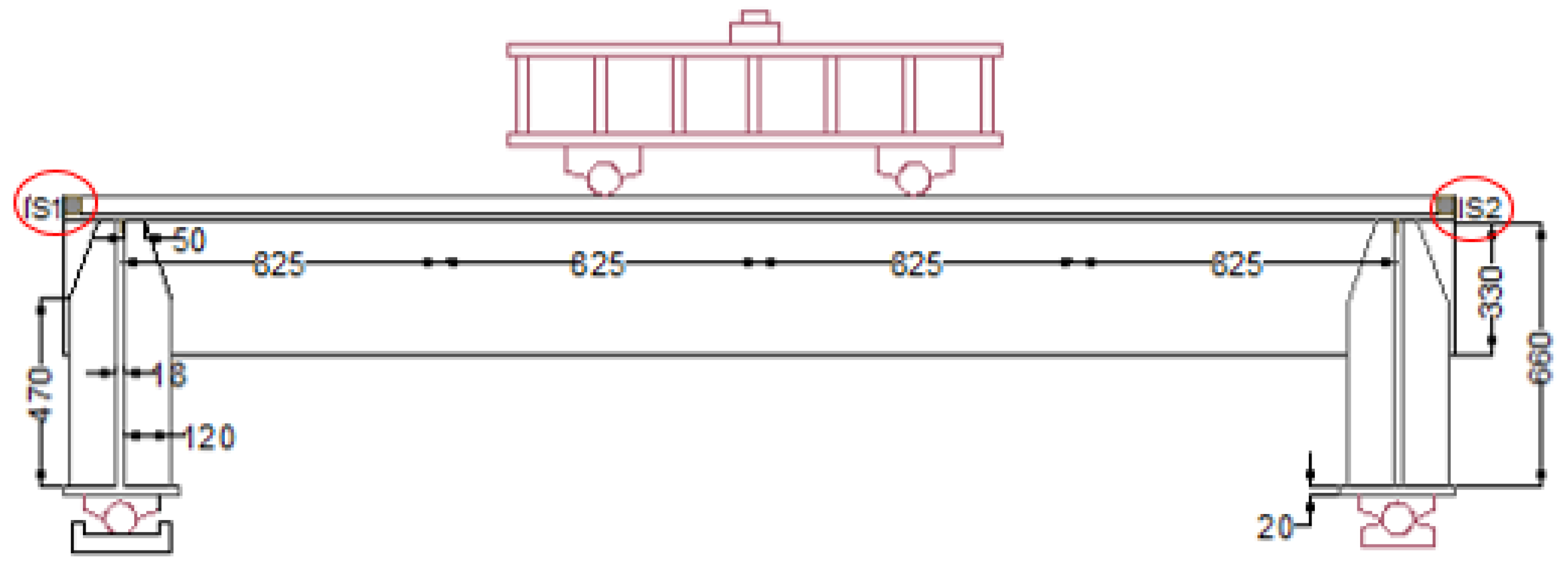
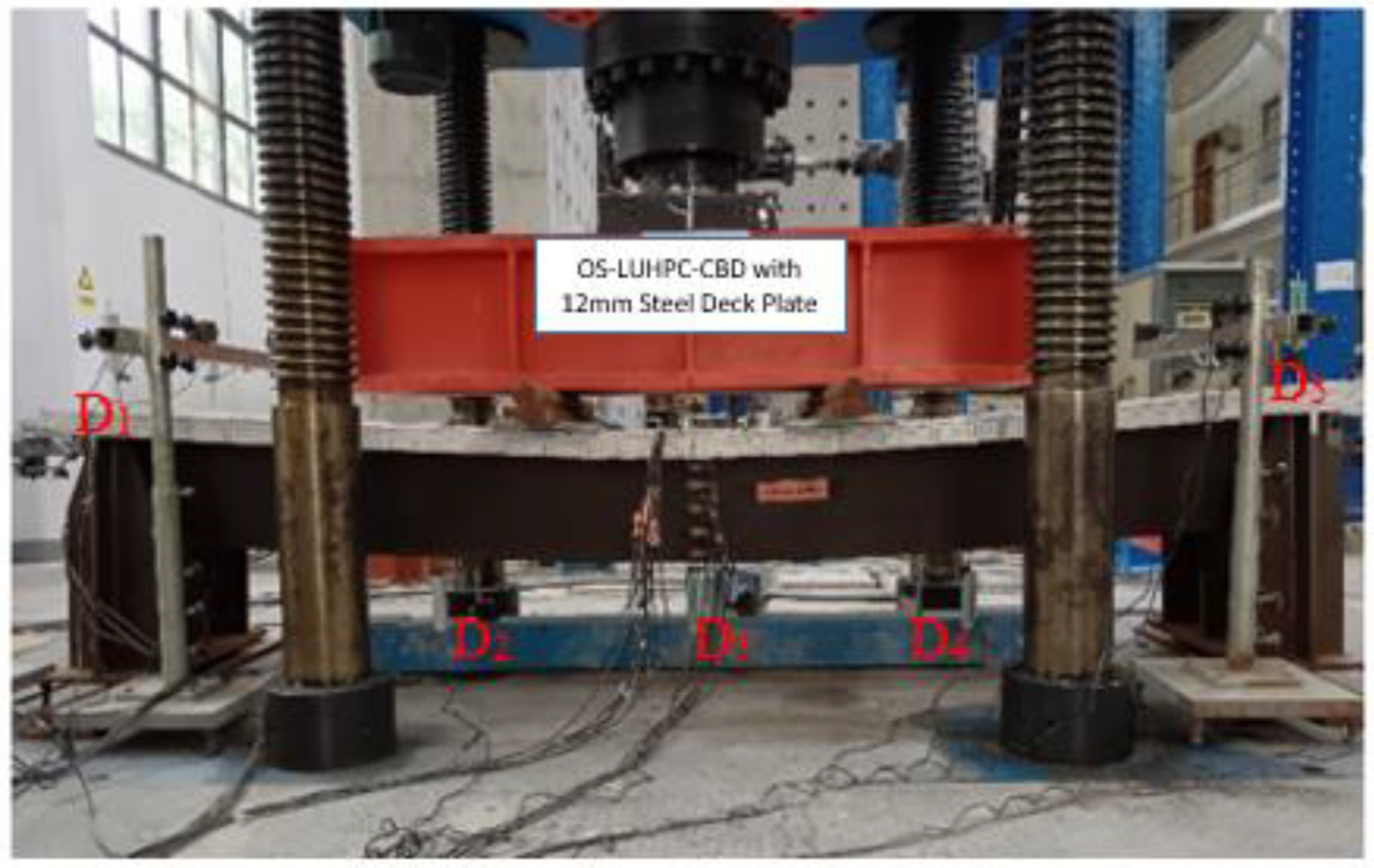
2.4. Test Setup, Loading Conditions, and Instrumentation
3. Experimental Results and Discussion
3.1. Failure Mode
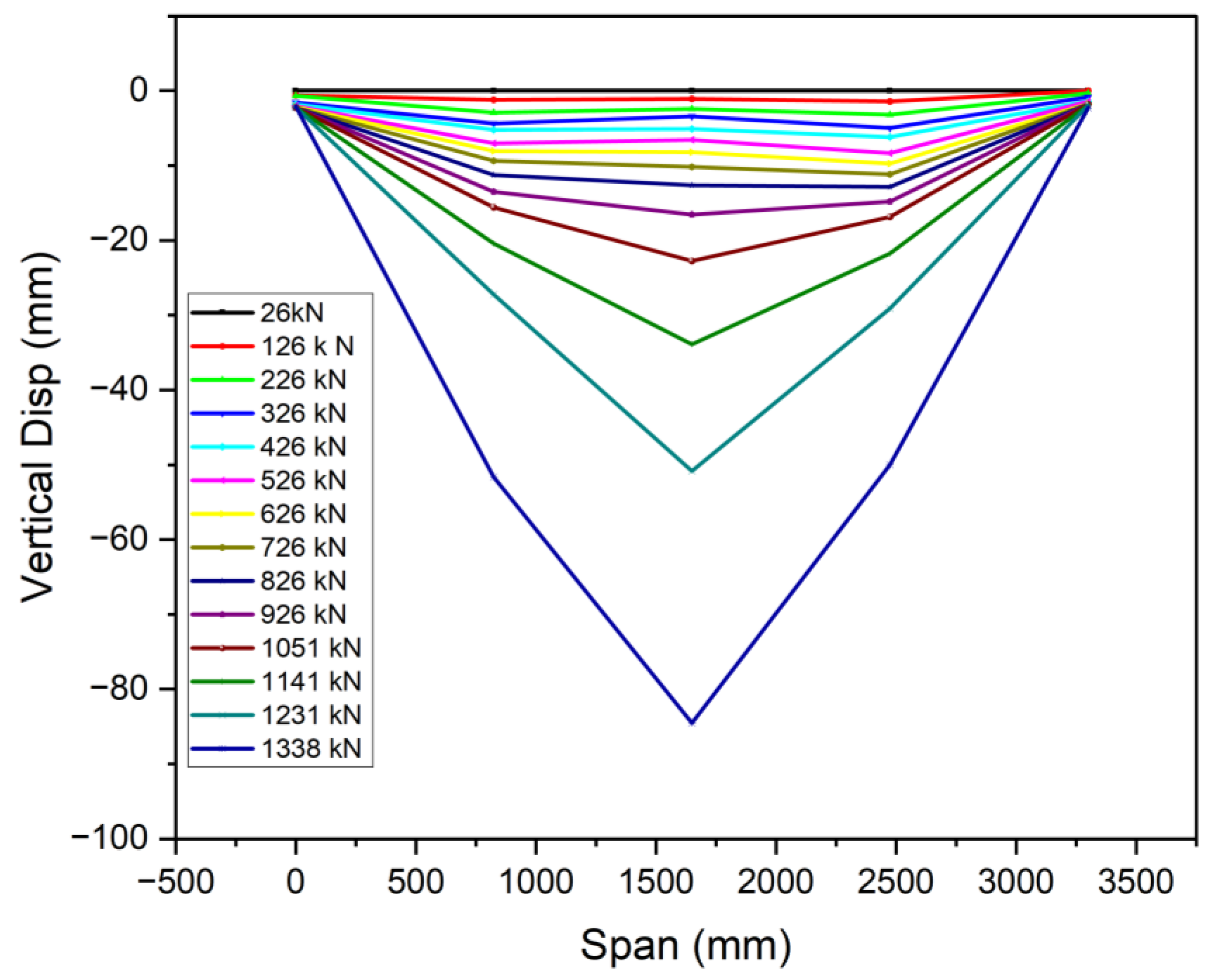
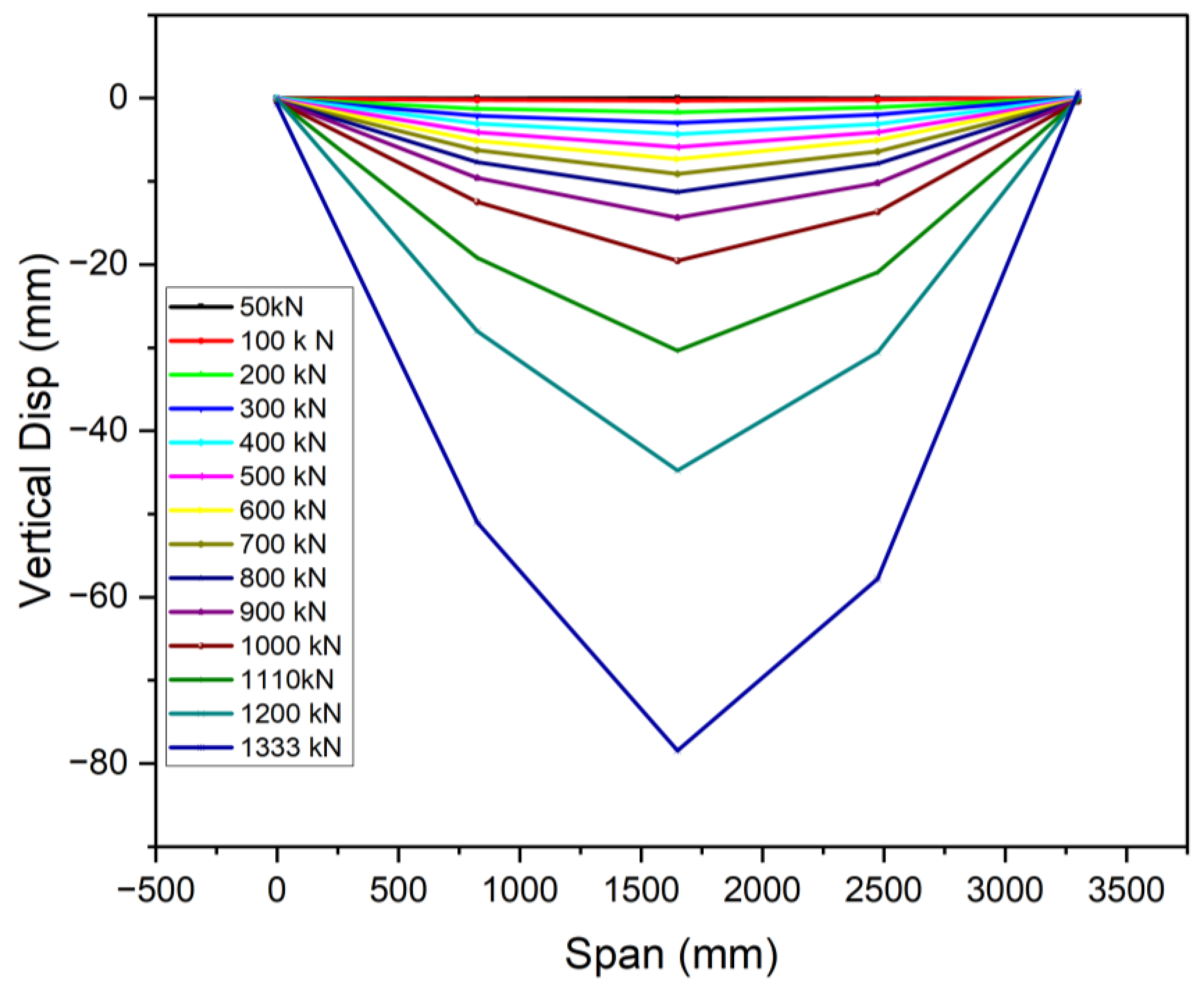
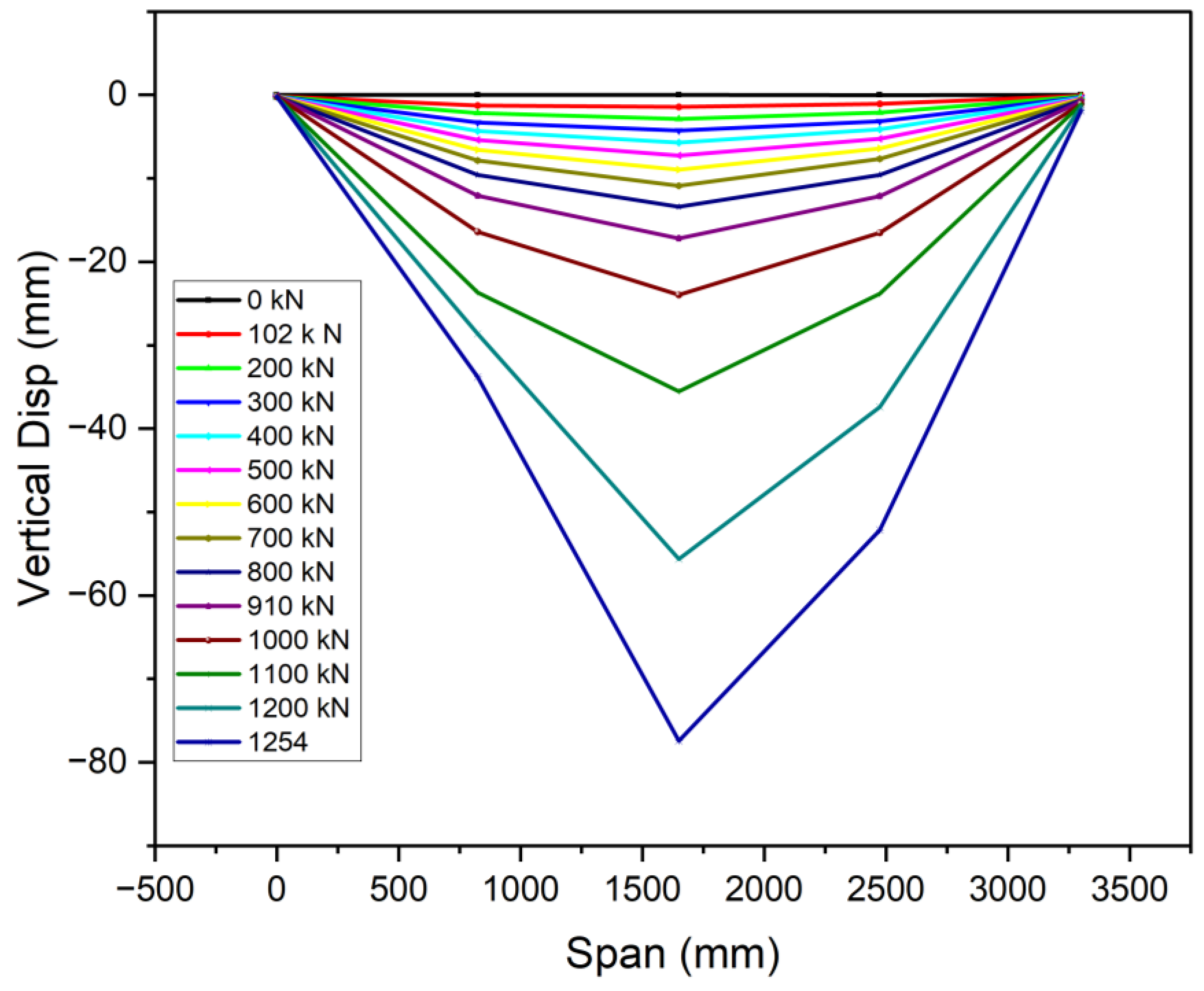
3.2. Load–Deflection Response
3.3. Ductile Performance
3.4. Load–Interfacial Slippage
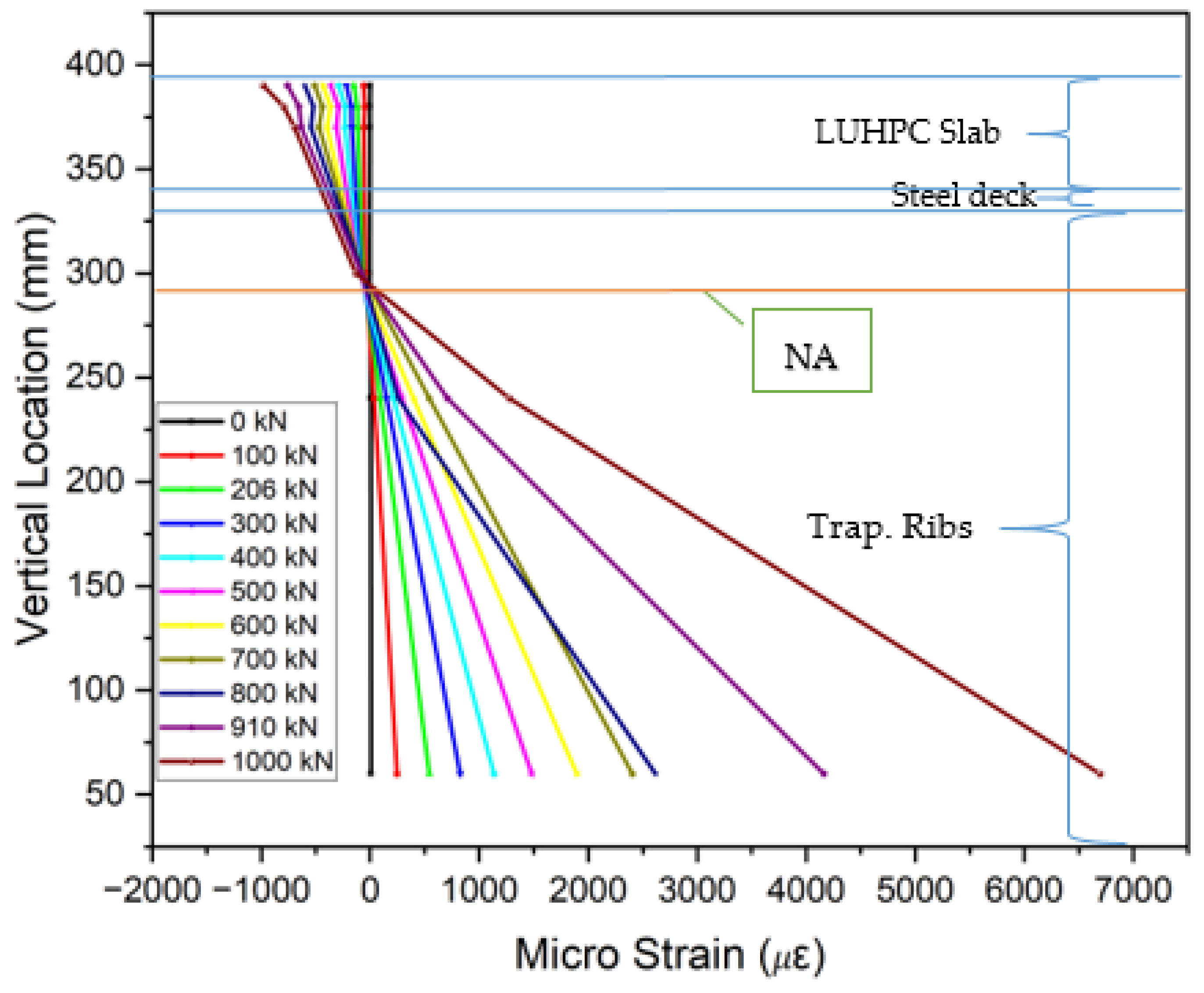
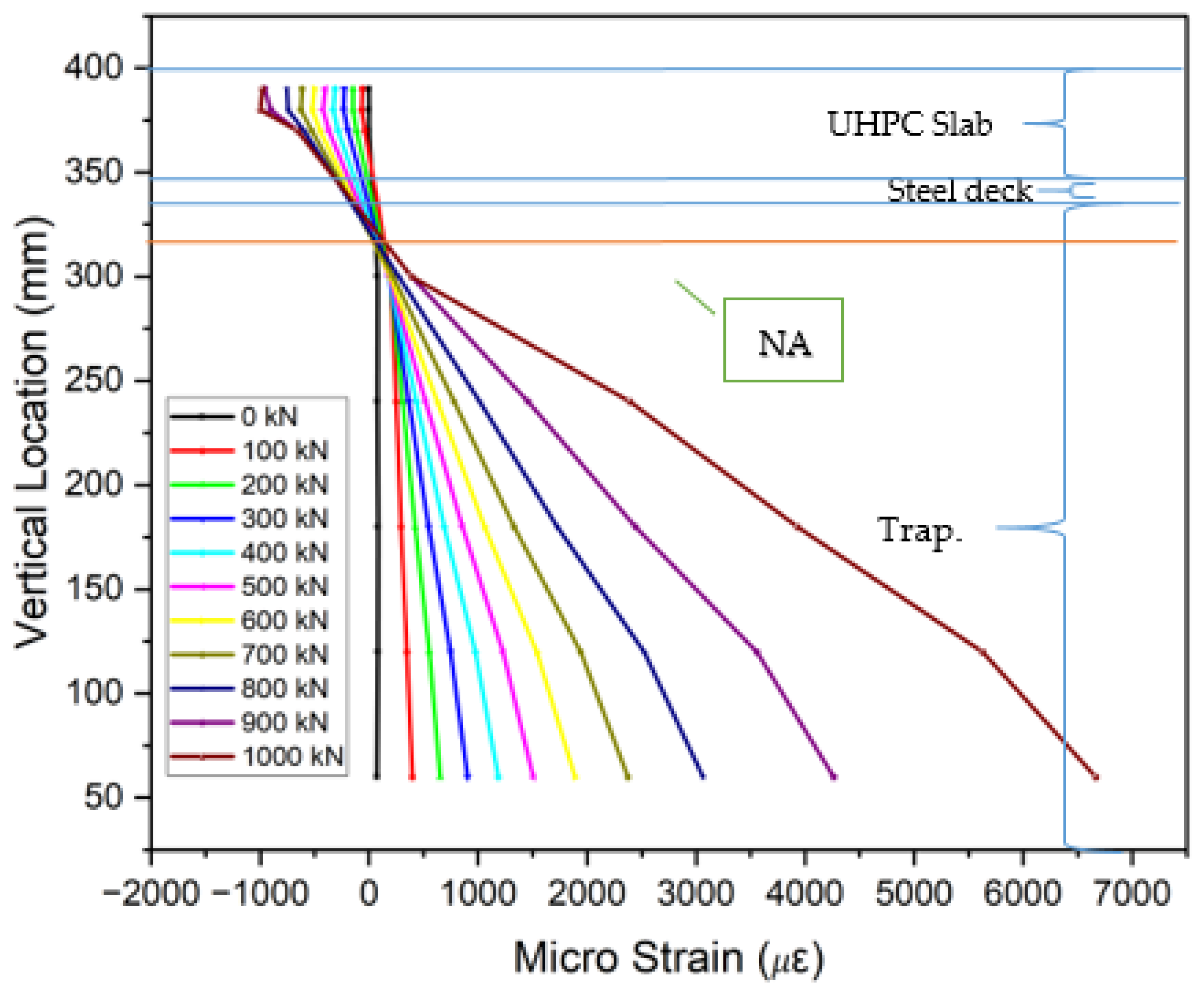
3.5. Strain Distribution
4. Numerical Analysis
4.1. Establishment of Finite Element Model
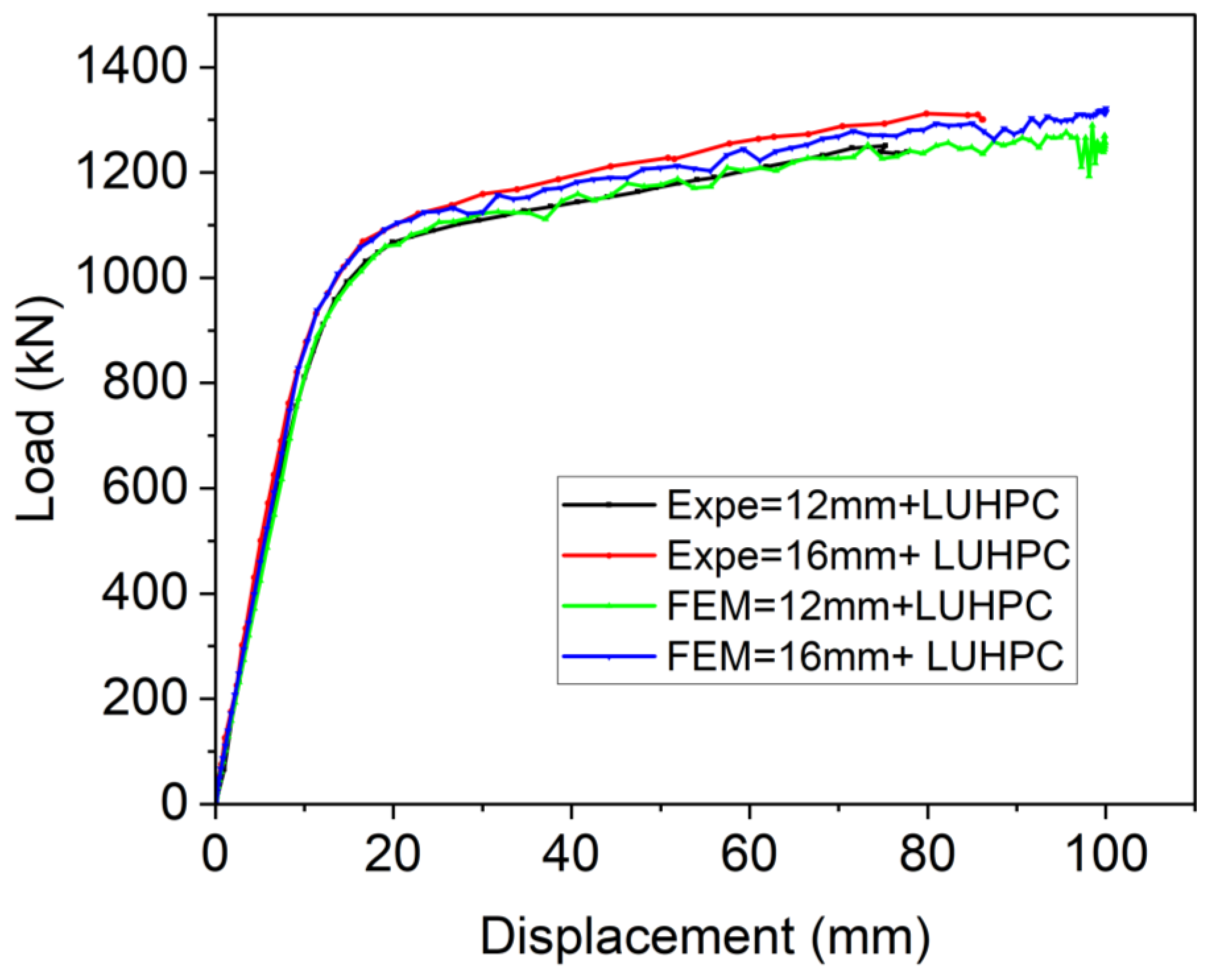
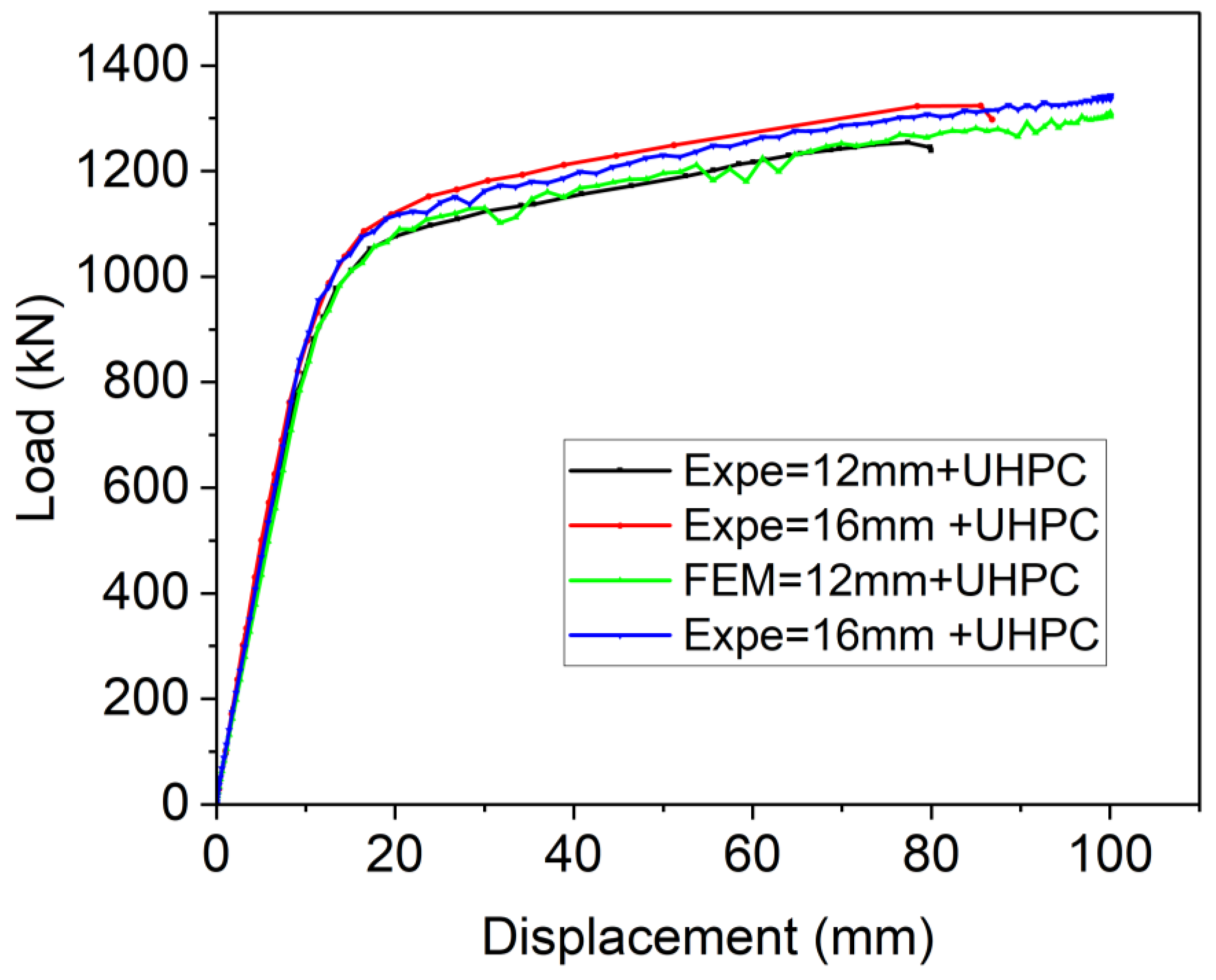
4.2. Model Validation
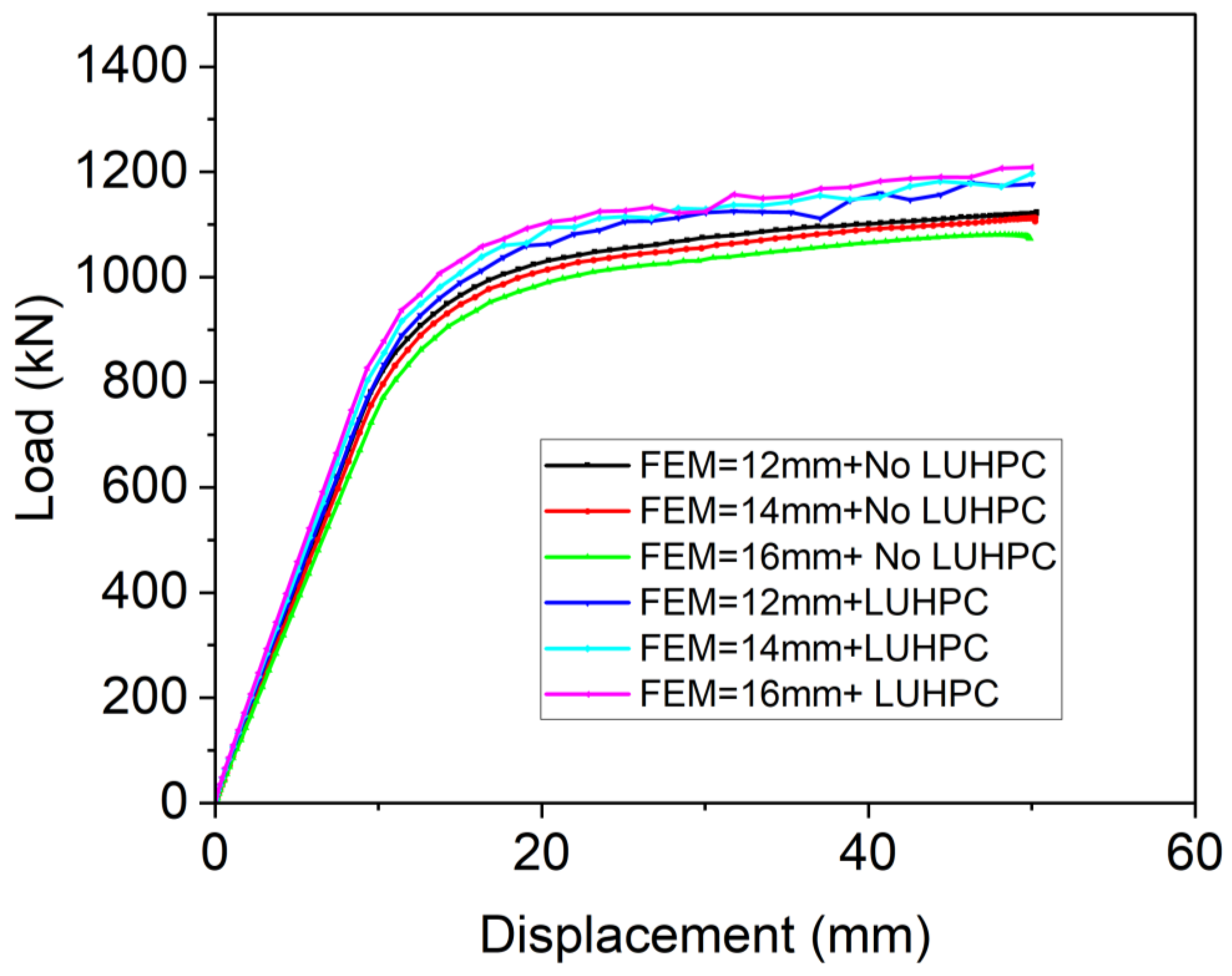
4.3. Parametric Study
5. Conclusions
- The experimental results demonstrated that the proposed OS-LUHPC-CBD has equivalent flexural capacity and improved ductility compared to the OS-UHPC-CBD, and is primarily characterized by flexural failure, as evidenced by the mid-span crushing of the LUHPC and UHPC slabs and yielding of the trapezoidal rib. The OS-LUHPC-CBD showed ductile flexural behavior under ultimate loads with the advantages of being 8.4% lighter in weight and 6.8% lower in cost while being just as easy to construct.
- The findings from this experimental investigation also show that when the steel deck thickness decreased from 16 mm to 12 mm in both the OS-LUHPC-CBD and OS-UHPC-CBD specimens, it resulted in a 6.2% and 5.9% decrease in the ultimate load-bearing capacity and 8.6% and 7.9% reduction in yield load, respectively, with no physically observable differences in their response upon ultimate load application.
- A finite element model with the use of concrete damage plasticity (CDP) was developed and validated, and showed strong correlation with the experimental data in predicting load–deflection, thus proving to be a reliable tool to numerically assess the performance of the OS-LUHPC-CBD and OS-UHPC-CBD systems.
- The parametric study conducted as part of the numerical analysis revealed the significant influence that important geometric parameters have, such as steel deck thickness, rib web thickness, and LUHPC and UHPC slab thickness. Increases in these thicknesses may increase the yielding load and ultimate load and their corresponding deformations.
- This research highlights the potential of the OS-LUHPC-CBD system as a transformative solution for use in long-span bridge decks, offering enhanced flexural performance with reduced material usage and addressing performance and economic concerns in modern infrastructure. Aside from the mentioned mechanical performance results, LUHPC has transformative potential for the design of long-span bridges through the facilitation of lower dead loads, remarkable durability, and novel structural designs. However, to guarantee practical viability, challenges related to large-scale implementation, such as the absence of standardization, need to be addressed. Future studies should focus on creating guidelines to facilitate industry adoption.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Acronyms
| ALE | Arbitrary Lagrangian–Eulerian |
| CDP | Concrete Damage Plasticity |
| C3D8R | 8-Node Hexahedral Solid Elements |
| LUHPC | Lightweight Ultra-High-Performance Concrete |
| NA | Neutral Axis |
| OSDs | Orthotropic Steel Decks |
| OSBDs | Orthotropic Steel Bridge Decks |
| OS-LUHPC-CBD | Orthotropic Steel Lightweight Ultra-High-Performance Composite Bridge Deck |
| OS-UHPC-CBD | Orthotropic Steel Ultra-High-Performance Composite Bridge Deck |
| RD | Rib to Deck Connection |
| RF | Rib to Floor Beam Connection |
| RPs | Reference Points |
| UHPC | Ultra-High-Performance Concrete |
References
- Zhou, X.; Zhang, X. Thoughts on the Development of Bridge Technology in China. Engineering 2019, 5, 1120–1130. [Google Scholar] [CrossRef]
- Zhu, Z.; Xiang, Z.; Li, J.; Huang, Y.; Ruan, S. Fatigue behavior of orthotropic bridge decks with two types of cutout geometry based on field monitoring and FEM analysis. Eng. Struct. 2020, 209, 109926. [Google Scholar] [CrossRef]
- Huang, W.; Minshan, P.; Liu, X.; Wei, Y. Design and construction of super-long span bridges in China: Review and future perspectives. Front. Struct. Civ. Eng. 2020, 14, 803–838. [Google Scholar] [CrossRef]
- Zhao, R.; Zheng, K.; Wei, X.; Jia, H.; Li, X.; Zhang, Q.; Xu, G.; Zhan, Y.; Shen, R.; Zhang, F.; et al. State-of-the-art and annual progress of bridge engineering in 2021. Adv. Bridge Eng. 2022, 3, 29. [Google Scholar] [CrossRef]
- Connor, R.J. Manual for Design, Construction, and Maintenance of Orthotropic Steel Deck Bridges; US Department of Transportation Federal Highway Administration: New Jersey, NJ, USA, 2012. [Google Scholar]
- Dahlberg, J.M.; Paterson, D.; Murphy, T.; Medlock, R.; Logan, T.; Sougata, R.; Artmont, F.A. Guide for Orthotropic Steel Deck Level 1 Design; Federal Highway Administration, Office of Bridge Technology, United States: New Jersey, NJ, USA, 2022. [Google Scholar]
- Lu, Z.; Wei, C.; Liu, M.; Deng, X. Risk Assessment Method for Cable System Construction of Long-Span Suspension Bridge Based on Cloud Model. Adv. Civ. Eng. 2019, 2019, 5720637. [Google Scholar] [CrossRef]
- Deng, X.; Liu, M. Nonlinear Stability Analysis of a Composite Girder Cable-Stayed Bridge with Three Pylons during Construction. Math. Probl. Eng. 2015, 2015, 978514. [Google Scholar] [CrossRef]
- Lou, T.; Liu, M.; Lopes, S.M.R.; Lopes, A.V. Moment redistribution in two-span prestressed NSC and HSC beams. Mater Struct 2017, 50, 246. [Google Scholar] [CrossRef]
- Luo, P.; Zhang, Q.; Bao, Y.; Bu, Y. Fatigue performance of welded joint between thickened-edge U-rib and deck in orthotropic steel deck. Eng. Struct. 2019, 181, 699–710. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, X.; Liu, R.; Chen, G. Design and Mechanical Properties of Steel-UHPC Lightweight Composite Decks. Adv. Civ. Eng. 2021, 2021, e8508795. [Google Scholar] [CrossRef]
- Di, J.; Ruan, X.; Zhou, X.; Wang, J.; Peng, X. Fatigue assessment of orthotropic steel bridge decks based on strain monitoring data. Eng. Struct. 2021, 228, 111437. [Google Scholar] [CrossRef]
- Huang, Z.; Lei, J.; Guo, S.; Tu, J. Fatigue performance of U-rib butt welds in orthotropic steel decks. Eng. Struct. 2020, 211, 110485. [Google Scholar] [CrossRef]
- Fehling, E.; Bunje, K.; Schmidt, M. Gärtnerplatz-Bridge over River Fulda in Kassel: Multispan Hybrid UHPC-Steel Bridge; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2011; 136p. [Google Scholar] [CrossRef]
- Sun, X.; Hu, J.; Li, Y.; Huang, G. Effects of Stiffener Type on Fatigue Resistance of Steel-UHPC Composite Bridge Decks. Adv. Civ. Eng. 2022, 2022, 6606129. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhu, R.; Xiang, Z. A Review on Behavior and Fatigue Performance of Orthotropic Steel–UHPC Composite Deck. Buildings 2023, 13, 1906. [Google Scholar] [CrossRef]
- Tong, J.-Z.; Chen, Y.-L.; Li, Q.-H.; Xu, S.-L.; Zeng, T.; Gao, W. Experimental study on flexural performance of steel-UHTCC composite bridge decks considering different shear connection degrees. Eng. Struct. 2023, 281, 115738. [Google Scholar] [CrossRef]
- Abellán-García, J.; Carvajal-Muñoz, J.S.; Ramírez-Munévar, C. Application of ultra-high-performance concrete as bridge pavement overlays: Literature review and case studies. Constr. Build. Mater. 2024, 410, 134221. [Google Scholar] [CrossRef]
- Shao, X.; Yi, D.; Huang, Z.; Zhao, H.; Chen, B.; Liu, M. Basic Performance of the Composite Deck System Composed of Orthotropic Steel Deck and Ultrathin RPC Layer. J. Bridge Eng. 2013, 18, 417–428. [Google Scholar] [CrossRef]
- Zhang, S.; Shao, X.; Cao, J.; Cui, J.; Hu, J.; Deng, L. Fatigue Performance of a Lightweight Composite Bridge Deck with Open Ribs. J. Bridge Eng. 2016, 21, 04016039. [Google Scholar] [CrossRef]
- Shao, X.; Qu, W.; Cao, J.; Yao, Y. Static and fatigue properties of the steel-UHPC lightweight composite bridge deck with large U ribs. J. Constr. Steel Res. 2018, 148, 491–507. [Google Scholar] [CrossRef]
- Abdal, S.; Mansour, W.; Agwa, I.; Nasr, M.; Abadel, A.; Özkılıç, Y.; Akeed, M.H. Application of Ultra-High-Performance Concrete in Bridge Engineering: Current Status, Limitations, Challenges, and Future Prospects. Buildings 2023, 13, 185. [Google Scholar] [CrossRef]
- Haber, Z.B.; Muñoz, J.F.; Muñoz, J.F.; De la Varga, I.; Graybeal, B.A. Bond characterization of UHPC overlays for concrete bridge decks: Laboratory and field testing. Constr. Build. Mater. 2018, 190, 1056–1068. [Google Scholar] [CrossRef]
- Ding, Q.; Hu, J.; Liu, Y.; Peng, C.; Zhang, G. Design and research of lightweight ultra-high performance concrete. In Proceedings of the 13th High Performance Concrete Symposium, Tangshan, China, 26 July 2019. [Google Scholar]
- Zhang, G.; Wei, Q.; Ding, Q.; Yang, J.; Ge, J.; Wang, Y. Mix design and volume stability of lightweight ultra-high performance concret. In Proceedings of the 13th High Performance Concrete Symposium, Tangshan, China, 26 July 2019. [Google Scholar]
- Li, H.; Song, J.; Ding, Q. Study on the influence of lightweight aggregate on the performance of lightweight ultra-high performance concrete. J. Wuhan Univ. Technol. 2020, 42. [Google Scholar]
- Hu, F.; Li, H.; Ding, Q.; Deng, C. Research on Shrinkage Control Technology of Lightweight Ultra-High Performance Concrete. J. Wuhan Univ. Technol. 2019, 41, 6. [Google Scholar]
- Lu, Z.; Jiang, Z.; Liu, M. Creep Performance and Model of Lightweight Ultra-High Performance Concrete. J. Huazhong Univ. Sci. Technol. (Nat. Sci. Ed.) 2023, 51, 128–133. [Google Scholar] [CrossRef]
- Lin, C.; Yan, L.; Lu, Z. Study on the Anti-cracking Performance of Steel-LUHPC Composite Beams. J. Wuhan Univ. Technol. 2019, 41. [Google Scholar]
- Liu, M.; Wu, F.; Zhang, Q.; Ma, R.; Wang, J. Experimental study on seismic performance of prefabricated bridge piers with LUHPC reinforced plastic hinges. J. Wuhan Univ. Technol. 2021, 43, 39–47. [Google Scholar]
- Zhan, J.; Peng, X.; Liu, M.; Cao, Y.; Zheng, G. Study on the Bonding Performance between Steel Bars and Lightweight Ultra-High Performance Concrete. J. Wuhan Univ. Technol. (Transp. Sci. Eng. Ed.) 2023, 47. [Google Scholar]
- Fu, J.; Xu, M.; Wang, Z.; Ding, Q. Study Basic Performance Low Shrinkage LUHPC Steel Bridge Deck Pavement Comb. J. Wuhan Univ. Technol. 2023, 40. [Google Scholar]
- Lu, Z.; Ou, X.; Liu, M.; Zhan, J.; Peng, X. Durability Study of Lightweight Ultra-High Performance Concrete (LUHPC). J. Wuhan Univ. Technol. 2023, 45. [Google Scholar]
- Cao, J.; Shao, X. Finite element analysis of headed studs embedded in thin UHPC. J. Constr. Steel Res. 2019, 161, 355–368. [Google Scholar] [CrossRef]
- Guan, Y.; Wu, J.; Sun, R.; Ge, Z.; Bi, Y.; Zhu, D. Shear behavior of short headed studs in Steel-ECC composite structure. Eng. Struct. 2022, 250, 113423. [Google Scholar] [CrossRef]
- Behnam, H.; Kuang, J.S.; Samali, B. Parametric finite element analysis of RC wide beam-column connections. Comput. Struct. 2018, 205, 28–44. [Google Scholar] [CrossRef]
- American Association of State Highway and Transportation Officials. AASHTO LRFD Bridge Design Specifications-SI Units, 4th ed.; American Association of State Highway and Transportation Officials (AASHTO): Washington, DC, USA, 2007. [Google Scholar]
- Du, J.; Meng, W.; Khayat, K.H.; Bao, Y.; Guo, P.; Lyu, Z.; Abu-Obeidah, A.; Nassif, H.; Wang, H. New development of ultra-high-performance concrete (UHPC). Compos. Part B Eng. 2021, 224, 109220. [Google Scholar] [CrossRef]
- Wu, N.; Ji, T.; Huang, P.; Fu, T.; Zheng, X.; Xu, Q. Use of sugar cane bagasse ash in ultra-high performance concrete (UHPC) as cement replacement. Constr. Build. Mater. 2022, 317, 125881. [Google Scholar] [CrossRef]
- Liu, K.; Yin, T.; Fan, D.; Wang, J.; Yu, R. Multiple effects of particle size distribution modulus (q) and maximum aggregate size (Dmax) on the characteristics of Ultra-High Performance concrete (UHPC): Experiments and modeling. Cem. Concr. Compos. 2022, 133, 104709. [Google Scholar] [CrossRef]
- Wang, J.N.; Yu, R.; Ji, D.D.; Tang, L.W.; Yang, S.C.; Fan, D.Q.; Shui, Z.H.; Leng, Y.; Liu, K.N. Effect of distribution modulus (q) on the properties and microstructure development of a sustainable Ultra-High Performance Concrete (UHPC). Cem. Concr. Compos. 2022, 125, 104335. [Google Scholar] [CrossRef]
- Lu, J.-X.; Shen, P.; Ali, H.A.; Poon, C.S. Mix design and performance of lightweight ultra high-performance concrete. Mater. Des. 2022, 216, 110553. [Google Scholar] [CrossRef]
- Meng, W.; Khayat, K. Effects of saturated lightweight sand content on key characteristics of ultra-high-performance concrete. Cem. Concr. Res. 2017, 101, 46–54. [Google Scholar] [CrossRef]
- Wu, J.; Ding, Q.; Liu, Y.; Mei, J.; Zhang, G. Mixture design, Preparation and Properties of Lightweight Ultra-high Performance Concrete. Int. Interact. Symp. Ultra-High Perform. Concr. 2019, 2. [Google Scholar]
- GB/T 31387-2015; Reactive Power Concrete. Ministry of House and Urban Rural Development of People’s Republic of China: Beijing, China, 2015.
- Hu, Y.; Meloni, M.; Cheng, Z.; Wang, J.; Xiu, H. Flexural performance of steel-UHPC composite beams with shear pockets. Structures 2020, 27, 570–582. [Google Scholar] [CrossRef]
- Tong, L.; Chen, L.; Wang, X.; Zhu, J.; Shao, X.; Zhao, Z. Experiment and finite element analysis of bending behavior of high strength steel-UHPC composite beams. Eng. Struct. 2022, 266, 114594. [Google Scholar] [CrossRef]
- Yang, T.; Zhou, X.; Liu, Y. Flexural performance of prefabricated composite beams with grouped bolt shear connectors under positive bending moments. Eng. Struct. 2023, 277, 115387. [Google Scholar] [CrossRef]
- Fang, Z.; Fang, H.; Li, P.; Jiang, H.; Chen, G. Interfacial shear and flexural performances of steel–precast UHPC composite beams: Full-depth slabs with studs vs. demountable slabs with bolts. Eng. Struct. 2022, 260, 114230. [Google Scholar] [CrossRef]
- Cheng, Z.; Zhang, Q.; Bao, Y.; Deng, P.; Wei, C.; Li, M. Flexural behavior of corrugated steel-UHPC composite bridge decks. Eng. Struct. 2021, 246, 113066. [Google Scholar] [CrossRef]
- Benedetty, C.A.; dos Santos, V.B.; Krahl, P.A.; Rossi, A.; Silva, F.d.A.; Cardoso, D.C.T.; Martins, C.H. Flexural and shear behavior of steel-UHPC composite beams: A review. Eng. Struct. 2023, 293, 116649. [Google Scholar] [CrossRef]
- Li, Z.; Zhu, H.; Zhen, X.; Wen, C.; Chen, G. Effects of steel fiber on the flexural behavior and ductility of concrete beams reinforced with BFRP rebars under repeated loading. Compos. Struct. 2021, 270, 114072. [Google Scholar] [CrossRef]
- Li, C.; Chen, B.; Hu, W.; Sennah, K. Push-out test of hybrid shear connector in steel-precast UHPC composite slab. Structures 2023, 50, 1767–1782. [Google Scholar] [CrossRef]
- Lin, J.-P.; Qiao, N.; Zhang, L.; Tang, J.; Zhou, L.; Huo, J.; Wang, G. Study on the mechanical properties of hybrid bonding shear connections with shear studs and epoxy mortar layer. Structures 2024, 70, 107642. [Google Scholar] [CrossRef]
- Graybeal, B. Compressive Behavior of Ultra-High-Performance Fiber-Reinforced Concrete. Aci Mater. J. 2007, 104, 146–152. [Google Scholar]
- Cao, Y.; Liu, M.; Zhang, Y.; Hu, J.; Yang, S. Effect of Strain Rates on the Stress–Strain Behavior of FRP-Confined Pre-Damaged Concrete. Materials 2020, 13, 1078. [Google Scholar] [CrossRef]
- Cao, Y.; Zhao, G.; Liu, M.; Jin, Q.; Tao, Z.; Jiang, C. FRP-confined rubber concrete with effect of strain rate: Tests and analysis-oriented stress–strain model. Constr. Build. Mater. 2023, 366, 130234. [Google Scholar] [CrossRef]
- Pavlović, M.; Marković, Z.; Veljković, M.; Buđevac, D. Bolted shear connectors vs. headed studs behaviour in push-out tests. J. Constr. Steel Res. 2013, 88, 134–149. [Google Scholar] [CrossRef]













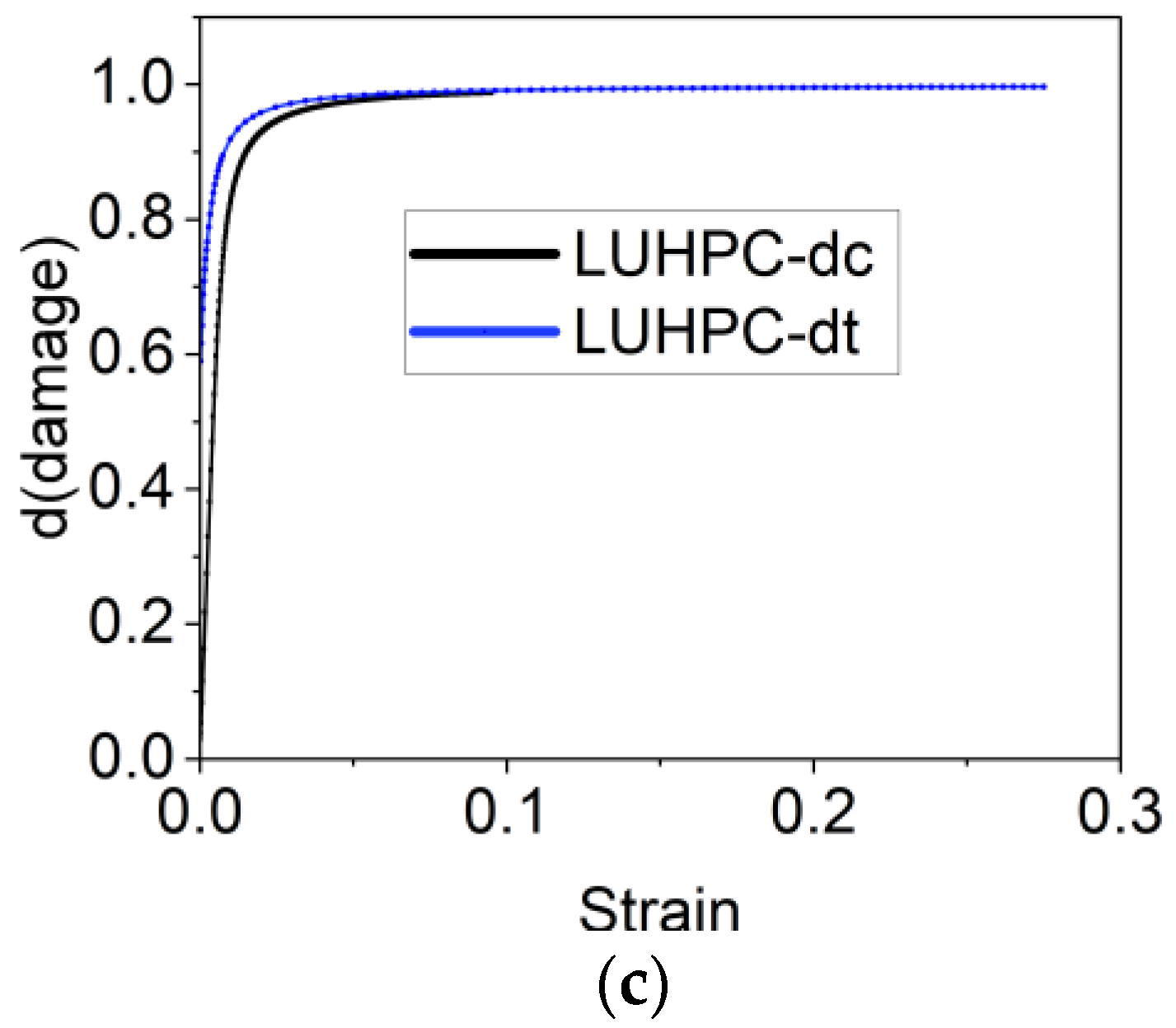
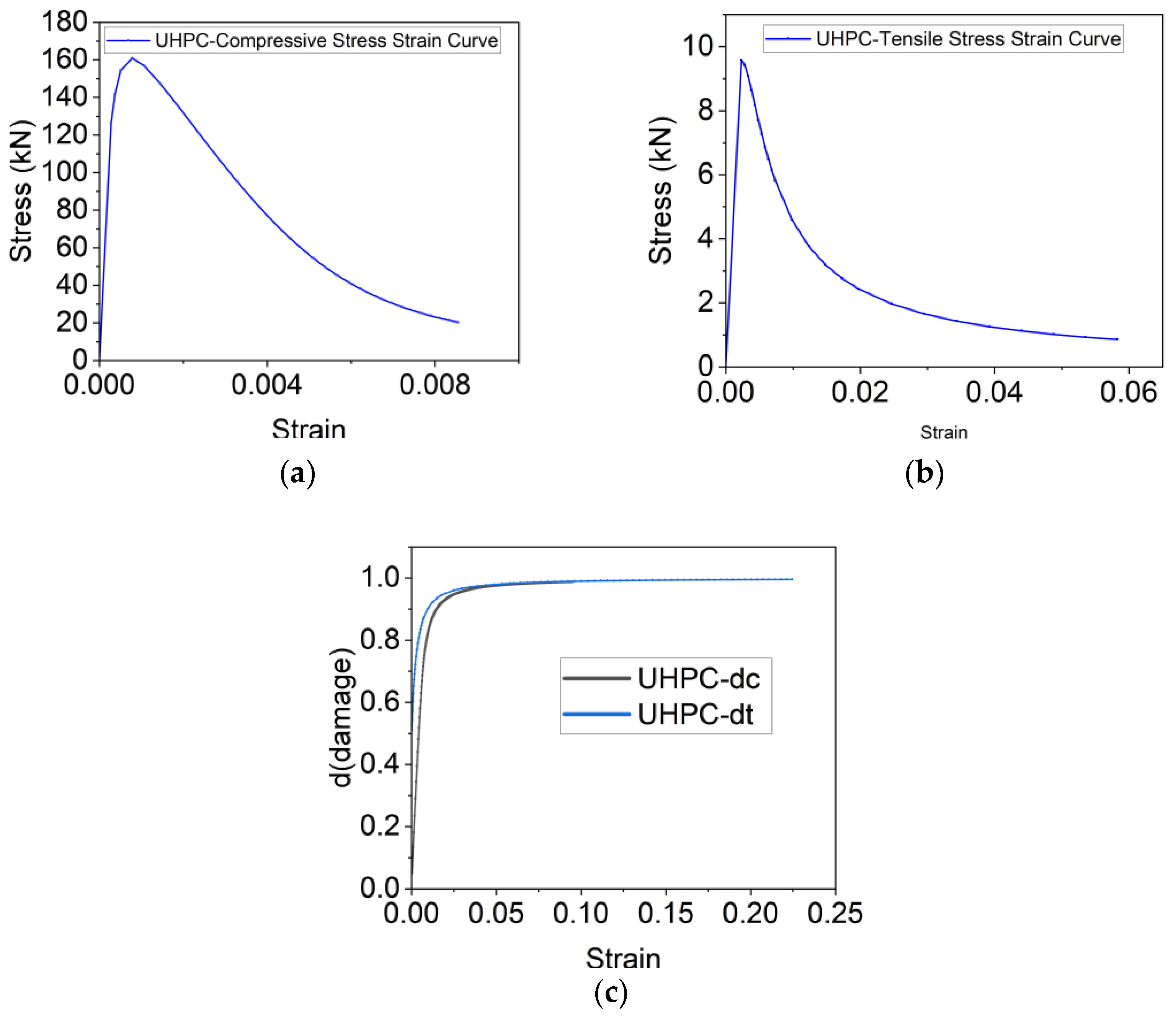




| Cement | Clay | Silica Fume | Fly Ash Microbeads | Water Reducing Agent | Water | Steel Fiber |
|---|---|---|---|---|---|---|
| 810 | 667 | 200 | 190 | 34 | 204 | 160 |
| Cement | Fly Ash Microbeads | Silica Fume | Quartz Sand (Mesh) | Steel Fiber | Water Reducing Agent | Expanding Agent | Water | ||
|---|---|---|---|---|---|---|---|---|---|
| 20–40 | 40–80 | 80–120 | |||||||
| 760 | 200 | 160 | 400 | 350 | 200 | 200 | 22 | 100 | 180 |
| Concrete Type | Density kg/m3 | Tensile Strength MPa | Compressive Strength MPa | Young’s Modulus GPa | Poisson’s Ratio |
|---|---|---|---|---|---|
| LUHPC | 2045 | 5.65 | 139 | 41.75 | 0.2 |
| UHPC | 2800 | 9.56 | 161 | 50.1 | 0.2 |
| Steel Type | Standard | Yield Strength MPa | Ultimate Strength MPa | Young’s Modulus Gpa | Poisson’s Ratio |
|---|---|---|---|---|---|
| OSD plates | Q425 | 425 | 525 | 206 | 0.3 |
| Reinforcement | HRB400 | 400 | 570 | 206 | 0.3 |
| Headed studs | h = 35 mm dia = 13 mm | 425 | 525 | 206 | 0.3 |
| Specimen | Yield Deflection (mm) | Ultimate Deflection (mm) | Deflection Ductility Coefficient μΔ |
|---|---|---|---|
| OS-LUHPC-CBD (12 mm steel deck) | 17.2 | 84.49 | 4.912 |
| OS-LUHPC-CBD (16 mm steel deck) | 16.3 | 78.43 | 4.813 |
| OS-UHPC-CBD (12 mm steel deck) | 16.01 | 77.4 | 4.834 |
| OS-UHPC-CBD (16 mm steel deck) | 16.91 | 68.18 | 4.032 |
| Concrete | Density kg/m3 | Tensile Strength Mpa | Compressive Strength Mpa | Young’s Modulus Gpa | Poisson’s Ratio |
|---|---|---|---|---|---|
| LUHPC | 2045 (Ding et al., 2019) [24] | 5.65 | 139 | 41.75 | 0.2 |
| UHPC | 2800 | 9.58 | 160.8 | 50.1 | 0.2 |
| Concrete | Dilation Angle | Eccentricity | fb0/fc0 | k | Viscosity |
|---|---|---|---|---|---|
| LUHPC | 51 | 0.1 | 1.1 | 0.66 | 0 |
| UHPC | 54 | 0.1 | 1.1 | 0.66 | 0 |
| Design Parameter | Symbol | Values Considered (mm) |
|---|---|---|
| Steel deck plate thickness (mm) | ts | 12, 14, 16 |
| Trapezoidal rib thickness (mm) | th | 8–10 |
| UHPC slab thickness (mm) | ts | 50, 60, 70 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Worku, Z.; Liu, M.; Wang, X.; Sheng, G. Flexural Behavior of Orthotropic Steel–LUHPC Composite Bridge Decks: Experimental and Numerical Study. Materials 2025, 18, 2106. https://doi.org/10.3390/ma18092106
Worku Z, Liu M, Wang X, Sheng G. Flexural Behavior of Orthotropic Steel–LUHPC Composite Bridge Decks: Experimental and Numerical Study. Materials. 2025; 18(9):2106. https://doi.org/10.3390/ma18092106
Chicago/Turabian StyleWorku, Zebene, Muyu Liu, Xin Wang, and Guangzu Sheng. 2025. "Flexural Behavior of Orthotropic Steel–LUHPC Composite Bridge Decks: Experimental and Numerical Study" Materials 18, no. 9: 2106. https://doi.org/10.3390/ma18092106
APA StyleWorku, Z., Liu, M., Wang, X., & Sheng, G. (2025). Flexural Behavior of Orthotropic Steel–LUHPC Composite Bridge Decks: Experimental and Numerical Study. Materials, 18(9), 2106. https://doi.org/10.3390/ma18092106






