Anisotropy in Creep Behavior of a Directionally Solidified Ni-Based Superalloy at 980 °C and 1070 °C
Abstract
:1. Introduction
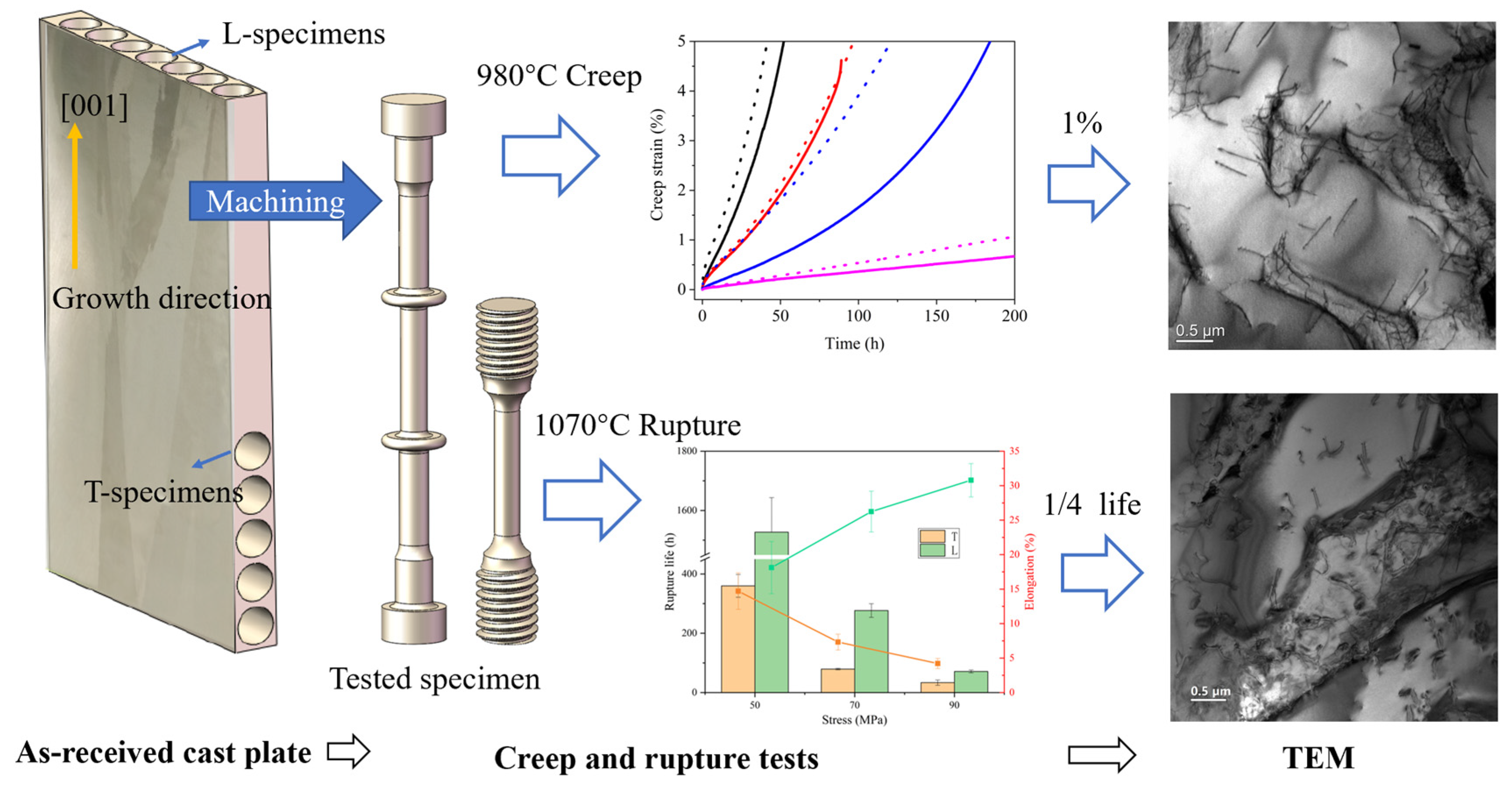
2. Materials and Methods
3. Results
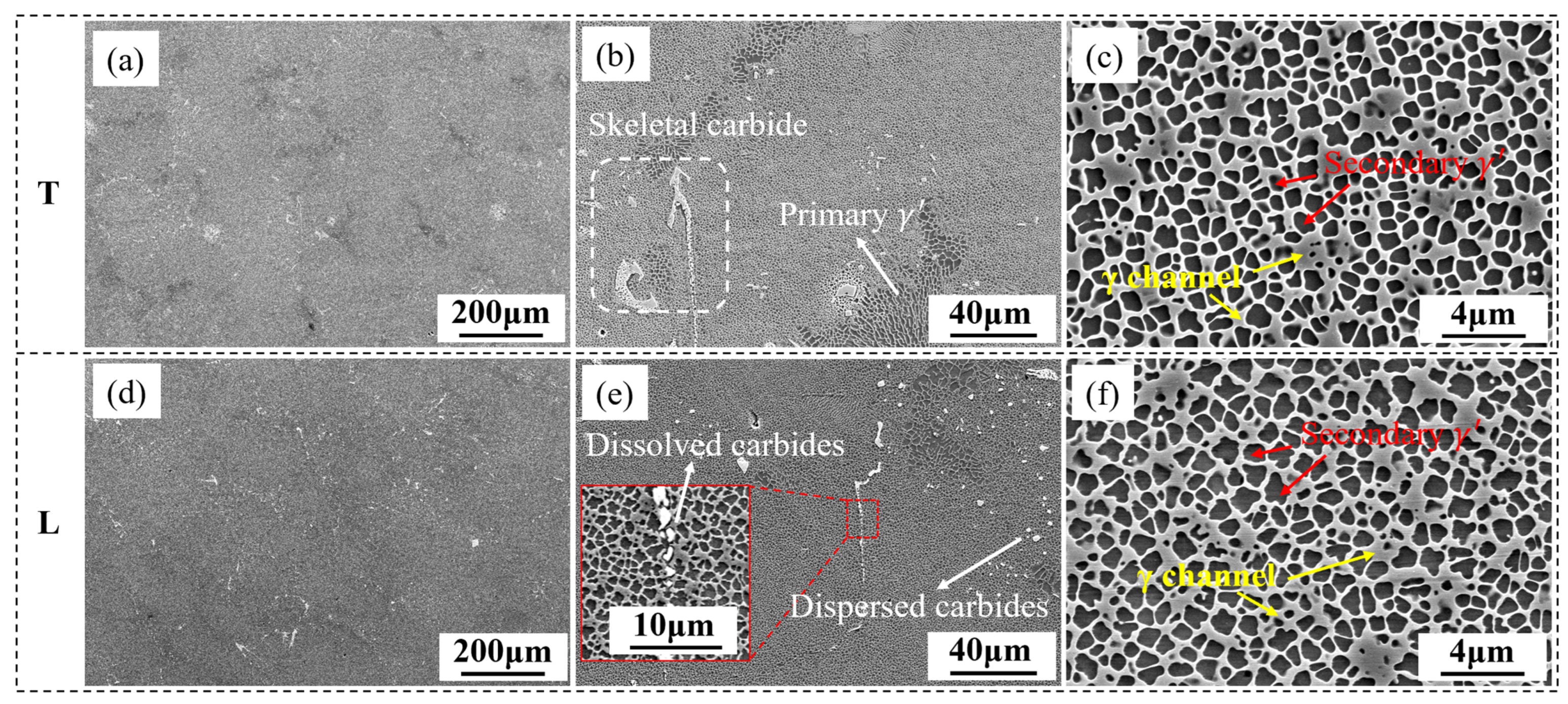
3.1. Original Microstructure
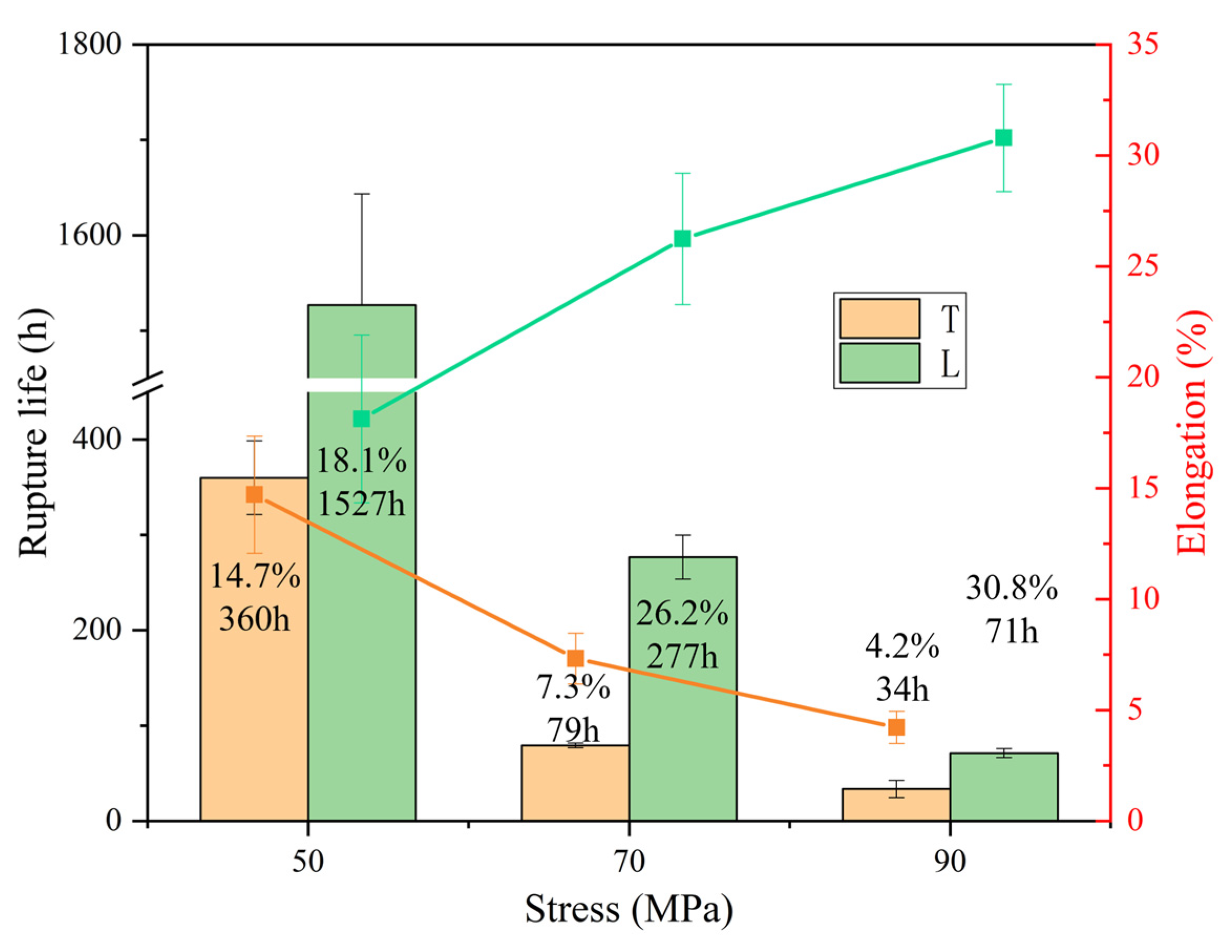
3.2. Creep/Rupture Properties at 980 °C/1070 °C
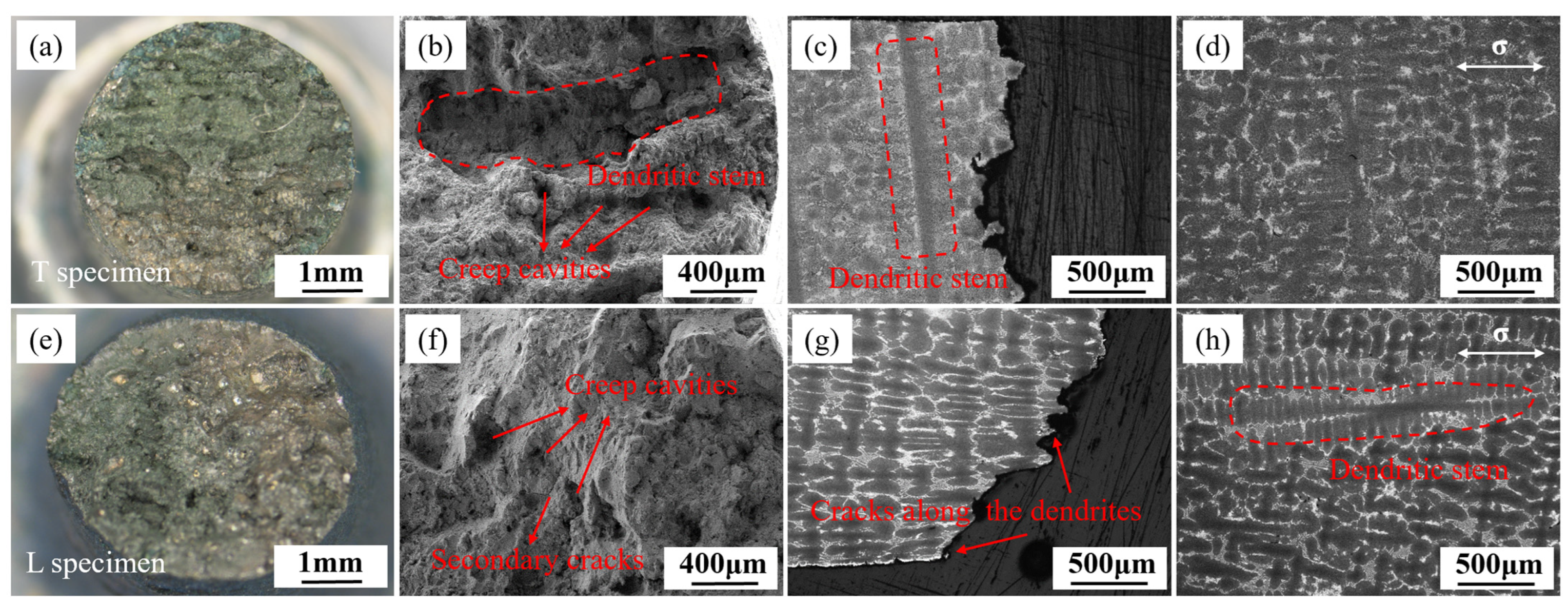
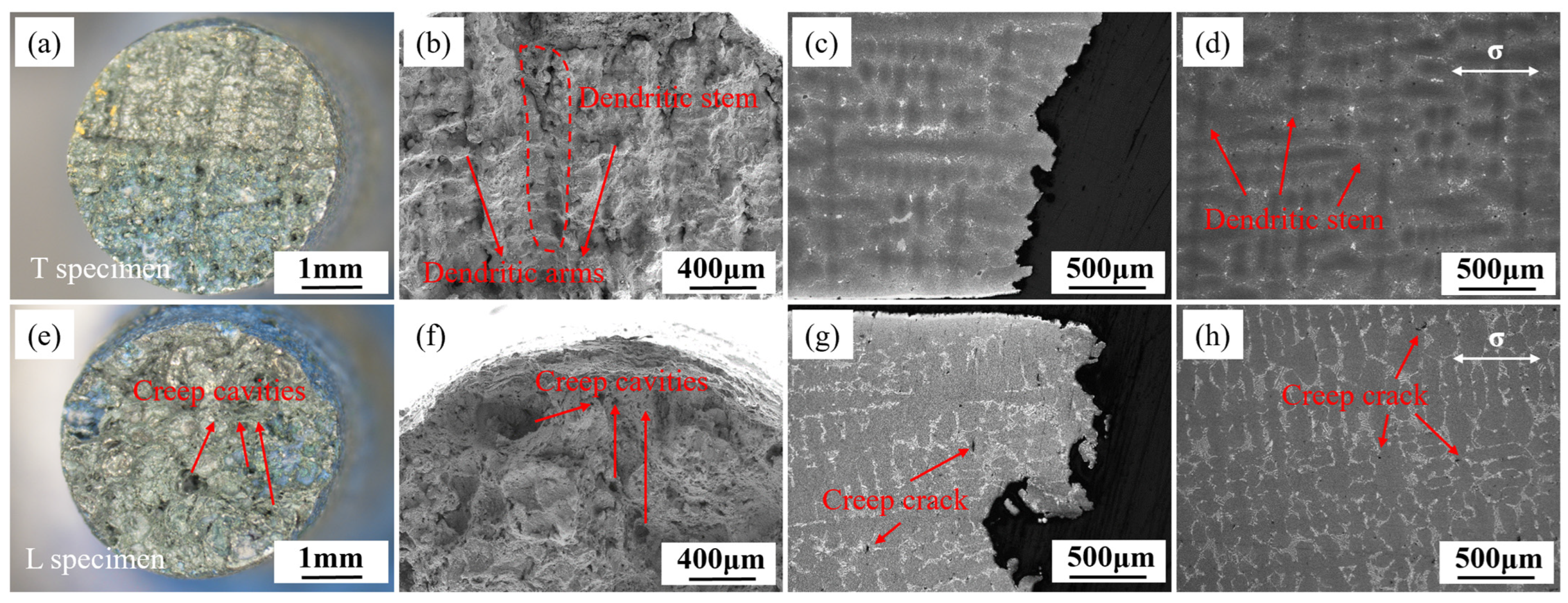
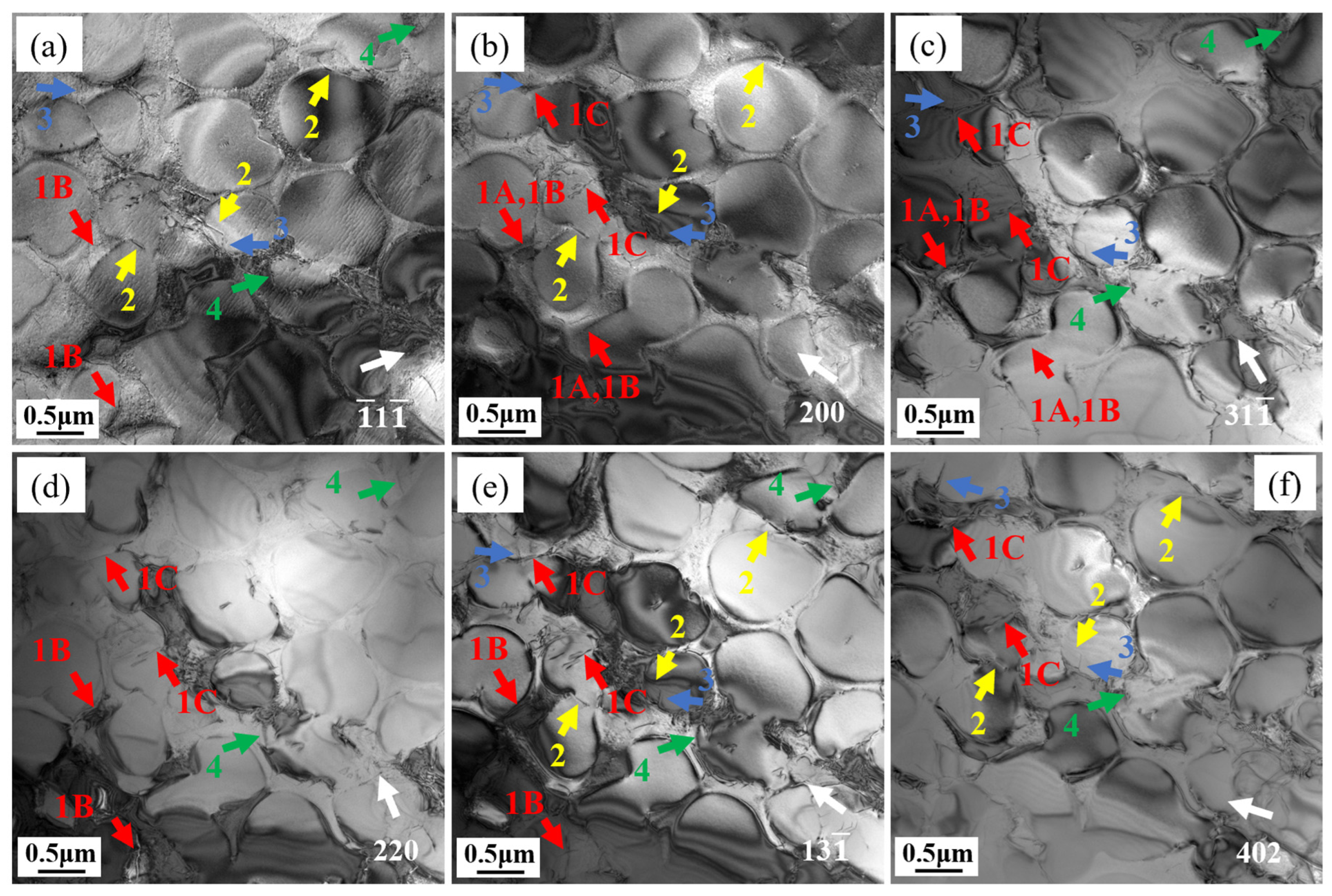
3.3. Microstructural Characteristics of Fractures
4. Discussion
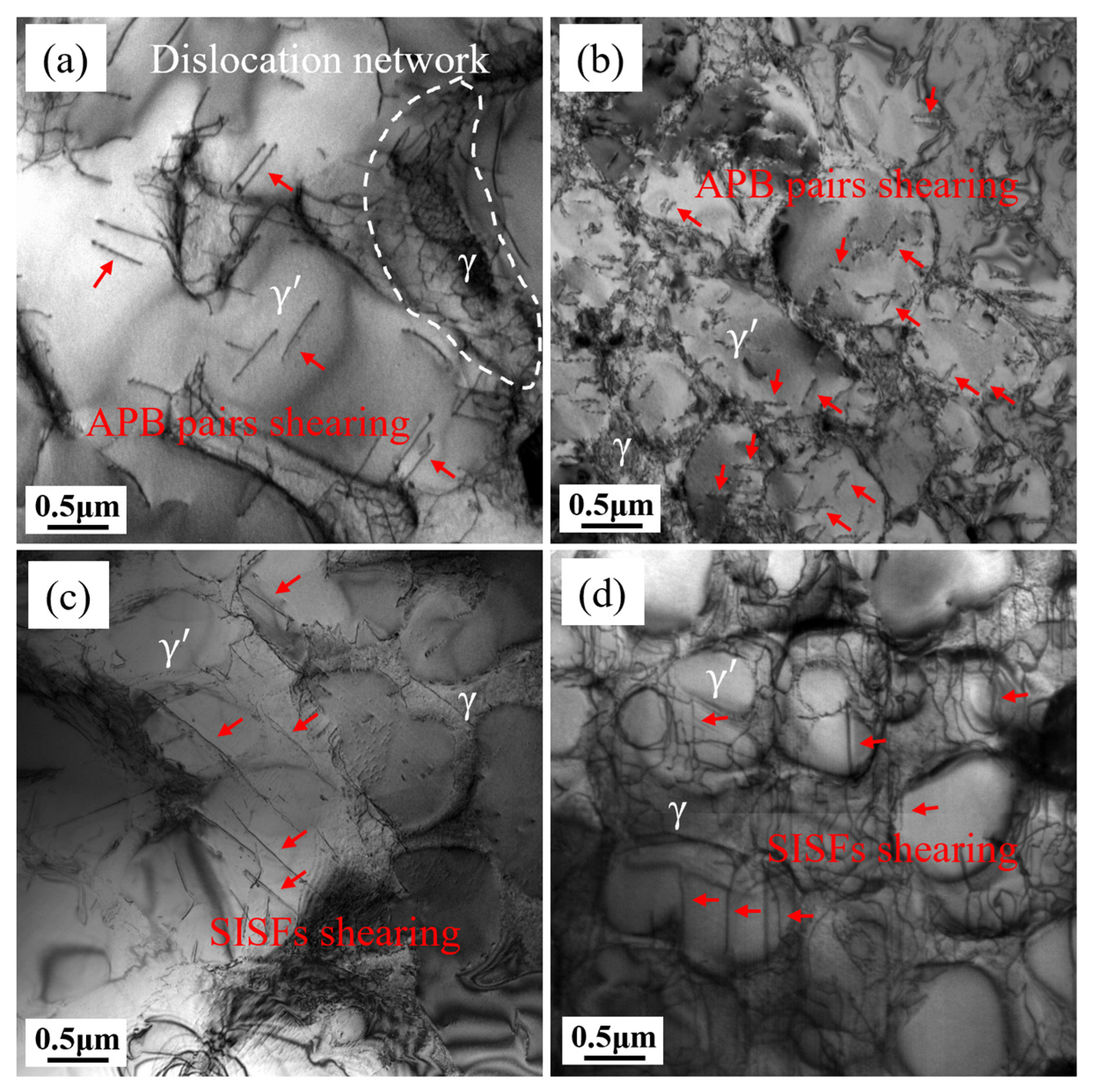
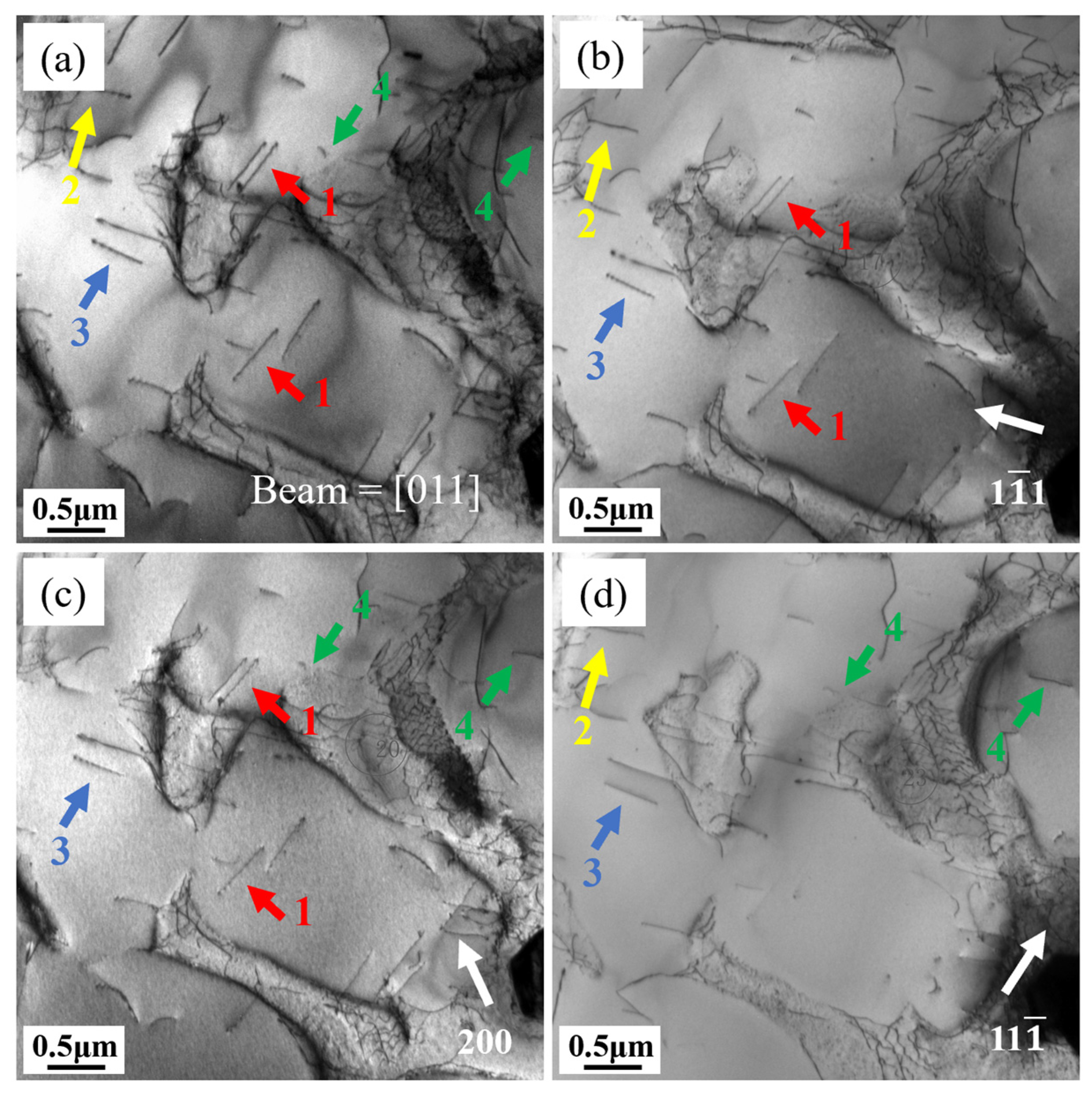
4.1. Deformation Mechanisms
4.1.1. Deformation Mechanisms of Creep at 980 °C/140 MPa
4.1.2. Deformation Mechanisms of Rupture at 1070 °C/50 MPa
4.1.3. Summary of Creep–Rupture Deformation Mechanisms
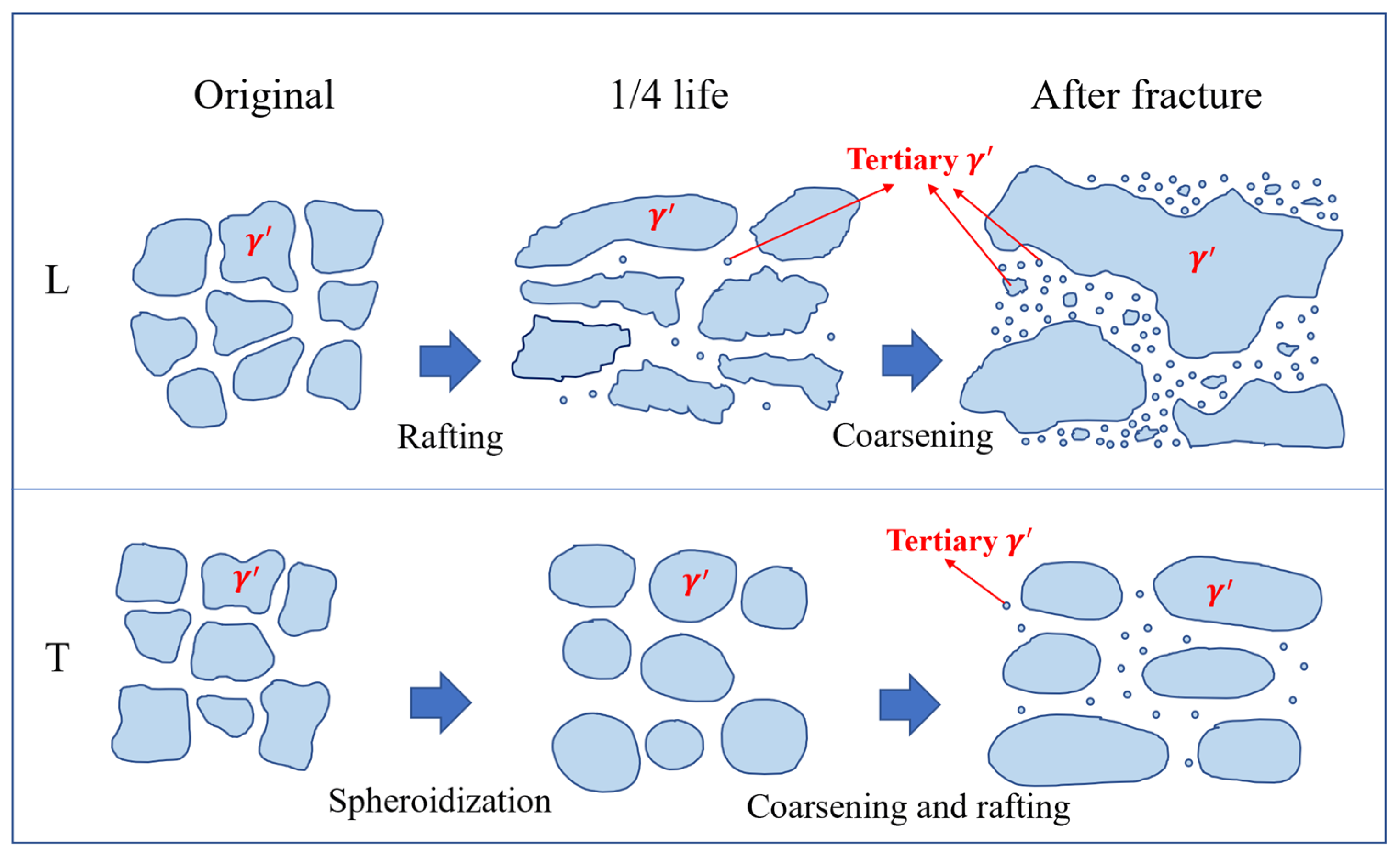
4.2. Microstructural Evolution
5. Conclusions
- (1)
- At 980 °C, the primary creep mechanism in the L specimen involves the operation of multiple <110>{111} slip systems, accompanied by APB pairs cutting through the γ′ phase. In contrast, the T specimen exhibits a combination of <110>{111} and <112>{111} slip system activation, where both APB and SISFs shearing take place concurrently. When the temperature increases to 1070 °C, the L specimen’s deformation behavior shifts to a combination of γ′ dislocation shearing and tertiary γ′ bypassing by dislocations. Meanwhile, the T specimen demonstrates dislocation climb within the γ channels along with dislocation shearing of the γ′.
- (2)
- Under creep conditions at 980 °C, neither the L nor T specimens demonstrated notable rafting behavior. However, the γ′ phase in the L specimen underwent coarsening, increasing from an initial size of 810 nm to 1470 nm. When subjected to rupture testing at 1070 °C, the L specimen displayed pronounced N-type rafting, accompanied by the formation of numerous tertiary γ′ within the widened γ channels. In contrast, the T specimen revealed distinct Ostwald ripening features, with a calculated ripening rate constant of 1.04 × 10−20 m3/h.
- (3)
- Nevertheless, this research is not without its limitations. One such constraint is the anisotropy of creep was not correlated with life prediction. In fact, a unified life prediction model for different orientations can be established through viscoplasticity theory. These also point the direction for our future research.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Reed, R. The Superalloys: Fundamentals and Applications; Cambridge University Press: Cambridge, UK, 2006; pp. 1–372. Available online: https://scholar.google.com/scholar_lookup?title=The+Superalloys:+Fundamentals+and+Applications&author=Reed,+R.&publication_year=2006 (accessed on 15 March 2025).
- Li, Y.M.; Tan, Z.H.; Guo, H.Y.; Wang, X.G.; Li, J.G.; Zhou, Y.Z.; Sun, X.F. In Enhancing Economic Affordability of Aircraft Engines: Development of a Low-Cost Single Crystal Superalloy DD93 Containing 3 Wt.% Re, Designed to Meet Third-Generation Superalloys Properties. In International Symposium on Superalloys; Springer: Berlin/Heidelberg, Germany, 2024; pp. 176–185. [Google Scholar] [CrossRef]
- Leng, F.; Lv, P.; Liu, L.R.; Zhao, Y.S.; Zhang, J. Creep properties of a low-cost 3rd generation nickel-based single crystal superalloy with orientation deviated from [001] direction. Mater. Sci. Eng. A 2024, 915, 147272. [Google Scholar] [CrossRef]
- Godha, A.; Das, D.; Ghosal, P.; Makineni, S.K. New insights on the interaction of solutes with the defects during creep deformation of CMSX4 Ni-based single crystal superalloy. Acta Mater. 2024, 281, 120360. [Google Scholar] [CrossRef]
- Wu, X.; Wollgramm, P.; Somsen, C.; Dlouhy, A.; Kostka, A.; Eggeler, G. Double minimum creep of single crystal Ni-base superalloys. Acta Mater. 2016, 112, 242–260. [Google Scholar] [CrossRef]
- Tan, Y.G.; Zhang, W.W.; Gao, N.; Reed, P. The finding of anisotropic compatibility of grain boundaries in a directionally solidified superalloy. J. Mater. Sci. 2024, 59, 11533–11545. [Google Scholar] [CrossRef]
- Wang, Z.C.; Li, J.R.; Liu, S.Z.; Zhao, J.Q.; Wang, X.G.; Yang, W.P. Investigation on freckle formation of nickel-based single crystal superalloy specimens with suddenly reduced cross section. J. Alloys Compd. 2022, 918, 165631. [Google Scholar] [CrossRef]
- Bortoluci Ormastroni, L.M. Crack Initiation Mechanisms in Very High Cycle Fatigue at High Temperatures of Nibased Single Crystal Superalloys: Transition from Internal Sites to the Surface. Ecole Nationale Superieure de Mechanique et de Aerotechnique. 2023. Available online: https://elib.dlr.de/191246/ (accessed on 15 March 2025).
- Wang, Z.C.; Li, J.R.; Liu, S.Z.; Yang, W.P.; Wang, X.G. Effects of shell mold heating temperature on microstructures and freckle formation of single crystal superalloys. Trans. Nonferrous Met. Soc. China 2024, 34, 1191–1203. [Google Scholar] [CrossRef]
- Lee, J.S.; Gu, J.H.; Jung, H.M.; Kim, E.H.; Jung, Y.G.; Lee, J.H. Directional solidification microstructure control in CM247LC superalloy. Mater. Today Proc. 2014, 1, 3–10. [Google Scholar] [CrossRef]
- Rottwinkel, B.; Pereira, A.; Alfred, I.; Noelke, C.; Wesling, V.; Kaierle, S. Turbine blade tip single crystalline clad deposition with applied remelting passes for well oriented volume extension. J. Laser Appl. 2017, 29, 022310. [Google Scholar] [CrossRef]
- Zhang, H.; Wen, W.; Cui, H.; Xu, Y. A modified Zerilli–Armstrong model for alloy IC10 over a wide range of temperatures and strain rates. J. Mater. Sci. Eng. A 2009, 527, 328–333. [Google Scholar] [CrossRef]
- Chiou, M.S.; Jian, S.R.; Yeh, A.C.; Kuo, C.M.; Juang, J.Y. High temperature creep properties of directionally solidified CM-247LC Ni-based superalloy. Mater. Sci. Eng. A 2016, 655, 237–243. [Google Scholar] [CrossRef]
- Knowles, D.M.; Chen, Q.Z. Superlattice stacking fault formation and twinning during creep in γ/γ′ single crystal superalloy CMSX-4. Mater. Sci. Eng. A 2003, 340, 88–102. [Google Scholar] [CrossRef]
- Guo, J.T.; Yuan, C.; Yang, H.C.; Lupinc, V.; Maldini, M. Creep-rupture behavior of a directionally solidified nickel-base superalloy. Metall. Mater. Trans. A 2001, 32, 1103–1110. [Google Scholar] [CrossRef]
- Li, J.C.; Tian, N.; Zhang, P.; Yu, F.; Zhao, G.Q.; Zhang, P. Creep damage and deformation mechanism of a directionally solidified alloy during moderate-temperature creep. Crystals 2021, 11, 646. [Google Scholar] [CrossRef]
- Rae, C.M.F.; Reed, R.C. Primary creep in single crystal superalloys: Origins, mechanisms and effects. Acta Mater. 2007, 55, 1067–1081. [Google Scholar] [CrossRef]
- Eggeler, G.; Dlouhy, A. On the formation of 〈010〉-dislocations in the γ′-phase of superalloy single crystals during high temperature low stress creep. Acta Mater. 1997, 45, 4251–4262. [Google Scholar] [CrossRef]
- Zhang, P.; Li, J.; Gong, X.F.; Yuan, Y.; Gu, Y.F.; Wang, J.C.; Yan, J.B.; Yin, H.F. Creep behavior and deformation mechanisms of a novel directionally solidified Ni-base superalloy at 900 °C. Mater. Charact. 2019, 148, 201–207. [Google Scholar] [CrossRef]
- Stinville, J.C.; Gallup, K.; Pollock, T.M. Transverse creep of nickel-base superalloy bicrystals. Metall. Mater. Trans. A 2015, 46, 2516–2529. [Google Scholar] [CrossRef]
- Mataveli Suave, L.; Cormier, J.; Villechaise, P.; Bertheau, D.; Benoit, G.; Cailletaud, G.; Marcin, L. Anisotropy in creep properties of DS200+ Hf alloy. Mater. High Temp. 2016, 33, 361–371. [Google Scholar] [CrossRef]
- Gupta, R.; Prasad, M.J.N.V.; Pant, P. Anisotropy in flow behaviour of directionally solidified Ni-based superalloy under compression at an elevated temperature. Materialia 2023, 32, 101920. [Google Scholar] [CrossRef]
- Fernandez-Zelaia, P.; Lee, Y.; Dryepondt, S.; Kirka, M.M. Creep anisotropy modeling and uncertainty quantification of an additively manufactured Ni-based superalloy. Int. J. Plast. 2022, 151, 103177. [Google Scholar] [CrossRef]
- Long, A.P.; Xiong, J.Y.; Wei, B.; Zhang, G.X.; Feng, G.J.; Guo, J.Z.; Liu, R.T. Creep Mechanism and Microstructure Evolution of a Directionally Solidified Ni-Based Superalloy with Different Orientations at 850 °C. Materials 2025, 18, 1540. [Google Scholar] [CrossRef]
- Chen, J.B.; Chen, J.Y.; Wang, Q.J.; Wu, Y.D.; Li, Q.; Xiao, C.B.; Li, S.L.; Wang, Y.D.; Hui, X.D. Enhanced creep resistance induced by minor Ti additions to a second generation nickel-based single crystal superalloy. Acta Mater. 2022, 232, 117938. [Google Scholar] [CrossRef]
- Wei, B.S.; Lin, Y.; Huang, Z.W.; Huang, L.; Zhou, K.C.; Zhang, L.F.; Zhang, L.J. A novel Re-free Ni-based single-crystal superalloy with enhanced creep resistance and microstructure stability. Acta Mater. 2022, 240, 118336. [Google Scholar] [CrossRef]
- Khoei, A.R.; Eshlaghi, G.T.; Shahoveisi, S. Atomistic simulation of creep deformation mechanisms in nickel-based single crystal superalloys. Mater. Sci. Eng. A 2021, 809, 140977. [Google Scholar] [CrossRef]
- Yu, X.M.; Yu, F.Y.; Wu, X.G.; Niu, H.Z.; Meng, L.; Zhang, B.W.; Zhang, D.L. Correlation between microstructural types and creep behavior of a PM near-α Ti–6Al–2Sn–4Zr–2Mo–0.1 Si–0.5 Y alloy. J. Alloys Compd. 2025, 1022, 179957. [Google Scholar] [CrossRef]
- Ghosh, S. Analysis of Creep Deformation. Preprints 2024, 1–25. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, J.Y.; Shang, H.S.; Sha, A.X.; Cheng, Y.Y.; Duan, H.L. Experiment and Modelling of the Pre-Strain Effect on the Creep Behaviour of P/M Ni-Based Superalloy FGH96. Materials 2023, 16, 3874. [Google Scholar] [CrossRef]
- Smith, T.M.; Rao, Y.; Wang, Y.; Ghazisaeidi, M.; Mills, M.J. Diffusion processes during creep at intermediate temperatures in a Ni-based superalloy. Acta Mater. 2017, 141, 261–272. [Google Scholar] [CrossRef]
- Wang, L.N.; Liu, Y.; Yu, J.J.; Xu, Y.; Sun, X.F.; Guan, H.R.; Hu, Z.Q. Orientation and temperature dependence of yielding and deformation behavior of a nickel-base single crystal superalloy. Mater. Sci. Eng. A 2009, 505, 144–150. [Google Scholar] [CrossRef]
- Yang, W.P.; Li, J.R.; Liu, S.Z.; Shi, Z.X.; Zhao, J.Q.; Wang, X.G. Orientation dependence of transverse tensile properties of nickel-based third generation single crystal superalloy DD9 from 760 to 1100 C. Trans. Nonferrous Met. Soc. China 2019, 29, 558–568. [Google Scholar] [CrossRef]
- Ardakani, M.G.; McLean, M.; Shollock, B.A. Twin formation during creep in single crystals of nickel-based superalloys. Acta Mater. 1999, 47, 2593–2602. [Google Scholar] [CrossRef]
- Pelleg, J. Introduction to Dislocations. In Mechanical Properties of Materials, 1st ed.; Springer: Dordrecht, The Netherlands, 2012; pp. 85–146. [Google Scholar] [CrossRef]
- Yoo, M.H.; King, A.H. Slip, twinning, and fracture at a grain boundary in the L1 2 ordered structure—AΣ= 9 tilt boundary. J. Mater. Res. 1988, 3, 848–855. [Google Scholar] [CrossRef]
- Shenoy, M.; Tjiptowidjojo, Y.; McDowell, D. Microstructure-sensitive modeling of polycrystalline IN 100. Int. J. Plast. 2008, 24, 1694–1730. [Google Scholar] [CrossRef]
- Wang, G.Y.; Zhang, S.; Tian, S.G.; Tian, N.; Zhao, G.Q.; Yan, H.J. Microstructure evolution and deformation mechanism of a [111]-oriented nickel-based single-crystal superalloy during high-temperature creep. J. Mater. Res. Technol. 2022, 16, 495–504. [Google Scholar] [CrossRef]
- Yang, H.; Li, Z.H.; Huang, M.S. Modeling dislocation cutting the precipitate in nickel-based single crystal superalloy via the discrete dislocation dynamics with SISF dissociation scheme. Comput. Mater. Sci. 2013, 75, 52–59. [Google Scholar] [CrossRef]
- Baker, I.; Schulson, E.M. The effect of temperature on dislocation structures in Ni3Al. Phys. Status Solidi A 1985, 89, 163–172. [Google Scholar] [CrossRef]
- Veyssiere, P.; Douin, J.; Beauchamp, P. On the presence of super lattice intrinsic stacking faults in plastically deformed Ni3Al. Philos. Mag. A 1985, 51, 469–483. [Google Scholar] [CrossRef]
- Yoo, M.H. Stability of superdislocations and shear faults in L12 ordered alloys. Acta Metall. 1987, 35, 1559–1569. [Google Scholar] [CrossRef]
- Milligan, W.W.; Antolovich, S.D. The mechanisms and temperature dependence of superlattice stacking fault formation in the single-crystal superalloy PWA 1480. Metall. Trans. A 1991, 22, 2309–2318. [Google Scholar] [CrossRef]
- Rémy, L.; Pineau, A.; Thomas, B. Temperature dependence of stacking fault energy in close-packed metals and alloys. Mater. Sci. Eng. 1978, 36, 47–63. [Google Scholar] [CrossRef]
- Yu, H.; Xu, W.; van der Zwaag, S. Microstructure and dislocation structure evolution during creep life of Ni-based single crystal superalloys. J. Mater. Sci. Technol. 2020, 45, 207–214. [Google Scholar] [CrossRef]
- Zhang, W.Q.; Chen, S.; Guo, G.H.; Liu, X.; Zhang, B.; Ye, X.J.; Wang, J.; Zhang, Y.F.; Zhao, X.B.; Zhang, Z. Study on plastic deformation mechanism of a fourth-generation nickel-based single crystal superalloy: In-situ SEM characterization and dislocation density-based model. Mater. Sci. Eng. A 2025, 930, 148132. [Google Scholar] [CrossRef]
- Yang, H.; Huang, M.S.; Li, Z.H. The influence of vacancies diffusion-induced dislocation climb on the creep and plasticity behaviors of nickel-based single crystal superalloy. Comput. Mater. Sci. 2015, 99, 348–360. [Google Scholar] [CrossRef]
- Ai, C.; Zhao, X.B.; Zhou, J.; Zhang, H.; Liu, L.; Pei, Y.L.; Li, S.S.; Gong, S.K. Application of a modified Ostwald ripening theory in coarsening of γ′ phases in Ni based single crystal superalloys. J. Alloys Compd. 2015, 632, 558–562. [Google Scholar] [CrossRef]
- Baldan, A. Review Progress in Ostwald ripening theories and their applications to the γ′-precipitates in nickel-base superalloys Part II Nickel-base superalloys. J. Mater. Sci. 2002, 37, 2379–2405. [Google Scholar] [CrossRef]
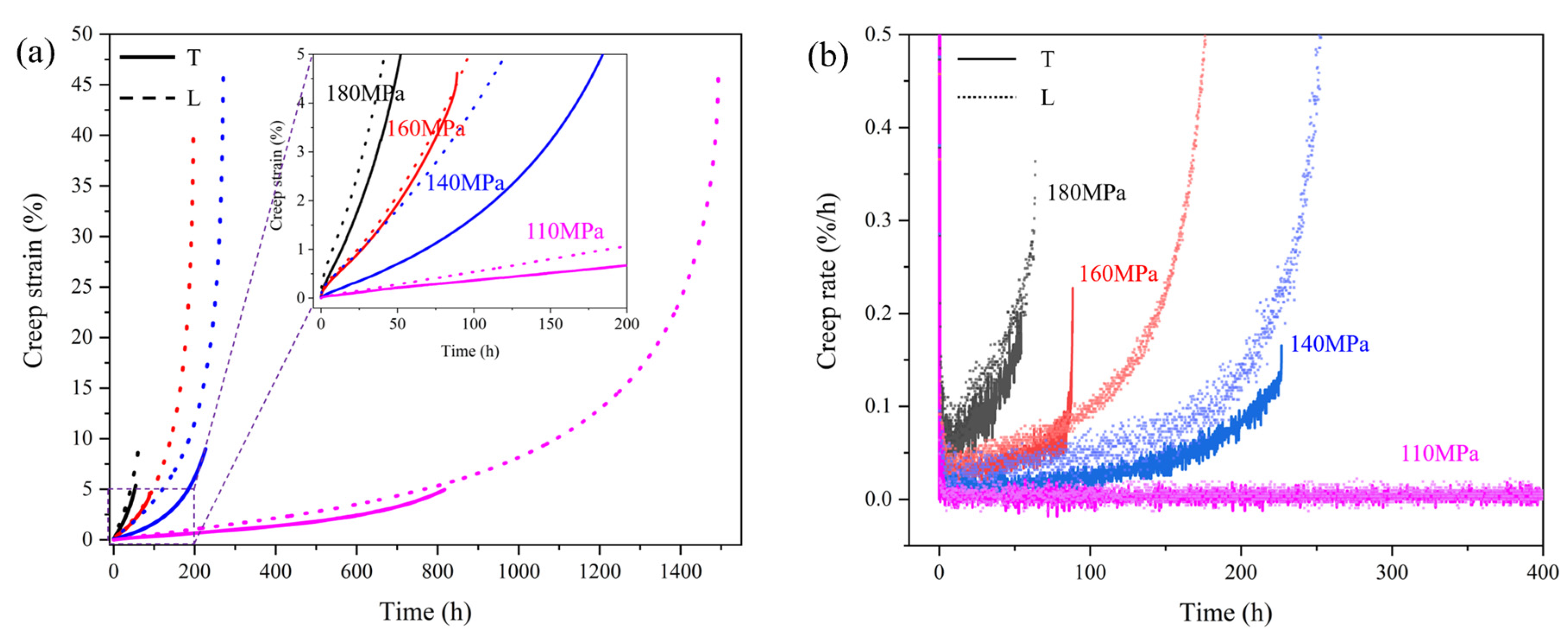




| Specimens | 110 MPa | 140 MPa | 160 MPa | 180 MPa | |
|---|---|---|---|---|---|
| T | Creep life, h | 794 ± 23 | 246 ± 19 | 86 ± 3.6 | 56 ± 1.8 |
| minimum creep rates, %/h | 0.0061 | 0.0113 | 0.0184 | 0.0599 | |
| L | Creep life, h | 1456 ± 37 | 272 ± 1.2 | 194 ± 7.5 | 64 ± 0.6 |
| minimum creep rates, %/h | 0.0086 | 0.0339 | 0.0438 | 0.1285 |
| Beam | g | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| [011] | 11 | √ | √ | √ | × |
| √ | × | √ | √ | ||
| 11 | × | √ | √ | √ | |
| Burgers vectors b | or | Unknow |
| Beam | g | 1A | 1B | 1C | 2 | 3 | 4 |
|---|---|---|---|---|---|---|---|
| [011] | × | √ | × | √ | √ | √ | |
| 200 | √ | √ | √ | √ | √ | × | |
| √ | √ | √ | × | √ | √ | ||
| [12] | 220 | × | √ | √ | × | × | √ |
| × | √ | √ | √ | √ | √ | ||
| 402 | × | × | √ | √ | √ | √ | |
| Burgers vectors b |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Long, A.; Liu, X.; Cheng, J.; Xiong, J.; Feng, G.; Guo, J.; Liu, R. Anisotropy in Creep Behavior of a Directionally Solidified Ni-Based Superalloy at 980 °C and 1070 °C. Materials 2025, 18, 1998. https://doi.org/10.3390/ma18091998
Long A, Liu X, Cheng J, Xiong J, Feng G, Guo J, Liu R. Anisotropy in Creep Behavior of a Directionally Solidified Ni-Based Superalloy at 980 °C and 1070 °C. Materials. 2025; 18(9):1998. https://doi.org/10.3390/ma18091998
Chicago/Turabian StyleLong, Anping, Xiaoshan Liu, Junyi Cheng, Jiangying Xiong, Ganjiang Feng, Jianzheng Guo, and Rutie Liu. 2025. "Anisotropy in Creep Behavior of a Directionally Solidified Ni-Based Superalloy at 980 °C and 1070 °C" Materials 18, no. 9: 1998. https://doi.org/10.3390/ma18091998
APA StyleLong, A., Liu, X., Cheng, J., Xiong, J., Feng, G., Guo, J., & Liu, R. (2025). Anisotropy in Creep Behavior of a Directionally Solidified Ni-Based Superalloy at 980 °C and 1070 °C. Materials, 18(9), 1998. https://doi.org/10.3390/ma18091998






