Thermo-Mechanical Phase-Field Modeling of Fracture in High-Burnup UO2 Fuels Under Transient Conditions
Abstract
:1. Introduction
2. Methodology
2.1. Multiphysics Phase-Field Modeling of Thermo-Mechanical Fracture as Phase Transition
2.1.1. Phase-Field Modeling of Fracture
2.1.2. Mechanical Equilibrium Equations
2.1.3. Heat Equation and Thermal Conductivity Correlation
2.1.4. Model Parameters
3. Results and Discussion
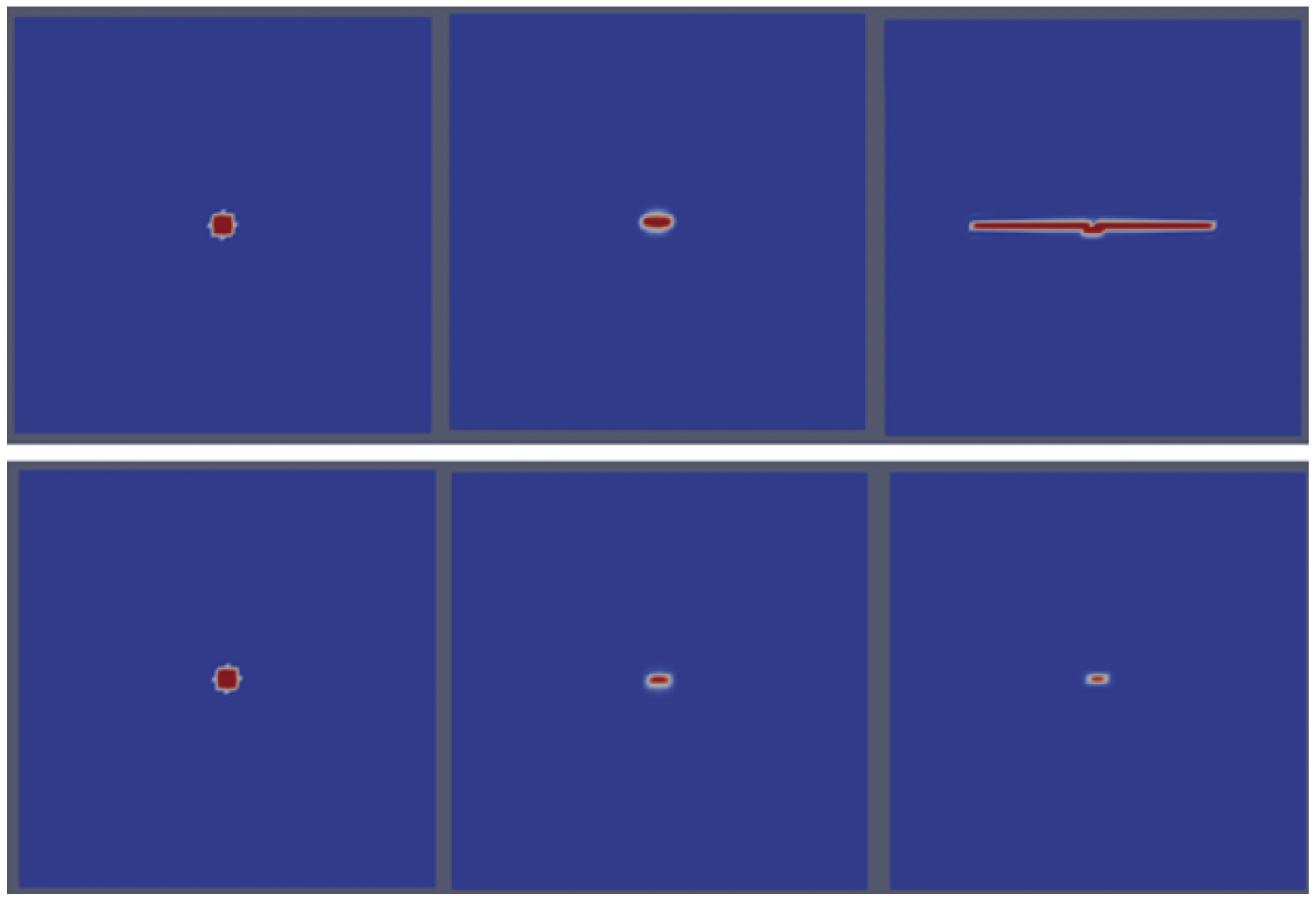
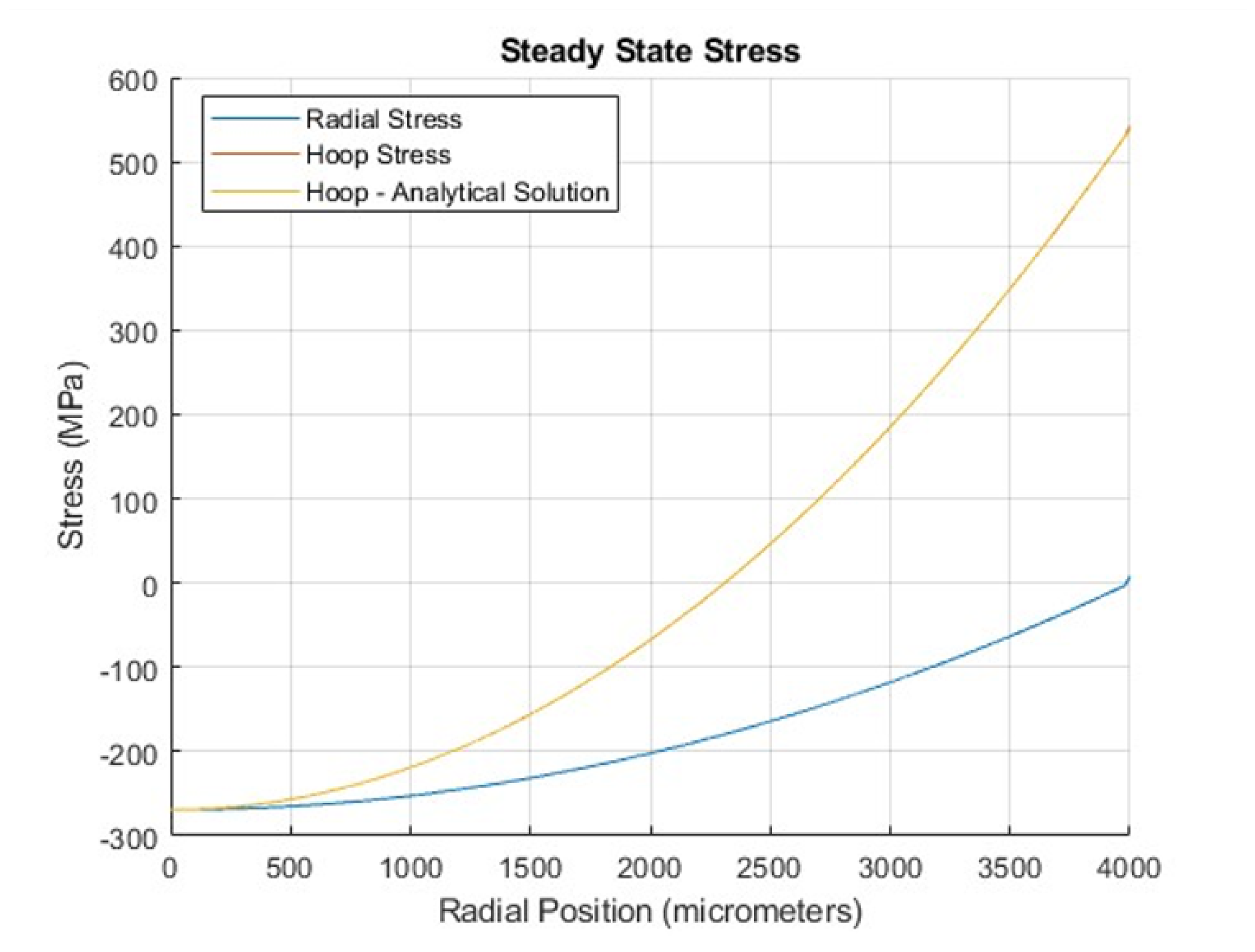
3.1. Benchmark Studies of Crack Initiation and Propagation
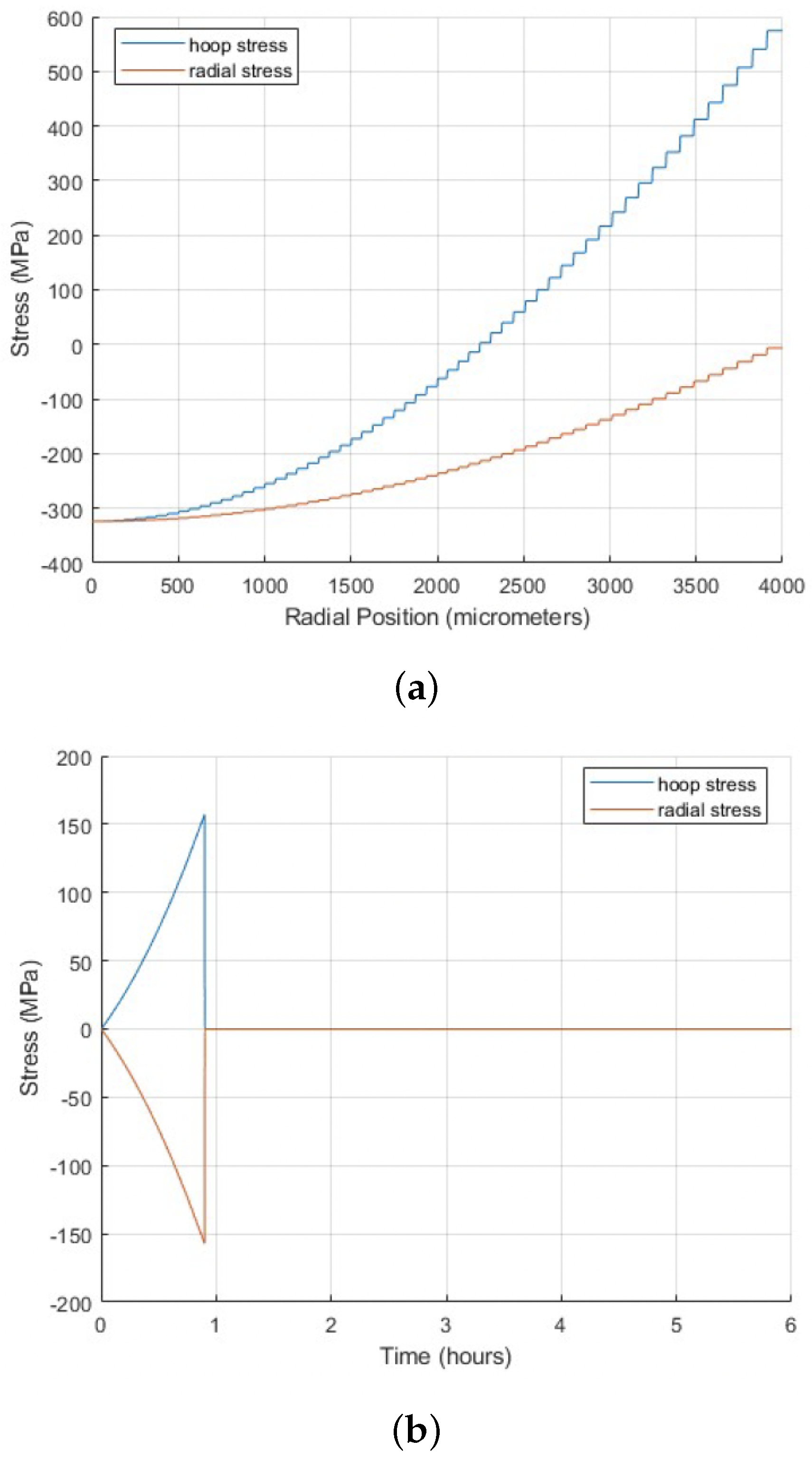
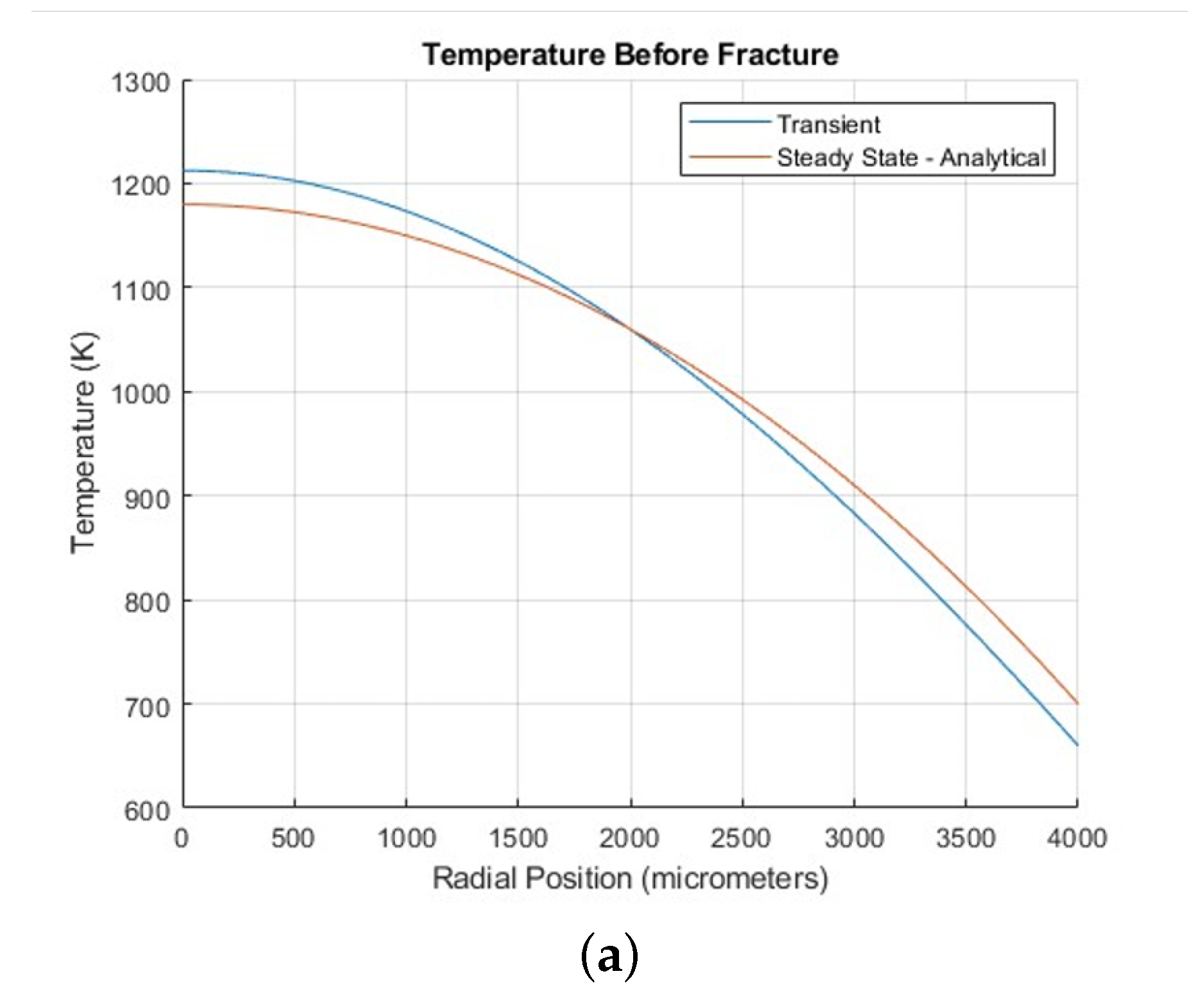
3.2. Transient Conditions: Start-Up and Power Ramp Phase
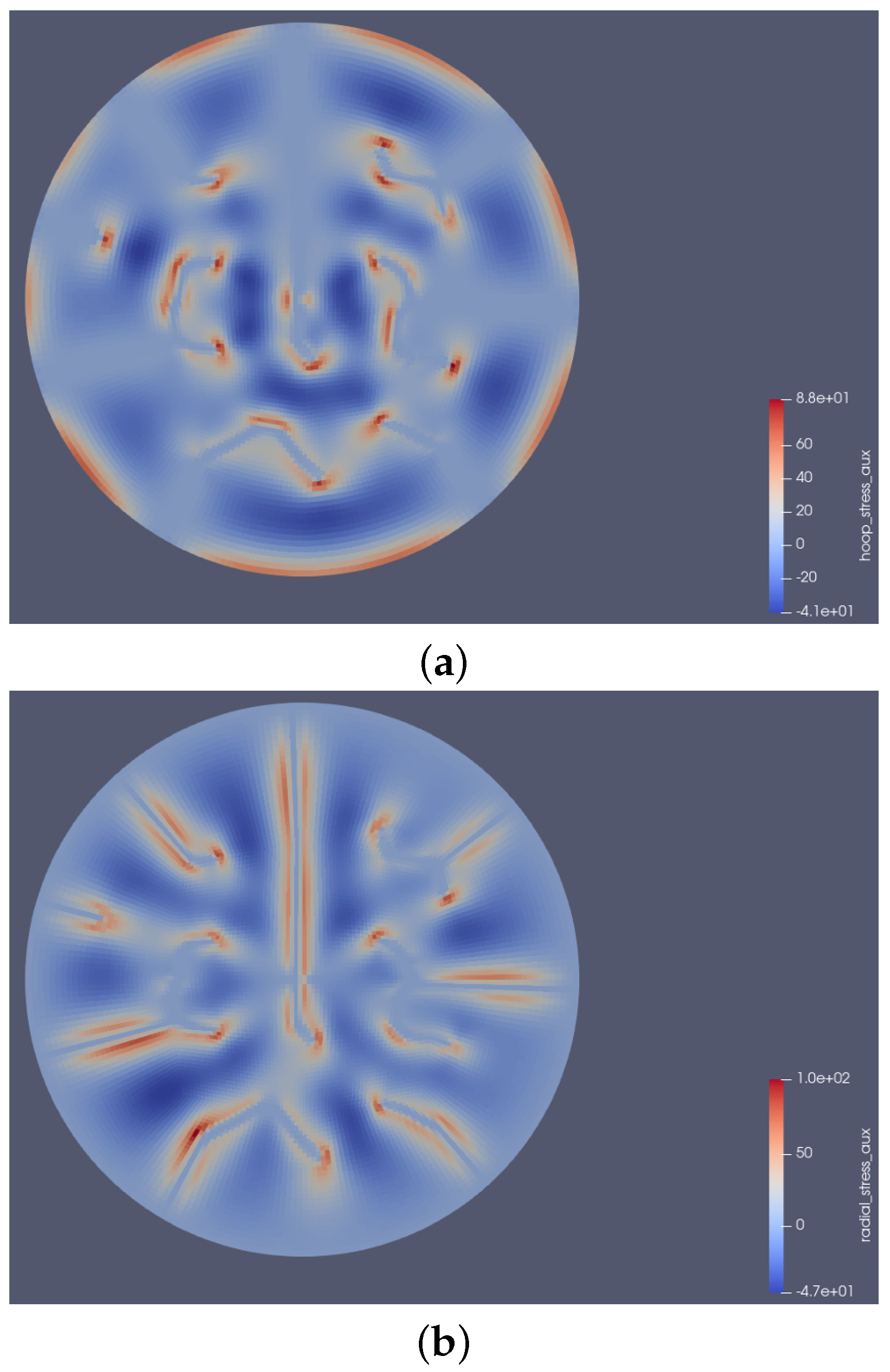
3.3. Modeling Distinct Fracture Patterns in UO2 Pellets
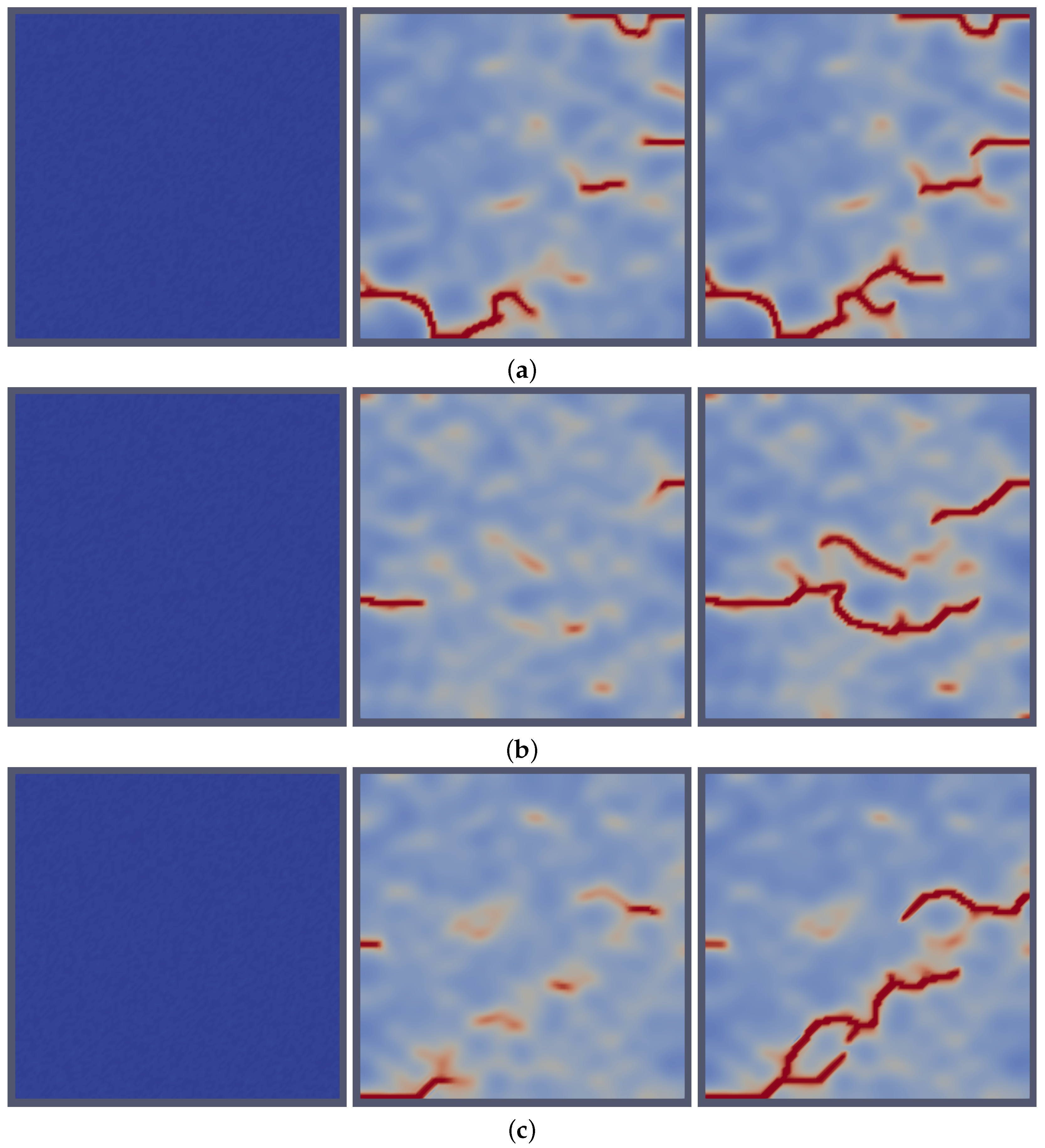
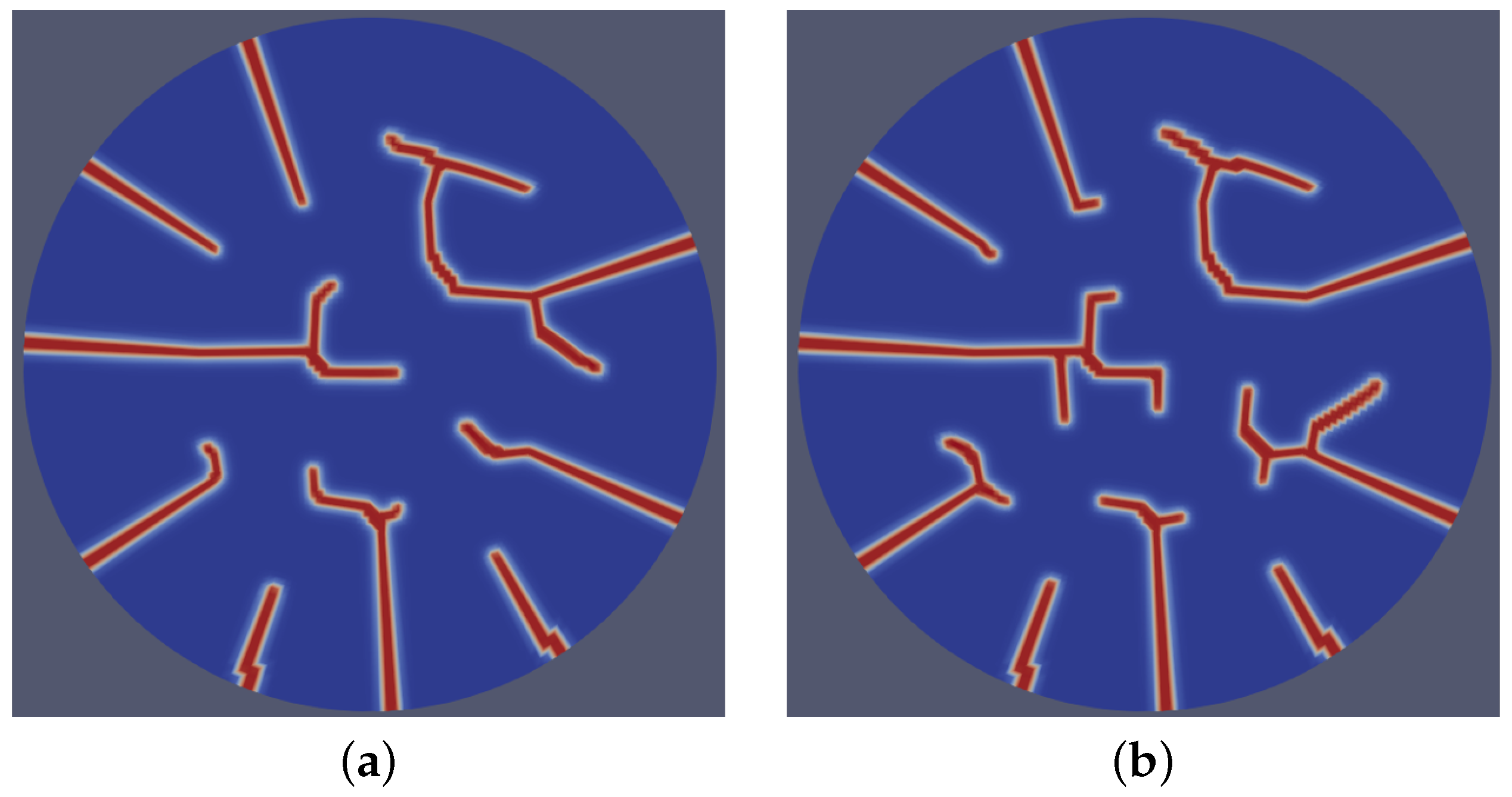
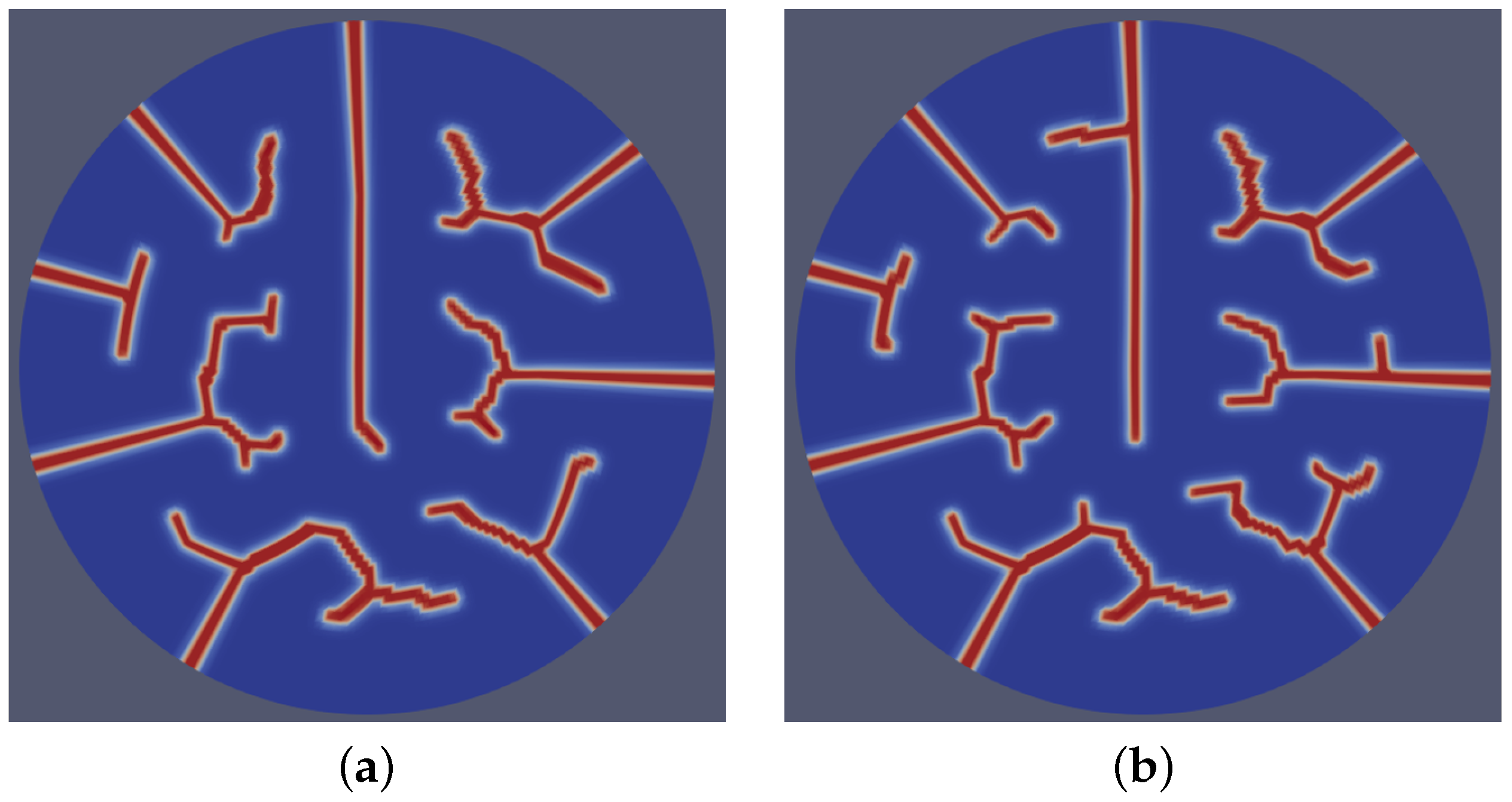
3.3.1. Incorporating the Effect of Randomness in Microstructure Evolution
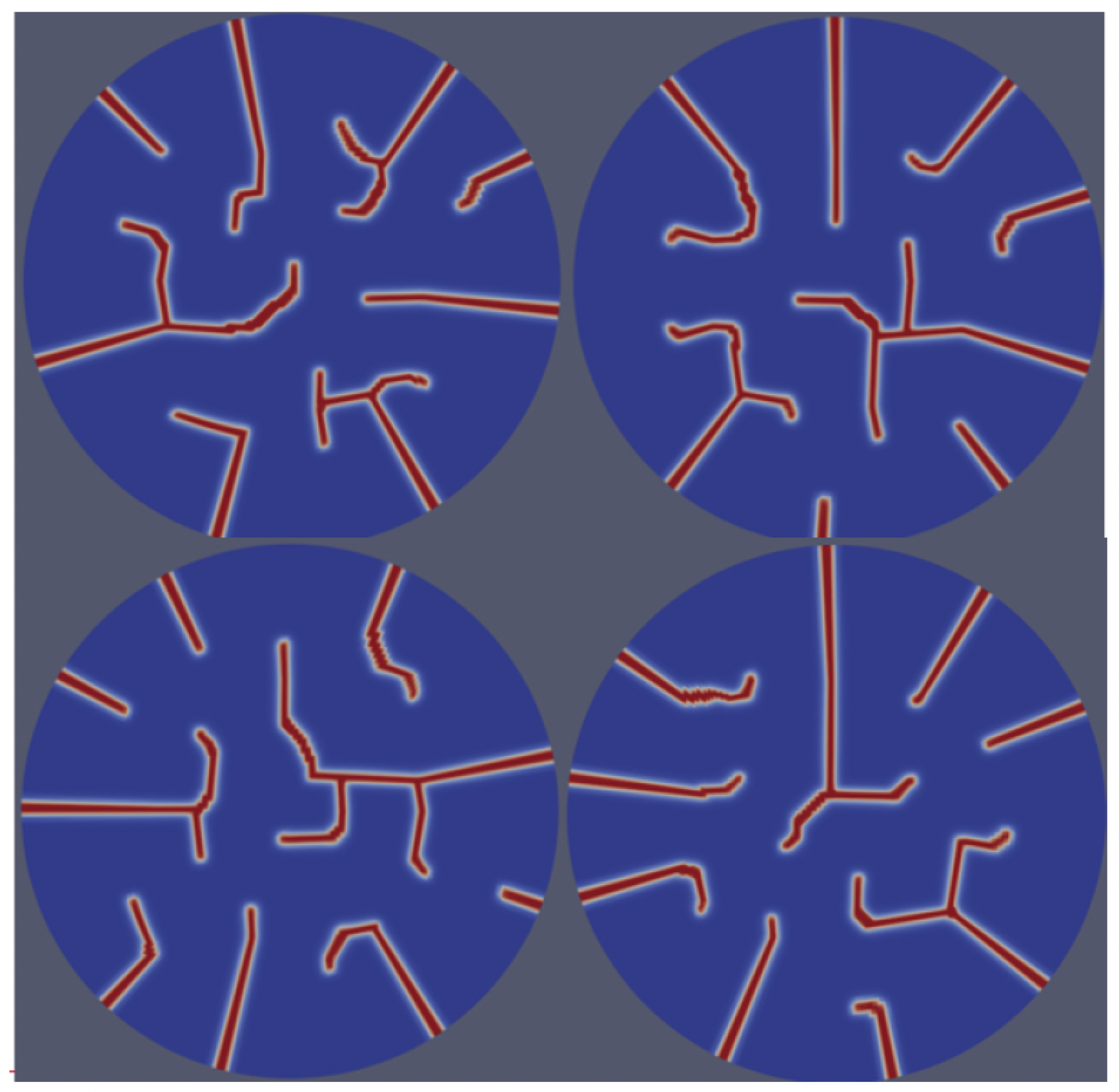
3.3.2. Accounting for the Initial Microstructural Characteristics
3.3.3. Effect of Burnup on Fracture Patterns
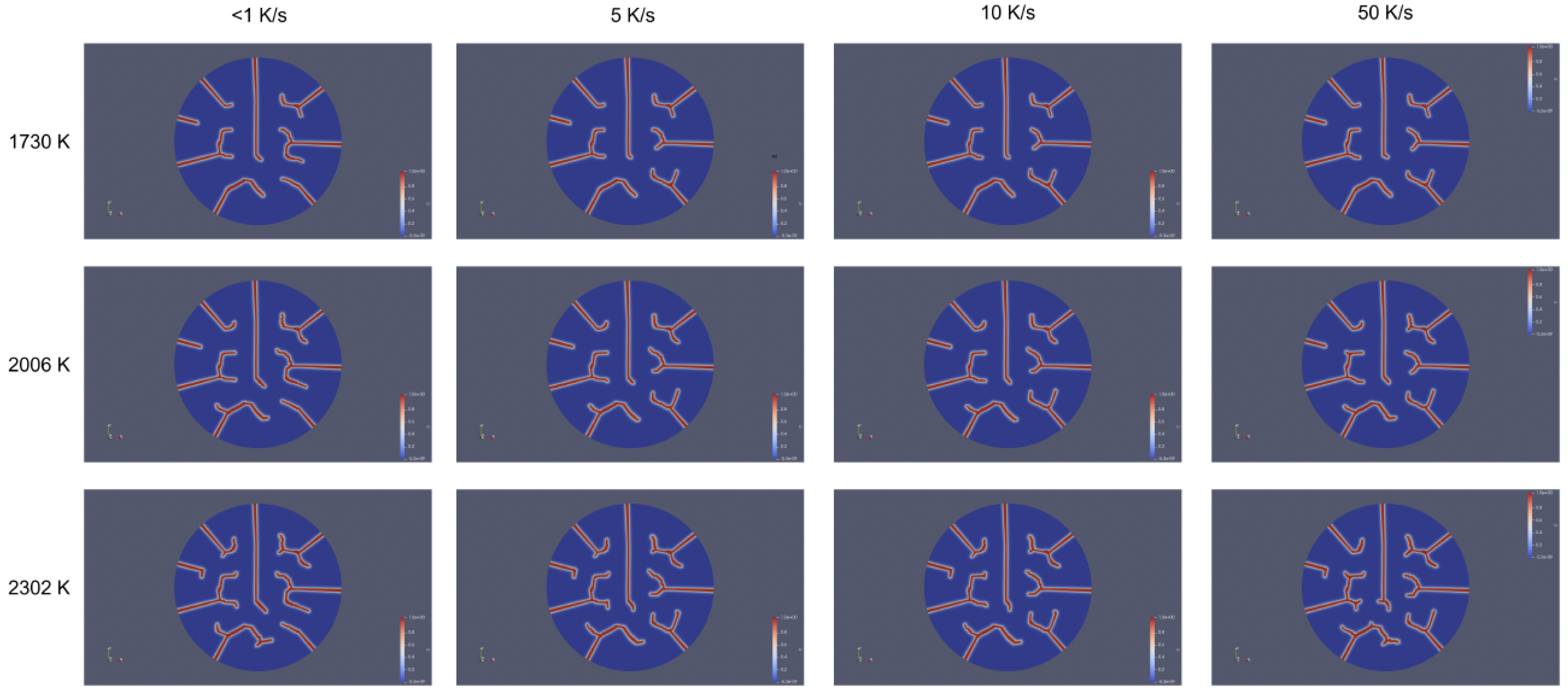
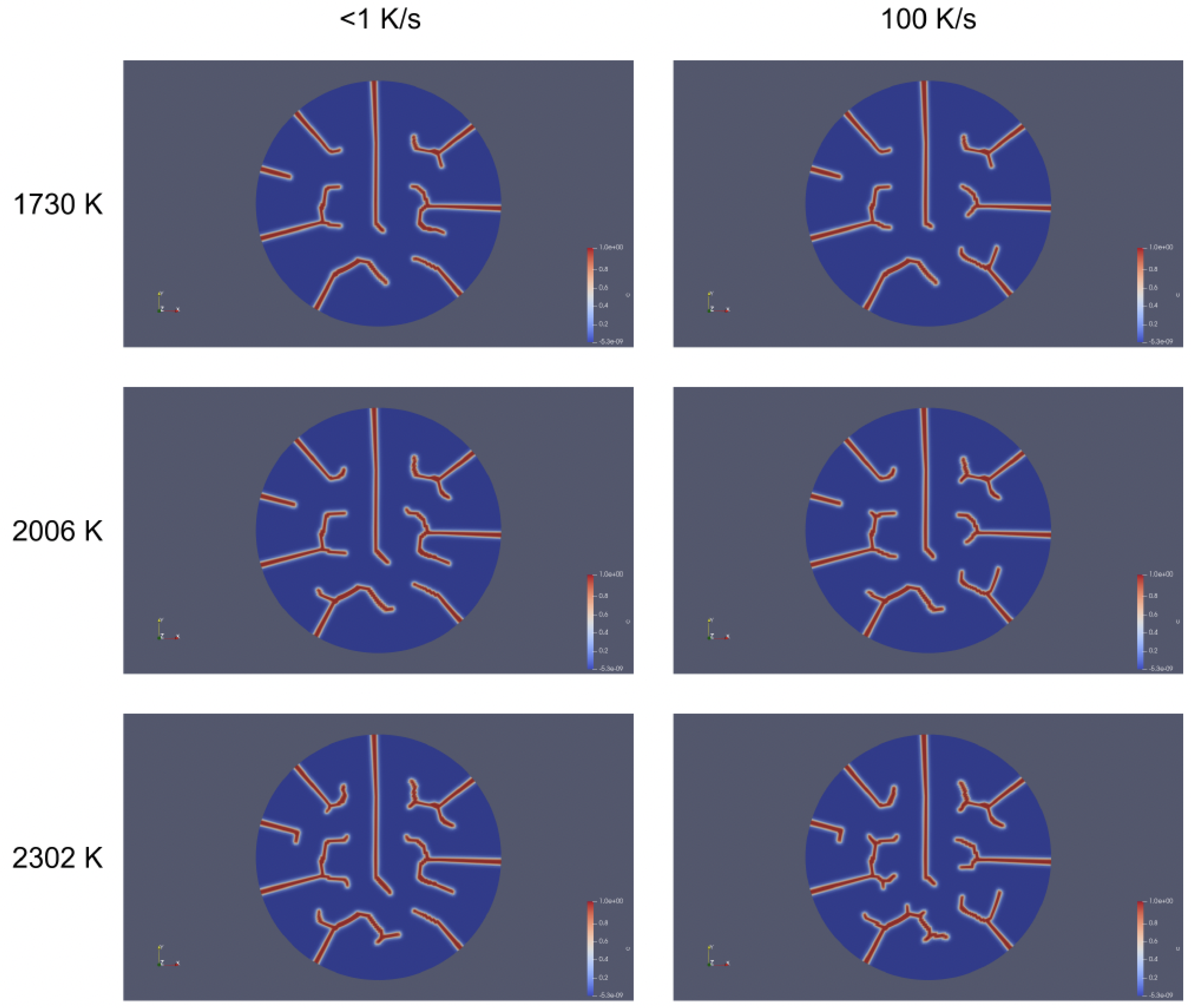
3.3.4. Crack Propagation Patterns in a UO2 Fuel Pellet at Different Heating Rates and Temperatures
4. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Geelhood, K. Fuel Performance Considerations and Data Needs for Burnup Above 62 GWd/MTU, PNNL-29368; Pacific Northwest National Laboratory: Richland, WA, USA, 2019. [Google Scholar]
- Hastings, I.; Smith, A.; Fehrenbach, P.; Carter, T. Fission gas release from power-ramped UO2 fuel. J. Nucl. Mater. 1986, 139, 106–112. [Google Scholar] [CrossRef]
- Bleiberg, M.; Berman, R.; Lustman, B. Effects of High Burn-Up on Oxide Ceramic Fuels; Effets de Taux de Combustion Eleves sur les Oxydes CeramiquesCombustibles; Efectos de un Elevado Grado de Combustión Sobre los Combustibles a Base de óxidos Cerámicos; IAEA International Atomic Energy Agency (IAEA): Vienna, Austria, 1963. [Google Scholar]
- Lassmann, K.; Walker, C.; Van De Laar, J.; Lindström, F. Modelling the high burnup UO2 structure in LWR fuel. J. Nucl. Mater. 1995, 226, 1–8. [Google Scholar] [CrossRef]
- Une, K.; Nogita, K.; Kashibe, S.; Imamura, M. Microstructural change and its influence on fission gas release in high burnup UO2 fuel. J. Nucl. Mater. 1992, 188, 65–72. [Google Scholar] [CrossRef]
- Nogita, K.; Une, K. Radiation-induced microstructural change in high burnup UO2 fuel pellets. In Nuclear Instruments and Methods in Physics Research Section B: Beam Interactions with Materials and Atoms; Elsevier: Amsterdam, The Netherlands, 1994; Volume 91, pp. 301–306. [Google Scholar] [CrossRef]
- Matzke, H.; Spino, J. Formation of the rim structure in high burnup fuel. J. Nucl. Mater. 1997, 248, 170–179. [Google Scholar] [CrossRef]
- Ray, I.; Matzke, H.; Thiele, H.; Kinoshita, M. An electron microscopy study of the RIM structure of a UO2 fuel with a high burnup of 7.9% FIMA. J. Nucl. Mater. 1997, 245, 115–123. [Google Scholar] [CrossRef]
- Thomas, L.E.; Beyer, C.E.; Chariot, L.A. Microstructural analysis of LWR spent fuels at high burnup. J. Nucl. Mater. 1992, 188, 80–89. [Google Scholar] [CrossRef]
- Barner, J.O.; Cunningham, M.E.; Freshley, M.D.; Lanning, D.D. High Burnup Effects Program; Technical Report; U.S. Department of Energy: Washington, DC, USA, 1990. [Google Scholar] [CrossRef]
- Raynaud, P. NUREG-2121: Fuel Fragmentation, Relocation, and Dispersal During the Loss-of-Coolant Accident; US Nuclear Regulatory Commission: Washington, DC, USA, 2012. [Google Scholar]
- NEA. Report on Fuel Fragmentation, Relocation and Dispersal (FFRD); OECD Publishing: Washington, DC, USA, 2016. [Google Scholar]
- Capps, N.; Jensen, C.; Cappia, F.; Harp, J.; Terrani, K.; Woolstenhulme, N.; Wachs, D. A Critical Review of High Burnup Fuel Fragmentation, Relocation, and Dispersal under Loss-of-Coolant Accident Conditions. J. Nucl. Mater. 2021, 546, 152750. [Google Scholar] [CrossRef]
- Bagger, C.; Mogensen, M.; Walker, C. Temperature measurements in high burnup UO2 nuclear fuel: Implications for thermal conductivity, grain growth and gas release. J. Nucl. Mater. 1994, 211, 11–29. [Google Scholar] [CrossRef]
- Nakamura, J.; Uchida, M.; Uetsuka, H.; Furuta, T. Thermal Diffusivity of High Burnup UO2 Pellet; Technical Report 1011-4289; International Atomic Energy Agency (IAEA): Vienna, Austria, 1998; IAEA-TECDOC–1036. [Google Scholar]
- International Atomic Energy Agency. Advances in Fuel Pellet Technology for Improved Performance at High Burnup Proceedings of a Technical Committee Meeting; Technical Report 1011-4289; International Atomic Energy Agency (IAEA): Vienna, Austria, 1998; IAEA-TECDOC–1036. [Google Scholar]
- Turnbull, J.A.; Yagnik, S.K.; Hirai, M.; Staicu, D.M.; Walker, C.T. An Assessment of the Fuel Pulverization Threshold During LOCA-Type Temperature Transients. Nucl. Sci. Eng. 2015, 179, 477–485. [Google Scholar] [CrossRef]
- Noirot, J.; Zacharie-Aubrun, I.; Blay, T. Focused ion beam–scanning electron microscope examination of high burn-up UO2 in the center of a pellet. Nucl. Eng. Technol. 2018, 50, 259–267. [Google Scholar] [CrossRef]
- Gerczak, T.J.; Parish, C.M.; Edmondson, P.D.; Baldwin, C.A.; Terrani, K.A. Restructuring in high burnup UO2 studied using modern electron microscopy. J. Nucl. Mater. 2018, 509, 245–259. [Google Scholar] [CrossRef]
- McKinney, C.; Seibert, R.; Werden, J.; Parish, C.; Gerczak, T.; Harp, J.; Capps, N. Characterization of the radial microstructural evolution in LWR UO2 using electron backscatter diffraction. J. Nucl. Mater. 2023, 585, 154605. [Google Scholar] [CrossRef]
- McClenny, L.D.; Butt, M.I.; Abdoelatef, M.G.; Pate, M.J.; Yee, K.L.; Harikrishnan, R.; Perez-Nunez, D.; Jiang, W.; Ortega, L.H.; McDeavitt, S.M.; et al. Experimentally validated multiphysics modeling of fracture induced by thermal shocks in sintered UO2 pellets. J. Nucl. Mater. 2022, 565, 153719. [Google Scholar] [CrossRef]
- Li, W.; Shirvan, K. Multiphysics phase-field modeling of quasi-static cracking in urania ceramic nuclear fuel. Ceram. Int. 2021, 47, 793–810. [Google Scholar] [CrossRef]
- Allen, S.M.; Cahn, J.W. A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening. Acta Metall. 1979, 27, 1085–1095. [Google Scholar] [CrossRef]
- Levitas, V.I.; Jafarzadeh, H.; Farrahi, G.H.; Javanbakht, M. Thermodynamically consistent and scale-dependent phase field approach for crack propagation allowing for surface stresses. Int. J. Plast. 2018, 111, 1–35. [Google Scholar] [CrossRef]
- Levitas, V.I.; Preston, D.L. Three-dimensional Landau theory for multivariant stress-induced martensitic phase transformations. I. Austenite ↔ martensite. Phys. Rev. B 2002, 66, 134206. [Google Scholar] [CrossRef]
- Griffith, A.A.; Taylor, G.I. VI. The phenomena of rupture and flow in solids. Philosophical Transactions of the Royal Society of London. Series A, Containing Papers of a Mathematical or Physical Character. Royal Soc. 1921, 221, 163–198. [Google Scholar] [CrossRef]
- Henry, R.; Zacharie-Aubrun, I.; Blay, T.; Chalal, S.; Gatt, J.M.; Langlois, C.; Meille, S. Fracture properties of an irradiated PWR UO2 fuel evaluated by micro-cantilever bending tests. J. Nucl. Mater. 2020, 538, 152209. [Google Scholar] [CrossRef]
- Wu, J.Y.; Nguyen, V.P.; Nguyen, C.T.; Sutula, D.; Sinaie, S.; Bordas, S.P. Phase-field modeling of fracture. In Advances in Applied Mechanics; Elsevier: Amsterdam, The Netherlands, 2020; Volume 53, pp. 1–183. [Google Scholar] [CrossRef]
- OECD; Nuclear Energy Agency. Thermal Performance of High Burn-Up LWR Fuel: Seminar Proceedings, Cadarache, France, 3–6 March 1998; OECD: Washington, DC, USA, 1999. [Google Scholar] [CrossRef]
- Staicu, D.; Rondinella, V.; Walker, C.; Papaioannou, D.; Konings, R.; Ronchi, C.; Sheindlin, M.; Sasahara, A.; Sonoda, T.; Kinoshita, M. Effect of burn-up on the thermal conductivity of uranium–gadolinium dioxide up to 100 GWd/tHM. J. Nucl. Mater. 2014, 453, 259–268. [Google Scholar] [CrossRef]
- Gaston, D.; Newman, C.; Hansen, G.; Lebrun-Grandié, D. MOOSE: A parallel computational framework for coupled systems of nonlinear equations. Nucl. Eng. Des. 2009, 239, 1768–1778. [Google Scholar] [CrossRef]
- Wachtman, J.B.; Wheat, M.L.; Anderson, H.J.; Bates, J.L. Elastic constants of single crystal UO2 at 25 °C. J. Nucl. Mater. 1965, 16, 39–41. [Google Scholar] [CrossRef]
- Kavazauri, R.; Pokrovskiy, S.A.; Baranov, V.G.; Tenishev, A.V. Thermal properties of nonstoichiometry uranium dioxide. IOP Conf. Ser. Mater. Sci. Eng. 2016, 130, 012025. [Google Scholar] [CrossRef]
- Fink, J.K.; Chasanov, M.G.; Leibowitz, L. Thermophysical properties of uranium dioxide. J. Nucl. Mater. 1981, 102, 17–25. [Google Scholar] [CrossRef]
- Oguma, M. Microstructure Effects on Fracture Strength of U02 Fuel Pellets. J. Nucl. Sci. Technol. 1982, 19, 1005–1014. [Google Scholar] [CrossRef]
- Williamson, R.; Knoll, D. Simulating Dynamic Fracture in Oxide Fuel Pellets Using Cohesive Zone Models; IASMiRT: Raleigh, NC, USA, 2009. [Google Scholar]
- Oguma, M. Cracking and relocation behavior of nuclear fuel pellets during rise to power. Nucl. Eng. Des. 1983, 76, 35–45. [Google Scholar] [CrossRef]
- Motta, A.; Olander, D.; Wirth, B. Light Water Reactor Materials; American Nuclear Society Scientific Publications; American Nuclear Society: Westmont, IL, USA, 2017. [Google Scholar]
- Gamble, K.; Knight, T.; Roberts, E.; Hales, J.; Spencer, B. Mechanistic verification of empirical UO2 fuel fracture models. J. Nucl. Mater. 2021, 556, 153163. [Google Scholar] [CrossRef]
- Onofri, C.; Sabathier, C.; Baumier, C.; Bachelet, C.; Drouan, D.; Gérardin, M.; Legros, M. Extended defect change in UO2 during in situ TEM annealing. Acta Mater. 2020, 196, 240–251. [Google Scholar] [CrossRef]
- Radford, K. Effect of fabrication parameters and microstructure on the mechanical strength of UO2 fuel pellets. J. Nucl. Mater. 1979, 84, 222–236. [Google Scholar] [CrossRef]
- Doitrand, A.; Henry, R.; Zacharie-Aubrun, I.; Gatt, J.M.; Meille, S. UO2 micron scale specimen fracture: Parameter identification and influence of porosities. Theor. Appl. Fract. Mech. 2020, 108, 102665. [Google Scholar] [CrossRef]
- Karb, E.H.; Prüßmann, M.; Sepold, L.; Hofmann, P.; Schanz, G. LWR Fuel Rod Behaviorin the FR2 Ln-Pile Tests Simulating the Heatup Phase of a LOCA; IAEA: Vienna, Austria, 1983. [Google Scholar]
- Oguma, M. Integrity degradation of UO2 pellets subjected to thermal shock. J. Nucl. Mater. 1985, 127, 67–76. [Google Scholar] [CrossRef]



| Parameter | Value |
|---|---|
| 0.1148 | |
| 0.0035 | |
| 0.0002474 | |
| C | 0.0132 |
| D | 0.00188 |
| Property | Symbol | Value | Reference |
|---|---|---|---|
| Fracture Stress | 200 MPa | [27,35] | |
| Elastic Modulus | E | 200 GPa | [32] |
| Poisson’s Ratio | 0.33 | [32] | |
| Thermal Conductivity | k | Halden (Equation (27)) | [29] |
| Density | 10,970 | [33] | |
| Specific Heat | 480 | [34] | |
| Thermal Expansion Coefficient | [21] |
| Parameter | Symbol | Value | Reference |
|---|---|---|---|
| Cohesion Contribution | A | 6 × Pa | This work |
| Double-Well Contribution | B | 4 × Pa | This work |
| 0.00045 J/m | This work | ||
| Surface Energy | 1 | [27] | |
| Length Scale | l | 0.3 mm | This work |
| Crack Mobility | L | 1 | This work |
| Applied Stress (MPa) | Strain | Strain Energy (Pa) | Crack Growth (Yes/No) |
|---|---|---|---|
| 200 | 0.001 | 100,000 | Yes |
| 100 | 0.0005 | 25,000 | Yes |
| 90 | 0.00045 | 20,250 | Yes |
| 80 | 0.0004 | 16,000 | Yes |
| 70 | 0.00035 | 12,250 | Yes |
| 67 | 0.000335 | 11,222.5 | Yes |
| 65 | 0.000325 | 10,562.5 | No |
| 62 | 0.00031 | 9610 | No |
| 60 | 0.0003 | 9000 | No |
| 50 | 0.00025 | 6250 | No |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gencturk, M.; Faulkner, N.; Ahmed, K. Thermo-Mechanical Phase-Field Modeling of Fracture in High-Burnup UO2 Fuels Under Transient Conditions. Materials 2025, 18, 1162. https://doi.org/10.3390/ma18051162
Gencturk M, Faulkner N, Ahmed K. Thermo-Mechanical Phase-Field Modeling of Fracture in High-Burnup UO2 Fuels Under Transient Conditions. Materials. 2025; 18(5):1162. https://doi.org/10.3390/ma18051162
Chicago/Turabian StyleGencturk, Merve, Nicholas Faulkner, and Karim Ahmed. 2025. "Thermo-Mechanical Phase-Field Modeling of Fracture in High-Burnup UO2 Fuels Under Transient Conditions" Materials 18, no. 5: 1162. https://doi.org/10.3390/ma18051162
APA StyleGencturk, M., Faulkner, N., & Ahmed, K. (2025). Thermo-Mechanical Phase-Field Modeling of Fracture in High-Burnup UO2 Fuels Under Transient Conditions. Materials, 18(5), 1162. https://doi.org/10.3390/ma18051162






