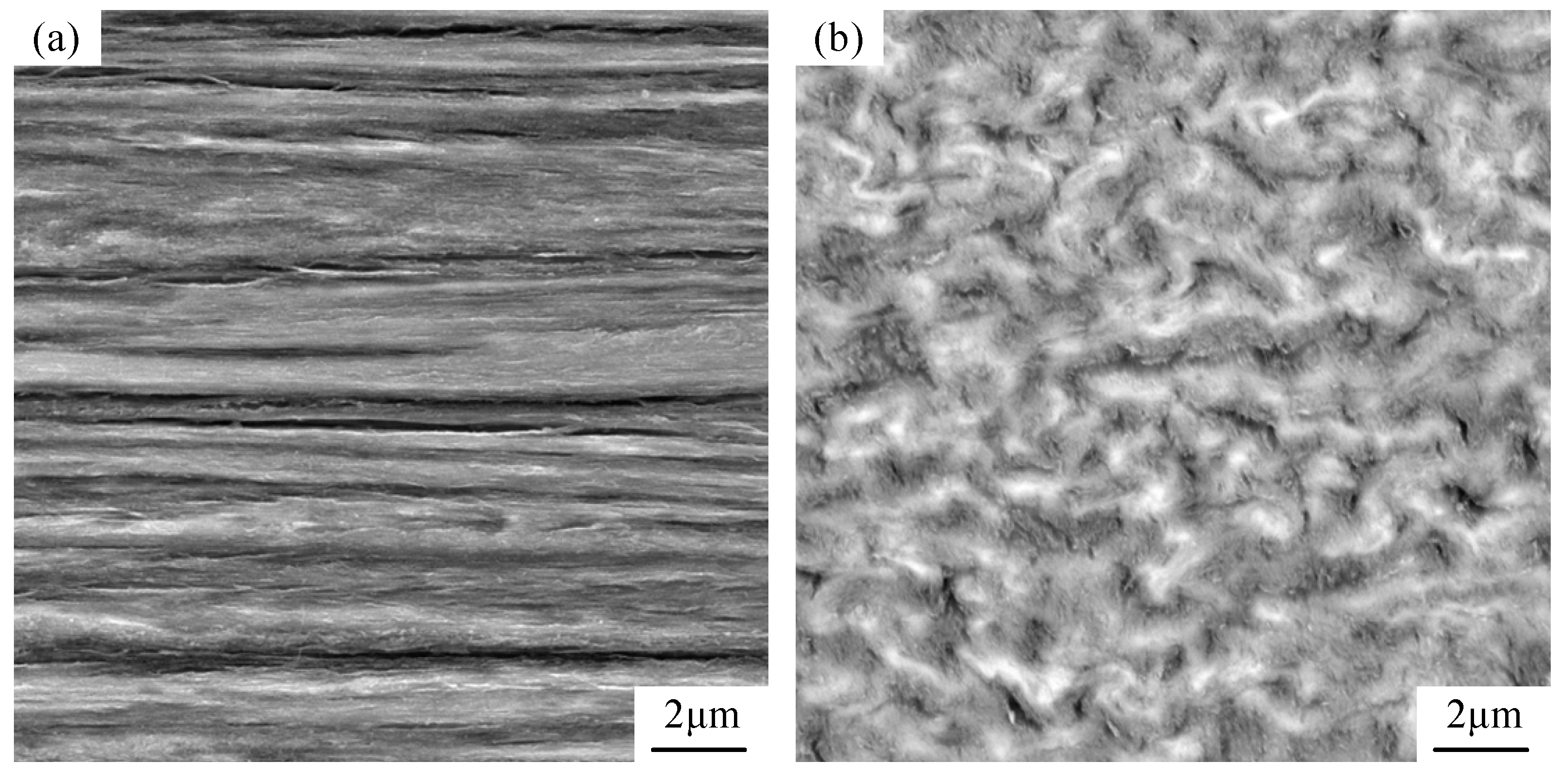
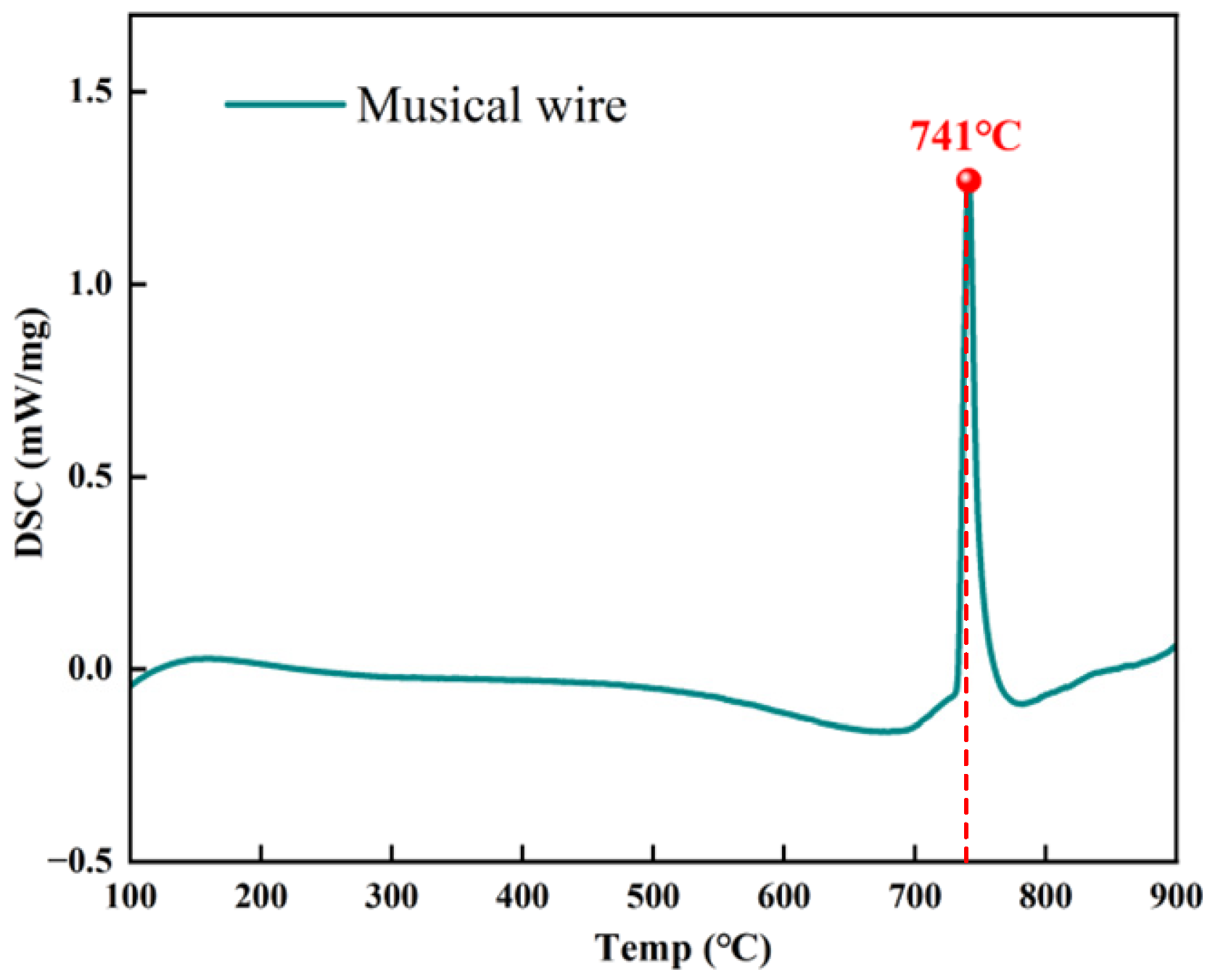
3.1. Microstructure Analysis of Music Wire After Low-Temperature Annealing
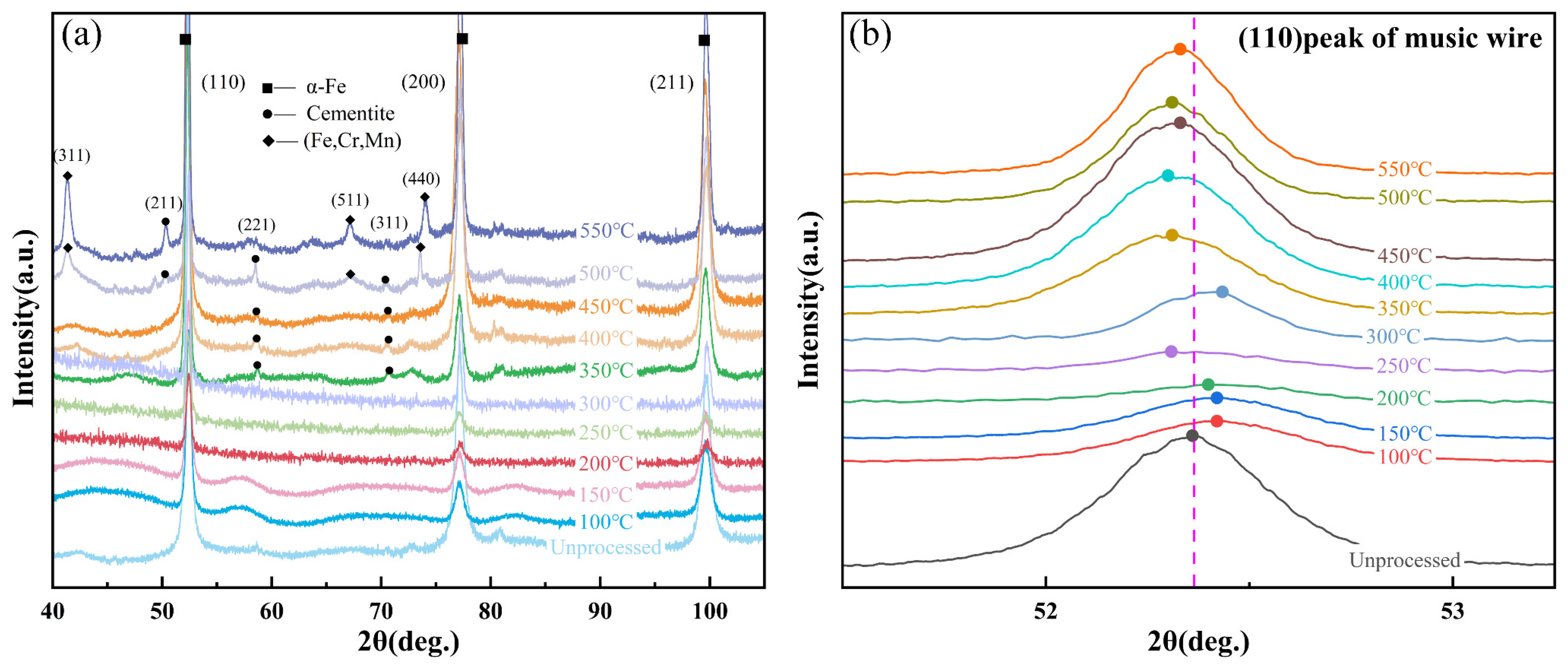
Figure 6 shows the XRD patterns of music steel wires and various post-annealing treatments. The calibration of the indices for ferrite is based on card number PDF#06-0696, while that for cementite is based on card number PDF#34-0001.
Figure 6a depicts the XRD patterns of music steel wire in various annealed states, whereas
Figure 6b illustrates the ferrite (110) crystal diffraction peaks of the wire in various annealed states.
As shown in
Figure 6a, at temperatures below 300 °C, the annealed music steel wire does not exhibit the diffuse cementite phase. Instead, C atoms are dissolved in the ferrite. As the annealing temperature increases, cementite is gradually precipitated. At 400 °C, precipitation of the Cr and Mn phases occurs. The diffraction peaks of the ferrite (110) appeared shifted, broadened, and significantly weakened, which is due to the nanostructures and residual internal stresses caused by the large deformation of the music steel wire after cold drawing, so that the internal ferrite lamella refinement and dislocation density of the music steel wire increased, and the occurrence of cementite lamella deformation and decomposition and the formation of carbide nanocrystalline particles and part of the amorphous cementite, which is reflected in the music steel wire having a higher tensile strength [
22].
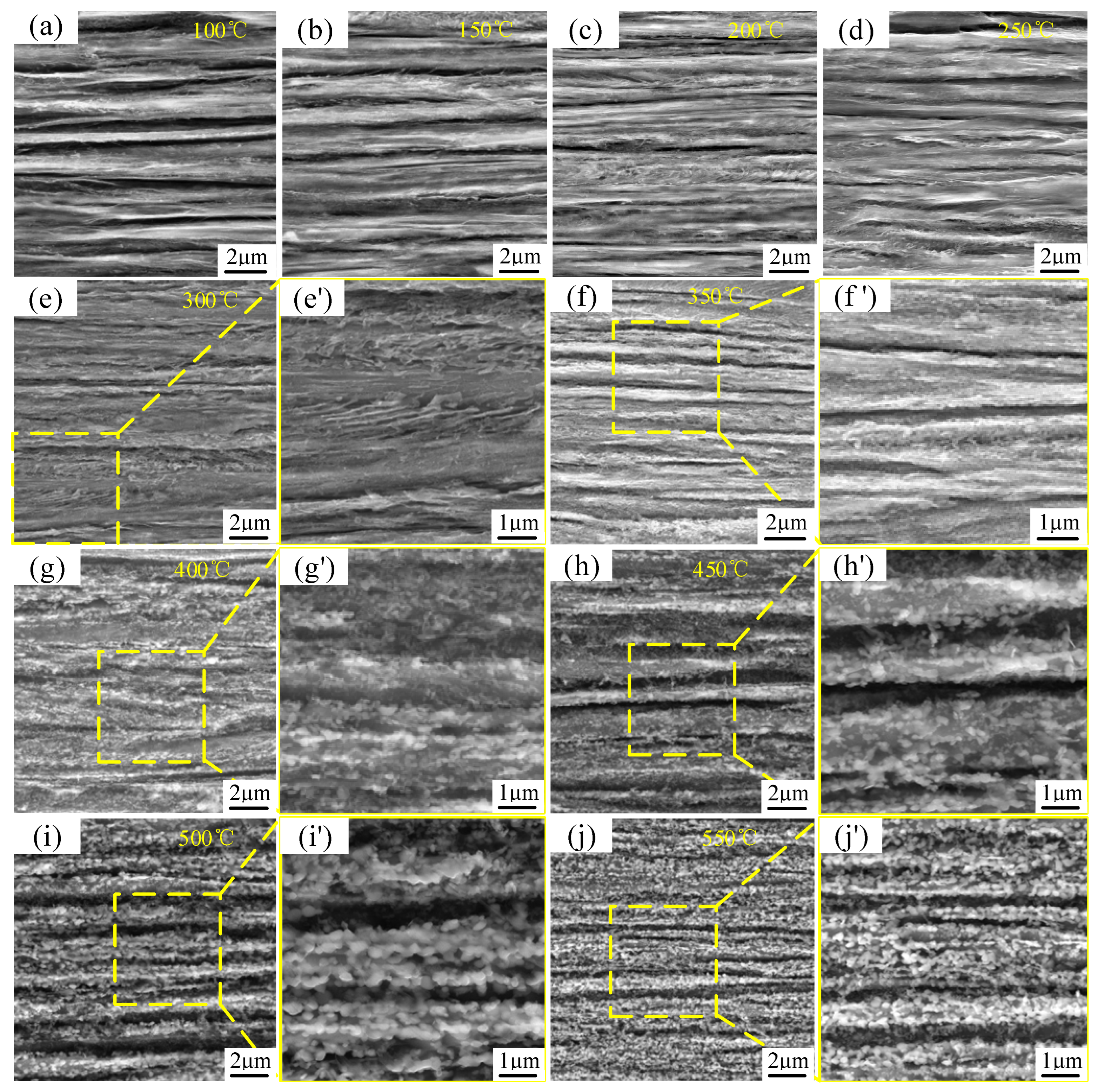
Figure 7 presents the SEM images of music steel wire magnified at 20,000× and localized SEM images at 50,000× magnification under varying annealing conditions. The longitudinal microstructure morphology of music steel wire was observed under low-temperature annealing at a conventional temperature for 30 min. It can be observed that, with the increase in annealing temperature, the internal microstructure of music steel wire is gradually spheroidized after annealing at 350 °C, and the internal microstructure of the steel wire is significantly spheroidized when it is annealed at 550 °C.
As shown in
Figure 7, the microstructure does not differ much from the original cold-drawn condition after annealing at 150 °C for holding 30 min. Although fragmentation of the cementite flakes can be observed, the lamellar structure is still dominant in the large-strain music steel wire after annealing at 250 °C for holding 30 min (
Figure 7d), and the lamellar structure remains stable, there is no spheroidization of the cementite and no recrystallization. A small amount of detached cementite was observed in the annealed wire at 350 °C (
Figure 7f). The internal microstructure of the music steel wire is still clearly lamellar-oriented before annealing below 400 °C (
Figure 7g) and, after heat treatment at 400 °C for 30 min, substantial changes in the microstructure are observed, accompanied by a notable reduction in lamellar spacing structure of the original lamellar pearlitic phase. In addition, the cementite flakes undergo spheroidization, mainly located in the interlayer spacing junctions. The internal lamellar structure of the music steel wire after annealing from 450 °C (
Figure 7h) is still visible in the axial direction, while cell/sub-boundaries were formed in the ferrite, and the cementite flakes started to spheroidize, with the appearance of small-diameter spheroidized carbides. Since the <110> fiber textile structure formed during wire drawing limits further sliding in each lamella to ordinary strain, the compatibility of adjacent grains can only be maintained by bending of the grains toward each other [
23], so that the cementite lamellae curl and fragment into numerous short segments or even round grains. This also proves that cementite flakes are capable of plastic deformation and even strain-induced fragmentation [
24]. Meanwhile, according to Y.J. Li [
25], it was shown that atom redistribution was not observed after annealing at 250 °C for 30 min, and during annealing at 450 °C (
Figure 7h), due to the uncertainty of the existence of layered cementite before annealing, there may be interactions between carbon atoms and dislocations, causing carbon atoms dissolved in ferrite to migrate to the pristine carbide. This migration is driven by a reduction in the ferrite/cementite phase boundary driven by a reduction in the ferrite/cementite phase boundary area, resulting in the formation of amorphous cementite crystallization generation and spheroidization of the cementite [
2,
26]. With increasing temperature, the spheroidization process becomes more pronounced and reaches its maximum at 550 °C (
Figure 7j), where the internal microstructure is completely transformed into a distinct spheroidal cementite phase, coexisting with the formation of highly deformed ferrite flakes which recrystallize and agglomerate into larger ferrite grains, with a blurred and almost invisible/cementite phase boundary.
3.2. Microstructure Analysis of Music Wire After High-Temperature Annealing
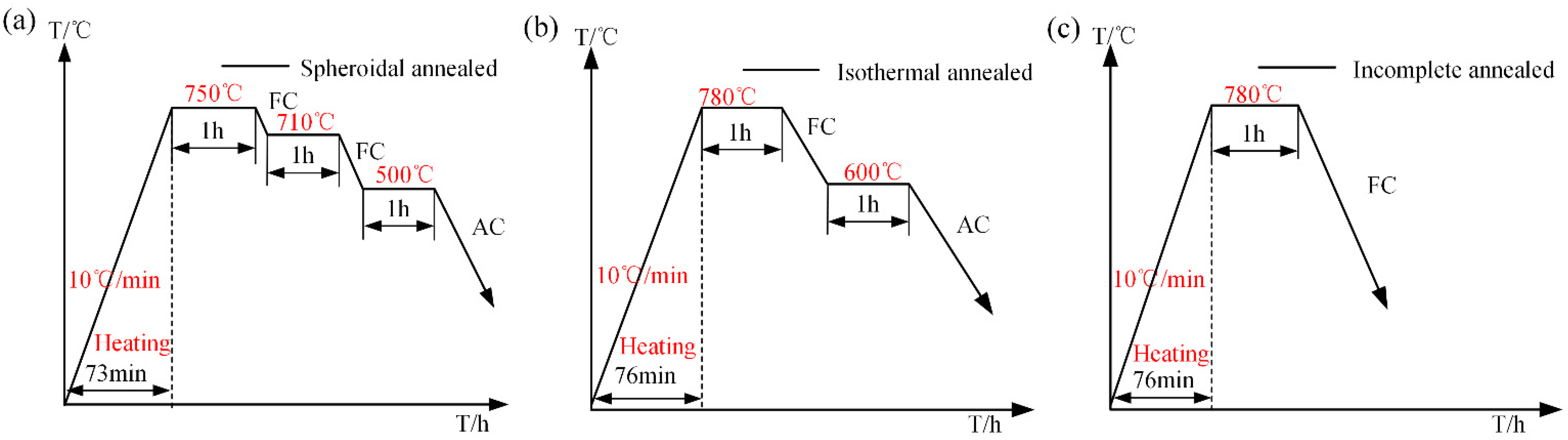
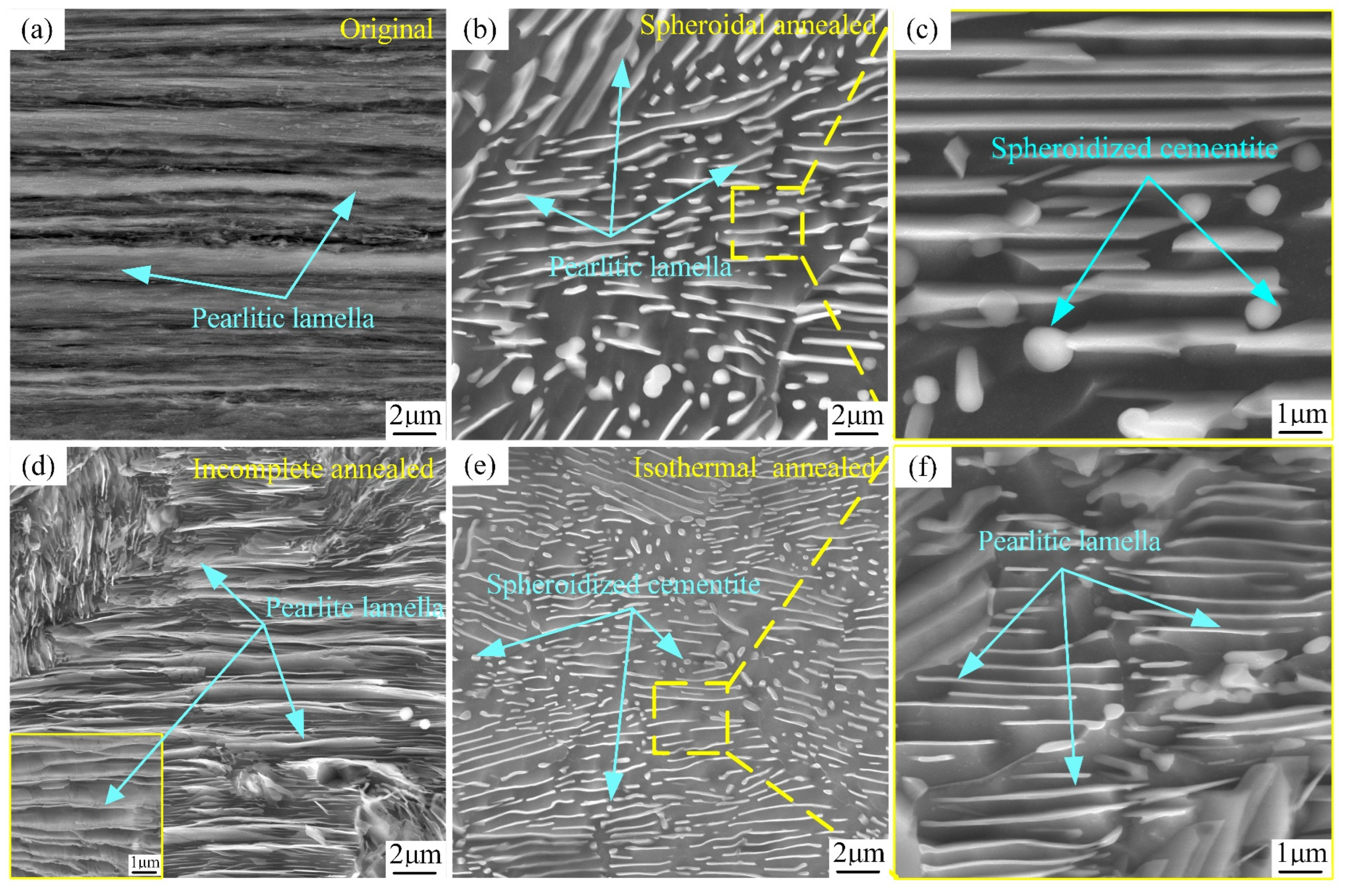
Figure 8 depicts the SEM microstructure of SWP-B music steel wire at 20,000× magnification following a high-temperature annealing treatment. The treatment processes of spheroidization annealing (
Figure 8b,c), isothermal annealing (
Figure 8e,f), and incomplete annealing (
Figure 8d) were employed for temperatures exceeding the phase transition point of music steel wire, respectively.
As illustrated in
Figure 8a, the microstructure of the music steel wire under 20 k magnification exhibits distinct axial lamellar pearlite spacing, as evidenced by the clear separation of the lamellae.
Figure 8b,c depicts the formation of multiple pearlite clusters with varying orientations resulting from the decomposition of eutectic austenite after reaching the spheroidization and annealing temperatures.
Figure 8c presents a magnified view of the interior of the clusters. It can be observed that the ferrite lamellae and cementite lamellae are randomly oriented, and the crystal orientations of the ferrite exhibit no strong tendency towards continuity or discontinuity. These are surrounded by minute particles of cementite [
27].
Figure 8d illustrates the formation of multiple pearlite clusters with varying orientations, derived from eutectic austenite decomposition following the attainment of an incomplete annealing temperature in the music steel wire. The pearlite lamella spacing within these clusters is considerable, exhibiting no spheroidization.
Figure 8e depicts the formation of multiple orientations of pearlite clusters in the music steel wire upon reaching the isothermal annealing temperature through eutectic austenite decomposition. The clusters of internal cementite plates have undergone a transformation, assuming the form of either short rods or spherical particles. The influence of cementite on the deformation of music steel wires is considered to be anisotropic due to the axially aligned laminar structure of the wire [
28]. The cementite plate is regarded as a barrier that prevents dislocations from passing through the ferrite, resulting in the deformation direction along the carbide plate being designated as the “soft” orientation of the plastic deformation [
29,
30]. Conversely, when stress is applied at an angle to the cementite plate, the plate is prone to fracturing, which in turn gives rise to the formation of high-density dislocation entanglement in the ferrite region in close proximity to the fractured cementite. This phenomenon gives rise to the bending of high-density dislocations within the ferrite. As a result, the internal microstructure of the music steel wire, following spheroidization annealing and isothermal annealing of the steel wire, displays a distinctive cementite and ferrite plate-bending phenomenon.
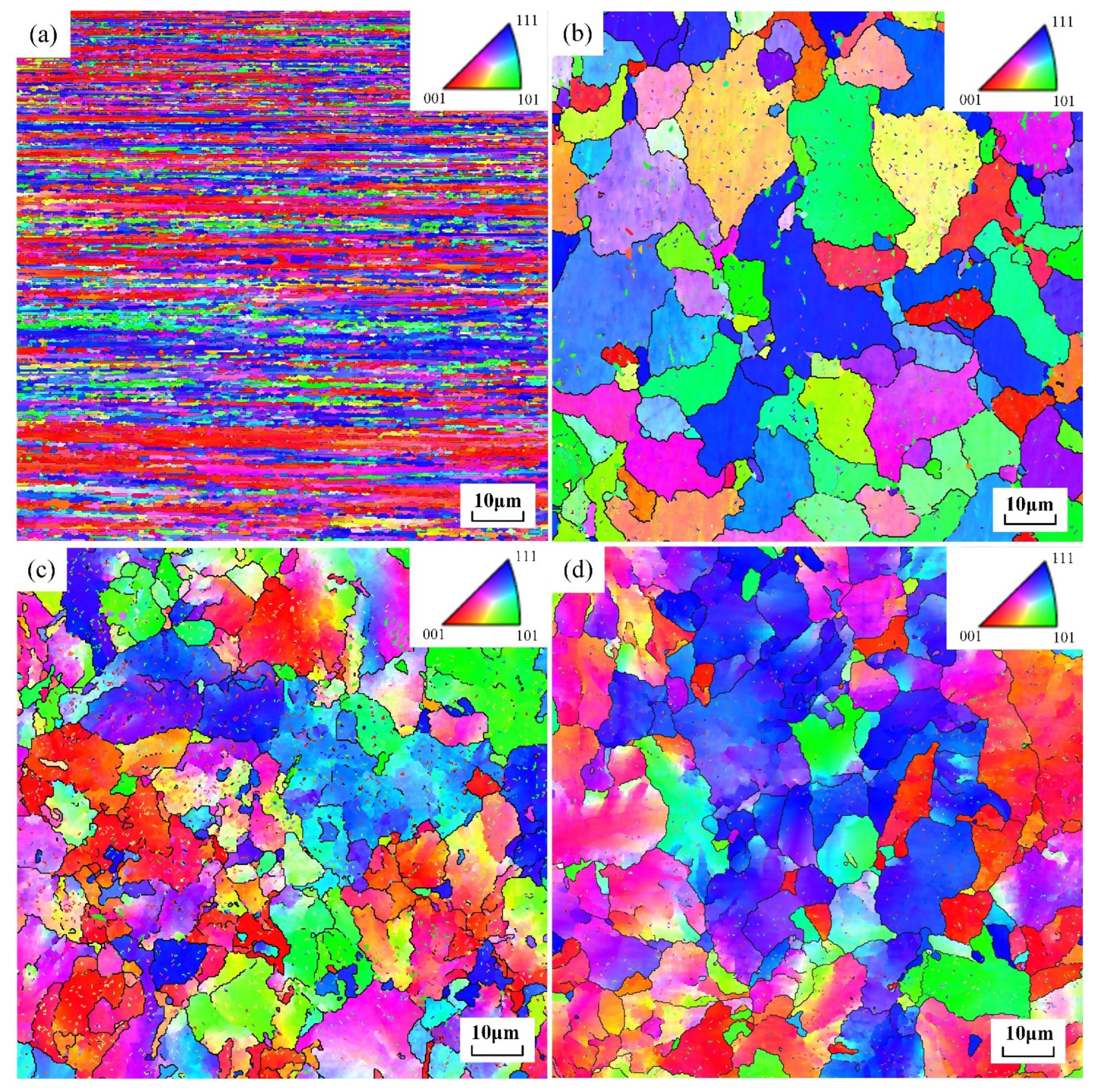
As illustrated in
Figure 9a, the original music steel wire exhibits a fiber texture that is nearly parallel to the axial direction, with fine grains displaying an anisotropic structure. Following three distinct high-temperature annealing treatments, the longitudinal cross-section IPF images of the music steel wire, as shown in
Figure 9b–d, reveal that, upon annealing of the music steel wire above the phase transition point, there is a notable shift in the internal organization of the material, specifically in the tensile direction of the parallel textile structure segmentation, which generates large-angle grain boundaries and multicrystalline grains. These observations suggest a tendency towards isotropy in each of these structures. Moreover, the recrystallized grains, which diverge from the original organization, exhibit pearlitic lamellar spacing and a drawing direction parallel to the anisotropic state. The latter display a random distribution of pearlitic crystallographic orientation, as the grains continue to grow, resulting in the formation of a fine pearlitic lamellar spacing structure within the grains, as illustrated in
Figure 8b–e. The grain size is more uniform following spheroidization annealing. Additionally, a considerable number of minute grain boundaries emerged within the metal wire following isothermal annealing. Furthermore, a combination of minute grain boundaries and larger grains was observed within the steel wire after incomplete annealing. As seen in
Figure 10, the content of the cementite phase during spheroidization annealing is the highest under different annealing methods and then decreases continuously, followed by an increase in the content of other precipitated phases.
Figure 11a–d,a′–d′ illustrate the PF plots of the ferrite phase and cementite phases of the original music steel wire, as well as the spheroidal annealing, isothermal annealing, and incomplete annealing treatments. The distribution of the phases can be clearly observed from the PF phase distribution diagrams. Additionally, the evolution of the internal microstructure of the music steel wire deformation texture under different annealing treatment levels is depicted by the polar diagrams of the ferrite and cementite phases.
The strengths of the ferrite <110> fiber texture of the unannealed music steel wire and the spheroidally annealed, isothermally annealed, and incompletely annealed music steel wires are quantified and presented in
Figure 11a–d.
Figure 11a illustrates that the strength of the ferrite <110> fiber texture of the original music steel wire is 9.56, which corresponds to a markedly robust strength arising from the situation. As the annealing temperature increases, the axial pearlitic lamella spacing inside the steel wire is destroyed, the ferrite phase is decomposed, and the strength of the <110> fiber texture is significantly reduced. This reduction in strength is observed until the incomplete annealing treatment, at which point the strength of the ferrite phase <110> fiber texture decreases to 2.62.
Figure 11a′–d′ demonstrate that the formation of spheroidized cementite results in an enhancement of the strength of the <110> phase of cementite. The isothermally annealed steel wire microstructure exhibits the highest strength of the cementite <010> phase, reaching 40.04, which can be attributed to its highest spheroidization rate after isothermal treatment.
Figure 12a–h illustrate the pearlite clusters of unannealed, spheroidally annealed, isothermally annealed, and incompletely annealed steel wires. Given that the music steel wire is a high-carbon steel wire, it follows that the mechanical properties of the high-carbon steel wire and the pearlitic lamellar spacing are inextricably linked. Pearlite is a mixture of ferrite and cementite, and the pearlite organization offers the benefits of high strength and good plasticity [
31]. The pearlitic rate serves as a crucial metric for assessing the organization of the steel wire. To ensure accurate measurement of the pearlitic lamellar spacing of the steel wires following different annealing treatments, four groups of pearlitic clusters were selected from each of the four groups visible in the 20k times scanning electron microscope images. The intercept method was employed to ascertain the pearlitic lamella spacing (ILS) [
32], with the red line representing an arbitrary intercept line, as illustrated in
Figure 12a–h. The measured results are presented in
Table 2.
The micrographs obtained via EBSD (
Figure 9) demonstrate that the size of the pearlite clusters remains consistent following both spheroidization annealing and isothermal annealing treatments. This is attributed to the comparable heat treatments administered prior to isothermal transformation (
Figure 4).
Figure 9b–d illustrate that the cluster size diminishes with a reduction in isothermal transformation temperature. The refinement of the pearlite cluster size is typically accompanied by an increase in the number of nucleation sites in the cementite at higher supercooling [
33]. The transformation of music steel wires at lower temperatures is distinguished by a reduction in interlayer spacing, which can be attributed to the increase in nucleation sites during supercooling and a concomitant decrease in the diffusion rate. The interlayer spacing of pearlite in SWP-B music steel wire exhibits the smallest value following isothermal annealing treatment. In comparison to the spheroidization annealing heat treatment process at an isothermal temperature of 710 °C, the isothermal annealing process at an isothermal temperature of 600 °C is relatively low (
Figure 4b), which results in a reduction in the ability of grain boundaries to move, a slowing down of the growth rate of pearlite, and a refinement of the final pearlite lamella spacing in comparison to the spheroidal annealing [
34]. The reduction of pearlitic lamellar spacing results in a slight increase in tensile strength for isothermally annealed music steel wire in comparison to spheroidally annealed wire. This phenomenon can be attributed to the fact when the wire is subjected to an external force, plastic deformation occurs within the ferrite. It is the cementite layer that plays a role in preventing this deformation, and its lamellar spacing represents the maximum slip distance. It can be reasonably deduced that a reduction in lamellar spacing will result in a reduction in slip distance, as well as in ferrite and cementite obstruction. Furthermore, the steel wire will demonstrate an increase in resistance to plastic deformation, which will consequently result in an increase in hardness.
3.3. Mechanical Properties Analysis
Figure 13 illustrates the variation in nanoindentation hardness and tensile strength of SWP-B musical steel wire following different heat treatments. The microstructure of the SWP-B musical steel wire is predominantly lamellar pearlite. The observed decline in hardness can be attributed to the alteration in the structural composition of pearlite and cementite as a consequence of elevated annealing temperatures.
The cementite present in the music steel wire is capable of withstanding the same degree of plastic strain as the ferrite during cold drawing, despite the inherent brittleness of the cementite itself [
27,
35,
36]. No notable reduction in strength was observed following annealing at 150 °C for 30 min (
Figure 13). Instead, a slight increase in strength was observed, reaching a peak of 2.7 GPa after annealing at 200 °C for 30 min. Following annealing and holding at 250 °C for 30 min, a slight decline in strength was observed. However, the level remained at approximately 2.5 GPa, indicating that no significant alterations in the microstructure were apparent. Moreover, it has also been demonstrated that, during annealing at relatively low temperatures (150–300 °C), reprecipitation, nanocrystals, and roughening of severely strained cementite occur [
2,
4]. Accordingly, the prevailing view in the literature is that the formation of cementite nanocrystals after a period of low-temperature annealing at approximately 250 °C results in wire hardening and a catastrophic deterioration of the torsional toughness of the wire [
37,
38]. This is evidenced by the fluctuating indentation hardness of the music steel wire between 150 °C and 300 °C as the annealing temperature increases, followed by a continuous decrease, and significant decomposition of the cementite occurs, while the lamellar structure remains dominant [
39]. At temperatures exceeding 400 °C, the original layered structure is replaced by a supersaturated ferrite substructure comprising grains [
8]. Furthermore, the coarsening of the ferrite substructure grains gradually becomes the dominant structure, exerting control over the strength of the material during subsequent annealing. This is the primary factor responsible for the observed decrease in strength during annealing. While the hardness measurements are predominantly surface-based, the observed decrease in hardness can be attributed to the dissolution of the amorphous mixed cementite structure, which was induced by the large deformation of the drawn music steel wires after annealing at 400 °C for 30 min, resulting in a significant reduction in their surface hardness [
40]. It is noteworthy that the spheroidization of cementite begins to predominate during annealing at 400 °C (
Figure 7). Following annealing at 450 °C for 30 min, the spheroidization of cementite intensifies, and the hardness of the music steel wires appears to decline, reaching 3.37 GPa (approximately 343 HV), which is about half of the hardness of the unannealed music steel wires, which is 6.94 GPa (approximately 707 HV). The internal lamellar structure of the music steel wire is no longer present after annealing at temperatures up to 550 °C, and the hardness also decreases to 1.6 GPa (approximately 163 HV).
Above the phase transition point, the high percentage of spheroidization of the isothermally spheroidized and isothermally annealed cementite, as well as the presence of the pearlitic lamellar spacing structure (
Figure 8b,c), resulted in a slightly higher hardness of the isothermally spheroidized and isothermally annealed microstructure than that of the incompletely annealed microstructure and that of the low-temperature annealed microstructure at 500 °C and 550 °C. In particular, the incompletely annealed microstructure of SWP-B music steel wire exhibits minimal spheroidization (
Figure 8d) and, consequently, the material exhibits the lowest recorded hardness value of 0.68 GPa (69 HV).
Figure 13 also demonstrates the correlation between tensile strength and annealing temperature. The tensile strength of the music steel wire at room temperature is 2578 GPa, which indicates that the wire is of a robust and high-strength construction. Following annealing at 200 °C for 30 min, the tensile strength is maintained with a slight increase to 2702 MPa. Following annealing at 250 °C for 30 min, a slight reduction in tensile strength is observed, although it remains at a high level. For annealing temperatures above this threshold, the relationship between tensile strength and annealing temperature is approximately linear, exhibiting a decrease in tensile strength. In high-temperature annealing above the phase transition point, the isothermal annealing microstructure and spheroidal annealing microstructure form large-angle grain boundaries, and their internal cementite spheroidization rate accounts for a significant percentage (
Figure 9b,c). Consequently, the tensile strength is significantly reduced. However, in the case of an incompletely annealed microstructure, the internal pearlitic lamellar spacing structure of the grain accounts for a considerable proportion of the grain, and the spheroidization rate is relatively low (
Figure 9d), resulting in a tensile strength of 1309 MPa. This is higher than that observed in the microstructure after spheroidal annealing and isothermal annealing.
The observed increase in tensile strength of the steel wire following annealing at temperatures below 200 °C for 30 min can be attributed to either static strain aging during low-temperature annealing or even during the storage of cold-deformed material at room temperature. The increase in wire strain serves to mitigate the effects of low-temperature annealing and subsequent strain aging in large-strain music steel wires. This phenomenon can be attributed to the supersaturation of ferrite with carbon during the cold drawing of large-strain music steel wires and the slightly higher temperatures that occur during the drawing process. These conditions allow for the intense polarization of carbon into individual dislocations in the presence of intense ferrite strain during wire drawing [
41]. This effect constrains the number of available sites on the carbon-modified dislocations during static storage at room temperature or annealing at low temperature. Furthermore, given that the formation of the ferrite dislocation cell/substructure is also decorated with carbon, the density of individual dislocations is observed to decrease with continued drawing [
25].
Interactions between interstitial solute atoms and dislocations also serve to determine a multitude of mechanical properties inherent to steel [
42]. As proposed by Y.J. Li, the formation of the cell/substructure grain in large-strain drawn steel wires after annealing at temperatures above 200 °C is not attributable to recrystallization. Rather, it can be attributed to pronounced recovery of the deformed microstructure. Upon annealing at temperatures above 250 °C, softening commences, accompanied by a pronounced decline in tensile strength. This phenomenon is primarily attributed to the static recovery of the deformed microstructure, manifested in the annihilation, rearrangement, or polygonization of ferrite dislocations [
2,
43]. Furthermore, additional microstructural modifications during annealing, including spheroidization of cementite, redistribution of alloying elements, recovery of point defects (vacancies and interstitials), and relatively minor coarsening of the cell/substructure grain, may also contribute to a reduction in tensile strength. The application of intense plastic deformation, such as cold drawing, results in the formation of a high dislocation density within the material. The majority of dislocations generated during cold drawing are stored at the interface between ferrite and cementite, where they form cell/substructure grains superimposed on the phase boundary [
25]. Concurrently, as a consequence of cementite decomposition, dislocations from adjacent ferrites may converge at the site of the original carburization, thereby forming dipole configurations [
44]. In conjunction with the accelerated diffusion process across the phase boundary resulting from severe plastic deformation, the rapid annihilation of dislocation dipoles during annealing becomes a viable phenomenon.
The inhibition of recrystallization in large-deformation SWP-B music wires can be attributed to their fiber texture [
45], which reduces the drive for recrystallization and decreases the mobility of grain boundary boundaries, as well as the formation of small-angle grain boundaries. The presence of a pearlitic lamellar spacing microstructure in music steel wires contributes to an increase in strength and hardness compared to wires containing spheroidized cementite. This is attributed to the fact that the latter material exhibits a smaller heterogeneous interfacial area fraction. During annealing at temperatures above 200 °C, the phase boundaries diminished by spheroidized cementite are replenished by the cell/substructure grain, which inhibits dislocation motion. Furthermore, the ferrite cell/substructure grain undergoes continuous coarsening in conjunction with the spheroidization of cementite throughout the annealing process [
2]. In the present case, it is challenging to quantify the reduction in tensile strength due to the loss of phase boundary density resulting from the spheroidization of the cementite. It is evident that the softening effect resulting from phase boundary loss is concurrent with the softening effect associated with cell/substructure grain coarsening.
3.4. Analysis of Musical Expression of Annealed Musical Wire
Without loss of generality,
Figure 14,
Figure 15,
Figure 16 and
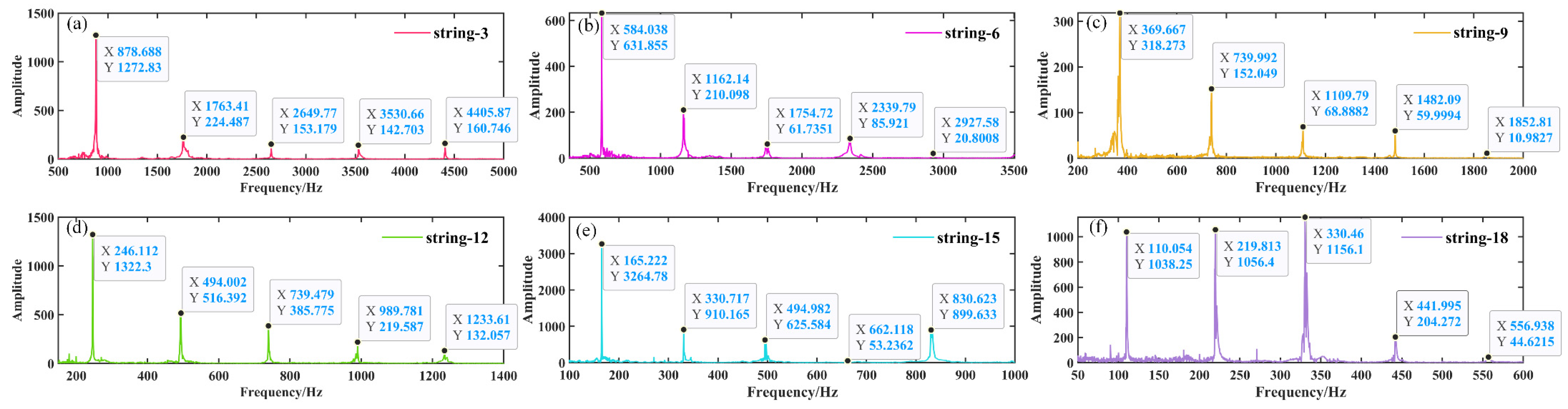
Figure 17 illustrate the selected nylon–steel composite wires of the guzheng, which are located on the 3rd string (pitched at 880 Hz) and 6th string (pitched at 493.8 Hz) in the treble region, the 9th string (pitched at 329 Hz) in the middle region, the 12th string (pitched at 220 Hz) and the 15th string (pitched at 146.8 Hz) in the bass region, and the 18th string (pitched at 110 Hz) in the double bass region. The strings are tuned in such a way that they are susceptible to excitation by the sound waves themselves, once they have been tuned. The frequency and time domain plots of the sound waves generated following a Fourier transform are presented herein.
As illustrated in
Figure 14,
Figure 15,
Figure 16 and
Figure 17, the guzheng strings are composed of nylon–steel composite wire, which produces discernible vibration signals and a prolonged aftertone. These strings are distinguished by a full and rounded timbre, a purity and softness of tone, brightness, and clarity. As the vibration of the guzheng string is a compound vibration, it exists in two distinct regions, namely the center and one third of the string. This results in the production of a compound tone, which includes both the fundamental frequency and overtones. The low-frequency overtones are of greater amplitude, resulting in a mellower tone that is more suitable for individuals with more sensitive hearing. However, the high-frequency output is less pronounced, and the tone may be perceived as more monotonous.
Figure 17 illustrates that the bass strings 12 and 15, in addition to the double bass string 18, exhibit a prolonged sustain and a gradual decay. In contrast, string 9 exhibits a more robust mid-frequency overtone, resulting in a tone that is more rounded and harmonious. In contrast, strings 3 and 6 exhibit augmented high-frequency overtones, which contribute to a brighter and more discernible tone. In the performance, the tone situated in the vicinity of the front yue-shan (a musical instrument part, specifically a horizontal wooden piece used to hold the strings on the forehead of the zither in ethnic musical instruments) is more robust, while the tone in the middle position of the zither code and the front yue-shan is comparatively softer. The entire vibration process of the guzheng’s articulation and the quantity and strength of the overtones generated have become the essential elements that define the guzheng’s distinctive tone. The tone is significantly influenced by the unique characteristics of the guzheng, including a clear and bright soprano, a round and full mid-range, a deep and dense bass, and a long aftertone.
Table 3 illustrates the mechanical property parameters that were evaluated for each of the selected nylon–steel composite wires, in addition to the bare steel wire, the 200 °C annealed steel wire, and the incomplete annealed steel wire.
As illustrated in
Table 3, the tensile strength of nylon–steel composite wire with a core steel wire diameter of φ = 0.6 mm, bare steel wire with a diameter of
φ = 0.6 mm, 200 °C annealed steel wire, and incompletely annealed steel wire is 460 MPa, 2578 MPa, 2702 MPa, and 1269 MPa, respectively. Furthermore, the Young’s modulus is 6.4 GPa, 24.1 GPa, 32.0 GPa, and 7.3 GPa, respectively. Following annealing at 200 °C, the tensile strength of the steel wire reached a maximum of 2.7 GPa. Subsequent low-temperature annealing treatment resulted in an even higher tensile strength of 1.2 GPa in the case of the incompletely annealed steel wire. The enhanced tensile strengths permit a greater degree of inharmonicity in the utilization of the musical steel wire [
18,
46], thus the steel wire strings that have undergone annealing at 200 °C and the incomplete annealed steel wire strings that have been subjected to high temperatures were selected for the assessment of the musical expression of the strings. The most significant factor influencing the functionality of a string is its stiffness. This term does not refer to the hardness of the string but rather to the external force required to produce a displacement within the string. The material properties and geometry of the string contribute to the overall stiffness of the string [
47]. In general, there is a proportional relationship between the stiffness of a material and its Young’s modulus,
E. Among the properties of a string, stiffness is of particular significance, given the strong correlation between the level of mechanical and physical properties and acoustic parameters. Moreover, the vibrational properties of the string are frequently employed to generate the sound of stringed instruments [
7,
47,
48].
Materials with high stiffness facilitate the transmission of sound waves with greater efficiency, resulting in a more robust and clearer audio output. It is evident that the stiffness of a material may be subject to alteration as a consequence of tensile forces. This alteration is of particular consequence in audio apparatus, as it can influence the velocity of sound wave propagation and the frequency response of the audio [
47,
49]. To illustrate, 200 °C annealed steel wire (
E = 24.1 GPa,
TS = 2702 MPa) and bare steel wire (
E = 32.0 GPa,
TS = 2578 MPa) exhibit increased modulus and tensile strength, allowing them to withstand greater stresses under tension. This results in superior tone retention and a richer, more articulate tone due to their capacity to efficiently conduct high-frequency vibrations. The audio spectral clarity and richness of high-frequency harmonics directly influence the musical expression of the instrument [
48]. In contrast, softer materials, such as nylon–steel composite wire (
E = 6.4 GPa,
TS = 460 MPa) and incomplete annealed steel wire (
E = 7.3 GPa,
TS = 1270 MPa), display greater susceptibility to vibration during stretching. This has an impact on the fullness of the sound quality and can result in a reduction in performance at higher pitches, which in turn affects the overall sound expression. Steel wire that has undergone annealing at 200 °C (with a Young’s modulus of 32 GPa) exhibits the highest Young’s modulus, which provides enhanced rigidity and stability. This makes it an optimal choice for generating higher frequencies and harmonics, which contribute to a more precise and clear sound quality. In comparison, incompletely annealed steel wire (with a Young’s modulus of 7.3 GPa) displays diminished rigidity, which gives rise to instability in its high-frequency expression and a mellifluous, warm tone that may be more conducive to specific applications. However, this is frequently accompanied by a reduction in volume and loudness.
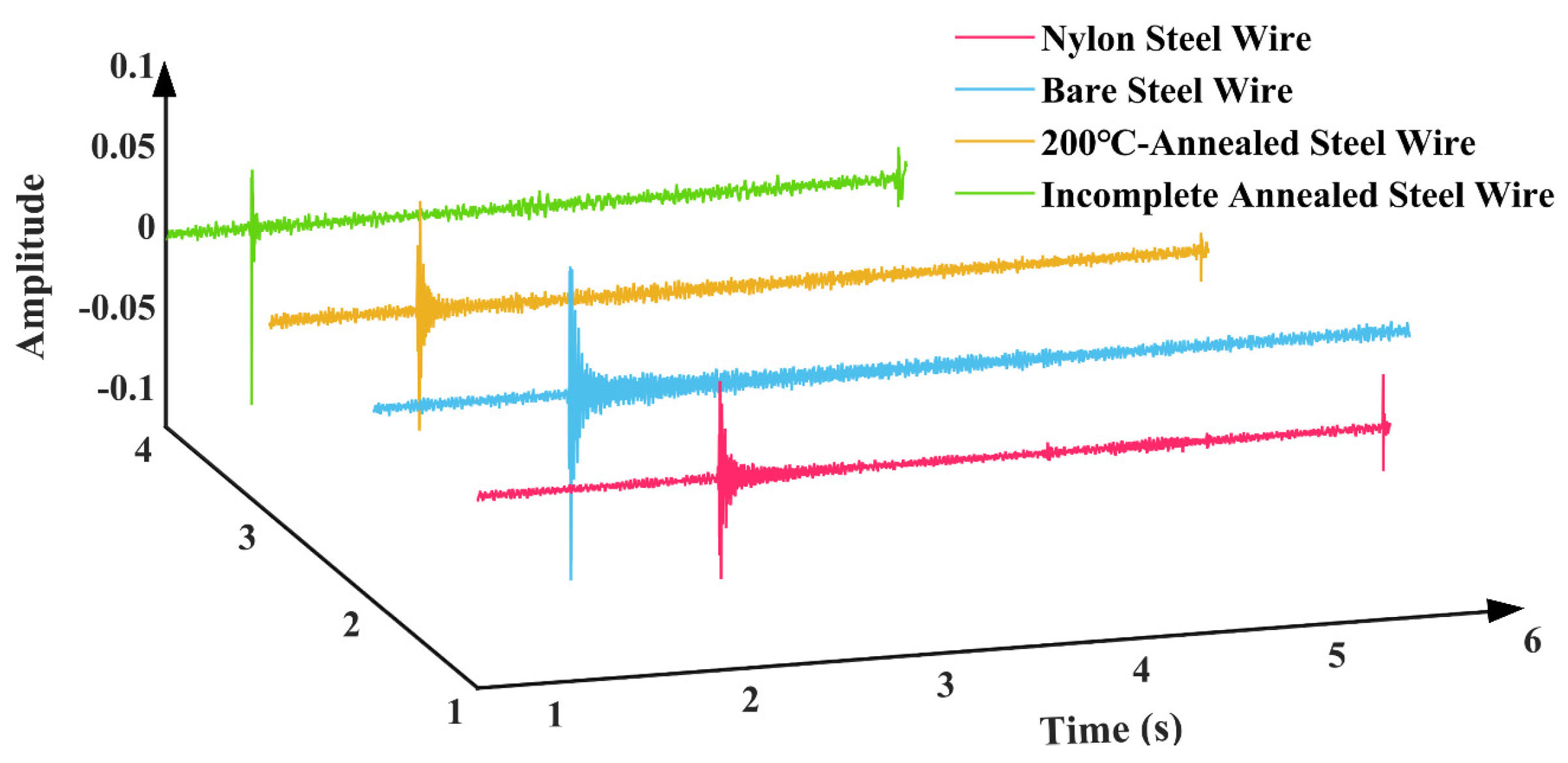
Figure 18,
Figure 19 and
Figure 20 illustrate the time domain, frequency domain plots, as well as the 3D spectrograms of four different string materials played under excitation for the A6 tone (standard fundamental frequency of 1760 Hz) after Fourier transformations for comparison.
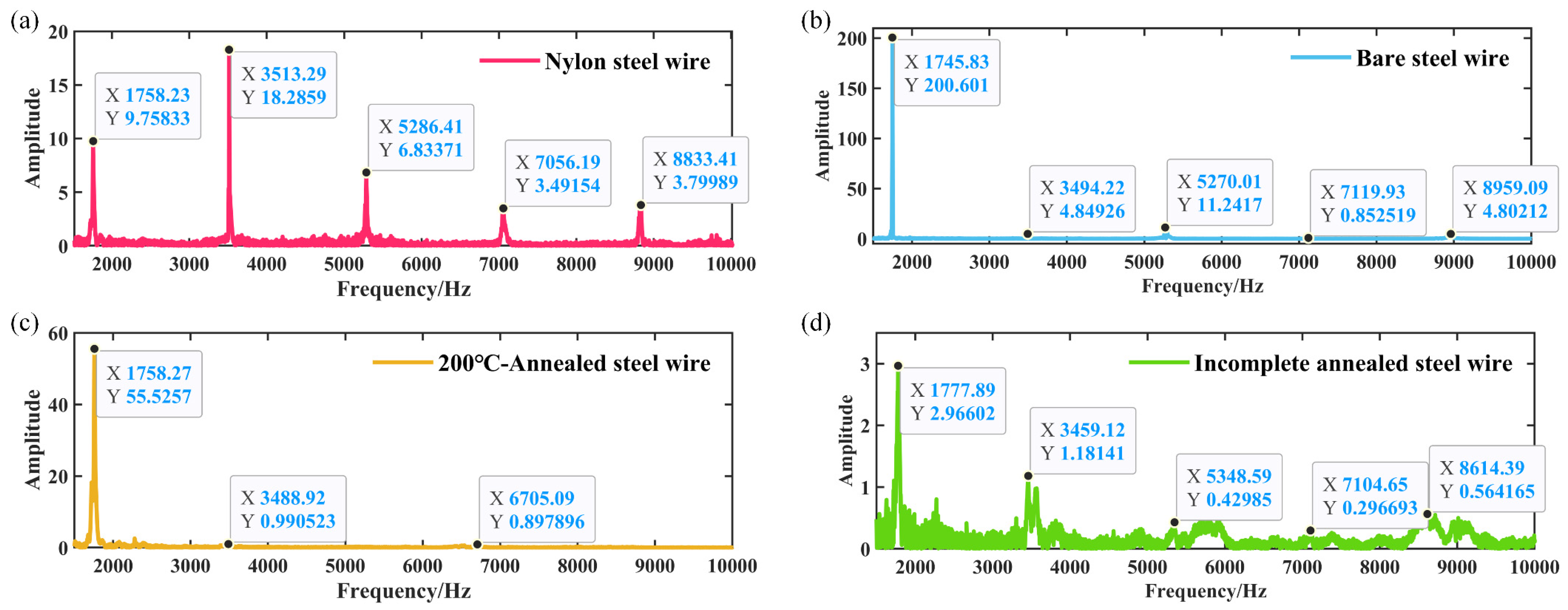
Table 4 presents the frequencies (amplitudes) of the first five harmonics of the A6 tone when played by the four wires that underwent distinct treatments.
As illustrated in
Figure 19 and
Figure 20 and
Table 4, musical wires composed of different materials demonstrate disparate frequency shifts between the various harmonic orders [
50]. The first harmonic (fundamental frequency) exhibits a range of approximately 1745 Hz to 1777 Hz, with the highest frequency (1777 Hz) observed in the incompletely annealed steel wire, resulting in a brighter tone. The second harmonic exhibits a relatively narrow frequency range, with the nylon–steel composite wire (3513 Hz) demonstrating superior stability compared to the other materials, indicative of enhanced frequency retention. The spectra of the various wire material distributions demonstrate that the annealing treatment has a considerable impact on the harmonic frequencies [
13]. In particular, the third harmonic lacks data for the bare steel wire annealed at 200 °C. However, the nylon–steel composite wire (5286 Hz) and incomplete annealed steel wire (5348 Hz) exhibit higher frequencies, indicating that both may be more effective in high-frequency timbres. Additionally, the fourth and fifth harmonics demonstrate a notable variation in frequency. The steel wire annealed at 200 °C (6705 Hz) exhibited relatively low frequencies at the fourth harmonic, which may contribute to a more profound aftertone. The amplitudes of the harmonics exhibited variability across the different materials. The amplitude characteristics of the bare steel wire and the nylon–steel composite wire are more pronounced in the higher harmonics, particularly the 5th harmonic. This may result in a richer tone and improved layering. The amplitude of the incomplete annealed wire is lower (8614 Hz), which affects the roundness and subtlety of the tone.
Figure 19 demonstrates that the steel wire that has undergone incomplete annealing displays a greater number of harmonics and a less discernible frequency amplitude. Upon the application of an excitation, the stabilization of the fundamental frequency of the nylon–steel composite wire occurs at a slower rate, while the stabilization of the fundamental frequency of the bare steel wire occurs at a faster rate. In conjunction with the Young’s modulus test, the nylon–steel composite wire and the incompletely annealed steel wire exhibited the lowest Young’s modulus value among all the strings, indicating that these materials possess relatively low stiffness and high deformability. This resulted in an instability and a less uniform vibration pattern when the strings were subjected to playing stimulation. Furthermore, the fundamental frequency demonstrated a prolonged stabilization period. The nylon–steel composite wire displays a greater richness of frequency components, a relatively weaker presence of high frequencies, and a notable prominence of spectral peaks in the low-frequency range. The fundamental frequency (1758 Hz) is robust and exhibits a gradual decay, while its harmonics (3513 Hz, 5286 Hz, 7056 Hz, 8833 Hz) are somewhat diminished and decay more rapidly, allowing for clear differentiation between scales. The timbre of nylon–steel composite wires is typically mellifluous with a warm quality. The phase response of these strings may result in relatively weak volume peaks at specific frequencies. These frequencies are distinguished by lower energy in the high frequencies and a more even distribution of harmonics, often with a greater proportion of low-frequency components. This can be attributed to the enhanced richness and layering of the string’s timbre resulting from the wrapping of the nylon and copper wires, which also increases the durability and abrasion resistance of the strings while maintaining pitch stability and reducing intonation fluctuations [
51].
In comparison to nylon–steel composite wire, bare steel wire displays enhanced stiffness, which results in a diminished amplitude and concentrated energy in the lower-frequency region. The fundamental frequency component in the spectrum is markedly amplified, accompanied by a more pronounced and powerful high-frequency component. Furthermore, the harmonics are more pronounced and robust, contributing to a brighter and clearer tone with a wider frequency distribution. The resonance effect and transient response of bare steel wire are typically more pronounced, with the potential for additional high-frequency peaks to appear within the frequency spectrum. Bare steel wires typically exhibit higher power and a more pronounced volume than nylon–steel composite wires and 200 °C annealed steel wires under the same playing force. This characteristic results in the generation of more penetrating acoustic effects. The spectral characteristics of 200 °C annealed steel wire are analogous to those of bare steel wire, albeit with a diminution in harmonics and a slight attenuation of the high-frequency range. The fundamental frequency and its harmonic energy are diminished, resulting in a less robust sound than that of ordinary steel wire strings. The initial stages of harmonic attenuation are accelerated, and the overall timbre is reminiscent of that nylon–steel composite wires. Nevertheless, the distinctive attributes of bare steel wire characteristics remain discernible. The spectrum of the incompletely annealed steel wire exhibits the most moderate characteristics. The application of high-temperature annealing can facilitate the release of internal stress, thereby enhancing the resonance and high-frequency gain. The spectrum displays a substantial high-frequency component and a complex harmonic structure. However, the energy is low and the sound quality is relatively poor, with a noticeable decrease in high-frequency power.
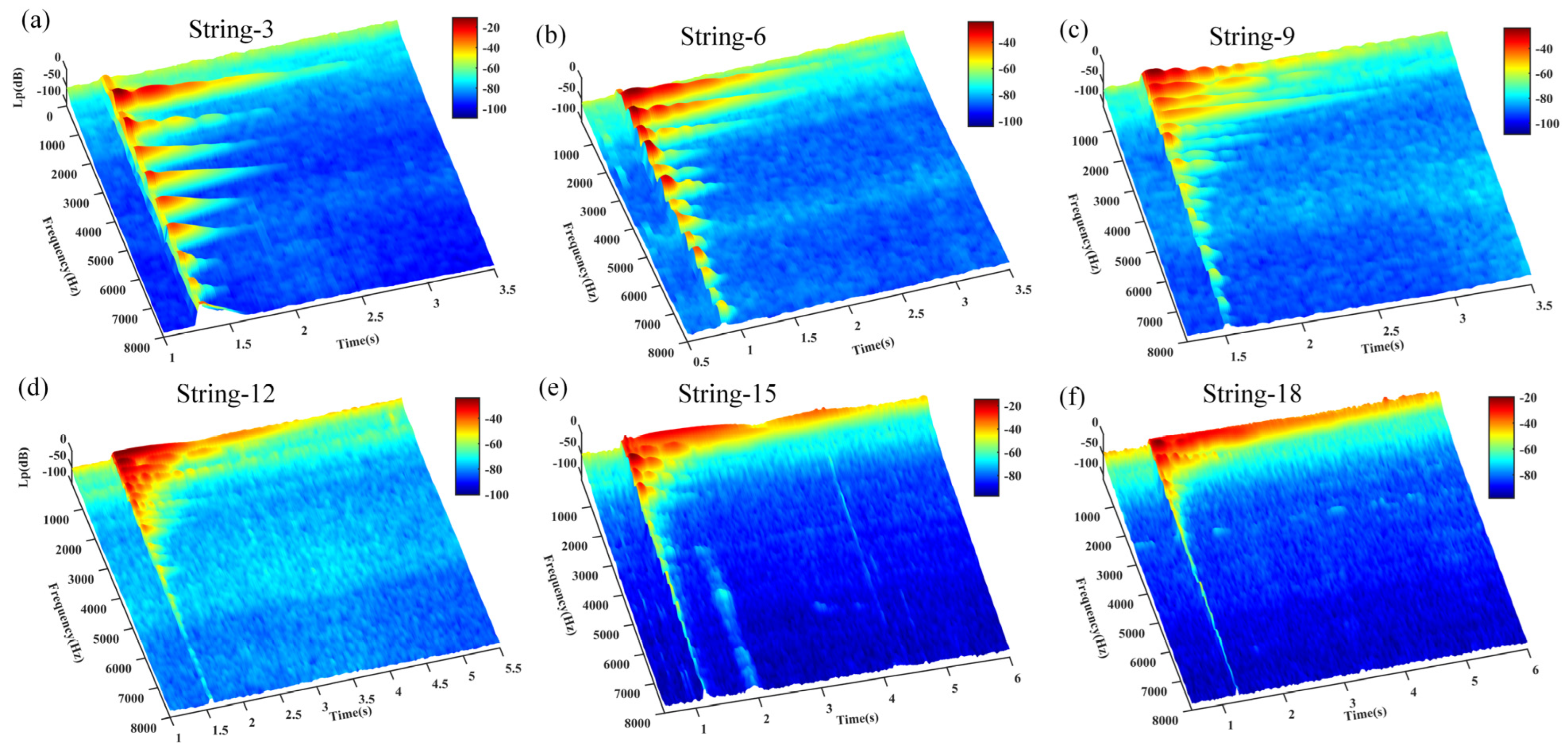
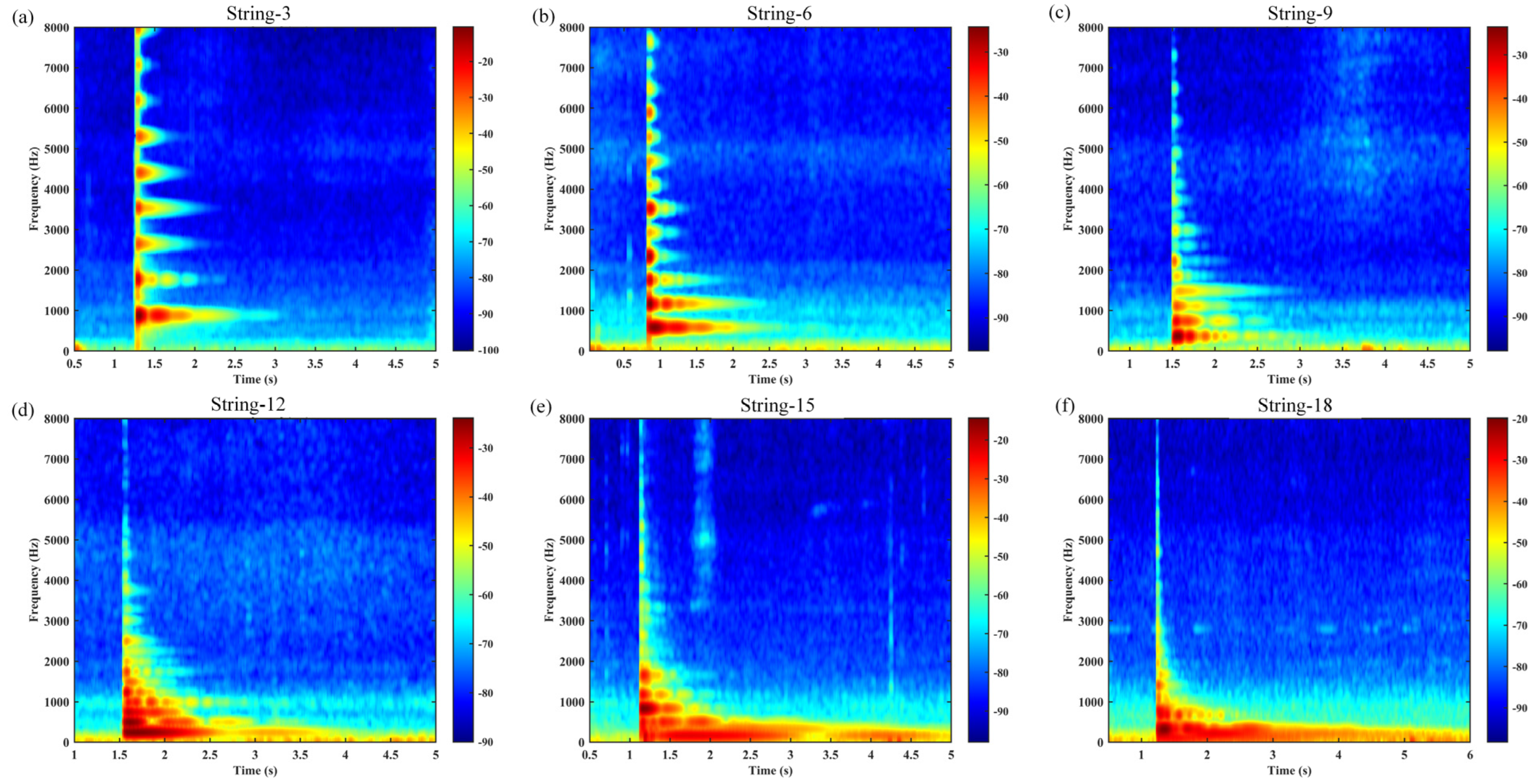
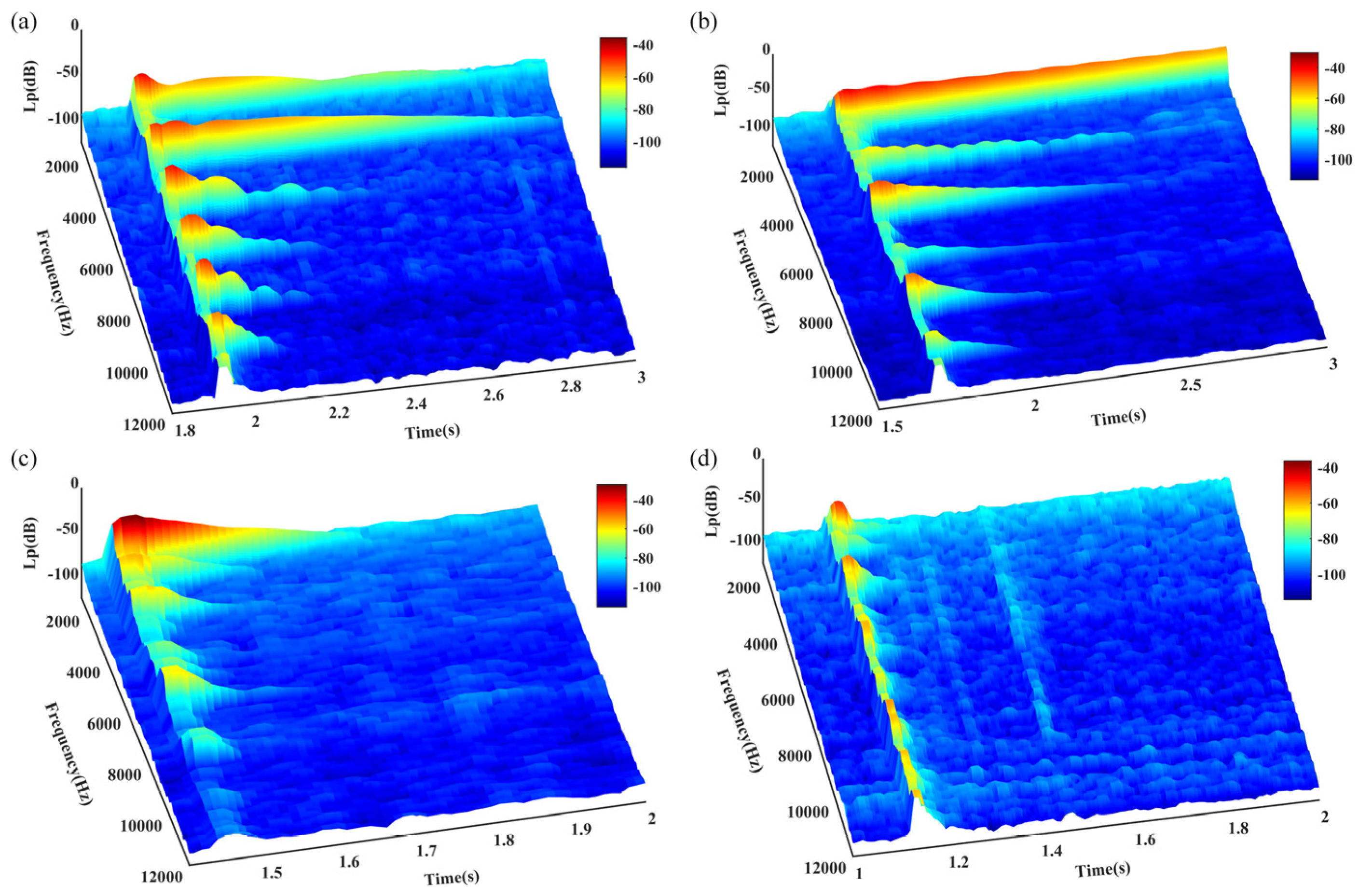
Figure 21 illustrates the time–frequency plots of the acoustic signals generated by each string of the aforementioned guzheng under plucking excitation following distinct heat treatments. These plots demonstrate the regularity of the changes in harmonic components over time.
Table 5 presents the calculated decay times of the fundamental frequency harmonic components of the acoustic signals generated during the vibration of four typical guzheng strings under excitation.
As illustrated in
Figure 21 and
Table 5, the duration of the intervals for all four strings of the guzheng exhibits variability to varying degrees. Consequently, the duration of the resulting sound signals is also subject to alteration. The fundamental wave components of all four strings of the guzheng reach their peak values with remarkable swiftness after the application of the plucking force and then undergo a rapid decay until they cease to exist [
12].
The various categories of wires exhibited notable differences in their respective decay times. The bare steel wire exhibited the longest decay time (319.7 ms), while the incompletely annealed steel wire demonstrated a markedly shorter decay time of 2.3 ms, indicating a rapid decay of vibration energy. These findings suggest that there are notable differences in vibration retention among various materials. This phenomenon is associated with the impact of attenuation on sound vibration, which is contingent upon the specific microstructure of the material in question [
52]. The decay time of the steel wire that has undergone a 200 °C annealing process is 41.6 ms, which indicates that it is better able to retain its acoustic properties. In contrast, the nylon–steel composite wire shows better sound retention in practical applications (96.5 ms), indicating that the material properties of the incompletely annealed steel wire are more favorable than those of the aforementioned wire, but not as optimal as those of the bare steel wire. The incorporation of a winding layer into the latter results in an increase in the weight of the string, the tension of the string, and the vibration characteristics of the steel wire core. This ensures a suitable amplitude and markedly improves the sound quality of the string.
The rest of the nylon–steel composite wire is shorter than that of bare steel wire. The material has a more readily dissipated sound, a relatively low sound beta value, a smaller volume, and is suitable for the expression of delicate, soft, and lyrical music. However, the material’s softness constrains the emotional richness that it is capable of expressing. The fundamental frequency of the initial excitation exhibits enhanced stability, however, it is susceptible to fluctuations resulting from fingering. The fundamental frequency stabilization of bare steel wire occurs more rapidly, allowing it to quickly reach a stable state. The pitch accuracy is higher, with a decibel value that is greater, a volume that is more pronounced, rich harmonics, an aftertaste that is longer, a relatively long decay time, and a slower auditory disappearance. Bare steel wire is capable of expressing a wide range of emotions, rendering it an optimal selection for fast-paced, high-intensity musical compositions. The bright sound quality of this instrument allows for the clear conveyance of a multitude of musical emotions.
Table 5 lends further support to the hypothesis that the frequency of annealed steel wire at 200 °C is analogous to that of nylon–steel composite wire, with the latter displaying a more pronounced first-order frequency (1758 Hz). However, the first harmonic decays more rapidly, resulting in a notable decline in the amplitude of high-order frequencies. Although these changes may be discernible to the human ear, the resulting lack of rhythm and lack of tone, along with the loss of musical beauty, are less perceptible. The microstructure of the 200 °C annealed material is more uniform, which results in enhanced energy propagation characteristics, improved mechanical properties, and a fuller, relatively warm tone. This renders it more conducive to the expression of emotionally rich music. The annealing process has the effect of reducing internal stresses, increasing the toughness and elasticity of the string, and enhancing its responsiveness when played. The annealing process alters the crystalline structure of the material, resulting in a refinement of the grain structure. This improvement in the material’s structure enhances the musical expression and sound quality of the string. A more uniform vibration results from a superior microstructure, which in turn gives rise to a richer harmonic characteristic and an enhanced overall acoustic effect. Consequently, the frequency of incompletely annealed steel wire is relatively high, and the amplitude is significantly lower in the low- and middle-frequency bands. This may result in a lack of warmth in the fundamental tone, rendering it suitable for the expression of certain high-frequency characteristics. Nevertheless, there are some limitations in regard to emotional expression.