Study on the Ultimate Load Failure Mechanism and Structural Optimization Design of Insulators
Abstract
1. Introduction
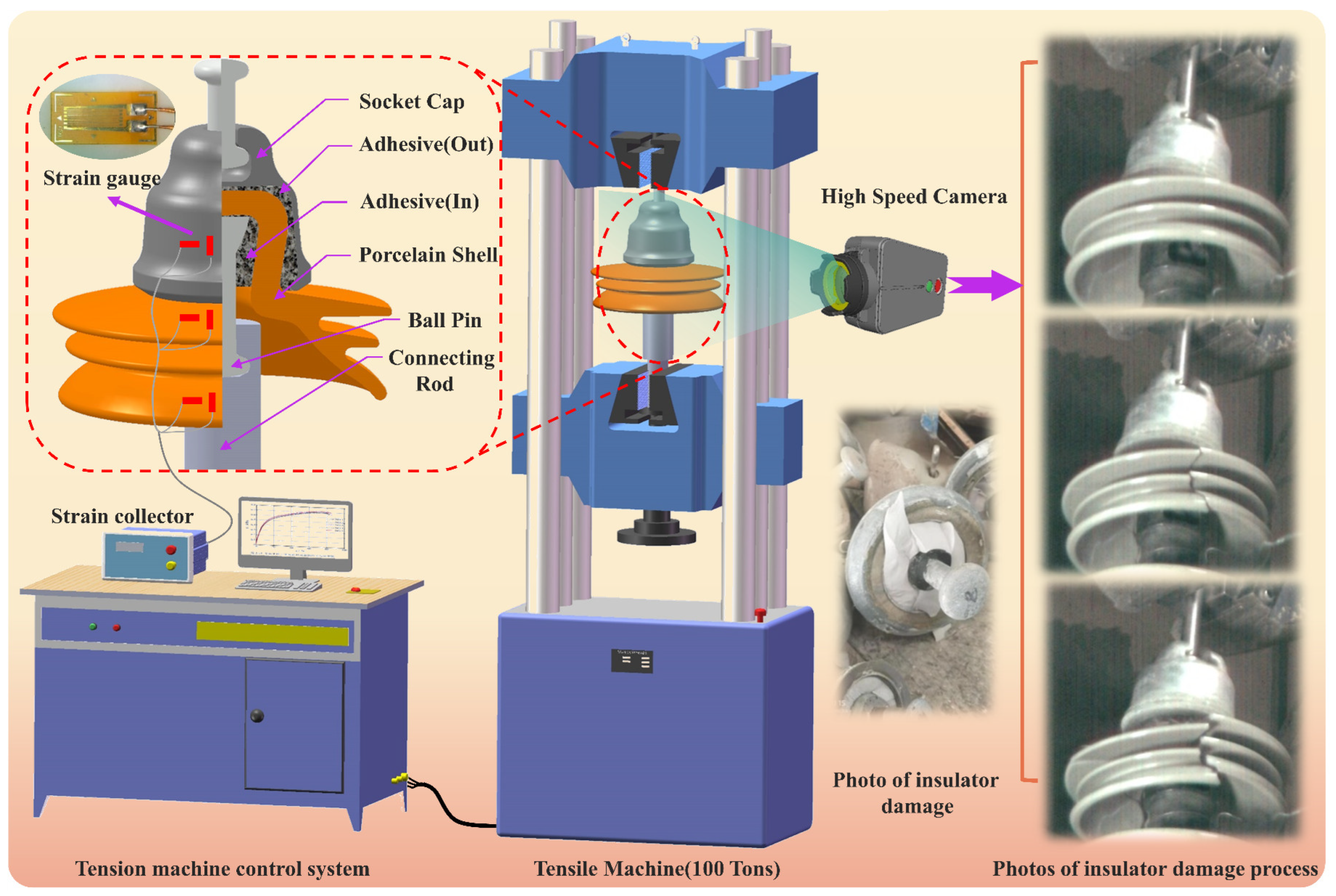
2. Insulator Tensile Testing
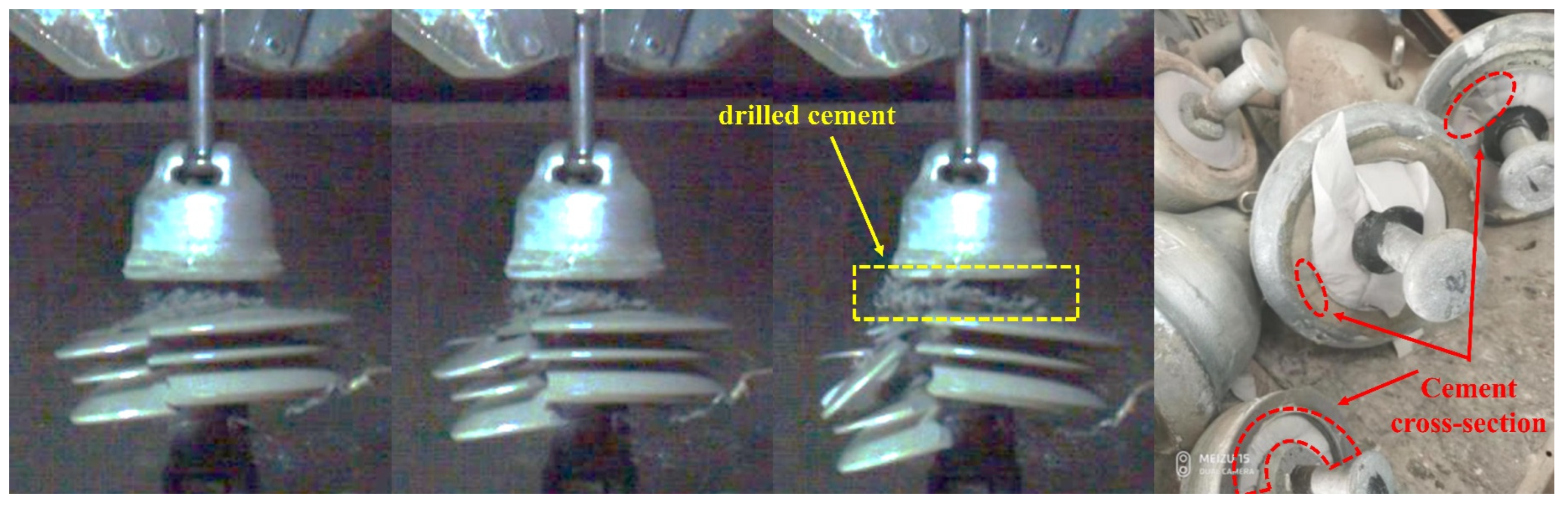
2.1. Insulator Tensile Test Results
3. Numerical Simulations
3.1. Pre-Processing
3.2. Simulation Results and Analysis
4. Simulation Validation Experiments
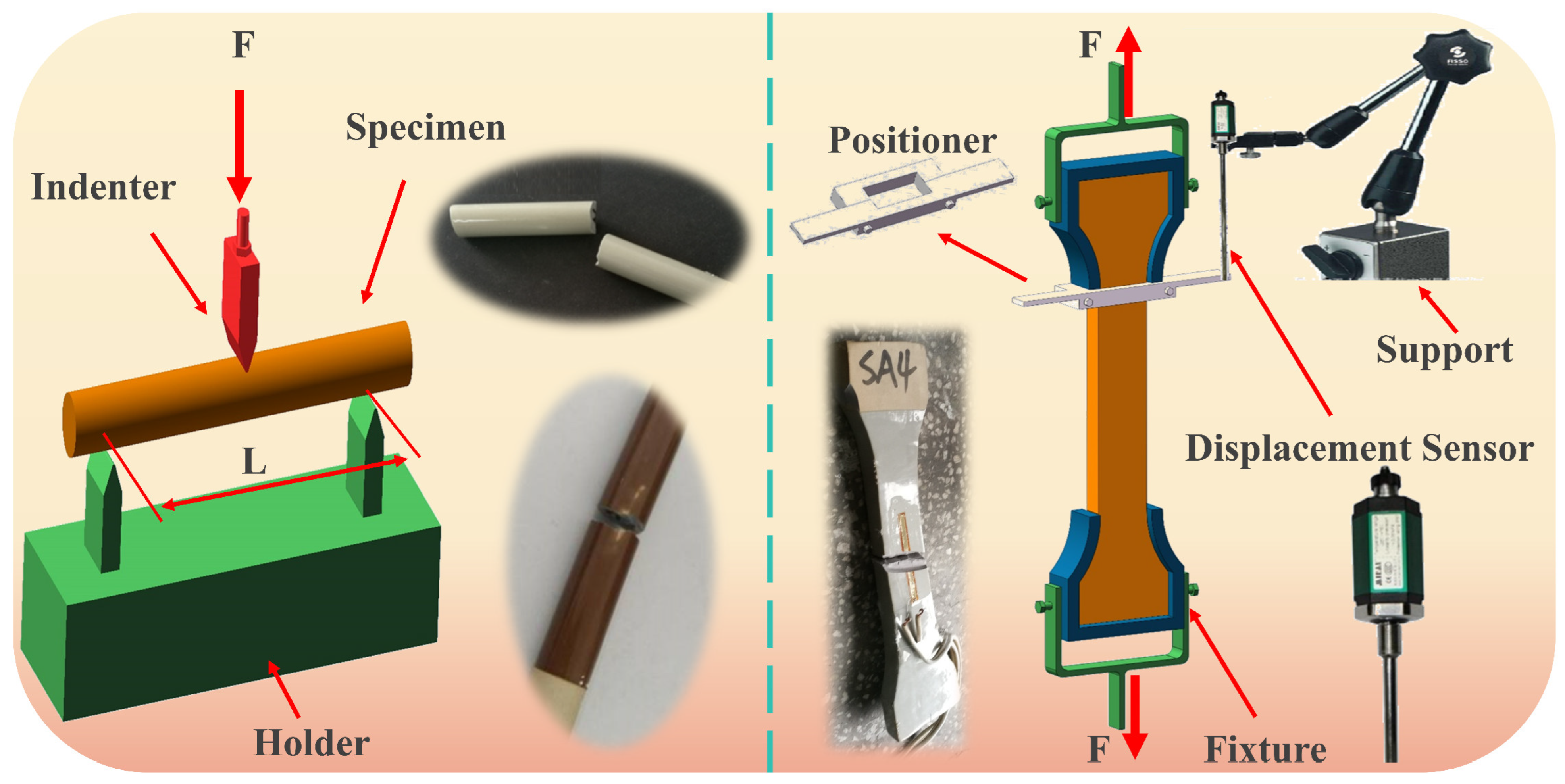
4.1. Verification of Simulated Strain
4.2. Verification of Simulation Stress
5. Simulation Validation Experiments
5.1. Introduction to Design Optimization
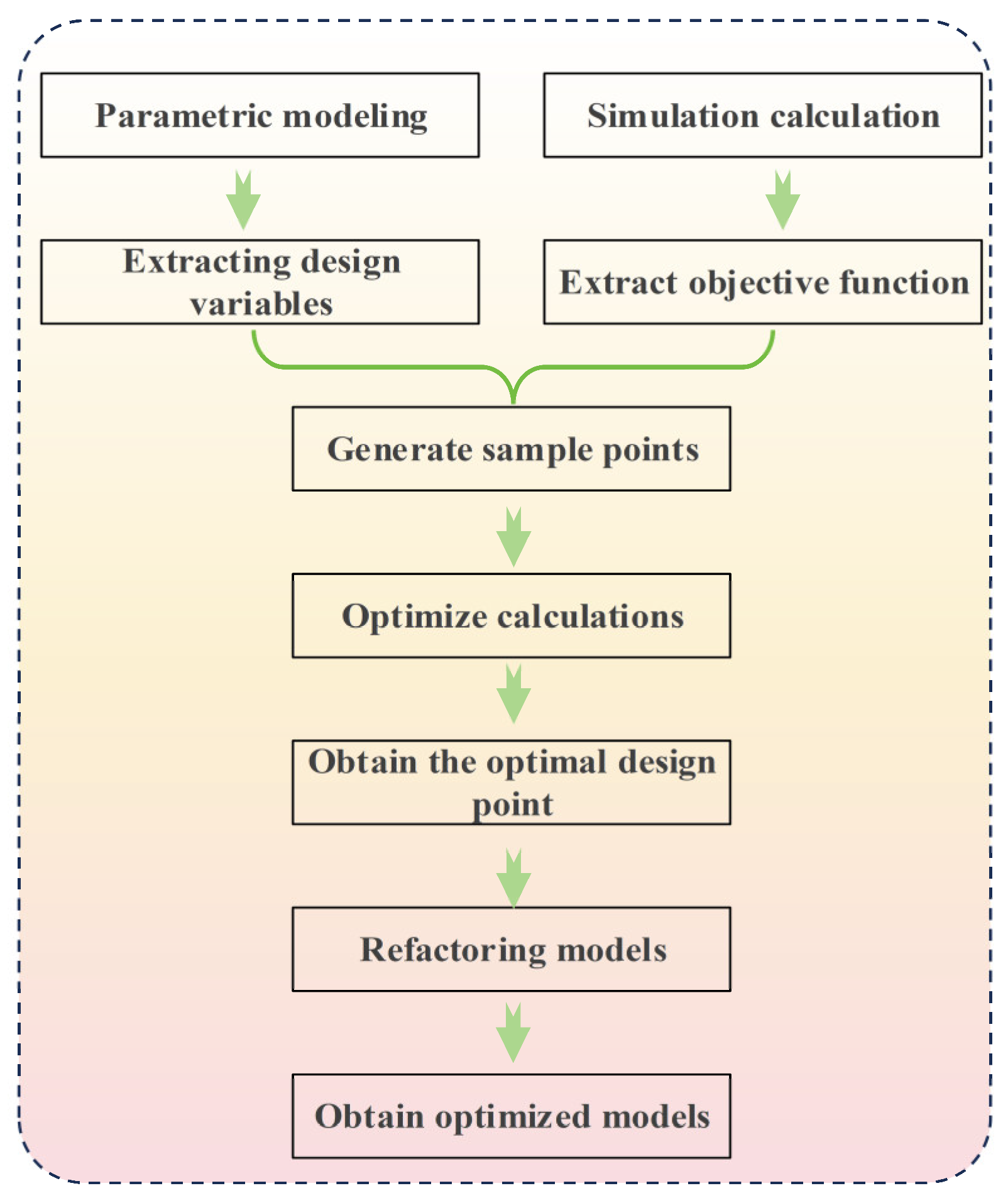
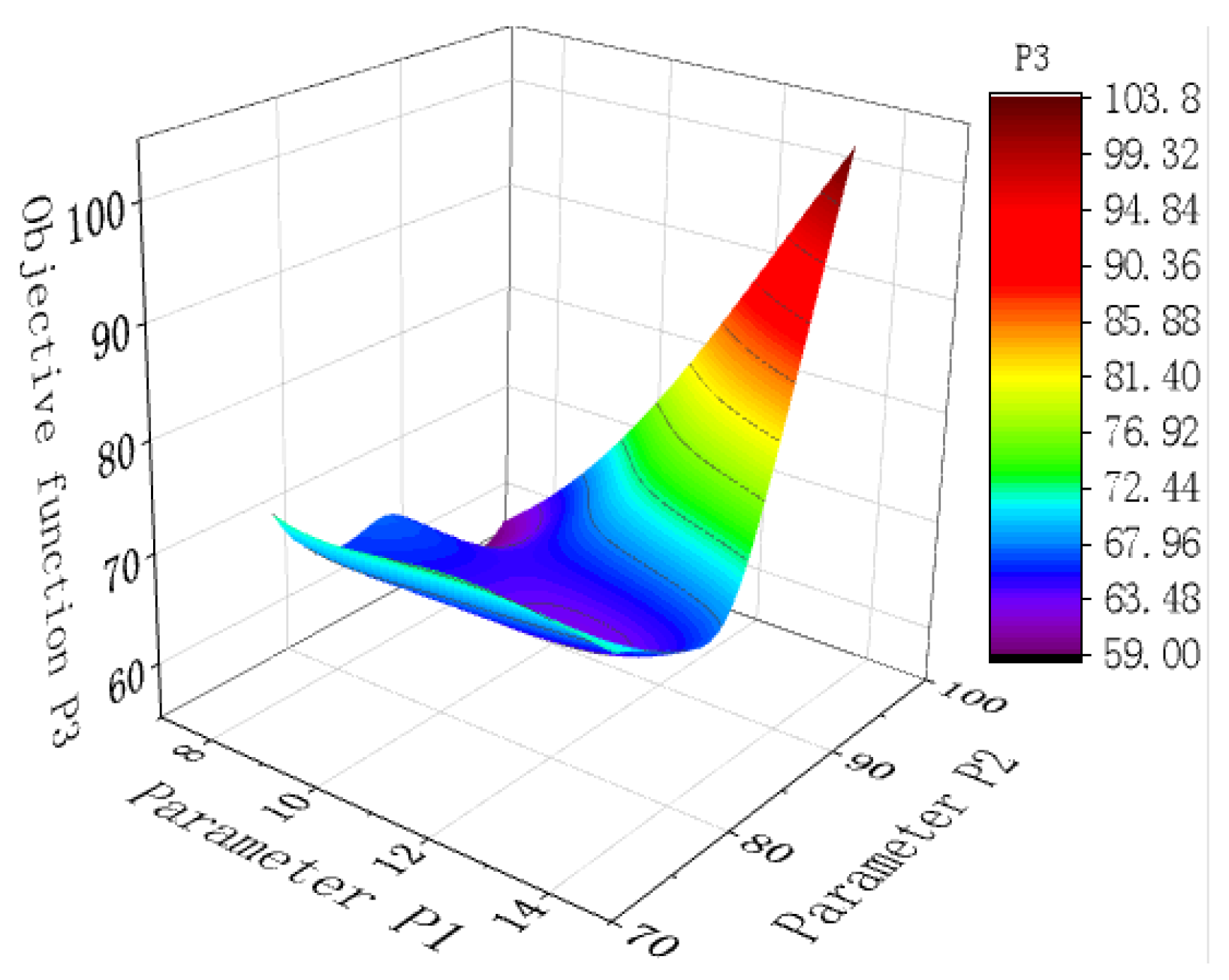
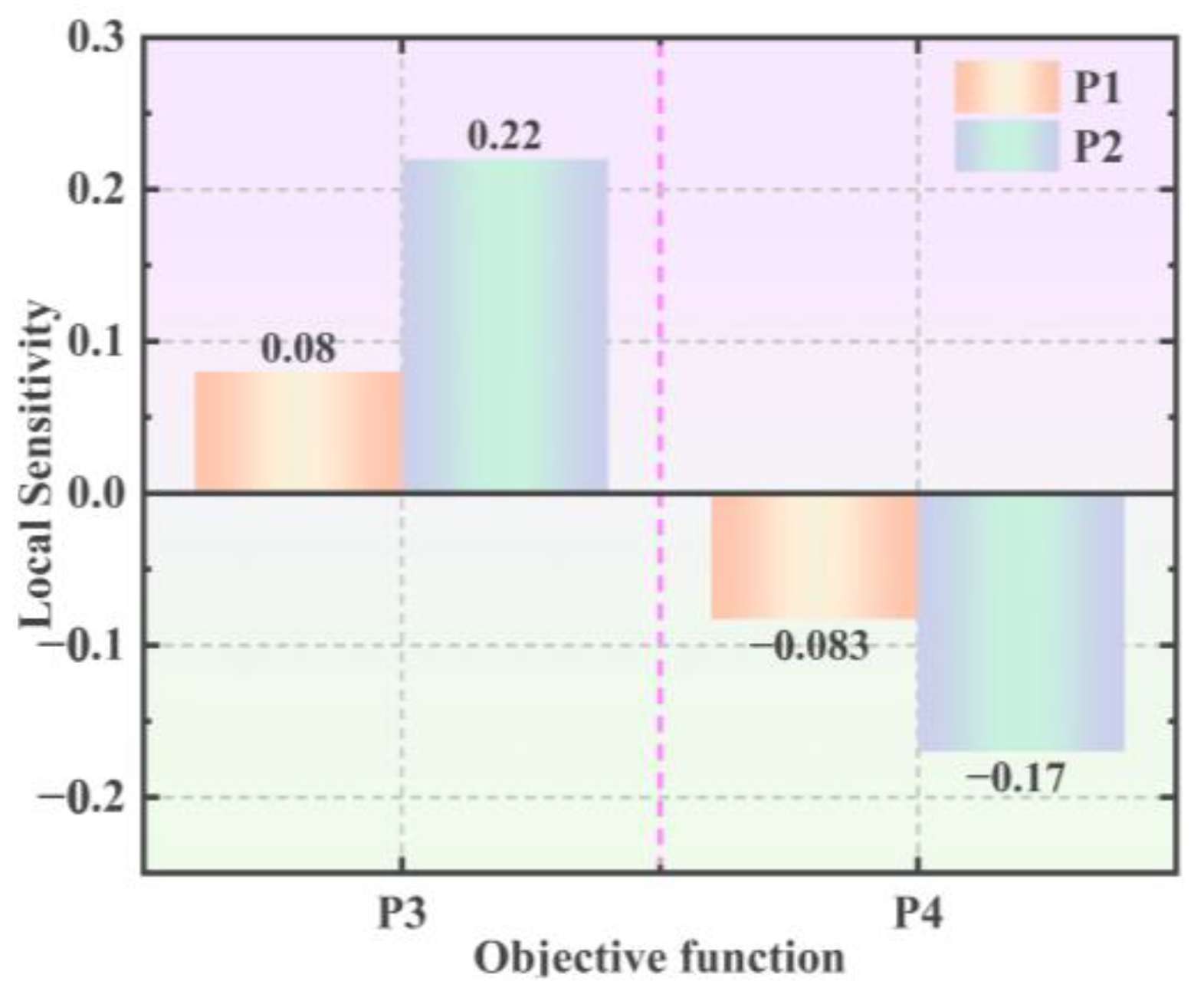
5.2. Optimization Process and Analysis of Results
6. Conclusions
- A tensile strength test conducted on a specific insulator model reveals the highest tensile force of 551 KN. The failure images indicate complete detachment of the porcelain shell, while the other components remain largely intact. These findings suggest that the porcelain shell acts as the weakest link among the insulator components.
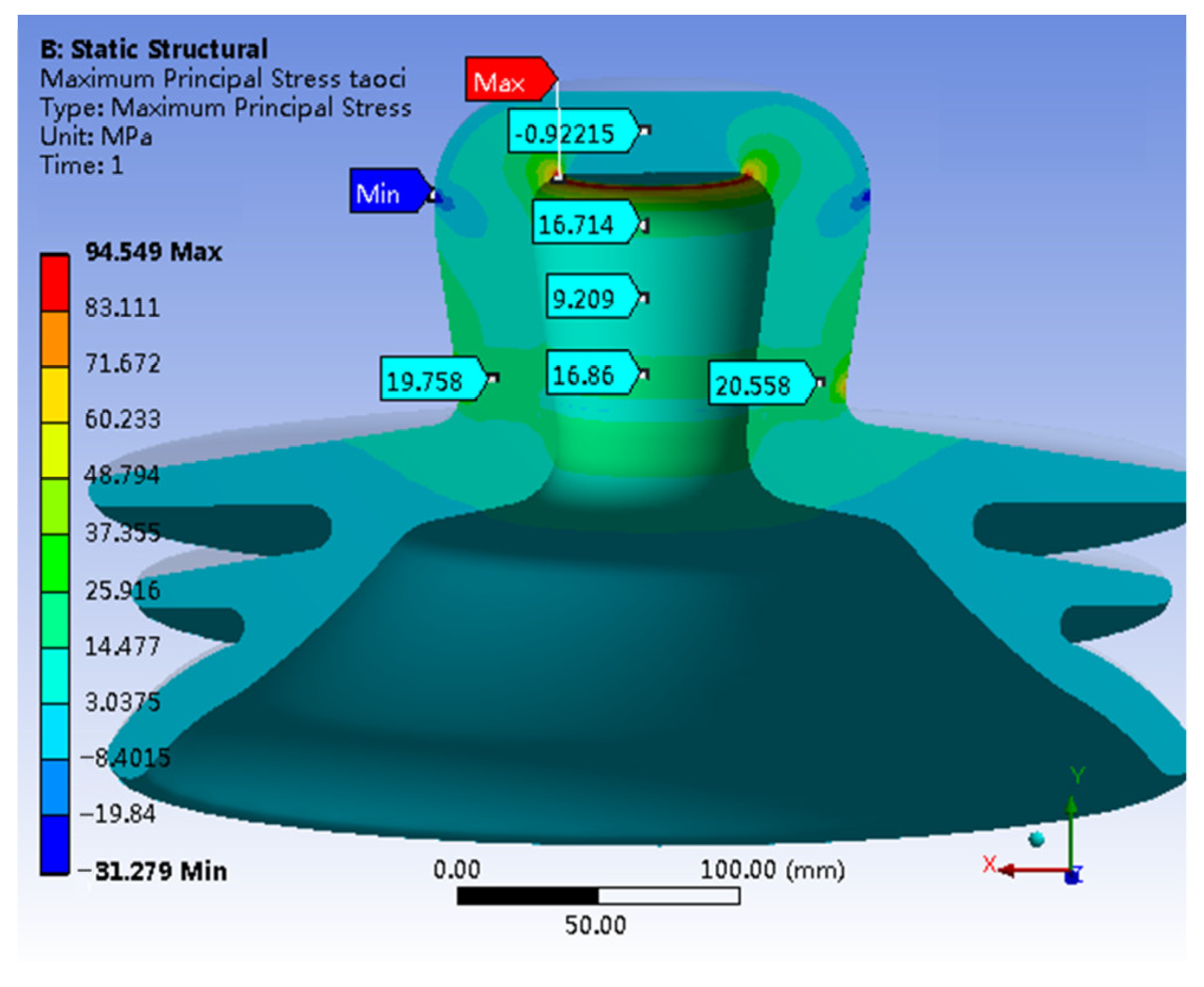
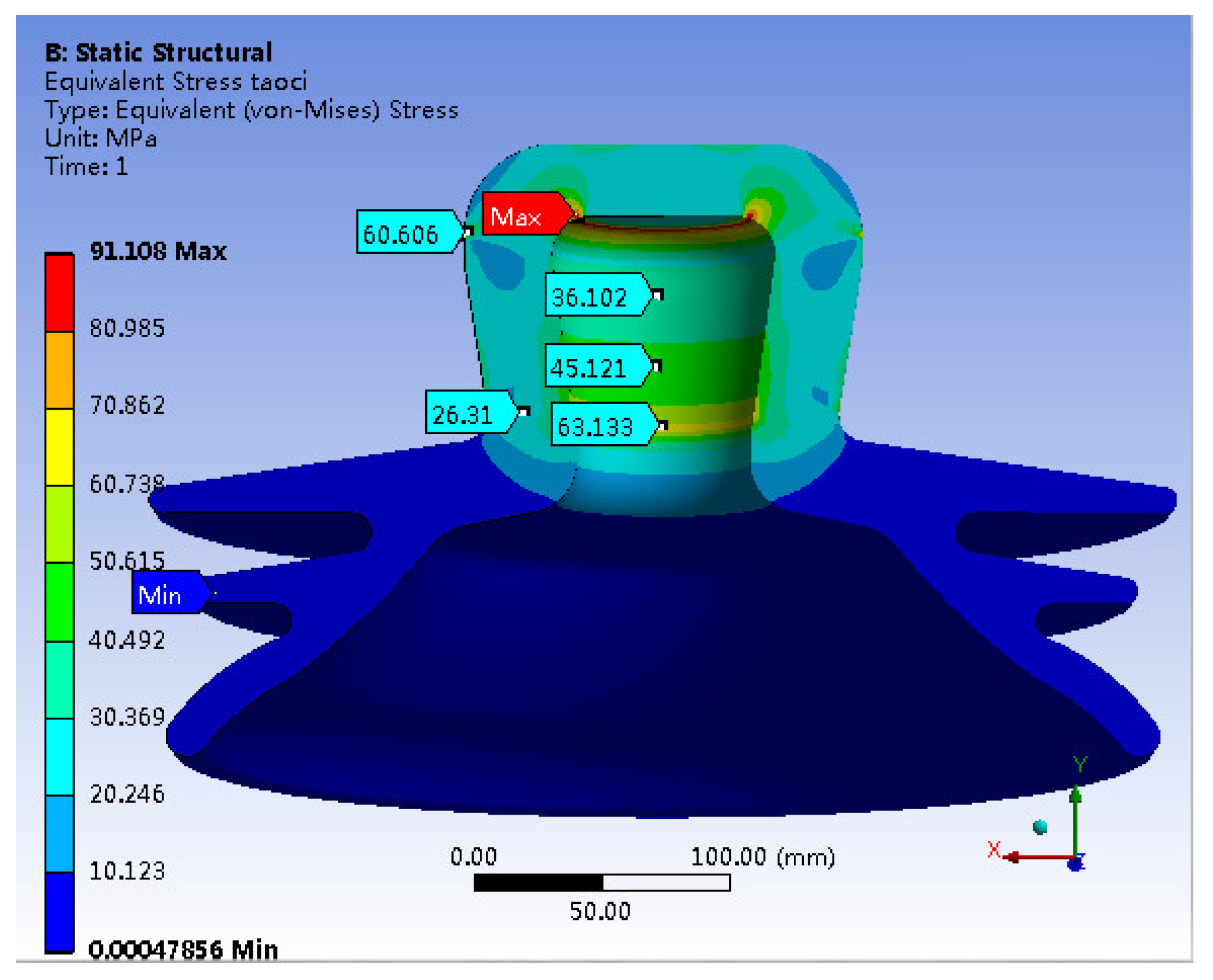
- Simulation results reveal that under an ultimate load of 551 KN, various components of the insulator exhibit notable stress concentrations. Specifically, the porcelain shell experiences the maximum first principal stress of 94.549 MPa and the maximum von Mises stress of 91.108 MPa.
- The simulation results highlight a stress distribution in the neck of the porcelain shell closely resembling that of a beam subjected to bending moments under the ultimate load. The insulator’s failure can occur when the porcelain shell exceeds its bending capacity.
- A comparison between experimental and simulation results reveals a ceramic bending strength of 100.52 MPa, while the maximum simulated first principal stress in the porcelain shell is 94.549 MPa, with a negligible error of only 5.49%. This confirms that insulator failure occurs when the porcelain shell exceeds its bending capacity, but also highlights the significant agreement between simulation and practical situations.
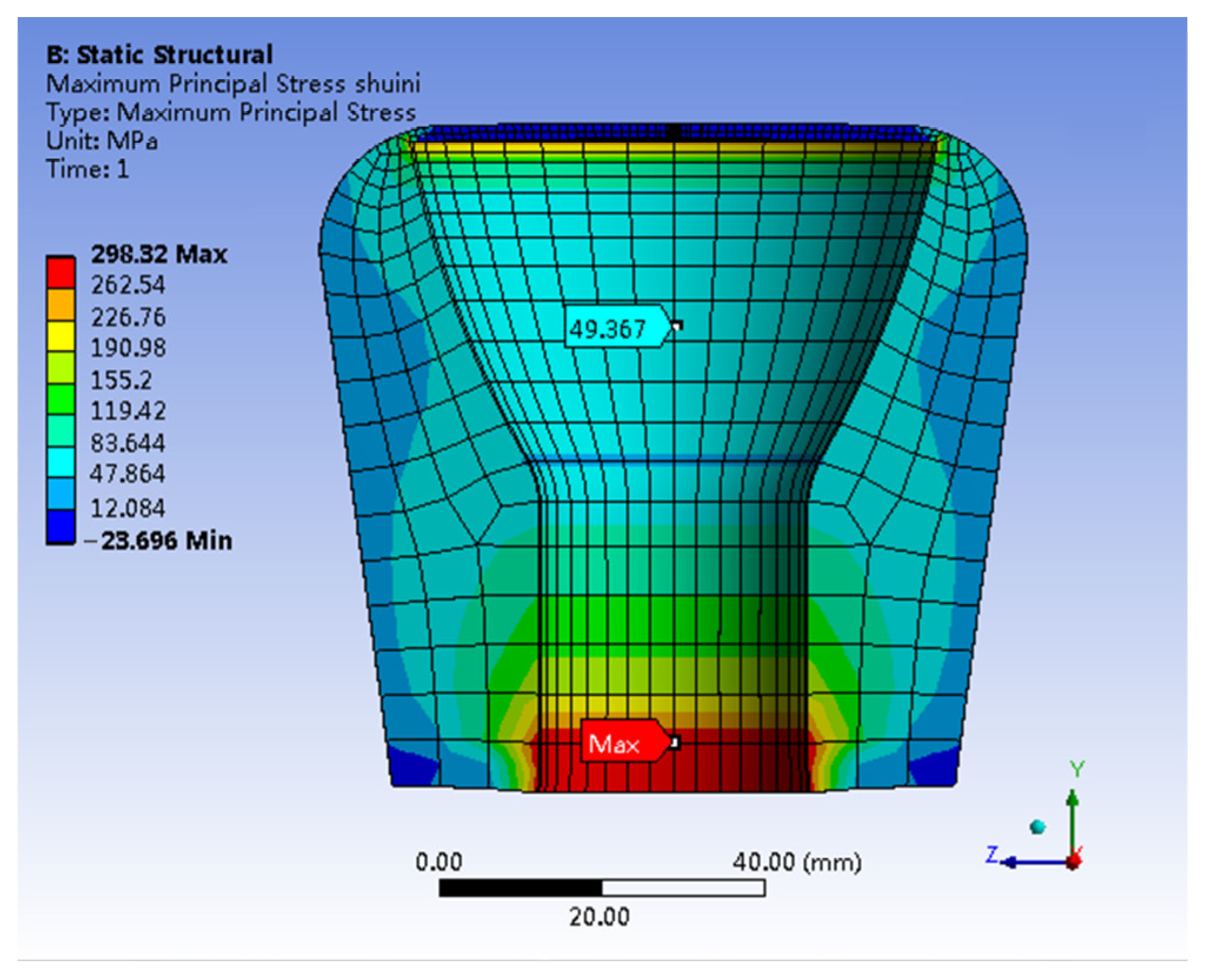
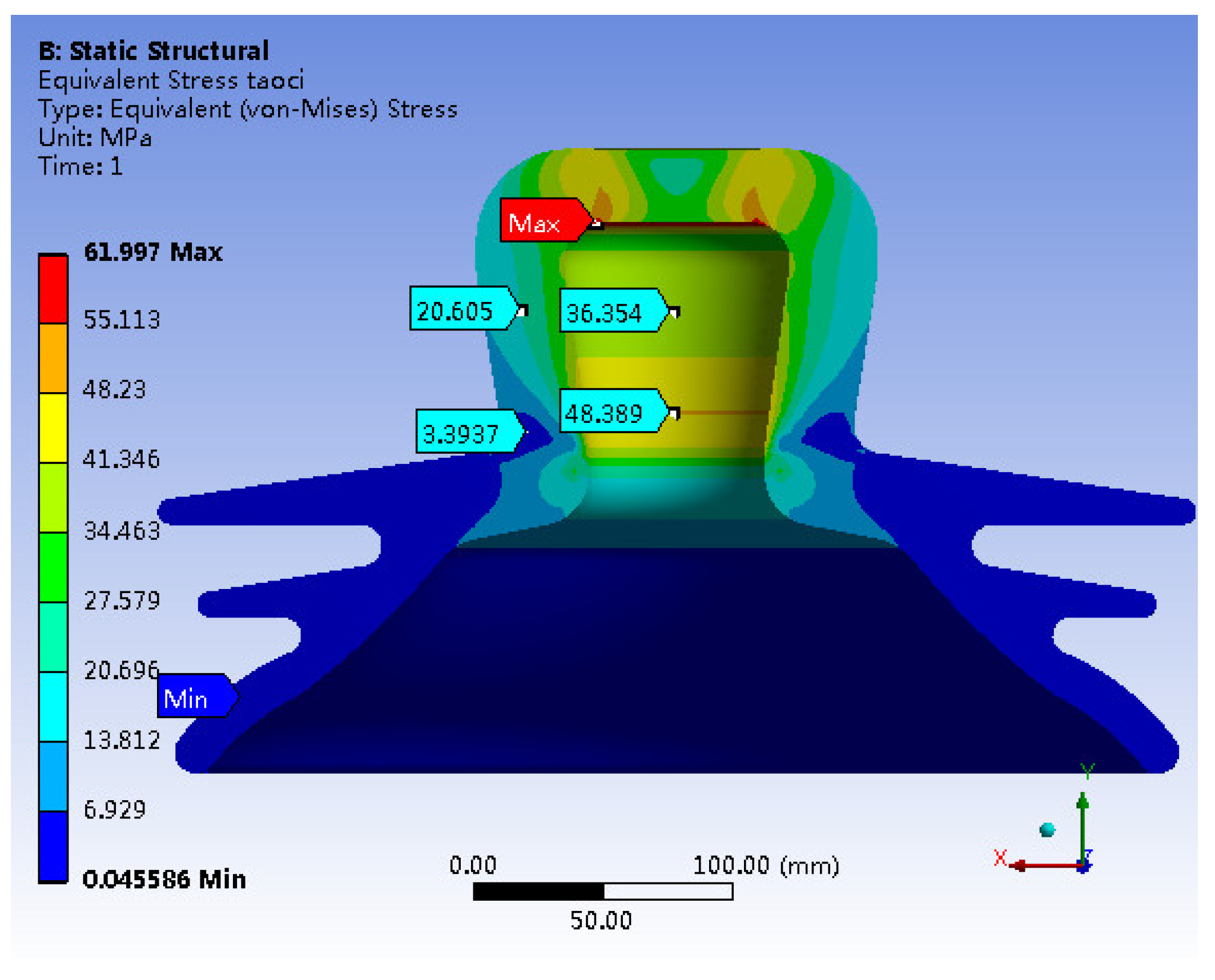
- Leveraging the design optimization feature of simulation software, the optimal solution is derived from numerous design alternatives. This leads to a substantial enhancement of insulator strength, reducing the maximum stress in the porcelain shell from 94.549 MPa to 49.481 MPa, a decrease of 47.6%. Additionally, the Mises stress decreases from 91.108 MPa to 61.997 MPa, signifying a reduction of 31.9%. The optimization significantly enhanced the overall strength of the insulator.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hackam, R. Outdoor HV composite polymeric insulators. IEEE Trans. Dielectr. Electr. Insul. 1999, 6, 557–585. [Google Scholar] [CrossRef]
- Akbar, M.; Mehmood, B. Global experience of HVDC composite insulators in outdoor and indoor environment. Rev. Adv. Mater. Sci. 2020, 59, 606–618. [Google Scholar] [CrossRef]
- Bonhôte, P.; Gmür, T.; Botsis, J.; Papailiou, K.O. Stress and damage analysis of composite–aluminium joints used in electrical insulators subject to traction and bending. Compos. Struct. 2004, 64, 359–367. [Google Scholar] [CrossRef]
- Klüss, J.; Rostaghi-Chalaki, M.; Whittington, W.; Rhee, H.; Whittington, S.; Yadollahi, A. Porcelain insulation—Defining the underlying mechanism of failure. High Volt. 2019, 4, 81–88. [Google Scholar] [CrossRef]
- Li, S.; Tsang, H.-H.; Cheng, Y.; Lu, Z. Seismic testing and modeling of cylindrical electrical equipment with GFRP composite insulators. Compos. Struct. 2018, 194, 454–467. [Google Scholar] [CrossRef]
- Bansal, A.; Schubert, A.; Balakrishnan, M.V.; Kumosa, M. Finite element analysis of substation composite insulators. Compos. Sci. Technol. 1995, 55, 375–389. [Google Scholar] [CrossRef]
- Han, G.; He, M.; Zhao, F.; Xu, Z.; Zhang, M.; Qin, L. Insulator detection and damage identification based on improved lightweight YOLOv4 network. Energy Rep. 2021, 7, 187–197. [Google Scholar] [CrossRef]
- Akbari, M.; Shayegani-Akmal, A.A. Experimental investigation on the accelerated aging of silicone rubber insulators based on thermal stress. Int. J. Electr. Power Energy Syst. 2023, 149, 109049. [Google Scholar] [CrossRef]
- Kim, T.; Sanyal, S.; Rabelo, M.; Choi, I.-H.; Geun Yoon, Y.; Oh, T.K.; Yi, J. Non-destructive analysis of power insulators by frequency response function and three dimensional-computed tomography. Mech. Syst. Signal Process. 2022, 177, 109310. [Google Scholar] [CrossRef]
- Qiu, Z.; Zhu, X.; Liao, C.; Shi, D.; Qu, W. Detection of Transmission Line Insulator Defects Based on an Improved Lightweight YOLOv4 Model. Appl. Sci. 2022, 12, 1207. [Google Scholar] [CrossRef]
- Prenleloup, A.; Gmür, T.; Botsis, J.; Papailiou, K.O.; Obrist, K. Stress and failure analysis of crimped metal–composite joints used in electrical insulators subjected to bending. Compos. Part A Appl. Sci. Manuf. 2009, 40, 644–652. [Google Scholar] [CrossRef]
- Pahl, R.J.; Shannon, M.A. Design of a cylindrical high-voltage high-temperature vacuum insulator. IEEE Trans. Dielectr. Electr. Insul. 2003, 10, 240–244. [Google Scholar] [CrossRef]
- Seman, L.O.; Stefenon, S.F.; Mariani, V.C.; Coelho, L.d.S. Ensemble learning methods using the Hodrick–Prescott filter for fault forecasting in insulators of the electrical power grids. Int. J. Electr. Power Energy Syst. 2023, 152, 109269. [Google Scholar] [CrossRef]
- Shanmugam, M.; Sivakumar, G.; Arunkumar, A.; Rajaraman, D.; Indhira, M. Fabrication and assessment of reinforced ceramic electrical insulator from bamboo leaf ash waste. J. Alloys Compd. 2020, 824, 153703. [Google Scholar] [CrossRef]
- Lanteigne, J.; Lalonde, S.; De Tourreil, C. Optimization of Stresses in the End-Fittings of Composite Insulators for Distribution and Transmission Lines. J. Reinf. Plast. Compos. 1996, 15, 467–478. [Google Scholar] [CrossRef]
- Ogbonna, V.E.; Popoola, A.P.I.; Popoola, O.M.; Adeosun, S.O. A review on corrosion, mechanical, and electrical properties of glass fiber-reinforced epoxy composites for high-voltage insulator core rod applications: Challenges and recommendations. Polym. Bull. 2022, 79, 6857–6884. [Google Scholar] [CrossRef]
- Ribeiro, A.C.; Soares, B.G.; Furtado, J.G.M.; Silva, A.A.; Couto, N.S.S.E. Superhydrophobic nanocomposite coatings based on different polysiloxane matrices designed for electrical insulators. Prog. Org. Coat. 2022, 168, 106867. [Google Scholar] [CrossRef]
- Shrimathi, H.P.; Mondal, M.; Mishra, P. Simulation based electric stress estimation on silicone rubber polymeric insulators under multi-environmental conditions. Electr. Power Syst. Res. 2023, 214, 108840. [Google Scholar] [CrossRef]
- Ahmed Salem, A.; Abd-rahman, R.; Abdul-Malek, Z.; Yiew Lau, K.; Ashida Salim, N. Controlling electrical stress of polymeric insulators using zinc oxide microvaristor. Mater. Lett. 2023, 352, 135202. [Google Scholar] [CrossRef]
- Ehsani, M.; Borsi, H.; Gockenbach, E.; Morshedian, J.; Bakhshandeh, G.R. An investigation of dynamic mechanical, thermal, and electrical properties of housing materials for outdoor polymeric insulators. Eur. Polym. J. 2004, 40, 2495–2503. [Google Scholar] [CrossRef]
- Pilan, N.; Marcuzzi, D.; Rizzolo, A.; Grando, L.; Gambetta, G.; Rosa, S.D.; Kraemer, V.; Quirmbach, T.; Chitarin, G.; Gobbo, R.; et al. Electrical and structural R&D activities on high voltage dc solid insulator in vacuum. Fusion Eng. Des. 2015, 96–97, 563–567. [Google Scholar] [CrossRef]
- Liu, J.; Hu, M.; Dong, J.; Lu, X. Summary of insulator defect detection based on deep learning. Electr. Power Syst. Res. 2023, 224, 109688. [Google Scholar] [CrossRef]
- Liu, C.; Wu, Y.; Liu, J.; Sun, Z.; Xu, H. Insulator Faults Detection in Aerial Images from High-Voltage Transmission Lines Based on Deep Learning Model. Appl. Sci. 2021, 11, 4647. [Google Scholar] [CrossRef]
- Han, S.W.; Cho, H.G.; Woo, B.C.; Han, D.H.; Lee, D.I.; Choi, I.H.; Shin, T.W. Simulation on interface mechanical stress in porcelain insulators for transmission line by cement growth using ANSYS/NASTRAN program. In Proceedings of the 7th International Conference on Properties and Applications of Dielectric Materials (Cat. No. 03CH37417), Nagoya, Japan, 1–5 June 2003; Volume 411, pp. 411–414. [Google Scholar]
- Tourreil, C.H.d. Response of composite insulators to dynamic mechanical loads. IEEE Trans. Power Deliv. 1990, 5, 379–383. [Google Scholar] [CrossRef]
- Epackachi, S.; Dolatshahi, K.M.; Oliveto, N.D.; Reinhorn, A.M. Mechanical behavior of electrical hollow composite post insulators: Experimental and analytical study. Eng. Struct. 2015, 93, 129–141. [Google Scholar] [CrossRef]
- Kumosa, M.; Armentrout, D.; Kumosa, L.; Han, Y.; Carpenter, S.H. Analyses of composite insulators with crimped end-fittings: Part II—Suitable crimping conditions. Compos. Sci. Technol. 2002, 62, 1209–1221. [Google Scholar] [CrossRef]
- Fallah-Arani, H.; Tehrani, F.S.; Koohani, H.; Elmdoust, B.; Nodoushan, N.J.; Shafiei, Z. Optimization of resin content to improve electrical and mechanical properties of polymer-concrete line-post insulators used in electrical distribution networks. Electr. Power Syst. Res. 2023, 220, 109357. [Google Scholar] [CrossRef]
- Hussain, M.K.; Alshadeedi, B.M. Optimal design of high voltage composite insulators with grading rings in different configurations. Electr. Power Syst. Res. 2023, 221, 109493. [Google Scholar] [CrossRef]
- Kim, T.; Jeon, S.; Lee, Y.J.; Yi, J.; Choi, I.H.; Son, J.A.; Choi, C.W. Three-dimensional computed tomography and composition analysis of porcelain insulators for 154 kV power transmission lines. IEEE Trans. Dielectr. Electr. Insul. 2019, 26, 115–119. [Google Scholar] [CrossRef]
- Rabelo, M.; Kim, T.; Sanyal, S.; Kim, K.; Seok, C.-S.; Choi, I.-H.; Yi, J. The impact of cap orientation on mechanical strength of high voltage devices and a novel design for improvement. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 65. [Google Scholar] [CrossRef]
- Choi, I.-H.; Kim, T.-K.; Yoon, Y.-B.; Kim, T.; Nguyen, H.T.T.; Yi, J. A Study on the Life-Time Assessment Ways and Various Failure Types of 154 kV Porcelain Insulators Installed in South Korea. Trans. Electr. Electron. Mater. 2018, 19, 188–194. [Google Scholar] [CrossRef]
- Moustafa, M.A.; Mosalam, K.M. Finite element modeling and assessment of seismic response of electrical substations porcelain post insulators. Soil Dyn. Earthq. Eng. 2021, 150, 106895. [Google Scholar] [CrossRef]
- Qu, X.; Deng, S.; Ma, Z.; Xiao, Y.; Li, Q.; Dai, Z.; He, G.; Liu, K.; Chen, X.; Wu, Y.; et al. Enhanced bending strength and microwave dielectric properties of Li2MgTi3O8 ceramics by adding Si3N4 reinforcing phase. Ceram. Int. 2022, 48, 29614–29619. [Google Scholar] [CrossRef]
- Zhang, M.; Zhao, L.; Guan, J.; Hao, Y. Fracture model of meso-ceramics based on relative size and prediction of fracture toughness and bending strength. Ceram. Int. 2023, 49, 35135–35144. [Google Scholar] [CrossRef]
- Bogomol, I.; Nishimura, T.; Nesterenko, Y.; Vasylkiv, O.; Sakka, Y.; Loboda, P. The bending strength temperature dependence of the directionally solidified eutectic LaB6–ZrB2 composite. J. Alloys Compd. 2011, 509, 6123–6129. [Google Scholar] [CrossRef]
- Muramoto, M.; Tatami, J.; Iijima, M.; Matsui, K.; Yahagi, T.; Takahashi, T.; Nakano, H.; Ohji, T. Deformation behavior and bending strength of single crystal 8 mol% yttria-stabilized zirconia at microscopic scale. J. Eur. Ceram. Soc. 2024, 44, 1061–1069. [Google Scholar] [CrossRef]
- Kim, H.S.; Kim, M.K.; Kang, S.B.; Ahn, S.H.; Nam, K.W. Bending strength and crack-healing behavior of Al2O3/SiC composites ceramics. Mater. Sci. Eng. A 2008, 483–484, 672–675. [Google Scholar] [CrossRef]
- Romaniv, O.M.; Vasyliv, B.D. Some features of formation of the structural strength of ceramic materials. Mater. Sci. 1998, 34, 149–161. [Google Scholar] [CrossRef]
- Ritchie, R.O. Mechanisms of fatigue-crack propagation in ductile and brittle solids. Int. J. Fract. 1999, 100, 55–83. [Google Scholar] [CrossRef]
- Azevêdo, A.S.d.C.; Ranjbarzadeh, S.; Gioria, R.d.S.; Carlos Nelli Silva, E.; Picelli, R. On the multi-objective perspective of discrete topology optimization in fluid-structure interaction problems. Appl. Math. Model. 2024, 127, 1–17. [Google Scholar] [CrossRef]
- Du, Z.; Guo, Y.; Liu, C.; Zhang, W.; Xue, R.; Guo, Y.; Tang, S.; Guo, X. Structural topology optimization of three-dimensional multi-material composite structures with finite deformation. Compos. Struct. 2024, 328, 117692. [Google Scholar] [CrossRef]
- Alfouneh, M.; Keshtegar, B. STO-DAMV: Sequential topology optimization and dynamical accelerated mean value for reliability-based topology optimization of continuous structures. Comput. Methods Appl. Mech. Eng. 2023, 417, 116429. [Google Scholar] [CrossRef]
- Liu, Y.; Gao, R.; Li, Y.; Fang, D. EMsFEM based concurrent topology optimization method for hierarchical structure with multiple substructures. Comput. Methods Appl. Mech. Eng. 2024, 418, 116549. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, C.; Mei, D.; Li, Y.; Sheng, K.; Wang, J.; Wang, L.; Zhu, S.; Guan, S. Optimized structure design of asymmetrical Mg alloy cerebrovascular stent with high flexibility. Smart Mater. Manuf. 2024, 2, 100040. [Google Scholar] [CrossRef]




| Component Name | Material | Density/(kg/m3) | Poisson Ratio μ | Elastic Modulus/MPa |
|---|---|---|---|---|
| Ball Pin | 45Mn2 | 7800 | 0.269 | 210 × 103 |
| Socket Cap | QT450 | 7860 | 0.3 | 178 × 103 |
| Adhesive | Concrete | 3100 | 0.3 | 40 × 103 |
| Porcelain Shell | Ceramics | 3700 | 0.3 | 60 × 103 |
| Socket Cap | Porcelain Shell | Ball Pin | ||||
|---|---|---|---|---|---|---|
| Y (Max) | X (Max) | Y (Max) | X (Max) | Y (Max) | X (Max) | |
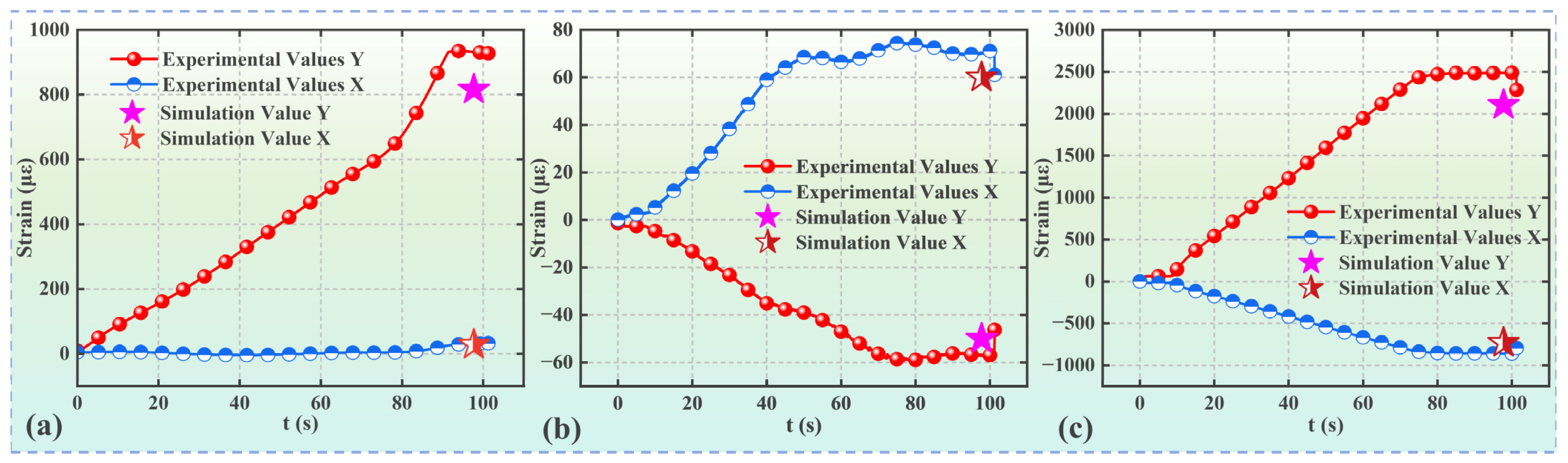
| Experimental Values | 927.8 με | 32.2 με | −56.9 με | 71.96 με | 2490.4 με | −859.8 με |
| Simulation Values | 846.3 με | 28.3 με | −51.3 με | 63.5 με | 2106.3 με | −732.6 με |
| Error | 8.7% | 12.1% | 9.8% | 11.7% | 15.4% | 14.8% |
| Sample Number | 1 | 2 | 3 | 4 | Mean Value |
|---|---|---|---|---|---|
| White glazed ceramics | 2.71 MPa | 2.90 MPa | 3.02 MPa | 3.14 MPa | 2.94 MPa |
| Yellow glazed ceramics | 2.23 MPa | 2.58 MPa | 2.63 MPa | 3.46 MPa | 2.73 MPa |
| Sample Number | 1 | 2 | 3 | 4 | Mean Value |
|---|---|---|---|---|---|
| White glazed ceramics | 105.03 MPa | 104.95 MPa | 92.16 MPa | 99.95 MPa | 100.52 MPa |
| Yellow glazed ceramics | 94.38 MPa | 94.67 MPa | 95.46 MPa | 104.66 MPa | 97.29 MPa |
| Name | Update Order | P1/° | P2/mm | P3/MPa | P4/MPa |
|---|---|---|---|---|---|
| 1 | 4 | 11 | 85 | 63.224 | 52.091 |
| 2 | 2 | 8 | 85 | 66.921 | 55.455 |
| 3 | 6 | 14 | 85 | 68.696 | 53.173 |
| 4 | 3 | 11 | 75 | 72.981 | 61.666 |
| 5 | 7 | 11 | 95 | 77.969 | 56.952 |
| 6 | 1 | 8 | 75 | 72.576 | 60.827 |
| 7 | 5 | 14 | 75 | 71.715 | 60.021 |
| 8 | 8 | 8 | 95 | 60.547 | 48.078 |
| 9 | 9 | 14 | 95 | 103.78 | 74.333 |
| 1 | Name | P3 | P4 |
|---|---|---|---|
| 2 | Goodness of Fit | ||
| 3 | Coefficient of Determination (Best Value = 1) |  1 1 |  1 1 |
| 4 | Maximum Relative Residual (Best Value = 0%) |  0 0 |  0 0 |
| 5 | Root Mean Square Error (Best Value = 0) | 1.45 × 10−7 | 9.24 × 10−8 |
| 6 | Relative Root Mean Square Error (Best Value = 0%) |  0 0 |  0 0 |
| 7 | Relative Maximum Absolute Error (Best Value = 0%) |  0 0 |  0 0 |
| 8 | Relative Average Absolute Error (Best Value = 0%) |  0 0 |  0 0 |
| Name | P1 | P2 | P3/MPa | P4/MPa | ||
|---|---|---|---|---|---|---|
| Parameter Value | Variation from Reference | Parameter Value | Variation from Reference | |||
| Candidate Point1 | 8 | 92.6 |  59.1 59.1 | −1.27% |  48.3 48.3 | −0.05% |
| Point1 (verified) |  68.7 68.7 | 14.82% |  57.3 57.3 | 18.53% | ||
| Candidate Point2 | 8 | 90.6 |  59.8 59.8 | 0.00% |  48.3 48.3 | 0.00% |
| Point2 (verified) |  62.0 62.0 | 3.64% |  49.5 49.5 | 2.41% | ||
| Candidate Point3 | 8.81 | 88.9 |  63.1 63.1 | 5.37% |  49.4 49.4 | 2.31% |
| Point3 (verified) |  62.4 62.4 | 3.88% |  49.9 49.9 | 3.18% | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, Y.; Li, Z.; Cao, P.; Li, X.; Wang, H.; Jiang, X.; Tian, L.; Zhang, T.; Jiang, H. Study on the Ultimate Load Failure Mechanism and Structural Optimization Design of Insulators. Materials 2024, 17, 351. https://doi.org/10.3390/ma17020351
Ji Y, Li Z, Cao P, Li X, Wang H, Jiang X, Tian L, Zhang T, Jiang H. Study on the Ultimate Load Failure Mechanism and Structural Optimization Design of Insulators. Materials. 2024; 17(2):351. https://doi.org/10.3390/ma17020351
Chicago/Turabian StyleJi, Yongchao, Zhuo Li, Peng Cao, Xinyu Li, Haoyu Wang, Xiaorui Jiang, Limin Tian, Tao Zhang, and Hao Jiang. 2024. "Study on the Ultimate Load Failure Mechanism and Structural Optimization Design of Insulators" Materials 17, no. 2: 351. https://doi.org/10.3390/ma17020351
APA StyleJi, Y., Li, Z., Cao, P., Li, X., Wang, H., Jiang, X., Tian, L., Zhang, T., & Jiang, H. (2024). Study on the Ultimate Load Failure Mechanism and Structural Optimization Design of Insulators. Materials, 17(2), 351. https://doi.org/10.3390/ma17020351






