Influences of Flood Conditions on Dynamic Characteristics of Novel 3D-Printed Porous Bridge Bearings
Abstract
1. Introduction
2. Novel 3D Printed Porous Bridge Bearings (3DPPBBs)
2.1. Design of a Novel Porous Bridge Bearing Model
2.2. Materials
2.3. The Preparation of the 3D-Printed Porous Bridge Bearing under Flood Conditions
3. Methods
3.1. Determination of Dynamic Modal Parameters of the 3DPPBB System Using an Impact Hammer
3.2. Simulating Floods in Novel Porous Bridge Bearing
3.3. Single-Degree-of-Freedom (SDOF) System
3.4. Impulse Response Function
4. Results
4.1. Experimental Measurements
4.2. The Impulse Forces
4.3. The Change of Acceleration
4.4. The Change of Frequency Response Function (FRF)
4.5. The Change of Natural Frequency
4.6. The Change of Dynamic Stiffness
4.7. The Change of Damping Ratio
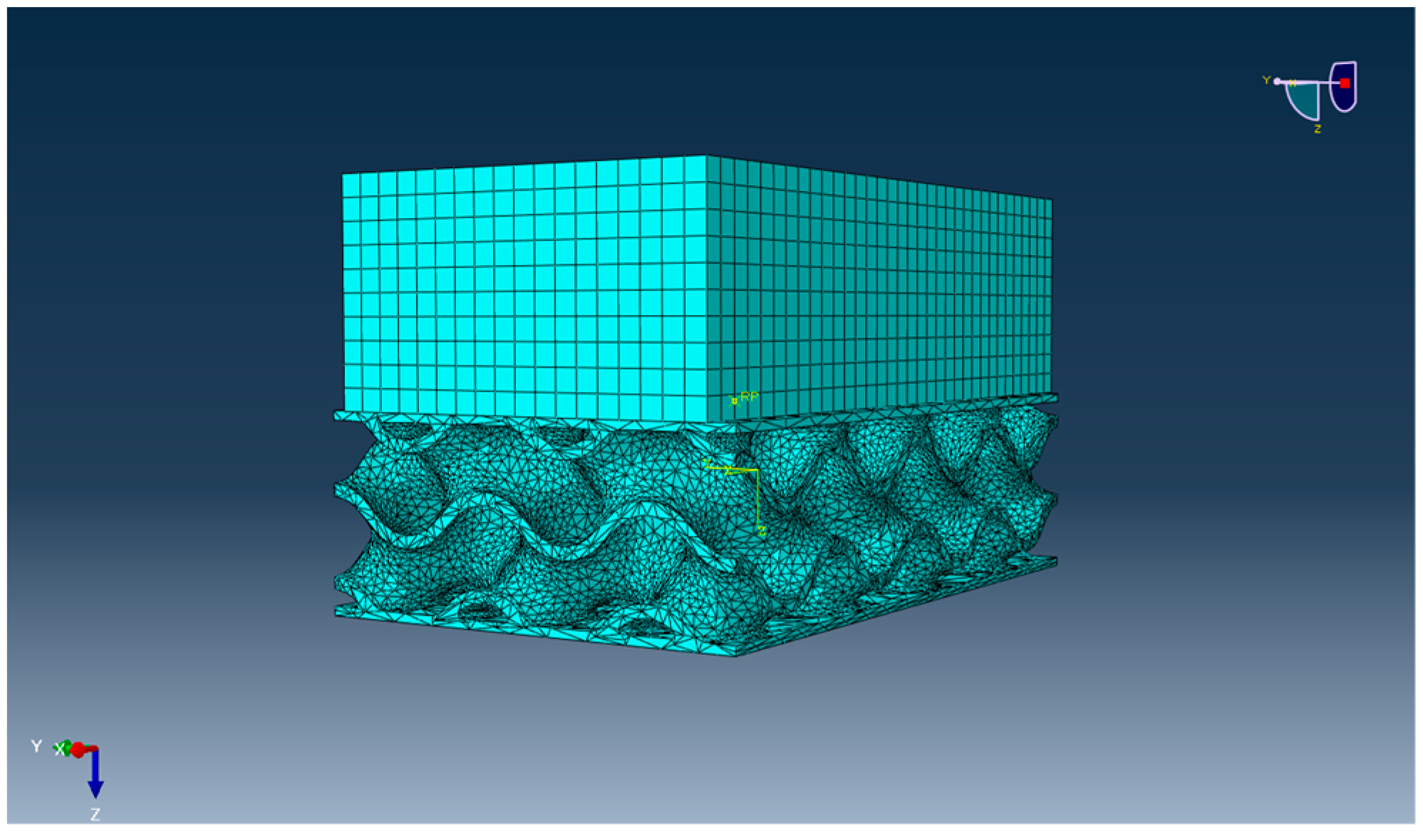
4.8. Finite Element Modelling of the Proposed Bridge Bearing
4.9. Experimental Justification
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sengsri, P.; Kaewunruen, S. Additive manufacturing meta-functional composites for engineered bridge bearings: A review. Constr. Build. Mater. 2020, 262, 120535. [Google Scholar] [CrossRef]
- Paul, A. Types & Functions Of Bearings for Bridges—CivilDigital. 2013. Available online: https://civildigital.com/types-functions-bearings-bridges (accessed on 15 May 2022).
- Krzysztofik, A. Elastomeric Bearing Pad and Its Role in Bridge Design, SimScale. 2021. Available online: https://www.simscale.com/blog/2015/07/elastomeric-bearing-pads-and-their-role-in-bridge-safety (accessed on 16 May 2022).
- Ashkezari, G.; Aghakouchak, A.; Kokabi, M. Design, manufacturing and evaluation of the performance of steel like fiber reinforced elastomeric seismic isolators. J. Mater. Process. Technol. 2008, 197, 140–150. [Google Scholar] [CrossRef]
- Stanton, J.; Roeder, C. Elastomeric Bearings Design, Construction, and Materials; Transportation Research Board: WA, USA, 1982. [Google Scholar]
- Gent, A.N.; Lindley, P.B. Internal rupture of bonded rubber cylinders in tension. Proc. R. Soc. London. Ser. A. Math. Phys. Sci. 1959, 249, 195–205. [Google Scholar] [CrossRef]
- Gent, A.N. Cavitation in Rubber: A Cautionary Tale. Rubber Chem. Technol. 1990, 63, 49–53. [Google Scholar] [CrossRef]
- Pond, T.J. Cavitation in bonded natural rubber cylinders repeatedly loaded in tension. J. Nat. Rubber Res. 1995, 10, 14–25. [Google Scholar]
- Dorfmann, A.; Burtscher, S.L. Aspects of Cavitation Damage in Seismic Bearings. J. Struct. Eng. 2000, 126, 573–579. [Google Scholar] [CrossRef]
- Sengsri, P.; Marsico, R.M.; Kaewunruen, S. Base isolation fibre-reinforced composite bearings using recycled rubber. IOP Conf. Ser. Mater. Sci. Eng. 2019, 603, 02206. [Google Scholar] [CrossRef]
- Sengsri, P.; Baniotopoulos, C.; Kaewunruen, S. Engineered model for the numerical investigation into vibration characteristics of a novel bridge bearing under free-free and fixed boundary condition. In Proceedings of the EURODYN 2020 XI International Conference on Structural Dynamics: EURODYN 2020. European Association for Structural Dynamics, Athens, Greece, 23 November 2020. [Google Scholar]
- Maskery, I.; Sturm, L.; Aremu, A.; Panesar, A.; Williams, C.; Tuck, C.; Wildman, R.; Ashcroft, I.; Hague, R. Insights into the mechanical properties of several triply periodic minimal surface lattice structures made by polymer additive manufacturing. Polymer 2018, 152, 62–71. [Google Scholar] [CrossRef]
- Abueidda, D.; Bakir, M.; Abu Al-Rub, R.; Bergström, J.; Sobh, N.; Jasiuk, I. Mechanical properties of 3D printed polymeric cellular materials with triply periodic minimal surface architectures. Mater. Des. 2017, 122, 255–267. [Google Scholar] [CrossRef]
- DeValk, T.; Lakes, R. Poisson’s Ratio and Modulus of Gyroid Lattices. Phys. Status Solidi (B) 2021, 258, 2100081. [Google Scholar] [CrossRef]
- Corkery, R.; Tyrode, E. On the colour of wing scales in butterflies: Iridescence and preferred orientation of single gyroid photonic crystals. Interface Focus 2017, 7, 20160154. [Google Scholar] [CrossRef]
- Abueidda, D.; Elhebeary, M.; Shiang, C.; Pang, S.; Abu Al-Rub, R.; Jasiuk, I. Mechanical properties of 3D printed polymeric Gyroid cellular structures: Experimental and finite element study. Mater. Des. 2019, 165, 107597. [Google Scholar] [CrossRef]
- Dolan, J.; Wilts, B.; Vignolini, S.; Baumberg, J.; Steiner, U.; Wilkinson, T. Optical Properties of Gyroid Structured Materials: From Photonic Crystals to Metamaterials. Adv. Opt. Mater. 2014, 3, 12–32. [Google Scholar] [CrossRef]
- Ko, Y.Y.; Chiou, J.S.; Tsai, Y.C.; Chen, C.H.; Wang, H.; Wang, C.Y. Evaluation of Flood-Resistant Capacity of Scoured Bridges. J. Perform. Constr. Facil. 2014, 28, 61–75. [Google Scholar] [CrossRef]
- Huang, H.B.; Yi, T.H.; Li, H.N.; Liu, H. Sparse Bayesian Identification of Temperature-Displacement Model for Performance Assessment and Early Warning of Bridge Bearings. J. Struct. Eng. 2022, 148, 04022052. [Google Scholar] [CrossRef]
- Sengsri, P.; Kaewunruen, S. Local Failure Modes and Critical Buckling Loads of a Meta-Functional Auxetic Sandwich Core for Composite Bridge Bearing Applications. Appl. Sci. 2021, 11, 10844. [Google Scholar] [CrossRef]
- Khosravani, M.R.; Berto, F.; Ayatollahi, M.R.; Reinicke, T. Characterization of 3D-printed PLA parts with different raster orientations and printing speeds. Sci. Rep. 2022, 12, 1016. [Google Scholar] [CrossRef]
- Fonseca, I.A.; Ferreira, M.F.S.F.; de Moura, M.; Machado, J.L. Alves, Study of the interlaminar fracture under mode I loading on FFF printed parts. Compos. Struct. 2019, 214, 316–324. [Google Scholar] [CrossRef]
- Sengsri, P.; Fu, H.; Kaewunruen, S. Mechanical Properties and Energy-Absorption Capability of a 3D-Printed TPMS Sandwich Lattice Model for Meta-Functional Composite Bridge Bearing Applications. J. Compos. Sci. 2022, 6, 71. [Google Scholar] [CrossRef]
- Sengsri, P.; Kaewunruen, S. Compression behaviour of an extremely lightweight structure with a gyroid core used for bridge bearings. In Proceedings of the International Conference on Advances in Construction Materials and Structures (ICCMS 2021), Virtual Mode, 15–17 December 2021. [Google Scholar]
- Sengsri, P.; Kaewunruen, S. Investigations into Nonlinear Effects of Normal Pressures on Dynamic Cyclic Responses of Novel 3D-Printed TPMS Bridge Bearings. Vibration 2023, 6, 65–81. [Google Scholar] [CrossRef]
- Ashby, M.F.; Medalist, R.M. The mechanical properties of cellular solids. Metall. Trans. 1983, 14, 1755–1769. [Google Scholar] [CrossRef]
- Kaewunruen, S.; Tang, T. Idealisations of Dynamic Modelling for Railway Ballast in Flood Conditions. Appl. Sci. 2019, 9, 1785. [Google Scholar] [CrossRef]
- Sengsri, P.; Ngamkhanong, C.; Melo, A.L.O.; Kaewunruen, S. Experimental and Numerical Investigations into Dynamic Modal Parameters of Fiber-Reinforced Foamed Urethane Composite Beams in Railway Switches and Crossings. Vibration 2020, 3, 174–188. [Google Scholar] [CrossRef]
- Standard Drawings for Highway Design and Construction 2015 Revision, Thai Version. BP-102. Available online: http://www.doh.go.th/uploads/tinymce/service/bid/doc_bid/STANDARD%20DRAWING%202015%20EDITION_2018.pdf (accessed on 28 May 2022).
- Wang, Q.A.; Dai, Y.; Ma, Z.G.; Ni, Y.Q.; Tang, J.Q.; Xu, X.Q.; Wu, Z.Y. Towards probabilistic data-driven damage detection in SHM using sparse Bayesian learning scheme. Struct. Control. Health Monit. 2022, 29, e3070. [Google Scholar] [CrossRef]
- Habib, S. What Is TPU Material, How Is TPU Plastic Made, TPU Filament Properties & Applications Types of TPU Films. PlasticRanger. 2022. Available online: https://plasticranger.com/what-is-tpu-material (accessed on 23 May 2022).
- Benedict, O. What Is TPU Material in 3D printing: Material properties, applications, and technologies. Wevolver. 2021. Available online: https://www.wevolver.com/article/tpu-3d-printing-material-properties-applications-and-technologies (accessed on 30 May 2022).
- Ultimaker TPU 95A Material: 3D Print Durable and Flexible Parts. Available online: https://ultimaker.com/materials/tpu-95a (accessed on 12 May 2022).
- TPU Carbon Fibre, Filament2Print. Available online: https://filament2print.com/gb/flexible-tpe-tpu/1411-tpu-carbon-fibre-smartfil.html (accessed on 12 May 2022).
- FFU. Synthetic Wood Railway Sleepers. Available online: https://www.sekisui-rail.com/en/technology.html (accessed on 1 April 2019).
- Remennikov, A.; Kaewunruen, S. Determination of Dynamic properties of rail pads using an instrumented hammer impact technique. Acoust. Aust. 2005, 33, 63–67. [Google Scholar]
- Gavin, H.P. Vibrations of Single Degree of Freedom Systems; Class Note CEE201; Department of Civil and Environmental Engineering, Duke University: Dehan, NC, USA, 2014. [Google Scholar]
- Chopra, A.K.; Chintanapakdee, C. Inelastic deformation ratios for design and evaluation of structures: Single-degree-of-freedom bilinear systems. J. Struct. Eng. 2004, 130, 1309–1319. [Google Scholar] [CrossRef]
- Kaewunruen, S.; Remennikov, A.M. An Alternative Rail Pad Tester for Measuring Dynamic Properties of Rail Pads Under Large Preloads. Exp Mech. 2008, 48, 55–64. [Google Scholar] [CrossRef]
- Kaewunruen, S.; Remennikov, A.M. Field trials for dynamic characteristics of railway track and its components using impact excitation technique. NDT E Int. 2007, 40, 510–519. [Google Scholar] [CrossRef]
- Li, Y.; Li, H.; Zhang, G.; Kaewunruen, S. Nonlinear responses of longitudinally coupled slab tracks exposed to extreme heat waves. Eng. Struct. 2023, 281, 115789. [Google Scholar] [CrossRef]



















| Thick Steel Plate | Bridge Bearing | |
|---|---|---|
| Material | Stainless Steel 304 | TPU 95A |
| Mass (kg) | 6 | 0.1 |
| Size (mm3) | 160 × 100 × 45 | 160 × 100 × 45 |
| Tensile modulus (MPa) | 190 | 26 |
| Tensile stress at yield (MPa) | 205 | 8.6 |
| Specific gravity | 7.9 | 1.22 |
| Method | Natural Frequency (Hz) | Error (%) |
|---|---|---|
| Experiment measurement | 65.00 | - |
| Numerical prediction | 65.10 | 0.15 |
| Types of Test | Water Level | Natural Frequency | Damping Ratio | Damped Natural Frequency | Stiffness |
|---|---|---|---|---|---|
| (%) | (Hz) | - | (Hz) | (kN/m) | |
| 0 | 53.53 | 0.204 | 52.40 | 216.02 | |
| Thick Steel Plate | 25 | 51.89 | 0.204 | 50.80 | 203.02 |
| on 3DPPBB | 50 | 51.99 | 0.204 | 50.90 | 203.82 |
| for test 1 | 75 | 51.77 | 0.211 | 50.60 | 202.04 |
| 100 | 51.57 | 0.212 | 50.40 | 200.54 | |
| 0 | 53.40 | 0.193 | 52.40 | 215.04 | |
| Thick Steel Plate | 25 | 52.72 | 0.205 | 51.60 | 209.56 |
| on 3DPPBB | 50 | 52.40 | 0.204 | 51.30 | 207.04 |
| for test 2 | 75 | 52.17 | 0.211 | 51.00 | 205.25 |
| 100 | 51.26 | 0.220 | 50.00 | 198.08 | |
| 0 | 53.46 | 0.198 | 52.40 | 215.47 | |
| Thick Steel Plate | 25 | 52.82 | 0.205 | 51.70 | 210.37 |
| on 3DPPBB | 50 | 52.27 | 0.201 | 51.20 | 205.97 |
| for test 3 | 75 | 52.67 | 0.210 | 51.50 | 209.20 |
| 100 | 51.64 | 0.218 | 50.40 | 201.08 | |
| 0 | 53.46 | 0.198 | 52.40 | 215.47 | |
| Thick Steel Plate | 25 | 52.48 | 0.205 | 51.37 | 207.70 |
| on 3DPPBB | 50 | 52.22 | 0.203 | 51.13 | 205.58 |
| for average test | 75 | 52.21 | 0.211 | 51.03 | 205.49 |
| 100 | 51.49 | 0.217 | 50.27 | 199.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sengsri, P.; Kaewunruen, S. Influences of Flood Conditions on Dynamic Characteristics of Novel 3D-Printed Porous Bridge Bearings. Materials 2023, 16, 2288. https://doi.org/10.3390/ma16062288
Sengsri P, Kaewunruen S. Influences of Flood Conditions on Dynamic Characteristics of Novel 3D-Printed Porous Bridge Bearings. Materials. 2023; 16(6):2288. https://doi.org/10.3390/ma16062288
Chicago/Turabian StyleSengsri, Pasakorn, and Sakdirat Kaewunruen. 2023. "Influences of Flood Conditions on Dynamic Characteristics of Novel 3D-Printed Porous Bridge Bearings" Materials 16, no. 6: 2288. https://doi.org/10.3390/ma16062288
APA StyleSengsri, P., & Kaewunruen, S. (2023). Influences of Flood Conditions on Dynamic Characteristics of Novel 3D-Printed Porous Bridge Bearings. Materials, 16(6), 2288. https://doi.org/10.3390/ma16062288







