A Phase-Field Study of Spinodal Decomposition Impeded by Irradiation in U-Mo and U-Mo-Zr Alloys
Abstract
:1. Introduction
2. Model and Method
2.1. Phase-Field Model of U-Mo and U-Mo-Zr Alloy System
2.2. Elastic Energy Model
2.3. Numerical Methods
| Parameter | Value | Reference |
|---|---|---|
| Gradient energy coefficient (mol·m2·J−1) | (In U-Mo) (In U-Mo-Zr) | [69] |
| Gas constant (J·mol−1·K−1) | This work | |
| Grid length (m) | (in U-Mo) (in U-Mo-Zr) | [64,66,67] |
| Intrinsic diffusion coefficient of U (m2·s−1) | [50] | |
| Intrinsic diffusion coefficient of Mo (m2·s−1) | [50] | |
| Intrinsic diffusion coefficient of Zr (m2·s−1) | [51] | |
| Lattice constant of γ-U (m) | [66] | |
| Molar volume of γ-U (m3·mol−1) | [66] | |
| Lattice constant of γ-Mo (m) | [64] | |
| Molar volume of γ-Mo (m3·mol−1) | [64] | |
| Lattice constant of β-Zr (m) | [67] | |
| Molar volume of β-Zr (m3·mol−1) | [67] | |
| Elastic constants of γ-U (GPa) | [66] | |
| Elastic constants of γ-Mo (GPa) | [68] | |
| Elastic constants of β-Zr (GPa) | [65] | |
| Replacements per displacement | [58,70] | |
| Average relocation distance (m) | (in U-Mo) (in U-Mo-Zr) | [64,66,67] |
| Time steps (s) | This work |
3. Results
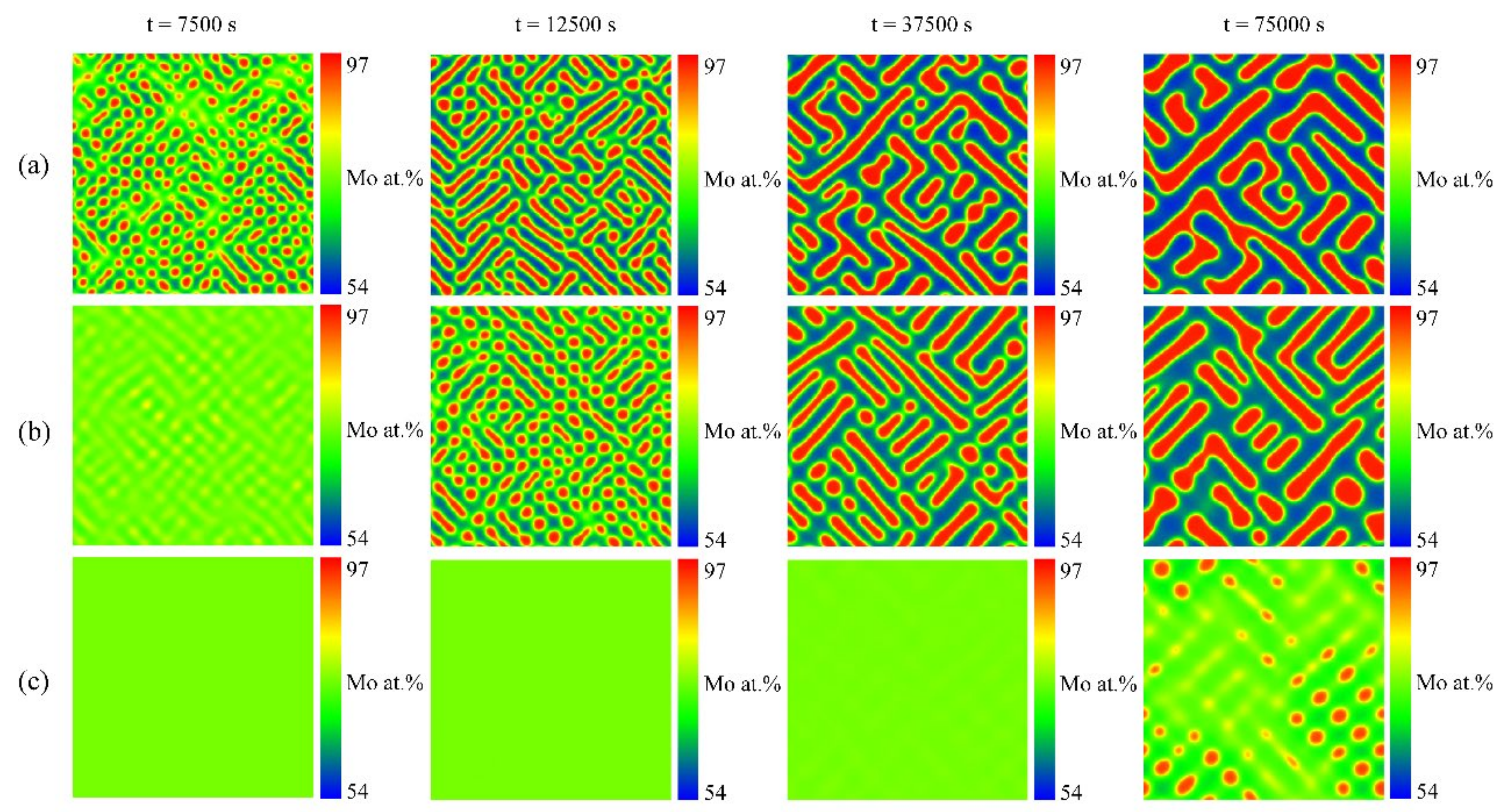
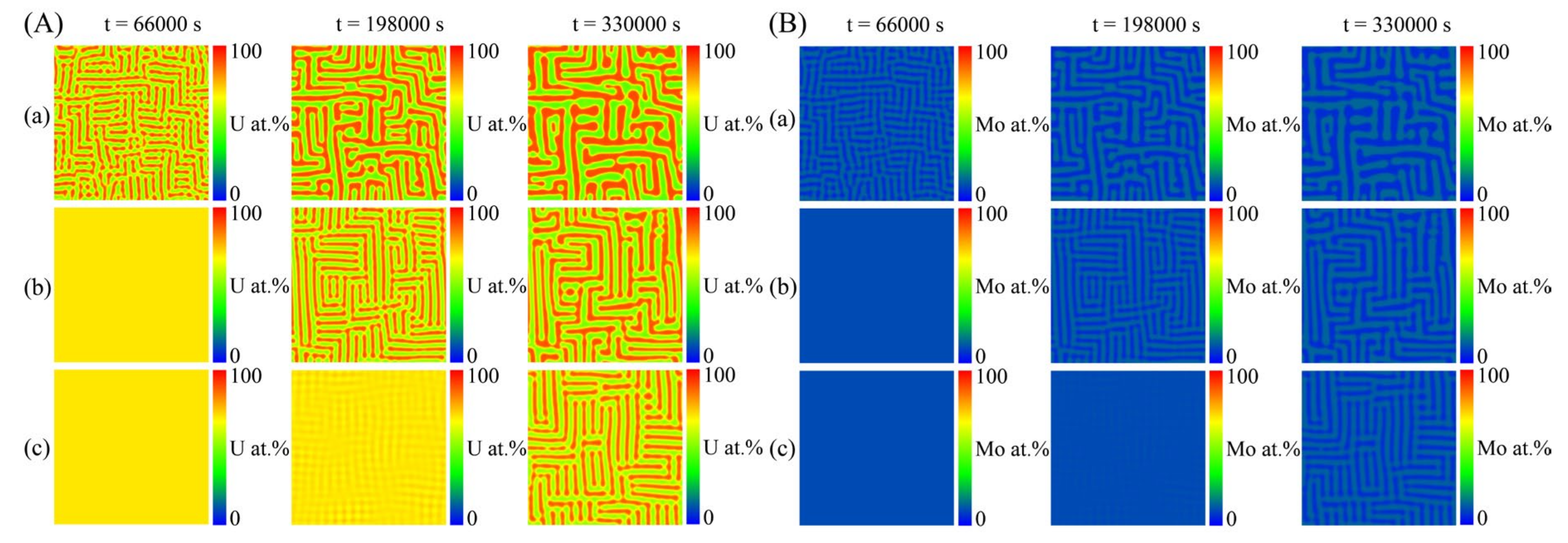
3.1. Simulation of Spinodal Decomposition in U-Mo and U-Mo-Zr Alloys
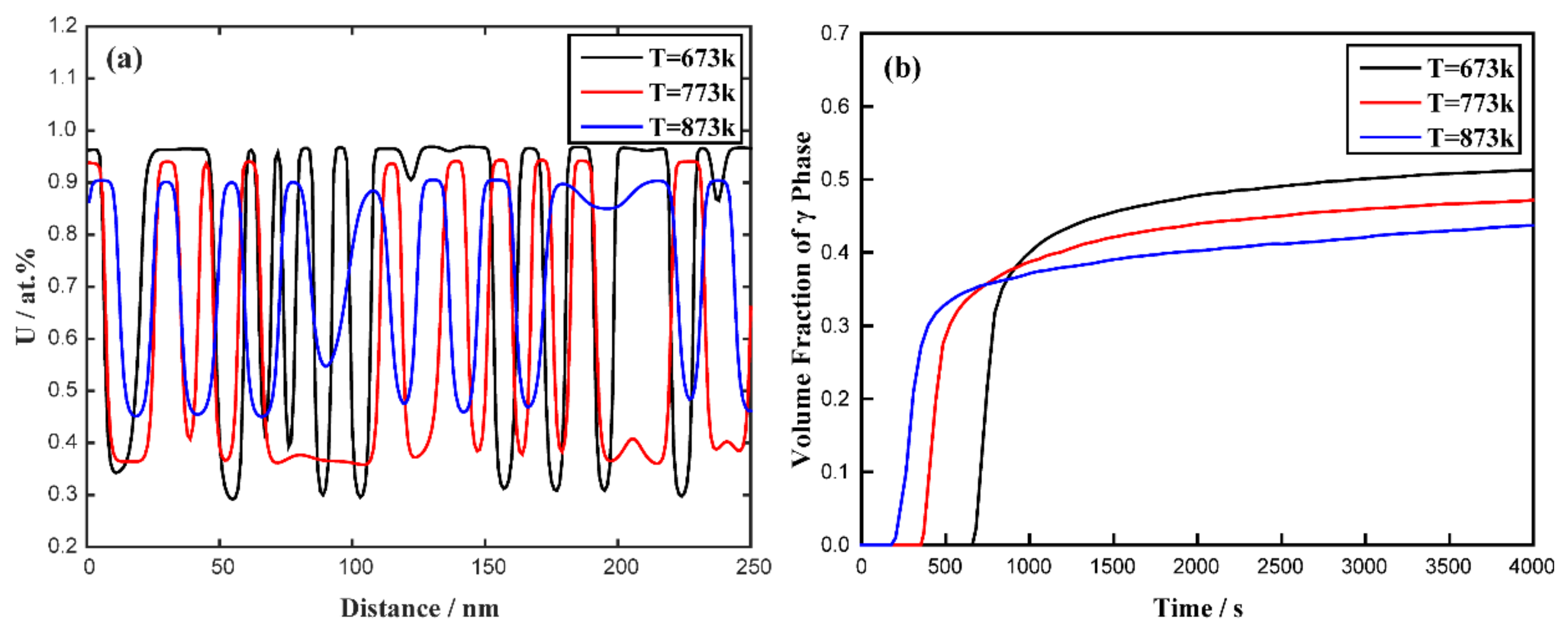
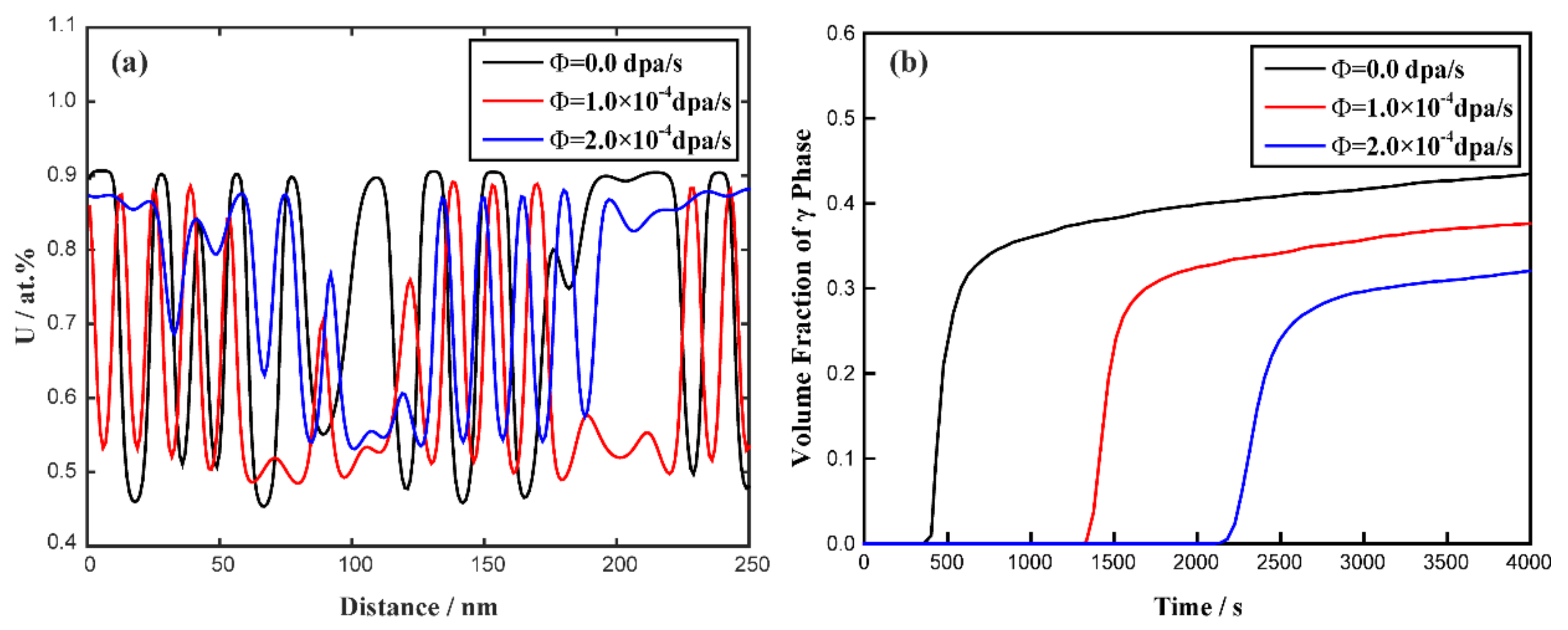
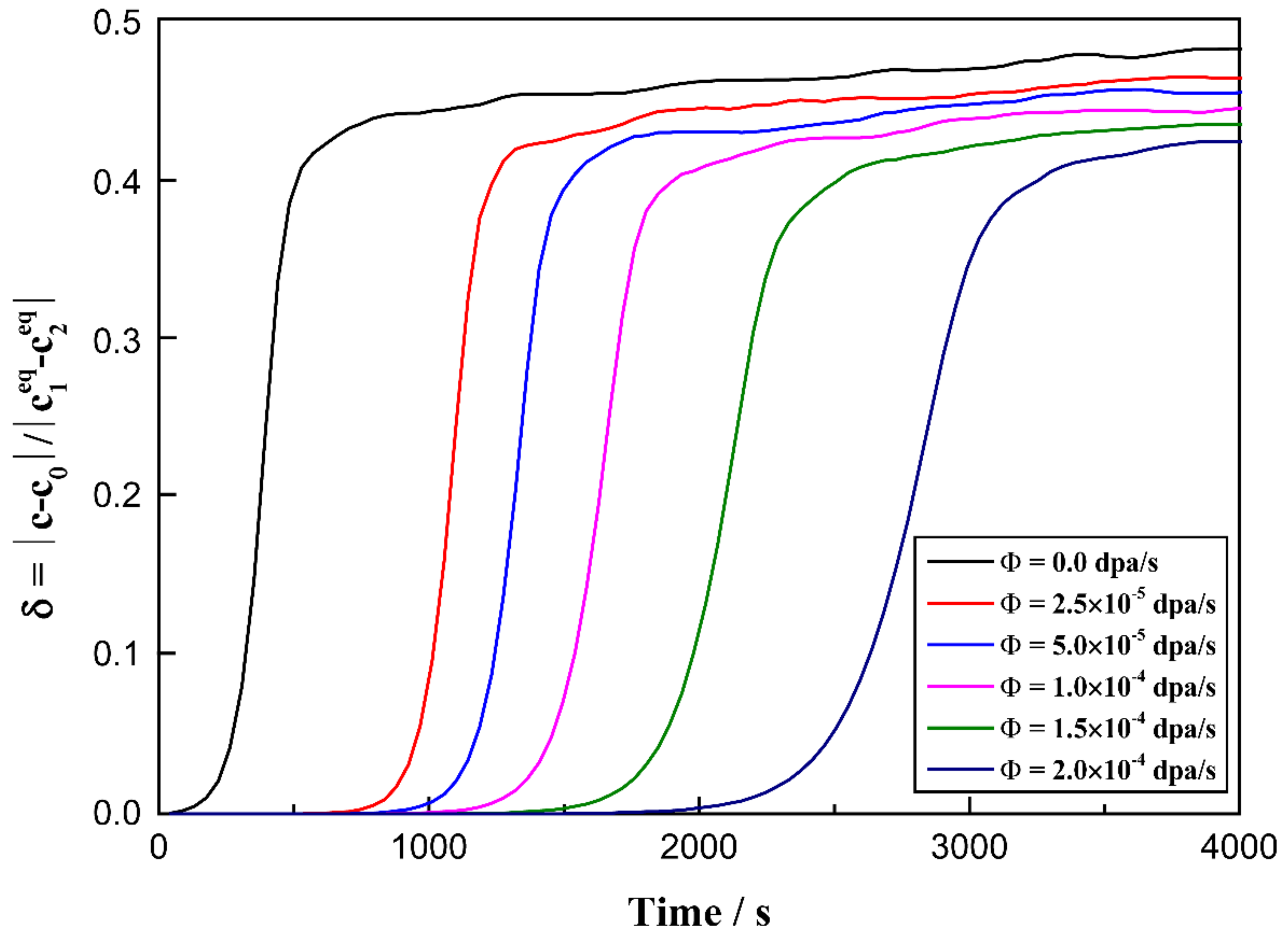
3.2. The Effect of Radiation on Spinodal Decomposition of U-Mo and U-Mo-Zr Alloys
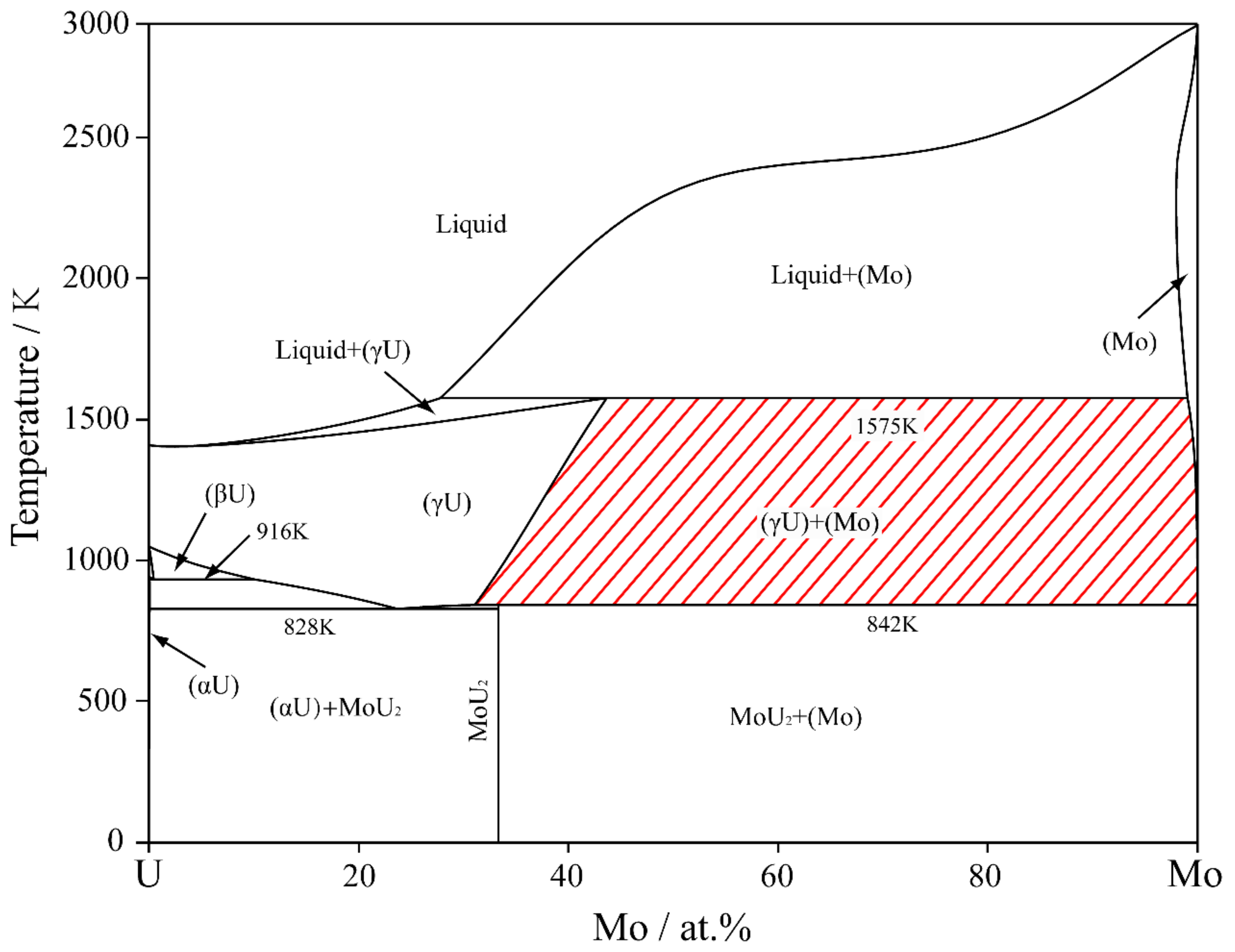
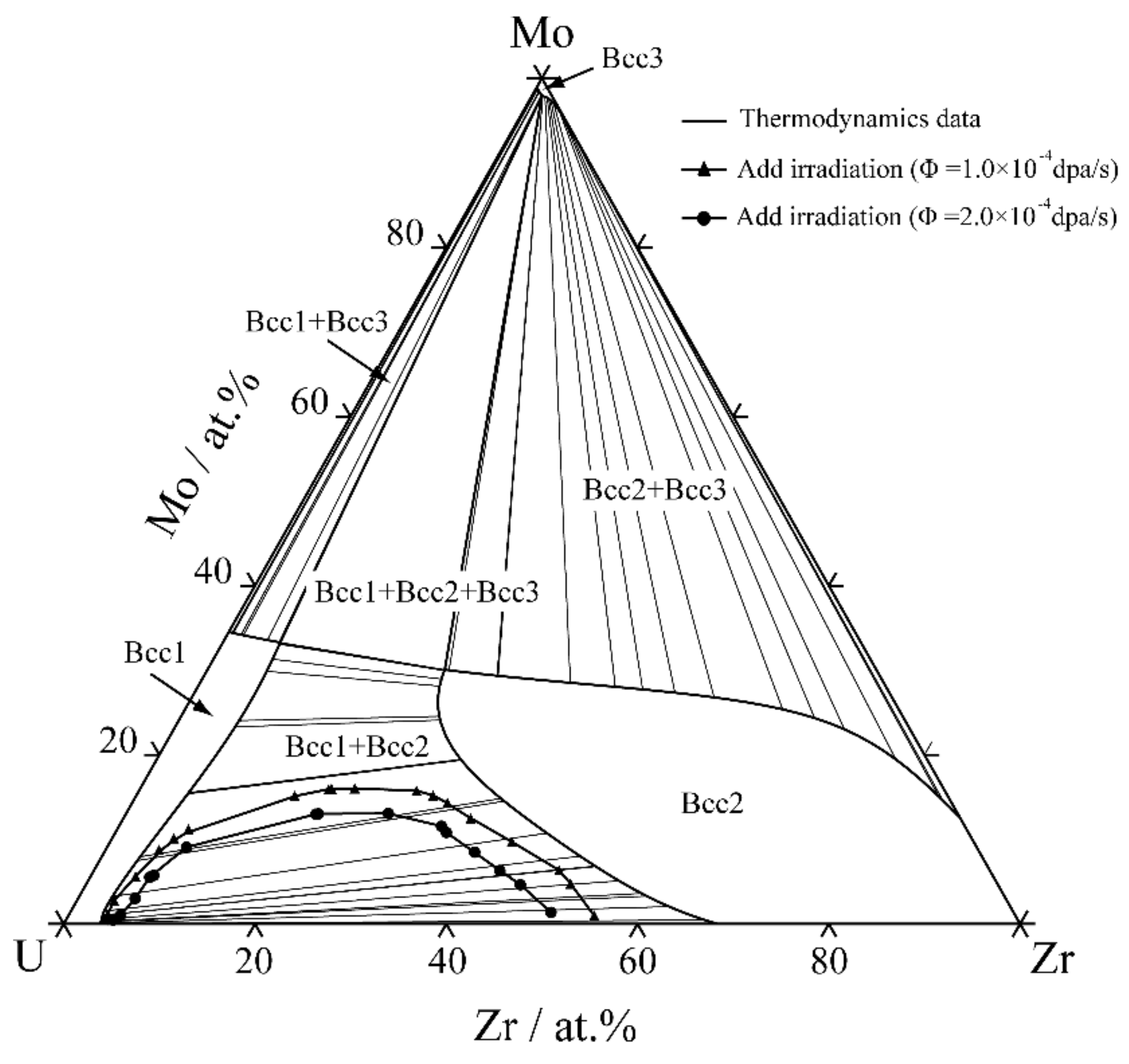
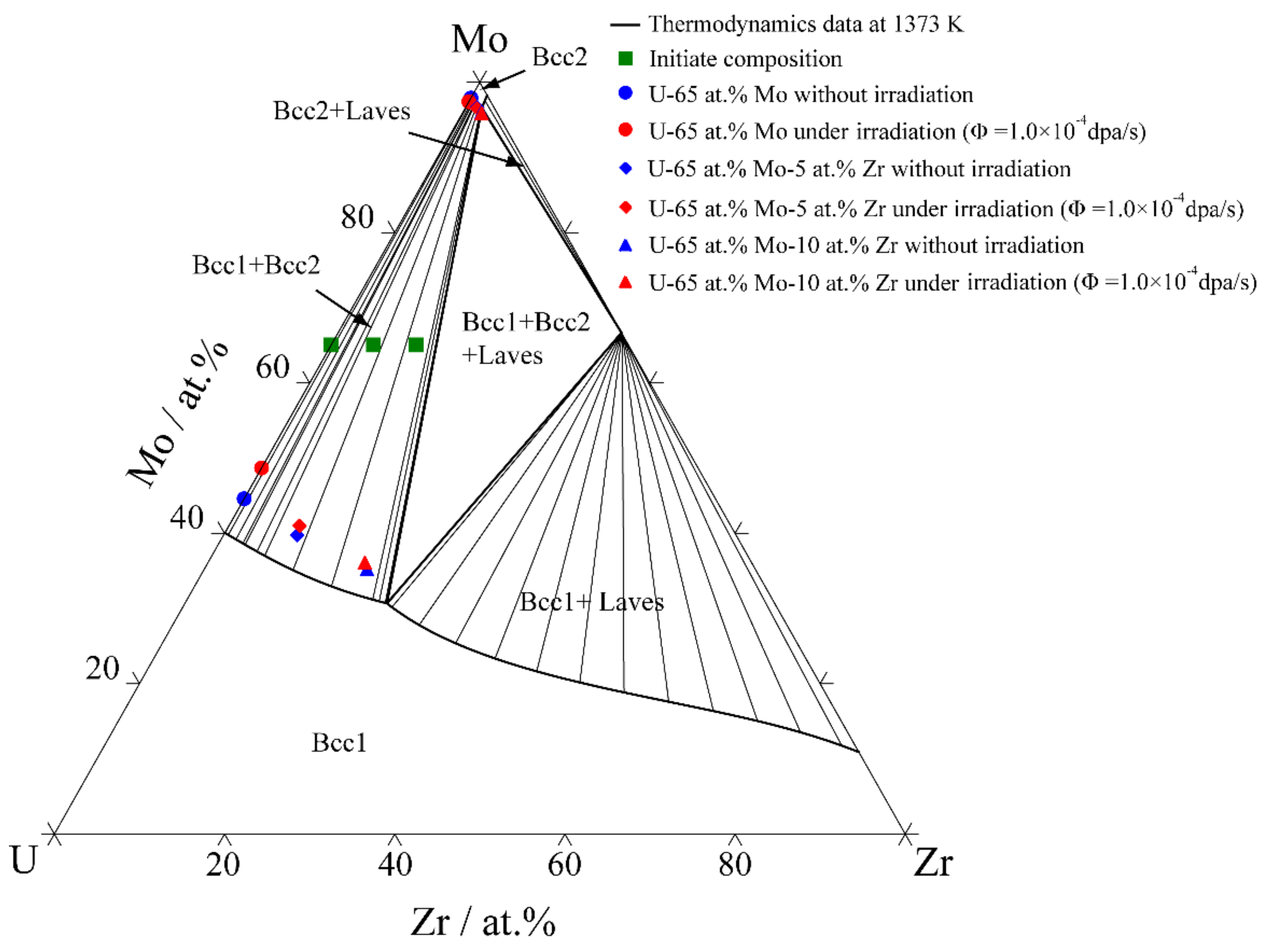
3.3. The Effect of Radiation on Phase Diagrams in U-Mo and U-Mo-Zr Alloys
4. Discussion
5. Conclusions
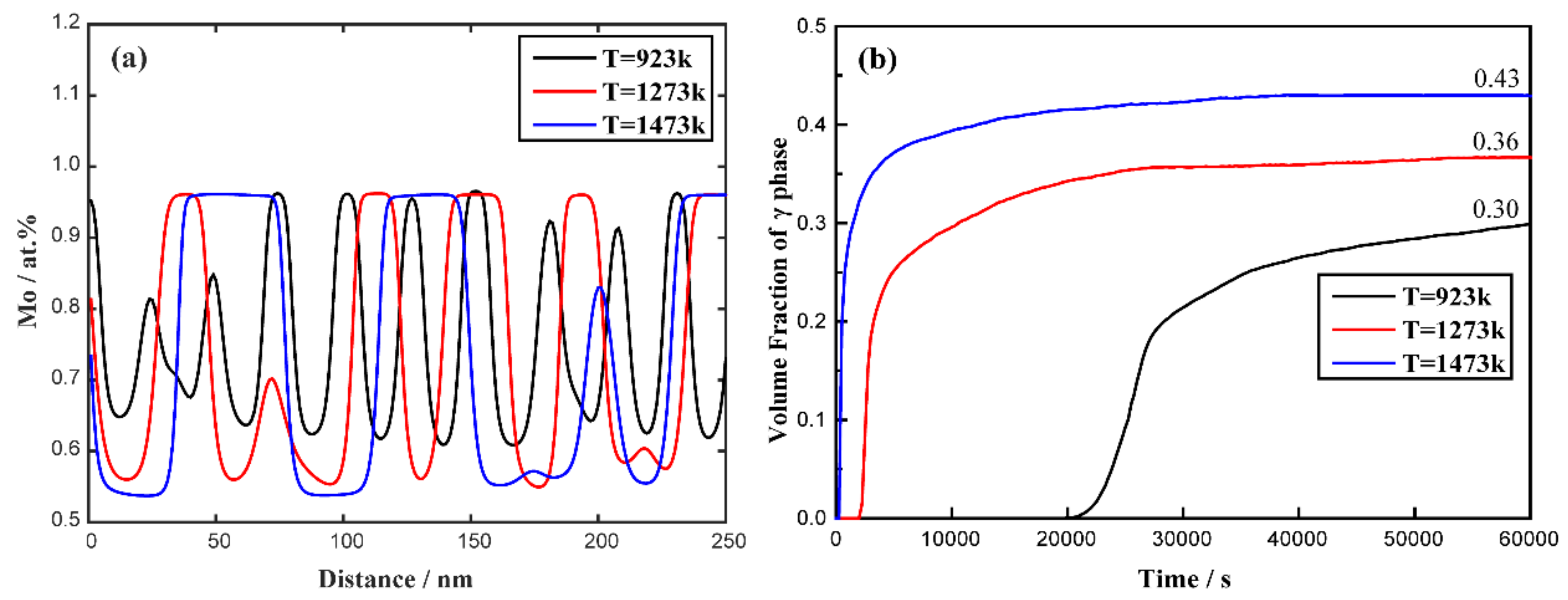
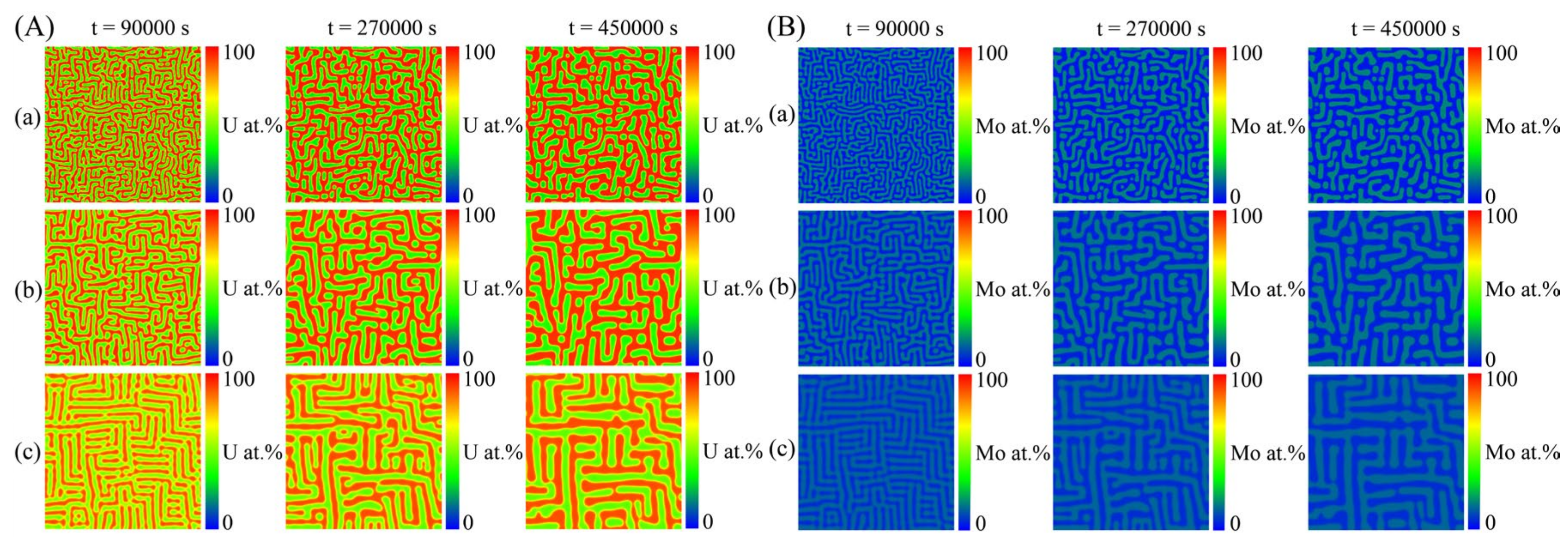
- As the temperature increases, the amplitude of component fluctuations and the volume fraction of the Mo-enriched γ-phase in the U-Mo binary alloy gradually increase after spinodal decomposition, and the morphology is in interconnected plane-like shapes. In the U-Mo-Zr ternary alloy, as the temperature increases, the microstructure obtained through spinodal decomposition is in interconnected maze-like shapes. Due to the decrease in the range of the miscibility gap with increasing temperature, the amplitude of component fluctuations and the volume fraction of the U-enriched γ-phase decrease.
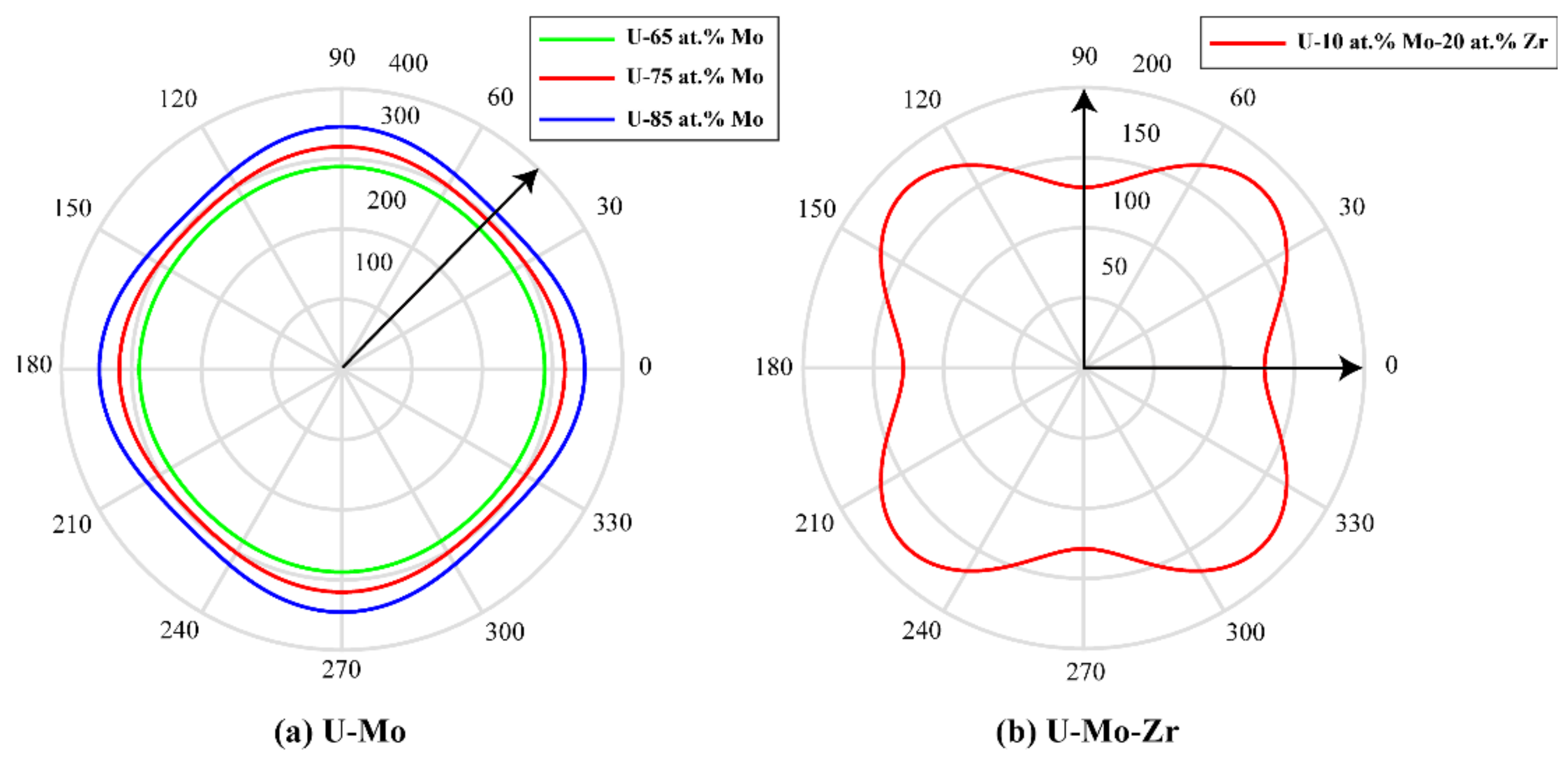
- Under the effect of elastic energy, the γ-phase in the U-75 at.% Mo alloy evolves with a preferential alignment along the <11> crystallographic orientation and the morphology is in interconnected plane-like shapes, while the orientation of the microstructure in the U-10 at.% Mo-20 at.% Zr alloy grows along the <10> and <01> directions, and the morphology is in interconnected maze-like shapes. Different irradiation intensities have little effect on the microstructure and morphology of U-Mo and U-Mo-Zr alloys after spinodal decomposition. As the irradiation intensity increases, the spinodal decomposition rate of U-Mo alloys and U-Mo Zr alloys decreases; the size and volume fraction of the γ-phase decrease; and the range of the miscibility gap after spinodal decomposition also decreases.
- Under irradiation conditions, the cascade mixing effect induced by irradiation “remixes” local atoms, suppressing their diffusion, contrary to the mechanism of spinodal decomposition. Therefore, the spinodal decomposition of U-Mo and U-Mo-Zr alloys under irradiation is the result of competition between thermodynamic spontaneous spinodal decomposition and irradiation-induced cascade mixing.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, W.; Shirvan, K. Multiphysics phase-field modeling of quasi-static cracking in urania ceramic nuclear fuel. Ceram. Int. 2021, 47, 793–810. [Google Scholar] [CrossRef]
- Sinha, V.P.; Hegde, P.V.; Prasad, G.J.; Dey, G.K.; Kamath, H.S. Phase transformation of metastable cubic γ-phase in U–Mo alloys. J. Alloys Compd. 2010, 506, 253–262. [Google Scholar] [CrossRef]
- Burkes, D.E.; Fielding, R.S.; Porter, D.L.; Crawford, D.C.; Meyer, M.K. A US perspective on fast reactor fuel fabrication technology and experience part I: Metal fuels and assembly design. J. Nucl. Mater. 2009, 389, 458–469. [Google Scholar] [CrossRef]
- Neogy, S.; Saify, M.T.; Jha, S.K.; Srivastava, D.; Hussain, M.M.; Dey, G.K.; Singh, R.P. Microstructural study of gamma phase stability in U-9 wt.% Mo alloy. J. Nucl. Mater. 2012, 422, 77–85. [Google Scholar] [CrossRef]
- Lopes, D.A.; Restivo, T.A.G.; Padilha, A.F. Mechanical and thermal behaviour of U-Mo and U-Nb-Zr Alloys. J. Nucl. Mater. 2013, 440, 304–309. [Google Scholar] [CrossRef]
- Meyer, M.K.; Gan, J.; Jue, J.F.; Keiser, D.D.; Perez, E.; Robinson, A.; Wachs, D.M.; Woolstenhulme, N.; Hofman, G.L.; Kim, Y.S. Irradiation performance of U-Mo monolithic fuel. Nucl. Eng. Technol. 2014, 46, 169–182. [Google Scholar] [CrossRef]
- Yao, T.; Capriotti, L.; Harp, J.M.; Liu, X.; Wang, Y.; Teng, F.; Murray, D.J.; Winston, A.J.; Gan, J.; Benson, M.T.; et al. α-U and ω-UZr2 in neutron irradiated U-10Zr annular metallic fuel. J. Nucl. Mater. 2020, 542, 152536. [Google Scholar] [CrossRef]
- Yao, T.; Wagner, A.R.; Liu, X.; EI-Azab, A.; Harp, J.M.; Gan, J.; Hurley, D.H.; Benson, M.T.; He, L. On spinodal-like phase decomposition in U–50Zr alloy. Materialia 2020, 9, 100592. [Google Scholar] [CrossRef]
- Okamoto, H. Mo-U (Molybdenum-Uranium). J. Phase Equilibria Diffus. 2012, 33, 497. [Google Scholar] [CrossRef]
- Liu, X.; Li, Z.; Wang, J.; Wang, C. Thermodynamic modeling of the U–Mn and U–Nb systems. J. Nucl. Mater. 2008, 380, 99–104. [Google Scholar] [CrossRef]
- Xiong, W.; Xie, W.; Shen, C.; Morgan, D. Thermodynamic modeling of the U–Zr system—A revisit. J. Nucl. Mater. 2013, 443, 331–341. [Google Scholar] [CrossRef]
- Lu, Y.; Tang, Q.Q.; Wang, C.P.; Li, Z.S.; Guo, Y.H.; Liu, X.J. The Application of CALPHAD Calculations to Uranium-Based Metallic Nuclear Fuels. J. Phase Equilibria Diffus. 2018, 39, 714–723. [Google Scholar] [CrossRef]
- Kim, Y.S.; Hofman, G.L.; Yacout, A.M.; Kim, T.K. U-Mo alloy fuel for TRU-burning advanced fast reactors. J. Nucl. Mater. 2013, 441, 520–524. [Google Scholar] [CrossRef]
- Kaity, S.; Banerjee, J.; Parida, S.C.; Behere, P.G.; Bhasin, V. Structural, microstructural and thermal analysis of U-(6-x)Zr-xMo alloys (x = 0, 2, 4, 6). J. Nucl. Mater. 2020, 532, 152046. [Google Scholar] [CrossRef]
- Morais, N.W.S.; Schön, C.G. Mechanical characterization of U-9Mo-3Zr and U-3Mo-9Zr alloys. J. Alloys Compd. 2021, 871, 159501. [Google Scholar] [CrossRef]
- Park, J.M.; Ryu, H.J.; Lee, G.G.; Kim, H.S.; Lee, Y.S.; Kim, C.K.; Kim, Y.S.; Hofman, G.L. Phase Stability and Diffusion Characteristics of U-Mo-X (S=Si, Al, Zr) Alloys; INIS-XA-C--121; International Atomic Energy Agency (IAEA): Vienna, Austria, 2005. [Google Scholar]
- Nicholas, E. Phase Transformations and Microstructural Evolution in the U-10 wt.% Mo Alloy with Various Zr Additions at 900 °C and 650 °C. Master’s Thesis, University of Central Florida, Orlando, FL, USA, 2015. [Google Scholar]
- Hills, R.F.; Butcher, B.R.; Heywood, J.A. A study of the effect of cooling rate on the decomposition of the γ-phase in uranium-low molybdenum alloys. J. Less Common Met. 1961, 3, 155–169. [Google Scholar] [CrossRef]
- Connor, W. Fabrication and Characterization of Uranium-Molybdenum-Zirconium Alloys. Master’s Thesis, Texas A & M University, College Station, TX, USA, 2014. [Google Scholar]
- Vatulin, A.V.; Morozov, A.V.; Suprun, V.B.; Petrov, Y.I.; Trifonov, Y.I. High-density U-Mo fuel for research reactors. Met. Sci. Heat Treat. 2004, 46, 484–489. [Google Scholar] [CrossRef]
- Chiang, H.Y.; Döblinger, M.; Park, S.H.; Beck, L.; Petry, W. Ion beam induced spinodal decomposition and amorphization in the immiscible bilayer system UMo/Mg. J. Nucl. Mater. 2014, 453, 41–47. [Google Scholar] [CrossRef]
- Lu, Y.; Guo, H.; Gong, H.; Jiang, Z.; Huang, X.; Liu, X.; Wang, C. Effect of irradiation-induced cascade mixing on spinodal decomposition in U–Nb and U–Zr alloys: A phase field study. RSC Adv. 2021, 11, 37612–37623. [Google Scholar] [CrossRef]
- Ma, X.Q.; Shi, S.Q.; Woo, C.H.; Chen, L.Q. Phase-field simulation of hydride precipitation in bi-crystalline zirconium. Scr. Mater. 2002, 47, 237–241. [Google Scholar] [CrossRef]
- Luo, Z.; Du, Y.; Liu, Y.; Tang, S.; Pan, Y.; Mao, H.; Peng, Y.; Liu, W.; Liu, Z. Phase field simulation of the phase separation in the TiC-ZrC-WC system. Calphad 2018, 63, 190–195. [Google Scholar] [CrossRef]
- Peng, Y.; Du, Y.; Stratmann, M.; Long, J.; Liu, Y.; Mao, H.; Zapolsky, H. Precipitation of γ′ in the γ binder phase of WC-Al-Co-Ni cemented carbide: A phase-field study. Calphad 2020, 68, 101717. [Google Scholar] [CrossRef]
- Yan, Z.; Shi, S.; Li, Y.; Chen, J.; Maqbool, S. Vacancy and interstitial atom evolution with the separation of the nanoscale phase in Fe-Cr alloys: Phase-field simulations. Phys. Chem. Chem. Phys. PCCP 2020, 22, 3611–3619. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Hu, S.; Li, C.; Li, Q.; Chen, J.; Shu, G.; Jr, C.H.; Weng, Y.; Xu, B.; Liu, W. Simulations of irradiated-enhanced segregation and phase separation in Fe-Cu-Mn alloys. Model. Simul. Mater. Sci. Eng. 2017, 25, 065007. [Google Scholar] [CrossRef]
- Hu, S.; Henager, C.H., Jr. Phase-field modeling of void lattice formation under irradiation. J. Nucl. Mater. 2009, 394, 155–159. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, Y.; Schwen, D.; Jiang, C.; Sun, C.; Gan, J. Formation and self-organization of void superlattices under irradiation: A phase field study. Materialia 2018, 1, 78–88. [Google Scholar] [CrossRef]
- Li, Y.; Hu, S.; Sun, X.; Gao, F.; Henager, C.H., Jr.; Khaleel, M. Phase-field modeling of void migration and growth kinetics in materials under irradiation and temperature field. J. Nucl. Mater. 2010, 407, 119–125. [Google Scholar] [CrossRef]
- Semenov, A.A.; Woo, C.H. Phase-field modeling of void formation and growth under irradiation. Acta Mater. 2012, 60, 6112–6119. [Google Scholar] [CrossRef]
- Hu, S.; Henager, C.H.; Heinisch, H.L.; Stan, M.; Baskes, M.I.; Valone, S.M. Phase-field modeling of gas bubbles and thermal conductivity evolution in nuclear fuels. J. Nucl. Mater. 2009, 392, 292–300. [Google Scholar] [CrossRef]
- Hu, S.; Burkes, D.E.; Lavender, C.A.; Senor, D.J.; Setyawan, W.; Xu, Z. Formation mechanism of gas bubble superlattice in U-Mo metal fuels: Phase-field modeling investigation. J. Nucl. Mater. 2016, 479, 202–216. [Google Scholar] [CrossRef]
- Hu, S.; Setyawan, W.; Beeler, B.W.; Gan, J.; Burkes, D.E. Defect cluster and nonequilibrium gas bubble associated growth in irradiated UMo fuels-A cluster dynamics and phase field model. J. Nucl. Mater. 2020, 542, 152441. [Google Scholar] [CrossRef]
- Gilles, D.; Sylvain, D.; Laurence, L.; David, S.; Vassilis, P. Irradiation-based design of mechanically resistant microstructures tuned via multiscale phase-field modeling. Sci. Rep. 2018, 8, 10237. [Google Scholar]
- Hu, S.; Casella, A.M.; Lavender, C.A.; Senor, D.J.; Burkes, D.E. Assessment of effective thermal conductivity in U-Mo metallic fuels with distributed gas bubbles. J. Nucl. Mater. 2015, 462, 64–76. [Google Scholar] [CrossRef]
- Liang, L.; Mei, Z.-G.; Kim, Y.S.; Anitescu, M.; Yacout, A.M. Three-dimensional phase-field simulations of intragranular gas bubble evolution in irradiated U-Mo fuel. Comput. Mater. Sci. 2018, 145, 86–95. [Google Scholar] [CrossRef]
- Khachaturyan, A.G. Theory of Structural Transformations in Solids; Courier Corporation: North Chelmsford, MA, USA, 2013. [Google Scholar]
- Wang, Y.U.; Jin, Y.M.; Khachaturyan, A.G. Phase field microelasticity theory and modeling of elastically and structurally inhomogeneous solid. J. Appl. Phys. 2002, 92, 1351–1360. [Google Scholar] [CrossRef]
- Wang, Y.; Li, J. Phase field modeling of defects and deformation. Acta Mater. 2010, 58, 1212–1235. [Google Scholar] [CrossRef]
- Cahn, J.W. Phase separation by spinodal decomposition in isotropic systems. J. Chem. Phys. 1965, 42, 93–99. [Google Scholar] [CrossRef]
- Cahn, J.W.; Hilliard, J.E. Spinodal decomposition: A reprise. Acta Metall. 1971, 19, 151–161. [Google Scholar] [CrossRef]
- Cahn, J.W.; Hilliard, J.E. Free energy of a nonuniform system. I. Interfacial free energy. J. Chem. Phys. 1958, 28, 258–267. [Google Scholar] [CrossRef]
- Cahn, J.W. On spinodal decomposition. Acta Metall. 1961, 9, 795–801. [Google Scholar] [CrossRef]
- Dinsdale, A.T. SGTE data for pure elements. Calphad 1991, 15, 317–425. [Google Scholar] [CrossRef]
- Berche, A.; Dupin, N.; Guéneau, C.; Rado, C.; Sundman, B.; Dumas, J.C. Calphad thermodynamic description of some binary systems involving U. J. Nucl. Mater. 2011, 411, 131–143. [Google Scholar] [CrossRef]
- Liu, W.; Li, Y.; Wu, X.; Hou, Z.; Hu, K. Phase-Field Simulation of the Separation Kinetics of a Nanoscale Phase in a Fe-Cr Alloy. J. Mater. Eng. Perform. 2016, 25, 1924–1930. [Google Scholar] [CrossRef]
- Mohanty, R.R.; Guyer, J.E.; Sohn, Y.H. Diffusion under temperature gradient: A phase-field model study. J. Appl. Phys. 2009, 106, 034912. [Google Scholar] [CrossRef]
- Koyama, T.; Onodera, H. Phase-Field simulation of phase decomposition in Fe-Cr-Co alloy under an external magnetic field. Met. Mater. Int. 2004, 10, 321–326. [Google Scholar] [CrossRef]
- Huang, K.; Keiser, D.D.; Sohn, Y. Interdiffusion, intrinsic diffusion, atomic mobility, and vacancy wind effect in γ(bcc) uranium-molybdenum alloy. Metall. Mater. Trans. A 2013, 44, 738–746. [Google Scholar] [CrossRef]
- Bian, B.; Zhou, P.; Wen, S.; Du, Y. Atomic mobilities and diffusivities in UX (X = Nb, Zr, Ti) bcc alloys. Calphad 2018, 61, 85–91. [Google Scholar] [CrossRef]
- Enrique, R.A.; Bellon, P. Compositional patterning in systems driven by competing dynamics of different length scale. Phys. Rev. Lett. 2000, 84, 2885. [Google Scholar] [CrossRef] [PubMed]
- Enrique, R.A.; Nordlund, K.; Averback, R.S.; Bellon, P. Simulations of dynamical stabilization of Ag-Cu nanocomposites by ion-beam processing. J. Appl. Phys. 2003, 93, 2917–2923. [Google Scholar] [CrossRef]
- Enrique, R.A.; Bellon, P. Compositional patterning in immiscible alloys driven by irradiation. Phys. Rev. B 2001, 63, 134111. [Google Scholar] [CrossRef]
- L’vov, P.E.; Svetukhin, V.V. Phase-field simulation of radiation-induced phase transition in binary alloys. Model. Simul. Mater. Sci. Eng. 2021, 29, 035013. [Google Scholar] [CrossRef]
- Demange, G.; Antoshchenkova, E.; Hayoun, M.; Lunéville, L.; Simeone, D. Simulating the ballistic effects of ion irradiation in the binary collision approximation: A first step toward the ion mixing framework. J. Nucl. Mater. 2017, 486, 26–33. [Google Scholar] [CrossRef]
- Shu, S.; Almirall, N.; Wells, P.B.; Yamamoto, T.; Odette, G.R.; Morgan, D.D. Precipitation in Fe-Cu and Fe-Cu-Mn model alloys under irradiation: Dose rate effects. Acta Mater. 2018, 157, 72–82. [Google Scholar] [CrossRef]
- Ke, J.-H.; Reese, E.R.; Marquis, E.A.; Odette, G.R.; Morgan, D. Flux effects in precipitation under irradiation-Simulation of Fe-Cr alloys. Acta Mater. 2019, 164, 586–601. [Google Scholar] [CrossRef]
- Bellon, P. Precipitate and Microstructural Stability in Alloys Subjected to Sustained Irradiation. In Materials Science with Ion Beams; Springer: Berlin/Heidelberg, Germany, 2009; pp. 29–52. [Google Scholar]
- Norgett, M.J.; Robinson, M.T.; Torrens, I.M. A proposed method of calculating displacement dose rates. Nucl. Eng. Des. 1975, 33, 50–54. [Google Scholar] [CrossRef]
- Hu, S.Y.; Chen, L.Q. A phase-field model for evolving microstructures with strong elastic inhomogeneity. Acta Mater. 2001, 49, 1879–1890. [Google Scholar] [CrossRef]
- Sugathan, S.; Bhattacharya, S. Effect of elastic anisotropy on phase separation in ternary alloys: A phase-field study. arXiv 2019, arXiv:1906.09637. [Google Scholar]
- Sugathan, S.; Bhattacharya, S. A phase-field study of elastic stress effects on phase separation in ternary alloys. Comput. Mater. Sci. 2020, 172, 109284. [Google Scholar] [CrossRef]
- Jiang, C.; Wolverton, C.; Sofo, J.; Chen, L.Q.; Liu, Z.K. First-principles study of binary bcc alloys using special quasirandom structures. Phys. Rev. B 2004, 69, 214202. [Google Scholar] [CrossRef]
- Wang, B.T.; Zhang, P.; Liu, H.Y.; Li, W.D.; Zhang, P. First-principles calculations of phase transition, elastic modulus, and superconductivity under pressure for zirconium. J. Appl. Phys. 2011, 109, 063514. [Google Scholar] [CrossRef]
- Beeler, B.; Deo, C.; Baskes, M.; Okuniewski, M. First principles calculations of the structure and elastic constants of α, β and γ uranium. J. Nucl. Mater. 2013, 433, 143–151. [Google Scholar] [CrossRef]
- Moore, A.P.; Beeler, B.; Deo, C.; Baskes, M.I.; Okuniewski, M.A. Atomistic modeling of high temperature uranium–zirconium alloy structure and thermodynamics. J. Nucl. Mater. 2015, 467, 802–819. [Google Scholar] [CrossRef]
- Mei, Z.-G.; Liang, L.; Kim, Y.S.; Wiencek, T.; O’Hare, E.; Yacout, A.M.; Hofman, G.; Anitescu, M. Grain growth in U–7Mo alloy: A combined first-principles and phase field study. J. Nucl. Mater. 2016, 473, 300–308. [Google Scholar] [CrossRef]
- Cahn, J.W.; Hilliard, J.E. Free energy of a nonuniform system. III. Nucleation in a two-component incompressible fluid. J. Chem. Phys. 1959, 31, 688–699. [Google Scholar] [CrossRef]
- Nordlund, K.; Zinkle, S.J.; Sand, A.E.; Granberg, F.; Averback, R.S.; Stoller, R.E.; Suzudo, T.; Malerba, L.; Banhart, F.; Weber, W.J.; et al. Primary radiation damage: A review of current understanding and models. J. Nucl. Mater. 2018, 512, 450–479. [Google Scholar] [CrossRef]
- Wang, Y.H.; Zhang, D.C.; Pi, Z.P.; Lin, J.G.; Wen, C. Phase field simulation of spinodal decomposition in Zr-Nb alloys for implant materials. J. Appl. Phys. 2019, 126, 085102. [Google Scholar] [CrossRef]
- Li, Y.; Yan, Z.; Zhou, X. Kinetics of initial phase separation and coarsening of nanoscale phase in Fe-Cr alloys. J. Nucl. Mater. 2017, 497, 154–460. [Google Scholar] [CrossRef]
- Ujihara, T.; Osamura, K. Kinetic analysis of spinodal decomposition process in Fe-Cr alloys by small angle neutron scattering. Acta Mater. 2000, 48, 1629–1637. [Google Scholar] [CrossRef]
- Bernas, H. Materials Science with Ion Beams; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Enrique, R.A.; Pascal, B. Phase stability under irradiation in alloys with a positive heat of mixing: Effective thermodynamics description. Phys. Rev. B 1999, 60, 14649. [Google Scholar] [CrossRef]
- Fujii, K.; Fukuya, K. Effects of radiation on spinodal decomposition of ferrite in duplex stainless steel. J. Nucl. Mater. 2013, 440, 612–616. [Google Scholar] [CrossRef]
- Schwen, D.; Schunert, S.; Jokisaari, A. Evolution of microstructures in radiation fields using a coupled binary-collision Monte Carlo phase field approach. Comput. Mater. Sci. 2021, 192, 110321. [Google Scholar] [CrossRef]
- Lu, Y.; Jiang, Z.; Li, L.; Wang, C.; Liu, X. Calculation of steady-state dynamical phase diagram in U-Mo binary system under irradiation. J. Nucl. Mater. 2021, 544, 152698. [Google Scholar] [CrossRef]
- Liu, X.J.; Zhao, Y.L.; Lu, Y.; Xu, W.W.; Jia, J.P.; Wang, C.P. Steady-state dynamical phase diagram calculation of U–Nb binary system under irradiation: Ballistic effect. J. Nucl. Mater. 2014, 451, 366–371. [Google Scholar] [CrossRef]
- Lu, Y.; Jiang, Z.; Li, L.; Wang, C.; Guo, Y.; Han, J.; Liu, X. Calculation of Dynamical Phase Diagram in U-Zr Binary System Under Irradiation. J. Nucl. Eng. Radiat. Sci. 2021, 7, 011605. [Google Scholar] [CrossRef]
- Zhou, N.; Wu, F.; Zhu, Y.; Li, X.; Wu, Q.; Wu, H. Defect production and segregation induced by collision cascades in U-10Zr alloy. J. Nucl. Mater. 2019, 526, 151769. [Google Scholar] [CrossRef]
- Moore, A.; Deo, C.; Baskes, M.; Okuniewski, M. Atomistic mechanisms of morphological evolution and segregation in U-Zr alloys. Acta Mater. 2016, 115, 178–188. [Google Scholar] [CrossRef]




| BCC_A2: (Mo,U,Zr)1(Va)3 |
|---|
| Alloy Composition | Aging Temperature | Irradiation Condition |
|---|---|---|
| U-65 at.% Mo | 1273 K | 0 dap/s |
| U-75 at.% Mo | 923 K | 0 dap/s |
| U-75 at.% Mo | 1273 K | 0 dap/s |
| U-75 at.% Mo | 1473 K | 0 dap/s |
| U-85 at.% Mo | 1273 K | 0 dap/s |
| U-65 at.% Mo | 1373 K | 1.0 × 10−4 dap/s |
| U-75 at.% Mo | 1273 K | 5.0 × 10−5 dap/s |
| U-75 at.% Mo | 1273 K | 1.0 × 10−4 dap/s |
| U-75 at.% Mo | 1273 K | 2.0 × 10−4 dap/s |
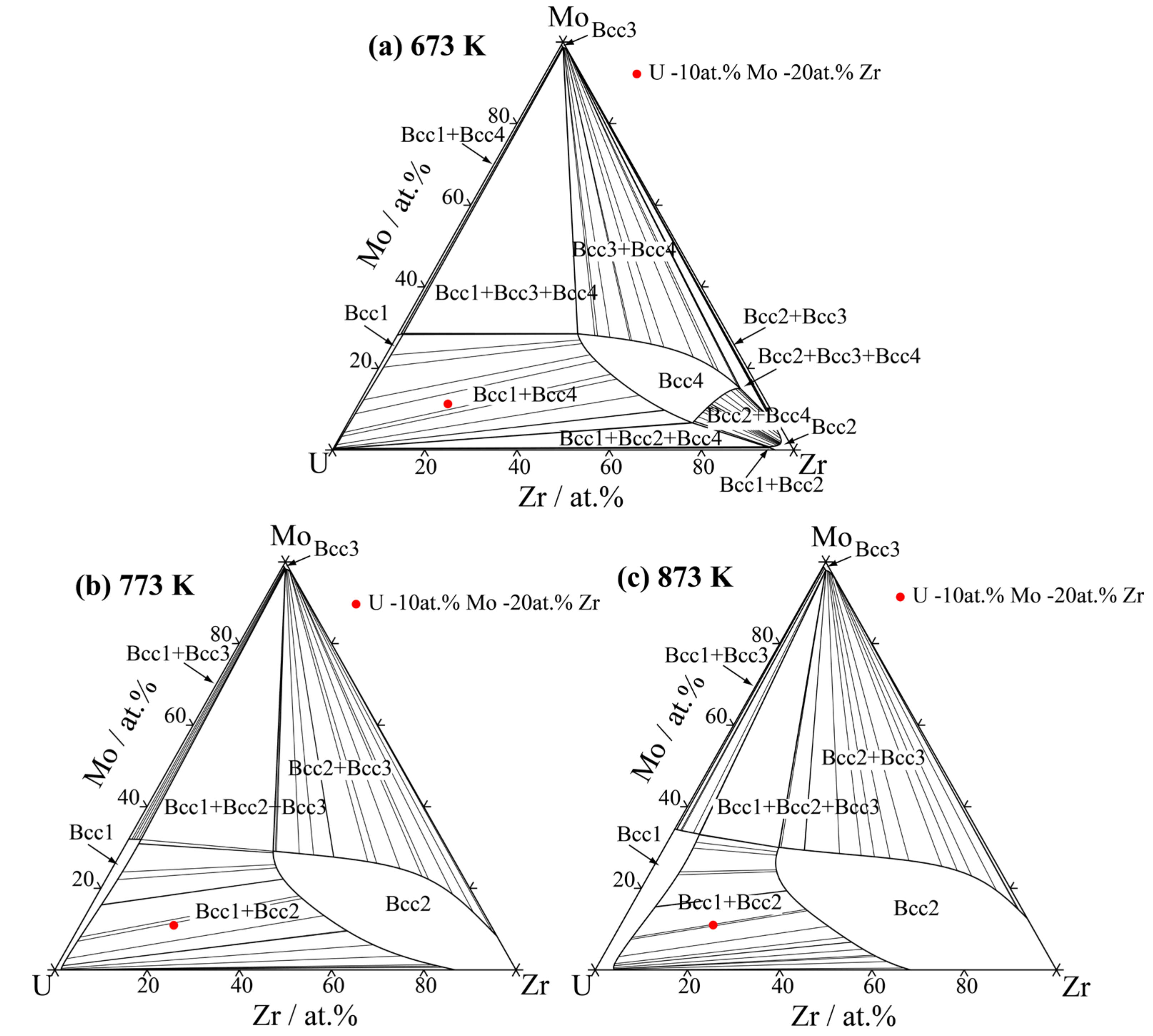
| U-10 at.% Mo-20 at.% Zr | 673 K | 0 dap/s |
| U-10 at.% Mo-20 at.% Zr | 773 K | 0 dap/s |
| U-10 at.% Mo-20 at.% Zr | 873 K | 0 dap/s |
| U-10 at.% Mo-20 at.% Zr | 873 K | 1.0 × 10−4 dap/s |
| U-10 at.% Mo-20 at.% Zr | 873 K | 2.0 × 10−4 dap/s |
| U-65 at.% Mo-5 at.% Zr | 1373 K | 1.0 × 10−4 dap/s |
| U-65 at.% Mo-10 at.% Zr | 1373 K | 1.0 × 10−4 dap/s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, Y.; Ni, X.; Guo, H.; Huang, X.; Sun, D.; Li, W.; Liu, X.; Wang, C. A Phase-Field Study of Spinodal Decomposition Impeded by Irradiation in U-Mo and U-Mo-Zr Alloys. Materials 2023, 16, 7546. https://doi.org/10.3390/ma16247546
Lu Y, Ni X, Guo H, Huang X, Sun D, Li W, Liu X, Wang C. A Phase-Field Study of Spinodal Decomposition Impeded by Irradiation in U-Mo and U-Mo-Zr Alloys. Materials. 2023; 16(24):7546. https://doi.org/10.3390/ma16247546
Chicago/Turabian StyleLu, Yong, Xue Ni, Honghao Guo, Xiaoyi Huang, Dan Sun, Wenjie Li, Xingjun Liu, and Cuiping Wang. 2023. "A Phase-Field Study of Spinodal Decomposition Impeded by Irradiation in U-Mo and U-Mo-Zr Alloys" Materials 16, no. 24: 7546. https://doi.org/10.3390/ma16247546
APA StyleLu, Y., Ni, X., Guo, H., Huang, X., Sun, D., Li, W., Liu, X., & Wang, C. (2023). A Phase-Field Study of Spinodal Decomposition Impeded by Irradiation in U-Mo and U-Mo-Zr Alloys. Materials, 16(24), 7546. https://doi.org/10.3390/ma16247546





