Analysis of Shear Model for Steel-Fiber-Reinforced High-Strength Concrete Corbels with Welded-Anchorage Longitudinal Reinforcement
Abstract
:1. Introduction
2. Experimental Study
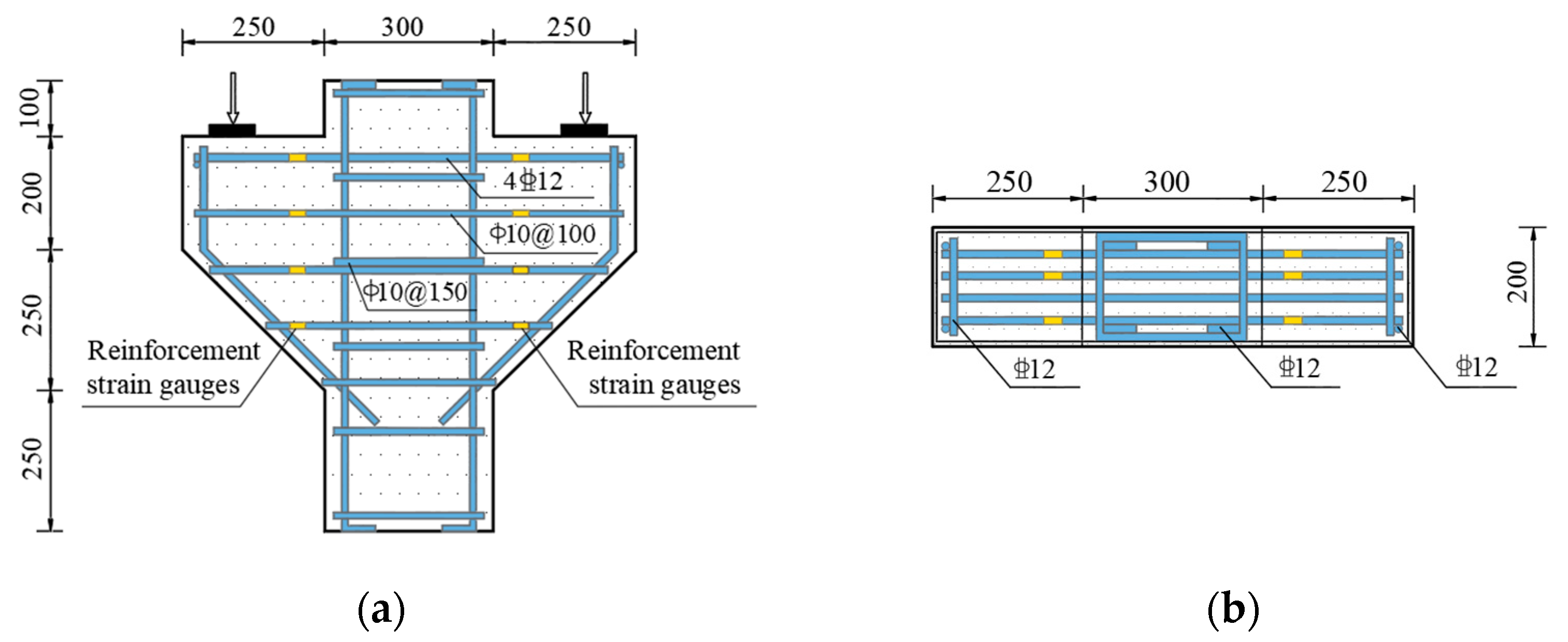
2.1. Specimen Design and Production
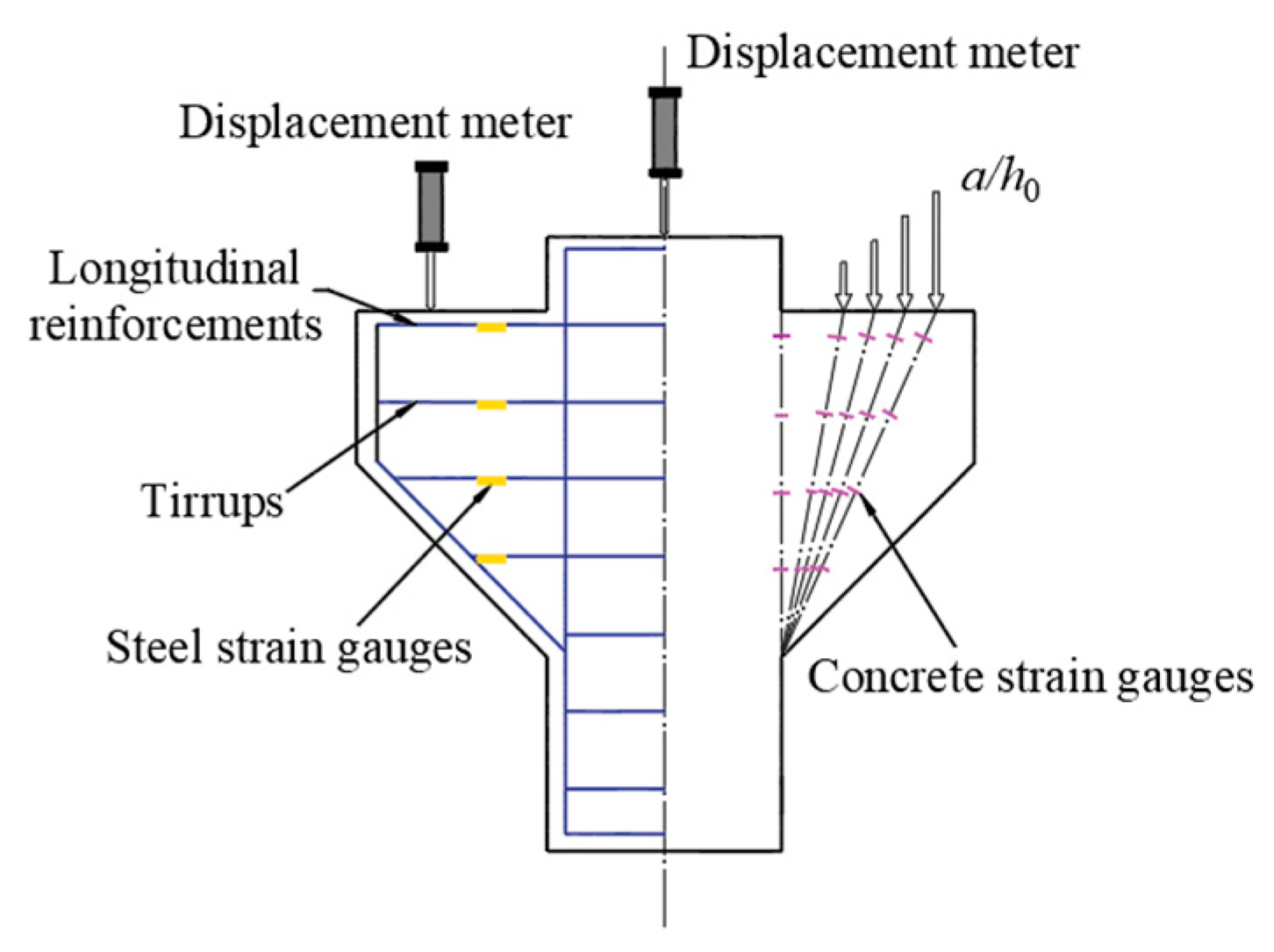
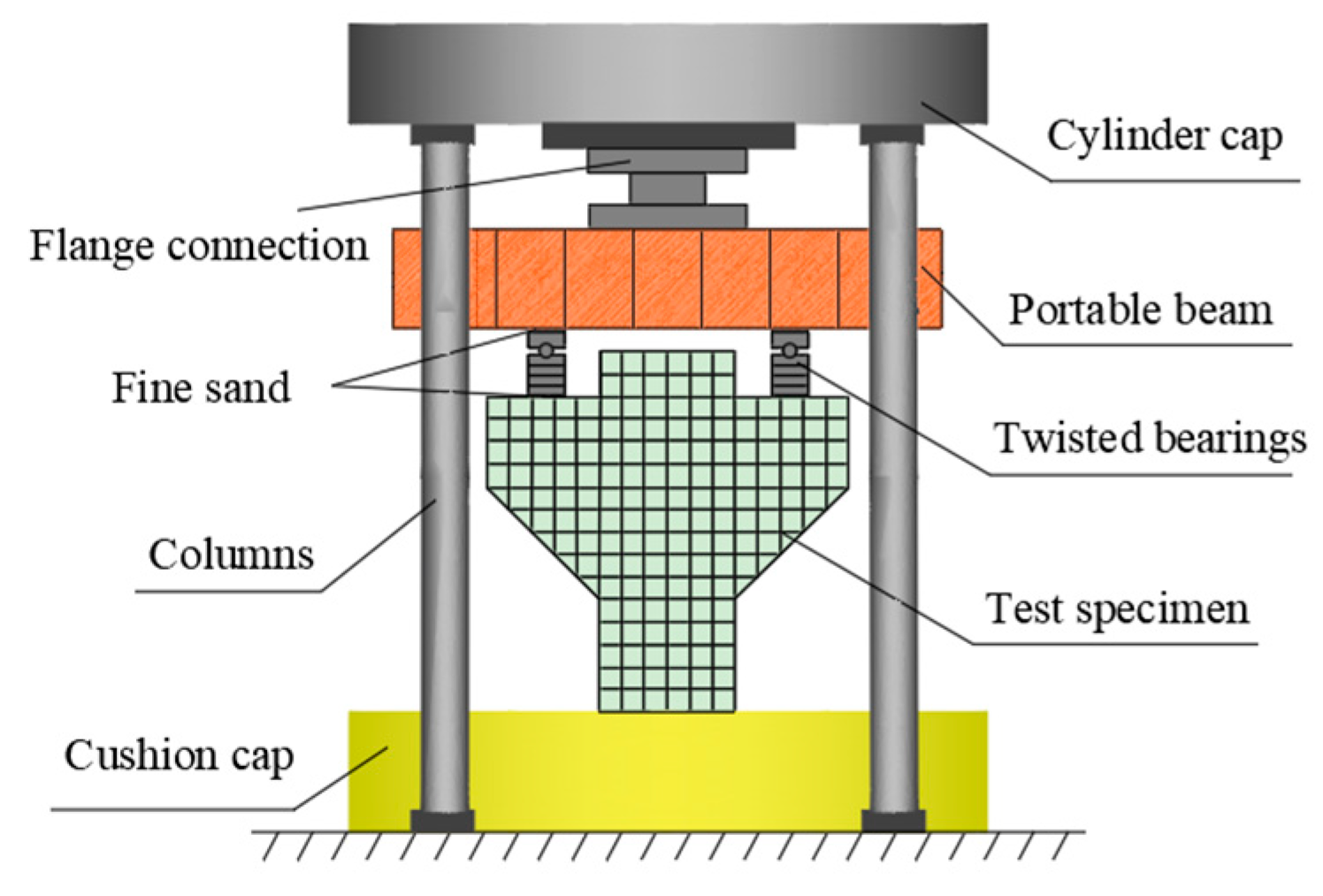
2.2. Measuring Points Arrangement and Loading Scheme
2.3. Test Results
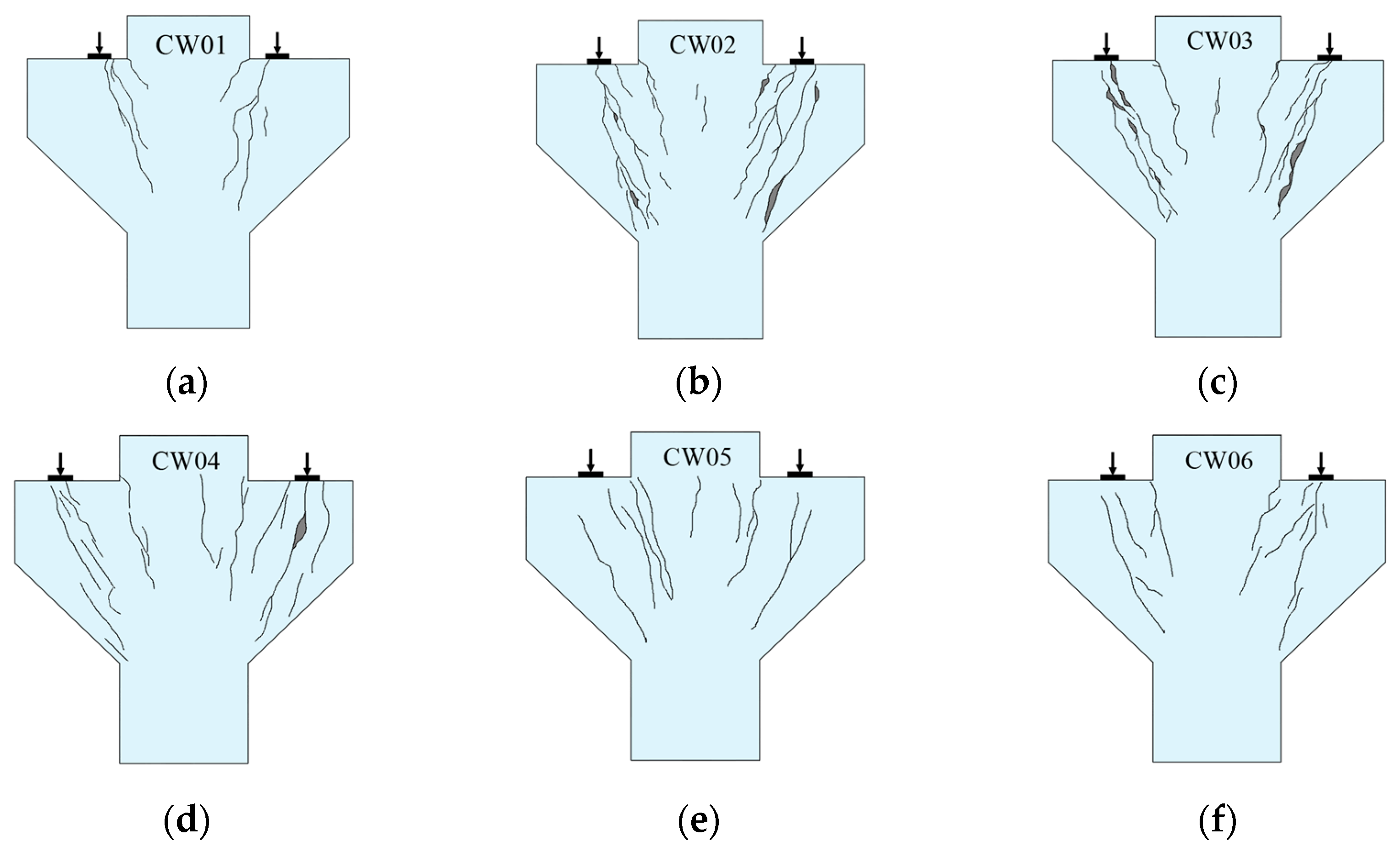
2.4. Failure Process and Failure Forms
2.5. Analysis of Influencing Factors
2.5.1. Shear Span Ratio
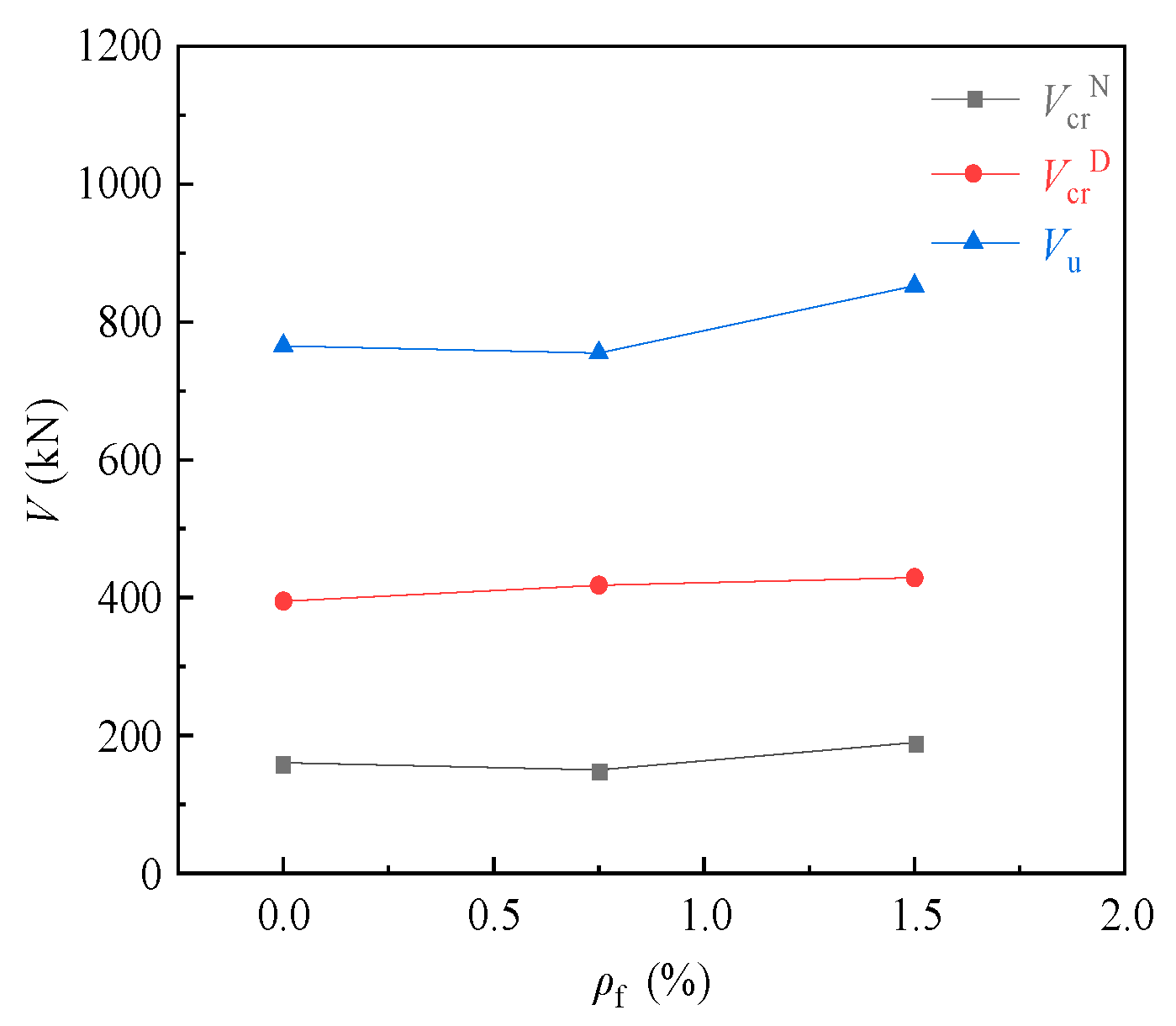
2.5.2. Steel Fiber Volume Fraction
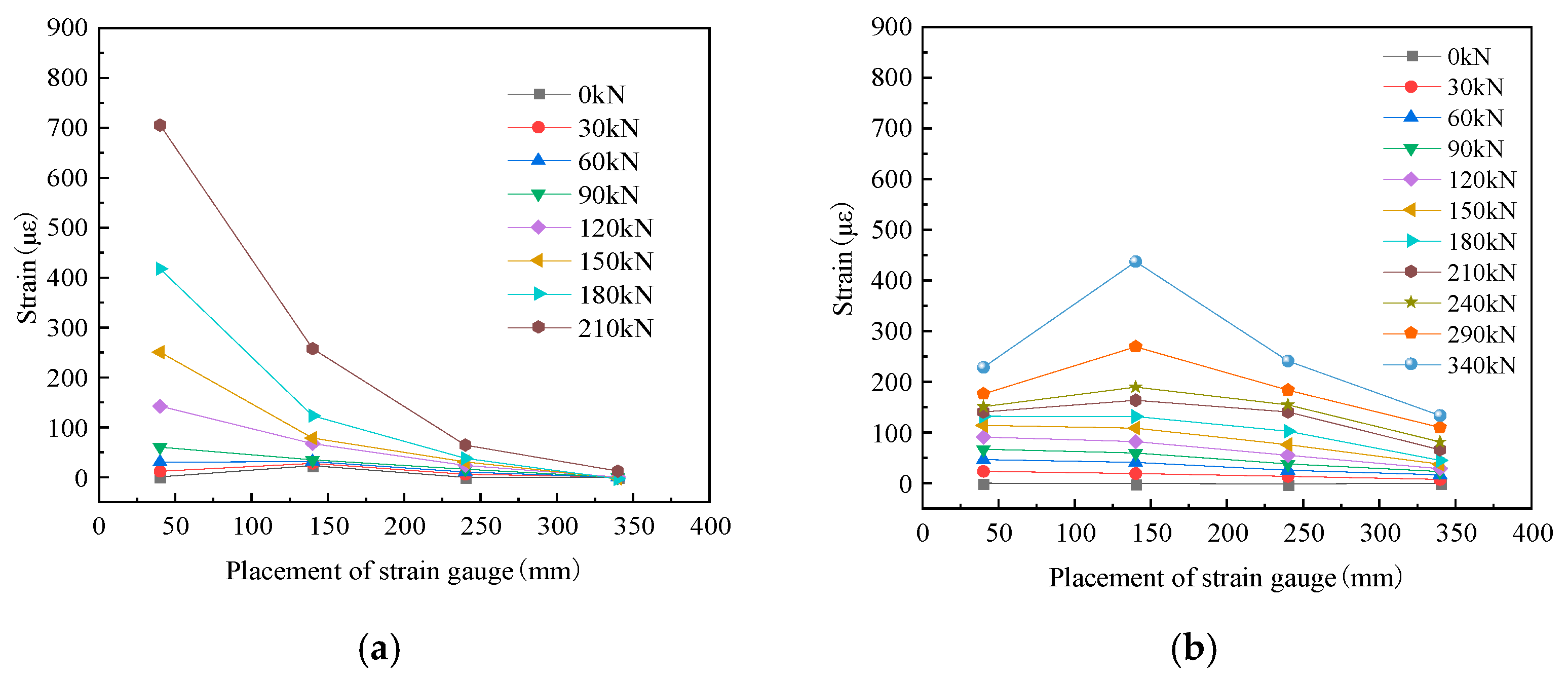
2.6. Concrete Strain
3. Calculation Methods of National Codes
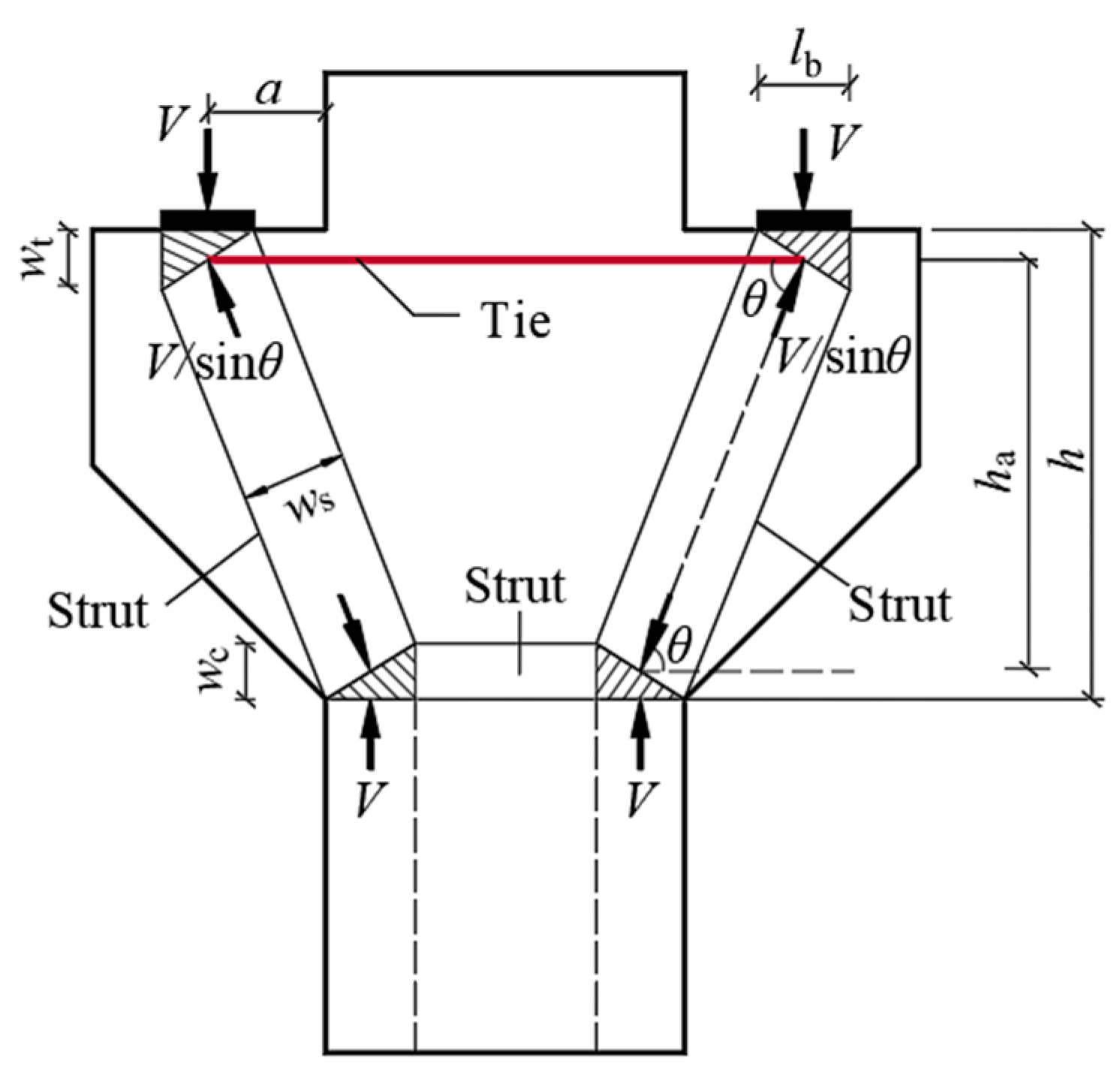
4. The Calculation Model of Shear Bearing Capacity of Corbels Based on Softened Strut-and-Tie Model (MSSTM)
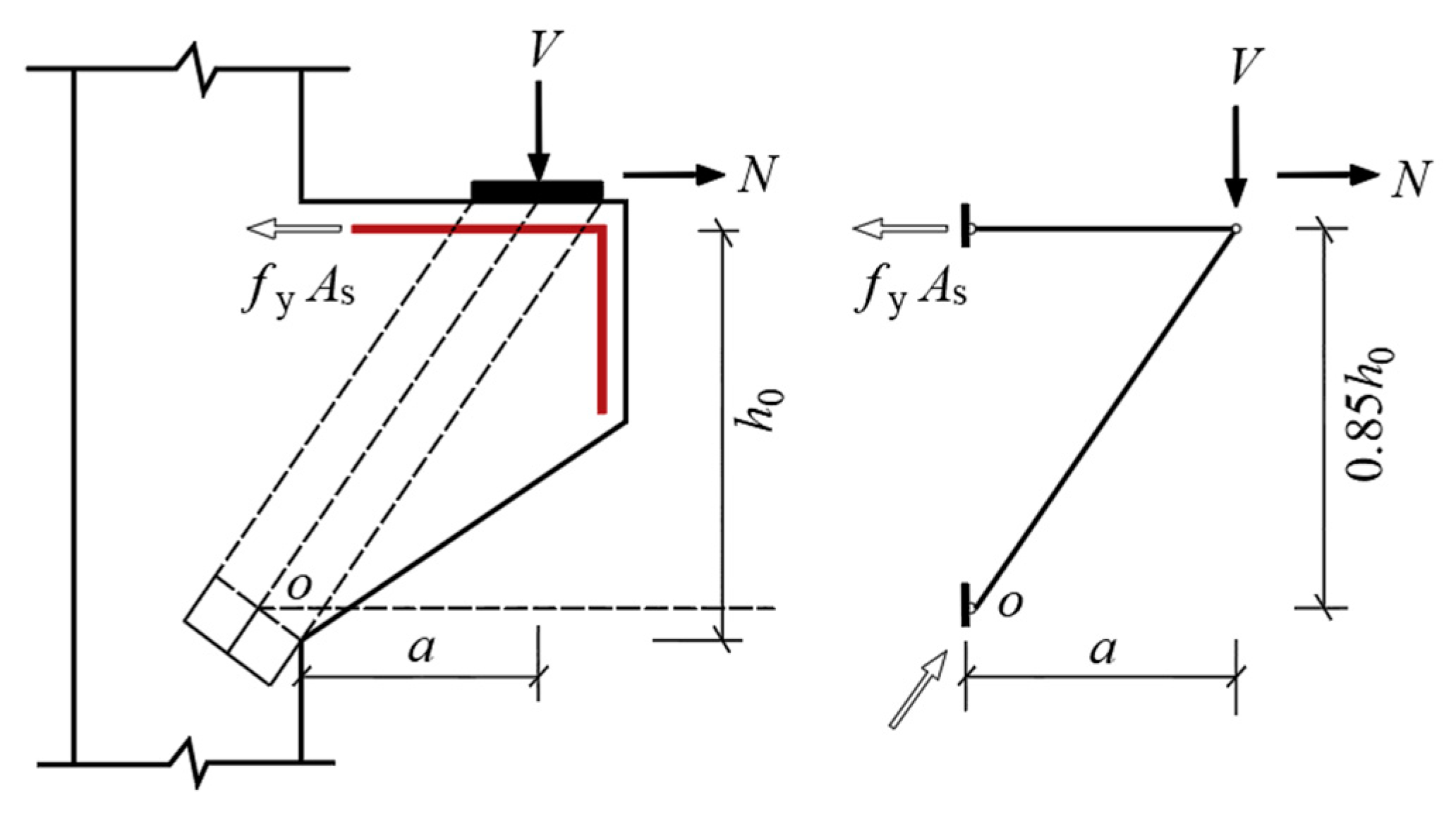
4.1. Shear Mechanism
4.2. Force Equilibrium
4.3. Constitutive Laws
4.4. Strain Compatibility
4.5. Solution Process
- (1)
- Input the basic parameters of the corbel: , , , , , , , etc.;
- (2)
- and are calculated through Equations (13) to (15);
- (3)
- Select the initial , and solve D and using Equations (10) and (12);
- (4)
- Calculate and through Equations (16) and (29), and take values of reasonably based on the size of the corbels;
- (5)
- Given reasonably, is solved using Equation (28), and then the softening coefficient ζ is solved using Equation (18);
- (6)
- is calculated using Formula (19), and then the value corresponding to a given is calculated by the steel bar constitutive Equation (17);
- (7)
- Compare the values of and , and if , repeat steps (3) to (6) until ;
- (8)
- After satisfying step (7), compare and . If , repeat steps (5) to (7) until , at which point the solving is completed. At this point, the maximum stress in the node zone has reached the strength limit of the concrete, and the is the bearing capacity value of the corbel.
5. Simplification of the Calculation Model for the Shear Bearing Capacity of the Corbels (SMSSTM)
5.1. Approximation of Softening Effect
5.2. Strut-and-Tie Index K
6. Example Verification
- (1)
- The mean values of the ratios of the test values to the calculated values of the GB50010-2010 code, the ACI318-19 code, the EN 1992-1-1 code and the CSA A23.3-19 code were 1.564, 1.373, 1.533 and 1.153, respectively, and the variances were 0.131, 0.025, 0.046 and 0.011, respectively. It can be seen that all the calculation results were lower than the experimental values, and the results were conservative, which indicates that the various national codes are applicable to the calculation of the shear capacity of steel-fiber-reinforced concrete corbels. However, the calculation results of the GB50010-2010 code were relatively discrete, while the variances of the calculation results of the ACI318-19 code, the EN 1992-1-1 code and the CSA A23.3-19 code based on the strut-and-tie model were relatively small.
- (2)
- The mean value of the ratio of the test value to the calculated value of the established shear bearing capacity calculation model was 0.965, and the variance was 0.016. The mean value of the ratio between the experimental value and calculation value of the simplified model was 1.014, and the variance was 0.008. The calculated results were in good agreement with the experimental values. The established model for calculating the shear bearing capacity of the corbel has a clear mechanical concept, which can be used for the calculation and prediction of the shear bearing capacity of steel-fiber-reinforced high-strength concrete corbels.
7. Conclusions
- The experimental studies showed that the steel-fiber-reinforced high-strength concrete corbels with shear span ratio from 0.2 to 0.5 mainly exhibit two typical failure modes: shear failure and diagonal compression failure. With an increase in shear span ratio, the bearing capacity of the corbel specimens decreases gradually, and the failure mode of specimens transitions from shear failure to diagonal compression failure. At the same time, with the same shear span ratio and the addition of steel fibers, the bearing capacity of the corbels increases with the increase in the steel fiber volume fraction.
- Steel fiber can improve the ductility of reinforced concrete corbels, but it has little effect on the failure mode of the diagonal section. The addition of steel fiber cannot change the characteristic that the normal section of the corbel does not comply with the plane section assumption.
- The shear mechanism of steel-fiber-reinforced high-strength concrete corbels can be described by the softened strut-and-tie model composed of diagonal struts and horizontal resistance mechanisms. In the analysis of shear capacity, randomly distributed steel fibers in concrete can be equivalent to an equal number of horizontal and vertical reinforcements, making the contribution of steel fiber dimensions to the bearing capacity of corbels more clear.
- The shear bearing capacity of steel-fiber-reinforced high-strength concrete corbels was calculated by using the national codes and the established corbel shear bearing capacity calculation model in this paper. The results showed that the calculation results of the national codes are lower than the experimental values, and the results are conservative. The national codes can be used for the shear bearing capacity calculation of steel-fiber-reinforced high-strength concrete corbels. However, the calculation results of the Chinese code (GB50010-2010) were more discrete, while the variances of the calculation results of the American code (ACI318-19), European code (EN 1992-1-1) and Canadian code (CSA A23.3-19), which are based on the strut-and-tie model, were relatively small. The theoretical calculation of the shear capacity calculation model for the corbels established in this paper was close to the experimental results. Moreover, the model has a clear mechanical concept considering the tensile properties of steel-fiber-reinforced concrete and the influence of horizontal stirrups, which can reasonably reflect the stress mechanism of the corbels and provide a theoretical reference for improving the design of steel-fiber-reinforced high-strength concrete corbels.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| shear-span ratio, dimensionless | ratio of the horizontal tie to the horizontal shear without a vertical tie, dimensionless | ||
| longitudinal reinforcement ratio, dimensionless | maximum compressive forces in the nodal zone | ||
| stirrup ratio, dimensionless | principal stress of concrete in the d-direction | ||
| steel fiber volume fraction, dimensionless | ζ | softening coefficient of steel-fiber-reinforced concrete | |
| cube compressive strength of concrete, MPa | principal compressive strain of concrete | ||
| prism compressive strength of concrete, MPa | principal tensile strain of concrete | ||
| tensile strength of concrete, MPa | peak strain of steel-fiber-reinforced concrete | ||
| elastic modulus of concrete, MPa | tensile stress of the horizontal stirrups, MPa | ||
| yield strength of reinforcement, MPa | tensile strain of the horizontal stirrups, dimensionless | ||
| elastic modulus of reinforcement, MPa | yield stress of horizontal stirrups, MPa | ||
| ultimate strength of reinforcement, MPa | yield strain of horizontal stirrups, dimensionless | ||
| yield strain of reinforcement, dimensionless | tensile stress of the steel fiber, MPa | ||
| cracking load of the normal section, kN | tensile stress of the horizontal steel fiber, MPa | ||
| cracking load of the diagonal section, kN | elastic modulus of steel fibers, MPa | ||
| ultimate load, kN | tensile strain of the steel fiber, dimensionless | ||
| inclination angle of two gentle struts in SSTM | tensile strain of horizontal steel fiber, dimensionless | ||
| lever arm, mm | maximum bond strength between steel fiber and concrete, MPa | ||
| modular ratio of elasticity, dimensionless | tensile strength of steel-fiber concrete, MPa | ||
| ratio of bending tensile reinforcement, dimensionless | influencing factor for different types of steel fibers, dimensionless | ||
| cross-sectional area of longitudinal reinforcement, mm² | surface area of the steel fiber, mm² | ||
| cross-sectional area of horizontal stirrup, mm² | diameter of steel fiber, mm | ||
| stirrup influence coefficient, dimensionless | effective anchorage length of steel fiber, mm | ||
| effective area of the diagonal strut, mm² | length of steel fiber, mm | ||
| width of the diagonal strut in SSTM, mm | average strain in the horizontal direction of steel-fiber-reinforced concrete, dimensionless | ||
| tension of the horizontal stirrup tie, kN | average strain in the vertical direction of steel-fiber-reinforced concrete, dimensionless | ||
| tension of the horizontal steel-fiber tie, kN | strut-and-tie index, dimensionless | ||
| cross-sectional area of horizontal steel-fiber tie, mm² | nominal diagonal compressive strength of corbels, kN | ||
| cross-sectional area of a single steel fiber, mm² | diagonal compression, kN | ||
| the number of equivalent horizontal steel fibers | diagonal strut index, dimensionless | ||
| effective coefficient of horizontal stirrup shear resistance, dimensionless | horizontal tie index with sufficient horizontal reinforcement, dimensionless | ||
| equivalent coefficient for steel fiber, dimensionless | balanced amount of the horizontal tie force, kN | ||
| resistance against the vertical shear, kN | horizontal tie index with insufficient horizontal reinforcement, dimensionless | ||
| resistance against the horizontal shear, kN | yield strength of the horizontal tie, kN | ||
| compression force in the diagonal strut, kN | yield stress of horizontal steel-fiber tie, MPa | ||
| tension force in the horizontal tie, kN | yield strain of horizontal tie, dimensionless | ||
| ratio of the corbel shear resisted by the diagonal mechanism, dimensionless | strut-and-tie index with sufficient horizontal reinforcement, dimensionless | ||
| ratio of the corbel shear resisted by the horizontal mechanism, dimensionless |
References
- Wang, Y.W.; Wang, M.T. An Experimental investigation of the Strength of Bracket Under the Mutual Actions of Vertical Loads and Horizontal Thrusts. J. Tsinghua Univ. (Sci. Technol.) 1975, 4, 93–118. [Google Scholar]
- Xu, L. Testing Research on Mechanic Properties of Steel Fiber Reinforced Concrete Corbel. Master’s Thesis, Zhengzhou University, Zhengzhou, China, 2002. [Google Scholar]
- Wilson, H.R.; Yousefpour, H. Investigation of Corbels Designed According to Strut-and-Tie and Empirical Methods. ACI Struct. J. 2018, 115, 813–824. [Google Scholar] [CrossRef]
- Fattuhi, N.I. Strength of SFRC corbels subjected to vertical load. J. Struct. Eng. 1990, 116, 701–718. [Google Scholar] [CrossRef]
- Fattuhi, N.I. Strength of FRC corbels in flexure. J. Struct. Eng. 1994, 120, 360–377. [Google Scholar] [CrossRef]
- Campione, G.; La Mendola, L.; Mangiavillano, M.L. Steel fiber-reinforced concrete corbels: Experimental behavior and shear strength prediction. ACI Struct. J. 2007, 104, 570–579. [Google Scholar]
- Yang, J.-M.; Lee, J.-H.; Yoon, Y.-S.; Cook, W.D.; Mitchell, D. Influence of steel fibers and headed bars on the serviceability of high-strength concrete corbels. J. Struct. Eng. 2012, 138, 123–129. [Google Scholar] [CrossRef]
- Gao, J.F.; Qiu, H.X.; Jiang, Y.S. Investigation on Design Method of the Steel-Fiber High-Strength Concrete Bracket. J. Southeast Univ. (Nat. Sci. Ed.) 1993, 23, 41–46. [Google Scholar]
- Gao, D.Y.; Zhao, J.; Zhu, H.T. Experimental study on shear capacity of reinforced concrete corbel with steel fiber. J. Build. Struct. 2006, 27, 100–106. [Google Scholar]
- Kurtoglu, A.E.; Gulsan, M.E.; Abdi, H.A.; Kamil, M.A.; Cevik, A. Fiber reinforced concrete corbels: Modeling shear strength via symbolic regression. Comp. Concr. 2017, 20, 1–10. [Google Scholar]
- Huang, Y.; Yin, W.M.; Yi, W.J. Study on Design Modification Factor for Shear Capacity of Reinforced Concrete Corbels. J. Hunan Univ. (Nat. Sci.) 2021, 48, 61–70. [Google Scholar]
- GB50010-2010; Code for Design of Concrete Structures. China Building Industry Press: Beijing, China, 2010.
- ACI Committee 318; Building Code Requirement for Structure Concrete (ACI318-19) and Commentary (ACI318R-19). American Concrete Institute: Farmington Hills, MI, USA, 2019.
- BS EN 1992–1–1:2004; Eurocode 2: Design of Concrete Structures. British Standards Institution: Brussels, Belgium, 2004.
- CSA A23.3-19; Design of Concrete Structures. Canadian Standards Association: Mississauga, ON, Canada, 2019.
- Zhou, L.Y.; Liu, Z.; He, Z.Q. Elastic-to-Plastic Strut-and-Tie Model for Deep Beams. J. Bridge Eng. 2018, 23, 04018007. [Google Scholar] [CrossRef]
- Chen, H.; Yi, W.J. Evaluation and modification of strut-and-tie model for simply-supported and continuous deep reinforced concrete beams without stirrups. J. Build. Struct. 2019, 40, 154–161. [Google Scholar]
- Zhou, M.; Zhong, J.T.; Wang, L.; Chen, H.T. Application of Evaluation System for Strut-and-Tie Models of Reinforced Concrete Structures. ACI Struct. J. 2021, 118, 17–30. [Google Scholar]
- Chetchotisak, P.; Teerawong, J.; Yindeesuk, S. Modified interactive strut-and-tie modeling of reinforced concrete deep beams and corbels. Structures 2022, 45, 284–298. [Google Scholar] [CrossRef]
- Yi, W.J.; Li, Y.; Chen, H.; Ma, Z.J.; Zhou, K.J.; Huang, Y.; Zhou, Y. Shear strength evaluation of RC D-Regions based on Single-Panel Strut-and-Tie model. Eng. Struct. 2022, 265, 114500. [Google Scholar] [CrossRef]
- Hwang, S.; Lee, H. Analytical model for predicting shear strengths of interior reinforced concrete beam-column joints for seismic resistance. ACI Struct. J. 2000, 97, 35–44. [Google Scholar]
- Hwang, S.; Lu, W.; Lee, H. Shear strength prediction for deep beams. ACI Struct. J. 2000, 97, 367–376. [Google Scholar]
- Hwang, S.J.; Fang, W.H.; Lee, H.J.; Yu, H.W. Analytical model for predicting shear strength of squat walls. J. Struct. Eng. 2001, 127, 43–50. [Google Scholar] [CrossRef]
- Hwang, S.J.; Lee, H.J. Strength prediction for discontinuity regions by softened strut-and-tie model. J. Struct. Eng. 2002, 128, 1519–1526. [Google Scholar] [CrossRef]
- Khosravikia, F.; Kim, H.S.; Yi, Y.; Wilson, H.; Yousefpour, H.; Hrynyk, T.; Bayrak, O. Experimental and numerical assessment of corbels designed based on strut-and-tie provisions. J. Struct. Eng. 2018, 144, 04018138. [Google Scholar] [CrossRef]
- Canha, R.M.F.; Kuchma, D.A.; El Debs, M.K.; de Souza, R.A. Numerical analysis of reinforced high strength concrete corbels. Eng. Struct. 2014, 74, 130–144. [Google Scholar] [CrossRef]
- Ding, B.Y. Calculation of Reinforced Concrete Corbels. Ind. Constr. 1974, 2, 31–36+51. [Google Scholar]
- He, Z.Q.; Liu, Z.; Ma, Z.J. Investigation of Load-Transfer Mechanisms in Deep Beams and Corbels. ACI Struct. J. 2012, 109, 467–476. [Google Scholar]
- Shen, Y. The Research of Shear Bearing Capacity of Reinforced Concrete Deep Beam Based on the Softened Strut-and-Tie Model. Master’s Thesis, Beijing Jiaotong University, Beijing, China, 2016. [Google Scholar]
- Hwang, S.J.; Lu, W.Y.; Lee, H.J. Shear strength prediction for reinforced concrete corbels. ACI Struct. J. 2000, 97, 543–552. [Google Scholar]
- Gao, D.Y.; Shi, K.; Zhao, S.B. Calculation method for shear capacity of steel fiber reinforced concrete beam-column joints based on softened strut-and-tie model. China Civ. Eng. J. 2014, 47, 101–109. [Google Scholar]
- Romualdi, J.P.; Mandel, J.A. Tensile strength of concrete affected by uniformly distributed and closely spaced short lengths of wire reinforcement. ACI Mater. J. 1964, 61, 657–672. [Google Scholar]
- Schấfer, K. Strut-and-Tie Models for the Design of Structural Concrete. National Cheng Kung University: Tainan, Taiwan, 1996. [Google Scholar]
- Zhang, L.X.B.; Hsu, T.T.C. Behavior and analysis of 100 MPa concrete membrane elements. J. Struct. Div. 1998, 124, 24–34. [Google Scholar] [CrossRef]
- Zhu, M.Q.; Fang, Z.; Chen, H.Y. Torsional behavior of reinforced SFHSC thin-walled box girder. J. Build. Struct. 2005, 26, 108–113. [Google Scholar]
- Tang, X.R.; Jiang, Y.S.; Ding, D.J. Application of the Theory of Softened Truss to Low-Rise Steel Fiber High Strength Concrete Shear Walls. J. Build. Struct. 1993, 14, 2–11. [Google Scholar]
- Foster, S.J.; Voo, J. Variable Engagement Model for Fibre Reinforced Concrete in Tension; University of New South Wales: Sydney, Australia, 2003. [Google Scholar]
- Khuntia, M.; Stojadinovic, B.; Goel, S.C. Shear strength of normal and high-strength fiber reinforced concrete beams without stirrups. ACI Struct. J. 1999, 96, 282–289. [Google Scholar]
- Cui, L.H. Behavior and Bearing Capacity of Steel Fiber Reinforced High-Strength Concrete Shear Wall. Master’s Thesis, Zhengzhou University, Zhengzhou, China, 2017. [Google Scholar]
- Wu, T.; Xing, G.H.; Liu, B.Q.; Huang, H. Shear Strength of RC Frame Joints Connecting Beams with Different Depths Using Softened Strut-and-Tie Model. Eng. Mech. 2010, 27, 201–208. [Google Scholar]
- Zhang, L.; Jirsa, J.O. A Study of Shear Behavior of Reinforced Concrete Beam-Column Joints; Phil M. Ferguson Structural Engineering Laboratory, University of Texas: Austin, TX, USA, 1982. [Google Scholar]
- Vecchio, F.J.; Collins, M.P. Compression response of cracked reinforced concrete. J. Struct. Div. 1993, 119, 3590–3610. [Google Scholar] [CrossRef]






| Specimen Number | Longitudinal Reinforcement | (%) | Stirrup | (%) | (%) | |
|---|---|---|---|---|---|---|
| CW01 | 0.2 | 4C12 | 0.55 | A10@100 | 0.78 | 1.50 |
| CW02 | 0.3 | 4C12 | 0.55 | A10@100 | 0.78 | 1.50 |
| CW03 | 0.4 | 4C12 | 0.55 | A10@100 | 0.78 | 1.50 |
| CW04 | 0.5 | 4C12 | 0.55 | A10@100 | 0.78 | 1.50 |
| CW05 | 0.3 | 4C12 | 0.55 | A10@100 | 0.78 | 0.75 |
| CW06 | 0.3 | 4C12 | 0.55 | A10@100 | 0.78 | 0 |
| (%) | Water (kg) | Cement (kg) | Sand (kg) | Pebble (kg) | Water Reducer (kg) | Steel Fiber (kg) | Gross Mass (kg) |
|---|---|---|---|---|---|---|---|
| 0 | 61.0 | 203.4 | 249.0 | 483.4 | 3.05 | 0 | 1000 |
| 0.75 | 65.1 | 216.9 | 285.7 | 428.8 | 3.26 | 23.80 | 1000 |
| 1.5 | 69.9 | 233.1 | 277.3 | 415.6 | 3.50 | 47.59 | 1000 |
| (%) | (MPa) | (MPa) | (MPa) | (MPa) |
|---|---|---|---|---|
| 0 | 73.2 | 55.9 | 4.0 | 38.7 |
| 0.75 | 69.8 | 51.7 | 3.3 | 37.6 |
| 1.5 | 72.6 | 49.8 | 3.6 | 37.2 |
| Reinforcement Type | (MPa) | (GPa) | (MPa) | ||
|---|---|---|---|---|---|
| A10 | 78.5 | 333.7 | 195.95 | 535.8 | 1703 |
| C12 | 113.1 | 425.2 | 172.60 | 541.4 | 2463 |
| Specimen Number | (%) | (kN) | (kN) | (kN) | (%) | (%) | Failure Mode | |
|---|---|---|---|---|---|---|---|---|
| CW01 | 0.2 | 1.50 | 210.0 | 460.0 | 1009.5 | 20.8 | 45.6 | Shear failure |
| CW02 | 0.3 | 1.50 | 190.0 | 429.0 | 852.0 | 22.3 | 50.4 | Diagonal compression |
| CW03 | 0.4 | 1.50 | 142.0 | 333.0 | 781.5 | 18.2 | 42.6 | Diagonal compression |
| CW04 | 0.5 | 1.50 | 120.0 | 310.0 | 767.5 | 15.6 | 40.4 | Diagonal compression |
| CW05 | 0.3 | 0.75 | 150.0 | 418.0 | 755.0 | 19.9 | 55.4 | Diagonal compression |
| CW06 | 0.3 | 0 | 160.0 | 395.0 | 765.0 | 20.9 | 51.6 | Diagonal compression |
| Formula Source | Calculation Formulas | Parameters |
|---|---|---|
| Chinese code [12] (GB50010-2010) | are the vertical load and the horizontal load acting on the top of the corbel, respectively; is the total area of longitudinal tensile reinforcements; is the yield strength of longitudinal tensile reinforcement; is the effective depth of corbel section; is the horizontal distance between vertical load and the edge of the corbel lower column. | |
| American code [13] (ACI318-19) | is the strut and node confinement modification factor; is the strut effective coefficient; is the compressive strength of concrete cylinders; is the section width of the corbel; is the width of strut; is the angle between strut and horizontal direction; is the vertical distance between the top node center and the bottom node center; is the width of the loading plate; are, respectively, the height of the node at the loading area and at the supporting area, and considering the simplicity of the calculation, the values of the two are equal (). | |
| European code [14] (EN 1992-1-1) | is the compressive stress in the concrete, the values of which are two cases: ① (when there is no transverse tensile stress or compressive stress); ② is the strength reduction factor for concrete cracked in shear; is the design value of concrete compressive strength; is the coefficient taking account of long-term effects on the compressive strength and of unfavorable effects resulting from the way the load is applied; is the partial safety factor for concrete; is the characteristic compressive cylinder strength of concrete at 28 days. | |
| Canadian code [15] (CSA A23.3-19) | is the resistance factor for concrete, taken as 1; is the limiting compressive stress in concrete strut; is the smallest angle between compressive strut and adjoining tensile ties; is the principal tensile strain in cracked concrete due to factored loads; to concrete strut, which ranges from 0.0012 to 0.0038; particularly, when the specified yield strength of the steel bar does not exceed 400 MPa, . |
| Specimen Number | (kN) | (kN) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| GB10 | ACI | EC2 | CSA | MSSTM | SMSSTM | GB10 | ACI | EN2 | CSA | MSSTM | SMSSTM | ||
| CW01 | 1009.5 | 858 | 591 | 515 | 740 | 1077 | 849 | 1.177 | 1.709 | 1.961 | 1.365 | 0.937 | 1.190 |
| CW02 | 852.0 | 572 | 605 | 528 | 733 | 968 | 828 | 1.490 | 1.407 | 1.615 | 1.162 | 0.880 | 1.029 |
| CW03 | 781.5 | 429 | 607 | 529 | 703 | 859 | 801 | 1.822 | 1.287 | 1.477 | 1.112 | 0.910 | 0.976 |
| CW04 | 767.5 | 343 | 598 | 521 | 655 | 630 | 738 | 2.236 | 1.284 | 1.473 | 1.171 | 1.218 | 1.039 |
| CW05 | 755.0 | 572 | 582 | 548 | 705 | 905 | 812 | 1.320 | 1.297 | 1.378 | 1.071 | 0.834 | 0.930 |
| CW06 | 765.0 | 572 | 610 | 592 | 739 | 756 | 831 | 1.337 | 1.253 | 1.292 | 1.035 | 1.012 | 0.921 |
| Mean | 1.564 | 1.373 | 1.533 | 1.153 | 0.965 | 1.014 | |||||||
| Variance | 0.131 | 0.025 | 0.046 | 0.011 | 0.016 | 0.008 | |||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.-S.; Peng, D.; Wang, H.; Zhang, F.-J.; Li, H.-M.; Xie, Y.-J.; Chen, A.-J.; Xie, W. Analysis of Shear Model for Steel-Fiber-Reinforced High-Strength Concrete Corbels with Welded-Anchorage Longitudinal Reinforcement. Materials 2023, 16, 4907. https://doi.org/10.3390/ma16144907
Li S-S, Peng D, Wang H, Zhang F-J, Li H-M, Xie Y-J, Chen A-J, Xie W. Analysis of Shear Model for Steel-Fiber-Reinforced High-Strength Concrete Corbels with Welded-Anchorage Longitudinal Reinforcement. Materials. 2023; 16(14):4907. https://doi.org/10.3390/ma16144907
Chicago/Turabian StyleLi, Shu-Shan, Die Peng, Heng Wang, Feng-Jian Zhang, Hong-Mei Li, Yi-Jun Xie, Ai-Jiu Chen, and Wei Xie. 2023. "Analysis of Shear Model for Steel-Fiber-Reinforced High-Strength Concrete Corbels with Welded-Anchorage Longitudinal Reinforcement" Materials 16, no. 14: 4907. https://doi.org/10.3390/ma16144907
APA StyleLi, S.-S., Peng, D., Wang, H., Zhang, F.-J., Li, H.-M., Xie, Y.-J., Chen, A.-J., & Xie, W. (2023). Analysis of Shear Model for Steel-Fiber-Reinforced High-Strength Concrete Corbels with Welded-Anchorage Longitudinal Reinforcement. Materials, 16(14), 4907. https://doi.org/10.3390/ma16144907






