Study on Crystallization Process of Li2O–Al2O3–SiO2 Glass-Ceramics Based on In Situ Analysis
Abstract
1. Introduction
2. Experiment
3. Results and Discussion
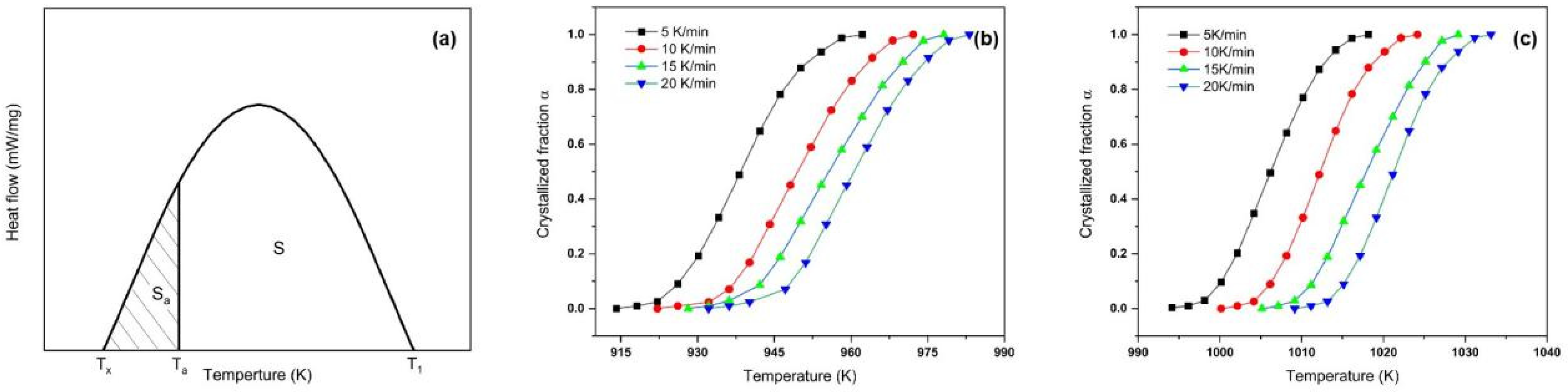
3.1. Differential Scanning Calorimetry (DSC)
3.2. Kine
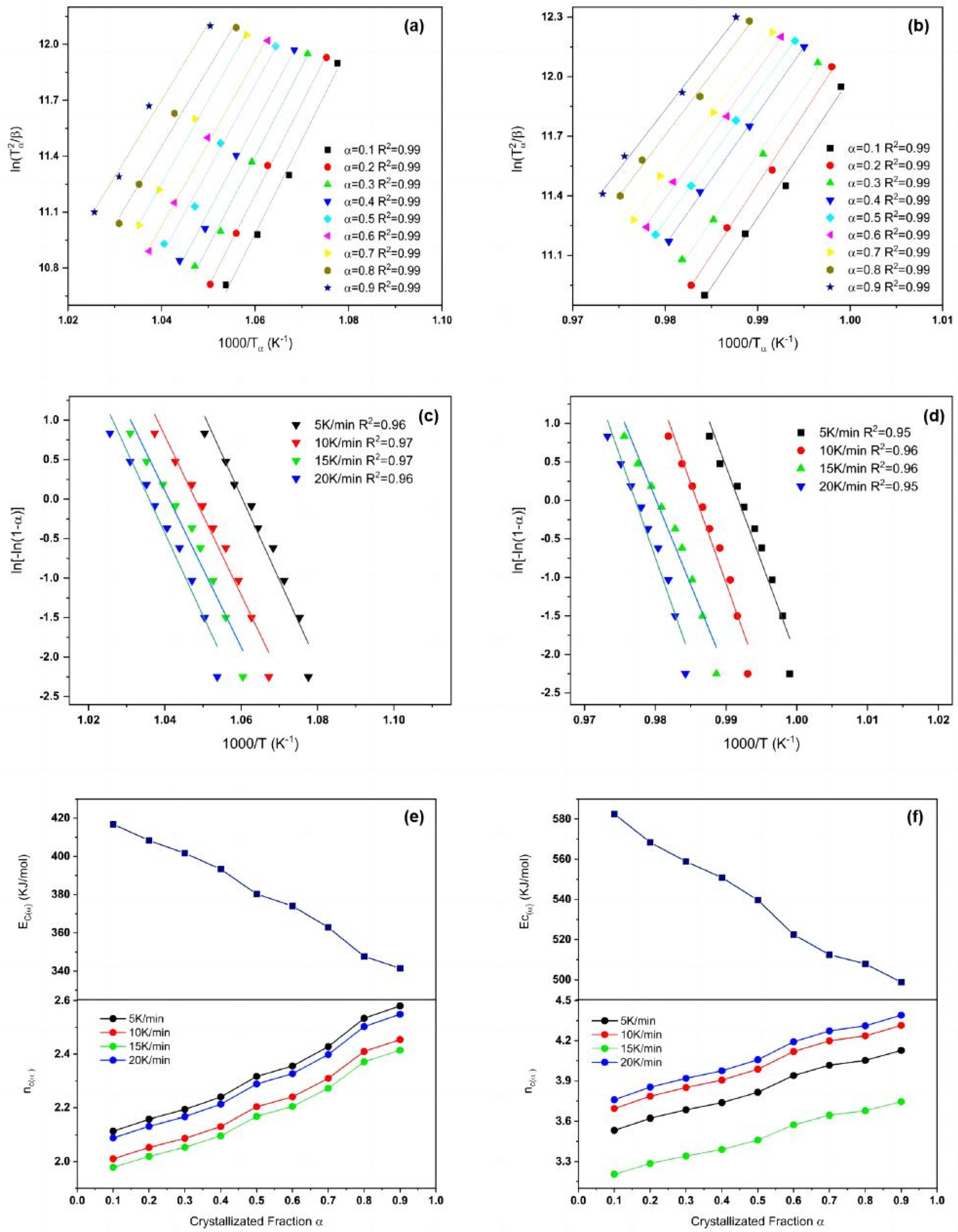
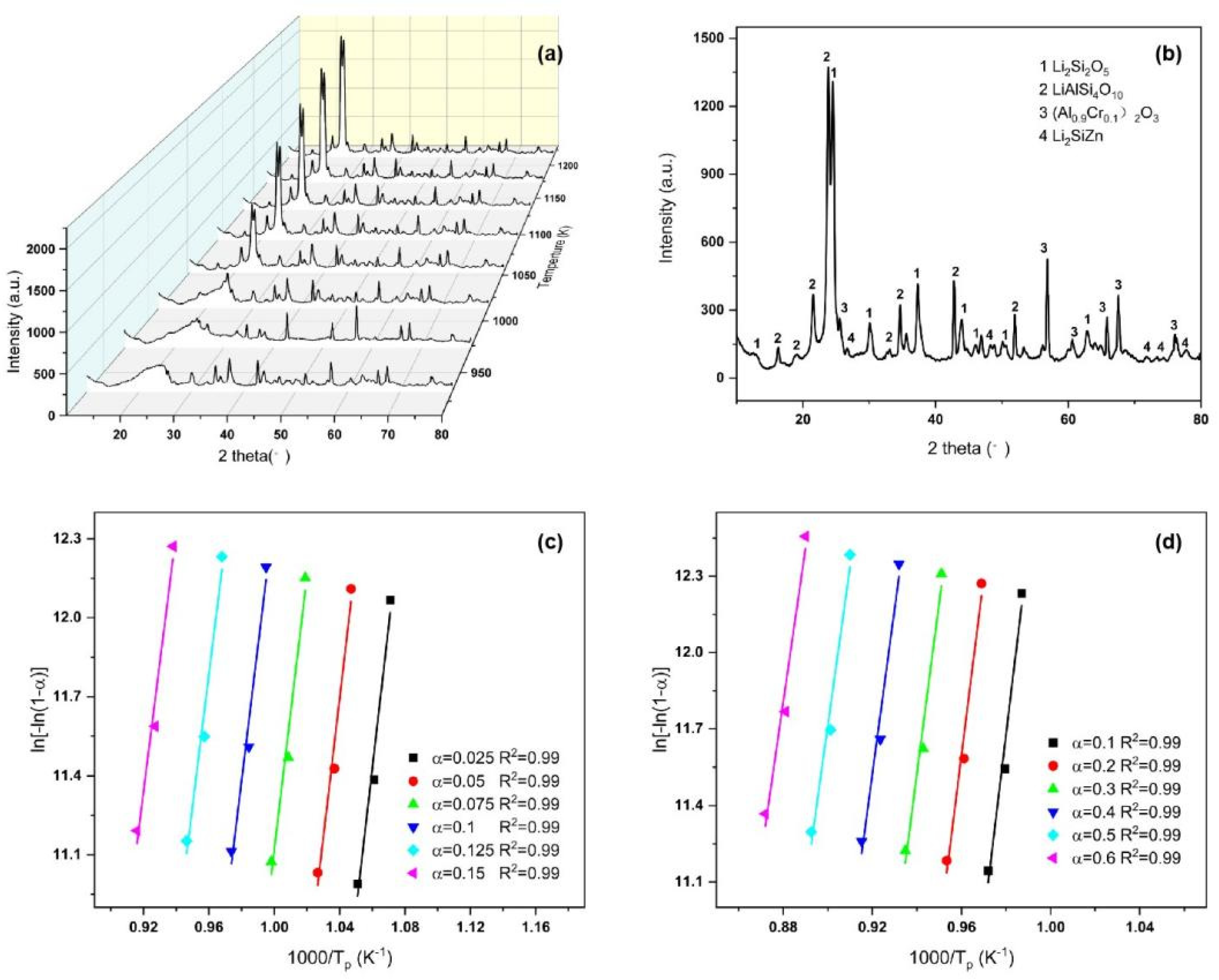
3.2.1. Kissinger Method
3.2.2. Kissinger–Akahira–Sunose (KAS) Method
3.3. HT-XRD
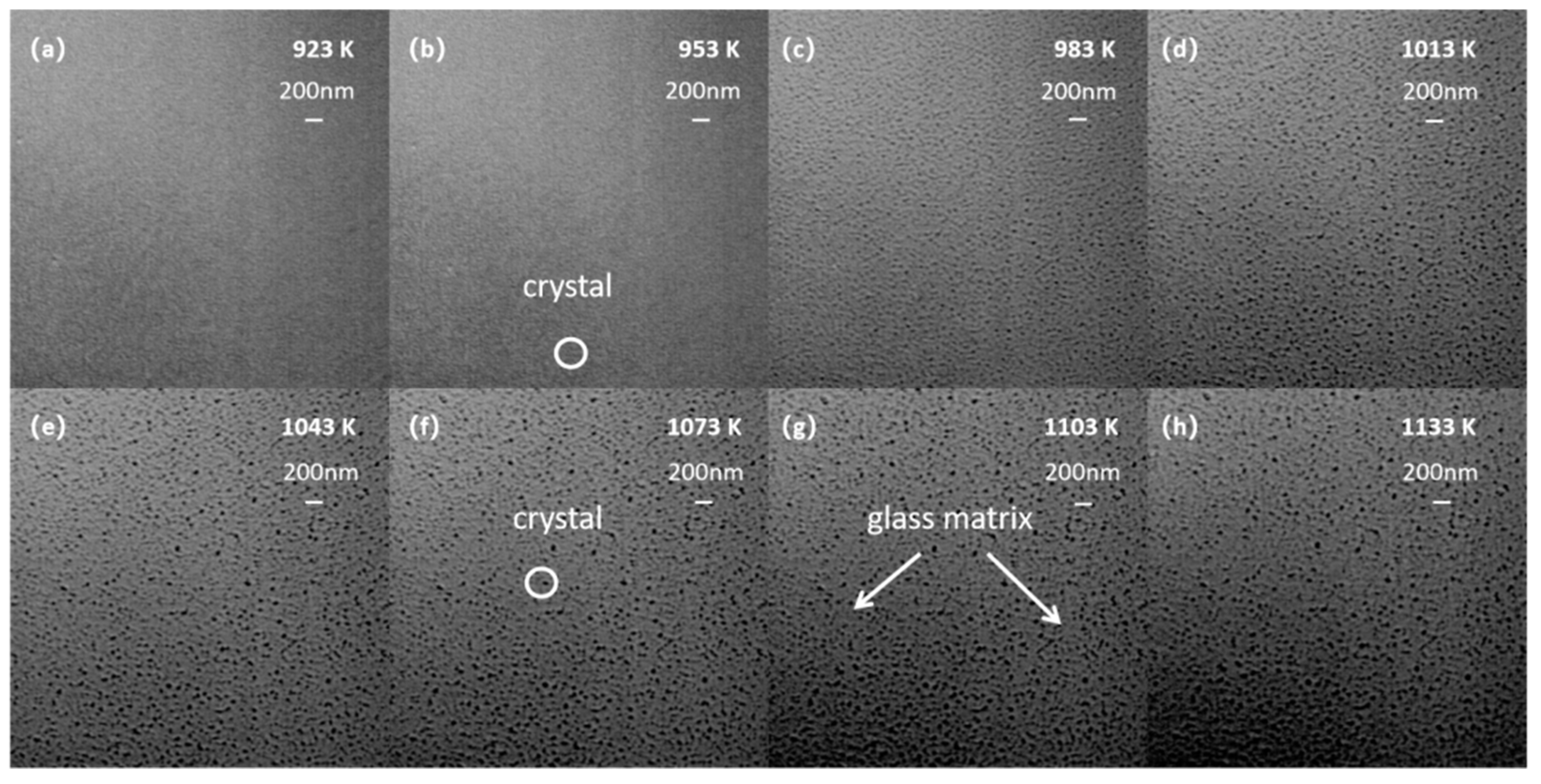
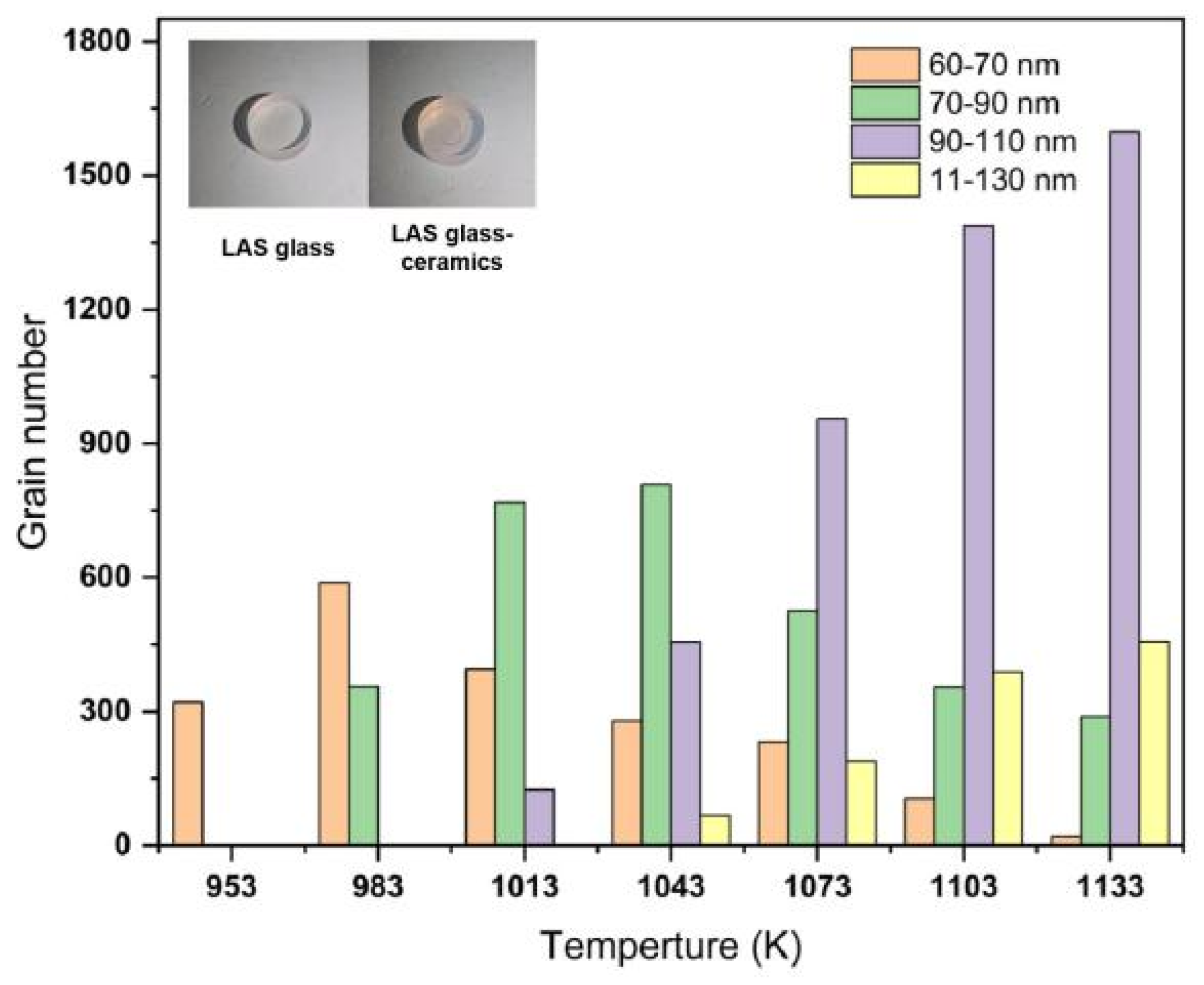
3.4. CLSM
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Zanotto, E.D. A bright future for glass-ceramics. Am. Ceram. Soc. Bull. 2010, 89, 19–27. [Google Scholar]
- Holand, W.; Beall, G.H. Glass Ceramic Technology; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar] [CrossRef]
- Chen, M.; He, F.; Shi, J. Low Li2O content study in Li2O-Al2O3-SiO2 glass-ceramics. J. Eur. Ceram. Soc. 2019, 39, 4988–4995. [Google Scholar] [CrossRef]
- Beall, G.H. Design and properties of glass-ceramics. Annu. Rev. Mater. Sci. 1992, 22, 91–119. [Google Scholar] [CrossRef]
- Roy, R.; Agrawal, D.K.; McKinstry, H.A. Very low thermal expansion coefficient materials. Annu. Rev. Mater. Sci. 1989, 19, 59–81. [Google Scholar] [CrossRef]
- Sava, B.A.; Elisa, M.; Bartha, C.; Iordanescu, R.; Feraru, I.; Plapcianu, C.; Patrascu, R. Non-isothermal free-models kinetic analysis on crystallization of europium-doped phosphate glasses. Ceram. Int. Part A 2014, 40, 12387–12395. [Google Scholar] [CrossRef]
- Al-Harbi, O.A. Influence of TiO2 and Cr2O3 on crystallinity and properties of Ca, Li aluminosilicate glass. Adv. Appl. Ceram. 2007, 106, 235–240. [Google Scholar] [CrossRef]
- Guo, Y.; Wang, J.; Jian, R.; Han, J.; Xie, J.; Liu, C. Microstructure and ion-exchange properties of glass-ceramics containing ZnAl2O4 and β-quartz solid solution nanocrystals. J. Eur. Ceram. Soc. 2021, 41, 5331–5340. [Google Scholar] [CrossRef]
- Kisilev, A.; Reisfeld, R.; Greenberg, E.; Buch, A.; Ish-Shalom, M. Spectroscopy of chromium(III) in β-quartz and petalite-like transparent glass ceramics: Ligand field strengths of chromium(III). Chem. Phys. Lett. 1984, 105, 405–408. [Google Scholar] [CrossRef]
- Kraipok, A.; Mamanee, T.; Ruangsuriya, J.; Leenakul, W. Investigation of phase formation and mechanical properties of lithium disilicate glass-ceramic doped CeO2. J. Non-Cryst. Solids 2021, 561, 120772. [Google Scholar] [CrossRef]
- Lilensten, L.; Fu, Q.; Wheaton, B.R.; Credle, A.J.; Stewart, R.L.; Kohli, J.T. Kinetic study on lithium-aluminosilicate (LAS) glass-ceramics containing MgO and ZnO. Ceram. Int. Part A 2014, 40, 11657–11661. [Google Scholar] [CrossRef]
- Montedo, O.R.K.; Hotza, D.; de Oliveira, A.P.N.; Meszaros, R.; Travitzky, N.; Greil, P. Crystallisation kinetics of a β-spodumene-based glass ceramic. Adv. Mater. Sci. Eng. 2012, 2012, 1–8. [Google Scholar] [CrossRef]
- Cheng, K. Determining crystallization kinetic parameters of Li2O–Al2O3–SiO2 glass from derivative differential thermal analysis curves. Mater. Sci. Eng. B 1999, 60, 194–199. [Google Scholar] [CrossRef]
- Guo, X.Z.; Yang, H.; Cao, M.; Han, C.; Song, F.F. Crystallinity and crystallization mechanism of lithium aluminosilicate glass by X-ray diffractometry. Trans. Nonferrous Met. Soc. China 2006, 16, 593–597. [Google Scholar] [CrossRef]
- Abu El-Oyoun, M. Effect of wide range of heating rate on the crystallization kinetic parameters of Se77Te2OSb3 glass. Thermochim. Acta 2009, 494, 129–135. [Google Scholar] [CrossRef]
- Cheng, Y. Study on Structure and Crystallization of Advanced Borate Glasses. Ph.D. Thesis, Hunan University, Changsha, China, 2006; p. 23. [Google Scholar]
- Kumar, A.; Chakrabarti, A.A.; Shekhawat, M.S. Transparent Ultra-Low Expansion Lithium Aluminosilicate Glass-Ceramics: Crystallization Kinetics, Structural and Optical Properties. Thermochim. Acta 2019, 676, 155–163. [Google Scholar] [CrossRef]
- Li, H.; Yang, L.; Cao, C.; Tao, Y.; Zhong, Q.; Xu, Z.; Luo, C.; Wang, W. In situ observation of Li2O-Al2O3-SiO2 glass crystallization process and kinetics analysis. J. Cryst. Growth 2020, 547, 125816. [Google Scholar] [CrossRef]
- Feichtinger, S.; Michelic, S.K.; Kang, Y.-B.; Bernhard, C. In Situ Observation of the Dissolution of SiO2 Particles in CaO-Al2O3-SiO2 Slags and Mathematical Analysis of its Dissolution Pattern. J. Am. Ceram. Soc. 2014, 97, 316–325. [Google Scholar] [CrossRef]
- Liu, L.; Hu, M.; Xu, Y.; Bai, C.; Gan, Y. Structure, Growth Process, and Growth Mechanism of Perovskite in High-Titanium-Bearing Blast Furnace Slag. Met. Mater. Trans. B. 2015, 46, 1751–1759. [Google Scholar] [CrossRef]
- Savabieh, H.; Alizadeh, P.; Nayebi, B.; Clemens, F.J. Kinetics of crystallization in 13.2Li2O-67.6SiO2-14.49Al2O3-3.3TiO2-0.4BaO-0.97ZnO glass ceramic powder: Part I: A model-free vs. model-fitting approach. Ceram. Int. Part A 2019, 45, 8856–8865. [Google Scholar] [CrossRef]
- Kissinger, H.E. Variation of peak temperature with heating rate in differential thermal analysis. J. Res. Natl. Bur. Stand. 1956, 57, 217–221. [Google Scholar] [CrossRef]
- Shi, J.; He, F.; Xie, J.; Liu, X.; Yang, H. Kinetic analysis of crystallization in Li2O-Al2O3-SiO2-B2O3-BaO glass-ceramics. J. Non-Cryst. Solids 2018, 491, 106–113. [Google Scholar] [CrossRef]
- Dressler, M.; Rüdinger, B.; Deubener, J. An In Situ High-Temperature X-Ray Diffraction Study of Early-Stage Crystallization in Lithium Alumosilicate Glass-Ceramics. J. Am. Ceram. Soc. 2011, 94, 1421–1426. [Google Scholar] [CrossRef]
- Shackelford, J.F.; Han, Y.-H.; Kim, S.; Kwon, S.-H. CRC Materials Science and Engineering Handbook; CRC Press: Boca Raton, FL, USA, 1994. [Google Scholar]
- Augis, J.A.; Bennett, J.E. Calculation of the Avrami parameters for heterogeneous solid state reactions using a modification of the Kissinger method. J. Therm. Anal. 1978, 13, 283–292. [Google Scholar] [CrossRef]
- Abu-Sehly, A.A.; Alamri, S.N.; Joraid, A.A. Measurements of DSC isothermal crystallization kinetics in amorphous selenium bulk samples. J. Alloy. Compd. 2009, 476, 348–351. [Google Scholar] [CrossRef]
- Liu, F.; Sommer, F.; Bos, C.; Mittemeijer, E.J. Analysis of solid state phase transformation kinetics: Models and recipes. Int. Mater. Rev. 2007, 52, 193–212. [Google Scholar] [CrossRef]
- Joraid, A.A.; Alamri, S.N.; Abu-Sehly, A.A. Model-free method for analysis of nonisothermal kinetics of a bulk sample of selenium. J. Non-Cryst. Solids 2008, 354, 3380–3387. [Google Scholar] [CrossRef]
- Arora, A.; Shaaban, E.R.; Singh, K.; Pandey, O. Non-isothermal crystallization kinetics of ZnO-BaO-B2O3-SiO2 glass. J. Non-Cryst. Solids 2008, 354, 3944–3951. [Google Scholar] [CrossRef]
- Ozawa, T. Applicability of Friedman plot. J. Therm. Anal. 1986, 31, 547–551. [Google Scholar] [CrossRef]
- Majhi, K.; Varma, K.B.R. Crystallization kinetic studies of CaBi2B2O7 glasses by nonisothermal methods. J. Mater. Sci. 2009, 44, 385–391. [Google Scholar] [CrossRef]
- Yinnon, H.; Uhlmann, D.R. Application of thermoanalytical techniques to the study of crystallization kinetics in glass-forming liquids, part I: Theory. J. Non-Cryst. Solids 1983, 54, 253–275. [Google Scholar] [CrossRef]
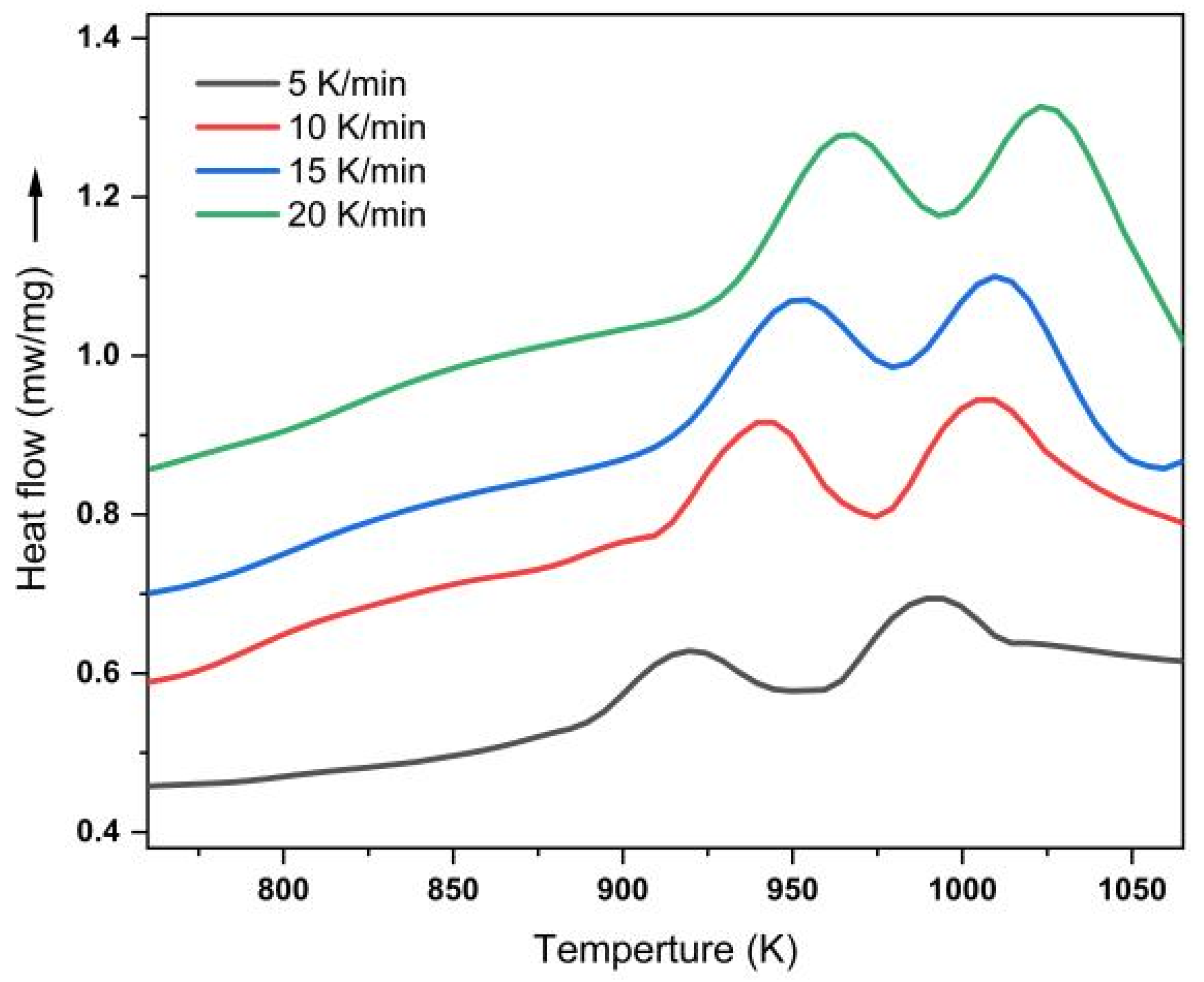

| Temperature (K) | The First Crystallization Peak | The Second Crystallization Peak | |||
|---|---|---|---|---|---|
| Heating Rate (K/min) | Tg | Tc1 | Tpc1 | Tc2 | Tpc2 |
| 5 | 789 | 918 | 934 | 994 | 1003 |
| 10 | 798 | 929 | 943 | 1000 | 1009 |
| 15 | 807 | 937 | 954 | 1006 | 1015 |
| 20 | 815 | 948 | 965 | 1011 | 1022 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, M.; Xiong, C.; Ma, Y.; Jiang, H. Study on Crystallization Process of Li2O–Al2O3–SiO2 Glass-Ceramics Based on In Situ Analysis. Materials 2022, 15, 8006. https://doi.org/10.3390/ma15228006
Li M, Xiong C, Ma Y, Jiang H. Study on Crystallization Process of Li2O–Al2O3–SiO2 Glass-Ceramics Based on In Situ Analysis. Materials. 2022; 15(22):8006. https://doi.org/10.3390/ma15228006
Chicago/Turabian StyleLi, Minghan, Chunrong Xiong, Yanping Ma, and Hong Jiang. 2022. "Study on Crystallization Process of Li2O–Al2O3–SiO2 Glass-Ceramics Based on In Situ Analysis" Materials 15, no. 22: 8006. https://doi.org/10.3390/ma15228006
APA StyleLi, M., Xiong, C., Ma, Y., & Jiang, H. (2022). Study on Crystallization Process of Li2O–Al2O3–SiO2 Glass-Ceramics Based on In Situ Analysis. Materials, 15(22), 8006. https://doi.org/10.3390/ma15228006





