1. Introduction
Functionally graded material (FGM) comes from the class of composite material with a gradual variation in their composition along a preferred direction. In 1984, FGM was introduced by Japanese material scientists as a composite material for thermal resistance [
1]. The continuous grading in the FGM plates results in the continuous material property variation across its thickness from one material phase to another material phase resulting in the uniform variation in the mechanical properties between both material phases. Laminated composite structures suffer from the abrupt change in the material properties at the separation layers of the laminae, which could result in transverse shear stress discontinuity causing delamination. These abrupt changes in transverse shear stress are avoided by the FGM due to its smooth and continuous gradation across the thickness. Many engineering areas, such as marine, aircraft, and automobile sectors utilize FGM extensively. With the increasing demand for FGM, there is a need to investigate and describe their behavior efficiently with more accurate computational methods.
Various studies have been undertaken to understand the structural behavior of FGM plates. Many theories were proposed to model the static response of the FGM plates under the application of the transverse load. Three types of theories can be distinguished: three-dimensional elastic theories, discrete layer theories, and equivalent single-layer theories. Three-dimensional elastic theories offer precise displacement and stress solutions. Vel [
2] proposed a precise three-dimensional solution for functionally graded plate vibrations. Kashtalyan [
3] suggested three-dimensional displacement and stress solutions for a functionally graded simply supported plate subjected to transverse loads. Jin et al. [
4] used three-dimensional elastic solutions for free vibrations of arbitrary thickness plates. Woodward and Kashtalyan [
5] considered exact three-dimensional solutions for transversely isotropic FGM plates. For the laminated composite plate analysis, Reddy [
6] suggested a simple higher-order shear deformation theory. For determining the stress and displacement of the FGM plate, Zhang et al. [
7] provided semi-analytical solutions. The finite element formulation of three-dimensional elastic theories for the static analysis of the FGM plate is quite complicated, and computing the results is extremely difficult and time-consuming. Various discrete theories have been presented to predict the behavior of composite laminates and compute displacement and stresses efficiently. The local deformation theory was used by Wu and Chen [
8] to analyze laminated composite plates. Cho et al. [
9] employed discrete layer deformation theory to analyze the plate vibration and buckling. Icardi and Ugo [
10] used an eight-node zig-zag element for the detection of stress and displacement in a laminated composite plate. The higher-order zig-zag theory was used by Ajay et al. [
11] to investigate the behavior of laminated composite and sandwich shells. These theories require a different set of unknowns for each layer of the laminated composite plates. Complex kinematic boundary conditions are usually recommended in these theories, which are substantially time-consuming and complicated for implementation to get accurate results.
At present, the equivalent single layer theories are preferably employed to develop the mathematical model for computing the displacement and stresses of the FGM plate. Kirchoff and Love proposed a simple classical plate theory for displacement and stress analysis [
12]. This theory does not account for the effect of shear; hence, it is used only for the analysis of thin plates. Reissner and Mindlin introduced the first-order shear deformation plate theory [
13] which considered the effect of shear but required additional computation of a shear correction factor. Various higher-order shear deformation theories (HSDT) [
14] have been proposed for the static analysis of FGM plates. HSDTs ensured traction-free boundary conditions on the plate’s top and bottom, eliminating the need to calculate the shear correction factor. For the static analysis of FGM plates, Talha and Singh [
15] utilized polynomial functions, Touratier [
16] used trigonometric functions, Thai et al. [
17] recommended inverse tangent function, Soldatos [
18] and Grover et al. [
19] applied the hyperbolic functions, Karama et al. [
20] used the exponential function, Li et al. [
21] proposed the combination of trigonometric functions and polynomial functions, and Mahi et al. [
22] applied the combination of hyperbolic function and polynomial functions as HSDT to describe the parabolic strain profile of the transverse shear strain.
Natarajan and Ganapathi [
23] proposed HSDT with 13 unknown functions, Nelson and Lorch [
24] presented HSDT with nine unknown functions, Neves et al. [
25] adopted nine unknown functions, and Mantari and Soares [
26] used six unknown functions for their HSDT. These theories were accurate, but computation was difficult because of the large number of unknown functions. Zenkour [
27] presented a trigonometric shear deformation theory for the analysis of functionally graded plates. Using higher-order shear deformation theory, Qian et al. [
28] calculated the static and dynamic deformation of a thick functionally graded elastic plate. Ferriera [
29] and Gooley et al. [
30] applied the MLPG method on thick FGM plates. Mechab et al. [
31] proposed a two-variable improved plate theory to study the behavior of functionally graded plates by reducing the number of shear deformation functions. Mahi et al. [
22] used five nodal unknowns to describe the hyperbolic shear deformation plate theory. The effect of stretching of thickness in the FGM plates and shell was investigated by Carrera et al. [
32] and Habib et al. [
33]. However, the equivalent single layer shear deformation theories generally use approximate mathematical formulations such as the Rayleigh–Ritz method and Fourier transformation to develop the solution, which is very specific to loading, shape, and displacement functions. Due to lack of generalization, inability to describe complicated shape and loading, and differences in findings when compared to 3-D solutions, numerical methods to simulate the behavior of functionally graded plates were developed.
To predict the behavior of the FGM plates, many researchers have chosen numerical methods. Singha et al. [
34] used high precision plate bending finite elements to examine the nonlinear behavior of FGM plates. Valizadeh et al. [
35] investigated the static and dynamic behavior of functionally graded plates using NURBs-based finite element analysis. Orakdogen et al. [
36] studied the coupling effect of the thickness and the FGM plate bending using the finite element method. Using the finite element model, Alshorbagy et al. [
37] examined the free vibration and flexure of the FGM plate. Pindera and Dunn [
38] presented the effect of the thermomechanical gradient on the FGM plate using Finite element modeling. For the investigation of the FGM plate, Nguyen et al. [
39] employed a triangular finite element computational algorithm. Can et al. [
40] applied finite element modeling for the calculation of the buckling load. Naghdabadi and Hosseini [
41] used a finite element-based model to analyze the FGM plates and shells. Talha and Singh [
15] incorporated the finite element method into HSDT to describe the FGM plate’s static and free vibration responses. Taj et al. [
42] modeled the FGM plate’s static response using nine node isoparametric finite elements with 10 nodal unknowns at each node.
From the literature review, it was observed that there is a need to develop the mathematical formulation with higher-order shear deformation theory considering the thickness stretching effect. Therefore, the hybrid hyperbolic and polynomial function based HSDT proposed by Mahi et al. [
22] is refined by incorporating the thickness stretching effect across the thickness to develop the mathematical formulation. In the present work, after mathematical model development, its finite element model implementation is undertaken. The C
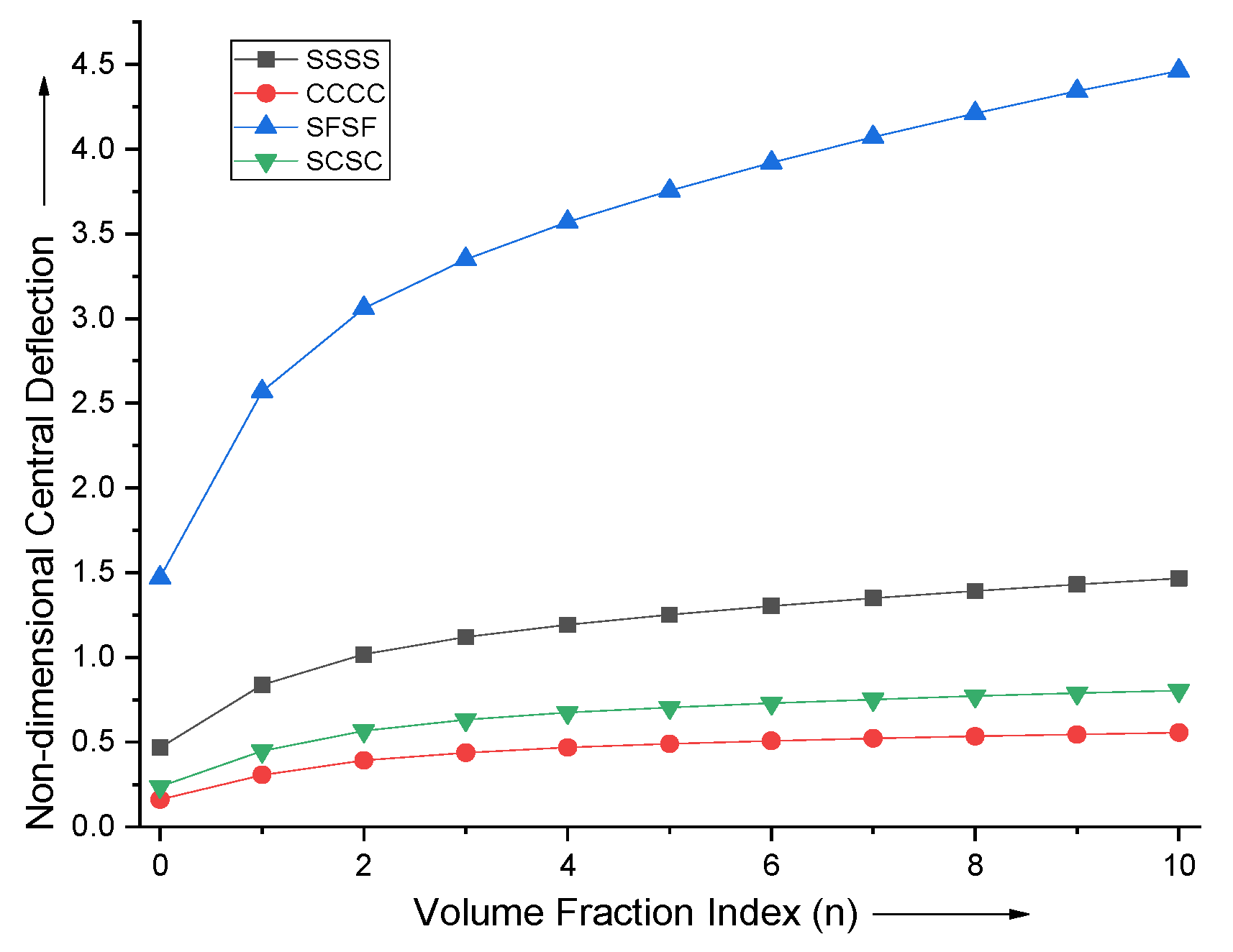
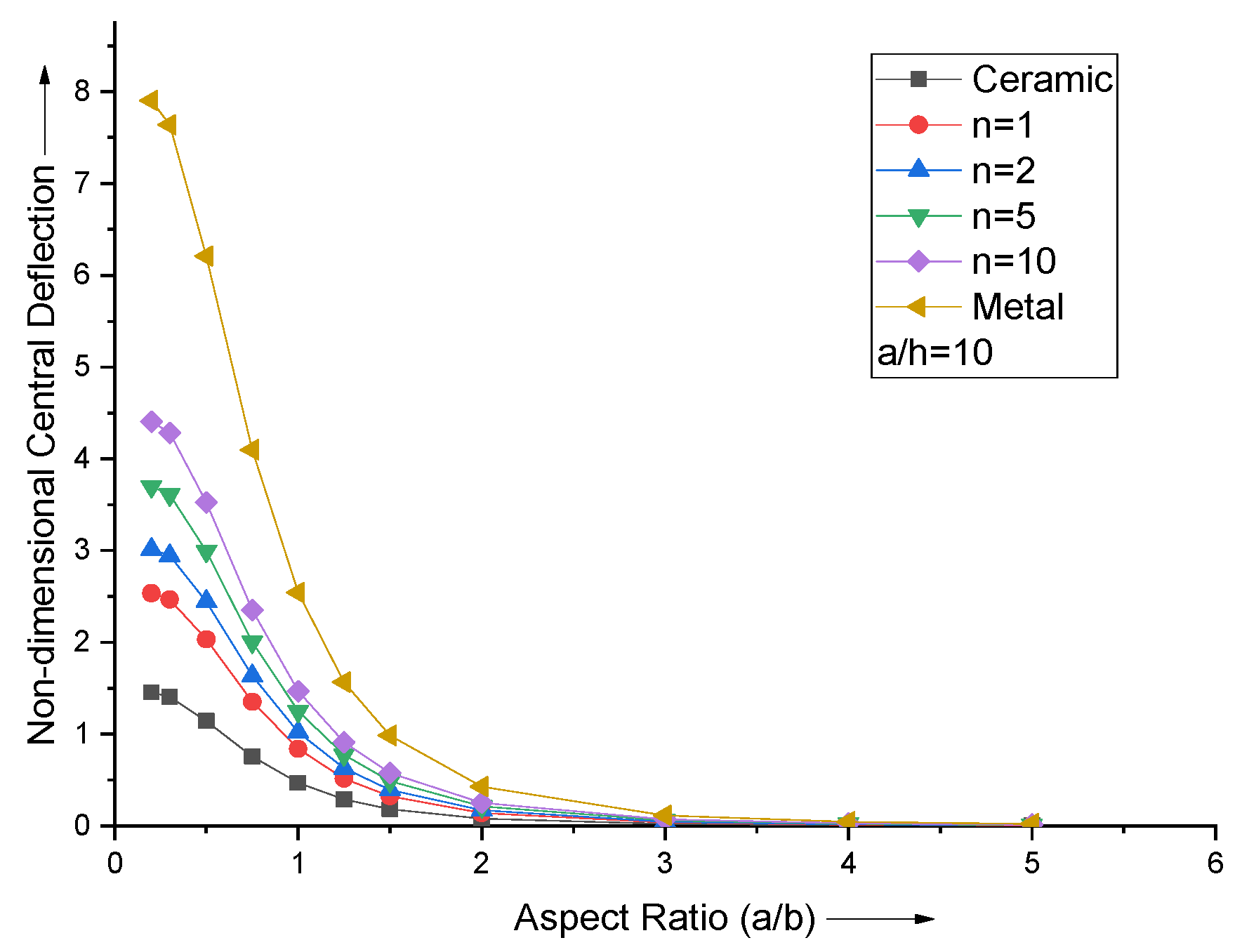
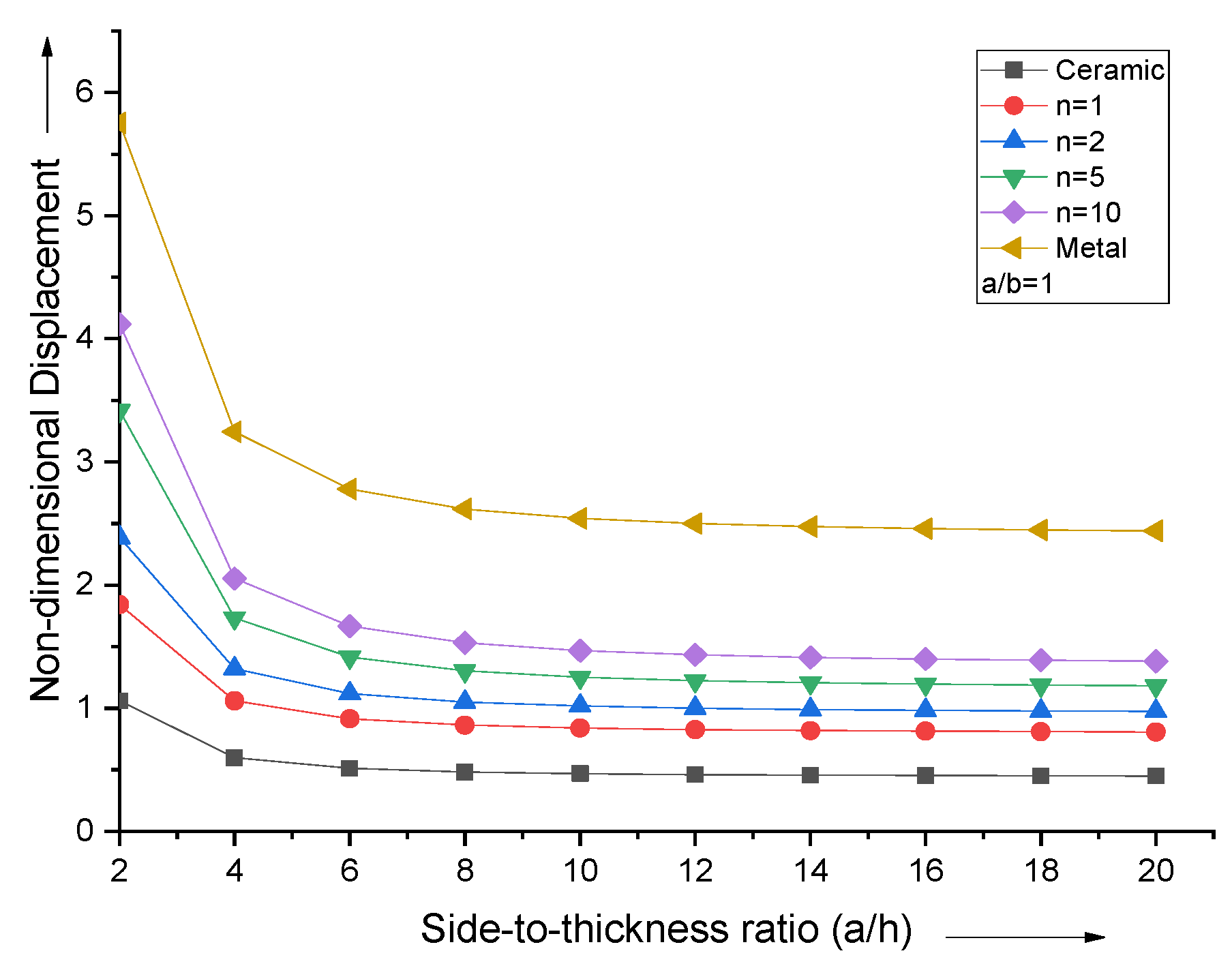
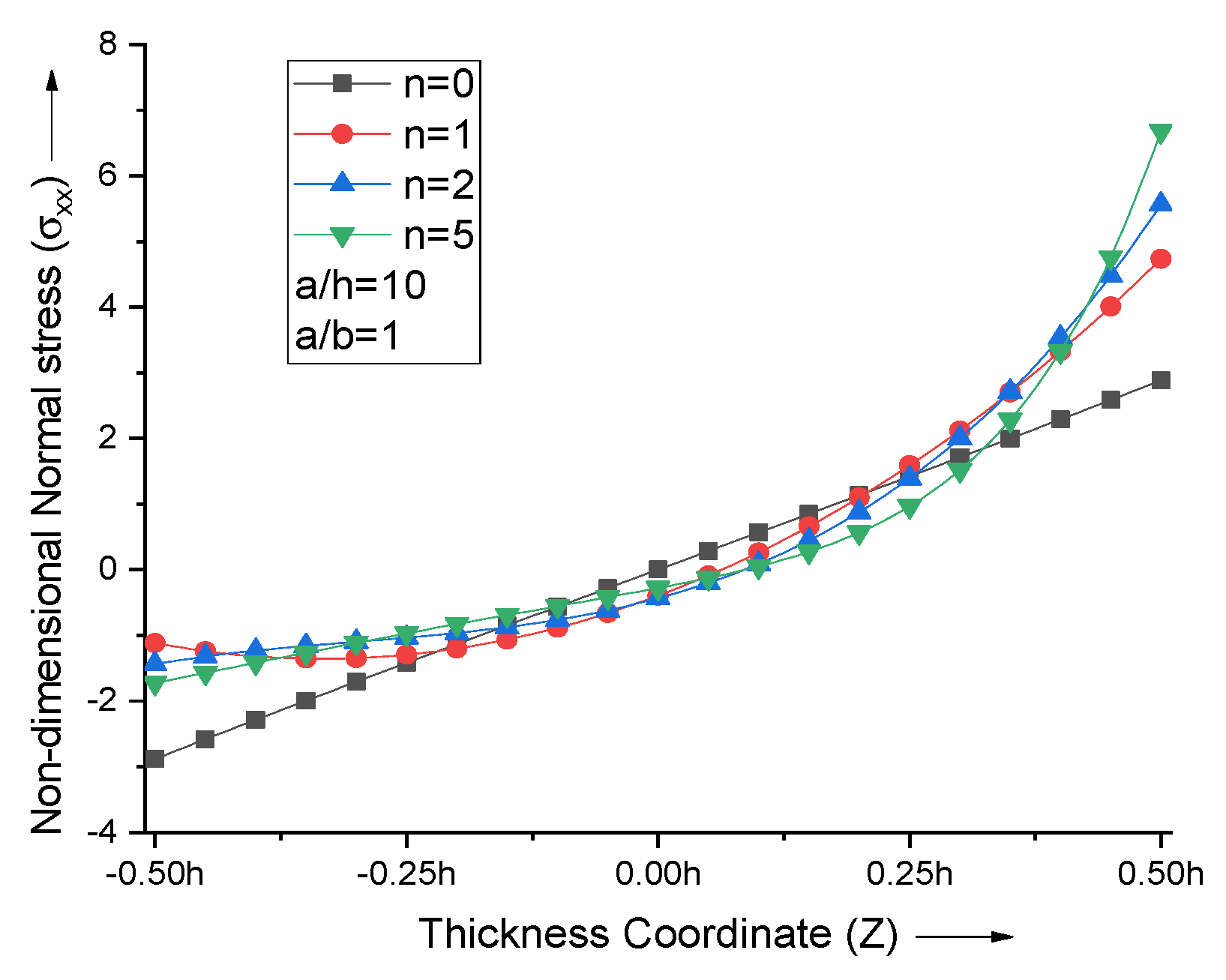
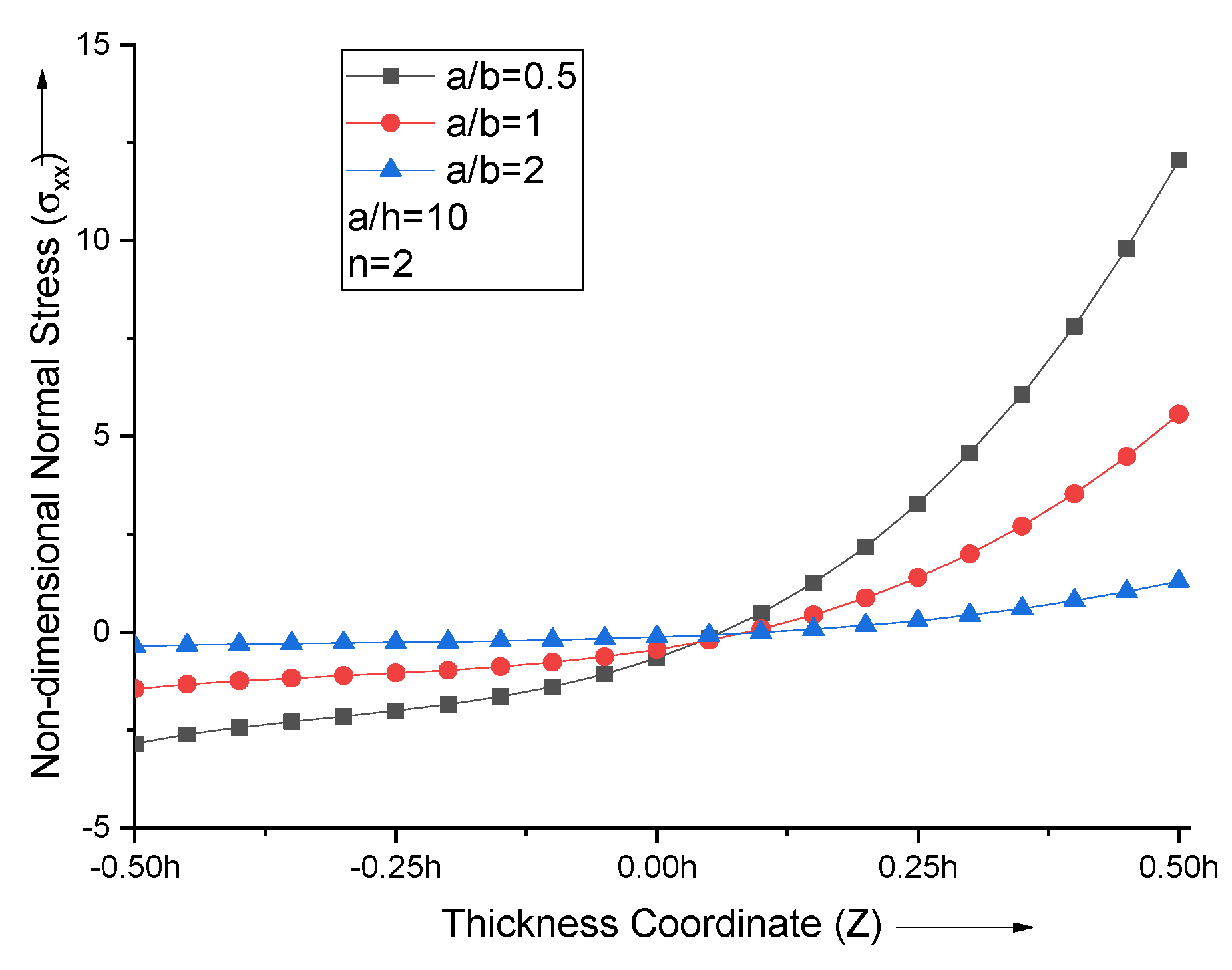
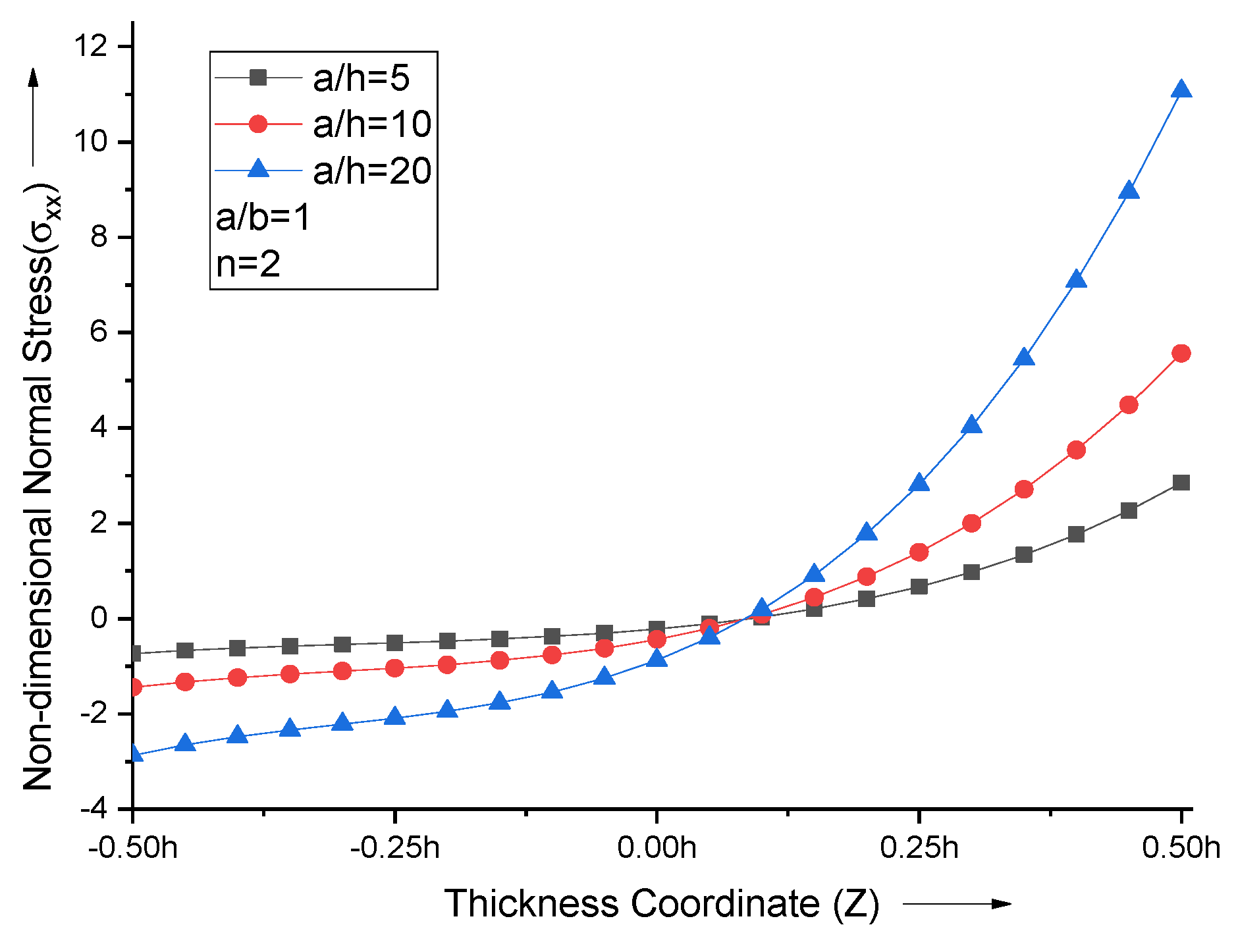
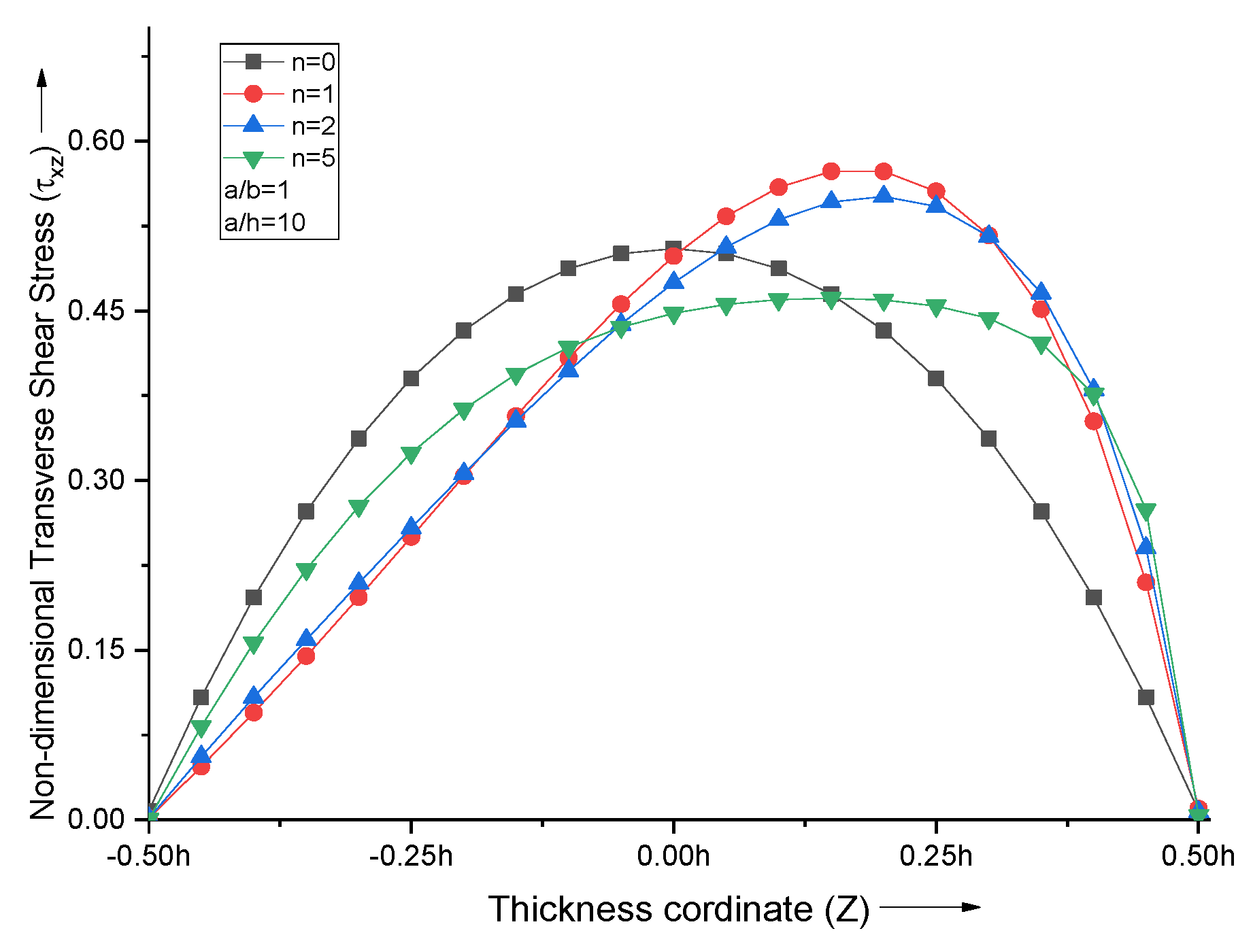
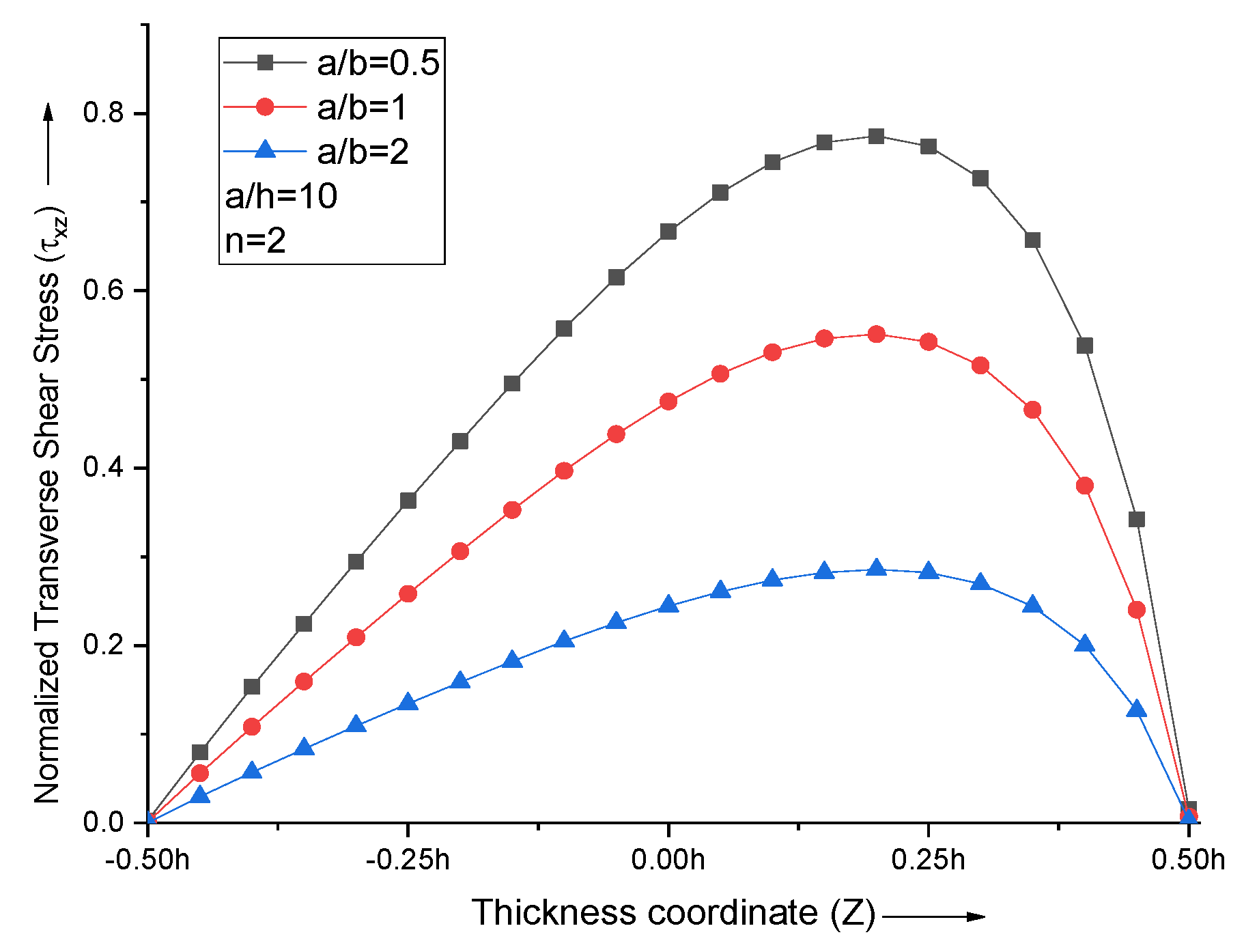
0 finite element model is developed by the authors for simplifying the computation of the displacements and stresses. The change in strain with plate thickness is addressed using an additional polynomial function, which was not implied in the earlier theory. The convergence study is performed to compute the displacement and stress results. The model is tested by analyzing the performance with published literature. The model is used for parametric investigations such as the fluctuation of non-dimensional displacement and stresses with the volume fraction index, aspect ratio, and plate depth.
3. Finite Element Formulation
The governing differential equation of the plate can be obtained using the application of the principal of virtual work on the strain energy U, external work W, and artificial constraint C of the FGM plate system as described in Equation (20).
The strain energy of the system can be expressed by Equation (21). The strain vectors can be represented as the product of thickness coordinate matrix [
H], differential operator matrix [B] described in
Appendix A, and mid-plane displacements {
Xo} described in Equation (22). Similarly, the work done by the external load is defined in Equation (23). The external load vector
q = [0 0 q
o 0 0 0 0 0] in which
qo (x, y) corresponds to the transverse load acting on the midplane.
The nine-node isoparametric Lagrangian shape functions were used in the development of the isoparametric finite element for the analysis of the FGM plate. The use of a nine-node element allows (3 × 3) a full integration scheme to be implemented and this results in improvement in the accuracy of the results. The midplane displacement can be interpolated using the isoparametric shape functions described in Equation (24).
The material rigidity matrix [D] is calculated using the constitutive matrix given in Equation (19) and thickness coordinate matrix [H]. This matrix helps in the implementation of the proposed HSDT as an equivalent single layer theory to convert the 3D domain to the 2D domain for the analysis.
Finally, the application of Equations (21)–(25) in Equation (20) results in the governing equation for the static analysis of the FGM plate described in Equation (26). The stiffness matrix [K], constraint stiffness matrix [K
c], and force matrix [F] can be derived from Equation (26) to establish the relationship between load and displacement expressed in Equation (28). Since the penalty approach was used for the finite element formulation, the effect of the artificial constraints on the displacements is negligible and the relationship K X
o = F holds true for the present static analysis.