Investigation of the Fatigue Stress of Orthotropic Steel Decks Based on an Arch Bridge with the Application of the Arlequin Method
Abstract
:1. Introduction
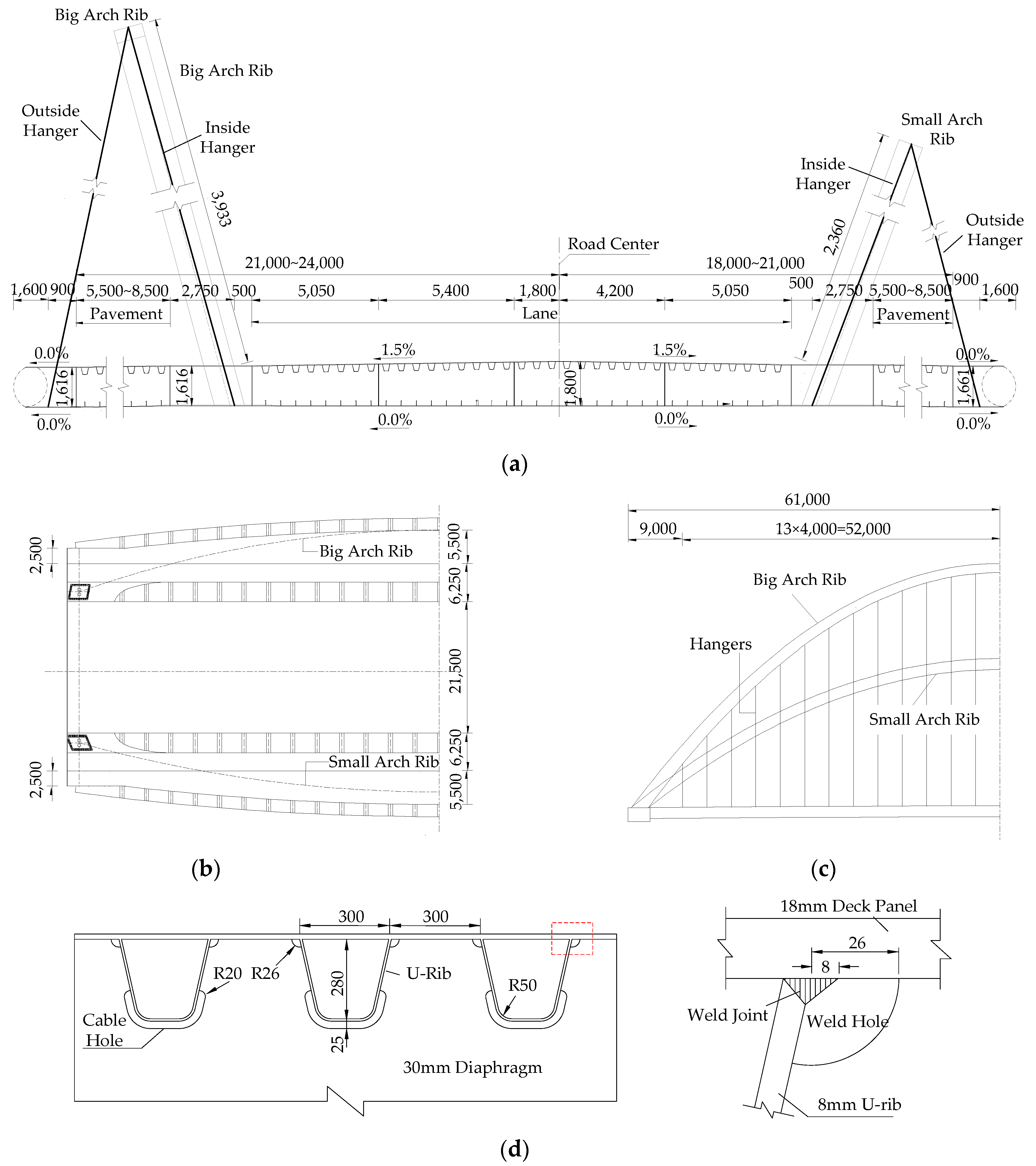
2. Description of the Bridge
3. Arlequin Method
4. Finite Element Models
4.1. Global Model
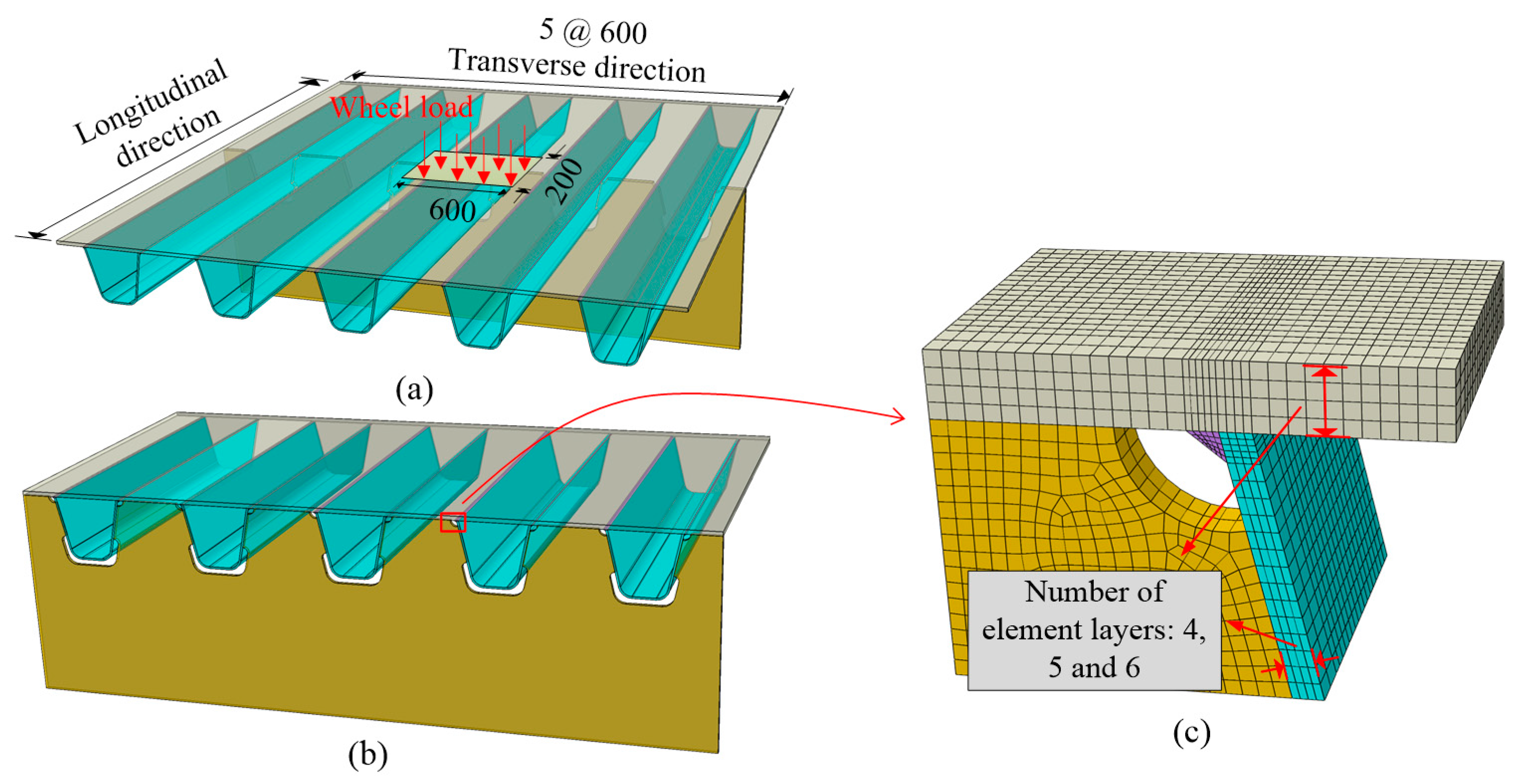
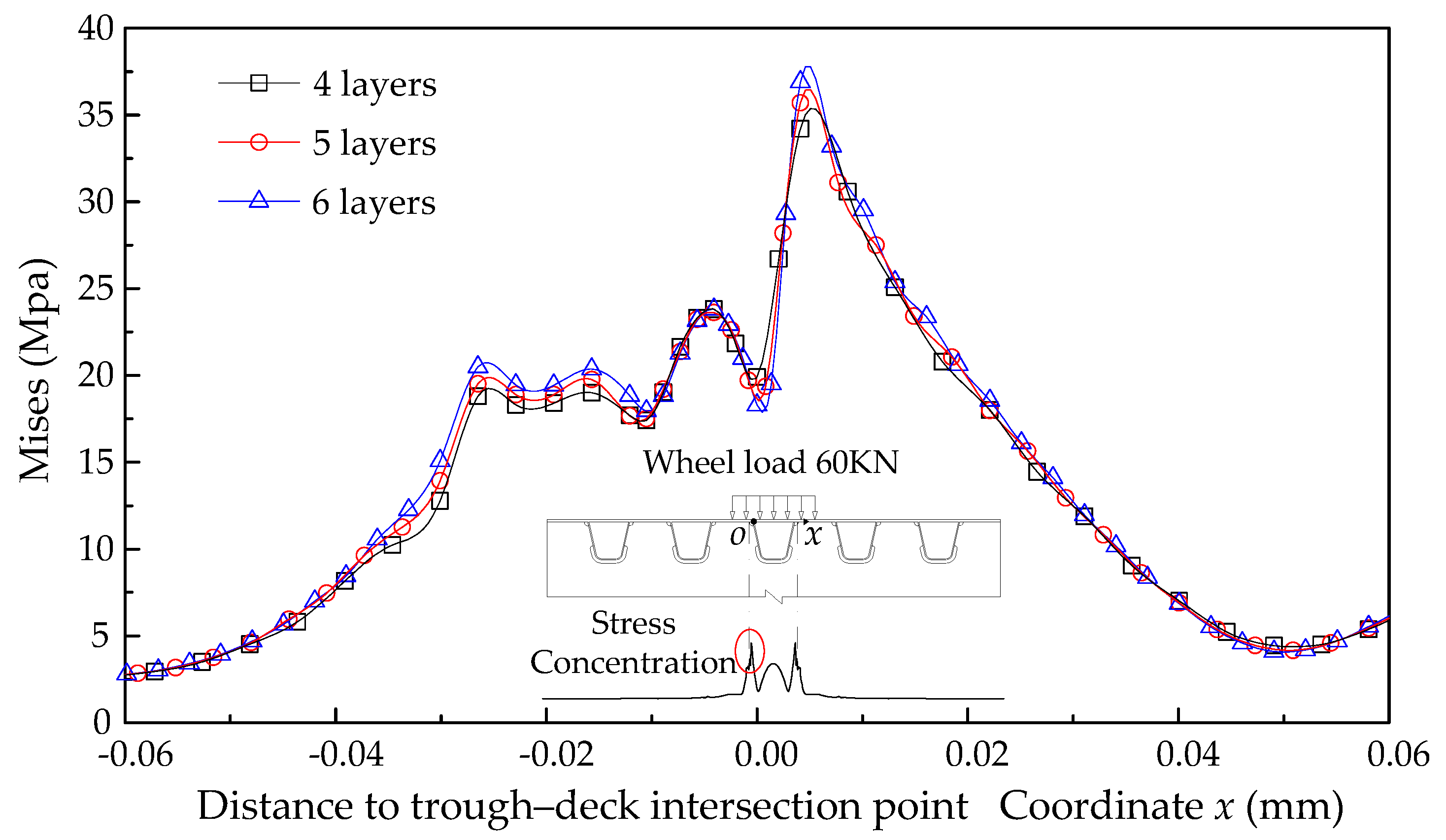
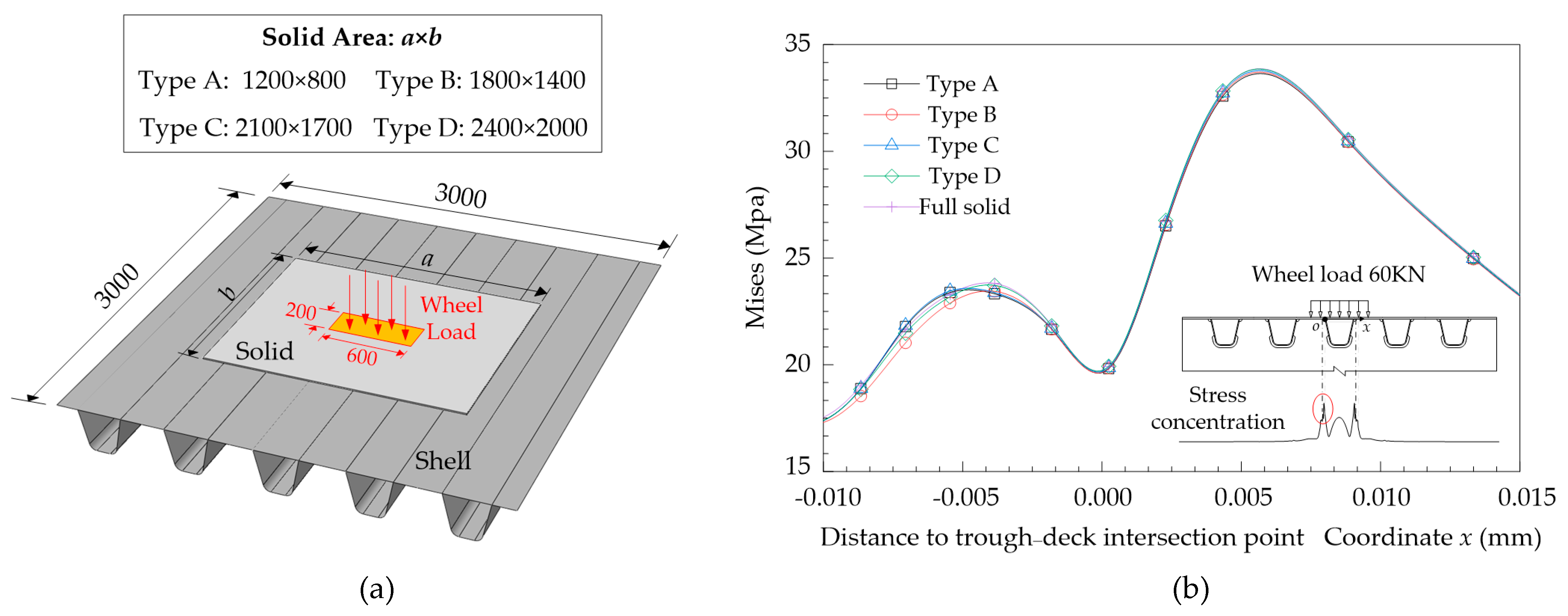
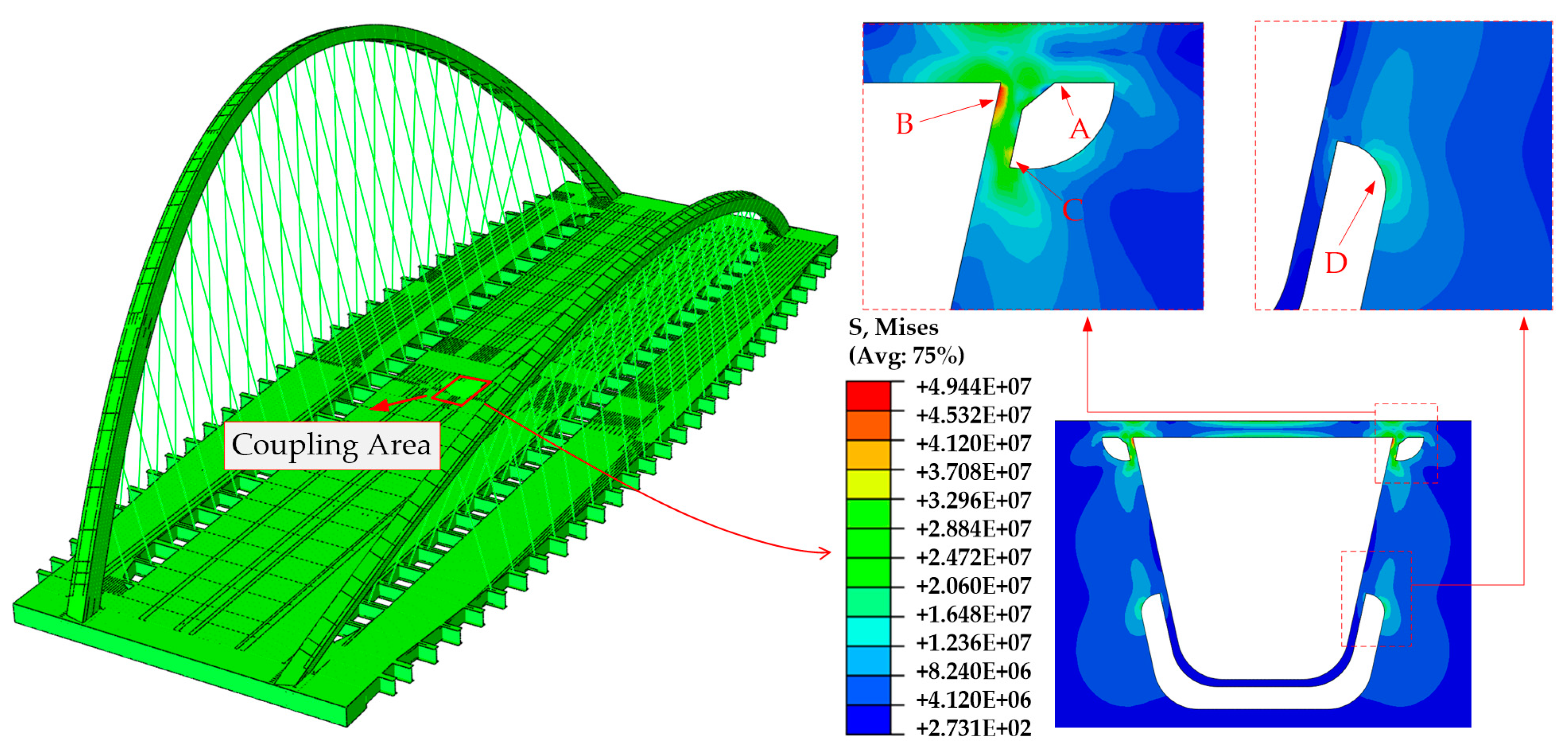
4.2. Local Model
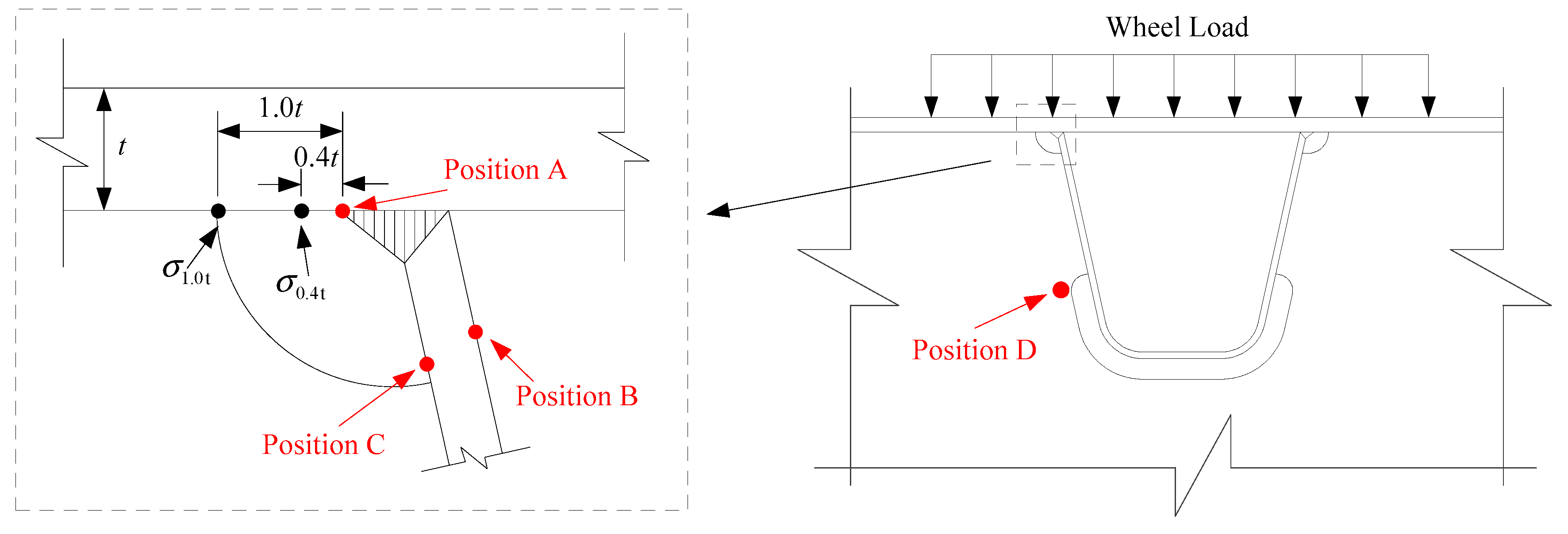
5. Fatigue Stress Analysis of Orthotropic Steel Deck
5.1. Fatigue Stresses of the Local and Global Models
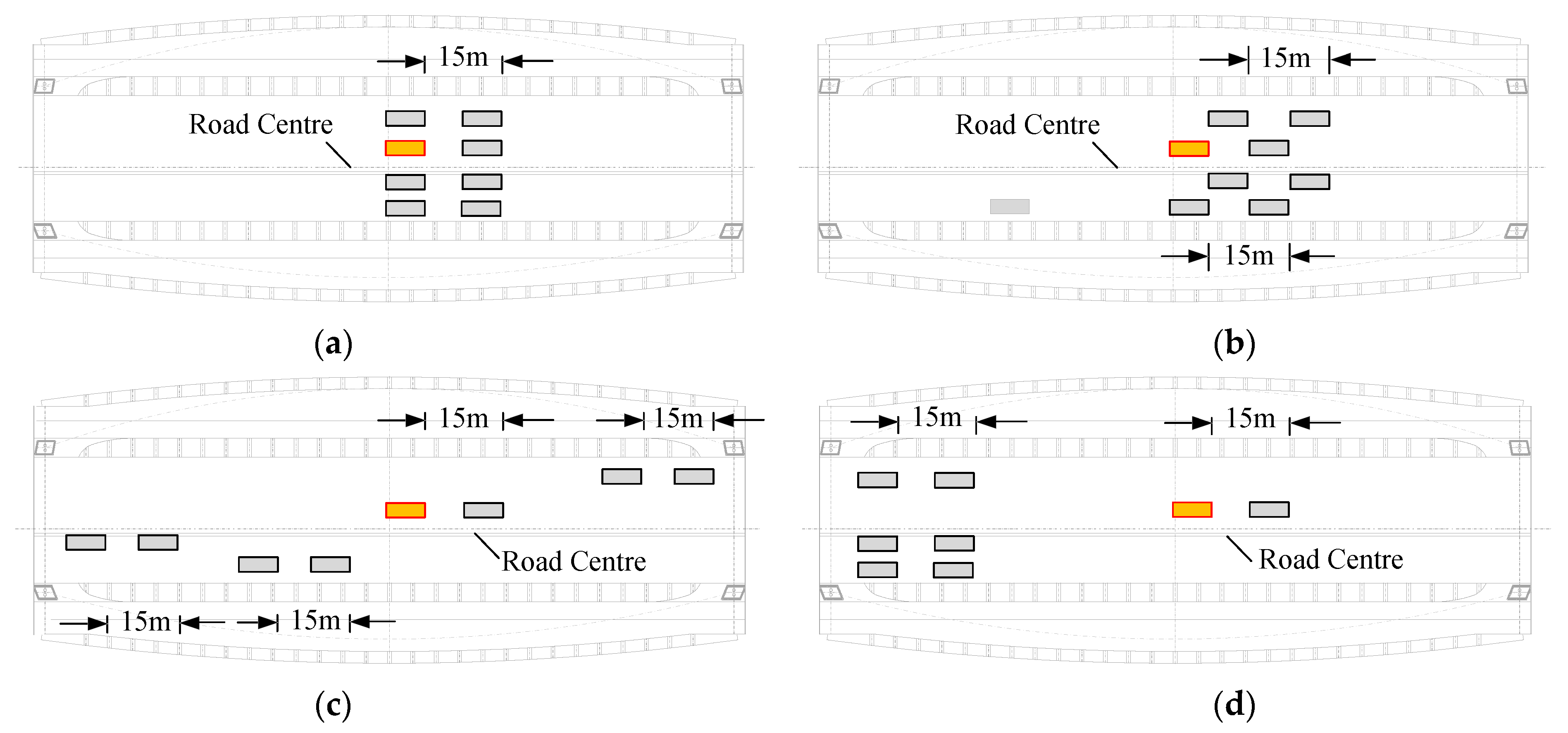
5.2. Fatigue Stresses Provoked by Other Vehicle Loads
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wolchuk, R. Lessons from weld cracks in orthotropic decks on three European bridges. J. Struct. Eng. 1990, 116, 75–84. [Google Scholar] [CrossRef]
- De Jong, F.B.P. Overview fatigue phenomenon in orthotropic bridge decks in the Netherlands. In Proceedings of the 1st international orthotropic bridge conference, Sacramento, CA, USA, 25–27 August 2004; pp. 489–512. [Google Scholar]
- Xu, W.; Zhang, X.N. Analysis of distress characters and design of steel orthotropic bridge. In Proceedings of the 2nd international orthotropic bridge conference, Sacramento, CA, USA, 25–29 August 2008; pp. 184–192. [Google Scholar]
- Xiao, Z.G.; Yamada, K.; Inoue, J.; Yamaguchi, K. Fatigue Cracks in Longitudinal Ribs of Steel Orthotropic Deck. Int. J. Fatigue 2005, 28, 409–416. [Google Scholar] [CrossRef]
- Ya, S.; Yamada, K.; Ishikawa, T. Fatigue Evaluation of Rib-to-Deck Welded Joints of Orthotropic Steel Bridge Deck. J. Bridge Eng. 2011, 18, 492–499. [Google Scholar] [CrossRef]
- Zhao, B. Research on Fatigue Behavior of Diaphragms for Orthotropic Decks of Steel Bridge Structures. Ph.D Thesis, Tianjin University, Tianjin, China, 2017. [Google Scholar]
- Nguyen, H.T.; Chu, Q.T.; Kim, S.E. Fatigue Analysis of a Pre-Fabricated Orthotropic Steel Deck for Light-Weight Vehicles. J. Constr. Steel Res. 2011, 67, 647–655. [Google Scholar] [CrossRef]
- Wang, D.; Xiang, C.; Ma, Y.; Chen, A.; Wang, B. Experimental Study on the Root-Deck Fatigue Crack on Orthotropic Steel Decks. Mater. Des. 2021, 203, 109601. [Google Scholar] [CrossRef]
- da Silva, L.S. Design of Steel Structures: Eurocode 3: Design of Steel Structures, Part 1–1 General Rules and Rules for Buildings; British Standard Institution: London, UK, 2010. [Google Scholar]
- American Railway Engineering and Maintenance-of-Way Association. AREMA Manual for Railway Engineering; American Railway Engineering and Maintenance-of-Way Association: Lanham, MD, USA, 2007. [Google Scholar]
- British Standard Institution. BS5400 Part 10: Code of Practice for Fatigue; British Standard Institution: London, UK, 1999. [Google Scholar]
- Ministry of Transport of the People’s Republic of China. JTG D64-2015: Specifications for Design of Highway Steel Bridge; China Communications Press: Beijing, China, 2015.
- Ministry of Housing and Urban-Rural Development of the People’s Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China. GB 50017-2017: Standard for Design of Steel Structures; China Construction Industry Press: Beijing, China, 2017.
- Japanese Road Association. Specifications for Highway Bridges, Part II: Steel Bridges; Tokyo Maruzen: Tokyo, Japan, 2017. [Google Scholar]
- Zeng, Z. Classification and Reasons of Typical Fatigue Cracks in Orthotropic Steel Deck. Steel Constr. 2011, 26, 9–15. [Google Scholar]
- Fisher, J.W.; Barsom, J.M. Evaluation of Cracking in the Rib-to-Deck Welds of the Bronx–Whitestone Bridge. J. Bridge Eng. 2016, 21, 04015065. [Google Scholar] [CrossRef]
- Zhong, W.; Ding, Y.L.; Song, Y.S.; Geng, F.F. Relaxation Behavior of Residual Stress on Deck-to-Rib Welded Joints by Fatigue Loading in an Orthotropic Bridge Deck. Period. Polytech. Civ. Eng. 2019, 63, 1125–1138. [Google Scholar] [CrossRef]
- Zhao, X. New Advance of Design Parameter and Structure Details of Orthotropic Deck. Steel Constr. 2010, 25, 1–7. [Google Scholar]
- Chen, B.; Chen, Z.; Xie, X.; Ye, X. Fatigue Performance Evaluation for an Orthotropic Steel Bridge Deck Based on Field Hotspot Stress Measurements. J. Test. Eval. 2020, 48, 20180565. [Google Scholar] [CrossRef]
- Tong, L.W. Study on Fatigue of Orthotropic Steel Deck. Ph.D Thesis, Tongji University, Shanghai, China, 1995. [Google Scholar]
- Xiang, H.F. Advanced Theory of Bridge Structures; People’s Communications Press: Beijing, China, 2001. [Google Scholar]
- Ichiro, K. Steel Bridge; Posts & Telecom Press: Beijing, China, 1980; Volume 8. [Google Scholar]
- Heins, C.P.; Firmage, D.A. Design of Modern Highway Steel Bridge; People’s Communications Press: Beijing, China, 1982. [Google Scholar]
- Tong, L.W.; Shen, Z.Y.; Chen, Z.Y. Structural analysis of orthotropic steel bridge deck in fatigue checking calculation. Shanghai J. Mechanics. 1998, 19, 3. [Google Scholar]
- Ji, B.; Liu, R.; Chen, C.; Maeno, H.; Chen, X. Evaluation on Root-Deck Fatigue of Orthotropic Steel Bridge Deck. J. Constr. Steel Res. 2013, 90, 174–183. [Google Scholar] [CrossRef]
- Fu, Z.; Ji, B.; Wang, M.; Zhang, Q. Analysis of Weld Fatigue Hot Spot Stress of Steel Bridge Deck Under Wheel Load. Ind. Constr. 2015, 45, 10. [Google Scholar]
- Song, Y.S.; Ding, Y.L. Influence of Local Geometric Parameters on Fatigue Performance of Orthotropic Steel Deck. J. Cent. South Univ. 2014, 21, 2091–2099. [Google Scholar] [CrossRef]
- Ayguel, M.; Al-Emrani, M.; Urushadze, S. Modelling and Fatigue Life Assessment of Orthotropic Bridge Deck Details Using FEM. Int. J. Fatigue 2012, 40, 129–142. [Google Scholar] [CrossRef]
- Heng, J.; Zheng, K.; Gou, C.; Zhang, Y.; Bao, Y. Fatigue Performance of Rib-to-Deck Joints in Orthotropic Steel Decks with Thickened Edge U-Ribs. J. Bridge. Eng. 2017, 22, 04017059. [Google Scholar] [CrossRef]
- Luo, P.J.; Zhang, Q.H.; Bao, Y.; Zhou, A.X. Fatigue evaluation of rib-to-deck welded joint using averaged strain energy density method. Eng. Struct. 2018, 177, 682–694. [Google Scholar] [CrossRef]
- Ben, D.H. Multiscale mechanical problems: The Arlequin method. Comptes Rendus L Académie Sci.-Ser. IIB-Mech.-Phys.-Astron. 1998, 326, 899–904. [Google Scholar]
- Qiao, H.; Chen, W.Q. Multi-Scale Simulation of Structures Based on Arlequin Method and Xfem. Eng. Mech. 2010, 27, 29–33. [Google Scholar]
- Qiao, H.; Chen, W.Q. Multi-Scale Numerical Simulation of Structuresbased On Arlequin Method. J. Zhejiang Univ. Eng. Sci. 2010, 44, 2314–2319. [Google Scholar]
- Kpogan, K.; Zahrouni, H.; Potier-Ferry, M.; Dhia, H.B. Buckling of Rolled Thin Sheets Under Residual Stresses by ANM and Arlequin Method. Int. J. Mater. Form. 2017, 10, 1–16. [Google Scholar] [CrossRef] [Green Version]
- General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China, Standardization Administration of the People’s Republic of China. GB/T 714-2015: Structural Steel for Bridge; Standards Press of China: Beijing, China, 2015. [Google Scholar]
- Ministry of Transport of the People’s Republic of China. JTG D60—2015: General Specifications for Design of Highway Bridges and Culverts; China Communications Press: Beijing, China, 2015.
- Shi, Y.P.; Zhou, Y.R. Detailed Explanation of ABAQUS Finite Element Analysis Examples; Mechanical Industry Press: Beijing, China, 2006; p. 54. [Google Scholar]
- Hobbacher, A.F. Recommendations for Fatigue Design of Welded Joints and Components; Springer International Publishing: Basel, Switzerland, 2016. [Google Scholar]
- Sun, Z.Q.; Pan, C.S.; Xu, J. Car-following Behavior of Passenger Cars on River Crossing Bridge Based on Naturalistic Driving Data. China J. Highw. Transp. 2020, 38, 154–162. [Google Scholar]
- Guo, H.B.; Qu, D.Y.; Hong, J.L.; Zhao, X.X. Study on traffic characteristics of expressway confluence area based on Full Velocity Difference Model. J. Qingdao Univ. Technol. 2021, 42, 8. [Google Scholar]



| E (MPa) | σy (MPa) | σu (MPa) | A (%) |
|---|---|---|---|
| 198,221 | 351.10 | 508.57 | 40.60 |
| Model | Position A | Position B | Position C | Position D | ||||
|---|---|---|---|---|---|---|---|---|
| Stress Range | δ | Stress Range | δ | Stress Range | δ | Stress Range | δ | |
| Local Model | 23.0 | - | 51.2 | - | 44.4 | - | 16.0 | - |
| MC | 27.8 | 20.87% | 62.3 | 21.68% | 55.7 | 25.45% | 24.3 | 51.87% |
| MS | 28.3 | 23.04% | 62.9 | 22.85% | 56.6 | 27.48% | 25.4 | 58.75% |
| QC | 27.5 | 19.57% | 61.7 | 20.51% | 55.2 | 24.32% | 23.2 | 45.00% |
| QS | 28.4 | 23.48% | 62.2 | 21.48% | 56.3 | 26.80% | 24.8 | 55.00% |
| Traffic Flow Case | Position A | Position B | ||||
| Stress Range | δ | δ1 | Stress Range | δ | δ1 | |
| Local Model | 23.0 | - | - | 51.2 | - | - |
| MC | 27.8 | - | - | 62.3 | - | - |
| Case 1 | 30.3 | 8.99% | 31.74% | 68.9 | 10.59% | 34.57% |
| Case 2 | 30.2 | 8.63% | 31.30% | 68.6 | 10.11% | 33.98% |
| Case 3 | 29.6 | 6.47% | 28.70% | 65.7 | 5.46% | 28.32% |
| Case 4 | 29.5 | 6.12% | 28.26% | 64.9 | 4.17% | 26.76% |
| Traffic Flow Case | Position C | Position D | ||||
| Stress Range | δ | δ1 | Stress Range | δ | δ1 | |
| Local Model | 44.4 | - | - | 16.0 | - | - |
| MC | 55.7 | - | - | 24.3 | - | - |
| Case 1 | 63.3 | 13.64% | 42.57% | 26.4 | 8.64% | 65.00% |
| Case 2 | 62.7 | 12.57% | 41.22% | 25.9 | 6.58% | 61.88% |
| Case 3 | 59.3 | 6.46% | 33.56% | 23.5 | −3.29% | 46.88% |
| Case 4 | 58.1 | 4.31% | 30.86% | 24.1 | 0.82% | 50.63% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, C.; Xie, X.; Yu, W. Investigation of the Fatigue Stress of Orthotropic Steel Decks Based on an Arch Bridge with the Application of the Arlequin Method. Materials 2021, 14, 7653. https://doi.org/10.3390/ma14247653
Cheng C, Xie X, Yu W. Investigation of the Fatigue Stress of Orthotropic Steel Decks Based on an Arch Bridge with the Application of the Arlequin Method. Materials. 2021; 14(24):7653. https://doi.org/10.3390/ma14247653
Chicago/Turabian StyleCheng, Cheng, Xu Xie, and Wentao Yu. 2021. "Investigation of the Fatigue Stress of Orthotropic Steel Decks Based on an Arch Bridge with the Application of the Arlequin Method" Materials 14, no. 24: 7653. https://doi.org/10.3390/ma14247653
APA StyleCheng, C., Xie, X., & Yu, W. (2021). Investigation of the Fatigue Stress of Orthotropic Steel Decks Based on an Arch Bridge with the Application of the Arlequin Method. Materials, 14(24), 7653. https://doi.org/10.3390/ma14247653







